Conductance enlargement in picoscale electroburnt graphene nanojunctions

Significance

Continuation of Moore’s law to the sub–10-nm scale requires the development of new technologies for creating electrode nanogaps, in architectures which allow a third electrostatic gate. Electroburnt graphene junctions (EGNs) have the potential to fulfill this need, provided their properties at the moment of gap formation can be understood and controlled. In contrast with mechanically controlled break junctions, whose conductance decreases monotonically as the junction approaches rupture, we show that EGNs exhibit a surprising conductance enlargement just before breaking, which signals the formation of a picoscale current path formed from a single sp² bond. Just as Schottky barriers are a common feature of semiconductor interfaces, conductance enlargement is a common property of EGNs and will be unavoidably encountered by all research groups working on the development of this new technology.

Abstract

Provided the electrical properties of electroburnt graphene junctions can be understood and controlled, they have the potential to underpin the development of a wide range of future sub-10-nm electrical devices. We examine both theoretically and experimentally the electrical conductance of electroburnt graphene junctions at the last stages of nanogap formation. We account for the appearance of a counterintuitive increase in electrical conductance just before the gap forms. This is a manifestation of room-temperature quantum interference and arises from a combination of the semimetallic band structure of graphene and a cross-over from electrodes with multiple-path connectivity to single-path connectivity just before breaking. Therefore, our results suggest that conductance enlargement before junction rupture is a signal of the formation of electroburnt junctions, with a picoscale current path formed from a single sp² bond.

Graphene nanojunctions are attractive as electrodes for electrical contact to single molecules (1–7), due to their excellent stability and conductivity up to high temperatures and a close match between their Fermi energy and the HOMO (highest occupied molecular orbital) or LUMO (lowest unoccupied molecular orbit) energy levels of organic materials. Graphene electrodes also facilitate electrostatic gating due to their reduced screening compared with more bulky metallic electrodes. Although different strategies for forming nanogaps in graphene such as atomic force microscopy, nanolithography (8), electrical breakdown (9), and mechanical stress (10) have been used, only electroburning delivers the required gap-size control below 10 nm (11–13). This new technology has the potential to overcome the challenges of making stable and reproducible single-molecule junctions with gating capabilities and compatibility with integrated circuit technology (14) and may provide the breakthrough that will enable molecular devices to compete with foreseeable developments in Moore’s law, at least for some niche applications (15–17).

One set of such applications is likely to be associated with room-temperature manifestations of quantum interference (QI), which are enabled by the small size of these junctions. If such interference effects could be harnessed in a single-molecule device, this would pave the way toward logic devices with energy consumption lower than the current state-of-the-art. Indirect evidence for such QI in single-molecule mechanically controlled break junctions has been reported recently in a number of papers (18), but direct control of QI has not been possible because electrostatic gating of such devices is difficult. Graphene electroburnt junctions have the potential to deliver direct control of QI in single molecules, but before this can be fully achieved, it is necessary to identify and differentiate intrinsic manifestations of room-temperature QI in the bare junctions, without molecules. In the present paper, we account for one such manifestation, which is a ubiquitous feature in the fabrication of picoscale gaps for molecular devices, namely an unexpected increase in the conductance before the formation of a tunnel gap.

Only a few groups in the world have been able to implement electroburning method to form nanogap-size junctions. In a recent study of electroburnt graphene junctions, Barreiro et al. (19) used real-time in situ transmission electron microscopy (TEM) to investigate this conductance enlargement in the last moment of gap formation and ruled out the effects of both extra edge scattering and impurities, which reduce the current density near breaking. Also, they showed that the graphene junctions can be free of contaminants before the formation of the nanogap. Having eliminated these effects, they suggested that the enlargement may arise from the formation of the seamless graphene bilayers. Here we show that the conductance enlargement occurs in monolayer graphene, which rules out an explanation based on bilayers. Moreover, we have observed the enlargement in a large number of nominally identical graphene devices and therefore we can rule out the possibility of device- or flake-specific features in the electroburning process. An alternative explanation was proposed by Lu et al. (20), who observed the enlargement in few-layer graphene nanoconstrictions fabricated using TEM. They attributed the enlargement to an improvement in the quality of few-layer graphene due to current annealing, which was simply ruled out by our experiments on electroburnt single-layer graphene. They also attributed this to the reduction of the edge scattering due to the orientation of the edges (i.e., zigzag edges). However, such edge effects have been ruled out by the TEM images of Barreiro et al. (19). Therefore, although this enlargement appears to be a common feature of graphene nanojunctions, so far the origin of the increase remains unexplained.

In what follows, our aim is to demonstrate that such conductance enlargement is a universal feature of electroburnt graphene junctions and arises from QI at the moment of breaking. Graphene provides an ideal platform for studying room-temperature QI effects (21) because, as well as being a suitable material for contacting single molecules, it can serve as a natural 2D grid of interfering pathways. By electroburning a graphene junction to the point where only a few carbon bonds connect the left and right electrodes, one can study the effect of QI in ring- and chain-like structures that are covalently bonded to the electrodes. In this paper, we perform feedback-controlled electroburning on single-layer graphene nanojunctions and confirm that there is an increase in conductance immediately before the formation of the tunnel junction. Transport calculations for a variety of different atomic configurations using the nonequilibrium Green’s function (NEGF) method coupled to density functional theory (DFT) show a similar behavior. To elucidate the origin of the effect, we provide a model for the observed increase in the conductance based on the transition from multipath connectivity to single-path connectivity, in close analogy to an optical double-slit experiment. The model suggests that the conductance increase is likely to occur whenever junctions are formed from any sp²-bonded material.

Conductance Through Constrictions

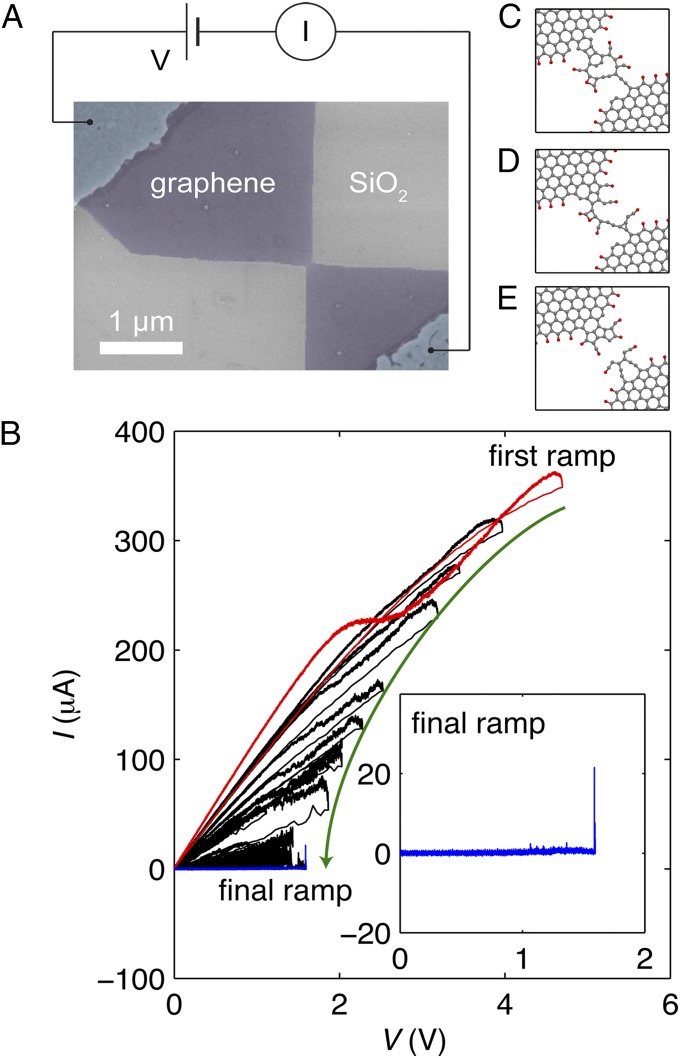
Experimentally we study the conductance jumps by applying the method of feedback-controlled electroburning to single-layer graphene (SLG) that was grown using chemical vapor deposition (CVD) and transferred onto a prepatterned silicon chip (Experimental Methods). The CVD graphene was patterned into 3-μm-wide ribbons with a 200-nm-wide constriction (Fig. 1A) using electron-beam lithography and oxygen plasma etching. Feedback-controlled electroburning has been demonstrated previously using few-layer graphene flakes that were deposited by mechanical exfoliation of kish graphite (11). However, by applying the method to an array of nominally identical single-layer graphene devices, we can rule out the possibility of device- or flake-specific features in the electroburning process.

Fig. 1.

(A) Scanning electron micrograph of the graphene device. (B) Measured current–voltage characteristic of the full I–V trace. (Inset) I–V trace of the final voltage ramp before the formation of the nanogap. This exhibits a sharp increase of the conductance just before the nanogap forms. (C–E) Three atomic configurations with two (C), one (D), and zero (E) pathways.

We form the nanogaps by ramping up the voltage that is applied across the graphene device. As the conductance starts to decrease due to the breakdown of the graphene, we ramp the voltage back to zero. This process is repeated until the nanogap is formed. The I–V traces of the voltage ramps, as shown in SI Appendix, Figs. S1–S4, closely resemble those recorded for mechanically exfoliated graphite flakes. As the constriction narrows, the conductance of the SLG device decreases. When the conductance becomes less than the conductance quantum G₀ = 2e²/h, the low-bias I–V traces are no longer Ohmic and start exhibiting random telegraph signal as the SLG device switches between different atomic configurations. Fig. 1B shows the full I–V trace and the final voltage ramp (Inset), which exhibits a sharp increase of the conductance just before the nanogap forms. This behavior is characteristic of many of the devices we have studied. Out of the 279 devices that were studied, 138 devices showed a sharp increase in the conductance before the formation of the nanogap (I–V traces for 12 devices are included in SI Appendix).

To investigate theoretically the transport characteristics of graphene junctions upon breaking, we used classical molecular-dynamics simulations to simulate a series of junctions with oxygen and hydrogen terminations as well as carbon-terminated edges and then used DFT combined with NEGF methods to compute the electrical conductance of each structure (Computational Methods). Fig. 1 C–E shows three examples of the resulting junctions with oxygen-terminated edges (which are the most likely to arise from the burning process), in which the left and right electrodes are connected via two (Fig. 1C), one (Fig. 1D), and zero (Fig. 1E) pathways.

Surprisingly, the conductance G through the single-path junction (Fig. 1D) is larger than the conductance through the double-path junction (Fig. 1C) (e.g., G = 18 µS for one path versus G = 0.4 µS for two paths in the low-bias regime V = 40 mV). For the nanogap junction shown in Fig. 1E, the conductance is less than both of these (G = 0.016 µS). We have calculated the conductance for 42 atomic junction configurations (SI Appendix, Figs. S6–S8), and commonly find that the conductance is larger for single-path junctions than for those with two or a few conductance paths. Approximately 40% of the total simulated junctions which were close to breaking exhibited the conductance enlargement, which is comparable to the experimental ratio of 49%.

The changes in the calculated conductances of junctions approaching rupture show a close resemblance to the experiments presented in this paper and by Barreiro et al. (19) and arise from the changes in the atomic configuration of the junction. We therefore attribute the experimentally observed jumps of the conductance to a transition from two- or few-path atomic configurations to single-path junctions, even though naive application of Ohm’s law would predict a factor of 2 decrease of the conductance upon changing from a double to a single pathway. In the remainder of this paper we will give a detailed analysis of the interference effects leading to the sudden conductance increase before the formation of a graphene nanogap.

Before proceeding to an analysis of QI effects, we first note that the conductance enlargement cannot be attributed to changes in the band structure near breaking. The band structures of the periodic chains and ribbons shown in Fig. 2 reveal that both are semimetallic, due to the formation of a π-band associated with the p orbital perpendicular to the plane of the structures. In fact, the ribbon (Fig. 2B) has more open conductance channels than the chain (Fig. 2A) around the Fermi energy (E = 0). The increase in conductance upon changing from a ribbon to a chain is therefore not due to a change in band structure, but rather due to QI in the different semimetallic pathways. A similar behavior is also found for structures with hydrogen termination and combined hydrogen–oxygen termination as shown in SI Appendix, Fig. S13.

Fig. 2.

Band structure of (A) C–O atomic chain, (B) C–O benzene chain. Gray atoms are carbon; red atoms are oxygen.

Fig. 3B shows the calculated current–voltage curves [corresponding transmission coefficients T(E) for electrons of energy E traversing the junctions are shown in SI Appendix, Fig. S10] for the five oxygen-terminated constrictions c₁–c₅ of Fig. 3A, with widths varying from 3 nm (c₅) down to a single atomic chain (c₁). The chains and ribbons in Fig. 3A are connected to two hydrogen-terminated zigzag graphene electrodes. The blue curve of Fig. 3B shows that the current through the chain c₁ is higher than the current through the ribbon c₂ (green curve in Fig. 3B), particularly at higher bias voltages. A nonequilibrium I–V calculation also confirms the same trend (SI Appendix, Fig. S10B). A similar behavior is found for structures with hydrogen termination and without edge termination as shown in SI Appendix, Figs. S11 and S12). Fig. 3C shows the I–V characteristic for junctions c₁ and c₂ plotted over a wider voltage range. At the penultimate stage of electroburning the c₂ curve is followed, until an electroburning event causes a switch from two carbon–carbon bonds to the single bond of structure c₁. At this point, the I–V jumps to that of structure c₁, as indicated in Fig. 3C by a dashed line.

Fig. 3.

(A) Ideal configuration with reduced junction width down to the atomic chain. (B) Calculated current–voltage relations in oxygen-terminated junctions. (C) I–V characteristic for junctions c₁ and c₂ over a wider voltage range. Dashed lines and arrows indicate the current jump from double bond of structure c₂ to that of structure c₁ when an electroburning event occurs.

To demonstrate that a two-path contact between two graphene electrodes typically has a lower conductance than a single-path contact, consider a graphene nanoribbon (Fig. 4 A–D, Left) connected to a carbon chain (Fig. 4 A and B, Right) or to hexagonal chains (Fig. 4 C and D). To calculate the current flow through the junctions Fig. 4 A–D and to study the effect of a bond breaking on the current when all other parameters are fixed, we built a tight-binding Hamiltonian of each system (Computational Methods). Starting from junctions Fig. 4 A and C with two pathways between the leads, we examined the effect of breaking a single bond to yield junction Fig. 4 B and D, respectively, with only one pathway each. As shown in Fig. 4, the current is increased when a bond is broken. (More detailed calculations are presented in SI Appendix.) This demonstrates that in a junction formed from strong covalent bonds, the current in the one-pathway junction can be higher than in junctions with more than one pathway. This captures the feature revealed by the DFT–NEGF calculations on the structures of Fig. 1 that if bonds break in a filament with many pathways connecting two electrodes from different points, the current flow can increase. This result is highly nonclassical and, as shown in the next section, is a consequence of constructive quantum interference in picoscale graphene junctions connected by a single sp² bond (of length ∼142 PM).

Fig. 4.

(A–D) Each shows an electrode formed from a graphene nanoribbon (Left) in contact with an electrode (Right) formed from a linear chain (A and B) or a chain of hexagons (C and D). For A and C the contact to the chain is via a two bond. For B and D the contact to the chain is via single bonds. For a voltage v = 20 mV, the red circles show the current through each structure. The arrows indicate that upon switching from a two-bond contact to a single-bond contact, the current increases. I₀ = 77.4 µA is the current carried by a quantum of conductance G₀ at 1 V.

QI in Atomic Chains and Rings

To illustrate analytically the consequences of QI in few-pathway junctions, consider the structure shown in Fig. 5A, which consists of an atomic chain (in Fig. 5A this comprises atoms 2 and 3) connected to a single-channel lead terminating at atom i = 1 and to a second single-channel lead terminating at atom j = 4. Now consider adding another atomic chain in parallel to the first, to yield the structure shown in Fig. 5B. In physics, the optical analog of such a structure is known as a Mach–Zehnder interferometer (22).

Fig. 5.

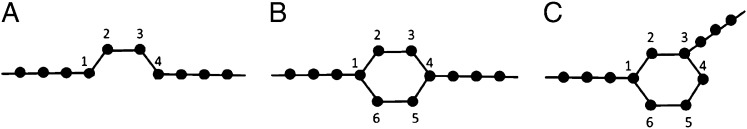
(A) A 1D chain connected to 1D semiinfinite leads on the left and right. (B) Two parallel chains forming a ring with para- coupling to the leads and (C) two parallel chains with meta- coupling to the leads.

In the following, we shall show that the single-path structure of Fig. 5A has the highest of the three conductances. This trend is the opposite of what would be expected if the lines were classical resistors (SI Appendix) and the circles were perfect connections. In that case Fig. 5A would have the lowest conductance and Fig. 5C the highest conductance. An intuitive understanding of why our case is different begins by noting that in the quantum case, electrical conductance is proportional to the transmission coefficient T(E) of de Broglie waves of energy E passing through a given structure. If we neglect the lattice nature of the system, and consider the paths simply as classical waveguides, then for a wave propagating from the left-hand end in each case, the bifurcations in Fig. 5 B and C present an impedance mismatch, so that a fraction of the wave is reflected. Considering a waveguide of impedance Z with a bifurcation into two waveguides, for unit incident amplitude the total transmitted amplitude is ($2\sqrt{2}/3$), and the transmitted intensity is $T=8/9$. A similar analysis can be applied to a 1D lattice formed of M semiinfinite chains. This is illustrated in Fig. 6A for M = 2 (a continuous chain) and Fig. 6B for M = 3 (a bifurcation).

Fig. 6.

(A) A system with M = 2 semiinfinite chains, centered on site 0. (B) A system with M = 3 semiinfinite chains, centered on site 0. In each case, a plane wave from the left is either reflected with reflection amplitude r, or transmitted with transmission amplitude t.

Within a tight-binding or Hückel description of such systems, the transmission and reflection amplitudes r and t are obtained from matching conditions at site “0.” Then for electron energies E at the band center (i.e., HOMO–LUMO gap center, which coincides with the charge neutrality point in our model), it can be shown (SI Appendix) that the transmission coefficient T = |t|² is given by

$$T=\frac{4\left(M-1\right)}{M^2}.$$ [1]

For M = 2, this formula yields T = 1, as expected, because system Fig. 6A is just a continuous chain with no scattering. Because T cannot exceed unity, any changes can only serve to decrease T. For a bifurcation (M = 3), Eq. 1 yields T = 8/9, which is the same result as a continuum bifurcated waveguide.

When the two branches of Fig. 6B come together again to form a ring, there can be further interference effects, associated with additional reflections where the branches rejoin. These may serve to decrease or increase the transmission. At most the transmission will increase to T = 1, but in general T will remain less than unity. It might be expected that the asymmetrical ring in Fig. 5C will be more likely to manifest destructive interference than the symmetrical ring in Fig. 5B. These intuitive conclusions from continuous and discrete models are confirmed by the following rigorous analysis based on a tight-binding model of the actual atomic configurations, which captures the key features of the full DFT–NEGF calculations.

We consider a simple tight-binding (Hückel) description, with a single orbital per atom of “site energy” ${\epsilon }_0$ and nearest-neighbor couplings –γ$–\gamma$. As an example, for an infinite chain of such atoms, the Schrodinger equation takes the form ${\epsilon }_0{\phi }_j-\gamma {\phi }_{j-1}-\gamma {\phi }_{j+1}=E{\phi }_j$ for $-\infty <j<\infty$. The solution to this equation is ${\phi }_j=e^{ikj}$, where $-\pi <k<\pi$ is wave vector. Substituting this into the Schrodinger equation yields the dispersion relation of $E={\epsilon }_0-2\gamma \cos k$. This means that such a 1D chain possesses a continuous band of energies between $E^{-}={\epsilon }_0-2\gamma$ and $E^{+}={\epsilon }_0+2\gamma$. Because the 1D leads in Fig. 5 are infinitely long and connected to macroscopic reservoirs, systems Fig. 5 A–C are open systems. In these cases, the transmission coefficient $T\left(E\right)$ for electrons of energy $E$ incident from the first lead is obtained by noting that the wave vector $k\left(E\right)$ of an electron of energy $E$ traversing the ring is given by $k\left(E\right)={\cos }^{-1}({\epsilon }_0-E)/2\gamma$. When $E$ coincides with the midpoint of the HOMO–LUMO gap of the bridge, i.e., when $E={\epsilon }_0$, this yields $k\left(E\right)=\pi /2$. Because $T\left(E\right)$ is proportional to ${\left|1+e^{ikL}\right|}^2$, where $L$ is the difference in path lengths between the upper and lower branches, for structure Fig. 5B, one obtains constructive interference, because $e^{ikL}=e^{i0}=1$ and for structure Fig. 5C destructive interference, because $e^{ikL}=e^{i2k}=-1$. This result is unsurprising, because it is well known that the meta-coupled ring Fig. 5C should have a lower conductance than the para-coupled ring Fig. 5B (23). More surprising is the fact that the single-chain structure Fig. 5A has a higher conductance than both Fig. 5B and Fig. 5C. To illustrate this feature, we note (see SI Appendix for more details) that the ratio of the Green’s function $G_{ring}$ of the structure of Fig. 5B to the Green’s function of the chain Fig. 5A, evaluated between the atoms 1 and 4, is

$$\frac{G_{ring}}{G_{chain}}=\frac{1}{2}[1-\alpha ],$$ [2]

where α is a small self-energy correction due to the attachment of the leads. For small α, this means that the transmission of the linear chain at the gap center is 4× higher than the transmission of a para- ring (because transmission is proportional to the square of the Green’s function), which demonstrates that the conductances of both the two-path para- and meta-coupled structures are lower than that of a single-path chain. This result is the opposite of the behavior discussed in ref. 24, where the conductance of two identical parallel chains was found to be 4× higher than that of a single chain. The prediction in ref. 24 is only applicable in the limit that the coupling of the branches to the nodes is weak, whereas in sp²-bonded graphene junctions, the coupling is strong.

Conclusion

We have addressed a hitherto mysterious feature of electroburnt graphene junctions, namely a ubiquitous conductance enlargement at the final stages before nanogap formation. Through a combined experimental and theoretical investigation of electroburnt graphene nanojunctions, we have demonstrated that conductance enlargement at the point of breaking is a consequence of a transition from multiple-path to single-path quantum transport. This fundamental role of quantum interference was demonstrated using calculations based on DFT–NEGF methods, tight-binding modeling, and analytic results for the structures of Fig. 5. Therefore, our results suggest that conductance jumps provide a tool for characterizing the atomic-scale properties of sp²-bonded junctions and in particular, conductance enlargement before junction rupture is a signal of the formation of electroburnt junctions, with a current path formed from a single sp² bond. Conductance enlargement is common, but does not occur in all electroburnt nanojunctions, because direct jumps from two-path to broken junctions can occur. With greater control of the electroburning feedback, our analysis suggests that one could create carbon-based atomic chains and filaments, which possess many of the characteristics of single molecules without the need for anchor groups, because the chains are already covalently bonded to electrodes.

Computational Methods

The Hamiltonian of the structures described in this paper was obtained using DFT as described below or constructed from a simple tight-binding model with a single orbital per atom of site energy ${\epsilon }_0=0$ and nearest-neighbor couplings $\gamma =-1$.

DFT Calculation.

The optimized geometry and ground-state Hamiltonian and overlap matrix elements of each structure were self-consistently obtained using the SIESTA (25) implementation of DFT. SIESTA employs norm-conserving pseudopotentials to account for the core electrons and linear combinations of atomic orbitals to construct the valence states. The generalized gradient approximation (GGA) of the exchange and correlation functional is used with the Perdew–Burke–Ernzerhof parameterization (26), a double-ζ polarized basis set, a real-space grid defined with an equivalent energy cutoff of 250 Ry. The geometry optimization for each structure is performed for the forces smaller than 40 meV/Å. For the band structure calculation, the given structure was sampled by a 1 × 1 × 500 Monkhorst–Pack k-point grid.

Transport Calculation.

The mean-field Hamiltonian obtained from the converged DFT calculation or a simple tight-binding Hamiltonian was combined with our implementation of the nonequilibrium Green’s function method [the GOLLUM (27)] to calculate the phase-coherent, elastic scattering properties of the each system consist of left (source) and right (drain) leads and the scattering region. The transmission coefficient T(E) for electrons of energy E (passing from the source to the drain) is calculated via the relation

$$T\left(E\right)=\mathrm{trace}\left({\mathrm{\Gamma }}_R\left(E\right)G^R\left(E\right){\mathrm{\Gamma }}_L\left(E\right)G^{R†}\left(E\right)\right).$$ [3]

In this expression,${\mathrm{\Gamma }}_{L,R}\left(E\right)=i\left({{{\displaystyle \sum }}^{\text{}}}_{L,R}\left(E\right)-{{\displaystyle \sum }}_{L,R}^{†}\left(E\right)\right)$ describes the level broadening due to the coupling between left (L) and right (R) electrodes and the central scattering region, ${{{\displaystyle \sum }}^{\text{}}}_{L,R}\left(E\right)$ are the retarded self-energies associated with this coupling, and $G^R={\left(ES-H-{{{\displaystyle \sum }}^{\text{}}}_L-{{{\displaystyle \sum }}^{\text{}}}_R\right)}^{-1}$ is the retarded Green’s function, where H is the Hamiltonian and S is overlap matrix. Using the obtained transmission coefficient [$T\left(E\right)$], the conductance could be calculated by the Landauer formula [$G=G_0{{\displaystyle \int }}^{\text{}}dET\left(E\right)\left(-\partial f/\partial E\right)$], where $G_0=2e^2/h$ is the conductance quantum. In addition, the current through the device at voltage V could be calculated as

$$I\left(V\right)=\frac{2e}{h}{\displaystyle \underset{-\left(V/2\right)}{\overset{+\left(V/2\right)}{\int }}dET\left(E\right)\left[f\left(E-\frac{V}{2}\right)-f\left(E+\frac{V}{2}\right)\right]},$$ [4]

where $f\left(E\right)={(1+\exp ((E-E_F)/k_{B}T))}^{-1}$ is the Fermi–Dirac distribution function, T is the temperature, and k_B= 8.6 × 10⁻⁵ eV/K is Boltzmann’s constant.

Molecular Dynamics.

Left and right leads (Fig. 1 C–E) were pulled in the transport direction by −0.1 Å and 0.1 Å every 40 fs (200 time steps) using the molecular dynamic code LAMMPS (28). Energy minimization of the system was achieved in each 200 time steps by iteratively adjusting atomic coordinates using the following parameters: the stopping energy of 0.2, the force tolerances of 10⁻⁸, the maximum minimizer iterations of 1,000, and the number of force–energy evaluations of 10,000. The atoms were treated in the REAX (reactive) force-field model with reax/c parameterization and charge equilibration method as described in ref. 28 with low and high cutoff of 0 and 10 for Taper radius and the charges equilibrated to a precision of 10⁻⁶. The atomic positions are updated in 0.02-fs time steps at 400 K with constant volume and energy. The snapshot of the atomic coordinates was sampled every 665 time steps. The whole procedure was performed twice and a total of 42 configurations was extracted. Each obtained set of coordinates was used as an initial set of coordinates for the subsequent self-consistent DFT loops as described above.

Experimental Methods

Similar to previous studies using few-layer graphene flakes, the feedback-controlled electroburning is performed in air at room temperature. The feedback-controlled electroburning of the SLG devices (29) is based on the same method as previously used for electroburning of few-layer graphene flakes (11) and electromigration of metal nanowires (30). A voltage (V) applied between the two metal electrodes is ramped up at a rate of 0.75 V/s, while the current (I) is recorded with a 200-µs sampling rate. When the feedback condition which is set at a drop ΔI of the current within the past 15 mV is met, the voltage is ramped down to zero at a rate of 225 V/s. After each voltage ramp the resistance of the SGL device is measured and the process is repeated until the low-bias resistance exceeds 500 MΩ. To prevent the SGL device from burning too abruptly at the initial voltage ramps we adjust the feedback condition for the each voltage ramp depending on the voltage at which the previous current drop occurred. The feedback conditions used were ΔI_set = 6, 9, 12, and 15 mA for V_th = 1.9, 1.6, 1.3, and 1.0 V, respectively.