The biomechanics of walking shape the use of visual information during locomotion over complex terrain

Abstract

The aim of this study was to examine how visual information is used to control stepping during locomotion over terrain that demands precision in the placement of the feet. More specifically, we sought to determine the point in the gait cycle at which visual information about a target is no longer needed to guide accurate foot placement. Subjects walked along a path while stepping as accurately as possible on a series of small, irregularly spaced target footholds. In various conditions, each of the targets became invisible either during the step to the target or during the step to the previous target. We found that making targets invisible after toe off of the step to the target had little to no effect on stepping accuracy. However, when targets disappeared during the step to the previous target, foot placement became less accurate and more variable. The findings suggest that visual information about a target is used prior to initiation of the step to that target but is not needed to continuously guide the foot throughout the swing phase. We propose that this style of control is rooted in the biomechanics of walking, which facilitates an energetically efficient strategy in which visual information is primarily used to initialize the mechanical state of the body leading into a ballistic movement toward the target foothold. Taken together with previous studies, the findings suggest the availability of visual information about the terrain near a particular step is most essential during the latter half of the preceding step, which constitutes a critical control phase in the bipedal gait cycle.

Introduction

Humans regularly traverse many types of terrain that require visually guided modifications of gait. For example, walking is sometimes made difficult due to the presence of unsafe or undesirable footholds (e.g., puddles, patches of mud or ice, potholes) on a flat ground surface. In such situations, successful locomotion requires walkers to use visual information to identify safe target footholds and modulate stride length, width, and timing to land on those footholds with precision and efficiency.

Given the demands for precision, one might assume that the trajectory of the legs and feet are controlled much like the trajectory of the hand is controlled during reaching to a target, that is, by actively and continuously guiding the effector for as much of the movement as possible. The importance of online control during reaching to stationary targets has been demonstrated by showing that aiming is more accurate when visual information is available throughout the movement compared to when the room lights are extinguished upon movement initiation (Elliott & Allard, 1985; Zelaznik, Hawkins, & Kisselburgh, 1983). Performance advantages with visual information are observed for all but the shortest movements (i.e., those lasting less than 100–150 ms; see Carlton, 1992, for a review), for which perceptual motor delays exceed movement time, preventing the use of visual feedback. Such findings have been interpreted as evidence of a rapid, closed-loop, visual feedback–based process for correcting errors in aiming trajectory (Elliott, Binsted, & Heath, 1999).

Studies of walking demonstrate that humans are capable of rapidly using visual information during the swing phase to improve the accuracy of stepping. When an obstacle suddenly appears (Patla, Beuter, & Prentice, 1991; Weerdesteyn, Nienhuis, Hampsink, & Duysens, 2004) or a target suddenly moves (Reynolds & Day, 2005a), the trajectory of the swinging leg is adjusted within about 120 ms. The extremely short latency of such adjustments compared to voluntary stride modifications and simple reaction time responses has been interpreted as evidence of the involvement of subcortical pathways (Reynolds & Day, 2005a; Weerdesteyn et al., 2004). Even when the foot target remains stationary, stepping is more accurate when visual information is available throughout the step compared to when vision is occluded at step initiation (Reynolds & Day, 2005b). Converging evidence for the use of visual information during the swing phase has been sought by studying gaze behavior during walking. For example, Hollands, Marple-Horvat, Henkes, and Rowan (1995) found that when humans walk over a series of irregularly spaced targets, they saccade to the upcoming target shortly before the step to that target is initiated and maintain fixation on the target until shortly before the foot lands on the target. Taken together, these findings provide support for the hypothesis that the accuracy with which humans step to targets is at least partly attributed to the ability to rapidly use visual information to modulate swing leg trajectory while the foot is moving toward the target.

However, when humans walk over extended stretches of complex terrain, there are at least two reasons why precision in the placement of the feet may be achieved in an entirely different manner. First, walkers need to be concerned not only with the upcoming step but also with the terrain further ahead. We recently demonstrated the importance of being able to see the terrain beyond the next step by showing that stepping accuracy degrades and walking speed decreases when the terrain does not become visible until it lies within a single step length (Matthis & Fajen, 2013, 2014). This finding raises questions about the relevance of some of the aforementioned studies for our understanding of visually guided walking over extended stretches of complex terrain. The task in those studies required subjects to take a single step to a target and then stop (Reynolds & Day, 2005a, 2005b) or step over a single obstacle and continue walking (Weerdesteyn et al., 2004). Although walkers may be able to make rapid adjustments to leg trajectory during a single step, it remains unclear whether visual information is used during the swing phase when walking over a series of irregularly spaced targets when the upcoming terrain must also be taken into account.

Second, during walking over extended stretches of complex terrain, the goal of landing on small targets must be satisfied while simultaneously walking in an energetically efficient manner, which is achieved by exploiting the passive physical forces acting on the body during locomotion. During the single support phase, the human body is mechanically similar to an inverted pendulum (Cavagna & Margaria, 1966) with a large center of mass (COM) supported over a point of rotation at the ankle joint. As the COM travels along an arc defined by the stance leg, the exchange between kinetic and potential energy is very efficient such that the total mechanical energy of the COM remains fairly stable over the course of a step. That is, the inverted pendulum–like structure of the human body makes it possible for energy to be largely conserved during the single support phase, which is central to the energetic efficiency of human walking (Kuo, 2007; Kuo & Donelan, 2010).

When walking over complex terrain, it may not be possible to allow the feet to land where the passive pendulum-like motion takes them. Nonetheless, energetic efficiency is just as important when walking over complex terrain. Therefore, the ability to walk efficiently in such environments is likely to be based on the same principles that allow walkers to be efficient in simpler environments. To take advantage of the body's inverted pendulum–like structure during walking over complex terrain, walkers could use visual information about the upcoming terrain to properly initialize each step so that the body can follow its natural trajectory to a safe foothold (Matthis & Fajen, 2013, 2014). The two determinants of the passive trajectory of the COM during a step are the position of the planted foot and the initial velocity of the COM. As such, to land on a target while exploiting one's inverted pendulum–like structure, a walker could properly position the foot on the preceding step and push off with the trailing leg to redirect the COM so that the passive motion takes the swinging leg toward the target. Indeed, the trajectory of the COM is similar to that of an inverted pendulum even during walking over complex terrain, provided that the walker can identify safe footholds at least two steps in advance, which is the minimum look-ahead distance needed to properly initialize each step (Matthis & Fajen, 2013).

To summarize, during walking over complex terrain, walkers can best exploit the inverted pendulum–like structure of their bodies by placing their feet and tailoring the push-off force during double support of the preceding step in such a way that energetically costly midflight corrections are not needed. In other words, it is to one's advantage to make adjustments before the step is initiated and let the passive forces guide the foot to the target. In this regard, the visual control of stepping on a target may be quite different than the visual control of reaching to a target. Whereas the energetic consequences of visually guided adjustments to the trajectory of the hand during reaching are relatively negligible, executing midflight adjustments to the trajectory of the foot during walking means fighting against more significant forces. Thus, although walkers are capable of using visual information to rapidly adjust the trajectory of the leg during the swing phase, a strategy that allows them to work with rather than against the passive forces that are generated during locomotion would be more energetically efficient.

The aim of the present study was to determine when visual information about a target foothold is no longer necessary to guide foot placement. Subjects walked across a path of irregularly spaced target footholds while attempting to step with a high degree of accuracy at each step. They performed this task in a full vision condition in which the targets were visible for the entire trial and in several limited visibility conditions in which each target became invisible at some point prior to foot placement on that target. If stepping onto a target with the foot is like reaching to a target with the hand (as suggested by Reynolds & Day, 2005b), then visual information should be used during the step to maximize stepping accuracy. On the other hand, if walkers attempt to exploit their inverted pendulum–like structure and follow a ballistic trajectory to the target, then it would be expected that visual information about the target is primarily used before the step is initiated. That is, visual information should no longer play a role once the leg is in the swing phase.

Methods

Subjects

Twelve subjects (four female, eight male; age [M ± SD]: 19.0 ± 1.3 years; height: 1.76 ± 0.1 m; weight: 72.2 ± 9.5 kg; leg length: 0.93 ± 0.04 m) participated in the study. Subjects were recruited from psychology courses and received extra credit for participating. All subjects reported no motor impairments and normal or corrected-to-normal vision. The protocol was approved by the Institutional Review Board at Rensselaer Polytechnic Institute and is in compliance with the Declaration of Helsinki. All subjects gave informed consent in writing before participating in the experiment.

Equipment

The experiment was conducted using a 14-camera Vicon motion-capture system running Vicon Nexus software. The motion-capture system tracked the positions of 34 retroreflective markers that were attached to a tightly fitting, elastic shirt and leggings worn by subjects. The markers were placed in accordance with the Vicon Plug-In Gait Full Body (SACR) marker set included in the Nexus software package. Subjects completed the experiment barefoot in order to avoid any irregularities caused by differences in footwear.

A Sanyo PLC-XP45 projector was used to display virtual obstacles onto the floor at a resolution of 1024 × 768 with a brightness of 3500 ANSI lumens. The projector was located 2.07 m behind the end position at a height of 1.45 m pointed at the ground at a relatively low angle of incidence (∼25°). The projector's range covered a trapezoid that was roughly 1.44 m at the end position nearest the projector and 4.00 m near the starting position (Figure 1).

Figure 1.

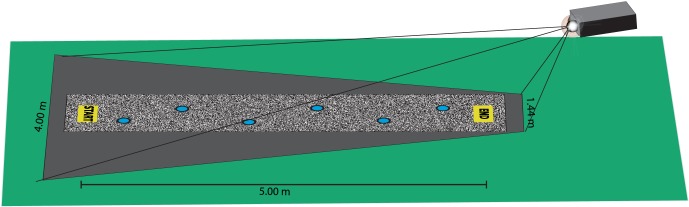
Experimental setup.

Subjects walked across a path of six small circular target footholds (radius: 50 mm) that spanned the space between the start and end locations. To define the target configurations used in this study, the experimenter recorded a normal footfall pattern when walking from the start to end location without targets. The target configurations were then obtained by randomly placing the targets within a 300 mm × 300 mm box centered on each step in this pattern of footfalls. In order to scale the task to each subject's body size, the distances between the targets were multiplied by the ratio of the subject's leg length to the leg length of the experimenter who defined the original footfall pattern. The path between the start and end boxes was covered by a scintillating white noise texture designed to prevent subjects from using irregularities on the carpet as landmarks to keep track of targets after they were made invisible. The projector system was synchronized to the motion-capture system using the method described in Matthis and Fajen (2014).

Task and procedure

Subjects began the experiment by completing 10 free-walking trials in which there were no targets present. They were asked to walk at a brisk pace from a start position (marked with tape on a carpeted floor) to an end position 5.0 m away. In the conditions following the free-walking trials, subjects walked across a path of six targets spanning the space between the start and end locations. At each step, if the Vicon marker that was attached to the second metatarsal head of the foot was within the target radius, the computer speakers played a high-pitched tone to indicate that the subject had successfully hit the target. Otherwise, a low-pitched tone was played to indicate that the target had been missed. Subjects were instructed to walk so as to place the foot marker as close to the center of the target as possible. Subjects clicked a button on a wireless mouse to begin each trial, after which they had 5 s to reach the end position. If they failed to reach the end position in 5 s, the trial was terminated and rerun. As such, subjects had to walk at an average speed of at least 1.0 m/s in order to successfully complete each trial.

Design

An experimental session consisted of two blocks of 100 trials each, for a total of 200 trials per subject. The first block comprised 10 different sets of 10 trials. The first set of trials was the free walking condition (in which there were no targets), and the remaining sets were a random ordering of the nine different visibility conditions: a full-vision control condition in which all targets were visible for the entire trial and the eight different limited-vision conditions in which the targets were made invisible at some point before the subject stepped on them. The nine sets were presented in a randomized order for each subject. The second block was identical to the first except that the order of the nine sets of trials following the free-walking condition was reversed. In all, there were a total of 20 repetitions of each condition.

In the limited-vision conditions, we defined a circular “invisibility trigger” surrounding each target (Figure 2). The circular trigger was not visible to subjects. The computer was programmed so that the targets were made invisible when the subject's foot moved inside the trigger's range. The radius of each invisibility trigger was defined as a proportion of the required stride length (SL), which was equal to the distance between the target at the center of the invisibility trigger and the target that was two targets back. For example, the foot landing on target₄ began from target₂, so the radius of the invisibility trigger for target₄ was a proportion of the distance between target₂ and target₄ (i.e., the stride length of the step that landed on target₄).

Figure 2.

Schematic depiction of the invisibility-trigger manipulation for four conditions. In the 0.5 invisibility-trigger condition (A), each target became invisible when the toe marker on the relevant foot entered a circular region centered on the target and with a radius equal to 0.5 of the stride length (SL) needed to reach that target (0.5 SL). Similarly, in the 0.75 (B) and 1.0 (C) invisibility-trigger conditions, targets became invisible when the relevant foot entered within 0.75 SL and 1.0 SL, respectively. When the invisibility trigger was larger than 1.0, targets were made invisible when the relevant toe marker intersected the invisibility-trigger radius of the previous target. For example, in the 1.5 invisibility-trigger condition (D), target₄ became invisible when the toe was within 0.5 SL of target₃. Note that the invisibility-trigger manipulation was applied to target₃ through target₆ although, for clarity, only the trigger affecting target₄ is shown here.

The size of the circular invisibility trigger surrounding each target was manipulated by varying the proportionality constant between 0.5 SLs and 2.0 SLs in increments of 0.25. When the proportionality constant was less than 1.0 SLs, each target became invisible when the foot that was about to step on that target was in the swing phase. For example, in the 0.5 trigger condition, each target was programmed to disappear when the foot was halfway through the step to that target (Figure 2A). In the 1.0 trigger condition, each target was programmed to disappear at the moment of toe-off of the step toward that target (Figure 2C). When the trigger was greater than 1.0, it controlled the disappearance of the subsequent target. For example, in the 1.5 trigger condition, target₄ disappeared when the foot was halfway to target₃ (Figure 2D). There was also a double support trigger condition in which the upcoming target_N disappeared when a collision was detected between the subject's foot and the previous target_N−1.

Due to processing time and the network communication between the computer running the motion-capture software and the computer controlling the projector, there was an ∼80-ms lag in the system. As such, targets turned off ∼80 ms after the foot actually reached the location defined by the invisibility trigger. For example, if the invisibility trigger was set to 0.5, then the actual location of the foot when the target disappeared was closer to one fourth of a stride length from the target. The precise location depends on how fast the foot was moving during the 80-ms period, which varied from step to step. By comparing motion-capture data to the visibility logs from the projector system, we were able to estimate the actual location of the feet when the targets were rendered invisible. This estimate was used to determine the actual percentage of each step that the target was invisible. The values shown on the abscissa of the results figure (Figure 3) are the mean invisibility triggers after adjusting for lag.

Figure 3.

Scatterplot of every recorded step showing stepping error versus calculated (lag-adjusted) invisibility trigger. Markers are color-coded according to invisibility-trigger condition.

Analyses

We analyzed accuracy of steps to the last four of the six targets (targets_3–6). Data from the first two targets were excluded because invisibility triggers larger than 1.0 did not affect the visibility of those targets. Therefore, each trial yielded accuracy data from a total of four steps. Each of the 12 subjects completed 20 repetitions of each of the nine visibility conditions (full vision and the eight invisibility triggers), resulting in 80 analyzable steps per condition and 720 total steps per subject. In total, the final analyses in this study examined 960 steps per visibility condition for a grand total of 8,640 steps. One subject accidentally took an extra step when traversing the six targets, so data from that trial were excluded from the final analyses.

Our primary measure of stepping error was calculated by taking the root mean square of the Euclidean distance between the vertical projection of the marker at the second metatarsal head of the relevant foot and the center of the target. This variable provides a measure of overall stepping error that combines error due to bias and variability along both the anterior–posterior (AP) and medial–lateral (ML) axes. In addition, we also carried out separate analyses on the different components of overall error: variable error, which reflects variability in stepping, and constant error, which reflects systematic bias in stepping. Variable error and constant error were calculated along both the AP and ML axes. Variable error was calculated by taking the standard deviation of signed error along the AP and ML axes and indicates the degree of consistency in the placement of the feet relative to the target. Constant error was calculated by taking the mean signed error along the AP and ML axes. Positive and negative constant errors in the AP direction indicate bias to overstep and understep to targets, respectively. Similarly, positive and negative constant errors in the ML direction indicate bias to step too wide and too narrow, respectively.

Results

Figure 3 is a scatterplot showing overall error on the ordinate and invisibility trigger (adjusted for each individual step to account for system lag) on the abscissa for all the steps taken by the 12 subjects throughout the experiment. Markers are color-coded according to invisibility trigger condition. This figure illustrates the degree of variability in trigger location within each condition and that trigger locations were reasonably tightly clustered around the mean with little overlap between conditions. Figure 3 also illustrates that there was a general trend toward larger stepping errors in longer invisibility trigger conditions. We explore this effect in the next section.

Overall stepping accuracy

Figure 4A shows mean overall error in each invisibility trigger condition. Note that the values on the abscissa were obtained by taking the mean of the invisibility triggers after adjusting for lag for each condition. This is equivalent to taking the mean of the set of x-coordinates of each marker of a given color in Figure 3. A univariate repeated-measures ANOVA was conducted on overall error. Mauchly's test revealed that the sphericity assumption was violated, so a Huynh-Feldt correction was applied. The ANOVA revealed a significant effect of the invisibility trigger manipulation on overall error, F(3.72, 40.88) = 35.38, p < 0.01, η² = .76. Planned comparisons at the p < 0.05 level were performed to compare subjects' performance in each invisibility trigger condition to the full vision control condition: adjusted trigger size (p value), 0.26 (0.69), 0.46 (0.36), 0.70 (0.38), 0.94 (0.01)*, 1.04 (<0.01)**, 1.25 (<0.01)**, 1.43 (<0.001)***, 1.68 (<0.001)***.

Figure 4.

Results for stepping error in the different invisibility trigger conditions. (A) Overall stepping error measured in two dimensions. (B) The contribution of variable error in the AP dimension to overall error, and (C) the contribution of constant error (bias) in the AP dimension. (D and E) The contributions of variable and constant error, respectively, in the ML dimension. The invisibility-trigger values shown along the abscissa are the mean calculated (lag-adjusted) visibility for each step taken in that condition (i.e., the mean of the horizontal position of each colored dot in Figure 3). Bars show ± 1 SEM. Asterisks denote significant difference from the full-vision condition.

The findings reveal that visibility triggers that rendered targets invisible during the step toward that target (0.26, 0.46, and 0.70 triggers) had no significant effect on mean stepping accuracy but that stepping error began to increase when targets became invisible near the moment of toe-off to the target (0.94 trigger). Stepping error increased when the targets became invisible just prior to foot contact with the previous target (1.04 trigger), rising sharply when targets disappeared during the preceding swing phase (1.25, 1.43, and 1.68 triggers). This result suggests that visual information about a target foothold is primarily used prior to initiation of the step to that target. Rendering targets invisible after the moment of toe-off had a negligible effect on stepping accuracy, but stepping error increased significantly when targets became invisible before the step began.

Overall error provides a useful index of performance on the stepping task, but does not tell us about the individual contributions of variability and bias to error in the visual control of step length and step width. To further investigate the components of stepping error, we examined the effects of the invisibility trigger manipulation on variable error and constant error along the AP and ML axes.

Variable and constant error along the AP axis

The effect of the invisibility trigger manipulation on variable error along the AP axis (VE_AP) mirrored that of overall error (Figure 4B). There was no significant change in VE_AP when targets became invisible during the step to the target (0.24, 0.40, 0.70 triggers), a small increase when targets became invisible at the point of toe-off (0.94 trigger), and a sharp increase in VE_AP when targets became invisible during the previous step (1.04, 1.25, 1.43, 1.68 triggers).

Figure 4C shows the analysis of constant error along the AP axis (CE_AP) with positive and negative errors indicating overshooting and undershooting of the targets, respectively. The analysis revealed a small positive constant error (suggesting overshooting of targets) in the full-vision condition and the short invisibility-trigger conditions. When targets disappeared before the step was initiated, constant error was significantly less compared to the full-vision condition and switched signs (indicating undershoot) in the longest invisibility-trigger conditions. The fact that the positive constant error was greatest in the full-vision condition suggests a source of bias that is unrelated to the invisibility-trigger manipulation. Subjects were instructed to place the part of the foot beneath the marker on the second metatarsal head on the center of the targets. However, they may have found it more natural to place the center of the foot, which was slightly behind the location of the marker, on targets. Because stepping error was measured relative to the location of the marker, aiming the center of the foot at targets would result in a small positive constant error in all conditions, including the full-vision condition.

What might account for the negative constant error, suggesting a bias to undershoot targets, in the longer invisibility-trigger conditions? One possibility is that landing on targets required subjects to slightly extend their step length beyond the biomechanically preferred step length but that their biomechanical preferences were more apparent when targets disappeared earlier. When humans walk on flat, obstacle-free terrain and foot placement is unconstrained, their preferred step length is approximately equal to that which minimizes energetic expenditure for the given walking speed (Kuo, 2001). Of course, gait parameters such as step length can be modulated when the terrain is more complex to ensure accurate foot placement on safe target footholds. However, when visual information about target location is made unavailable before the step is initiated, the influence of the walker's biomechanical preferences may be more apparent.

To test this interpretation, we compared subjects' step lengths in each condition (including the target-free walking condition) to a theory-driven prediction of preferred step length. The prediction was based on the following equation, which was used by Kuo (2001) to capture the relationship between step length and walking speed:

s is unitless step length (normalized by leg length, l), and w is unitless walking speed (normalized by 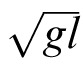, where g is gravitational acceleration). We used Equation 1 to predict subjects' biomechanically preferred step length from their walking speed in the various conditions. We then compared this predicted step length to the actual step lengths recorded in each condition.¹

Figure 5 shows the mean difference between subjects' actual step lengths in each condition and step length predicted by Equation 1. As expected, when targets remained visible or disappeared after the step to that target was initiated, the difference between actual step length and preferred step length was consistently greater than it was in the free-walking condition (indicated by the solid horizontal line in Figure 5). This reflects the demands of the task, which required subjects to slightly extend step length to land on the targets. However, when targets disappeared before the step was initiated (i.e., in the 1.04, 1.25, 1.43, and 1.68 trigger conditions), subjects' step lengths were as well predicted by the step length/walking speed relationship captured by Equation 1 as the steps they took in the free-walking condition. Thus, it appears that when visual information about the upcoming path is not available at the critical time, subjects' inability to adapt their gait to the upcoming terrain effected a more pronounced influence of their biomechanical preferences.

Figure 5.

Difference between actual and predicted step length in each invisibility-trigger condition. Predicted step length was based on Equation 1. Error bars show ± 1 SEM. Thick horizontal line corresponds to the difference in step length in the free-walking condition (dotted lines are ± 1 SEM).

Variable and constant error along the ML axis

The effect of the invisibility trigger manipulation on variable error along the ML axis was similar to that along the AP axis, in that the largest deviations from full-vision performance occurred when targets disappeared during the preceding step (Figure 4D). However, unlike error along the AP axis, there was a more consistent trend toward an increase in variable error in the conditions in which targets disappeared during the swing phase of the step to the target (0.46, 0.7, and 0.94 trigger conditions). Similarly, the analysis of constant error along the ML axis revealed a weak trend toward narrower steps in all but the 0.24 invisibility-trigger condition (Figure 4E). Although these trends did not reach statistical significance, the notable dissimilarity between these results along the ML axis and those along the AP axis are worthy of further consideration. We return to this issue in the Discussion.

Discussion

The findings of the present study differ from those reported by Reynolds and Day (2005b), who found that stepping accuracy degraded when visual information became unavailable after step initiation. We attribute the difference in findings to the fact that subjects in Reynolds and Day's experiment took a single step to a target and then stopped whereas the task in our experiment required subjects to step on a series of targets along a walking path. In the latter case, which is more representative of natural walking behavior, walkers may not be able to focus entirely on guiding the foot to the upcoming target because they must simultaneously adapt their movements to the terrain up to two steps ahead (Matthis & Fajen, 2013, 2014). In addition, when it is necessary to take more than a single step, walkers are better able to exploit the physical dynamics of bipedal walking when they can use visual information further in advance (i.e., during the preceding step; Matthis & Fajen, 2013).

The role of visual information during stepping versus reaching

It is well established that the ability to rapidly use visual feedback to adjust the trajectory of the effector plays an important role in the accuracy of reaching to a target with the hand (Carlton, 1992; Elliott & Allard, 1985; Zelaznik et al., 1983). Given the similarities between reaching and stepping (i.e., both require one to move an effector to a target with precision; Georgopoulos & Grillner, 1989), one might expect that visual information would be used in a similar manner to guide the trajectory of the foot. The findings of the present study suggest otherwise. The difference may reflect the dynamics of the limbs involved in the two tasks. Whereas each arm (including the hand) comprises just 5% of the mass of the human body, each leg (including the foot) comprises 16% (Winter, 2009). As such, changes to the trajectory of the body during locomotion involve far greater forces than those involved in reaching. Furthermore, the structure of the human body during walking (i.e., the inverted pendulum–like structure of the planted foot and the COM, and the pendulum-like structure of the swinging leg) facilitates a style of control in which visual information is primarily used to initialize the mechanical state of the body leading into a ballistic single-support phase. To our knowledge, there is nothing analogous about the structure of the arms and hands during reaching that would promote such a ballistic style of control.

The broader significance of this finding is that the way in which visual information is used, and most likely the nature of the visual information itself, is shaped not only by the task but also by the underlying biomechanical structure and physical dynamics of bipedal walking (i.e., the “intrinsic dynamics”). Given the success with which humans accommodate complex terrain, it would be easy to overlook the significance of the intrinsic dynamics and assume that they govern behavior only during walking over flat, obstacle-free terrain. However, the influence of the intrinsic dynamics during walking over complex terrain was revealed in the present study in the bias to undershoot targets in the longer invisibility trigger conditions (Figure 4C). When targets disappeared during the previous step (i.e., when the visual information was degraded), the influence of the underlying biomechanics was made apparent in the form of a walking speed/step length relationship that more closely approximated that displayed during unconstrained walking over target-free terrain (Figure 5). Thus, the intrinsic dynamics do not become irrelevant when the terrain demands visually guided gait modulations. Rather, such modulations are achieved by coupling information to parameters of the gait cycle, which itself emerges from the underlying biomechanics of bipedal locomotion.

Visual information and the control of step width

There was a weak trend suggesting larger variable error in step width and a small bias toward narrower steps even in the smaller invisibility-trigger conditions. Neither of these trends was statistically significant, but they stand out in contrast to both overall error and variable error in the AP direction, which showed little to no such error for conditions in which targets disappeared during the swing phase.

It is possible that these trends arise from an underlying difference in the control of foot placement along the AP and ML axes. Although walking is passively stable along the AP axis, there is evidence that active, sensory-driven regulation of step width may be necessary to ensure stability along the ML axis (Bauby & Kuo, 2000; Kuo, 1999; O'Connor & Kuo, 2009). If so, there may be a role for active feedback-driven regulation of step width even after the step has been initiated. This interpretation would suggest the existence of two modes of control operating to ensure accurate foot placement. One mode of control involves the use of visual information about the upcoming terrain to initialize the mechanical state of the body prior to toe-off so that the physical dynamics of the body will carry the walker along a desirable trajectory during the upcoming single-support phase. Such a control strategy would break down in conditions in which targets disappear during the step to the preceding target, leading to the increase in stepping error observed when the invisibility trigger was larger than 1.0. The second mode of control is purely active and relies on visual information to make minor adjustments to the trajectory of the foot to ensure proper placement along the ML axis. Because this mode of control would operate during the swing phase, its effectiveness would be compromised when information about target location is made unavailable during the step. This could account for the trend toward greater error along the ML axis in the short invisibility trigger conditions. Nonetheless, this is a post hoc explanation of a trend that did not reach statistical significance and, as such, must be viewed as speculative until further evidence can be obtained.

The critical phase for visual control of stepping

Let us now place the results of the present study, along with those of Matthis and Fajen (2013, 2014), in the context of the biomechanical analysis of walking presented in the Introduction. In this study, we found that visual information about target location is not necessary after toe-off of the step to the target. In Matthis and Fajen, we found that walkers need to see at least two step lengths ahead to walk at the same level of performance as they do when vision is unrestricted. Taken together, these two results suggest that the availability of visual information about the terrain near a particular step is most essential during the latter half of the preceding step, that is, the second half of step_N−1 is a critical phase for the visual control of foot placement onto a target at the end of step_N (Figure 6).

Figure 6.

Based on the results of the current experiment and the results reported in Matthis and Fajen (2013, 2014), it appears that the critical phase for the visual control of foot placement occurs during the latter half of the preceding step.

Interestingly, the critical control phase hypothesis can also be derived from the previously mentioned idea that walkers use visual information about the upcoming terrain to initialize each step in order to let the physical dynamics of their body carry them toward the desired foothold. Recall that the initialization of individual steps entails adjusting the two determinants of the passive trajectory of the COM: (a) the position of the planted foot, which defines the base of the inverted pendulum, and (b) the push-off force from the trailing leg, which defines the energetics of the COM leading into that step. Because both determinants are still under the walker's control during the last half of step_N−1, it follows that this is the phase of the gait cycle during which visual information about the terrain at step_N is most useful. Thus, the biomechanical analysis of walking and the empirical findings converge onto the same idea—that the critical phase for the visual control of step_N occurs during the latter part of step_N−1.

The notion of a biomechanically specified critical control phase provides a useful context within which to understand findings from other studies on the visual control of locomotion. Laurent and Thomson (1988) found that subjects were able to step to a single target without detriment when the target was only visible during the stance phase of the foot that would eventually step to the target (i.e., during the hypothesized critical control phase) but that stepping was more variable when the target was only visible during the swing phase. Furthermore, in a series of studies, Hollands and colleagues reported that stepping accuracy is robust to intermittent manipulations of visual information about the location of a target, especially when visual information is removed during the swing phase. They also found that subjects tended to step more accurately when they saccaded to the upcoming foothold while the foot to be moved was still in stance (Hollands & Marple-Horvat, 2001; Hollands et al., 1995), which is again consistent with the proposed critical control phase of the human gait cycle.

Interestingly, the critical control phase may also apply when locomotion is guided by nonvisual information. Blind individuals who are being taught how to walk with a cane traditionally learn the Hoover method (or two-touch method) for long cane use, which involves tapping the ground at the location of the upcoming foothold during the swing phase of the opposite foot (Kim, Emerson, & Curtis, 2009; J. Miller, 1967; M. E. Miller & Hoover, 1946; Wall, 2002). Such a technique would efficiently provide haptic information about the elevation, slant, and traction of the upcoming terrain precisely during the hypothesized critical control phase of the bipedal gait cycle.

Conclusion

We presented evidence that visual information about target location is not necessary after the step to that target has been initiated. This finding is conspicuously different from the literature on the visual control of reaching, which holds that visual feedback is used to fine tune the trajectory of the hand throughout the entire reaching movement. We attribute this discrepancy to the unique structure and organization of the human body during walking, which promotes an energetically efficient, ballistic style of control. Rather than making energetically costly midflight adjustments to the trajectory of the foot, walkers use visual information further in advance (i.e., during the critical control phase) to initialize the upcoming step so that the body can follow its natural trajectory to a safe foothold.