Mechanical Strength of the Side-to-Side Tendon Attachment for Mismatched Tendon Sizes and Shapes

Summary

Certain combinations are advised against in tendon transfers due to size or shape mismatches between donor and recipient tendons. In this study, ultimate load, stiffness and Young’s modulus were measured in two tendon-to-tendon attachments with intentionally mismatched donor and recipient tendons - pronator teres (PT)-to-extensor carpi radialis brevis (ECRB) and flexor carpi ulnaris (FCU)-to-extensor digitorum communis (EDC). FCU-EDC attachments failed at higher loads than PT-to-ECRB attachments but they had similar modulus and stiffness values. Ultimate tensile strength of the tendon attachments exceeded the maximum predicted contraction force of any of the affected muscles, with safety factors of 4x and 2x for the FCU-to-EDC and PT-to-ECRB constructs, respectively. This implies that size and shape mismatch should not be a contraindication to tendon attachment in transfers. Further, these safety factors strongly suggest that no postoperative immobilization of these attachments is necessary.

Introduction

After spinal cord injury, peripheral nerve injury and other upper motor neuron lesions, hand function can be improved by suturing appropriate donor tendons from functioning muscles into appropriate recipient tendons of paralyzed muscles (Fridén, 2005, Tsiampa et al., 2012). In contrast to the many surgical techniques reported to repair flexor tendon injuries (Boyer et al., 2005), there is relatively sparse objective information available about different tendon-to-tendon attachment techniques used for tendon transfer surgery. Such information as tendon-to-tendon attachment strength, suturing method, tendon dimensions, and possible tendon donor options are critical to enable surgeons to make informed decisions regarding repair and rehabilitation strategies. Recent studies in both human and animal tendons demonstrated the tremendous strength achieved by using a double-sided, back and forth running suture with 3 to 5 cm of tendon overlap, suggesting that immediate mobilization after these types of attachment is feasible (Brown et al., 2010, Tsiampa et al., 2012). Clinical application of this suturing approach to restore arm and hand function in tetraplegia confirmed its success in clinical outcome studies (Fridén and Reinholdt, 2008, Wangdell and Friden, 2012). Although, the feasibility of this type of suturing has been clearly demonstrated for the posterior deltoid tendon-triceps combination (Wangdell and Friden, 2012) and flexor digitorum superficialis-flexor digitorum profundus combination (Brown et al., 2010), other donor-recipient combinations can be envisioned that could provide more diverse options for surgical restoration. Unfortunately, these other combinations are sometimes not advised since there is often a mismatch between donor and recipient tendons in the form of size, shape or both. Therefore, in the current study, we measured the mechanical integrity (as indicated by ultimate tensile strength, elastic modulus and stiffness) of two tendon-to-tendon attachments in which the donor and recipient tendons were intentionally mismatched—pronator teres (PT)-to-extensor carpi radialis brevis (ECRB) and flexor carpi ulnaris (FCU)-to-extensor digitorum communis (EDC). The PT-ECRB transfer represents a flat donor into a round and solid recipient while the second represents a round donor and multiple flat recipient tendons. Both of these transfers are used commonly in tendon transfer surgery but little objective information about the mechanical integrity of these transfers exists in the literature. Therefore, the purpose of this investigation was to quantify the strength of transfers that have mismatched donor and recipient tendons.

Methods

Tendon harvest and repairs

Pronator teres (PT), extensor carpi radialis brevis (ECRB), flexor carpi ulnaris (FCU) and extensor digitorum communis (EDC) tendons were each harvested from one side (7 left, 3 right) of each of 10 formalin-fixed human cadavers (6 male, 4 female, average age 75 years) for a total of 40 tendons. PT tendons were taken from proximal to the musculotendinous junction to the radial insertion; ECRB tendons were taken from the musculotendinous junction to the radiocarpal joint; FCU tendons were taken from the musculotendinous junction to the insertion onto the pisiform; EDC tendons were taken from the musculotendinous junction to the extensor retinaculum.

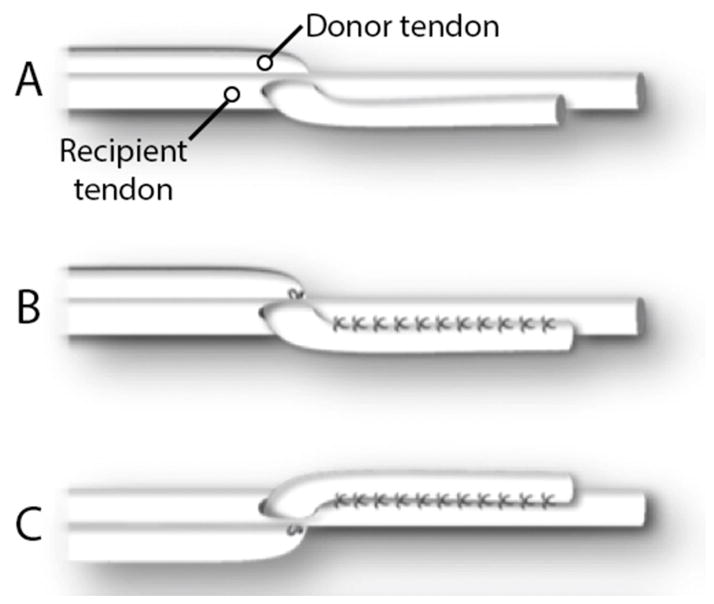
To simulate in vivo FCU to EDC transfers, the distal FCU tendon was placed at a 30 degree angle to the collection of EDC tendons, corresponding to the approximate angle at which an FCU tendon would lie if wrapped around the ulna to the dorsal compartment of the forearm. EDC tendons were individually incised at distally progressing locations (corresponding to the sites of overlap with FCU); incisions were parallel with the coronal plane in typical anatomic positioning. Approximately 3 cm of FCU tendon was passed through these incisions and a double looped knot was placed in each EDC tendon; these knots acted as stay sutures to keep the FCU tendon in proper position. After the FCU was positioned appropriately, it was wrapped, superficial to the EDC tendons, in an ulnar direction. It was sutured to the EDC tendons in a manner very similar to a suture method previously described (Brown et al., 2010). The general strategy underlying this suture method is summarized in Fig. 1. Briefly, using 3-0 Ti-Cron (Syneture, Covidien, Dublin, Ireland) one double looped knot with 3 throws was placed at the proximal end of the overlapping tendons. Ti-Cron was chosen because it is a commonly used in tendon transfer surgeries by the primary surgeon of this study (JF). The tail end was left long and the remaining suture was used to place 10 throws of running suture, progressing distally, at intervals of approximately 3 mm. After these 10 throws, another 10 throws were placed, in a distal to proximal fashion, overlapping the original 10 throws, forming 10 cross-stitches. The remaining suture was used to tie a final surgeon’s knot with the tail from the first knot. This sequence was performed on both the ulnar and radial sides of the tendon overlap (Fig. 2A).

Figure 1.

Generalized scheme of the side-to-side tendon attachment method. Stay sutures are placed at the proximal point of overlap (A). Running sutures are placed from proximal to distal and then overlapped from distal to proximal (B). Equivalent overlapping running sutures are placed on the other side of the construct.

Figure 2.

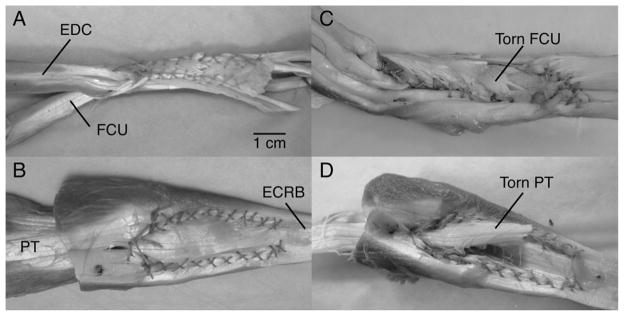
Photographs of the attachments of FCU-to-EDC (A and C) and PT-to-ECRB (B and D), in their intact states (A and B) and after mechanical loading (C and D). Note that failures occur by the suture effectively “splitting” one of the tendons, allowing it to migrate away from the other. Note also that the scale bar is approximate.

For suturing PT into ECRB, an incision was made in the proximal end of the ECRB tendon, near the musculotendinous junction. The distal end of the PT tendon was passed through this division in a manner that would reflect a “deep to superficial” path if these two tendons were at their in situ locations. The PT tendon was passed distally far enough such that approximately 3 cm of tendon were visible, superficial to the ECRB tendon. The suture method used was very similar to one previously described for tendon-tendon fixation (Brown et al., 2010) and for FCU to EDC transfer, as above (Fig. 2B). 10 of each construct type (FCU-to-EDC and PT-to-ECRB) were created for this study. All tendon attachments were performed by the same individual, a hand surgeon with extensive experience in tendon transfer surgeries, and more than 25 years of operative experience.

Cross sectional areas (CSA) of the tendons used in each construct were measured using digital calipers at the distal FCU (proximal to the attachment) and the proximal EDC (distal to the attachment). The anterior-posterior and medial-lateral dimensions of each tendon were measured and CSA was calculated as a rectangular area based on these measurements.

Mechanical properties of tendon constructs

Mechanical properties of the tendon transfer constructs were measured using a tensile testing machine (Instron Model 5565A; Instron, Norwood, MA) equipped with a 5,000 N tension/compression load cell. Sandpaper was glued to the instrument clamps and free tendon ends were wrapped in moist gauze to increase clamp purchase on tendons. A force of 1 kN was applied by the clamps to secure the tendons. The tendon repair testing protocol consisted of 5 consecutive preconditioning cycles of 5% strain followed by elongation at the relatively slow deformation rate of 10 mm/min (0.27%/s strain rate), until failure. The slow rate was chosen to emphasize the elastic rather than the viscous properties of the attachment and because it has been used previously to study the strength of tendon attachment techniques (Brown et al., 2010). Categories of failure modes were not predetermined because these attachment techniques have not been well studied. Thus, categories were determined based on observation and subjective grouping.

Data collection and analysis

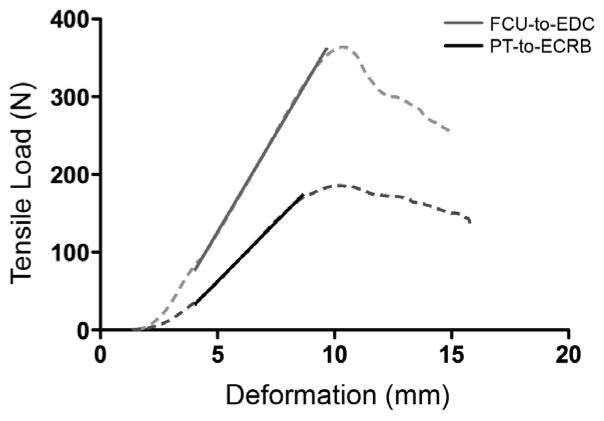
Constructs were alternately tested by attachment types; specimen order within each type was randomized. Main outcomes of interest were maximum load, modulus, and stiffness. Raw data collected from material testing were load, extension, and time. Construct failure was defined as a 30% drop relative to peak force; maximum load was the load associated with peak force. Stiffness and Young’s modulus were calculated from the linear region of the load-extension curve. This region was selected using a MATLAB (MathWorks, Natick, MA, USA) computer algorithm which defined the largest interval over which a first order polynomial fit yielded an r² value of greater than 0.999 (Fig. 3). Modulus is the quotient of the change in stress and the change in strain, and stiffness is the quotient of the change in force and the change in length.

Figure 3.

Sample load-deformation curves (solid) for FCU-to-EDC (blue) and PT-to-ECRB (red) attachments. The dashed black line overlaying each curve represents the period over which data were used to calculate modulus and stiffness values for the attachment constructs. Note that the FCU-to-EDC attachment is both stiffer and bears a higher ultimate load before failure.

Descriptive statistics of the morphology are given as mean and standard deviation, while experimental outcome parameters (load, modulus, stiffness) are reported as mean and standard error of the mean. Unpaired T-tests were used to compare values between the two constructs and significance level (α) was set to 0.05.

Results

Attachment Morphology

Morphologic measurements of the attachments are summarized in Table 1. Briefly, the ulnar side of the FCU-EDC attachments had 29.6 (1.1) mm overlap while radial side overlap was 30.4 (1.5) mm (Fig. 2A) and the angle formed between the donor and recipient averaged 28.9 (3.3) degrees. FCU CSA averaged 26.1 (10.3) mm² while EDC CSA values were 4.6 (1.8) mm² (index finger), 8.8 (4.7) mm² (middle finger), 5.8 (2.0) mm² (ring finger), 3.8 (2.2) mm² (small finger).

Table 1.

Morphologies of donor and acceptor tendons, as well as overlap measurements.

	FCU -> EDC		PT -> ECRB
Overlap (mm)
- Radial	29.6 (1.1)	- Total	30.8 (1.8)
- Ulnar	30.4 (1.5)
Overlap angle (deg.)	28.9 (3.3)
Cross sectional area (mm2)
- FCU	26.1 (10.3)	- PT	19.4 (5.7)
- EDC		- ECRB	32.8 (14.3)
- Index	4.6 (1.8)
- Middle	8.8 (4.7)
- Ring	5.8 (2.0)
- Small	3.8 (2.2)

PT-ECRB attachments had 30.8 (1.8) mm of overlap. PT CSA was 19.4 (5.7) mm² while ECRB CSA was 32.8 (14.3) mm², measured in the same manner as the FCU-EDC transfers. These morphological measurements were made to try to determine the basis for differences in ultimate tensile load (see Discussion).

Mechanical properties

Load-deformation curves showed the typical pattern observed for tendons (Butler et al., 1978), with a toe region at low deformation, a linear region and then a failure region (Fig. 3). Our algorithm was easily able to identify an extensive linear region from which modulus could be quantified (dotted lines in Fig. 3). This region was typically greater than 50% of the total load-deformation data set.

FCU-EDC attachments failed at a higher maximum load than PT-ECRB attachments (FCU-EDC: 338 (23.3) N, PT-ECRB: 185 (14.9) N, Fig. 4A, p<0.001). They had similar modulus (FCU-EDC: 50.3 (5.8) MPa, PT-ECRB: 44.7 (7.0) MPa, Fig. 4B, p=0.55, β=0.09) and stiffness values (FCU-EDC: 36.6 (3.0) N/mm, PT-ECRB: 33.7 (2.2) N/mm, Fig. 4C, p=0.45, β=0.11).

Figure 4.

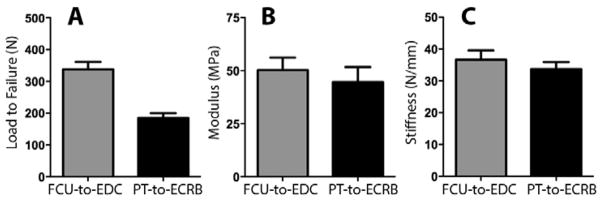
Maximum load (A), modulus (B) and stiffness (C) of the FCU-to-EDC (blue) and PT-to-ECRB (red) attachments. The FCU-to-EDC bears significantly higher loads (as indicated by the * symbol), but the two constructs have similar modulus and stiffness values. Error bars represent standard error.

Failure modes

In all cases, failure occurred at the attachment site in one of the tendons (no suture failures were observed; Figs 2C and 2D). In effect, the sutures (which stayed intact), “split” through one of the tendons, allowing it to slip and migrate away from the other while under load. Failure location was thus defined as the tendon in which a “split” was observed. Of the 10 FCU-EDC attachments, seven failed at the EDC, two failed at the FCU, and one showed failure in both tendons. Of the 10 PT-ECRB attachments, 9 failed at the PT while one failed in both tendons.

Discussion

The purpose of this paper was to determine the ultimate mechanical properties of tendon constructs created from two tendons whose sizes, shapes, and configurations were intentionally mismatched. It is important that such a test be performed because tendons mismatched in size and shape are often connected during surgical tendon transfers, and ultimate tensile strength guides specific post-operative remobilization and rehabilitation protocols. Indeed, tendon attachment mechanics strongly influenced the development of rehabilitation strategies after flexor tendon attachment (Boyer et al., 2005). Should tendon attachment strength be low, extensive immobilization time would be required to allow tendon healing to occur. However, potential complications of immobilization include loss of neural drive to the affected muscle (Gondin et al., 2004, Lundbye-Jensen and Nielsen, 2008) and tendon adhesions (Verdan, 1972). We have thus endeavored to create suturing methods that provide sufficient strength to allow rapid postoperative mobilization, as previously shown when we compared a traditional Pulvertaft weave to our so-called side-to-side (SS) method (Brown et al., 2010).

The current data demonstrated that the ultimate tensile strength of the mismatched SS construct exceeded the maximum predicted contraction force of any of the affected muscles. The maximal predicted tetanic force that a muscle can produce is calculated as the product of the physiological cross sectional area and the specific tension of the muscle. The PT and FCU have physiological cross sectional areas of 4.13 cm² (Lieber et al., 1992) and 3.42 cm² (Lieber et al., 1990), respectively. The specific tension for muscle, as determined in a guinea pig model, is 22.5 N/cm² (Powell et al., 1984). Based on these two factors, we calculate the maximum tetanic tension produced by the PT and FCU as 92.9 N and 77.0 N, respectively. The maximum predicted tetanic force of the PT is approximately 50% of the ultimate load borne by the PT-to-ECRB constructs in our study (185 N). We therefore state that that the PT-to-ECRB attachment provides a two-fold margin of safety. Equivalent analysis for the FCU demonstrates that its maximum predicted tetanic force (77.0 N) is approximately 23% of the load borne by the FCU-to-EDC constructs (338 N), which represents a 4X safety factor for the construct. There is, thus, virtually no chance that voluntary activation of the FCU or PT could disrupt these constructs. Given the limited ability of patients to voluntarily activate these muscles postoperatively, the safety factor for both PT and FCU strongly suggest that no postoperative immobilization of these attachments is necessary. Having used immediate postoperative mobilization under other tendon transfer situations (Wangdell and Friden, 2012), we recommend the use of SS suturing for tendon attachment after tendon transfer to permit immediate postoperative mobilization to counteract the detrimental effects of long term postoperative immobilization.

The results of this study in which the donor and recipient tendons were intentionally mismatched is relevant to hand surgery since mismatching is very common in tendon transfers. For example, brachioradialis (BR) has a small distal tendon, which is sutured into ECRB distal tendon, which is wide and thin, for C-6 spinal cord injury patients. Similarly, FCU, which has a round distal tendon, is sutured into an EDC, which is flat with multiple slips, in the case of radial nerve palsy. Finally, PT, which is flat, thin and wide, is often sutured into the thick ECRB for radial nerve palsy, representing another mismatch. In other words, it is almost impossible to avoid performing surgical reconstruction without considering mismatched tendons. The general issue is to define the donor muscle’s peak force (usually based on architecture), and the tendon construct strength. Based on this study and our previous study (Brown et al., 2010) we now know that average construct strength is about 250 N, which represents a greater force than can be generated by any muscle in the forearm (Lieber et al., 1990) (by way of comparison, the maximum contractile force of the biceps brachii is 100 N).

Origin of Construct Strength

Tendon attachment strength has been studied primarily in the case of the flexor tendon, where it has been shown that there are a great number of variables that contribute to the strength of the tendinous attachment, including suture technique and “number of throws (Boyer et al., 2005). While these factors are probably relevant for small digital flexor tendons, they do not explain the strength differences among our various constructs. All of our construct attachments had 10 cross-stitches (each composed of two throws) per side, and 20 per sample. We measured various aspects of our attachments to try to define which factors were related most to ultimate strength. For example, the construct thickness for PT-to-ECRB was about 70 mm² compared to the FCU-to-EDC, which had a thickness of only 60 mm². Thus, given the PT-to-ECRB attachment was half the strength of the FCU-to-EDC attachment this cannot explain the differential. It is also not the angulation, which could potentially produce shear stresses in muscle. There is no angulation in the PT-to-ECRB attachment, but there was a 30° angulation with the stronger FCU-to-EDC construct. Similarly, the overlap ratio in both the proximal and distal attachment sites was higher with the FCU and, therefore, overlap areas do not simply explain these discrepancies. At this point, we suggest that the discrepancies are due to the fact that the FCU tendon was wrapped around the EDC and this may perhaps provide a stronger interface. At this point, this is purely conjecture.

Limitations

While we made every effort to use these cadaveric samples to perform clinically relevant research, there are a number of limitations in this study. Most importantly, while we intentionally mismatched tendon shapes in the two constructs studied with the mismatch including size, shape, relative orientation, donor-recipient anatomy and construct geometry, we did not test every possible combination of these variables. There are three main factors that represent potential for mismatch between tendons - size, number of slips in the recipient tendon, and the presence or absence of folding. Varying these parameters corresponds to eight possible combinations, two of which we have tested here. FCU-to-EDC represents a combination of size mismatches, multiple slips in recipient tendon, and presence of folding. PT-to-ECRB represents a combination of size mismatch, a single slip in the recipient tendon, and absence of folding. It is thus possible that a unifying theme will emerge when other combinations are tested. Also, our suggestion that postoperative immobilization may not be necessary only addresses immobilization as a means of protecting the patient from damaging attachment sites from force generated by their own muscles. A role for immobilization as protection from external or extrinsic forces may still exist, depending on patient compliance.

In addition, in this study, as well as previous biomechanical studies carried out using cadaveric tissue, the effects of tendon healing and changes that may occur based on tendon suturing are not considered. Further, strength of transfers is a function of tissue strength and suture strength. No suture failures were observed in this experiment but results may not be applicable to repairs conducted with smaller suture sizes, weaker suture types, or rapidly absorbing suture types. Lastly, the tissue used in these studies was obtained from fixed specimens. In our previous study of the digital flexors (Brown et al., 2010), the tendons were obtained from fresh-frozen specimens. Therefore, extrapolation of our results to clinical scenarios must take into consideration the effects of tissue fixation on its response to mechanical load. One study (Viidik and Lewin, 1966) summarizes a body of work, carried out in the field of leather chemistry, that examines the effect of formaldehyde on mechanical properties of tendons, both human and animal. The findings of these studies fell into two categories: formaldehyde-fixed tendons exhibited decreased tensile strength or no significant changes in tensile strength. Among the six studies that were reviewed, none found that formaldehyde fixation enabled tendons to bear higher loads that native tissue. This supports our claim that these constructs may be used in conjunction with immediate postoperative mobilization. At worst, our tendon strengths in this study are not significantly different from normal tendons; at best, our formaldehyde-fixed tendons are weaker than normal ones so in vivo, these attachments would likely be able to bear even more load.