Changing Material Properties of the Tree Shrew Sclera During Minus Lens Compensation and Recovery

Abstract

Purpose.

To estimate two collagen-specific material properties (crimp angle and elastic modulus of collagen fibrils) of the remodeling tree shrew sclera during monocular −5 diopter (D) lens wear and recovery.

Methods.

Tensile tests were performed on scleral strips obtained from juvenile tree shrews exposed to three different visual conditions: normal, monocular −5 D lens wear to induce myopia, and recovery. Collagen fibrils are crimped in the unloaded sclera and uncrimp as the tissue stiffens under load. Inverse numerical analyses were performed to estimate the (unloaded) crimp angle and elastic modulus of collagen fibrils using a microstructure-based constitutive model.

Results.

Compared with the control eye, the crimp angle was significantly higher in the treated eye after 2 days and remained significantly higher until 21 days of lens wear (P < 0.05). The difference between the crimp angle of the treated and control eye rapidly vanished during recovery in concert with the changes in axial elongation rate. A rapid and extensive increase in the elastic modulus was seen in both eyes after starting and stopping the lens wear.

Conclusions.

The estimated change in the crimp of scleral collagen fibrils is temporally associated with the change in axial elongation rate during myopia development and recovery. This finding suggests that axial elongation may be controlled by a remodeling mechanism that modulates the collagen fibril crimp. The observed binocular changes in scleral stiffness are not temporally associated with the axial elongation rate, indicating that scleral stiffening may not be causally related to myopia.

We have identified transient changes in the material properties of tree shrew scleras during induced myopia and recovery. The results suggest that axial elongation may be controlled by a remodeling mechanism that modulates the collagen fibril crimp.

Introduction

Myopia (“nearsightedness”) is the most common type of refractive error affecting approximately 40% of the US adult population¹ and more than 80% of some Asian populations.^2,3 High myopia¹ is associated with an increased risk of blinding diseases such as glaucoma.^1,4–6 A myopic eye is too long for its current focal length, which is typically characterized by an elongated posterior scleral shell.⁷ The purpose of this article is to gain further insight into the remodeling mechanism underlying scleral elongation in the mammalian eye by estimating the changing collagen-specific material properties of tree shrew scleras during negative power lens-induced myopia and recovery from myopia.

There is increasing evidence from animal studies in support of an active emmetropization mechanism that involves active growth and remodeling of the sclera to match the eye's axial length to its optical power, producing eyes with retinal focus (emmetropia).^8–11 Here, we define growth as a mechanism that changes the amount (volume) of scleral tissue. We define remodeling as a mechanism that involves internal changes in the scleral extracellular structure, such as collagen sliding or realignment. The literature around the field of myopia often does not distinguish between scleral growth and remodeling as an underlying mechanism controlling axial elongation. However, deciphering the underlying mechanisms will be critical to design an effective myopia treatment.

The structure of the sclera, as well as the mechanisms used to adjust the axial length of the eye, varies across species. We use the tree shrew model of myopia, which has a fibrous sclera that is similar to humans. The fibrous sclera of tree shrews, humans, and other mammals is mainly composed of interwoven lamellae of type I collagen.^12–15 Its extracellular matrix contains elastin, proteoglycans (PGs), and glycosaminoglycans (GAGs). Surprisingly, the tree shrew sclera contains aggrecan, a proteoglycan normally found in cartilage. Aggrecan also is present in the human sclera, but its role in scleral biomechanics or myopic remodeling is still unclear. Induced myopia in the tree shrew (by either using a negative power lens or a diffuser) has been shown to impact the sclera by scleral thinning (Norton TT, Kang RN. IOVS 1995;36:ARVO Abstract 760); reduction in dry weight (3%–5%)^16,17; lower hyaluronan and sulfated GAG levels¹⁷; numerous changes in gene expression,^18–21 including upregulation of matrix metalloproteinase (MMP)-2 and membrane-type (MT)1-MMP, and downregulation of tissue inhibitor of metalloproteinase (TIMP)-3 and aggrecan; and a higher creep rate.²² One would expect a higher dry weight, lower MMP levels, and higher TIMP levels if a growth mechanism is driving scleral elongation by synthesizing more tissue matter. The reported findings show the opposite, pointing toward an active remodeling mechanism that leads to scleral elongation of the mammalian eye due to alterations in tissue composition and material properties. Note that the sclera of avians, such as the chick, consists of two layers: a mammal-like fibrous layer and a cartilage layer, which contains collagen type II and large PGs. Although similar responses have been observed in the fibrous sclera, the axial length of the chick eye seems to be mainly controlled by a growth mechanism, which modulates the growth and differentiation of the scleral cartilage layer in the posterior pole.^23–25

Inverse computational models were developed to estimate the crimp angle and elastic modulus of collagen fibrils from load-displacement measurements performed on scleral strips. Collagen fibril crimping is a well-known phenomenon that occurs when collagenous soft tissues are unloaded.^26–29 Tensile loading of the tissue leads to uncrimping and straightening of the collagen fibrils, which in turn leads to a typical nonlinear stiffening response of the tissue. We have previously proposed a computational model for crimped collagen fibrils³⁰ that has been used to investigate the biomechanical response of the sclera, cornea, lamina cribrosa, and the heart.^31–37 We use this model in the present study to estimate the changing collagen-specific material properties of the tree shrew sclera during minus lens compensation and recovery. The computational model was fitted to load-displacement data of scleral strips, which were collected alongside our previously published creep experiments.²²

Mechanical testing protocols for scleral tissue vary greatly in the literature. Some protocols include 3 to 20 load cycles to reach a repeatable experimental state,^38–42 whereas in other studies no preconditioning is performed.^22,43–46 Geraghty et al.³⁹ have shown that repeatable results can be obtained after two to three load cycles for the human sclera using uniaxial strip test. Coudrillier et al.,⁴⁷ Tong et al.,⁴⁸ and Myers et al.⁴⁹ have shown that the mechanical response of human, porcine, and bovine sclera exhibits minimal preconditioning effects during inflation testing. The preconditioning effect is characterized by a softening response of the tissue, which depends on the load history.^50–53 The preconditioning effect in tree shrew sclera is unknown, so cyclic load-displacement tests were performed at physiological and supraphysiological loads.

In addition, an axisymmetric computer model of the tree shrew eye is presented in this article to investigate the potential implication of the changing material properties on the axial length of the eye.

Methods

Approximately half the data used in this study originates from our previous work on the visual-guided regulation of the scleral creep.²² Additional data were collected for this study, where the additional experimental groups were treated and measured following exactly the same protocols. Consequently, the description of our experimental procedures is presented in a condensed form in the following and we refer to our previous publication for detailed descriptions.²²

Subjects

Subject data of 49 tree shrews (Tupaia glisbelangeri) were used here. The shrews were bred and raised in a colony at the University of Alabama at Birmingham in accordance with The American Association for the Advancement of Laboratory Animal Care guidelines. The subjects used in this study were exposed to three different visual conditions: (1) normal development (24, 28, 35, and 45 days of visual experience (VE, days after natural eyelid opening at ~19 days of age; n = 3 per group); (2) monocular −5 diopter (D) lens wear to induce axial elongation and myopia (1, 2, 4, 11, and 21 days of lens wear; n = 5 per group); and (3) recovery from the myopia (1, 2, 4, and 10 days of recovery with no lens after 11 days of lens wear; n = 3 per group). The first day of lens treatment in experimental groups 2 and 3 was performed at 24 days of VE, where VE days were counted once the lids of both eyes were fully opened. Axial length was measured using A-scan ultrasonography.^14,54,55 The 1-day myopia group and the recovery groups represent new data, whereas the data of the remaining experimental groups originates from our previous publication.²² The same protocols were followed for the new experimental groups. A detailed description of the subject care and the experimental techniques, including the goggle system and ocular measurements can be found in our previous publications.^{14,22,54–56} In addition to the above-mentioned subjects, five normal subjects (28 VE) were used to study the preconditioning effect of the juvenile tree shrew sclera.

Tissue Preparation

Both eyes were enucleated after the animal had received a lethal injection of nembutal anesthesia. A 3-mm-wide × approximately 15-mm-long strip was cut from the posterior sclera approximately 1 mm away from the optic nerve head of each tree shrew eye (axial length ~8 mm) using a razor blade jig. The strips were cut at a similar orientation (approximately superior-inferior) to minimize any difference due to local differences in tissue anisotropy (Fig. 1).²² To obtain the thickness of the strips, each strip was placed in a pool of phosphate-buffered saline (PBS) between two coverslips. The thickness was estimated using a microscope (Orthoplan, Leitz, Wetzlar, Germany) by measuring the distance between the top surface of the bottom cover slip and bottom surface of the top coverslip.²²

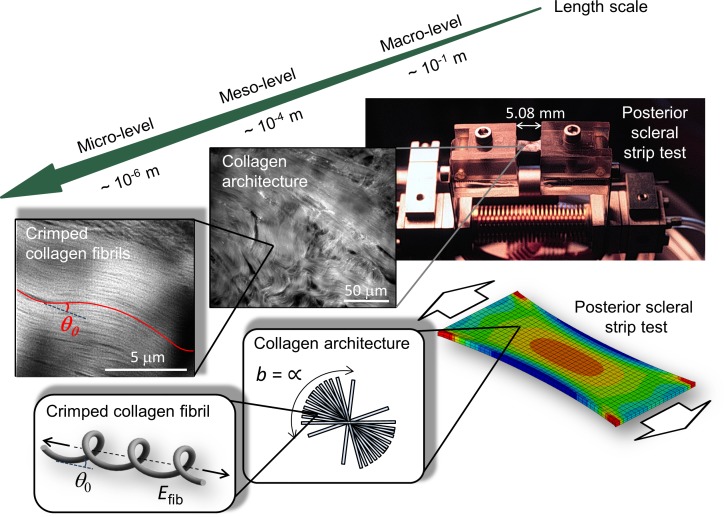
Figure 1.

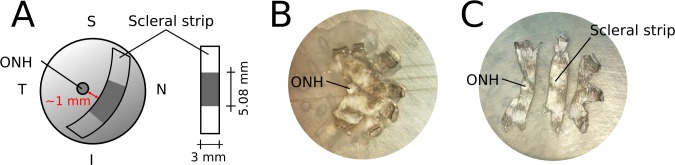
(A) Schematic of the location and orientation of the scleral strips used for mechanical testing. Each strip was approximately 15 mm long, where 5.08 mm was the initial clamp-to-clamp distance. The width of the strips was 3 mm. (B, C) Images of a flattened posterior scleral shell before (B) and after the strip was cut (C).

Mechanical Testing

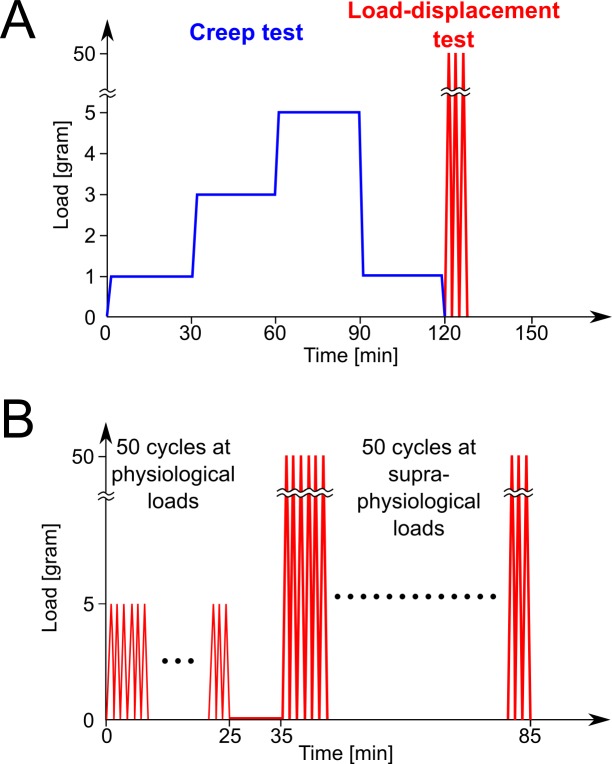
Uniaxial tensile testing was performed on each scleral strip using a Vitrodyne V-200 material tester (Liveco, Inc., Burlington, VT, USA). The load and deformation resolutions were 0.01 g and 2.5 μm, respectively. To minimize any clamping-related prestress of the tissue, each unloaded strip was placed on a platform floating in PBS while it was carefully mounted at a known initial clamp-to-clamp length of 5.08 mm (Fig. 2, top right). Once the strips were clamped, the platform was removed and mechanical testing was performed in horizontal position while the strips remained immersed in PBS. Both eyes of one subject were consecutively tested within 4 hours postmortem. The sclera that was tested second was kept in PBS at 4°C. Mechanical testing was performed at room temperature according to Load Protocol A (Fig. 3A). First, a creep test was performed, where the strips were subjected to 1, 3, and 5 g of tension for 30 minutes at each tension. The results of this creep test were presented and discussed in a previous publication.²² The scleral strips were allowed to rest at 1 g for 30 minutes at the end of the creep test, before the load-displacement test was performed. The load-displacement test consisted of three cycles of loading and unloading from 0 g to 50 g and back to 0 g in 60 seconds. The data from the load-displacement test were used in this study for inverse computational analysis. Supraphysiological loads were applied during the load-displacement test to ensure that the linear region of the stress-strain response was included in the data. The inclusion of linear region is essential, as we aim fit the collagen-specific material properties that typically dominate the linear region of the stress-strain response at high loads.⁵⁷ All scleral strips were processed and mechanically tested identically. The only difference between the samples was the treatment condition of the different experimental groups outlined before.

Figure 2.

Tissue structures at multiple scales in the experimental (top) and computational model (bottom). Macro-level: The posterior scleral strip clamped into the uniaxial tensile testing device and the finite element model of the scleral strip test. Meso-level: The collagen architecture showing a random alignment of scleral lamellae in the plane of the sclera, which is represented by a von Mises distribution with concentration parameter b = ∞ in the computer model. Micro-level: Crimped collagen fibrils in the unloaded tree shrew sclera and in the computational model.

Figure 3.

Experimental Load Protocols A and B. (A) First, creep tests were performed (30 minutes each) at 1, 3, and 5 g. At the end of the third creep test, the loading was reduced to 1 g for 30 minutes and three subsequent load-displacement tests were performed from 0 to 50 g at 60 seconds per cycle. The results of the creep test were previously published.²³ Only the loading path of the load-displacement tests were used in this study. All strips that were tested under Load Protocol A were processed and mechanically tested in the same way. (B) To investigate the preconditioning effect of the tree shrew scleral strips, 50 load-displacement cycles were performed at physiological (0 to 5 g, 30 seconds per cycle) and subsequently at supraphysiological loading conditions (0 to 50 g, 60 seconds per cycle). All strips that were tested under loading Protocol B were processed and mechanically tested in the same way.

Preconditioning

Five scleral strips of five normal animals at 28 days of VE were used to study the preconditioning effect of the juvenile tree shrew sclera. The tissues were processed, clamped, and mechanically tested as described above except that Load Protocol B was applied (Fig. 3B). Cyclic uniaxial tensile tests were performed first at physiological loads (50 cycles, 0–5 g, 30 seconds per cycle) and, subsequently, after 10 minutes rest, at supraphysiological loads (50 cycles, 0–50 g, 60 seconds per cycle). The preconditioning effect was studied by calculating the strain at maximum load (ε_max) at each cycle and how this strain changes from one load cycle to the subsequent load cycle (Δε_max). Generalized estimating equation models were constructed to estimate the mean trend of the cyclic change in strain at physiological and supraphysiological loads. A repeatable and preconditioned sate was defined as the cycle at which the mean cyclic change in strain diminishes (reaches zero).

Inverse Computational Analysis

A multiscale computational model was generated for each strip test (Fig. 2) that was tested under Load Protocol A. The computer model was fitted to the loading curve of each load cycle independently. The computer model consisted of a finite element mesh representing the macrostructure of the strip. The mesh was composed of 400 quadratic hexahedral elements of serendipity-type. Please note that only one-fourth of the model shown in Figure 2 (bottom right) was used in the calculation, accounting for the symmetry of the boundary value problem. We used our microstructure-based, hyperelastic constitutive model to account for the hierarchical collagen structure of the sclera.^30,35,58 At the microscale, collagen fibrils are assumed to crimp and buckle when the sclera is unloaded. When tension is applied to the sclera, this crimp is removed and the sclera stiffens as the collagen fibrils straightened. At the mesoscale, collagen fibrils form scleral lamellae that are strongly interwoven. We assume that the lamellae are randomly oriented tangential to the scleral surface. The random orientation of the scleral lamellae are represented by a von Mises distribution with concentration parameter b = ∞ in the computer model. We assume that the scleral lamellae are embedded in an isotropic tissue matrix, which represents all noncollagenous tissue components (e.g., elastin, GAGs, PGs, cells, and fluids). The matrix is mostly composed of tissue water and therefore assumed to be isotropic and incompressible, and its elastic contribution described by the shear modulus μ and bulk modulus. Nearly incompressible deformation behavior is achieved by setting the bulk modulus to 1000 times the value of the tissue's shear modulus. The constitutive model consists of four unknown parameters: the elastic modulus of collagen fibrils E_fib; the crimp angle of collagen fibril in the unloaded sclera θ₀; the ratio between the crimp amplitude and the fibril cross-sectional radius R₀/r₀; and the shear modulus of the sclera μ. We applied the experimentally recorded deformations as boundary conditions to the computer model and computed the reaction forces at the clamps. The four model parameters were independently fitted for each load cycle by minimizing the sum of squared residuals between the computationally predicted and experimentally measured boundary forces. The fitting was performed by using the Nelder-Mead simplex algorithm, which is freely available through the Open Source Library of Scientific Tools (SciPy). We varied the initial guess of unknown model parameters over a wide range of values (E_fib = [0.1, 300.0 MPa]; θ₀ = [0.1°, 50°]; R₀/r₀ = [1.0, 50.0]; μ = [0.0001, 1.0 MPa]) to verify that the fitting algorithm converged to the global minimum. Detailed derivations of the constitutive model and its implementation into a finite element code can be found in our previous publications.^30,35,58

Statistical Analysis

Paired and unpaired t-tests between experimental groups were performed to analyze the effect of the −5 D lens and the recovery from the lens on the material parameters. We observed continuous cyclic softening of the juvenile sclera with repeated load cycles. To minimize the impact of the experimentally induced softening, the fitted material parameters of the first load cycle were used for the statistical group tests.

Predictive Computer Simulation

To investigate the potential impact of the changing material properties on the axial length, an axisymmetric computer model of the tree shrew eye was proposed. The corneo-scleral geometry and thickness of the computer model was approximated by using histological measurements of normal tree shrews.⁵⁹ The computer model was subjected to 15 mm Hg IOP using our previously published prestressing method.³⁴ Within the same simulation, the material properties of the posterior sclera were changed from the values obtained for the control eye to the values of the treated eye while keeping IOP constant.

Results

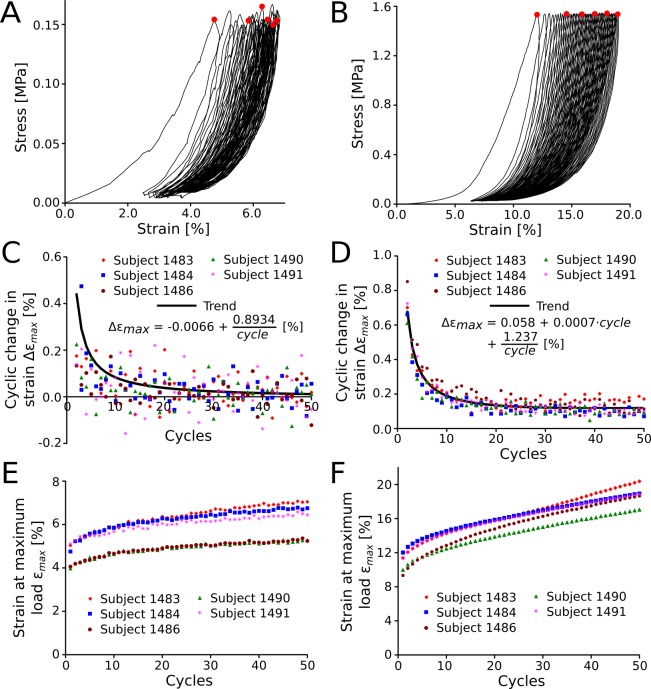
The results of the investigation of the preconditioning effect (Load Protocol B) are shown in Figure 4. The cyclic stress-strain response at physiological (Fig. 4A) and supraphysiological loading conditions (Fig. 4B) are plotted for one representative subject. The cyclic change in strain (Δε_max) at physiological and supraphysiological loading conditions is plotted for all five subjects in Figures 4C and 4D, respectively. Several nonlinear models with linear coefficients were tested to fit the average cyclic change in strain. At supraphysiological loading conditions, all scleral strips were characterized by a continuous cyclic softening response throughout all cycles. The average cyclic change in strain Δε_max was best described by the function 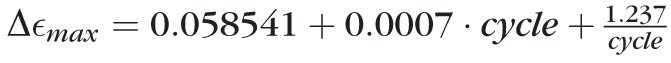[%] (P < 0.05 for all coefficients). The cyclic change in strain decreased during the first 42 cycles followed by a slow increase for subsequent cycles. This result suggests that the juvenile tree shrew sclera never reaches a repeatable load-displacement cycle and, therefore, cannot be preconditioned at supraphysiological loading conditions using strip testing. At physiological loading conditions, the average cyclic change in strain was best described by the function 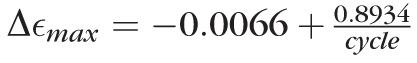[%] (P < 0.05 for all coefficients), suggesting that a preconditioned state (Δε_max = 0) is reached after 135 cycles. Figures 4E and 4F show the strain at maximum load (ε_max) for physiological and supraphysiological loading conditions, respectively. At physiological load, the maximum strain increased with increasing cycles as the tissues precondition while the variation of ε_max between the different subjects remained mostly constant (Fig. 4E). This was not the case at supraphysiological loads, where subject-specific tissue damage seemed to cause variable trends of ε_max with increasing cycles (Fig. 4F).

Figure 4.

Preconditioning response of the juvenile tree shrew sclera using Load Protocol B. Representative stress-strain curves of one subject (1484) are shown for 50 load cycles at physiological (A) and supraphysiological loading conditions (B). The red circles mark the maximum stress value of load cycles 1, 10, 20, 30, 40, and 50. The change in strain at maximum load from one load cycle to the subsequent load cycle is shown for physiological (C) and supraphysiological loading conditions (D). At supraphysiological loading conditions, the juvenile tree shrew sclera undergoes continuous cyclic softening throughout all load cycles (B, D). Substantial softening also is seen at physiological loading conditions (A, C). The strain at maximum load shows similar variability between subjects throughout all cycles at physiological loads (E) but variable trends at supraphysiological loading conditions (F).

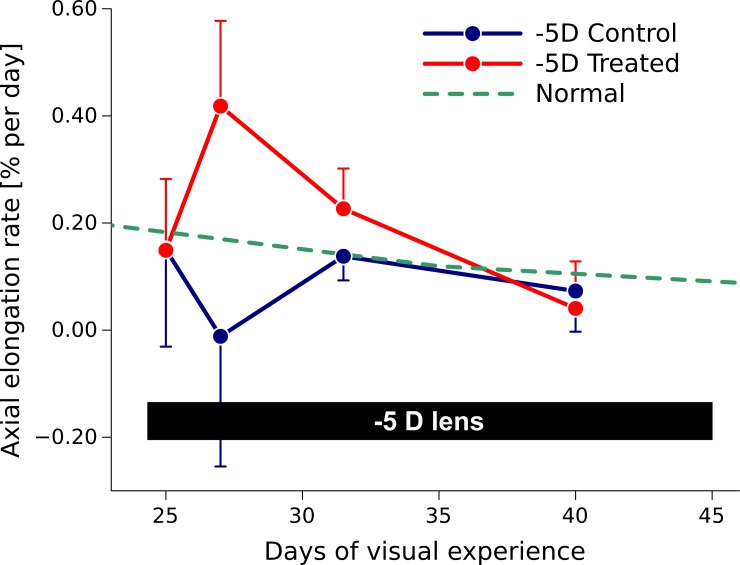
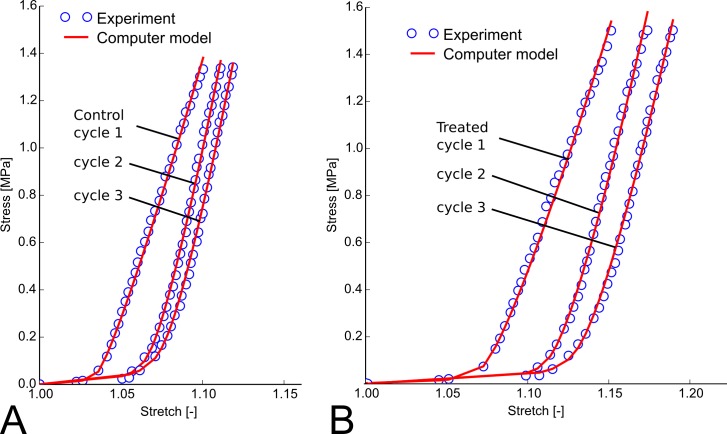
As reported before,²² the −5 D lens induced axial elongation in the treated eye, where the axial elongation rate increased rapidly during the first 3 days of lens wear and decreased to normal values as the eye adapted its axial length to the lens (Fig. 5). Figure 6 shows typical stress-stretch curves of a treated and control eye after 4 days of −5 D lens wear using Load Protocol A. The computational model matched the experimental stress-stretch curves well. The curves of the treated eye appeared shifted to higher stretch levels. For both eyes (treated and control) the stress-stretch curve shifted to higher stretch levels with increasing cycles as seen in the preconditioning experiment. Figure 7 shows the differences in the fitted elastic modulus and crimp angle of collagen fibrils between the load-displacement cycles of Load Protocol A using all investigated scleras including normal, control, and treated eyes. The elastic modulus of collagen fibrils increased from cycle 1 to 2 but remained in average unchanged from cycle 2 to 3. The increase in elastic modulus from cycle 1 to 2 was linearly dependent on the initial value of the elastic modulus at the first cycle. In contrast to the elastic modulus, the crimp angle of collagen fibrils increased throughout the three cycles due to the continuous softening of the tissue with increasing cycles. The increase in crimp angle from cycle 1 to 2 was higher for smaller values at the first cycle.

Figure 5.

Axial elongation rate in normal animals and animals in which one eye was treated with a −5 D lens (−5 D Treated) while the other eye was left as control (−5 D Control). The axial elongation rate increased rapidly in the treated eye, and then gradually decreased to normal values as the eye adapted to the −5 D lens (data reproduced from Siegwart and Norton²³).

Figure 6.

Typical stress-stretch curves showing the three loading cycles (Load Protocol A) of the control (A) and −5 D lens–treated sclera (B) of one animal after 4 days of −5 D lens wear (lens was worn from 24 to 28 days of VE). The computational model was fitted to each loading curve independently. The computational fits (red lines) matched the experimental measurements (blue circles) well. With increasing load cycles, the stress-stretch curves of both, the control and treated sclera, shift to the right suggesting that the tissue undergoes cyclic softening. The stress-stretch curves of the treated sclera were shifted to the right showing a more pronounced toe region compared with the control tissue.

Figure 7.

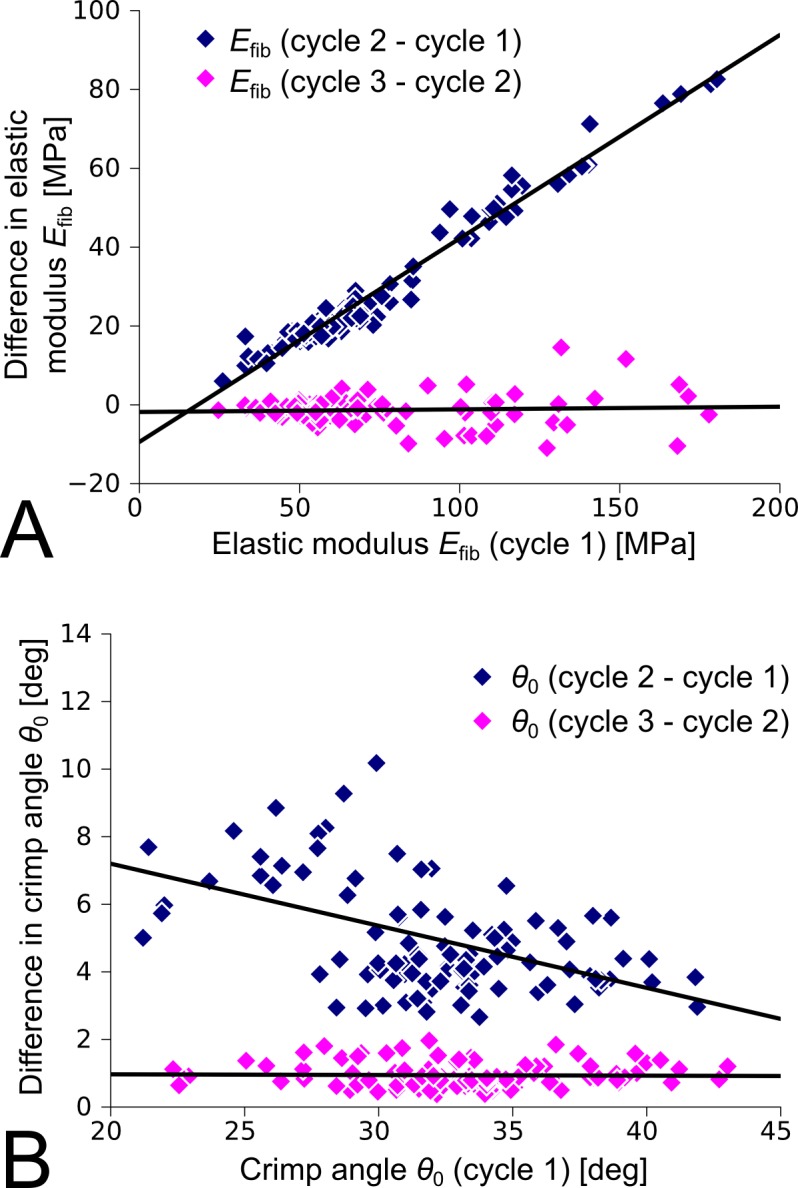
Differences in the material parameters between load-displacement cycles of all investigated scleras (normal, control, and treated) tested under Load Protocol A. (A) The elastic modulus of collagen fibrils increased from cycle 1 to 2. This increase was higher for higher elastic moduli at cycle 1. No further changes were seen between cycle 2 and cycle 3. (B) The crimp angle of collagen fibrils increased from cycle 1 to 2. This increase was lower for higher values at cycle 1. The crimp angle further increased from cycle 2 to 3, suggesting continued softening of the tissue as observed in Figure 4.

The Table summarizes the four fitted model parameters (mean, SD) of load cycle 1 for each experimental group. The fitted shear modulus μ was very low (0.0001–0.039 MPa, 5th–95th percentile) in all fitted eyes compared with mature to elderly human scleras³⁵ (0.11–0.71 MPa, 5th–95th percentile). The low shear modulus reflects the very low shear stiffness of the juvenile tree shrew sclera. The shear modulus μ and the ratio R₀/r₀ (2.1 to 18.2, 5th–95th percentile) showed no significant differences due to treatment or cycling loading and were therefore not further investigated here.

Table. .

Fitted Model Parameters for Load Cycle 1 of Load Protocol A (Mean, SD)

Experimental Group	Day of VE	θ0, deg	Efib, MPa	μ, kPa	R0/r0, −
Normal (L&R)	24	27.2 ± 6.3	29.4 ± 2.0	9.8 ± 8.4	8.1 ± 4.7
	28	31.1 ± 2.8	39.8 ± 3.3	8.8 ± 2.1	11.0 ± 2.4
	35	25.0 ± 5.6	37.1 ± 5.2	23.4 ± 34.7	8.7 ± 3.3
	45	20.1 ± 6.6	34.4 ± 3.2	43.8 ± 83.3	5.8 ± 3.1
−5 D control	25	28.7 ± 1.1	68.5 ± 12.6	7.1 ± 4.8	12.9 ± 5.6
	26	30.5 ± 3.0	44.6 ± 10.7	8.9 ± 2.4	10.2 ± 1.3
	28	23.3 ± 5.3	40.4 ± 4.2	27.0 ± 27.1	7.5 ± 4.6
	35	23.9 ± 5.1	38.9 ± 5.7	20.6 ± 15.5	10.2 ± 9.9
	45	20.2 ± 7.1	35.4 ± 4.2	14.2 ± 6.6	4.3 ± 3.6
−5 D treated	25	27.5 ± 2.4	82.2 ± 18.3	5.0 ± 3.6	11.4 ± 2.3
	26	34.0 ± 3.0	45.7 ± 4.2	5.8 ± 4.0	12.3 ± 3.0
	28	31.3 ± 6.6	38.5 ± 5.5	10.0 ± 4.3	8.8 ± 3.1
	35	29.1 ± 4.5	35.2 ± 5.5	25.3 ± 24.6	21.8 ± 18.6
	45	22.8 ± 7.5	35.8 ± 6.2	13.6 ± 15.7	9.8 ± 12.3
Recovery control	36	27.9 ± 2.2	71.9 ± 6.6	2.8 ± 4.6	10.5 ± 1.2
	37	28.2 ± 1.4	68.7 ± 15.9	6.0 ± 5.3	12.1 ± 2.5
	39	28.2 ± 1.0	60.8 ± 0.8	6.5 ± 5.3	11.2 ± 0.8
	45	26.2 ± 3.3	47.3 ± 5.3	7.9 ± 6.7	7.8 ± 0.9
Recovery treated	36	30.2 ± 3.6	59.5 ± 17.0	5.3 ± 5.8	11.5 ± 1.5
	37	30.0 ± 2.3	55.5 ± 5.2	15.0 ± 7.4	11.9 ± 2.6
	39	29.3 ± 0.2	55.3 ± 7.1	5.3 ± 4.6	10.9 ± 2.1
	45	25.2 ± 1.1	42.0 ± 2.5	9.3 ± 2.5	7.4 ± 0.7

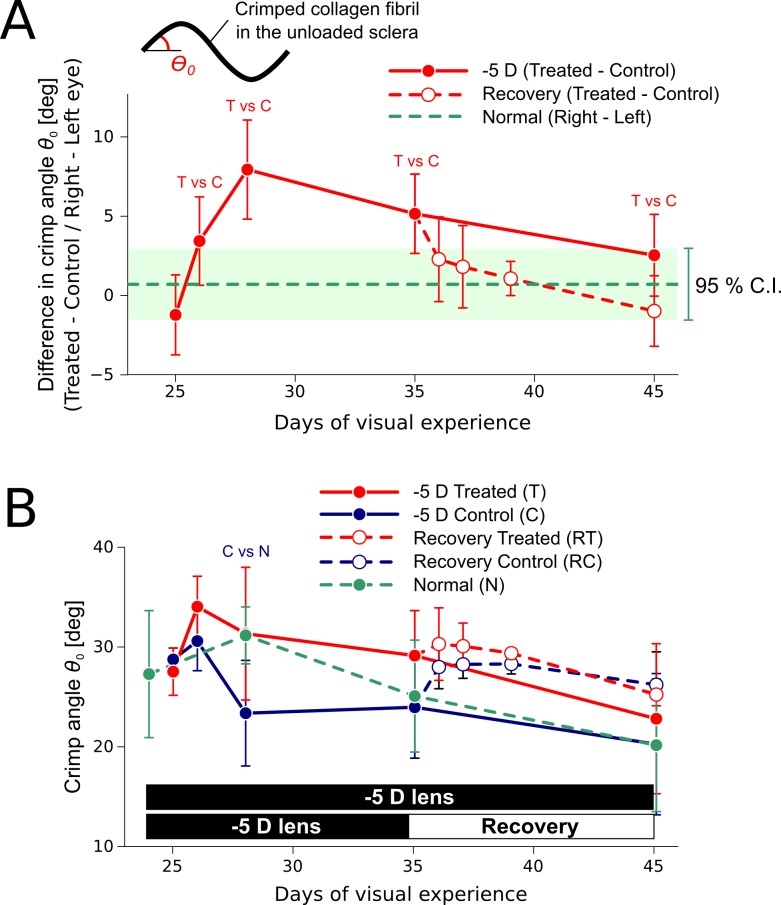
The fitted crimp angle θ₀ of the juvenile tree shrew sclera (16.2°–34.8°, 5th–95th percentile) was high compared to mature/elderly human sclera³⁵ (3.6°–7.0°, 5th–95th percentile). Figure 8A shows the difference in the fitted collagen fibril crimp angle between the treated eye and the control eye for load cycle 1. The difference in crimp angle between the left and right eyes of normal animals was small, suggesting that the material properties are similar for both eyes of a normal animal. Compared with the control eye, the fitted collagen fibril crimp angle was significantly higher in the treated eye after 2 days and peaked after 4 days of −5 D lens wear (paired t-test, P < 0.05). This difference was slowly reduced with continuous −5 D lens wear but the crimp angle remained significantly higher in the treated eye up to 11 days of lens wear. In contrast, the difference in crimp angle rapidly decreased after the lens was removed and was not significant after 1 day of recovery. Figure 8B shows the absolute values of the fitted crimp angle. The crimp angle of the control eye was significantly lower compared with the normal group at 28 days of VE (unpaired t-test, P < 0.05), whereas the fitted crimp angle of the lens-treated eye was not significantly different from normal eyes. During recovery, the crimp angle of the control eye seems to increase to match the crimp angle of the treated eye. However, the crimp angle of the recovering and its control eye were not significantly different from normal eyes.

Figure 8.

Fitted crimp angle parameter (Load Protocol A, load cycle 1, mean, SD) during compensation for a −5 D lens (n = 5 per group) and recovery (n = 3 per group). (A) Difference in the fitted crimp angle parameter between the treated or recovering eye and the control eye. “T vs C” indicates a significant difference (paired t-test, P < 0.05) between the lens-treated eye and the control eye. No significant differences were found between the recovering eye and its control. The average value and 95% confidence interval are shown for the difference between the right and left eyes of normal animals. (B) Absolute values of the fitted crimp angle. “C vs N” indicates a significant difference (unpaired t-test, P < 0.05) between control and normal eyes. No significant differences were found between normal eyes and the lens-treated, the recovering, or the recovering control eye. Please note that each data point is based on an independent set of subjects.

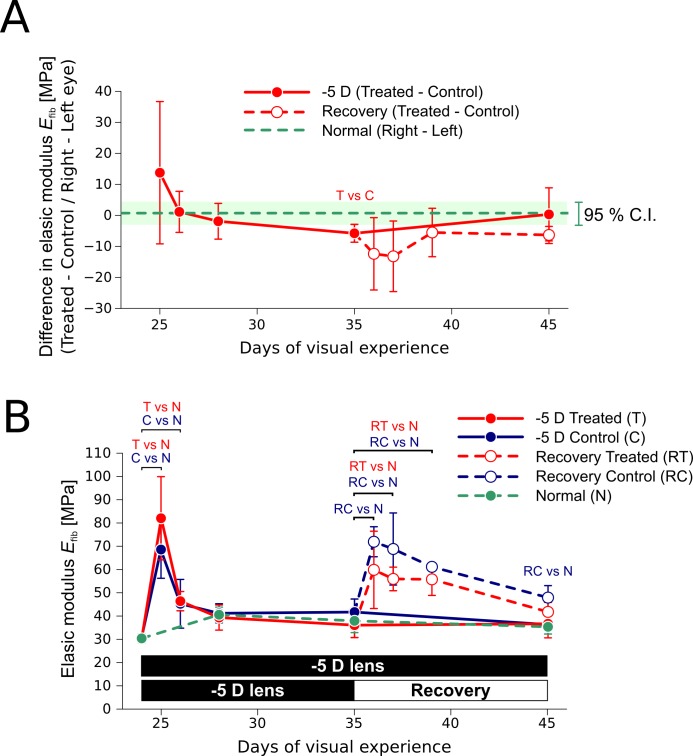
The fitted elastic modulus of juvenile tree shrew scleral collagen fibrils E_fib (29.2–78.2 MPa, 5th–95th percentile) was in a similar range compared with mature elderly human sclera³⁵ (18.9–76.0 MPa, 5th–95th percentile). Figure 9 shows the fitted elastic modulus of collagen fibrils for load cycle 1 in normal animals, during −5 D lens wear, and recovery. The elastic moduli of the lens-treated and recovering eyes were not significantly different from its control except at day 35 of VE, where the elastic modulus of the treated eye was significantly lower compared with the control (paired t-test, P < 0.05, Fig. 9A). Compared with normally developing eyes, a rapid increase in the elastic modulus (up to 2- to 3-fold) was seen in both eyes (control and treated) after starting or stopping the −5 D lens wear (Fig. 9B). The increase was highly transient during lens wear, but more sustained during recovery. Compared with normally developing eyes, this stiffening effect was significant (unpaired t-test, P < 0.05) during the first 2 days of monocular −5 D lens wear in both eyes, whereas it remained significant up to day 4 and 10 of recovery in the treated and control eye, respectively.

Figure 9.

Fitted elastic modulus of collagen fibrils (Load Protocol A, load cycle 1, mean, SD) during compensation for a −5 D lens (n = 5 per group) and recovery (n = 3 per group). (A) Difference in the fitted elastic modulus between the treated or recovering eye and the control eye. “T vs C” indicates a significant difference (paired t-test, P < 0.05) between the lens-treated eye and the control eye. No significant differences were found between the recovering eye and its control. The average value and 95% confidence interval are shown for the difference between the right and left eyes of normal animals. (B) Absolute values of the fitted elastic modulus. Compared with normal sclera, significant differences (unpaired t-test, P < 0.05) are indicated with text labels for the lens-treated eye (T vs N), the control eye (C vs N), the recovering eye (RT vs N), and the recovering control eye (RC vs N). Please note that each data point is based on an independent set of subjects.

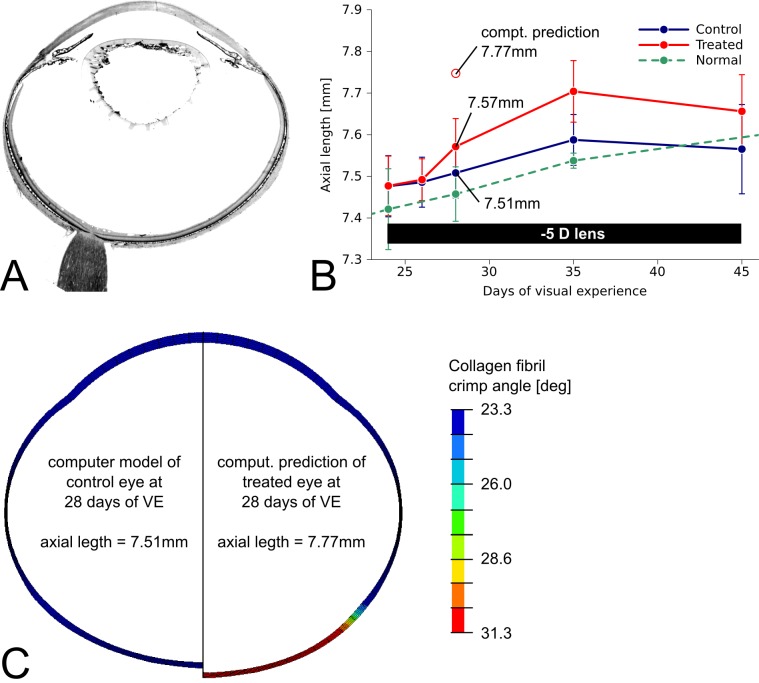
An axisymmetric computer model (Fig. 10C) of the tree shrew eye was derived from a histological section (Fig. 10A) and local thickness measurements of the sclera.⁵⁹ The axial length of the control eye model (left-hand side of Fig. 10C) was adjusted to match the mean axial length of the control eye at 28 days of VE (4 days of −5 D lens wear) shown in Figure 10B. Although keeping IOP constant at 15 mm Hg, changing the crimp angle parameter in the posterior sclera from the fitted mean value of the control eye (23.3°) to the fitted mean value of the treated eye (31.3°) increased the axial length of the computer model from 7.51 to 7.77 mm (Fig. 10C). The predicted increase overestimated the experimentally observed difference in the axial length (Fig. 10B).

Figure 10.

(A) Histologic section of a tree shrew eye. (B) Axial length versus days of VE in normal, control, and −5 D lens–treated eyes (mean, SD) showing an increase in axial length in the treated eyes. (C) Axisymmetric computer models of the control (left-hand side) and the treated (right-hand side) tree shrew eye at 28 days of VE (4 days of −5 D lens wear). The contour plot represents the local distribution of the crimp angle parameter in the computer model. The experimentally obtained axial length (plot B) and fitted material parameters of the (mean) control eye were used to define the computer model of the control eye (left-hand side of C). Starting with the model of the control eye, the crimp angle parameter was changed from the fitted values of the control eye to the values of the treated eye in posterior sclera while IOP was kept constant (15 mm Hg). Increasing the crimp angle led to an increase in axial length in the computer model shown on the right-hand side of (C). The computationally predicted axial length overestimated the experimentally observed increase in axial length in the treated eye (see plot B).

Discussion

We have presented an inverse computational analysis to identify material properties from experimental tensile tests of tree shrew scleral strips. The computational model is based on a mechanistic constitutive formulation³¹ that derives the scleral material properties from its collagen fibril microstructure. The results suggest that the sclera remodels during induced myopia and recovery, and the underlying remodeling mechanism changes the crimping response of scleral collagen fibrils. In animals that wore a monocular −5 D lens, the unloaded crimp angle increased, and then decreased, in concert with the increase, and decrease, in axial elongation rate as the treated eye compensated for the lens. The difference in the unloaded crimp angle between the treated and control eye rapidly decreased to normal values during recovery from myopia. In addition, a computer model of the tree shrew eye was presented showing that the estimated change in collagen fibril crimp angle leads to an increase in axial length. These findings suggest that axial eye elongation may be controlled by a remodeling mechanism that modulates the collagen fibril crimp as well as the creep rate.²²

The presented results revealed a binocular change in the elastic modulus of collagen fibrils during monocular −5 D lens wear and recovery. A rapid 2- to 3-fold increase in the elastic modulus was seen in both eyes after starting or stopping the −5 D lens wear. Compared with normal eyes, this increase was highly transient after starting the lens wear (2 days in both eyes), but more sustained during recovery (treated eye: 4 days; control eye: 10 days). We are confident in this result because this stiffening effect was consistent in all subjects. The rapid and extensive changes in collagen fibril elastic modulus suggests the existence of a dynamic biomechanical mechanism that leads to transient scleral stiffening. This binocular stiffening is surprising, and its cause and potential role in scleral remodeling is unclear. The increase in E_fib during the first 2 days of lens treatment is especially surprising, as the increase in stiffness should lead to a shorter axial length. However, the binocular changes in scleral stiffness during monocular lens treatment and recovery are not temporally associated with the change in axial elongation, indicating that scleral stiffening may not be causally related to axial elongation in myopia.

The observed transient changes in collagen fibril crimp angle further support the notion that scleral remodeling and not scleral growth controls axial elongation in myopia in the mammalian eye. Collagen sliding due to relative deformations of adjacent scleral lamellae has been suggested as a potential remodeling mechanism in myopia.^20,22,60 Collagen sliding may be induced by weakening the extracellular matrix (e.g., by decreasing collagen crosslinks and altering the sulfated GAG levels). Note that the prevention of collagen crosslinking is known to increase axial elongation rate during form-deprivation myopia in tree shrew.⁶¹ The previously reported upregulation of MMP-2, MT1-MMP, and downregulation of TIMP-3 mRNA levels^18–20 in myopia may reduce the integrity of the collagen network and promote collagen sliding. Collagen sliding in turn may lead to scleral elongation and thinning,^16,62 as observed in induced myopia. If elastin maintains its ability to pull the elongated sclera back to its original unloaded state (before collagen sliding occurred), the collagen fibril crimp should increase in the unloaded treated sclera as predicted here. As the eye stays in its elongated state, new collagen crosslinks may be formed, thereby reducing the amount of elastic recoil energy and decreasing collagen fibril crimping. The remodeling mechanism that underlies myopia remains unclear, however, and further experimental investigation is required to elucidate if collagen sliding underlies scleral remodeling in myopia.

The mechanism underlying the observed stiffening of the sclera after starting or stopping the −5 D lens wear and its role in scleral remodeling remains unclear. To the best knowledge of the authors, a similar rapid and extensive stiffening effect of a remodeling soft tissue has not been reported previously. Changes in scleral hydration could lead to transient stiffening. Hatami-Marbini and Rahimi⁶³ have shown that cornea strips significantly stiffened in bathing solution that reduced cornea hydration. Our experimental procedure should not have caused variations in tissue hydration, as all scleral strips were treated identically during mechanical testing (immersed in PBS). However, the previously reported reduction of GAG and PG levels in myopic scleras^17,19,60 might have changed the hydration of the sclera in vivo due to lens treatment. Other biochemical stimuli might also lead to a transient stiffening of scleral collagen fibrils.⁶⁴

The PG and GAG levels are known to change during induced myopia,^17,19,60 but their impact on the biomechanics of the sclera and its remodeling is mostly unknown. Aggrecan appears to be located primarily between scleral lamellae, whereas decorin and biglycan are located throughout the lamellae.^8,60 The expression of aggrecan was shown to be strongly differentially modulated in the sclera during experimentally induced myopia (downregulated) and recovery (upregulated).^17,19,60 Also hyaluronan, which interacts with aggrecan at its G1 domain,⁶⁵ shows a rapid decrease during induced myopia with a rapid return to normal values in recovery.^17,66 The modulation of aggrecan and hyaluronan may control collagen crimping and sliding in the sclera. However, controversial opinions exist in the literature on the role of PGs and their GAG sidechains in soft tissue mechanics, suggesting that PGs may mechanically couple neighboring collagen fibrils and reduce collagen sliding^67–73 or alternatively isolate them and promote collagen sliding.^74,75 Recently, Ahmadzadeh et al.⁷⁶ proposed a new shear-lag model, suggesting that GAG depletion promotes collagen sliding only if collagen fibrils are shorter than a characteristic length. Multiple authors have shown that the hyperelastic and viscoelastic material properties remain unchanged in tendon^77–79 and ligament^80,81 after enzymatic GAG depletion. Note that the above-cited works focused primarily on smaller PGs (decorin and biglycan) and the results were based on acute loading experiments at high strain. The role of the PG aggrecan on the biomechanics of dense collagenous soft tissues is largely unknown, as it is mostly absent in soft tissues except for cartilage. Although our computational model does not directly link the scleral material properties to GAG or PG concentrations, the reported increase in the collagen fibril crimp angle is closely related to the right-ward shift of the linear region of the stress-strain curve seen here during myopia development (Fig. 6). A similar pronounced shift of the stress-strain curve was recently seen by Murienne et al.⁶⁶ in porcine scleras after GAG degradation. These two findings support the notion the modulation of GAGs may play an important role in scleral remodeling during myopia development and underlie the increase in collagen fibril crimp reported here.

The sclera contains myofibroblasts, which may be actively involved in scleral remodeling during lens-induced myopia and recovery. Myofibroblast-induced fibrosis may cause scleral stiffening. However, it is unlikely that scleral fibrosis can increase the sclera stiffness by a factor of two to three within 1 day of lens wear and decrease it to the initial value after another day as seen here. Jobling et al.⁸² proposed the idea that scleral cell contraction may be involved in myopic scleral remodeling. In eyes developing myopia, α-smooth muscle actin levels were found to increase, suggesting increased numbers of contractile myofibroblasts, and decrease in eyes recovering from myopia.⁸² Although cell contraction may play a role in the observed changes of collagen fibril crimp, it is unlikely to underlie the stiffening effect seen here, as the sclera strips were tested between 2 to 4 hours after enucleation.

Preconditioning is applied to achieve a repeatable stress-strain state during mechanical testing, which is typically reached after 3 to 10 cycles.^39,83,84 Our investigation of the preconditioning effect using 50 load cycles at physiological and supraphysiological loads (Load Protocol B) showed that cyclic uniaxial loading leads to continued softening of the juvenile tree shrew sclera at supraphysiological loads and substantial softening at physiological loads when using uniaxial strip testing. This result suggests that the juvenile tree shrew sclera cannot be preconditioned at supraphysiological loads and requires a high number of load cycles (135) to reach a repeatable response at physiological loads. This result was unexpected, as Geraghty et al.³⁹ saw repeatable stress-strain curves after two to three load cycles using uniaxial strip tests and human sclera. This profound difference may be due to differences in the collagen architecture or tissue composition between tree shrew and human sclera. Furthermore, Geraghty et al.³⁹ tested human scleras of adult and elderly donors (age range, 51 to 84 years), whereas we tested juvenile tree shrew scleras. Collagen crosslinks are known to increase with age,⁸⁵ and the relatively small degree of crosslinking at juvenile age may underlie the profound cyclic softening response seen here. Coudrillier et al.,⁴⁷ Tong et al.,⁴⁸ and Myers et al.⁴⁹ have reported that minimal preconditioning effects are seen when inflation testing porcine, human, and bovine sclera. It remains unclear if cutting strips out of the tree shrew scleral shell has enhanced the softening response we report. To answer this question, inflation tests of tree shrew scleral shells have to be performed. Please note that a pronounced preconditioning effect that persists even after a long stretch duration or many cycles (up to 1000 cycles) also has been observed in tendons.^52,86,87 Considering the profound cyclic softening seen here, it is likely that the first load cycle has the smallest amount of experimentally induced scleral softening and is, therefore, the most relevant cycle with respect to the in vivo properties.

A load-cycle–dependent response also was seen in the fitted material parameters obtained from Load Protocol A. The elastic modulus of collagen fibrils increased during the first two load cycles, but did not change from load cycle two to three. In contrast, the crimp angle parameter changed throughout the three load cycles of Load Protocol A. The increase in stiffness during the first two cycles is likely due to a reorientation of collagen fibrils toward the loading direction, as reported previously.^88,89 The continued increase in the crimp angle parameter is due to the cyclic softening of the tree shrew sclera discussed in the previous paragraph. It has been proposed that breaking of collagen crosslinks underlies the softening seen with repeated cyclic loading.^90,91 The breaking of collagen crosslinks may promote collagen sliding due to relative deformations of adjacent scleral lamellae or interfibrillar deformation within the scleral lamellae. If cycling loading induces additional collagen sliding, the stress-stretch curve should continue to shift to a higher stretch level, increasing the fitted crimp angle parameter as predicted by the inverse model.

We saw no statistically significant changes during lens treatment or recovery in two material parameters, the shear modulus and the ratio R₀/r₀. The tissue stiffness at low strains was very low in all scleral strips of the juvenile tree shrew, which led to a very small shear stiffness in all strips irrespective of the experimental groups. The variation of the parameter R₀/r₀ was high (10.1 ± 6.6, mean value and SD of all strips), as its impact on the stress-strain response is typically small compared with E_fib and θ₀, as discussed before.³⁵ The fitted shear modulus was low and the crimp angle parameter was high compared with our previous fits of human sclera.^35,92 The profound difference in these two parameters may be due to the structural differences in the collagen architecture or alterations in the tissue composition (e.g., the crosslink, GAG, or PG density) between human and tree shrew sclera.

Several limitations should be considered when interpreting the results of the present study. Cutting the scleral strips out of the eye may have released residual stresses and altered the mechanical properties of the sclera from its in vivo condition. In vivo, the scleral shell is subjected to an IOP-induced biaxial tensile state. In contrast, the scleral strips were subjected to uniaxial tension in our strip tests, which may lead to a more compliant response of the tissue. Furthermore, our loading protocol (Load Protocol A) included creep tests at physiological loading conditions (1, 3, and 5 g of tension for 30 minutes at each tension) before the load-displacement tests that were investigated in the present article. Our investigation of the preconditioning effect showed that physiological loads do not change the strain variability between subjects (Fig. 4E). Consequently, the creep tests may have affected our estimates of the absolute values of scleral material parameters but not the relative difference between samples or treatments. Based on the limitations of this study, the absolute material properties obtained from uniaxial tensile testing are likely to differ from the in vivo properties of the sclera, which may explain the overestimation of the axial length in the computational model of the tree shrew eye (Fig. 10). Inflation testing may provide better estimates of the in vivo material properties.^{35,40,47,49,93–96} However, all tissue samples were processed and tested in exactly the same way and reported trends in material property differences among treated, control, and normal eyes should hold even if the absolute values may differ.

It is important to note that the estimated changes in collagen fibril crimp are based on an inverse computational model and not on a direct measurement of the microstructure. However, the crimp angle parameter of the constitutive model used here has been validated against direct measurements in rat tail tendons.^30,68 Due to the highly anisotropic collagen architecture of the sclera, direct measurement of scleral collagen fibril crimp is challenging. Recently, Sigal et al. (Sigal et al. IOVS 2013;53:ARVO E-Abstract 3158) presented the first experimental study to measure collagen fibril crimping in ocular tissues. They reported crimp angles between 2° and 12° in the unloaded lamina cribrosa of sheep. Our fitted crimped angle values of the tree shrew sclera are higher (17.2°–38.7°, 5th–95th percentile) but not unusual for soft tissues. Omens et al.⁹⁷ measured collagen fibril tortuosities in myocardium of 1.1, which relates to a crimp angle values of 25° in our model. Please note that the crimp angle in our model is directly related to the tortuosity of collagen fibrils through the so-called locking stretch.³⁰ Voorhees and Han³⁷ used inverse fitting and estimated a crimp angle of 25.5° in heart tissue. Rezakhaniha et al.⁹⁸ measured the waviness of collagen fibers in the arterial adventitia and reported a mean value of 0.72 for their straightness parameter, which is just the inverse of tortuosity and relates to a crimp angle of 38.7° in our model. Consequently, the predicted crimp angle values for tree shrew sclera are in the range of values seen in soft tissues, but direct experimental measurements need to be performed to validate our computational predictions. Furthermore, our computational model is based on many simplifying assumptions, and a multitude of constitutive models exist to simulate the response of soft tissues.^99–103 Using a different constitutive model may result in different collagen fibril crimp and stiffness estimations.

In conclusion, we have identified transient changes in the material properties of tree shrew scleras during induced myopia and recovery. The estimated changes in the crimp angle of scleral collagen fibrils were found to be temporally associated with the changes in the axial elongation rate. The computational simulation of increasing the collagen fibril crimp angle in the posterior sclera led to an increase in axial length, suggesting that axial elongation may be controlled by a remodeling mechanism that modulates the collagen fibril crimp. Further experimental investigations are required to gain insight into the scleral remodeling mechanism that underlies axial elongation in myopia.