Evaluation and mitigation of the interplay effects for intensity modulated proton therapy for lung cancer in a clinical setting

Abstract

Purpose

The primary aim of this study was to evaluate the impact of interplay effects for intensity-modulated proton therapy (IMPT) plans for lung cancer in the clinical setting. The secondary aim was to explore the technique of iso-layered re-scanning for mitigating these interplay effects.

Methods and Materials

Single-fraction 4D dynamic dose without considering re-scanning (1FX dynamic dose) was used as a metric to determine the magnitude of dosimetric degradation caused by 4D interplay effects. The 1FX dynamic dose was calculated by simulating the machine delivery processes of proton spot scanning on moving patient described by 4D computed tomography (4DCT) during the IMPT delivery. The dose contributed from an individual spot was fully calculated on the respiratory phase corresponding to the life span of that spot, and the final dose was accumulated to a reference CT phase by using deformable image registration. The 1FX dynamic dose was compared with the 4D composite dose. Seven patients with various tumor volumes and motions were selected.

Results

The CTV prescription coverage for the 7 patients were 95.04%, 95.38%, 95.39%, 95.24%, 95.65%, 95.90%, and 95.53%, calculated with use of the 4D composite dose, and were 89.30%, 94.70%, 85.47%, 94.09%, 79.69%, 91.20%, and 94.19% with use of the 1FX dynamic dose. For the 7 patients, the CTV coverage, calculated by using single-fraction dynamic dose, were 95.52%, 95.32%, 96.36%, 95.28%, 94.32%, 95.53%, and 95.78%, using maximum MU limit value of 0.005. In other words, by increasing the number of delivered spots in each fraction, the degradation of CTV coverage improved up to 14.6%.

Conclusions

Single-fraction 4D dynamic dose without re-scanning was validated as a surrogate to evaluate the interplay effects for IMPT for lung cancer in the clinical setting. The interplay effects can be potentially mitigated by increasing the number of iso-layered re-scanning in each fraction delivery.

Introduction

Lung cancer remains the leading cause of cancer-related deaths. High doses of radiotherapy are required to eradicate the tumor within the lung. However, the critical normal tissue surrounding the tumor requires maximal protection. For patients with stage III non–small cell lung cancer (NSCLC), previous clinical studies have demonstrated that intensity-modulated proton therapy (IMPT), using scanning proton beams, allows further dose escalation while keeping all parameters of normal tissue sparing lower than or similar to those of passively scattered proton therapy (PSPT).^1,2 Because of substantial dosimetric improvements achieved with use of IMPT for lung cancer, IMPT is being recommended for more and more patients in our center.

For spot scanning techniques, a magnetically deflected particle beam scans the tumor volume laterally by sequentially delivering a series of scanning spots and longitudinally layer by layer via altering proton energy. If the target moves at the same time that the scanning beam is delivered, the motion of the pencil beam might interfere with the delivery of the intended dose distribution, causing deviations from the planned dose distributions. This interference usually results in local regions of under- and overdoses, referred to as interplay effects. In patients with lung cancer, intrafractional motion of tumors and organs is highly correlated with respiration. Since typical breathing periods are on the same time scale with the time needed to deliver spot scanning and change the energy between two adjacent layers, interplay between breathing and the scanning sequence can cause deviations from the planned dose distribution in individual treatment fractions.

Currently, there is no standard approach to managing the motion uncertainty caused by interplay effects. In our center, patients with breathing motion of less than 5 mm are recommended for IMPT. However, the 5-mm motion criterion should be modified to allow for flexibility in the clinical decision of patient selection for IMPT. This criterion does not reflect the magnitude of interplay effects, which varies among patients who have different tumor locations and sizes with similar breathing motion.

Because of the urgent need for our clinicians to manage the interplay effects associated with the IMPT technique for lung cancer, we aim 1) to introduce clinically applicable metric with which to evaluate the interplay effect, and 2) to find a clinically feasible way for our center to mitigate the interplay effect if this effect is found to be large.

One of the consequences of interplay effect is that the doses received by a voxel in each of the fraction are no longer equal resulting in non-uniform fractionation. Previous studies have shown that interplay effect can be washed out during fractional delivery and the biological effect is not largely influenced by the non-uniform fractionation.^3,4 However, in our clinical practice, we attempt to achieve as uniform dose as possible in every fraction.

Our strategy to manage the interplay effect was based on studies that showed that interplay effect can be reduced by volumetric or non-volumetric re-scanning.^5–9 Volumetric re-scanning could be performed across the target volume by irradiating the complete volume once before the next scan commences.⁸ Non-volumetric re-scanning, also known as layered re-scanning, performs multiple scans per iso-energy slice before the energy is changed.

Use of 4D composite doses calculated with 4DCT images and deformable image registration is a well-accepted method of evaluating the actual delivered dose for PSPT in the presence of respiratory motion.¹⁰ For the IMPT technique with dynamic delivery, it is important to incorporate the details of spot delivery into the dose calculation, such as the time stamp of each spot. Previous studies also indicated that dynamic dose converges to the 4D composite dose in multiple deliveries despite interplay.¹¹

The metric that was used to evaluate the magnitude of the interplay effects was target coverage of the single-fraction dynamic 4D dose without considering re-scanning, denoted 1FX dynamic dose. The 4D dynamic dose simulator calculates the dose on the basis of treatment planning procedures and beam-delivery system details at our institution. Thus, target coverage of the 1FX dynamic dose, calculated with use of our 4D dynamic dose simulator, has become our clinical standard for evaluating the magnitude of interplay effects. If the uncertainties of the interplay effects were found to be large, based on the 1FX dynamic dose, we determined whether/how iso-layered re-scanning could reduce the interplay effects so that patient could still undergo IMPT.

In the Methods and material section, we describe our clinical workflow to evaluate and manage the interplay effects for NSCLC patients. In the Results section, we use several patient examples to demonstrate the effectiveness of our method. The uniqueness, implications, and significance of our work are described in the Discussion section.

Methods and materials

Clinical workflow to evaluate and manage interplay effect for NSCLC patients

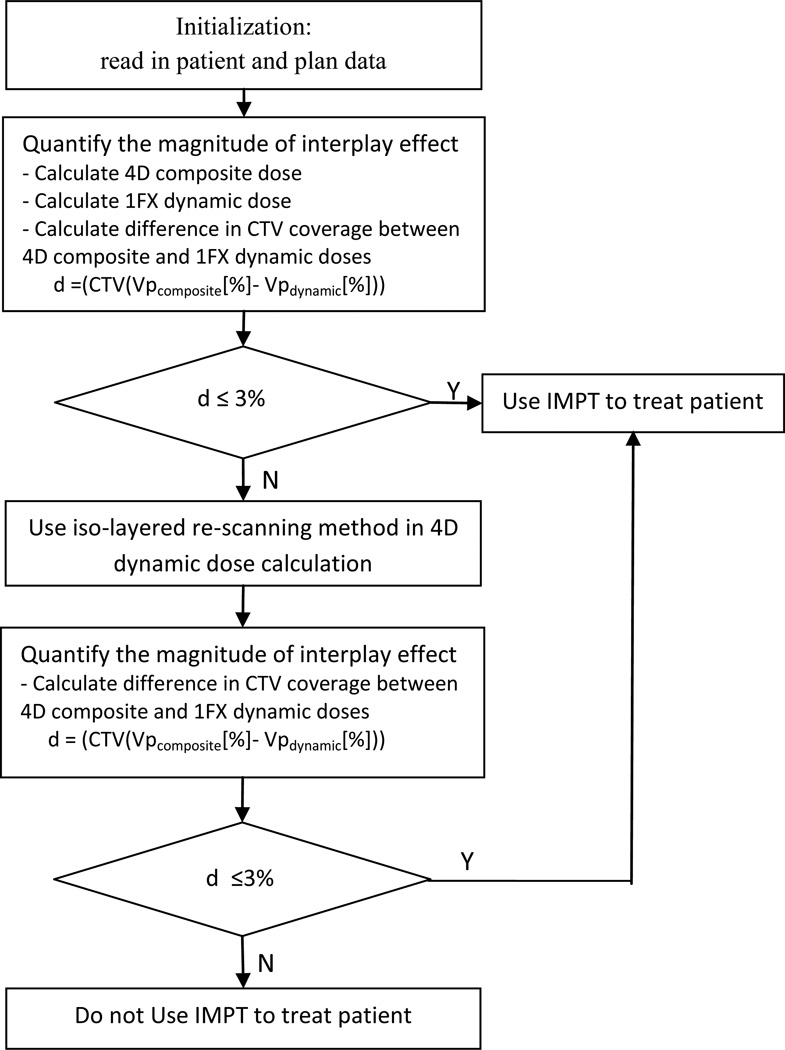
The proposed workflow framework of IMPT for NSCLC is shown in Figure 1. The procedure requires some initialization functions, which involve acquiring relevant data such as CT datasets for all 10 breathing phases, patient breathing motion data, patient breathing pattern, and spot positions and weights. The 4D composite and 4D dynamic dose distributions are calculated for each patient. The 4D composite dose is the equally weighted average of the doses computed on respiratory phases of a 4DCT. The 4D dynamic dose is an estimation of the delivered dose under the influence of interplay between spot-scanning proton beam and respiratory motion. The 4D dynamic dose delivered in single fraction without considering re-scanning is defined as 1FX dynamic dose. The static dose distribution is calculated on the average CT images with which the treatment plans is designed.

Figure 1.

Clinical workflow to evaluate and manage interplay effect for NSCLC patients (Vp: volume of CTV that receives at least the prescription dose).

The magnitude of the interplay effect is quantified by calculating the difference in clinical target volume (CTV) coverage between the 4D composite and 1FX dynamic doses. If the difference does not exceed a threshold value (e.g., 3%), IMPT is recommended for the patient; otherwise, an iso-layered re-scanning method is used to mitigate the interplay effect, and the threshold condition is checked again.

Patient data

We identified seven patients with stage III NSCLC who had previously undergone intensity-modulated radiation therapy or PSPT at our center. For each patient, motion data were derived from the 4DCT dataset of the thorax comprising 10 respiratory phases. These patients had been found to be subject to small (<2.5 mm), medium (>2.5 and <5 mm), or large (> 5 mm) tumor motion during respiration. Table 1 lists the tumor location, tumor size, amount of tumor motion, and the prescribed radiation dose for each patient.

Table 1.

Tumor location, size, motion, and the prescribed dose

Patient no.	Tumor location	Tumor volume (cc)	Tumor motion (mm)	Prescribed dose fxs × Gy/fx
1	RM	472.2	16.6	35 × 2
2	RL	545.1	10.0	35 × 2
3	RU	236.8	8.5	35 × 2
4	LM	358.2	4.6	35 × 2
5	LM	40.8	4.3	35 × 2
6	LM	20.6	2.1	35 × 2
7	RM	26.2	1.4	35 × 2

R, right; L, left; U, upper; L, lower; M, middle; fx, fraction

The prescribed dose was listed as the number of fractions (fxs) times the dose per fraction

IMPT plan

Treatment plans were optimized on the basis of 4DCT datasets and contours of the CTV in each motion phase. CTVs were created by expanding 8 mm from corresponding gross tumor volume of primary tumor and diseased lymph nodes. CTVs of all motion phases were combined to form an internal target volume (ITV) accounting for respiratory motion. For each patient, an individualized treatment plan consisting of three coplanar fields was designed with use of the average CT images. The treatment plan data were calculated by means of the Eclipse treatment planning system (TPS) (Varian Medical Systems, Palo Alto, CA). The data comprised the initial spot positions and intensities. All of this information, along with patient contours and CT images, was then exported as DICOM files to an in-house IMPT research system which implements robust optimization algorithm to optimize the spot intensities according to clinically accepted dose criteria.

For the robust optimization algorithm, different dose distributions with or without consideration of set-up and range uncertainties were computed. The worst dose distribution was then obtained by assigning the lowest dose among all doses to each voxel in the ITV and the highest dose to each voxel outside the ITV. The spot weights were then optimized based on the worst-case dose distribution.¹²

4DCT and 4D composite dose

4DCT images of the patients were acquired at the time of radiotherapy. Multiple CT images acquired at each table position were binned retrospectively according to respiratory signals recorded externally by using a real-time position management (RPM) system. Each respiratory period is divided equally into 10 phases, T0 to T90, wherein T0 represents the end-of-inspiration CT and T50 represents the end-of-expiration CT. All other phases are between these two extremes.

A “demons” deformable image registration algorithm¹³ was applied to define the voxel-by-voxel displacement vector between the image of the exhale phase (T50), serving as a reference phase, and the images of the residual breathing phases. To calculate the 4D composite dose, the treatment plan was first transferred to all 10 breathing phases of the 4DCT. Using the 3D deformation vectors from the exhale phase to all other phases, the dose distributions from all breathing phases were transferred to and averaged on the exhale CT image to create a composite dose for the whole breathing cycle.

Proton spot scanning system

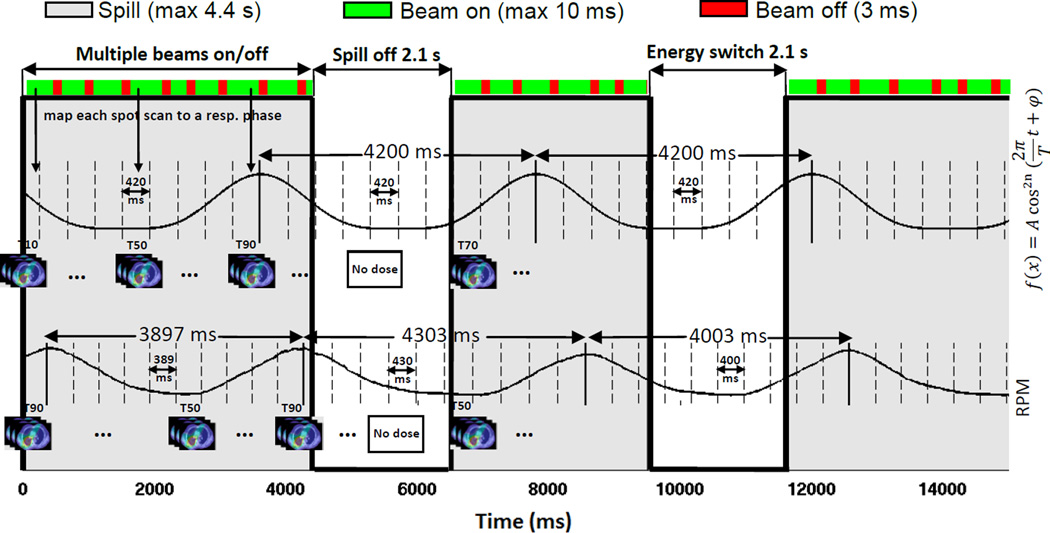
The beam delivery systems (Hitachi ProBeat) available at our center, provides discrete spot scanning by using a synchrotron with active energy variation.¹⁴ In each acceleration cycle, the synchrotron generates a spill of proton beams with the maximum duration of 4.4 s. A switch of energy requires a new spill. We assume that 2.1 s is required to change the energy and fill the synchrotron with protons. Within each spill, the beam is turned on and off to deliver different scanning spots with the same energy. The irradiation time per spot is from 1 to 10 ms, depending on spot monitor units (MU). The beam off time of 3 ms is considered between two neighboring spots from one spill. The full width at half maximum of a scanning spot ranges from 12.8 to 34.3 mm in air at the isocenter as the proton energy varies from 221.8 to 72.5 MeV.

Simulation of interplay effect

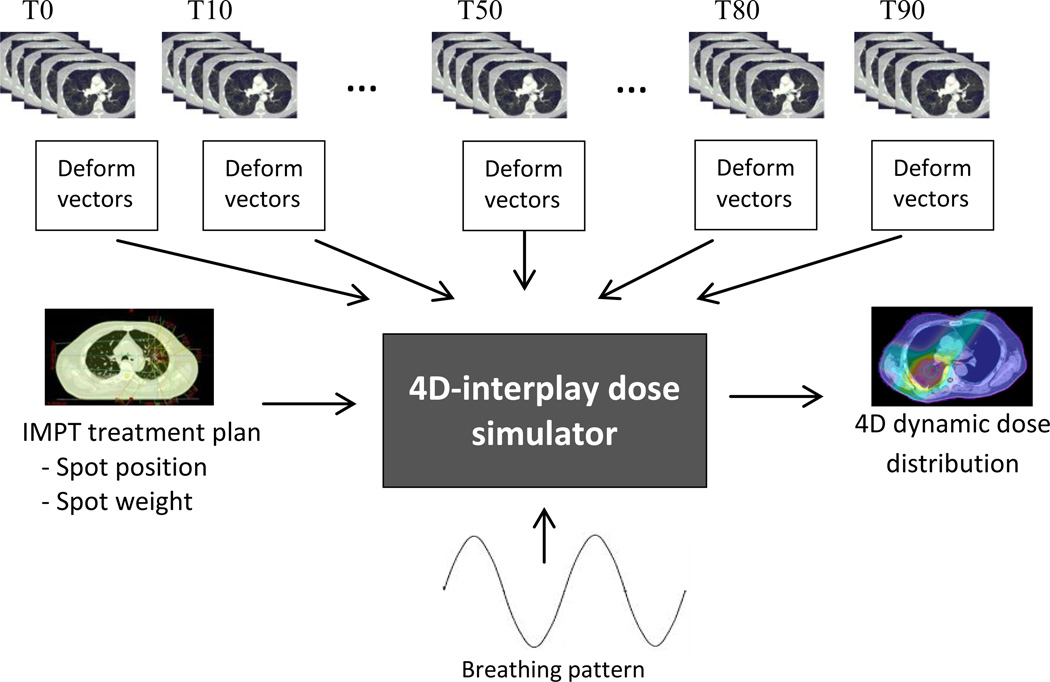
In this study, we used the dose algorithm implemented by Li et al.¹⁵ to develop our simulation tool for interplay effect. Figure 2 shows the inputs and output of the 4D dynamic dose simulator. Spot positions and weights, CT datasets, patient motion data, and patient breathing patterns served as input to the 4D dynamic dose simulator tool.

Figure 2.

Schematic plot of the 4D-interplay dose simulator: Inputs are the IMPT plan which contains the spot positions and weights, 4DCT, deformation vectors of 4D phase to the reference phase (T50 in our simulation), and breathing pattern (either regular or realistic pattern from RPM). Output is the 4D dynamic dose distribution.

To simulate interplay effects, the time spent by each spot scanning step (e.g., turning on and off beams, switching energies, and starting new spills) was tracked as the scanning process proceeded, and a respiratory phase was assigned to each spot according to the recorded timestamp (Figure 3). At the beginning of each fraction and field, a random respiratory phase was assigned. With each spot fully calculated on its assigned respiratory phase, doses of different phases from all spots were summed up to finally yield the dynamic dose distribution on the reference phase by using deformation vectors generated by the deformable image registration.

Figure 3.

Process of the interplay effects simulation. One regular respiratory pattern (upper signal) with the fixed cycle of 4.2 s and one realistic respiratory pattern (lower signal) with different cycles from breath-to-breath are shown. Each respiratory cycle was divided equally into ten phases (dashed lines) and an appropriate 4DCT phase image was assigned to each phase. At the delivery of each fraction and field, scanning would start at a random phase. The time spent by each delivery step was tracked, and a breathing phase was assigned to each spot according to the recorded time stamp. Notice that the period of the respiratory motion can change for the RPM pattern. The amplitude of the respiratory pattern was not used and is explicitly included in the 4DCT.

Iso-layered re-scanning scenarios

There are several options to deliver the dose of an energy level. Zenklusen et al.¹⁶ have identified two approaches for distributing the dose per spot into each re-scan: 1) scaled re-scanning, in which the total dose to a spot is divided by the number of re-scans; and 2) iso-layered re-scanning, in which the irradiation time per spot is limited by a maximal value. The system used presently for patient treatment at our center is based on the iso-layered re-scanning method. The upper limit of the time per spot visit can range from 1 to 10 ms, corresponding to the minimum allowed MU of 0.005 and maximum allowed MU of 0.04. Decreasing the dwell time per spot visit (i.e., decreasing the MU value) can increase the magnitude of re-scanning. To calculate the 4D dynamic dose considering the iso-layered re-scanning, the initial phase for each re-scanning was determined from the current breathing phase after the previous scan, considering the delay to prepare another synchrotron spill or beam off time.

Study design

To quantify the magnitude of interplay effects on the planned dose distribution, the 4D dynamic dose distribution delivered in a single fraction without considering spot re-scanning (1FX dynamic dose) was simulated. To investigate the impact of respiratory motion parameters on the 1FX dynamic dose distribution, simulations were performed under various respiratory motion variation scenarios (e.g., different initial phases and respiratory patterns). A regular breathing pattern, modeled by an asymmetrical sinusoidal function¹⁷, and three realistic breathing patterns extracted from patient RPM data with various irregularities, characterized by the standard deviation (SD) in the period of breathing cycles (0.13, 0.46, and 0.93 s), were included. Note that different periods of respiratory cycles result in different durations of each respiratory phase in the simulation process. The regular respiratory pattern was modeled as $f(x)=A{\mathit{\text{cos}}}^{2n}(\frac{2\pi }{T}t+\phi )$, where A is the motion amplitude, T is the period of respiratory cycle, n is a parameter that determines general shape of the model, and φ is the initial phase of respiratory cycle. In our calculation, only a period T=4.2 s and a random initial phase were used. The amplitude A and parameter n were naturally included in the 4DCT. In our simulation, we relied on a single 4DCT scan which assumes that patient breathes with the same amplitude during the treatment. Changes in the value of parameter n were considered by using different respiratory patterns.

The dose distributions resulting from dynamic deliveries were compared with the dose distributions of the 4D composite dose. If the uncertainties of the interplay effects were found to be large, we examined how iso-layered re-scanning can reduce the interplay effects. In our center, the number of layer-by-layer re-scanning required to deliver a spot is determined by the hardware limitations of the machine. We investigated how a lower value of MUs resulting in a higher number of re-scanning required to deliver a spot can improve the dose distribution of a single fraction. Simulations were performed by incorporating different maximum MU limit values including the maximum (0.04) and minimum (0.005) of the parameter as well as a value in this range (0.01).Results for single-fraction deliveries were normalized in order to be compared with the 4D composite doses of about 70 Gy.

Results

The percentage of CTV that received at least the prescription dose (V70) was used as a measure of target coverage. Organ volumes receiving doses of at least 5 Gy (V5), 10 Gy (V10), 20 Gy (V20), and 30 Gy (V30) were calculated for total lung. The V40 and V55 for heart and esophagus, respectively, and the maximum dose to the spinal cord were also calculated.

Interplay effects without motion mitigation

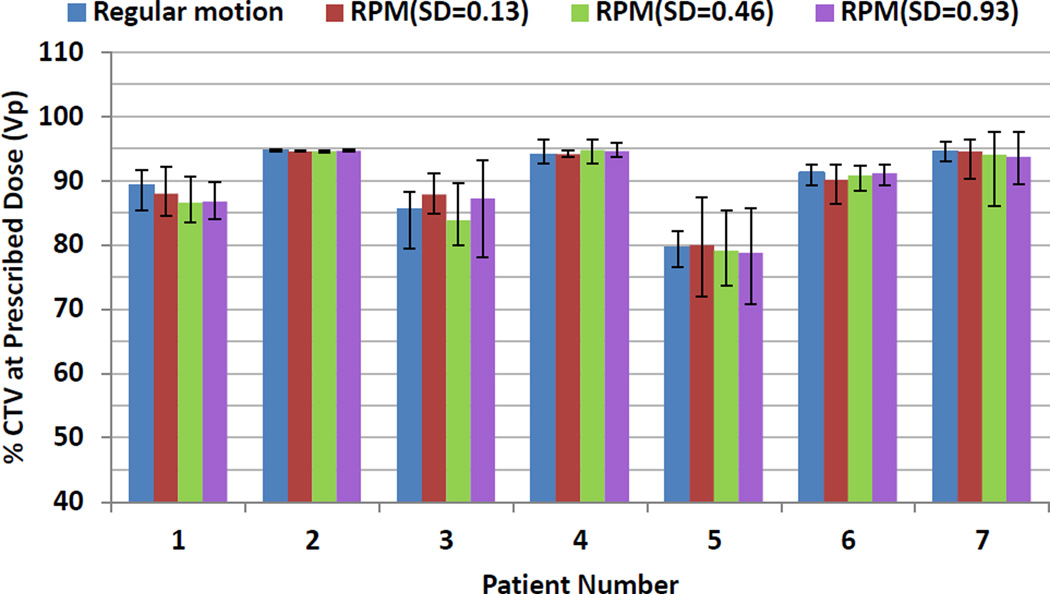
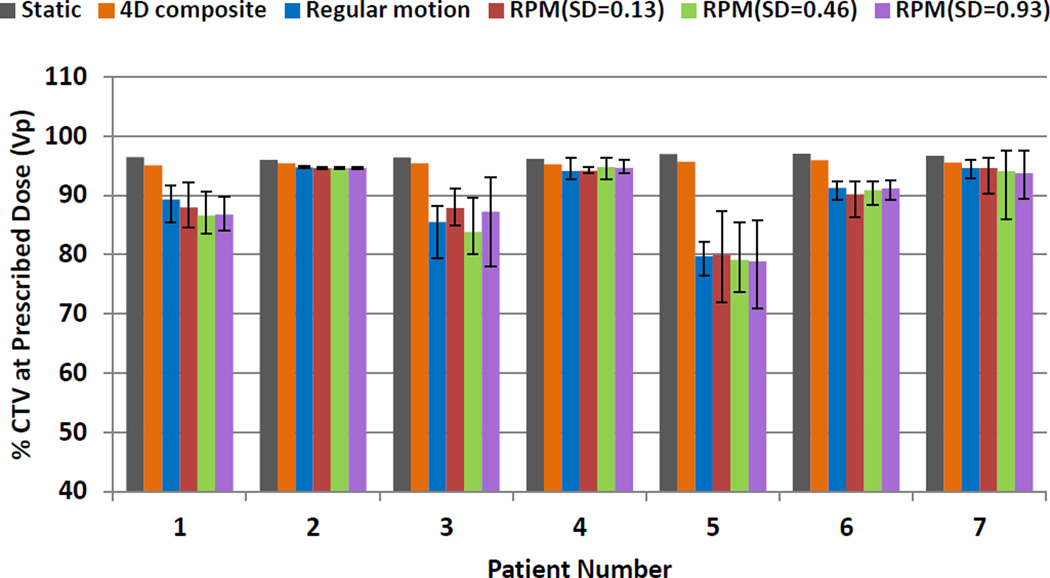
Figure 4 shows the average target coverage using 1FX dynamic doses when different initial starting phases and respiratory patterns were considered. For each patient, 10 runs with random initial phases were conducted for each respiratory pattern. The error bars indicate the maximum and minimum of target coverage. As shown in Figure 4, the changes of the CTV prescription coverage due to different respiratory patterns and different starting phases were not statistically significant different. The stability of the average target coverage indicates that the dosimetric impact on the target coverage was mainly due to interplay. Figure 4 also shows that there is tendency that the larger the interplay effects (patients 3 and 5), the larger the variation caused by the different starting phases.

Figure 4.

The impact of the motion patterns and starting phases on the interplay effects. Average CTV prescription coverage in 1FX dynamic doses for different initial starting phases and respiratory patterns. The error bars represent the maximum and minimum values for CTV coverage due to different starting phases

Figure 5 compares the CTV coverage of the 4D composite and 1FX dynamic doses. The CTV coverage values for the seven patients were 95.04%, 95.38%, 95.39%, 95.24%, 95.65%, 95.90%, and 95.53%, calculated with use of the 4D composite dose, and were on average 87.62%, 94.62%, 86.09%, 94.36, 79.50%, 90.85%, and 94.23% over all respiratory patterns with the use of 1FX dynamic dose.

Figure 5.

Comparison of CTV prescription coverage between 4D composite and 1FX dynamic doses considering different respiratory patterns, where the error bars indicate the range of CTV coverage due to different starting phases.

The average CTV coverage of the 1FX dynamic dose for seven patients were 89.30%, 94.70%, 85.47%, 94.09%, 79.69%, 91.20%, and 94.19% when regular respiratory pattern was considered. For the regular respiratory pattern, the differences in average CTV coverage between the 4D composite and the 1FX dynamic doses were 0.68%, 1.15%, and 1.34% for patients 2, 4, and 7, respectively. These patients will be recommended for IMPT since the average differences in CTV coverage between the 4D composite and 1FX dynamic dose were less than our predefined threshold value of 3%. 1FX dynamic doses yielded on average 5.74%, 9.92%, 15.96%, and 4.70% less CTV coverage than the 4D composite doses yielded for patients 1, 3, 5, and 6, respectively. Therefore, these patients will not be recommended for IMPT unless a motion mitigation technique is used.

Note that in the first two motion categories in Table 1 (i.e., motion > 5 mm or 2.5 mm < motion < 5 mm) for which we included both small and large tumor volumes, the interplay effects were more pronounced for patients with smaller tumor volumes. The difference was most noticeable for patient 5, with tumor motion of 4.3 mm and tumor volume of 40.8 cc. Comparison of the dose distribution of patient 4, with tumor motion of 4.6 mm and tumor volume of 358.2 cc, with that of patient 5 suggests that a decrease in tumor volume can lead to an increase in the interplay effect.

For the entire group of seven patients, the maximum differences between the 4D composite and 1FX dynamic doses were 4.4 Gy, 0.2%, 0.88%, 0.4%, 0.16%, 0.19%, and 0.21%, respectively, for the maximum spinal cord dose, heart V40, esophagus V55, and lung V5, V10, V20, and V30.

Interplay effects with re-scanning

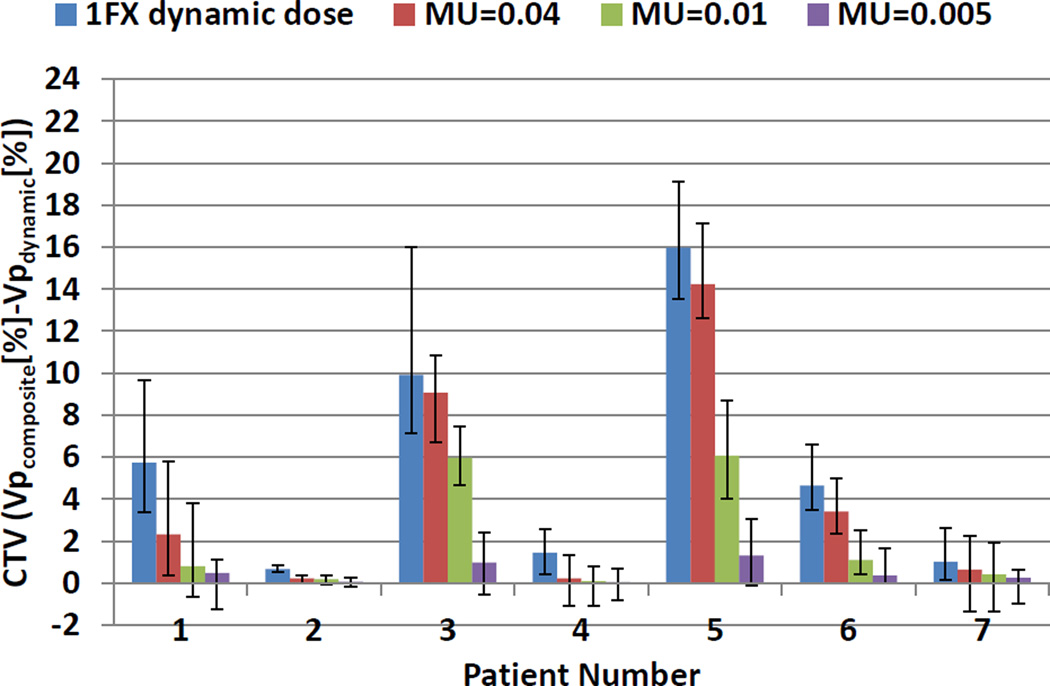
Figure 6 shows the differences in CTV coverage between 4D composite and dynamic doses for regular respiratory pattern when the iso-layered re-scanning method, with various maximum MU limit values equal to 0.04, 0.01, and 0.005, was used. Results confirmed that re-scanning yielded lower differences between 4D composite and dynamic doses for all patients.

Figure 6.

Average differences in CTV prescription coverage between 4D composite and single-fraction dynamic doses with and without iso-layered re-scanning for regular respiratory pattern. The degree of iso-layered re-scanning is reflected in the values of maximum MU limit. The error bars show the range of the differences due to different starting phases.

As the maximum MU limit value decreased, the 4D dynamic doses converged more to 4D composite doses. Our results showed that single-fraction dose delivery using maximum MU limit values as small as 0.01 might not allow adequate CTV coverage for patients 3 and 5 with relatively small tumor size and large tumor motion. For these two patients, a higher order of re-scanning was required to reduce the difference in CTV coverage between 4D composite and dynamic doses to below 3%. Dwell time of 1 ms (i.e., Maximum MU limit value of 0.005) was necessary to wash out hot and cold spots in these two cases. For the seven patients, the average CTV prescription coverage, calculated by using single-fraction dynamic dose, were 95.52%, 95.32%, 96.36%, 95.28%, 94.32%, 95.53%, and 95.78% using maximum MU limit value of 0.005.

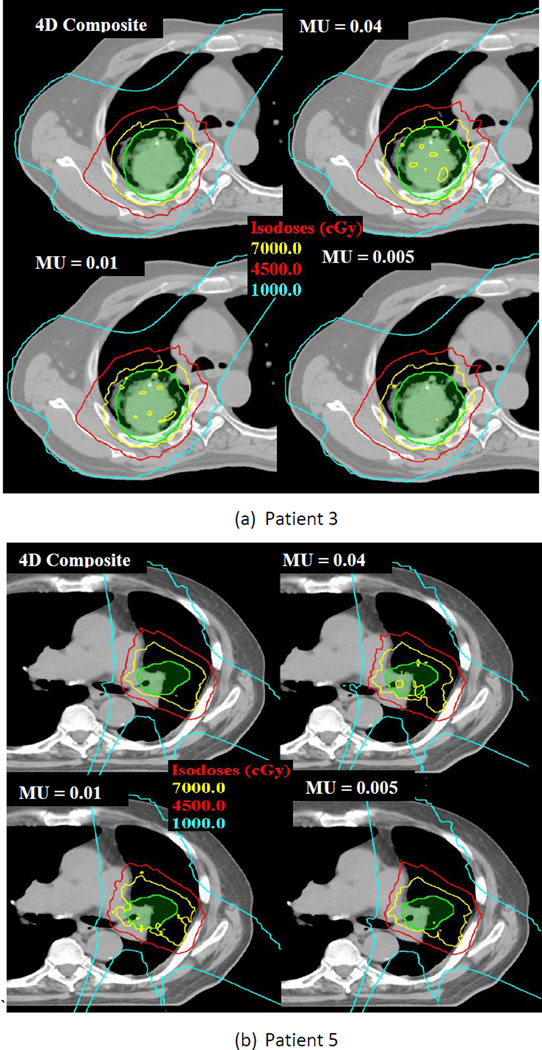
When iso-layered re-scanning with a maximum MU limit value of 0.005 was used, the maximum differences between the 4D composite and 4D dynamic doses decreased to 0.6 Gy, 0.01%, 0.02%, 0.09%, 0.09%, 0.08%, and 0.08%, respectively, for the maximum spinal cord dose, heart V40, esophagus V55, and lung V5, V10, V20, and V30. Figures 7(a) and 7(b) show how relatively large dose spreads for target volumes of patients 3 and 5 in single fractions were smoothed out when the number of re-scanning increased.

Figure 7.

Dose distributions of 4D composite and single-fraction 4D dynamic doses with different iso-layered re-scanning. The green color wash is the clinical tumor volume (CTV); the inner yellow line indicates the prescription isodose line (70 Gy); the red line indicates the 45 Gy isodose line; and the blue line contains the 10-Gy dose regions. Non-uniform doses in single-fraction dynamic doses for regular respiratory pattern were smoothed when maximum MU limit of 0.005 was used.

Discussion

A clinically applicable metric, target coverage of single-fraction dynamic dose without considering re-scanning, was introduced to assess the dosimetric effects of respiratory motion on the final dose distribution in NSCLC patients. The impact of tumor motion uncertainty on the dose distribution of patients with different tumor volumes and motion amplitudes has been evaluated. The magnitude of interplay effect using scanning proton beams has been reported in previous studies and it has been shown that proton dose could be enormously impacted by the interplay effect for tumor motions around or larger than 10 mm and relatively small spot sizes.^{4–6,18–20} In this study, we found tumor size also influences the dose distribution. Our results showed that a decrease in tumor size as well as an increase in tumor motion increases the CTV coverage deviation from the values for the 4D composite dose. Up to 15.96% of difference in CTV coverage was noted between the 4D composite and 1FX dynamic doses for patients with smaller tumor volumes. This study indicated that the current center-to-center motion criterion alone may not be a good measure of the interplay effect because it does not reflect the magnitude of interplay effect for individual patients with different tumor sizes. We observed situations in which motion of less than 5 mm and small tumor size led to relative large uncertainties caused by interplay effect in a single fraction. In contrast, for some patients with motion larger than 5 mm and large tumor size, the interplay effect was small.

We defined a threshold value of 3% for the difference in CTV coverage between the 4D composite and 1FX dynamic dose. For every individual patient, if the difference was less than the threshold value, that patient was considered able to undergo IMPT; otherwise the iso-layered re-scanning approach was used to mitigate the interplay effect. Iso-layered re-scanning has been found to consistently mitigate the interplay effect. We found a trend in the influence on the dose distribution for maximum MU limit values. We demonstrated that higher numbers of iso-layered re-scannings led to less distorted dose distributions confirmed by a reduced deviation of all dose values from the 4D composite doses. We found re-scanning to reduce interplay effects by up to 14.6%. Based on our results, faster spot scanning due to decreasing maximum MU limit value was able to reduce the interplay effect but at the price of longer delivery times. However, readers should be cautioned that the above observation was based on the spot sizes that may be large relative to those adopted by other centers. Therefore, we urge that institution-specific study may be necessary for establishing and verifying institution-specific treatment procedures.

We used the 4D composite dose to validate the 4D dynamic doses calculated by 4D dynamic dose simulator. Based on the results of this study, we recommend that an IMPT plan be carefully evaluated by using the 4D composite dose for cases with respiratory motion. The interplay analysis tool described in this study can be used to validate selected proton scanning plans, especially for cases with large respiratory motion and/or small tumor volumes.