Diastolic Function in Heart Failure

Abstract

Heart failure has reached epidemic proportions, and diastolic heart failure or heart failure with preserved ejection fraction (HFpEF) constitutes about 50% of all heart failure admissions. Long-term prognosis of both reduced ejection fraction heart failure and HFpEF are similarly dismal. No pharmacologic agent has been developed that actually treats or repairs the physiologic deficit(s) responsible for HFpEF. Because the physiology of diastole is both subtle and counterintuitive, its role in heart failure has received insufficient attention. In this review, the focus is on the physiology of diastole in heart failure, the dominant physiologic laws that govern the process in all hearts, how all hearts work as a suction pump, and, therefore, the elucidation and characterization of what actually is meant by “diastolic function”. The intent is for the reader to understand what diastolic function actually is, what it is not, and how to measure it. Proper measurement of diastolic function requires one to go beyond the usual E/A, E/E′, etc. phenomenological metrics and employ more rigorous causality (mathematical modeling) based parameters of diastolic function. The method simultaneously provides new physiologic insight into the meaning of in vivo “equilibrium volume” of the left ventricle (LV), longitudinal versus transverse volume accommodation of the chamber, diastatic “ringing” of the mitral annulus, and the mechanism of L-wave generation, as well as availability of a load-independent index of diastolic function (LIIDF). One important consequence of understanding what diastolic function is, is the recognition that all that current therapies can do is basically alter the load, rather than actually “repair” the functional components (chamber stiffness, chamber relaxation). If beneficial (biological/structural/metabolic) remodeling due to therapy does manifest ultimately as improved diastolic function, it is due to resumption of normal physiology (as in alleviation of ischemia) or activation of compensatory pathways already devised by evolution. In summary, meaningful quantitative characterization of diastolic function in any clinical setting, including heart failure, requires metrics based on physiologic mechanisms that quantify the suction pump attribute of the heart. This requires advancing beyond phenomenological global indexes such as E/A, E/E′, Vp, etc. and employing causality (mathematical modeling) based parameters of diastolic function easily obtained via the parametrized diastolic function (PDF) formalism.

Overview

The overall focus of this review of diastolic function in heart failure is for the reader to have a better intuitive and conceptual grasp of how the heart works when it fills. Rather than describe the features of “diastolic function,” as is the current conventional tradition, in order to gain deeper understanding it is more useful to view diastolic function as a problem to be solved! Once the basic problem of normal physiology of diastole has been mastered, it is nearly self-evident as to what the issues are in clinical heart failure and which physiologic mechanism and its hard-wired compensatory pathways one is confronted with. Extensive literature exists on the therapeutic options to be employed in every conceivable clinical setting where heart failure has been diagnosed; therefore, the focus here will be on physiology and pathophysiology, and, more importantly, on what diastolic function actually is, what it is not, and how to measure it.

Heart failure (HF), often called congestive heart failure (CHF), is commonly defined as a clinical diagnosis in a setting of impaired pump function when the ejection fraction has been reduced and the heart muscle has weakened and is unable to pump sufficient volume of blood at the required pressure to maintain normal physiologic function. Actually, heart failure is more nuanced, and has been characterized by clinicians in terms of “left-sided heart failure”, where “left” refers to left ventricle (LV), “right-sided heart failure”, where “right” refers to the right ventricle (RV), “systolic heart failure”, implying overall inadequate pumping, and diastolic heart failure (DHF) or heart failure with preserved ejection fraction (HFpEF) where the ventricle(s) are unable to fully relax while they fill or fill incompletely at a sufficiently low pressure, to avoid pulmonary congestion in the time available for filling. It is relevant that, if patients are classified according to HF with reduced EF (HFrEF) or HFpEF, it is very likely that they have multiple common comorbidities that are likely to affect both the management and the ultimate outcome. For example, pulmonary disease, diabetes, anemia, and obesity tend to be more prevalent in HFpEF than in HFrEF, but the prevalence of renal dysfunction and breathing disorders during sleep are similar between the groups. Furthermore, common cellular pathophysiologic mechanisms are likely to include inflammation and fibrosis at both the systemic and myocardial level.¹

The general causal explanation for the clinical state of HF commonly involves coronary artery disease, ischemia and associated myocardial infarction, hypertension, diabetes, diseases of the valves (valvulopathy), non-ischemic cardiomyopathy, myocarditis, gradual adverse (rather than beneficial) remodeling of the chamber due to chronic arrhythmias (atrial fibrillation for example), alcoholism, obesity, and other less common ailments such as infiltrative myopathies (amyloidosis), hyperthyroidism, hypothyroidism, emphysema, hemochromatosis, and congenital heart disease.

It immediately follows from the above description that the cellular and intracellular details of heart failure of any cause are biologically and conceptually complicated. Because of the electromechanic (conduction system → myocyte) mechanism, which Nature has evolved for the design of the myocardium, a design that allows for the presence of mechanoelectric feedback (stretch-activated ion channels), only selected aspects of how the entire system functions are understood at various dimensional scales (from molecular to organ level). As is characteristic of biology, at the molecular and biochemical level all intracellular pathways [metabolism to generate adenosine triphosphate (ATP), intracellular component turnover (DNA, RNA, etc)] are coupled and interact in a myriad of known (and as yet unknown) ways and have evolved both feed-forward control, feedback, and compensatory mechanisms. In spite of the obvious complexity of the system as evidenced by ∼10⁵ sarcomeres (about 2 μm in length) per myocyte, with ≈10⁹ myocytes per LV, intracellular Ca⁺⁺ ion concentration oscillates from micromolar to millimolar in tens of milliseconds, t-tubules form a three-dimensional Cartesian grid² to ensure simultaneity of mechanical contraction (cross-bridge cycling) of a single cell via triggering of Ca⁺⁺ channels and ryanodine receptors, the giant protein titin can have “stiff” or “soft” extensible region isoforms, myocytes are coupled mechanically by “rivets” (desmosomes) and electrically by connexins (of various types), and fibroblasts (25% of LV volume, 60%–70% by number, small 1 × 2 × 10 μm) exceed myocytes (75% by volume, but 30%–40% by cell number, large 20 μm diameter × 60 μm length) numerically in the myocardium and manage the extracellular matrix (ECM) in addition to having biochemical, structural, and electrical activity.^3,4

Basic Governing Physiologic Principles of How the Heart Works When it Fills

In order to fully comprehend the elegance of diastolic function in normal physiology and how it is modified in heart failure, it is of value to review the basic principles that govern the macroscopic function of the intact human heart.

The heart is a mechanical (muscle-powered) oscillator

To appreciate its design, it is useful to compare it to other components of human anatomy that are examples of muscle-powered oscillatory function – for example, clenching the fist and then opening the fist to show the palm. This oscillatory action requires two muscle groups that reside in the forearm: the extensors and the flexors. The action is voluntary, but when one group contracts, the other automatically relaxes, and vice versa. The duration, strength of closing, and opening the fist are under voluntary control. It is possible to close the fist and hold it in that form for an extended period. The four- chambered heart is also a muscle-powered oscillator. However, the LV is a single (woven) muscle group (shaped as a bowl), but it has no opposing muscle group. In spite of lacking an opposing muscle group (for precision, a technical correction here: in normal sinus rhythm atrial systole, the Doppler A- wave performs an opposing muscle group function by stretching the post-diastatic chamber. However, atrial contraction is not required for LV filling via the E-wave – as evidenced by rate-controlled atrial fibrillation), it decreases chamber size in systole when it contracts, and even when in isolation (such as in a Langendorf prep) it expands on its own (diastole) without another muscle group interacting with it, or when the source pressure for filling is zero (as in a freshly excised rat heart freely submersed in Krebs that swims around like a squid!). The method by which the chamber expands in early diastole presented a puzzle historically, because the muscle was known to contract (in systole), but no mechanism was known that made it “push” in diastole! This issue has been resolved, and there is general agreement that extracellular and intracellular elastic elements (essentially springs) are loaded in systole, which cause the chamber (myocytes, ECM, etc.) to return to its “equilibrium” configuration in diastole. These elastic elements include the ECM⁵ (primarily made of collagen and elastin), the intracellular giant protein titin,⁶ and the visceral pericardium, which acts like a “shrink-wrap” and stores elastic energy in systole, contributing to the elastic recoil in diastole.⁷

The four-chambered heart is a (nearly perfect) constant-volume pump

More precisely, what remains nearly constant volume during the cardiac cycle is the content of the pericardial sac.⁸ The obvious consequence is that an A/P chest X-ray [by which heart size (cardiac silhouette) is often determined while holding one’s breath (to avoid diaphragmatic motion)] can be taken anytime during the cardiac cycle, without the need to gate the X-ray machine to the electrocardiogram (ECG)! The volume of the pericardial sac includes the blood pool in the atria and ventricles, the roots of the great vessels, and the muscle. It is self-evident that the muscle mass remains constant during the cardiac cycle (except for a tiny correction due to cardiac lymphatics and coronary artery and vein caliber changes); therefore, the (near perfect) constancy of pericardial sack volume requires that the atrioventricular volumes reciprocate, meaning, when the ventricles eject, the atria must fill.

The (near) constant volume, four-chamber physiology has been shown to apply to the left- and right-sided chambers separately. This is not too surprising in light of the fact that the time-averaged spatial location of the midline of the interatrial septum and the LV septum remains essentially fixed in space during the cardiac cycle rather than shifting a large amount to the left or to the right. If the left heart was indeed a perfect constant-volume pump in systole and diastole, atrial filling volume during ventricular systole (Doppler pulmonary vein S-wave, when the closed mitral valve plane is pulled downward by the contracting LV toward the apex, much like a piston in a syringe, and oxygenated blood is aspirated by the descending closed mitral valve plane from the lung to fill the atrium) would equal the volume ejected by the LV. If this S-wave volume were to be equal to the LV systolic stroke volume, there would be no need for the existence of a pulmonary vein D-wave! Why? Because the aspirated atrial volume would enter the LV as the mitral annulus ascended. But the LV stroke volume is always more than the pulmonary S-wave volume because of the slight epicardial displacement during systole.⁹ Because the ejected LV stroke volume in systole is always greater than the simultaneously aspirated pulmonary vein S-wave volume entering atrium, the difference must be made up during the next Doppler E-wave as the (simultaneous) pulmonary vein D-wave. This explains why the four-chamber heart is – to within 95% – a constant-volume pump and it explains the conduit (blood flowing from lung to left heart during the E-wave) function of the left atrium!¹⁰ The difference can be easily understood based on conservation of volume principles and visually appreciated as the “crescent effect” when the epicardial displacement of the LV during systole is compared to its location in diastole.

The difference between “volume pumping” and “pressure pumping”

To appreciate these attributes, it is useful to refer to the Wiggers Diagram for both the left and right ventricles, shown in the next column (http://starklab.slu.edu/Physio/Circulation.htm).

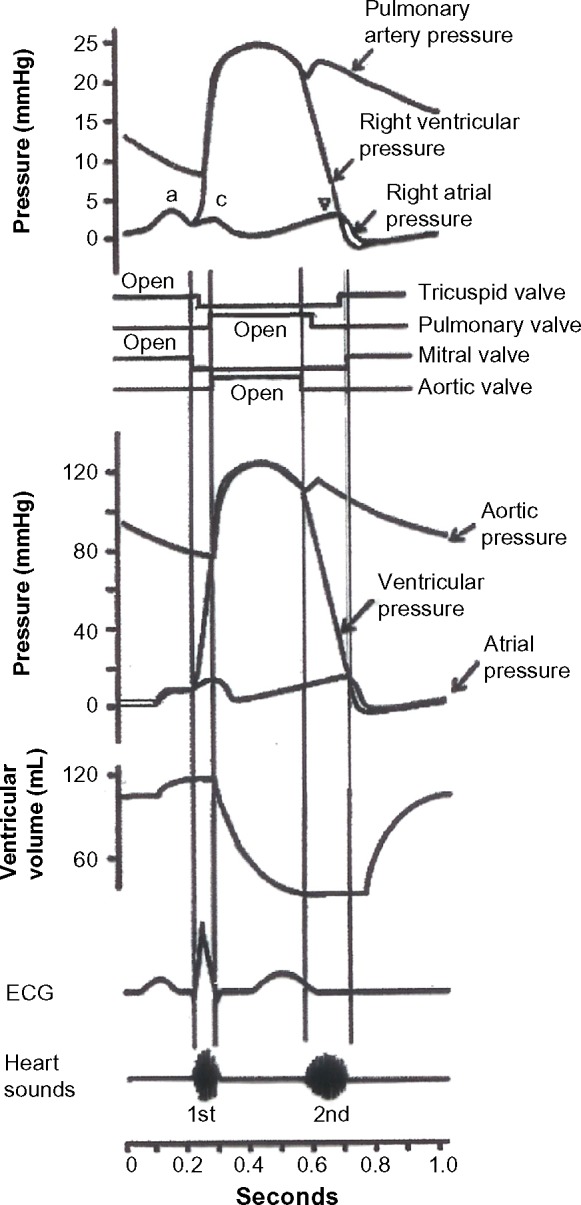
Wiggers diagram.

It is self-evident that the right heart – the right ventricle in particular – is essentially a pure volume pump in both systole and diastole. This is because the pressure variation in the RV is about 15 mmHg – meaning that blood enters at about 5–10 mmHg and leaves the RV at a pressure of 15–25 mmHg. In diastole, the RV pressure is lower than the right atrial pressure (RAP) during early rapid filling (tricuspid E-wave acceleration portion on Doppler) and the temporary diastolic atrioventricular gradient is about 3–5 mmHg. Essentially, the RV accepts blood at a low venous pressure and transports it to the lung with only a slight elevation of pressure, so that the mean PA pressure is 9–18 mmHg. The primary volume pumping attribute of the right heart is underscored by the observation that, due to the primarily longitudinal orientation of RV free wall muscle fibers, the oscillatory apex to base displacement of the tricuspid annulus during the cardiac cycle is greater (by at least 1 cm) than the apex to base oscillatory displacement of the mitral annulus.¹¹ In contrast, for the left heart, the left atrium is always a volume pump, but the LV is a volume (suction) pump in diastole but a pressure–volume pump in systole. That is because the LV fills at a low (mean left atrial) pressure of about 10–15 mmHg, but empties at a high (mean) arterial pressure of >70 mmHg. In order for the LV to be a pressure pump in systole, additional circumferential weave is included in the mid-wall in addition to the helical fiber orientation that varies from endo to epi by 160º. Accordingly, mitral annular excursion is less than tricuspid annular excursion.

In early diastole all LVs are mechanical suction pumps

The history of how the heart works when it fills has generated controversy, and, amazingly, some controversy remains to this day!! Careful consideration of the physiology of diastole and relating the physiology to the above-enumerated principles allows a definitive, causal-mechanism-based formalism by which diastolic function can be described, analyzed, taught, and (noninvasively) quantitated.

In 1930, in experiments in turtle hearts, Katz¹² noted that during early diastole, after valve opening, the chamber continued to decrease its pressure while it increased its volume. In physiologic terms, dP/dt <0, while simultaneously dV/dt >0, hence the chamber stiffness dP/dV <0! But how should one interpret a negative chamber stiffness? Katz correctly concluded that the chamber was expanding (recoiling) faster than it could fill. He noted the same physiology (dP/dV <0) in the mammalian (dog) heart and correctly concluded that the mammalian LV initiated filling by being a mechanical suction pump. The mechanism that explained this physiologic behavior was unknown to him. By 1985, the role of the heart as a suction pump⁵ was noted, with the elastic ECM (collagen, elastin) as the sole (recoiling spring) analog. Subsequently, intracellular spring elements (titin⁶) and extracellular attributes of the visceral pericardium⁷ have been shown to contribute to the mechanical recoiling of the LV wall responsible for recoil of the wall and filling of the chamber. The suction-pump physiology was often confused by the erroneous interpretation that the LV is a suction pump only when the left ventricular pressure (LVP) is below the atmospheric pressure (zero gauge pressure). This is misleading, since the source pressure for LV filling is (the mean) LAP rather than atmospheric pressure. Therefore, as long as the LV is able to decrease its pressure below LAP at mitral valve opening and for a bit thereafter, so that dP/dV <0, all ventricles initiate filling by being mechanical suction pumps. This implies that all ventricles fill by the same physiologic and kinematic principles and that diastasis corresponds to the in vivo equilibrium volume of the chamber.¹³ What vary from one ventricle to another are the load and the parameters that govern the recoil/suction process (assuming a normal mitral valve).

Diastolic function

The above basic principles immediately demonstrate that systole and diastole must be mechanically coupled. In other words, ventricular contraction stores elastic energy in the tissue (as in compressing an equivalent spring), which powers the chamber wall recoil process. Implicit in this conceptual framework is that relaxation (sarcomere cross-bridge uncoupling) proceeds in a quantifiable manner. The key mechanistic advantage of the aforementioned framework is that both chamber properties of stiffness (property of equivalent spring to generate recoil) and relaxation (rate and extent of cross-bridge uncoupling that modulates recoil process) simultaneously determine global diastolic function. It is self-evident that diastolic function depends on the load (ie, the volume ejected affects end-systolic strain and the storage of elastic potential energy in tissue).

Common misconceptions remain about how the heart works when it fills. Diastolic function can be understood in a more intuitive way by recalling that the LV initiates filling by being a mechanical suction pump at, and for a little while after, mitral valve opening. That means that at mitral valve opening, blood does not get pushed into the ventricle by the atrium, but is instead mechanically aspirated by the ventricle.^5,12,14 The answer to the question: “Where does the energy that drives suction come from?” is, systole. As the chamber contracts, overcomes the arterial load, and ejects, it simultaneously compresses the elastic elements. This stored elastic strain energy is released when the cardiac muscle relaxes, and the elastic elements generate the recoil of the ventricle until a fully relaxed equilibrium configuration of the chamber is achieved – usually during diastasis.

To fill effectively, the ventricle must become compliant during filling (not be too stiff), quickly relax the “cramp” that was the previous systole (effective cross-bridge uncoupling to achieve relaxation), and physically accommodate the aspirated blood volume. Thus, stiffness and relaxation are intrinsic ventricular properties that determine how the tissue moves and are useful lumped parameters that determine and thereby quantitate diastolic function. It also follows that volumetric load (also termed preload) represents another extrinsic parameter that modulates the filling process.

Conventional Measures of Global Diastolic Function

The preferred method is echocardiography and, more specifically, Doppler measurement of transmitral flow. An excellent review is available¹⁵ Recommendations for LV DF assessment have been published jointly by European Association of Echocardiography (EAE) and American Society of Echocardiography (ASE).¹⁶

Briefly, as a first approximation, E- and A-wave contours are simplified as having triangular shape, and triangle-based indexes, such as peak heights of the E- and A-waves and their ratio (E_peak, A_peak, E_peak/A_peak), the deceleration time and width of the E-wave (DT, E_dur), and the velocity time integral (VTI) of the E- and A-waves (Fig. 1A), are routinely measured. The triangular approximation to E-wave shape is convenient, but current imaging technology allows the curvature of E-wave contours to be discerned (see Fig. 1B). Regrettably, the physiologically important curvature features of the E-wave are usually disregarded. However, the benefit in understanding the physical and physiologic principles that govern E-wave shape and its curvature allows additional information to be extracted as detailed below [the parametrized diastolic filling (PDF) formalism].

Figure 1.

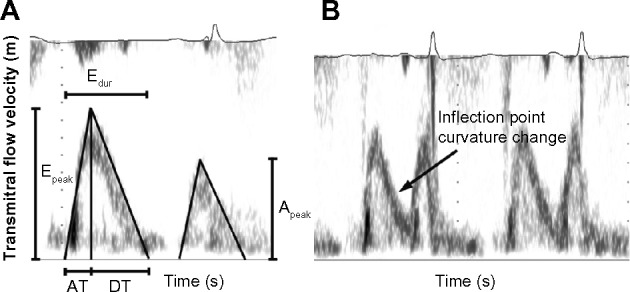
(A) The current conventional triangle approximation to characterize E- and A-wave shapes. Using this approximation, E_peak, A_peak, the duration of E-wave (E_dur), AT, DT, E_peak/A_peak, and E_VTI/A_VTI are measured. (B) The often observed curvature change (inflection point) of the E-wave contour that is not a feature of the triangle approximation to E-wave shape. See text for details.

The measurement of even smaller (ie, tissue) velocities permits measurement of the longitudinal mitral annular motion. The shapes of mitral annular Doppler tissue imaging (DTI) velocity contours are assumed (incorrectly) to be triangles, with measurement restricted only of the peak E’. E’ had value for estimation of end-diastolic pressure.¹⁴ It is possible to extract physiologic information from the curvature and oscillations of the E’-wave, as discussed below.

Additional capabilities include speckle tracking,¹⁷ which provides strain (change in length/per unit length) and strain rate (change in length/per unite length/per second) measurements. Importantly, speckle tracking is necessarily a local or regional metric, and its utility in determining global diastolic function remains an area of investigation.

Causality-Based Measures of Global Diastolic Function: the PDF Formalism

Using triangles to approximate E-wave contours has obvious limitations. Even less sensitive are metrics such as E/A or E-wave deceleration time.

By considering the heart as a suction pump and mathematically modeling its bulk motion, it is possible to gain deeper understanding into the physiologic determinants of the E-wave and its features such as its curvature.

The filling process can be modeled in analogy to the motion of a recoiling spring (a mechanical oscillator) that has an inertial load and is moving against a resistance.¹⁸ The model is the PDF formalism. More specifically, the formalism (kinematic model) is a way to analyze the Doppler E-wave and is derived from Newton’s laws of motion. It characterizes the E-wave in terms of a driving force (the atrioventricular pressure gradient), a damping/viscous force (relaxation or cross-bridge uncoupling that modulates recoil) resisting or opposing transmitral flow, and an inertial load due to tissue and blood.

The model involves solving the associated (differential) equation of motion, whose solution provides mitral flow velocity as a mathematical prediction of the solution to the equation of motion. Equivalently stated – all ventricles are mechanical suction pumps and fill by obeying the same law of motion. The features that differentiate diastolic function in one heart from another are the parameters that specify the motion, and they vary from heart to heart.

Each transmitral flow velocity (E-wave) contour is uniquely determined by three PDF model parameters: the initial displacement of the analogous spring x_o (cm) [linearly related to E-wave VTI (ie, volumetric preload)],¹⁴ the stiffness of the “spring” denoted by parameter k (g/s²) that is linearly related to chamber stiffness (dP/dV),¹⁹ and a resistance parameter or chamber viscoelasticity index c (g/s), which accounts for energy losses that – by modulating recoil through relaxation – effectively act to oppose the filling process.¹⁴ In geometric terms, the spring constant k primarily determines the width of the E-wave. A high spring constant (stiff spring) generates a tall, narrow E-wave, as seen in the “constrictive-restrictive” pattern. The resistance/damping/relaxation parameter c is mainly responsible for modulating the deceleration portion or tail of the E-wave and is directly responsible for the inflection point (change in curvature from “cup-down” to “cup-up”) in the deceleration portion. An elevated value for the resistance/damping parameter in general, relative to normal, gives rise to E-wave having the “delayed relaxation” pattern.

The three PDF model parameters (x_o, c, and k) for each E-wave can be obtained through model-based image processing (MBIP), detailed in Figure 2. Briefly, after the E-wave is selected, its maximum velocity envelope is identified and is fit numerically by the solution to the PDF equation of motion, yielding the three best fit PDF parameters (x_o, c, k) and a measure of goodness of fit. The details of the method and instructions as to its use by anyone seeking to perform E-wave analysis have been detailed elsewhere.²⁰

Figure 2.

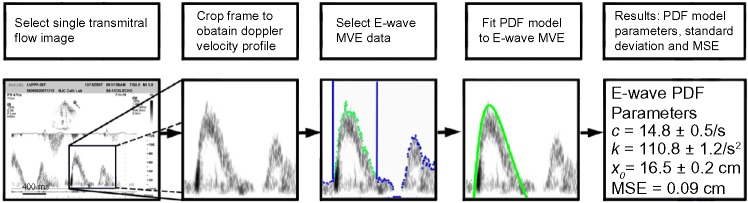
Quantitation of diastolic function via the PDF formalism. As the sequence of panels shows, the raw echo (DICOM) image is frame-grabbed, the E-wave is cropped, and the maximum velocity envelope (MVE) is identified and fitted numerically by the solution to the PDF model, yielding the three unique best fit PDF parameters of load, relaxation and chamber stiffness (x_o, c, k) and a measure of goodness of fit. See text for details.

Diastolic function quantitation by analysis of E-wave using the PDF has shown that diabetes increases the resistance/relaxation parameter c in both rats²¹ and humans.²² Importantly, these two studies showed that for hearts with normal left ventricular ejection fraction (LVEF), in contrast to the relaxation/viscosity parameter c, conventional E-wave indexes such as E_peak or DT were unable to differentiate between normal and diabetic subjects. A further insight provided by the approach is that, because energy must be lost during the filling process due to the resistance/relaxation (cross-bridge uncoupling that modulates recoil) term in the equation of motion, all ventricles fill to a diastatic volume that must be less than the maximum (lossless) ideal filling volume. An index that characterizes this efficiency of filling, the kinematic filling efficiency index (KFEI), has been shown to be lower in normal LVEF diabetic hearts compared to nondiabetic normal controls.²³ Furthermore, the PDF parameter c has been shown to be linearly related to simultaneous chamber relaxation characterized by the (invasively determined) time-constant of isovolumic relaxation.²⁴

Doppler Tissue Imaging

DTI has become routine in diastolic function assessment, including the ability to image the E’-wave and its subsequent multiple annular oscillations, which have been previously noted.^25,26 Such oscillations may have been considered to be noise. Importantly, these annular oscillations obey the laws of oscillatory motion and therefore are amenable to quantitation.^27–29 The presence or absence of annular oscillations is often encountered in clinical imaging using DTI.

The ability to image longitudinal motion and radial motion to high precision has permitted the characterization of spatially distinct compensatory mechanisms in diastole. In other words, because maintenance of cardiac output via maintenance of stroke volume constitutes the prime directives for survival, it follows that impairment of longitudinal motion should be accompanied by compensation in radial motion in order to preserve stroke volume.

The information obtained from DTI holds great potential for diagnosis of diastolic relaxation abnormalities. The absence of mitral annulus oscillation (MAO) is a good predictor of relaxation-related diastolic dysfunction. In prior work,³⁰ we found that patient groups without MAO tend to have prolonged τ and isovolumic relaxation time (IVRT), lower E_peak/A_peak and E’-waves, and increased E_peak/E′.

Solution of the Load-independent Index of Diastolic Function Problem

An obvious problem in the quantitation of diastolic function relates to the load dependence of the conventional metrics proposed to date. Some correlations regarding only a limited dependence on load have been made regarding E′, but these are merely correlation, rather than causal-mechanism-based. The traditional (E_peak/A_peak, VTI, AT, DT) and newer echo-derived indices (E/E′, strain, strain rate, Vp, etc) are known to be load-dependent. Hence, a load-independent index of diastolic function (LIIDF), though highly sought and desired, has not been available.

Because a LIIDF is not available, clinical practice has averaged measurements from multiple beats. Specifically, for E_peak, several consecutive E-waves are recorded, and then reported as the average E_peak. Thus DF information present in beat to beat, ie, load variation, is lost due to averaging.

The kinematic modeling approach to diastolic function characterization has allowed us to solve the LIIDF problem.³¹ The key insight lies in analyzing not just one, but several E-waves, acquired at varying load states, and, rather than averaging and losing load-dependent information, making use of the variability of each E-wave in response to load. To determine the LIIDF, we compute the three PDF parameters: stiffness (k), damping (c), and initial load parameters (x_o), for each E-wave. In addition, E_peak is recorded. For each E-wave, the product of k and x_o provides model-predicted maximum driving force, which is the analog of the peak instantaneous atrioventricular pressure gradient. The product of each E-wave’s c and E_peak defines the maximum resistive force opposing filling. Thus, for each load-varying E-wave, we compute kx_o and the associated cE_peak, and for each E-wave we plot a point in the kx_o versus cE_peak coordinate system. The LIIDF is the slope, called M, of the linear regression between all (cE_peak, kx_o) points, where each point is generated by a separate E-wave obtained under a different load.

In healthy volunteers undergoing tilt-table maneuvers, despite the dramatic visual E-wave shape variation, all associated (cE_peak, kx_o) points remained colinear (R² = 0.98), demonstrating that the slope of the kx_o versus cE_peak relation is independent of load. Furthermore, we found that, in subjects undergoing cardiac catheterization, the LIIDF was significantly lower in subjects having diastolic dysfunction (elevated filling pressures >18 mmHg), compared to subjects with normal diastolic function. Thus, the LIIDF is easily determined from a series of load-varying E-waves; it is constant in the setting of load variation, and differentiates between normal versus diastolic dysfunction subjects. The practical utility and clinical power of the LIIDF resides in its application in sequential studies where each subject serves as his/her own control. Hence, whether therapy has resulted in “beneficial” or “adverse remodeling” of the chamber can be assessed in load-independent terms. Furthermore, the LIIDF in and of itself can serve as a therapeutic target.

Summary

In addressing the issue of diastolic function in heart failure, emphasis has been on viewing diastolic function as a problem to be solved, rather than correlating mere features of transmitral waveforms with clinical features. By understanding that diastolic function is actually a measure of the suction pump attribute of the chamber, causally determined by chamber properties of stiffness, relaxation, and their response to load, it is possible to characterize diastolic function in accordance with the laws of motion that apply to all hearts. Measurement of diastolic function then becomes the act of analyzing E-waves (or E’-waves) via the PDF formalism and determining the model-derived indexes of stiffness, relaxation, and load. These metrics allow sensitive and specific differentiation of diastolic dysfunction states relative to controls³² and provide further opportunity to assess chamber remodeling via the LIIDF. The method is ideally suited to more fully characterize the physiology of HFpEF^33,34 using echocardiography.