The Effects of Walking Speed on Tibiofemoral Loading Estimated Via Musculoskeletal Modeling

Abstract

Net muscle moments (NMMs) have been used as proxy measures of joint loading, but musculoskeletal models can estimate contact forces within joints. The purpose of this study was to use a musculoskeletal model to estimate tibiofemoral forces and to examine the relationship between NMMs and tibiofemoral forces across walking speeds. We collected kinematic, kinetic, and electromyographic data as ten adult participants walked on a dual-belt force-measuring treadmill at 0.75, 1.25, and 1.50 m/s. We scaled a musculoskeletal model to each participant and used OpenSim to calculate the NMMs and muscle forces through inverse dynamics and weighted static optimization, respectively. We determined tibiofemoral forces from the vector sum of intersegmental and muscle forces crossing the knee. Estimated tibiofemoral forces increased with walking speed. Peak early-stance compressive tibiofemoral forces increased 52% as walking speed increased from 0.75 to 1.50 m/s, whereas peak knee extension NMMs increased by 168%. During late stance, peak compressive tibiofemoral forces increased by 18% as speed increased. Although compressive loads at the knee did not increase in direct proportion to NMMs, faster walking resulted in greater compressive forces during weight acceptance and increased compressive and anterior/posterior tibiofemoral loading rates in addition to a greater abduction NMM.

Walking is the most common form of physical activity and is generally assumed to expose participants to relatively small risk of musculoskeletal injury.¹ However, walking-related musculoskeletal injuries, particularly at the knee joint, are not uncommon, affecting approximately 18% of those who walk for exercise each year.² These injuries may be due, at least in part, to relatively large loads distributed across the knee joint. In addition, it is hypothesized that repetitive compressive loading may be a key mechanical factor preceding the onset of osteoarthritis (OA).³ Surprisingly, there is little data regarding how walking conditions (eg, speed, grade) affect loads (ie, contact forces) across lower extremity joints. Musculoskeletal injuries and excessive or abnormal loading have both been linked to the onset and progression of OA,^3,4 therefore, improving our understanding of how walking speeds affect knee joint loading will enhance our ability to recommend effective walking-based physical activities.

Indirect estimates of knee joint loading suggest that loads increase with walking speed. Knee net muscle moments (NMMs), derived via inverse dynamics, have been used as a proxy measure of knee joint loads. Specifically, sagittal and frontal plane knee NMMs have been used to gain insight into compressive and mediolateral distribution of knee joint loads.^5–7 Peak early stance knee extension NMMs have been reported to increase by 140% to 250% when walking speed is increased from 0.75 m·s⁻¹ to 1.5 m·s⁻¹,^8,9 primarily due to increased knee flexion angles and ground reaction forces as walking speeds increase.^10,11 Knee extensor muscle activity has also been shown to increase with walking speed,^10,12 suggesting that greater knee extension NMMs require increased knee extensor muscle forces.¹³ Because these muscle forces are the primary contributors to the compressive tibiofemoral joint contact force, greater extension NMMs should be associated with greater compressive tibiofemoral contact forces during walking.¹⁴ However, NMMs do not account for antagonist co-contraction, which may limit their ability to estimate these joint loads. Peak early stance knee abduction NMM, a measure of mediolateral distribution of knee joint loads, increases with walking speed.¹⁵ This suggests that medial compartment knee joint loads increase with walking speed. Given that stance time decreases while ground reaction forces and NMMs increase as walking speed increases, the rate of joint loading would also increase with walking speed.⁵ Combined, these results demonstrate that the loading environment (magnitude, distribution and rate) in the knee is affected by walking speed.

The advent of artificial joint replacements with force transducers and telemetry systems has allowed researchers the ability to measure in vivo loading characteristics of the knee during gait.^16–18 Only one study to date has quantified the effects of walking speed on tibiofemoral contact forces using a force measuring implant. D’Lima et al¹⁸ reported no significant changes in contact forces while individuals walked at speeds ranging from 0.47 m·s⁻¹ to 1.34 m·s⁻¹; however, they did report a significant increase in contact forces (from 2.2–3.0 times body weight [BW]) when speed was increased from 1.34 to 1.79 m·s⁻¹. Unfortunately, these results may not be generalizable to healthy adults due to the limited participant sample size, altered knee architecture, and age/pathology of the participants.

Musculoskeletal modeling offers researchers a means to estimate in vivo contact forces in non-total knee arthroplasty populations. Simplified mathematical models, such as described by DeVita and Hortobagyi,^19,20 have been used to estimate the compressive (axial) and anterior/posterior (A/P) shear tibiofemoral contact force distribution during walking. While these models are superior to using only sagittal plane NMMs for estimating contact forces, they are limited in that they use only a few major muscle groups (eg, quadriceps), allow only limited co-contraction, and do not include a patella-femoral joint. Recently, more robust musculoskeletal models, which allow computation of individual muscle forces and estimates of joint loading, have become more widely available.^21–24 These models have been used to examine individual muscle force contributions to axial knee contact forces,¹⁴ as well as the function of individual muscles with speed.^24–26 To date, however, studies which have used musculoskeletal modeling to investigate joint loading across walking speeds are limited. While validating a musculoskeletal model against an instrumented knee implant for one subject, Kim et al²⁷ reported 35% increase (approximately 2.1 BW to 2.85 BW) in estimated tibiofemoral forces from 0.80 m·s⁻¹ to 1.52 m·s⁻¹, with very close agreement to measured tibiofemoral forces. To our knowledge, no studies have directly quantified the effects of speed on model-estimated tibiofemoral forces and compared these estimated forces to proxy measures of loading, such as sagittal plane NMMs in healthy adults.

The purpose of this study was to use musculoskeletal modeling to examine how the loading environment of the knee joint (primarily magnitude, mediolateral distribution, and rate of tibiofemoral loading) changes with walking speed. A secondary purpose was to determine the relationship between knee extension NMMs and compressive tibiofemoral forces. We hypothesized that tibiofemoral forces would increase with walking speed. However, based on differences in the magnitude of change between reported NMMs and measured in vivo tibiofemoral contact forces, we further hypothesized that increases in knee extension NMMs would be larger than increases in model-estimated tibiofemoral contact forces.

Methods

Subjects

Data from 10 participants (7 male and 3 female) was used in this experiment. Participants were in good health with no known acute/chronic disease or limitations to physical activity, sedentary to lightly active (<4 hours of physical activity per week),²⁸ and nonobese, with a body mass index (BMI) of less than 25 kg/m². Physical characteristics of the participants are shown in Table 1. Subjects gave written informed consent approved by the Colorado State University human research institutional review board.

Table 1.

Physical characteristics of participants

Item	Mean (SD)
Age (years)	23.6 (2.5)
Height (m)	1.78 (0.09)
Body Mass (kg)	67.2 (12.0)
BMI (kg/m2)	21.2 (2.1)

Experimental Protocol

As part of a larger study, each participant attended three experimental sessions on separate days, which have been described in detail previously, but are outlined briefly here.⁵ During the first visit, subjects completed a health screening, body composition assessment via dual X-ray absorptiometry (DEXA, Hologic Discovery, Bedford, MA), and a graded exercise stress test to determine maximal oxygen uptake (VO₂max). During the second and third sessions subjects walked, wearing shoes, at 16 randomized walking speed/grade combinations (8 per session). Treadmill speeds ranged from 0.50 m·s⁻¹ to 1.75 m·s⁻¹ and grades ranged from −3° to 9°. Trials were 6 minutes in duration and subjects rested for 5 minutes between trials. Before data collection, subjects were given an acclimatization period, where they walked at a comfortable, self-selected, pace for up to 10 minutes. Data from level walking at 0.75, 1.25 and 1.50 m·s⁻¹ were used for the current study.

Experimental Data

To record kinematic and kinetic data, we used a seven-camera, three-dimensional motion capture system (Nexus, Vicon, Centennial, CO) and a dual-belt, inclinable, force-measuring treadmill (Fully Instrumented Treadmill; Bertec Corp, Columbus, OH). To identify anatomical landmarks and delineate lower extremity segments, we placed lightweight retro-reflective, spherical markers on each subject in accordance with a modified Helen Hayes marker set. Markers were placed on the sacrum, left and right anterior superior iliac spines, sternum, clavicle, 10th thoracic vertebra, and 7th cervical vertebra. Additional markers were placed on the left and right midthigh, femoral epicondyles, midshank, lateral malleoli, and the 2nd metatarsal head and calcaneus of each foot. To account for any adipose tissue over the anterior pelvis, pelvic width was measured via the DEXA scan digital image. A pelvic depth to pelvic width ratio of 83.7% for females and 74.3% for males was used to estimate pelvic depth.²⁹ Two virtual anterior superior iliac spine markers were created anterior to the sacrum marker by a distance equivalent to pelvic depth calculated by the previous ratios and laterally by one half the distance of the DEXA measured pelvic width. Marker trajectories were recorded at 100 Hz while ground reaction force and moment data were recorded at 1000 Hz by force platforms embedded underneath each treadmill belt. We also recorded electromyographic (EMG) data (Noraxon, Scottsdale, Arizona) using International Society for Electrophysiology and Kinesiology standard procedures.³⁰ We used bipolar surface electrodes and recorded data from medial gastrocnemius, vastus lateralis, vastus medialis, biceps femoris long head, and semimembranosus muscles at 2000 Hz. The data were band-pass filtered (16–380 Hz), fully rectified and low-pass filtered at 7 Hz to generate a linear envelope. We collected motion capture and EMG data for 30 seconds during the final minute of each trial. Coordinate and kinetic data were digitally low-pass filtered at 5 Hz and 12 Hz, respectively, using fourth order zero-lag Butterworth filters.

Musculoskeletal Modeling

We used a generic OpenSim musculoskeletal model that was scaled for each subject to account for the anthropometrics of each individual.³¹ The 12 segment, 19 degree of freedom, 92 muscle Open-Sim musculoskeletal model used in the study is based on a lower extremity musculoskeletal model, originally developed by Delp et al,³¹ combined with an upper body segment connected to the pelvis by a 3 degree of freedom ball and socket joint. The tibiofemoral joint was modeled as a single degree of freedom planar joint, with anterior/posterior and superior/inferior translations prescribed as a function of knee flexion. The knee joint in this model also included a planar patellofemoral joint based on kinematics from Yamaguchi et al³² and Delp et al.³¹ However, the knee mechanism in this augmented model differed in that the patella articulated with the femur, rather than the tibia, yielding more physiologically relevant tibiofemoral and patellofemoral joint contacts.³³ The quadriceps muscles (vastus lateralis, vastus intermedius, vastus medius, and rectus femoris) wrapped around the patella as if it were a frictionless pulley, then terminated on the tibial tuberosity. To report the NMMs in the frontal plane at the knee, the knee joint definition of each musculoskeletal model was customized to include an optional abduction/adduction degree of freedom. This abduction/adduction degree of freedom was only included when calculating NMMs. However, since the model lacked constraints in the frontal plane (ie, ligaments and soft tissue) to control knee abduction/adduction, this degree of freedom was locked while calculating the model kinematics. Thus, the same kinematic solution was applied during the computation of NMMs, muscle forces, and joint contact forces. Three force actuators and three torque actuators were applied to the pelvis to account for dynamic inconsistencies between experimental forces, measured kinematics, and model parameters (segment geometry, segment mass distribution, lack of arms, etc.).

We used OpenSim’s inverse kinematics analysis to determine the joint angles across each gait cycle by minimizing the errors between the experimental and virtual marker trajectories and the markers defined on each scaled model. OpenSim’s inverse dynamics analysis was used to calculate the model generalized forces that reproduced the measured kinematics given the ground reaction forces at 0.75, 1.25, and 1.50 m·s⁻¹.²¹ The knee extension and abduction/adduction generalized forces represent the knee extension and abduction/ adduction NMMs, respectively. OpenSim’s Joint Reaction analysis was used to compute the intersegmental reaction forces using the generalized forces as inputs.³³

Muscle forces were estimated using the OpenSim static optimization algorithm that minimizes the sum of squared muscle activations while reproducing the required NMMs at all joints in the model.²¹ In addition, the static optimization algorithm used individual muscle weighting constants (integer values that can range from 1 to 10) for the major flexor and extensor muscles that cross the knee joint. We used weighting constants of seven for the gastrocnemius, three for the hamstrings and one for all other muscles in the model as these constants have previously resulted in good agreement between model estimated tibiofemoral forces with those experimentally measured from an individual with an instrumented total knee replacement.^33,34 Tibiofemoral contact forces were computed using the Joint Reaction analyses with the muscle forces from static optimization as inputs.³³ These tibiofemoral forces represent the resultant forces and moments that the tibiofemoral joint structure carries due to all muscle forces, external loads, and inertial loads of the model. The compressive tibiofemoral force was computed as the component of the resultant force acting on the tibia and parallel to the long axis of the tibia, while the anterior-posterior and mediolateral shear components of the contact force were orthogonal to the axial component. Joint contact force, muscle forces, and intersegmental forces were normalized to BW of each subject, while NMMs were normalized to body mass. We calculated axial and A/P tibiofemoral loading rates for each subject as the difference between the maximum and minimum tibiofemoral forces in each direction during the first 20% of the gait cycle divided by the time duration between the extremes. All data were collected from the right leg for five gait cycles, normalized to each gait cycle, averaged across gait cycles for each subject, and then averaged across subjects to obtain group means at each speed.

Statistical Analysis

One-way repeated-measures ANOVA analyses were used for comparisons of NMMs and tibiofemoral forces between speeds. When a significant main effect was observed, post hoc comparisons were made using the Holm-Sidak method. A criterion of P < .05 defined significance. We also used linear regression to determine the relationship between peak early stance knee extension NMMs and the associated compressive tibiofemoral force.

Results

All participants completed the three trials; hence we present mean values based on the data from ten participants. Mean and peak residual forces applied at the pelvis averaged less than 2.8% and 18% BW, respectively, indicating that our musculoskeletal simulations were relatively dynamically consistent.

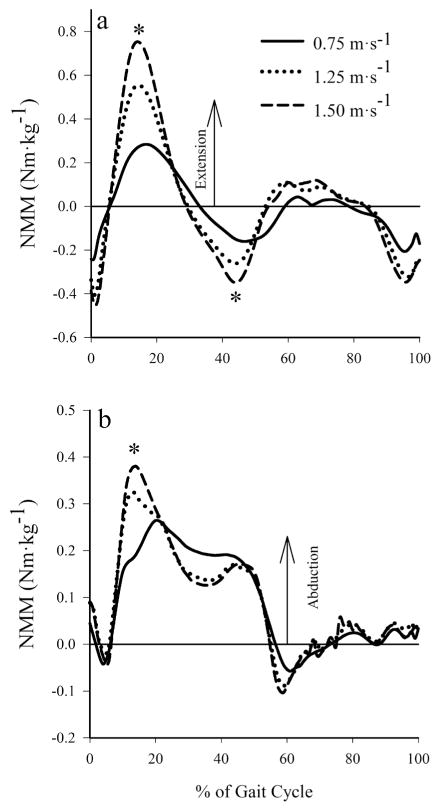
Peak NMMs increased with walking speed (Table 2, Figure 1). Peak knee extension NMMs occurred slightly earlier during stance as speed was increased (14% of the gait cycle at 1.50 m·s⁻¹ versus 16% at 0.75 m·s⁻¹) and increased 168% when walking speed increased 0.75 m·s⁻¹ to 1.50 m·s⁻¹ (P < .001; Figure 1a). The peak knee flexion NMM occurred during late stance and increased by 63% when walking speed increased from 0.75 m·s⁻¹ to 1.50 m·s⁻¹ (P = 0.001; Figure 1a). The peak magnitude of the internal abduction moment during early stance increased by 41% across walking speeds (P = .003; Figure 1b).

Table 2.

Peak knee extension/flexion and abduction NMMs at the knee across walking speeds

Speed (m·s−1)	Knee Extension (N·m·kg−1)	Knee Flexion (N·m·kg−1)	Knee Abduction (N·m·kg−1)
0.75	0.32 (.10)	−0.22 (.07)	0.28 (.04)
1.25	0.57 (.10)*	−0.28 (.07)*	0.35 (.02)
1.50	0.77 (.08)*	−0.36 (.08)*	0.41 (.02)*

Note. Values are mean (SE).

^*indicates significant difference from 0.75 m·s⁻¹.

Figure 1.

Mean knee net muscle moments at 0.75, 1.25, and 1.50 m·s⁻¹: (a) knee extension NMM. (b) internal abduction NMM. Peak NMMs at the knee showed a significant positive trend with walking speed. *Significant main effect of speed on NMM.

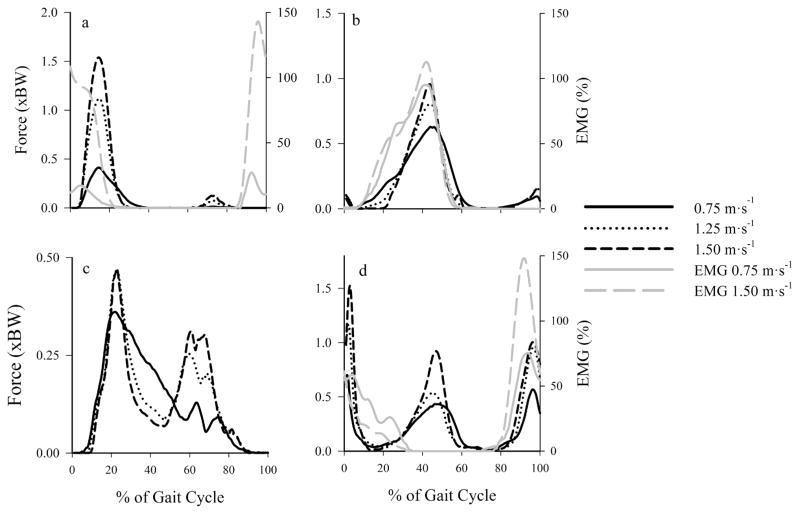
Estimated muscle force production generally increased with speed, while the timing of model estimated muscle activity remained relatively constant across walking speeds (Figure 2). We qualitatively compared the estimated muscle forces with our EMG data and found relatively good agreement between these two variables. The vasti muscle group showed the largest force production increase (approximately 300%) across walking speeds (Figure 2a), while other muscle groups, such as the gastrocnemius group (Figure 2b), showed relatively smaller (50%) increases in force production with speed. Estimated hamstring muscle force output ended earlier during stance than indicated by semimembranosus EMG while quadriceps muscle force output began in early stance compared with onset of EMG during late swing. Thus, compressive tibiofemoral forces during these periods of the gait cycle may be underestimated.

Figure 2.

Mean estimated muscle forces (black) and EMG (gray) crossing the knee joint at 0.75 m·s⁻¹, 1.25 m·s⁻¹, and 1.50 m·s⁻¹. Muscles grouped and summed by similar activation patterns: (a) vasti (vasti lateralis, vasti medialis, vastus intermedius), (b) gastrocnemius, (c) rectus femoris, and (d) hamstrings (semimembranosus, semitendinosus, and biceps femoris short/long head). Gracilis, sartorius, and tensor fascia latae not shown due to small force output (<0.10 BW across speeds). The EMG was normalized to peak values recorded during walking at 1.25 m·s⁻¹ and are expressed as a percentage. The EMG is from muscles in bold. Rectus femoris EMG was not available. The mid-gait-cycle hamstring muscle force production was due to the biceps femoris short head, which was not assessed with EMG.

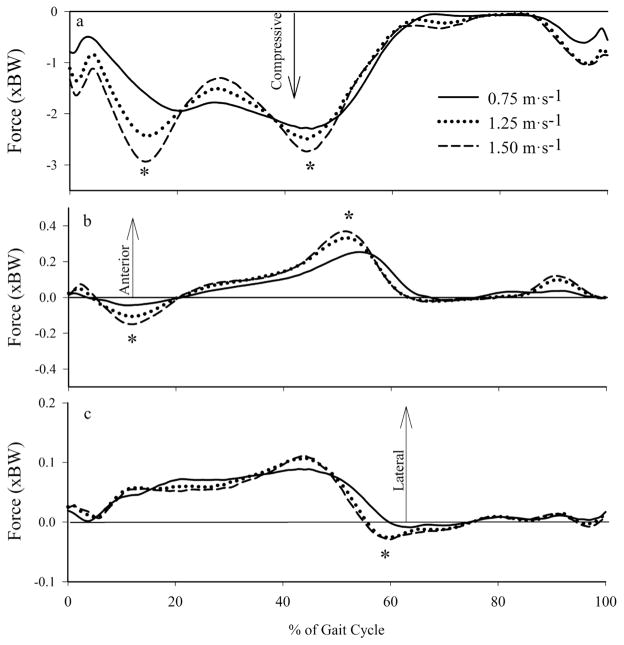
Tibiofemoral forces increased with walking speed (Table 3, Figure 3). Peak compressive tibiofemoral forces during early stance increased by 52% as walking speed increased from 0.75 to 1.50 m·s⁻¹ (P < .001). Peak late stance compressive tibiofemoral forces also increased significantly between 0.75 and 1.50 m·s⁻¹ (P = .008). At the instant associated with the peak knee extension NMM during early stance, compressive tibiofemoral forces increased approximately 59% from 1.83BW to 2.91BW. Peak anterior/posterior shear forces at the tibiofemoral joint during early and late stance increased across all walking speeds (P < .001; Figure 3b). Peak mediolateral tibiofemoral force increased significantly during early (P = .018) and late stance (P < .001) with walking speed (Figure 3c). The compressive and axial tibiofemoral loading rates both increased approximately 3-fold between the slowest and fastest speeds (P < .001), from 6.58 BW·s⁻¹ to 19.59 BW·s⁻¹ and 1.04 BW·s⁻¹ to 3.05 BW·s⁻¹ at 0.75 m·s⁻¹ and 1.50 m·s⁻¹, respectively.

Table 3.

Peak compressive and anterior/posterior tibiofemoral forces (TF) across walking speeds

Speed (m·s−1)	Early Stance TF Force (BW)	Late Stance TF Force (BW)	Early Stance A/P TF Force (BW)	Late Stance A/P TF Force (BW)
0.75	−2.00 (.15)	−2.37 (.19)	−0.06 (.01)	0.26 (.02)
1.25	−2.56 (.16)*	−2.56 (.17)	−0.13 (.02)*	0.34 (.02)
1.50	−3.04 (.22)*§	−2.79 (.21)*	−0.16 (.02)*§	0.40 (.03)*

Note. Values are mean (SE).

^*indicates significant difference from 0.75 m·s⁻¹;

^§indicates significant difference from 1.25 m·s⁻¹.

Figure 3.

Mean tibiofemoral forces at 0.75, 1.25, and 1.50 m·s⁻¹: (a) axial, (b) anterior/posterior, and (c) medial/lateral forces. *Significant main effect of speed on joint contact/shear forces.

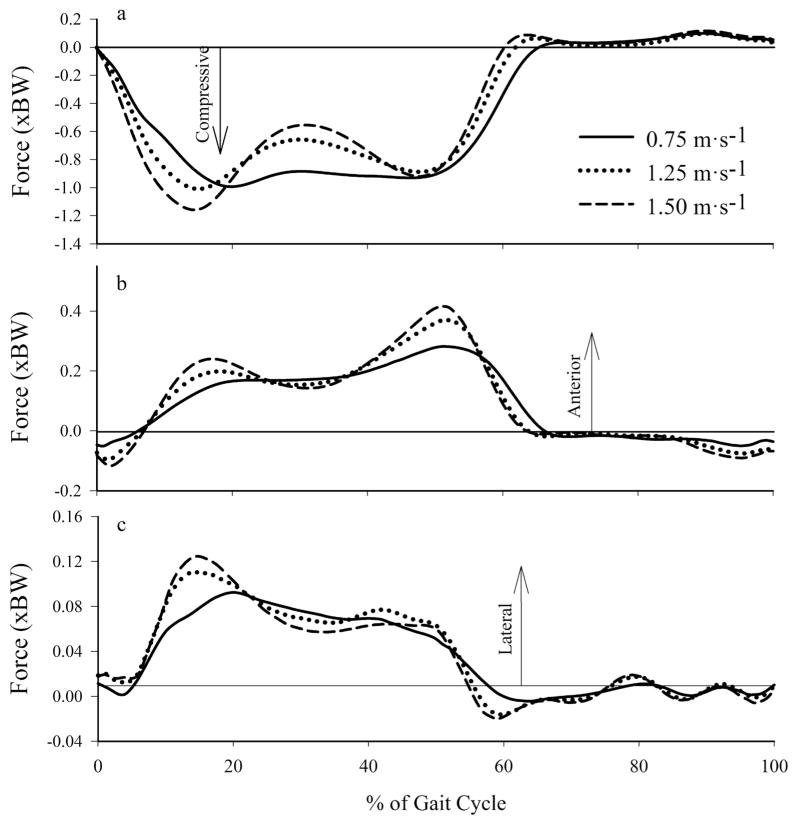
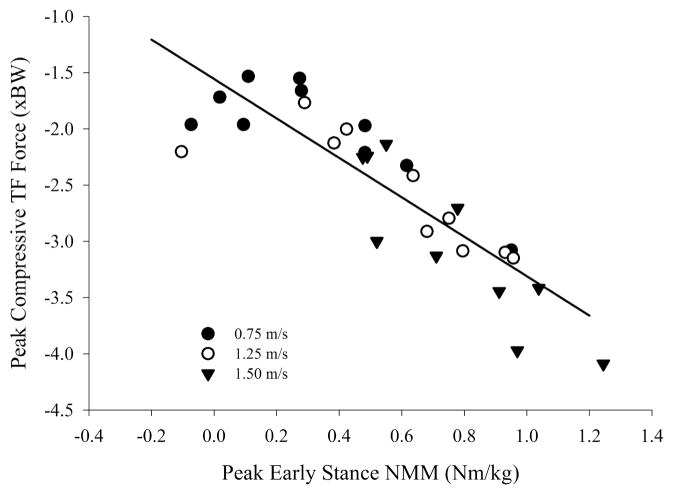
Based on the difference between the total contact force and intersegmental forces, we found that muscles spanning the knee joint were the primary contributors to the compressive tibiofemoral force, and increased their relative contributions with increased walking velocity. The axial intersegmental force (computed via inverse dynamics) at the tibiofemoral joint (Figure 4a) was responsible for approximately 51% and 41% of the peak compressive tibiofemoral force occurring during early and late stance, respectively, at 0.75 m·s⁻¹ and approximately 39% and 32% during early and late stance, respectively, at 1.50 m·s⁻¹. Linear regression analysis showed that peak knee extension NMM explained approximately 75% of the variance in compressive tibiofemoral force (Figure 5).

Figure 4.

Mean (a) axial, (b) anterior/posterior, and (c) medial/lateral intersegmental forces at the tibiofemoral joint across walking speeds.

Figure 5.

Peak knee extension NMM vs. early-stance axial tibiofemoral force. Tibiofemoral forces increased with knee extension NMM (R² = .75, P < .001). The linear regression equation is F_TF = −1.557 – (1.753 × M_EXT). F_TF: early-stance peak axial tibiofemoral forces, and M_EXT: peak extensor NMMs.

Discussion

We accept our first hypothesis that peak compressive tibiofemoral forces would increase with walking speed. During early stance, peak knee extension NMMs were 170% greater at 1.50 m·s⁻¹ than 0.75 m·s⁻¹, while the corresponding compressive tibiofemoral force at the same instant increased by 52%. During late stance, there was a 63% increase in the peak knee flexion NMM, while peak compressive tibiofemoral forces increased by only 18%. This supports our secondary hypothesis that knee extension NMMs would increase by a greater magnitude than compressive tibiofemoral forces estimated via a musculoskeletal model.

Our model-based estimates of compressive tibiofemoral loading yielded two distinct peaks during stance, the first occurring during early stance (15% of the gait cycle) and the second during late stance (45% of the gait cycle). This pattern and magnitude (peaks of approximately 2.0–3.0 BW) is consistent with reported in-vivo measurements,^16,35 and model-based estimations^14,27 of compressive tibiofemoral loads during gait, though smaller in magnitude than some estimations derived via a computed muscle control approach in OpenSim (3.5–4.5 BW).³⁶ We observed increases in model-based estimates of compressive tibiofemoral forces across walking speeds that are similar to those measured via instrumented knee implants. D’Lima et al¹⁸ reported that peak tibiofemoral joint contact forces increased 35% (2.2 BW to 3.0 BW) between 0.45 m·s⁻¹ and 1.79 m·s⁻¹. While D’Lima et al reported similar tibiofemoral forces across slower speeds with a greater force at the fastest speed; our results show an increase in peak forces as speed increased. It may be that individuals who have undergone joint replacement have learned to adopt a gait pattern (ie, slower speeds with less flexed stance) to reduce joint loads, but at higher speeds are not able to maintain this strategy and thus joint loads increase. In contrast, our healthy participants gradually increased knee flexion with walking speed, and this may explain why we observed a more consistent increase in compressive loading with speed.

Changes in sagittal plane NMMs did not directly reflect the magnitude of changes in compressive joint loading associated with walking speed. The peak extension NMM increased by 170% between 0.75 and 1.50 m·s⁻¹, while the compressive tibiofemoral force increased 52% at the same instant. In addition, peak flexion NMMs increased by approximately 63% between 0.75 and 1.50 m·s⁻¹, while the peak compressive tibiofemoral force during late stance increased by only 18%. The significant effects of speed on sagittal plane NMMs reported in this study are supported throughout the literature. Schwartz et al¹⁰ showed a 175% increase in sagittal-plane extension NMMs from slow to fast walking speeds, and a nearly 75% increase in peak sagittal-plane flexor NMMs across speeds. In addition, Lelas et al⁹ document large (250%) increases in knee extension moments across a range of speeds similar to those used in this study. NMMs and compressive tibiofemoral forces both increase in magnitude with speed, and a regression analysis of peak extension NMMs and peak early-stance compressive tibiofemoral forces shows a good correlation between these two measures (R² = .75, Figure 5). Therefore, peak knee extension NMM may offer relatively good predictive ability of peak tibiofemoral forces, but they do not increase in direct proportion.

Three factors may have contributed to the larger increase in peak knee extension NMM compared with compressive tibiofemoral force across walking speeds. First, as walking speed increased, vasti and rectus femoris muscle force production increased while early gastrocnemius force output during this part of the gait cycle decreased (less co-contraction). The reduced contribution by the biarticular gastrocnemius to the compressive tibiofemoral force at fast speeds may explain why the compressive tibiofemoral force does not increase by the same amount as the knee extension NMM. Second, knee flexion increased by approximately 10 degrees as walking speed increased from 0.75 to 1.50 m·s⁻¹. Thus, the distribution of tibiofemoral forces would change slightly such that the anterior-posterior shear force component would increase and the compressive force component would decrease at faster speeds. Third, compressive intersegmental knee joint forces, which are primarily due to the vertical ground reaction force, only increased by 16% as walking speed increased. Thus, increases in compressive tibiofemoral forces due to intersegmental forces were relatively modest, a finding similar to that reported by Fey and Neptune.³⁷

The gastrocnemius muscle was the largest contributor to the compressive tibiofemoral force during late stance and increased force production by 50% as walking speed increased. This increase in gastrocnemius force production, especially the relatively small increase between 1.25 and 1.50 m·s⁻¹, is supported by our EMG data and is consistent with results of Neptune and Sasaki, who reported that gastrocnemius muscle force production was impaired by intrinsic muscle properties above self-selected walking speeds.²⁶ In addition, force production by the antagonist rectus femoris muscle coincident with peak late stance compressive tibiofemoral force decreased at faster walking speeds. Because the gastrocnemius only increased force output by 50% and the rectus femoris force output decreased, the net force increase from muscles spanning the knee joint across speeds during late stance was only 20%. Combined with modest increases in the intersegmental forces at the tibiofemoral joint, this accounts for the relatively small (18%) increase in the compressive tibiofemoral forces during terminal stance, despite a relatively large increase in the peak sagittal plane flexion NMM.

While these results show that peak compressive tibiofemoral forces increase modestly across walking speeds (compared with NMMs), the loading environment of the knee joint is still significantly different between slow and fast walking speeds. At the same instant during early stance weight acceptance (0% to 15% of the gait cycle), compressive tibiofemoral forces were 0.52–1.23 BW greater at 1.50 m·s⁻¹ than at 0.75 m·s⁻¹. This represents a 100% increase in compressive tibiofemoral forces at 10% of the gait cycle across walking speeds. In addition, internal abduction NMMs increased 31% with speed, which may be indicative of a greater proportion of the compressive tibiofemoral force being distributed in the medial compartment of the knee,⁷ which has been implicated with the development and progression of osteoarthritis.^38,39 At faster walking speeds, the shear components of the tibiofemoral force, especially in the A/P direction, increase in magnitude by 180% during early stance, which could potentially place greater stress on ligamentous structures of the knee joint and increase risk of injury. Due to shorter stance durations and higher tibiofemoral forces at faster walking speeds, tibiofemoral loading rates also increased. Compressive tibiofemoral loading rates increased 200%, from the slowest to fastest walking speeds. Increased rate of loading could potentially increase the risk of acute musculoskeletal injury or development of OA during walking.⁴⁰ Along with increases in tibiofemoral loading, patellofemoral loading would likely also increase with walking speed due to a more flexed knee and higher vasti muscle forces. The results from this model suggest that slower walking reduces early stance compressive loading, shear loading, rate of loading, and medial distribution of loads during walking. However, due to the decreased metabolic intensity of slower walking speeds; it would likely be a poor choice of physical activity for most populations.⁴¹

There are several limitations to this study which should be noted. First, we used a generic musculoskeletal model scaled to the anthropometries of each subject. This model contained several simplifications, including joints with limited/no degrees of freedom and generic muscle paths. Therefore our results should be considered estimates. Clearly, as more sophisticated models are developed, muscle and joint contact force estimates should improve. While the average peak residuals (18% BW) were not small, they were generally instantaneous in nature and present only during instances of the gait cycle prone to high accelerations (eg, heal strike). Thus, the effect of model simplifications/limitations and errors in the experimental data on peak muscle and joint contact force estimates are likely minimal. Second, there is no direct way to validate the individual muscle forces predicted by the musculoskeletal model used in this study and trends of force production with speed. Nonetheless, when compared with our EMG data, muscle force output showed similar trends across speeds (Figure 2). Finally, the model and methodologies used in this study were chosen based on minimizing the differences between model estimates of loading and in vivo data from a subject with an instrumented knee replacement.³³ This is currently the best means for model/loading validation, though research exploring how differences in gait may affect muscle force prediction validity is needed to apply these models to a healthy population as accurately as possible.

In summary, we observed increases in compressive tibiofemoral loading with walking speed, though not in direct proportion to increases in knee extension NMMs. The loading environment was, however, still significantly different across walking speeds. At faster speeds we observed significantly greater compressive loads during weight acceptance, a likely greater medial distribution of loads, and greatly increased compressive and A/P tibiofemoral loading rates.