Crowding by a repeating pattern

Abstract

Theinability to recognize a peripheral target among flankers is called crowding. For a foveal target, crowding can be distinguished from overlap masking by its sparing of detection, linear scaling with eccentricity, and invariance with target size.Crowding depends on the proximity and similarity of the flankers to the target. Flankers that are far from or dissimilar to the target do not crowd it. On a gray page, text whose neighboring letters have different colors, alternately black and white, has enough dissimilarity that it might escape crowding. Since reading speed is normally limited by crowding, escape from crowding should allow faster reading. Yet reading speed is unchanged (Chung & Mansfield, 2009). Why? A recent vernier study found that using alternating-color flankers produces strong crowding (Manassi, Sayim, & Herzog, 2012). Might that effect occur with letters and reading? Critical spacing is the minimum center-to-center target–flanker spacing needed to correctly identify the target. We measure it for a target letter surrounded by several equidistant flanker letters of the same polarity, opposite polarity, or mixed polarity: alternately white and black. We find strong crowding in the alternating condition, even though each flanker letter is beyond its own critical spacing (as measured in a separate condition). Thus a periodic repeating pattern can produce crowding even when the individual elements do not. Further, in all conditions we find that, once a periodic pattern repeats (two cycles), further repetition does not affect critical spacing of the innermost flanker.

Introduction

Crowding is the failure to identify a target because of neighboring clutter (e.g., Bouma, 1970; Flom, Heath, & Takahashi, 1963; Townsend, Taylor, & Brown, 1971). The perceived object seems to include features not only from the target but from the flankers as well (Levi, Hariharan, & Klein, 2002; Parkes, Lund, Angelucci, Solomon, & Morgan, 2001; Pelli, Palomares, & Majaj, 2004). Crowding depends on the distance between the target and flankers. The minimal center-to-center distance needed between a target and its flankers, in order to avoid crowding, is called the critical spacing. Critical spacing increases linearly with eccentricity (Bouma, 1970; Jacobs, 1979; Latham & Whitaker, 1996; Toet & Levi, 1992). Beyond the fovea, the linear growth is practically proportional to eccentricity. The degree of crowding depends on the ratio of target–flanker spacing to critical spacing. In everyday life, we minimize crowding by moving our eyes to recognize the target in our central vision, where critical spacing is smallest.

Reading speed is limited by crowding (Pelli & Tillman, 2008; Pelli et al., 2007). Reading consists of successive glimpses, about four per second. In each glimpse, central vision allows us to perceive several letters without crowding. This is the uncrowded window. Letters farther in the periphery are crowded because their spacing is less than the observer's critical spacing at that eccentricity. The uncrowded window determines the number of letters read in each glimpse. The number of letters we can see in one glimpse, without moving our eyes, is the visual span. Since the rate of glimpses is roughly four per second, reading speed is proportional to the visual span (Legge et al., 2007; Pelli & Tillman, 2008). Reducing crowding would increase one's visual span, allowing one to see more letters per glimpse and read faster. So how does one reduce crowding?

Contrary to intuition, increasing the letter spacing of text does not relieve crowding (Pelli et al., 2007). Crowding depends on spacing and on eccentricity (which determines critical spacing), but crowding is conserved by proportionally changing spacing and eccentricity. Crowding depends on the ratio of the letter spacing to the observer's critical spacing. The letter spacing of ordinary text is independent of eccentricity, while the observer's critical spacing is proportional to eccentricity. We can relieve crowding in a particular pair of letters by increasing their spacing without changing their eccentricity. However, for fixation on any given letter in a line of text, increasing the letter spacing of all the text will increase the eccentricity and spacing of any pair of letters on that line by the same proportion. Recall that critical spacing is proportional to eccentricity, and that crowding is determined by the ratio of spacing to critical spacing. Thus, loosening text spacing increases both the numerator and denominator by the same proportion, so the ratio is unchanged, and the crowding of each letter pair is unchanged. Thus the number of letters in the visual span is unchanged by increasing the letter spacing.

The simplest way to change spacing is to magnify or approach the page. Changing text size affects both spacing and size of letters, but crowding depends only on spacing, independent of size (Levi, Song, & Pelli, 2007; Pelli et al., 2004). Thus, the visual span's independence from spacing is consistent with the finding that reading speed changes little over a wide range of sizes (Legge, Pelli, Rubin, & Schleske, 1985).

Crowding is reduced by decreasing the similarity of the target to the flankers (e.g., Andriessen & Bouma, 1976; Bouma, 1969; Kooi, Toet, Tripathy, & Levi, 1994). As shown in Figure 1, flankers must be similar to a target in order to crowd it. Using text consisting of letters with alternating color reduces similarity and thus should reduce crowding and increase reading speed. However, reading speed for alternating-polarity text is the same as for normal black text (Chung & Mansfield, 2009). How can this be?

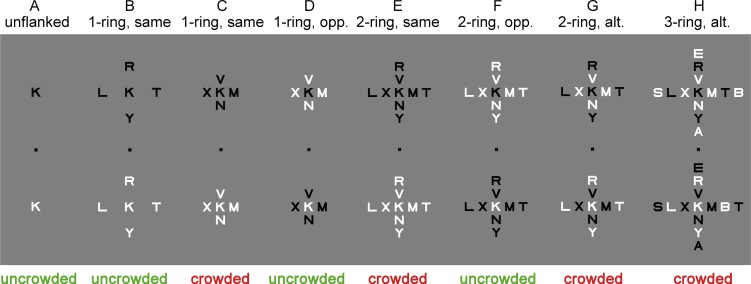
Figure 1.

Crowding demos. In each column, while fixating the black square, try to identify the center letter in the upper or lower demo. That is the target. It is black in the upper demo and white in the lower demo. Crowding scales with eccentricity, so these demos work at any viewing distance. (The 10° target eccentricity used in the Experiment reported in Figure 2 corresponds to viewing this figure at 100% magnification from about 6 cm, or at 400% from 24 cm. But the viewing distance hardly matters.) Critical spacing varies among people. Our demos work for most people, but if you are particularly susceptible or resistant to crowding, the demos might be too hard or too easy. You can compensate for this by fixating a bit above or below the black fixation square, to adjust the eccentricity of the target. (A) With no flankers, it is easy to identify the target letter. (B) One ring of same-polarity flankers, far away, does not crowd the target. (C) One ring of same-polarity flankers, nearby, crowds the target. Panels B and C show the same condition at different spacings to demonstrate the effect of proximity on crowding. (D) One ring of opposite-polarity flankers does not crowd a target. (E) Two rings of same-polarity flankers do crowd a target. (F) Two rings of opposite-polarity flankers do not crowd a target. (G) Surprisingly, a near ring of opposite-polarity flankers and a far ring of same-polarity flankers, both of which do not produce crowding alone, do crowd a target when presented together. (H) Three rings of alternating-polarity flankers crowd a target as strongly as two rings of alternating-polarity flankers. (B–H) For each condition we test many spacings, ranging from near to far, controlled by QUEST, to determine threshold, i.e., critical spacing.

This article focuses on the effect of pattern grouping on crowding. Many gestalt laws of grouping have been shown to promote crowding (e.g., Banks, Larson, & Prinzmetal, 1979; Banks & White, 1984; Livne & Sagi, 2007; Manassi, Sayim, & Herzog, 2012, 2013; Prinzmetal & Banks, 1977; Rosen, Chakravarthi, & Pelli, 2015; Saarela, Sayim, Westheimer, & Herzog, 2009; Saarela, Westheimer, & Herzog, 2010; Wolford & Chambers, 1983). See Rosen & Pelli (2015) for a review.

The deleterious effect of embedding a target in an alternating-color pattern of flankers was originally reported as grouping, with no mention of crowding. This came out of a series of studies of the effects of Gestalt grouping on foveal vernier acuity (e.g., Herzog & Fahle, 2002; Herzog, Schmonsees, & Fahle, 2003; Malania, Herzog, & Westheimer, 2007; Sayim, Westheimer, & Herzog, 2008, 2010). More precisely, in a foveal study using a vernier target flanked by lines at intervals of 1.33′ (much smaller than both the 5′ letter-acuity size and the 8′ critical spacing¹), Sayim et al. (2008) reported that alternating-color flankers impair vernier acuity due to “perceptual grouping.” Crowding was not mentioned. Crowding is relieved by expansion (at fixed target eccentricity) and exacerbated by increasing target eccentricity, while grouping is unaffected by those manipulations. Sayim et al. did not vary size or eccentricity.

The idea that an alternating-color array of flankers produces crowding was first presented at the 2011 European Conference on Visual Perception by two groups of investigators. Manassi et al. (2011, 2012) showed that the foveal paradigm of Sayim et al. also works peripherally at 3.88° eccentricity using lines at intervals of 23.33′ (much bigger than the 11′ acuity at that eccentricity, but still smaller than the now 78′ critical spacing) and concluded that their results were due to crowding. They found that when a red vernier target is flanked by an array of alternating green and red flankers, crowding is strong despite the fact that when the same red or green flankers are removed from the alternating configuration, they produce little crowding. At the same meeting, we reported the alternating-color letter results presented here, including the large critical spacing, which is diagnostic of crowding (Rosen & Pelli, 2011). The good agreement between the effects of alternating-color flankers on vernier acuity and letter recognition is encouraging evidence of generality.

Experiment

We foreshadow the presentation of our objective performance measurements (Results) by demonstrations. Using target and flankers of opposite polarity (black target and white flankers, or white target and black flankers) greatly reduces crowding (Chakravarthi & Cavanagh, 2007; Hess, Dakin, Kapoor, & Tewfik, 2000; Kooi et al., 1994). To confirm this, we place one flanker in each of four directions: to the left of, to the right of, above, and below the target. We measure crowding for target and flankers of the same polarity (one ring, same, Figure 1B, C) and for target and flankers of opposite polarity (one ring, opposite, Figure 1D). Next we add a second ring of flankers, so that there are now two flankers in each of the four directions. Here we test two polarity conditions: target and flankers of the same polarity (two rings, same, Figure 1E) and target and flankers of opposite polarity (two rings, opposite, Figure 1F).

In our main experimental condition, we present two rings of flankers that alternate in polarity with the target (two rings, alternating, Figure 1G). In a final condition, we present three rings of flankers that alternate in polarity with the target (three rings, alternating, Figure 1H).

Methods

Observers

Three experienced observers (two male, one female), including the first author, aged 24–34, with normal or corrected-to-normal vision participated in the experiment. Observers gave written informed consent in accordance with the procedures and protocols approved by the University Committee on Activities Involving Human Subjects at New York University.

Stimuli

Stimuli are generated using MATLAB with the Psychtoolbox extensions (Brainard, 1997; Pelli, 1997) running on an Apple G4 Macintosh computer and presented on an 18-in. CRT monitor with a resolution of 1024 × 768 pixels and a frame rate of 90 Hz. The display is 38 cm from the eyes of the observer, whose head is stabilized with a chin and forehead rest.

Stimuli consist of the 26 English letters (A–Z). We use an extended Sloan font with 26 letters in the same style as Sloan's original 10 letters (http://psych.nyu.edu/pelli/software.html). Each letter is rendered in the extended Sloan font and subtends 1° × 1°. The letters are either black (2 cd/m²) or white (98 cd/m²) and are presented against a gray background (50 cd/m²). All letters are uppercase. The target letter is always presented at the center of the screen. A small black square (0.78°), serving as the fixation mark, is presented 10° above the target letter, so that all stimuli are presented in the lower visual field. We test six conditions (which can be seen in Figure 1): one ring, same polarity; one ring, opposite polarity; two rings, same polarity; two rings, opposite polarity; two rings, alternating polarity; three rings, alternating polarity. For each condition, half of the trials have a black target and half of the trials have a white target. The sequence of trials is random. When flankers are present, there are an equal number of flankers (either one, two, or three) on each side of the target (top, bottom, left, and right). We refer to each set of four flankers as a ring of flankers. From the target, there are four radial axes, two vertical and two horizontal. All distances between letters are measured center to center. Along each axis, the target and flankers are evenly spaced. Thus, when two or three rings of flankers are present, the second ring is twice as far from the target as the innermost ring, and the third ring is three times as far from the target as the innermost ring. The target and flanker letters are randomly selected on each trial. Observers are asked to report the identity of the target by pressing that letter on a keyboard.

Procedure

Each condition is tested in a block of trials. Condition order is randomized. The stimulus parameters and procedures are the same for all conditions. We run four blocks of 40 trials per condition (20 of these trials have a black target and 20 of these trials have a white target). Trial order is randomized. The minimum distance needed between a target and its flankers in order to avoid crowding is called critical spacing. Critical spacing is a convenient and standard way to characterize crowding. We measure critical spacing from the center of the target to the center of a near flanker. We obtain critical spacing (target–flanker separation at which performance is at 70% accuracy) estimates for each block using the QUEST algorithm (Watson & Pelli, 1983). When more than one ring of flankers is present, all rings are spaced equally so that the innermost ring is at 1× spacing, the second ring is at 2× spacing, and the third ring (if present) is at 3× spacing.

Each trial progresses as follows: A black fixation square appears at the top of the screen throughout the whole block. Each block begins with a press of the space bar. The target and flankers are presented at the center of the screen, 10° below the fixation square. The observer reports the identity of the target (uppercase letter A–Z) with a key press. Based on whether this response is right or wrong, the QUEST algorithm sets the target–flanker separation for the next trial. The next trial is presented after an intertrial interval of 1 s. The QUEST algorithm provides an estimate of critical spacing at the end of each 40-trial block. We report the average of the four estimates obtained for each condition.

Results

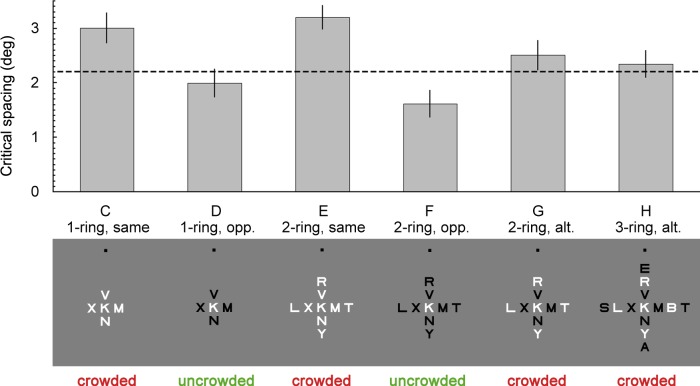
Results are plotted in Figure 2. The objective measurement of critical spacing is consistent with the crowded/uncrowded predictions of the demos. Conditions that produce a crowded demo also have large critical spacing.

Figure 2.

Results. Critical spacing (in degrees) is measured from the center of the target to the center of a flanker in the innermost ring of flankers. Each gray bar shows the mean (± standard error) critical spacing for the condition presented in the corresponding demo below it. The conditions, C through H, are carried over from Figure 1. The dashed line indicates the spacing of the demos below (equivalent to 2.2° at an eccentricity of 10°). For each condition, the demo is crowded if and only if its spacing (dashed line) is less than the measured critical spacing (gray bar). Error bars indicate plus or minus one standard error of the mean. Three observers.

A repeated-measures ANOVA shows a significant effect of condition, F(5, 10) = 61.45, p < 0.001. Paired t tests show that for a target surrounded by one ring of flankers, the critical spacing is significantly less for opposite-polarity flankers (critical spacing = 1.99° ± 0.25°) than for same-polarity flankers (critical spacing = 3.01° ± 0.27°), t(2) = 8.16, p < 0.05. Adding a second ring of flankers, like the first, has negligible effect whether they are both opposite polarity (critical spacing = 1.61° ± 0.24°) or same polarity (critical spacing = 3.20° ± 0.21°), t(2) = 15.38, p < 0.01. Regardless of whether one or two rings are present, using flankers of opposite polarity to the target yields lower critical spacing than using same-polarity flankers.

Using paired t tests to compare the one- and the two-ring conditions, we find that adding an extra ring of same-polarity flankers does not affect critical spacing, t(2) = 1.74, p = 0.22. Adding an extra ring of opposite polarity is barely significant, t(2) = 4.20, p = 0.05, but, doing a within-observer t test for each observer, we find that the difference between these two conditions is significant in only one of the three observers (p = 0.36, p < 0.01, p = 0.35). Thus, for most observers, there is no effect of adding a further ring like the first.

Paired t tests show that the critical spacing of the two-ring, alternating-polarity condition (2.51° ± 0.27°) is less than that of the two-ring, same-polarity condition, t(2) = 7.11, p < 0.05, and greater than that of the two-ring, opposite-polarity condition, t(2) = 24.24, p < 0.01. However, the two-ring, alternating-polarity condition is not significantly different from the one-ring, same-polarity condition, t(2) = 2.59, p = 0.12, or the three-ring, alternating-polarity condition (2.34° ± 0.24°), t(2) = 2.71, p = 0.11.

Discussion

A line of text consists of closely spaced letters. Reading speed is limited by peripheral crowding of those letters. Reducing that crowding would increase reading speed. Crowding experiments, with one target surrounded by flankers, show that crowding is relieved if the flankers have a different color than the target. So it has been surprising that visual span profiles and reading speed are not improved by using alternating-polarity text (Chung & Mansfield, 2009). Unlike traditional crowding experiments, here we use several rings of flankers to better replicate the extended patterns found in lines of text. We find that the relief of crowding due to alternating color that occurs with one ring of flankers disappears with two. Measuring critical spacing for each condition assays the crowding effect of each kind of flanker, and connects our results to the crowding literature.

Our results using one or two rings of same- or opposite-polarity flankers are in line with previous findings: Regardless of whether one or two rings of flankers are present, using flankers of opposite polarity (e.g., white target with black flankers) reduces critical spacing compared to using flankers of the same polarity (e.g., Bouma, 1969; Kooi et al., 1994). In the one-ring conditions, the critical spacing is 3° for same polarity and 2° for opposite polarity. This is the well-known dependence of crowding on similarity. In this case, flankers that are similar to a target have a critical spacing that is 1.5× that of flankers that are dissimilar to the target. When two rings of flanker are present, the critical spacing is 1.6° for opposite-polarity flankers and 3.2° for same-polarity flankers. Here, making the flankers similar to the target doubles the critical spacing.

Synergy

In the two-ring, alternating-polarity condition, the critical spacing of the inner (opposite-polarity) flankers is 2.5°. When presented alone, the same inner ring has a critical spacing of 2°. Thus adding the outer ring increases the critical spacing from 2° to 2.5°, t(2) = 7.46, p < 0.05. In fact, when the two-ring, alternating condition is critically spaced, the spacing of the inner ring (which is opposite polarity) is no different than the critical spacing for the same-polarity single ring, t(2) = 2.59, p = 0.12, and the spacing of the outer ring (same-polarity flankers) is significantly larger than the same flankers in the one-ring condition, t(2) = 5.27, p < 0.05. The demos show that, with a 2° spacing, the alternating condition crowds (Figure 1G), but neither ring alone does (Figure 1B, D). The measurements bear this out, finding that at the critical spacing of the alternating condition, the inner and outer rings are each at a spacing significantly larger than their spacing measured alone (recall that the outer ring is at 2× the value plotted). This result for identifying a letter among letters parallels the finding for vernier acuity flanked by alternating-color lines (Manassi et al., 2012; Sayim et al., 2008). We quantify crowding in the standard way, as critical spacing.

Additional pattern repetitions do not affect critical spacing

For both same- and opposite-polarity conditions, we also find that adding an extra ring of flankers with the same polarity as the first ring does not alter critical spacing (in two of three observers in one condition and in all three observers in the other). This is in line with past reports that adding displaced copies of similar flankers at regular intervals does not affect crowding (Manassi et al., 2012; Pelli et al., 2004; Wilkinson, Wilson, & Ellemberg, 1997). (The degree of similarity matters: Extra flankers that are dissimilar in length to the target do affect crowding, reducing it; Manassi et al., 2012.)

For alternating-polarity flankers, at least two flankers are required on each side of the target in order to create a pattern. When only one flanker is present on each side, this is the one-ring, opposite-polarity condition and there is little crowding. Beyond two alternating rings, adding more flankers does not alter critical spacing: The two- and three-ring, alternating-polarity conditions both have the same critical spacing. We tested a target surrounded by either same- or alternating-polarity flankers. In both cases, we find that once the pattern repeats once (two cycles), further repetition, by adding more flankers, does not affect critical spacing of the innermost flanker.

In the case of a one-element period (a target surrounded by same-polarity flankers) the target plus one flanker on each side of the target is already three cycles. For a two-element period (a target surrounded by alternating-polarity flankers) the target plus two flankers on each side of the target is two and a half cycles. In both cases, adding further flankers, so as to continue the repeating pattern, does not affect critical spacing.

Centroid spacing is not conserved

The critical spacing of crowding is usually measured to the target center. However, sometimes crowding is determined by the distance from target to the centroid (geometric center) of a group of flankers (Levi & Carney, 2009). That is, when multiple flankers crowd a target, if the flankers group, the group may act as a flanker. A centroid theory of crowding predicts that the distance between the target and the centroid of the flankers remains constant across objects. Do our results conserve centroid spacing? No. Suppose that adjacent same-color flankers group together along each radial axis. The one-ring, same-polarity condition has a critical spacing of 3.0°, which is much smaller than the 4.8° critical spacing of the centroid of the two-ring, same-polarity condition, t(2) = 14.41, p < 0.005. Thus, critical spacing of centroids is not conserved when comparing one versus two rings of same-polarity flankers. Further, the two- and three-ring, alternating-polarity conditions have the same critical spacing but different centroid spacings. The finding that centroid spacing is not conserved when adding additional flankers is in line with previous findings (Manassi et al., 2012; Saarela et al., 2010).

Reading speed is unchanged

As we note at the beginning, since crowding is known to limit reading, and crowding depends on similarity and proximity, it was widely expected that alternating letter polarity would reduce crowding and speed up reading. However, reading speed is unchanged when using alternating letter polarity (Chung & Mansfield, 2009). Our results show that two cycles of an alternating-color pattern crowd as much as same-color neighbors. There is no relief of crowding, so reading speed is unchanged.

Reading speed is improved

Avoiding creation of a periodic pattern, Rosen and Pelli (2012) found a way to selectively relieve crowding and speed up reading. Two letters are contrast-reversed in a gaze-contingent display of text. The observer's normal visual span (uncrowded window) is about nine characters, which this method extends by an additional character on each side, to about 11 characters. The text display reverses the contrast of the two flanking letters on either side of the extended span, which is centered on the observer's current fixation. This relieves crowding at the ends of the extended span, increasing the visual span and reading speed by 20%.

Saarela et al. (2010) found that presenting targets and flankers in a regular spacing increases crowding compared to presenting target and flankers in irregular spacings. We do not know whether imposing irregular spacing on text might speed reading. Of course, only monospaced fonts, like Courier, have regular spacing. Most of what we read has proportionally spaced fonts, whose center-to-center letter spacing is erratic. Any speed benefit of reading proportionally spaced text might be partly due to that.

Cortex

Crowding is a computational limit on feature combination for object recognition. As objects get farther into the periphery, they need to be spaced farther apart in order to be recognized. The world is mapped retinotopically onto the visual cortex (V1), but the cortical magnification factor drops with increasing eccentricity, so that fewer and fewer neurons are devoted to each square degree of visual field. The scaling of crowding with eccentricity matches the cortical magnification factor so that critical spacing is a fixed distance, about 6 mm, on the surface of primary visual cortex (Pelli, 2008). Objects must be sufficiently separated on the visual cortex in order to be recognized. A pattern can increase the critical spacing at the visual field and thus at the cortex. Thus, a pattern can produce a longer-range interaction in the cortex. This joins several other cases of long-distance crowding (Harrison, Retell, Remington, & Mattingley, 2013; Manassi et al., 2012, 2013; Pelli & Cavanagh, 2013; Vickery, Shim, Chakravarthi, Jiang, & Luedeman, 2009).

Critical spacing marks the boundary just beyond the greatest distance at which the flanker affects recognition of the target. In our results, the greatest distance at which the similar flankers are effective is 3° when presented alone, or 2 × 2.5° = 5° when presented as a second ring around a dissimilar first ring. Increasing critical spacing from 3° to 5° at the visual field will proportionally increase critical spacing at V1 from 6 mm to 10 mm.

Conclusion

Conventional wisdom says that a target is only crowded by nearby similar flankers. We find that embedding the target in a simple alternating pattern overrides local dissimilarity, producing crowding without nearby similar flankers. Our finding for letter identification parallels a similar finding for vernier acuity (Manassi et al., 2012). The periodic repetition of the pattern overrides the local dissimilarity, producing a longer-range neural interaction. This explains why alternating the polarity of text does not speed up reading.