Gain and phase of perceived virtual rotation evoked by electrical vestibular stimuli

Abstract

Galvanic vestibular stimulation (GVS) evokes a perception of rotation; however, very few quantitative data exist on the matter. We performed psychophysical experiments on virtual rotations experienced when binaural bipolar electrical stimulation is applied over the mastoids. We also performed analogous real whole body yaw rotation experiments, allowing us to compare the frequency response of vestibular perception with (real) and without (virtual) natural mechanical stimulation of the semicircular canals. To estimate the gain of vestibular perception, we measured direction discrimination thresholds for virtual and real rotations. Real direction discrimination thresholds decreased at higher frequencies, confirming multiple previous studies. Conversely, virtual direction discrimination thresholds increased at higher frequencies, implying low-pass filtering of the virtual perception process occurring potentially anywhere between afferent transduction and cortical responses. To estimate the phase of vestibular perception, participants manually tracked their perceived position during sinusoidal virtual and real kinetic stimulation. For real rotations, perceived velocity was approximately in phase with actual velocity across all frequencies. Perceived virtual velocity was in phase with the GVS waveform at low frequencies (0.05 and 0.1 Hz). As frequency was increased to 1 Hz, the phase of perceived velocity advanced relative to the GVS waveform. Therefore, at low frequencies GVS is interpreted as an angular velocity signal and at higher frequencies GVS becomes interpreted increasingly as an angular position signal. These estimated gain and phase spectra for vestibular perception are a first step toward generating well-controlled virtual vestibular percepts, an endeavor that may reveal the usefulness of GVS in the areas of clinical assessment, neuroprosthetics, and virtual reality.

with the body restrained, galvanic vestibular stimulation (GVS) evokes an illusory motion percept that we and others term “virtual rotation” (St. George et al. 2011; Wardman et al. 2003). Human GVS involves delivering percutaneous electrical current binaurally or monaurally through the mastoid processes (for review see Fitzpatrick and Day 2004). Based on the nonhuman primate literature, it is commonly assumed that firing rates of irregular vestibular afferents, and to a lesser extent regular vestibular afferents, increase with cathodal current and decrease with anodal current (Goldberg et al. 1982, 1984). It has further been argued that the site of transduction for galvanic current is the spike triggering zone on the individual afferent terminals (Goldberg et al. 1984), bypassing natural mechanotransduction in the vestibular labyrinth. Once this input enters the central nervous system (CNS), it can give rise to a perception of head movement that is judged to be similar qualitatively to a real perturbation of the head. Because of this similarity between virtual and real kinetic perception, we wanted to investigate the potential usefulness of GVS as a more practical and simple method of measuring vestibular function and evoking controlled vestibular percepts in general. As a first step toward this goal, it is essential to know the gain and phase of virtual vestibular perception as a function of frequency, knowledge that is presently lacking in the literature.

The current model of GVS transduction suggests that all vestibular afferents, both otolith and canal based, are uniformly stimulated by the applied current (Fitzpatrick and Day 2004), with each afferent signaling its preferred linear or angular head motion vector. Thus, by computing a vector sum across all otolith and canal afferents bilaterally, Fitzpatrick and Day (2004) arrived at an estimate of a net GVS-evoked rotation vector passing ∼19° below Reid's plane through the center of the skull (see Fig. 1)—a prediction that has been confirmed experimentally (Day and Fitzpatrick 2005). Signals from otolith afferents cancel bilaterally in the commonly used binaural bipolar electrode configuration (Mian et al. 2010); therefore, the illusory motion evoked by GVS is felt as largely that of an angular head rotation about the net GVS-evoked vector. Researchers have taken advantage of the GVS-evoked rotation vector by pitching the head down ∼71° such that this vector aligns with Earth-vertical, eliciting the perception of whole body yaw rotation (Day and Fitzpatrick 2005; Fitzpatrick et al. 2002; St. George et al. 2011). In the present study, we utilize this head pitch manipulation to probe the perception of virtual whole body yaw rotation for the first time with standard psychophysical methods.

Fig. 1.

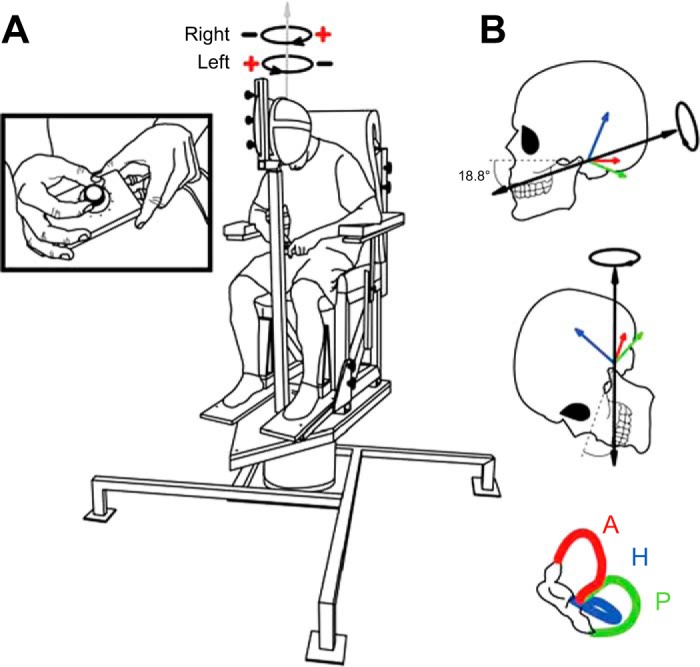
Illustration of rotary chair and head pitch. A: participants were seated comfortably atop a memory foam-padded rotary chair. Additional foam was added for real rotation experiments (not depicted). The chair was custom-designed to provide Earth-vertical yaw rotations via a large servo-controlled electric motor. Depicted schematically above the participant is the perceived virtual direction for the 2 different electrode polarities; virtual head rotation was always felt toward the cathode side for suprathreshold stimuli. B: head pitch angle was specifically chosen to align the galvanic vestibular stimulation (GVS)-evoked net rotational vector (see Fitzpatrick and Day 2004) with the rotary chair's axis of rotation, producing the illusion that the chair was rotating when binaural bipolar GVS was applied over the mastoids (cathode right/anode left = rightward virtual rotation, cathode left/anode right = leftward virtual rotation). Anterior (A), horizontal (H), and posterior (P) semicircular canal rotational vectors are drawn for the left side; the net GVS-evoked rotation vector summed across all 6 canals bilaterally is shown as a solid black arrow.

One open question is whether sensitivity to virtual rotations would be altered by stimulus frequency as previously reported for real rotations (Benson et al. 1989; Grabherr et al. 2008; Soyka et al. 2012). Estimating the gain spectrum of virtual and real kinetic perception was accomplished by quantifying direction discrimination thresholds as a function of frequency (0.05–2 Hz). For real rotations, it is well established that human discriminability of direction improves with increasing frequency, as would be predicted from the high-frequency gain of the vestibular system (e.g., whole body yaw rotations; Benson et al. 1989; Grabherr et al. 2008; Soyka et al. 2012). In each of these previous yaw rotation studies, the head was upright, facing forward, activating mainly the horizontal canals; therefore, since we had the head pitched down in the present study, it was important to confirm that thresholds still decrease at higher frequencies because mainly the anterior and posterior canals are activated in this posture. Previous neurophysiological studies (Goldberg et al. 1982) have demonstrated that the gain of real kinetic perception is predicted by the afferent transfer function. Therefore, given that animal studies suggest that GVS-to-afferent response gain is not influenced by, or increases a very small amount over, the range of frequencies we tested (Goldberg et al. 1982), we hypothesized that the gain of virtual rotation perception would also not be influenced by stimulus frequency.

Estimating the phase spectrum of virtual and real kinetic perception was accomplished with a novel manual tracking task that measured perceived angular velocity throughout continuous sinusoidal stimulation. Very little is known about the phase of perception relative to real kinetic stimulation; however, given that neural responses in the vestibular nucleus (Dickman and Angelaki 2004), thalamus (Büttner et al. 1977), and cortex (Grüsser et al. 1990) all lead angular velocity by 0–30°, we predicted that perceived velocity will lead actual velocity by a similar magnitude. Owing to a lack of neurophysiological data on central processing of GVS, it is unclear whether the perception of GVS would be in phase with perceived position, velocity, or acceleration, although previous work would suggest the latter (St. George et al. 2011).

MATERIALS AND METHODS

Participants

Fourteen healthy subjects (10 men, 4 women) between the ages of 21 and 40 yr (mean = 27.8 yr, SD = 5.9) with no known history of neurological disease or injury participated in this study. The experimental protocol was explained to each subject, and their written, informed consent was obtained. All procedures conformed to the standards of the Declaration of Helsinki and were approved by the University of British Columbia's clinical research ethics board.

Galvanic Vestibular Stimuli

Electrical vestibular stimulation was delivered through a binaural bipolar electrode configuration. Carbon rubber electrodes (9 cm²) coated with Spectra 360 electrode gel (Parker Laboratories) were secured over participants' mastoid processes with surgical tape and an elastic headband. Vestibular stimuli were generated on a PC computer with custom LabVIEW software (National Instruments) and were sent directly to a constant-current isolation unit (STMISOLA, Biopac Systems) via a multifunction data acquisition board (PXI-6289, National Instruments). In the first experiment [see Direction discrimination thresholds (session 1)], vestibular stimuli consisted of raised-cosine bell curves, with the peak current amplitude adaptively adjusted (from 0.1 to 5 mA) across trials. In the second experiment, continuous sinusoidal stimuli were used [see Phase lag of perception (session 2)]. To minimize any nonvestibular cues associated with skin tingling under the electrodes, especially because the higher frequencies we tested required relatively high levels of current to detect (up to 3–5 mA for some participants), we anesthetized the skin over the mastoid processes bilaterally with AMETOP [tetracaine HCl gel 4% (wt/wt), Smith & Nephew] applied 30–45 min prior to each experiment. Testing was carried out in a dark, electrically shielded room, with the eyes closed and earplugs in. Ten participants performed the virtual rotation experiments, three of whom also participated in the real kinetic rotation experiments described below.

Real Kinetic Rotations

To deliver whole body yaw rotations, we used a custom-built rotary chair (see Fig. 1A), which we drove with a real-time motion controller (PXI-7350 Motion Controller, National Instruments; Universal Motion Interface UMI-7774, National Instruments) running in-house LabVIEW software built with the NI Motion programming suite (National Instruments). The motion controller sent torque commands to a servo amplifier (SGDV-200A01A, Yaskawa), which powered a large AC motor (SGMCS-1EN3A31, Yaskawa; encoder angular resolution 0.00034°, continuous torque 150 Nm). In the first experiment [see Direction discrimination thresholds (session 1)], vestibular stimuli consisted of raised-cosine bell curves, with the peak velocity adaptively adjusted (from 0.1 to 7°/s) across trials. In the second experiment, continuous sinusoidal stimuli were used [see Phase lag of perception (session 2)]. To mitigate nonvestibular (somatosensory) cues, additional dual-layer memory foam padding (not depicted in Fig. 1A) was added beneath the participant's socked feet and around the chest, forearms, and shanks and was firmly secured to the chair with adjustable strapping. The participant was further strapped to the chair with a five-point racing harness. Testing was carried out in a dark, electrically shielded room, with the eyes closed and earbuds in. Ocean sounds were played through the earbuds to mask the low-amplitude, high-frequency sound emitted by the motor during testing. Seven participants performed real kinetic rotation experiments.

Experimental Procedures

Direction discrimination thresholds (session 1).

In this experiment, participants attempted to discern whether the direction of virtual or real whole body rotation was to the right or left. Participants were seated comfortably atop the rotary chair with their head facing down toward their lap and immobilized. The experimenter ensured that the head was pitched down 71°, aligning the angle of the GVS-evoked rotational vector with a binaural bipolar electrode configuration (Fitzpatrick and Day 2004) with an Earth-vertical axis through the center of the chair's axis of rotation (see Fig. 1B). Previous research has shown that pitching the head downward evokes an illusion of whole body rotation in the yaw plane, providing the sensation of spinning on a turntable (Day and Fitzpatrick 2005; St. George et al. 2011). In all experiments, the participant's head was held fixed in place with a helmet (Pro-Tec) that was solidly braced to the rotary chair carriage. Correct head position was confirmed by using a protractor to measure head pitch numerous times throughout testing.

We asked participants to complete a series of trials in which they were given a single cycle of a raised-cosine bell GVS or real velocity pulse (with electrode polarity and rotation direction randomized across trials) and were required (forced choice) to indicate which direction they were rotated with a verbal response (“right” or “left”). With GVS, participants reported vivid sensations of actually being rotated in the chair, although the chair remained stationary. The direction of virtual rotation was always toward the cathode side for suprathreshold stimuli (see Fig. 1A). Each participant completed 6 blocks of 40 trials, each block with a different frequency of GVS/real velocity (0.05, 0.1, 0.2, 0.5, 1, and 2 Hz; block order randomized). The frequency range of 0.05–2 Hz encompasses the normal operating range of the vestibular system (Goldberg et al. 2012; Mayne 1974), over which gain remains relatively constant and the phase shift is negligible.

We adaptively varied the peak GVS current and velocity across trials, using a Bayesian adaptive procedure (Kontesevich and Tyler 1999). This psychophysical testing method efficiently estimates each participant's function, relating stimulus amplitude (in mA or °/s) to his/her proportion of correct direction discriminations, and from this function a threshold level of performance can be extracted (see Fig. 2). We parameterized each participant's sigmoidal psychometric function as a modified Weibull function (Tong et al. 2013),

$$P\left(correct|x\right)=\gamma +\left(1-\gamma -\delta \right)\left(1-2^{-{\left(x/a\right)}^b}\right)$$

Fig. 2.

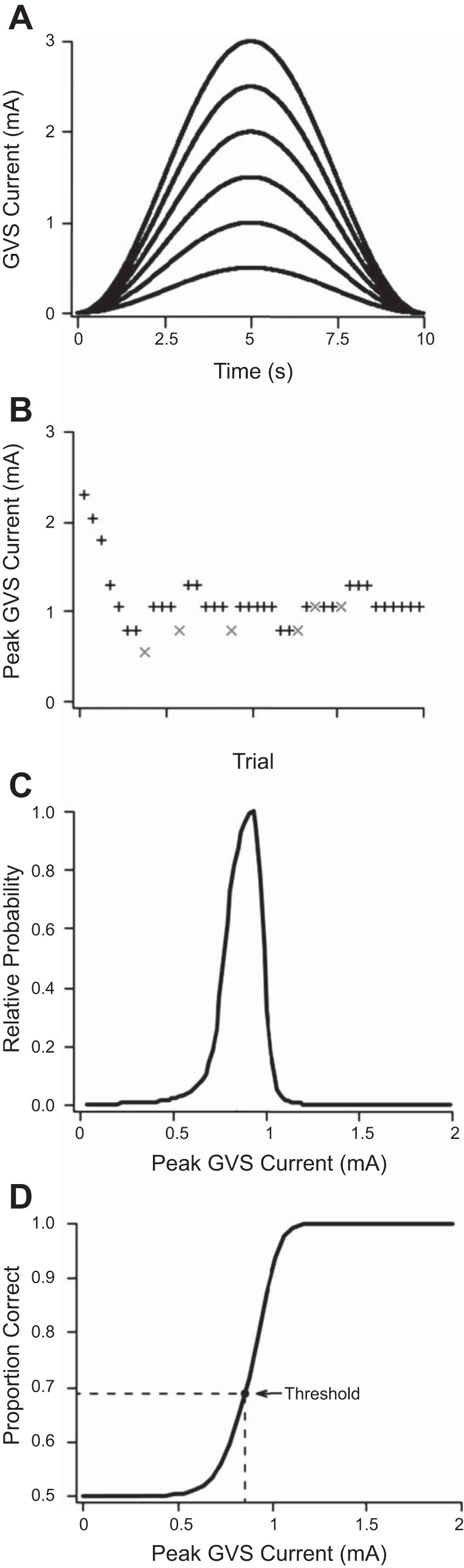
The Bayesian adaptive procedure for estimating real and virtual motion direction discrimination thresholds. A: examples of different peak-amplitude GVS waveforms (raised-cosine bell curves) used to evoke virtual rotations (stimulus frequency = 0.1 Hz). B: performance plot showing how peak amplitude was adaptively adjusted across 40-trial blocks with the Bayesian adaptive procedure (+ = correct response; × = incorrect response). C: the end-of-block a parameter probability distribution function (normalized to the maximum value for plotting purposes) corresponding to the performance plot shown in B. D: best-estimated psychometric function relating the peak GVS current to the proportion of correct responses for the same block of trials as B and C.

Here the γ parameter sets the y-intercept of the curve, the a parameter determines the lateral position of the curve along the x-axis, the b parameter determines the shape (slope) of the curve, and the δ parameter represents the lapse rate. The lapse rate term accounts for the realistic possibility of occasional attention lapses, resulting in 50% correct response probability, regardless of the peak stimulus amplitude. The algorithm, which we programmed in LabVIEW (National Instruments), adaptively adjusted the peak stimulus level from trial to trial, presenting the stimulus expected to yield the greatest information regarding the participant's psychometric function parameters (expected entropy minimization; Kontesevich and Tyler 1999). The γ parameter was held fixed at 0.5 for this experiment because this represents chance performance; thus the Bayesian adaptive procedure made hypotheses only on the possible a, b, and δ parameters of each participant's psychometric function and returned the joint posterior probability distribution function (PDF) over these three parameters, along with the best-estimated psychometric function.

The direction discrimination threshold was defined as the peak stimulus level at which the participant could correctly discriminate direction with 69% probability, which corresponds to d′ = 1 on this single-interval task (Gescheider 1997). In this case, the discriminabilty index, d′, refers to the theoretical separation between response distributions for rightward and leftward rotations normalized by the SD (which is assumed to be equal for both directions). To extract the 69% correct threshold, we marginalized each participant's joint posterior PDF over the δ parameter, plotted the best-fit psychometric function for each (a,b) pair, and interpolated to find the stimulus amplitude corresponding to 69% correct performance. We then averaged the 69% correct stimulus amplitude across the (a,b) posterior PDF and took this as the participant's threshold estimate.

Phase lag of perception (session 2).

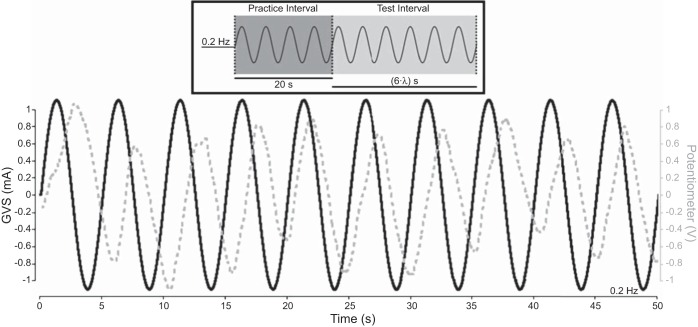
To determine the phase relationship between perceived movements and the actual input stimulus, we used a single-interval manual tracking task. The participant's task was simply to move a potentiometer along with his/her perceived position during stimulation. It is common to report phases relative to velocity (Büttner et al. 1977; Dickman and Angelaki 2004; Goldberg et al. 1982; Grüsser et al. 1990); therefore, we differentiated the potentiometer traces to obtain the participant's perceived velocity (i.e., a phase difference of 0 means GVS or real kinetic velocity is in phase with perceived velocity). As depicted in Fig. 3, each trial consisted of a 20-s practice interval of continuous sinusoidal vestibular stimulation (virtual or real) in which the participant was free to begin moving the potentiometer whenever he or she was ready; this was followed by an additional six cycles (“test interval”). Participants completed 3 trials at 6 frequencies (0.05, 0.1, 0.2, 0.5, 1, and 2 Hz; order randomized), yielding 18 cycles total (6 final cycles from 3 trials) that were used in the analysis for each frequency. For the virtual rotation experiments, one participant was only able to give four to six cycles of movement at the two lowest frequencies tested because he struggled with tracking the virtual movements; thus the final four to six cycles of potentiometer movement were used in the analysis for this participant.

Fig. 3.

Sample manual tracking trial timeline. Within 1 or 2 stimulus cycles, participants began moving the potentiometer at a consistent phase relative to the stimulus. For the virtual and real manual tracking experiments, each trial consisted of a 20-s practice interval in which participants began moving the potentiometer, followed immediately by 6 cycles of the test stimulus (test interval). In this example, the stimulus frequency was 0.2 Hz (λ = 5 s); therefore, the test interval duration was 30 s (see inset). Data from 3 test intervals at each frequency were concatenated together in the analysis.

The sinusoidal stimuli had an amplitude equal to twice the participant's direction discrimination threshold (as determined by the first experiment) at each frequency. In a few cases, the participant still reported that he or she had difficulty clearly tracking the real/virtual chair oscillations even at twice his/her direction discrimination threshold; in these cases we incremented the peak velocity by 0.2°/s or peak current by 0.2 mA until the participant was having a clear perception of rotation. Only one participant in the real kinetic experiments needed an increment, and this was only for a single frequency (0.2 Hz); the increment the participant needed was quite large (2.6°/s). This participant's threshold at 0.2 Hz was unexpectedly low given the group mean; thus it was not surprising that a large increment from twice the participant's threshold was needed to accurately track position. Seven participants requested an amplitude increment for the virtual rotation experiments; the mean increment across all participants was 0.1 mA (SD = 0.3 mA).

Coherence, a measure of correlated frequency components in the input (GVS waveform or rotary chair velocity) and output (derivative of potentiometer waveform) signals (Amjad et al. 1997; Halliday et al. 1995; Rosenberg et al. 1989), was calculated at the test frequency (λ) as

$${\left|R_{\text{AB}}\left(\lambda \right)\right|}^2=\frac{{\left|f_{\text{AB}}\left(\lambda \right)\right|}^2}{f_{\text{AA}}\left(\lambda \right)f_{\text{BB}}\left(\lambda \right)}$$

Coherence is a unitless measure bounded between 0 and 1, with 1 indicating a perfect linear relationship and 0 indicating independence. Phase was calculated at the stimulus frequency as the angle of the complex coherency function. The GVS and the potentiometer traces were sampled at 2,048 Hz, and real angular position and velocity were sampled at 100 Hz from the rotary chair encoder. The encoder data were then upsampled to 2,048 Hz for the analysis.

Coherence was analyzed on a participant-by-participant basis to ensure that the phase estimates were reliable. If coherence was not significant for a given participant, the phase data were not analyzed (n = 3 for GVS and n = 1 for real rotation). We chose not to present the 2-Hz trials here, as these were too difficult for participants to track. This limitation was expected given previous real rotation research (Mergner et al. 1981; see discussion). Difficulty tracking 2-Hz oscillations is evident in our finding that all of the participants in the virtual rotation experiment failed to show significant coherence (critical 95% CI value = 0.16) between the GVS and the derivative of the potentiometer waveforms at 2 Hz. For the other frequencies the coherence was strong (∼0.6–0.8) at the test frequency. The mean coherence values across participants for virtual rotations were 0.68 (SD = 0.29) at 0.05 Hz, 0.62 (SD = 0.26) at 0.1 Hz, 0.8 (SD = 0.07) at 0.2 Hz, 0.77 (SD = 0.29) at 0.5 Hz, 0.65 (SD = 0.33) at 1 Hz, and only 0.02 (SD = 0.02) at 2 Hz. Because the real rotation data were collected after the virtual rotation experiments, we only collected phase data for real rotations using 0.05–1 Hz. The mean coherence values across participants for real rotations were 0.79 (SD = 0.21) at 0.05 Hz, 0.79 (SD = 0.23) at 0.1 Hz, 0.89 (SD = 0.07) at 0.2 Hz, 0.8 (SD = 0.21) at 0.5 Hz, and 0.74 (SD = 0.27) at 1 Hz.

Statistical Analysis

The dependent variables we extracted were 1) the mean 69%-correct direction discrimination threshold for virtual and real rotations at each frequency tested and 2) the mean phase relationship between the sinusoidal GVS current/chair velocity and the derivative of the potentiometer movement made by the participant at each frequency tested. To analyze the threshold data, we performed two one-way repeated-measures ANOVAs (1 for each type of stimulus) with 69%-correct discrimination threshold as the dependent variable and frequency as the independent variable. To analyze the phase data, we performed two one-way repeated-measures ANOVAs with phase as the dependent variable and frequency as the independent variable. Each ANOVA showing a significant effect of frequency was supplemented with linear trend analysis to evaluate increases or decreases across stimulus frequency. Contrast weights were adjusted to account for unequal spacing of the log-transformed frequency intervals. Statistical analyses were performed with STATISTICA 6.0 (StatSoft), and in all cases we used an α level of 0.05 for assessing statistical significance.

RESULTS

Direction Discrimination Thresholds

We used the Bayesian adaptive procedure to map each participant's sensitivity to virtual motion direction. All participants reported the sensation of the chair rotating toward the cathode side when GVS was applied. As the frequency of the applied raised-cosine bell curve GVS current increased, thresholds doubled from 0.7 mA at 0.05 Hz to 1.4 mA at 2 Hz. This can be seen in the rightward shift of the psychometric function estimates as frequency increases (down Fig. 4, left), as well as in the mean threshold values plotted over frequency in Fig. 5A. To test whether stimulus frequency significantly affected performance, we conducted a one-way repeated-measures ANOVA with 69%-correct threshold as the dependent variable and GVS frequency as the independent variable. This returned a significant effect of frequency (F_5,45 = 6.502, P = 0.00013), indicating that direction discrimination thresholds were significantly affected by stimulus frequency. Linear contrast analysis on the log-transformed frequency intervals revealed a significant trend (P = 0.0123), indicating that thresholds to virtual rotation increased linearly as a function of the log-transformed stimulus frequency.

Fig. 4.

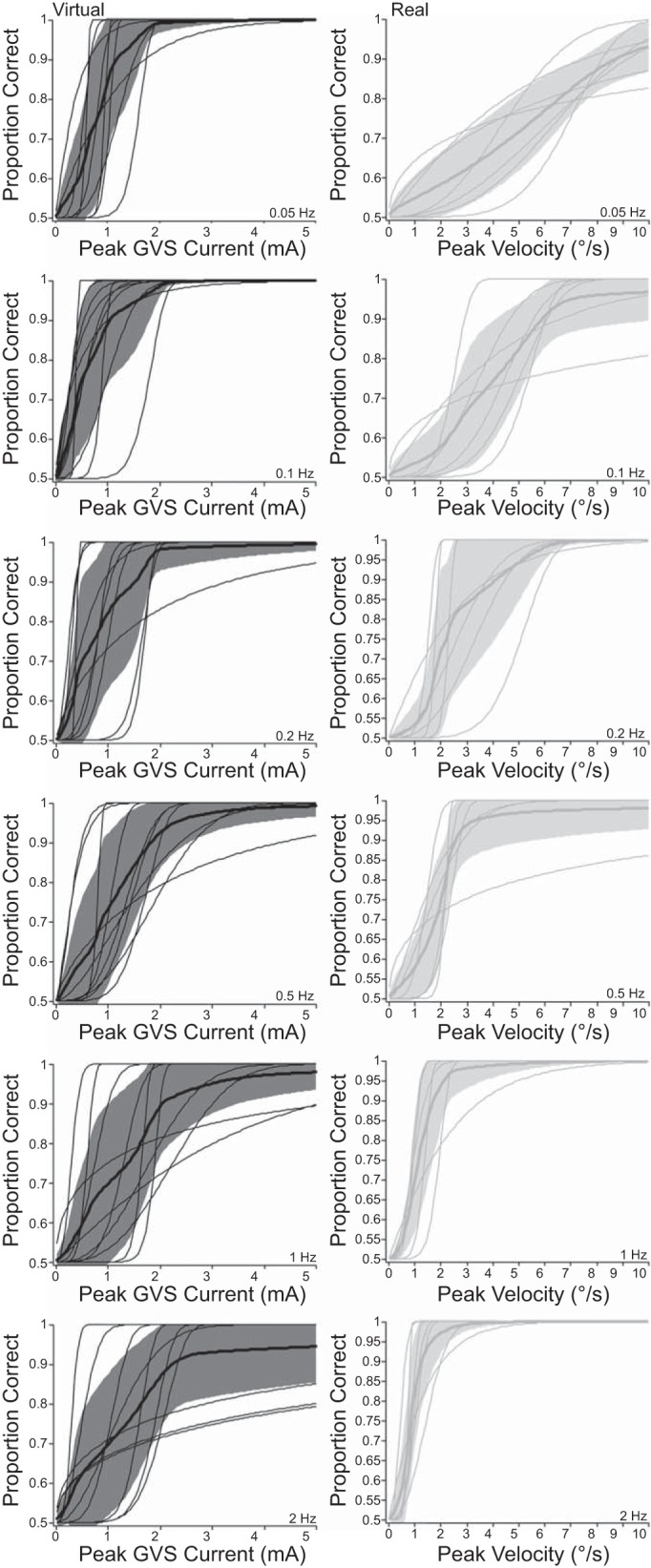
Individual and mean psychometric functions for virtual and real direction discrimination task. Individual participant (thin) and mean (thick) best-estimated psychometric functions (shaded regions: ±1 SD). Left: virtual rotations (black curves and shaded regions). Right: real rotations (gray curves and shaded regions). Different stimulation frequencies are plotted in each row.

Fig. 5.

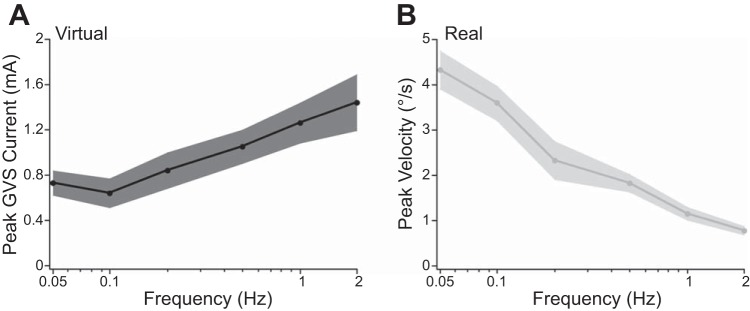
Direction discrimination thresholds as a function of frequency. A: virtual rotation thresholds. Solid black curve plots the mean threshold value as a function of frequency (black shaded region = ±1 SE). B: real rotation thresholds. Solid gray curve plots the mean threshold value as a function of frequency (gray shaded region = ±1 SE).

We additionally measured real direction discrimination thresholds, using the Bayesian adaptive procedure. As expected based on previous whole body yaw rotation studies performed with the head upright and facing forward, the discriminability of real rotation direction improves with increasing frequency. As the frequency of the raised-cosine bell curve velocity profiles increased, thresholds reduced from 4.3°/s at 0.05 Hz to 0.8°/s at 2 Hz. This can be seen in the leftward shift of the psychometric function estimates as frequency increases (down Fig. 4, right), as well as in the mean threshold values plotted over frequency in Fig. 5B. To test whether stimulus frequency significantly affected performance, we conducted a repeated-measures one-way ANOVA with 69%-correct threshold as the dependent variable and real rotation frequency as the independent variable. This returned a significant effect of frequency (F_5,30 = 26.153, P < 0.0001). A linear contrast analysis on the log-transformed frequency intervals revealed a significant trend (P = 0.0001), indicating that thresholds to real rotation decreased linearly as a function of the log-transformed stimulus frequency.

Manual Tracking of Continuous Virtual and Real Sinusoidal Oscillations

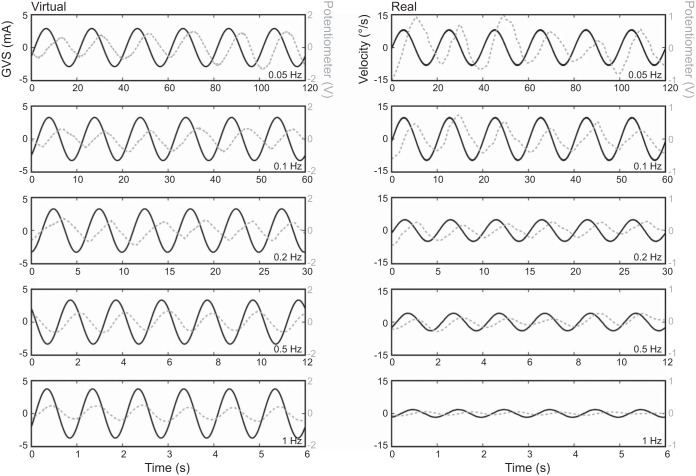
We used a single-interval manual tracking paradigm to measure the phase relationship between participants' perceived virtual velocity (derivative of potentiometer trace) and the GVS waveform. Representative data from one participant are shown in Fig. 6, left. The time delays between GVS and perceived position ranged from 5.3 s at 0.05 Hz to 0.17 s at 1 Hz. As depicted in Fig. 7A, perceived virtual velocity was in phase with the GVS waveform at low frequencies (0.05 and 0.1 Hz) and the phase of perceived velocity advanced relative to the GVS waveform as the frequency increased. The corresponding time delays are depicted in Fig. 7B. To compare phase across frequencies, we conducted a one-way repeated-measures ANOVA with mean phase as the dependent variable and GVS frequency as the independent variable. Three participants were removed from the repeated-measures ANOVA because coherence failed to reach the 95% confidence level for at least one frequency. This returned a significant effect of frequency (F_4,24 = 12.359, P < 0.0001). A linear contrast analysis on the log-transformed frequency intervals revealed a significant positive trend (P = 0.00536), indicating that the phase increased linearly over the log-transformed stimulus frequency.

Fig. 6.

Representative manual tracking data for virtual and real sinusoidal rotations. GVS/encoder velocity (solid black) and potentiometer voltage (dashed gray) are plotted together on the same timescale. Note that the potentiometer traces illustrated are raw signals; however, these signals were differentiated for all analyses. Left: virtual rotations. Right: real rotations. Different stimulation frequencies are plotted in each row.

Fig. 7.

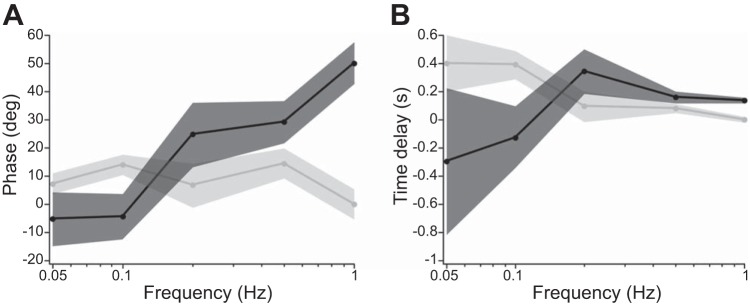
Phase of virtual and real kinetic perception relative to stimulus waveform. A: mean phase of perceived velocity (i.e., the derivative of potentiometer trace) relative to the GVS waveform and encoder velocity. B: corresponding time differences for data plotted in A. Black and gray shaded regions = ±1 SE for GVS and real rotations, respectively.

Using a homologous single-interval manual tracking paradigm, we measured the phase relationship between perceived velocity and the actual velocity of the rotary chair during sinusoidal real whole body rotations. Representative data from one participant are shown in Fig. 6, right. As depicted in Fig. 7A, perceived real velocity was in phase with the actual rotary chair velocity across all test frequencies. Figure 7A shows that on average the phase shift between perceived velocity and actual velocity was near 0° (mean = 8.5°; SD = 5.9°) regardless of stimulus frequency. To compare phase across frequencies, we conducted a one-way repeated-measures ANOVA with mean phase as the dependent variable and real rotation frequency as the independent variable. One participant was removed from the repeated-measures ANOVA because coherence failed to reach the 95% confidence level for one frequency. This failed to return a significant effect of frequency (F_4,20 = 1.4059, P = 0.268). Therefore, the phase of real kinetic perception was independent of stimulus frequency over the 0.05–1 Hz range.

DISCUSSION

These results characterize the gain and phase of vestibular perception relative to real and virtual whole body yaw rotations. We hypothesized that virtual perception gain and phase would not be influenced by frequency based on nonhuman primate afferent recordings (Goldberg et al. 1982) over the frequency range we tested; however, we found that the gain of virtual perception decreased with increasing stimulus frequency (above 0.5 Hz) and the phase of velocity perception relative to the GVS waveform advanced with increasing frequency (above 0.1 Hz). Additionally, although real direction discrimination thresholds are well known, information on the phase of perception relative to real kinetic stimulation is scarce. We found that real velocity perception was in phase with the angular velocity of the input stimulus, in line with the neocortical responses reported in nonhuman primates (Grüsser et al. 1990) that show a small phase lead relative to stimulus angular velocity. This also confirms previous reports, based on a different methodology, that perceived that velocity and real velocity are nearly in phase (Mergner et al. 1981, 1991). It is clear from these results that these two modes of vestibular stimulation have dramatically divergent stimulus-to-perception transfer functions. While filtering of the stimulus could occur at both the peripheral and central levels, we speculate that these differences are mostly related to differences in stimulus transduction for electrical vs. real kinetic stimulation.

Human Discriminability for Rotation Direction

As predicted from the high-frequency gain of the vestibular system, real rotation thresholds reduced with increasing frequency (Benson et al. 1989; Grabherr et al. 2008; Soyka et al. 2012). We observed this effect with the head pitched down; therefore, stimulating different semicircular canals (horizontal in previous studies; anterior and posterior in the present study) did not affect perceptual sensitivity to whole body yaw rotations. Conversely, to reach the same threshold level of performance using virtual rotations, on average, humans required larger amplitudes of current at higher stimulation frequencies.

The decrease in human sensitivity to virtual rotations with increasing frequency contrasts with the gain spectrum of the GVS-to-afferent transfer function reported in nonhuman primates, which was insensitive to, or increased slightly with, increasing stimulus frequency (Goldberg et al. 1982). At higher frequencies (1–10 Hz), the GVS-to-afferent response appears to increase further (Kim et al. 2011). This stands in stark contrast to our direction discrimination experiment results, which would suggest that the GVS-to-afferent gain is decreasing with increasing stimulus frequency. Differences in electrical stimulation protocols likely led to this discrepancy; in animal studies current was injected directly into the perilymphatic space of the vestibule, whereas in human GVS studies current is injected percutaneously. Low-pass filtering of the electrical stimulus may occur as it travels through tissue, bone, and cerebrospinal fluid to reach the underlying vestibular afferents. Individual differences in mastoid thickness, for example, could account for the larger variability observed in the virtual rotation data relative to the real rotation data. The animal literature supports progressive filtering of GVS from skin surface to afferents; vestibular afferents were found to be 10 times more sensitive to direct current injected into the tensor tympani than at the surface (Kim and Curthoys 2004), and vestibular afferents were found to be slightly less sensitive to alternating current injected into the vestibule than into the internal auditory meatus (Goldberg et al. 1984). Postural research also supports low-pass filtering of GVS (Peterka 2012); however, the filter cutoff reported in that study was sharper than that measured for perception here, potentially because of additional low-pass filtering by the musculoskeletal system (Dakin et al. 2010; Fitzpatrick et al. 1996).

Our finding of reduced sensitivity to virtual rotations at higher frequencies is corroborated by Wardman et al. (2003), who tested the ability to detect leftward vs. rightward virtual tilt evoked by a variable-duration (0.04–5 s) 1-mA square-wave current step in both free standing and immobilized standing participants. When participants were free standing they accurately perceived the postural response toward the anode; when participants were immobilized they perceived virtual movement toward the cathode. Importantly, the perception of virtual movement direction worsened when stimulus duration was decreased and was abolished for current steps lasting <0.4 s in free standing and <1 s in immobilized participants. This result from Wardman et al. (2003) agrees well with the present finding that yaw rotation direction discrimination thresholds worsen at higher frequencies (i.e., 1–2 Hz).

Phase Lag of Perception

Perception of real rotations follows predictions based on the phase of responses at the level of the neocortex (Grüsser et al. 1990)—perceived velocity led angular velocity by a small amount (8.5°) regardless of frequency over the 0.05–1 Hz range. Neural responses in the vestibular nucleus (Dickman and Angelaki 2004), thalamus (Büttner et al. 1977), and neocortex (Grüsser et al. 1990) all lead real velocity by 0–30° across tested frequencies, setting up a strong prediction that perception would also lead real velocity. Our results confirmed this prediction, lending support to the notion that our manual tracking task was tapping into neural processing in the vestibular system.

In sharp contrast to real rotations, we further demonstrate that perceived velocity during a continuous sinusoidal virtual movement is in phase with the GVS current being injected at low frequencies (0.05–0.1 Hz) and that the phase of perceived velocity advances as the frequency is increased. This phase shift strongly suggests that humans perceive GVS as an angular velocity signal at low frequencies and perceive GVS increasingly as a position signal at higher frequencies. Importantly, the phase spectrum for GVS perception measured here spans an important range of natural head movement frequencies (Grossman et al. 1988; Mayne 1974; Pozzo et al. 1990). Previous research investigating the perception of GVS used DC current, which represents a somewhat unnatural vestibular stimulus (Wardman et al. 2003; St. George et al. 2011). While St. George et al. (2011) concluded that GVS is interpreted as an angular acceleration signal, we suggest that this result does not generalize to naturalistic head movement frequencies. In pilot studies, we additionally measured the phase shift of perception at 0.01 Hz and found that perceived velocity was still in phase with the GVS waveform. This suggests that GVS continues to be interpreted as an angular velocity signal as stimulus frequency approaches 0 Hz (DC). Furthermore, we note that St. George et al. (2011) indeed showed evidence that humans interpret DC current as a velocity for at least 30 s, when neural adaptation appears to drive the perception of rotation to zero over ∼100 s; however, comparison between studies is difficult because St. George et al. (2011) had participants report their “perception of rotation” on a 5-point scale, whereas here we directly asked participants to track angular position.

Although our method for measuring the phase lag of virtual and real rotation perception is somewhat novel, the general approach is well grounded in the literature on synchronization of tapping along to an auditory stimulus (for review see Repp 2005), as well as in the literature on manual tracking (Cliff 1973; Gerisch et al. 2013; Ivry et al. 1988; Netick and Klapp 1994; Stepp 2009; Vercher and Gauthier 1992). Both of these lines of research suggest that humans are able to preemptively send motor commands such that the output movement (tapping, tracking, turning a knob, etc.) is synchronized with perception (Blouin et al. 2004; Paillard 1955). Trainor et al. (2009) used GVS to disambiguate perceived auditory rhythms. They utilized a tapping task similar in principle to the manual tracking task used here to confirm that the head was perceived to move at the same rate as the GVS stimulus. Perceived delays in the range of 170–260 ms were reported, a range that agrees well with the temporal lags we observed for virtual rotations. Although simple in nature, our phase experiment demonstrates something fundamental about how GVS is interpreted by the CNS. At 0.05–0.1 Hz our results suggest that GVS is interpreted as an angular velocity signal; GVS becomes increasingly interpreted as an angular position signal at higher frequencies. We observed phase lags between the potentiometer trace (the subject's perceived position) and the GVS waveform of up to 5.3 s for 0.05 Hz, demonstrating that the effect is not merely a tracking error or a delay in the motor command. It should be noted that measuring the phase of perception in this manner is limited to a frequency bandwidth of ∼0.01–1 Hz. Mergner et al. (1981) also found that participants were no longer able to track their vestibular perception via vocalizations at 1 Hz and above because of the task demands.

Our findings for the phase of perceived virtual and real rotation partly agree with related research into the temporal processing of virtual and real vestibular inputs. Vestibular input is perceived slowly (Barnett-Cowan et al. 2012; Barnett-Cowan and Harris 2009, 2011; Baxter and Travis 1938; Chang et al. 2012; Sanders et al. 2011; for review see Barnett-Cowan 2013). When paired with visual, tactile, and auditory inputs, virtual and real vestibular inputs must be presented far in advance of the other modality in order to be subjectively perceived as simultaneous. For temporal order and simultaneity judgments, GVS must be delivered ∼160 ms prior to a touch, light, or sound in order for the two stimuli to be subjectively experienced as simultaneous (Barnett-Cowan and Harris 2009). Whole body rotations at 0.5 Hz must precede auditory tones in one study by ∼60 ms for simultaneity judgments and by 91 ms for temporal order judgments (Chang et al. 2012; Sanders et al. 2011).

While these temporal tasks have important differences, they are nonetheless related to our findings. Indeed, Soyka et al. (2013) have shown that reaction times can be predicted from direction discrimination thresholds. Our experiment allowed synchronization with a continuous vestibular input, while previous work has relied primarily on reaction times and perceived temporal order/synchrony between discrete inputs. It is important to note that participants were able to compensate and track real position/velocity, even though previous work using temporal order and simultaneity judgments would suggest that there should be a lag of real kinetic perception, not a slight lead as observed here. We did observe a substantial lag for GVS that agrees with the previous finding that GVS is perceived even later than kinetic stimulation for temporal order/synchrony judgments (Barnett-Cowan and Harris 2009). Interestingly, our finding that perceived position lagged the GVS waveform by ∼170 ms at 1 Hz agrees well with the stimulus onset asynchrony of ∼160 ms needed for a 1.2-Hz GVS pulse to be felt synchronously with a touch, light, or sound (Barnett-Cowan and Harris 2009).

Comparing Virtual and Real Rotation Perception

The discriminability of virtual rotation direction stands in stark contrast to the discriminability of real rotation direction. Sensitivity to real yaw rotations corroborates the nonhuman primate physiology—thresholds decrease at higher stimulation frequencies, likely because of the high-frequency gain of the vestibular system (Büttner et al. 1977; Dickman and Angelaki 2004; Goldberg et al. 1982; Grüsser et al. 1990). Therefore, the differences observed in sensitivity to electrical and kinetic stimulation of the vestibular system appear to be due mainly to differences in transduction early on in the vestibular pathway, since kinetic stimulation is transduced in a natural manner (deflection of hair cells) whereas GVS bypasses cupular mechanotransduction (Goldberg et al. 1984).

It should be noted that vestibular tests using real rotation have an unavoidable limitation: nonvestibular (e.g., somatosensory, interoceptive, and auditory) cues may enhance perception. Strain distributions over the skin and muscle proprioceptors, air passing over the face, interaural time and level differences, and visual optic flow all serve as potential cues to whole body motion that must be removed to obtain solely vestibular stimulation. Researchers have attempted to control such nonvestibular cues, for example, by using foam padding and layers of clothing to dampen somatosensory sensations, blowing air onto the face with fans or using a face shield to reduce air movement cues, playing white noise through headphones, and occluding vision (e.g., see Benson et al. 1989; Grabherr et al. 2008; Soyka et al. 2012). Although we took similar precautions (see materials and methods), contributions of nonvestibular cues cannot be entirely excluded. As is the case, it has been proposed that GVS derives its usefulness as a tool because it allows researchers to investigate the vestibular system in isolation (Day 1999; Fitzpatrick and Day 2004) while avoiding some of the limitations associated with real (whole body) vestibular stimuli. In fact, one must wonder whether the high-frequency gain of the vestibular system to real kinetic rotation is partly due to nonvestibular cues, given that virtual rotation sensitivity showed lower gain at higher frequencies. However, we doubt this is the case, given the report of a vestibular-deficient patient requiring a velocity of 38°/s to reach threshold-level performance at 0.5 Hz using nonvestibular cues alone (Mallery et al. 2010).

The usefulness of GVS in clinical assessment of vestibular function deserves consideration, even though it is largely an assessment of central processing mechanisms. It has been suggested that real rotation testing could provide a “vestibulogram” akin to the commonly performed clinical audiogram (Grabherr et al. 2008); however, virtual rotation testing would be far more economical and practical in a clinical setting. GVS, however, presents other challenges because it uniformly stimulates afferents from all canals and otolith organs (Fitzpatrick and Day 2004) and preferentially activates irregular afferents (Goldberg et al. 1982, 1984). Nevertheless, the findings presented here represent a step forward for future applications of GVS. Standard psychophysical thresholds and the phase of perception for virtual rotations provide the quantitative details required to know approximately how much current should be delivered, and at what time lag, to evoke the perception of arbitrary, yet well-defined virtual whole body motion. Our results suggest that a 1-Hz sinusoidal GVS current with a peak amplitude of 1 mA is perceived to be roughly identical in magnitude to a 1-Hz sinusoidal real velocity pulse with a peak velocity of 1°/s.

GRANTS

This work was funded by the Natural Sciences and Engineering Research Council (NSERC) Discovery Grants of J.-S. Blouin and J. T. Inglis. J.-S. Blouin received additional support from the Canadian Chiropractic Research Foundation and salary support from the Michael Smith Foundation for Health Research. R. M. Peters received salary support from NSERC funding granted to J. T. Inglis.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the author(s).

AUTHOR CONTRIBUTIONS

Author contributions: R.M.P., B.G.R., J.T.I., and J.-S.B. conception and design of research; R.M.P. and B.G.R. performed experiments; R.M.P. analyzed data; R.M.P., B.G.R., J.T.I., and J.-S.B. interpreted results of experiments; R.M.P. prepared figures; R.M.P. drafted manuscript; R.M.P., B.G.R., J.T.I., and J.-S.B. edited and revised manuscript; R.M.P., B.G.R., J.T.I., and J.-S.B. approved final version of manuscript.