ENDOR spectroscopy and DFT calculations: evidence for the hydrogen bond network within α2 in the PCET of E. coli ribonucleotide reductase

Abstract

E.coli class I ribonucleotide reductase (RNR) catalyzes the conversion of nucleotides to deoxynucleotides and is composed of two subunits: α2 and β2. β2 contains a stable di-iron tyrosyl radical (Y₁₂₂^•) cofactor required to generate a thiyl radical (C₄₃₉^•) in α2 over a distance of 35 Å, which in turn initiates the chemistry of the reduction process. The radical transfer process is proposed to occur by proton-coupled electron transfer (PCET) via a specific pathway: Y₁₂₂ ⇆ W₄₈[?] ⇆ Y₃₅₆ in β2, across the subunit interface to Y₇₃₁⇆ Y₇₃₀ ⇆ C₄₃₉ in α2. Within α2 a co-linear PCET model has been proposed. To obtain evidence for this model, 3-amino tyrosine (NH₂Y) replaced Y₇₃₀ in α2 and this mutant was incubated with β2, CDP and ATP to generate a (NH₂Y₇₃₀•) in D₂O. [²H]-Electron-nuclear double resonance (ENDOR) spectra at 94 GHz of this intermediate were obtained and together with DFT models of α2 and quantum chemical calculations allowed assignment of the prominent ENDOR features to two hydrogen bonds likely associated with C₄₃₉ and Y₇₃₁. A third proton was assigned to a water molecule in close proximity (2.2 Å O-H---O distance) to residue 730. The calculations also suggest that the unusual g-values measured for NH₂Y₇₃₀• are consistent with the combined effect of the hydrogen bonds to Cys₄₃₉ and Tyr₇₃₁, both nearly perpendicular to the ring plane of NH₂Y₇₃₀. The results provide the first experimental evidence for the hydrogen bond network between the pathway residues in α2 of the active RNR complex, for which no structural data is available.

Introduction

Ribonucleotide reductases (RNRs) convert nucleotides (NDPs) to their corresponding deoxynucleotides (dNDPs) in all organisms based on a catalytic mechanism which involves highly controlled radical chemistry.^1,2 These enzymes are responsible for generating a balanced pool of the deoxynucleoside triphosphates (dNTPs) and provide the monomeric precursors for DNA replication and repair³. The E. coli class Ia RNR is composed of two homodimeric subunits α2 and β2, which form an α2β2 complex during nucleotide turnover. β2 harbours a stable di-iron tyrosyl radical (Y₁₂₂^•) cofactor, which is essential for catalysis. Each turnover requires radical propagation from Y₁₂₂^• to the active site in α2, where a cysteinyl radical C₄₃₉^• is generated and initiates nucleotide reduction. A model for radical propagation has been postulated (Figure 1) based on in silico docking of the X-ray structures of the individual subunits, and requires that Y₁₂₂^• is ∼ 35 Å removed from C₄₃₉^{• 4,5}. Using PELDOR spectroscopy, our distance measurements between Y₁₂₂^• and a nitrogen radical from the mechanism-based inhibitor 2′-azido-2′-deoxyuridine 5′-diphosphate trapped in the active site of α2 provided the first evidence for the long distance between the two sites.⁶

Fig 1.

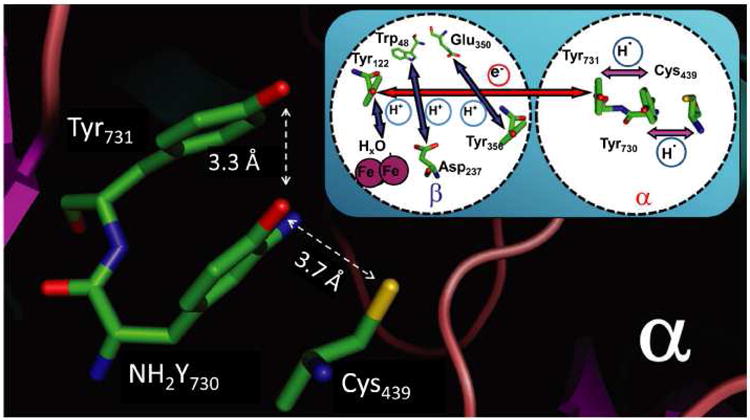
Crystal structure of the Y₇₃₀NH₂Y-α2 mutant of E. coli RNR from¹⁹ (molecule A) showing the residues Y₇₃₁, NH₂Y₇₃₀ and C₄₃₉ in the PCET pathway. The O-O distance and the O-S distance between the essential amino acid residues are marked. The inset shows the current model for the long-range (∼ 35 Å), reversible PCET starting at Y₁₂₂ in β2 and reaching C₄₃₉ in α2. Note that the position of Y₃₅₆ is unknown as the C-terminus is disordered in all X-ray structures of β2.

The unprecedented mechanism for radical initiation over a pathway of conserved redox active amino acids, Y₁₂₂^• ⇆ [W₄₈?] ⇆ Y₃₅₆ on β2 and Y₇₃₁⇆ Y₇₃₀ ⇆ C₄₃₉ in α2, has attracted considerable attention in the past decade. Mutagenesis studies provided the first experimental support for the importance of the proposed pathway residues.^7,8 However, recent studies using site-specifically incorporated unnatural tyrosine analogs with altered redox potentials and pKas in place of pathway residues^9-11 and studies with photo-initiated RNRs¹² have suggested a more detailed chemical mechanism for this long-range oxidation. Specifically co-linear and orthogonal proton-coupling to the electron transfer (PCET) steps within α2 and β2 respectively, have been proposed.^13,16 The mechanism of oxidation across the subunit interface remains unclear, as the C-terminal 35 amino acids of E. coli β2, which include pathway residue Y₃₅₆, are not visible in the structure.

The original crystallographic data of α2,- indicated that the orientation of Y₇₃₁ and Y₇₃₀ relative to each other (Figure 1, inset) is unusual and suggested a co-linear PCET mechanism^14-17 The importance of Y731 and Y730 in the initiation process is supported by a number of different types of experiments. Mutagenesis studies in which Y₇₃₁ and Y₇₃₀ were replaced by F were inactive and the structures of the mutant-α2s were not perturbed, demonstrating their importance.⁸ The first direct support for the redox role of Y₃₅₆, Y₇₃₀ and Y₇₃₁ was obtained by site-specific incorporation of a variety of tyrosine analogues using either expressed protein ligation¹⁸ or the suppressor tRNA/aminoacyl-tRNA synthetase (RS) methodology¹¹. The insertion of 3-aminotyrosine (NH₂Y) in place of each of these Ys, for example, revealed that the Y₁₂₂• can oxidize NH₂Y to an amino tyrosyl radical (NH₂Y•) in the presence of the appropriate substrate and allosteric effector pair, while retaining low levels of enzymatic activity.^11,19,20 Thus, the NH₂Y•s, produced in a kinetically competent fashion, represent excellent mechanistic probes of a pathway radical intermediate involved in nucleotide reduction.

The radical generated in NH₂Y₇₃₀-α2 E. coli RNR was recently characterized by multifrequency EPR spectroscopy²¹ and revealed unprecedented g-values (g_x = 2.0052, g_y = 2.0042 and g_z = 2.0022) suggesting that it resides in an environment with unusual electrostatic and H-bond interactions.²² This study prompted us to examine in more detail the structure around NH₂Y₇₃₀^• to find evidence for the proposed intermolecular hydrogen bonding network to the next essential pathway residues, Y₇₃₁ and C₄₃₉. For this purpose we have performed electron-nuclear double resonance spectroscopy (ENDOR) at high fields and frequencies (3.4 Tesla/94 GHz) to detect the hyperfine coupling to protons that were exchanged to deuterons using D₂O buffer. Specifically, the method at high fields permits separation of the resonances of the deuterons from the ¹H resonances of non-exchangeable protons or of other low-gamma (γ) nuclei such as ¹⁴N. Furthermore, the high-field/high-frequency approach allows orientation-selective experiments to be carried out.²³ Here, only a few molecular orientations are excited within individual experiments permitting the reconstruction not only of the magnetic hyperfine tensors, but also of their orientations in the molecular frame. Nevertheless, the interpretation of the EPR data in terms of a structure requires the use of sophisticated quantum chemical calculations, as was shown in previous work.^24-27 DFT calculations can generate possible molecular structures of the trapped intermediate that serve as a model(s) for the simulation of the EPR data. The calculated EPR parameters can then be employed for the interpretation of the spin-Hamiltonian parameters of the intermediate. In the present study we demonstrate that the combination of the ENDOR and DFT methods has allowed the assignment of exchangeable protons with their distances and orientations around the NH₂Y₇₃₀^• intermediate. Moreover, our study detects an exchangeable proton that given the X-ray structures and the observed magnetic parameters is very likely a conserved water molecule at close distance (2.2 Å O-H---O distance) from the oxygen of NH₂Y₇₃₀^•. This water molecule was previously mentioned by Siegbahn and co-workers, but was not used in their model¹⁴ and has not been considered to date in a possible PCET mechanism within α2. The present work has used combined experimental and theoretical approaches to establish the hydrogen bond network in the PCET pathway in α2 of an active RNR complex for which no X-ray structure is available. The results are used to rationalize the radical transfer between Y₇₃₁⇆ Y₇₃₀ ⇆ C₄₃₉ in α2.

Results

94 GHz ENDOR spectra of exchangeable protons coupled to NH2Y₇₃₀^•

ENDOR spectra of a radical reveal the hyperfine couplings to magnetic nuclei that are in the vicinity (usually approx. ≤ 5 Å) of this center. When the buffer is exchanged with D₂O, a deuterium ENDOR spectrum displays the resonances of exchangeable protons, such as those attached to heteroatoms including water molecules and amide protons in proximity. Each deuteron contributes to the spectrum a line pattern characterized by a hyperfine and quadrupole splitting (quartet). A representative deuterium ENDOR spectrum of the NH₂Y₇₃₀^• radical trapped during reaction of NH₂Y₇₃₀-α2, β2, CDP/ATP in D₂O buffer is displayed in Figure 2. The spectrum shows a complex deuterium ENDOR signal with a spectral width (± ∼ 2 MHz) consistent with the strong deuterium resonances of the ND₂-group of the NH₂Y^• probe, as previously reported from the analysis of the EPR spectra²¹, and other overlapping signals. Nevertheless, the most prominent features are two strong peaks at around ± 0.6 MHz and a Pake pattern of at least one weakly coupled deuteron around the deuterium Larmor frequency. The strong peaks at ± 0.6 MHz lie in a region expected for coupled hydrogen bonds.^28,29 To gain insight into the possible number of exchangeable protons that could contribute to the ENDOR spectrum, the crystal structure of the NH₂Y₇₃₀-α2 mutant in the resting state was examined. However, it should be kept in mind that the X-ray structure of the active α2β2 RNR complex is unknown. The predominant structure reported of NH₂Y-α2¹⁹ (Figure 1) is very similar to the wt structure (see also overlaid structures in SI, Fig.S8) and suggests that several exchangeable protons might be found in a distance range of ≤ 5 Å (Figure 2, inset) in the active complex. First, the oxygen of Y₇₃₁, the sulfur of C439 and the oxygen of Y₄₁₃ are all protonated and could contribute H bonding interactions with NH₂Y₇₃₀^•. Second, two water molecules reside at distances < ∼ 5 Å, but only one is conserved in the structure of resting wt α2.

Fig. 2.

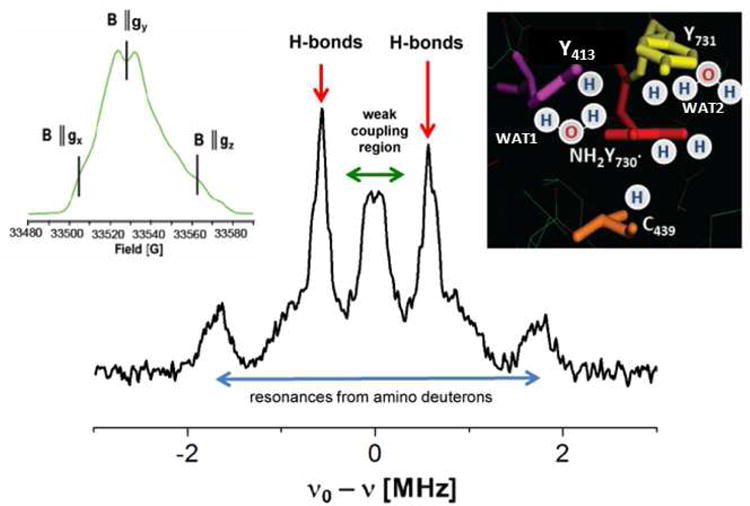
94 GHz ²H-Mims ENDOR spectrum of NH₂Y₇₃₀^• in D₂O assay buffer generated after reaction of NH₂Y₇₃₀ with CDP/ATP and quenching after 30 s. The spectrum was recorded at B‖g_y (see EPR spectrum on the inset left). Different resonant regions are indicated with arrows and discussed in the text. The stars denote blind spots given by the Mims ENDOR sequence. The inset reveals the resting structure of NH₂Y₇₃₀-α2 from¹⁹ and possible protons around this residue. The water molecules have been denoted WAT1 and WAT2, as described in the text. Exp. conditions (for field sweep): T = 70 K, MW (π/2) = 32 ns, τ = 260 ns, MW (π) = 64 ns, repetition time = 3 ms, shots/point = 50, scans = 700. Conditions (for ENDOR): T = 10 K, MW pulse (π/2) = 20 ns, τ = 360 ns, RF pulse = 40μs, repetition time = 150 ms, 1700 scans.

Couplings to external H-bonds

To increase the resolution of the ENDOR experiment we recorded ENDOR spectra of the segments in the region of ± 1.5 MHz together with their orientation selection (Fig.3b). The unusual intensity of the peaks occurring at ± 0.6 MHz suggested that they could constitute the perpendicular component of an axially symmetric hyperfine tensor (A_⊥) arising from more than one deuteron. If this tensor was purely dipolar, the wings at about 1-1.2 MHz could represent the A_‖ component; however the amino deuterons also contribute in this region and are not distinguishable. We note that the precise form of the ND₂ tensors is still to be established, as previous EPR simulations²¹ were not sensitive to small tensor components (i.e. ≤ 6 MHz for ¹H or about ≤ 1 MHz for ²H).

Fig. 3.

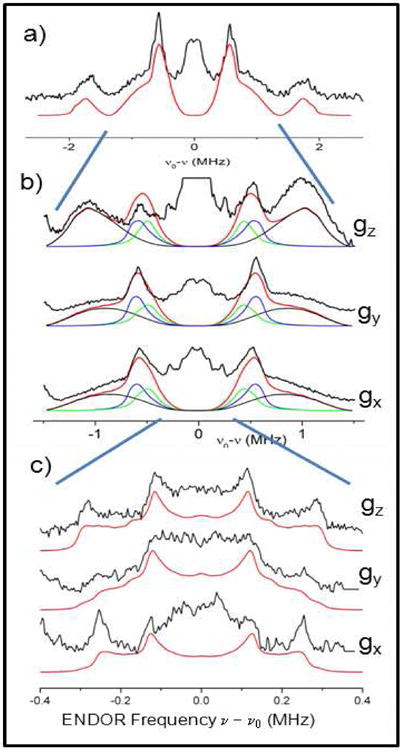
(a) ²H-Mims ENDOR spectrum of NH₂Y₇₃₀^• in D₂O assay buffer of the weakly coupled regions (black, from Fig. 2) and simulation (red). (b,c) Orientation selected ENDOR spectra recorded across the EPR line (Fig.2, inset). The blue lines connect the spectra segments from (a) to (c), which have been expanded. (b) Conditions: T = 10 K, MW pulse (π/2) = 20 ns, τ = 320 ns, RF pulse = 40 μs, repetition time = 150 ms, 2000-3000 scans/spectrum, 1 shot/point, random RF acquisition. The simulation (red) has been decomposed in the three separate simulations with the parameters for Y₇₃₁-²HO (blue spectra) and C₄₃₉-²HO (green spectra) and the amino deuterons (²H)₂N (black) as listed in Table 1. (c) Exp. conditions as in (b) but τ = 360 ns. Simulation parameters are reported in Table 1. Average rmsd were about 0.09 for (b) and 0.07 for (c), as explained in Methods.

The orientation selective spectra in Figure 3b displayed a pronounced attenuation of the ± 0.6 MHz peaks at B ‖ g_z. Attempts to simulate the spectra using either one or two deuterons with a pure dipolar tensor of 2A_⊥ = A_‖ ≈ 1.2 MHz and g_z ‖ A_‖ did not reproduce the observed intensities, when the absorption of the amino deuterons was taken into account as previously reported.²¹ We also note that part of the tensor belonging to the ± 0.6 MHz resonances might be hidden in the central part of the ENDOR spectrum, where the intensities are attenuated by the central hole of the Mims sequence³⁰ and overlap with the weakly coupled deuteron(s). Therefore, the results indicated that the complete form of the hyperfine tensors cannot be easily disentangled from the observed ENDOR spectra.

The orientation selected ENDOR spectra in Figure 3c display the region of ± 0.4 MHz with higher resolution. In this region, the ENDOR line shape is reminiscent of a dipolar powder pattern from mainly one deuteron, with the orientation selectivity leading to sharpness of the spectra at B ‖ g_x and g_z. The position of the outer edges shifts only slightly between 0.25 MHz (g_x) and 0.3 MHz (g_z), suggesting a counteracting effect of orientation dependent hyperfine and quadrupole splittings. As in the high-field limit the quadrupole coupling splits the ENDOR line symmetrically around the hyperfine value, the observed line shape sets boundaries for the hyperfine tensor of this weakly coupled deuteron with A_‖ ≈ 0.4 MHz. On the other hand, a further determination of the tensor elements and particularly their orientations is aggravated by the uncertainty about the number of weakly coupled deuterons contributing to this region.

Overall, the ENDOR spectra of Fig. 3 contain the fingerprints of the hydrogen bond network around NH₂Y₇₃₀^• however spectral simulations could not be performed a priori due to the large and unknown number of possible couplings. We note that the ENDOR simulation requires at least eight independent parameters for each deuteron, i.e. three hyperfine and two quadrupole tensor components, three Euler angles relating A and g tensors if A and Q are assumed to be collinear. Not much rationale can be applied for the choice of these parameters, if a structural model and subsequent prediction of the related EPR parameters is lacking. Such a model can be obtained from DFT calculations, if possible energy minimized structures of the radical intermediate within its protein environment are examined.

Optimized structure of NH₂Y₇₃₀^• from combined DFT and ENDOR results

In order to find an optimized structure with magnetic parameters compatible to the ENDOR spectra, six different models were investigated by DFT. All structures used the coordinates of α-NH₂Y₇₃₀ as input, but differed in the number and arrangement of the water molecules. Because in the X-ray structure of NH₂Y₇₃₀-α₂ the amino tyrosine is reduced and the enzyme is not active, energy-minimized structures were evaluated on the basis of their computed EPR parameters for NH₂Y₇₃₀^• as compared with the previously reported EPR parameters²¹, i.e. the g-values and the large hyperfine couplings to the β-methylene hydrogens and the ¹⁴N nucleus of the amino group.

Specifically, two structures contained only the water molecule (WAT1) located between NH₂Y₇₃₀ and Y₄₁₃ (Fig. 2, inset), which is conserved in most X-ray structures,^5,19,31,32, whereas three other structures took into account a second water molecule visible in α-NH₂Y₇₃₀ and located at ∼ 5 Å from both the nitrogen and the oxygen atom of NH₂Y^• (Fig. 2, inset). We note that the second water molecule is not conserved in the wt structure, but could be present in the NH₂Y-mutant and contribute to the ENDOR spectra. The optimized cluster models are illustrated in the SI, Fig. S1- S5 and in Figure 4. However, the three structures (in SI models 1, 2 and 3) with two water molecules were discarded and not analysed further because they show that the second water molecule would interact strongly with either the carbonyl group of residue S₆₉₄ (Fig. S1) or with the amino group of NH₂Y^• (Fig. S2 and S3). Both situations would give rise to nitrogen and C_β methylene proton tensors for NH₂Y^• that are not compatible with our EPR data.²¹ Structure 5 (see Fig. S4, SI) with only one water shows this water molecule with one hydrogen at a 1.9 Å from the oxygen of NH₂Y^•. The calculated dipolar tensor for this deuteron has eigenvalues that by far exceed the experimentally observed values in Figure 3C. Finally, structure 4 (Figure 4) shows the water molecule with a proton at a distance of 2.2 Å. This arrangement generates a dipolar coupling tensor for this proton that is quite close to the experimental results (Table 1). Also, the DFT calculation of structure 4 predicts that both hydrogen bonds to the next essential pathway residues, Y₇₃₁ and C439, should be observable in the ENDOR experiments. The calculated couplings are reported in Table 1. The hydrogen bond lengths to Y₇₃₁ and C439 are quite different from one another (1.8 Å and 2.0 Å respectively, Figure 4). The form of the hyperfine tensor strongly depends on the out-of-plane angle between the bond vector and the NH₂Y^• ring, as indicated from systematic calculations that explore this dependency (data not shown; this behaviour has previously been described for a similar system, a quinone radical anion³³). This dependency is readily rationalized when considering that the H-bond starts to overlap with the π-orbitals of the NH₂Y^• for large out-of-plane angles, as was already reported³³. Thus, the form of the hyperfine tensor can significantly deviate from a typical axially symmetric dipolar tensor that we observed for the H-bond to the essential tyrosyl radical diiron-cofactor in S. cerevisiae RNR²⁹ Also, for large out-of-plane angles, the point-dipole model cannot be used to derive the hydrogen bond length from the hyperfine couplings.

Fig. 4.

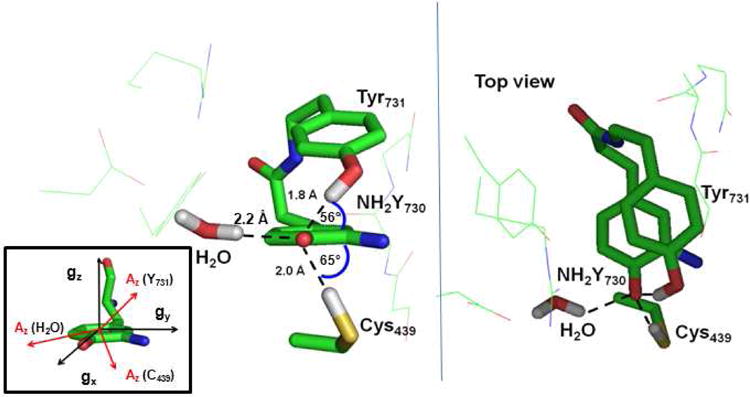
Combined EPR and DFT model for the hydrogen bond network around NH₂Y₇₃₀^• in α2. Left (side view): distances and out-of-plane angles of the hydrogen bonds and to WAT1 within the optimized DFT model 4. Right: top view. Inset: Location of the g tensor within the NH₂Y• structure. The direction of the hyperfine tensor component A_z of the three hydrogen/deuteron couplings is also schematically drawn.

Table 1. Summary of magnetic and structural parameters of the exchangeable deuterons coupled to NH₂Y^• from combined HF-ENDOR and DFT calculations.

	Ax	Ay	Az	α	β	γ	Q1	Q2	Q3	rO - D
Cys439-SD
Sim	-1.1	- 0.95	0.6	-17	38	-44	-0.03	- 0.02	0.06
DFT	-1.0	- 0.8	0.6	-17	38	-44	-0.04	- 0.03	0.07	2.0
Tyr731-OD
Sim	-1.25	- 1.15	0.7	-166	47	102	- 0.02	- 0.03	0.06
DFT	- 1.70	- 1.15	0.73	-166	47	102	- 0.04	- 0.06	0.11	1.8
2H2O
Sim H(1)	- 0.21	- 0.21	0.42	6	-81	10	0.14	- 0.06	- 0.05
DFT H(1)	- 0.31	- 0.28	0.64	6	-81	55	0.14	- 0.06	- 0.07	2.2
2H2N
Sim H(1)	-3.3	0	-2.7	7	6	75	-0.04	0.11	-0.06
DFT H(1)	-3.3	-0.1	-2.7	7	6	75	-0.05	0.13	-0.07
Sim H(2)	-3.8	0	-2.9	0	-5	-30	0.14	-0.08	-0.06
DFT H(2)	-4.0	0.1	-2.6	4	5	-30	0.14	-0.08	-0.06

All hyperfine and quadrupole values are given in MHz. The signs of the couplings from the simulation are only relative to each other within one tensor. The Euler angles (α, β, γ) in degrees are defined from the A to the g tensor with the second rotation around the y axis, counterclockwise. The orientation of the g tensor as well as the orientation of the A_z direction for the three weakly coupled deuterons is illustrated in Figure 4. The latter is defined such that it coincides with the bond direction. Instead, for the amino deuterons, the N-H bond defines the y direction. Distances are given in Å. Uncertainties in the experiment and calculations are estimated up to 20%. The spin distribution, as derived from the Loewdin population analysis of the DFT calculations of model 4, is as follows: O(NH₂Y^•) 0.21, N(NH₂Y^•) 0.16, carbon skeleton(NH₂Y^•) 0.54, S(Cys₄₃₉) 0.01, O(Tyr₇₃₁) 0.01.

The calculated EPR parameters derived from model 4 for five deuterons, i.e. Tyr₇₃₁-OD Cys₄₃₉-OD WAT1-OD and the amino deuterons, were used as input for the simulations of the ENDOR spectra. Specifically, all Euler angles predicted by the DFT calculation for the couplings to Tyr₇₃₁-OD and Cys₄₃₉-OD were kept fixed and the hyperfine and quadrupole tensors were allowed to change as much as ± 20-30 % in order to reproduce the position and the relative intensities of the ENDOR lines. The values obtained in this way should represent boundaries for the magnitude of the hyperfine tensors. The obtained simulations are presented in Fig. 3, and the simulation parameters are compared with the DFT values in Table 1. As a result, only minor refinement of the DFT values was required to obtain reasonable agreement between simulations and experimental spectra, with rmsd less than 0.1 (for spectra normalized to unity). The simulation of the strong peaks at ± 0.6 MHz is reproduced by the A_x ≈ A_y components of almost axially symmetric tensors, as predicted for the hydrogen bonds to Tyr₇₃₁ and Cys₄₃₉. However, in contrast to typical dipolar tensors, here we find |A_x,y| > |A_z| due to a non-zero isotropic coupling. Notably, the two tensors cannot be distinguished in the experimental spectra, but the intensity of both deuterons is required to reproduce the overall line shape, as also illustrated by the simulation of the single components in Fig.3b. The form of the tensors for these hydrogen bonds is consistent with the observed attenuation of the strong peaks (A_x,_y) at B‖g_z, as g_z is close to A_z, with the latter pointing to Tyr₇₃₁ and/or Cys₄₃₉ located above and/or below the NH₂Y₇₃₀^• ring (Fig.4). The resonance of the A_z component(s) remains invisible in the spectrum due to the overlap with the matrix peak. This region is also not reproduced by the simulation because of the hole caused by the Mims ENDOR preparation function.

The simulation of the weakly coupled deuteron required an overall scaling of the hyperfine tensor of about 30%, which is likely caused by a minor deviation of the DFT predicted distance with respect to the experimental distance. On the other hand, the orientation dependence of the spectra (Fig. 3c) was consistent with the calculated direction of the A and Q tensors for the closest deuteron of WAT1. Indeed A_z for this interaction lies almost in the tyrosine plane and points between g_x and –g_y (Figure 4). Thus, the results strongly support the assignment of this deuteron to WAT1. We observe that the simulation requires A_z closer to g_x than predicted by the DFT (Euler angle γ). Again, the deviation might suggest a small displacement of the water, but could also be within the combined experimental and computational uncertainty.

The simulation of the weakly coupled deuteron in 3c does not entirely reproduce the matrix peak, which indicates that some other deuterons (presumably the second deuteron of WAT1) contribute to the matrix peak. Finally, the A_y tensor components of the ND₂ resonances are also predicted around zero frequencies but not distinguishable. These resonances likely contribute the offset observed under the deuteron spectrum of WAT1. Moreover, the tensors of ND₂ extend over the entire ENDOR spectrum, with the remaining principal axis values A_x and A_z predicted far outside the resonances of the hydrogen bonds. This further supports our assignment of the strong peaks at ± 0.6 MHz to the external H-bonds.

DFT calculations of the g-tensor support strong hydrogen bond interactions to NH₂Y₇₃₀^•

We used the optimized structure (model 4) and further DFT calculations to rationalize the g-tensor observed for NH₂Y₇₃₀^•, which is the EPR parameter that is most sensitive to the electrostatic environment of a radical. Table 2 displays the g-tensors calculated for a set of model systems (see Table 2, entries 2-9) compared to the experimental value for the RNR mutant (see Table 2, entry 1).

Table 2. Comparison of computed g-values from DFT models with experiment.

		gx	gy	gz
1	Experiment	20052	2.0042	2.0022
	DFT model	Deviation from experiment [in ppt]
2	Model 4 (with WAT1)	-0.3	-0.1	-0.4
3	Model 6 (wirhout WATl)	-0.1	-0.1	-0.3
4		-0,6	-0.3	-0,5
5		-0.2	-02	-0.4
6		0.3	0.2	-0.2
7		0.8	0,1	-0,4
S		0.9	0.3	0.0
9		1.7	0,4	0.0

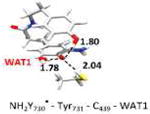
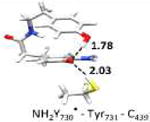
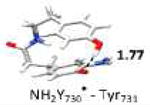
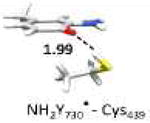
The optimized structure without water (entry 3, model 6) is shown in Figure S5. The optimized structure with water (entry 2, model 4) is shown in Figure 4. Small model systems were obtained by omitting residues of model 4, which are not involved in the PCET pathway. Hydrogen bond lengths are given in Å. Geometries and EPR parameters are calculated using COSMO (ε = 4.0). The uncertainty in the experimental values (entry 9) at 180 GHz is ± 0.1 ppt. The unit ppt refers as part per thousand of the g-value.

In the largest and most realistic model systems investigated here, 8 amino acid residues surrounding NH₂Y₇₃₀^• are included (Fig. S6a). The initial coordinates were taken from the predominant structure of α-NH₂Y₇₃₀ (see Figure 1)¹⁹ and subjected to constrained geometry optimization. The water molecule (WAT1) between Y₄₁₃ and NH₂Y₇₃₀^• is present in model 4 (entry 2), but was omitted in model 6 (entry 3; see also Figure S5 for comparison). For both models 4 and 6 a g_x-value is obtained that is very close to our experimental results (within the inherent accuracy of the theoretical methodology, i.e. ± 0.5 ppt). This shows on one hand that the model size is sufficiently large, but on the other hand that the g-tensor alone cannot be used to decide on the existence of the water molecule in the experimental structure. When comparing the experimental g_x-value to the calculated g_x-value of the isolated radical (entry 8) a substantial difference is observed (Δg_x = 0.9 ppt). This is likely indicative for strong hydrogen bond interactions from the protein environment. In order to rationalize the effect of the intermolecular hydrogen bonds in the protein on the g_x-value, the large models were systematically reduced in size (starting from entry 2) and the effect on the g-values calculated. Entry 4 shows the results for a model containing only the PCET pathway residues and WAT1, i.e. without the second sphere residues present in entries 2 and 3. With this model the g_x-value is decreased compared to entry 2. This is due to the missing effect of the surrounding residues on the strength of the hydrogen bond between WAT1 and NH₂Y₇₃₀^• (for more information see SI and Fig.S7). Removing WAT1 (entry 5) increases the g_x-value and brings it again back into the range of the experimental results. Removing the hydrogen bonding partners Cys₄₃₉ (entry 6) or Tyr₇₃₁ (entry 7) increases the g_x-value further by 0.5 and 1.0 ppt, respectively. Comparing entries 3 and 8 shows that it is mostly the combined effect of the hydrogen bonds to Cys₄₃₉ and Tyr₇₃₁, both nearly perpendicular to the ring plane of NH₂Y₇₃₀^•, which substantially shifts the predicted g_x-value towards the experimental value. The hydrogen bond to WAT1 also has an effect on the g_x-value, but it is strongly influenced by the second sphere residues.

The isolated radical (entry 8) contains an additional intra-molecular hydrogen bond and we expected a further increase of the g_x-value when removing this hydrogen bond. In order to analyze this we replaced the hydrogens of the amino-group in silico with methyl groups, thus removing the intramolecular hydrogen bond. As expected, an upward shift of the g_x-value is observed (entry 9).

Discussion

In order to obtain structural insight into a putative hydrogen bond network in the Y₇₃₁↔Y₇₃₀↔C₄₃₉ triad of E. coli RNR during radical propagation, 3-aminotyrosine (NH₂Y) was site-specifically incorporated at position 730 using the in vivo nonsense suppressor t-RNA/RS methodology.^34,35 Because the modified tyrosine residue has E°′ of 640 mV (pH 7), which is 190 mV¹¹ less than that of Y at pH 7, NH₂Y serves as a spin trap in radical transport through the α2-subunit. The first spectroscopic evidence for the formation of a NH₂Y^• in Y₇₃₀NH₂Y-α2 was provided by earlier studies using X-band EPR spectroscopy, stopped-flow UV-Vis kinetics¹¹ and PELDOR spectroscopy.²⁰ We have further characterized the electronic structure of the trapped radical at position 730 by multi-frequency EPR spectroscopy and have determined its g-values.²¹ The latter analysis suggested strong intermolecular H-bonding interactions around NH₂Y^• that could be essential for the PCET in the α-subunit.

The mutant employed here, Y₇₃₀NH₂Y-α2, has activity 5-8 % that of wt-α2¹⁹ and provides a means to obtain structural insight in the PCET pathway within the α2-subunit of the α2β2 complex. Our high-field ENDOR spectra illustrate couplings to several exchangeable protons in a distance range < 5 Å to the oxygen of NH₂Y^•. Several spectral features, but particularly strong resonances at ± 0.6 MHz and a weakly coupled deuteron(s) with 2A_⊥ = A_‖ ≈ 0.4 MHz, provide fingerprints of the hydrogen bonding interactions of this intermediate. The DFT calculation on the basis of the X-ray structure in the resting state predicts an optimized structure of the NH₂Y₇₃₀^•, in which three external deuterons should be visible in the ENDOR spectrum (i.e. with A_x,y,z ≥ 0.1 MHz), i.e. both exchangeable hydrogens of Y₇₃₁ and C439 and one deuteron of a water molecule located between NH₂Y₇₃₀ and Y₄₁₃ that is conserved in the wt structure. Spectral simulations illustrated that the hyperfine and quadrupole tensors predicted by DFT for this model are in agreement with the experimental spectra within 20% uncertainty. A more detailed DFT analysis of the g-values revealed that the observed unusual g-shifts are mostly due to the combined effect of the hydrogen bonds to Cys₄₃9 and Tyr₇₃₁, both nearly perpendicular to the ring plane of NH₂Y₇₃₀. This is the first combined experimental and theoretical evidence for a hydrogen bond network between the essential residues in the α2 subunit of E. coli RNR in an active complex.

The hydrogen bonds and hyperfine couplings observed in this study are assigned to residues that are conserved in the wt structure. Because of the strong similarities in the X-ray structures of wt and NH₂Y-α2 mutant around residue 730 (Fig. S8), our results strongly suggest that the observed hydrogen bond network is conserved also in the ‘active’ wt enzyme. The work establishes that the X-ray structure of the wt-α2 subunit alone with the unusual configuration of Y₇₃₁ and Y₇₃₀ is present in the active α2β2 complex in solution. We also note that the DFT optimized structure of the intermediate state (Fig. 4) reveals distances between the pathway residues Cys₄₃₉-NH₂Y₇₃₀-Y₇₃₁ that are considerably shorter than in the X-ray structure of the resting state (Fig.1). Specifically, the O---O distance between NH₂Y₇₃₀ and Y₇₃₁ decreases from 3.3 Å (Fig.1) to 2.7 Å (Fig. 4) and the O---S distance between NH₂Y₇₃₀ and Cys₄₃₉ from 3.7 Å to 3.4 Å. The obtained structural data can now be employed to rationalize the proposed mechanism of proton coupled electron transfer (PCET) within <x2.

For this goal, the energetics for the radical transfer between Y₇₃₁⇆Y₇₃₀⇆C₄₃₉ were computed as a function of suitably chosen reaction coordinates. Relaxed surface scans were carried out by pulling the hydrogen atom from Y731/C439 towards Y730^• via reducing the O(Y730^•) - H bond length. For this purpose, an even larger model than 4 (Figure 4) was constructed in order to include the residues around Cys₄₃₉ and the sugar of the substrate GDP (PDB code 4R1R³¹, model 7 with WAT1 and model 8 without WAT1, in Fig S6b SI); a total of 209 and 212 atoms, respectively, were considered in the quantum calculations. We display in Fig. 5 the energetics of the radical transfer using models 7 and 8 and consider explicitly the effect of the water molecule observed in this study. In the calculation with the water molecule, both Y₇₃₁^• and Y₇₃₀^• are isoenergetic, whereas radical transfer to Cys₄₃₉ is endergonic by about 3-4 kcal/mol. From these data it can be estimated that the oxidation potential of Cys₄₃₉ is about 120 mV greater than that of the tyrosines in the protein environment. Very recent studies on the distribution of the Y• between residues 356 in β2 and 731 and 730 in α2, suggested within error that Y731 and Y730 are isoenergetic³⁶. These results are consistent with the similarities of the reduction potentials of Y₇₃₁^• and Y₇₃₀^• obtained in this work. Until now no experimental data is available on the redox gradient towards C₄₃₉. Kinetic studies of tyrosyl radical reduction by the tripeptide glutathione indicate that the redox potential of the glutathione thiyl radical and the tyrosyl radical are nearly the same at pH 7³⁷. However this model system might be too simple to be compared with the Y₇₃₀^• and C₄₃₉^• in the active RNR complex.

Fig. 5.

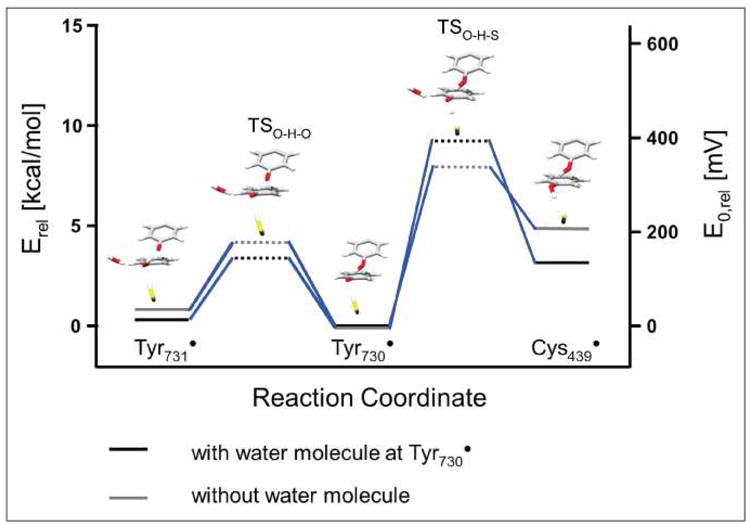
Energy level diagram including the transition states for the PCET pathway residues Y₇₃₁-Y₇₃₀-C₄₃₉. The reaction coordinate was investigated using the large models 7 and 8 (Table 2), with and without the water molecule. Shown are only parts of the optimized structures of model 7 (with water molecule).

Upon removal of the water molecule, a destabilization of Y₇₃₁^• and Cys₄₃₉^• is observed in the calculation. The activation barrier is diminished for the PCET from Y₇₃₀^• to Cys₄₃₉^• by 1.2 kcal/mol (from 9.2 to 8.0 kcal/mol), whereas the barrier for the PCET from Y₇₃₁^• to Y₇₃₀^• is only slightly increased (from 3.1 to 3.4 kcal/mol). We point out that the absolute errors for energy barriers are certainly not less than about 2 kcal/mol in these DFT calculations. However, when comparing trends in very similar systems, as done here, the errors should be much smaller and an energy shift of 1.2 kcal/mol between two closely related structures is considered significant. By removing the water molecule, the kinetics of the radical transfer from Y₇₃₁^• to Cys₄₃₉^• is accelerated by about one order of magnitude. The difference is small as compared to the much higher energy barrier dominating the overall kinetic behaviour in RNR (the turnover number of 2-10 s^-1 corresponds to a rate-determining step with a barrier of 15 to 16 kcal/mol). Nevertheless, changing a rate constant in a chemical mechanism perturbs the balance between different possible reaction channels and could allow off-pathway reactions to compete. We should mention that in the previous work of Siegbahn et al. the transfer from Y₇₃₁^• to Cys₄₃₉^• was studied, but with much smaller model systems and without WAT1¹⁴. Their calculated barrier for the transfer from Y₇₃₀^• to Cys₄₃₉^• is almost identical (8.1 kcal/mol), whereas the barrier for the PCET from Y₇₃₁^• to Y₇₃₀^• is 1.5 kcal/mol higher than the one predicted by our calculations (4.9 kcal/mol versus 3.4 kcal/mol). In their early calculations, the dispersion interaction was not taken into account and affects the interaction between both tyrosine residues (e.g. the tyrosine-tyrosine distance in the transition state for the radical transfer from Y₇₃₁^• to Y₇₃₀^•, measured by the C(-OH)-C(-OH) distance, is 3.25 Å in their calculations and 2.97 Å in ours). We further note that the determination of transition states in quantum chemical calculations makes no a priori assumption about the actual mechanism (e.g. H-atom transfer or PCET of any kind). The calculations simply provide a transition state structure and associated energy which have the mathematically and physically well-defined property of representing a point on the potential energy surface that is a local maximum in exactly one degree of freedom. The important question of how the electronic structure of the system is to be interpreted at this point is an entirely different subject. In the present case we find the perhaps surprising result that the calculated transition state does not reflect classical hydrogen atom transfer HAT. If it would be HAT, the calculation would have to show positive spin population on both tyrosine residues and negative spin at the transferring hydrogen. Rather one observes essentially no spin on the transferring hydrogen (see Figures S9) and half of the spin density each on both tyrosine π-electron systems (see Figures S9).³⁸ This means that the reaction is best described as a PCET in which the proton and electron move separately. It is noted that our results are in agreement with a recent study by Kaila and Hummer, who used dispersion corrected DFT for the calculation of the radical transfer between two stacked tyrosine residues.¹⁷ Their results, like ours for RNR, are in agreement with a co-linear PCET mechanism. Similar observations hold for the Y₇₃₀↔C₄₃₉ radical transfer (see Figure S9b). A more detailed theoretical analysis would involve going significantly beyond the Born-Oppenheimer approximation and is outside the scope of the present study.

Conclusion

We have presented using a combination of ENDOR spectroscopy and density functional calculations evidence for the structure of the hydrogen bond network around NH₂Y₇₃₀^• in an NH₂Y₇₃₀-α2β2 complex of E. coli RNR competent in nucleotide reduction. The short distances and hydrogen bonding interactions revealed along the radical transfer pathway Y₇₃₁⇆Y₇₃₀⇆C₄₃₉ support the current model of a co-linear PCET in the active α2. Our results also suggest the involvement of a water molecule near Y₇₃₀ in the active complex, which is conserved in available X-ray structures of the wt α2-subunit. The results give insight into the complexity of H-bond interactions, which could regulate the energetics of PCET at the molecular level.

Methods

Preparation of high-field EPR samples

Y₇₃₀NH₂Y-α2 was prepared as previously described.¹¹ Pre-reduced Y₇₃₀NH₂Y-α2 and β2 were combined in an equimolar ratio and concentrated at 4 °C in a Minicon concentration device (YM-30 membrane) to a final complex concentration of 100 μM (ε₂₈₀ nm (α2+β2)) = 320 mM^-1cm^-1) in D₂O buffer consisting 50 mM Hepes, 15 mM MgSO₄ and 1 mM EDTA (assay buffer) at pD 8.0. The concentrated samples were divided into 100 μL aliquots, placed in 1.5 mL Eppendorf tubes, and flash-frozen in liquid N₂. High-field EPR samples were prepared by thawing each aliquot on ice and adding CDP and ATP to final concentrations of 2 and 6 mM in a 20 μL sample volume. Each reaction was allowed to proceed for ∼ 30 s at room temperature and was then quenched in an EPR tube by hand-freezing with liquid N₂. The reaction in D₂O was carried out after exchanging the H₂O buffer of prereduced α2-NH₂Y₇₃₀ and β2 into deuterated assay buffer by 5 concentration/dilution cycles at 4 °C using a Minicon concentration device and a YM-30 membrane until the solution consisted of > 99% deuterated buffer. Nucleotide stock solutions were also prepared in deuterated buffer.

High-frequency pulsed EPR and ENDOR on NH₂Y₇₃₀^• in D₂O buffer

Samples for 94 GHz EPR and ENDOR spectroscopy contained a volume of ∼ 0.25 μL in a Suprasil quartz tube from Wilmad (OD 0.5 mm) and 0.5 μL in a quartz tube from Bruker (OD 0.9 mm), respectively. Echo-detected EPR spectra at 94 GHz were recorded at 70 K on a Bruker E680 W-band with 400 mW output power. At 70 K only the ND₂Y₇₃₀^• contributes to the pulsed EPR spectra due to the fast relaxation of the Y₁₂₂^• in the vicinity of the di-iron cluster ³⁹. In order to get a spectrum with high signal-to-noise ratio, the shot per points (SPP), number of field sweeps and repetition times had to be adjusted appropriately at 70 K. Experimental conditions are given in the caption of Figure 2. 94 GHz ²H ENDOR measurements were carried out with the Mims ENDOR sequence (π/2 - τ - π/2 - RF - π/2 - τ - echo) at 10 K and random radio-frequency acquisition. RF pulses were produced with a 250 W RF amplifier from Amplifier Research (AR). τ and π/2 values were optimized for each experiment and spectral range as described in the figure captions. As the EPR/ENDOR spectra at 10 K also contain about 50-60% contribution of Y₁₂₂^•, the matrix peak of Y₁₂₂^• was recorded in a separate experiment and subtracted for each spectrum of Fig.3C. However, no H-bonds are observed in Y₁₂₂^•, as known from previous studies.^22,29,40 All displayed ENDOR spectra were normalized for comparison with simulations.

Simulations of ENDOR spectra

94 GHz deuterium ENDOR spectra were simulated using a MATLAB routine developed in-house that is based on a first order Hamiltonian (high-field condition) for the hyperfine and quadrupolar interaction^23,29. Previous simulations of the EPR spectra at two different frequencies (9 and 94 GHz)²¹ provided a set of hyperfine tensor principal values (A_x, A_y, A_z) and Euler angles (α,β,γ) for the strongly coupled nuclei of NH₂Y₇₃₀^• in D₂O and H₂O buffer, i.e. the β-methylene proton, the ¹⁴N of the amino group. The blind spots produced by the Mims ENDOR sequence were included by multiplying the calculated ENDOR powder pattern with an envelope function given by I_ENDOR = 1 - cos(2πAτ).⁴¹ This is valid for the I = 1 nuclei considered here, as all quadrupole couplings are much smaller than the hyperfine values.³⁰ The simulations of spectra 3A and 3B did not include the weakly coupled deuteron due to the central hole produced in the simulation by the blind spots of the Mims sequence. For the same reason, the simulation of spectra 3c was performed without convolution with the blind spot function.

RMSD values for the orientation selected traces within one kind of experiment (Fig.3b or c) were summed up and subsequently divided by the number of traces. In both cases, the central part of the spectrum was not considered, i.e. the region of ± 0.37 MHz in (b) and ± 0.08 MHz in (c). Fitting procedures were not used due to the too large number of parameters involved.

Computational methodology

Cluster models 1 – 8

The cluster models were generated from the crystal structure of the Y₇₃₀NH₂Y-α2 mutant of E.coli RNR from¹⁹ (PDB code 2XO4). Models 1 to 6 incorporated, besides the residues NH₂Y₇₃₀^•, Y₇₃₁ and C₄₃₉ that are directly involved in the PCET process, the surrounding residues D₃₃₄, R₄₁₁, Y₄₁₃, S₆₉₄, A₆₉₅ and N₆₉₆ (see Figure S6 in SI). Models 4 – 5 additionally incorporated the water molecule (WAT1 in Figure S6), which is present between NH₂Y₇₃₀• and D₃₃₄ in all three molecules of the asymmetric unit of the crystal structure. Models 1 – 3 contain, in addition to WAT1, a second water molecule that is present in only two out of the three molecules in the asymmetric unit of the crystal structure (WAT2 in Figure S6). It is located between NH₂Y₇₃₀^• and S₆₉₄.

For the calculation of the PCET barriers for the triad Y₇₃₁↔Y₇₃₀↔C₄₃₉ more extended models were used in which the residues surrounding C₄₃₉ and the ribose of GDP are also included. The cluster models were generated from the crystal structure of E.coli RNR with bound substrate (PDB code 4R1R).³¹ The position of the backbone atoms of 4R1R and 2XO4 show practically no deviation in the region of interest (see Figure S8 in SI). Besides the region already incorporated in models 5 and 6 the residues T₃₀₃, L₄₃₈, E₄₄₁, C₂₂₅, C₄₆₂ and ribose as a mimic for the substrate GDP were taken into account for the extended cluster models. Model 7 (see Figure S6b in SI) includes WAT1 in the same position as in model 4, in model 8 this water molecule is not included (similar to model 6). Because the interactions studied here are very local in nature, we believe that the size of the cluster choosen here should be large enough to cover effects from the protein electrostatics.

For all model structures the side chains of the residues were always fully incorporated. Bonds were truncated in the backbone region between C_α and C(C=O) and between C_α and N(N-H), respectively, and replaced by a C-H bond. If the neighbouring residue is present in the model structure (as for S₆₉₄ – N₆₉₆ and NH₂Y₇₃₀^• – Y₇₃₁) the respective backbone bonds were not cut. In order to mimic the steric influence of the protein in the native enzyme structure Cartesian constraints were included in the geometry optimization of the model structures. For all residues the coordinates of the C_α and C_β atoms were kept fixed. In case of the truncated GDP model, the bonds between C4 and C5 of the ribose as well as the bond between C1 of the ribose and the base were replaced by a C-H bond. In order to preserve the orientation of ribose in the vicinity of C₄₃₉, Cartesian constraints were imposed on these C-H bonds.

For the simulation of the radical transfer relaxed surface scans were carried out by pulling the hydrogen atom from Y₇₃₁/C₄₃₉ towards Y₇₃₀^•. Transition state optimizations were performed using the P-RFO algorithm with an approximate Hessian matrix as an initial Hessian as implemented in ORCA ^42,43.

Small cluster model

Small model systems were constructed in order to analyze the influence of Y₇₃₁ and C₄₃₉ on the g-values of NH₂Y₇₃₀^•. For this purpose, large parts of model 4 were removed. The simplest model contains only the 2-amino-4-methyl-phenol radical (2-AMPR) representing NH₂Y₇₃₀^• (Table 2, entry 8). Y₇₃₁ and C₄₃₉ are mimicked by the bound neighbouring tyrosine (including the peptide bond) and cysteine, respectively. This gives rise to three additional models which are labelled as followed: NH₂Y₇₃₀^• – C₄₃₉, NH₂Y₇₃₀^• – Y₇₃₁, NH₂Y₇₃₀^• – Y₇₃₁ – C₄₃₉ (Table 2, entry 5-7). Model NH₂Y₇₃₀^• – Y₇₃₁ – C₄₃₉–WAT1 (Table 2, entry 4) contains an additional water molecule (in comparison to Table 2, entry 5). The effect of the intra-molecular hydrogen bond in NH₂Y₇₃₀^• was also investigated in one small cluster model (Table 2, entry 9). In this model the intra-molecular hydrogen bond is disrupted by substituting the hydrogen atoms of the amino group with methyl groups. In order to mimic the effect of the protein on the geometry, the C_α and C_β atom coordinates (C_β and C_γ for 2-AMPR without backbone) were kept fixed during the geometry optimization.

QM methodology

The optimizations were performed with spin unrestricted RI-BP using the def2-TZVP basis set in conjunction with the def2-TZVP/J auxiliary basis set.^44-48 Single-point energy calculations were carried out with RIJCOSX²⁴-B3LYP^48-51 using the def2-TZVPP basis set in conjunction with the def2-TZVPP/J as auxiliary basis set for all atoms. The long range electrostatic effects of the protein environment were crudely modeled using a dielectric continuum approach on the basis of the conductor like screening model (COSMO⁵²) with a dielectric constant (ε) of 4.0.⁵³ The EPR calculations were carried out using RIJCOSX-B3LYP using the EPR-II basis set (IGLO-II for sulfur) in accordance with def2-TZVPP/JK as auxiliary basis set for all atoms^54-56. Grimme's 2006 semiempirical dispersion correction (keyword VDW06 in ORCA) was included to account for dispersion effects⁵⁷. Numerical frequency calculations were carried out at the same level of theory as for the optimization. From these frequency calculations the zero point energies (ZPE) for the relative energies were derived.