The Bouma law of crowding, revised: Critical spacing is equal across parts, not objects

Abstract

Crowding is the inability to identify an object among flankers in the periphery. It is due to inappropriate incorporation of features from flanking objects in perception of the target. Crowding is characterized by measuring critical spacing, the minimum distance needed between a target and flankers to allow recognition. The existing Bouma law states that, at a given point and direction in the visual field, critical spacing, measured from the center of a target object to the center of a similar flanking object, is the same for all objects (Pelli & Tillman, 2008). Because flipping an object about its center preserves its center-to-center spacing to other objects, according to the Bouma law, crowding should be unaffected. However, because crowding is a result of feature combination, the location of features within an object might matter. In a series of experiments, we find that critical spacing is affected by the location of features within the flanker. For some flankers, a flip greatly reduces crowding even though it maintains target–flanker spacing and similarity. Our results suggest that the existing Bouma law applies to simple one-part objects, such as a single roman letter or a Gabor patch. Many objects consist of multiple parts; for example, a word is composed of multiple letters that crowd each other. To cope with such complex objects, we revise the Bouma law to say that critical spacing is equal across parts, rather than objects. This accounts for old and new findings.

Introduction

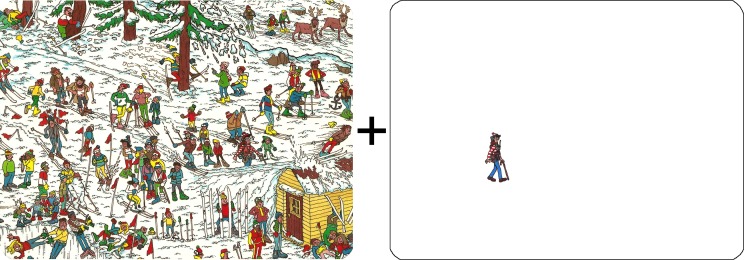
When presented with a cluttered scene, such as the one in Figure 1 (left panel), you can easily fixate any object and detect and combine its features to identify it. However, when the image is in the periphery, the same object can no longer be identified. This is not an acuity problem. While fixating the plus sign, Waldo is easily identified in isolation (Figure 1, right panel) but is impossible to identify in clutter (left panel). This phenomenon is called crowding—a breakdown of object recognition thought to be due to combining extraneous features from neighboring objects with those of the target.

Figure 1.

Crowding demo. In the cluttered scene (left panel), Waldo is impossible to identify while fixing your eyes on the plus sign. Removing the clutter reveals Waldo. This is not an acuity problem; it is crowding. (For further discussion of the role of crowding in search, see Balas, Nakano, & Rosenholtz, 2009; Motter & Simoni, 2007; Rosenholtz, Huang, Raj, Balas, & Ilie, 2012). (Ski slope scene and Waldo images reproduced from Where's Waldo? The Phenomenal Postcard Book, by Martin Handford, illustration copyright © 1987, 1997, 2007, and Where's Waldo?, by Martin Handford, illustration copyright © 1987, 1997, by permission of the publisher, Candlewick Press, Somerville, MA, on behalf of Walker Books London.)

To correctly identify an object, we combine its features, excluding those of any nearby objects. However, in the periphery, the features of nearby objects may be inappropriately combined with those of the target object, leading to a jumbled percept that cannot be identified. This is crowding: feature combination over an inappropriately large area (Bouma, 1970; Levi, 2008; Pelli, Palomares, & Majaj, 2004; Pelli & Tillman, 2008; Stuart & Burian, 1962; Toet & Levi, 1992). The mechanisms underlying crowding are not yet well understood (Hanus & Vul, 2013; Levi, 2008). Some explanations include feature pooling (Levi, Hariharan, & Klein, 2002; Parkes, Lund, Angelucci, Solomon, & Morgan, 2001; Wilkinson, Wilson, & Ellemberg, 1997), excessive feature combination (Levi et al., 2002; Pelli et al., 2004), feature averaging (Greenwood, Bex, & Dakin, 2009; Parkes et al., 2001), substitution of features or whole objects (Nandy & Tjan, 2007; Strasburger, 2005; Strasburger, Harvey, & Rentschler, 1991; Zhang, Zhang, Liu, & Yu, 2012), and limits of attentional resolution (He, Cavanagh, & Intriligator, 1996; Intriligator & Cavanagh, 2001).

The bounds of the area within which features are combined is specified by the critical spacing, which is the minimum center-to-center spacing needed between objects in order for the target object to be recognized. Critical spacing is a succinct measure of crowding.

Pelli and Tillman (2008) suggested that the known empirical rules of critical spacing be called “the Bouma law” in honor of Herman Bouma. Here, we distinguish several revisions (mark 1, 2, and 3) of this evolving law. Mark (abbreviated mk) traditionally designates the version of a product, including cars and computers. Each successive revision of this law describes a wider set of results while including the previous version as a special case.

Bouma law mk 1

Based on his results for small letters, Bouma (1970) said, “For complete visual isolation of a letter presented at an eccentricity of φ deg, … no other letters should be present within (roughly) 0.5 φ deg … [T]he adverse interaction is stronger … at the peripheral side … than the foveal side. The area of interaction is thus … egg-shaped towards the retinal periphery.”

Bouma later reduced his estimate of the proportionality constant from 0.5 to 0.4 (Andriessen & Bouma, 1976). The exact value of the proportionality constant, later named b, after Bouma, depends on the similarity of the flankers to the target, the number of possible targets, and the arbitrary threshold criterion (Pelli & Tillman, 2008). Furthermore, measurements in the fovea demand that Bouma's proportionality be generalized to linearity, incorporating an additive offset to account for the nonzero critical spacing at zero eccentricity (Liu & Arditi, 2000; Strasburger, Rentschler, & Jüttner, 2011; Toet & Levi, 1992). Bouma measured critical spacings that were much larger than his small letters (x-height: 0.2°), so it hardly mattered what point on each letter was used to measure the critical spacing of target and flanker. Later work showed that the critical spacing is independent of letter size, provided it is measured from the center of the target to the center of the flanker (Pelli et al., 2004; Strasburger et al., 1991; Tripathy & Cavanagh, 2002).

Bouma law mk 2

Reviewing a wide range of published critical spacings of simple objects, such as roman letters and Gabors, Pelli and Tillman (2008) proposed, “The Bouma law: For an object that can be identified in isolation, our ability to identify it among similar objects depends solely on the ratio of the object spacing to the observer's critical spacing at that location. The object is crowded whenever the ratio is less than one. For each observer, the critical spacing is independent of what the object is and depends only on where the object is in the visual field and the direction from target object to flanker object.”

Note that mk 2 of the law includes mk 1 as the special case of small letters in the periphery.

In general, when something is independent of other changes, we can say it is “conserved.” The most quoted principles of physics are laws of conservation (of energy, momentum, charge, etc.). The Bouma law mk 2 says that the critical spacing for a given location and direction in the visual field is conserved, equal for all objects and sizes, provided the flankers are similar to the target.

The Bouma law is counterintuitive. Vision science has largely studied visual interactions, like overlap masking, that scale with the signal and are independent of eccentricity (e.g., Legge & Foley, 1980). Crowding is an exception. The original Bouma law makes the unorthodox observation that the critical spacing of small letters scales with eccentricity. Mk 2 notes that this critical spacing is universal, conserved across the kind and size of object. Mk 2 adds this universality to mk 1's eccentricity.

In crowding studies, spacing is usually measured center to center, from the center of a target object to the center of a flanking object. Because the Bouma law refers only to objects, not features, it implies that crowding is unaffected by the location of features within the target and flanker.

Might the location of the features within each object affect the strength of crowding? For example, if a flanker object has an asymmetric distribution of features with most features on one edge, will crowding depend on whether the feature-rich or feature-poor edge is nearest to the target (Figure 2)?

Figure 2.

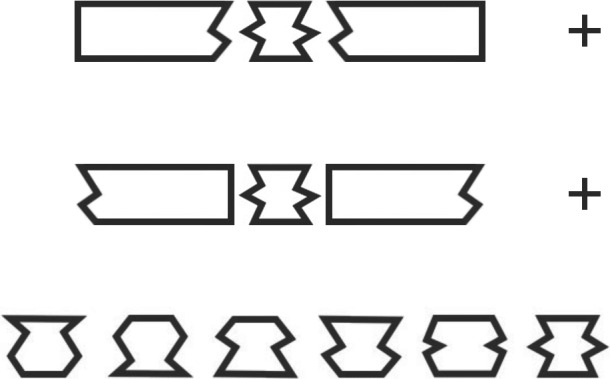
Crowding and flipping demo. The Bouma law mk 2 says that, for any given location and direction in the visual field, critical spacing, measured between the centers of the target and the flankers, is conserved. Thus, crowding should be unaffected by any change that does not affect center-to-center spacing, such as flipping an object about its center. Here we present a demo that appears to contradict this law. In each test row, we will ask you to identify a target. The bottom row shows you all six possible targets. In the first and second rows, while fixating the plus sign on the right, try to identify the target (the middle object). Identifying is hard when the jagged (feature-rich) edge of the flanker is near to the target (first row) and easier when it is far (second row) even though center-to-center spacing (and the set of features) is unchanged. This breaks the Bouma law mk 2. Crowding seems to depend specifically on the distance of the jagged edge of the flanker, not the distance of the flanker itself, from the target.

The demo in Figure 2 shows that lopsided flankers break the Bouma law mk 2: Critical spacing is not conserved. The problem is obvious. The flanker is nearly innocuous except for its jagged edge. Crowding seems to depend specifically on the distance of the jagged edge of the flanker, not the distance of the flanker itself, from the target. Our three experiments parametrically investigate several flanker properties that affect crowding drastically: (a) length, (b) size, and (c) orientation. We first present the whole story quickly, with demonstrations, in the Summary. Then each experiment is presented with its own Method and Results. Finally, we close with a General discussion and Conclusion, in which we show how to modify the Bouma law based on the results of our experiments to cope with complex objects in general and lopsided flankers in particular.

Summary with demos

The critical spacing of crowding is particularly well suited to informal testing with paper and pencil. Simple demos allow us to explore a wide range of ideas quickly. Here we explore how critical spacing is affected by length, size, and orientation of the flankers. We present the demos first to help the reader understand the experimental results.

Critical spacing varies among people. Our demos work for most people, but if you are particularly susceptible or particularly resistant to crowding, the demos might be too hard or too easy. You can compensate for this by fixating left or right of the fixation mark, to fixate a little closer to or farther from the target.

We measure spacing in three ways:

• Center spacing—center-to-center spacing (Figure 3). The center of each object is the center of its bounding box; its x center is the average of its two most extreme x coordinates, and its y center is the average of its two most extreme y coordinates.

• Edge spacing—edge-to-edge spacing (Figure 3). Edge spacing is the minimum distance between extremes of the target and flanker along the line from center of target to center of flanker.

• Centroid spacing is defined in General methods.

Figure 3.

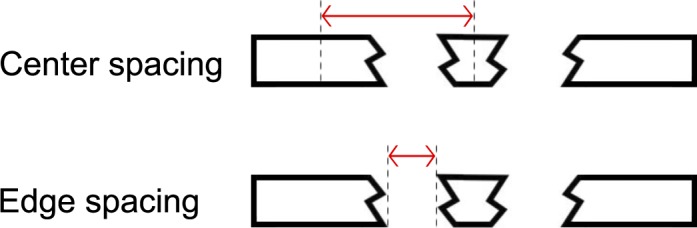
Center and edge measurements. Center spacing is the distance from the center of the target to the center of the flanker. The x-axis, the axis of crowding, is the axis running through the center of the target and flanker. It is horizontal in this diagram. The x-center of the flanker is the average of its two most extreme x-coordinates; y is orthogonal to the axis of crowding and is vertical in this diagram. The y-center is the average of its two most extreme y-coordinates. Edge spacing is the minimum x-difference (i.e., along the axis of crowding) between extremes of the target and flanker.

In the length experiment (Experiment 1), we lengthen the flankers to discover which of our measures most accurately predicts crowding. Supposing that the flanker's features correspond to its corners, lengthening merely displaces the corner features. Consider a flanker with a jagged edge near the target. If we fix the center of the flanker, then lengthening the flanker will move the jagged edge toward the target (Figure 4, rows 1 and 2). Thus, this manipulation decreases edge spacing without affecting center spacing. If, instead, we fix the edge of the flanker while lengthening, then the location of the center of the flanker will move away from the target (Figure 4, rows 1 and 3). Thus, this manipulation increases center spacing without affecting edge spacing. In general, lengthening the flanker affects the two measures of spacing differently, providing a test for which critical spacing, center or edge, is better conserved. A conserved spacing measure has the same critical value across all objects. The length experiment measures critical spacing as a function of flanker length. Our demo in Figure 4 suggests that across variations in length, critical edge spacing is conserved for lopsided rectangles.

Figure 4.

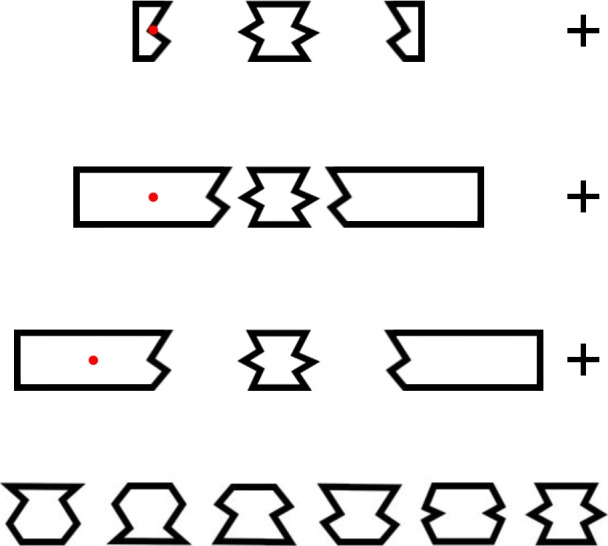
Length demo (Experiment 1). When we lengthen the flanker, how might we change its position so as to preserve its crowding of the target? A red dot marks the center of the left flanker. In each row, fixate the plus sign and try to identify the middle object. The set of possible targets is shown in the bottom row. In the first row, you can identify the target. In the second row, the center spacing is the same as in the first row, but it is very hard, if not impossible, to identify the target. In the third row, the edge spacing is the same as in the first row, and it is just as easy to identify the target as in the first row. For these lopsided rectangles, equal edge spacing preserves crowding.

Our stimuli differ from traditional crowding stimuli in that they have one edge that is feature-rich and another that is feature-poor. Much of the past work on crowding used letters. To link new and old, in the size experiment (Experiment 2) we put them head to head, comparing crowding of lopsided rectangles and letters. Lengthening letters would distort their shapes and make them unfamiliar, so we just make them bigger. In the size experiment, we vary flanker size, scaling the whole flanker proportionally instead of just its length. We test both lopsided rectangles and letters. Our demos in Figures 5 and 6 suggest that across variations in size, critical edge spacing is conserved for lopsided rectangles (Figure 5), and critical center spacing is conserved for letters (Figure 6).

Figure 5.

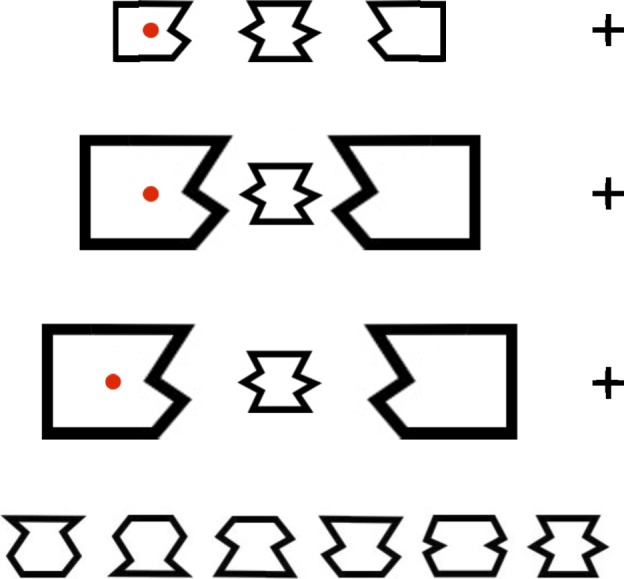
Size demo (Experiment 2). A red dot marks the center of the left flanker. In each row, fixate the plus sign and try to identify the middle object from the set of possible targets shown in the bottom row. In the first row, you can identify the target. In the second row, the center spacing is the same as in the first row, but it is very hard, if not impossible, to identify the target. In the third row, the edge spacing is the same as in the first row, and it is just as easy to identify the target as in the first row. For lopsided rectangles, equal edge spacing preserves crowding.

Figure 6.

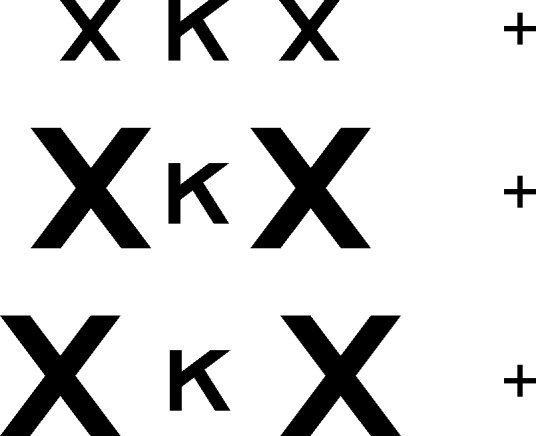
Size demo with letters (Experiment 2). In each row, fixate the plus sign and try to identify the middle letter. In the first row, it is hard to identify the target. In the second row, the center spacing is the same as in the first row, and again, the target is barely identifiable. This spacing is sufficient to barely identify the target. In the third row, the edge spacing is the same as in the first row, but the target is now easy to identify. For letters, equal center spacing preserves crowding.

Summarizing the demonstrations, critical center spacing is conserved for letters but not for the lopsided rectangles. In that case, only critical edge spacing is conserved. Can we use rotation to make the lopsided rectangles act like letters? If we rotate a lopsided flanker 90°, then the flanker will no longer be lopsided with respect to the target. Because the asymmetry is gone, critical center spacing might now be conserved.

In the orientation experiment (Experiment 3), we rotate the target and flankers by 90° so that the jagged edges that were formerly adjacent are now roughly collinear (Figure 7). Our demo in Figure 8 suggests that after rotation, critical center spacing is conserved for lopsided rectangles, just like letters. Table 1 summarizes our predictions of conservation based on the demos.

Figure 7.

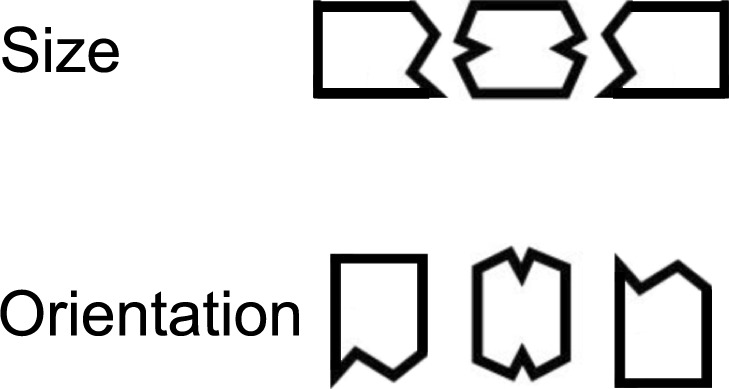
Rotated lopsided rectangles. The first row displays a typical stimulus from the size experiment. In the second row, all the objects have been rotated clockwise by 90°. Such rotated objects are used in the orientation experiment. We predict that this rotation will cause the lopsided rectangles to act like letters: Critical center spacing will be conserved.

Figure 8.

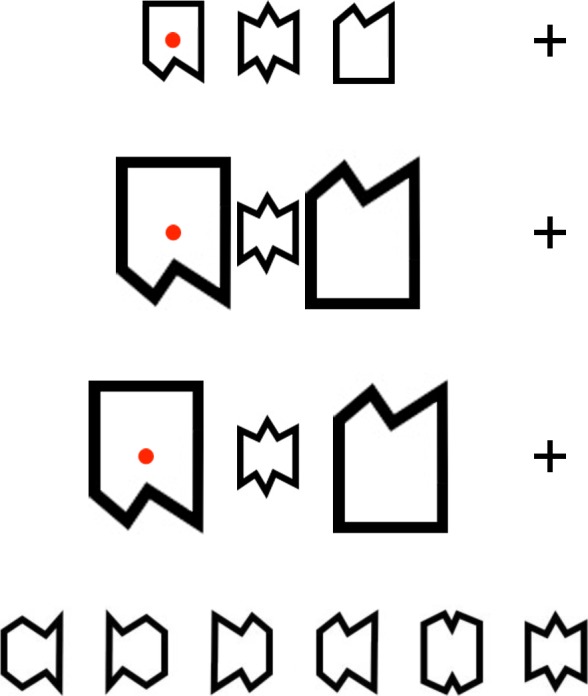
Orientation demo (Experiment 3). A red dot marks the center of each left flanker. In each row, fixate the plus sign and try to identify the middle object from the set of possible targets shown in the bottom row. In the first row, it is hard to identify the target. In the second row, the center spacing is the same as in the first row, and again, the target is barely identifiable. This spacing is sufficient to barely identify the target. In the third row, the edge spacing is the same as in the first row, but the target is now easy to identify. For rotated lopsided rectangles, equal center spacing preserves crowding, the same as for letters.

Table 1.

Predictions of conservation. Notes: Based on the demos, the table predicts the experimental outcomes, noting whether the flanker features are symmetrical or not and which critical spacing is conserved across manipulations. Symmetry is assessed along the axis of crowding.

Experiment	Flanker	Symmetrical distribution of features?	Conserved critical spacing
1: Length	Lopsided	No	Edge
2: Size	Lopsided	No	Edge
2: Size	Letter	Yes	Center
3: Orientation	Rotated lopsided	Yes	Center

General methods

We create flankers that consist of a rectangle with one jagged edge. We suppose that most of the features lie on the jagged edge, so that the flanker's feature distribution is lopsided. Unlike the lopsided flanker, the target rectangle is symmetric with two jagged edges at opposite ends, each a mirror image of the other. Examples of such targets and flankers can be seen in Figure 4.

Observers identify a peripheral target object between two flankers. Across conditions, we test various target–flanker spacings. We generate a psychometric function by plotting the observer's proportion of correct identifications of the target as a function of target–flanker separation. We summarize each psychometric function by the observer's threshold spacing, the minimum target–flanker distance needed for 65% target identification. This is the critical spacing.

According to the Bouma law mk 2, critical spacing is independent of object type, provided the target and flankers are similar (Pelli & Tillman, 2008). If critical spacing is conserved, then the psychometric function should be independent of the type of flanker. Using the same trial data, we create psychometric functions for several spacing measures in order to determine which type of critical spacing is conserved:

• Center spacing is defined in the Summary.

• Centroid spacing—centroid-to-centroid spacing. Intuitively, one might want to estimate the average location of all the object's features. Thus, we define each object's “centroid” as the average position of all its corners.

• Edge spacing is defined in the Summary.

Observers

Three experienced observers (two male, one female), including the first and second authors, aged 22–33 years with normal or corrected-to-normal vision participated in the experiments. Observers gave written informed consent in accordance with the procedures and protocols approved by the human observers review committee of New York University.

Stimuli

Stimuli are generated using MATLAB with the Psychtoolbox extensions (Brainard, 1997; Pelli, 1997) running on an Apple G4 Macintosh computer and presented on an 18-in. CRT monitor with a resolution of 1024 × 768 pixels and a frame rate of 100 Hz. The display is placed 57 cm from the observer, whose head is stabilized with a chin and forehead rest. At this distance, there are 29 pixels/deg.

We design six flanker objects, each consisting of a rectangle with one jagged edge. We suppose that most of the features lie on the jagged edge so that the flanker's feature distribution is lopsided. We also design six target objects, each a rectangle with two matching jagged edges on parallel sides. The target is symmetric. The two jagged edges are reflections of each other. The jagged edges of the targets are drawn from the same set as those used for the flankers.

In separate conditions, the experiment is performed in the lower and in the left visual fields. Within each of these visual fields, in separate blocks, stimuli are tested in each configuration, radial and circumferential (i.e., in each location, stimuli are tested in each configuration, horizontal and vertical). Relative to the horizontal configuation, in the vertical configuration, the entire stimulus is rotated 90 deg about fixation so that all spacings along the axis of crowding are preserved. However, letters are always presented upright. We perform the test in two different visual fields and two different target–flanker arrangements because crowding is known to display asymmetries. For example, critical spacing is greater in the radial direction than in the tangential direction (Petrov & Popple, 2007; Toet & Levi, 1992). As noted above, the Bouma law predicts that critical spacing is independent of object type, provided the target and flanker are similar. Hence, changing the location of features within a flanker without changing the target–flanker center-to-center spacing should leave crowding intact. This should hold true for all areas of the visual field and in all configurations.

The target object, in the middle, is flanked by two flankers, each a mirror image of the other, presented on a uniform gray background with luminance 37.4 cd/m². The center of the target object is 12° from the center of the black fixation square (0.5° × 0.5°).

Procedure

For each test location (radial and circumferential orientation in both the lower and left visual fields), trials are divided into multiple conditions, one for each flanker length or size. Each condition is tested with seven spacings. Each physical spacing can be expressed in three ways: center, centroid, and edge spacing. We present proportion correct as a function of all three spacing measures. Each condition is tested in a separate block of 40 trials. All blocks are run twice. The block order is randomly determined for each observer. Each block contains 40 trials of each of the seven spacings for a total of 280 trials. Within each block, the trial order is randomized. Each block begins with the press of the space bar. A black 0.5° × 0.5° fixation square is presented at the center of the screen for the entire duration of the block. In each trial, the target and flanker objects are presented for 150 ms in the same field and orientation. The target and flanker objects are chosen randomly for each trial. After stimulus presentation, the screen displays the set of all six possible targets, and the observer has unlimited time to identify the target with a mouse click (chance = 0.167). The next trial is presented after an intertrial interval of 1 s.

Results

For each condition, proportion correct is plotted as a function of spacing. The same data are plotted against each of the three measures of spacing. The data are then fit with an inverse cumulative normal distribution function, otherwise known as a probit function. To summarize the data, we find the critical spacing (65% threshold) for each observer for each kind of flanker and plot this as a function of flanker length or size. Results are consistent across all observers and across both visual fields and orientations.

In a given experiment, we ask which spacing measure (center, centroid, or edge) is most predictive of crowding across flanker length or size. When comparing critical spacing across flanker lengths or sizes in the summary plot, the conserved measure should produce a slope of zero. Threshold spacing should be the same for all flanker conditions.

Experiment 1: Length

To determine which of the three measures most accurately predicts crowding, we lengthen the flankers (Figure 4). Lengthening the flanker affects the measures of spacing differently, providing a test for which critical spacing—center, centroid, or edge—is better conserved. If critical spacing is conserved, unaffected by lengthening flankers, then we expect the psychometric function (performance vs. spacing) to be conserved as well.

Methods

In the horizontal configuration, all targets are 1.5° high and 2° wide. All flankers are 1.5° high. We use three flanker lengths: 1°, 2°, and 4° (flanker lengths of 1° and 4° are shown in Figure 4). Flankers are presented so that the jagged edge is nearest the target. For each flanker length, we test seven different target–flanker spacings. For each spacing, we plot performance as a function of center, centroid, and edge spacing. The spacings are listed in Table 2. Note that each spacing measure (center, centroid, and edge) is a transformation of the other measures. For example, in the horizontal configuration, center spacing = edge spacing + ½ target width + ½ flanker width.

Table 2.

Spacings tested. Notes: Performance is measured for each flanker length (1°, 2°, and 4°) and position (radial lower visual field, circumferential lower visual field, radial left visual field, circumferential left visual field). We test seven different target–flanker spacings. For each condition, spacing is calculated in three ways (center spacing, centroid spacing, and edge spacing). Performance is analyzed as a function of each kind of spacing.

Flanker length	Spacing
1°	Center	1.57°	2.02°	2.53°	3.57°	4.60°	5.64°	6.67°
2°		2.07°	2.52°	3.03°	4.07°	5.10°	6.14°	7.17°
4°		3.07°	3.52°	4.03°	5.07°	6.10°	7.14°	8.17°
1°	Centroid	1.49°	1.94°	2.45°	3.49°	4.52°	5.56°	6.59°
2°		1.83°	2.28°	2.79°	3.83°	4.86°	5.90°	6.93°
4°		2.45°	2.90°	3.41°	4.45°	5.48°	6.52°	7.55°
1°	Edge	0.07°	0.52°	1.03°	2.07°	3.10°	4.14°	5.17°
2°		0.07°	0.52°	1.03°	2.07°	3.10°	4.14°	5.17°
4°		0.07°	0.52°	1.03°	2.07°	3.10°	4.14°	5.17°

Results

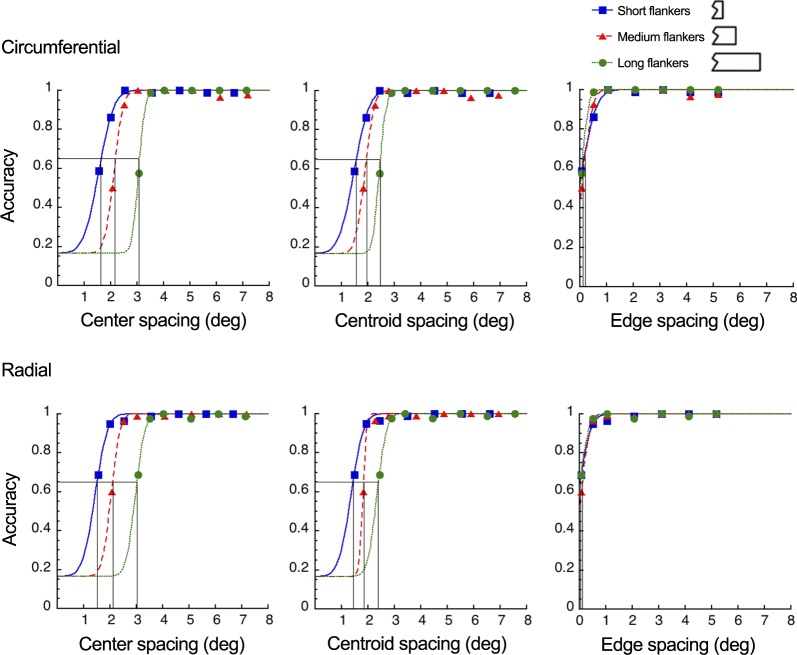
The plots for observer NB, who was naive as to the purpose of the experiment, are shown in Figure 9. The psychometric curves superimpose only in the edge-spacing graphs, indicating that only critical edge spacing is conserved. Summary plots are shown in Figure 10. The slope is zero only for edge spacing (Table 3). Thus, edge spacing is more predictive of crowding than center or centroid spacing.

Figure 9.

Experiment 1: Length. The results for observer NB in the left visual field are shown above. Each graph plots three psychometric functions, one for each flanker length: 1°, 2°, and 4° (corresponding to short, medium, and long flankers). The horizontal and vertical black lines mark the 65% threshold for each condition. In both radial and circumferential configurations, only critical edge spacing is conserved.

Figure 10.

Experiment 1: Length. Only critical edge spacing (blue) is conserved. 65% thresholds are estimated from each psychometric function. Thresholds for center, centroid, and edge spacing (red, green, and blue, respectively) are compared. Thresholds are consistent across flanker lengths only when measured in terms of edge spacing. This is true in both visual fields for both the circumferential and radial configurations. Error bars indicate plus or minus one standard error about the mean (three observers). The fits are linear regressions.

Table 3.

Experiment 1: Length. Conservation of critical spacing. Notes: Each slope is the average across circumferential and radial arrangement in lower and left visual fields. Conservation predicts zero slope. Instances of conservation (yes) are emphasized by italics.

Critical spacing	Slope (mean ± SE)	Conserved?
Center	0.47 ± 0.06	no
Centroid	0.27 ± 0.04	no
Edge	0.02 ± 0.05	yes

A one-way repeated-measures ANOVA shows that critical spacing differs significantly across flanker lengths when measured in terms of center or centroid spacing. For center spacing: left visual field circumferential, F(2, 4) = 54.64, p < 0.001; left visual field radial, F(2, 4) = 300.5, p < 0.001; lower visual field circumferential, F(2, 4) = 274.75, p < 0.001; lower visual field radial, F(2, 4) = 390.25, p < 0.001. For centroid spacing: left visual field circumferential, F(2, 4) = 22.53, p < 0.01; left visual field radial, F(2, 4) = 134.13, p < 0.001; lower visual field circumferential, F(2, 4) = 88.41, p < 0.001; lower visual field radial, F(2, 4) = 178.06, p < 0.001. Pair-wise t tests with Bonferroni corrections confirm these findings.

Discussion

This experiment bears out what was apparent in the Figure 4 demo. When the distribution of features in the flanker is lopsided, crowding depends solely on the distance of the clump of features, regardless of where the center of the flanker is. Center spacing is a poor predictor of the crowding produced by our lopsided flankers.

Critical center spacing has been used to characterize crowding for 40 years without uncovering this problem. However, most past experiments used simple objects, especially Gabors and letters, which presumably have a fairly uniform distribution of features within the object. Although some letters, such as the letter “P” are not symmetrical, these studies either used individual symmetrical letters, such as a “T,” or averaged across all letters.

Similarity is known to affect crowding (Kennedy & Whitaker, 2010; Kooi, Toet, Tripathy, & Levi, 1994; Nazir, 1992; Põder, 2007). Lengthening the flanker may affect target–flanker similarity somewhat, but it seems unlikely that these changes in similarity affected crowding here. The target is the same size as the medium-length flanker. If length-dependent similarity matters here, then we would expect the medium-length flankers, which are the most similar to the target, to crowd the target most. Instead, the longest flankers crowd the target most. Thus, the effect of length on crowding does not seem to be mediated by changes in similarity. We confirm this again in Experiment 2, which scales the flankers rather than stretching them.

Experiment 2: Size

As noted in the Introduction, one way in which our stimuli differ from traditional crowding stimuli is that they have one edge that is feature-rich and another that is feature-poor. Previous work on crowding has often relied on symmetrical or quasisymmetrical stimuli, such as letters. To compare our results with previous results, this size experiment puts them head to head, comparing asymmetrical lopsided rectangles with symmetrical letters.

In the length experiment (Experiment 1), we stretch the flankers to manipulate edge spacing while keeping the center spacing the same. We do not want to stretch the letters, as that would distort their shapes and make them unfamiliar. Instead, we make them bigger. Previous studies have found that if objects are scaled, then critical center spacing is conserved (Pelli et al., 2004; Strasburger et al., 1991; Tripathy & Cavanagh, 2002). What happens when our lopsided flankers grow in size? Is critical spacing conserved? Which critical spacing? We use the same procedure as in the length experiment except that we vary flanker size, scaling the whole flanker proportionally instead of just its length.

Methods

In addition to lopsided rectangles, we also test letters (displayed in the Sloan font, see Figure 6). We manipulate the size of the flanker rather than just its length. To determine target and flanker size, we measure acuity of both lopsided rectangles and letters at 12° eccentricity in both the left and lower visual field. We use QUEST to determine threshold acuity (82% accuracy for each condition) (Watson & Pelli, 1983). We test four blocks of 40 trials for each location in the visual field. Averaging across observers for our lopsided rectangles, the measured acuity is 0.62° ± 0.04° for the left visual field and 0.74° ± 0.03° for the lower visual field. For letters, the measured acuity is 0.28° ± 0.03° for the left visual field and 0.33° ± 0.03° for the lower visual field.

Targets are 1.25 times acuity, and flankers are either 1.25 times acuity (small flanker condition) or 2.5 times acuity (large flanker condition). The tested edge spacings are 0.07°, 0.52°, 1.03°, 2.07°, 3.10°, 4.14°, and 5.17°. The corresponding center and centroid spacings depend on flanker size (which varies for each observer as it is based on acuity). These various spacings are interleaved for a total of 280 trials per block (40 for each spacing). For letters, centroid spacing is practically the same as center spacing, so it is not calculated.

Results

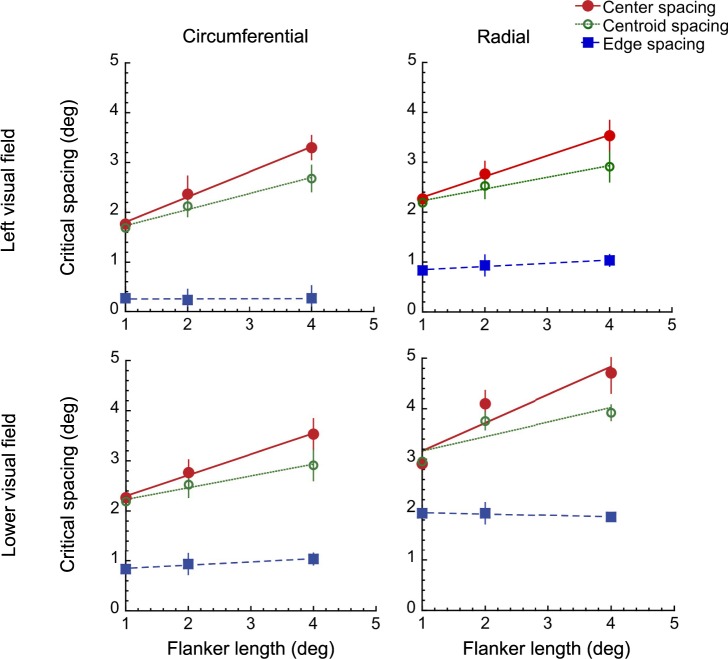
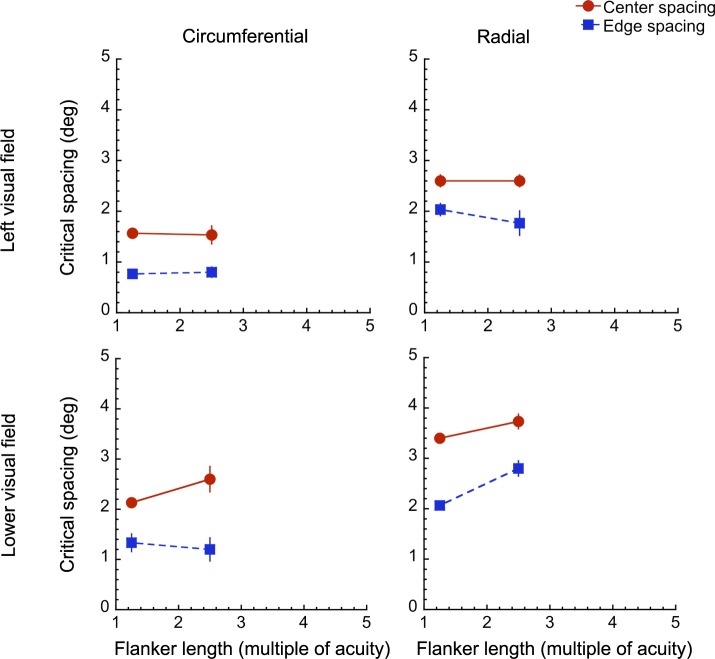
Summary plots for lopsided rectangles are shown in Figure 11. The slope is zero only for edge spacing (Table 4). For lopsided rectangles, edge spacing is more predictive of crowding than center or centroid spacing.

Figure 11.

Experiment 2: Size, jagged rectangles. Critical edge spacing (blue) is conserved. 65% thresholds are estimated from each observer's psychometric functions. Thresholds for center, edge, and centroid spacing (red, blue, and green) are compared. Thresholds are only consistent when measured in terms of edge spacing in both visual fields for both the circumferential and radial configurations. Error bars indicate plus or minus one standard error about the mean (three observers). Linear fits are shown.

Table 4.

Experiment 2: Size, jagged rectangles. Conservation of critical spacing. Notes: Each slope is the average across circumferential and radial arrangement in lower and left visual fields. Conservation predicts zero slope. Instances of conservation (yes) are emphasized by italics.

Critical spacing	Slope	Conserved?
Center	0.61 ± 0.09	no
Centroid	0.41 ± 0.09	no
Edge	−0.02 ± 0.03	yes

Thresholds differ significantly across the two flanker size conditions when measured in terms of both center spacing and centroid spacing in both visual fields for both the circumferential configuration, center: left visual field, t(2) = −19.00, p < 0.005; lower visual field, t(2) = −15.59, p < 0.005; centroid: left visual field, t(2) = −11.76, p < 0.01; lower visual field, t(2) = −11.41, p < 0.05, and the radial configuration, center: left visual field, t(2) = −11.00, p < 0.05; lower visual field, t(2) = −8.69, p < 0.05; centroid: left visual field, t(2) = −7.38, p < 0.05; lower visual field, t(2) = 5.96, p < 0.05.

Summary plots for letters are shown in Figure 12. The slope is zero for center and edge spacing (Table 5). For letters, centroid spacing (not shown) is practically the same as center spacing and is not calculated. Edge spacing thresholds differ significantly in the radial configuration in the lower visual field, t(2) = −8.32, p = 0.05, making edge spacing less conserved than center spacing (bottom right plot in Figure 12).

Figure 12.

Experiment 2: Size, letters. Both critical spacings are mostly conserved (red and blue). 65% thresholds are estimated from each observer's psychometric functions. Thresholds for center spacing (red) and edge spacing (blue) are compared for conditions with small and large flankers. Targets are always small. Centroid spacing (not shown) is practically the same as center spacing. Thresholds do not differ significantly when measured in terms of center spacing. Error bars indicate plus or minus one standard error about the mean (three observers). Linear fits are shown.

Table 5.

Experiment 2: Size, letters. Conservation of critical spacing. Notes: Each slope is the average across circumferential and radial arrangement in lower and left visual fields. Conservation predicts zero slope. Instances of conservation (yes) are emphasized by italics.

Critical spacing	Slope	Conserved?
Center	0.15 ± 0.18	yes
Edge	0.20 ± 0.30	yes

Discussion

For lopsided rectangles, only critical edge spacing is conserved across size whereas for letters it is the critical center spacing that is better conserved. The results for edge and center spacing for letters are quite similar. Our stimuli are presented at 1.25 and 2.5 times acuity. Across observers and stimuli, the average acuity for letters (at 12° eccentricity, where the target is always presented) is 0.31°, so, on average, the target size is 0.39° and the flanker size is 0.39° or 0.78°. The critical center spacing (roughly 2°) is much larger than the 0.39° object size. Because the object size is only a small fraction of the center spacing, there is only a small difference between center and edge spacings (on average, 0.2°). Thus, it is not surprising that we find little difference between edge and center spacing for letters. At much larger letter sizes, we predict that only center spacing is conserved. This is demonstrated in Figure 6.

Experiment 3: Orientation

Summarizing our demos and data, critical center (not edge) spacing is conserved for many simple objects, including letters and Gabors, but not for our lopsided rectangles. In that case, only critical edge spacing is conserved. These findings show that the location of features within the flanker can affect crowding.

If the location of features matters, then rotating the lopsided rectangles might change our results. Will a 90° rotation make the lopsided rectangles act like letters? If we rotate a lopsided flanker 90°, then the flanker will no longer be lopsided along the axis of crowding. Because the asymmetry is gone, critical center spacing may now be conserved.

In this experiment, we rotate the target and flankers by 90° so that the jagged edges that were formerly adjacent are now roughly collinear (Figure 8). Centroid spacing is practically the same as center spacing, so it is not measured. We use the same method as in the size experiment. Our hypothesis predicts that this rotation should reverse our earlier results so that critical center spacing, rather than critical edge spacing, is conserved.

Methods

We rotate the target and flankers by 90° so that the jagged edges that were formerly adjacent are now roughly collinear (Figure 8). Centroid spacing is practically the same as center spacing, so it is not calculated. We measure acuity as in the size experiment. Averaging across observers, for our rotated lopsided rectangles, the measured acuity is 0.64° ± 0.03° for the left visual field and 0.87° ± 0.01° for the lower visual field. Targets are 1.25 times acuity, and flankers are either 1.25 times acuity (small flanker condition) or 2.5 times acuity (large flanker condition).

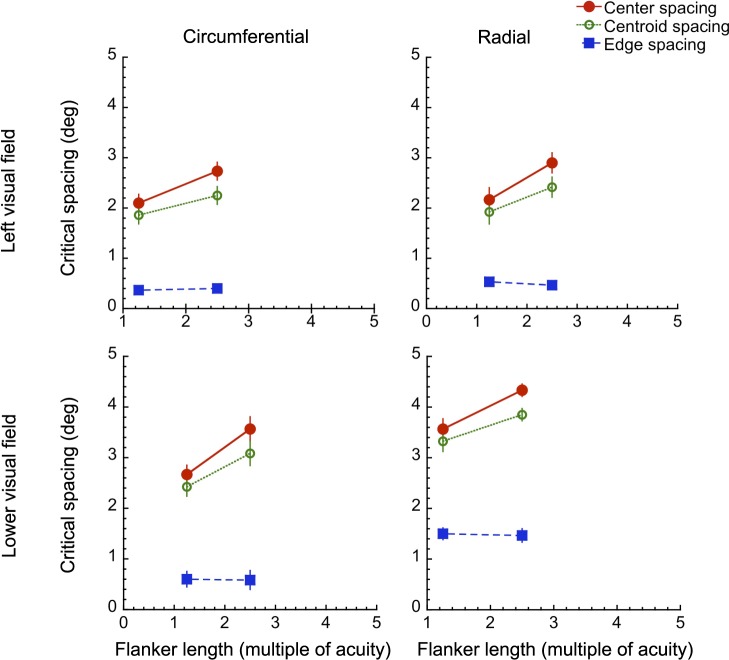
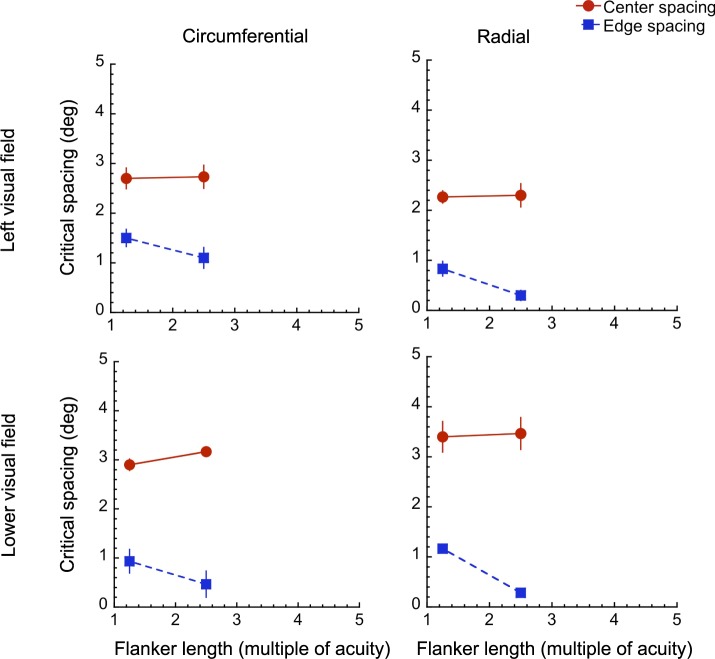
Results

Summary plots for rotated lopsided rectangles are shown in Figure 13. The slope is zero only for center spacing (Table 6). For rotated lopsided rectangles, center spacing is more predictive of crowding than center or spacing.

Figure 13.

Experiment 3: Orientation. Conservation of critical spacing (red). 65% thresholds are estimated from each observer's psychometric functions. Thresholds for center spacing (red) and edge spacing (blue) are compared for conditions with small and large flankers. Targets are always small. Thresholds do not differ significantly when measured in terms of center distances in both visual fields for both the circumferential configuration and the radial configuration. Error bars indicate plus or minus one standard error about the mean (three observers). Linear fits are shown.

Table 6.

Experiment 3: Orientation. Conservation of critical spacing. Notes: Each slope is the average across circumferential and radial arrangement in lower and left visual fields. Conservation predicts zero slope. Instances of conservation (yes) are emphasized by italics.

Critical spacing	Slope	Conserved?
Center	0.08 ± 0.09	yes
Edge	−0.46 ± 0.17	no

Thresholds differ significantly when measured in terms of edge spacing in both visual fields for both the circumferential configuration, left visual field, t(2) = 6.93, p < 0.05; lower visual field, t(2) = 7, p < 0.05; and the radial configuration, left visual field, t(2) = 8, p < 0.05; lower visual field, t(2) = 10.60, p < 0.01.

Discussion

While only critical edge spacing is conserved for lopsided rectangles in Experiments 1 and 2, Experiment 3 shows that after rotating each rectangle by 90° only critical center spacing is conserved. Because the target and flankers underwent the same 90° rotation, this manipulation does not affect their similarity to one another. Rotation changes only the location of features within the flanker, which shows that location of the features within the flanker can affect crowding of the target.

General discussion

Critical spacing of crowding is conserved across many manipulations when measured from the center of one object to the center of another object (see Pelli & Tillman, 2008). However, here we report a case, using lopsided flankers, in which critical center spacing is not conserved, and instead, critical edge spacing is. Rotating each object by 90° makes the flankers symmetric and dramatically affects crowding. The orientation of these targets and flankers determines whether critical edge or critical center spacing is conserved across changes in size. This suggests that the visual system's ability to isolate and combine features depends on the locations of features in the objects, not just the locations of the objects.

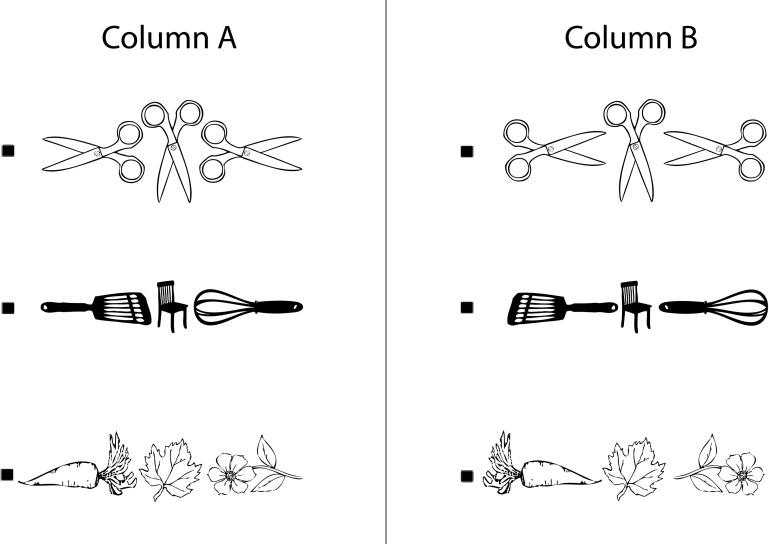
Figure 14 demonstrates crowding that neither edge spacing nor center spacing can predict. Flipping the flankers horizontally maintains both center and edge spacing yet turns crowding on and off. This breaks the Bouma law mk 2, which declares that critical spacing of simple similar objects depends only on target eccentricity and direction of the flanker, independent of the kind of object (Pelli & Tillman, 2008).

Figure 14.

Familiar objects. Fixating on the black square, you will be unable to identify the middle object in each row of column A, but it's easy in column B even though center and edge spacing are unchanged. Column B was created by flipping the flankers in column A. This moves the target-similar part of the flanker away from the target. (Images of objects in rows 1 and 3 are from Snodgrass & Vanderwart, 1980.)

The Bouma law mk 2 provides a very concise summary of what is becoming a large literature on crowding. Can we tweak the law to extend its embrace to include our new findings with lopsided flankers?

As previously mentioned, crowding studies usually use simple objects, such as roman letters and Gabors. An isolated simple object is not crowded, but complex objects suffer from “self-crowding” (Martelli, Majaj, & Pelli, 2005). To identify a complex object (such as a word or a face), the parts (such as letters or facial features) must have the same critical spacing as simple objects. The Bouma law mk 2 does not account for self-crowding.

Thus we propose revising the law to

Bouma law of crowding mk 3: Objects may have parts that are identified independently and contribute independently to crowding. For a target part that can be identified in isolation, our ability to identify it among similar parts depends solely on the ratio of the part spacing (measured center to center) to the observer's critical spacing at that location and direction. The part is crowded whenever the ratio is less than one. For each observer, the critical spacing is conserved, independent of what the part is, depending only on where the part is in the visual field and the direction to the nearest similar part.

Note that mk 3 of the law includes mk 2 as a special case when applied to simple one-part objects, such as roman letters and Gabors.

In light of the new law, the most parsimonious account for our new experimental findings is that our lopsided flanker is complex, having multiple parts. One part is the feature-rich edge; the rest of the object (containing one or more parts) is not similar to the target and is therefore irrelevant for crowding of that particular target.

All of our results show conservation of critical spacing of parts. In the length and size experiments, when jagged edges are facing each other, we find that only edge spacing is conserved for lopsided rectangles. Because edge spacing is measured from one jagged edge to the other, it is measured between parts. Note that we measured edge spacing from the extreme of the jagged edge, not its center. However, because the jagged edge is quite narrow (less than 25% of the object length), its center is very close to its extreme. In the size experiment, we also measure critical spacing for letters. We find that center spacing is conserved for letters. Because letters are simple objects with only one part (Martelli et al., 2005), center spacing for letters is measured between parts. Finally in the orientation experiment, once we rotate our lopsided rectangles to be symmetric and no longer lopsided with respect to the target, we find that center spacing is conserved. After rotation, the jagged edges of the target and flanker are in line with each other. The objects and their jagged edges now have the same center-to-center spacing.

Conclusion

Even though crowding is operationally defined by failure to identify an object, we find that the mechanism of crowding is not about objects. It's about parts. Critical spacing of similar parts is conserved.