Simulation studies of circular muscle contraction, longitudinal muscle shortening, and their coordination in esophageal transport

Abstract

On the basis of a fully coupled active musculomechanical model for esophageal transport, we aimed to find the roles of circular muscle (CM) contraction and longitudinal muscle (LM) shortening in esophageal transport, and the influence of their coordination. Two groups of studies were conducted using a computational model. In the first group, bolus transport with only CM contraction, only LM shortening, or both was simulated. Overall features and detailed information on pressure and the cross-sectional area (CSA) of mucosal and the two muscle layers were analyzed. In the second group, bolus transport with varying delay in CM contraction or LM shortening was simulated. The effect of delay on esophageal transport was studied. For cases showing abnormal transport, pressure and CSA were further analyzed. CM contraction by itself was sufficient to transport bolus, but LM shortening by itself was not. CM contraction decreased the CSA and the radius of the muscle layer locally, but LM shortening increased the CSA. Synchronized CM contraction and LM shortening led to overlapping of muscle CSA and pressure peaks. Advancing LM shortening adversely influenced bolus transport, whereas lagging LM shortening was irrelevant to bolus transport. In conclusion, CM contraction generates high squeezing pressure, which plays a primary role in esophageal transport. LM shortening increases muscle CSA, which helps to strengthen CM contraction. Advancing LM shortening decreases esophageal distensibility in the bolus region. Lagging LM shortening no longer helps esophageal transport. Synchronized CM contraction and LM shortening seems to be most effective for esophageal transport.

the esophagus is a muscular conduit that transports food bolus aborally. In the supine position, bolus transport, also called esophageal transport here, originates from neurally controlled muscle activation. Experiments have shown that muscle activation during esophageal transport involves sequential activation and inhibition of two types of muscles: the inner circular muscle (CM) contraction and outer longitudinal muscle (LM) shortening, called CM contraction and LM shortening, respectively (4, 19, 23). Several questions arise: What are the roles of CM contraction and LM shortening in esophageal transport? Are both necessary? How does each influence esophageal transport? These questions have only been partially addressed in the literature. CM contraction has been extensively studied with manometric measurements of luminal pressure (14, 15). It is generally believed that the role of CM contraction is to generate a squeezing pressure to close the lumen and propel the bolus (14, 15). The study of LM shortening involves two approaches: 1) to place radiopaque clips on the mucosal surface of the esophagus to quantify the trajectories of material points on the luminal surface (4, 11, 23), or 2) to measure the change of muscle cross-sectional area (CSA) from ultrasound images (19, 21) and deduce the local shortening based on mass conservation. The role of LM is relatively unclear. On the basis of mass conservation, Nicosia et al. (21) proposed that LM shortening, by concentrating circular muscle fibers, can reduce the tension per fiber to maintain the required closure pressure. Pal and Brasseur (22) proposed that another potential benefit of LM shortening was to reduce the required luminal pressure. They argued that the LM shortening caused axial motion of mucosa and, based on a mechanical model, axial motion of mucosal surface would reduce the required luminal closure pressure.

We hypothesize that CM contraction plays a primary role in bolus transport whereas LM shortening is secondary and may help strengthen the contracted CM layer. We also hypothesize that CM contraction by itself will lead to a change of axial length and local CSA. This implies that the geometry change measured in experiments is a combination of the influence of both LM shortening and CM contraction, and quantifying the former should involve subtraction of the latter from experimental measurements. In the first part of this work, we will address the above questions and test our hypotheses. We will also discuss our findings in relation to current opinions.

The other issue addressed in this work is related to the effect of coordination, or synchronization, between CM contraction and LM shortening, on esophageal transport. Clinical studies (19, 21, 23) have shown that LM and CM activations are in near perfect synchronization in normal subjects. This is indicated by a spatiotemporal overlap between luminal pressure and muscle CSA peaks. However, in some patients discoordination was observed (17). Jung et al. (8) found that patients with a nutcracker esophagus show LM shortening preceding CM contraction. This suggests that discoordination might play a role in the genesis of esophageal motility disorders (17, 18). Here, we hypothesize that the coordination between CM contraction and LM shortening is important in esophageal transport, and discoordination could cause abnormal transport. This hypothesis will be tested in the second part of this work by case studies in which CM contraction or LM shortening is delayed. The effect on bolus transport is presented and a mechanics-based explanation is given. All studies were conducted by a fully resolved computational model we have developed and explained below.

MATERIALS AND METHODS

Model Components

We have developed a fully resolved computational model of esophageal transport. The model integrates three essential aspects (liquidlike bolus, esophageal wall, and neural-controlled muscle activation) into a single simulation and is therefore capable of fully resolving interactions among the three. Here we summarize only the essential information relevant to the problem of esophageal transport. For other technical details, please refer to Ref. 13.

Since peristalsis was the main focus, only the section of the esophagus between upper esophageal sphincter and lower esophageal sphincter, excluding the sphincters, was modeled. The modeled esophagus was considered to be a multilayered deformable tube, with its top end anchored in place, and its bottom end free to move. Initially, the proximal esophageal section was distended by a swallowed liquid bolus. Distal to the bolus, the esophageal wall was at rest with a thin liquid film lining the lumen. The overall setup is illustrated in Fig. 1A. The total length of the modeled esophagus was 18 cm, which is consistent with clinical data (16). The bolus was modeled as a Newtonian fluid with a viscosity of 10 centipoise and a volume of ∼1 ml. This is within the range of properties used in clinical studies (3). The specific problem we were trying to simulate was: How is the bolus transported to the distal esophagus after muscle activation wave is initiated?

Fig. 1.

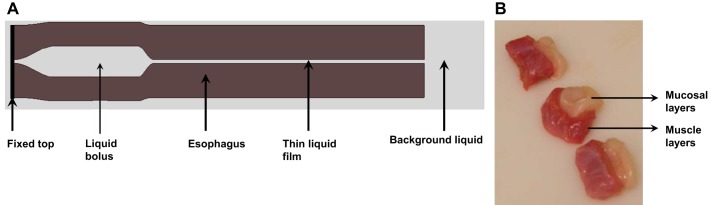
A: schematic (not drawn to scale) of the overall problem setup consisting of the elastic esophagus (brown) and a viscous liquid (light gray). The elastic esophagus was modeled as a cylindrical tube immersed in the background fluid. The top end of the esophagus was fixed in place. The outer surface and lower end of the esophagus were free of constraint, so that the lateral deformation and axial movement of the esophagus during bolus transport could be captured. The upper esophagus was initially filled with a bolus and the lower part was filled with a thin liquid layer in the lumen. B: segments of pig esophagus showing pronounced sliding between mucosal (white) and muscle layers (red).

Anatomical Model of the Esophageal Wall: Geometry and Structure

The esophageal wall is a long hollow tube that consists of mucosa, submucosa (here, collectively called “mucosal” layer), CM, and LM. From an in vitro test on pig esophagus, we found that the mucosal layer could easily slide relative to the CM as shown in Fig. 1B. Presumably, this sliding is indicative of weak attachment between these layers that has also been reported to allow easy separation of these layers in vitro (7, 27). Hence, the modeled esophageal wall consisted of three layers: mucosa, CM, and LM (see Material Model of the Esophageal Wall for details). In the resting state, the esophageal lumen was filled with a thin liquid film (5). The thickness of each layer was deduced on the basis of data from ultrasound images and the principle of mass conservation. In particular, we adopted experimental data reported by Puckett et al. (24). With these data, the thickness of each esophageal layer was deduced on the basis of the conservation of CSA. This was based on assumptions that 1) the esophagus was incompressible, and thus its volume did not change; 2) the change in the esophageal length, resulting from inserting a catheter, was negligible, because of which volume conservation also implied CSA conservation; and 3) the esophageal lumen and all layers were circular for simplicity. Data on geometry from in vivo ultrasound images used in our model are listed in Table 1.

Table 1.

Lumen radius and layer thickness of esophagus

	Lumen Radius	Thickness of Mucosal Layers	Thickness of CM Layer	Thickness of LM Layer
Experimental data (mm)	3.5	1.85	0.55	0.49
Data used in the model (mm)	0.3	3.8	0.6	0.6

In the model, it was assumed the lumen in the resting state only contained a thin liquid film with radius as 0.3 mm. Geometric data used in the model were obtained based on experimental data and conservation of cross-sectional area (CSA). Experimental data are from Ref. 24. CM, circular muscle; LM, longitudinal muscle.

Material Model of the Esophageal Wall: Passive Elastic Properties of Muscle and Mucosal Layers

As demonstrated experimentally, the CM and LM layers consist of circumferentially and longitudinally oriented muscle fibers, respectively (6). The mucosal layers are composed of collagen fibrils and connective tissue (20). Motivated by this structural information, we modeled the CM, LM, and mucosal layers separately as 3D axially circumferentially radially arranged fiber networks. Each fiber could generate an elastic force when subjected to stretching, compression, or bending. Hence, the assembled fiber network could characterize the effective passive elastic property (i.e., the elastic property without muscle activation). The compliance of each esophageal layer depends on its material property, in particular the fiber's modulus. For muscle layers, reported modulus values based on in vitro tests varied widely from several kPa to several MPa (25–27). Ghosh et al. (5) assumed esophageal body stiffness of several kPa (i.e., 2–20 kPa), depending on the stretch ratio. Here, we used fiber moduli for the CM and LM layers in three orientations as 4 kPa. For the mucosa, we used fiber moduli of 0.004 kPa in radial and circumferential orientations and 0.04 kPa in the axial direction. This was because the mucosal layers are highly buckled at rest to occlude the lumen, leading to high compliance. To model the sliding between mucosal and muscle layers, the outermost layer of mucosa was modeled with only radial fibers that were connected to the CM. The thickness of this outermost layer was assumed to be 0.6 mm, and the modulus of those radial fibers was the weakest, at 0.0004 kPa.

Muscle Activation Model

The sequential pattern of muscle activation is associated with neurophysiological activity that regulates the contraction/relaxation of muscle fibers. However, a quantitative model to characterize this regulation is unavailable owing to its complexity. In our computer model, we “mimicked” the neurally controlled contraction/relaxation events by dynamically changing the rest lengths of fibers in the corresponding layers, i.e., circular fibers in the CM layer and longitudinal fibers in the LM layer. Specifically, a muscle fiber was in a contracted state when its rest length was reduced because a fiber with a reduced rest length will tend to shorten to attain the new rest length. An inhibited or relaxed muscle fiber was the one with its initial rest length. A sequential contraction was modeled with a sequential wave of changing muscle fibers' rest length, defined as a “contraction wave,” propagating from the proximal to the distal esophagus. These simple features allowed us to clearly distinguish active muscle behavior from passive muscle behavior and facilitated control of each muscle type, i.e., we could “turn on or turn off” either CM contraction or LM shortening or both. Note that, because of the large scale of our fully resolved computational model, we used a contraction wave speed of 10 cm/s, which is faster than that of bolus transit imaged in a supine position. On the basis of extensive numerical testing, the muscle activation model was shown to capture the features of esophageal transport (13).

Methods for Case Studies

Two groups of case studies were conducted based on the fully coupled computational model. The first group investigated the roles of CM contraction and LM shortening, which included three cases: case 1, CM contraction, but no LM shortening; case 2, LM shortening, but no CM contraction; case 3, CM contraction and LM shortening. For each case, we first investigated the overall behavior by plotting the pressure field and axial bolus velocity using the software Visit (2). Next, we quantified the geometric and luminal pressure information in the axial direction using MATLAB (The MathWorks, Natick, MA).

The second group of studies examined the influence of coordination between CM contraction and LM shortening. In particular, two subgroups of studies were conducted. Subgroup 1 concerned esophageal transport when CM contraction was delayed relative to LM shortening, and subgroup 2 concerned esophageal transport when LM shortening was delayed. For each subgroup, we first studied two cases with delay times of 0.3 and 0.6 s, representing cases with intermediate delay and large delay, respectively. If an obvious effect on esophageal transport was seen in either subgroup, further case studies were conducted with delay times ranging from 0.1 to 0.6 s at 0.1-s increments.

RESULTS

Roles of CM Contraction and LM Shortening

Overall characteristics: role of CM contraction.

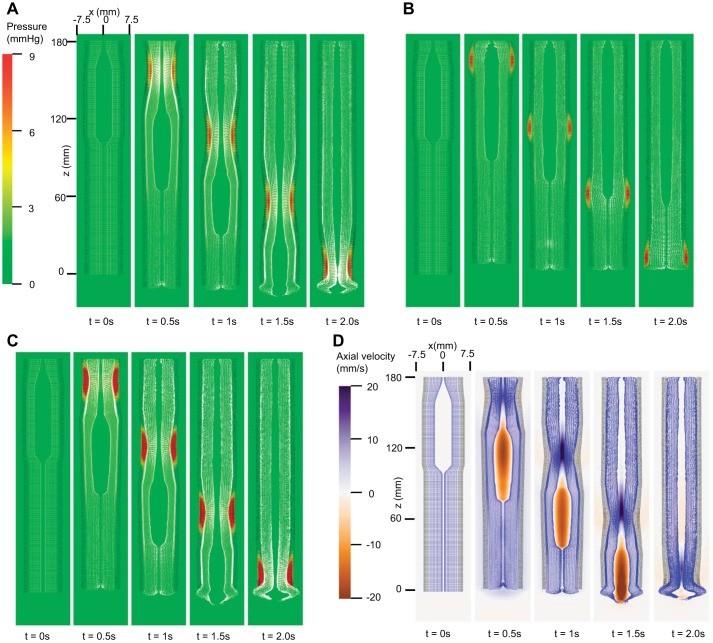
As evidenced in Fig. 2A, esophageal transport succeeded with only CM contraction characterized by a distinctive high-pressure zone proximal to the bolus tail. Also note that the esophagus lengthened because of the local effect within the contraction segment. On the other hand, with only LM shortening (Fig. 2B), no effective bolus transport was observed. The case with both LM and CM contraction is shown in Fig. 2, C and D. Good bolus transport was observed. A greater pressure within the contractile region was seen due to the combined muscle tension.

Fig. 2.

Overall features of esophageal transport plotted in the plane y = 0 (i.e., x–z plane) at different time. In our 3D model, z-axis represented the axial direction and x- and y-axes the 2 orthogonal lateral directions. To better visualize the information, the esophageal wall is colored as a 2-layered wall. The outer layer denotes muscle layers including circular muscle (CM) and longitudinal muscle (LM) layers, and the inner layer denotes mucosal layer. A, B, and C show pressure field with the deformed esophageal wall for CM contraction (case 1), LM shortening (case 2), and CM contraction and LM shortening (case 3), respectively (the outer layer is colored black, and the inner layer white). D: axial velocity for CM contraction and LM shortening (case 3) (the outer layer is colored black and the inner layer purple).

The above three cases suggest that CM contraction plays a primary role in esophageal transport by generating a squeezing pressure. However, these cases did not give a clear understanding of the role of LM shortening. Hence, we further analyzed the geometry, discussed below.

Geometry: role of LM shortening.

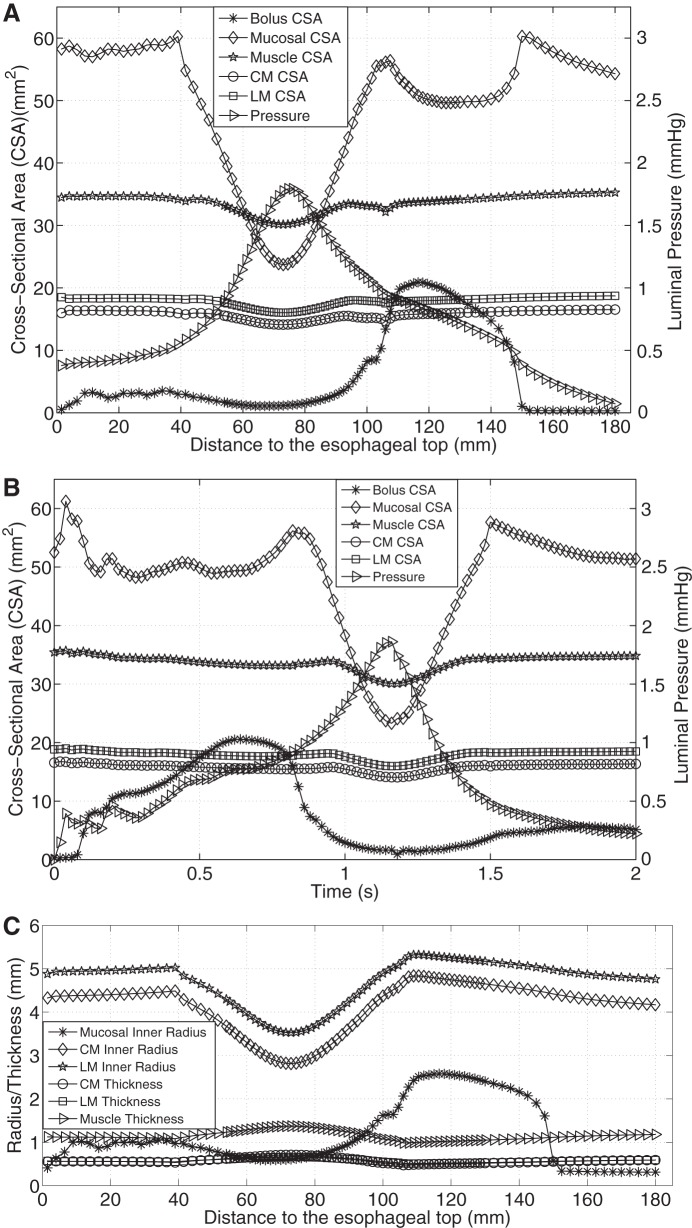
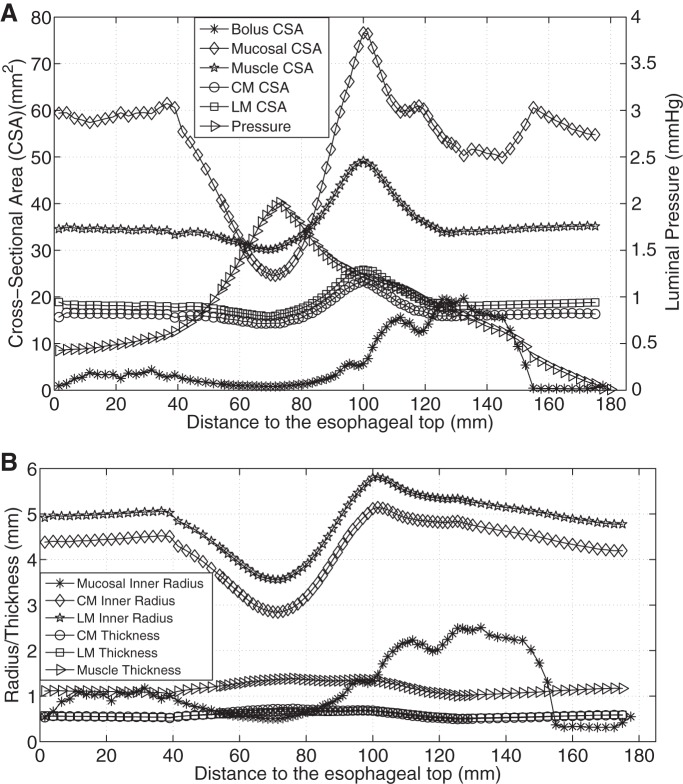
Figure 3A shows the axial distribution of CSA of bolus, mucosal, and muscle layers, as well as pressure, at time = 1 s (time = 0 s is defined as the time when muscle activation starts) for case 1 (CM contraction). Figure 3B shows the time-history of the above quantities at a fixed position z = 90 mm (z = 0 mm is defined as the initial position of the lower esophageal end) for case 1. Comparing Fig. 3, A and B, it can be seen the overall spatial profile of pressure and CSA, at a fixed time, was roughly the mirror image of the time-history profile of these same quantities. In particular, Fig. 3A shows a high-pressure region on the left of the bolus region, indicating that the moving bolus was driven by the proximal squeezing pressure. And Fig. 3B shows a high-pressure region on the right of the bolus region, as a typical esophageal locus was firstly filled with the bolus and then contracted owing to sequential muscle activation. The similarity between spatial profile and time-history profile of those quantities results from the sequential nature of activation and relaxation of esophageal muscle. Note that this similarity also occurred for case 2 (LM shortening) and case 3 (CM contraction and LM shortening). On the basis of these consistent similarities, the time-history characteristics can be reasonably appreciated by examining only the spatial profile.

Fig. 3.

Luminal pressure and geometrical information for CM contraction (case 1). A: luminal pressure and the cross-sectional area (CSA) of bolus, mucosal, and muscle layers along the axial direction at time = 1 s. B: temporal profile of the luminal pressure and the CSA of bolus, mucosal, and muscle layers of the esophageal segment that is located 90 mm proximal to the initial esophageal bottom. C: radius of mucosal and muscle layers, and the thickness of muscle layers along the axial direction at time = 1 s.

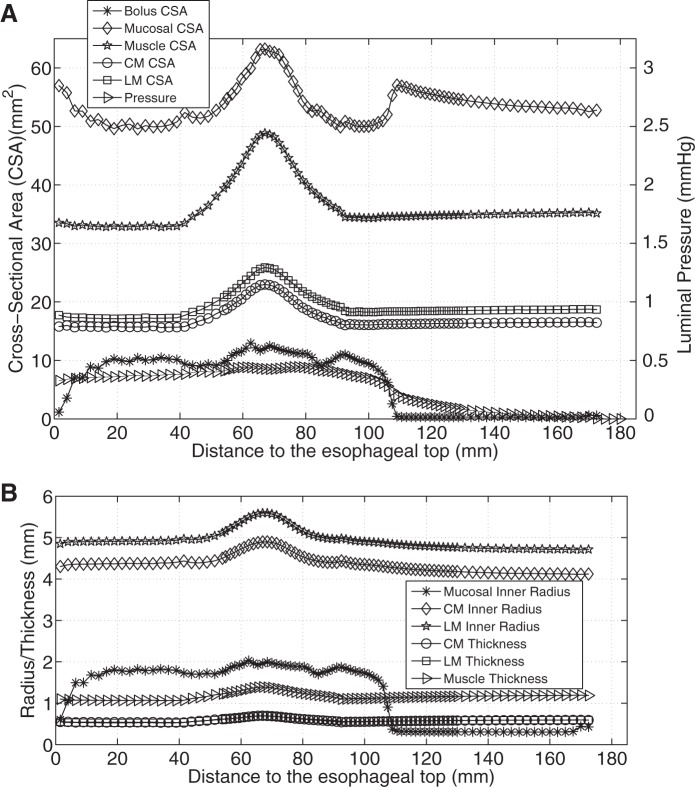
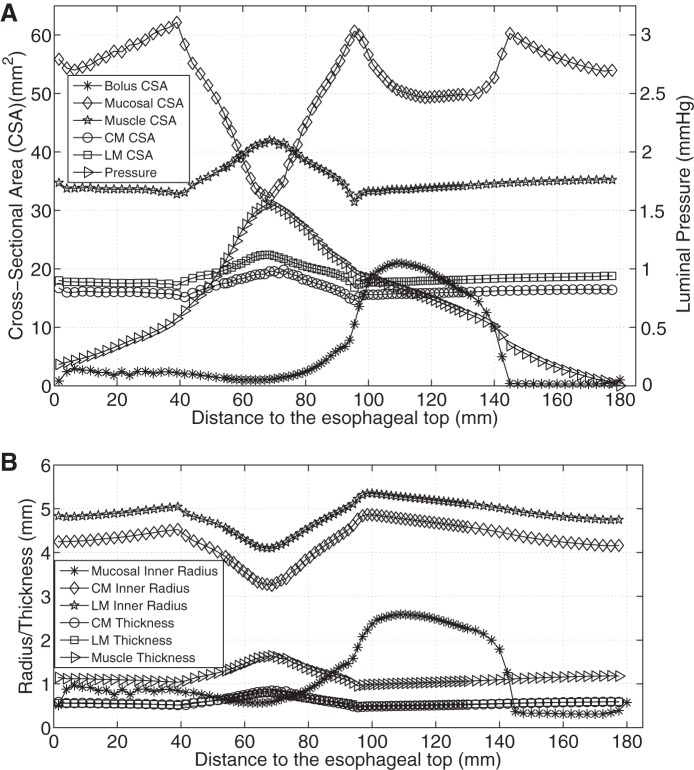
Figure 3, A and B, shows that with only CM contraction, muscle CSA decreased at the contracting region, indicated by the luminal pressure peak. Note that the decrease of muscle CSA resulted from the decrease in contracted radius, even though the thickness increased slightly (see Fig. 3C). In contrast, Fig. 4A shows that with only LM shortening, the CSA of all esophageal layers increased in the shortening region. There was no associated luminal pressure peak, which likely explains the absence of effective bolus transport in Fig. 2B. Figure 4B illustrates that the increase of muscle CSA was due to increases of both muscle radius and thickness. With synchronized LM shortening and CM contraction, a pressure peak proximal to the moving bolus overlapped the muscle CSA peaks of both the CM and the LM (Fig. 5A). This suggests that local LM shortening can increase the muscle CSA in the contracting region, which otherwise would be decreased due to CM contraction. Figure 5B further shows that the increase of muscle CSA was a consequence of an increased muscle thickening contributed primarily by LM shortening (though the muscle layer radius would still diminish to narrow the lumen and squeeze the bolus). Table 2 summarizes the characteristics in terms of geometry and pressure profile for the three cases and shows that both LM shortening and CM contraction must be involved to generate the coexisting muscle CSA and pressure peaks.

Fig. 4.

Luminal pressure and geometrical information for LM shortening (case 2). A: luminal pressure and the CSA of bolus, mucosal, and muscle layers along the axial direction at time = 1 s. B: radius of mucosal and muscle layers, and the thickness of muscle layers along the axial direction at time = 1 s.

Fig. 5.

Luminal pressure and geometrical information for CM contraction and LM shortening (case 3). A: luminal pressure and the CSA of bolus, mucosal, and muscle layers along the axial direction at time = 1 s. B: radius of mucosal and muscle layers, and the thickness of muscle layers along the axial direction at time = 1 s.

Table 2.

Characteristics of esophageal transport

	Overall Length of Esophageal Muscle Layer	Muscle CSA Profile in the Activation Region	Muscle Thickness	Muscle Average Radius	Pressure Profile in the Activation Region	Bolus Transport
Case 1	Increase	Trough	Slight increase	Decrease	Peak	Effective
Case 2	Decrease	Peak	Increase	Increase	Plateau	Not effective
Case 3	Slight Decrease	Peak	Increase	Decrease	Peak	Effective

Case 1, CM contraction; Case 2, LM shortening; Case 3, CM contraction and LM shortening.

Influence of Discoordination between LM and CM Activations

Esophageal transport if LM shortening precedes CM contraction.

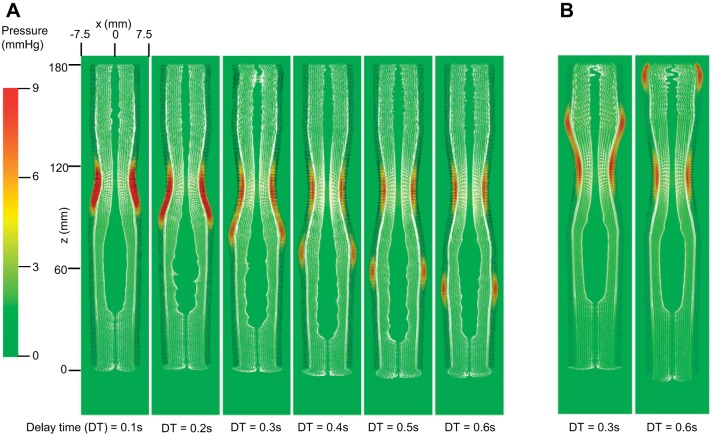
The pressure field for cases in which the CM contraction wave is delayed is shown in Fig. 6A. With the increase in delay time and the associated CM and LM discoordination, two high-pressure regions in the muscle layers became apparent. Bolus transport seems to be affected, indicated by the irregular shape of the mucosal surface over the moving bolus. This suggests that if the CM contraction wave is delayed, the bolus transport could be adversely affected. The detailed geometric information and the pressure profile for the case with delay time = 0.3 s was further examined as shown in Fig. 7A. Indicated by the pressure peak, the CM contraction wave was ∼70 mm distal to the top of the esophagus. The preceding LM wave was ∼100 mm distal on the basis of the muscle CSA peak. The moving bolus was in the region between 80 and 155 mm distal from where the bolus CSA was significantly large. This implies that when CM and LM are not synchronized it is the CM contraction wave, not the LM shortening wave, that determines the locus of the tail of the moving bolus. Hence the preceding segment of LM shortening will overlap with the bolus region. In the overlapped region (∼90–110 mm distal), the esophageal wall's CSA and thickness, including that of mucosal and the two muscle layers, was increased (see Fig. 7A). This was a consequence of local shortening. Thus the wall's compliance was reduced, leading to decreased distensibility. This can also be seen by comparing the CSA of the bolus and the esophageal components in the region between 90 and 110 mm distal in Figs. 7A and 5A. From the viewpoint of mechanics, the reduced compliance in the bolus region due to LM shortening may account for the unstable shape of the moving bolus observed in Fig. 6A.

Fig. 6.

Pressure field with the deformed esophageal wall in the plane y = 0 (i.e., x–z plane) for cases that CM contraction was delayed (A), and that LM shortening was delayed (B). A: from left to right, the delay time (DT) increased from 0.1 s to 0.6 s, and the snapshot for each case was taken at time = 1 s + DT, i.e., when the center of CM contraction wave in all cases arrived at the same level (i.e., z = 110 mm). B: from the left to right, the DT was 0.3 and 0.6 s, respectively. The snapshot for each case was taken at time = 1 s, i.e., when the center of CM contraction wave arrived at the same level (i.e., z = 110 mm). The esophageal wall is colored as a 2-layered wall. The outer layer (black) denotes muscle layers including CM and LM layers, and the inner layer (white) denotes mucosal layer.

Fig. 7.

Luminal pressure and geometrical information for the case that CM contraction was delayed by 0.3 s. A: luminal pressure and the CSA of bolus, mucosal, and muscle layers along the axial direction at time = 1.15 s. B: radius of mucosal and muscle layers, and the thickness of muscle layers along the axial direction at time = 1.15 s.

Esophageal transport if CM contraction precedes LM shortening.

Figure 6B illustrates the pressure and axial velocity fields for cases with LM shortening delayed by 0.3 and 0.6 s relative to CM contraction. In both cases, bolus transport seemed unaffected. Clearly, the locus of the tail of the moving bolus was determined by the wavefront of CM contraction. Thus the lagging LM shortening wave became irrelevant to bolus transport. Therefore, it is conjectured that bolus transport with the CM contraction wave preceding LM shortening will approximate the case with CM contraction and no LM shortening.

DISCUSSION

Esophageal transport is a complicated physiological and mechanical process that involves interactions among the esophageal wall, neurally controlled muscle activation, and a bolus. The neurally controlled muscle activation involves two coordinated aborally propagating waves in normal subjects: CM contraction and LM shortening. Here we utilized a fully coupled active musculomechanical model in an attempt to address two questions: 1) What are the roles of CM contraction and LM shortening in esophageal transport? and 2) How does discoordination between the two muscle activations influence esophageal transport? We remark that an experimental approach to directly answer the above questions can at the very least be difficult since it involves exciting one muscle activation while inhibiting (question 1) or delaying (question 2) the other. However, the fully coupled simulation approach offers a feasible tool to address them with a fairly easy method such as a parametric study.

As Fig. 2A shows, CM contraction plays primary roles in esophageal transport, by contracting the lumen and generating high luminal pressure to force the bolus aborally. LM shortening, when collaborating with CM contraction, will increase the muscle CSA in the contracting region, which would otherwise be decreased with only CM contraction. Thus LM shortening can thicken and strengthen the contracted muscle to sustain the high squeezing pressure during bolus transport. Nicosia et al. (21) proposed, on the basis of mass conservation, that LM shortening also helps reduce required tension per muscle fiber or, equivalently, increase the luminal pressure. However, this may not necessarily be the case because LM shortening will also increase the radius of the muscle layer or expand the esophageal wall (see Figs. 2B and 4B). Contracting an expanded esophageal wall means additional amount of elastic deformation is involved and larger elastic force needs to be balanced by the active muscle force. This reduces the luminal pressure peak as seen by comparing Figs. 5A and 3A. Another role of LM shortening proposed by Brasseur and colleagues (1, 22) is to generate axial motion of mucosal surface, but this was not supported by our study.

How well CM contraction and LM shortening collaborate depends on their coordination. If LM shortening preceded CM contraction, the transport was adversely impacted as the bolus became unstable, showing irregular shape in Figs. 6A and 7A. This may cause dysmotility. Related clinical data support that conjecture. Jung et al. (8) showed that in some patients LM shortening preceded CM contraction, and they speculated that the discoordination (or asynchrony) might cause dysfunction. On the other hand, if CM contraction preceded LM shortening wave, the lagging LM shortening became irrelevant in bolus transport, and it no longer strengthened the contracted CM segment. In summary, from the viewpoint of mechanics, it seems that the near-synchronized CM contraction and LM shortening that occurs in the normal physiology of bolus transport is the optimal among all cases studied.

In addition to addressing the above two questions, our simulations also confirmed a number of experimental observations on esophageal peristalsis: 1) that, consistent with the observation from concurrent manometry and videofluoroscopy (9), a high CM squeezing pressure region was always located near the tail of the moving bolus; 2) consistent with observations from concurrent manometric and high-frequency intraluminal ultrasound studies (HFIUS) (19), there was a spatial and temporal overlap between muscle CSA peak and pressure peak, which occurred after bolus passage; and 3), also as observed in HFIUS study on some patients (8), for cases in which LM shortening preceded the pressure peak, abnormal peristalsis occurred. Moreover, the simulations also provided novel observations and interpretations. First, the case studies with individual muscle activation showed that the high-pressure zone observed experimentally is associated with CM contraction, not LM shortening. Second, the muscle CSA increase results from LM shortening, not CM contraction. Hence, the experimentally observed synchrony of muscle CSA and pressure peaks indeed indicates synchronized LM shortening and CM contraction. Third, the experimentally observed abnormality of esophageal peristalsis associated with LM shortening preceding CM contraction probably relates to the decreased compliance of esophageal wall in the bolus region. The underlying mechanism of this relates to two factors: 1) overlap between the bolus region and the region with activated LM shortening; and 2) thickening of the esophageal wall resulting from LM shortening.

Understanding the roles of the two muscle contractions could provide guidance on clinical diagnosis. First, CM contraction plays a primary role in esophageal transport and it is sufficient to transport the bolus. This suggests that examining the behavior of CM contraction, compared with that of LM shortening, should be of high priority in the diagnosis of the esophageal motility. This may further highlight the significance of manometry-based metrics, such as Chicago Classification criteria (10), in clinical practice. This is because the characteristics of sequential CM contraction can be well manifested by the spatiotemporal variation of the pressure field obtained from high-resolution esophageal pressure topography. Second, as listed in Table 2, both LM shortening and CM contraction can cause a change in geometry. Hence, the quantification of LM shortening characteristics based on geometry change in experimental studies, such as the CSA from ultrasound (19, 21), should involve the subtraction of geometry change due to CM contraction. For example, LM shortening could still possibly occur in the contracting region where the muscle CSA is only slightly decreased.

Results on discoordination may shed light on the correlation between motor dysfunction and motility disorders. In particular, an irregular shape of a moving bolus due to an advancing LM shortening may indicate the emergence of lateral movement of bolus. This might be an early sign of bolus obstruction. Depending on the sensory and motor mechanism, other physiological compensations such as an increased level of muscle contraction may develop to attempt to overcome the obstruction. This might explain why advancing LM shortening was seen in nutcracker esophagus (8) or eosinophilic esophagitis (12). Another consequence of discoordination is the loss of the benefit of muscle thickening from LM shortening in the contracting segment. Strength will be reduced simply because of less muscle available to sustain the peak luminal pressure. The esophageal wall is more likely to develop a weak spot, leading to the formation of an esophageal diverticulum. This has also been speculated by Jung et al. (8).

Our computational model has limitations. First, we take the wavespeed of muscle activation to be 10 cm/s instead of ∼3 cm/s, observed in reality (15), to reduce computational cost. Second, in the interest of simplicity we assume that the muscle tension is from the contraction of muscle fibers with the same stiffness as in the passive state. This may underpredict the magnitude of muscle tension and luminal pressure. However, we present our results and conclusions in a way that they do not depend on the absolute magnitude of pressure.

GRANTS

This work was supported by National Institute of Diabetes and Digestive and Kidney Diseases Grants DK056033 (to P. J. Kahrilas) and DK079902 (to J. E. Pandolfino).

DISCLOSURES

J. E. Pandolfino discloses consulting and educational association with Given Imaging and Sandhill Scientific. No relevant competing financial and other interests exist for other authors.

AUTHOR CONTRIBUTIONS

W.K., J.E.P., P.J.K., and N.A.P. conception and design of research; W.K. and N.A.P. performed experiments; W.K. and N.A.P. analyzed data; W.K., J.E.P., P.J.K., and N.A.P. interpreted results of experiments; W.K. prepared figures; W.K. drafted manuscript; W.K., J.E.P., P.J.K., and N.A.P. edited and revised manuscript; W.K., J.E.P., P.J.K., and N.A.P. approved final version of manuscript.