Abstract
Several structural and physical changes in foodstuffs are the consequence of water removal during the drying process. Porosity (volume fraction of pores) is one of the key parameter that affects the quality and other properties of foods (such as apple and potato). To understand the effect of dehydration in apple and potato, in the present study an arbitrary small cubic volume element is considered which contains pores (intracellular spaces) distributed in it. Further, it is assumed that each pore in the cubic volume element is spherical. A mathematical relation is developed between porosity (volume fraction of pores) and pressure generated (due to contraction of cells during water removal) in outward direction on the surface of spherical elements containing pore. The developed relation is satisfactory in respect of experimental observations given in the literature. For the given pressure range, acquired porosity range is 0.1 to 0.92 for apple and 0.03 to 0.89 for potato which is matched with the existing experimental values. The results showed that the porosity is increasing with the increasing values of pressure, as expected, during moisture removal. Further, it is observed that the current porosity is depended on the initial porosity for both apple and potato.
Keywords: Apple and potato, Dehydration, Porosity, Pressure, Strain invariants, Strain energy function
Introduction
Quality and texture of food products can be characterized by their structural properties during dehydration without effecting their taste and appearance. The dehydration process is frequently used to extend shelf-life by increasing stability of foods (for example, apple and potato) during storage, since it reduces microbiological activity, decreases extensively water activity of the material and minimizes physical changes (Mayor and Sereno 2004). There are various types of structural properties such as moisture content, shrinkage, porosity, density and surface area that are affected by dehydration process.
Several physical and structural changes can be seen in food materials due to moisture removal during drying process (Koç et al. 2008). Ratti (1994) reported that transport property and dimensions of individual particles modified during drying that caused changes in shape and size of food materials. Numerous studies are available in literature which investigated these physical and structural changes in various foodstuffs. Among the diverse-studied properties for different drying methods, some of the properties are listed in Table 1 for selected fruits and vegetables. However, in the present work we have focused on the study of porosity of apple and potato only because porosity is the most important physical property that characterizes the quality and texture of dry and moisture foods (Sahin and Sumnu 2006). Porosity of apple and potato largely depends on the temperature, moisture contents, humidity, air velocity, drying method and initial porosity (Rahman et al. 2005; Krokida and Maroulis 2001; Oikonomopoulou and Krokida 2013).
Table 1.
Various examined parameters in different drying experiments for various foodstuffs
| Examined parameters | Examined products | Drying methods | References |
|---|---|---|---|
| Porosity, moisture content | Apple | Air drying | Lozano et al. (1980) |
| Porosity, shrinkage, density | Potato, Carrot, Garlic, Pear, Sweet potato | Air drying | Lozano et al. (1983) |
| Porosity, density, shrinkage | Apple, Potato, Carrot | Air drying | Zogzas et al. (1994) |
| Porosity, structure, density | Potato | Air drying | Wang and Brennan (1995) |
| Porosity, density, specific surface area, pore size, pore size distribution | Apple, Potato Cabbage, Carrot | Air and Freeze drying | Karathanos et al. (1996) |
| Porosity, density, specific volume | Apple, Potato, Banana, Carrot | Conventional, Vacuum, Osmotic, Microwave, Freeze Drying | Krokida and Maroulis (1997) |
| Porosity, density, specific volume, moisture contents, pressures | Apple, Potato, Banana, Carrot | Vacuum dehydration | Krokida et al. (1997) |
| Porosity, shrinkage, moisture contents | Potato | Air drying | McMinn and Magee (1997) |
| Porosity, shrinkage, density, moisture contents | Apple | Osmotic drying | Mavroudis et al. (1998) |
| Porosity, density, color and viscoelastic behavior | Apple, Potato, Banana, Carrot | Microwave and Microwave-vacuum drying | Krokida and Maroulis (1999) |
| Porosity, density | Apple, Potato, Abalone, Sates | Freeze drying | Sablani and Rahman (2002) |
| Porosity, densities | Apple | Osmotic dehydration | Nieto et al. (2004) |
| Porosity, density, pore size distribution | Potatoes, Mushrooms, Strawberries | Freeze dried | Oikonomopoulou and Krokida (2012) |
For the quality characterization of dehydrated food products the study of structural properties such as density and porosity are important. These properties have profound effects on other properties, namely, permeability, diffusivity and thermal conductivity (Krokida and Maroulis 2001). Among the various changes in food materials, the most significant change which occurs during drying is the reduction of its external volume. Therefore, the change in microstructure i.e. shrinkage and formation of pores is the consequence of loss of water and heating that causes stress in cellular material (Mayor and Sereno 2004). Bai et al. (2002) studied the structural changes in apple rings during convective drying. They found that the water removal leads to cell rupture and the formation of cracks in the inner structure which is caused of the microstresses in the cell membrane and the cell wall in the process of dehydration. Further, Sinha (2011) mentioned that during dehydration, the more the water loss, the more the contraction stresses are originated in the material that generates the pressure outward on the cavity surfaces. As a result, cavities of different size and shape in food products build up (Mayor et al. 2008) that increase the porosity in the product.
Numerous studies are available in the literature (Dissa et al. 2010; Karathanos et al. 1996; Katekawa and Silva 2004; Krokida et al. 1998; Liu et al. 2012; May and Perre 2002; McMinn and Magee 1997; Rahman 2001, 2003; Rahman et al. 1996; Wang and Brennan 1995) which reported that the formation of porosity is the consequence of evaporation of water from cellular products during dehydration. Further, it is also reported that development and increment of porosity in foods during drying is strongly affected by initial porosity, initial material moisture content, relative humidity, composition, drying methods and conditions (Zogzas et al. 1994).
Rahman (2003) mentioned that the factors which affect the development of pores can be grouped as (i) extrinsic and (ii) intrinsic factors. Relative humidity, temperature, gas atmosphere, pressure, electromagnetic radiation, and air circulation are including in extrinsic factors, whereas the intrinsic factors are initial structure and chemical composition. The shrinkage is considered as the ideal during drying if the decrease in volume of material is proportional to the loss of mass. On the other hand, porosity comes into view if the volume reduction of material is lesser than volume of water loss (Madiouli et al. 2007, 2012). During drying, Mattea et al. (1989) analyzed the shrinkage of apple tissues by means of computer model.
As drying process proceeds and temperature increases, moisture content in apple and potato decreases consequently internal pressure in these products generated by water vapor which is responsible for pore formation. The most common terminology used in characterizing pores is porosity (volume fraction of pores) (Rahman 2001). For the prediction of porosity during dehydration, the aim of present work is therefore two-fold: (i) to develop a mathematical relation between porosity (volume fraction of pores) and pressure (a contraction stress generated in the cells due to water removal during dehydration) with the help of strain energy function for apple and potato, and (ii) to examine porosity behavior with respect to pressure and initial porosity.
Mathematical development
Problem formulation
Natural solid foodstuffs constitute systems of cellular tissues, and therefore, may be regarded as peculiar porous systems (McMinn and Magee 1997). Rahman (2001) described various factors (such as surface tension, pore pressure, structure, environment pressure, and mechanisms of moisture transport) that play important roles in explaining the pore formation during drying of food products. Further, Rahman (2001) hypothesized that as capillary force is the main force responsible for collapse, so counterbalancing this force causes formation of pores and lower shrinkage. The counterbalancing forces are due to generation of internal pressure due to vaporization of water or other solvents, variation in moisture transport mechanism, and pressure outside the material. Further, it is reported in literature (Khalloufi et al. 2011; Ratti 1994) that food products undergo deformations that can be characterized by changes in volume, shape, porosity, density, shrinkage and/or collapse phenomena during drying. To study the porosity behavior and deformation of porous material (apple and potato), let us consider an arbitrary small cubic volume element containing pores distributed in it (Fig. 1a). For the mathematical analysis it is assumed that every pore in the cubic volume element surrounded by a spherical surface which is lying completely in the material matrix at every instant. Suppose that this porous material is subjected to the pressure that is responsible for counterbalancing forces and generated on the surface of porous matrix as depicted in Fig. 1c. Therefore, the problem under consideration is the study of deformation of a sphere consisted of spherical pore and analysis of the growth of voids (i.e. pores or cavities) in deformed material. Due to spherical symmetry, the pressure on the surface of porous matrix prescribed radial deformation of the spherical solid element enclosing pore. Fig. 1b, c depicts the undeformed and deformed configurations of the spherical material, respectively. Suppose that in the undeformed configuration, the radius of a spherical void cell is denoted by A0 (inner radius) and that of the bulk material by B0 (outer radius), while in the deformed configuration, the radius of the void cell and material cell is denoted by A (inner radius) and B (outer radius), respectively. Using spherical polar coordinates, the position of a particle x in the undeformed configuration is denoted by (R, Θ, Φ) and after deformation; suppose it takes the new position y and is denoted by (r, θ, ϕ). For the study of cavitations in porous hyperelastic solid, the radial symmetric deformation is defined as (Ball 1982; Hou 1990; Lopez-Pamies et al. 2011; Sivaloganathan 1986)
| 1 |
Fig. 1.
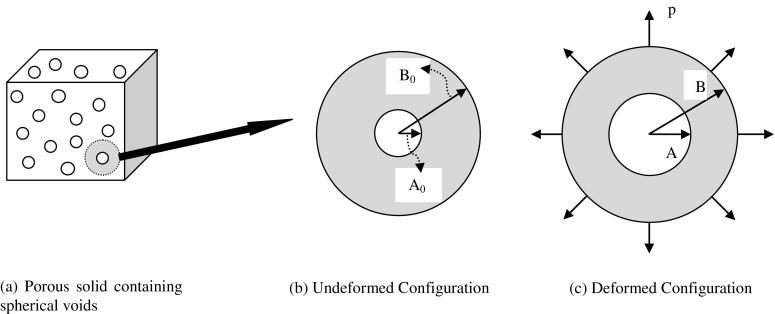
The structure of porous material (a) cubic porous solid containing spherical voids (b) undeformed configuration (c) deformed configuration
where
and F(R) is a function of R which will be determined.
Since deformation is radialy symmetric, therefore
| 2 |
Deformation gradient tensor F associated with deformation (1) is given by
| 3 |
where
| 4 |
where F′(R) is derivative of F(R) and δij is Kronecker delta. By the assumption of incompressibility, we have
| 5 |
on solving Eqs. (3) and (5) (for details, see Appendix A), one can get
| 6 |
where C is constant of integration.
During deformation, the point R = A0 deformed into the point r = F(R) = A, therefore, Eq. (6) reduced to
| 7 |
If A = A0, Eq. (7) implies that r = R, i.e. no deformation in the solid matrix.
Constitutive relation
To study the mechanical properties of apple and potato tissues, Singh et al. (2013) proposed a strain energy function by considering tissues as isotropic, incompressible and hyperelastic material. Further, the influence of turgor pressure on apple and potato tissues as a function of stretch ratio was discussed by using this strain energy function (Singh et al. 2014). In the present work, therefore, we have considered this strain energy function to obtain the desired mathematical relation for the prediction of porosity in apple and potato. The proposed strain energy function was
| 8 |
where b, C1 and C2 are material constant, and I1, I2 are strain invariants which are defined as
| 9 |
B is the left Cauchy-Green deformation tensor calculated from B = FFT = V2, F is given by Eq. (3). Principal stretches λr , λθ , λϕ (i.e. eigenvalues of V) are given by
| 10 |
using incompressibility condition λrλ2θ = 1, Eq. (9) can be written as
| 11 |
where
| 12 |
| 13 |
For the isotropic, incompressible hyperelastic material, Cauchy stress tensor is defined by (Holzapfel 2000)
| 14 |
where ρ is the hydrostatic pressure. From Eq. (14), the principal components of Cauchy stress can be obtained as
| 15 |
| 16 |
Equilibrium equation
Now, the stress distribution within the material during deformation can be solved with the help of equilibrium equation. For the radial deformation, the equilibrium equation can be written as (Chang and Pan 1997, 2001; Hou 1990; Lopez-Pamies et al. 2011; Murphy and Biwa 1997; Polignone and Horgan 1993)
| 17 |
Boundary conditions
The pressure p developed on the surface of the porous sphere (which is responsible for counterbalancing force in formation of pores) based on deformed configuration is related to radial stress by the relation (Chang and Pan 2001; Polignone and Horgan 1993)
or by using Eqs. (5) and (13), τrr can be rewritten as (for details, see Appendix B)
| 18 |
It is also assumed that the cavity surface is traction free, which gives
| 19 |
Final desired relation
The difference between τrr and τθθ can be calculated from Eqs. (15) and (16) as
| 20 |
Hence, the problem in hand is to solve the Eq. (17) with boundary conditions (18) and (19). Therefore,
| 21 |
on integrating Eq. (21) from r = A to r = B, we get
| 22 |
by using boundary conditions (18) and (19); and change the variable of integration from r to λr with the help of Eq. (12), we can get
| 23 |
where Ā, (with initial porosity f0 = (A0/B0)3 and current porosity f = (A/B0)3) and λθ (using Eq. B3) are given by
| 24 |
hence, by using Eq. (8) with (11), Eq. (23) reduced to
| 25 |
with the use of Eq. (24), Eq. (25) gives the relation between current porosity and pressure in terms of initial porosity during dehydration in apple and potato.
Porosity measurement methods
Porosity plays an important role in characterizing of the quality and the texture of dry and intermediate moisture foods. It has significant effect on the physical (such as thermal conductivity, thermal diffusivity and diffusion coefficient), textural and mechanical properties of foods (Rahman 2001). Porosity is defined as the volume fraction of the air or the void fraction in the sample and expressed as:
Various methods that can be used for porosity measurement of food products were reported by Sahin and Sumnu (2006) and are summarized as follows:
Direct method: This method is used to determine the porosity by calculating the difference of bulk volume of a sample of porous material and the volume measured after destruction of all voids by compression. The porosity of very soft material can be determined by using this method.
Optical method: In this method, porosity is determined from the microscopic view of a section of the porous medium. This method is appropriate if the porosity is uniform throughout the sample.
-
Density method: In this method, porosity is measured from the densities of materials.
where bulk density and particle density can be measured by the methods given in the work of Krokida and Maroulis (1997). In another way, if the densities data of the material is not available then porosity can be calculated from its pore volume. Liquid or gas displacement method (Sahin and Sumnu 2006) can be used for pore volume measurement.
Gas pycnometer method: In this method, air comparison pycnometer is used to measure the porosity by measuring the volume fraction of air.
Using porosimeters: Mercury porosimetry can be used to determine the porosity, pore’s characteristics and pore size distribution. Porosimeters are based on the principle of either liquid extrusion from the pores or liquid intrusion into pores. In liquid intrusion method, liquid (such as oil, mercury or water) is forced into the pores by applying pressure and intrusion volume is measured. On the other hand, in extrusion porosimetry, pores in the porous materials are filled by wetting liquids and pressure is applied to displace the liquid from the pores. Further, volume change of extruded liquid is measured with pressure.
Among these methods, for porosity measurement, density method was extensively used by researchers to measure the porosity of apple, carrot and potato (Zogzas et al. 1994), potato (Wang and Brennan 1995), banana, apple, carrot and potato (Krokida and Maroulis 1997; Krokida et al. 1997), however, Gas pycnometer and porosimeters method was used for corn, wheat, and sorghum kernels (Chang 1988), starch materials (Karathanos and Saravacos 1993), apple, potato, cabbage, and carrot (Karathanos et al. 1996) and apple (Rahman et al. 2005) during drying. These methods can also be used to measure porosity of food products before and after drying process.
In the present study, the porosity data used to validate the model is measured by density method. Finally, the details of density method can be found in literature (Krokida and Maroulis 1997; Krokida et al. 1997; Wang and Brennan 1995; Zogzas et al. 1994) for porosity measurement of apple and potato experimentally.
Results and discussion
Analysis of cavity growth
To discuss the growth of cavity (pores) during dehydration in apple and potato, we have considered two possible cases for the value of A (i.e. growth of cavity). It may be possible that either A = A0 or A > A0.
-
(i)
When A = A0
It is evident from literature (Joardder et al. 2014; May and Perre 2002; Weinberg 2007) that fresh apple and potato contains certain amount of arbitrarily distributed voids and pores in it. However, the cavities are small as compared to the whole structure of the product. For instance, if we assume that initial porosity in these products is zero (i.e. A0 = 0) which leads to A = 0, the Eqs. (2) and (7) describes a trivial deformation in which the body remains undeformed. Also the value A = 0 contradicts the results regarding formation of pores during dehydration which was reported in several experimental studies (Rahman et al. 2005; Lozano et al. 1980; Krokida et al. 1997). Therefore, the assumption A0 = 0 is not valid and hence A0 > 0. Again, if A = A0 > 0, then Eqs. (2) and (7) describes a trivial deformation.
-
(ii)
When A > A0
In this case, cavity formation and size of cavity depends on the values of A0. If we assume that A0 = 0 (i.e. absence of initial porosity), then the value of p calculated from Eq. (25) is very large for formation of pores in dried products. Practically, no such large pressure can be generated during dehydration in these products. Therefore, it is reasonable to consider some positive value of initial porosity (i.e. A0 > 0). Also the value of A depends on p that should not exceed a suitable limit. The case A = A0 is not possible as discussed above. Only the possibility remains is A > A0. For practically valid situations, we have explored all possible conditions for A. During dehydration when amount of water in apple and potato decreases, internal pressure in cells increases consequently radius of cavity (A) increases.
Numerical solution
Equation (25) presents the relation between current porosity and pressure (in kPa) which is (responsible for counterbalancing force in formation of pores) developed on the surface of spherical elements containing pores formed by water evaporation during dehydration in apple and potato. The integral in Eq. (25) is evaluated numerically by using Gauss-Legendre quadrature rule. The values of constants b = 1, C1 = 0.3524 (MPa), C2 = 6.4440 (MPa) for apple and b = 1, C1 = 0.0283 (MPa), C2 = 3.0118 (MPa) for potato are taken from literature (Singh et al. 2013). The obtained relation (Eq. 25) is used to predict the porosity variation in apple and potato for varying pressure values during drying. During dehydration, as moisture content decreases in apple and potato, generated pressure (contraction stress) increases on the cell-wall, as a results cells shrink which causes increment in the porosity.
Experimental data
For various drying methods and temperature, experimental works had been carried out for diverse food products (for example, apple, banana, carrot, and potato) by several group of researchers (Krokida et al. 1999, 2000; Krokida and Maroulis 1999), and a relationship between moisture content and maximum stress and maximum strain in dehydrated products was reported. This stress is analogy to the pressure generated on the cell-wall or in other words on the surface of spherical elements containing pores. The values of pressure generated on the spherical surface are varying in different drying methods; therefore, a range (from minimum value to maximum value) for pressure is considered in the present work. In the acquired range of pressure, we have done our calculation and obtained a range for porosity which is recumbence in between the range (values of porosity) which is given in literature. For different experimental studies, the values of initial porosity for apple and potato are presented in the Table 2. Krokida and Maroulis (2001) and Oikonomopoulou and Krokida (2013) collected data of porosity for various foodstuffs, temperature, conditions and drying methods from literature and adduced in a table form. It is observed that porosity is varying from 0.1 to 0.92 and 0.03 to 0.89 for apple and potato, respectively.
Table 2.
Initial porosity of apple and potato
| Product | Initial porosity | References |
|---|---|---|
| Apple | 0.15 | Krokida and Maroulis (1997) |
| 0.182 | Lozano et al. (1980) | |
| 0.194 | Sablani and Rahman (2002) | |
| 0.2 | Mavroudis et al. (1998); Rahman (2008) | |
| 0.22 | Rahman et al. (2005) | |
| Potato | 0.03 | Mavroudis et al. (1998) |
| 0.04 | Lozano et al. (1983) | |
| 0.05 | Suzuki et al. (1976); Zogzas et al. (1994) | |
| 0.08 | Krokida and Maroulis (1997) |
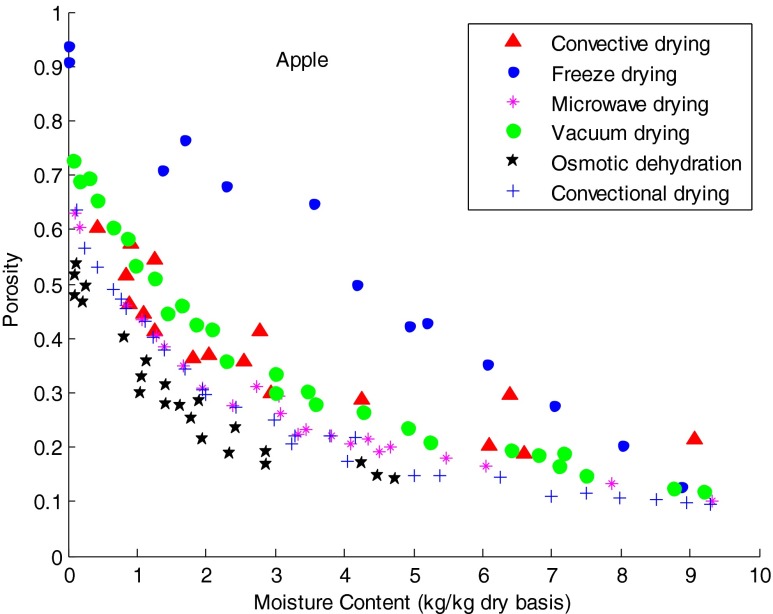
In the present study, for the validity of the developed mathematical relation data have been extracted from the experimental works (Krokida and Maroulis 1997; Wang and Brennan 1995; Zogzas et al. 1994) for apple and potato during dehydration. Figure 2 shows the experimental data (Krokida and Maroulis 1997; Zogzas et al. 1994) of porosity as a function of moisture content for convective, freeze, microwave, vacuum, osmotic and convectional drying of apple. It can be seen from the Fig. 2 that the value of porosity increases from 0.1 to 0.92 as moisture content decreases in various drying methods. Further, the porosity increment with decrease in moisture content is high in freeze drying whereas it is low in osmotic dehydration.
Fig. 2.
Porosity versus moisture content for apple. Data have been taken from literature- Zogzas, et al. (1994) (convective drying) and Krokida and Maroulis (1997) (freeze, microwave, vacuum, osmotic and convectional drying)
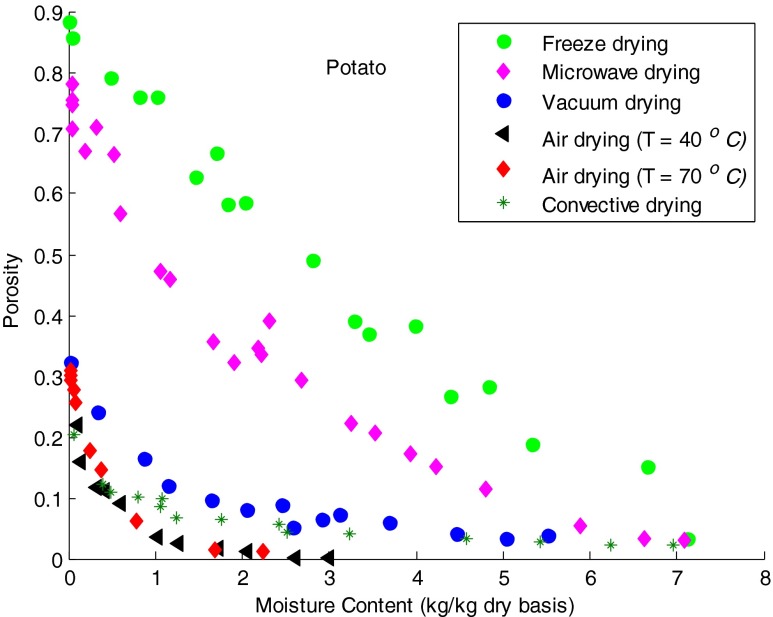
Figure 3 shows the porosity changes as the function of moisture content from the experimental data (Krokida and Maroulis 1997; Wang and Brennan 1995) for air drying at temperature 40 °C and 70 °C and for freeze, microwave and vacuum drying of potato. The porosity variation is seemed from 0.03 to 0.89 as moisture content decreases. During potato drying, the porosity increment is high for freeze drying whereas it is low for air drying at temperature 40 °C for decreasing value of moisture content.
Fig. 3.
Porosity versus moisture content for potato. Data have been taken from literature-Krokida and Maroulis (1997) (freeze, microwave, vacuum drying) and Wang and Brennan (1995) (air drying 40 °C and 70 °C)
Model validation
The relation between porosity and pressure developed in the present work is based on the strain energy function (Eq. 8). To study the mechanical properties of apple and potato, the adequacy of this strain energy function is described well in our previous works (Singh et al. 2013, 2014). In these works mechanical properties (tension-stretch ratio relation, turgor pressure-stretch ratio relation) of apple and potato were studied and the model was validated with the help of existing experimental data. A good agreement between predicted values and experimental data was obtained. It was reported that this strain energy function is appropriate to study the mechanical properties of apple and potato. Therefore, in the present work, the strain energy function given in Eq. (8) has been used for the development of the desired relation. Further, by considering the range of pressure given in literature (Krokida et al. 1999, 2000), the values of porosity are calculated from Eq. (25) for various drying methods. The obtained values of porosity are checked and found in the range which is given in literature (Krokida and Maroulis 1997; Wang and Brennan 1995; Zogzas et al. 1994). Therefore, it can be concluded that the developed relation in the present study is appropriate for study the porosity behavior as a function of pressure.
Porosity variation in different drying methods
By using Eq. (25), the values of current porosity are calculated for the different values of pressure. For different drying methods, the behavior of porosity as a function of initial porosity is presented in Figs. 4, 5, 6, 7 and 8 for apple and Figs. 9, 10, 11, 12 and 13 for potato. Before starting the drying process, when there is no moisture loss, the porosity (i.e. the initial porosity) is shown in Figs. 4, 5, 6, 7, 8, 9, 10, 11, 12 and 13 corresponding to pressure p = 0. As drying proceeds, pressure increases by water loss as a result current porosity increases. Further, current porosity also increases for increasing values of initial porosity during dehydration in these products.
Fig. 4.
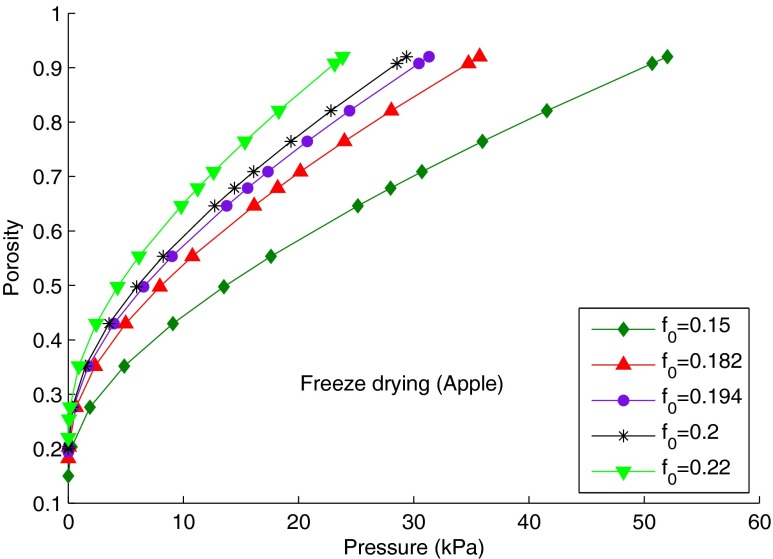
Porosity variation with respect to initial porosity and pressure developed due to water removal during freeze drying of apple
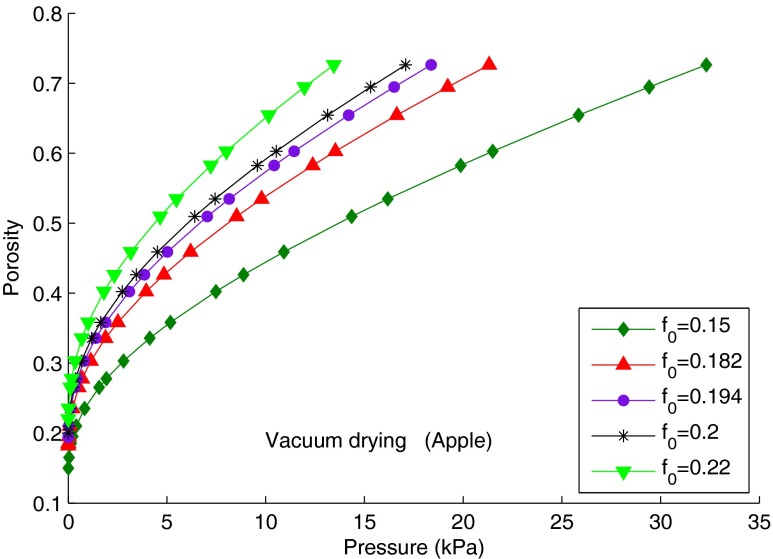
Fig. 5.
Porosity variation with respect to initial porosity and pressure developed due to water removal during vacuum drying of apple
Fig. 6.
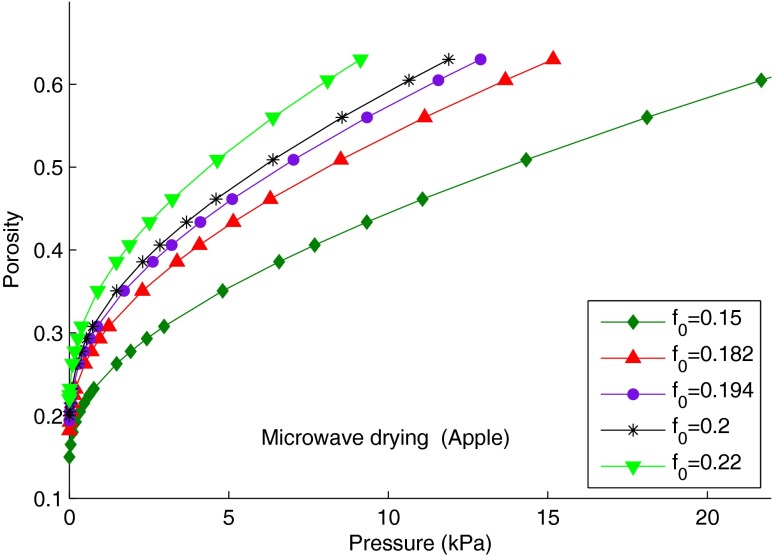
Porosity variation with respect to initial porosity and pressure developed due to water removal during microwave drying of apple
Fig. 7.
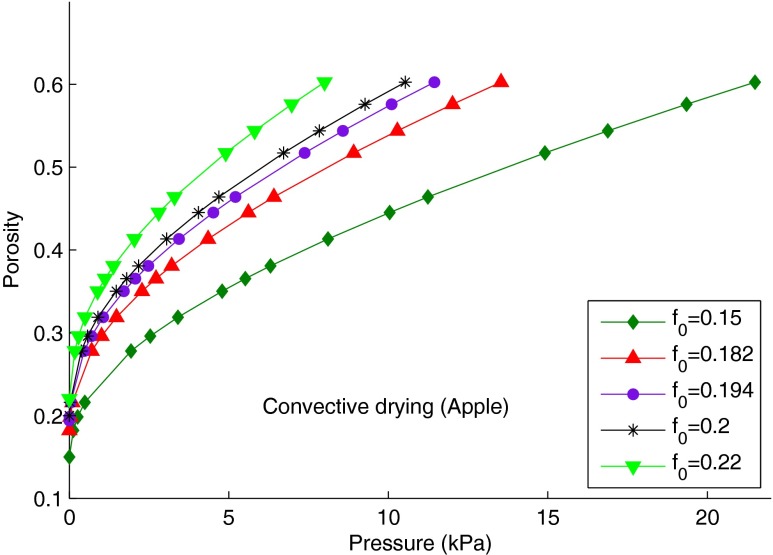
Porosity variation with respect to initial porosity and pressure developed due to water removal during convective drying of apple
Fig. 9.
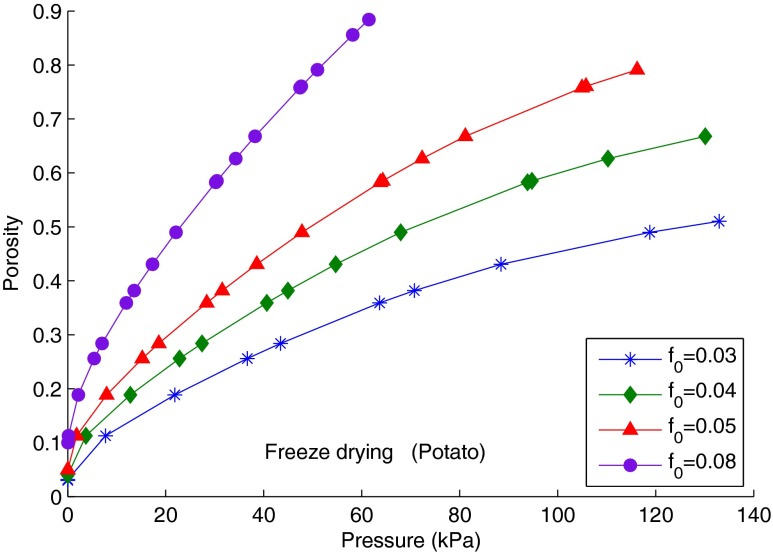
Porosity variation with respect to initial porosity and pressure developed due to water removal during freeze drying of potato
Fig. 10.
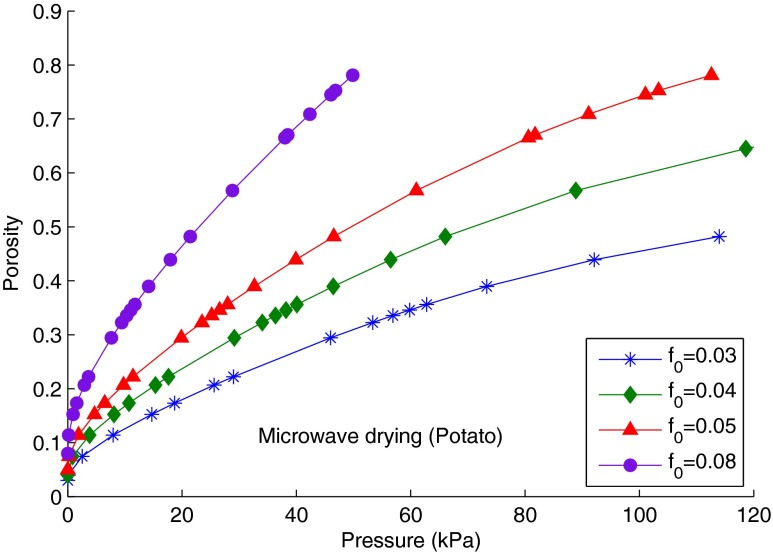
Porosity variation with respect to initial porosity and pressure developed due to water removal during microwave drying of potato
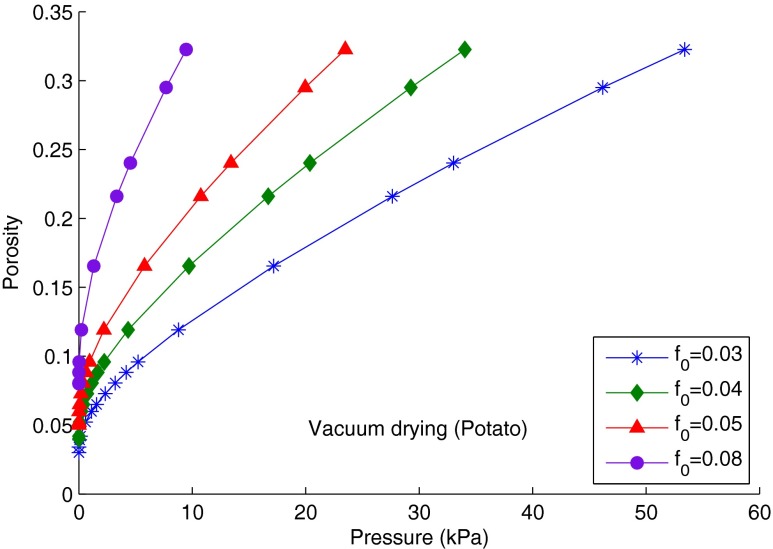
Fig. 11.
Porosity variation with respect to initial porosity and pressure developed due to water removal during vacuum drying of potato
Fig. 12.
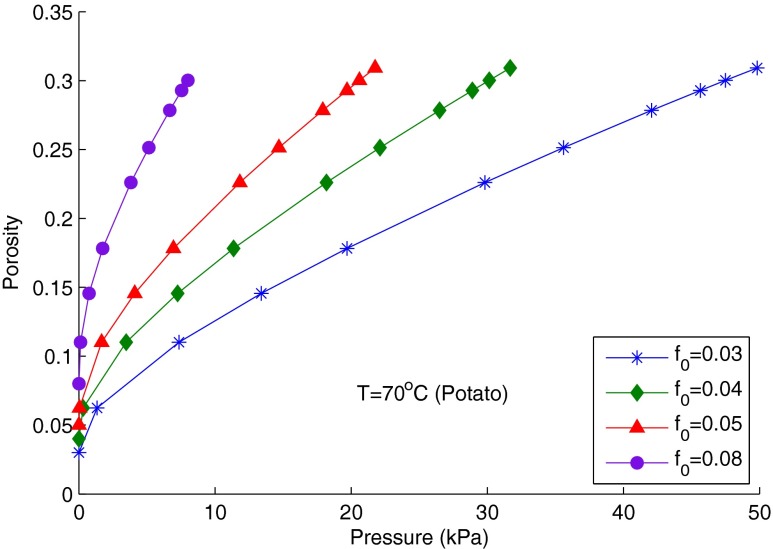
Porosity variation with respect to initial porosity and pressure developed due to water removal during drying potato at 70 °C
Fig. 8.
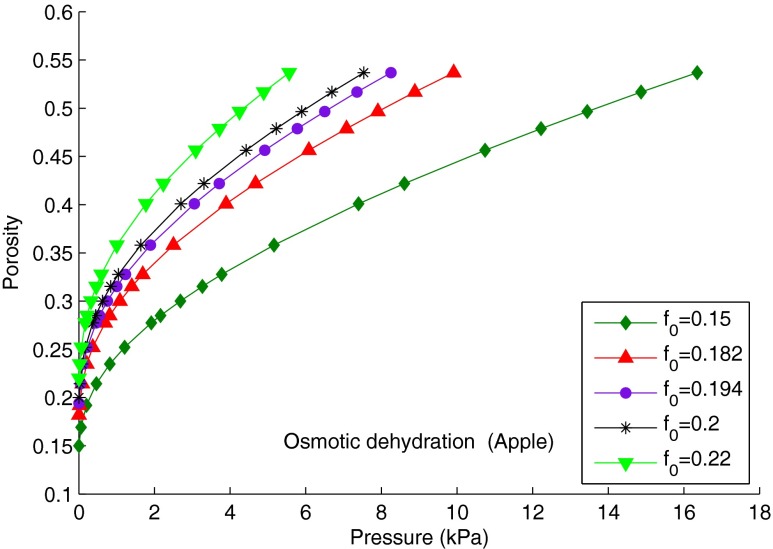
Porosity variation with respect to initial porosity and pressure developed due to water removal during osmotic dehydration of apple
Fig. 13.
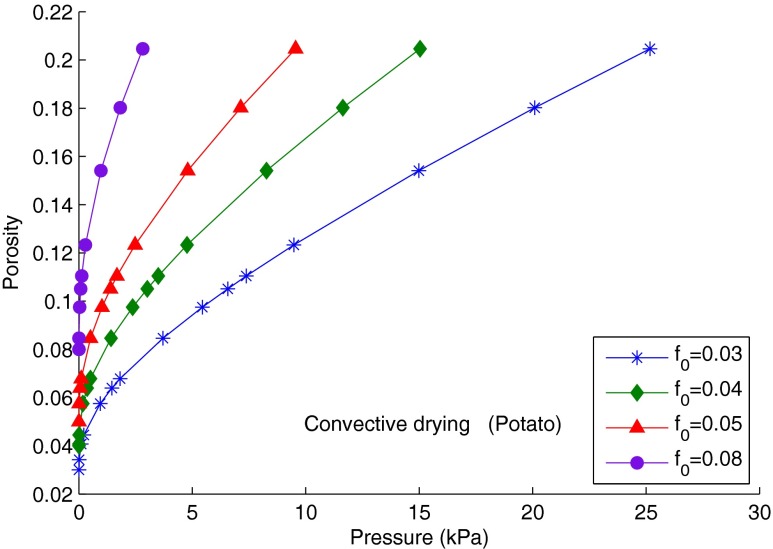
Porosity variation with respect to initial porosity and pressure developed due to water removal during convective drying of potato
Porosity variation in apple
The variation in current porosity with respect to initial porosity and pressure is depicted in Figs. 4, 5, 6, 7 and 8 for freeze drying, vacuum drying, microwave drying, convective drying and osmotic dehydration, respectively. It can be seen from the figures that the current porosity ranges from 0.15 to 0.93 in case of freeze drying; from 0.15 to 0.72 in case of vacuum drying; from 0.15 to 0.63 in case of microwave drying; from 0.15 to 0.60 in case of convective drying and from 0.15 to 0.54 in case of osmotic dehydration. It is clear from the figures that the initial porosity and pressure have significant effects on the porosity variation for all the drying methods discussed above. Further, the porosity is high in freeze drying, whereas it is low in osmotic dehydration. The high porosity in freeze drying of foodstuffs is the consequence of absence of capillary forces during sublimation of the frozen solvent (Madiouli et al. 2012). In the different drying methods, maximum value of generated pressure is different. It is maximum in freeze drying whereas minimum in osmotic dehydration.
The above-mentioned range of porosity for apple is reported in literature (Krokida and Maroulis 2001; Oikonomopoulou and Krokida 2013) for different drying methods, temperature and conditions. The continuous process of drying caused the increase in porosity with pressure, as expected. The rate of increment of porosity is depending on the initial porosity and it is high for the greater value of the initial porosity. It is also obvious from the figures that for the high value of initial porosity, the less amount of pressure is sufficient to attain the greater value of current porosity.
In the freeze drying when the initial porosity is 0.15, the value of pressure is very high (Fig. 4). In the initial hours of drying of these products, current porosity increases rapidly (even for the small values of pressure), however, the increment become slow for latter drying hours. As the initial porosity increases, the generated pressure is low comparatively for reaching the highest value of porosity. In the vacuum drying, values of pressure and current porosity are low as compare to freezing drying for the same value of initial porosity (Fig. 5). Further, as initial porosity increases, the value of pressure decreases. In the microwave and convective drying, the values of pressure and current porosity are low as compare to vacuum drying (Figs. 6 and 7). Again, the value of pressure decreases as initial porosity increases; correspondingly, current porosity increases. The values of pressure and current porosity are low as compare to microwave and convective drying in case of osmotic dehydration (Fig. 8). Current porosity and generated pressure are depending on the initial porosity.
In the early stage of drying, the porosity increases rapidly for all values of the initial porosity and after that its increasing rate became slow. The open space in the structure formed by the water evaporation is caused of the incapability of structure to collapse perfectly as water evaporated (Karathanos et al. 1996). The cause for collapse may be the interfacial tension of tissue’s walls filled with water.
Porosity variation in potato
The current porosity and generated pressure in potato are shown in Figs. 9, 10, 11, 12 and 13 for freeze drying, microwave drying, vacuum drying, drying at temperature 70 °C and convective drying, respectively, for different values of initial porosity. For potato, current porosity ranges from 0.03 to 0.88 in case of freeze drying; from 0.03 to 0.78 in case for microwave drying; from 0.03 to 0.32 in case of vacuum drying; from 0.03 to 0.31 in case of drying at temperature 70 °C and from 0.03 to 0.20 in case of convective drying for the given values of pressure. This range of porosity for potato is reported in literature (Krokida and Maroulis 2001; Oikonomopoulou and Krokida 2013) for different drying methods, temperature and conditions. As the drying process proceeds, pressure on the spherical surface (or contraction stress in cells/tissues) increases that caused the increase in porosity.
The value of current porosity is highest in freezing drying (Fig. 9) whereas it is lowest in convective drying (Fig. 13). For the initial porosity 0.03 and 0.04, the value of pressure is very large. However, as initial porosity increases, the value of pressure decreases. Similar behavior of porosity and pressure can be seen in case of microwave drying (Fig. 10); however, the values of these parameters are less as compare to freezing drying. The current porosity increases rapidly in starting drying hours of potato in case of vacuum drying, convective drying and drying at temperature 70 °C for initial porosities 0.05 and 0.08, whereas, its value is lower for initial porosities 0.03 and 0.04. The values of porosity and pressure in vacuum drying are higher than convective and at 70 °C drying but lower than freeze and microwave drying.
The porosity characteristic presented the nonlinear variation with respect to pressure, as expressed in the form of Eq. (25). Further, as can be seen from Figs. 9, 10, 11, 12 and 13 that current porosity is depending on the initial porosity and rapidly increases for slight change in initial porosity. It is also observed that potato is more sensitive than apple against drying temperature. The rate of porosity increment in potato drying is high as compare to apple drying. The possible reason of the formation of porosity in foodstuffs explained by Krokida et al. (1997) is given as: in the initial hours of drying, cellular tissues are sufficiently elastic to shrink into the space left by the evaporated water. As the drying process proceeds, the water is replaced by air and, hence, structural changes occur in the cellular tissue that results in a more rigid skeleton, thus favoring the development of porosity.
Comparison of different drying techniques
The understanding of pore formation process in food products during storage and processing is necessary for the characterization and process design of the final processed product with desired properties. Several drying methods are generally liable for formation of different porous micro - and macrostructures in a different way due to different process parameters.
During freeze drying of apple and potato, internal water from these products removed in two steps: in the first step, product is frozen by keeping pressure and temperature low and then water is removed by sublimation. In the vacuum drying method, drying is performed using low temperature and different pressure. In the microwave drying method, heat inside the material is generated using electromagnetic waves. This method is applied by food industries to dry the products at the reduced drying time using dielectric heating with microwaves. Convective air drying is the most widely applied method for food dehydration. In this method, high temperature is applied for drying food products and the moisture is removed from the center to the surface of the product. In the osmotic dehydration method, apple and potato is immersed in the concentrated solutions, as a result, products of intermediate moisture content obtain due to natural water removal. The details of various drying technique can be found in literature (Krokida and Maroulis 2001; Oikonomopoulou and Krokida 2013).
The drying methods have great effect on the porosity of apple and potato. The final porosity for apple is the highest (92 %) in freeze drying (Fig. 4) and the lowest (54 %) in osmotic dehydration (Fig. 8). The higher porosity value for apple means that the greater volume of pores is formed during freeze drying. The larger pores creation is the consequence of larger ice crystal development during freeze drying. Further, the porosity of apple during other drying technique such as vacuum drying, microwave drying and convective drying is 73 %, 63 % and 60 %, respectively. It is clear that the microwave dried apple developed lower porosity than vacuum dried apple and greater porosity than convective dried apple (Figs. 5, 6 and 7). Similar results for apple porosity were reported by (Krokida and Maroulis 1999).
In the case of potato drying, the freeze dried potato (Fig. 9) developed the highest porosity (89 %), whereas the lowest porosity (21 %) is obtained in convective drying (Fig. 13). Result of the lowest porosity in convective drying method is supported by (Zogzas et al. 1994). For the other drying methods, for example microwave drying, vacuum drying and air drying at temperature 70 °C, the porosity of potato is 78 %, 33 % and 31 %, respectively. The porosity of vacuum dried potato is observed greater than the porosity of air dried potato and lower than the porosity of microwave dried potato (Figs. 10, 11 and 12). Similar results were reported by (Krokida and Maroulis 1999, 2001) for porosity of potato.
It is evident from the results discussed above for porosity of apple and potato that dried apple developed higher porosity than dried potato in freeze drying, vacuum drying and convective drying. This means that the apple is more capable to shrink as compare to potato. These results may be helpful for food drying industries to choose an appropriate drying technique to obtain the desired quality, appearance and texture of final product.
General remarks
Increment in contraction stress (pressure) in cells of apple and potato due to water removal is practically valid. Therefore, the present study justified the physical behavior of apple and potato in respect of change of porosity and pressure during dehydration. From above discussion, it is clear that calculated values of porosity (Figs. 4, 5, 6, 7, 8 and 9, 10, 11, 12 and 13) are similar to the experimental values of porosity (Figs. 2 and 3) for both apple and potato, respectively. However, the nature of the graphs is seems different because of the different behavior of porosity with respect to moisture content and pressure. Further, it is difficult to comprise the experimental and calculated values of porosity through the same graph due to different scale of porosity-moisture content and porosity-pressure measure.
Besides aforementioned drying (methods, conditions, temperature etc.), moisture removal also comes into the picture during postharvest handling, storage and transportation of apple and potato (in general fruits and vegetables). The processes of moisture loss from fresh fruits and vegetables termed as transpiration. The transpiration process includes the evaporation of moisture from the surface of the fruits or vegetables to the surrounding air during storage (Chakraverty et al. 2003). Due to this process, moisture loss induces shrinkage, increase in porosity, wilting, and loss of firmness and crispness of apple and potato, and thus contrarily affects the texture, flavor, appearance, and the mass of these products. During storage, the formation of pores in fresh apple and potato is the consequence of water removal that is largely dependent on the air velocity, relative humidity, and the heat of respiration and bulk density. This loss of moisture can be reduced by minimizing the water vapor pressure difference between the air and the produce and hence porosity increment can be minimized (Chakraverty et al. 2003). Additionally, at packaging time, various moisture-proof films and skin coatings can be used. As a result, mass loss and shrinkage can be reduced in these produce that caused an improvement in firmness, appearance and overall storage life. Furthermore, the textural and sensory properties should be controlled which changes the consumer’s opinion regarding food quality.
Conclusions
In the present work, a mathematical relation for the prediction of porosity (volume fraction of pores) variation in apple and potato is developed as the function of initial porosity and pressure which is (responsible for the counterbalancing forces) developed on the surface of porous spherical structure during dehydration. Current porosity is largely depended on the initial porosity. For the different values of initial porosity, the effect of pressure on the current porosity is obtained. The obtained porosity range for apple and potato is satisfactory and agreed by the previously published experimental observations. Additionally, due to close resemblance between experimental and theoretical results, the developed mathematical relation is appropriate for the study of porosity behavior of apple and potato. The developed relation may be helpful in further studies for the calculation of porosity and structural properties of apples and potatoes, and furthermore, it can be extended to examine the porosity behavior for other fruits and vegetables during dehydration.
Acknowledgments
One of the authors is very thankful to Council of Scientific & Industrial Research (CSIR) New Delhi, India for providing financial support during preparation of this manuscript. Authors also wishes to acknowledge Mrs. Ravita for her useful help during the development of this work.
A bold faced quantity denotes a vector or a tensor
- A0
Radius of pores (internal radius) in undeformed configuration (μm)
- B0
Radius of bulk material (external radius) in undeformed configuration (μm)
- A
Radius of pores (internal radius) in deformed configuration (μm)
- B
Radius of bulk material (external radius) in deformed configuration (μm)
- C
Constant of integration
- F
Function
- F
Deformation gradient tensor
- x(x1, x2, x3)
Particle position vector in undeformed configuration
- y(y1, y2, y3)
Particle position vector in deformed configuration
- R
Particle distance from the origin in undeformed configuration
- r
Particle distance from the origin in deformed configuratio
- (R, Θ, Φ)
Particle position in undeformed configuration
- (r, θ, φ)
Particle position in deformed configuration
- W
Strain energy function
- b, C1, C2
Material constants
- B
Left Cauchy-Green deformation tensor
- V
Left stretching tensor
- I1 , I2 , I3
Invariants of left Cauchy-Green deformation tensor
- λr , λθ , λφ
Principal stretches
- τ
Cauchy stress tensor
- τrr , τθθ
Principal component of Cauchy stress
- ρ
Hydrostatic pressure (kPa)
- p
Pressure (a contraction stress generated in the cells due to water removal) (kPa)
- f0
Initial porosity (volume fraction of pores)
- f
Current porosity (volume fraction of pores)
- δij
Kronecker delta
Appendix A
In the terms of components, the deformation gradient tensor can be written as
| A1 |
where y = (y1, y2, y3), x = (x1, x2, x3), and
| A2 |
on substituting Eq. (A2) in Eq. (A1) and after some simplifications we can find
| A3 |
By using incompressibility condition Det(F) = 1 (from Eq. 5), Eq. (A3) can be solved to get
| A4 |
Appendix B
From Eq. (5) we have B3 − B30 = A3 − A30, substituting the value of A3 − A30 in Eq. (13), we can get
| B1 |
Now, for r = B, Eq. (B1) reduced to
| B2 |
Again, substituting the value of B from Eq. (5) into Eq. (B2)
| B3 |
References
- Bai Y, Rahman MS, Perera CO, Smith B, Melton LD. Structural changes in apple rings during convection air-drying with controlled temperature and humidity. J Agric Food Chem. 2002;50:3179–3185. doi: 10.1021/jf011354s. [DOI] [PubMed] [Google Scholar]
- Ball JM. Discontinuous equilibrium solutions and cavitation in nonlinear elasticity. Philos Trans R Soc A. 1982;306:557–611. doi: 10.1098/rsta.1982.0095. [DOI] [Google Scholar]
- Chakraverty A, Mujumdar AS, Raghavan GSV, Ramaswamy HS. Handbook of postharvest technology: cereals, fruits, vegetables, tea, and spices. New York: CRC Press; 2003. [Google Scholar]
- Chang CS. Measuring density and porosity of grain kernels using a gas pycnometer. Cereal Chem. 1988;65:13–15. [Google Scholar]
- Chang WJ, Pan J. Cavitation instabilities in plastics and rubber-modified plastics. Int J Fract. 1997;88:61–86. doi: 10.1023/A:1007446104430. [DOI] [Google Scholar]
- Chang WJ, Pan J. Cavitation instability in rubber with consideration of failure. J Mater Sci. 2001;36:1901–1909. doi: 10.1023/A:1017581420879. [DOI] [Google Scholar]
- Dissa AO, Desmorieux H, Savadogo PW, Segda BG, Koulidiati J. Shrinkage, porosity and density behaviour during convective drying of spirulina. J Food Eng. 2010;97:410–418. doi: 10.1016/j.jfoodeng.2009.10.036. [DOI] [Google Scholar]
- Holzapfel GA. Nonlinear solid mechanics: a continuum approach for engineering. Chichester: Wiley; 2000. [Google Scholar]
- Hou HS. Cavitation instability in solids, solid mechanics. China: Zhejiang University Hangzhou; 1990. [Google Scholar]
- Joardder MUH, Karim A, Kumar C (2014) Brown RJ Effect of cell wall properties of plant tissue on porosity and shrinkage of dried apple. In: Proceedings of the 2014 International Conference on Food Properties (ICFP2014)
- Karathanos VT, Saravacos GD (1993) Porosity and pore size distribution of starch materials. J Food Eng 18:259–280 doi:10.1016/0260-8774(93)90090-7
- Karathanos VT, Kanellopoulos NK, Belessiotis VG. Development of porous structure during air drying of agricultural plant products. J Food Eng. 1996;29:167–183. doi: 10.1016/0260-8774(95)00058-5. [DOI] [Google Scholar]
- Katekawa ME, Silva MA (2004) Study of porosity behavior in convective drying of bananas. In: Proceedings of the 14th. International Drying Symposium (IDS 2004), Sao-Paulo, Brazil, 22–25 August 2004. pp 1427–1434
- Khalloufi S, Almeida-Rivera C, Jansen J, Van-Der-Vaart M, Peter Bongers P (2011) Sensitivity of shrinkage and collapse functions involved in pore formation during drying. Paper presented at the 21st European Symposium on Computer Aided Process Engineering – ESCAPE 21
- Koç B, Eren İ, Kaymak Ertekin F. Modelling bulk density, porosity and shrinkage of quince during drying: the effect of drying method. J Food Eng. 2008;85:340–349. doi: 10.1016/j.jfoodeng.2007.07.030. [DOI] [Google Scholar]
- Krokida MK, Maroulis ZB. Effect of drying method on shrinkage and porosity. Dry Technol. 1997;15:2441–2458. doi: 10.1080/07373939708917369. [DOI] [Google Scholar]
- Krokida MK, Maroulis ZB. Effect of microwave drying on some quality properties of dehydrated products. Dry Technol. 1999;17:449–466. doi: 10.1080/07373939908917545. [DOI] [Google Scholar]
- Krokida M, Maroulis Z. Quality changes during drying of food materials. In: Mujumdar AS, editor. Drying technology in agriculture and food science. USA: Science Publishers; 2001. [Google Scholar]
- Krokida MK, Zogzas NP, Maroulis ZB. Modelling shrinkage and porosity during vacuum dehydration. Int J Food Sci Technol. 1997;32:445–458. doi: 10.1111/j.1365-2621.1997.tb02119.x. [DOI] [Google Scholar]
- Krokida MK, Karathanos VT, Maroulis ZB. Effect of freeze-drying conditions on shrinkage and porosity of dehydrated agricultural products. J Food Eng. 1998;35:369–380. doi: 10.1016/S0260-8774(98)00031-4. [DOI] [Google Scholar]
- Krokida MK, Kiranoudis CT, Maroulis ZB. Viscoelastic behaviour of dehydrated products during rehydration. J Food Eng. 1999;40:269–277. doi: 10.1016/S0260-8774(99)00063-1. [DOI] [Google Scholar]
- Krokida MK, Karathanos VT, Maroulis ZB. Effect of osmotic dehydration on viscoelastic properties of apple and banana. Dry Technol. 2000;18:951–966. doi: 10.1080/07373930008917746. [DOI] [Google Scholar]
- Liu G, Chen J, Liu M, Wan X. Shrinkage, porosity and density behaviour during convective drying of bio-porous material. Procedia Eng. 2012;31:634–640. doi: 10.1016/j.proeng.2012.01.1078. [DOI] [Google Scholar]
- Lopez-Pamies O, Idiart MI, Nakamura T. Cavitation in elastomeric solids: I—a defect-growth theory. J Mech Phys Solids. 2011;59:1464–1487. doi: 10.1016/j.jmps.2011.04.015. [DOI] [Google Scholar]
- Lozano JE, Rotstein E, Urbicain MJ. Total porosity and open-pore porosity in the drying of fruits. J Food Sci. 1980;45:1403–1407. doi: 10.1111/j.1365-2621.1980.tb06564.x. [DOI] [Google Scholar]
- Lozano JE, Rotstein E, Urbicain MJ (1983) Shrinkage, porosity and bulk-density of foodstuffs at changing moisture contents. J Food Sci 48:1497–1502 & 1553 doi: 10.1111/j.1365-2621.1983.tb03524.x
- Madiouli J, Lecomte D, Nganya T, Chavez S, Sghaier J, Sammouda H. A method for determination of porosity change from shrinkage curves of deformable materials. Dry Technol. 2007;25:621–628. doi: 10.1080/07373930701227185. [DOI] [Google Scholar]
- Madiouli J, Sghaier J, Lecomte D, Sammouda H. Determination of porosity change from shrinkage curves during drying of food material. Food Bioprod Process. 2012;90:43–51. doi: 10.1016/j.fbp.2010.12.002. [DOI] [Google Scholar]
- Mattea M, Urbicain MJ, Rotstein E. Computer-model of shrinkage and deformation of cellular tissue during dehydration. Chem Eng Sci. 1989;44:2853–2859. doi: 10.1016/0009-2509(89)85095-X. [DOI] [Google Scholar]
- Mavroudis NE, Gekas V, Sjoholm I. Osmotic dehydration of apples. Shrinkage phenomena and the significance of initial structure on mass transfer rates. J Food Eng. 1998;38:101–123. doi: 10.1016/S0260-8774(98)00090-9. [DOI] [Google Scholar]
- May BK, Perre P. The importance of considering exchange surface area reduction to exhibit a constant drying flux period in foodstuffs. J Food Eng. 2002;54:271–282. doi: 10.1016/S0260-8774(01)00213-8. [DOI] [Google Scholar]
- Mayor L, Sereno AM. Modelling shrinkage during convective drying of food materials: a review. J Food Eng. 2004;61:373–386. doi: 10.1016/S0260-8774(03)00144-4. [DOI] [Google Scholar]
- Mayor L, Pissarra J, Sereno AM. Microstructural changes during osmotic dehydration of parenchymatic pumpkin tissue. J Food Eng. 2008;85:326–339. doi: 10.1016/j.jfoodeng.2007.06.038. [DOI] [Google Scholar]
- McMinn WAM, Magee TRA. Physical characteristics of dehydrated potatoes - part I. J Food Eng. 1997;33:37–48. doi: 10.1016/S0260-8774(97)00039-3. [DOI] [Google Scholar]
- Murphy JG, Biwa S. Nonmonotonic cavity growth in finite, compressible elasticity. Int J Solids Struct. 1997;34:3859–3872. doi: 10.1016/S0020-7683(96)00237-5. [DOI] [Google Scholar]
- Nieto AB, Salvatori DM, Castro MA, Alzamora SM. Structural changes in apple tissue during glucose and sucrose osmotic dehydration: shrinkage, porosity, density and microscopic features. J Food Eng. 2004;61:269–278. doi: 10.1016/S0260-8774(03)00108-0. [DOI] [Google Scholar]
- Oikonomopoulou VP, Krokida MK. Structural properties of dried potatoes, mushrooms, and strawberries as a function of freeze-drying pressure. Dry Technol. 2012;30:351–361. doi: 10.1080/07373937.2011.639475. [DOI] [Google Scholar]
- Oikonomopoulou VP, Krokida MK. Novel aspects of formation of food structure during drying. Dry Technol. 2013;31:990–1007. doi: 10.1080/07373937.2013.771186. [DOI] [Google Scholar]
- Polignone DA, Horgan CO. Cavitation for incompressible anisotropic nonlinearly elastic spheres. J Elast. 1993;33:27–65. doi: 10.1007/BF00042634. [DOI] [Google Scholar]
- Rahman MS. Toward prediction of porosity in foods during drying: a brief review. Dry Technol. 2001;19:1–13. doi: 10.1081/DRT-100001349. [DOI] [Google Scholar]
- Rahman MS. A theoretical model to predict the formation of pores in foods during drying. Int J Food Prop. 2003;6:61–72. doi: 10.1081/JFP-120016624. [DOI] [Google Scholar]
- Rahman MS. Food properties handbook. 2. New York: CRC Press, Taylor & Francis Group, LLC; 2008. [Google Scholar]
- Rahman MS, Perera CO, Chen XD, Driscoll RH, Potluri PL. Density, shrinkage and porosity of calamari mantle meat during air drying in a cabinet dryer as a function of water content. J Food Eng. 1996;30:135–145. doi: 10.1016/S0260-8774(96)00013-1. [DOI] [Google Scholar]
- Rahman MS, Al-Zakwani I, Guizani N. Pore formation in apple during air-drying as a function of temperature: porosity and pore-size distribution. J Sci Food Agric. 2005;85:979–989. doi: 10.1002/jsfa.2056. [DOI] [Google Scholar]
- Ratti C. Shrinkage during drying of foodstuffs. J Food Eng. 1994;23:91–105. doi: 10.1016/0260-8774(94)90125-2. [DOI] [Google Scholar]
- Sablani SS, Rahman MS. Pore formation in selected foods as a function of shelf temperature during freeze drying. Dry Technol. 2002;20:1379–1391. doi: 10.1081/DRT-120005857. [DOI] [Google Scholar]
- Sahin S, Sumnu SG. Physical properties of foods. New York: Springer Science + Business Media, LLC.; 2006. [Google Scholar]
- Singh F, Katiyar VK, Singh BP. A new strain energy function to characterize apple and potato tissues. J Food Eng. 2013;118:178–187. doi: 10.1016/j.jfoodeng.2013.04.006. [DOI] [Google Scholar]
- Singh F, Katiyar VK, Singh BP. Analytical study of turgor pressure in apple and potato tissues. Postharvest Biol Technol. 2014;89:44–48. doi: 10.1016/j.postharvbio.2013.11.007. [DOI] [Google Scholar]
- Sinha NK. Handbook of vegetables and vegetable processing. USA: Wiley; 2011. [Google Scholar]
- Sivaloganathan J. Uniqueness of regular and singular equilibria for spherically symmetric problems of nonlinear elasticity. Arch Ration Mech Anal. 1986;96:97–136. doi: 10.1007/BF00251407. [DOI] [Google Scholar]
- Suzuki K, Kubota K, Hasegawa T, Hosaka H. Shrinkage in dehydration of root vegetables. J Food Sci. 1976;41:1189–1193. doi: 10.1111/j.1365-2621.1976.tb14414.x. [DOI] [Google Scholar]
- Wang N, Brennan JG. Changes in structure, density and porosity of potato during dehydration. J Food Eng. 1995;24:61–76. doi: 10.1016/0260-8774(94)P1608-Z. [DOI] [Google Scholar]
- Weinberg IK (2007) Material modeling of microstructured solids, theory, numeric and applications. Technical University of Berlin
- Zogzas NP, Maroulis ZB, Marinoskouris D. Densities, shrinkage and porosity of some vegetables during air-drying. Dry Technol. 1994;12:1653–1666. doi: 10.1080/07373939408962191. [DOI] [Google Scholar]