Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2015 Oct 1;5:14604. doi: 10.1038/srep14604

Stroboscopic phenomena in superconductors with dynamic pinning landscape

Ž L Jelić 1,2,a, M V Milošević 1,b, J Van de Vondel 3, A V Silhanek 2
PMCID: PMC4589687  PMID: 26423610

Abstract

Introducing artificial pinning centers is a well established strategy to trap quantum vortices and increase the maximal magnetic field and applied electric current that a superconductor can sustain without dissipation. In case of spatially periodic pinning, a clear enhancement of the superconducting critical current arises when commensurability between the vortex configurations and the pinning landscape occurs. With recent achievements in (ultrafast) optics and nanoengineered plasmonics it has become possible to exploit the interaction of light with superconductivity, and create not only spatially periodic imprints on the superconducting condensate, but also temporally periodic ones. Here we show that in the latter case, temporal matching phenomena develop, caused by stroboscopic commensurability between the characteristic frequency of the vortex motion under applied current and the frequency of the dynamic pinning. The matching resonances persist in a broad parameter space, including magnetic field, driving current, or material purity, giving rise to unusual features such as externally variable resistance/impedance and Shapiro steps in current-voltage characteristics. All features are tunable by the frequency of the dynamic pinning landscape. These findings open further exploration avenues for using flashing, spatially engineered, and/or mobile excitations on superconductors, permitting us to achieve advanced functionalities.

A type-II superconductor can allow penetration of external magnetic field in terms of vortices, quantized filaments of magnetic flux inside which superconductivity is locally depleted. The whirl of superconducting current around this topological defect, responsible for its name, interacts with an applied electric current causing the motion of vortices in a manner analogous to the Lorentz force1. Unfortunately, this dissipative motion results in progressive Joule heating and quenching of superconductivity.

Over the past decades, the main strategy to prevent (or at least decrease) the described energy dissipation was to artificially anchor the vortices, using specifically designed arrays of pinning centers. In that respect, many alternatives were employed—e.g. pinning centers produced by irradiation with heavy ions2, chemically grown defects3, nanostructured perforations4, or permanent nanomagnets5. Regardless of the exact nature of pinning, the common idea has always been to create a spatial inhomogeneity in the superconducting condensate, i.e. locally suppress superconductivity on a scale comparable to the size of the vortex core. If then a periodic arrangement of the pinning centers is made, the superconductor can sustain a particularly high critical current at so-called matching fields, where the ideally homogeneous lattice of vortices is interlocked with the periodic lattice of the pinning centers6,7. Properties of such periodical pinning landscapes have been extensively investigated in the literature, with particular attention on the appearance of resonant features in the presence of the ac force8,9,10,11,12.

To date, all used pinning strategies involved an energy landscape imposed on permanent basis—i.e. neither its intensity nor its spatial distribution could be further modified. The question arises—how would a dynamic pinning landscape affect the superconducting properties? Clearly, the added degrees of freedom in terms of frequency and strength of the pinning, as well as its possible mobility and speed, next to its spatial geometry, could trigger new phenomena unattainable with static pinning. Such a study is not only interesting but also timely, since such dynamic and highly controllable pinning potential can now be achieved, for instance, by inhomogeneous light distribution (created by arrays of lasers13 or plasmonic nanostructures14,15,16) or by intensity modulation of the laser light.

The interaction of light with superconductivity has been of interest for decades, and it is now very well established that in most cases it leads to local heating and depletion of the superconducting condensate (a feature employed in superconducting single-photon detectors17,18,19,20,21), though at frequencies close to the superconducting gap light can interfere with the recombination of Cooper-pairs and even enhance superconductivity22. Interaction of laser light with vortices has also been studied, to form the base of the modern imaging technique of low-temperature laser scanning microscopy (LTLSM)23. However, light as a source of a time-dependent pinning potential in superconductors has not been explored to date, although it has been utilized for dynamic optical scanning in Bose-Einstein condensates (BECs)24, and for flashing ratchets in colloidal systems25,26,27,28,29,30,31.

Therefore, in this work, we investigate the fundamental consequences of a temporally periodic pinning landscape imprinted on a superconducting condensate. As a simple but exemplary case, we consider a superconducting stripe with longitudinally applied current, in magnetic field perpendicular to its plane, and with an oscillating depletion line along its middle (depicted in Fig. 1). We focus on the range of currents and fields where vortex motion induces a ‘resistive’ state32,33,34, and show the rich emergent phenomena as a function of the frequency of the pinning landscape.

Figure 1. Layout of the studied system.

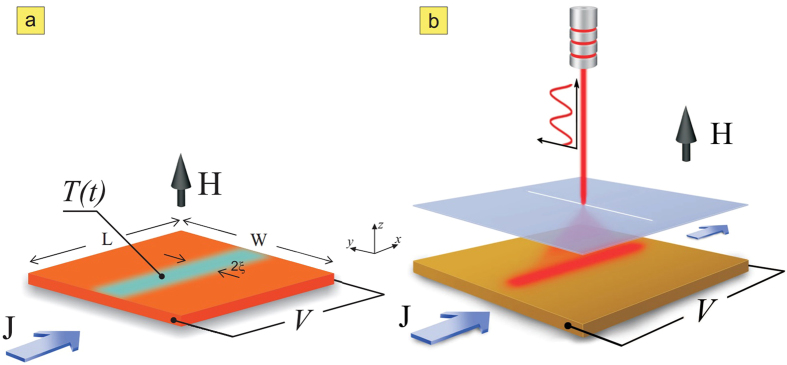
Figure 1

Open in a new tab

(a) A superconducting stripe of width W with a central, time-dependent, pinning line of width comparable to the vortex size (ξ is the superconducting coherence length), and a 4-point probe applied dc current (with density J), and measured voltage (V, at contacts separated by distance L). A depletion region is simulated as sinusoidally oscillating local heating up to the critical temperature Tc and back to working temperature, with frequency ω (see Methods). (b) Possible experimental setup corresponding to (a), by using a laser light at the far field passing through a metallic mask to create time-dependent and spatially modulated depletion of the superconducting condensate.

Results

What phenomenology should one expect from the frequency dependence of the pinning landscape? Clearly, if the change between the ON and OFF state of the pinning potential (further denoted as period τ) is faster than the characteristic relaxation time of the superconducting order parameter (i.e. τ ≤ τGL, where τGL is the Ginzburg-Landau relaxation time, see Methods), then a recovery of the superconducting condensate is not possible—leading to permanent depletion and effectively static pinning. When the frequency of pinning oscillations is below this ultrafast limit, but still faster than the vortex velocity (or in terms of the characteristic time scales, 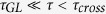Inline graphic, where τcross is the average time needed for a vortex to cross the pinning-free sample at certain magnetic field and applied current), there will be a noticeable change. Even though superconductivity has sufficient time to fully recover over one pinning cycle, the duration of the ON state is still not long enough to fully trap the moving vortex, hence the interaction between the flux quanta and the dynamic pinning can be considered weak (we refer to this regime as ‘vortex tapping’). At further decreased pinning frequencies, where τ becomes comparable with τcross, one reaches the ‘vortex pinning’ regime where the motion of the flux quanta is greatly influenced by the variations in the pinning landscape. This is the richest part of the frequency phase diagram, and the main focus of our study. Finally, in the limit where τ→∞, the system becomes a simple alternation of two long-lasting states: the one where the pinning is OFF and the structure behaves as the pinning-free sample, and the second one with the pinning ON behaving as the static case extensively studied in literature.

Stroboscopic resonances

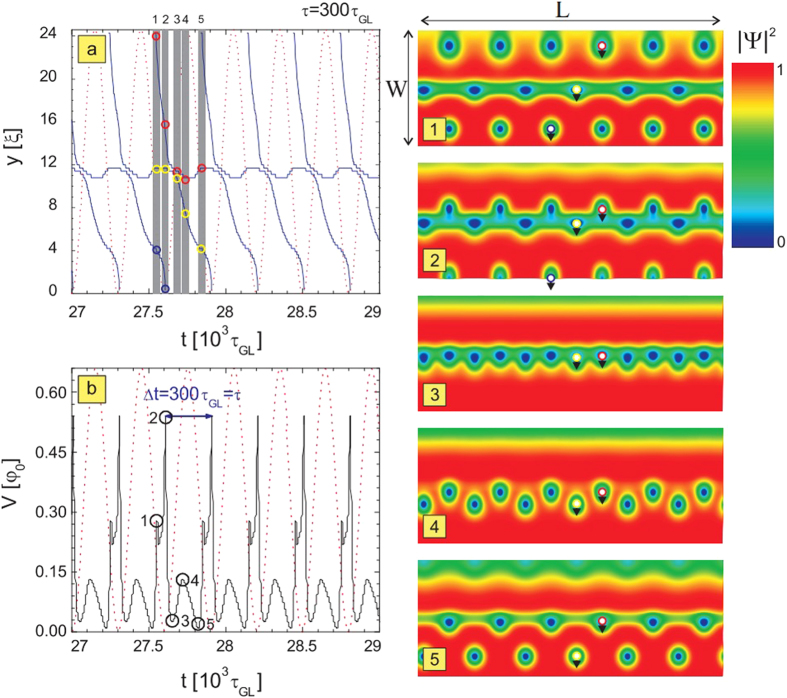
In what follows, we study in detail the regimes where pinning landscape and moving vortices interact most. In Fig. 2, we present the diagram of the calculated voltage on the sample (with taken size L × W = 72ξ × 24ξ, sample is periodic in L, see Fig. 1 and Methods) as a function of the period of the pinning oscillations τ, varied between 100 and 1000τGL. The voltage is a measure of dissipation for the given current, hence is intimately related to the vortex motion. Fig. 2(a) reveals clear indications of resonant behavior, namely, all V(τ) characteristics show well-defined occurrence of extendedly stable states perfectly and continuously linking the curves for different applied currents. This resonant behavior at different currents is highlighted by black lines in Fig. 2(a), and follows 1/τ dependence as evidenced in Fig. 2(b). To see the origin of this behavior, we chose one point on the curve (for τ = 300τGL and applied current density J = 0.066j0, where j0 is the unit of the current, see Methods) and monitored the vortex motion y(t) and voltage as a function of time (shown in Fig. 3(a,b)). For the chosen length of the simulation region L and the considered magnetic field, we actually had Nv = 6 parallel vortices simultaneously moving in a single row, as shown in the contourplots of the Cooper-pair density in the right panel of Fig. 3. There, points 1–5 are used to denote one period of the vortex dynamics, marking the characteristic instances: the entry of a new vortex row (beginning of the cycle—1), the exit of a single row of the previously present vortices (2), the trapping of the vortex row in the depletion region, together with remaining preexisting vortices (3), depinning of the second row of the previously present vortices from the depletion region (4), and finally, exit of the last preexisting vortices (5). As one can observe in Fig. 3, at the chosen resonance the frequency of pinning is exactly synchronized with the vortex motion—the period of the pinning matches exactly the period of the measured voltage (see Fig. 3(b)), and during one period effectively one vortex row crosses the sample from one edge to another, while one vortex row remains pinned all the time (see Fig. 3(a)), or in words of Faraday’s law—the voltage corresponds to the change of magnetic flux over period τ of exactly one flux quantum, multiplied by Nv. For more information, we refer the reader to full animated data and description of vortex dynamics at resonances in the supplementary materials.

Figure 2. Voltage resonances.

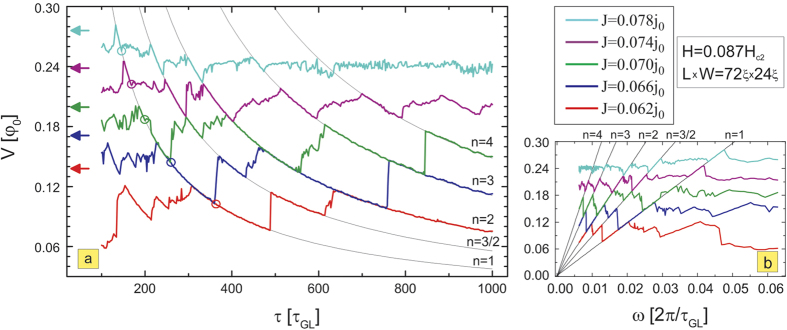
Figure 2

Open in a new tab

(a) Voltage is plotted versus the period τ of the oscillating pinning landscape shown in Fig. 1, for a given sample size and magnetic field, and for five different values of the applied current. Arrows indicate the voltage in absence of pinning for the corresponding current, while dots indicate the transition between two dynamic regimes: vortex tapping at low τ and vortex pinning at high τ. The black lines highlight the resonances where 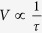Inline graphic as further shown in (b) where voltage is plotted against pinning frequency ω = 2π/τ, and clearly shows a linear dependence when resonance conditions are met.

Figure 3. Vortex trajectory and temporal voltage response at the first resonance.

Figure 3

Open in a new tab

The location of the vortex core traveling across the sample as a function of time (a), and the corresponding change in the voltage (b), for one example of the system at the first resonance (in particular, for J = 0.066j0 and 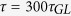Inline graphic, see Fig. 2). Dotted lines show the profile of the time-dependent pinning potential, where minima of the dotted lines represent the ON state of the pinning, and the maxima represent the OFF state. Points 1–5 denote one cycle of the vortex dynamics, marking the instances when the new vortex row enters (cycle begins) (1), some of the the previously existing vortices exit (2), the vortex row is trapped in the depletion region (3), the remaining preexisting vortices are depinned from the depletion region (4), and the remaining previously existing vortices exit the sample (5). Corresponding snapshots of the Cooper-pair density for states 1–5 are given in the right panel, showing rows containing six vortices in parallel and simultaneous motion. The resulting periodicity of the voltage during vortex motion exactly matches the period of the pinning, and entering/exiting vortices provide a flux change of exactly one flux-quantum per vortex row during that period.

Therefore, we revealed a temporal matching effect which is in essence stroboscopic, i.e. caused by synchronization between pinning and vortex dynamics. Multiple resonances are possible, depending on the number of vortices that participate in the characteristic dynamics during one period of the pinning oscillations (further denoted by n). Every integer number n leaves the resonant fingerprint on the V(τ) characteristics, but also fractional resonances are possible—for example for n = 3/2, where 3 magnetic flux quanta (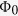Inline graphic) cross the sample over a period of 2τ (per vortex row). At all resonances, the voltage exhibits V ∝ 1/τ behavior (specifically 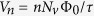Inline graphic, i.e. 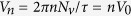Inline graphic in dimensionless units, see Methods), hence it has linear dependence on pinning frequency, as shown directly in Fig. 2(b). Please note that in realistic experimental conditions, and significantly longer samples than shown in our simulation, the number Nv of simultaneously moving vortex rows at the resonance will be larger, hence the measured voltage at resonance will be proportionally larger than shown in our results—which facilitates the observation of the reported phenomenon.

The initiated reader will immediately notice the resemblance of this temporal matching events to the spatial commensurability phenomena employed in the past to enhance the critical parameters of superconductors. In our case, the system is always in a dynamic regime, but matching effects in resonances do decrease the voltage, hence also decrease the overall dissipation. Looking at the n = 1 resonance in Fig. 2, we note the possible tuning of resistance in a very broad range by simultaneous adjustment of τ and applied current—both externally controllable parameters.

Shapiro steps

Another careful look at the resonances shown in Fig. 2 reveals more important details. Particularly, we observe that voltage curves obtained for different currents can overlap at certain pinning periods. In other words, for specific τ the system can exhibit identical voltage at two different bias currents. One of such cases is examined in Fig. 4, where we constructed the current-voltage J–V characteristics for 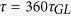Inline graphic. For J = 0.062 − 0.066j0 the system remains in the same n = 1 resonance, as seen in Fig. 2, and consequently J–V characteristics show a Shapiro step at these currents. At larger currents more Shapiro steps are found, corresponding to higher resonances and exhibiting exactly quantized voltages—equal to nV0, thus tunable by τ.

Figure 4. Shapiro steps.

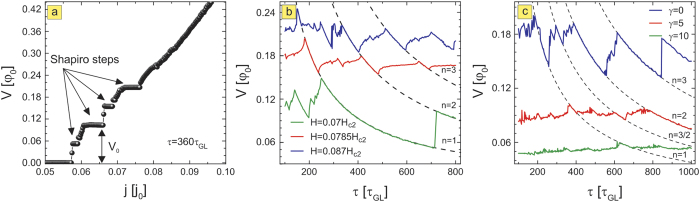
Figure 4

Open in a new tab

(a) The Shapiro steps in V(j) characteristics for the pinning period of 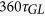Inline graphic. V0 indicates the Shapiro step at first resonance. (b) The resonant behavior in V(τ) for different values of magnetic field indicates possible Shapiro steps in magnetoresistivity. (c) The resonant behavior in V(τ) is also found for varied disorder, i.e. varied inelastic phonon-electron scattering time, with effective influence on the viscosity of the superconducting condensate (parameter γ in the theory, see Methods).

It is actually a well-known fact that when a superconductor in the presence of periodic potential is driven with the superimposed dc and ac force, Shapiro steps can be experimentally found in the I–V characteristics35,36,37,38. In our case Shapiro steps appear due to fact that we apply dc Lorentz force via magnetic field and current, and on the other side the time-dependent pinning potential is contributing with the ac component. The presence of the pinning potential reflects in the variation of the superconducting order parameter, which then translates in the ac variation of the supercurrent. In addition, due to the stripe-like profile of the depletion region, our structure is a time-dependent Josephson junction (S − N(t) − S junction), hence the relevance of Shapiro physics is clear.

Interestingly, in the reported investigation we observe Shapiro steps not only in current-voltage characteristics, but also with varying practically any parameter instead of current. An analogy can be drawn here with the case of static periodic pinning and an ac excitation (both for superconducting vortices and colloids), for which resonant features have been reported8,10,12,25. In our case, the reason is that the origin of the stroboscopic phenomenon is the synchronization of vortex motion and time-dependent pinning, and vortex motion can be influenced in more ways than just by current. In Fig. 4(b) we demonstrate the dependence of the V(τ) characteristics on the applied magnetic field, for fixed applied current. We again note the presence of the resonances, which obey the exact same 1/τ behavior as in Fig. 2(a). This leads us to the conclusion that Shapiro steps should also be expected in V(H) characteristics, i.e. in magnetoresistance. For additional check, we conducted simulations for varied inelastic phonon-electron scattering time of the superconductor, such that the effective viscosity for vortex motion changes. Even then resonances are observed to follow the same 1/τ behavior and where, with increased viscosity (parameter γ in the theory, see Methods), the vortices slow down, and voltage slides down on the resonant curve (see Fig. 4(c)). Further relations between vortex velocity and voltage will be explored in the Discussions section.

Discussion

Voltage-phase relation

In a region where there is no interconversion between supercurrent and normal current densities (i.e., wherever ∇·js = 0, thus well outside moving vortex cores) the electric field E and the gauge-invariant vector potential AS are related by the simple gauge-independent equation

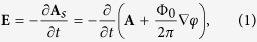graphic file with name srep14604-m6.jpg

where A is the vector potential within a chosen gauge, and φ is the phase of the order parameter in the same gauge. Our calculations show that the contribution of ∇φ to As is far larger than that due to A. This argument arises from the fact that the magnetic-flux contributions can be neglected relative to kinetic-energy contributions when the linear dimensions of the nanocircuits under consideration are small by comparison with the Pearl length39. For this reason, at least for relatively narrow nanocircuits it is an excellent approximation to write

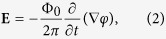graphic file with name srep14604-m7.jpg

hence

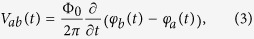graphic file with name srep14604-m8.jpg

which is the standard Josephson relation between voltage and phase (a and b being the arbitrary points between which the voltage is measured). For additional check, we plotted in Fig. 5(a) the change of phase φ12 =φ2 − φ1 between the points where the voltage is measured, together with the time derivative of φ12, and confirmed that 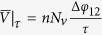Inline graphic, as stipulated by Eq. (3). As also shown in e.g. Ref. 40, the dc average of V(t) during vortex crossing will be Vdc = Φ0R, where R is the rate of vortex crossings. The latter corresponds exactly to our resonant conditions.

Figure 5. Relation of voltage to phase and vortex velocity.

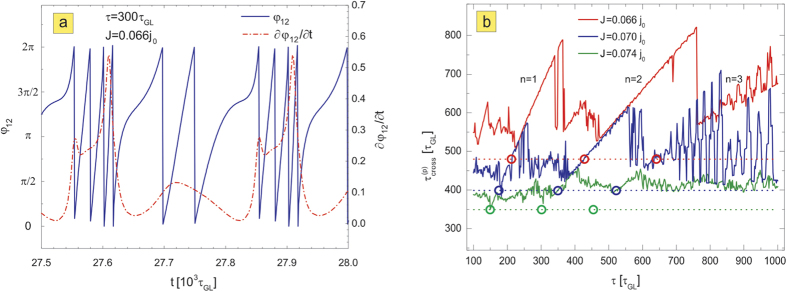
Figure 5

Open in a new tab

(a) The temporal evolution of the phase difference (blue line) and the temporal derivative of the phase difference (red dash-dots) measured between the voltage contacts, for the situation corresponding to Fig. 3. (b) The crossing time of one vortex as a function of the pinning period τ, for different applied current. Horizontal dashed lines represent the vortex crossing time in the pinning-free sample. Open dots indicate τ = τ*, the period of the pinning for which the vortex crossing time becomes the same as in the absence of pinning (consequently, the corresponding voltages match, c.f. Fig. 2).

Effects on vortex dynamics and velocity

The description of the vortex dynamics corresponding to Fig. 3, and the higher resonances involving more vortices is presented in the Supplementary Materials. Essentially, the voltage associated with vortex crossing Vab(t) is proportional to the vortex velocity v(t) (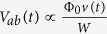Inline graphic, where W is the width of the sample), and in the case of multiple crossing vortices the total voltage is the sum of their individual contributions. Knowing this, and looking at the time-averaged voltage shown in Fig. 2, one can establish relations between time-averaged vortex velocities at different currents, with or without pinning.

Usually, in experiments the vortex velocity can only be reliably estimated at high magnetic field41,42,43, since vortex-vortex interaction dominates over vortex-pinning and therefore the velocity distribution function is very narrow. In our numerical simulations we tracked the velocities of individual vortices, by developing a software capable of mapping the vortex trajectory in real time (shown in Fig. 3(a)). From it, we can also obtain the average crossing time in the presence of the pinning, 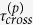Inline graphic, which includes the time vortices spend trapped in the flashing pinning potential. At the nth resonance, the average time a vortex spends in motion during 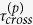Inline graphic is exactly τ/n, and the average vortex velocity during its motion is therefore 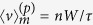Inline graphic. The time a vortex spends pinned is also approximately τ/n, as can be seen in Fig. 3(a) and the Supplementary Material. Hence, at resonance, 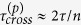Inline graphic. This is indeed verified in Fig. 5(b), where we show the dependence 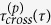Inline graphic, corresponding to the cases considered in Fig. 2. Therefore, if voltage is measured experimentally for varied τ and applied current, one can establish where voltage in absence of pinning (indicated by arrows in Fig. 2) meets the V(τ) curve during the resonance, and determine τ* for which time-averaged vortex velocities with and without pinning are the same, i.e. 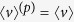Inline graphic, hence 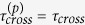Inline graphic. Then, the time-averaged vortex velocity without pinning can be conveniently estimated in experiment as 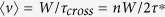Inline graphic. Remarkably, for pinning flashing with period τ = τ*, the average vortex velocity when in motion 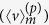Inline graphic will be twice larger than the average vortex velocity in absence of pinning.

Here we provide some estimates of the relevant quantities for NbN frequently used for superconducting single-photon detectors44. Taking Tc = 12.7 K and the working temperature T = 5.5 K, and coherence length ξ(0) = 4 nm, we estimate 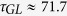Inline graphic fs and upper critical field 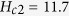Inline graphic T. Since τcross is more than hundred times the τGL, this translates into τcross ≥ 10 ps. Range of the considered pinning periods τ for this particular case goes from 7.2 ps up to 72 ps, which means that the highest considered pinning frequency is 140 GHz. If the width of the sample is increased, all of the resonances are shifted towards higher τ, making them feasible at frequencies below 20 GHz. These values are comparable with the parameters of mode-locked lasers where pulse duration goes up to 5 fs, and with achievable repetition rates of 100 GHz45. Expected value of the resonant voltage V0 is of the order of 10–100 μV. A depleted region of several ξ could be imprinted using the far field excitation of a slit graved in a thick metallic mirror as schematically shown in Fig. 1(b). However, in case the size of the depleted region should be smaller than the wavelength of the impinging light, it would be necessary to work in the optical near-field.

Finally, we take a step back to the case of faster oscillations of the pinning, the so called ‘tapping’ mode. The reason is that a careful look at Fig. 2(a) reveals the possibility of having a larger voltage at the resonance (see beginning of the n = 1 curve) than in the case without any pinning in the sample (indicated by an arrow in the vertical axis). This is highly counterintuitive, as adding pinning to superconductors is supposed to anchor vortices and decrease dissipation. This unusual feature is only possible in the tapping regime (see the dots separating the tapping from the pinning regime in Fig. 2). Namely, the conditions to obtain this phenomenon are such that when the vortex enters the sample, the pinning is at its maximum, but weakens as vortex approaches. Therefore, the attractive force between the vortex and the depletion region will accelerate the vortex, only to tap it and release as if there is no pinning. Hence the average vortex velocity is larger than in the case without pinning, which is a unique case of pinning-enhanced dissipation.

In summary, we presented the very first consideration of the effects of dynamic pinning landscape on resistive state in superconductors. We revealed stroboscopic matching between vortex dynamics and pinning oscillations, which leaves unexpected signature as resonances in the measured voltage versus the period of the pinning, continuous in wider parameter space (for different applied current, magnetic field, microscopic sample parameters). Most of these parameters are externally variable, hence system can be easily tuned along the resonant condition, to achieve beneficial resistive conditions, Shapiro physics without any nanostructuring, or advanced control of flux quanta, all of which deserve further experimental and theoretical pursuit.

Methods

In this work, the time-dependent Ginzburg-Landau (TDGL) equations are used to calculate the voltage as a function of the applied current, magnetic field, material parameters and the period of the time dependent pinning46,47. The TDGL model is known to provide excellent description of the superconductive dynamics close to the critical temperature Tc. The TDGL equations we solved read:

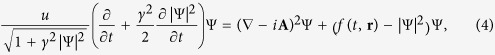graphic file with name srep14604-m22.jpg
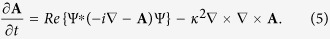graphic file with name srep14604-m23.jpg

These equations are given in the gauge of zero electric potential, and are expressed in the dimensionless units, where the order parameter, Ψ, is given in the units of 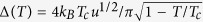Inline graphic at temperature T (kB is the Boltzmann constant, u = 5.79 is the ratio of the relaxation time of the order parameter phase and the relaxation time of the order parameter amplitude46,48), and the vector potential, A, is given in units of Φ0/2πξ(T) (Φ0 is the flux quantum). The parameter γ = 2τinΔ(T)/ħ (ħ is the Planck constant) is determined by the product of the inelastic collision time τin for electron-phonon scattering and Δ(T), and κ is the Ginzburg-Landau parameter which is by its definition dimensionless. Time is expressed in the units of Ginzburg-Landau relaxation time, τGL = πħ/8kB(Tc − T)u, and all of the distances are scaled with the coherence length, ξ(T). The transport current was introduced via the boundary condition for the vector potential in the y direction: 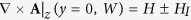Inline graphic, where 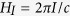Inline graphic is the magnetic field induced by the current I. From the vector potential it is possible to obtain the voltage by using the 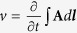Inline graphic. The applied current is given in units of 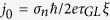Inline graphic (σn is the normal-state conductivity), the magnetic field in units of 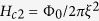Inline graphic, and the voltage scale is given by 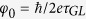Inline graphic. In these units, j0 can be related to the theoretical depairing current jdp, as 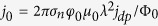Inline graphic, where μ0 is magnetic permeability of vacuum, and λ is the superconducting penetration depth.

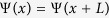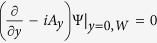In order to simulate a long sample, we apply periodic boundary conditions in the direction of the applied current, Inline graphic and A(x) = A(x + L), where L = 72ξ is the length of the simulated rectangular unit cell (with width W = 24ξ). In y direction the superconductor-vacuum boundary conditions were applied: Inline graphic.

The function f(r, t) is the time-dependent pinning potential:

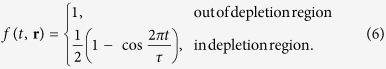graphic file with name srep14604-m34.jpg

The term 2π/τ in Eq. (6) is the angular frequency ω of the pinning. This time-dependent pinning potential arises from temperature variation due to the local heating from some external source (e.g. laser setup). From there the temperature can be varied around its critical value, so we are able to create a region of depleted superconductivity in the middle of the sample. The function f(t, r) provides oscillations of the order parameter in the depletion region, which shifts between the superconducting state (f = 1), and normal state (f = 0). A relation between the temperature and f(t, r) is given through 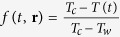Inline graphic, where Tw is the superconductor working temperature outside of the depletion region. By adding the additional terms in the pinning potential f(t, r) it is also possible to account for the impact of the non-equilibrium quasi-particle distribution, where such distribution is calculated outside of TDGL theoretical frame18.

In order to properly describe the possible temperature oscillations in the sample, it is required to couple the heat-balance equation (HBE) to the existing TDGL model as it was done in the work of Vodolazov et al.49 This task is far from trivial since the characteristic thermal coefficients appearing in the HBE (thermal conductivity of the material, the specific heat and the heat removal through the substrate) are themselves temperature dependent. The case described in the present work corresponds to the situation where heat removal is very efficient due to the negligible heat diffusion within the sample. By decreasing the heat removal through the substrate, eventually the order parameter oscillations in the depletion region will transform into a hot-spot where the order parameter will become strictly zero. Under these circumstances oscillations will be lost and so will be the Shapiro steps. Consequently, a purely bolometric effect will rule the behavior of the system, which may also serve as a pinning center, but the dynamic aspect will be simply lost. Notice also that HBE does not appear natively from Ginzburg-Landau theory, nor from the derivation of TDGL equations from BCS theory, and therefore we cannot determine the exact microscopic nature of the thermal coefficients at question.

Numerical simulations were all performed in the same manner, starting from the Meissner state (zero-field, zero-current cooled), then the magnetic field was applied, and subsequently current was gradually raised to the desired value over a period of 5000τGL. Total duration of the simulation for each set of the parameters was the same, 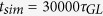Inline graphic.

In order to determine the vortex velocity, both local and average, we developed a vortex tracking software capable of locating the individual vortex and then tracing vortices in real time. As a result we simultaneously obtained information about the vortex paths, vortex crossing time and vortex velocity, for all participating vortices.

Additional Information

How to cite this article: Jelić, Ž. L. et al. Stroboscopic phenomena in superconductors with dynamic pinning landscape. Sci. Rep. 5, 14604; doi: 10.1038/srep14604 (2015).

Supplementary Material

Supplementary Animation 1
srep14604-s1.gif (4.1MB, gif)
Supplementary Animation 2
srep14604-s2.gif (4MB, gif)
Supplementary Animation 3
srep14604-s3.gif (4.2MB, gif)
Supplementary Information
srep14604-s4.pdf (340.3KB, pdf)

Acknowledgments

This work was supported by the Methusalem Funding of the Flemish Government, the Research Foundation-Flanders (FWO) and the COST Action MP1201. The work of Ž.L.J. and A.V.S. was partially supported by “Mandat d’Impulsion Scientifique” of the F.R.S.-FNRS.

Footnotes

Author Contributions All authors discussed the results and contributed to the scientific statement of the manuscript. A.V.S. initiated and managed the project, Ž.L.J. and M.V.M. performed simulations and wrote the manuscript with input from J.V.d.V. and A.V.S.

References

  1. Chen D. X., Moreno J. J., Hernando A., Sanchez A. & Li B. Z. Nature of the driving force on an Abrikosov vortex. Phys. Rev. B 57, 5059 (1998). [Google Scholar]
  2. Maiorov B. et al. Synergetic combination of different types of defect to optimize pinning landscape using BaZrO3-doped YBa2Cu3O7. Nature Mater. 8, 398–404 (2009). [DOI] [PubMed] [Google Scholar]
  3. Macmanus-Driscoll J. L. et al. Strongly enhanced current densities in superconducting coated conductors of YBa2Cu3O7x + BaZrO3. Nature Mater. 3, 439–443 (2004). [DOI] [PubMed] [Google Scholar]
  4. Nanoscience and Engineering in Superconductivity (eds Moshchalkov V. V., Woerdenweber R. & Lang W.) ISBN 978-3-642-15137-8 Springer (2010). [Google Scholar]
  5. Kramer R. B. G., Silhanek A. V., Gillijns W. & Moshchalkov V. V. Imaging the Statics and Dynamics of Superconducting Vortices and Antivortices Induced by Magnetic Microdisks. Phys. Rev. X 1, 021004 (2011). [Google Scholar]
  6. Rosenstein B., Shapiro I. & Shapiro B. Y. Maximal persistent current in a type-II superconductor with an artificial pinning array at the matching magnetic field. Phys. Rev. B 81, 064507 (2010). [Google Scholar]
  7. Rosenstein B., Shapiro I. & Shapiro B. Y. Transport current carrying superconducting film with periodic pinning array under strong magnetic fields. Phys. Rev. B 83, 064512 (2011). [Google Scholar]
  8. Zhu B. Y., Marchesoni F., Moshchalkov V. V. & Nori F. Controllable step motors and rectifiers of magnetic flux quanta using periodic arrays of asymmetric pinning defects. Phys. Rev. B 68, 014514 (2003). [Google Scholar]
  9. Zhu B. Y., Marchesoni F. & Nori F. Controlling the Motion of Magnetic Flux Quanta. Phys. Rev. Lett. 92, 180602 (2004). [DOI] [PubMed] [Google Scholar]
  10. Shklovskij V. A., Sosedkin V. V. & Dobrovolskiy O. V. Vortex ratchet reversal in an asymmetric washboard pinning potential subject to combined dc and ac stimuli. J. Phys. Cond. Matt. 26, 025703 (2014). [DOI] [PubMed] [Google Scholar]
  11. Shklovskij V. A. & Dobrovolskiy O. V. ac-driven vortices and the Hall effect in a superconductor with a tilted washboard pinning potential. Phys. Rev. B 78, 104526 (2008). [Google Scholar]
  12. Shklovskij V. A. & Dobrovolskiy O. V. Frequency-dependent ratchet effect in superconducting films with a tilted washboard pinning potential. Phys. Rev. B 84, 054515 (2011). [Google Scholar]
  13. Rodrigo P. J., Eriksen R. L., Daria V. R. & Glückstad J. Interactive light-driven and parallel manipulation of inhomogeneous particles. Opt. Expr. 10, 26 (2002). [DOI] [PubMed] [Google Scholar]
  14. Shegai T., Brian B., Miljković V. D. & Käll M. Angular Distribution of Surface-Enhanced Raman Scattering from Individual Au Nanoparticle Aggregates. ASC Nano 5(3), 2036–2041 (2011). [DOI] [PubMed] [Google Scholar]
  15. Vercruysse D. et al. Unidirectional Side Scattering of Light by a Single-Element Nanoantenna. Nano Lett. 13(8), 3843–3849 (2013). [DOI] [PubMed] [Google Scholar]
  16. Valev V. K. et al. Distributing the optical near-field for efficient field-enhancements in nanostructures. Adv. Mater. 24, OP208–OP215 (2012). [DOI] [PubMed] [Google Scholar]
  17. Zotova A. N. & Vodolazov D. Y. Photon detection by current-carrying superconducting film: A time-dependent Ginzburg-Landau approach. Phys. Rev. B 85, 024509 (2012). [Google Scholar]
  18. Zotova A. N. & Vodolazov D. Y. Intrinsic detection efficiency of superconducting nanowire single photon detector in the modified hot spot model. Supercond. Sci. Technol. 27, 125001 (2014). [Google Scholar]
  19. Lusche R. et al. Effect of the wire width on the intrinsic detection efficiency of superconducting-nanowire single-photon detectors. J. Appl. Phys. 116, 043906 (2014). [Google Scholar]
  20. Hofherr M. et al. Time-Tagged Multiplexing of Serially Biased Superconducting Nanowire Single-Photon Detectors. IEEE Trans. on Appl. Supercond. 23, 2501205 (2013). [Google Scholar]
  21. Engel A. et al. Tantalum nitride superconducting single-photon detectors with low cut-off energy. Appl. Phys. Lett. 100, 062601 (2012). [Google Scholar]
  22. Lara A., Aliev F. G., Silhanek A. V. & Moshchalkov V. V. Microwave-stimulated superconductivity due to presence of vortices. Sci. Rep. 5, 9187 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  23. Sivakov A. G., Zhuravel’ A. P., Turutanov O. G. & Dmitrenko I. M. Spatially resolved characterization of superconducting films and cryoelectronic devices by means of low temperature scanning laser microscope. Appl. Surf. Sci. 106, 390–395 (1996). [Google Scholar]
  24. Davis M. C. et al. Manipulation of vortices by localized impurities in Bose-Einstein condensates. Phys. Rev. A 80, 023604 (2009). [Google Scholar]
  25. Libál A., Reichhardt C., Jankó B. & Olson Reichhardt C. J. Dynamics, Rectification, and Fractionation for Colloids on Flashing Substrates. Phys. Rev. Lett. 96, 188301 (2006). [DOI] [PubMed] [Google Scholar]
  26. Grier D. G. A revolution in optical manipulation. Nature (London) 424, 810 (2003). [DOI] [PubMed] [Google Scholar]
  27. Koss B. A. & Grier D. G. Optical Peristalsis. Appl. Phys. Lett. 82, 3985 (2003). [Google Scholar]
  28. Lee S. H., Ladovac K., Polin M. & Grier D. G. Observation of Flux Reversal in a Symmetric Optical Thermal Ratchet. Phys. Rev. Lett 94, 110601 (2005). [DOI] [PubMed] [Google Scholar]
  29. Lee S. H. & Grier D. G. Flux reversal in a two-state symmetric optical thermal ratchet. Phys. Rev. E 71, 060102(R) (2005). [DOI] [PubMed] [Google Scholar]
  30. Babič D. & Bechinger C. Noise-Enhanced Performance of Ratchet Cellular Automata. Phys. Rev. Lett. 94, 148303 (2005). [DOI] [PubMed] [Google Scholar]
  31. Babič D., Schmitt C. & Bechinger C. Colloids as model systems for problems in statistical physics. Chaos 15, 026114 (2005). [DOI] [PubMed] [Google Scholar]
  32. Shmidt V. V. & Mkrtchyan G. S. Vortices in type-II superconductors. Usp. Fiz. Nauk 112, 459–490 (1974). [Google Scholar]
  33. Halperin B. I. & Nelson D. R. Resistive transition in superconducting films. Journ. of Low Temp. Phys. 36, 599–616 (1979). [Google Scholar]
  34. Ivlev B. I. & Kopnin N. B. Resistive state of superconductors. JETP Lett. 28, 10 (1978). [Google Scholar]
  35. Van de Vondel J. et al. Vortex core deformation and stepper motor behavior in a superconducting ratchet. Phys. Rev. Lett. 106, 137003 (2011). [DOI] [PubMed] [Google Scholar]
  36. Van Look L. et al. Shapiro steps in a superconducting film with an antidot lattice. Phys. Rev. B 60, 10 (1999). [Google Scholar]
  37. Van de Vondel J. et al. Self-organized mode-locking effect in superconductor/ferromagnet hybrids. Phys. Rev. B 79, 054527 (2009). [Google Scholar]
  38. Silhanek A. V. et al. Local probing of the vortex-antivortex dynamics in superconductor/ferromagnet hybrid structures. Supercond. Sci. Technol. 24, 024007 (2011). [Google Scholar]
  39. Clem J. R. & Berggren K. K. Geometry-dependent critical currents in superconducting nanocircuits. Phys. Rev. B 84, 174510 (2011). [Google Scholar]
  40. Bulaevskii L. N., Graf M. J., Batista C. D. & Kogan V. G. Vortex-induced dissipation in narrow current-biased thin-film superconducting strips. Phys. Rev. B 83, 144526 (2011). [Google Scholar]
  41. Dorsey A. T. Vortex motion and the Hall effect in type-II superconductors: A time-dependent Ginzburg-Landau theory approach. Phys. Rev. B 46, 13 (1992). [DOI] [PubMed] [Google Scholar]
  42. Villegas J. E. et al. A Superconducting Reversible Rectifier That Controls the Motion of Magnetic Flux Quanta. Science 302, 5648 (2003). [DOI] [PubMed] [Google Scholar]
  43. Martin J. I., Velez M., Nogues J. & Schuller I. K. Flux Pinning in a Superconductor by an Array of Submicrometer Magnetic Dots. Phys. Rev. Lett. 79, 10 (1997). [Google Scholar]
  44. Bartolf H. et al. Current-assisted thermally activated flux liberation in ultrathin nanopatterned NbN superconducting meander structures. Phys. Rev. B 81, 024502 (2010). [Google Scholar]
  45. Keller U. Recent developments in compact ultrafast lasers. Nature 424, 831–838 (2003). [DOI] [PubMed] [Google Scholar]
  46. Kramer L. & Watts-Tobin R. J. Theory of Dissipative Current-Carrying States in Superconducting Filaments. Phys. Rev. Lett. 40, 1041 (1978). [Google Scholar]
  47. Vodolazov D. Y. & Peeters F. M. Rearrangement of the vortex lattice due to instabilities of vortex flow. Phys. Rev. B 76, 014522 (2007). [Google Scholar]
  48. Baranov V. V., Balanov A. G. & Kabanov V. V. Current-voltage characteristic of narrow superconducting wires: Bifurcation phenomena. Phys. Rev. B 84 094527 (2011). [Google Scholar]
  49. Vodolazov D. Y., Peeters F. M., Morelle M. & Moshchalkov V. V. Masking effect of heat dissipation on the current-voltage characteristics of a mesoscopic superconducting sample with leads. Phys. Rev. B 71, 184502 (2005). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Animation 1
srep14604-s1.gif (4.1MB, gif)
Supplementary Animation 2
srep14604-s2.gif (4MB, gif)
Supplementary Animation 3
srep14604-s3.gif (4.2MB, gif)
Supplementary Information
srep14604-s4.pdf (340.3KB, pdf)

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES