Purpose:
To evaluate the biomechanics of corneal ring implants by providing a related mathematical theory and biomechanical model for the treatment of myopia and keratoconus.
Methods:
The spherical dome model considers the inhomogeneity of the tunica of the eye, dimensions of the cornea, lamellar structure of the corneal stroma, and asphericity of the cornea. It is used in this study for calculating a strengthening factor sf for the characterization of different ring-shaped corneal implant designs. The strengthening factor is a measure of the amount of strengthening of the cornea induced by the implant.
Results:
For ring segments and incomplete rings, sf = 1.0, which indicates that these implants are not able to strengthen the cornea. The intracorneal continuous complete ring (MyoRing) has a strengthening factor of up to sf = 3.2. The MyoRing is, therefore, able to strengthen the cornea significantly.
Conclusions:
The result of the presented biomechanical analysis of different ring-shaped corneal implant designs can explain the different postoperative clinical results of different implant types in myopia and keratoconus.
Key Words: keratoconus, cornea, MyoRing, ring segments, progression, ectasia, biomechanics, corneal strength, corneal rings, ICRS
Intracorneal implants for the correction of refractive errors and ectatic corneal diseases can be grouped into 2 categories: (1) intracorneal ring segments (ICRS) of up to 355 degree arc length such as the Ferrara ring (Ferrara Ophthalmics, Brazil), Intacs (Addition Technology Inc, Lombard, IL), and Keraring (Mediphacos, Brazil)1–5 and (2) the intracorneal continuous complete ring MyoRing (Dioptex GmbH, Austria)6,7 (Fig. 1). The fundamental mechanism of action behind all these devices is characterized by an arc-shortening effect within the cornea because of the added implant volume to the cornea. While ICRS are implanted into a circular tunnel, the MyoRing is implanted into a corneal pocket through a small corneal incision. Intracorneal ring implants mainly produce deformation of the cornea according to the refractive need. Understanding the biomechanical interaction between intracorneal ring implants and the cornea is therefore important.
FIGURE 1.
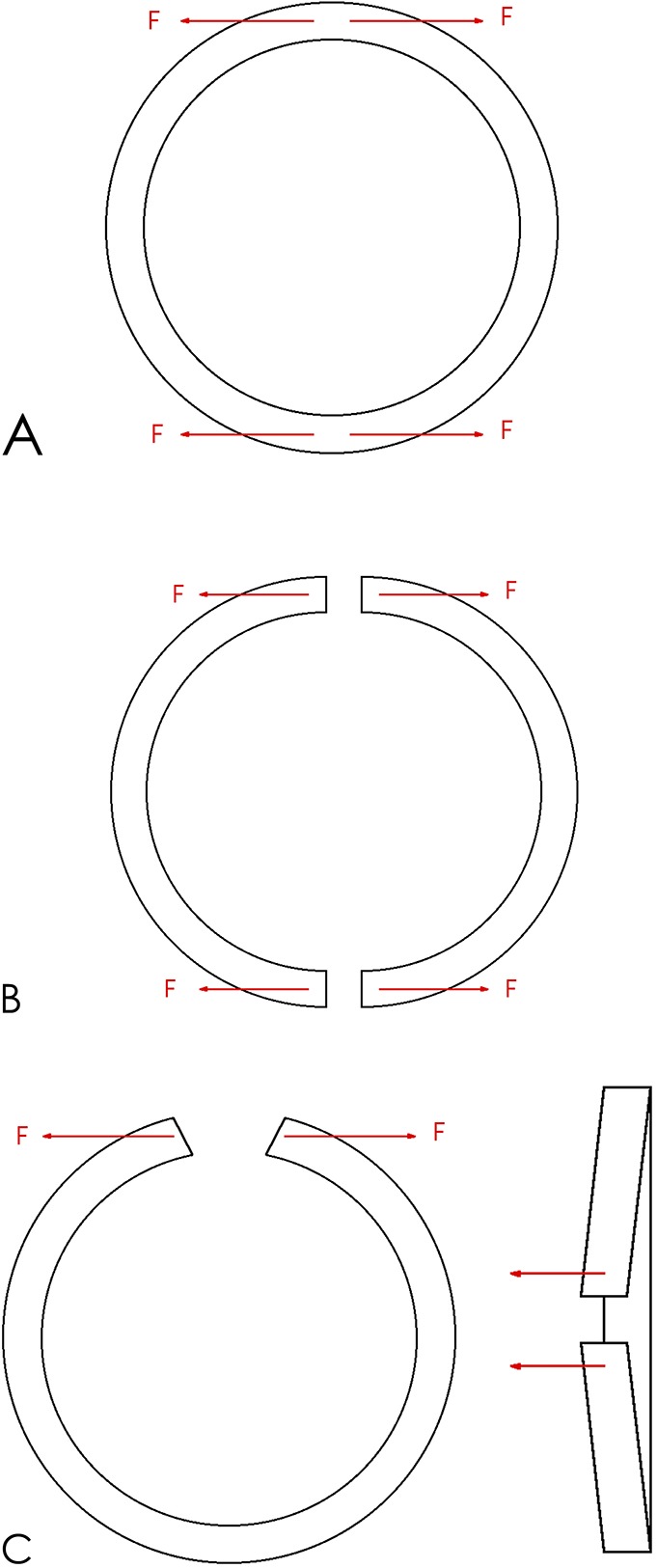
A, The MyoRing is a full ring implant without discontinuity along the entire circumference where the force F required to separate 2 parts of the implant is extremely high. The endings of (B) intra corneal ring segments (ICRS) and (C) incomplete rings can be separated easily by a force F of virtually zero.
MATERIALS AND METHODS
The Spherical Dome Model after Daxer8
 |
is an effective and easy-to-use mathematical model of the cornea, which considers (1) the structural and biomechanical heterogeneity of the tunica of the eye, allowing to distinguish between the cornea, limbus, and sclera, as well as (2) the anisotropy and asphericity of the cornea. It therefore facilitates an independent biomechanical analysis of the cornea. Here, σ represents the stress inside the cornea, ρ the isotropically acting intraocular pressure, D the diameter of the cornea (limbus), d the thickness of the cornea, and f is an anisotropy factor ranging between 0 and 1.
In the nonexisting case of structural and biomechanical isotropy within the corneal tissue, when 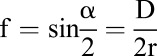 ,with α representing the opening angle of the cornea, and r the corneal radius, the spherical dome model8 converges to the special case of the elastic sphere model after Laplace,9 which is an excellent proof of the model.
,with α representing the opening angle of the cornea, and r the corneal radius, the spherical dome model8 converges to the special case of the elastic sphere model after Laplace,9 which is an excellent proof of the model.
In this study, I used the spherical dome model with an appropriate anisotropy factor of f = 1, which results in
 |
An anisotropy factor of f = 1 means that the corneal local cross-sectional area contributes entirely to the stress uptake inside the tissue and not only the projection area into the direction of the resulting force vector, which is aligned roughly in the direction of the optical axis. This is equivalent to an increased strength of the cornea because of the reinforcement in the lamellar direction by the collagen fibrils.10
The spherical dome model is also based on the fact that the Young modulus of the limbus is reported to be up to 13 MPa, and thus comparatively higher than the Young modulus of the cornea of roughly 0.3 MPa, which allows us to consider the limbus as a biomechanical border of the cornea.8,11–14
The corneal implants investigated in this study are made of types of PMMA. PMMA has a Young modulus between 1800 MPa and 3100 MPa, which is even higher than that of the limbus.15 The different implant designs can, however, significantly affect the way in which the comparatively high Young modulus of the material of the implants can be used to stabilize the cornea. The difference in the biomechanical effect of the different implant designs result from whether or not the implant has discontinuity along the circumference or not. This quality determines whether the implant can be considered an additional (artificial) limbus or not. In the case of no discontinuity (MyoRing) the implant can use the enormous Young modulus and act as an additional (artificial) limbus inside the cornea. Accordingly, the postoperative value for D in Equation 2 is represented by the inner diameter of the MyoRing DMRI and by the corneal diameter Dc preoperatively. In the case of ICRS and incomplete rings, both the preoperative and postoperative value for D in Equation 2 is represented by the anatomical corneal diameter Dc.
This allows the calculation of a strengthening factor sf, which is identical to the term “relative corneal strength” used throughout this study, and which is defined as the ratio between the intracorneal stress before and after treatment according to
The intracorneal stress σ is calculated using Equation 2.
RESULTS
MyoRing
Because every part of the implant is connected to the adjoining part with the full mechanical strength of the material along the entire circumference (Fig. 1A), the MyoRing can be biomechanically considered as a further (artificial) limbus, which separates the load on the cornea resulting from the intraocular pressure into an independent load inside the inner diameter of the implant and an independent load between the outer diameter of the implant and the limbus (corneal diameter). Therefore, D in Equation 2 is the diameter of the cornea preoperatively (Dc) and the inner diameter of the MyoRing DMRI (artificial limbus) postoperatively. The corneal strengthening factor sfc of the central cornea is, therefore
 |
Figure 2A shows sfc for different corneal diameters and implant geometries.
FIGURE 2.
A, Corneal strengthening factor for the central corneal area (centrally to the MyoRing) of different MyoRing diameters as a function of the corneal diameter. B, Corneal strengthening factor for the peripheral corneal area (outside the MyoRing) of different MyoRing diameters as a function of the corneal diameter.
For the corneal periphery outside the MyoRing (ie, between MyoRing and limbus), the corneal strengthening factor is given by
 |
where DMRA is the outer MyoRing diameter (Fig. 2B).
The peripheral area is, however, not the sensitive area in corneal refractive surgery and in ectatic corneal diseases (Fig. 3). Therefore, the relevant corneal strengthening factor is sf = sfc.
FIGURE 3.
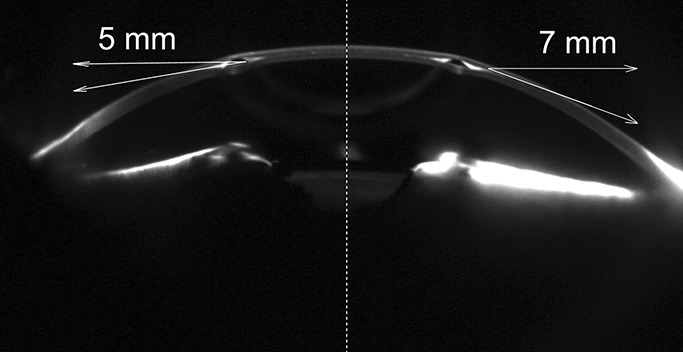
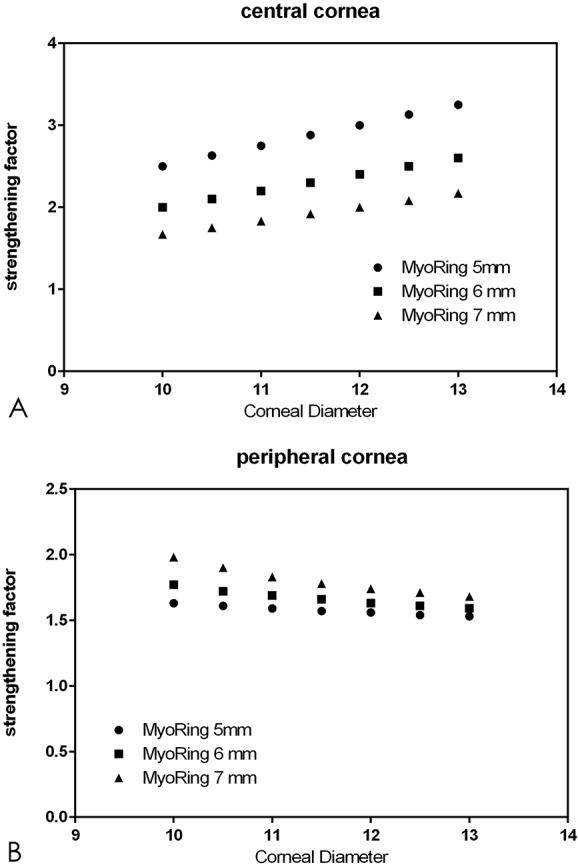
Cornea with a 5-mm MyoRing. The arrows indicate the stress vector components within the cornea for a 5-mm MyoRing (left) and a 7-mm MyoRing (right). The true stress vector runs tangentially to local corneal curvature at the position of the implant. The horizontal vectors indicate the component of the intracorneal stress acting within the implant plane, which is the effective plane to take up the intracorneal stress.
As a result, the cornea is strengthened by a factor of 3.0 with a 5-mm MyoRing, by a factor of 2.4 with a 6-mm MyoRing and by a factor of 2.0 with a 7-mm MyoRing, using a corneal diameter of 12 mm in Equation 2 (Fig. 2A). The inner diameter of the MyoRing is 1 mm less than the outer one.
As long as the lamellar incision for the insertion of the implant is small, it is not expected to contribute significantly to the biomechanical modification of the tissue.7 It is, therefore, important to remain significantly below an opening angle of 45 degrees when creating a 9-mm pocket at 300-μm depth. The opening width w therefore has to be less than 5.5 mm. In no case should the outer diameter of the MyoRing rest beyond the connection line w between the 2 opposite incision limits. Therefore, the incision width w should meet the following 3 criteria to guarantee an unaltered strength of the corneal tissue (Fig. 4): Range 1: w ≤ DMRA and w < 5.5 mm; range 2: w ≤ Dp·sin(arccos(DMRA/Dp)), where Dp is the diameter of the pocket of 9 mm, and DMRA is the outer diameter of the MyoRing.
FIGURE 4.
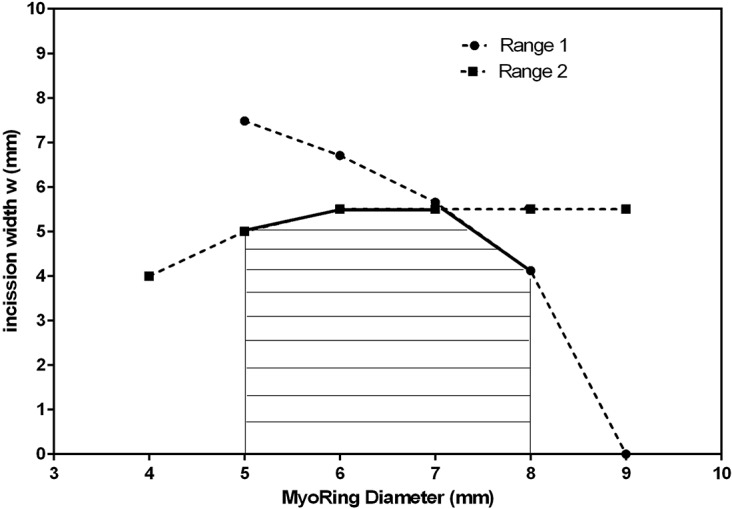
Pocket incision width w as a function of the MyoRing diameter. The area with horizontal lines is the range of safe application.
ICRS AND INCOMPLETE RINGS
The situation is totally different when considering incomplete ring geometry such as in ICRS. Virtually no force is required to separate the endings (Figs. 1B, C). In such a case, the implant cannot act as an artificial limbus to stabilize the cornea. D in Equation 2 is, therefore, represented by the real anatomical corneal diameter preoperatively and postoperatively, which results in a constant strengthening factor of sf = 1. Consequently, ICRS and incomplete rings have no strengthening effect on the cornea whatsoever.
DISCUSSION
Various reports suggest that MyoRing implantation in keratoconic corneas can not only achieve visual rehabilitation but also perhaps stop the progression of the disease.16–18 In contrast to the clinical data obtained after MyoRing implantation for keratoconus, the clinical data after ICRS implantation do not indicate stopping disease progression.19,20 So far, only corneal cross-linking (CXL) has been shown to stop progression of the disease, yet without achieving visual rehabilitation.21–23 The strengthening factor of CXL in humans was reported to be approximately 4.5.24
PMMA is characterized by a comparatively high Young modulus (“stiffness”) that can be used by the MyoRing but not by the ICRS. In this respect, Figure 1 and Figure 3 relate these characteristics to the mechanism responsible for stabilization (strengthening) of the cornea by the MyoRing.
Although the strengthening of the cornea in CXL is achieved on an ultrastructural level resulting in a stiffening of the tissue by increasing the Young modulus, the mechanism of strengthening the cornea by means of MyoRing insertion is different. A cornea treated by MyoRing implantation can be compared to a load-bearing ceiling beam that evenly distributes the load resting on the ceiling over 2 separate compartments, each of which requires only partial strength to take up the load. An interrupted ceiling beam, which is equivalent to ring segments, is unable to fulfill this function.
A comparison of different refractive procedures with respect to the strengthening factor is shown in Figure 5. ICRS treatment including incomplete rings is biomechanically neutral as long as the radial cut is sutured. MyoRing treatment strengthens the cornea considerably. It is therefore possible to combine MyoRing treatment with excimer laser surface ablation without biomechanically harming the cornea.25 However, it is no longer necessary to combine MyoRing treatment with CXL in progressive keratoconus26,27 because MyoRing implantation alone seems to be sufficient to strengthen the cornea. In contrast to early experiments on complete rings,28 the design of the MyoRing combines 2 a priori conflicting qualities in one device: it is rigid enough to modify and stabilize the corneal shape according to the refractive needs, and flexible with a shape memory to allow quick, easy, and reversible implantation into the corneal pocket through a small entrance.7 The model presented in Equations 1 and 2 draws on an anisotropic cornea.8
FIGURE 5.
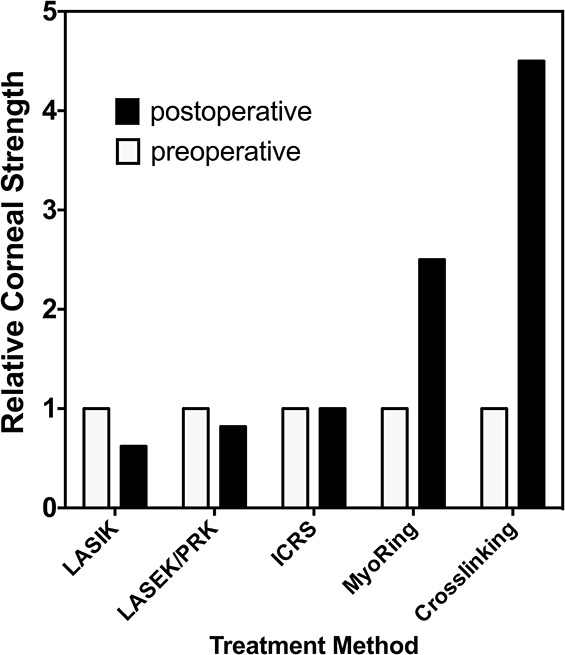
Postoperative relative corneal strength (black bars) compared with preoperative relative corneal strength (white bars) for different refractive treatments. A postoperative value of less than 1 means weakening of the cornea, whereas a value higher than 1 means strengthening. For the laser treatments, −7.75 D correction according to a case presented in the model of Reinstein et al29 was used. The MyoRing case assumes a 6-mm MyoRing (outer diameter) and a corneal diameter of 12 mm. The strengthening factor for CXL was taken from experiments performed by Wollensak et al.24
Mathematically speaking, choosing f = 1 in the basic equation (Eq. 1) for the lamellar cornea is equivalent to biomechanical domination of the lamellar direction within a stiffness tensor (stress tensor) applied to the cornea. It is unimportant for the resulting stress σ in Equation 1 whether the relative strengthening (reduced σ) in the lamellar direction is mathematically achieved by an increased Young modulus in the lamellar direction within the stress tensor or by an increase in the related cross-sectional area (f = 1).
The fact that the model8 converges to the law of Laplace9 in the special case of an isotropic assumption and that the results for laser vision correction are in excellent agreement with other models29 indicates that the model is valid.
The assumption of a depth-dependent Young modulus may have an impact on the corneal biomechanics after tissue-removing refractive procedures,29 but not when considering corneal ring implants in which case the relative composition of the corneal tissue remains unchanged.
The model presented is an approximation and does not incorporate higher-order effects. Such higher-order effects may result from nonlinearity of the stress–strain relation of the tissue. Because it is assumed that the preoperative intraocular pressure corresponds to the postoperative one and the cornea is not exposed to a dynamic load, the presented results should not be sensitive to this effect.
The true stress vector within the tissue is represented by the tangential arrows in Figure 3. The MyoRing is, however, only able to take up the component acting within the plane of the implant according to Figure 1A, which is represented by the horizontal arrows in Figure 3. This modifies the strengthening factor, however, only minimally: from 3.0 to 2.95 for a 5-mm implant and from 2.0 to 1.88 for a 7-mm implant.
Sophisticated numerical approaches to simulate corneal biomechanics are laborious and applicable to specific problems only.30,31 There are also no valuable measurement techniques and trustable biomechanical parameters of the human cornea.11–14,30,31 Therefore, even “sophisticated computational methods” are burdened with related uncertainties. The results in this study are relative values obtained from 2 different states (preoperatively and postoperatively) to reduce such uncertainties.
Long-term studies on MyoRing treatment are currently being conducted to clinically prove the presented theory.
Footnotes
Supported in part by the Austrian Research Fund (FFG).
The author has an investment interest in Dioptex GmbH.
The data have been presented in part at the IV Expert Meeting for Keratoconus in Amsterdam, 2013, and at the Congress of the ESCRS in London, 2014.
REFERENCES
- 1.Blavatskaya ED. Intralamellar homoplasty for the purpose of relaxation of refraction of the eye. Arch Soc Am Ophthalmol Optom. 1968;6:311–325. (translated from Oftalmol Zh 1966;7:530-537). [Google Scholar]
- 2.Nose W, Neves RA, Schanzlin DJ, et al. Intrastromal corneal ring—one year results of first implants in humans: a preliminary non-functional eye study. Refract Corneal Surg. 1993;9:452–458. [PubMed] [Google Scholar]
- 3.Ruckhofer J, Stoiber J, Twa MD, et al. Correction of astigmatism with short arc-length intrastromal corneal ring segments: preliminary results. Ophthalmology. 2003;110:516–524. [DOI] [PubMed] [Google Scholar]
- 4.Siganos D, Ferrara P, Chatzinikolas K, et al. Ferrara intrastromal corneal rings for the correction of keratoconus. J Cataract Refract Surg. 2002;28:1947–1951. [DOI] [PubMed] [Google Scholar]
- 5.Gharaibeh AM, Muhsen SM, AbuKhader IB, et al. KeraRing intrastromal corneal ring segments for correction of keratoconus. Cornea. 2012;31:115–120. [DOI] [PubMed] [Google Scholar]
- 6.Daxer A. Corneal intrastromal implantation surgery for the treatment of moderate and high myopia. J Cataract Refract Surg. 2008;34:194–198. [DOI] [PubMed] [Google Scholar]
- 7.Daxer A. Adustable intracorneal ring in a lamellar pocket for keratoconus. J Refract Surg. 2010;26:217–221. [DOI] [PubMed] [Google Scholar]
- 8.Daxer A. Biomechanics of the cornea. Int J Kerat Ect Cor Dis. 2014;3:57–62. [Google Scholar]
- 9.Dawson DG, Ubels JL, Edelhauser HF. Cornea and sclera. In: Levin LA, ed. Adlers Physiology of the Eye. 11th ed Edinburgh, Scotland: Elsevier; 2011. [Google Scholar]
- 10.Daxer A, Fratzl P. Collagen fibril orientation in the human corneal stroma and its implications in keratoconus. Invest Ophthalmol Vis Sci. 1997;38:121–129. [PubMed] [Google Scholar]
- 11.Ruberti JW, Zieske JD, Trinkhaus-Randall V. Corneal-tissue replacement. In: Lanza RP, Langer R, Vacanti J, eds. Principles of Tissue Engineering. 3rd ed New York: Elsevier, Inc; 2007. [Google Scholar]
- 12.Friberg TR, Lace JW. A comparison of the elastic properties of human choroid and sclera. Exp Eye Res. 1988;47:429–436. [DOI] [PubMed] [Google Scholar]
- 13.Liu J, Roberts CJ. Influence of corneal biomechanical properties on intraocular pressure measurement: quantitative analysis. J Cataract Refract Surg. 2005;31:146–155. [DOI] [PubMed] [Google Scholar]
- 14.Meek KM. The cornea and sclera. In: Fratzl P, ed. Collagen: Structure and Mechanics. New York: Springer; 2010. [Google Scholar]
- 15.Available at: http://en.wikipedia.org/wiki/Poly(methyl_methacrylate). Accessed November 28, 2014, 20.00h CET.
- 16.Alio JL, Pinero DP, Daxer A. Clinical outcomes after complete ring implantation in corneal ectasia using the femtosecond laser technology. A pilot study. Ophthalmology. 2011;118:1282–1290. [DOI] [PubMed] [Google Scholar]
- 17.Jabbarvand M, Salamatrad A, Hashemian H, et al. Continuous corneal intrastromal ring implantation for treatment in keratoconus in an Iranian population. Am J Ophthalmol. 2013;155:837–842. [DOI] [PubMed] [Google Scholar]
- 18.Daxer A. Corneal thickness after MyoRing implantation for keratoconus. Int J Kerat Ect Cor Dis. 2014;3:15–19. [Google Scholar]
- 19.Alio JL, Vega-Estrada A, Esperanza S, et al. Intrastromal corneal ring segments: how successful is the surgical treatment of keratoconus? Middle East Afr J Ophthalmol. 2014;21:3–9. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Chhadva P, Yesilirmak N, Cabot F, et al. Intrastromal corneal ring segment explantation in patients with keratoconus: causes, technique, and outcomes. J Refract Surg. 2015;31:362–397. [DOI] [PubMed] [Google Scholar]
- 21.Spoerl E, Huhle M, Seiler T. Induction of cross-links in corneal tissue. Exp Eye Res. 1998;66:97–103. [DOI] [PubMed] [Google Scholar]
- 22.Vinciguerra P, Albe E, Trazza S, et al. Refractive, topographic, tomographic, and abberometric analysis of keratoconic eyes undergoing corneal cross-linking. Ophthalmology. 2009;116:369–378. [DOI] [PubMed] [Google Scholar]
- 23.Kanellopoulos AJ, Pamel GJ. Review of current indications for combined very high fluence collagen cross-linking and laser in situ keratomileusis surgery. Indian J Ophthalmol. 2013;61:430–432. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Wollensak G, Spoerl E, Seiler T. Stress-strain measurements of human and porcine corneas after riboflavin-ultraviolet-A-induced cross-linking. J Cataract Refract Surg. 2003;29:1780–1785. [DOI] [PubMed] [Google Scholar]
- 25.Daxer A. MyoRing treatment for cases of myopia not eligible for Laser Vision Correction. Int J Kerat Ect Cor Dis. 2014;3:20–22. [Google Scholar]
- 26.Daxer A, Mahmoud HA, Venkateswaran RS. Corneal crosslinking and visual rehabilitation in keratoconus in one session without epithelial debridement: new technique. Cornea. 2010;29:1176–1179. [DOI] [PubMed] [Google Scholar]
- 27.Studeny P, Krizova D, Stranak Z. Clinical outcomes after complete Intracorneal ring implantation and corneal collagen cross-linking in an intrastomal pocket in one session for keratoconus. J Ophthalmol. 2014;2014:568128. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 28.Binder PS. Hydrogel implants for the correction of myopia. Curr Eye Res. 1982-1983;2:435–441. [DOI] [PubMed] [Google Scholar]
- 29.Reinstein DZ, Archer TJ, Randleman JB. Mathematical model to compare the relative tensile strength of the cornea after PRK, LASIK, and small incision lenticule extraction. J Refract Surg. 2013;29:454–460. [DOI] [PubMed] [Google Scholar]
- 30.Kling S, Marcos S. Finite-element modeling of intrastromal ring segment implantation into a hyperelastic cornea. Invest Ophthalmol Vis Sci. 2013;54:881–889. [DOI] [PubMed] [Google Scholar]
- 31.Abhijit SR, Dupps WJ, Roberts CJ. Comparison of biomechanical effects of small-incision lenticule extraction and laser in situ keratomileusis: finite-element analysis. J Cataract Refract Surg. 2014;40:971–980. [DOI] [PMC free article] [PubMed] [Google Scholar]


