Dynamic criteria of plankton jumping out of water

Abstract

In nature, jumping out of water is a behaviour commonly observed in aquatic species to either escape from predators or hunt prey. However, not all aquatic species are capable of jumping out, especially small organisms whose length scales are comparable to the capillary length (approx. 2.7 mm for water). Some aquatic animals smaller than the capillary length are able to jump out while others are not, as observed in some marine copepods. To understand the dynamics of jumping out of the water–air interface, we perform physical experiments by shooting a spherical particle towards the liquid–air interface from below. Experimental results show that the particle either penetrates or bounces back from the interface, depending on the particle and fluid properties, and the impact velocity. The transition from bouncing to penetration regimes, which is theoretically predicted based on a particle force balance, is in good agreement with both physical experiments and plankton behavioural data.

1. Introduction

A number of aquatic species across a broad range of taxonomic groups can be commonly observed ‘jumping’ through the water surface for different hypothetical reasons [1,2]. For example, salmonid fish leap out of the river occasionally when they encounter obstacles during upstream spawning migrations [3]. Humpback whales (Megaptera novaeangliae), spinner dolphins (Stenella longirostris) and gulf sturgeons (Acipenser oxyrinchus desotoi) also jump out of the water presumably in order to communicate within the group by creating sounds at the moment of impact [4–6]. Some other aquatic species break through the water surface to evade predators or to capture prey [1,7]. Some predators, such as dolphinfish (Coryphaena) and arowana (Osteoglossum bicirrhosum) jump to catch prey above the surface of the water [7,8]. On the other hand, jumping prey take advantage of the fact that predators can lose visual contact and are thus unable to predict the re-entry position due to light refraction across the water–air interface [1].

The aforementioned examples of aquatic species have relatively large characteristic length scales varying from several centimetres to several metres. In contrast to these large animals, a few planktonic species (i.e. copepods) less than 3 mm in length have been observed to successfully jump to avoid predatory fish [9]. The primary benefit of jumping is a reduced drag force in air relative to water. Lower drag allows plankton to temporarily achieve greater velocities and cover longer distances [9]. This is important to minimize the chance of remaining in the predator's perceptive field upon re-entry into the water. Unlike larger animals whose inertia and gravity are large enough to neglect the surface tension, plankton need to overcome the surface tension of the water–air interface in order to break through [9]. While detailed observations of this behaviour have been made, the question of whether observed escape speeds alone are sufficient to break the surface remains unresolved.

Surface tension force is proportional to the characteristic body length (l), while gravity is proportional to its volume (approx. l³). The capillary length is defined as the resultant length scale from balancing these two forces (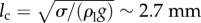). Here, ρ_l and σ are the density and the surface tension coefficient of water, respectively. For animals larger than l_c, gravity is the only important barrier that must be overcome in order to make an aerial jump [10]. However, for smaller organisms like plankton with l ≤ l_c, the surface tension force becomes a critical factor in breaking the water surface. To overcome the surface tension force, animals should generate high enough jumping speeds or inertia. For example, Labidocera aestiva with l ∼ 3 mm is able to jump out of the water with high speeds, approximately 1 m s⁻¹, whereas the similarly sized Daphnia magna cannot break the water surface when moving at low speeds, approximately 0.1 m s⁻¹ (see electronic supplementary material, movie S1). Despite a number of studies describing animals interacting with and breaking through the water–air interface, the underlying fluid mechanics needs to be better resolved to rationalize the dynamical and critical behaviour between jumping and non-jumping plankton.

Moreover, even though this study is motivated by biological questions, the dynamics of a particle penetrating a fluid interface shares a common framework with multiphase separation processes present in the oil industry [11]. For example, a technique called ‘flotation’ employs air bubbles to extract oil or valuable ores from oil shale or raw minerals [11–13]. An underlying mechanism in the flotation processes is interaction and subsequent attachment of a particle on either a liquid–liquid or a liquid–air interface, which share similar physical ingredients with this study [14].

In this study, we investigate the minimum inertia required for an object to jump out of water against gravity and surface tension. First, we designed and performed physical experiments of shooting spherical particles towards the liquid surface to mimic planktonic copepods jumping as described in §2. In §3.1, we have developed a simple theoretical model using a force balance that describes the instant at which the particle impacts the interface. This model allows us to estimate the critical impact velocity in §3.2. Section 3.2 shows that dynamic criteria from our model are in good agreement with the particle shooting experiments as well as the existing plankton jumping data. The summary and future directions are included in §4.

2. Material and methods

2.1. Materials

Physical experiments were executed using spherical particles to represent plankton as depicted in figure 1c–e. We used three different types of particles, purchased from McMaster-Carr, consisting of polymer, steel and tungsten, with densities, ρ_s, of 1336, 7935 and 19 250 kg m⁻³, respectively. The sphere diameters (D) were 1.6, 2.0, 2.1, 2.2, 2.4, 2.8, 3.2, 3.6, 4.0, 4.8, 5.6 and 6.4 mm. Furthermore, two different liquids, distilled water and ethanol, were used to study the effect of liquid–air surface tension, σ, of 0.073 and 0.022 N m⁻¹, respectively.

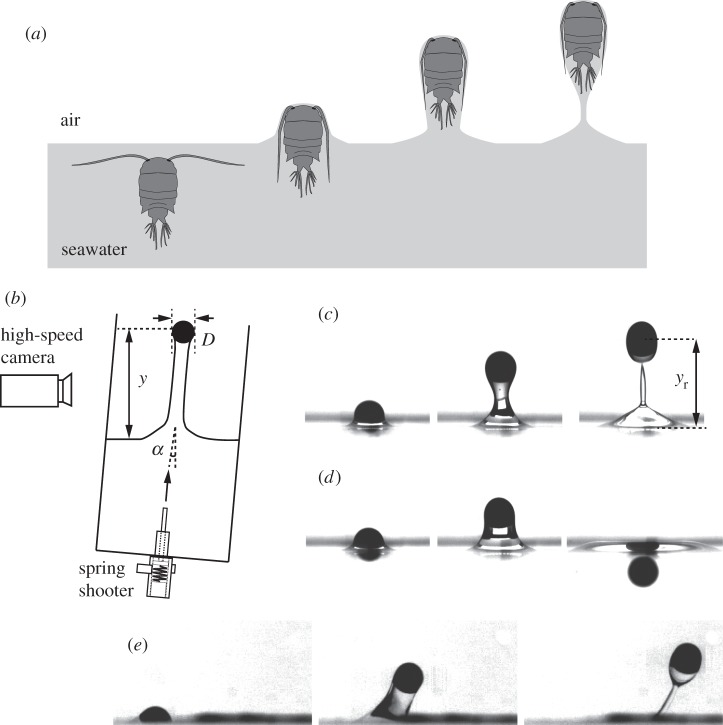
Figure 1.

(a) Schematic of a plankton jumping out of seawater. (b) Schematic of physical experiments. (c,d) Sequential experimental images of shooting experiments of the polymer sphere with D = 2.4 mm impacting the water–air interface with α = 0° which show qualitatively different behaviours of (c) penetration through the interface when V_im = 0.77 m s⁻¹ and (d) bouncing-off when V_im = 0.60 m s⁻¹ (see electronic supplementary material, movie S1). (e) The penetration of a steel sphere with D = 2.8 mm, α = 36° and V_im = 0.49 m s⁻¹.

The jumping plankton of L. aestiva and the non-jumping plankton of D. magna, Pseudodiaptomus salina and Acartia tonsa were also used in the experiments. Data for L. aestiva [9] and A. tonsa [15] were re-collected from previously published data. Daphnia magna and P. salina were obtained from Carolina Biological and AlgaGen L.L.C., respectively, and their behaviours were recorded in the laboratory using a high-speed camera (MotionXtra N3/Integrated Design Tools, Inc.) at a frame rate up to 500 Hz. For the plankton data, the diameter scale of D is estimated as the mean value of major and minor lengths of the elliptic body, and the body density is approximated to the density of seawater, ρ_l = 1027 kg m⁻³, and the surface tension of air–seawater is given by σ = 0.075 N m⁻¹ [9,16].

2.2. Experimental set-up and method

To shoot the particles, a spring system was designed, a schematic of which is shown in figure 1b. Another set of experiments was performed to quantify surface deformations. In the latter experiments, a linear stage (BiSlide MB10-0150/Velmex, Inc.) was used to move the particles with constant speed as shown in figure 2a. In our experiments, the impact velocity, V_im, on the liquid–air interface was controlled to be in the range of 0.074–1.8 m s⁻¹ by adjusting the compressed length of the spring or the input of the linear stage. Corresponding Reynolds number of 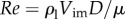ranged from 240 to 4950 in the experiment, and the impact angle of α was controlled up to 36° by tilting the spring shooter and the liquid tank. As each particle was set in motion, the position (y) of the particle and the deformation of the liquid–air interface were recorded with a high-speed camera at a frame rate up to 3000 Hz. Then, the position of a particle and the interfacial deformation were tracked and estimated with an in-house Mathematica code. Figure 1c,d shows the representative image sequences of shooting experiments with α = 0° for (c) the penetration case, in which the particle exhibits the ‘jumping-out’ behaviour, and (d) the bouncing case, which is representative of ‘non-jumping’ plankton. Figure 1e shows the penetration case of a steel particle with D = 2.8 mm and V_im = 0.49 m s⁻¹ impacting the water–air interface with α = 36°. For the biological experiments, in order to stimulate a plankton to jump out of water, a photic startle response was performed by a rapid change in light intensity [15].

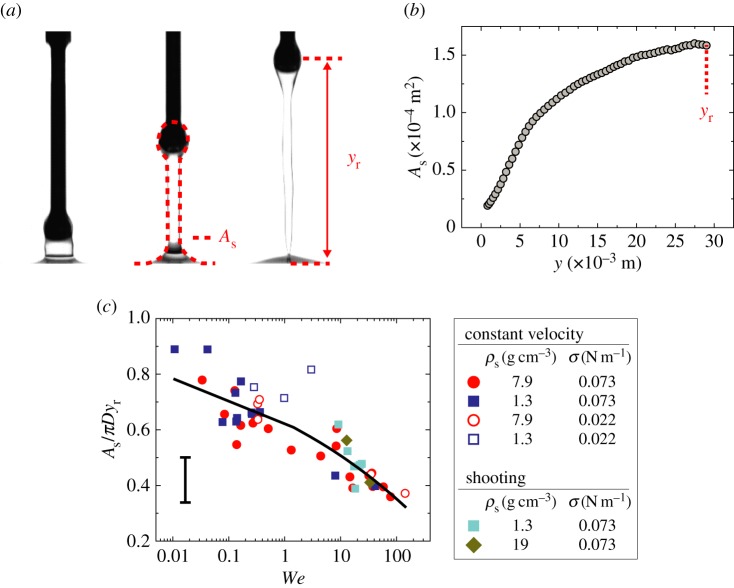
Figure 2.

Linear stage experiment pulling up the attached particle with constant speed. (a) Experimental images, where the red dotted line in the middle image indicates the interfacial area of A_s and y_r in the right image is the rupturing position. (b) The typical spatial evolution of surface deformation up to y_r, where the steel particle of D = 4.0 mm impacts the water–air interface with V_im = 1.0 m s⁻¹. (c) Normalized surface deformation rate as the function of a single dimensionless variable, We. The characteristic error bar is estimated based on experimental errors due to optical distortions near the free surface. (Online version in colour.)

3. Results

3.1. Governing equation of motion

A particle impacting a liquid–air interface can be described by the following equation of motion along the vertical direction as a function of its vertical position, y:

3.1

where m_s is the particle mass, m_l is the liquid mass displaced by the particle and a is the particle acceleration. Forcing terms consist of the surface tension force, F_s, and the drag force acting on the particle, F_d. The added mass effect arises when the surrounding fluid is accelerated as a consequence of the acceleration of the solid particle. In this study, the added mass for a spherical particle, m_a, is assumed to be m_a = 0.5m_l, which has been previously used in fully immersed cases [17]. It should be noted that m_a is presumably a decreasing function of time, t, due to the combined effects of the interface and gravity. However, despite its dependency on t, the leading-order estimate of m_a = 0.5 m_l does not appear to affect the overall result analogous to the water-entry problem [18].

The surface tension force, F_s, resists the deformation of the liquid–air interface. Note that the apparent contact line on the particle is not observed in the present experiments. Therefore, we cannot use the uncompensated Young's force to calculate the surface tension force, as has been done in examples of falling particles with a manifest liquid–air interface [19]. Moreover, compared to similar examples of the water-entry problem, which has been analytically solved by virtue of the radial flow approximation with well-posed boundary conditions [18,20], the liquid column deformation entrained by a sphere in the present system is difficult to estimate analytically due to an unknown boundary condition at the bottom of the column. Therefore, we attempt to estimate F_s based on the gradient in surface energy, namely 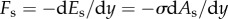, where E_s is the surface energy and A_s is the liquid–air interfacial area. A_s is calculated by assuming that the deformed interface is axis-symmetric along the vertical axis. The liquid–air area is expressed as 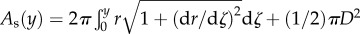, where r and ζ are the radial and vertical coordinates of the interface, respectively. Figure 2b shows the plot of A_s as a function of y, to illustrate the typical evolution of an deforming interface during impact; its slope (∂A_s/∂y) is the direct measure of F_s when multiplied by σ. It is observed that, for small y, A_s increases quadratically with y, as a similar behaviour has been observed in the deformation of a soap film impacted by a liquid droplet [21]. However, as the particle moves up further ( 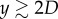as in figure 2b), the nonlinear behaviour of A_s takes place presumably due to the liquid column drainage, resulting in a decreasing value of ∂A_s/∂y.

To simplify the problem, we average the gradient of interfacial area up to the rupturing position, y_r, as 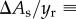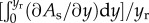, which leads to 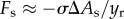. Then, based on the dimensionless analysis with constant speed, 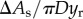can be correlated to two-dimensionless parameters from the five experimental parameters ρ_l, σ, V_im, D and g. The first is the Weber number, 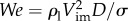, and the second is the Froude number, 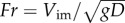. The Weber number quantifies the relative magnitude of inertia over surface tension, while the Froude number represents the relative magnitude of inertia over gravity. In our experiments, the range of particle size (1.6–6.4 mm) was chosen to be of the same order of magnitude as zooplankton (0.78–3.3 mm). This small range of particle size around the capillary length (D ∼ l_c) yields We ∼ Fr² due to the lack of size dependency. Therefore, we may eliminate one of the two-dimensionless parameters and use only We as the primary dimensionless variable in the analysis to follow. Then, we can empirically find the mean interfacial deformation rate (ΔA_s/y_r) as a function of We, after normalizing by πD:

3.2

where k_s1 and k_s2 are determined to be 0.38 and 0.12, respectively, from the best fit in figure 2c. The empirical relation above states that the surface tension force increases with D and inversely with the Weber number. As We increases, the second term in equation (3.2) captures the gradual reduction of the column's diameter with an increase of We due to the gravitational and capillary drainage. The scatter in figure 2c is attributed to experimental errors in estimating A_s due to optical distortions near the free surface, especially in the small Weber number limit.

In addition to the interfacial resistance from above, the surrounding fluid near the particle provides a drag force, F_d. In general, drag force is estimated using the empirical relation, 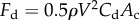, where C_d is the drag coefficient and A_c is the projected cross-sectional area normal to the direction of motion. Our present situation is further complicated by the fact that the particle is impacting the liquid–air interface. In this study, the drag is assumed to be 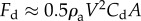, where ρ_a is the air density. The experimental results in the next section will show the validation of this drag assumption. Then, according to characteristic experimental parameters, F_d is estimated to be at least two orders of magnitudes smaller than the other resistances and is, hence, neglected.

Therefore, equation (3.1) can be rewritten as

3.3

This equation will be non-dimensionalized with 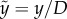and 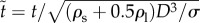, where the tilde indicates the dimensionless quantity. With 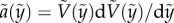based on the chain rule, equation (3.3) becomes a simple first-order differential equation for the solid velocity, :

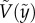3.4

where Bond number, 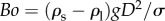, represents the ratio of gravity over surface tension force. By noting 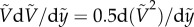, equation (3.4) states that the spatial rate of change in the kinetic energy of a particle is given by the change in the gravitational and capillary potential energies. Equation (3.4) with the initial condition, 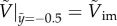, yields the following solution;

3.5

where 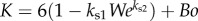. Here, K can be interpreted as the estimate of resistance to the upward motion of the sphere, which decreases with We but increases with Bo. Also, the ± sign indicates the solutions that describe the velocity of either a rising or falling particle.

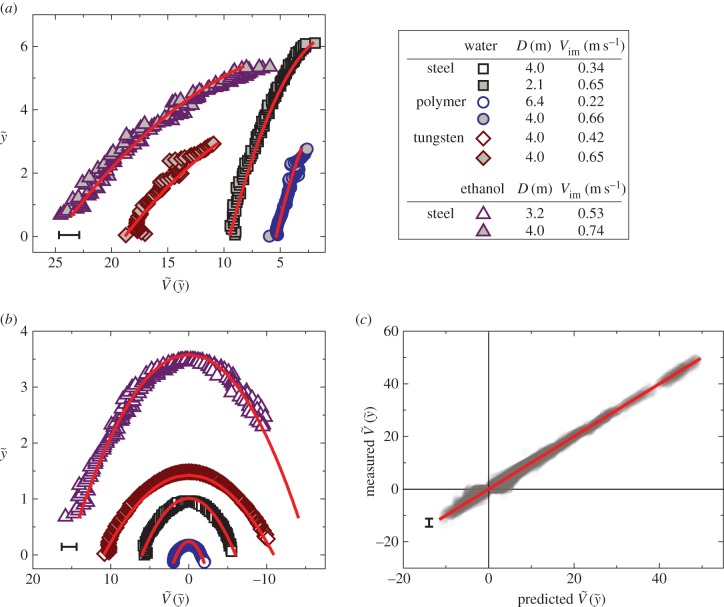
The particle shooting experiments have been performed to verify the solution of equation (3.5). Figure 3a,b shows the plot of the particle position (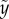) versus its corresponding vertical speed (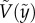) of eight representative cases: four penetration and four bouncing-off cases. Here, the red curves are from the predicted 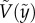as in equation (3.5). All experimental velocities (N = 102) are presented with respect to predicted velocities from equation (3.5) in figure 3c, which shows good agreement. This implies that our assumptions of neglecting drag and using the averaged interfacial area are able to capture the particle dynamics for both penetration and bouncing cases.

Figure 3.

(a,b) Plots of representative eight cases; (a) four penetration cases and (b) four bouncing-off cases in terms of the vertical position (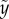) and the vertical speed 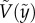. Symbols were experimentally measured values. Red curves indicate the theoretical predictions based on equation (3.5). (c) Comparison of experimentally measured 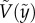and predicted 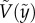by equation (3.5) over 102 different experiments. The red straight line indicates the perfect matching with the prediction. The characteristic error bars are estimated based on experimental errors due to optical distortions near the free surface. (Online version in colour.)

3.2. Critical impact velocity

Two states of particular interest are penetration (or jumping-out) and bouncing-off (or non-jumping). Equation (3.5) is rearranged to estimate the maximum height reached by the particle as

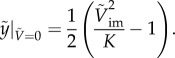3.6

The penetration regime corresponds to the case in which the rupturing height of the liquid column must be less than the maximum height reached by the particle, thereby yielding 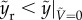. For bouncing-off case, 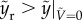. To explicitly express the transition from bouncing-off to penetration, the rupturing height 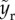needs to be determined.

To further investigate the rupturing height in different regimes, we experimentally pull up a particle at constant speed using the linear stage: quasi-static regime when We < 1 and dynamic regime when We > 1. Rupture in the quasi-static regime is primarily driven by the capillary force [22,23], which shows y_r ∼ D and independent of We (figure 4a).

Figure 4.

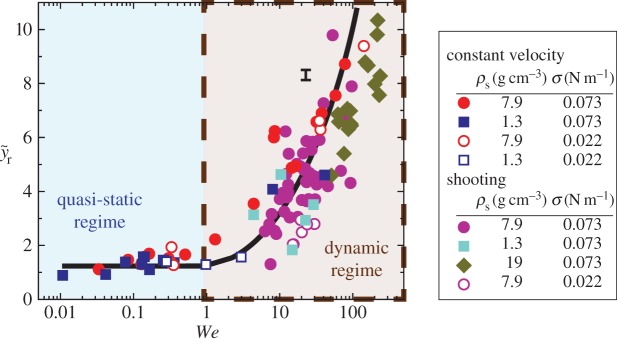
The ruptured position data for shooting and linear stage experiments with constant rise velocity. Two different regimes of quasi-static and dynamic rupture depending on We. The characteristic error bar is estimated based on experimental errors due to optical distortions near the free surface. (Online version in colour.)

When We > 1, the rupturing position varies with inertia. To understand 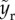in the regime of We > 1, two different timescales are considered: the rising timescale, y_r/V_im, and the pinch-off capillary timescale, 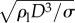[24]. When the two timescales are balanced, the dimensionless rupturing height becomes 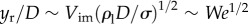. Therefore, the rupturing position can be expressed in a simple form with a prefactor, k_r,

3.7

Experiments with a constant rising velocity yield k_r = 1.01, or close to unity, with a moderate adjusted R² = 0.39. All experiments of penetrating shooting particles belong to the dynamic regime, or We >1, as shown in figure 4. The scatter in the particle shooting data might be due to the particle deceleration which is not accounted for in equation (3.7).

Combining equations (3.7) and (3.6), the critical impact velocity can be computed by solving

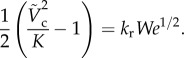3.8

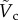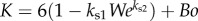Please note that both K and We are also functions of as and 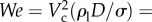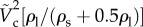, with 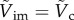at the critical condition. This allows us to construct a phase diagram that categorizes the penetration (light blue area) and the bouncing-off cases (light red area) of the particle, as well as the jumping plankton (L. aestiva) and the non-jumping plankton (D. magna, P. salina and A. tonsa) as shown in figure 5a. Different plankton regions are highlighted by ellipses with different colours (blue for the jumping and red for the non-jumping). The physical data of the plankton are listed in table 1. The phase diagram shows reasonable agreement with the theoretical prediction. Furthermore, the ratio of the impact velocity to the critical velocity is plotted against an impact angle (α) from the vertical axis in figure 5b. Experimental results show that our model predicts the transition well regardless of α in the range of 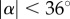. It indicates that the vertical force balance we have developed here is valid, independent of the horizontal force balance. Equation (3.8) shows the dependency of 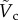on the two-dimensionless quantities of DR and Bo, where DR is the density ratio of 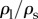. When DR is fixed as constant, 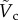becomes dependent on Bo only. For the specific case of DR = 0.13 corresponding to the case of the steel particle, 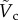is plotted as a solid curve in the inset of figure 5a. For 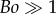, equation (3.8) can be approximated as 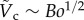, which is illustrated as the triangular slope in the inset of figure 5a.

Figure 5.

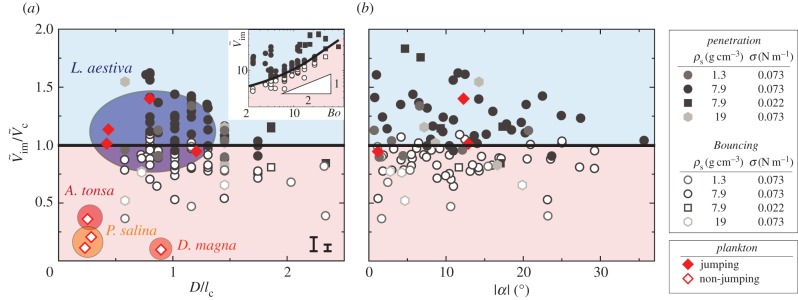
The phase diagram of the penetration case (light blue area) through the interface and bouncing-off case (light red area) from the interface of the particles, as well as the jumping plankton (L. aestiva) and the non-jumping plankton (D. magna, P. salina and A. tonsa), where D is scaled by the capillary length of 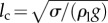. Different plankton regions are highlighted by ellipses with different colours (blue for the jumping and red for the non-jumping). The inset in (a) shows 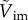versus Bo when 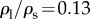and a solid curve for 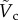. Two characteristic error bars for 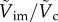are estimated based on experimental errors due to optical distortions near the free surface (left) and 50% reduced added mass (right). (Online version in colour.)

Table 1.

Physical properties of the plankton.

		lengths (mm)		velocity (m s−1)a
		major	minor
jumping plankton	Labidocera aestiva	1.7–4.6	0.62–2.0	0.6–1.1
non-jumping plankton	Daphnia magna	3.5	1.4	0.071
	Pseudodiaptomus salina	0.87–1.1	0.39–0.43	0.16–0.27
	Acartia tonsa	1.0	0.38	0.50

^aIt is estimated by the impact velocity for the jumping plankton and by the maximum swimming velocity for the non-jumping plankton.

4. Conclusion

Physical experiments of a particle impacting the liquid–air interface have been performed to elucidate how different physical properties, such as body size and vertical velocity, affect the jumping and non-jumping plankton. The simple model derived from the force balance equation describes the particle trajectories for both penetration and bouncing-off cases. Surface tension plays a crucial role in resisting the particle's penetration by deforming the liquid–air interface. Furthermore, the scaling analysis of the rupturing position has allowed us to acquire an estimate of the critical impact velocity, which categorizes the jumping and non-jumping plankton. The experimental results based on the shooting particle and the jumping plankton verify our predicted critical velocity () to be a reasonable estimate and provide a physical basis for the plankton's minimum impact speed required to jump out of the water.

The results of these experiments may also suggest a plausible explanation as to why neustonic copepods tend to be larger than most other species of copepod. Even if moving at a similar relative speed (0.5–0.8 m s⁻¹), the results show that a small copepod (≈1 mm) will not possess enough kinetic energy to break the surface tension and instead bounce back. This would be highly problematic for copepods living at the surface because not only do they have pigmentation as protection from UV exposure [25], which makes them more visually conspicuous to visual fish predators [26], but escape options are limited as predators approach from below. Thus, while a larger body size may increase visual conspicuousness, it could have been positively selected due to the ability to break the surface tension and make an aerial jump.

Additionally, the results suggest that it is unlikely that aerial jumping copepods require special adaptations to their body surface properties in order to make it easier for them to jump out of the water. Without properties such as super-hydrophobicity, which is a well-known feature of the integument of water-walking arthropods [27], to increase the contact angle or chemicals produced by the copepods to reduce surface tension, polymer spheres that possess similar size and mass to copepods can break the surface when travelling at comparable speeds to live copepods. Even though the present work is motivated by plankton, the illustrated theoretical model is relevant across multiple disciplines, due to the fundamental interest in the particle–interface interaction and the corresponding potential engineering applications.

Authors' contributions

S.K., J.H. and S.J. performed the physical experiments; S.K., S.L. and S.J. did the theoretical work; S.K., B.J.G. and S.J. performed the biological experiments: S.K., J.H., B.J.G., S.L. and S.J. wrote the paper.

Competing interests

We declare we have no competing interests.

Funding

This work was supported by the National Science Foundation (PHY-1205642, CBET-1336038) and VT-ICTAS.