The evolution of avian wing shape and previously unrecognized trends in covert feathering

Abstract

Avian wing shape has been related to flight performance, migration, foraging behaviour and display. Historically, linear measurements of the feathered aerofoil and skeletal proportions have been used to describe this shape. While the distribution of covert feathers, layered over the anterior wing, has long been assumed to contribute to aerofoil properties, to our knowledge no previous studies of trends in avian wing shape assessed their variation. Here, these trends are explored using a geometric–morphometric approach with landmarks describing the wing outline as well as the extent of dorsal and ventral covert feathers for 105 avian species. We find that most of the observed variation is explained by phylogeny and ecology but shows only a weak relationship with previously described flight style categories, wing loading and an investigated set of aerodynamic variables. Most of the recovered variation is in greater primary covert feather extent, followed by secondary feather length and the shape of the wing tip. Although often considered a plastic character strongly linked to flight style, the estimated ancestral wing morphology is found to be generally conservative among basal parts of most major avian lineages. The radiation of birds is characterized by successive diversification into largely distinct areas of morphospace. However, aquatic taxa show convergence in feathering despite differences in flight style, and songbirds move into a region of morphospace also occupied by basal taxa but at markedly different body sizes. These results have implications for the proposed inference of flight style in extinct taxa.

1. Introduction

Wing shape has been proposed to be related to many aspects of avian ecology and life history, including migratory behaviour [1–6], age-class [7,8], foraging behaviours and sexual selection [9–14]. Aerodynamic theory has also yielded an array of predictions regarding the relationship between flight performance and wing shape in birds [14–31]. To date, analyses of the relationship between wing shape and flight style or flight behaviour [5,19,20,23,31–34] have been based on simple numerical indices derived from linear measurements (e.g. wing length, wing chord, aspect ratio and wing pointedness) or used surface area estimates (e.g. wing loading). Other studies have investigated how skeletal measures and primary feather length relate to wing shape, flight style and wing kinematics [35–41]. However, many of these linear measurements have been found to be significantly related to size [15,22,36,37], and capture relatively little information about wing geometry [42]. By contrast, landmark-based morphometric methods assess shape independent of size [42]. Orientation (or rotation) and scale effects attributed to each specimen are removed during analysis [43], and subtle sources of variation that are not easily summarized by simple linear measurements are more completely captured [42,44–46]. Although landmark-based morphometric analyses have been widely used to investigate the relationships between form and function in insects [47], the sole previous study in birds investigated only six closely related species [46]. So far to our knowledge, no landmark-based morphometric analysis has been used to investigate broader patterns of variation in wing shape across Aves.

Previous work quantifying aerofoil shape using wing area, bony measurement proxies or geometric morphometric approaches has focused exclusively on the wing outline, described by the tips of primary, secondary and tertiary feathers. Covert feathers, layered over the anterior wing, cover large areas of both the dorsal and ventral wing surface and, in some taxa, extend nearly the length of the flight feathers (figure 1). While long assumed to contribute importantly to aerofoil shape [48,49], no previous assessment of wing geometry in Aves has considered their variation. Here, we use 90 dimensionless pseudo-landmarks to describe the wing outline as well as the tips of the greater upperwing (dorsal) and underwing (ventral) covert feathers (figure 1 and electronic supplementary material, Methods). To assess both functional and phylogenetic signal in our shape descriptors, branch lengths and topologies from two of the most-inclusive analyses of avian relationships were used [50,51].

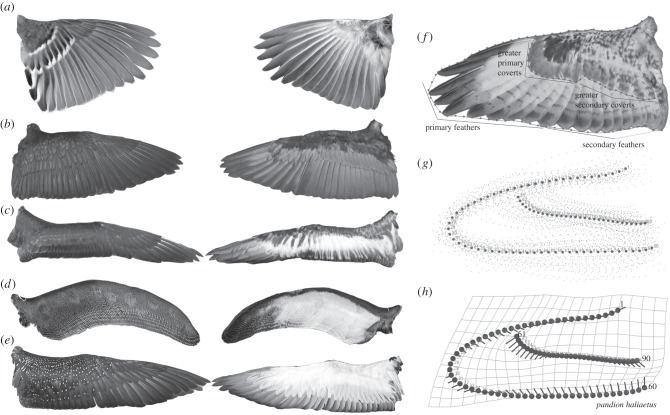
Figure 1.

Dorsal (left) and ventral (right) wings show variation in relative covert length and geometric morphometric assessment of this variation. Representative taxa and specimens: (a) Spizella arborea, American tree sparrow; (b) Phalacrocorax auritus, double-crested cormorant; (c) Phoebastria immutabilis, Laysan albatross; (d) Aptenodytes patagonicus, king penguin; (e) Gavia immer, common loon. Pseudo-landmarks plotted on all wings in the study (f); consensus wing shape based on 105-taxa, 90 pseudo-landmarks (g); an example of vector-based output used to visualize how shape differed from the consensus wing (h). Each landmark of the consensus wing represents the vector base, and arrows show the direction and magnitude of difference at any point along the wing. Wings are not to scale.

Here, we test the hypothesis that the wing outline and the covert distribution on the wing vary systematically with flight style, wing loading, body size and/or flight speed. Based on previous analyses using primarily linear measurements [20,30–34], we expected the most observed variation to be in the wing tip and wing outline. We had no a priori expectation for patterns of variation in the covert feathering; previously proposed hypotheses for covert function based on experimental data for single species (serving a sensory role [52], sealing off the bases of the flight feathers [53], as Krueger flaps [54]) have yielded no specific hypotheses concerning their variation across Aves. Whether the distribution of dorsal covert feathers on the wing would be expected to covary with that of the ventral has not been discussed. However, given their different proposed functions (e.g. dorsal covert feathers as playing a sensory role [52], and ventral covert feathers as lift enhancing devices [54]), they were expected to show distinct and independent patterns across Aves.

2. Results

(a). Covert and secondary feather length show more variation than the primary proportions

The majority of shape variation in both dorsal and ventral wing datasets is summarized by the first two principal component (PC) axes (62.93% and 15.5% of the total variance for the dorsal dataset; 64.4% and 13.09% for the ventral dataset (figure 2), respectively). Greater primary covert feather extent (relative to the trailing wing edge; figure 1) and secondary feather extent (relative to greater secondary covert extent) are most heavily weighted on PC1, which explain most of the variance (30.8% for the dorsal and 40.06% for the ventral primary coverts). PC2 explains much less of the variance and mainly reflects wing tip shape and secondary covert extent. The relative contributions of the landmarks are consistent with the results of the PC analyses; landmarks that describe the covert extent (landmarks 61–89) vary the most (ventral wing, 67.18%; dorsal wing, 60.8% of variation), with 40.06% (ventral) and 30.8% (dorsal) being explained by landmarks that describe greater primary coverts (landmarks 61–75). Landmark numbers are given in figure 1. Those describing the wing tip (landmarks 23–32) contribute 16.26% (ventral) and 24.2% (dorsal) of the observed variation. The rest of the landmarks contribute 15.56% (ventral) and 15% (dorsal).

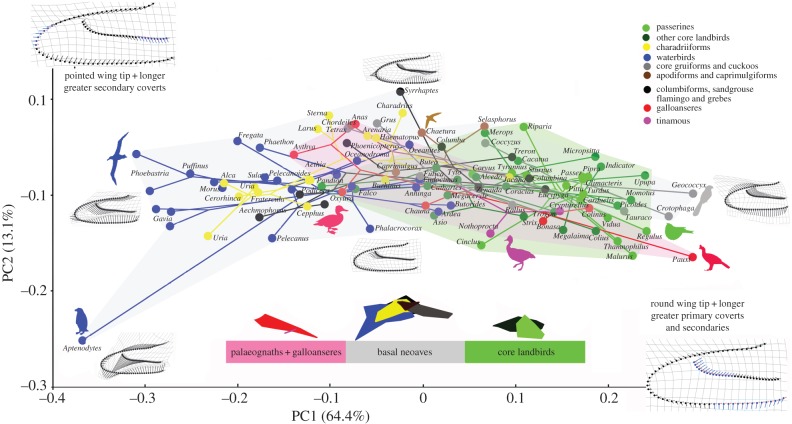
Figure 2.

Major avian clades plotted in a wing phylomorphospace described by principal components (PC) 1 and 2 for the 105 taxon sample. The phylogenetic tree [51] is mapped in this morphospace, with internal nodes placed according to a squared-change parsimony optimization [55]. Major changes in wing shape along the two PC axes are depicted on deformation grids from the consensus wing (insets). Each landmark on the consensus wing represents the vector base, and arrows show the direction and magnitude of variation at any point along the wing. In the wing insets, changes (blue) along PC1 reflect a shortening of the greater primary coverts and greater secondary feather length. Changes (blue) along PC2 reflect a narrowing of the wing tip. Dot colours for individual taxa reference major avian subclades recovered in Jarvis et al. [51] (see also key). The morphospace occupied basal and successively more nested clades in Aves is depicted along the base of the figure. Passerine birds and other core landbirds move into a morphospace occupied by more basal taxa but at significantly different body sizes.

We find that the relative extent of the greater dorsal covert feathers on the wing is strongly correlated with the ventral covert extent (r² = 0.89; p < 0.001), a pattern that is not predicted from the different sensory and aeroelastic functions previously proposed for these feathers [52–54]. Only a small number of species show differences in the relative length of these feathers and the extent of their coverage of the wing surface (figure 3). These taxa are ecologically diverse and are not phylogenetically clustered (figure 3). Diving taxa Aythya affinis, Aethia pusilla, Pelecanoides urinatrix and Gavia stellata, as well as the ruffed grouse, Bonasa umbellus, show markedly longer ventral coverts. By contrast, a heron (Ardea herodias), an antshrike (Thamnophilus caerulescens) and a sandgrouse (Syrrhaptes paradoxus) show distinctly longer dorsal coverts. These taxa do not differ significantly from other Aves in any of the evaluated flight parameters. Both the correlation of relative greater ventral and dorsal covert feather extent in the majority of avian taxa and the set of taxa deviating from this pattern, remain unexplained.

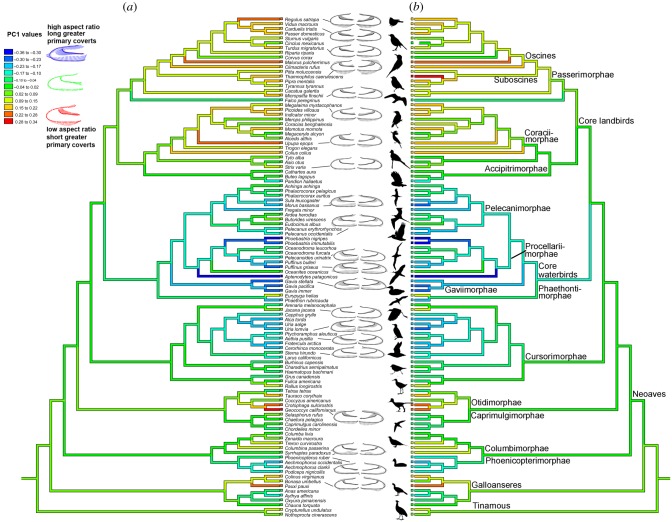
Figure 3.

Changes in wing shape across Aves. Ancestral state estimation for wing shape based on (a) ventral PC1 scores and (b) dorsal PC1 scores using weighted squared-change parsimony and the Jarvis et al. [51] phylogenetic tree. Vector-based output was used to visualize how shape differed across the phylogeny. Each landmark of the consensus wing represents the vector base, and arrows show the direction and magnitude of variation at any point along the wing. Anseriformes, Podicipediformes, Charadriiformes and waterbirds (Aequornithia) show a trend towards more elongate primary covert feathers and shorter secondary feathers, regardless of flight style or body size. Core ‘higher landbirds’ show a trend toward shorter primary coverts.

(b). Ancestral state reconstructions reveal phylogenetic and apparent ecological signal in wing shape

Ancestral state reconstructions of PC1 values for the dorsal and ventral wing show similar patterns (figure 3 and electronic supplementary material). In general, the greater primary coverts are short, and secondary feathers are relatively long in highly nested avian clades such as Passeriformes and Psittaciformes. By contrast, core waterbirds (Aquornithia of [51]) and Charadriiformes are characterized by relatively longer greater primary coverts along with shorter secondary feathers (F = 65.7669, p = 0.001). Relatively more basal clades (e.g. Tinamidae, Galloanserae) show more variation in relative greater covert and secondary feather length (F = 3.5, p < 0.01). For example, short coverts and elongate secondary feathers are present in Tinamidae, Galliformes and Cuculidae, but long primary coverts and short secondaries are present in ducks (Anatidae) and grebes (Podicipediformes). These patterns hold, regardless of whether the Hackett et al. [50] or the Jarvis et al. [51] phylogeny and branch lengths are used (figure 3 and electronic supplementary material, S1).

Wing shape variation is significantly correlated with phylogeny for both the Hackett et al. [50] and the Jarvis et al. [51] phylogenies (electronic supplementary material, figure S2 and table 1). Procrustes coordinates that represent wing shape show significant phylogenetic signal in permutation test in MorphoJ (tree length = 1.43900338, p < 0.001). Pagel's lambda and Blomberg's K indicate that PC1, which describes the relative length, or extent, of the greater primary covert feathers, exhibits significant correlation with phylogeny. PC2, which describes primarily wing tip shape, is weakly correlated with phylogeny (table 1). A two-dimensional phylomorphospace ([59], PC1 versus PC2) for the ventral wing dataset is depicted in figure 2. Overall, we recover an increase in avian morphospace through time with basal clades occupying largely distinct areas of this morphospace. However, taxa typically referred to as ‘higher land birds’ including passerines move back into an area of morphospace also occupied by more basal taxa but at smaller body sizes (figure 2). Anseriformes lie in a part of morphospace otherwise occupied primarily by Charadriiformes and waterbirds.

Table 1.

Assessment of phylogenetic signal for wing shape (PC scores) using Pagel's lambda [56], Blomberg's K [57] and a permutation test [58] for the Hackett et al. [50], ‘H’ and Jarvis et al. [51], ‘J’ topologies. (Strong phylogenetic signal detected for (PC1d) dorsal and (PC1v) ventral PC1 values.)

PCs	Pagel's lambda						Blomberg's K
	lambda		log-likelihood		p-value		K		p-value
	H	J	H	J	H	J	H	J	H	J
PC1v	0.99	0.99	94.25	91.48	<0.001	<0.001	1.25	1.09	0.001	0.001
PC1d	0.97	0.98	91.4	89.7	<0.001	<0.001	1.1	0.96	0.001	0.001
PC2v	0.79	0.84	142.72	141.43	0.005	0.02	0.51	0.48	0.001	0.014
PC2d	0.72	0.78	139.41	138.68	0.01	0.10	0.51	0.51	0.007	0.003

Covert feathers vary with ecology but not with most identified flight style categories (figure 2 and electronic supplementary material, S2). We find that all included aquatic clades (e.g. all waterbirds, grebes, ducks, gulls, auks) have significantly longer greater coverts (relative to trailing wing edge) than all included taxa that occupy complex terrestrial environments such as forests (e.g. passerines, parrots, grouse, tinamous; F = 65.77, p = 0.001; F = 58.56, p = 0.001; but excluding more littoral/wading clades (e.g. charadriiforms and ciconiiforms; see the electronic supplementary material).

Elongate covert feathers are also a feature of specialized wing-propelled diving birds (t = −12.42, p = 0.003), such as penguins (Spheniscidae), auks and puffins (Alcidae), and diving-petrels, as well as of taxa that only occasionally show this locomotor mode (e.g. gannets and shearwaters; figure 2). Penguins have highly specialized, flipper-like forelimbs with stiff, elongate covert feathers that are closely packed into multiple layers and are not distinguishable from remiges in morphology (figure 1; [60]). However, ancestral state reconstructions support elongation of the covert feathers preceding the origin of penguins and further modifications linked to the evolution of this novel locomotor mode (figure 3). These results are consistent with innovations in wing shape and covert feathering predating the loss of penguin flight, a result consistent with shifts observed in linear proportions of bony elements of the wing early in the evolution of waterbirds [41].

We also find that in wing shape morphospace, loons, with distinctive long primary coverts, cluster with wing-propelled divers (e.g. auks, shearwaters, Morus) and are closer to penguins than any other taxon (figure 2). Whether this result may reflect wing proportions plesiomorphic for the waterbird clade or a functional signal is unclear as loons are foot propelled divers that have not been observed using the wing in underwater propulsion [41]. Similarities between loons and wing-propelled divers (especially penguins) are also seen in carpometacarpal morphology and forelimb proportions [41]. By contrast, the American dipper (Cinclus mexicanus), which also uses its forelimbs in underwater propulsion, [61], shares the wing shape and covert feather arrangement of other songbirds described as exhibiting ‘passerine-type’ flight [62].

(c). The relationship between wing shape, flight style and aerodynamic variables

Wing shape has been found to vary among bird species in relation to flight style and body size [5,15,22,30,31,62]. By contrast, here phylogenetically informed linear regressions did not find a strong relationship between PC1 and PC2 values and previously evaluated aerodynamic parameters (i.e. flight speed and wing beat frequency) for the taxa for which these data are available (Methods, electronic supplementary material, table S1 and figure S3). Traditional wing shape parameters (AR, aspect ratio; Q, wing loading: body mass/wing area) are found to be weakly correlated with PC1 values, but body mass is not found to be correlated with these values (electronic supplementary material, table S1 figure S3). Interestingly, it is only in the comparison of PC values with aerodynamic variables that phylogeny choice affects our results. With the whole genome tree of Jarvis et al. [51] r²-values for aspect ratio/PC1 for both the dorsal and ventral wing increase to 0.50 and 0.53 from 0.32 and 0.34, respectively, for the Hackett et al. [50] tree. By contrast, they are markedly lower for wing loading with this topology (electronic supplementary material, table S1 and figure S3).

A phylogenetic ANOVA shows that PC1 values (dorsal and ventral wing) vary significantly among four previously described flight styles (electronic supplementary material, table S2). However, further post hoc analyses show that PC1 values are only significantly different between taxa with ‘passerine-type’ (PT) flight [62] and taxa included in each of the other flight style categories (p = 0.006). However, this is the only flight style category that by definition [62] included all parts of a major avian subclade. No significant difference is recovered when any other flight style categories are compared with each other (electronic supplementary material, table S2).

(d). Implications for assessment of Archaeopteryx

While wing shape in fossil taxa has been commonly discussed, fossil preservation rarely allows detailed assessment of wing geometry or covert feather organization. The wing feathering of the Jurassic ‘urvogel’, Archaeopteryx, known from many exceptionally preserved specimens, has been actively debated [15,63–70]. Many studies have concluded that although the wing of Archaeopteryx may look ‘modern’ in general features, the arrangement, especially of the covert feathers, may have been different from those of living birds [64–66]. Specifically, some authors proposed that the coverts were almost the length of the primaries [67]. These hypotheses were recently revisited in the light of the newly reported 11th specimen [69], and the proposed extremely elongate coverts [67] were assessed to be a taphonomic artefact [68,69]. The dorsal primary coverts have most recently been described [69] as about half the length of the primaries, consistent with the wing reconstructions by Rietschel [64] and Wellnhofer [66] based on the Berlin specimen. However, published reconstructions, including those by Rietschel and Wellnhofer, differ markedly in the shape of the wing tip and estimated secondary feather length from each of them (electronic supplementary material, figure S4).

Using the ventral wing construction of Rietschel [64] supported by the 11th specimen [69], Archaeopteryx is recovered in a space not occupied by any living taxa (electronic supplementary material, figure S4). Primary covert length is most similar to that of waterbirds and Charadriiformes. The proposal that elongate coverts, if present, are consistent with passive gliding in Archaeopteryx [67], is not supported based on comparison with extant taxa. As noted above, elongate coverts are differentially seen in aquatic taxa and are not correlated with gliding or soaring behaviours. While present in taxa like albatross, they are as commonly seen in taxa that flap continuously or use the wing in underwater propulsion (electronic supplementary material, S4 and figure 2). Further data on primary and secondary covert length are necessary to inform a new reconstruction of the Archaeopteryx wing or to infer its aerodynamic performance. For other fossil taxa lacking a fully extended wing, ratio descriptions of the greater primary or secondary covert length might be considered.

3. Discussion and conclusion

Aspect ratio, wing loading and wing tip shape have been considered to be strongly linked to flight style and aerodynamic performance [5,15,18,22,23,25,29]. However, here wing geometry is found to be only weakly correlated with previously described flight style categories and flight speed. Instead, strong phylogenetic signal is recovered (table 1). Consistent with these results, a previous study of cruising flight speed found that body mass and wing loading explained only limited variation in flight speed while phylogenetic affinities explained the most [71]. Wing loading, assessing the surface area of the wing relative to body weight, is recovered as only weakly related to the most heavily weighted variables in the PC analyses, relative primary covert length and secondary length (figure 2 and electronic supplementary material). Further, it shows no relationship with PC2, which primarily reflects wing tip shape and secondary covert length.

Low recovered variance in wing tip geometry across Aves was unexpected given previously noted variation [5,15]. However, the one other study that used geometric morphometrics to describe avian wing shape [46], also found that the curvature of the middle of the wing, described by its leading and trailing edges, and the width of the wing base explained the majority of observed variation (82.6%), whereas variation in wing tip shape was much more limited (13.3% in reference [46]). One explanation for this pattern may be the extreme biomechanical demands of flight; those wing shape characteristics (e.g. primary feathers, wing tip shape) that are functionally central to flight performance may not show as much variation among taxa precisely because of their aerodynamic importance. Our conclusions are limited, however, because differences in the wing tip owing to separation among distal primary feathers are not effectively captured by pseudo-landmark-based approaches (see the electronic supplementary material for explanation), and we may be underestimating total variation across Aves. For example, in most soaring birds (e.g. vultures, storks) and small agile taxa (e.g. wood warblers) separation of the primary tips, or slotting, functions to reduce induced drag [72].

Avian wing morphospace, as visualized in PC space, is characterized by successive expansions into novel areas of that morphospace in basal clades (figure 2). Passerines, by contrast, move back into a morphospace also occupied by basal taxa (Galliformes, Tinamidae) but at very different body sizes. Many characteristics, including a novel flight style [62], have been proposed to explain the impressive diversity within passerines, which make up approximately half of the species of the approximately 10 000 species of living birds. Here, we find that the wing shape associated with ‘passerine flight style’ (with short primary coverts and long secondary feathers) is significantly different from that of all other living birds when controlling for phylogeny (electronic supplementary material, Results). Wing geometry is estimated to evolve comparatively early in major clades during the radiation of living birds and to relatively rarely shift within clades even as flight behaviour changes. Thus, our results do not fit a model where the feathered portion of the wing is highly plastic and evolves readily or abruptly with changes in flight style and aerodynamic demands associated with those described flight styles.

Just as we observed marked clade-specific patterns in living birds, so we may expect early stem birds to show similar variation. However, we need to better understand potential developmental or phylogenetic constraints on the evolution of wing shape and feathering. The strong phylogenetic signal in wing shape and poor fit of wing geometry with described extant flight styles also problematizes the inference of aerodynamic performance or flight behaviour from descriptions of wing feathering in extinct taxa. Because extended wings are rarely well preserved, flight style in extinct taxa has long been inferred from linear skeletal measurements [35–41]. However, like the wing shape parameters investigated here, wing bone proportions have shown comparatively strong phylogenetic signal and weak functional signal [41,73]. There is a need for more detailed descriptors of flight behaviours and reassessment of existing flight style categories. Birds change wing shape in a single stroke and may employ more than one flight mode [49]; it is not clear that existing categories capture this variation. Investigated variables such as flight speed may vary significantly in these behaviours, and so far detailed data are lacking for many avian species.

In the avian flight literature, the variation in, and potential function of, covert feathering has been rarely and lightly discussed [22,25,36,48,49]. Only a handful of studies have treated these feathers in some detail, primarily in single species [52–54]. Specifically, Brown & Fedde [52] found that dorsal wing coverts on the propatagium are associated with mechanoreceptors and may function as air flow sensors. They did not explicitly comment on the greater coverts associated with the remiges. Muller & Patone [53] proposed that coverts may function in creating a more planiform wing by sealing off the base of the flight feathers. By contrast, Carruthers et al. [54] found the separation of the greater dorsal coverts during certain conditions and flight behaviours consistent with a sensory and/or aerodynamic function. The greater ventral coverts were proposed to operate as an automatic high-lift device, analogous to a Krueger flap on an aircraft during landing [54]. Here, we find three striking and previously unremarked patterns. First, covert feathers are among the most variable parts of the wing assessed (figure 2). Second, relative dorsal greater covert feather length tracks ventral covert length across birds with very few exceptions (figure 3). Third, in taxa showing a more aquatic habitus but varying in foraging behaviour and flight style, covert feathers are elongate and secondary feathers are relatively short. This trend is exaggerated in non-passerine taxa that co-opt the flight stroke for underwater propulsion but is supported as originating before the origin of this novel locomotor mode in both in Charadriiformes and waterbirds (figure 3).

Covert feather function clearly demands further experimental research. Current sensory and aeroelastic functions are insufficient to explain the observed variation. For example, we need to consider whether developmental or aerodynamic constraint may explain the similarity of relative dorsal and ventral covert extent across Aves; these feathers show different behaviour in flight and have different proposed functions [52–54]. Elongation of covert feathers in taxa with aquatic ecologies could be related to behavioural modifications linked to this ecology (e.g. take off from the water surface or take off angle), but the reason for such elongation remains wholly uninvestigated. The potential function of these feathers in affecting the wing profile and camber is important [64] but unexplored; the longest coverts are observed in taxa that use the wing effectively like a flipper in water, a medium much more dense and viscous than air. The shortest coverts are seen in passerine birds and are recovered associated with a flight style proposed to be distinctive in flap-gliding behaviours involving partially or fully flexed wings [62]. While linear or surface area measurements of the avian wing may be inadequate to describe the deformable and feathered aerofoils of birds, two-dimensional morphometrics offer a more detailed assessment of wing geometry and feathering. However, it is clear that three-dimensional approaches will be needed to capture the wing profile and to further investigate the potential function of coverts in creating a cambered wing.

4. Material and methods

(a). Dataset

Wing shape data were collected for 105 extant species from all major clades supported in recent avian phylogenies [50,51] (see the electronic supplementary material). All species are represented by male and female adults that were not in moult. Photographs of extended right spread wings are from the image collections of the Slater Museum of Natural History or were taken of specimens at the Burke Museum of Natural History (electronic supplementary material). All images were taken from directly above the wing; limited distortion was corrected in Photoshop using the ‘lens correction’ function. One to six specimens were sampled for each species depending upon availability (electronic supplementary material). Dorsal and ventral surfaces for each specimen were digitized twice.

(b). Morphometric analysis

We followed the methods of Brewer & Hertel [46], describing wing shape with a thin-plate spline and using 90 equally spaced points (pseudo-landmarks) with 60 points to define the wing outline and 30 points to define the distribution of covert feathers for both dorsal and ventral wing (figure 1). To obtain the points, we first obtained a dense sampling of points around the two curves (one for the wing outline, one for the coverts) by manually tracing, and then used interpolation methods to reduce this set of points to 60 (for wing outline) and 30 (for coverts) respectively for each curve [74–76]. Each tracing of the wing outline began at the most proximal anterior point and ended at the tip of the most proximal feather on the trailing edge of the wing. The curve delineating the coverts began at the tip of first greater primary covert and ended at the tip of the last greater secondary covert. These points provided a sufficient number of points to eliminate the need for sliding landmarks [46]. No linear dimensions were measured. The landmarks were digitized with tpsDig (v. 2.171, [77]). Datasets (one to six specimens) for each species were run through the tpsRelw program (v. 1.26) and combined into one consensus wing. This set of landmark data was used in subsequent analyses.

Given the size of the dataset we used here, the amount of variation among specimens was first assessed using tpsSmall [77] to make sure that it was not too large to use thin-plate spline methods (see below; figure 1). To quantify variation in wing morphology, we used general procrustes analysis (GPA; [78,79]). For the analysis, the second consensus wing for all species was first calculated using a generalized Procrustes superimposition analysis. All samples were then aligned to this consensus wing using generalized least-squares fitting [79]. Finally, differences in size within and among species, and variation in orientation of each wing were standardized by scaling each sample to centroid size at 1 (centroid size is a size measure calculated as the square root of the sum of the squared distances among all the landmarks in each configuration [80]). This procedure produced a set of GPA-corrected landmark coordinates, which were then converted into a covariance matrix and subjected to PC analysis. This method summarizes the multidimensional information generated by the superimposition in linear combinations (PCs) of the original variables (90 landmarks in two-dimensional yield 2 × 90 = 180 dimensions). The PC scores are orthogonal and they can be viewed as separate features of shape variation and interpreted biologically [81]. The combination of PCs yields a graphic ordination of the studied specimens (an empirical morphospace [82]), and the first two dimensions often account for the most meaningful information in the data. The PCs (coefficient values) were represented graphically using transformation grids based from the thin-plate spline interpolation function, with vectors showing changes in landmark position from average [80]. PCs for both ventral (PCsv) and dorsal wing (PCsd) were used in the analyses. All geometric morphometric analyses were performed with the software MorphoJ (v. 1.05f [83]). The relative contribution of each landmark was quantified using tpsRelw (v. 1.49 [77]). All analyses were done for both topologies ([50,51]; figure 1 and electronic supplementary material).

(c). Phylogenetic signal and ancestral state reconstruction

We used three methods to assess phylogenetic signal in the morphometric dataset based for two phylogenetic hypotheses for Aves ([50,51]; electronic supplementary material). Permutation tests were conducted in MorphoJ [58]. Test for Pagel's lambda [56] and Blomberg's K [57] were performed in R v. 3.0.1 [84] using the Phytools package (function phylosig, [85]). Mesquite (v. 2.75; [86]) was used to map PC 1 and 2 which contained the wing shape information onto the reference phylogeny. Each character was traced onto the tree using the ‘reconstruct ancestral state’ module of Mesquite with weighted squared-change parsimony [55]. See the electronic supplementary material for further information.

(d). Statistical analysis

All statistical analyses controlled for phylogeny. Phylogenetic ANOVA including a post hoc test (using the Holm–Bonferroni method) on means was conducted in R using the package Phytools [85] to see if wing shape (represented by PCs) was significantly different between any two flight styles or ecologies. Phylogenetic generalized least-squares were performed in R package Caper [87] to assess the relationship between wing shape (PCs) and aerodynamic parameters (i.e. aspect ratio, wing loading, wing beat frequency, flight speed). Wing shape (represented by PC values) was also regressed on body mass (electronic supplementary material). Taxa included in the ‘terrestrial’ and ‘aquatic’ ecological categories are given in the electronic supplementary material, table S3; phylogenetic ANOVA was run twice on the dataset using both the more and less inclusive ‘aquatic’ categories.

Body mass data are from Dunning [88]. Wing aspect ratio (AR, aspect ratio, wing span²/wing area) and wing loading (Q, wing loading, body mass/wing area) measurements for 54 taxa are from a dataset used in [89] and [62] (electronic supplementary material, table S4). Wingspan and wing area include the area of the body equal to the proximal wing chord. Cruising speed (V, 30 taxa) and wing beat frequency (Hz, 32 taxa) data are from the literature ([62,90,91]; electronic supplementary material, table S4). Four flight styles for living birds were defined most recently by Bruderer et al. [62]. These categories, ‘continuous flapping’; ‘flapping and soaring’; ‘flapping and gliding’ and ‘PT’ flight, and the assignment of taxa to these categories are based on this recent review by Bruderer et al. [62].

Ethics

All the specimens used in this study are from the spread-wing collection of the Burke Museum of Natural History and Culture. No live animals were used.

Data accessibility

Electronic supplementary material is available online.

Authors' contributions

J.A.C. and X.W. designed the study. X.W collected and analysed data and prepared figures. X.W and J.A.C. wrote the manuscript.

Competing interests

The authors declare no competing interests.

Funding

This project was funded by the National Science Foundation (DEB 0949897 and EAR 1355292 to J.A.C.).