Abstract
Many current research efforts in strongly correlated systems focus on the interplay between magnetism and superconductivity. Here we report on coexistence of both cooperative ordered states in recently discovered stoichiometric and fully inversion symmetric heavy fermion compound Ce3PdIn11 at ambient pressure. Thermodynamic and transport measurements reveal two successive magnetic transitions at T1 = 1.67 K and TN = 1.53 K into antiferromagnetic type of ordered states. Below Tc = 0.42 K the compound enters a superconducting state. The large initial slope of dBc2/dT ≈ – 8.6 T/K indicates that heavy quasiparticles form the Cooper pairs. The origin of the two magnetic transitions and the coexistence of magnetism and superconductivity is briefly discussed in the context of the coexistence of the two inequivalent Ce-sublattices in the unit cell of Ce3PdIn11 with different Kondo couplings to the conduction electrons.
The vast majority of cerium intermetallic compounds investigated to date have been compounds which have only one crystallographic site for the Ce-ion. As such, the physical properties of these dense Kondo lattices are determined by the competition between the magnetic inter-site interaction, i.e., the indirect RKKY exchange interaction, and the demagnetizing on-site Kondo interaction. This competition is well described by the Doniach diagram1; the ground state depends on the relative value of the respective RKKY and Kondo energies, 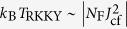 and
and  , with NF being the conduction band density of states at the Fermi level and Jcf the coupling constant (hybridization) between 4f (Ce) and conduction electrons. For small values of
, with NF being the conduction band density of states at the Fermi level and Jcf the coupling constant (hybridization) between 4f (Ce) and conduction electrons. For small values of 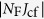 the compound’s ground state is magnetic while, for high values of
the compound’s ground state is magnetic while, for high values of 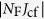 , the Kondo effect dominates and the ground state is nonmagnetic. The value of Jcf itself depends critically on the local environment of the 4f electron. This aspect plays a crucial role in compounds exhibiting inequivalent crystallographic Ce-sites per unit cell constituting different Ce–sublattices. The different local symmetry of each inequivalent Ce-ion results in a different hybridization strength and hence each corresponding Ce–sublattice is characterized by its own Kondo temperature. Therefore, it gives the possibility of having different ground states for each sublattice. Examples are for instance Ce7Ni3, Ce5Ni6In11 and Ce3Pd20Si6. The first-mentioned compound crystallizes in the hexagonal Th7Fe3 structure in which the Ce-ions reside in three inequivalent sites: (i) one atom in sublattice Ce1 (Wyckoff site 2b: 3 Ni and 12 Ce neighbors); (ii) three in sublattice Ce2 (6c: 4 Ni, 11 Ce) and (iii) three in sublattice Ce3 (6c: 4 Ni, 11 Ce but different distances than for Ce2). From thermodynamic and magnetic studies it was suggested that at low-T the Ce1 ions are responsible for antiferromagnetic (AFM) order at TN = 1.8 K, the Ce2 ions are associated with heavy fermion (HF) behavior (TK ≈ 4 K) and the Ce3 ones give rise to intermediate valence contributions2. The second compound, Ce5Ni6In11, has a base centered orthorhombic unit cell. There are ten Ce-ions per unit cell. They occupy two different Ce-sites. Eight Ce-ions sit at Wyckoff position 8p (Ce1) while the remaining two occupy the 2c site (Ce2). The compound has been classified as an intermediate HF system with γ ≈ 145 mJ/(molCe · K2) and exhibits two successive AFM magnetic phase transitions at TN2 = 1.10 K and TN1 = 0.63 K which have been attributed to the Ce1 and Ce2 sublattices, respectively3. The analysis of the entropy indicated a weak interaction between the two magnetic sublattices below TN2. The latter compound, Ce3Pd20Si6, has recently attracted much interest because of its unusual quantum critical behavior4. The compound crystallizes in the cubic structure of space group
, the Kondo effect dominates and the ground state is nonmagnetic. The value of Jcf itself depends critically on the local environment of the 4f electron. This aspect plays a crucial role in compounds exhibiting inequivalent crystallographic Ce-sites per unit cell constituting different Ce–sublattices. The different local symmetry of each inequivalent Ce-ion results in a different hybridization strength and hence each corresponding Ce–sublattice is characterized by its own Kondo temperature. Therefore, it gives the possibility of having different ground states for each sublattice. Examples are for instance Ce7Ni3, Ce5Ni6In11 and Ce3Pd20Si6. The first-mentioned compound crystallizes in the hexagonal Th7Fe3 structure in which the Ce-ions reside in three inequivalent sites: (i) one atom in sublattice Ce1 (Wyckoff site 2b: 3 Ni and 12 Ce neighbors); (ii) three in sublattice Ce2 (6c: 4 Ni, 11 Ce) and (iii) three in sublattice Ce3 (6c: 4 Ni, 11 Ce but different distances than for Ce2). From thermodynamic and magnetic studies it was suggested that at low-T the Ce1 ions are responsible for antiferromagnetic (AFM) order at TN = 1.8 K, the Ce2 ions are associated with heavy fermion (HF) behavior (TK ≈ 4 K) and the Ce3 ones give rise to intermediate valence contributions2. The second compound, Ce5Ni6In11, has a base centered orthorhombic unit cell. There are ten Ce-ions per unit cell. They occupy two different Ce-sites. Eight Ce-ions sit at Wyckoff position 8p (Ce1) while the remaining two occupy the 2c site (Ce2). The compound has been classified as an intermediate HF system with γ ≈ 145 mJ/(molCe · K2) and exhibits two successive AFM magnetic phase transitions at TN2 = 1.10 K and TN1 = 0.63 K which have been attributed to the Ce1 and Ce2 sublattices, respectively3. The analysis of the entropy indicated a weak interaction between the two magnetic sublattices below TN2. The latter compound, Ce3Pd20Si6, has recently attracted much interest because of its unusual quantum critical behavior4. The compound crystallizes in the cubic structure of space group 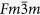 . It harbors two different crystallographic Ce-sites, both with cubic symmetry but one with coordination 16 (tetrahedral 8c: all Pd) and one with coordination 18 (octahedral 4a: 12 Pd, 6 Si). At T = 0.5 K, Ce3Pd20Si6 undergoes a quadrupolar phase transition and at TN = 0.31 K a transition in an AFM ordered state5. Each ordered state has been associated with a particular sublattice, meaning that the quadrupolar state and the dipolar coexist at low temperatures.
. It harbors two different crystallographic Ce-sites, both with cubic symmetry but one with coordination 16 (tetrahedral 8c: all Pd) and one with coordination 18 (octahedral 4a: 12 Pd, 6 Si). At T = 0.5 K, Ce3Pd20Si6 undergoes a quadrupolar phase transition and at TN = 0.31 K a transition in an AFM ordered state5. Each ordered state has been associated with a particular sublattice, meaning that the quadrupolar state and the dipolar coexist at low temperatures.
Yet, the field of Kondo lattice compounds with multiple distinct local moments (4f (Ce)) per unit cell is still uncharted terrain. This despite the fact that interplay between different ground states, which in these compounds might come from interaction between sublattices with different electronic or magnetic order, often give rise to new interesting phenomena. Indeed recent theoretical work of Benlarga et al. examines the question of a Kondo lattice with two distinct Kondo ions6. Their model describes a unit cell with two inequivalent Ce-sites. Each experiences different hybridization 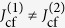 which reflects in different (sublattice) Kondo temperatures TK1≠TK2, respectively. For simplicity of the discussion it was assumed TK1>TK2
which reflects in different (sublattice) Kondo temperatures TK1≠TK2, respectively. For simplicity of the discussion it was assumed TK1>TK2
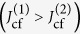 . Long-range magnetic ordering was induced by allowing for Ce1-Ce2 interaction and a schematic phase diagram has been constructed. Particular interesting becomes the situation TK1>T>TK2 where one enters a region in the phase diagram of so-called partial Kondo screening. Partial refers to the fact that only 4f (Ce1) moments are effectively screened by Kondo effect forming heavy particle states. Hence, as has been pointed out by the authors, any magnetic phase in this regime will manifest characteristics of a weakly polarized HF phase coexisting with properties of typical local-moment magnetism originating from the strongly polarized and little screened 4f (Ce2)-moments. The compound Ce7Ni3 mentioned earlier shows exactly such behavior. Although not explicitly discussed, in a broader sense one can speculate that under certain conditions the HF sublattice becomes superconducting while the second sublattice remains magnetically ordered.
. Long-range magnetic ordering was induced by allowing for Ce1-Ce2 interaction and a schematic phase diagram has been constructed. Particular interesting becomes the situation TK1>T>TK2 where one enters a region in the phase diagram of so-called partial Kondo screening. Partial refers to the fact that only 4f (Ce1) moments are effectively screened by Kondo effect forming heavy particle states. Hence, as has been pointed out by the authors, any magnetic phase in this regime will manifest characteristics of a weakly polarized HF phase coexisting with properties of typical local-moment magnetism originating from the strongly polarized and little screened 4f (Ce2)-moments. The compound Ce7Ni3 mentioned earlier shows exactly such behavior. Although not explicitly discussed, in a broader sense one can speculate that under certain conditions the HF sublattice becomes superconducting while the second sublattice remains magnetically ordered.
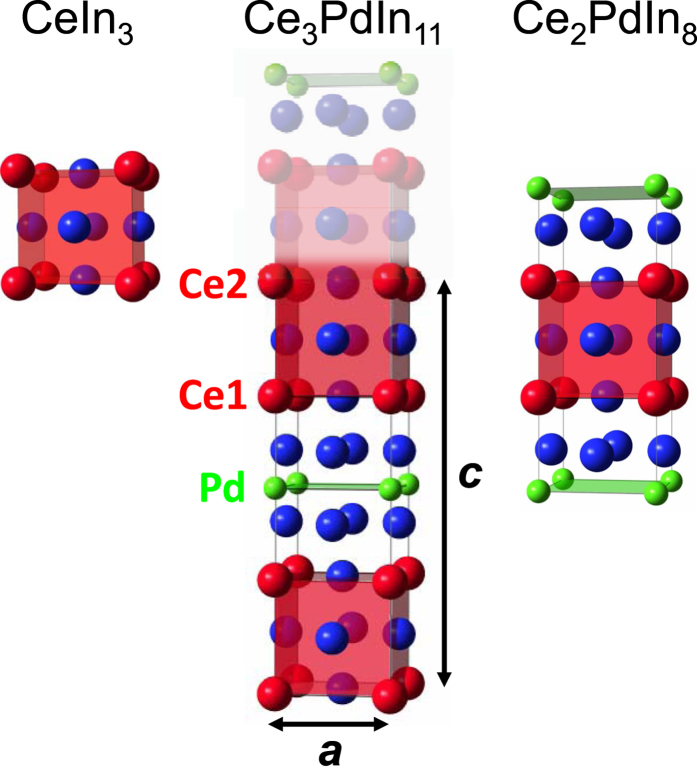
In this work we present first low-temperature magnetization, specific heat and resistivity measurements on the recently7,8 discovered HF system Ce3PdIn11. The compound belongs to the CenTmIn3n+2m class of layered materials which comprises a numerous amount of compounds including CeCoIn5, CeRhIn5 and Ce2RhIn8 9,10,11. Ce3PdIn11 can be regarded as “intermediate step” between the cubic CeIn3 which orders antiferromagnetically at TN = 10.1 K12 and tetragonal Ce2PdIn8 being a HF superconductor with Tc between 0.45 and 0.7 K depending on the crystal quality13,14,15,16. It crystallizes in the typical tetragonal structure (space group P4/mmm) based on the AuPt3-type (CeIn3-block) and PtHg2-type (Tin2) units alternating along the [001]-direction as depicted in the Fig. 1. The unit cell possesses two inequivalent crystallographic Ce-sites denoted by Ce1 and Ce2. What distinguishes Ce3PdIn11 from previously investigated multi-site compounds is that the Ce1–Ce1 nearest-neighbor distance is exactly equal to the Ce2–Ce2 nearest-neighbor distance. That means, the distance as a parameter to explain the various behaviors of the sublattices like in other compounds plays no role17. Instead, the ground state properties of the sublattices are solely determined by the respective hybridization of the Ce-ions in our compound. A second point which outrivals Ce3PdIn11 from previous multi-site Ce-compounds is that the local environment of both Ce-ions are not uniquely related to Ce3PdIn11 but already exists in closely related compounds. As displayed in Fig. 1, Ce2 resides in the 1a Wyckoff site (C4v symmetry) featuring CeIn3 environment, whereas the Ce1-site occupies the 2g position (D4h symmetry). Its surrounding atoms are identical with those of the Ce-ions in Ce2PdIn8. Preliminary results on Ce3PdIn11 revealed two successive magnetic transitions at T1 ≈ 1.7 K and TN ≈ 1.5 K and a pronounced enhancement of the Sommerfeld coefficient8. Our extended low-T experiments presented here show that below Tc = 0.42 K the compound enters a HF superconducting state, suggesting coexistence of long-range magnetic order and HF superconductivity in Ce3PdIn11 at ambient pressure.
Figure 1. The crystal structure of Ce3PdIn11 (middle) pointing out the two inequivalent Cerium sites Ce1 and Ce2.
The red shaded part emphasizes the CeIn3 building blocks. The lattice parameters yield a = 4.6896(11) Å and c = 16.891(3) Å. To show the local environment of the Ce2-ion more clearly, the unit cell has been extended along the [001]-direction (semi-transparent part). The unit cells of CeIn3 (left) and Ce2PdIn8 (right) are displayed for comparison.
Results
The electrical resistivity ρ(T) of Ce3PdIn11 with current applied along different crystallographic axes is shown in Fig. 2. At high temperatures above 80 K the resistivity ρ(T) is weakly temperature dependent with dρ/dT>0, passes through a shallow maximum at Tmax ≈ 60 K (j ‖ [100]) with lowering temperature, and then rapidly decreases signaling the formation of coherent Bloch states at low temperatures. At T = 2 K the resistivity reaches ρ(2 K) ~ 16 μΩ cm, 11 μΩ cm and 10 μΩ cm for j ‖ [100], ‖[001] and ‖[011], respectively. The overall behavior is similar to that reported from a previous study performed on polycrystalline material7. The room temperature resistivity value corresponds well to ours, i.e., ρ = 55 μΩ cm has been reported compared to 52 μΩ cm measured for j ‖ [100] measured in our study. The maximum structure at Tmax is at 30 K and more pronounced in the polycrystal. The low temperature resistivity value, however, differs significantly being worse for the polycrystal (ρ(2 K) ≈ 34 μΩ cm) which is an indication that our single crystal is of higher quality. From our single crystal data we observe that the resistivity is fairly isotropic despite the complex tetragonal structure.
Figure 2. The electrical resistivity ρ(T) of Ce3PdIn11 with current j || [100] (triangles), ||[110] (open squares) and ||[001] (circles) from room temperature down to 2 K.
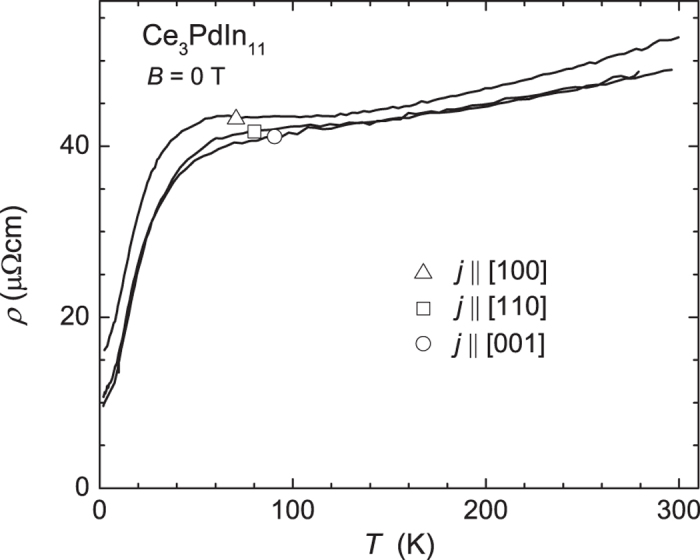
The residual resistivity ratio RRR = ρ300K/ρ2K equals 5.
The magnetic susceptibility χ(T) displays similar isotropic behavior. At T = 4 K the ratio between parallel and perpendicular [001]-axis yields χ[001]/χ[100] = 1.2. This suggests a strong influence of the cubic CeIn3 structure unit on the magnetic correlations in this compound. In Fig. 3 we plotted the inverse susceptibility 1/χ = B/M as function of temperature along both crystallographic directions in applied field of B = 1 T. For temperatures T >85 K and B ‖ [100] and for T >100 K and B ‖ [001] the susceptibility follows Curie-Weiss law. As inferred from Fig. 3 the data 1/χ(T) for both directions are parallel resulting in an equal effective moment of μeff = 2.43 μB per Ce-ion. The value is close to the Hund’s rule value of 2.54 μB for a free Ce3+ ion. In addition, because of the nearly magnetocrystalline isotropy of Ce3PdIn11 the extrapolated Weiss temperatures are close, yielding 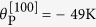 and
and 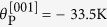 . The upper inset of Fig. 3 displays the low-temperature susceptibility for B ‖ [001] in greater detail. Below about 6 K χ(T) gradually deviates from the high-temperature behavior and exhibits a broad hump with a maximum at T = 2.4 K. In fact, Ce-based compounds (J = 5/2) commonly exhibit a low-T maximum in χ(T). This is expected from the theory of orbitally degenerate Kondo impurities18. However, we have to account for the crystal electric field (CEF) level scheme. In tetragonal crystal symmetry the J = 5/2 line splits into three doublets,
. The upper inset of Fig. 3 displays the low-temperature susceptibility for B ‖ [001] in greater detail. Below about 6 K χ(T) gradually deviates from the high-temperature behavior and exhibits a broad hump with a maximum at T = 2.4 K. In fact, Ce-based compounds (J = 5/2) commonly exhibit a low-T maximum in χ(T). This is expected from the theory of orbitally degenerate Kondo impurities18. However, we have to account for the crystal electric field (CEF) level scheme. In tetragonal crystal symmetry the J = 5/2 line splits into three doublets, 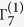 ,
, 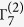 and Γ6. Assuming that the Ce1-ion in Ce3PdIn11 has similar CEF scheme as Ce in Ce2PdIn8, it follows that the first excited level is located at about ΔCEF ≈ 60 K13. The situation for Ce2-ion is more unclear. It exhibits tetragonal symmetry contrary to cubic symmetry of the Ce-ion in CeIn3. The first excited CEF level is at ΔCEF ≈ 140 K19. In a rough approximation we may assume a similar level splitting of the J = 5/2 line for Ce2. Because the Kondo temperature in Ce2PdIn8 and CeIn3 is much smaller than the CEF splitting being of the order of TK ≈ 10 K13,19 only the ground state doublet is relevant and the effective degeneracy of the moments becomes J = 1/2. In that case the Kondo effect produces a maximum in susceptibility at T = 0. We therefore attribute the maximum structure to the presence of short-range AFM correlations and determine the transition into long-range AFM ordered state in accordance with work of Fisher20: for a mean-field transition, the dc susceptibility should show a cusp at TN, whereas for classical critical behavior the derivative d(χT)/dT should diverge at TN with a specific exponent (i.e.,
and Γ6. Assuming that the Ce1-ion in Ce3PdIn11 has similar CEF scheme as Ce in Ce2PdIn8, it follows that the first excited level is located at about ΔCEF ≈ 60 K13. The situation for Ce2-ion is more unclear. It exhibits tetragonal symmetry contrary to cubic symmetry of the Ce-ion in CeIn3. The first excited CEF level is at ΔCEF ≈ 140 K19. In a rough approximation we may assume a similar level splitting of the J = 5/2 line for Ce2. Because the Kondo temperature in Ce2PdIn8 and CeIn3 is much smaller than the CEF splitting being of the order of TK ≈ 10 K13,19 only the ground state doublet is relevant and the effective degeneracy of the moments becomes J = 1/2. In that case the Kondo effect produces a maximum in susceptibility at T = 0. We therefore attribute the maximum structure to the presence of short-range AFM correlations and determine the transition into long-range AFM ordered state in accordance with work of Fisher20: for a mean-field transition, the dc susceptibility should show a cusp at TN, whereas for classical critical behavior the derivative d(χT)/dT should diverge at TN with a specific exponent (i.e.,  ) so that TN occurs at an inflection point below a rounded maximum. From specific heat measurements two inflection points in χ at T1 = 1.67 K and TN = 1.53 K can be expected8. We observe only one at approximately 1.45 ± 0.1 K as displayed in the lower inset of Fig. 3. The value coincides well with the lower specific heat jump at TN. The upper T1-anomaly seems not to be reflect in χ(T) (B ‖ [001]) but it is not excluded that it might be visible for perpendicular direction. Alternatively, the T1–signature is too small, and beyond resolution of our current measurement setup.
) so that TN occurs at an inflection point below a rounded maximum. From specific heat measurements two inflection points in χ at T1 = 1.67 K and TN = 1.53 K can be expected8. We observe only one at approximately 1.45 ± 0.1 K as displayed in the lower inset of Fig. 3. The value coincides well with the lower specific heat jump at TN. The upper T1-anomaly seems not to be reflect in χ(T) (B ‖ [001]) but it is not excluded that it might be visible for perpendicular direction. Alternatively, the T1–signature is too small, and beyond resolution of our current measurement setup.
Figure 3. Temperature dependence of the inverse of the susceptibilities 1/χ(T) of Ce3PdIn11 for the [100] (triangles) and [001] (circles) directions in an applied field of B = 1 T.
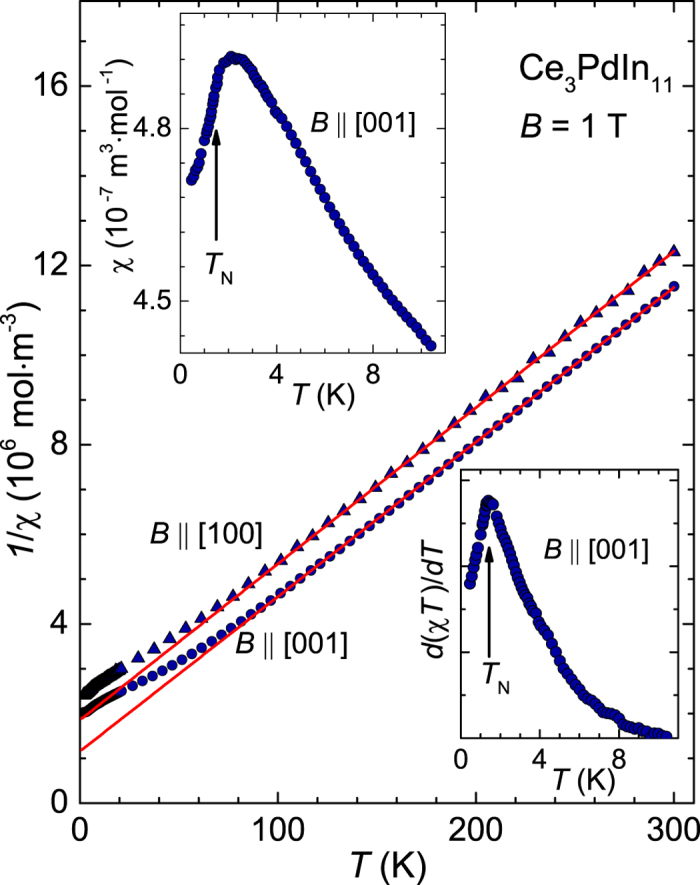
The solid (red) lines are Curie-Weiss fits to the data. The upper left inset shows the low temperature susceptibility for B ‖ [001] in more detail. The lower right inset displays the corresponding derivative d(χT)/dT.
We continued our investigation down to lower temperatures by means of specific heat (C/T) and resistivity experiments. Measurements were performed on the same single crystal to avoid sample dependent effects. In order to trace the associated anomalies and to complement the methods among each other the results of C(T)/T and ρ(T) in zero field are displayed one below the other in Fig. 4. The synthesis of the respective non-magnetic La and Y–compounds was unsuccessful so far. In order to retrieve the electronic part of the specific heat, Cel/T, we approximated the specific heat data in the interval 10<T<25 K by C(T)/T = Cphon/T + γ, where the Debye expression, 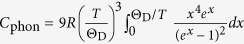 , accounts for the phononic and the Sommerfeld term (γ) for the the electronic contribution. The fit yields a characteristic temperature of ΘD ~ 182 K and γ = 0.890 J/(mol · K2). Cphon/T was subtracted from the total specific heat data and we obtained Cel/T presented in Fig. 4a. In our treatment we have disregarded the contribution arising from CEF. A more accurate analysis including this term is not possible because of the limited fitting interval and because of the fact that the exact CEF level scheme for Ce3PdIn11 is unknown. The CEF contribution, CCEF/T, is somehow captured by Cphon/T and γ. To give an estimate about CCEF/T we might take the CEF scheme of Ce2PdIn8 for comparison. The first excited level (ΔCEF ≈ 60 K) gives rise to a Schottky-type of anomaly with a maximum at around 25 K (CCEF/T ≈ 0.17 J/(molCe · K2))14. Below it falls off exponentially and at 10 K reaches a value of CCEF/T ≈ 0.05 J/(molCe · K2). We can assume that similar values are reached for Ce3PdIn11. This means, that our simplified fitting of the heat capacity data leads to an overestimation of the phononic and electronic contribution due to the inclusion of the CEF contribution. Towards lower temperatures, because CCEF/T and Cphon/T become small, the error in Cel/T displayed in Fig. 4 becomes negligible. Figure 4a shows three distinct features. Upon cooling down a first anomaly appears at T1 = 1.67 K which is immediately followed up by a second one at 1.53 K, being marked as TN and visible by a λ-like structure in the Cel/T data. Like in susceptibility, the higher transition T1 is not observed in ρ(T) within the accuracy of the measurement. TN is noticeable (Fig. 4b). It is manifested by a subtle change of the slope as shown more clearly by the temperature derivative d(ρ/ρ2K)/dT (inset of Fig. 4b). More intriguing is the third feature appearing in Cel/T at approximately 0.4 K. The jump marks the entrance into a superconducting phase, as it is evident from the corresponding resistivity data. The transition is fairly broaden which makes a proper analysis impossible. It resembles the first results on UPt3, where after improving sample quality it turned out that the broad specific heat jump actually consists of two very close superconducting jumps21. A more likely explanation is that the broadening results from an artifact caused by a strong increase of Cel/T of a normal state background. Consequently the expected decrease becomes compensated. Such an effect can be observed for instance in CeIr(In1−xCdx)5 22. To extract some numbers for comparison, we define the SC transition temperature being at half of the jump height, Tc = 0.42 K, which corresponds roughly with the onset of a second step in the resistivity data. The measured specific heat discontinuity, ΔC divided by the linear term in the specific heat at the transition (γTc) equals 0.73 (γ = 0.55 ± 0.03 J/(molCe · K2)). This value is well below that expected from BCS theory (ΔC/γTc = 1.43). However, such downsized number is not unusual when the transition is particularly broad, see for instance ref. 23,24.
, accounts for the phononic and the Sommerfeld term (γ) for the the electronic contribution. The fit yields a characteristic temperature of ΘD ~ 182 K and γ = 0.890 J/(mol · K2). Cphon/T was subtracted from the total specific heat data and we obtained Cel/T presented in Fig. 4a. In our treatment we have disregarded the contribution arising from CEF. A more accurate analysis including this term is not possible because of the limited fitting interval and because of the fact that the exact CEF level scheme for Ce3PdIn11 is unknown. The CEF contribution, CCEF/T, is somehow captured by Cphon/T and γ. To give an estimate about CCEF/T we might take the CEF scheme of Ce2PdIn8 for comparison. The first excited level (ΔCEF ≈ 60 K) gives rise to a Schottky-type of anomaly with a maximum at around 25 K (CCEF/T ≈ 0.17 J/(molCe · K2))14. Below it falls off exponentially and at 10 K reaches a value of CCEF/T ≈ 0.05 J/(molCe · K2). We can assume that similar values are reached for Ce3PdIn11. This means, that our simplified fitting of the heat capacity data leads to an overestimation of the phononic and electronic contribution due to the inclusion of the CEF contribution. Towards lower temperatures, because CCEF/T and Cphon/T become small, the error in Cel/T displayed in Fig. 4 becomes negligible. Figure 4a shows three distinct features. Upon cooling down a first anomaly appears at T1 = 1.67 K which is immediately followed up by a second one at 1.53 K, being marked as TN and visible by a λ-like structure in the Cel/T data. Like in susceptibility, the higher transition T1 is not observed in ρ(T) within the accuracy of the measurement. TN is noticeable (Fig. 4b). It is manifested by a subtle change of the slope as shown more clearly by the temperature derivative d(ρ/ρ2K)/dT (inset of Fig. 4b). More intriguing is the third feature appearing in Cel/T at approximately 0.4 K. The jump marks the entrance into a superconducting phase, as it is evident from the corresponding resistivity data. The transition is fairly broaden which makes a proper analysis impossible. It resembles the first results on UPt3, where after improving sample quality it turned out that the broad specific heat jump actually consists of two very close superconducting jumps21. A more likely explanation is that the broadening results from an artifact caused by a strong increase of Cel/T of a normal state background. Consequently the expected decrease becomes compensated. Such an effect can be observed for instance in CeIr(In1−xCdx)5 22. To extract some numbers for comparison, we define the SC transition temperature being at half of the jump height, Tc = 0.42 K, which corresponds roughly with the onset of a second step in the resistivity data. The measured specific heat discontinuity, ΔC divided by the linear term in the specific heat at the transition (γTc) equals 0.73 (γ = 0.55 ± 0.03 J/(molCe · K2)). This value is well below that expected from BCS theory (ΔC/γTc = 1.43). However, such downsized number is not unusual when the transition is particularly broad, see for instance ref. 23,24.
Figure 4. Zero field low-temperature results on Ce3PdIn11.
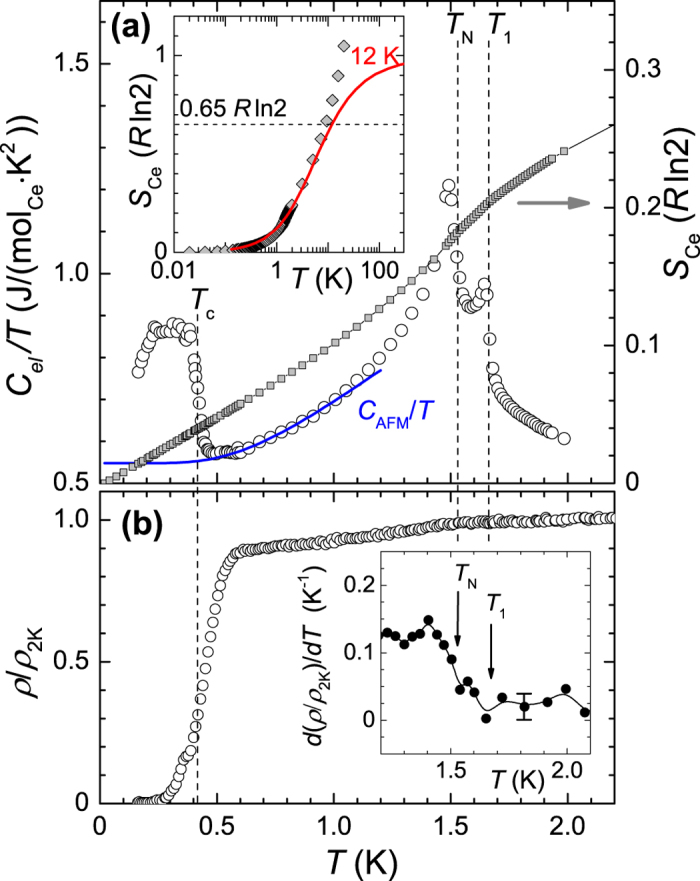
(a) the specific heat Cel/T vs. T (open symbols) in units of J/(molCe · K2). The transitions are clearly visible at T1 = 1.67 K, TN = 1.53 K and Tc = 0.42 K. The solid line represents the function 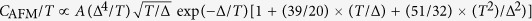 with values for A and Δ given in the text. The right ordinate displays the presumable magnetic entropy SCe per molCe in units of Rln2. The inset compares SCe with the spin-1/2 Kondo model of Desgranges and Schotte with TK = 12 K (red curve)30. (b) The temperature dependence of the electrical resistivity normalized to 2 K. The current was applied j ⊥ [001]. Inset: temperature derivative of ρ/ρ2K in the temperature region around the magnetic transitions. The error bar indicates scattering of the data points.
with values for A and Δ given in the text. The right ordinate displays the presumable magnetic entropy SCe per molCe in units of Rln2. The inset compares SCe with the spin-1/2 Kondo model of Desgranges and Schotte with TK = 12 K (red curve)30. (b) The temperature dependence of the electrical resistivity normalized to 2 K. The current was applied j ⊥ [001]. Inset: temperature derivative of ρ/ρ2K in the temperature region around the magnetic transitions. The error bar indicates scattering of the data points.
Finally, we estimated the magnetic entropy  by cutting off the superconducting transition and extrapolating the low-temperature tail of the TN–transition towards zero. For this we employed a fit expression accounting for the cooperative effect of spin-wave excitations25, with A = 0.0109 J/(mol · K2) per Ce and Δ = 2.74 K (solid line in Fig. 4a). It is fair to state that a second-order mean-field BCS-type of fit26 gives similar result due to the limited fitting range of 0.5–1.2 K. SCe(T) is plotted as closed symbols in Fig. 4a. The entropy deliberated at T1 is only 0.2Rln2 per molCe. This is well below the value (Rln2) associated with the lifting of the degeneracy of the ground state doublet suggesting magnetic ordering with substantially reduced magnetic moments.
by cutting off the superconducting transition and extrapolating the low-temperature tail of the TN–transition towards zero. For this we employed a fit expression accounting for the cooperative effect of spin-wave excitations25, with A = 0.0109 J/(mol · K2) per Ce and Δ = 2.74 K (solid line in Fig. 4a). It is fair to state that a second-order mean-field BCS-type of fit26 gives similar result due to the limited fitting range of 0.5–1.2 K. SCe(T) is plotted as closed symbols in Fig. 4a. The entropy deliberated at T1 is only 0.2Rln2 per molCe. This is well below the value (Rln2) associated with the lifting of the degeneracy of the ground state doublet suggesting magnetic ordering with substantially reduced magnetic moments.
B – T Magnetic Phase Diagram
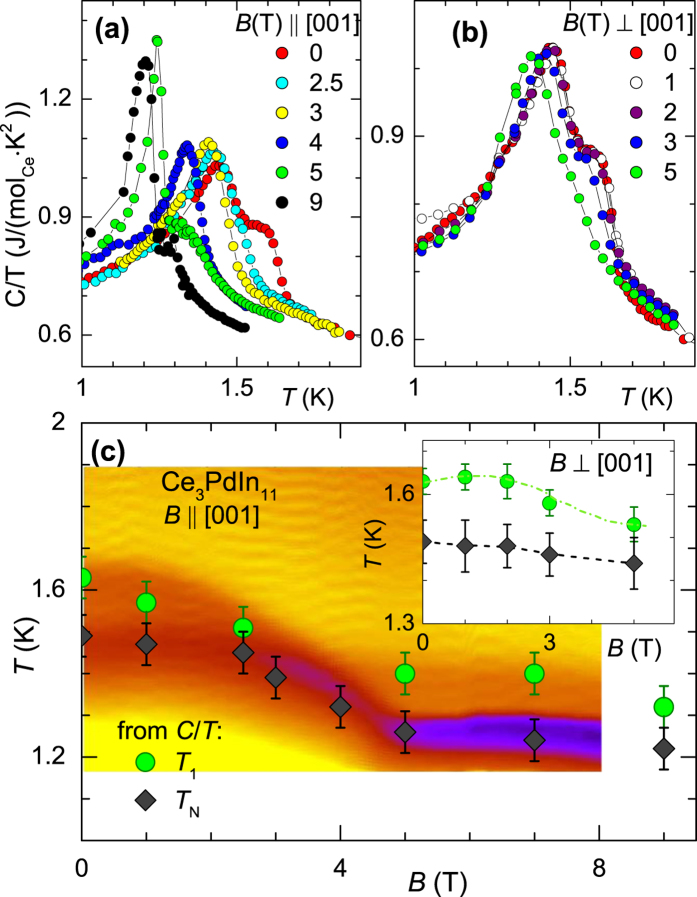
The influence of an applied magnetic field on the magnetic transitions was studied by means of specific heat experiments. The magnetic transitions are most pronounced in this measurement. The subtraction of the phonon and CEF terms has been omitted because we are only interested in the change of the transition temperatures. Experiments were conducted on a sample from a different batch than the one shown in Fig. 4. Figure 5 cumulates the results. We first focus on the behavior for B ‖ [001]. From Fig. 5a it can be seen that in small fields B < 2.5 T the transition T1 shifts to lower temperatures while TN remains almost unaffected. At B ≈ 3 T both transitions seem to merge. In higher fields B >4 T we observe again two transitions. Whether or not these two transitions indeed correspond to the low-field transitions as suggested by the figure is subject of further investigation. Our attention was devoted to the measurements in B = 5 T and above. In this field region the transition at TN shows a sharp λ-like shape, typical for a first-order type transition. Such increase in sharpening and peak size has also been found for the field-induced transition in CeRhIn5 11.
Figure 5.
Specific heat as function of temperature for various values of applied magnetic fields (a) B || [001] and (b) B ⊥ [001]. The temperature – magnetic field phase diagram (c) for B ‖ [001] mapped out by the thermal response technique (see text). The green circles and black diamonds show T1 and TN, respectively, as obtained from the data present in (a). Inset depicts the B − T phase diagram for B ⊥ [001] axis.
Figure 5b shows the specific heat for applied field perpendicular to [001]-axis. The T1-transition and the successive ordering at TN are clearly observed in low fields. T1 first shows a tiny increase, likely caused by a realignment of the moments, before gradually decreasing with increasing field. The lower transition temperature remains almost unchanged for B <5 T and moves monotonically to lower temperatures in larger fields. The constructed B − T phase diagrams are presented Fig. 5c. The phase diagram for B ‖ [001] has been measured in more detail by the method of analyzing the response of the compound to thermal heat pulses. By this method a heat pulse resulting in a ΔT = 1 K temperature change of the sample (mounted quasi-adiabatically) to the bath temperature Tbath was applied. The subsequent thermalization of the sample temperature to a temperature T was monitored over time t. The color plot shows the derivative d(ΔT)/dt as a function of the bath temperature and field (yellow: small change in dT/dt; blue/violet: large change in dT/dt). The thermal relaxation result is complemented by data points of T1 and TN obtained from specific heat experiments (T1: circles, TN: diamonds). The inset displays the respective phase diagram for B ⊥ [001].
From Fig. 5c it becomes more evident that the two transitions, T1 and TN, merge at ≈3 T. Moreover, in fields >4 T the TN anomaly sharpens as inferred by the rapid change in color code. Such a behavior indicative of a pronounced first-order type of transition and hence corroborates our observation already made in C/T. An intriguing aspect is that the sensitivity of the magnetic transitions with respect to the applied field is weakest for B ⊥ [001] and strongest for B ‖ [001]. For the two other magnetically ordered compounds of the CenTmIn3n+2m class of materials, CeRhIn5 and Ce2RhIn8, it is vice versa11.
The Superconducting State
The observation of ambient pressure bulk superconductivity below TN gives Ce3PdIn11 a unique place within the CenTmIn3n+2m family. So far pressure or doping was necessary to induce SC in a magnetically ordered compound. Corresponding examples are CeRhIn5, Ce2RhIn8, Ce(Rh1−xIrx)In5 or CeCoIn5 doped with Sn and Cd22,27,28. Figure 6 shows the superconducting phase diagram of Ce3PdIn11 for B ‖ [001] and j ‖ [100]. The jump in resistivity in various external fields is plotted in the inset of Fig. 6. The data are normalized to the value at 1.5 K (ρ(T)/ρ(1.5 K)). We defined the second step in the resistivity drop as Tc as discussed earlier. Within the accuracy of the measurement, the value of Tc fairly well corresponds with that obtained from the transition temperature in the specific heat. As expected, the application of magnetic fields reduces Tc, giving rise to a rather large change of the initial slope of the upper critical field dBc2/dT ≈ −8.6 T/K. Extrapolation of the field dependent transition temperatures to T → 0 using a simple mean-field type expression Bc2(T) = Bc2(0)[1 − (T/Tc)2] yields Bc2(0) ≈ 2.3 T.
Figure 6. Temperature dependence of the upper critical field of Bc2 as derived from resistivity experiments on Ce3PdIn11.
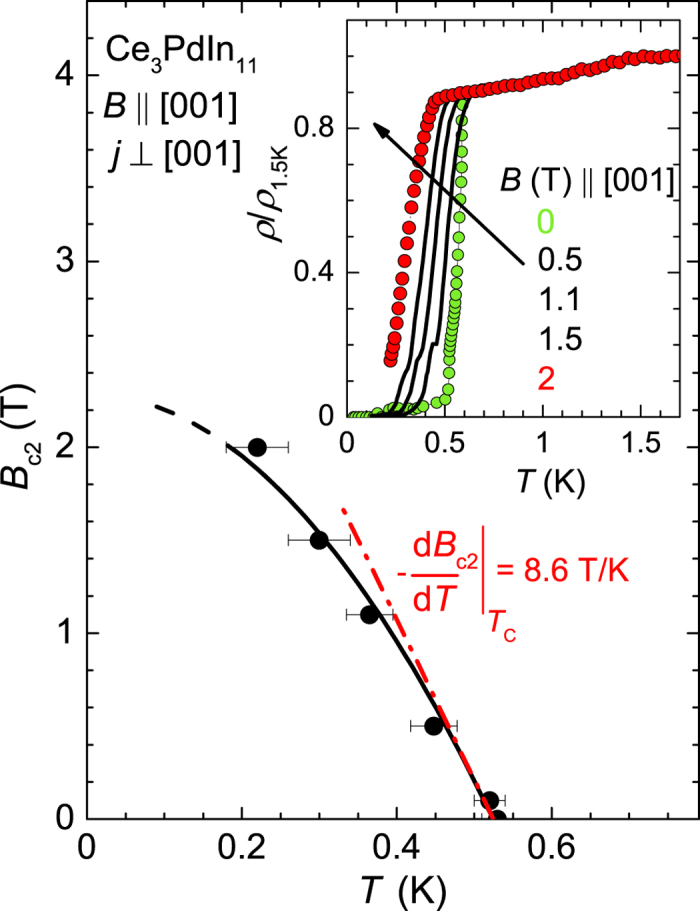
Typical curves are shown in the inset. Current was j ⊥ [001] and the field was applied parallel to the [001]-axis. The red solid line yields –dBc2/dT = 8.6 T/K.
Discussion
Our experimental results show that Ce3PdIn11 undergoes two successive magnetic transitions into antiferromagnetic-type ordered states at T1 = 1.67 K and TN = 1.53 K. At lower temperatures the compound becomes superconducting (Tc = 0.42 K). Beyond that the data reveal that the magnetic ground state exhibits a reduced magnetic moment and suggest a heavy fermion superconducting state. Hence, Ce3PdIn11 takes a unique place being the first known full inversion symmetrical stoichiometric Ce-based compound which shows coexistence of magnetic order and superconductivity at ambient pressure. As we mentioned in the introduction the existence of two inequivalent Ce-sites in Kondo lattice systems can lead to the observation of unusual ground states and complex phase diagrams (e. g., ref. 2, 3, 4). Such an expectation found some general justification in the framework of the Kondo lattice model describing the consequences of the coexistence of two inequivalent local moment Ce sublattices on the ground state properties, when linked to different Kondo couplings to the conduction electrons6. Since Ce3PdIn11 belongs to this class of Kondo systems, we will try in the following to provide an explanation of the novel phenomena in the context of two Ce sublattices.
The tetragonal structure of Ce3PdIn11 comprises two crystallographically inequivalent Ce-sublattices. The Ce-ions on the Ce1-sublattice (2 ions per f.u.) exhibit a Ce2PdIn8 environment and the Ce-ions on the Ce2-sublattice (1 ion per f.u.) possess a CeIn3 surrounding. Due to different local environments, each sublattice will have its individual CEF scheme and Kondo scale (TK1 and TK2). As a result complex interacting mechanisms are expected in Ce3PdIn11. In a first approach we can gain some insight by a quantitative analysis of C(T)/T and S(T). As shown in Fig. 4 the electronic specific heat coefficient γ of Ce3PdIn11 is found to be 0.55 ± 0.03 J/(molCe · K2) when extrapolated to zero temperature. This large γ places Ce3PdIn11 into the heavy fermion category. However, since there are two kinds of Ce-ions (Ce1 and Ce2) with different site symmetries and neighboring ions it is quite likely that the two do not contribute equally to the density of states at the Fermi level, i.e., γ = 0.55 J/(molCe · K2) is an average value. The same argument accounts for the magnetic entropy SCe which is depicted in the same figure. The average SCe per mol of cerium deliberated at T1 equals 0.2Rln2, that is one-fifth of the value expected for a ground state doublet. Thus there is a considerable reduction of the magnetic entropy. We relate the deficiency of SCe (T1) to the presence of the Kondo effect which partially lifts the twofold degeneracy above T1. In such a case it can be shown that SCe(T1) = SK(T1/TK), where SK(T1/TK) is the Kondo entropy at T1 29. The relation holds if the first exited level 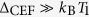 and
and 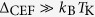 . From earlier discussion of the temperature dependence of the susceptibility we concluded that ΔCEF ≈ 60 K. Thus the former condition is satisfied in Ce3PdIn11. Typical Kondo scales in the CenTmIn3n+2m class of materials are in the range between 2 and 10 K suggesting that also the second condition is fulfilled10. Further, using the Bethe ansatz for spin-1/2 Kondo model, Desgranges and Schotte calculated the specific heat and the corresponding magnetic entropy30. By inserting the experimentally found SCe into the above given relation we obtain the ratio T1/TK. The estimated Kondo temperature for Ce3PdIn11 hence yields 12 K. This Kondo temperature is an average of TK1 and TK2. The value agrees well with the estimated Kondo temperature from the relation
. From earlier discussion of the temperature dependence of the susceptibility we concluded that ΔCEF ≈ 60 K. Thus the former condition is satisfied in Ce3PdIn11. Typical Kondo scales in the CenTmIn3n+2m class of materials are in the range between 2 and 10 K suggesting that also the second condition is fulfilled10. Further, using the Bethe ansatz for spin-1/2 Kondo model, Desgranges and Schotte calculated the specific heat and the corresponding magnetic entropy30. By inserting the experimentally found SCe into the above given relation we obtain the ratio T1/TK. The estimated Kondo temperature for Ce3PdIn11 hence yields 12 K. This Kondo temperature is an average of TK1 and TK2. The value agrees well with the estimated Kondo temperature from the relation 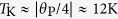 31. The inset in Fig. 4a compares SCe with the calculated values of the spin-1/2 Kondo model30. We observe that below T1 the experimental data fall under the theoretical curve as expected (entropy is absorbed in the transition). For
31. The inset in Fig. 4a compares SCe with the calculated values of the spin-1/2 Kondo model30. We observe that below T1 the experimental data fall under the theoretical curve as expected (entropy is absorbed in the transition). For 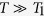 the SCe values exceed the calculated ones which is an indication for the presence of additional contributions to the specific heat, most likely originating from excited CEF levels which have not been subtracted from the C/T data.
the SCe values exceed the calculated ones which is an indication for the presence of additional contributions to the specific heat, most likely originating from excited CEF levels which have not been subtracted from the C/T data.
The small entropy release of ~0.2Rln2 at T1 implies a strong screening of the 4f-moment which gives rise to a small ordered moment in the magnetic state. This is consistent with the observation of a small signal in χ(T) at the magnetic transition temperatures. The nature of these two phase transitions at T1 and TN needs to be studied further in particular with regard to the two Ce-sites. We notice that with an increasing field parallel to [001] both T1 and TN decrease which is consistent with the fact that both phase transitions are of antiferromagnetic type. It is thus expected that the magnetic moments of both Ce–sublattices are mutually interacting (RKKY-type interaction). The long-range magnetic interaction on the other hand competes with the Kondo interaction. In Ce3PdIn11 TK will be in turn determined by the effective strength and type of interplay of the two sublattice Kondo temperatures TK1 and TK2 6. The complexity of the interactions may account for the intriguing magnetic phase diagram of Ce3PdIn11 being distinct different from the other two magnetically ordered compounds of the CenTmIn3n+2m family (CeRhIn5 and Ce2RhIn8) which have only one Ce-site per f.u.11.
Next we briefly discuss the superconducting state. A remarkable close analogy is found between the SC properties of Ce3PdIn11 and Ce2PdIn8. The SC transition temperature of our compound is Tc = 0.42 K while for Ce2PdIn8 values between 0.45 and 0.7 K have been reported13,14,15,16,32. In addition, the upper critical field yields Bc2 = 2.3 T for Ce3PdIn11 and 2.5 T for Ce2PdIn8 which is very similar32. Difference is solely observed in the initial slope of Bc2 being dBc2/dT ≈ −8.6 T/K and dBc2/dT ≈ −13.5 T/K, respectively.
Before we discuss such a similarity to Ce2PdIn8 and to avoid confusion we want to exclude the possibility of having Ce2PdIn8 impurity phase in our samples. As we recently reported, our single crystal Ce3PdIn11 samples have been carefully analyzed by X-ray diffraction and Scanning Electron Microscopy with an Energy Dispersive X-ray detector (see Methods section) and clearly excludes the presence of any Ce2PdIn8 inclusions.
The large observed SC discontinuity of ΔC/Tc ≈ 0.89 J/(mol · K2) in Ce3PdIn11 corresponds to a SC jump ΔC/(γTc) = 0.73 in Ce3PdIn11 and amounts to 51% of the BCS-value. Such a high ratio suggests that a large portion of the sample is in the superconducting state. One possibility to understand the observed similarities of the superconducting properties could be the fact that Ce3PdIn11 has a layer structure and the Ce1-site in Ce3PdIn11 exhibits a Ce2PdIn8 environment (see above). However this needs further microscopic proof.
In summary, at ambient pressure Ce3PdIn11 undergoes magnetic transitions into an AFM state at T1 = 1.67 K and an order-to-order transition into a second AFM state at TN = 1.53 K. The entropy analysis shows an magnetic entropy release at T1 of only 0.2Rln2 indicating that the ordered moment is small. The strong reduction of the magnetic moment is due to Kondo effect. Below Tc = 0.42 K the compound becomes superconducting. The size of the jump in specific heat and the large value of initial slope dBc2/dT ≈ −8.6 T/K indicate that the Cooper pairs are formed of heavy quasiparticles. In this sense Ce3PdIn11 is unique being the first known full inversion symmetrical stoichiometric heavy fermion material based on Ce exhibiting coexistence of both attributes, magnetism and superconductivity at ambient pressure. In fact, the crystal structure of Ce3PdIn11 possesses two inequivalent Ce-sites. The Ce1-site exhibits Ce2PdIn8 surrounding and the Ce2-site has CeIn3-like environment. Each Ce-sublattice is expected to have a different Kondo temperature and different RKKY interaction within the sublattice. The interplay between these two sublattices in Ce3PdIn11 is likely strong and gives rise the complex ground state. To unravel and to gain deeper insight in the particular mechanisms at work, microscopic methods are indispensable. For instance 105Pd and 115In NQR and NMR can provide information about the magnetic moment on the Ce-ion but also about the superconducting gap structure which is an important quantity to reveal the mechanism behind Cooper-pairing. On the other hand neutron experiments can resolve the magnetic structure. Finally we feel that our experimental results would stimulate further experimental and theoretical efforts to explore the nature of electronic correlation and their theoretical understanding in this class of compounds.
Methods
Single crystal growth
Plate-like single crystals with mass up to ≈1 mg were grown by indium self-flux method8. High-purity starting materials (Ce 99.9%, Pd 99.995% and In 99.999%) in the ratio 3:25:50 were placed in high purity alumina crucibles and sealed under vacuum in quartz glass tubes together with another crucible filled with quartz wool plug for later flux removal. The crucibles were heated up to a temperature of 750 °C for several hours (8–10 h) and slowly (3 °C/h) cooled down to 550 °C. At this temperature, the material was decanted to separate the single crystals from the remaining In-flux.
The quality of the single crystals was checked by X-ray Laue diffraction in reflection geometry. The chemical composition was verified employing a Tescan Mira I LMH scanning electron microscope (SEM). The instrument is equipped with a Bruker AXS energy dispersive X-ray detector (EDX). Elemental mapping has confirmed composition homogeneity8. Several pieces were pulverized and examined at room temperature by means of powder X-ray diffraction (Bruker D8 Advance diffractometer with Cu-Kα radiation). The obtained diffraction patterns were refined by Rietveld analysis using FullProf Suite. In addition, in order to refine the structural parameters more precisely and to confirm phase homogeneity, several samples were investigated by single crystal X-ray diffraction using a X-ray diffractometer Gemini with Mo-K radiation. The crystal structure was resolved by charge flipping program Superflip and adjacent refinement was done by full-matrix least-squares based on F2 using Jana2006. Experiments were performed on samples from different batches.
Experimental Details
The physical properties of the Ce3PdIn11 samples were attained employing standard equipment. The magnetic susceptibility was determined using a MPMS 7 T SQUID magnetometer (Quantum Design) with an applied field of 1 T. Additionally, in the temperature range between 0.5 and 4 K magnetization measurements were performed using a Hall-probe which is sensitive to the dipole field created by the sample magnetization. In order to obtain absolute values the Hall probe data were scaled in the overlapping region (2–10 K) with the data measured by the MPMS. The method is still in testing stage. Resistivity was measured utilizing standard four point a. c. technique. Low-temperature measurements up to T ≈ 2 K were performed in a Leiden Cryogenics MCK72 dilution refrigerator. The setup is equipped with a 9 T magnet. Measurements above 0.4 K up to room temperature were done in a Physical Property Measurement System (PPMS) from Quantum Design. The low-T range of the PPMS guarantees sufficient overlap of low- and high-temperatures data sets. In similar manner specific heat experiments were conducted in PPMS down to 0.45 K and were continued to lower temperatures using a Quantum Design dilution refrigerator heat capacity puck mounted to the MCK72 dilution refrigerator.
Additional Information
How to cite this article: Kratochvílová, M. et al. Coexistence of Antiferromagnetism and Superconductivity in Heavy Fermion Cerium Compound Ce3PdIn11. Sci. Rep. 5, 15904; doi: 10.1038/srep15904 (2015).
Acknowledgments
The authors would like to thank Mohsen M. Abd-Elmeguid for critical reading of the manuscript. J.C. thanks Julian Sereni and Christoph Geibel for valuable discussions. This work was supported by the Grant Agency of the Czech Science Foundation (Project P203/12/1201). Experiments were performed in MLTL (http://mltl.eu/) which is supported within the program of Czech Research Infrastructures (project no. LM2011025). The crystallography was supported by project P204/11/0809 of the Czech Science Foundation.
Footnotes
Author Contributions M.K. and K.U. prepared the samples. M.K., M.D. and K.U. characterized the single crystals. M.K., V.T. and J.P. performed the specific heat, resistivity, thermal heat-pulse and magnetization experiments. V.S. and J.C. wrote the manuscript in consultation with all authors.
References
- Doniach S. The kondo lattice and weak antiferromagnetism. Physica B + C 91, 231–234 (1977). [Google Scholar]
- Sereni J. et al. Low temperature magnetic behavior of Ce7Ni3. Physica B 199 & 200, 567–569 (1994). [Google Scholar]
- Tang J. et al. Ce5Ni6In11: An intermediate heavy-fermion system. Phys. Rev. B 52, 7328–7333 (1995). [DOI] [PubMed] [Google Scholar]
- Custers J. et al. Destruction of the kondo effect in the cubic heavy-fermion compound Ce3Pd20Si6. Nature. Mater. 11, 189–194 (2012). [DOI] [PubMed] [Google Scholar]
- Strydom A., Pikul A., Steglich F. & Paschen S. Possible field-induced quantum criticality in Ce3Pd20Si6. J. Phys.: Conf. Ser. 51, 239–242 (2006). [Google Scholar]
- Benlagra A., Fritz L. & Vojta M. Kondo lattices with inequivalent local moments: Competitive versus cooperative kondo screening. Phys. Rev. B 84, 075126 (2011). [Google Scholar]
- Tursina A., Neterenko S., Seropegin Y., Noël H. & Kaczorowski D. Ce2PdIn8, Ce3PdIn11 and Ce5Pd2In19?members of homological series based on AuCu3- and PtHg2-type structural units. J. Solid State Chem. 200, 7–12 (2013). [Google Scholar]
- Kratochvílová M. et al. Single crystal study of the layered heavy fermion compounds Ce2PdIn8, Ce3PdIn11, Ce2PtIn8 and Ce3PtIn11. J. Cryst. Growth 387, 47–52 (2014). [Google Scholar]
- Knebel G., Aoki D. & Flouquet J. Antiferromagnetism and superconductivity in cerium based heavy-fermion compounds. C. R. Physique 12, 542–566 (2011). [Google Scholar]
- Thompson J. & Fisk Z. Progress in heavy-fermion superconductivity: Ce115 and related materials. J. Phys. Soc. Jpn. 81, 011002 (2012). [Google Scholar]
- Cornelius A. L., Pagliuso P. G., Hundley M. F. & Sarrao J. L. Field-induced magnetic transitions in the quasi-two-dimensional heavy-fermion antiferromagnets CenRhIn3n+2 (n=1 or 2). Phys. Rev. B 64, 144411 (2001). [Google Scholar]
- Walker I., Grosche F., Freye D. & Lonzarich G. The normal and superconducting states of CeIn3 near the border of antiferromagnetic order. Physica C 282–287, 303–306 (1997). [Google Scholar]
- Kaczorowski D., Pikul A., Belan B., Sojka L. & Kalychak Y. Non-fermi liquid behavior in polycrystalline Ce2PdIn8. Physica B 404, 2975–2977 (2009). [Google Scholar]
- Kaczorowski D., Pikul A. P., Gnida D. & Tran V. H. Emergence of a superconducting state from an antiferromagnetic phase in single crystals of the heavy fermion compound Ce2PdIn8. Phys. Rev. Lett. 103, 027003 (2009). [DOI] [PubMed] [Google Scholar]
- Uhlířová K., Prokleška J. & Sechovský V. Comment on “emergence of a superconducting state from an antiferromagnetic phase in single crystals of the heavy fermion compound Ce2PdIn8”. Phys. Rev. Lett. 104, 059701 (2010). [DOI] [PubMed] [Google Scholar]
- Uhlířová K., Prokleška J., Sechovský V. & Daniš S. Solution growth of Ce–Pd–In single crystals: Characterization of the heavy-fermion superconductor Ce2PdIn8. Intermetallics 18, 2025–2029 (2010). [Google Scholar]
- Gschneidner K. Jr. & Pecharsky V. Cerium intermetallic compounds with two inequivalent crystallographic sites. Physica B 223–224, 131–134 (1996). [Google Scholar]
- Rajan V. T. Magnetic susceptibility and specific heat of the coqblin-schrieffer model. Phys. Rev. Lett. 51, 308–311 (1983). [Google Scholar]
- Knafo W. et al. Study of low-energy magnetic excitations in single-crystalline CeIn3 by inelastic neutron scattering. J. Phys.: Condens. Matter 15, 3741–3749 (2003). [Google Scholar]
- Fisher M. Relation between the specific heat and susceptibility of an antiferromagnet. Phil. Mag. 7, 1731–1743 (1962). [Google Scholar]
- Fisher R. A. et al. Specific heat of UPt3: Evidence for unconventional superconductivity. Phys. Rev. Lett. 62, 1411–1414 (1989). [DOI] [PubMed] [Google Scholar]
- Pham L. D., Park T., Maquilon S., Thompson J. D. & Fisk Z. Reversible tuning of the heavy-fermion ground state in CeCoIn5. Phys. Rev. Lett. 97, 056404 (2006). [DOI] [PubMed] [Google Scholar]
- Bauer E. et al. Heavy fermion superconductivity and magnetic order in noncentrosymmetric CePt3Si. Phys. Rev. Lett. 92, 027003 (2004). [DOI] [PubMed] [Google Scholar]
- Stewart G. R., Fisk Z., Willis J. O. & Smith J. L. Possibility of coexistence of bulk superconductivity and spin fluctuations in UPt3. Phys. Rev. Lett. 52, 679–682 (1984). [Google Scholar]
- Continentino M. A., Medeiros S. N. d., Orlando M. T. D., Fontes M. B. & Baggio-Saitovitch E. M. Anisotropic quantum critical behavior in CeCoGe3−xSix. Phys. Rev. B 64, 012404 (2001). [Google Scholar]
- Maple M. B. et al. Partially gapped fermi surface in the heavy-electron superconductor URu2Si2. Phys. Rev. Lett. 56, 185–188 (1986). [DOI] [PubMed] [Google Scholar]
- Pagliuso P. G. et al. Coexistence of magnetism and superconductivity in CeRh1−xIrxIn5. Phys. Rev. B 64, 100503 (2001). [Google Scholar]
- Nicklas M. et al. Magnetism and superconductivity in Ce2RhIn8. Phys. Rev. B 67, 020506 (2003). [Google Scholar]
- Mori H., Yashima H. & Sato N. A new dense kondo system: CeyLal−yGe2. Resistivity, specific heat, and susceptibility studies. J. Low Temp. Phys. 58, 513–531 (1985). [Google Scholar]
- Desgranges H. & Schotte K. Specific heat of the kondo model. Phys. Lett. 91A, 240–242 (1982). [Google Scholar]
- Hewson A. The Kondo Problem to Heavy Fermions (Cambridge University Press, Cambridge, 1993). [Google Scholar]
- Kaczorowski D., Gnida D., Pikul A. & Tran V. Heavy-fermion superconductivity in Ce2PdIn8. Solid. State Commun. 150, 411–414 (2010). [Google Scholar]