Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2015 Nov 20;5:17080. doi: 10.1038/srep17080

Electrochemical gating-induced reversible and drastic resistance switching in VO2 nanowires

Tsubasa Sasaki 1, Hiroki Ueda 1, Teruo Kanki 1,a, Hidekazu Tanaka 1,b
PMCID: PMC4653652  PMID: 26584679

Abstract

Reversible and drastic modulation of the transport properties in vanadium dioxide (VO2) nanowires by electric field-induced hydrogenation at room temperature was demonstrated using the nanogaps separated by humid air in field-effect transistors with planer-type gates (PG-FET). These PG-FETs allowed us to investigate behavior of revealed hydrogen intercalation and diffusion aspects with time and spatial evolutions in nanowires. These results show that air nanogaps can operate as an electrochemical reaction field, even in a gaseous atmosphere, and offer new directions to explore emerging functions for electronic and energy devices in oxides.

The transport characteristics of transition metal oxides are sensitive to redox reactions because the valence numbers of the transition metal ions are easily changed by the reactions, which affect the carrier density and/or stabilization of the crystal structure1,2,3,4. Of the prototypical materials, VO2 is promising as it undergoes a metal-insulator transition (MIT) and the resistance changes by orders of magnitude around 340 K. In VO2 nano to microstructures5,6,7,8,9,10,11,12,13, the coupling of the MIT with mechanical7,8,9, optical13, thermal12 and electronic properties9,11 can be used in tunable resonators, optical switchers, electronic and thermo-sensing devices. Furthermore, only a few atomic percent of the hydrogen- or oxygen-intercalation and -desorption in VO2 cause drastic changes in the transport properties, equal to that caused by inducing the MIT14,15,16,17,18,19,20. Conventionally, controlling the amount of hydrogen/oxygen ions in an oxide has been conducted by annealing the samples under redox gas atmospheres1,4,15,16, in an aqueous solution17,18 and with a hydrogen spillover method14,19. Every method requires high temperatures of at least 150 °C. Recently, on the other hand, an electric field has been shown to be a means of both hydrogenation and oxidization in oxides at room temperature20,21,22,23. For example, a strong electric field in ionic liquid (IL) gates induces oxygen vacancy formation in VO222. In IL or CAN (amorphous 12CaO·7Al2O3 with a nanoporous structure) gates including water21,24, furthermore, hydrogen ions can be intercalated by positive electric field. Thus electrochemical gating including water electrolysis has a full potential to allow tuning of doping level at room temperature. In this paper, we report investigation of systematic transport modulation in VO2 nanowires by electrochemical gating via air gap under humid condition and establishment of the ion diffusion model, giving the hydrogen ion intercalation and diffusion aspects with time and spatial evolutions in VO2.

Results

Proposed device structure

As a proper device structure to perform this experiment, we have prepared planer-type field effect transistor with side gates and a nanoscaled wire channel separated by air nanogaps (PG-FET) illustrated in Fig. 1a. This type of device has following advantages: An electric field can be applied under various different gas and vapor atmospheres through the air nanogap. In this study, air with various humidity levels and dry air were used. Secondly, the electric-field induced ion intercalation and diffusion aspects with the time and spatial evolutions in the vicinity of interface can be systematically investigated because ions are intercalated from channel edges of both side and diffuse from the sides in VO2. Especially a narrower channel often enhances interface effect. An atomic force microscope image of a device is shown in Fig. 1a and the height profile is in Fig. 1b. The thicknesses of the channels and the gap distance were roughly 35 nm and 400 nm, respectively. The channel widths (w) were systematically changed from 3 μm to 400 nm (see Methods and Supplementary Information section A for details). Figure 1c shows a cross-sectional electric field-map, obtained using a Finite Element simulation using typical experimental parameters (see Supplementary Information section B). The electric field converged at the edges of the channels, providing the strongest effect on the electrochemical reactions. Thus the field-induced ion intercalation begins at the lateral sides of the nanowires

Figure 1. VO2 nanowire device with planer-type gates.

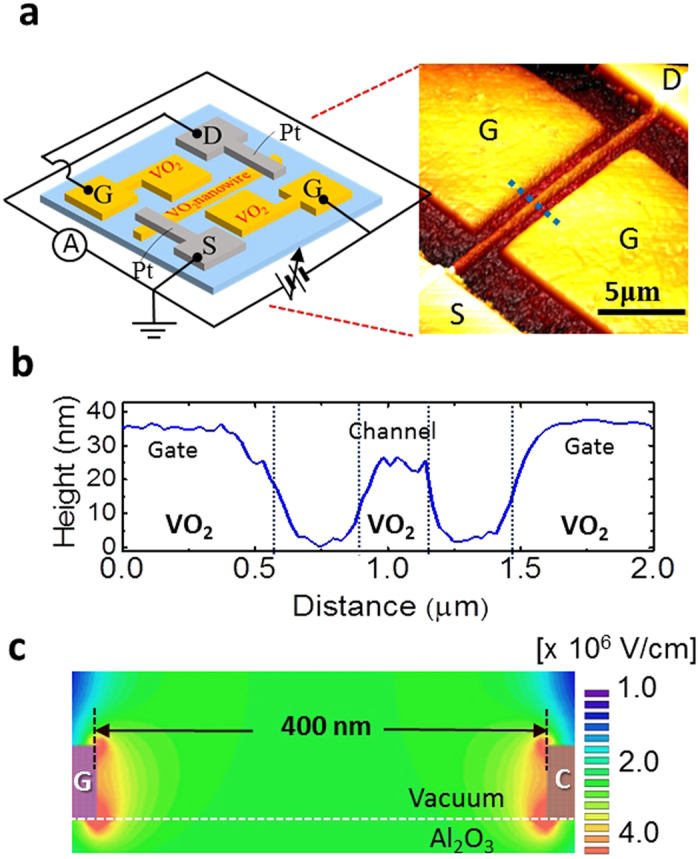
Figure 1

Open in a new tab

(a) The typical device architecture and an atomic force microscope (AFM) image of the VO2 channel area. S, D and G indicate the source, drain and gate electrodes, respectively. (b) Cross-sectional AFM image, taken at the blue dashed line in (a). (c) Cross-sectional electric field map, determined using finite element analysis at VG = 100 V through the 400-nm vacuum gap between G and the channel (c) on an Al2O3 substrate under vacuum.

Drastic resistance modulation by electrochemical induced-hydrogenation

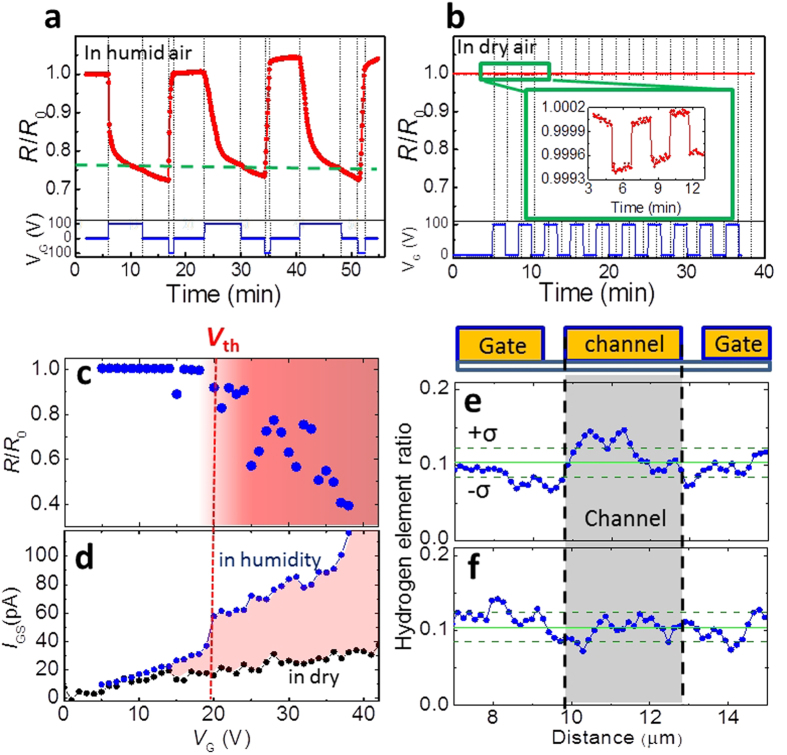
Figure 2a shows the reversible and non-volatile resistance modulation in a VO2 nanowire channel (w = 500 nm), found by applying a positive and negative VG at 300 K under a humidity of around 50%. The normalized resistance (R/R0), where R and R0 are the resistance and the pristine resistance before applying a VG at 300 K, respectively, slowly continued to drop during the application of VG = + 100 V down to the saturation line at roughly R/R0 = 0.75. This state was held after removal of the VG. Namely, it exhibited a non-volatile memory effect. In contrast, the resistance increased again at VG = −100 V. Regarding slow resistive drops by an electric field, it is suggested that the origin would be related to mechanical relaxation or slow traps according to some reports25,26,27. In our PG-FET devices, on the other hand, such slow changes in the resistance were not observed under dry air condition (Fig. 2b). A steep resistance-switching of 0.06% occurred without any non-volatile memory effects, as shown in the inset of Fig. 2b. Approximately 1012 cm−2 electrons should be accumulated at VG = 100 V and 400 nm-gap distance. From Hall measurements in VO2 thin films on Al2O3 substrates28, the carrier density was evaluated at ~4 × 1018 cm−3, equivalent to the Debye length of ~2 nm. Given modulation of resistivity in both lateral sides in our device geometry, the calculated modulation rate would be ideally 0.4%. Thus, it is considered that a pure carrier accumulation acts at lateral sides in dry air condition though the efficiency on the electrostatic effects was not so high compared with the ideal case. From the presence of the steep resistive switching in Fig. 2b, the origin of slow resistive drops under humid air conditions is not likely to attribute to the mechanical relaxation or slow traps as mentioned above, but rather to electrochemical reaction with intercalation of hydrogen ions21,24,27,29, which can significantly reduce resistivity in 3d-orbital sensitive systems30 (see Supplementary Information C for the intercalation (VG = 100 V) and non-intercalation (VG = 0 V) cases in detail) and/or color-switching properties known as electrochromism31. In VO2 intercalated hydrogen ions, a strong H-O bond induces electron transfer from hydrogen onto the oxygen atom, resulting in higher 3d-orbital occupancy of vanadium from V4+(3d1) to V3+(3d2)5. Figure 2c shows VG dependence of R/R0 20 minutes after applying the VG to investigate the magnitude of resistance changes with variety of VG. The threshold voltage starting to the reduction (Vth) was approximately 20 V and the magnitude of the resistance changes was enhanced with increasing VG. The resistance fluctuation in over 20 V would be due to fluctuation for level of humidity. The resistance modulation is sensitive to the level of humidity. In addition, repetitive intercalation and desorption of hydrogen ions may slightly change crystallinity of VO2 channels, causing the resistive fluctuation. In response with the resistance changes, the current between the gate and source electrodes (IGS) suddenly increased at around 20 V as seen in Fig. 2d, corresponding to the Vth in Fig. 2c. Subtracting the current recorded under humid conditions from that recorded under dry conditions gives the current generated by the electrolysis of water. Accordingly, the density of generated hydrogen ions increases with increasing VG and the number of intercalated hydrogen ions in VO2 increases. Additionally, Fig. 2e shows the ratio of hydrogen elements in a device after applying VG = 100 V, investigated by ToF-SIMS (time-of-flight secondary ion mass spectrometer). It can be indicated that hydrogen content in VO2 channel is higher than that in other area in a device after applying the VG, though the hydrogen content is roughly averaged because spatial resolution in the measurement is several hundred nanometer at most, whereas the hydrogen contents remain unchanged in a pristine device in Fig. 2f (see Supplementary Information section D in detail).

Figure 2. Effect of the electric field on the transport properties and hydrogen intercalations in a VO2 channel.

Figure 2

Open in a new tab

(a,b) Time dependence of the normalized resistance (R/R0, where R0 is the pristine resistance of a non-treated VO2 channel at 300 K with applied VG values of 100, 0 and −100 V) (a) in humid air and (b) in dry air. The green dashed line in (a) indicates the rough saturation of R/R0. The inset in (b) is a magnified view. (c) VG dependence of R/R0 after applying a VG for 20 minutes. (d) VG dependence of the current between the gate and source electrodes (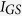Inline graphic) under a humidity of 60% (blue dotted-line) and in dry air (black line). (e,f) The relative elemental ratios for hydrogen normalized by oxygen (e) in a device after applying VG = 100 V and (f) in a pristine device. The solid and dashed green lines represent the averages of the hydrogen atom profiles and the standard deviations, respectively.

Establishment in ion diffusion model by electrochemical gating

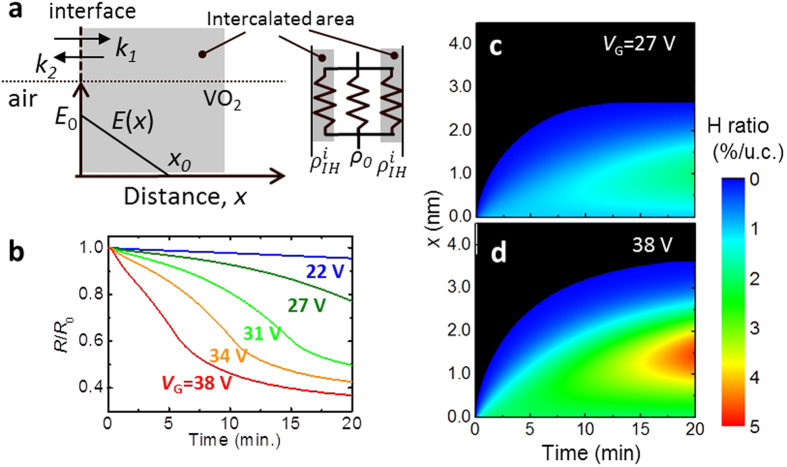
It is known that a 1% hydrogen intercalation per VO2 unit cell induces almost one order of magnitude reduction in resistivity17,18. Based on the empirical facts and the intercalation from the channel edge by applying electric gate from lateral sides, the amount of intercalated hydrogen ions and the effect on the diffusive aspect in VO2 can be evaluated by investigating the resistive behavior with a variety of VG values. Figure 3 shows the time dependence of the resistive modulations with various VG under a humidity of 60%. The resistance began to decrease above VG = 22 V, approximately corresponding to the Vth. In the characteristic features, the magnitude of resistance reduction rate increases with increasing VG and the initial reduction speed is faster in the larger VG, while the reduction speed became slower with time.

Figure 3. Transport properties from redox reactions in VO2 by the electrolysis of water with various VG values.

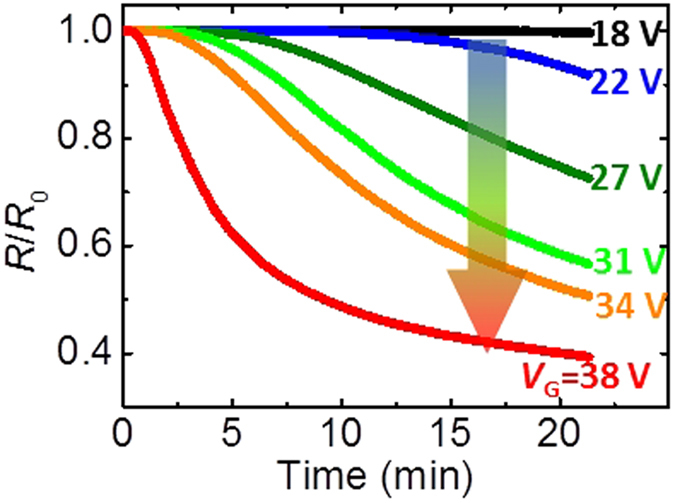
Figure 3

Open in a new tab

Time dependence of R/R0 for different VG values under a humidity of 60% at 300 K.

To understand such transport behaviors in a variety of VG, theoretical investigations have been carried out with a combination of chemical reaction kinetics at the interface and ion diffusion model under an electric field32. The external hydrogen ions stochastically react with VO2 at the interface. The reaction rate depends on the external hydrogen ion concentration (nH+) generated by electrolysis of the absorbed water. The nH+ tends to increase with increasing current between the gate and channel electrodes (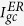Inline graphic), promoting formation of HVO2. While at the same time, desorption of hydrogen, namely return to VO2, would occur because of the natural recovery of resistivity by the thermal energy as seen in Supplementary Information section E. Thus assuming the reversible reaction, the time evolution-dependence of the concentration of intercalated ions inside VO2 at the interface (ninter) can be written as a differential equation with respect to time (t):

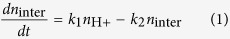graphic file with name srep17080-m2.jpg

where k1 and k2 are the forward and reverse reaction rate constants, depending on the activation energy at the interface and temperature. Next, we consider how the intercalated ions diffuse in VO2. Theoretically, for ion diffusion, the ionic fluxes likely arise from the gradients of the ion concentration and the electric gradients in solid-state materials32. 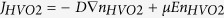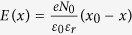Thus, as nHVO2 is the hydrogen ion concentration in VO2, the hydrogen ion flux (JHVO2) can be described as: Inline graphic, where D is the diffusivity, μ is the mobility and E is the internal electric field in VO2. The first and second terms represent ion diffusion by the ion concentration gradient and by an electric field, respectively. 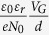The E resulting from VG is screened by mobile electrons in VO2 according to Poisson’s equation, given as a function of the distance (x) from the interface (x = 0), namely, Inline graphic, where e is the elementary charge, N0 is the carrier density in VO2, and εr and ε0 are the relative permittivity of VO2 and the permittivity of a vacuum, respectively. 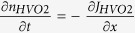x0 can be expressed as: Inline graphic as a function of VG, where d is the distance between the gate and channel. N0 and εr change with depending on the magnitude of intercalated hydrogen ions, however, N0/εr can be treated as a constant value because the change rate of εr is roughly proportional to that of N033. Thus, the length of x0 would be determined only by the magnitude of VG, and E linearly decreases as a function of x and become zero at x0, as shown in Fig. 4a. To conduct the unsteady state analysis, we use Fick’s second law in the one dimensional case, namely, Inline graphic, which predicts the time and spatial evolutions of the ion concentration. With this the following equation was obtained:

Figure 4. Simulated transport properties determined by the diffusion of intercalated ions.

Figure 4

Open in a new tab

(a) Schematic of the ion intercalation at the interface between air and VO2, resulting from chemical kinetics and the diffusion area (gray region), found using Fick’s diffusion model under an electric field (E(x)) derived by Poisson’s equation. The simulated channel resistance was calculated using the parallel resistor model for the intercalated (ρIH) and non-intercalated (ρ0) resistivities and by taking each width into consideration, as shown on the right side in (a). (b) Time dependence of the simulated R/R0 with a variety of VG values for 500 nm wide VO2 channels. (c,d) Spatial- and time-evolution maps of the hydrogen ion concentration at (d) VG = 27 V and (e) 38 V. x = 0 indicates the interface.

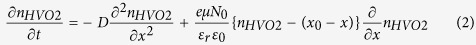graphic file with name srep17080-m7.jpg

Furthermore, using a parallel resister model for the resistivity in the intercalated (ρIH) and non-intercalated (ρ0) parts in Fig. 4a and assuming a 1% hydrogen intercalation per VO2 unit cell induces one order of magnitude reduction in the resistivity17,18, R/R0 could be evaluated as the following equation:

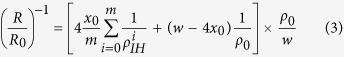graphic file with name srep17080-m8.jpg

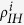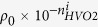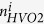where Inline graphic is the part of the spatially divided resistivity in the diffuse area taking 2 × 0 in consideration of ion diffusion by the ion concentration gradient, which is divided into i, and is given as: Inline graphic, where Inline graphic represents the hydrogen concentration in segment i (i: integral), derived using the finite difference method in equation (2) and the boundary conditions in equation (1). A more detailed derivation is outlined in the Supplementary Information section F.

The experimental results in Fig. 3 are significantly reproduced in Fig. 4b as k1, k2 and μ are fitting constants, though the more perfect reproduction requires more consideration efforts in the setting parameters, for example, considering k1 and k2 parameters depending on VG, which make enhanced the reduction rate of resistivity with increasing VG, and more precise resistive simulation like a random resistor network. Furthermore, this simulation enough includes the important essence of ion diffusion behavior, time and spatial evolutions of the ion diffuse in VO2 are shown in Fig. 4c,d, which are in the cases at VG = 27 and 38 V, respectively, where x = 0 indicates a channel edge. Hydrogen ions expand with time and the concentration increase with increasing VG. Within the frame work of this model, interestingly, hydrogen ions accumulate in an inner area, clearly observed at VG = 38 V in Fig. 4d. This is caused by the continuous non-equilibrium states of the ion intercalation and diffusion by an electric field. In more detail, this accumulation is induced by the slower ion-diffusion rate in the inner part, depending on deduction of internal electric field with increasing x. As a token of this scenario, following the removal of any VG, this accumulation fades away in time due to the ion-diffusion by concentration gradient and finally the concentration become homogeneous and equilibrium states. This diffusion behavior at VG = 0 is significantly reproducible for the persisting resistance decrease even after removal of the VG in Fig. 2a, shown by the simulation in Supplementary Information section G. Thus this device suggests to be a kind of proton pumps in solid-state system.

Drastic resistance modulation in narrower nano-channel

Moreover, this model predict that the magnitude of resistance reduction rate enhance with decreasing the channel width (w) because the effect on the interface diffusion due to the electrochemical gating from lateral sides become more prominent in narrower w. Figure 5 shows the time dependence of R/R0 with a variety of wire widths (w = 400, 1500 and 3000 nm) at VG = 100 V and 300 K. The saturation values for R/R0 were enhanced with decreasing w and the sharpness of the resistance deduction in the initial process differs among the three devices as seen in the left inset of Fig. 5, magnified view from 0 to 2 minutes of Fig. 5. This behavior is in agreement with the simulation in the right inset of Fig. 5. Thus the further narrower channel will provide perfect electrochemical gating causing metal-insulator transition in whole channel area.

Figure 5. Enhancement of R/R0 with decreasing w.

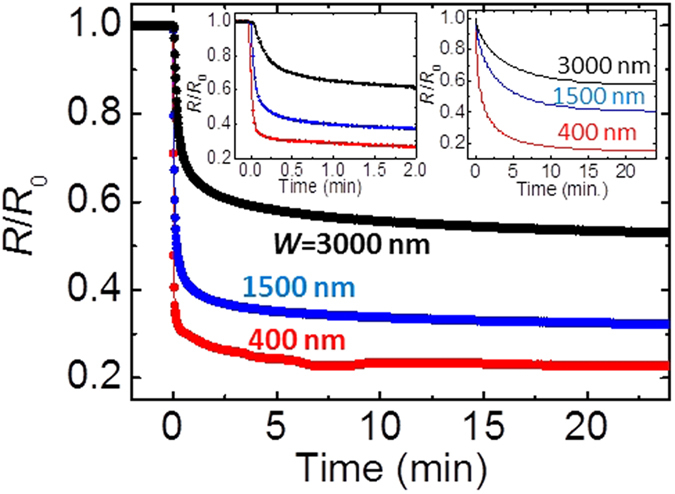
Figure 5

Open in a new tab

Time dependence of the simulated R/R0 values for 400 nm, 1500 nm and 3000 nm wide VO2 channels at VG = 100 V. The left and right insets show the magnified view of Fig. 5 and the simulation results, respectively.

Discussion

These results show that an air nanogap significantly works as an electrochemical reaction field, even in a gaseous atmosphere, and it is expected that the intercalated elements have an impact on reversibly changing in the physical properties of VO2. This interfacial effect was more enhanced in smaller nanoscaled channels. This offers a new way to both investigate the fundamental physical properties on the effect of intercalation and non-equilibrium ion diffusion for a wide range of materials and may lead to the realization of new gas-sensing, storage applications and also ion pumps in solid-state materials.

Methods

Thin film growth

35-nm-thick VO2 films were prepared on Al2O3(0001) single crystal substrates by pulsed laser deposition using an ArF excimer laser at 450 °C under an oxygen pressure of 1.0 Pa. Using X-ray diffraction measurements, it was confirmed that the films were b-axis-oriented without any impurity phases.

Device fabrication

The films were patterned into nanowire channels with planer-type field gates by nanoimprint lithography and reactive ion etching using O2 and SF6 gases. As advantages of this method, we can easily obtain 200 nm to 400 nm-air gaps between VO2 gate electrodes and VO2 channels, and fabricate many PG-FETs at one process. Also, since the resistivity of VO2 is roughly 1 ohm cm even in insulating region at room temperature, VO2 gate should enough work as gate electrodes in electrostatic effect. Pt/Cr electrodes were deposited by radio-frequency sputtering. Ohmic contacts between the VO2 films and electrodes were confirmed.

Electrical measurements

The transport characteristics were measured using a two terminal method with a Keithley 2635A. VG was applied using a Keithley 236 and the currents between the gate and source electrodes were monitored simultaneously. The temperature of the device was controlled by a Peltier-based temperature stage (T95, Linkam). The gaseous conditions, from dry air to 80% humidity were controlled in a glovebox. The humidity fluctuation was within ±2%.

Additional Information

How to cite this article: Sasaki, T. et al. Electrochemical gating-induced reversible and drastic resistance switching in VO2 nanowires. Sci. Rep. 5, 17080; doi: 10.1038/srep17080 (2015).

Supplementary Material

Supplementary Information
srep17080-s1.pdf (864KB, pdf)

Acknowledgments

This work was supported by a Grant-in-Aid for Scientific Research A (No. 26246013), a Grant-in-Aid for Scientific Research B (No. 25286058) from the Japan Society for the Promotion of Science (JSPS) and the Nanotechnology Platform Project (Nanotechnology Open Facilities in Osaka University) from the Ministry of Education, Culture, Sports, Science and Technology, Japan (MEXT) (F-14-OS-0010, S-14-OS-0007).

Footnotes

The authors declare no competing financial interests.

Author Contributions T.S. fabricated the devices, conducted the measurements and analyzed the data. H.U. contributed to the nanofabrication processes used to prepare the devices and the experimental setup. T.K. and H.T. planned and supervised the research. T.S., T.K. and H.T wrote the manuscript. All authors discussed the results.

References

  1. Malavasi L., Mozzati M. C., Azzoni C. B., Chiodelli G. & Flor G. Role of oxygen content on the transport and magnetic properties of La1−xCaxMnO3+δ manganites. Solid State Commun. 123, 321–326 (2002). [Google Scholar]
  2. Nagashima M., Wada H., Tanikawa K. & Shirahata H. The electronic behaviors of oxygen-deficient VO2 thin films in low temperature region. Jpn. J. Appl. Phys. 37, 4433–4438 (1998). [Google Scholar]
  3. Zhao Y. et al. Electrical transport and magnetic properties of La0.5Ca0.5MnO3-y with varying oxygen content. Phys. Rev. B 65, 144406 (2002). [Google Scholar]
  4. Poulsen F. Defect chemistry modelling of oxygen-stoichiometry, vacancy concentrations, and conductivity of (La1−xSrx)yMnO3±δ. Solid State Ionics 129, 145–162 (2000). [Google Scholar]
  5. Wu J. et al. Strain-Induced Self Organization of Metal-Insulator Domains in Single-Crystalline VO2 Nanobeams. Nano Lett. 6, 2313–2317 (2006). [DOI] [PubMed] [Google Scholar]
  6. Cheng C. et al. Heat Transfer across the Interface between Nanoscale Solids and Gas. ASC Nano 5, 10102–10107 (2011). [DOI] [PubMed] [Google Scholar]
  7. Pellegrino L. et al. Multistate Memory Devices Based on Free-standing VO2/TiO2 Microstructures Driven by Joule Self-Heating. Adv. Mater. 24, 2929–2934 (2012). [DOI] [PubMed] [Google Scholar]
  8. Cheng C., Liu K., Xiang B., Suh J. & Wu J. Ultra-long, free-standing, single-crystalline vanadium dioxide micro/nanowires grown by simple thermal evaporation. Appl. Phys. Lett. 100, 103111 (2012). [Google Scholar]
  9. Manca N. et al. Programmable Mechanical Resonances in MEMS by Localized Joule Heating of Phase Change Materials. Adv. Mater. 25, 6430–6435 (2013). [DOI] [PubMed] [Google Scholar]
  10. Cheng C. et al. Self-Assembly and Horizontal Orientation Growth of VO2 Nanowires. Sci. Rep. 4, 5456 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Takami H., Kanki T. & Tanaka H. Multistep metal insulator transition in VO2 nanowires on Al2O3(0001) substrates. Appl. Phys. Lett. 104, 023104 (2014). [Google Scholar]
  12. Guo H. et al. Vanadium dioxide nanowire-based microthermometer for quantitative evaluation of electron beam heating. Nat. Commun. 5, 4986 (2015). [DOI] [PubMed] [Google Scholar]
  13. Matsui H. et al. Mid-infrared Plasmonic Resonances in 2D VO2 Nanosquare Arrays. Adv. Opt. Mater. In-press doi: 10.1002/adom.201500322. [DOI] [Google Scholar]
  14. Wei J., Ji H., Guo W., Nevidomskyy A. H. & Natelson D. Hydrogen stabilization of metallic vanadium dioxide in single-crystal nanobeams. Nat. Nanotechnol. 7, 357–362 (2012). [Google Scholar]
  15. Pan X., Zhao Y., Ren G. & Fan Z. Highly conductive VO2 treated with hydrogen for supercapacitors. Chem. Commun. (Camb). 49, 3943 (2013). [DOI] [PubMed] [Google Scholar]
  16. Hong W. et al. Hydrogen-induced morphotropic phase transformation of single-crystalline vanadium dioxide nanobeams. Nano Lett. 13, 1822 (2013). [DOI] [PubMed] [Google Scholar]
  17. Andreev V. N., Kapralova V. M. & Klimov V. A. Effect of hydrogenation on the metal-semiconductor phase transition in vanadium dioxide thin films. Phys. Solid State 49, 2318–2322 (2007). [Google Scholar]
  18. Andreev V. N., Klimov V. A. & Kompan M. E. Influence of hydrogenation on electrical conductivity of vanadium dioxide thin films. Phys. Solid State 54, 601–606 (2012). [Google Scholar]
  19. Filinchuk Y. et al. In situ diffraction study of catalytic hydrogenation of VO2: Stable phases and origins of metallicity. J. Am. Chem. Soc. 136, 8100–8109 (2014). [DOI] [PubMed] [Google Scholar]
  20. Sim J. S., Zhou Y. & Ramanathan S. Suspended sub-50 nm vanadium dioxide membrane transistors: fabrication and ionic liquid gating studies. Nanoscale 4, 7056–7062 (2012). [DOI] [PubMed] [Google Scholar]
  21. Ji H., Wei J. & Natelson D. Modulation of the electrical properties of VO2 nanobeams using an ionic liquid as a gating medium. Nano Lett. 12, 2988–2992 (2012). [DOI] [PubMed] [Google Scholar]
  22. Jeong J. et al. Suppression of metal-insulator transition in VO2 by electric field-induced oxygen vacancy formation. Science 339, 1402–1405 (2013). [DOI] [PubMed] [Google Scholar]
  23. Liu K. et al. Dense electron system from gate-controlled surface metal-insulator transition. Nano Lett. 12, 6272–6277 (2012). [DOI] [PubMed] [Google Scholar]
  24. Ohta H. et al. Field-induced water electrolysis switches an oxide semiconductor from an insulator to a metal. Nat. Commun. 1, 118 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  25. Ruzmetov D. et al. Three-terminal field effect devices utilizing thin film vanadium oxide as the channel layer. J. Appl. Phys. 107, 114516 (2010). [Google Scholar]
  26. Zhou Y. & Ranamanathan S. Relaxation dynamics of ionic liquid – VO2 interfaces and influence in electric double-layer transistors. J. Appl. Phys. 111, 084508 (2012). [Google Scholar]
  27. Sengupta S. et al. Field-effect modulation of conductance in VO2 nanobeam transistors with HfO2 as the gate dielectric. Appl. Phys. Lett. 99, 062114 (2011). [Google Scholar]
  28. Ruzmetov D. et al. Hall carrier density and magnetoresistance measurements in thin-film vanadium dioxide across the metal-insulator transition. Phys. Rev. B 79, 153107 (2009). [Google Scholar]
  29. Yuan H. et al. Hydrogenation-induced surface polarity recognition and proton memory behavior at protic-ionic-liquid/oxide electric-double-layer interfaces. J. Am. Chem. Soc. 132, 6672–6678 (2010). [DOI] [PubMed] [Google Scholar]
  30. Shi J., Zhou Y. & Ramanathan S. Colossal resistance switching and band gap modulation in a perovskite nickelate by electron doping. Nat. Commun. 5, 4860 (2014). [DOI] [PubMed] [Google Scholar]
  31. Mortimer R. J. Electrochromic Materials. Annu. Rev. Mater. Res. 41, 241–68 (2011). [Google Scholar]
  32. Yang Z. et al. Dielectric and carrier transport properties of vanadium dioxide thin films across the phase transition utilizing gated capacitor devices. Phys. Rev. B 82, 205101 (2010). [Google Scholar]
  33. Zhu B. & Mellander B.-E. fProton conduction and diffusion in Li2SO4. Solid State Ionics 97, 535–540 (1997). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information
srep17080-s1.pdf (864KB, pdf)

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES