Effects of dynamic shear and transmural pressure on wall shear stress sensitivity in collecting lymphatic vessels

Abstract

Given the known mechanosensitivity of the lymphatic vasculature, we sought to investigate the effects of dynamic wall shear stress (WSS) on collecting lymphatic vessels while controlling for transmural pressure. Using a previously developed ex vivo lymphatic perfusion system (ELPS) capable of independently controlling both transaxial pressure gradient and average transmural pressure on an isolated lymphatic vessel, we imposed a multitude of flow conditions on rat thoracic ducts, while controlling for transmural pressure and measuring diameter changes. By gradually increasing the imposed flow through a vessel, we determined the WSS at which the vessel first shows sign of contraction inhibition, defining this point as the shear stress sensitivity of the vessel. The shear stress threshold that triggered a contractile response was significantly greater at a transmural pressure of 5 cmH₂O (0.97 dyne/cm²) than at 3 cmH₂O (0.64 dyne/cm²). While contraction frequency was reduced when a steady WSS was applied, this inhibition was reversed when the applied WSS oscillated, even though the mean wall shear stresses between the conditions were not significantly different. When the applied oscillatory WSS was large enough, flow itself synchronized the lymphatic contractions to the exact frequency of the applied waveform. Both transmural pressure and the rate of change of WSS have significant impacts on the contractile response of lymphatic vessels to flow. Specifically, time-varying shear stress can alter the inhibition of phasic contraction frequency and even coordinate contractions, providing evidence that dynamic shear could play an important role in the contractile function of collecting lymphatic vessels.

the lymphatic system supports some of the human body's most critical functions, including maintaining tissue fluid balance (59), providing a route for immune cell and antigen transport to and from the lymph node (52), and transporting lipid from the gut to the blood (14), among others. Specifically, the network of vessels begins in the interstitial tissue spaces, subsequently merging downstream as it branches into larger vessels termed the collecting lymphatic vessels. These collecting vessels consist of luminal lymphatic endothelial cells (LECs) surrounded by muscle cells that actively contract to transport fluid toward the left subclavian vein (66). Like in the heart, these rapid phasic contractions are crucial in lymphatic transport, since interstitial fluid pressure alone is normally insufficient to move lymph against the adverse pressure gradient present in the system (4). However, like blood vessels, the collecting lymphatic vessels also actively alter their tone on a slower time scale in a manner that promotes passive lymph transport from externally applied pressure gradients (19, 49). The collecting lymphatic vessels are separated by one-way valves into unit segments known as lymphangions, which close under adverse pressure gradients to minimize backflow (11). Together, these two features operate in synchrony to control lymph transport throughout the body, which recent estimates put at almost 8 l/day for humans (37), However, unlike most veins, these vessels can rapidly contract to quickly eject fluid from one lymphangion to another, sometimes up to 75% of their diameter (13), a phenomenon achieved through the specialized muscle cells surrounding the collecting lymphatic vessel (44).

The contraction dynamics of collecting lymphatic vessels, which are categorized as either phasic or tonic and often referred to as the intrinsic pump, are highly sensitive to the mechanical forces imposed on the vessel (10, 19, 40, 42). Since lymph formation can vary widely, even during normal, physiological circumstances due to extrinsic factors (35) (such as digestion, skeletal muscle contraction, respiration, and interstitial fluid formation), it has been hypothesized that the lymphatic vessel's sensitivity to the local mechanical environment aids in optimizing lymph transport (19). This ability to sense and respond to local mechanical forces would certainly be beneficial to the vessels' intrinsic pumping, allowing it to optimize its performance for the rapidly varying loads in vivo. For instance, like the heart, collecting lymphatic vessels have been shown to quickly react to different levels of transmural pressure (23, 40) and preload/afterload (12, 57), parameters that change continuously on the basis of levels of lymph formation, body position, skeletal muscle activity, etc. Much like in the heart, the lymphangions' response to these loads appears tuned in such a way to maximize fluid output and minimize energy expenditure (21).

In addition to a contractile dependency on transmural pressure, isolated vessel and in vivo studies have also demonstrated inhibition of lymphatic pump function in response to luminal fluid shear stress (3, 18, 19, 30, 50). In isolated vessel studies, higher magnitudes of applied transaxial pressure gradient resulted in higher levels of inhibition for these contractile parameters. These responses are mediated by the lymphatic endothelium and have been shown to use similar mechanisms that regulate vasoactive responses in the blood vasculature (29). Likewise, the transition of flow direction has also been shown to modulate lymphatic contractility. Specifically, Gashev et al. (19) demonstrated that rapidly changing from orthograde to retrograde flow (as well as from orthograde or retrograde flow to no flow) temporarily increased contraction frequency in isolated lymphatic vessels. However, actual values of flow and, thus, wall shear stress have been difficult to obtain in isolated vessels, making it difficult to determine the exact magnitude of wall sheer stress (WSS) that can elicit a dilatory response in lymphatic vessels. Additionally, although lymphatic flow has been shown to be rapidly time-varying (and often oscillatory) in vivo (13), no one has yet to verify this fast chronotropic response in a collecting lymphatic vessel exposed to a continuously varying transaxial pressure gradient waveform (while also controlling for the effects of average transmural pressure). Moreover, since shear stress has previously been shown as a self-regulatory element in isolated lymphatic vessels (19, 21), the dynamic transaxial pressure gradient that occurs in vivo could play an essential role in the contractile coordination observed among lymphangions (65), a possibility that could greatly enhance lymph transport efficiency.

Despite these questions regarding both the effects of dynamic shear stress on contractility and of transmural pressure on shear sensitivity, it is very difficult to simultaneously address these issues due to the lack of adequate experimental tools. For instance, because the flow rates present in the lymphatic vessels are very low (13, 28), measuring fluid shear stress is difficult in these isolated vessel preparations due to the lack of adequate commercial flow sensors. Additionally, no one has been able to construct an ex vivo perfusion system capable of imposing independent transaxial pressure gradient and average transmural pressure waveforms dynamically to perform single-factor shear stress studies. In this respect, we have recently developed an ex vivo lymphatic perfusion system (ELPS) capable of dynamically estimating fluid shear stress and independently controlling both transaxial pressure gradient and average transmural pressure on an isolated lymphatic vessel (31). Here, we use this system to 1) determine the threshold at which lymphatic vessels exhibit a contractile response to WSS and investigate how it is affected by transmural pressure, 2) demonstrate that imposed oscillatory shear stress has a differential effect on lymphatic contractility compared with a steady shear stress of the same average magnitude, and 3) demonstrate that oscillatory transaxial pressure gradient waveforms can coordinate the phasic lymphatic contractile response to synchronize its frequency and minimize resistance to flow.

MATERIALS AND METHODS

Experimental hardware.

The ELPS consists of two independently actuated glass syringes connected in a closed-loop fashion on each side of an isolated vessel preparation. The system is connected by 1/16“ ID, 1/8” OD Tygon tubing with the exception of the small segments (silicon) placed in the two three-way pinch solenoid valves (Cole-Parmer, Vernon Hills, IL), which are joined in such a way to effectively form a four-way valve. In this way, when one syringe has expelled a majority of its fluid, the four-way solenoid valve switches, while the syringes begin to propagate in the opposite direction. Thus, the system can maintain the directionality of flow with respect to the isolated vessel for an indefinite period of time (25). Each syringe is 100 μl in volume (Hamilton, Reno, NV) and is actuated independently by a MX80L brushless linear stage motor (Parker Hannifin, Rohnert Park, CA), which permits the generation of independent transaxial pressure gradient (ΔP = P₁ − P₂) and average transmural pressure [P_avg = (P₁ + P₂)/2] waveforms (31).

The controller hardware consists of a chipKIT Uno32 microcontroller development board (Digilent, Pullman, WA), which is based on the Arduino Uno software platform (16, 32). The controller receives desired waveform values sent from a computer and uses feedback from the pressure sensors located on each side of the cannula pipettes in the flow loop to control the movement of the two syringes and, thus, in essence, control both the flow through the vessel and the average transmural pressure. Upon the start of an experiment, the diameter tracing is recorded in real time via a custom LabView program, using data from a bright-field camera capturing at 30 fps, as in other studies (18). Both diameter data and other sensor data are synchronized postexperiment using recorded time stamps.

Animals and isolated vessel preparation.

The extraction and cannulation technique of the thoracic ducts was similar to previous studies (18, 19), where a ∼1-cm segment (free of valves) was taken from a Sprague-Dawley rat and cannulated on two resistance-matched glass pipettes 350–500 μm in tip diameter. In total, we examined the contractile activity of thoracic ducts from nine Sprague-Dawley rats weighing roughly 300 g. All protocols were performed under established IACUC guidelines for the location of the given experiment (Georgia Tech or Texas A&M).

Once exteriorized, each thoracic duct segment was cannulated in a warm (38°C) bath of PSS (in mM: 145.0 NaCl, 4.7 KCl, 2.0 CaCl₂, 1.17 MgSO₄, 1.2 NaH₂PO₄, 5.0 dextrose, 2.0 sodium pyruvate, 0.02 ETA, and 3.0 MOPS) that was pH adjusted to 7.4. After the procedure was completed, the cannulation chamber was carefully integrated into the ELPS tubing (already perfused with warm PSS), so as to avoid forming bubbles and then placed onto the microscope stage. Once positioned, each vessel was allowed to equilibrate at 38°C for 20 min at a transmural pressure of 3 cmH₂O, while regular contractile activity was established. To confirm that the vessel preparation was functioning correctly, we sequentially imposed an average transmural pressure of 1, 3, 5, and then 3 cmH₂O with no transaxial pressure gradient and then a transaxial pressure gradient of 1 and 3 cmH₂O, with an average transmural pressure of 3 cmH₂O for 5 min each (30 min total). In addition to serving as control conditions for that vessel, we could ensure that decreased contractile function occurred during applied transaxial pressure gradients and that increased contractile frequency occurred during high transmural pressures (18, 19). At the end of the experiment, each vessel was equilibrated in Ca²⁺-free PSS for 20 min and subsequently exposed to an average transmural pressure of 1, 3, and 5 cmH₂O to observe the resting diameter (for calculating tone) at each pressure.

Fluid shear stress responsiveness.

To estimate the WSS imposed on the vessel, we employed the Poiseuille flow constitutive relation:

$${\tau }_w=\frac{32\mu Q}{\pi D^3}$$ (1)

where τ_w is the WSS imposed by the fluid, μ is the dynamic viscosity of the working fluid (taken to be that of water at 38°C, which is a reasonable estimate for PSS since it contains no protein), Q is the flow rate through the vessel, and D is the experimentally measured outer diastolic diameter of the vessel at the respective transmural pressure. This relationship has been shown to be sufficient for describing lymphatic fluid flow both experimentally (13) and computationally (51) because of the fact that both the Reynolds number and Womersley number are very low. We needed only to estimate the flow rate, Q, through the vessel to determine the applied WSS. Fortunately, because of the utilization of precision syringes and position encoders in the ELPS, it is possible to estimate the average flow rate through the vessel in sequential 5-s time windows, ignoring potential transient dynamics within each window (31). In other words, the average volume of fluid displaced from one syringe into the other during each 5-s time window should accurately approximate the flow rate through the vessel imposed by the system over that time.

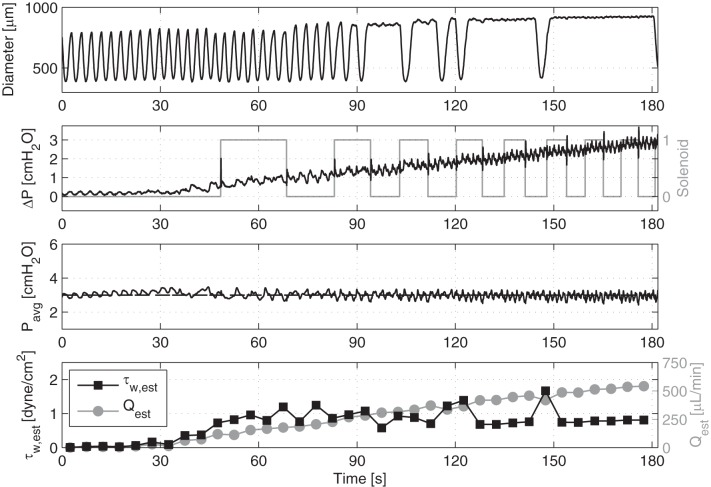
With these values in hand, we used the ELPS to impose a transaxial pressure gradient ramping from 0 to 3 cmH₂O over 3 min, while simultaneously holding the average transmural pressure constant at both 3 and 5 cmH₂O. Each ramp was preceded by 5 min of a zero transaxial pressure gradient at that respective average transmural pressure to allow the vessel to equilibrate again and resume contraction. In this way, the flow rate through the vessel (and, hence, the shear stress imposed on the vessel) could be estimated post hoc, and we could determine at which point contractile inhibition occurs through observation of the vessel diameter. We interpreted this information as the shear sensitivity of the vessel—in other words, the shear stress threshold at which the vessel begins to actively adjust its diameter based on the incoming fluid flow. However, to accomplish this, we first had to define an objective metric to determine at what point this contractile inhibition begins to occur. To do this, we used a peak detection algorithm in MATLAB to determine the time interval between each systolic peak in the diameter waveform. We then determined the point during the ramp in which the time between systolic peaks first presented with a significant delay (Fig. 1). This was defined as the first time in which the interval between two systolic peaks was greater than twice the average systolic interval during the no flow period. Since we can estimate the imposed flow rate that was occurring at this time, we can estimate the value of the imposed wall shear stress using Eq. 1.

Fig. 1.

Example showing an isolated vessel's diameter response to a ΔP ramp going from 0 to 3 cmH₂O over 3 min with a constant P_avg = 3 cmH₂O. Also shown is the resulting flow rate and shear stress values estimated over sequential 5-s time windows, as well as the rate of solenoid valve switching.

Application of transaxial pressure gradient sine functions.

To study the contractile response of lymphatic vessels to time-varying transaxial pressure gradients, we used the ELPS to apply the following function:

$$\Delta P=A\hspace{0.17em}\sin \hspace{0.17em}(2\pi ft)+C$$ (2)

where A is the amplitude [cmH₂O], f is the frequency [Hz], and C is the offset [cmH₂O]. This waveform was applied after 5 min of no applied transaxial pressure gradient with an average transmural pressure of 3 cmH₂O. Two separate conditions were tested following the shear stress ramps: f = 0.0525 and 0.125 Hz [or 3.75 and 7.5 min⁻¹, which are within the observed range of lymphatic contraction in vivo (13, 28)] for 5 min each. A in Eq. 2 was set to 0.5 cmH₂O and C was set to 1 cmH₂O to ensure that an average positive transaxial pressure gradient was imposed with a peak-to-peak amplitude of 1 cmH₂O. The average transmural pressure was held constant at P_avg = 3 cmH₂O. For each condition, the flow rate, wall shear stress, contraction frequency, and vessel tone were calculated. For comparison purposes, the mean shear stress, normalized contraction frequency, and tone were calculated for the steady flow control (ΔP = 1 cmH₂O and P_avg = 3 cmH₂O for 5 min).

On four separate vessel preparations, we investigated the vessel contractile response to a sine function of larger amplitude, but still within the range of the pressure amplitude experienced during phasic lymphatic contractions (3). Specifically, a condition consisting of A = 2 cmH₂O, f = 0.125 Hz [7.5 contractions per minute (min⁻¹)], and C = 2 cmH₂O (Eq. 2), with a constant average transmural pressure of 3 cmH₂O that was applied for 3 min. In addition, on two of these vessels, this condition was followed immediately by a similar sine function at half the frequency (3.75 min⁻¹). Each large-amplitude sine function was preceded by a 5-min condition with no applied transaxial pressure gradient and an average transmural pressure of 3 cmH₂O to allow the vessel to equilibrate between conditions. To analyze the vessel's response to this oscillatory transaxial pressure gradient, we calculated the point-to-point contraction frequency over time. In this way, the contractile activity of each vessel could be investigated upon the onset of a large, periodic transaxial pressure gradient waveform.

To check endothelial involvement in response to dynamic shear stress, we applied imposed flow waveforms to vessels with a disrupted endothelial layer and compared the response of these vessels to vessels with an intact endothelium. The vessel was allowed to equilibrate for 30 min at a transmural pressure 3 cmH₂O. An isolated thoracic duct (TD) segment with intact endothelium was exposed to an average transmural pressure of 1, 3, 5, and then 3 cmH₂O with no transaxial pressure gradient, then to transaxial pressure gradients of 1 and 3 cmH₂O with an average transmural pressure of 3 cmH₂O for 5 min each (30 min total). After this, sinusoidal flow waveforms (0.125 and 0.0625 Hz for 3 min each) using a large-amplitude transaxial pressure gradient (from 0 to 4 cm H₂O), were applied to the TD segment in PSS (control), while holding the transmural pressure constant. Each sinusoidal function was preceded by a 5-min period with no applied transaxial pressure gradient and an average transmural pressure of 3 cmH₂O to allow the vessel to equilibrate. A denudation of the endothelium was performed as previously described (17a). Shortly, the fluid inside of the TD segment was replaced with an air bolus for 10–12 s using a 1-ml syringe, and then the vessel was immediately flushed with PSS before further experimentation. Then the same steps as mentioned immediately above were applied to the TD segment with the disrupted endothelial layer.

To check whether the small changes in transmural pressure due to noise in the control algorithm were driving the contractile response rather than the applied flow, we applied a sinusoidal function to the transmural pressure with an average of 3 cmH₂O and a peak-to-peak amplitude of 0.5 cmH₂O, while holding the transaxial pressure gradient constant. After a 30-min equilibration period and the application of a range of transmural pressure and flow steps (as described above), sinusoidal average transmural pressure waveforms with frequency of 0.125 Hz and 0.0625 Hz with zero transaxial pressure gradient were applied for 3 min each.

Nitric oxide, a known endothelium-derived relaxation factor (EDRF) in the lymphatic vasculature, has been extensively studied and shows an inhibitory effect on tonic and phasic lymphatic vessel contraction (19, 21, 24, 43). To block NO production we used N^G-nitro-l-arginine methyl ester (l-NAME) (Sigma-Aldrich, St. Louis, MO) at 100 μM, which has been shown to be an effective concentration for inducing a vasoactive response in isolated lymphatic vessels (5, 21, 45). After completing the testing protocol described above to ensure proper vessel functionality in PSS (control), we set the transmural pressure at 3 cmH₂O and replaced PSS in the chamber with PSS containing l-NAME for 15 min. Then, the same protocol was repeated.

Histamine was recently shown as another lymphatic EDRF, as it demonstrates inhibitory effects on lymphatic vessel contractility similar to NO (47). However, histamine acts through a relaxing mechanism that is independent of NO synthesis (34). To block histamine production, we used α-methyl-dl-distidine dihydrochloride (α-MHD) (Sigma-Aldrich, St. Louis, MO) at 10 μM overnight, an effective histidine decarboxylase (HDC) blocker, which is a major enzyme involved in histamine production (17). Since completely depleting a histamine store may take up to 4 to 6 h (6), we used a protocol for overnight pharmacological blockage of HDC. That technique demonstrates maximum depletion of histamine stores in lymphatic vessels (47). Thoracic duct segments were separated into two groups: control and α-MHD-treated. One segment after dissection was placed in a 35-mm petri dish, filled with DMEM/F-12 solution at ∼37°C with an antibiotic mixture (Life Technologies, Grand Island, NY) to achieve a concentration of 100 U of penicillin and 100 μg streptomycin/ml of DMEM/F12. Another TD segment was placed separately in a 35-mm petri dish with the same solution plus α-MHD and both cultured overnight. The mechanical loading protocol, as described above, was executed the next day in control, HDC blockage, and HDC blockage + l-NAME-treated vessels.

In addition, we checked whether the soluble guanylyl cyclase (sGC) pathway is involved in the TD response to dynamic shear stress. It has been shown that the sGC inhibitor 1H-[1,2,4]Oxadiazolo[4,3-a]quinoxalin-1-one (ODQ) induced changes in the TD contractility similar to NO synthase blockade and successfully blocks all effects of the NO donor (20). Additionally, NO-independent activation of sGC has also been shown in response to histamine in lymphatic vessels (34) and NO-like compounds in the blood vasculature (48). To block sGC, we used the inhibitor ODQ (Sigma-Aldrich, St. Louis, MO) at a concentration of 30 μM (20, 34). The same protocol as mentioned above for l-NAME was implemented for ODQ treatment.

Statistics.

For making comparisons between the WSS threshold for a vessel at different transmural pressures, we used a two-tailed paired ratio Student's t-test. When multiple comparisons were performed, we used a paired one-way ANOVA with a Dunnett multiple-comparison correction. For all cases, significance was defined as P < 0.05 (*) or P < 0.01 (**) or P < 0.001 (***). For comparison between control and pharmacologically treated vessel responses to control and dynamic WSS, we used a two-way ANOVA followed by a Dunnett multiple-comparison correction.

RESULTS

Determination of lymphatic wall shear stress sensitivity.

Figure 1 is an example of the ELPS, applying a transaxial pressure gradient ramp from 0 to 3 cmH₂O over 3 min, while simultaneously holding the average transmural pressure constant at 3 cmH₂O. The rate of the solenoid valve switching (1 for ON and 0 for OFF), which is indicative of the volume of fluid being moved through the vessel, is also shown. As expected, the transaxial pressure gradient ramp also produced a linearly increasing flow rate, which was estimated every 5 s using the solenoid valve state and the position of the syringes. To verify the instantaneous Poiseuille flow assumption, both the Reynolds number and Womersley number were calculated for all cases to be less than 20 and 0.8, respectively, indicating that this shear stress constitutive relationship is perfectly valid since the flow is laminar and “steady-in-the-mean”.

Since shear stress is known to inhibit the intrinsic lymphatic pacemaker, we used an increase in the delay time between systolic peaks to estimate the shear stress at which the lymphatic vessel first showed a contractile response to shear. Thus, we were able to determine the minimum shear stress required for a vasoactive response. In general, this shear stress threshold always occurred at larger shears when the transmural pressure was 5 cmH₂O compared with 3 cmH₂O. Although there was a fairly wide range of shear threshold values between vessels (values ranged between 0.1 and 2.5 dynes/cm²), every vessel exhibited an increased shear threshold when the transmural pressure was raised. Thus, the mean shear threshold of the vessels at a transmural pressure of 5 cmH₂O (0.97 ± 0.36 dyne/cm²) was significantly higher (P < 0.05) than the shear threshold at an average transmural pressure of 3 cmH₂O (0.64 ± 0.22 dyne/cm²) (Fig. 2). One vessel exhibited no signs of shear inhibition, as defined in the methods and, thus, was excluded from the analysis.

Fig. 2.

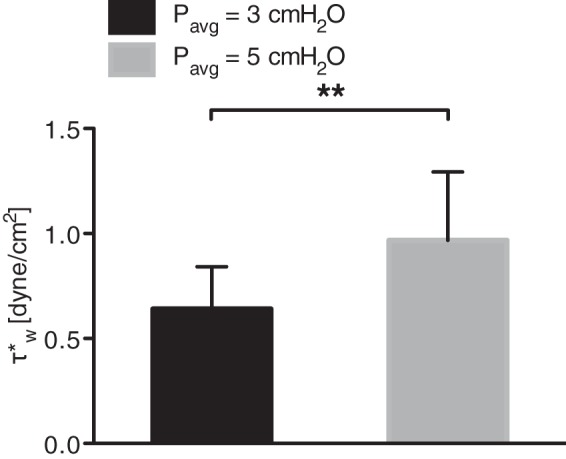
The mean shear threshold for contraction inhibition, ${\tau }_{\mathrm{w,}}^{*}$ at an average transmural pressure of 5 cmH₂O, 0.97 dyne/cm², was significantly higher than at an average transmural pressure of 3 cmH₂O, 0.64 dyne/cm². **P < 0.01.

Loss of shear inhibition under dynamic shear.

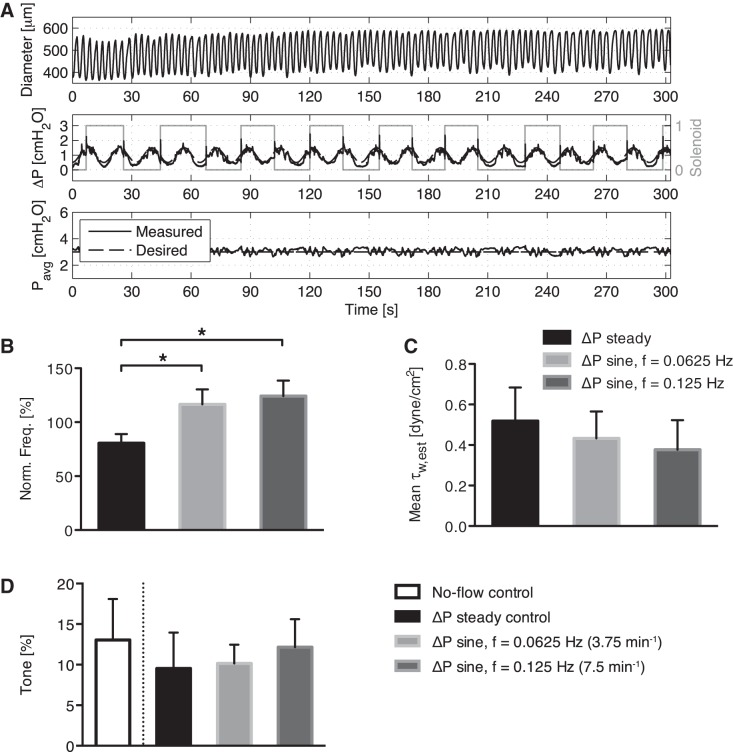
When the imposed flow through the vessels oscillates at frequencies within the range of in vivo lymphatic contractions (3.75–7.5 min⁻¹) and the imposed flow gradient is kept relatively low (while at the same time the average transmural pressure was kept constant at 3 cmH₂O), the vessel no longer exhibits inhibition of the contraction frequency set by the intrinsic pacemaker (see Fig. 3A for a representative tracing at 3.75 min⁻¹), as is typically seen in response to a steady applied WSS [data not shown but reported previously (31)]. The mean normalized contraction frequency (normalized to the no-flow control for that vessel) of the steady transaxial pressure gradient (91%) was significantly less (P < 0.05) than that seen in both the 3.75 min⁻¹ condition (114%) and the 7.5 min⁻¹ condition (121%) (Fig. 3B). However, the mean wall shear stresses for each imposed flow waveform (steady, 3.75 min⁻¹, and 7.5 min⁻¹) were relatively similar and not significantly different (Fig. 3C). If anything, the trend is for the contraction frequency to go above the control pacemaker frequency when an oscillatory WSS is imposed on the vessel. There were no statistical differences in vessel tone between any of the applied WSS conditions. (Fig. 3D).

Fig. 3.

A: example showing an isolated vessel's diameter response to a ΔP sine function with a frequency of 3.75 min⁻¹ and a constant average transmural pressure of 3 cmH₂O. Despite this lack of frequency inhibition under dynamic flow (B), the mean wall shear stress across all imposed flow conditions (C) (steady, 3.75 and 7.5 min⁻¹) was relatively similar and not significantly different. D: no statistical differences in vessel tone were observed for the various imposed flow conditions. *P < 0.05.

Contraction synchronization under dynamic shear.

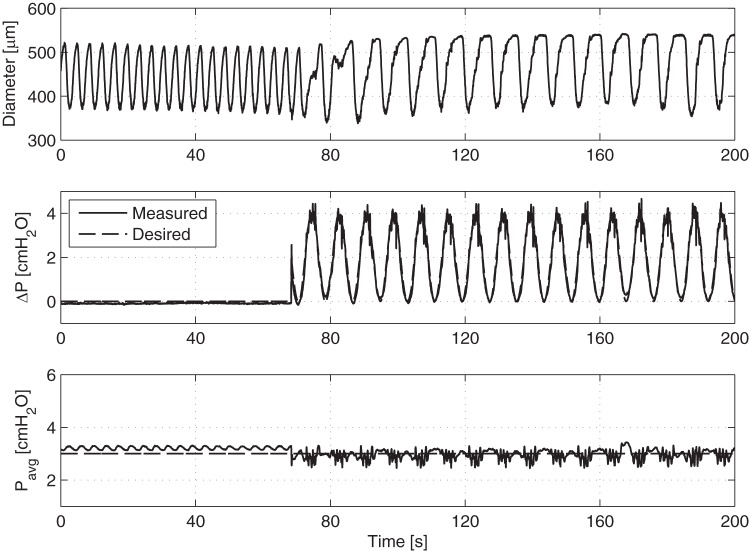
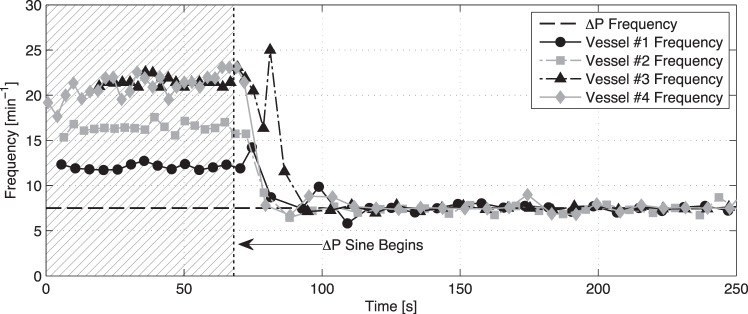
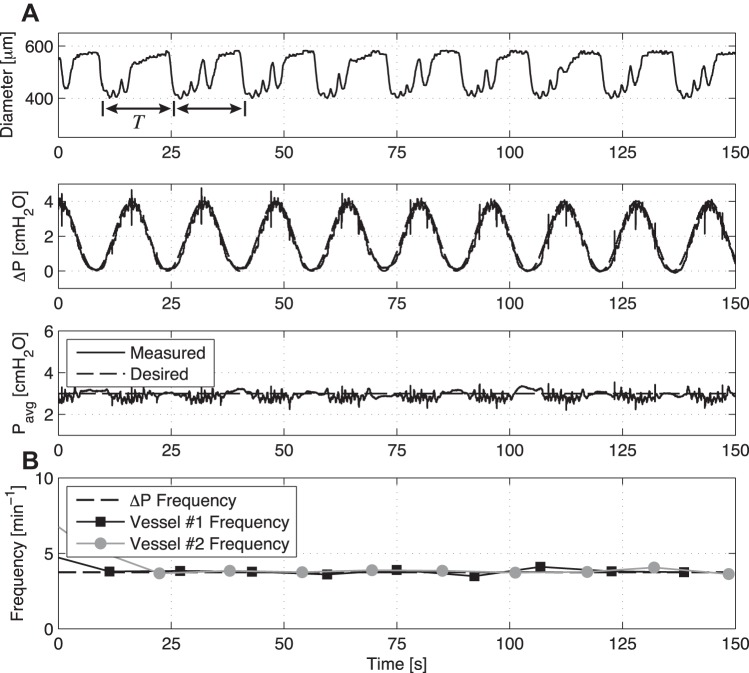
When the vessels were exposed to a time-varying flow waveform using a larger peak-to-peak amplitude transaxial pressure gradient (i.e., 4 cmH₂O instead of 1 cmH₂O), the vessel immediately begins to contract at the same frequency as that of the imposed flow waveform (Fig. 4). This phenomenon is more clearly seen in Fig. 5, which shows the point-to-point contraction frequency over time for four separate vessel preparations before and after the application of the large ΔP sinusoid (resulting in mean shear stresses of 4.03, 2.60, 2.02, and 4.12 dyne/cm², respectively, for these four vessels over the duration of the imposed sine wave). In all of these cases, the contraction frequency of each vessel changes relatively quickly from its corresponding intrinsic pumping frequency to that of the applied sine function. In addition to the large ΔP sine function at 7.5 min⁻¹, Fig. 6 shows the application of a similar sine function but at 3.75 min⁻¹. In this case, the vessel contracts at the same frequency of the applied sine wave. However, because of the lower frequency of the sine wave (3.75 vs. 7.5 min⁻¹), the vessel exhibits several small phasic contractions between each of the major contraction/inhibition cycles associated with the applied shear. Counting just the large-amplitude contractions, the contraction frequency over time matches the prescribed ΔP frequency exactly for two separate vessel preparations.

Fig. 4.

Wall shear can modulate lymphatic vessel contraction frequency. Representative tracing demonstrating an isolated vessel's diameter response to the onset of a ΔP sine function of large amplitude at a frequency of 7.5 min⁻¹. After the sine function is applied, the vessel begins to contract at the same frequency.

Fig. 5.

Point-to-point frequency data over time for four separate isolated vessel preparations upon the onset of the ΔP sine function from Fig. 7. Even though each vessel's baseline contraction frequency differs when flow is not imposed through the vessel, they all begin to contract at the same frequency of the imposed flow rate once it is applied (7.5 min⁻¹).

Fig. 6.

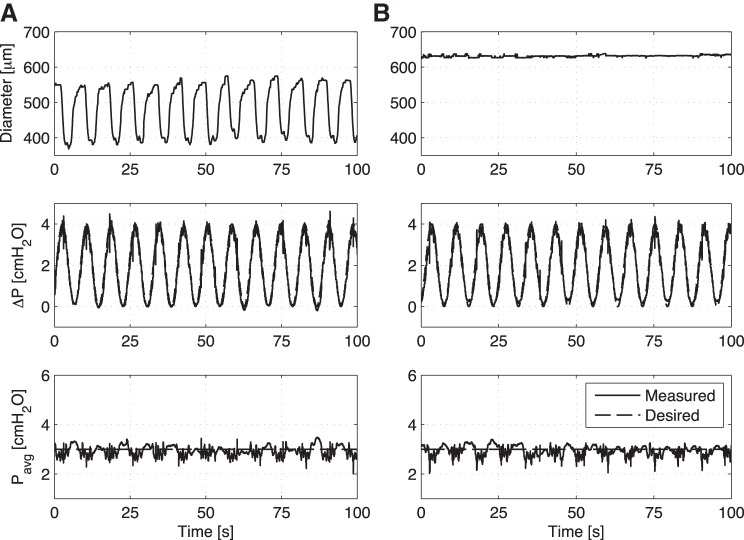
A: example demonstrating an isolated vessel's diameter response to a sine function of large amplitude with a frequency half that of Fig. 5, or 3.75 min⁻¹. Similar to before, the vessel has significant contractions at the same frequency of the applied sine wave; however, because of its slower nature, the vessel is able to attempt several phasic contractions between each significant contraction/inhibition cycle. B: counting just these significant contractions, the contraction frequency over time exactly matches the imposed flow frequency (3.75 min⁻¹) for two separate vessel preparations.

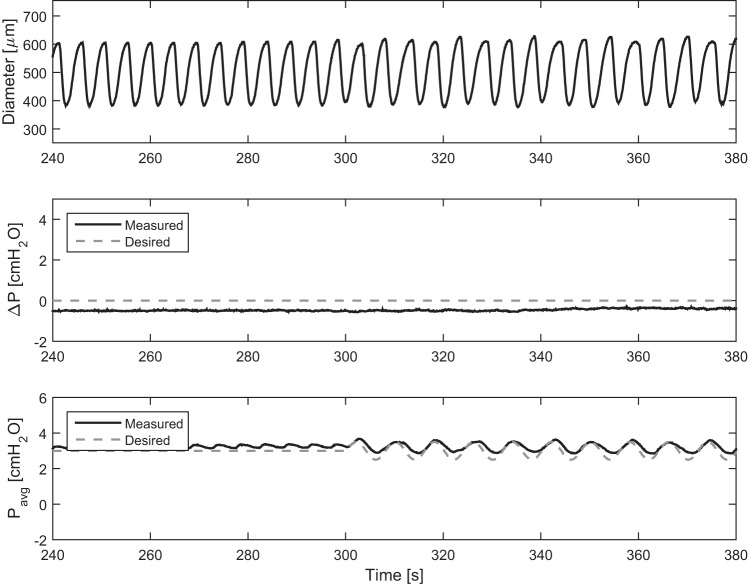
To ensure that the vessel responses to these large sine functions were not diameter responses artificially induced by the ELPS, the f = 7.5 min⁻¹ case was applied to a vessel equilibrated in calcium-free PSS to eliminate active tone and contractions (Fig. 7). Although the applied ΔP sine function resulted in contractions of similar frequency (Fig. 7A), after the application of calcium-free PSS, the passive vessel diameter was not affected by the condition (Fig. 7B). Hence, the vessel syncing observed was confirmed to be an active response from the vessel. To determine whether this active response could be due to the small fluctuations in transmural pressure that are a result of the noise in the control algorithm instead of the applied shear, we exposed a vessel to time-varying transmural pressures of similar magnitude to that of the noise of the pressure control system. These small pressure fluctuations do not alter the contraction of the vessel, further confirming that the response was due to fluid shear (Fig. 8).

Fig. 7.

To ensure that the vessel syncing was not artificially induced by the ELPS, a sine function of large amplitude [f = 0.125 Hz (7.5 min⁻¹), A = 2 dynes/cm², and C = 2 dyne/cm²] (Eq. 3) was applied to a vessel (A) with PSS and then (B) with calcium-free PSS. A: in PSS, the applied ΔP sine function resulted in contractions of similar frequency. B: however, after the application of calcium-free PSS, the passive vessel diameter was not affected by imposed flow pattern.

Fig. 8.

Small transmural pressure fluctuations do not cause changes in the contractile frequency. Without the large dynamic flow waveform (middle), the vessel does not shift its contractile frequency, as we imposed a small oscillatory transmural pressure (at time >300 s) to simulate the small changes observed in our other dynamic flow protocols (e.g., Fig. 4).

Contractile synchronization to wall shear is endothelium dependent.

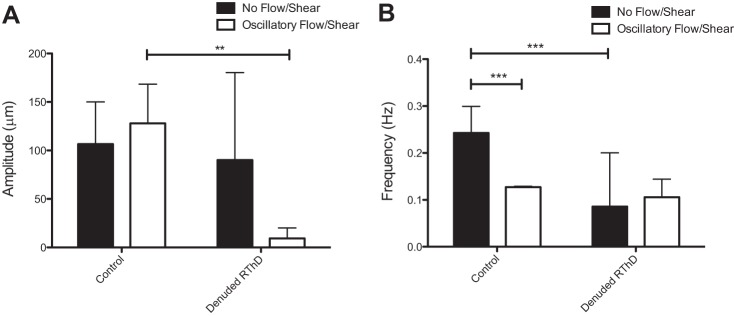
To demonstrate that the synchronization of the vessel contraction to dynamic wall shear stress involves lymphatic endothelial cells, a procedure was performed to mechanically remove most of the LEC from the lumen of the vessel. When the vessel is pressurized, but flow is not imposed, the vessel exhibits strong intrinsic contractions, although the frequency is lower and more erratic than when compared with static controls, in which the endothelium is completely intact. Once the dynamic shear is applied through the denuded vessel, the vessel does contract at the same frequency of the imposed flow waveform, but can no longer generate strong intrinsic contractions synced with the wall shear stress frequency, as is evident by the significantly reduced amplitude (Fig. 9), indicating that the LECs are required for producing strong synchronized contractions, perhaps by actively releasing a vasoactive substance when the fluid decelerates to near zero velocity.

Fig. 9.

Contractile synchronization is endothelium dependent. LECs were removed from the lumen of the vessel. A: while the vessel showed strong intrinsic contractions before dynamic flow is imposed, as is evidenced by the contractile amplitude, the vessel no longer displays strong intrinsic contractions phase-locked with the dynamic flow waveform when the endothelium has been physically disturbed. B: when the endothelium is mechanically damaged, the vessel has a reduced and highly variable contraction frequency under no flow. When dynamic shear is imposed on the vessel, the vessel still contracts at a frequency similar to the imposed waveform. **P < 0.01; ***P < 0.001.

Contractile synchronization is not regulated by histamine or endothelial nitric oxide synthase.

To determine whether histamine or endothelial nitric oxide synthase (eNOS), known endothelium-dependent regulators involved in the shear inhibition of contractile function in lymphatic vessels, is responsible for the synchronization of the lymphatic contraction to applied shear, vessels were exposed to a variety of inhibitors to these agents. Blocking NO production with l-NAME, blocking histamine production with α-MHD, or simultaneous blocking with both inhibitors had no effect on the synchronization of the lymphatic contractile wave to the applied WSS waveform (Fig. 10). To further demonstrate that NO is not involved in this response, we used ODQ to block sGC, the downstream receptor for nitric oxide responsible for its vasodilatory effects. This too, had no effect on the lymphatic contractile synchronization (Fig. 10).

Fig. 10.

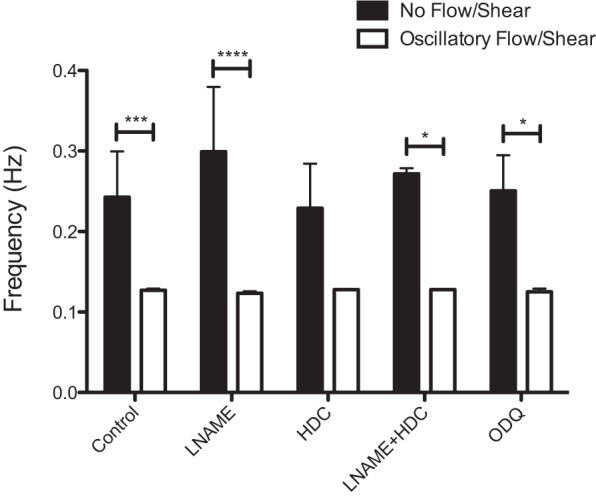
Histamine and nitric oxide are not responsible for contractile synchronization with flow. Vessels treated with 100 μM N^G-nitro-l-arginine methyl ester (l-NAME), 10 μM HDC incubated overnight to block histamine, both l-NAME (100 μM) and histidine decarboxylase (HDC; 10 μM), and 30 μM 1H-[1,2,4]Oxadiazolo[4,3-a]quinoxalin-1-one (ODQ) to block downstream effects of nitric oxide via the soluble guanylyl cyclase (sGC) pathway, all exhibited synchronization to the applied WSS waveform. *P < 0.05; ***P < 0.001; ****P < 0.0001.

DISCUSSION

As mentioned previously, one of the primary reasons that investigators have been unable to answer questions regarding shear stress sensitivity and shear stress dynamics in collecting lymphatic vessels is the lack of adequate experimental tools. In this respect, the ELPS has the unique ability to independently control the two primary mechanical stimuli imposed on a lymphatic vessel: average transmural pressure, P_avg, which affects circumferential stress, and transaxial pressure gradient, ΔP, which affects fluid shear stress via flow rate in a dynamic fashion (31). This capability is significant because independent control affords the ultimate flexibility to perform a wide range of experiments, including single-factor studies that aim to isolate shear-mediated mechanisms. Additionally, the ability to measure flow rate through the vessel allows investigators to calculate wall shear stress values empirically for the first time.

To quantify approximate levels of the shear stress threshold, we first needed to propose an objective metric to determine the magnitude of shear stress at which contraction inhibition first occurs. In brief, we utilized the linear relationship between wall shear stress and flow rate in the Poiseuille flow equation (Eq. 1) to define the metric. The shear thresholds reported (Table 1) do corroborate with shear stress ranges found in previous studies both in vitro and in vivo. In particular, Kawai et al. (29) found that eNOS activation in LECs (via calcium flux from the P_2X/P_2Y receptor) occurred above the threshold of 0.5 dyne/cm² and more recently Baeyens et al. (2) determined that a shear stress value of 4–6 dynes/cm² served as a “set-point” in which the WSS was large enough to cause the LECs to remodel and align in the direction of flow. Furthermore, Dixon et al. (13) measured the average fluid shear stress in vivo (0.64 dyne/cm²) within the rat mesentery, and Rahbar et al. (50) recently reported average shear stresses of 0.12 and 1.5 dyne/cm² for control and edemagenic stress conditions, respectively, within the rat mesentery in vivo. Additionally, given that the vessel cannulation chamber for this set-up is similar to the set-up used in the previously reported flow inhibition studies (18, 19), the applied transaxial pressure gradients used in other reports to inhibit flow were also likely within the physiological range of wall shear stress experienced by lymphatic vessels in vivo. All of these in vivo experiments are based on an assumption of constant viscosity, as it currently remains unclear how lymph viscosity might vary in vivo under normal or inflammatory conditions. Additionally, it is worth noting that the estimates of WSS are based on measurements of the outer diameter, which is easily obtainable throughout the experiment using the recorded video sequence. Although the true WSS would depend on the inner wall diameter, rat thoracic ducts are fairly thin with reasonably low variability in their thickness (32.2 ± 1.6 μm, as recently reported by Ref. 7), thus approximating WSS with the outer diameter, will tend to slightly underestimate the true WSS.

Table 1.

Shear stress sensitivity values, τ_w^*, calculated for the vessels used in the study at the average transmural pressures of 3 and 5 cmH₂O

		Shear sensitivity, τw*, dyne/cm2
Vessel	Diameter, μm	Pavg = 3	Pavg = 5
1	666	0.91	0.98
2	1069	0.29	0.43
3	964	0.39	0.57
4	857	0.08	0.46
5	612	1.45	2.54
6	665	0.72	0.82

As a reference, the calcium-free diameter at 5 cmH₂O is also provided.

Overall, the shear threshold was found to increase when the transmural pressure increased (Fig. 2), suggesting a dependency of the flow response on the transmural pressure, as was shown in previous isolated blood vessel studies (33, 58). With respect to the magnitude of fluid shear stress in lymphatic vessels, the levels of shear experienced by LECs in vivo are much less compared with similarly sized blood microvessels (13, 46); thus, the shear regulation of lymphatic contractile function appears significantly more sensitive. Blood endothelial cell responses to fluid shear stress are known to be partly mediated by junctional proteins (specifically, VE-cadherin, PECAM-1) in association with vascular endothelial growth factor receptor-2 (VEGFR-2) (60), with fluid shear stress recently being shown to increase the force on the junctional protein PECAM-1 (9). This difference in sensitivity could be attributed, in part, to differences in this junctional complex that result from the LEC-specific expression of VEGFR-3 (2) and differences in PECAM-1 expression, which are typically lower in LECs than blood endothelial cells (BECs). Altogether, this evidence suggests that the transmural pressure within a collecting lymphatic, which certainly affects circumferential stress within the endothelium, and, thus, the force exerted on the junctional proteins, could assist in mediating the vessel's shear sensitivity. Further investigation is needed to provide better insight into the physiological mechanisms of this phenomenon.

The difference in shear stress thresholds at different transmural pressures is particularly interesting in the context of lymphatic pathologies that alter the mechanical environment surrounding the vessels. One such pathology is lymphedema, a disease characterized by gross swelling of the tissue estimated to affect over 130 million people worldwide (53, 54). Lymphedema has been shown to result in elevated transmural pressure in the affected area (22), and the accumulation of fibrotic tissue and adipocytes due to lymph stasis can drastically alter the mechanical environment surrounding the vessels (55). In this context our results suggest that the chronic disruption of the mechanical state of the collecting lymphatic vessels via transmural pressure could have a significant effect on their shear stress sensitivity. Whether a loss of shear sensitivity is detrimental to lymphatic function remains experimentally unexplored; however, computational models have suggested that flow-mediated dilation is important for enhancing lymph transport in situations of elevated lymph flow (49). Interestingly, impaired flow-mediated dilation has been a hallmark of endothelial dysfunction and is routinely used as a clinical diagnostic in the blood vasculature (27), and thus, further investigation is certainly warranted to determine whether a similar mechanism is in play in lymphatic disease.

Furthermore, investigators have recently shown that the unique biomechanical environment of lymphatic vessels is essential in guiding the lymphatic system's development (8, 56, 59a); thus, the presence of chronically high pressures could result in shifts of shear sensitivity toward another phenotype, such as veins. However, extrapolating these results to pathological cases remains difficult since current data on the mechanical sensitivity of lymphatic vessels have only been collected across relatively short time scales. Like mechanically induced growth and remodeling displayed in the blood vasculature that occurs over days and weeks (26), lymphatic vessels have also been qualitatively shown to remodel in response to pathological levels of mechanical loading (8, 15, 41). Hence, future work in this area should focus on longer-term studies relating the consequences of vessel remodeling in lymphatic disease to the mechanosensitivity of the intrinsic lymphatic pump.

The lymphatic response to WSS is not only dependent on the transmural pressure, but the rate of the applied WSS also plays a significant role, as the control condition of steady imposed flow resulted in inhibition of the pacemaker frequency, while the applied dynamic shear of the same average magnitude did not (Fig. 3). Thus, imposing a low-magnitude oscillatory flow rate (average WSS ∼1 dyne/cm²) within a physiological frequency range (13) produces results different than the typical frequency inhibition that occurs in response to a steady imposed shear stress. This result would support the recent in vivo findings of Rahbar et al. (50), who found that, in a rat model of edemagenic stress, the contraction frequency did not decrease compared with control, although the wall shear stress was almost an order of magnitude higher (50). Of course, another major difference is that in these isolated vessel studies, the transmural pressure was held constant to only account for the effects of WSS. However, in the cited in vivo model of edemagenic stress, the transmural pressure in the lymphatics goes up after volume loading, which according to our results would in itself raise the WSS necessary to inhibit contractile function (3). The results in this current study also confirm the original observation by Gashev et al. (19) that transaxial pressure gradient transitions can temporarily reverse flow inhibition in isolated lymphatic vessels. As originally proposed in that paper, this lack of phasic contractile inhibition could be beneficial during normal pump function, in which each lymphangion experiences rapidly changing flow rates and wall shear stresses.

However, unlike what Gashev et al. (19) originally proposed, a reversal of the transaxial pressure gradient (or fluid shear stress) was not necessary to observe this effect on contraction frequency in the current studies. Although it has been previously established that collecting lymphatic vessels are exposed to oscillatory flow rates during normal contractile function (13, 28), the two ΔP sine functions that we imposed (Fig. 3) were strictly positive and contained no flow reversal. Hence, reversal of flow direction was unnecessary to impede the phasic frequency inhibition normally observed in response to fluid shear stress. Since Gashev et al. (19) only observed this fast chronotropic response when the transaxial pressure was altered after flow was already established (in either direction, orthograde, or retrograde), perhaps fluid deceleration could play a role in these observations.

Both the frequency and magnitude of the WSS during the imposed oscillatory transaxial pressure gradient profoundly affect the contractile response. When the average magnitude of the oscillatory shear was ∼1 dyne/cm² (Fig. 3), we did not observe phase locking of the contraction frequency to the imposed shear frequency nor did we observe any signs of inhibition of the pacemaking frequency as is seen with steady WSS. However, when the oscillatory WSS is large enough (but still well within the reported in vivo range), it actually has the ability to match the frequency of contraction to that of the imposed oscillatory shears (Figs. 4 and 5). Although the vessels studied did not exhibit similar contraction amplitude trends, contractile tone was decreased in all cases and contractile events were synchronized with the applied transaxial pressure gradient waveform, even though the transmural pressure was held essentially constant. In addition, this syncing effect was not just unique to one frequency (i.e., 7.5 min⁻¹), as it was seen in two vessels that were also exposed to a flow rate at half the frequency (3.75 min⁻¹) (Fig. 6). While this response requires the endothelium (Fig. 9), which is not surprising given that this is the primary cell type that experiences the dynamic WSS, the underlying molecular mechanisms remain unknown. Compared with the blood vasculature, very little is known regarding the role of endothelium-derived relaxing factors in lymphatic vasoregulation; thus, we chose to focus on the two EDRF that have been established in the literature to participate in the shear-mediated response in lymphatics: nitric oxide and histamine (18, 19, 21, 30, 34, 47). Interestingly, neither of these appears to play a role in the phase locking response to dynamic shear (Fig. 10). The fact that vessels have significantly reduced contraction amplitude in response to flow when the endothelium is disturbed suggests that LEC produce vasoconstrictor substances to facilitate contractile coordination. This is a completely unexplored area of lymphatic physiology and warrants future study to determine the existence of such substances released by LEC and their role in the shear-mediated lymphatic response.

Computational models of lymphatic pumping have produced conflicting results. While one model of a lymphangion chain suggested that coordination of contraction does not assist in helping promote lymph flow (62), more recent models that incorporate experimentally measured mechanical properties and more realistic values of physical properties of the lymphatics (e.g., valve hysteresis, dependence of valve resistance on vessel diameter, nonlinear pressure-diameter relationships) demonstrated computationally that the phase difference between individual contractions along a chain of lymphangions is a significant parameter in the overall pumping efficiency (4). This model along with the fact that most experimental observations of lymphatic vessel chains in vivo and in isolated vessels show coordination (39, 42, 65, 67), suggests that some level of coordination among vessel segments would be beneficial. Practically speaking, it would be advantageous for a downstream lymphangion (or perhaps multiple downstream lymphangions) to be dilated as the upstream lymphangion ejects its contents downstream during systole. Alternatively, in tissue beds where extrinsic mechanisms play an important role in driving lymph flow (e.g., intestinal peristalsis, respiration, and skeletal muscle contractions), an immediate dilation of the lymphatic vessel in response to an extrinsically driven packet of lymph flow, would lower resistance to flow and improve overall transport. This may play important roles in maintaining efficient overall lymph flow through impedance matching within the lymphatic network. For instance, it is feasible that oscillatory lymph flows, driven by skeletal muscular contractions, respiratory movements, or other similar mechanisms, could override the intrinsic pacemaking of downstream lymphatics and produce phase locking to the oscillatory flows that we demonstrate here. Such events are qualitatively observed in the behavior of packet flow that has been described with near-infrared lymphatic imaging (36, 63, 64). Lymphatic vessel segments are capable of contractile entrainment, and it is hypothesized that the electrical coupling between lymphangions plays an important role (39, 65). Moreover, it has been shown that 80–90% of rat lymphatic contractions are coordinated between adjacent lymphangions, and this coordination is due, in part, to gap junction communication (39, 65). A recent report utilized computational modeling to demonstrate, similar to our experimental results here, that wall shear stress could coordinate lymphatic contractile function (33a). While this paper proposed that this would be achieved through LEC release of nitric oxide (NO), our results suggest that, at least in the thoracic duct, NO is not responsible.

While the evidence is clear that there are pacemaking-like regions along the lymphatic where the lymphatic contraction initiates (1, 38, 39, 61) and that pressure/stretch and flow/shear can alter the pacemaker frequency (18, 19, 30, 40), the extent that the frequency of pacing can be driven by shear forces alone remains unexplored. Details of the biophysical mechanisms that are responsible for lymphatic contractile coordination have eluded researchers. The results in this current study provide direct evidence that fluid shear stress has the capacity to regulate the coordination of lymphatic pumping activity between lymphangions, further supporting the hypothesis that wall shear stress is a potential self-regulatory mechanism in the rat thoracic duct that optimizes lymphatic pumping (21). Although care should be taken when extrapolating these results in vivo, as there are a variety of mechanical and biochemical factors that are constantly changing around the lymphatic microenvironment, and it is difficult to determine the relative importance of the WSS response when other competing factors are at play.

Perspectives and Significance

The mechanical forces that the lymphatics are exposed to in vivo are highly dynamic and typically an order of magnitude or two lower than those experienced by the blood endothelium, and yet studies have demonstrated a remarkable level of mechanosensitivity of lymphatics. In light of the complexity of the mechanical environment experienced by lymphatics, we used our recently developed ELPS system to test isolated lymphatics for the first time under physiologically realistic dynamic waveforms. The new capabilities provided by the ELPS to both estimate fluid shear stress and independently (and dynamically) vary ΔP and P_avg—the factors modulating the two primary mechanical forces exerted on lymphatics: fluid shear stress and circumferential stress—allow for new single-factor studies to be performed that would be nearly impossible with in vivo models. In particular, the ability to dynamically vary ΔP (hence shear stress), while controlling for average transmural pressure, has allowed us to explore the interactions between transmural pressure and shear sensitivity, the effects of dynamically varying shear on contraction frequency, as well as the existence of shear-specific contractile coordination. In addition to providing these interesting insights into the functional role of shear stress on the collecting lymphatics, we believe the ELPS provides a promising platform to perform new studies aimed at isolating the effects of fluid shear stress and transmural pressure. By unraveling the difficult and complex interactions comprising the biomechanical state of lymphatic vessels, we hope this platform will spark novel discoveries in the future with regard to both physiological and diseased conditions of lymphatic fluid transport.

GRANTS

This material is based upon work supported under a National Science Foundation Graduate Research Fellowship. Any opinions, findings, conclusions or recommendations expressed in this publication are those of the author(s) and do not necessarily reflect the views of the National Science Foundation. This study was also funded by the National Institutes of Health (R00HL-091133, R01HL-113061, R01HL-094269, R01HL-096552, and U01HL-123420).

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the authors.

AUTHOR CONTRIBUTIONS

Author contributions: J.A.K., Z.N., O.Y.G., D.C.Z., and J.B.D. conception and design of research; J.A.K., Z.N., and O.Y.G. performed experiments; J.A.K., Z.N., A.M., and J.B.D. analyzed data; J.A.K., Z.N., D.C.Z., and J.B.D. interpreted results of experiments; J.A.K., Z.N., and A.M. prepared figures; J.A.K. drafted manuscript; J.A.K., Z.N., O.Y.G., A.M., D.C.Z., and J.B.D. edited and revised manuscript; J.A.K., Z.N., O.Y.G., A.M., D.C.Z., and J.B.D. approved final version of manuscript.