Skip to main content
PMC home page
NIHPA Author Manuscripts logoLink to NIHPA Author Manuscripts
. Author manuscript; available in PMC: 2015 Dec 6.
Published in final edited form as: Psychol Sci. 2012 Apr 5;23(5):453–458. doi: 10.1177/0956797611430096

How to Be Proactive About Interference: Lessons From Animal Memory

Anthony A Wright 1, Jeffrey S Katz 2, Wei Ji Ma 3
PMCID: PMC4670779  NIHMSID: NIHMS735467  PMID: 22491142

Abstract

Processes of proactive interference were explored using the pigeon as a model system of memory. This study shows that proactive interference extends back in time at least 16 trials (and as many minutes), revealing a continuum of interference and providing a framework for studying memory. Pigeons were tested in a delayed same/different task containing trial-unique pictures. On interference trials, sample pictures from previous trials reappeared as test pictures on different trials. Proactive-interference functions showed greatest interference from the most recent trial and with the longer of two delays (10 s vs. 1 s). These interference functions are accounted for by a time-estimation model based on signal detection theory. The model predicts that accuracy at test is determined solely by the ratio of the elapsed time since the offset of the current-trial sample to the elapsed time since the offset of the interfering sample. Implications for comparing memory of different species and different types of memory (e.g., familiarity vs. recollection) are discussed.

Keywords: comparative psychology, memory, time perception, cognitive processes, signal detection theory

The study of short-term memory has a history of focusing on decay and forgetting in order to account for performance decreases over time (Baddeley, 1992; Brown, 1958; Peterson & Peterson, 1959). Capacity-limited models of short-term memory continue this trend of focusing on decay and forgetting (e.g., Atkinson & Shiffrin, 1968; Cowan, 2001, 2005; Rensink, 2002). A limited-capacity short-term memory would rapidly turn over as other stimuli in the environment are encountered. By contrast, no decline in memory performance (i.e., no memory decay or short-term turnover) was found by Keppel and Underwood (1962) on the first tests of stimuli, but substantial declines occurred, particularly at longer delays, after only three repetitions of those stimuli. The authors concluded that repeating the same stimuli produced proactive interference on subsequent trials and thereby caused the observed decline in memory performance.

Proactive interference has been shown in recognition-memory procedures using small sets of repeating stimuli with humans and (nonhuman) animals when stimuli to be identified as “different” (from the to-be-remembered stimuli) have been seen on the immediately preceding trial (e.g., Makovski & Jiang, 2008; Roberts & Grant, 1976). It is somewhat remarkable that any stimulus-specific interference effects were discernible in these studies because the stimuli from these small sets had been seen hundreds of times, which likely produced near-saturation of proactive interference. To more thoroughly explore proactive interference—that is, explore proactive interference from stimuli further back in time than just the preceding trial—requires that the background level of proactive interference on baseline trials be lowered by using a large stimulus set and minimizing stimulus repetitions. If the background level of proactive interference is minimized, then the effects of controlled interference can be judged against a baseline of no interference to reveal the extent and limits (e.g., the capacity) of different types of memory (e.g., short-term and long-term memory) with and without proactive interference, which will perhaps lead to improved comparisons of memory among species.

In same/different and serial-probe-recognition tasks, proactive interference occurs when previously seen sample pictures are later re-presented as test pictures on trials with nonmatching sample pictures (i.e., different trials). Having seen the test picture previously tends to create confusion as to whether this picture was the sample picture in the current trial or in some previous trial. In the experiment reported here, we used a large set of pictures (1,024 pictures of man-made objects, natural objects, animals, scenes, etc.) selected to be trial unique for at least 2 weeks of testing (except for stimuli on the proactive-interference tests). We tested interference of memory at two delays by systematically placing interfering stimuli 1, 2, 4, 8, or 16 trials prior to interference tests in a delayed same/different task with trial-unique baseline trials. Surprisingly, interference was greater at the longer delay, despite the interfering stimuli being encountered considerably further in the past than at the shorter delay.

Experiment

The 4 pigeons in the current study were trained to perform a delayed same/different task with pairs of pictures (selected from the set of 1,024 pictures; for more details, see Subjects and Apparatus in the Supplemental Material available online; also see Katz & Wright, 2006, for a similar training procedure). The pigeons pecked a sample picture presented in the upper half of a computer screen 20 times (a fixed-ratio 20, or FR 20, schedule), and then the screen went blank. Following a delay, a test picture plus a white rectangle were presented in the lower half of the screen (see Fig. 1). If the two pictures were the same, then a peck to the test picture was correct; if they were different, then a peck to the white rectangle was correct. All the pigeons showed accurate transfer to novel stimuli, demonstrating abstract-concept learning. They were then trained extensively, with increasing delays between the offset of the sample picture and the onset of the test picture, prior to proactive-interference testing at 1-s and 10-s delays.

Fig. 1.

Fig. 1

Open in a new tab

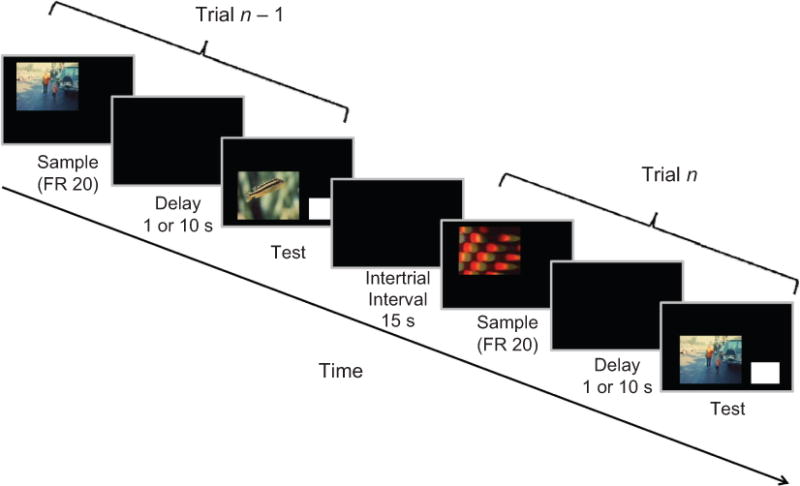
Example trial sequence for proactive-interference testing. In this example, an interference stimulus is presented as the sample on trial n − 1. This same stimulus appears again on the next trial (n) as the test stimulus. The correct response on trial n is a “different” response (a peck to the white rectangle). However, having seen this stimulus on the previous trial increases the chances of an error (making a “same” response by pecking the test picture) due to confusing the previous sample (on trial n − 1) with the current sample (on trial n). On each trial, pigeons were required to peck the sample picture 20 times (a fixed-ratio 20, or FR 20, schedule) before the delay began. The relative sizes of the pictures, white rectangle, and monitors in this illustration are not to scale.

Proactive-interference testing began with a block of 24 sessions at the 1-s delay, followed by a block of 24 sessions at the 10-s delay. We then repeated these tests (using different interference pictures and picture sequences) to assess reproducibility and any effects of testing order. Each daily session consisted of 64 trials with five interference tests (one test each at the 1-, 2-, 4-, 8-, and 16-trial separations). Trials other than those of the interference tests (i.e., same and different baseline trials) contained randomly selected (without replacement) pictures from the 1,024-picture set. There were overall totals of 32 same and 32 different trials in each session. Pictures used to test for interference had not been presented for at least 10 sessions (2 weeks) and had not been used as previous interference-test pictures.

Results

Figure 2 shows the major results from the proactive-interference tests. The percentage of correct responses was analyzed in a three-way Testing Order (first two blocks, second two blocks) × Delay (1 s, 10 s) × Interfering-Stimulus Separation (n − 1, n − 2, n − 4, n − 8, n − 16, no-PI) repeated measures analysis of variance (ANOVA). For the no-PI, baseline condition, only different-trial performance was used in this and other analyses because PI trials were different trials (see Statistical Analyses of Baseline Performance in the Supplemental Material). This analysis showed significant main effects of delay, F(1, 3) = 25.95, p < .016, ηp2 = .896; interfering-stimulus separation, F(5, 15) = 19.91, p < .001, ηp2 = .869; and the Delay × Interfering-Stimulus Separation interaction, F(5, 15) = 4.05, p < .017, ηp2 = .574. There was no significant effect of testing order, F(1, 3) = 1.1, p > .37, and there were no interactions between testing order and other factors, Fs < 1.02; therefore, results from the two tests at each delay were combined in Figure 2 (see Statistical Analyses of Baseline Performance in the Supplemental Material).

Fig. 2.

Fig. 2

Open in a new tab

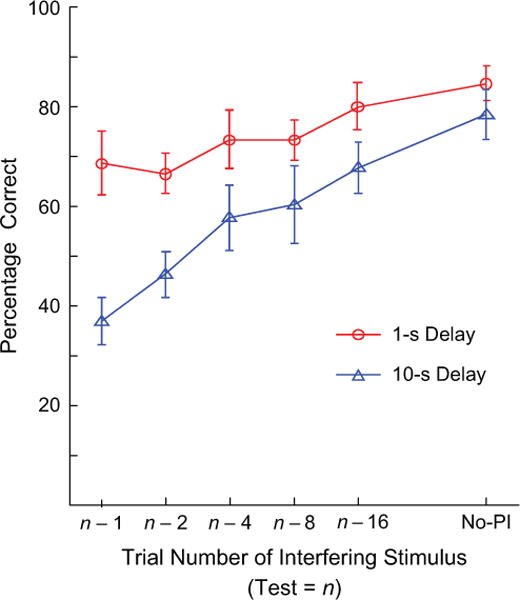
Mean results of 4 pigeons on the same/different task at 1-s and 10-s delays between the offset of the sample stimulus and onset of the test stimulus. Percentage correct is shown as a function of the number of trials separating the interfering stimulus and the test. On the left of the x-axis, “n − 1” refers to the condition in which the interfering stimulus occurred on the immediately preceding trial. On the right of the x-axis, “no-PI” refers to the no-interference, or different-trial baseline, condition. Error bars represent standard errors of the mean.

At the shorter delay of 1s (Fig. 2), there was a 17.1% interference effect for stimuli presented on the immediately preceding two trials (n − 1, n − 2) relative to baseline (no-PI) accuracy, t(3) = 4.22, p < .025. This interference effect largely dissipated when the interfering picture was presented 16 trials prior to test: Performance was 80.2% accurate with the 16-trial interfering-stimulus separation, compared with 84.7% in the no-PI (baseline) condition, t(3) = 1.99, p > .14. Trend analyses confirmed that accuracy significantly increased as a function of the number of intervening trials for both the 1-s and the 10-s delays, Fs(1, 3) > 14.54, ps < .04.

At the longer delay of 10 s (Fig. 2), the interference effect for stimuli presented on the immediately preceding trial (n − 1) was much greater (41.7%), t(3) = 5.4, p < .013, than at the shorter delay of 1 s (17.1%). As trial separation at the 10-s delay gradually increased from 1 to 16 trials, accuracy rose a substantial 30% (from 37.0% to 67.7%, respectively), t(3) = 5.94, p < .011. Nevertheless, there was still an 11% interference effect for stimuli presented 16 trials prior (and 16 min prior) to the test (see Elapsed Times in the Supplemental Material). It is important to note that below-chance performance (37% in this case) is meaningful because proactive interference could theoretically have driven accuracy to 0%.

Discussion

Our finding of greater interference of memory at the longer (10-s) delay than at the shorter (1-s) delay is counterintuitive. Although the interfering stimuli at the 10-s delay were encountered more distantly in the past than were the interfering stimuli at the 1-s delay (> 200 s more distantly for n − 16; see Elapsed Times in the Supplemental Material), they nevertheless produced greater interference. Encountering stimuli more distantly in the past should, according to models of decay or limited capacity, translate to more forgetting and therefore less interference. But just the opposite occurred.

We used a model based on signal detection theory to explain why the longer (10-s) delay produced greater interference. A direct consequence of this model is that interference depends on time ratios, not absolute times. According to the model, the subject retrieves memory of sample pictures, including not only the most recent sample picture but also other recently viewed sample pictures. However, the subject’s memory about when these sample pictures were seen is “noisy,” which results in some probability of the subject mixing up the temporal order of the current and a past sample picture. When a sample picture from a previous trial is identified as a match to the current test picture, the subject may erroneously make a “same” response.

The internal representation of elapsed time is assumed to follow the well-known Weber-Fechner law, or log scale of time (Buhusi & Meck, 2005; Gibbon, 1977), and to vary from trial to trial, as shown in Figure 3a. The subject makes its decision on a given trial according to a likelihood ratio determined on the basis of noisy evidence and a criterion for responding “same” or “different.” Therefore, the model accounts for response biases (“same” or “different” biases); in addition, it incorporates a measure of random guessing, because pigeons’ performance (like that of other nonhuman animals) is seldom 100% accurate. Performance is predicted to depend only on the ratio of the time elapsed since the offset of the current sample (i.e., delay time, denoted TC) to the time elapsed since the offset of the interfering sample (denoted TI; see Elapsed Times in the Supplemental Material). The model is expressed in the following equation:

PCmodel=12+12(1−g)erf−logTCTI−b2σ,

where PCmodel is the proportion of correct responses predicted by the model, erf is the error function, g is the guessing rate, b is the response bias, and σ is the standard deviation of the noise (for the derivation, see Signal Detection Theory Model of Proactive Interference in the Supplemental Material). The model was fit to the functions for both the 1-s and the 10-s delay, simultaneously for each subject, which provided considerable constraint to each fit. The means of the individual fits are shown in Figure 3b (see Model Fitting in the Supplemental Material for individual subjects’ fits). The model provides an excellent quantitative match (R2 = .95) to the proactive-interference functions from both delays and also, importantly, to the results for the no-PI condition.

Fig. 3.

Fig. 3

Open in a new tab

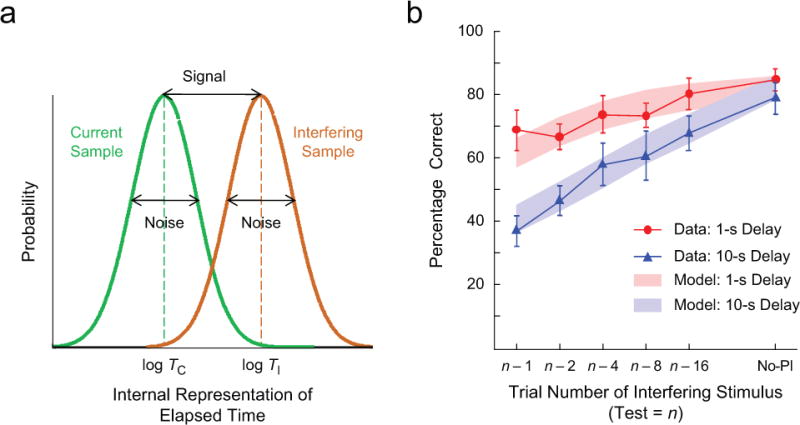
Model and data fit. In our signal detection model of proactive interference (a), the signal is given by the difference between the log of the retention-delay time (log TC) and the log of the time between the offset of the interfering sample presented in a previous trial and the onset of the test-trial picture (log TI). The observer’s internal representation of elapsed time is noisy, as indicated by the width of the normal distributions. The graph (b) shows the mean model fit to the data from Figure 2. The model was fit simultaneously to the proactive-interference functions for both delays for each individual subject. On the right of the x-axis, “no-PI” refers to the no-interference, or different-trial baseline, condition. The shaded areas show 1 SEM of the individual fits (see Model Fitting in the Supplemental Material).

According to this model, proactive interference should be equally affected by viewing time, intertrial-interval time, response time, and reinforcement (reward or punishment) time. One implication of this model is that increased processing of the current sample should not, in itself, combat proactive interference (i.e., facilitate discrimination of old from new) any more than should an equivalent amount of time added to the intertrial interval. Another implication is that time-outs following incorrect responses (popular in the training of animal subjects) should hasten learning by reducing proactive interference, in addition to any effect of delaying the time to the next opportunity for reinforcement—a popular explanation in animal learning.

It is important to note that the data cannot be explained by alternative familiarity models, which assume that the subject simply reports whether the test stimulus was or was not seen before, including models based on decaying familiarity. According to such models, performance would depend only on the absolute time from the interfering stimulus, not the critical time ratio shown here.

Explaining memory on the basis of time ratios has a substantial history in human-memory research for effects occurring within individual trials, including stimulus-distinctiveness effects (e.g., Cowan, 2001, 2005; Murdock, 1960; Nairne, Neath, Serra, & Byun, 1997), primacy effects (e.g., Bjork, 2001; Nairne et al., 1997; Neath, 1993), and recency effects (e.g., Glenberg, Bradley, Kraus, & Renzaglia, 1983; Howard & Kahana, 2002). Our results show that time ratios are crucial well beyond the current trial and account for effects on memory resulting from events that occurred as many as 16 trials prior.

Some human-memory researchers have used a time-distance metaphor of telephone poles appearing closer together as they recede into the distance to explain these effects (e.g., Nairne, 2002). Although this time-distance metaphor has previously been applied to events within single trials, it can also be applied to the proactive-interference effects observed in our study, which spanned several trials. The first (nearest) telephone pole would be the test stimulus on the test trial, and the second pole would be the sample stimulus on that test trial. Consequently, the gap between these first two poles would be 10 times greater for the 10-s delay condition than for the 1-s delay condition. This greater gap (i.e., the 10-s delay) would make the second pole (the sample on the test trial) relatively closer to the other poles receding into the distance, thereby making the current-trial sample more confusable with past samples and more vulnerable to proactive interference.

Although our experiment and model are based on tests of pigeon memory, implications of these findings may extend well beyond pigeons and familiarity. We argue that pigeons are not limited to indiscriminate familiarity because proactive interference dissipates after several (e.g., 16) trials and its dissipation did not result from forgetting, as shown by the robust proactive interference at these same trial numbers at the longer (10-s) delay.

The proactive-interference functions observed in our experiment have implications for the vast majority of memory studies using small stimulus sets, because proactive interference rapidly builds and saturates (e.g., Keppel & Underwood, 1962). Studies of pigeon and monkey memory using only two colored stimuli (which repeat in different combinations as samples and distractors over many trials and sessions) have shown nearly complete forgetting in as short a time as 30 s (e.g., Overman & Doty, 1980; Roberts & Grant, 1976; see Wright, 2007, for review). Additional evidence from Overman and Doty’s (1980) study, however, showed that this apparent rapid forgetting was actually due to interference, not forgetting. In two follow-up studies, these authors showed that tests with larger stimulus sets (100 or more pictures) reduced repetition of stimuli across trials, which in turn reduced interference and dramatically improved memory performance (85% accuracy at 180-s delays and even ≥ 70% accuracy at a 24-hr delay). Likewise, animal list-memory studies with small stimulus sets (6 pictures) showed relatively mediocre (70%) 3-item memory performance (Devine & Jones, 1975; Sands & Wright, 1980), but increasing the size of the stimulus sets (to 111 pictures) reduced stimulus repetition and proactive interference and dramatically improved memory performance (from 70% accuracy to 93% accuracy; Sands & Wright, 1980). These comparisons show that repeating stimuli from small stimulus sets has a powerful and detrimental effect on animal memory performance.

Studies of human memory, including popular change-detection studies conducted to evaluate human memory capacity (e.g., 4 ± 1 items), also typically use small stimulus sets—for example, six colored squares, letters, polygons, or shaded cubes (e.g., Alvarez & Cavanagh, 2004; Eng, Chen, & Jiang, 2005). However, proactive interference is only rarely evaluated in such studies (but see Hartshorne, 2008; Makovski & Jiang, 2008). Although the typical use of short retention delays (0.5–1.0 s) in these change-detection studies would tend to minimize interference, the short stimulus-presentation times and intertrial intervals (e.g., 0.5 s) would tend to enhance interference. Perhaps systematically manipulating these time variables and testing proactive interference in change-detection studies might reveal that proactive interference can affect visual short-term memory capacity. Any effects of long-term memory on capacity, including proactive interference, would pose challenges for fixed-capacity theories of visual short-term memory (cf. Brady, Konkle, & Alvarez, 2011; Cowan, Johnson, & Saults, 2005; Hintzman, 2011).

Supplementary Material

1

Acknowledgments

The authors would like to thank Jacquelyne J. Rivera, Thomas A. Daniel, Adam M. Goodman, and John F. Magnotti for their assistance with data collection and Anne B. Sereno, Saumil Patel, and L. Caitlin Elmore for their comments and suggestions on an earlier draft of this manuscript.

Funding

This research and the preparation of this article were supported by National Institutes of Health Grants R01MH072616 and R01MH091038 (to A. A. W.) and R01EY020958 (to W. J. M.).

Footnotes

Declaration of Conflicting Interests

The authors declared that they had no conflicts of interest with respect to their authorship or the publication of this article.

Supplemental Material

Additional supporting information may be found at http:/pss.sagepub.com/content/by/supplemental-data

References

  1. Alvarez GA, Cavanagh P. The capacity of visual short-term memory is set both by visual information load and by number of objects. Psychological Science. 2004;15:106–111. doi: 10.1111/j.0963-7214.2004.01502006.x. [DOI] [PubMed] [Google Scholar]
  2. Atkinson RC, Shiffrin RM. Human memory: A proposed system and its control processes. In: Spence KW, Spence JT, editors. The psychology of learning and motivation. Vol. 2. New York, NY: Academic Press; 1968. pp. 89–105. [Google Scholar]
  3. Baddeley AD. Working memory. Science. 1992;255:556–559. doi: 10.1126/science.1736359. [DOI] [PubMed] [Google Scholar]
  4. Bjork RA. Recency and recovery in human memory. In: Roediger HL III, Nairne JS, Neath I, Surprenant AM, editors. The nature of remembering: Essays in honor of Robert G Crowder. Washington, DC: American Psychological Association; 2001. pp. 211–232. [Google Scholar]
  5. Brady TF, Konkle T, Alvarez GA. A review of visual memory capacity: Beyond individual items and toward structured representations. Journal of Vision. 2011;11(5) doi: 10.1167/11.5.4. Article 4. Retrieved from http://journalofvision.org/11/5/4. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Brown J. Some tests of the decay theory of immediate memory. Quarterly Journal of Experimental Psychology. 1958;10:12–21. doi: 10.1080/17470218.2014.914546. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. Buhusi CV, Meck WH. What makes us tick? Functional and neural mechanisms of interval timing. Nature Reviews Neuroscience. 2005;6:755–765. doi: 10.1038/nrn1764. [DOI] [PubMed] [Google Scholar]
  8. Cowan N. The magical number 4 in short-term memory: A reconsideration of mental storage capacity [Target article and commentaries] Behavioral and Brain Sciences. 2001;24:87–185. doi: 10.1017/s0140525x01003922. [DOI] [PubMed] [Google Scholar]
  9. Cowan N. Working memory capacity. Hove, England: Psychology Press; 2005. [Google Scholar]
  10. Cowan N, Johnson TD, Saults JS. Capacity limits in list item recognition: Evidence from proactive interference. Memory. 2005;13:293–299. doi: 10.1080/09658210344000206. [DOI] [PubMed] [Google Scholar]
  11. Devine JV, Jones LC. Matching to successive samples: A multiple-unit memory task with rhesus monkeys. Behavior Research Methods & Instrumentation. 1975;7:438–440. [Google Scholar]
  12. Eng HY, Chen D, Jiang Y. Visual working memory for simple and complex visual stimuli. Psychonomic Bulletin & Review. 2005;12:1127–1133. doi: 10.3758/bf03206454. [DOI] [PubMed] [Google Scholar]
  13. Gibbon J. Scalar expectancy theory and Weber’s law in animal timing. Psychological Review. 1977;84:279–325. [Google Scholar]
  14. Glenberg AM, Bradley MM, Kraus TA, Renzaglia GJ. Studies of the long-term recency effect: Support for a contextually guided retrieval hypothesis. Journal of Experimental Psychology: Learning, Memory, and Cognition. 1983;9:231–255. [Google Scholar]
  15. Hartshorne JK. Visual working memory capacity and proactive interference. PLoS One. 2008;3(7):e2716. doi: 10.1371/journal.pone.0002716. Retrieved from http://www.plosone.org/article/info:doi/10.1371/journal.pone.0002716. [DOI] [PMC free article] [PubMed] [Google Scholar]
  16. Hintzman DL. Research strategy in the study of memory: Fads, fallacies, and the search for the “coordinates of truth”. Perspectives on Psychological Science. 2011;6:253–271. doi: 10.1177/1745691611406924. [DOI] [PubMed] [Google Scholar]
  17. Howard MW, Kahana MJ. A distributed representation of temporal context. Journal of Mathematical Psychology. 2002;46:269–299. [Google Scholar]
  18. Katz JS, Wright AA. Same/different abstract-concept learning by pigeons. Journal of Experimental Psychology: Animal Behavior Processes. 2006;32:80–86. doi: 10.1037/0097-7403.32.1.80. [DOI] [PubMed] [Google Scholar]
  19. Keppel G, Underwood BJ. Proactive inhibition in short-term retention of single items. Journal of Verbal Learning and Verbal Behavior. 1962;1:153–161. [Google Scholar]
  20. Makovski T, Jiang YV. Proactive interference from items previously stored in visual working memory. Memory & Cognition. 2008;36:43–52. doi: 10.3758/mc.36.1.43. [DOI] [PubMed] [Google Scholar]
  21. Murdock BB., Jr The distinctiveness of stimuli. Psychological Review. 1960;67:16–31. doi: 10.1037/h0042382. [DOI] [PubMed] [Google Scholar]
  22. Nairne JS. Remembering over the short-term: The case against the standard model. Annual Review of Psychology. 2002;53:53–81. doi: 10.1146/annurev.psych.53.100901.135131. [DOI] [PubMed] [Google Scholar]
  23. Nairne JS, Neath I, Serra M, Byun E. Positional distinctiveness and the ratio rule in free recall. Journal of Memory and Language. 1997;37:155–166. [Google Scholar]
  24. Neath I. Distinctiveness and serial position effects in recognition. Memory & Cognition. 1993;21:689–698. doi: 10.3758/bf03197199. [DOI] [PubMed] [Google Scholar]
  25. Overman WH, Doty RW. Prolonged visual memory in macaques and man. Neuroscience. 1980;5:1825–1831. doi: 10.1016/0306-4522(80)90032-9. [DOI] [PubMed] [Google Scholar]
  26. Peterson LR, Peterson MJ. Short-term retention of individual verbal items. Journal of Experimental Psychology. 1959;58:193–198. doi: 10.1037/h0049234. [DOI] [PubMed] [Google Scholar]
  27. Rensink RA. Change detection. Annual Review of Psychology. 2002;53:245–277. doi: 10.1146/annurev.psych.53.100901.135125. [DOI] [PubMed] [Google Scholar]
  28. Roberts WA, Grant DS. Studies of short-term memory in the pigeon using the delayed matching-to-sample procedure. In: Medin DL, Roberts WA, Davis RT, editors. Processes of animal memory. Hillsdale, NJ: Erlbaum; 1976. pp. 79–112. [Google Scholar]
  29. Sands SF, Wright AA. Primate memory: Retention of serial list items by a rhesus monkey. Science. 1980;209:938–940. doi: 10.1126/science.6773143. [DOI] [PubMed] [Google Scholar]
  30. Wright AA. An experimental analysis of memory processing. Journal of the Experimental Analysis of Behavior. 2007;88:405–433. doi: 10.1901/jeab.2007.88-405. [DOI] [PMC free article] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

1
NIHMS735467-supplement-1.pdf (57.2KB, pdf)

RESOURCES