Abstract
Objective
This study investigated the effect of body size and shape and harness fit on suspension tolerance time.
Background
Fall victims may develop suspension trauma, a potentially fatal reduction of return blood flow from legs to the heart and brain, after a successfully arrested fall if they are not rescued quickly or the harness does not fit them well.
Method
For this study, 20 men and 17 women with construction experience were suspended from the dorsal D-ring of a full-body fall-arrest harness. Their suspension tolerance time, physical characteristics, and harness fit levels were assessed.
Results
Body characteristics (i.e., weight, stature, upper- and lower-torso depths) were associated with decreased suspension tolerance time (r = −.36 ~ –.45, p ≤ .03). In addition, harness fit affected suspension tolerance time; workers with a torso angle of suspension greater than 35°, a thigh strap angle greater than 50°, or a poorly fitting harness size had shorter suspension tolerance time (mean differences = 14, 11, and 9.8 min, respectively, p ≤ .05).
Conclusion
Body size and harness fit were predictors of suspension tolerance time. Selecting well-fit harnesses and establishing a 9-min rescue plan are suggested to ensure that no more than 5% of workers would experience suspension trauma.
Applications
The study provides a basis for harness designers, standards writers, and manufacturers to improve harness configurations and testing requirements for better worker protection against suspension trauma.
Keywords: 3-D scan, body shape, harness fit, suspension, anthropometry, fall arrest, rescue
INTRODUCTION
In 1995, the Occupational Safety and Health Administration (OSHA) mandated a construction standard specifying that full-body harnesses replace waist belts for fall arrest in a personal fall arrest system effective in 1998 (U.S. Department of Labor, 2011), which has resulted in an increase in the use of full-body harnesses for fall protection. Along with the OSHA mandate, safety professionals and scientists have furthered our knowledge on fall protection in the past decade, including the postfall suspension and rescue issues.
After a worker’s fall has been successfully arrested by a full-body harness, the suspended worker needs to be rescued promptly. A prolonged suspension can cause the pooling of blood in the legs and the reduction of the return blood flow to the heart. The restrictions of the femoral arteries and veins caused by the harness straps can worsen venous pooling. Other factors, such as immobilization because of injury, neurological disorders, aging, dehydration, traumatic shock, or norepinephrine-transporter deficiency, can further speed the detrimental effects, which may damage vital organs, such as the kidneys (Robertson, 2008; Seddon, 2002; Shannon et al., 2000). This phenomenon of venous pooling is known as suspension trauma.
Some studies have investigated the tolerance of participants to motionless suspension, mainly that of men wearing full-body harnesses (Brinkley, 1988; Weber & Michels-Brendel,1990). With small sample sizes, suspension tolerance was, as measured, 3.5 to 60 min, with about half the trials in these studies terminated voluntarily by the participants, possibly because of discomfort caused by poor harness design or fit. Although these researchers have speculated about the harness-fit effect on suspension tolerance, that issue was not explored. There is an urgent need to investigate the role of harness design and fit on suspension trauma in workers (Noel, Ardouin, Archer, Amphoux, & Sevin, 1991).
Harness fit can be attributed to four key parameters: harness ring location, torso angle of suspension, strap configuration, and human body characteristics (i.e., size and shape; Hsiao, Whitestone, Taylor, Godby, & Guan, 2009). For harness ring location, there is a general understanding that the harness dorsal D-ring should be positioned between the inferior and superior borders of the scapula while the user is standing. The chest ring is to be placed at the sternum area. Harness manufacturers have more detailed ring location specifications, which are generally included in product manuals. The correlation between these fit criteria and suspension tolerance has not been investigated.
The second element of harness fit to the user’s body is the torso angle of suspension (Hsiao, Whitestone, et al., 2009). The angle of suspension is measured between the torso center line and the harness vertical suspension line. Using a rigid torso dummy for drop testing, the American National Standards Institute’s (ANSI) Z359.1 standard specifies that the angle at rest shall not exceed 30° (American Society of Safety Engineers, 1992). Canadian Standards Association’s (CSA) CAN/CSA-Z259.10-06 standard also allows a maximum angle of 30° (CSA, 2006). The International Organization of Standards (ISO) 10333-1 (ISO, 2002) and European EN 361 (European Committee for Standardization, 1992) standards allow maximum angles of 45° and 50°, respectively. These standards, however, do not specify why these angle limits were specified and how they affect suspension tolerance (Seddon, 2002).
The third element for harness fit is strap configuration fit. Strap configuration fit refers to the shoulder strap location (in relation to the neck base and acromion) and thigh strap angle (defined as the angle between the thigh strap and the sagittal plane). A study of 216 participants using an overhead-style harness showed no major fit concerns with the shoulder strap configurations for the tested harness (Hsiao, Whitestone, & Kau, 2007). The same study revealed that the group of participants who received a fail fit rating had a greater mean thigh strap angle from the sagittal plane than the group who received a pass fit rating. It is unknown whether this fit outcome affects suspension tolerance.
The last element for harness fit is human body size and shape. Literature has reported that body weight is a fair predictor for suspension tolerance (Seddon, 2002; Turner, Wassell, Whisler, & Zwiener, 2008) and that a relatively bulky chest combined with a short torso is associated with a poor harness-fit rating (Hsiao, Friess, Bradtmiller, & Rohlf, 2009). However, very limited information is available in the literature on the impact of body shape, harness size selection, and gender of a user on suspension tolerance.
The current study was conducted to determine the effects of harness fit on suspension tolerance time to make recommendations for harness design and rescue plans. The hypotheses were that (a) there would be a significant relationship between suspension tolerance time and body weight, stature, gender, harness static fit, torso angle of suspension, upper- and lower-torso depths, thigh strap angles, and harness size match level; (b) torso angles of suspension would not change during the course of a suspension; (c) harness static fit (harness ring locations) and suspension fit (torso angles of suspension) would be worse for women than for men; (d) workers with a large suspension angle (>35°) or thigh strap angle (>50°) would have a shorter suspension tolerance; (e) participants who use a size of harness that is different from the best-fit size would have shorter suspension tolerance time; and (f) rescue should occur within 10 min to assure that no more than 5% of workers would experience suspension trauma symptoms.
METHOD
Dependent Variable
Suspension tolerance
Suspension tolerance was defined as the duration of motionless suspended time until (a) any sign of medical orthostatic intolerance or (b) a voluntary termination by the participant because of extreme discomfort. The signs of orthostatic intolerance included (a) a systolic blood pressure (BP) decrease of more than 20 mmHg below the pretest value, (b) a diastolic BP decrease of more than 10 mmHg below the pretest value, (c) a heart rate (HR) increase of more than 28 bpm over the pretest value, (d) an HR decrease of more than 10 bpm from baseline, (e) a pulse pressure decrease to less than 18 mmHg, or (f) an observed shortness of breath, nausea, dizziness, or diastolic BP greater than 100 mm Hg (Streeten, 1987).
Independent Variables
Gender
New roles for women in the traditionally male-dominated construction work-force make it necessary that both female and male body dimensions be considered in the assessment of harnesses fit and suspension tolerance.
Body weight
The harnesses used in this study were designed to accommodate body weights varying from 50 kg to 141 kg. Participation recruitment was set in this range. The actual weight variation of the participants was 50 kg to 107.6 kg.
Stature
The harnesses used in this study were designed to accommodate stature varying from 150 cm to 200 cm. The actual variation of the participants was 158.6 cm to 194.7 cm.
Harness static fit
Harness static fit was evaluated on the basis of the locations of the chest D-ring and the dorsal D-ring while standing, per the harness manufacturer’s instructions. If the chest D-ring base was 0 to10 cm below the armpits and the dorsal D-ring was 0 to 5 cm above or below the armpits, the static fit was recorded as pass. Otherwise, it was recorded as fail.
Angle of suspension and suspension fit
The angles of suspension (Figure 1) were measured from the sagittal plane of 3-D scan images, which were registered by a full-body scanner (Figure 2). Participants were scanned at the beginning of the suspension and then every 5 min thereafter during their course of suspension. Suspension fit was defined as pass if the angle of suspension at the beginning of a suspension was no more than 35°.
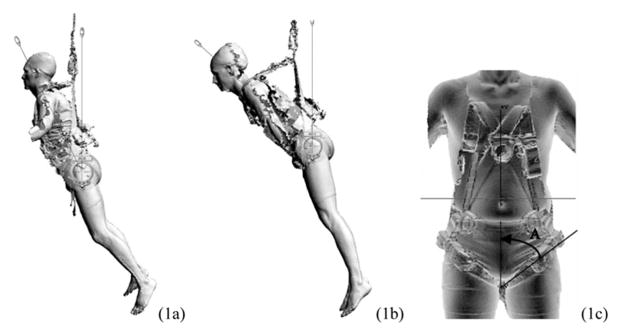
Figure 1.
The angle of suspension is measured between the torso centerline and the harness vertical suspension line. In 1a, the suspension fit rating is a pass (angle of suspension = 26°); in 1b, the suspension fit rating is a fail (angle of suspension = 48°). The thigh strap angle A in 1c is defined by the line that connects the highest point and the lowest point of the thigh strap and in reference to the body sagittal plane.
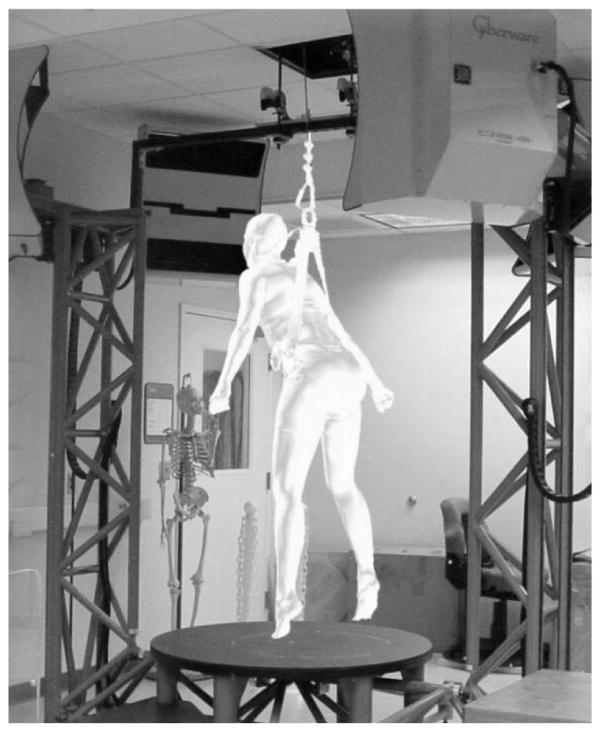
Figure 2.
The CyberwareWB4 3-dimensional full-body scanner (Monterey, CA) with a custom-made suspension system was used to suspend the participant and register the interface between the harness and participant.
Thigh strap angles
The thigh strap angle was defined by the line that connects the highest point and the lowest point of the thigh strap and in reference to the body sagittal plane when the participant was standing erect while wearing a harness (Figure 1).
Torso shape and size and best-fit harness size
Four torso shape and size dimensions (upper-torso breadth, upper-torso depth, lower-torso breadth, and lower-torso depth) were extracted from the 3-D torso scan images of the participants. The 10th rib locations were used to separate the torso images into the upper- and lower-torso regions. These dimensions were empirically tested and were found to suitably explain the interaction of the general body shape and dimensions with harness fit (for details, see Hsiao et al., 2007).
These four dimensions, along with stature, weight, and gender, were used as input parameters to four body-shape-based harness size prediction equations to determine the best-fit harness size. Standard size (STD) was set as the reference size; the resulting probability for choosing a specific size larger or smaller than the STD fell between 0 and 1.
| (1) |
| (2) |
| (3) |
| (4) |
in which breadthlow, breadthup, depthlow, and depthup are lower-torso breadth, upper-torso breadth, lower-torso depth, and upper-torso depth, respectively (Hsiao et al., 2007).
Predicted harness size by body weight and stature
When purchasing a harness, one needs a sizing chart for the harness user and vendor to identify the best-fit size in a fast and reasonably accurate manner. The chart must be based on dimensions that are readily known or easily measured by the harness user or vendor. Body weight and stature are well known to individual workers and have fine correlations with harness fit and thus have been widely used as indices by harness manufacturers (Hsiao, Bradtmiller, & Whitestone, 2003). Equations 1 through 4 were simplified to become Equations 5 through 8 in presenting an updated, body-size-based sizing system.
| (5) |
| (6) |
| (7) |
| (8) |
in which Equations 5 and 6 defined the boundary between XSM and STD sizes for men and women, respectively and Equations 7 and 8 defined the boundary between STD and XLG sizes for men and women correspondingly (Figures 3a and 3b; Hsiao, Whitestone, et al., 2009).
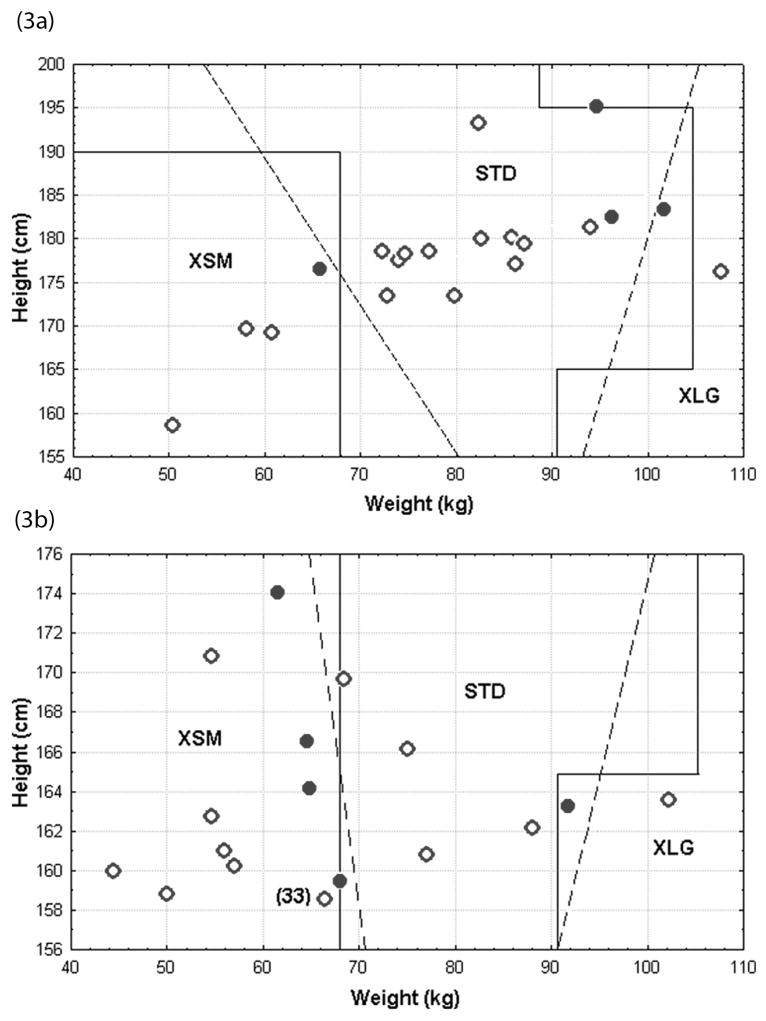
Figure 3.
The distributions of harness size (circles and dots) by weight and height for men (3a) and women (3b), respectively. The solid lines indicate the size boundaries of the current sizing scheme. The dotted lines indicate the updated size boundaries based on Hsiao, Whitestone, and Kau (2007). Each solid dot denotes a participant whose assigned harness size (per the current sizing scheme) is different from the best-fit harness size based on either body shape or body size.
Harness size match level
The sizing charts included in the manufacturer’s product manual are presented in Figures 3a and 3b. Participants were assigned their harness size on the basis of the existing sizing chart. If their assigned size matched the best-fit harness size (based on Equations 1 through 4) and the predicted size by body weight and stature (based on Equations 5 through 8), the individuals had a matched harness size. Otherwise, the harness assigned was an unmatched one.
Sample Size
Body size and shape differences between genders were the most challenging issue for this suspension tolerance study, and their sample size must be determined separately. The number of participants needed for each gender group can be estimated by , where n is the sample size, δ is the eccentricity (1.96 for 5% two-sided probability); σ is the standard deviation of the group, |X̄ − υ| is within-group accuracy; X̄ is the sample mean of the group, and υ is the true mean of the group. A military study of 13 participants on suspension tolerance showed a standard deviation of 8.01 min (Brinkley, 1988). It was expected that the standard deviation for civilians would be larger because of greater variation in body size and shape; 12 min was used in this simple size estimation. With the desired group accuracy of 6 min, the estimated sample size was 16 for each gender. For our research, 17 women (weight, M = 67.3 kg, SD = 15.5; stature, M = 163.6 cm, SD = 4.5) and 20 men (weight, M = 80.2 kg, SD = 14.7; stature, M = 178.1 cm, SD = 7.8) completed the study.
Apparatus
Traditional anthropometry measurement device
An anthropometer (GPM Instruments, Switzerland) and a digital scale (Mettler-Toledo, Worthington, OH) were used to measure stature and body weight.
Three-dimensional (3-D) body scanner
A Cyberware WB4 3-D full-body scanner (Cyberware, Monterey, CA) was used to register the interface between the harness and participant during normal standing and while suspended (Figure 2). The accuracy of the scanning system was tested to an average error of 2.9 mm, varying from +6 to −6 mm (Hsiao et al., 2003).
Physiology recording system
An automated system (Dinamap Pro 1000V3 monitor, GE, Milwaukee, WI) was used to record HR, electrocardiogram (ECG), pulse oximetry, and BP.
On-screen protractor
A digital screen protractor (Iconico, New York, NY) was used to measure and register the torso angle of suspension and thigh strap angle.
Procedures
After arrival, participants viewed a Web page that described the details of the study and the tasks that they were to perform. Each participant signed an informed consent. The participant was then taken to a dressing room, where he or she changed from street clothes into bicycle shorts and sport bra for women. Then a 3-D full-body scan was performed while the participant was standing to register his or her torso dimensions.
A tower harness (MSA TechnaCurv, Pittsburgh, PA) with an overhead design was then donned for tests. A 3-D full-body scan was performed again while the participant was standing and wearing the harness to register his or her harness-body interface, particularly, the thigh strap angle and the harness static fit. The participant was then suspended with an anchored suspension system. Measurement of suspension time commenced after the participant was raised 5 cm from the floor. All participants were asked to remain motionless, simulating unconsciousness for as long as they could, during suspension tests. They were instructed that they could terminate the suspension at any time without penalty. HR, ECG, and pulse oximetry were continuously monitored, and BP was measured automatically every 2 min at heart level by an automated monitor (Dinamap Pro 1000V3, Milwaukee, WI). A 3-D full-body scan was performed during the 1st min of suspension and every 5 min thereafter for up to eight scans.
The study was approved by the National Institute for Occupational Safety and Health (NIOSH) Institutional Review Board; as a safety measure, a medical practitioner was in the laboratory with the participants during the study, and an emergency medical service unit with an ambulance was at standby mode outside of the laboratory building. Tests were terminated for medical criteria or participant discomfort.
Data Analyses
Pearson correlations of suspension tolerance time with body weight, stature, angle of suspension, upper- and lower-torso depths, and thigh strap angle were calculated. Mean suspension tolerance time comparisons were also calculated by gender, harness static fit, suspension fit, and harness size match level. Normality of suspension tolerance time was confirmed with the Shapiro-Wilk test (W = 0.983, p = .839); therefore, we conducted estimation of suspension duration for the 5th-percentile worker using the normal distribution approach in lieu of a nonparametric survival function analysis. Finally, a mixed model with repeated measures was performed on torso suspension angles during the time suspended.
RESULTS
Factors Associated With Suspension Tolerance Time
Pearson correlation coefficient analyses show significant negative correlations of suspension tolerance time with body weight (r = −.45, p = .005), stature (r = −.43, p = .007), torso angle of suspension (r = −.43, p = .008), upper-torso depth (r = −.37, p = .02), lower-torso depth (r = −.36, p = .03), and thigh strap angle (r = −.36, p = .03).
Gender Effect
A t test for mean suspension tolerance time comparison by gender revealed no significant difference (p = .261). The mean suspension tolerance time was 27.1 min for men (95% confidence interval [CI] [21.2, 33.0]; n = 20) and 31.6 min for women (95% CI [25.7, 37.6]; n = 17).
Effect of Harness Static Fit
Women had a higher fail rating than men for harness static fit, with 35% versus 15%, respectively. A t test for mean suspension tolerance time comparison by harness static fit showed no significant difference (p = .44) between the pass group (M = 30.1 min; 95% CI [25.1, 35.1]; n = 28) and the fail group (M = 26.4 min; 95% CI [19.1, 33.8]; n = 9).
Torso Angle of Suspension and Suspension Fit
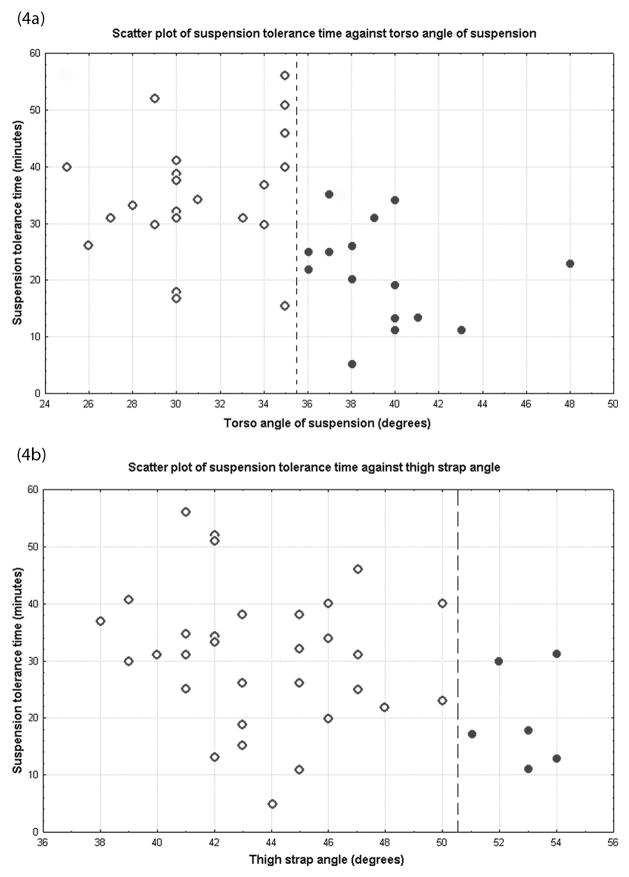
The scatter plot in Figure 4a shows the association between torso angle of suspension and suspension tolerance time. The dashed line indicates a natural point (about 35°), above which the suspension tolerance time decreased consistently. A t test for mean suspension tolerance time comparison by harness suspension fit showed a significant difference between the two levels (p < .001) with mean of 34.9 min (95% CI [30.1, 39.6]; n = 22) for the pass group (angle of suspension ≤ 35°) and 20.9 min (95% CI [15.9, 25.8]; n = 15) for the fail group (angle of suspension > 35°). However, the hypothesis that harness suspension fit would be worse for women than for men was not confirmed; 23.5% of women and 55% of men received a fail rating.
Figure 4.
In 4a, the dashed line indicates a natural point, about 35°, where the data set forms two obvious clusters (i.e., ≤35° and >35° of torso angles of suspension). There is no clear cut point of torso angle of suspension between 25° and 35° at which the suspension tolerance time is clearly dropping. For the angles of suspension greater than 35°, the corresponding suspension tolerance time drops substantially in proportion to the torso angle of suspension. When the two clusters of data are combined, suspension angle still has a correlation with suspension tolerance time (r = −.43, p = .008). In 4b, thigh strap angle has a correlation with suspension tolerance time (r = −.36, p = .03). The dashed line indicates a natural point, about 50°, above which the suspension tolerance time is significantly less than that of the ≤50° cluster.
An F test of the torso angle of suspension measurements over time revealed that there were significant effects of time, F(1, 140) = 18.8, p < .001; gender, F(2, 35) = 1179.1, p < .001; and Time × Gender interaction, F(1, 140) = 4.86, p = .029, on torso angles over time. There was a trend that torso angles of suspension increased slightly during suspension; men had a rate of 0.13° increase per minute and women 0.04° per minute.
Effect of Thigh Strap Angle
Figure 4b affirms that thigh strap angle had an effect on suspension tolerance. The dashed line reveals a natural point (around 50°), above which the suspension tolerance time decreased consistently. A t test for mean suspension tolerance time comparison by thigh strap angles showed a significant difference (p = .041) between the levels above and below 50° with a mean of 31.0 min (95% CI for mean [26.6, 35.4]; n = 31) for the ≤50° group and 20.0 min (95% CI for mean [11.0, 29.0]; n = 6) for the >50° group.
Effect of Harness Size Match Level
Figures 3a and 3b show the distribution of harness size by body weight and stature for men and women, respectively. The solid lines indicate the size boundaries of the current sizing system. The dotted lines indicate the size boundaries based on Equations 5 through 8. Each solid dot in the charts represents a participant whose assigned harness size (per the current sizing system) was different from either the best-fit harness size (based on Equations 1 through 4) or the predicted size by weight and height (based on Equations 5 through 8). In other words, each solid dot denotes an unmatched harness size, and each circle represents a matched size. Participant 33 was excluded from this analysis. She was to be assigned an XSM harness on the basis of her body weight and height; however, the harness size could not fit her for her torso shape. She used an STD harness to complete the study. A t test for mean suspension tolerance time comparison by harness-size match level showed significant difference (p = .03) between the matched and unmatched groups, with a mean of 31.0 min (SD = 11.6; n = 27) for the matched group and 21.2 min (SD = 8.9; n = 9) for the unmatched group.
Rescue Time
Of the 37 tests, 81% were terminated because of medical criteria; 19% (4 men and 3 women) were terminated for discomfort. With a normal distribution of the suspension tolerance data, the mean and standard deviation of suspension tolerance time were 29.2 and 12.1 min, respectively. To ensure that no more than 5% of workers would experience suspension trauma, a 9-min rescue plan was to take place, which was estimated by T0.05 = M − (1.645*SD) = 29.2 − 1.645*12.1 = 9.26 min.
DISCUSSION
Factors Associated With Suspension Tolerance Time
The study results show that body weight, stature, torso angle of suspension, thigh strap angles, upper- and lower-torso depths, and harness-size match level all had a correlation with suspension tolerance time, whereas gender and harness static fit did not demonstrate a similar association. Literature has reported that body weight (Turner et al., 2008) and stature (Seddon, 2002) were predictors for suspension tolerance. This study contributes additional information to the literature on the impact of harness fit on suspension tolerance.
An absence of gender difference in the ability to tolerate harness suspension has been reported elsewhere (Weber & Michels-Brendel, 1990); however, the true effect cannot be determined in that there were only 3 and 4 women in the two tests of the Weber and Michels-Brendel (1990) study. In the current study, the larger sample size (17 women) allowed us to examine further the gender effect. A challenge, however, remains. This was not a matched-pairs study; 71% of the female participants weighed less than 70 kg, whereas 80% of male participants weighed more than 70 kg. With the confounding between gender and body weight, the insignificant effect of gender (p = .26) cannot be confidently confirmed.
Effect of Static Fit
In general, static harness fit was worse for women than for men in the current study, a finding that supports previous research (Hsiao et al., 2003). However, static harness fit did not relate to the ability to tolerate harness suspension. Harness users need to be aware that a static fit does not guarantee that a person suspended motionless in such a harness will be exempt from suspension trauma.
Suspension Fit
Participants with a suspension angle >35° had shorter suspension tolerance time than those with smaller suspension angles, an outcome that supports the ANSI Z359.1 standard and CAN/CSA-Z259.1006 standard of 30° or less but not the ISO 10333-1 and EN 361standards of 45° ~ 50°. In a critical review, Seddon (2002) was puzzled by why the maximum angle requirements were set differently in different standards. From the aspect of biomechanics, increased torso suspension angle will increase the moment arm for compressive force at the low back, which in turn can cause back discomfort and increased consumption of oxygen and thus poses an increased risk for suspension trauma. When the torso suspension angle is greater than 30°, the imposed force by the weight of the upper body will increase dramatically, according to the basic trigonometric concept. The study result supports this concept. The harness industry and fall protection professional community may want to consider setting body harness interface suspension testing criteria, using a series of anthropometric manikins during suspension, in lieu of relying on traditional static-fit testing criteria to provide an increased protection against suspension trauma.
Although the increase of torso angles of suspension across the duration of suspension was statistically significant at 0.13° per minute for men and 0.04° for women, the practical implication of these amounts is limited. Given that a rescue plan is suggested to occur in 9 min, the change in angle of suspension would be in the 1° range. The harness industry could use the torso angle of suspension at the beginning of the suspension test as the testing criterion for economic reason without losing its representation of real-world situations.
Effect of Thigh Strap Angle
Literature has shown that a greater thigh strap angle from the sagittal plane was correlated with an increase in torso suspension angle, hence in fit failure (Hsiao et al., 2007). This study has further demonstrated that both thigh strap angle and torso suspension angle were a precursor to suspension trauma; thigh strap angle may be considered as a fit criterion to possibly minimize the need for a suspension test to predict angle of suspension and suspension tolerance if so desired.
Effect of Harness Match Level
This study showed that participants who used a size of harness that was different from the best-fit size had shorter suspension tolerance times. Previous studies have reported that large changes have taken place in the past decades in body dimensions among the U.S. civilian population (U.S. Department of Health and Human Services, 2001), and NIOSH researchers have proposed updated harness sizing systems for the harness industry and fall protection community to use (Hsiao, Friess, et al., 2009; Hsiao, Whitestone, et al., 2009). This study not only confirmed the effect of harness fit on suspension tolerance, as suggested by Noel et al. (1991), but also demonstrated the need to update the existing harness sizing scheme, as proposed by Hsiao, Whitestone, et al. (2009) to improve protection for harness users.
Workers, whose combination of body weight and stature is at the proximity of the size boundaries of the sizing scheme, particularly need to try on more than one size to select the best-fit size, because the overall combination of their body dimensions (i.e., body shape) governs the size; some of their body dimensions may fall on different sides of the boundaries. When purchasing a safety harness, clearly, the harness should be tested while suspended under supervision so that one can find a model that fits well the shape of his or her body, thus delaying the onset of suspension trauma (Bariod & Théry, 1997, as cited in Seddon, 2002).
Tolerance Time and Rescue Time
In this study, the suspension tolerance time was measured to be an average of 29.1 min but evidenced large variability (SD = 12.1 min, N = 37) with a minimum to maximum of 5 to 56 min. Literature has shown some variant results regarding tolerance time for the use of similar full-body harnesses: from 8 to 45 min (N = 5; Noel et al., 1991), from 6 to 37 min (N = 2; Bariod & Théry, 1997), and from 5.1 to 30.1 min (M = 14.38, N = 13; Brinkley, 1988). Small sample sizes may explain some of the variation. Literature also has reported differences in suspension tolerance time for different styles of full-body harness, with an average of 17.1 min (minimum to maximum of 3.5 to 32) to 28.4 min (minimum to maximum of 10.2 to 49.8) for four harness types (N = 10; Brinkley, 1988), as well as median suspension durations of 20 to 27 min for five styles of harness (N = 15; Weber & Michels-Brendel, 1990). In any case, suspension tolerance varies considerably from person to person.
With a comparatively larger sample size (N = 37), this study has provided further data for estimating the time constraints in rescuing a worker suspended after a fall. It is worth noting that the range of the participant body weight in this study was 50 kg to 107.6 kg, although the harnesses have been designed to accommodate 50 kg to 141 kg. Given that body weight has a negative correlation with suspension tolerance time, harness users with the body weight greater than 107.6 kg may experience even shorter suspension tolerance time than that reported in this study.
CONCLUSION
Body weight, stature, and upper- and lower-torso depths were negatively correlated with suspension tolerance time. Harness suspension angle, thigh strap angle, and harness-size match level were precursors for suspension tolerance, whereas static fit on harness ring locations were not. Selecting well-fitting harnesses and establishing a 9-min rescue plan are suggested to ensure that no more than 5% of workers would experience suspension trauma. This short rescue time is challenging. A self-deployable suspension trauma relief accessory or mechanism that can be integrated into harness design to further harness-user protection would be useful. The study results provide a basis for harness designers, standards writers, and manufacturers to improve harness configurations and testing requirements for better worker protection against suspension trauma.
KEY POINTS.
Body characteristics (i.e., weight, stature, upper- and lower-torso depths) were associated with decreased suspension tolerance time.
Harness fit affected suspension tolerance; a torso angle of suspension greater than 35°, a thigh strap angle greater than 50°, or a poorly fitting harness size were associated with shorter suspension tolerance times.
Safety harnesses need to be tested while the user is suspended in lieu of the traditional static fit evaluation to provide an increased protection against suspension trauma.
Selecting well-fitting harnesses and establishing a 9-min rescue plan are suggested to ensure that no more than 5% of workers would experience suspension trauma symptoms. The allowable rescue time is short for suspension trauma control. A self-deployable suspension trauma relief accessory or mechanism that is integrated into harness design to further harness user protection would be useful.
Acknowledgments
We extend our special appreciation to Tsui-Ying Kau of the University of Michigan for her statistical consultation of the study.
Biographies
Hongwei Hsiao is a distinguished consultant and chief of the Protective Technology Branch at the National Institute for Occupational Safety and Health, and an adjunct professor at West Virginia University in Morgantown, West Virginia. He received his PhD in industrial engineering from the University of Michigan, in Ann Arbor, in 1990.
Nina Turner is the lead physiologist in the Technology Research Branch, National Personal Protective Technology Laboratory, at the National Institute for Occupational Safety and Health in Pittsburgh, Pennsylvania. She received her PhD in physiology from Penn State in University Park, Pennsylvania, in 1991.
Richard Whisler is an information technology specialist in the Technology Development Team of the Protective Technology Branch at the National Institute for Occupational Safety and Health. He received two associate degrees, one in computer information management from the Computer Tech in 1993 and the other in computer animation and multimedia from the Art Institute of Pittsburgh in 1995.
Joyce Zwiener is a health scientist in the Protective Technology Branch at the National Institute for Occupational Safety and Health. She received her MS in occupational hygiene and occupational safety from the College of Engineering and Mineral Resources of West Virginia University, in Morgantown, in 1999.
Footnotes
The authors of this article are U.S. government employees and created the article within the scope of their employment. As a work of the U.S. federal government, the content of the article is in the public domain.
The findings and conclusions in this article are those of the authors and do not necessarily represent the views of the National Institute for Occupational Safety and Health (NIOSH). Mention of company names or products does not imply an endorsement from NIOSH.
References
- American Society of Safety Engineers. American National Standard Institute ANSI Z359.1-1992: Safety requirements for personal fall arrest systems, subsystems and components. Des Plaines, IL: Author; 1992. [Google Scholar]
- Bariod J, Théry B. Medizinische Auswirkungen des Hängens in Sicherheitsgurten [The medical effects of being suspended in safety harnesses], BG Magazine. Norwich, UK: Her Majesty’s Stationery Office; 1997. French to German translation then German to English translation by HSE Language Services, trans no. 16366(A). Cited from Seddon, P. (2002). Harness suspension: Review and evaluation of existing information (Health and Safety Executive Contract Research Report 451/2002) [Google Scholar]
- Brinkley JW. Experimental studies of fall protection equipment. Proceedings of the 1st International Fall Protection Symposium; Toronto, Canada: International Society for Fall Protection; 1988. pp. 51–65. [Google Scholar]
- Canadian Standards Association. Canadian Standard CAN/ CSA-Z259.10-06: Full body harnesses. Etobicoke, Canada: Author; 2006. [Google Scholar]
- European Committee for Standardization. European Standard EN 361: 1992: Personal protective equipment against falls from a height—Full body harnesses. Brussels, Belgium: Author; [Google Scholar]
- Hsiao H, Bradtmiller B, Whitestone J. Sizing and fit of fall-protection harnesses. Ergonomics. 2003;46:1233–1258. doi: 10.1080/00140130310001593595. [DOI] [PubMed] [Google Scholar]
- Hsiao H, Friess M, Bradtmiller B, Rohlf J. Development of sizing structure for fall arrest harness design. Ergonomics. 2009;52:1128–1143. doi: 10.1080/00140130902919105. [DOI] [PubMed] [Google Scholar]
- Hsiao H, Whitestone J, Kau T. Evaluation of fall-arrest harness sizing schemes. Human Factors. 2007;49:447–464. doi: 10.1518/001872007X200094. [DOI] [PubMed] [Google Scholar]
- Hsiao H, Whitestone J, Taylor S, Godby M, Guan J. Harness sizing and strap adjustment configurations. Human Factors. 2009;51:497–518. doi: 10.1177/0018720809346320. [DOI] [PubMed] [Google Scholar]
- International Standards Organization. ISO 10333-1: 2000 + AMD 1, 2002: Personal fall-arrest systems. Part 1: Full-body harnesses. Geneva, Switzerland: Author; 2002. [Google Scholar]
- Noel G, Ardouin MG, Archer P, Amphoux M, Sevin A. Some aspects of fall protection equipment employed in construction and public works industries. In: Sulowski AC, editor. Fundamentals of fall protection. Toronto, Canada: International Society for Fall Protection; 1991. pp. 1–32. [Google Scholar]
- Robertson D. The pathophysiology and diagnosis of orthostatic hypotension. Clinical Autonomic Research. 2008;18(Suppl 1):2–7. doi: 10.1007/s10286-007-1004-0. [DOI] [PubMed] [Google Scholar]
- Seddon P. Harness suspension: Review and evaluation of existing information (Health and Safety Executive Contract Research Report 451/2002) Norwich, UK: Her Majesty’s Stationery Office; 2002. [Google Scholar]
- Shannon J, Flattem N, Jordan J, Jacob G, Black B, Biaggioni I, Blakely R, Robertson D. Orthostatic intolerance and tachycardia associated with norepinephrine-transporter deficiency. New England Journal of Medicine. 2000;342:541–549. doi: 10.1056/NEJM200002243420803. [DOI] [PubMed] [Google Scholar]
- Streeten D. Orthostatic disorders of the circulation: Mechanisms, manifestations, and treatment. New York, NY: PlenumMedical Book Company; 1987. [Google Scholar]
- Turner N, Wassell J, Whisler R, Zwiener J. Suspension tolerance in a full-body safety harness, and a prototype harness accessory. Journal of Occupational and Environmental Hygiene. 2008;5:227–231. doi: 10.1080/15459620801894386. [DOI] [PubMed] [Google Scholar]
- U.S. Department of Health and Human Services. The surgeon general’s call to action to prevent and decrease overweight and obesity. Washington, DC: U.S. Government Printing Office; 2001. [Google Scholar]
- U.S. Department of Labor. Safety and health regulations for construction, Subpart M: Fall protection, 1926.502(d) Washington, DC: Author; 2011. Retrieved from http://www.osha.gov/pls/oshaweb/owadisp.show_document?p_table=STANDARDS&p_id=10758. [Google Scholar]
- Weber P, Michels-Brendel G. Physiologiebeanspruchungenbeimhägen in Auffanggurten [Physiological limits of suspension in harnesses] Frankfurt, Germany: Johann Wolfgang Goethe University; 1990. [Google Scholar]