Shape of chondrocytes within articular cartilage affects the solid but not the fluid microenvironment under unconfined compression

Abstract

Metabolic activity of the chondrocytes in articular cartilage is strongly related to their zone-specific shape and the composition and mechanical properties of their surrounding extracellular matrix (ECM). However the mechanisms by which cell shape influences the response of the ECM microenvironment to mechanical loading is yet to be elucidated. This relationship was studied using a biphasic multiscale finite element model of different shaped chondrocytes in the superficial and deep zones of the ECM during unconfined stress relaxation. For chondrocytes in the superficial zone, increasing the cell’s initial aspect ratio (length/height) increased the deformation and solid stresses of the chondrocyte and pericellular matrix (PCM) during the loading phase; for chondrocytes in the deep zone the effect of the cell shape on the solid microenvironment was time and variable dependent. However, for superficial and deep zone chondrocytes the cell shape did not affect the fluid pressure and fluid shear stress. These results suggest that mechanotransduction of chondrocytes in articular cartilage may be regulated through the solid phase rather than the fluid phase, and that high stresses and deformations in the solid microenvironment in the superficial zone may be essential for the zone-specific biosynthetic activity of the chondrocyte. The biphasic multiscale computational analysis suggests that maintaining the cell shape is critical for regulating the microenvironment and metabolic activity of the chondrocyte in tissue engineering constructs.

Graphical Abstract

1 Introduction

Articular cartilage is a low-friction, load-bearing material covering the surfaces of diarthrodial joints. Mature cartilage has a zone-dependent inhomogeneous composition: collagen fibers are oriented parallel to the cartilage surface in the superficial zone (SZ), become more randomly oriented in the middle zone (MZ), and align perpendicular to the subchondral bone in the deep zone (DZ) [1, 2]. Chondrocytes are the only cell type in articular cartilage and are responsible for the homeostasis of the extracellular matrix (ECM), including the synthesis of type II collagen, proteoglycan and other proteins [3]. Chondrocytes also exhibit zonal shape dependency: flattened or disc-shaped cells aligned with the articular surface in the SZ, spherical shaped and randomly spaced in the MZ, and somewhat elongated cells arranged in columns aligned perpendicular to the subchondral bone in the DZ [4]. Mechanical stimuli modulate the metabolic response of the chondrocytes [5–9], which is influenced by the local profile of interstitial fluid flow and matrix deformation [10–12] and thus their location within the different zones [13].

Chondrocyte mechanotransduction, the mechanism by which chondrocytes convert mechanical signals into biochemical responses [14], involves the integration and transduction of multiple biophysical signals, such as solid stresses and strains, fluid pressurization, fluid shear stress, and osmotic pressure [15]. Articular cartilage responds to mechanical compression by initiating intracellular calcium transients during and immediately following tissue compression [16]. Calcium is a second messenger directly involved in many cellular processes, including matrix synthesis, cytoskeletal remodeling, cell hyperpolarization and cell death [17]. The zone-specific metabolic activity and gene expression of chondrocytes is strongly related to chondrocyte shape [18], yet the mechanisms by which chondrocyte shape and function are related to the mechanical response of their ECM microenvironment when subjected to external joint loading is yet to be elucidated.

In an attempt to repair a cartilage defect to its native zonal organization, stratified tissue engineering techniques use zonal chondrocyte subpopulations to generate cartilage constructs with zonal features and biomechanical properties [19–22]. Yet, to date, a relatively small number of in vitro studies have used this technique, and current in vivo treatment strategies do not reflect native zonal differences [23]. Understanding the relationship between cell shape and their microenvironment is important for understanding cell mechanobiology, not only for regulation of cell phenotype in tissue engineered constructs but, as important, for understanding changes in normal chondrocyte function after post-traumatic injury and in the initiation and progression of osteoarthritis.

Because of the difficultly using experimental studies to quantify chondrocyte biomechanical behavior within its ECM, computational models were developed to investigate the biomechanical microenvironment of the chondrocyte [24]. Most computational studies modeled the chondrocytes as spherical in all zones, ignoring the true zonal morphologies [25]. The aim of this study was to investigate how the different shape of chondrocytes in the SZ and DZ would affect the mechanical response of the chondrocytes and their pericellular and extracellular microenvironments when the articular cartilage was loaded in unconfined stress relaxation. The chondrocytes, pericellular matrix (PCM), and ECM were modeled using an inhomogeneous finite-deformation biphasic multiscale finite element analysis. The solid deformation, stress and strain, and the fluid pressure of the chondrocytes and PCM in the SZ and DZ were investigated.

2 Methods

Finite deformation biphasic theory

The current study used the hyperelastic biphasic theory proposed by Holmes and Mow [26]. The governing equations are

$$\nabla ·({\mathrm{\sigma }}^{\mathrm{e}}-\mathrm{p}I)=0$$ (1)

$$\nabla ·({\mathrm{\nu }}^{\mathrm{s}}-\mathrm{k}\nabla \mathrm{p})=0$$ (2)

where p is the fluid pressure, I the identity tensor, ${\mathrm{\nu }}^{\mathrm{s}}=\frac{\text{du}}{\text{dt}}$ the solid phase velocity, k the permeability, and σ^e the effective stress of the solid matrix, defined as

$${\mathrm{\sigma }}^{\mathrm{e}}=\frac{1}{\mathrm{J}}\mathrm{F}·\frac{\partial {\mathrm{\Psi }}^{\mathrm{s}}}{\partial \mathrm{\epsilon }}·{\mathrm{F}}^{\mathrm{T}}$$ (3)

where J is the volume ratio, J= det(F), F the Jacobian determinant of the deformation gradient, and $\epsilon =\frac{1}{2}(\nabla \mathrm{u}+{(\nabla \mathrm{u})}^{\mathrm{T}}+\nabla \mathrm{u}{(\nabla \mathrm{u})}^{\mathrm{T}})$ the Green-Lagrangian strain tensor [27]. The strain energy density function is defined by [26]

$${\mathrm{\Psi }}^s={\alpha }_0\frac{e^{{\alpha }_1(I_1-3)+{\alpha }_2(I_2-3)}}{I_3^{\beta }}$$ (4)

where I₁, I₂, and I₃ are the invariants of the right Cauchy-Green deformation tensor, C = F^TF, α₀, α₁, and α₂ positive material parameters, and the dimensionless nonlinear stiffening coefficient β = α₁+2α₂. The parameters α₀, α₁, and α₂ are related to β, aggregate modulus, H_A, and Poisson’s ratio, ν, by

$${\alpha }_0=\frac{H_A}{\beta },{\alpha }_1=\frac{1-3\nu }{1-\nu }\beta ,{\alpha }_2=\frac{\nu \beta }{1-\nu }$$ (5)

The deformation-dependent permeability [28] is defined by

$$k=k_0{J}^m$$ (6)

where k₀ is the initial permeability in the undeformed state, and m a material parameter. The fluid shear stress [29] is estimated by

$$\tau ={\omega }_{\infty }\sqrt{{\mu }_f/k}$$ (7)

where ω_∞ is the fluid velocity, and μ_f the viscosity of the fluid (1 mPa*s for water).

Biphasic multiscale finite element simulations

The hyperelastic biphasic theory was implemented in COMSOL Multiphysics (Burlington, MA). Solid mechanics in the Structural Mechanics Module and Darcy’s Law in the Earth Science Module were used [30, 31]. User-defined function in COMSOL was used to input the strain energy density function (Eq. 4) [32].

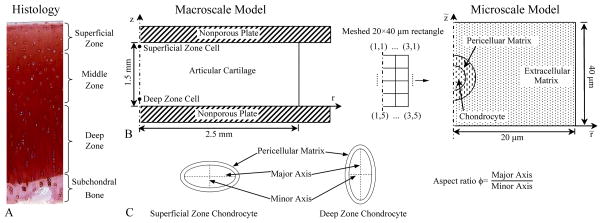
In the biphasic multiscale approach, an axisymmetric macroscale model (Fig. 1, left side) representing articular cartilage in unconfined compression was first analyzed to determine the solid displacement and fluid pressure at each node of the finite element model. The width and thickness of the tissue were 2.5 mm and 1.5 mm, respectively. Quad meshes with a size of 10×10 μm were used (~ size of chondrocytes). A displacement of 0.075 mm (5% axial compression) was linearly applied in 100 seconds to the top surface of the articular cartilage and thereafter held as constant. The top and bottom boundaries of the cartilage were assumed frictionless, with the top boundary free to slide in the radial direction while a roller boundary condition was applied to the bottom boundary. A free draining boundary condition was applied to the peripheral (vertical) boundary of the macroscale model. The model was solved to 3000 seconds at which time an equilibrium condition was achieved. Thereafter the solid displacement and fluid pressure at each chondrocyte location (i.e. 20×40 μm rectangle) were extracted (Fig. 1, middle).

Fig. 1.

(A) Histology of articular cartilage and subchondral bone. Schematic diagrams of the biphasic multiscale finite element method (B) and chondrocyte shape (C) used in the current study. A superficial zone (SZ) chondrocyte and a deep zone chondrocyte were studied. Aspect ratio of the chondrocyte was defined as the ratio of the major axis to the minor axis. The major axis of the chondrocyte was fixed as 15 μm and was in the radial direction for the SZ chondrocyte and axial direction for the DZ chondrocyte. Pericellular matrix (PCM) had a uniform thickness of 2.5 μm.

Two chondrocytes, one in the superficial zone and one in the deep zone, were studied in detail; their radial and axial (r, z) coordinates (in mm) were (0, 1.44) and (0, 0.15), respectively. Since gradients of solid displacement and fluid pressure around a cell at the axisymmetric axis of the macroscale model were less than 0.1% [25], average solid displacement and fluid pressure at the boundaries of the 20×40 μm rectangle were used as boundary conditions for the microscale model (Fig. 1, right side). In the microscale model, the chondrocyte and PCM were modeled as ellipses, with the PCM having a uniform thickness of 2.5 μm and the chondrocyte filling the entire area within the PCM. The initial aspect ratio of the chondrocyte ϕ₀ was defined as the ratio of the major axis to the minor axis. The major axis of the SZ chondrocyte was in the radial direction, while that of the DZ chondrocyte was in the axial direction. The length of major axis for both chondrocytes was fixed as 15 μm. Five aspect ratios, from 1 to 5, were studied for each chondrocyte.

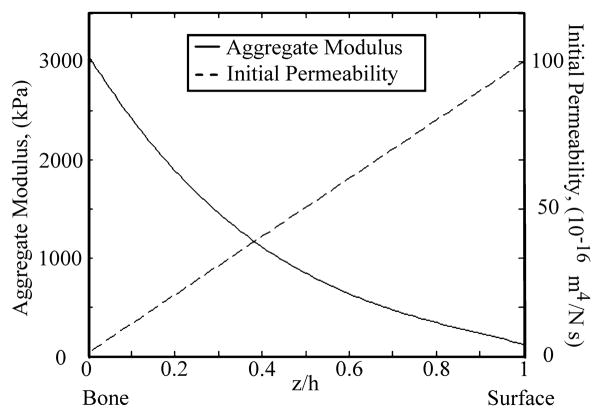
The zone-dependent aggregate modulus for articular cartilage was obtained from published measurements [33–35] (Fig. 2). The initial permeability k₀ was assumed to linearly decrease from 1×10⁻¹⁴ m⁴/Ns at the articular surface to 1×10⁻¹⁶ m⁴/Ns at the cartilage-bone interface (Fig. 2) [25]. In the microscale model, the aggregate modulus and initial permeability of the ECM were based on the zonal location. Material properties for the chondrocyte and PCM were obtained from pervious measurements (Table 1) [36–40] while values for β and m were assumed to be the same as those of the articular cartilage since they have not been previously determined.

Fig. 2.

Zone-dependent aggregate modulus [33–35] and initial permeability of the articular cartilage used in the present study. The initial permeability k₀ was assumed to linearly decrease from 1×10⁻¹⁴ m⁴/Ns at the articular surface to 1×10⁻¹⁶ m⁴/Ns at the cartilage-bone interface [25].

Table 1.

Material properties of the biphasic multiscale models

	Aggregate modulus (kPa)		Poisson’s ratio ν	β	Initial permeability k0 (m4/Ns)	m
	superficial	deep
Chondrocyte	0.42 [36]	0.24 [36]	0.38 [37]	0.35	5×10−15 [38]	2.2
PCM	40 [39, 40]		0.04 [40]	0.35	4.19×10−17 [40]	2.2
ECM/Cartilage	see Fig. 2		0.1 [34]	0.35 [34]	see Fig. 2	2.2 [34]

Computational results that were investigated as functions of time were: normalized aspect ratio (ϕ/ϕ₀) of the chondrocyte; volume deformation (i.e. percent of volume change), average effective axial stress, average effective radial stress, average axial strain, average radial strain, average fluid pressure, and fluid shear stress of the chondrocyte and PCM; distributions of stresses and strains.

3 Results

3.1 Aspect ratio and volume deformation

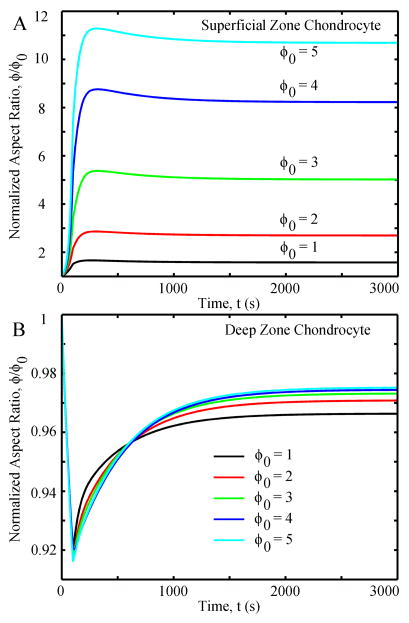
In the first 300 seconds, the SZ chondrocyte’s aspect ratio ϕ rapidly increased for all cases, thereafter slowly decreasing by a small amount until reaching equilibrium at 3,000 seconds (Fig. 3A). With increasing initial aspect ratios ϕ₀ from 1 to 5, the normalized aspect ratios ϕ/ϕ₀ increased from ~1.5 to ~11, respectively. For the DZ chondrocyte, all aspect ratios rapidly decreased in the first 100 seconds and then slowly increased until equilibrium (Fig. 3B). The change in the equilibrium normalized aspect ratios were relatively small (<8%) and similar (< 5% difference) in all the cases.

Fig. 3.

Normalized aspect ratio as functions of time in different cases: (A) superficial zone chondrocyte and (B) deep zone chondrocyte. ϕ₀ is the initial aspect ratio of the chondrocyte.

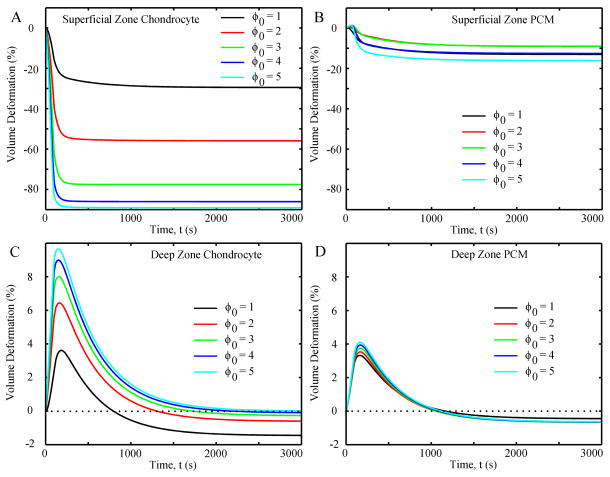
Volume deformation of the SZ chondrocyte for all ϕ₀ values rapidly decreased in the first 100 seconds (~ −25% to −85% for ϕ₀ = 1 to 5, respectively), with small decreases thereafter (~−30% to −90% at equilibrium) (Fig. 4A). For the DZ chondrocyte (Fig. 4C), the volume rapidly increased in the first 100 seconds (~ +4% to +10%) and then rapidly decreased until equilibrium, where all volumes were slightly less than their initial values. The PCM in the SZ and DZ had much smaller changes in volume deformation than the chondrocytes (Fig. 4B and D), both of which were little affected by changes in the initial aspect ratio.

Fig. 4.

Volume deformation (i.e. percent of volume change) of chondrocytes and PCM as functions of time in different cases: (A) superficial zone chondrocyte, (B) superficial zone PCM, (C) deep zone chondrocyte, and (D) deep zone PCM. Negative value means volume decrease and positive value means volume increase.

3.2 Stresses and strains

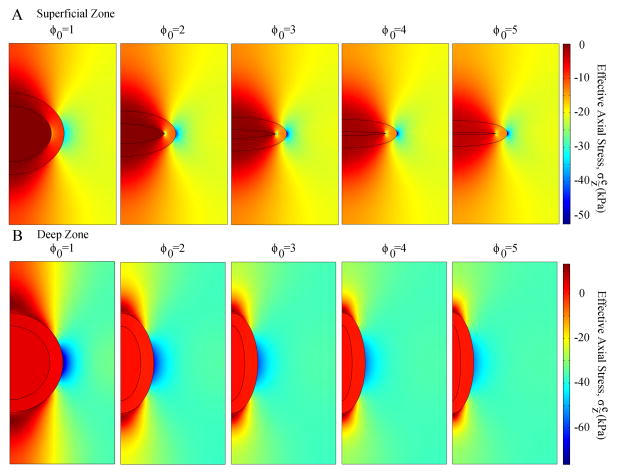
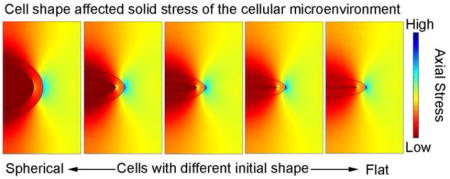
Microscale models for the SZ and DZ chondrocytes with different aspect ratios had similar stress distributions but different peak values (effective axial stresses at t=100 seconds are shown in Fig. 5). High effective axial stresses were found in the ECM adjacent to the radial apex of the PCM, and low effective axial stresses adjacent to the two vertical apexes of the PCM. Peak effective axial stresses in the SZ chondrocyte increased with the initial aspect ratio, while those of the DZ chondrocyte decreased.

Fig. 5.

Distributions of effective axial stress in the microscale model of different cases at t=100 seconds (end of the ramp displacement or peak ramp): (A) superficial zone and (B) deep zone.

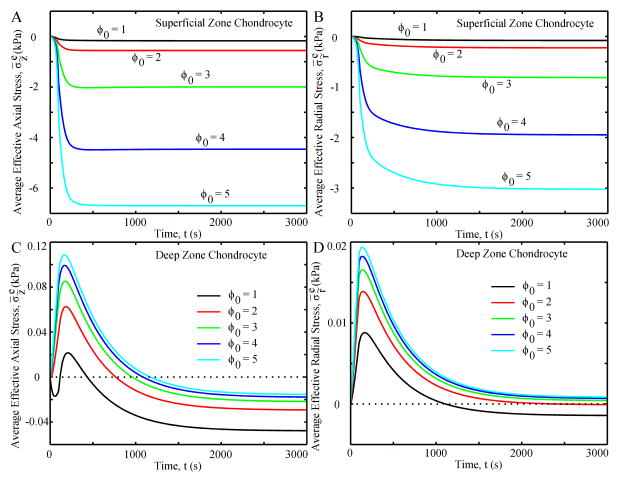
Average effective axial and radial stresses for the SZ chondrocyte were always compressive and showed creep behaviors, rapidly increasing in the first 300 seconds and then slowly increasing until equilibrium (Fig. 6A and B, respectively). As the initial aspect ratio increased, so did the stresses of the SZ chondrocyte. For the DZ chondrocyte with ϕ₀ = 1, the average effective axial stress changed from compression to tension and back to compression in the first 300 seconds (Fig. 6C), while chondrocytes with ϕ₀>1 went from tensile to compressive axial stress with the transition at ~ 800–1,000 seconds. Increasing initial aspect ratio resulted in greater tensile axial stresses and lower compressive axial stresses. In all cases, the average effective radial stress increased to a positive peak at ~150 seconds then decreased until equilibrium. At equilibrium, DZ chondrocytes with ϕ₀ = 1 and 2 had a compressive radial stress while the other chondrocytes had a tensile radial stress (Fig 6D).

Fig. 6.

Average effective axial stress (A and C) and average effective radial stress (B and D) of the chondrocyte as functions of time.

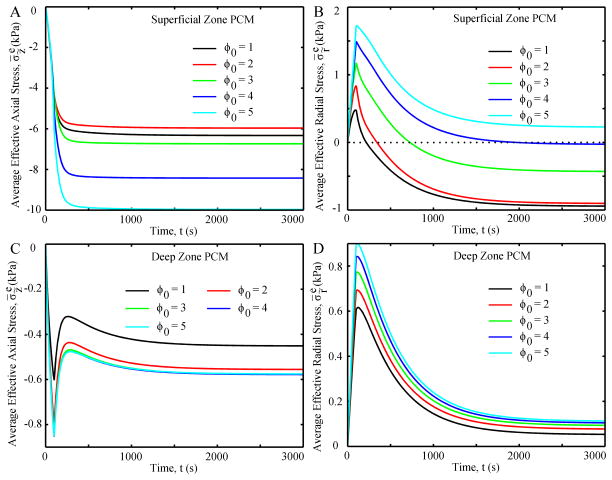
The PCM in the SZ and DZ always had greater stresses than their corresponding chondrocytes (Fig. 6 and 7). Average effective axial stress of the PCM in the SZ had a similar variation pattern as that in the SZ chondrocyte, while the difference in the average effective axial stresses between the PCM and chondrocyte decreased with initial aspect ratio (Fig. 6A and 7A). The average radial stresses in the PCM in the SZ increased to a positive peak at 100 seconds and then decreased to negative values at equilibrium, except for the case with an initial value of 5, which had a positive value at equilibrium (Fig. 7B). Average effective axial stresses in the PCM in the DZ were always compressive, with a peak and rebound in the first 300 seconds followed by a small increase to equilibrium (Fig. 7C). Average effective radial stresses in the PCM in the DZ were tensile in all cases and increased with initial aspect ratio (Fig. 7D).

Fig. 7.

Average effective axial stress (A and C) and average effective radial stress (B and D) of the PCM as functions of time.

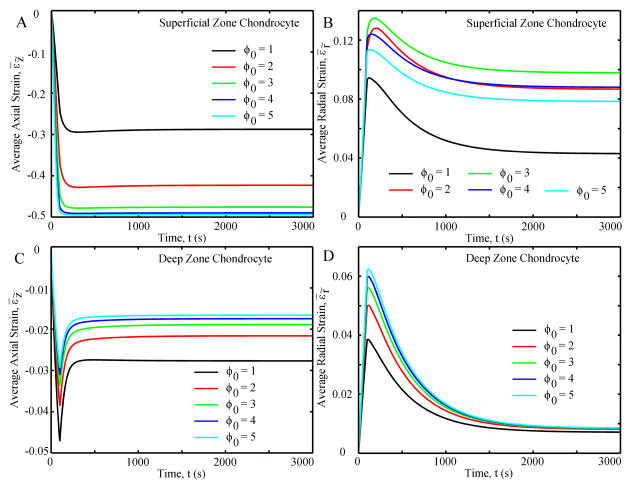
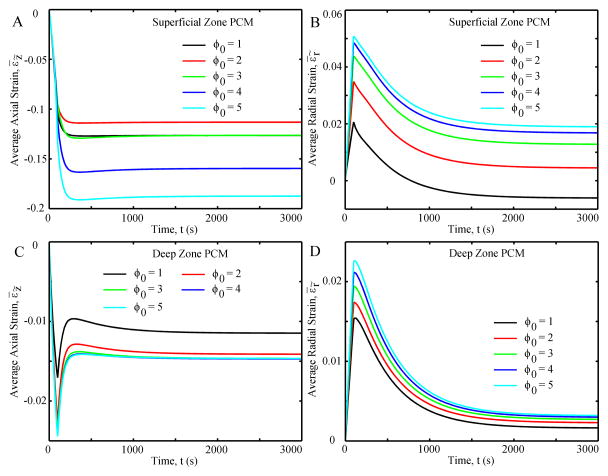
For all SZ chondrocytes (Fig. 8A), the average axial strains were compressive, increasing to ~300 seconds and remaining constant thereafter; the magnitude of the strains increased with initial aspect ratio. The average radial strains were tensile, increasing to a positive peak at ~100 seconds and then decreasing to equilibrium (Fig. 8B). The chondrocyte with ϕ₀ = 1 had the lowest radial strain while that with ϕ₀ = 3 had the greatest radial strain. For the DZ chondrocyte (Fig. 8C), the average axial strain was compressive and increased to ~100 seconds, then decreased until equilibrium (~300 seconds). The greater ϕ₀, the lower the average axial strain. Finally, the average radial strain was tensile, increasing to ~100 seconds and then decreasing to equilibrium (Fig. 8D).

Fig. 8.

Average axial strain (A and C) and average radial strain (B and D) of the chondrocyte as functions of time.

3.3 Fluid pressure and shear stress

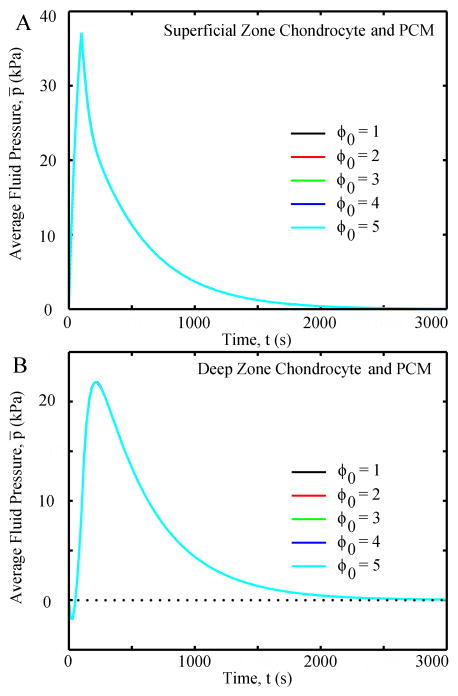
Chondrocytes and PCMs in the same zone had the same average fluid pressure (Fig. 10), and chondrocyte shape did not affect the fluid pressure of the chondrocyte and it’s PCM. For the SZ chondrocyte, fluid pressure increased in the first 100 seconds and then gradually decreased to zero at equilibrium. On the other hand, the DZ chondrocyte had a negative fluid pressure for ~50 seconds, which increased to a positive peak at ~200 seconds and then gradually decreased to zero at equilibrium. In all cases, fluid flux in the chondrocytes and PCMs were very small with peak velocities of ~4 nm/s and peak fluid shear stresses of ~0.02 Pa.

Fig. 10.

Chondrocyte shape did not affect the average fluid pressure of the chondrocyte and PCM: (A) SZ and (B) DZ. Chondrocyte and its PCM had the same average fluid pressure, thus the lines overlap with each other.

4 Discussion

Using a biphasic multiscale finite element analysis, we investigated changes in the mechanical microenvironments of chondrocytes in the superficial and deep zones of articular cartilage, as a function of cell shape, when the ECM was compressed to 5% unconfined compression. We used a multiscale approach by first analyzing an axisymmetric, inhomogeneous, macroscale model of articular cartilage to extract the solid displacements and fluid pressure at each chondrocyte location, and then used these as boundary conditions in a microscale finite element model (Fig, 1) to calculate the volume deformations, stresses, strains and fluid pressures in the chondrocytes and their surrounding PCMs. Independent of cell shape, the chondrocyte and PCM in the SZ had large volumetric decreases, high axial and radial stresses and strains, and high fluid pressures, as compared to the chondrocyte and PCM in the DZ. In almost all cases, the SZ values were an order of magnitude greater (10-fold) than in the DZ. For the SZ chondrocyte, increasing the cell’s aspect ratio from 1 to 5 (spherical to ellipsoidal) increased the volume deformation, normalized aspect ratio and solid stresses and strains of the chondrocyte’s solid microenvironment. The effect of the cell shape on the solid microenvironment of the DZ chondrocyte was more variable with the initial aspect ratio. During the loading phase the volume change and tensile effective axial stress increased with the initial aspect ratio while at equilibrium the volume and compressive effective axial stress decreased with the initial aspect ratio. Normalized aspect ratios of the DZ chondrocyte were similar (< 5% difference) in all the cases. Chondrocytes in the DZ were subjected to tensile and compressive radial and axial stresses and strains depending on the initial aspect ratio. Finally, the shape of the SZ and DZ chondrocytes did not affect the fluid pressures in the chondrocytes and its PCM.

Of interest was that when the ECM was compressed, the volume decrease of the chondrocyte in the SZ increased 3-fold from ~30% for an initial aspect ratio of 1 to ~90% for an initial aspect ratio of 5. Previous studies have shown a strong correlation between volume change and intracellular calcium signaling [41], and that increasing volume deformation increases the intracellular calcium signaling [42]. The large changes in deformation and volume of the chondrocyte with different initial shapes could also produce large differences in membrane expansion and unfolding events, both of which have been associated with calcium signaling and mechanotransduction [42]. The results of the current study suggest a mechanism by which cell shape and function may be related: under compression chondrocytes with larger aspect ratios (ϕ₀>1) had greater deformations and stresses compared to spherical shape chondrocytes (ϕ₀=1), suggesting they may have greater intracellular calcium signaling and activated more stress-sensitive ion channels, both of which could affect the biosynthesis of anabolic and catabolic metabolites by chondrocytes, such as ECM components and degradative enzymes, respectively.

As found by others, the chondrocytes in our study had lower stresses and higher strains compared to their surrounding microenvironments (PCM and ECM) [25, 38, 39, 43, 44]. This is due to the chondrocyte being more compliant than the PCM and ECM. We also found a larger volumetric decrease in the SZ chondrocytes than in their PCM, thus the connections between the chondrocyte’s integrin and PCM (collagen and fibronectin) will be stretched to possibly expose hidden domains of proteins in the cell cytoskeleton and PCM. Previous studies have shown that stretching of fibronectin will unfold the fibronectin type III domain to expose buried binding sites that serve as nucleation sites for the assembly of fibronectin into functional fibrils [45, 46], leading to possible loss of cell signaling. The current study also found that the difference in volumetric change between SZ chondrocytes and their PCM increased with the initial aspect ratio, indicating that PCM proteins connecting to a flat chondrocyte in the SZ are stretched more than PCM proteins connecting to a spherical chondrocyte. These results suggest that the shape of the chondrocyte, especially in the SZ, can affect the mechanical microenvironment of the chondrocyte, leading to alternations of biological functions which are critical for extracellular mechanotransduction [47].

Though the macroscale model was in unconfined stress relaxation, the effective stresses in the SZ chondrocyte exhibited creep behavior (Fig. 6A and B), and the DZ chondrocyte had increased volume and tensile effective axial stresses during the loading phase (Fig. 4B and 6C). These distinctly different behaviors at the tissue (macro) and cell (micro) levels were probably caused by the inhomogeneity of the articular cartilage, where the aggregate modulus increased over 25 times from the surface to the cartilage-bone interface. In the macroscale model, after the compressive displacement was held constant, the middle and deep zones relaxed and compressed the softer (lower aggregate modulus, higher permeability) SZ. These results emphasize that, even under a simple compressive loading condition, the cellular microenvironment can be very different from the tissue macroenvironment behavior, and that the inhomogeneous and nonlinear material properties of the articular cartilage are critical for the biphasic multiscale analysis in the cellular microenvironment.

In unconfined compression, the SZ chondrocyte was subjected to over 5 times greater deformations, stresses and strains compared to the DZ chondrocyte, which is consistent with previous experimental [12, 48] and computational studies [25, 38, 49, 50]. The volume of the SZ chondrocyte always decreased, while that of the DZ chondrocyte increased in the loading phase and decreased at equilibrium. The SZ chondrocyte was always subjected to compressive stresses, while the DZ chondrocyte was subjected to tensile stresses during the loading process and compressive stresses at equilibrium. These differences in the mechanical microenvironments in the superficial and deep zones most likely affect the metabolic activity and gene expression of chondrocytes from the altered mechanical stresses [14]. The high stress microenvironment in the SZ may activate stress-sensitive ion channels at the chondrocyte membrane to modify specific mechanotransduction and biological functions. The tensile stresses and increased volume of the DZ chondrocyte may activate stretch-activated ion channels, which are key factors in the regulation of cytoskeletal function and intracellular mechanotransduction [47].

A unique finding of the current study was that the cell shape did not affect the fluid pressure within the cell and PCM in either zone. Though previous studies have shown that high fluid shear stress and interstitial fluid flow can alter chondrocyte metabolism [9, 10], the fluid shear stresses calculated in this study were over 100-fold smaller than the stress caused by the peak solid deformation, therefore the fluid shear stresses were negligible compared to the solid stresses found from the quasi-static loading condition studied here and by others [25, 51]. The low interstitial fluid flow is caused by the frictional drag effect of the porous-permeable solid matrix [3]. These results suggest that chondrocyte mechanotransduction is regulated through the solid phase rather than the fluid phase.

Using a novel biphasic multiscale analysis, the current study shows the potential mechanism by which cell shape influences the response of the cellular microenvironment to mechanical loading. The findings of the current study are critical not only for understanding mechanotransduction in normal chondrocyte, but, as important, for regulation of cell phenotype in tissue engineered constructs. The findings that cell shape and zonal location affect the cellular microenvironment provide theoretical evidence favoring stratified tissue engineering approaches for cartilage repair. For instance, zonal chondrocyte subpopulations may be needed to recreate the zonal cellular microenvironment to properly guide metabolic activity of the chondrocytes to regenerate cartilage constructs with zonal features and biomechanical properties similar to normal articular cartilages. In future studies, the multiscale modeling approach of the current study can also be used to design new tissue engineering constructs. By changing the material properties of the construct the model is able to mimic the cellular microenvironment within the extracellular matrix, such that a statistically-augmented finite element approach [52, 53] can then be used to systematically investigate the influence of the material properties of the tissue construct on the cellular microenvironment.

In summary, the current study presented a novel biphasic multiscale analysis of the relationship between cell shape and cellular microenvironment, which is critical for understanding cell mechanobiology and regulation of cell phenotype in tissue engineering applications. The shape of the chondrocytes were found to affect the solid but not the fluid microenvironment, which suggests that differences in the deformation of the chondrocyte and its PCM in different zones of the articular cartilage will have unique contributions to mechanobiological health and degeneration of the tissue. Maintaining the cell shape is critical for regulating the microenvironment and metabolic activity of the chondrocyte in native cartilage and tissue engineering constructs.

Fig. 9.

Average axial strain (A and C) and average radial strain (B and D) of the PCM as functions of time.