Kinetics of CO₂ Reduction over Nonstoichiometric Ceria

Abstract

The kinetics of CO₂ reduction over nonstoichimetric ceria, CeO_2−δ, a material of high potential for thermochemical conversion of sunlight to fuel, has been investigated for a wide range of nonstoichiometries (0.02 ≤ δ ≤ 0.25), temperatures (693 ≤ T ≤ 1273 K), and CO₂ concentrations (0.005 ≤ p_CO2 ≤ 0.4 atm). Samples were reduced thermally at 1773 K to probe low nonstoichiometries (δ < 0.05) and chemically at lower temperatures in a H₂ atmosphere to prevent particle sintering and probe the effect of higher nonstoichiometries (δ < 0.25). For extents greater than δ = 0.2, oxidation rates at a given nonstoichiometry are hindered for the duration of the reaction, presumably because of near-order changes, such as lattice compression, as confirmed via Raman Spectroscopy. Importantly, this behavior is reversible and oxidation rates are not affected at lower δ. Following thermal reduction at very low δ, however, oxidation rates are an order of magnitude slower than those of chemically reduced samples, and rates monotonically increase with the initial nonstoichiometry (up to δ = 0.05). This dependence may be attributed to the formation of stable defect complexes formed between oxygen vacancies and polarons. When the same experiments are performed with 10 mol % Gd³⁺ doped ceria, in which defect complexes are less prevalent than in pure ceria, this dependence is not observed.

1. Introduction

Conversion and storage of sunlight into renewable fuels using ceria-based solar thermochemical redox cycles has gained considerable interest in recent years.¹⁻⁸ Ceria and its solid solutions are promising redox materials because of their ability to show high oxygen storage and release capacities,⁹⁻¹¹ fast redox kinetics,¹²⁻¹⁴ single-phase stability at elevated temperatures, and high nonstoichiometries^15,16 and abundant existence in Earth’s ore deposits.¹⁷ Two-step thermochemical redox cycling comprises (1) an endothermic reduction of ceria driven by concentrated solar energy at elevated temperatures (T_red > 1673 K) and low oxygen partial pressures and (2) an exothermic oxidation of reduced ceria by splitting H₂O and CO₂ into H₂ and CO (syngas) at lower temperatures (T < T_red).^14,18−20 If desired, syngas can further be processed to liquid fuels with well-developed catalytic conversion procedures, viz. by Fischer–Tropsch process.²¹

In general, solar-to-fuel energy conversion efficiency relies on the oxygen storage and release capacity, the radiative heat absorptivity, and kinetics of ceria toward CO₂. Various studies have investigated reduction and oxidation kinetics of pure and doped ceria solid solutions.^{5,13,14,22−25} Using the weight relaxation methodology, Ackermann et al.²³ determined ambipolar diffusion coefficients of pure ceria pellets at high temperatures (T < 1773 K). Based on the results, reduction reaction times less than 10 s are estimated for bulk diffusion length scales of 0.4 mm and less. Because of such rapid reaction rates and relatively small characteristic diffusion lengths, reduction within solar reactors is most likely limited by the heating rate rather than chemical kinetics. Such behavior has also been observed experimentally.^3,4 Oxidation rates, in contrast, are shown to be primarily dictated by the chemical kinetics of splitting CO₂ and H₂O. For example, Gopal and Haile¹³ used an electrical conductivity relaxation technique for determination of bulk oxygen diffusivity within Sm-doped ceria and reported 40 times larger surface reaction rate constants for splitting CO₂ compared to H₂O. Chueh et al.¹⁴ examined activation energies and determined the average fuel production rates (H₂O/CO₂ = 2:1) by measuring temporal downstream gas concentrations for nonstoichiometric porous Sm-doped ceria placed in a packed bed reactor. In contrast to Gopal and Haile,¹³ H₂ production rates were generally higher compared to CO production rates, and a lower activation energy for dissociation of H₂O was observed compared to CO₂. Furler et al.⁵ reported an increase in CO₂ splitting rates when surface area is increased by using structures containing micrometer-sized interconnected pores. Similar observations were confirmed by Venstrom et al.²⁵ and Petkovich et al.²⁴ with structures consisting of three-dimensionally ordered and interconnected submicrometer-sized pores. Additionally, Furler et al.⁵ showed decreasing rates with increasing temperature beyond 1000 K because of a strong influence of the backward reaction with product gas CO. Sørensen^26,27 reported changes in oxidation rates and characteristic activation energies with CO₂ for different subphases formed at high reduction extents by using quasi-isothermal thermogravimetry at very low oxygen partial pressures (p_O2 < 10^–10 atm). To date, such phase changes have not been considered for thermochemical fuel conversion applications.

In summary, there is a general consensus that oxidation rates are limited by the specific surface area, whereas reduction rates are typically limited by the rate of heat transfer because of rapid ambipolar diffusion at such elevated temperatures. Despite the promising proof-of-principles in applying the material in a reactor and initial kinetics studies, investigation of the splitting kinetics and associated near-order atomistic changes for the oxide require further attention. Other than the work by Sørensen,^26,27 there is little work that has investigated the effect of nonstoichiometry on oxidation rates, yet this data is critical for the design of solar reactors. Therefore, within the framework of this investigation, we elucidate CO₂ reduction rates over nonstoichiometric ceria for fine powder and sintered pellets, a very broad range of temperatures and CO₂ concentrations, and a large variation of nonstoichiometries. To gain insight into morphology, structure, and chemistry with respect to nonstoichiometry and CO₂ reactivity, scanning electron microscopy (SEM), Raman spectroscopy, and thermogravimetry are utilized.

2. Experimental Section

2.1. Sample Fabrication

Ceria samples with different length scales were used, namely, fine powder (Sigma-Aldrich, <5 μm, 99.9% trace metals basis) and sintered pellets made from the same powder or 10 mol % Gd doped ceria (Sigma-Aldrich, 5–10 nm, Ce_0.9Gd_0.1O_1.95). The pellets have a diameter and thickness of 8.8 mm and 1.5 mm, respectively, and were uniaxially cold pressed at 8 tons. The pellets were sintered for 5 h at 1873 K under atmospheric conditions resulting in a porosity <0.05. The grain size was 20–50 μm and 3–10 μm for the undoped and Gd-doped ceria pellets, respectively. The mass of the ceria pellets and powder samples were ∼600 mg and 25–100 mg, respectively.

2.2. Thermogravimetric Analysis

Isothermal oxidation experiments were conducted using a thermogravimetric analysis instrument (TGA, NETZSCH STA 409 CD). The ceria sample was reduced at elevated temperatures and then cooled at a rate of −20 K/min to the desired oxidation temperature followed by isothermal oxidation with CO₂. Two different methods were applied to reduce ceria: (1) thermal reduction at 1773 K at low oxygen partial pressure ∼10^–4 atm (pellets) and (2) chemical reduction at 1373 K enhanced with p_H2 = 0.02 atm (powder and pellets) to prevent sintering. From the absolute sample mass, m_s, and temporal weight loss, Δm(t), nonstoichiometry, δ(t), was calculated as shown in eq 1.

1

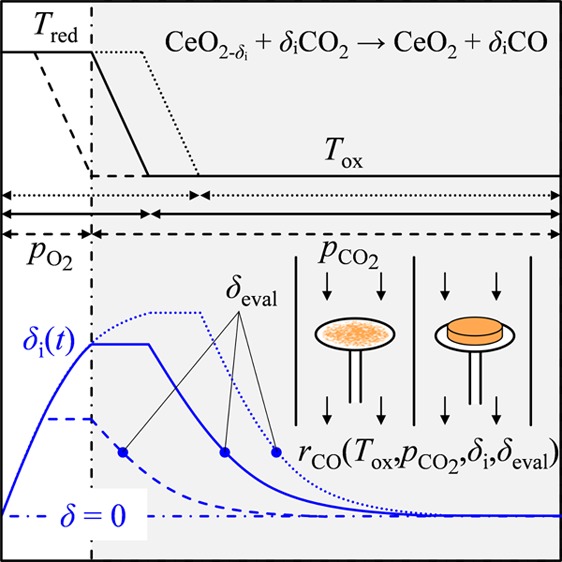
where M_CeO2 and M_O are the molar mass of ceria and oxygen, respectively. The nonstoichiometry right before starting oxidation with CO₂ is denoted as the initial nonstoichiometry, δ_i, as schematically shown in Figure 1. It is changed by varying reduction temperature, reduction duration, and gas flow composition (low p_O2 or p_H2 = 0.02 atm). Rate of CO production was evaluated at constant nonstoichiometry, denoted as δ_eval, which represents an averaged value for the sample according to eq 1, and is necessarily smaller than δ_i (see Figure 1). Buoyancy effects were corrected with blank experiments using equivalent masses of inert zirconium(IV) oxide powder (Sigma-Aldrich, 5 μm, 99% trace metals basis). Additionally, ceria powder oxidation rates were corrected for rate contributions from small oxygen levels present in the system.

Figure 1.

Rate of CO production was evaluated at constant nonstoichiometries, δ_eval, for oxidations that start from different initial nonstoichiometries, δ_i, where δ_i = f(T_red, t, p_O2, p_H2).

2.3. Analytical Analysis

A Hitachi TM-1000 and Zeiss Supra VP55 SEM instrument were used to optically characterize the samples. Raman spectroscopy was performed using a WITec CRM200 confocal Raman microscope. The spectra were generated with an excitation wavelength of 532 nm by taking the average of three repetitions with an integration time of 20 s.

3. Results and Discussion

3.1. TGA: Ceria Powder

Initial oxidation experiments were performed to examine external mass-transfer limitations. All samples described in this section were reduced for 30 min at 1373 K with p_H2 = 0.02 atm in argon at a total volumetric flow rate of 320 mL min^–1, leading to an average δ_i of 0.1. Figure 2 shows δ versus time during oxidation for two different powder masses, 26.8 mg (white triangles) and 54.6 mg (black circles) for different temperatures and CO₂ concentrations. From the visual similarity of the weight relaxation curves, external mass-transfer limitations are excluded at these operation conditions.

Figure 2.

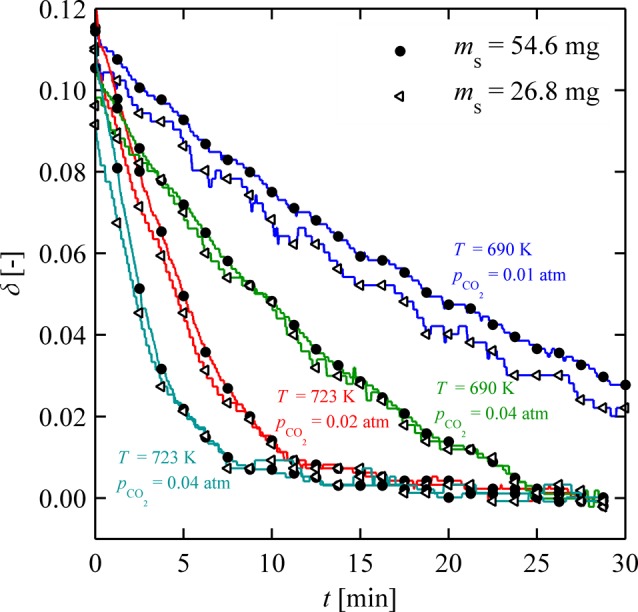
Isothermal weight relaxation curves of ceria oxidation for two different sample masses of 26.8 mg (white triangles) and 54.6 mg (black circles) at different temperatures and CO₂ concentrations.

Figure 3a shows relative mass changes as a function of time during oxidation for various temperatures ranging from 693 to 773 K and a constant p_CO2 (0.02 atm). In general, oxidation rates increase with increasing temperature, and the oxidation time decreases from 25 to 3 min. At higher temperatures where the reaction is more rapid, however, external mass-transfer limitations and/or the onset of the reverse reaction is eventually observed. As seen in Figure 3b for T > 783 K, rates are less dependent on temperature and eventually decrease with increasing temperature. For example, oxidation rates between 793 and 853 K are nearly identical with an oxidation time of ∼2 min. Further kinetic analysis of the powder samples is limited to temperatures below 773 K where these limitations are not observed.

Figure 3.

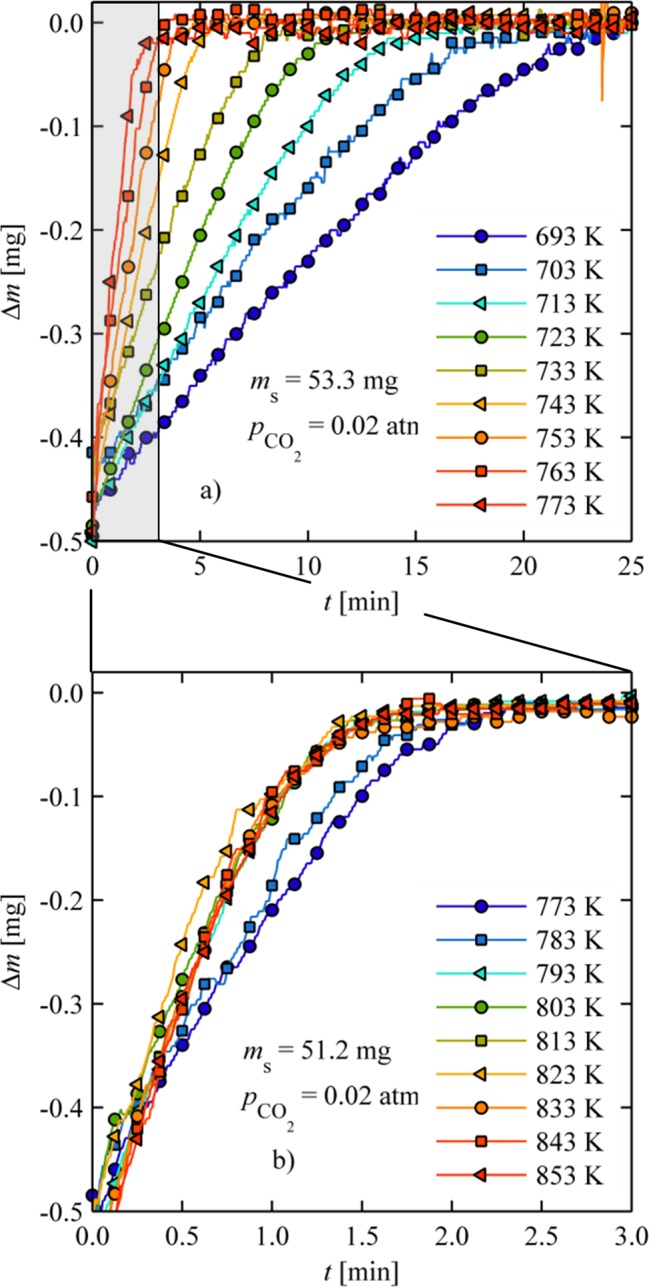
Isothermal oxidation of ceria powder between (a) 693 and 773 K with p_CO2 = 0.02 atm and (b) 793 and 853 K with p_CO2 = 0.02 atm.

Additional experiments were conducted for p_CO2 = 0.005, 0.01, and 0.04 atm, as well as for mixtures of ceria powder and inert zirconia powder with mass loadings (m_CeO2/m_s) of 50%, 75%, or 100% for p_CO2 = 0.02 atm. In Figure 4, the natural logarithm of CO production rates (r_CO) evaluated at δ_eval = 0.04 is shown versus inverse temperature. r_CO increases with increasing temperature and CO₂ concentration as expected (see least-squares fitted trend lines). There is little dependence on the inert particle mass, giving us further confidence that data are not limited by external mass transfer.

Figure 4.

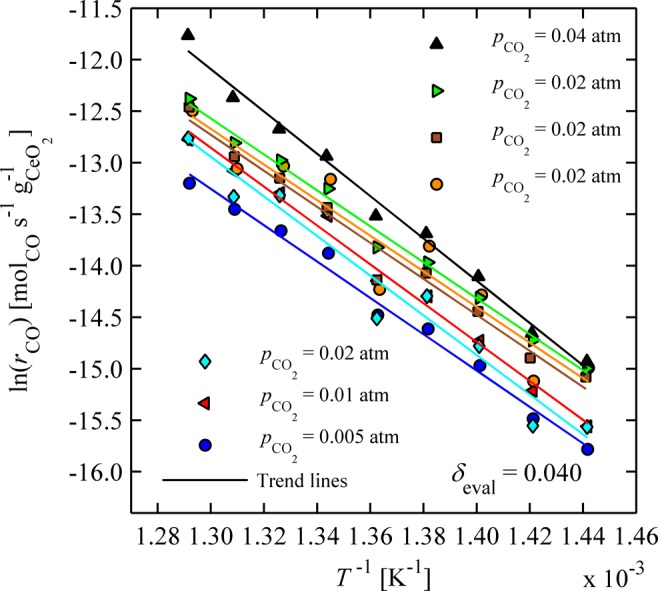
Rates of CO production over oxidizing ceria powder plotted as a function of the inverse temperature for different CO₂ concentrations at a constant nonstoichiometry. Orange circles and blue diamonds are results from ceria powder mixed with inert zirconia powder with a ceria mass loading of 75 and 50%, respectively.

Activation energy, E_A, was extracted using a simplified rate equation for a more detailed analysis of data. The simplified kinetic rate expression for the case of CO₂ reduction over nonstoichiometric ceria, r_CO, was defined as the product of the reaction rate constant, k(T), the reaction mechanism, f(δ), and the CO₂ concentration, C_CO2, with reaction order, n, as shown in eq 2.²⁸

2

The CO₂ concentration is related to the p_CO2 through the following relationship:

3

For constant composition, the slope of the natural logarithm of k·f as a function of inverse temperature yields the activation energy of the reaction. Figure 5a shows natural logarithm of k·f as a function of inverse temperature for several p_CO2 data sets at δ_eval = 0.015 and 0.06 calculated from rate data according to eqs 2 and 3. The data confirms Arrhenius type dependency and shows no dependency on CO₂ concentration for n ≈ 0.45 (numerically determined through a least-squares minimization). It should be noted that the function used to describe the reaction mechanism has no bearing on the determination of activation energy because it is evaluated at constant composition.

Figure 5.

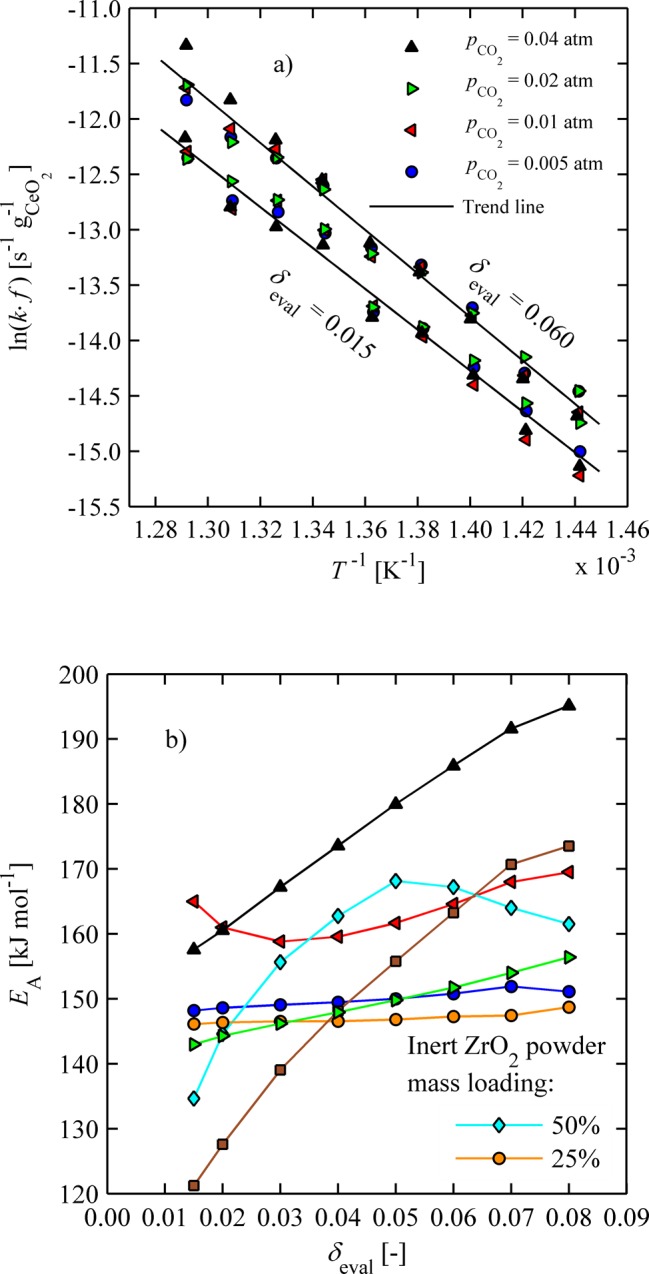
(a) Arrhenius plot of oxidizing ceria powder for several CO₂ concentrations at constant nonstoichiometries; (b) activation energy of oxidizing ceria powder as a function of nonstoichiometry (see Figure 4 for legend).

Figure 5b shows E_A, extracted from the data sets presented in Figure 4, as a function of δ_eval. In general, E_A stays almost constant over all δ_eval for lower p_CO2 values, with a mean value of ∼145 kJ mol^–1. For p_CO2 = 0.04, E_A increases with increasing δ_eval from 155 to 190 kJ mol^–1. One may hypothesize that in this case, where reactions are most rapid, there is insufficient heat dissipation leading to increased activation energy, in accordance with ref (5). In effect, measured temperatures are probably lower than those observed locally. An additional measurement error is related to the small mass changes due to oxidation in comparison to the natural drifts of the system with time. Furler et al.⁵ reported activation energies from 90 up to 136 kJ mol^–1 for pure ceria at δ_eval = 0.015. However, oxidation rates were determined from highly porous structures and may be partly affected by pore diffusion limitations resulting in a lower apparent activation energy.^29,30

3.2. TGA: Ceria Pellet

3.2.1. Chemical Reduction

To investigate oxidation behavior for T > 783 K, isothermal weight relaxation experiments were conducted with dense ceria pellets where oxidation rates were much slower than for the particles. Experimental errors associated with small mass changes during oxidation could be suppressed because substantially larger sample masses were utilized. Initially, pellets were reduced to different nonstoichiometries and subsequently oxidized at different temperatures. Figure 6a shows r_CO (δ_eval = 0.04) in log space as a function of inverse temperature (913 ≤ T ≤ 1053 K, p_CO2 = 0.2 atm, δ_eval = 0.04) for four different initial nonstoichiometries, namely, δ_i = 0.07, 0.154, 0.19, and 0.214. Interestingly, rates were strongly dependent on the initial nonstoichiometry, increasing by orders of magnitude with decreasing δ_i for the lowest temperatures. At higher temperatures the effect was suppressed. Here there is not a linear increase of rates with increasing temperature, as was observed for lower temperatures, presumably because of the onset of the reverse reaction. Figure 6b shows the same data presented in Figure 6a, but as a function of δ_i for varying temperature. Here, the transition in rates at high nonstoichiometries is more easily observed.

Figure 6.

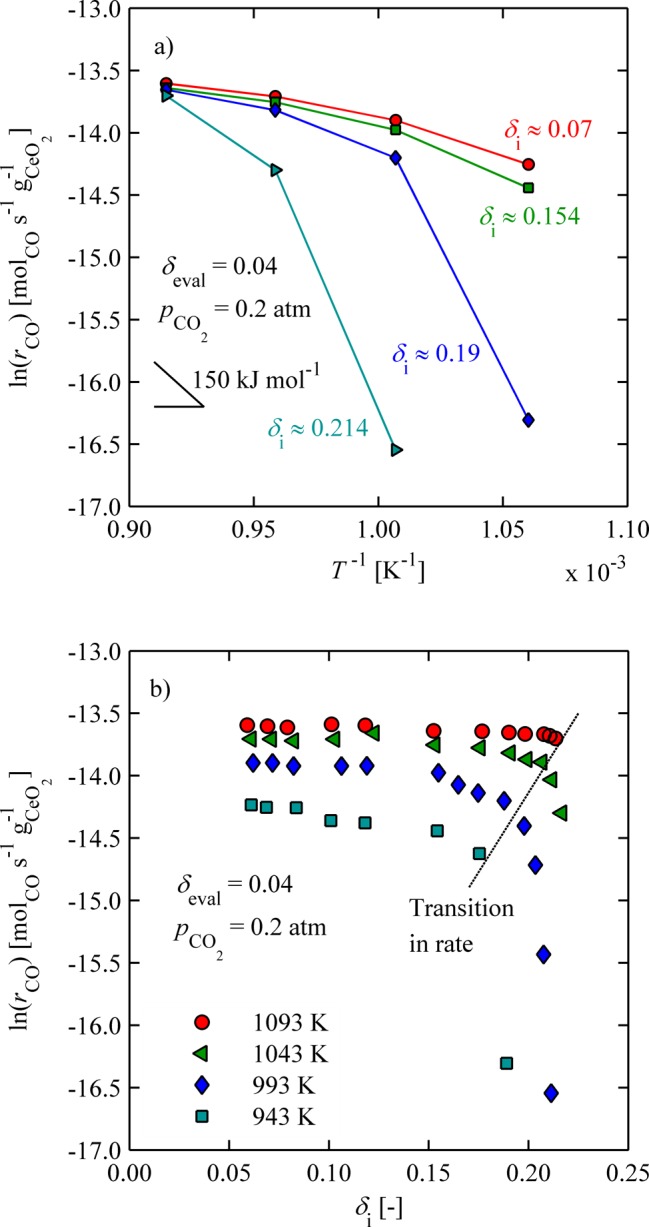
CO production rates for p_CO2 = 0.2 atm evaluated at δ_eval = 0.04: (a) as a function of inverse temperature for several δ_i; (b) as a function of δ_i for several temperatures.

Such behavior may be based on a phase change and is a typical explanation for a sudden transition in a physical property of solid oxides. Normally, ceria is assumed to be a single-phase material for the purpose of thermochemical applications; however, several changes in local near order have been reported.^27,31−33 For example, Bevan and Kordis³¹ used sudden changes in thermodynamic properties of ceria to construct a phase diagram between temperatures of 909 and 1442 K and δ between 0 and 0.5. Subphase changes have also been hypothesized by Sorenson²⁷ to explain sudden changes in the slope of partial Gibbs free energies at high nonstoichiometries. Several ordered intermediate phases were proposed within the ά-phase region, each consisting of its own apparent nonstoichiometric single phase. In fact, the rate transition region shown in Figure 6b is found to be very close to a phase boundary, as shown in Figure 7. Here, the phase diagram of CeO_2−δ, determined from specific heat measurements reported by Riess and co-workers³² with y = 2 – δ, is plotted alongside colored dots representing the transition region extracted from Figure 6b at 943, 993, 1043, and 1093 K. Each point is shifted slightly to lower δ_i, yet parallel to a phase boundary. One explanation for a shift toward lower δ compared to the boundary might be because the samples were not fully equilibrated. Thus, the surface where the reaction with CO₂ takes place might be reduced to nonstoichiometries above the phase boundary, whereas some of the bulk remains below. We do not believe the hindered rates are due to carbonate formation because the mass balance between released O₂ and produced CO is shown to be closed in this work and under similar reaction conditions in other works.^4,5 Additionally, Li et al.³⁴ showed with Fourier transform infrared spectroscopy that carbonates thermally desorb for T > 773 K. Furthermore, we saw no evidence of carbonate formation from unreasonable mass changes observed during oxidation.

Figure 7.

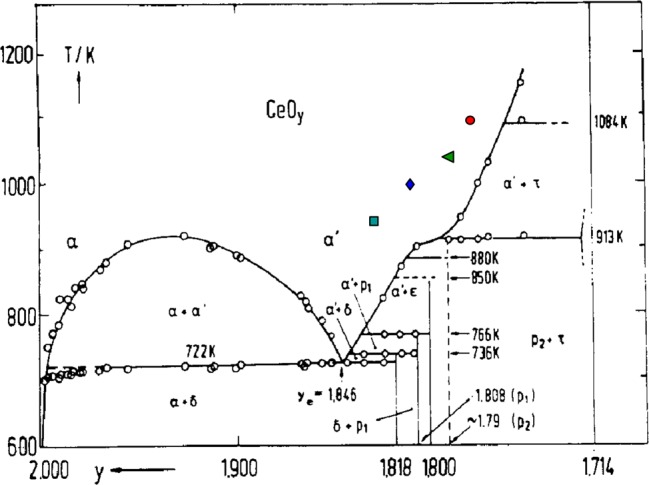
Phase diagram of pure CeO₂ determined from specific heat measurements.^32,33 Colored points represent initial nonstoichiometries of pellets where transition for drastic decrease in CO production rate is observed with TGA measurements (see Figure 6b).

Raman spectroscopy was employed to study the structural near-order oxygen anionic–cationic changes for the ceria pellets with respect to the oxygen nonstoichiometry. For this, we compare (i) a freshly sintered oxidized pellet, (ii) two chemically reduced pellets with different reduction durations at 1373 K using p_H2 = 0.02 atm, and (iii) two thermally reduced samples with different reduction durations at 1773 K at low oxygen partial pressures as shown in Table 1. During cooling, chemically and thermally reduced samples were held under pure argon for 15 min at 993 and 1073 K, respectively. Chemical reduction at 1373 K for 120 min resulted in δ_i ≈ 0.253, which is clearly above the transition point of drastic decrease in CO production rate (see Figure 6b).

Table 1. Experimental Conditions and Initial Nonstoichiometries of Ceria Pellets for Performing Raman Spectroscopy of Surface.

reduction methodology	none	chemical pH2 = 0.02 atm	chemical pH2 = 0.02 atm	thermal pO2 ≪ 1 atm	thermal pO2 ≪ 1 atm
Tred (K)	–	1373	1373	1773	1773
duration (min)	–	0	120	5	180
δi (−)	0	0.128	0.253	0.034	0.057

For a given cubic fluorite crystal structure such as in undoped ceria, there is only a single characteristic cationic–anionic stretching vibration mode with Raman activity, namely, the F_2g triply degenerated Raman mode, which is viewed as a symmetric breathing mode of the oxygen ions around each cerium cation in the lattice, see refs (35−37) for details. This breathing mode is sensitive to the “chemo-mechanic” property changes such as oxygen nonstoichiometry–bond strength changes, namely, the F_2g mode shifts in wavenumber with respect to the nonstoichiometry of the material.^38,39

Figure 8a shows the normalized Raman intensity as a function of wavelength for all samples. All spectra reveal a strong and single vibration mode around 465 cm^–1. No additional bands were detected, clearly confirming the single-phase nature of the ceria independent of the oxygen nonstoichiometry over the range of δ_i = 0–0.253. This main vibration band is assigned to the symmetric oxygen anionic–cationic breathing Raman F_2g mode and is in accordance with earlier literature references by McBride et al.,³⁵ Weber et al.³⁷, and Rupp et al.⁴⁰ Extraction of the F_2g peak position and full width at half-maximum as a function of δ_i was obtained through fitting data with a sixth-order polynomial function. Increasing the oxygen nonstoichiometry lowers the F_2g Raman peak position by half a wavenumber from 465 cm^–1 (δ_i ≈ 0) to 464.5 cm^–1 (δ_i ≈ 0.253), Figure 8b. This trend is accompanied by a broadening of full width at half-maximum with increasing oxygen nonstoichiometry from Δω ≈ 7 to 9.5 cm^–1 for δ_i ≈ 0 to 0.253, respectively, Figure 8c. Conventionally, one would expect that with increasing oxygen nonstoichiometry and lattice expansion of the ceria, the F_2g signature peak would shift up to higher wavenumbers and peak width would broaden because of mixing of the band with second-order phonon scatter effects, see McBride et al. for details.³⁵ In contrast, we report a lowering of the F_2g mode in its wavenumber upon reduction of the ceria for the rather unusually high oxygen nonstoichiometries of 0.253. This reflects an active compression of the oxygen anion–cation near order and ceria lattice. In agreement with the ceria phase diagram,³² this is close to the phase transition regime of face-centered cubic cells with long-range defect–defect interactions (α′-phase) to intermediate rhombohedral cell structures (τ-phase).^27,31 At this stage, the material may develop a phase instability that thermodynamically benefits an increased association energy of the reduced Ce³⁺ ions (Ce_Ce^′) with oxygen vacancies (V_o) to form dimer associates (Ce_Ce^′/V_o). Very recently, Marrocchelli et al.⁴¹ highlighted how changes in chemical expansion may significantly alter lattice bond strength by atomic scale simulations in the ceria system; for example, compressively straining the lattice can lead to shifts in the association energies and thereby result in oxygen anionic–cationic bond compression, in agreement with refs (38, and 42−44).

Figure 8.

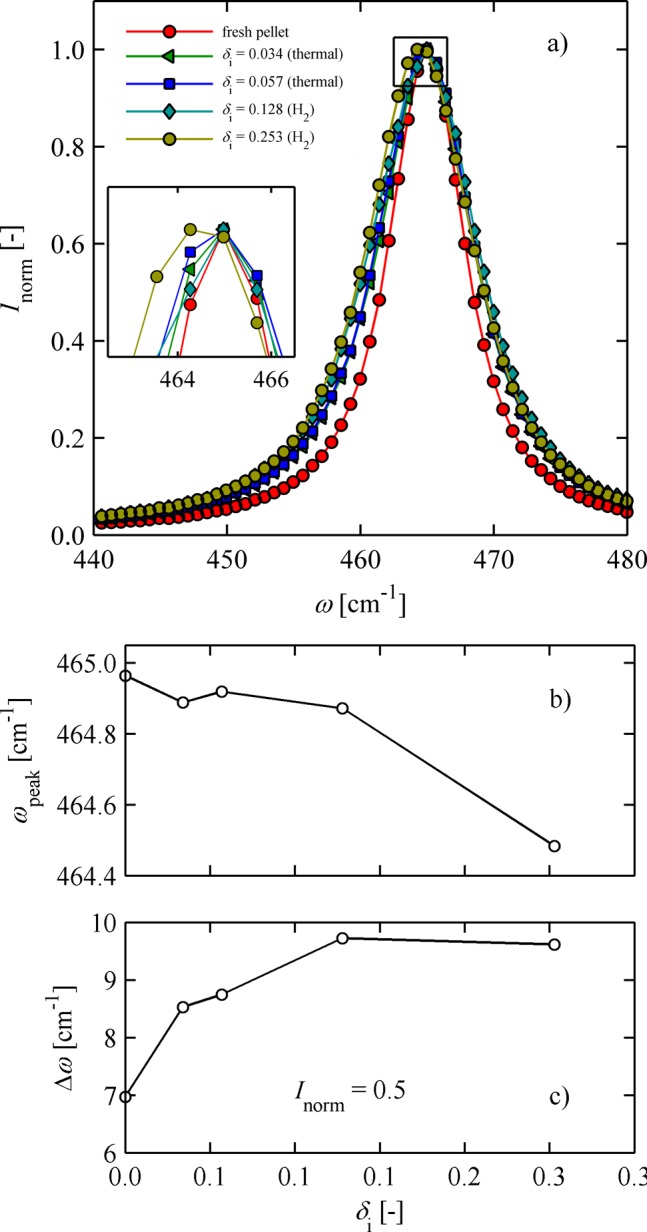
Raman spectroscopy of ceria pellet surface reduced to different δ_i: (a) single F_2g peak at ω ≈ 465 cm^–1; (b) decreasing peak wavelength position with increasing δ_i; (c) increasing full width at half-maximum intensity with increasing δ_i.

3.2.2. Thermal Reduction

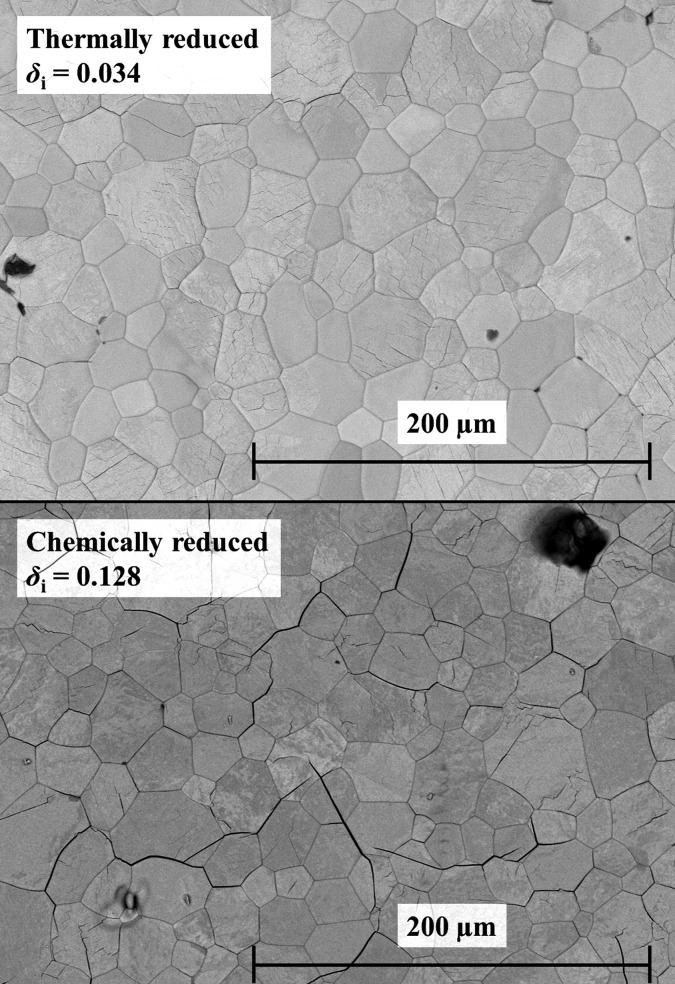
To study oxidation behavior following thermal reduction, sintered ceria pellets were thermally reduced at 1773 K and p_O2 ≈ 2.5 × 10^–4 atm for different reduction times.⁹ Compared to reduction with H₂, oxidation rates are shown to be around 1 order of magnitude lower. These slower rates are attributed to the fact that fewer small cracks are induced. This can be seen in Figure 9 where we show SEM pictures of the surface of the thermally reduced pellet in the top panel (δ_i = 0.034) and the chemically reduced in the bottom panel (δ_i = 0.128). We were not able to measure the Brunauer–Emmett–Teller (BET) surface area without destroying their morphology and therefore could not quantify the change in BET surface area induced by cracks.

Figure 9.

SEM pictures of (top panel) grain surface of a ceria pellet thermally reduced to δ_i = 0.034 and (bottom panel) grain surface of a ceria pellet chemically reduced to δ_i = 0.128.

Figure 10a shows r_CO in log space at δ_eval = 0.02 as a function of inverse temperature for 973 ≤ T ≤ 1273 K for CO₂ concentrations ranging from 0.1 to 0.4 atm. Increasing rates are observed with increasing CO₂ concentration, whereas a clear turnover of r_CO with increasing temperature is observed for all CO₂ concentrations. Similar behavior was observed after chemical reduction at these elevated temperatures, and is likely due to a shift in the chemical equilibrium.⁵ Increasing CO₂ concentrations shift the transition to higher temperatures because higher CO₂ concentrations favor the forward reaction.

Figure 10.

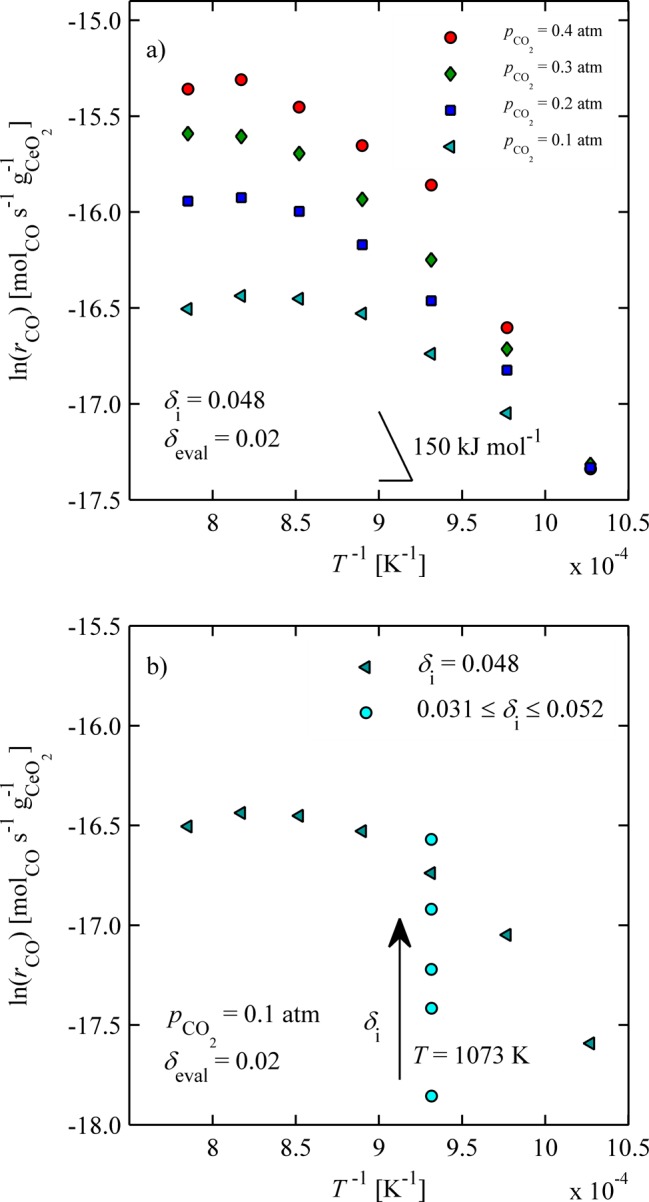
(a) CO production rates at δ_eval = 0.02 for 973 ≤ T ≤ 1273 K and 0.1 ≤ p_CO2 ≤ 0.4 atm concentrations for δ_i = 0.048; (b) increasing CO production rate with increasing δ_i at T = 1073 K and p_CO2 = 0.1 atm.

Oxidation rates were also strongly dependent on the initial nonstoichiometry and increased with δ_i over the range of nonstoichiometries probed (δ_i < 0.06). This is shown in Figure 10b, in which the oxidation rates are shown at T = 1073 K for initial nonstoichiometries increasing from 0.031 to 0.052. For reference, the rate versus inverse temperature is also shown for a single δ_i, extracted from Figure 10a. During chemical reduction in this reduction range, there was no observable effect on oxidation rates. The rates were affected only at higher δ, but in a contrasting way, that is, rates increased with increasing initial nonstoichiometry.

lnr_CO increases almost linearly with δ_i for a range of operating conditions, as shown in Figure 11. Here, r_CO is shown in log space as a function of δ_i for δ_eval = 0.02, p_CO2 = 0.1 and 0.2 atm, and T = 1123 and 1173 K. Rates at δ_i ≈ 0.055 are almost twice as large as rates at δ_i ≈ 0.035. On the basis of the Raman results and phase diagram discussed above, this behavior is probably not due to near-order phase changes. One possible explanation is an increase in charge-driven defect interactions caused by increasing electron and vacancy concentrations. This would necessarily affect the dominant transport processes occurring in the lattice. In an effort to test this hypothesis, similar experiments were performed with a 10 mol % Gd doped ceria pellet that is expected to have less defect interactions under the conditions investigated.^10,45−47 In fact, for Ce_0.9Gd_0.1O_1.95−δ an ideal solution model accounting only for doubly ionized oxygen vacancies and localized electrons on the cerium lattice sites has been shown to be valid above 1073 K for δ < 0.04.⁴⁶ In the case of pure ceria, such a model is not applicable except in the case of very low nonstoichiometries (δ < 0.01).^9,12,48 Results are also shown in Figure 11, and in this case oxidation rates are mostly constant for δ_i < 0.04. Initial nonstoichiometries greater than δ_i = 0.04 were not possible in this system because of limitations in the operating temperature and baseline oxygen level.

Figure 11.

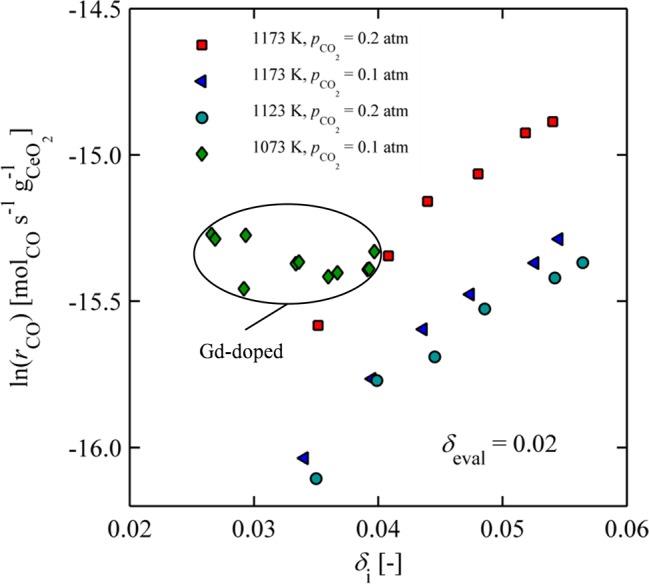
CO production rates as a function of increasing initial nonstoichiometry for different temperatures and CO₂ concentrations for ceria and 10 mol % Gd doped ceria.

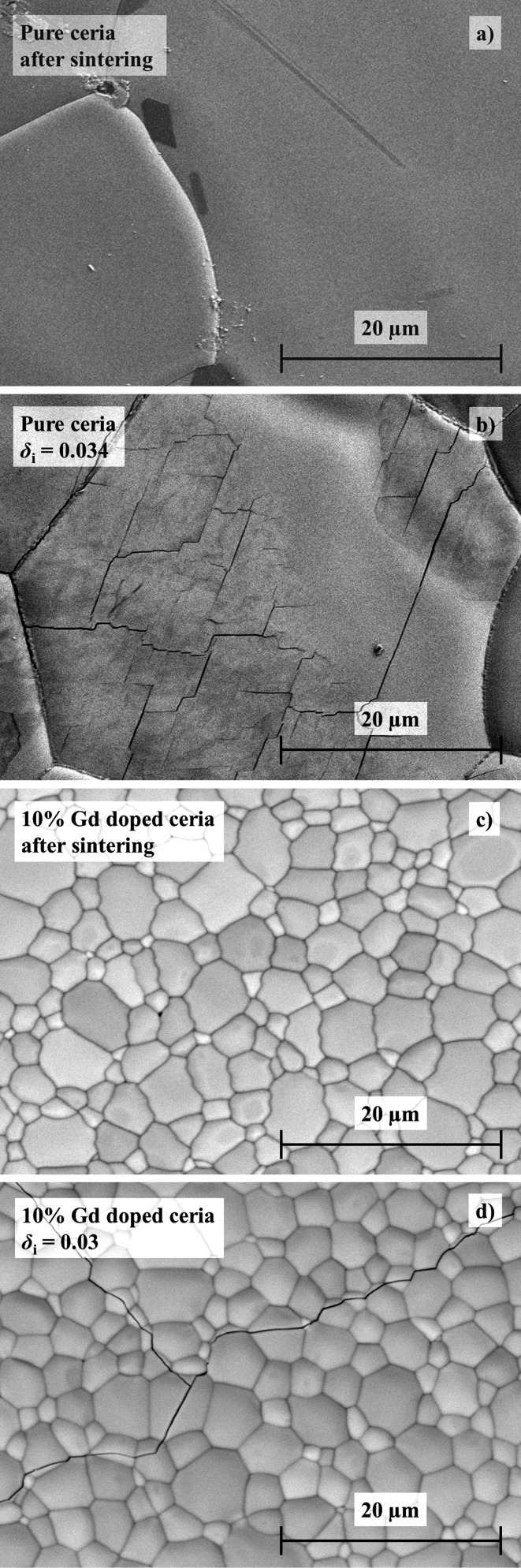
Another possible explanation for increased rates with nonstoichiometry may be related to the increase in chemical expansion with increasing δ_i.^{11,41,49−51} Prior to reduction, the grain sizes within the pure ceria pellet are larger (20–50 μm) compared to the grain sizes of 10 mol % Gd doped ceria (3–10 μm), and no cracks are visible (Figure 12a,c). However, following reduction, cracks are formed within pure ceria grains (Figure 12b) which probably lead to an increased surface area. No such small intragrain cracks can be observed on grain surface of the reduced 10 mol % Gd doped ceria pellet, as shown in Figure 12d, probably because the small grains can accommodate chemical expansion more easily than larger grains. However, there is noticeable crack propagation across grains. Unfortunately, because the same degree of crack propagation does not occur in Gd-doped ceria as in pure ceria, it is not possible to point toward a likely explanation for increasing oxidation rates with nonstoichiometry. Therefore, we can only hypothesize that it is due to either increasing defect interactions or increasing surface area caused by chemical expansion.

Figure 12.

SEM images: (a) grain surface of a stoichiometric pure ceria pellet; (b) grain surface of pure ceria pellet reduced to δ_i = 0.034; (c) grain surface of a stoichiometric 10 mol % Gd doped ceria pellet; and (d) grain surface of a stoichiometric 10 mol % Gd doped ceria pellet reduced to δ_i = 0.03.

4. Conclusion

It is shown that ceria oxidation rates are not only dependent on temperature and CO₂ concentration but also strongly dependent on reduction extent and surface morphology of the sample. For sintered ceria pellets reduced with H₂ to extreme nonstoichiometries (δ > 0.2), a transition toward very slow oxidation rates is observed. Specifically, as nonstoichiometry increased, the oxidation rate for a fixed oxygen concentration (constant δ) decreased. The nonstoichiometry where the transition occurs slightly increases with temperature and agrees well with a near-order phase change reported in literature. Results are further supported with Raman spectroscopy results that indicate an active compression of the oxygen anion–cation near order and ceria lattice in this region. For nonstoichiometries <0.06, achieved during higher-temperature thermal reduction with low oxygen partial pressure, the opposite behavior is observed, and oxidation rates increase almost linearly with increasing nonstoichiometry for pure ceria. We attribute this trend to one of two phenomena: (1) the formation of defect clusters that affect bulk transport, primarily because such behavior is not observed for 10 mol % Gd doped ceria where defect interactions are known to be less prevalent or (2) slight changes in morphology due to chemical expansion. While these results shed new light on oxidation rates of ceria under a broad range of operating conditions, further work is required to obtain more quantitative information about the rates and their relationship to nonstoichiometry so that they may ultimately be applied in system models to improve overall performance. For example, it will be important to decouple intrinsic reaction rates from any changes in subtle morphology during reduction (i.e., expansion, crack propagation). Already, extensive data exists describing the chemical and thermal expansion behavior of these materials,^{41,49,50,52,53} but it will also be important to quantify how cracks propagate as a function of grain size and nonstoichiometry.

Glossary

Nomenclature

C_CO2

CO₂ concentration (mol m^–3)

E_A

Activation energy of reaction (J mol^–1)

f(δ)

Reaction mechanism (−)

I_norm

Normalized Raman intensity (−)

k(T)

Reaction rate constant (s^–1)

m_CeO2

Reactive sample mass (mg)

m_s

Total sample mass (mg)

M_CeO2

Molar mass of ceria (g mol^–1)

M_O

Molar mass of oxygen (g mol^–1)

n

Reaction order (−)

p_CO2

CO₂ partial pressure (atm)

r_CO

Rate of production of gaseous CO (mol_CO s^–1 g_CeO2^–1)

R_gas

Universal gas constant, 8.314 (J mol^–1 K^–1)

T

Temperature (K)

T_red

Reduction temperature (K)

δ

Oxygen nonstoichiometry (−)

δ_eval

Oxygen nonstoichiometry at which rates are evaluated (−)

δ_i

Initial oxidation nonstoichiometry (−)

Δm

Mass change of sample (mg)

Δω

Full wavenumber width at half-maximum intensity (cm^–1)

ω

Wave number (cm^–1)

ω_peak

Wave number of maximum peak intensity (cm^–1)

The authors declare no competing financial interest.