Abstract
Plants in multiple symbioses are exploited by symbionts that consume their resources without providing services. Discriminating hosts are thought to stabilize mutualism by preferentially allocating resources into anatomical structures (modules) where services are generated, with examples of modules including the entire inflorescences of figs and the root nodules of legumes. Modules are often colonized by multiple symbiotic partners, such that exploiters that co-occur with mutualists within mixed modules can share rewards generated by their mutualist competitors. We developed a meta-population model to answer how the population dynamics of mutualists and exploiters change when they interact with hosts with different module occupancies (number of colonists per module) and functionally different patterns of allocation into mixed modules. We find that as module occupancy increases, hosts must increase the magnitude of preferentially allocated resources in order to sustain comparable populations of mutualists. Further, we find that mixed colonization can result in the coexistence of mutualist and exploiter partners, but only when preferential allocation follows a saturating function of the number of mutualists in a module. Finally, using published data from the fig–wasp mutualism as an illustrative example, we derive model predictions that approximate the proportion of exploiter, non-pollinating wasps observed in the field.
Keywords: host sanctions, preferential allocation, meta-population dynamics, modularity, stability of mutualism
1. Background
Most plant species rely on symbionts to provide essential services, including acquiring nutrients, dispersing pollen and defending against pests and disease. This can pose a problem, as symbionts vary in their capacity to provide these services [1–3]. Further, exploitative symbionts that allocate resources primarily to their own survival and reproduction can outcompete mutualists that provision some of their resources to providing services [4–9]. Discriminating hosts are thought to stabilize mutualist populations by preferentially allocating resources to mutualists and/or terminating resource allocation to exploiters (sanctions) [5]. However, rather than allocating resources directly into individual symbionts, hosts generally allocate resources into coarse features of their anatomy where symbiotic services are generated. We refer to these features as modules, with examples including the entire inflorescences of actively pollinated figs [3,10], root nodules of legumes [11–12] and the fine roots of arbuscular mycorrhizal plants [8,13]. Each of these modules can be colonized by multiple symbionts, such that exploiters that co-occur with mutualists within a module can escape sanctions and share rewards generated by their mutualist competitors [3,8,10,14]. The purpose of this study is to predict how mixed colonization of modules influences the population dynamics of mutualist and exploiter symbionts.
Exploiters provide less service to their hosts than mutualists, but can have higher fitness when they co-occur with a mutualist within the same module. For example, in the nutritional symbioses between plants and mycorrhizal fungi, exploitative fungi that do not invest in nutrient acquisition have been shown to outcompete mutualists when they co-occur in mixed modules [7–8]. Similarly, in the legume–rhizobium symbiosis, mutualist bacteria pay a metabolic cost to fix nitrogen that could otherwise be allocated towards their own reproduction [6,15–16], which may allow exploiter strains to outcompete mutualists in mixed modules [14,17]. This dynamic may also apply to plant–pollinator symbioses; for example, pollinating fig wasps collect pollen and disperse it between trees that are 10–15 km apart [18], whereas non-pollinating wasps incubate their offspring inside developing figs without providing this service. If pollen dispersal is expensive and sanctions act on whole inflorescences (e.g. [10]), exploiter wasps would outcompete mutualists in mixed modules.
Mixed colonization by mutualists and exploiters can occur whenever at least two symbionts colonize individual modules. However, while a previous model considered the population dynamics of mutualists and exploiters in terms of the proportion of mixed modules with exactly two colonists each [17], hosts in plant–pollinator and microbial symbioses develop modules that are frequently colonized by more than two symbionts [19–21]. Higher module occupancies (N = number of colonists per module) should be associated with a greater proportion of mixed modules by increasing the chance that mutualists and exploiters both arrive and establish in the same module. Further, at higher module occupancies, mixed colonization follows a more continuous distribution, with exploiters comprising from 1/N to (N − 1)/N of the module (e.g. 1/6 to 5/6 of modules with an occupancy of 6). A general model of module-level discrimination must accommodate both this increase in the overall proportion of mixed modules and the continuity of their distribution, particularly given variability in module occupancy both within and among symbioses.
Measuring module occupancy requires identifying the smallest structure where hosts discriminate against exploiters and counting the number of symbionts associated with it. For example, many actively pollinated fig species can either abort or reduce resource allocation to whole inflorescences that are exploited by non-pollinating wasps of the pollinating species (hereafter referred to as ‘non-pollinating wasps', which are distinct from wasp species that oviposit from outside developing inflorescences) [3,10,21]. The average number of wasp foundresses that colonize the modules of different fig species ranges from one to more than six wasps [19]. Moreover, fig species colonized by a greater number of foundresses are associated with higher proportions of non-pollinating wasps [3], suggesting that the most susceptible hosts are those that develop modules that increase both the extent and distribution of mixed colonization.
Similar ranges of occupancies can be estimated in plant–microbial symbioses by measuring the number of different symbionts that can co-occur at the scale of host discrimination. Thus, plants can preferentially allocate carbon to regions of their root system colonized by mutualist fungi that enhance nutrient uptake [8,13,22–23]. While some plants can preferentially allocate carbon into different (approx. 2 cm) sections of the same fine root [13,22], others do so on the coarser scale of whole branched root sections [8]. Considering that single fine roots can host as many as 6–12 species of mycorrhizal fungi [20], coarse preferential allocation should correspond to similarly high module occupancies, whereas fine-scale preferential allocation should correspond to lower occupancies. Likewise, soya bean (a legume) can reduce oxygen allocation to whole nodules that are colonized by rhizobia that are prevented from fixing nitrogen [11]. If other legumes similarly discriminate at the scale of whole nodules, then variability in module occupancy among legume species can be inferred by comparing the proportion of nodules that are colonized by multiple bacterial strains, with low proportions being consistent with low module occupancy, and vice versa. Legume species vary 10-fold in the proportion of nodules that are colonized by multiple bacterial strains [14], from 7.2% [24] to 74% [25], suggesting that they would span a gradient of module occupancy from 1 to approximately 3 (electronic supplementary material).
When symbiotic services are costly, exploiters should outperform mutualists in every module in which they co-occur. However, this within-module advantage of exploitation is potentially offset by an among-module advantage of mutualism, such that hosts can compensate mutualists for the costs of symbiotic services by allocating a greater amount of resources into modules with a greater proportion of mutualists. The resulting dynamics of mutualist and exploiter populations is a balance between these within- and among-module fitness components, with symbionts structured into meta-populations of modules with different proportions of mutualists. Here, we explore how the number of partners capable of colonizing a host's modules (occupancy) influences the stability of mutualism. In addition, we assess different functions of how hosts preferentially allocate resources into modules with increased proportions of mutualists versus exploiters. The resulting meta-population model describes how measurable properties of the host influence the fitness of mutualists and exploiter populations in ways that promote fixation of one or the other, stable coexistence, or fixation conditional on the initial proportions of mutualists.
2. Material and methods
(a). Mutualist and exploiter equilibrium with precise discrimination
We begin our model by solving for the simplest condition: precise discrimination to the unit of the individual organism (e.g. figs with inflorescences that host only single wasps [10]). First, we specify the dynamics of a population with proportion m of mutualists and 1 − m of exploiters using the replicator equation [26], such that
 |
2.1 |
The population is at equilibrium when equation (2.1) is set equal to 0, which occurs whenever mutualists and exploiters have the same fitness (Wm = We).
In our model, mutualists pay a symbiotic service cost (z), while exploiters do not. Hosts allocate a certain amount of resource into symbionts after they arrive in module in order to initiate resource exchange, which we define as v. In addition, hosts preferentially allocate resources (b) into modules colonized by mutualists, such that b = allocation to mutualists − allocation to exploiters. Thus, the fitness of mutualists (Wm) is equal to v + b − z (initial resource allocation + preferential resource allocation − symbiotic cost), while the fitness of exploiters (We) is equal to v (these and all subsequent model terms are defined in table 1).
Table 1.
A glossary of model terms and their definitions.
| model term | definition |
|---|---|
| module occupancy (N) | the number of colonists per module |
| preferential allocation (b) | the difference between the amount of resources allocated to modules colonized entirely by mutualists and modules colonized entirely by exploiters, expressed in units of per capita fitness |
| symbiotic service cost (z) | the cost that mutualists pay to generate host benefits; equal to the difference in mutualist and exploiter fitness within a mixed module |
| initial allocation (v) | the initial resources allocated to all symbionts within a module (mutualist and exploiter) in order to establish symbiosis |
| half-saturation constant (α) | the proportion of preferential allocation that goes into modules with a 50 : 50 mix of mutualists and exploiters, determining whether the function is linear (α = 0.5), saturating (α > 0.5) or accelerating (α < 0.5) |
| mutualists (m versus M) | symbionts that provide a costly service to their hosts; the proportion of mutualists in the population is m, while the number of mutualists within a module is M |
| exploiters (1 − m versus N − M) | symbionts that consume host resources without generating any services; the proportion of exploiters in the population is 1 − m, while the number of exploiters within a module is N − M |
| proportion of module occupancy N (PN) | an empirically determined frequency of modules with N colonists |
Setting these two quantities equal to one another and solving for b demonstrates that mutualists will go to fixation whenever preferential allocation exceeds the cost of symbiotic services (b > z). In contrast, when preferential allocation falls short of symbiotic service costs, exploiters go to fixation. In the absence of mixed colonization, coexistence of mutualists and exploiters is impossible.
Note that the value of v is immaterial to the dynamics of this and all of our subsequent examples, as this component of fitness is equally available to mutualists and exploiters, and thus does not give one a competitive edge over the other. However, as we shall explore later, measuring the value of v is important when estimating model terms from available data. For now, we will assume that v ≥ z, such that both mutualists and exploiters have positive growth rates in all modules.
(b). Equations for preferential allocation with mixed colonization
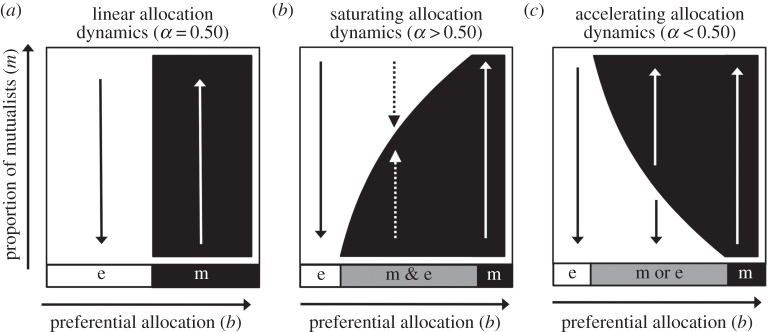
Discriminating hosts allocate more resources into modules that contain a higher proportion of mutualists. Here, we contrast three qualitatively different ways this can occur, designated as linear, saturating and accelerating functions, respectively (figure 1). The benefit to each symbiont within a module with M mutualists out of a total of N occupants is
 |
2.2 |
Figure 1.
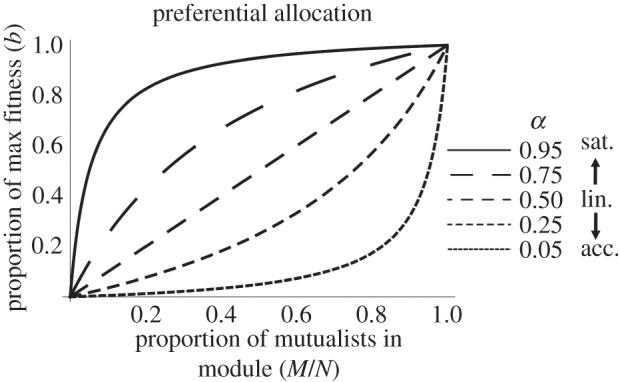
Preferential allocation to modules according to their proportion of mutualists (M/N) with linear (α = 0.50), saturating (α > 0.50) and accelerating (α < 0.50) functions. The maximum amount in each case is equal to b.
where the value of α determines the proportion of the maximum benefit (b) allocated to a module with a 50 : 50 mixture of mutualists and exploiters. We assume that there is no difference in allocation to mutualists and exploiters within a module, which is supported empirically in the fig–wasp symbiosis [10]. Thus, when α = 0.50, we have a linear function where host allocation increases steadily with the proportion of mutualists within a module. This type of allocation has been demonstrated in the legume–rhizobium mutualism, in which rhizobium within nodules that fix nitrogen at 50% of the maximum rate (simulating M/N = 0.50) have roughly intermediate fitness between those within nodules fixing nitrogen at 0 and 100% of the maximum rate (simulating M/N = 0 and 1, respectively) [27]. Assuming that allocation functions reflect host remuneration for symbiotic services [28], linear functions are consistent with individual mutualists providing similar services whether they are rare or common within a module.
When α > 0.50, we have a saturating function, where host allocation increases rapidly with the proportion of mutualists initially but then tapers off at higher proportions of mutualists. For example, in the fig–wasp mutualism, discriminating hosts target completely unpollinated inflorescences with floral abortions and reductions in resource allocation [3]; however, wasps within modules colonized by one pollinating (mutualist) and one non-pollinating (exploiter) wasps (M/N = 0.5) can largely escape these sanctions [10]. This results in wasp fitness following a saturating function. In addition to other plant–pollinator mutualisms [29], saturating functions for preferential allocation may be appropriate for plant and herbivore defence mutualisms where only completely unprotected modules face strong sanctions [30]. Further, saturating functions should apply whenever mutualists are physiologically redundant at high densities—including in nutritional symbioses where increasing the abundance of mutualists beyond a threshold results in only marginal returns in host nutrition [31].
Finally, when α < 0.50, we have an accelerating function, where host allocation increases slowly with the proportion of mutualists initially but then increases rapidly at higher proportions of mutualists. This positive-frequency-dependent allocation is analogous to positive-density-dependent interactions described as the Allee effect [32]. For example, accelerating functions could describe host responses to services that can only be rendered by mutualists that are spatially aggregated or working together in a group. However, there are currently too few datasets available to provide an illustrative example of accelerating preferential allocation.
(c). Module occupancy and binomial distribution
To specify the process that sorts partners into modules with different numbers of mutualists and exploiters, we make the simplifying assumptions that colonization is an independent and unbiased process (neither mutualists nor exploiters are better at getting into modules). This allows us to calculate the proportion of modules with M mutualists from a binomial distribution, where the proportion of mutualists and exploiters in the population are equal to m and 1 − m, respectively. Specifically, the proportion of modules with M mutualists, given they have an occupancy of N and the overall proportion of mutualists m, is equal to
 |
Symbiont fitness is the sum of the product of the proportion of modules with M mutualists and the fitness of mutualists and exploiters within these modules (determined by the preferential allocation function, equation (2.2)), or
 |
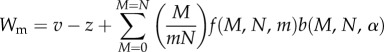2.3 |
and
 |
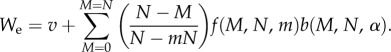2.4 |
Note the fitness equations for mutualists and exploiters differ in two ways: first, mutualists pay the symbiotic service cost; second, the two equations have different weighting terms [M/mN and (N − M)/(N − mN) for mutualists and exploiters, respectively]. The difference in the weighting terms reflects that the proportion of mutualists and exploiters present in modules with exactly M mutualists are usually different. For example, no exploiters are present in modules when M = N, while no mutualists are in modules when M = 0.
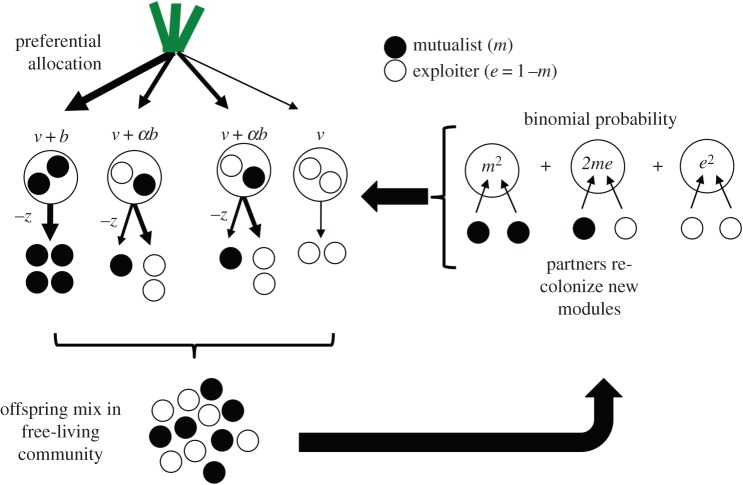
The conceptual model in figure 2 illustrates the three components of the model: (i) preferential allocation depending on the proportion of mutualists in a module; (ii) mixing of mutualists and exploiters in the free-living community; and (iii) re-colonization of newly developed modules according to the binomial distribution. All the population dynamics we describe here are derived from this basic framework, changing only the different types of allocation patterns (linear, saturating and accelerating) and the module occupancies (N = 2, 4 and 6).
Figure 2.
A conceptual representation of our model using a plant host that discriminates among modules with an occupancy of 2. Exploiters outcompete mutualists in mixed modules but have equal fitness to mutualists overall due to the relatively higher fitness of mutualists in modules with two mutualists relative to exploiters in modules with two exploiters. This iteration depicts a linear function of preferential allocation at equilibrium (m = 0.5, v = 1, z = 1, b = 2, α = 0.5). (Online version in colour.)
3. Results
(a). Qualitative differences among linear, saturating and exponential functions of preferential allocation
In the model for precise discrimination (N = 1), we solved for mutualist and exploiter equilibrium in terms of the magnitude of preferential allocation (the solution was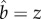 
), while the only equilibrium proportions of mutualists were 0 and 1. In contrast, when discrimination is imprecise (N > 1), it is possible for the proportions of mutualists and exploiters to reach an interior equilibrium point (0 < 
< 1). We can numerically solve for the equilibrium proportion of mutualists as a function of preferential allocation, or 
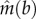(see electronic supplementary material for general solutions). By plotting this function, we can determine whether the equilibriums are stable (coexistence) or unstable (conditional dynamics) graphically. In figure 3, we contrast three of these plots, one each for linear, saturating and accelerating functions of preferential allocation. Mutualists have a greater fitness when preferential allocation exceeds equilibrium (right of the line, black), while exploiters have a higher fitness when preferential allocation is less than equilibrium (left of the line, white).
Figure 3.
The equilibrium level of mutualism depicted versus preferential allocation for (a) linear, (b) saturating and (c) accelerating functions. Preferential allocation values to the left of the line (white with black arrows) indicate that exploiters have higher fitness, while preferential allocation to the right of the line (black with white arrows) indicate that mutualists have higher fitness. Dotted arrows indicate symbiont dynamics that lead to a stable coexistence of mutualists and exploiters, which is only possible with saturating preferential allocation (b), whereas positive frequency dependence leads to alternative stable states of 100% mutualists or exploiters with accelerating preferential allocation (c).
When preferential allocation is linear, the equilibrium level of preferential allocation (b) is a perpendicular line, such that stable equilibrium occurs only when m = 1 and m = 0, with the outcome determined by whether b < Nz (exploiters go to fixation) or b > Nz (mutualists go to fixation; figure 3a). In contrast, when preferential allocation is saturating, the equilibrium level of mutualism increases with preferential allocation. This can result in stable coexistence of mutualists and exploiters at intermediate values of preferential allocation, with mutualists and exploiters exhibiting negative frequency dependence around the interior equilibrium (figure 3b). Conversely, accelerating functions of preferential allocation exhibit the opposite pattern, with the equilibrium proportion of mutualists decreasing with preferential allocation. This results in positive-frequency-dependent population dynamics, with alternative stable states of either 100% mutualists or exploiters depending on the initial proportion of mutualists (figure 3c).
The explanation for why population dynamics differ among these three functions of preferential allocation is best explored with an analogy. Imagine exploiters within a module can transition into mutualists (and vice versa) when it is advantageous for them to do so. When preferential allocation is linear, an exploiter that transitions to a mutualist pays the symbiotic service cost (z) and receives an additional b/N resource in return for its investment, where b/N is the slope of the preferential allocation line. This transition is worthwhile for the exploiter when the increase in preferential allocation outweighs the symbiotic service cost, such that b/N > z or b > Nz. On the other hand, mutualists should transition into exploiters if b < Nz, as the cost of services exceeds the marginal return of preferential allocation. This analogy can be extended to saturating and accelerating functions of preferential allocation, but the outcomes differ because the reward an exploiter receives for transitioning into a mutualist depends on the modular frequency of mutalists (M/N).
Unlike linear functions of preferential allocation, saturating and accelerating functions do not have a constant slope. Thus, the reward to an exploiter for transitioning to a mutualist is not a constant b/N, but varies depending on the frequency of mutualists within a module. With saturating preferential allocation, the slope of the preferential allocation function is the greatest when mutualists are at low proportions within a module (low M/N) and decreases as this proportion increases (high M/N). This means that the reward for transitioning to a mutualist is greatest in modules where mutualists are rare and relatively marginal in modules when they are common, which can result in coexistence via stabilizing negative feedback. Conversely, with accelerating preferential allocation, the slope of the preferential allocation function is lowest when mutualists are at low proportions within a module and greatest when they are at higher proportions, which can result in alternative stable states via destabilizing positive feedback.
In practice, our model does allow symbionts the option of transitioning between strategies. However, competitive dynamics between fixed strategies produce the same qualitative results—coexistence between mutualists and exploiters is possible only with saturating functions of preferential allocation.
(b). Quantitative differences among linear, saturating and accelerating preferential allocation
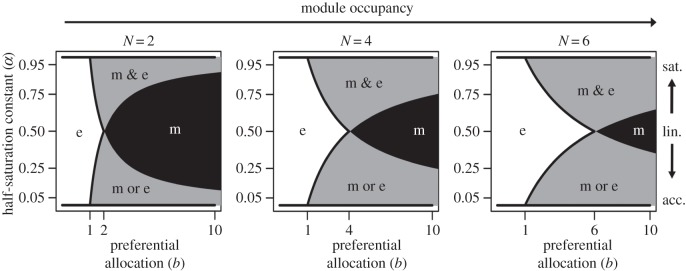
In figure 4, we plot qualitatively different symbiont population dynamics for three different module occupancies across a range of preferential allocation and half-saturation constants. When preferential allocation is linear, mutualism collapses whenever b < Nz. This limit is high relative to saturating functions of preferential allocation, where mutualists can stably coexist with exploiters even when preferential allocation is below Nz. However, while mutualists go to fixation with linear functions whenever b > Nz, with saturating functions a range of preferential allocation values in excess of Nz is associated with stable mixed communities of mutualists and exploiters. This range of preferential allocation increases as the functions become increasingly saturated (from α = 0.50 to 1). Thus, relative to linear functions, saturating functions are associated with a greater proportion of mutualists when preferential allocation is low (mixed communities versus mutualism collapse, b < Nz) and a lesser proportion of mutualists when preferential allocation is high (mixed communities versus mutualism fixation, b > Nz). In all cases, as module occupancy increases higher levels of preferential allocation are required to drive mutualists to fixation regardless of starting conditions.
Figure 4.
The population dynamics of mutualists and exploiters as a function of preferential allocation (b, x-axis) and the half-saturation constant of preferential allocation (α, y-axis). Exploiters dominate at low preferential allocation values (‘e’). The symbiotic service cost (z) in each plot is equal to 1. At higher values of b, mutualists go to fixation (‘m’, linear functions, α = 0.50), coexistence between mutualist and exploiters occurs (‘m & e’, saturating functions, α > 0.50), or conditional dynamics drive either mutualists or exploiters to fixation (‘m or e’, accelerating functions, α < 0.50). Increasing module occupancy results in exploiters dominating at higher values of b, while increasing nonlinearity expands the region of values associated with stable or unstable equilibrium.
(c). Predictions when hosts have a distribution of module occupancies
Although we have considered the population dynamics of mutualists and exploiters in modules with single levels of occupancies, in nature hosts have a distribution of occupancies, with modules with occupancy N accounting for a proportion PN of the total [19]. We can amend our model to this complexity by calculating mutualist and exploiter fitness as the weighted average of their fitness in each module, such that
 |
3.1 |
and
 |
3.2 |
where WNm and WNe are the fitness of mutualists and exploiters at occupancy N (equations (2.3) and (2.4)). The weighting involves calculating the proportion of symbionts in the modules with different occupancies. For example, even if the proportion of modules with 1 and 2 occupants were equal to one another, there would be twice as many symbionts in the N = 2 modules than the N = 1 ones.
This refined model predicts the same relationship between qualitative population dynamics and linear, saturating and accelerating preferential allocation (figure 4). However, whereas with single occupancies linear preferential allocation is capable of driving mutualists to fixation when b > Nz, with a distribution of module occupancies this limit is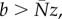 
where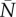 
is the average module occupancy, or
 |
Using this refined model, it is now possible to parametrize model terms using experimental data.
(d). Parametrizing the model
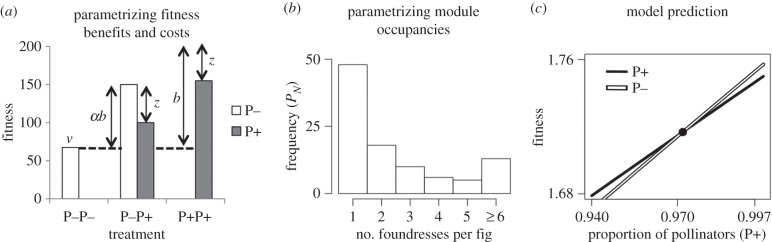
Here, we provide an illustrative example of how to quantify preferential allocation, half-saturation constant, symbiotic service cost and module occupancy. We use data on the fig–wasp mutualism from Jandér et al. [10], who introduced wasps of the pollinating species that either carried pollen (P+, mutualists) or had pollen experimentally removed (P−, exploiters) into the inflorescences of Ficus nymphaeifolia in the following way: figs received two non-pollinating wasps (P−P−), one non-pollinating and one pollinating wasp (P−P+), or two pollinating wasps (P+P+). By counting the offspring of the wasp foundresses and assessing maternity with molecular tools, Jandér et al. [10] measured the fitness of P− and/or P+ wasp foundresses in modules from each of these three treatments. However, because the experiment used artificial P− wasps, we note that these data are not appropriate for measuring symbiotic service costs relative to naturally occurring exploiters. Nevertheless, they are the best available to quantify the other model terms and act as a tutorial for future tests of model predictions.
In figure 5a, we plot wasp fitness averaged over two experiments conducted on two F. nymphaeifolia trees from Panama's Barro Colorado Island. Arrows correspond to how these measurements relate to our model terms. Briefly, allocation that is available to both mutualists and exploiters (intercept value), v, is the fitness of a P− wasp in a P−P− module; the symbiotic service cost, z, is estimated as the difference between the fitness of a P− and P+ wasp in the P−P+ module; preferential allocation, b, is the fitness of a P+ wasp in a P+ module plus the symbiotic service cost and minus the intercept; the half-saturation constant, α, is the ratio of the fitness of a P− wasp in a P−P+ module minus the intercept (giving αb) and preferential allocation.
Figure 5.
(a) Mean fitness of pollinating (P+) and non-pollinating (P−) wasps from two experimental trees of F. nymphaeifolia (adapted from [3]). Arrows illustrate how model terms can be derived from experimental data. (b) A frequency histogram of the number of wasp foundresses per fig (adapted from [19]), which are used to predict the fitness of P+ and P− wasps. (c) The model predicts that P+ and P− wasps will reach a single stable equilbrium when P+ wasps are approximately 97% of the population. The half-saturation constant (α) is 0.6, resulting in stable coexistence.
Once we have these values, we can solve for the equilibrium predictions at any module occupancy. In figure 5b, we plot a histogram of the frequency of inflorescences with 1 to 6 or more foundresses for F. nymphaeifolia (data from [19]). Using equations (3.1) and (3.2), we can then plot the fitness of P+ and P− wasps as a function of the frequency of P+ wasps in the population (figure 5c). Equilibrium occurs when the two fitnesses are equal to one another. As the value of the half-saturation constant (α) was estimated at 0.6, this equilibrium results in stable coexistence.
The model predicts that 97% of wasps associated with F. nymphaeifolia should be pollinators versus 99.7% observed in the field [3], where the proportions of naturally occurring P+ and P− wasps are from the same species. This fit is promising, such that symbiotic service costs similar to those illustrated in figure 5a would reproduce the actual frequencies of P+ and P− wasps. The model can be additionally used to indirectly estimate symbiotic service costs from experiments where they are not directly measured (electronic supplementary material). These examples illustrate the potential empirical utility of the model in an experimental system.
4. Discussion
Host discrimination is marked by varying degrees of imprecision. The unit of preferential allocation—the module—can contain a diverse assemblage of mutualists and exploiters. Thus, the inflorescences of figs can be colonized by a combination of pollinating and non-pollinating wasps, the nodules of legumes by nitrogen-fixing and non-nitrogen-fixing bacteria, and the fine roots of arbuscular mycorrhizal plants by growth-promoting and non-growth-promoting fungi. We demonstrate that the number of colonization events per module and the extent to which mixed modules are allocated resources are important determinants of the effectiveness of discrimination, and therefore the stability of mutualism.
Our model is consistent with experimental work that establishes the effectiveness of precise discrimination in modules with low levels of mixed infection [3,8]. Decreasing the module occupancy prevents exploiters from finding a haven in mixed modules where they enjoy higher fitness than mutualists. At higher module occupancies, mixed infection both increases overall and follows a more continuous distribution (from 1/N to N − 1/N). As the cost of symbiotic services prevents mutualists from outcompeting exploiters within a mixed module, the stability of mutualism is dependent on the fitness among modules. Therefore, mutualists can outcompete exploiters in other modules (with a lower proportion of mutualists), even when they cannot outcompete exploiters in their own module. Our model demonstrates that when preferential allocation is high enough relative to the cost of symbiotic services, this among-module component is sufficient to bring mutualists to fixation even when they are initially rare. We further demonstrate that the amount of preferential allocation necessary to drive mutualists to fixation increases as module occupancy increases.
Although the mixed module has been described as a cheater haven capable of fostering the coexistence of mutualists and exploiters [4,5], we find that coexistence is a special case of saturating preferential allocation. This occurs with saturating functions alone because the advantage mutualists gain in among-module competition with exploiters is maximized when mutualists are rare and extinguished when they are common. In contrast, we find that accelerating preferential allocation—where hosts are relatively unresponsive to mutualists when they are at low proportions within a module—can destabilize mutualist and exploiter populations, resulting in alternative stable states where either mutualists or exploiters are driven to fixation. This instability may account for why we were unable to find examples of accelerating models of preferential allocation in the literature.
Our results regarding the relationship between preferential allocation and symbiont population dynamics are similar to those from game theoretical models of nonlinear public goods [33–34]. Both models predict that only nonlinear models of public goods (preferential allocation) can allow for the coexistence of cooperators and defectors (mutualists and exploiters). However, our model differs in considering population dynamics explicitly in terms of host–symbiont interactions. As a result, we are able to easily shift from a heuristic model to one parametrized to empirical data. In addition, our model terms correspond to host traits that have been shown to vary in natural populations. This provides a context for evaluating discriminating hosts in terms of the mechanisms that determine how resources are allocated to mixed modules and how many symbionts can colonize each module.
The ability of plants to adjust the three different types of preferential allocation will depend on the sensitivity of hosts to differences in services rendered among modules. For example, in the fig–wasp mutualism, hosts discriminate against exploiters using a combination of abortion of completely unpollinated inflorescences and reductions in resource allocation to mixed modules (resulting in reduced wasp offspring) [3,10]. Hosts that abort only completely unpollinated figs will tend to allocate resources to modules according to saturating function with high α. Conversely, increasing the extent to which mixed modules are additionally penalized with reductions in resource allocation could reduce α, resulting in a more linear function of preferential allocation. Finally, at the extreme where hosts abort all but completely pollinated figs, preferential allocation would be accelerating.
Hosts can also manipulate module occupancy, which is the product of multiple features of the host anatomy and physiology. Larger modules should have a larger occupancy than small modules, as size corresponds both to the surface available for colonization and the volume where resource exchange takes place. Consistent with this, in the fig–wasp mutualism, fig species with larger inflorescences host more foundresses than fig species with smaller inflorescences [19]. Additionally, a large module that admits colonists across its entire surface may have a greater occupancy than a similar module that forces them through specialized entry points (or a single bottleneck). For example, many plants force arbuscular mycorrhiza through specialized passage cells before they can enter the root cortex where resource exchange occurs [35]. Similarly, fig wasps enter inflorescences through an opening called the ostiole that can be closed after foundresses enter, preventing further colonization [36].
Discriminating hosts can also increase the among-module benefit of being a mutualist by increasing the magnitude of preferential allocation. The magnitude of preferential allocation likely has an environmentally plastic component [37]; for example, shade prevents plants from preferentially allocating carbon into roots colonized by beneficial mycorrhizal fungi [23]. In addition, hosts can exhibit fixed differences in their responsiveness to their partner communities [38]. In our model, an unresponsive host that only marginally enhances resource allocation into modules colonized by mutualists (low b) would tend to favour exploiters. In contrast, a responsive host that dramatically increases resource allocation into modules colonized by mutualists (high b) would tend to interact with mutualists [3]. Consistent with this prediction, in an ant–plant mutualism, more responsive hosts tend to have a higher proportion of defensive ants [39].
We parametrized our model to experimental data from the fig–wasp mutualism [10] and used it to derive equilibrium predictions for pollinating and non-pollinating wasps on the host tree F. nymphaeifolia. Our model predicts that P+ and P− (exploiter) wasps of the pollinating species should coexist, with exploiters in the minority. Both of these predictions are qualitatively consistent with measurements of non-pollinating wasps associated with F. nymphaeifolia [3,10]. However, as the magnitude of symbiotic service costs is difficult to estimate from the available experimental data, more extensive experimentation is required to test the model. Further, as estimating the magnitude of symbiotic service costs is essential to predicting mutualist and exploiter dynamics, testing the model will require using exploiters that are adapted to avoid these costs rather than simulating exploiters by artificially preventing mutualists from performing services (e.g. removing pollen from fig wasps [3,10] or preventing rhizobia from fixing nitrogen by removing N2 gas from the atmosphere [11,27]).
Although we did not consider the physiological feedbacks between partners and their hosts, or the feedbacks of hosts on one another, it is possible to integrate our approach here into models that do (e.g. [31,37]). Our model also does not consider the impact of imperfect mixing in the partner community—a process that could lead to positive associations and repeat encounters among the offspring of individual modules. Such associations would be likely to increase the effectiveness of preferential allocation at maintaining mutualists. In contrast, if exploiters adapted to occupy only those modules that had been previously colonized by mutualists, then they would prevent being isolated in modules with other exploiters. This would be likely to reduce their among-module disadvantage relative to mutualists, decreasing the effectiveness of preferential allocation. As imperfect mixing and exploiter sophistication are likely to act as opposing forces on the population dynamics of mutualists and exploiters, we suggest that violations of the assumption of random colonization be addressed in system-specific ways.
We demonstrate that mutualist and exploiter meta-population dynamics are determined by module occupancy, the magnitude of preferential allocation, the cost of symbiotic services and the extent to which resources are preferentially allocated to mixed modules (determined by the half-saturation constant of preferential allocation). All other things being equal, higher preferential allocation, lower module occupancies and lower symbiotic service costs all increase the fitness of mutualists relative to exploiters. In contrast, the half-saturation constant determines the qualitative dynamics of symbiont populations, with coexistence of mutualists and exploiters occurring only with saturating preferential allocation. Overall, we conclude that saturating preferential allocation and mixed colonization of modules contribute to the resolution of the evolutionary paradox of mutualisms [40–41] by explaining how imperfect discrimination can foster diversity in symbiont quality.
Supplementary Material
Acknowledgements
We acknowledge McKenna Kelly, the James Bever laboratory group, Charlotte Jandér and one anonymous reviewer for editing and commenting on this manuscript.
Authors' contributions
B.S.S. designed the model and wrote the manuscript; J.D.B. provided critical revisions and model interpretations.
Competing interests
We have no competing interests.
Funding
We received financial support from NSF DEB-1405347.
References
- 1.Heath KD, Tiffin P. 2007. Context dependence in the coevolution of plant and rhizobial mutualists. Proc. R. Soc. B 274, 1905–1912. ( 10.1098/rspb.2007.0495) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 2.van der Heijden MGA, Klironomos JN, Ursic M, Moutoglis P, Streitwolf-Engel R, Boller T, Wiemken A, Sanders IR. 1998. Mycorrhizal fungal diversity determines plant biodiversity, ecosystem variability and productivity. Nature 396, 69–72. ( 10.1038/23932) [DOI] [Google Scholar]
- 3.Jandér KC, Herre EA. 2010. Host sanctions and pollinator cheating in the fig tree–fig wasp mutualism. Proc. R. Soc. B 277, 1481–1488. ( 10.1098/rspb.2009.2157) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 4.Yu DW. 2001. Parasites of mutualisms. Biol. J. Linn. Soc. 72, 529–549. ( 10.1111/j.1095-8312.2001.tb01336.x) [DOI] [Google Scholar]
- 5.Kiers ET, Denison RF. 2008. Sanctions, cooperation, and the stability of plant–rhizosphere mutualisms. Annu. Rev. Ecol. Evol. Syst. 39, 215–236. ( 10.1146/annurev.ecolsys.39.110707.173423) [DOI] [Google Scholar]
- 6.Ratcliff WC, Kadam SV, Denison RF. 2008. Poly-3-hydroxybutyrate (PHB) supports survival and reproduction in starving rhizobia. FEMS Microb. Ecol. 65, 391–399. ( 10.1111/j.1574-6941.2008.00544.x) [DOI] [PubMed] [Google Scholar]
- 7.Bennett AE, Bever JD. 2009. Trade-offs between arbuscular mycorrhizal fungal competitive ability and host growth promotion in Plantago lanceolata. Oecologia 160, 807–816. ( 10.1007/s00442-009-1345-6) [DOI] [PubMed] [Google Scholar]
- 8.Bever JD, Richardson SC, Lawrence BM, Holmes J, Watson M. 2009. Preferential allocations to beneficial symbiont with spatial structure maintains mycorrhizal mutualism. Ecol. Lett. 12, 13–21. ( 10.1111/j.1461-0248.2008.01254.x) [DOI] [PubMed] [Google Scholar]
- 9.Ghoul M, Griffin AF, West SA. 2013. Toward an evolutionary definition of cheating. Evolution 68, 318–331. ( 10.1111/evo.12266) [DOI] [PubMed] [Google Scholar]
- 10.Jandér KC, Herre EA, Simms EL. 2012. Precision of host sanctions in the fig tree–fig wasp mutualism: consequences for uncooperative symbionts. Ecol. Lett. 15, 1362–1369. ( 10.1111/j.1461-0248.2012.01857.x) [DOI] [PubMed] [Google Scholar]
- 11.Kiers ET, Rousseau RA, West SA, Denison RF. 2003. Host sanctions and the legume–rhizobium mutualism. Nature 425, 78–81. ( 10.1038/nature01931) [DOI] [PubMed] [Google Scholar]
- 12.Simms EL, Taylor DL, Povich J, Schefferson RP, Sachs JL, Urbina M, Tausczik Y. 2006. An empirical test of partner choice mechanisms in a wild legume–rhizobium interaction. Proc. R. Soc. B 273, 77–81. ( 10.1098/rspb.2005.3292) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Kiers ET, et al. 2011. Reciprocal rewards stabilize cooperation in the mycorrhizal symbiosis. Science 333, 880–882. ( 10.1126/science.1208473) [DOI] [PubMed] [Google Scholar]
- 14.Denison RF. 2000. Legume sanctions and the evolution of symbiotic cooperation by rhizobia. Am. Nat. 156, 567–576. ( 10.1086/316994) [DOI] [PubMed] [Google Scholar]
- 15.Gutschick VP. 1981. Evolved strategies in nitrogen acquisition by plants. Am. Nat. 118, 607–637. ( 10.1086/283858) [DOI] [Google Scholar]
- 16.Kretovich WL, Romanov VI, Yshokova LA, Shramko VI, Fedulova NG. 1977. Nitrogen-fixation and poly-beta-hydroxybutyric acid content in bacteroids of Rhizobium lupini and Rhizobium leguminosarum. Plant Soil 48, 291–302. ( 10.1007/BF02187241) [DOI] [Google Scholar]
- 17.Friesen ML, Mathias A. 2009. Mixed infections may promote diversification of mutualistic symbionts: why are there ineffective rhizobia. J. Evol. Biol. 23, 323–334. ( 10.1111/j.1420-9101.2009.01902.x) [DOI] [PubMed] [Google Scholar]
- 18.Nason JD, Herre EA, Hamrick JL. 1998. Paternity analysis of the breeding structure of strangler fig populations: evidence for substantial long-distance wasp dispersal. J. Biogeogr. 23, 401–512. [Google Scholar]
- 19.Herre EA. 1989. Coevolution of reproductive characteristics in 12 species of new world figs and their pollinator wasps. Experentia 45, 637–647. ( 10.1007/BF01975680) [DOI] [Google Scholar]
- 20.Vandenkoornhuyse P, Ridgway KP, Watson IJ, Fitter AH, Young JP. 2003. Co-existing grass species have distinctive arbuscular mycorrhizal communities. Mol. Ecol. 11, 3085–3095. ( 10.1046/j.1365-294X.2003.01967.x) [DOI] [PubMed] [Google Scholar]
- 21.Wang RW, Dunn DW, Sun BF. 2014. Discriminative host sanctions in a fig-wasp mutualism. Ecology 95, 1384–1393. ( 10.1890/13-0749.1) [DOI] [PubMed] [Google Scholar]
- 22.Verbruggen E, Mouden CE, Jansa J, Akkermans G, Bücking H, West SA, Kiers ET. 2012. Spatial structure and interspecific cooperation: theory and an empirical test using the mycorrhizal mutualism. Am. Nat. 179, 133–146. ( 10.1086/665032) [DOI] [PubMed] [Google Scholar]
- 23.Zheng C, Zhang J, Zhang F, Bever JD. 2015. Shading decreases plant carbon preferential allocation towards the most beneficial mycorrhizal mutualist. New Phytol. 205, 361–368. ( 10.1111/nph.13025) [DOI] [PubMed] [Google Scholar]
- 24.Gage DJ. 2002. Analysis of infection thread development using Gfp- and DsRed-expressing Sinorhizobium meliloti. J. Bacteriol. 184, 7042–7046. ( 10.1128/JB.184.24.7042-7046.2002) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Demezas DH, Bottomly PJ. 1986. Interstrain competition between representatives of indigenous serotypes of Rhizobium trifolii. Appl. Environ. Microbiol. 52, 1020–1025. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26.Hofbauer J, Sigmund K. 1998. Evolutionary games and population dynamics. Cambridge, UK: Cambridge University Press. [Google Scholar]
- 27.Kiers ET, Rousseau RA, Denison RF. 2006. Measured sanctions: legume hosts detect quantitative variation in rhizobium cooperation and punish accordingly. Evol. Ecol. Res. 8, 1077–1086. [Google Scholar]
- 28.Olsson PA, Rahm J, Aliasgharzad N. 2010. Carbon dynamics in mycorrhizal symbioses is linked to carbon costs and phosphorus benefits. FEMS Microb. Ecol. 72, 125–131. ( 10.1111/j.1574-6941.2009.00833.x) [DOI] [PubMed] [Google Scholar]
- 29.Pellymyr O, Huth CJ. 1994. Evolutionary stability of mutualism between yuccas and yucca moths. Nature 372, 257–260. ( 10.1038/372257a0) [DOI] [Google Scholar]
- 30.Edwards DP, Hassall M, Sutherland WJ, Yu DW. 2006. Selection for protection in an ant–plant mutualism: host sanctions, host modularity, and the principal agent game. Proc. R. Soc. B 273, 595–602. ( 10.1098/rspb.2005.3273) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31.Bever JD. 2015. Preferential allocation, physio-evolutionary freedbacks, and the stability and environmental patterns of mutualism between plants and their root symbionts. New Phytol. 205, 1503–1514. ( 10.1111/nph.13239) [DOI] [PubMed] [Google Scholar]
- 32.Courchamp F, Clutton-Brock T, Grenfell B. 1999. Inverse density dependence and the Allee effect. Trends Ecol. Evol. 14, 405–410. ( 10.1016/S0169-5347(99)01683-3) [DOI] [PubMed] [Google Scholar]
- 33.Archetti M, Sheurling I. 2012. Game theory of public goods in one-shot social dilemmas without assortment. J. Thoer. Biol. 299, 9–20. ( 10.1016/j.jtbi.2011.06.018) [DOI] [PubMed] [Google Scholar]
- 34.Archetti M, Scheuring I, Hoffman M, Frederickson ME, Pierce NE, Yu DW. 2011. Economic game theory for mutualism and cooperation. Ecol. Lett. 14, 1300–1312. ( 10.1111/j.1461-0248.2011.01697.x) [DOI] [PubMed] [Google Scholar]
- 35.Sharda JN, Koide RT. 2008. Can hypodermal passage cell distribution limit root penetration by mycorrhizal fungi? New Phytol. 180, 696–701. ( 10.1111/j.1469-8137.2008.02600.x) [DOI] [PubMed] [Google Scholar]
- 36.Wang RW, Ridley J, Sun BF, Zheng Q, Dunn DW, Cook J, Shi L, Zhang YD, Yu DW. 2009. Interference competition and high temperatures reduce the virulence of fig wasps and stabilize a fig–wasp mutualism. PLoS ONE 4, e7802 ( 10.1371/journal.pone.0007802) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 37.Steidinger BS, Bever JD. 2014. The coexistence of hosts with different abilities to discriminate against cheaters: an evolutionary game theory approach. Am. Nat. 183, 762–770. ( 10.1086/675859) [DOI] [PubMed] [Google Scholar]
- 38.Grman E. 2012. Plant species differ in their ability to reduce allocation to non-beneficial arbuscular mycorrhizal fungi. Ecology 93, 711–718. ( 10.1890/11-1358.1) [DOI] [PubMed] [Google Scholar]
- 39.Heil M, Gonzalez-Teuber M, Clement L, Kautz S, Verhaagh M, Silva-Bueno J. 2009. Divergent investment strategies of Acacia myrmecophytes and the coexistence of mutualists and cheaters. Proc. Natl Acad. Sci. USA 106, 18 091–18 096. ( 10.1073/pnas.0904304106) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 40.Foster KR, Kokko H. 2006. Cheating can stabilize cooperation in mutualisms. Proc. R. Soc. B 273, 2233–2239. ( 10.1098/rspb.2006.3571) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Heath KD, Stinchcombe JR. 2013. Explaining mutualism variation: a new evolutionary paradox? Evolution 68, 309–317. ( 10.1111/evo.12292) [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.