Tuning to Binaural Cues in Human Auditory Cortex

Abstract

Interaural level and time differences (ILD and ITD), the primary binaural cues for sound localization in azimuth, are known to modulate the tuned responses of neurons in mammalian auditory cortex (AC). The majority of these neurons respond best to cue values that favor the contralateral ear, such that contralateral bias is evident in the overall population response and thereby expected in population-level functional imaging data. Human neuroimaging studies, however, have not consistently found contralaterally biased binaural response patterns. Here, we used functional magnetic resonance imaging (fMRI) to parametrically measure ILD and ITD tuning in human AC. For ILD, contralateral tuning was observed, using both univariate and multivoxel analyses, in posterior superior temporal gyrus (pSTG) in both hemispheres. Response-ILD functions were U-shaped, revealing responsiveness to both contralateral and—to a lesser degree—ipsilateral ILD values, consistent with rate coding by unequal populations of contralaterally and ipsilaterally tuned neurons. In contrast, for ITD, univariate analyses showed modest contralateral tuning only in left pSTG, characterized by a monotonic response-ITD function. A multivoxel classifier, however, revealed ITD coding in both hemispheres. Although sensitivity to ILD and ITD was distributed in similar AC regions, the differently shaped response functions and different response patterns across hemispheres suggest that basic ILD and ITD processes are not fully integrated in human AC. The results support opponent-channel theories of ILD but not necessarily ITD coding, the latter of which may involve multiple types of representation that differ across hemispheres.

INTRODUCTION

The capacity to localize sound sources is vital both for environmental awareness and for separating signal from noise in complex listening environments. To a significant extent, this ability relies on the neural coding of differences in the acoustic intensity and arrival time of sound at the two ears, termed interaural level and time differences (ILD and ITD, respectively). These cues subserve sound localization and, in human listeners, speech intelligibility in background noise (Licklider 1948; Culling et al. 2004).

Sound location is not coded topographically on the cochlea but is instead computed, initially in brainstem circuits that combine and compare inputs from the two ears. This information is maintained by successively higher auditory nuclei, including auditory cortex (AC). Lesion and neurophysiological data indicate that AC plays an essential role in mediating mammalian sound localization behaviors (Middlebrooks and Pettigrew 1981; Jenkins and Masterton 1982; Thompson and Cortez 1983; Heffner 1997; Stecker et al. 2003, 2005a; Lomber et al. 2007; Harrington et al. 2008). However, the cortical representations of ITD and ILD, and their interactions, remain poorly understood. Human studies investigating AC sensitivity to ILD and ITD, in particular, have yielded inconsistent results.

In the ascending auditory pathway, lateralized binaural tuning is well established in the form of contralateral tuning to ITD and ILD in the inferior colliculus (e.g., Palmer and Kuwada 2005; Lau et al. 2013). But evidence for contralateral bias at the level of the AC is more variable, depending on cue type (ILD versus ITD, or combinations thereof), mammalian species, and experimental method employed. In response to ILD variation, neurophysiological recordings in cat, rat, ferret, and marmoset show contralaterally biased AC tuning (Phillips and Irvine 1981; Zhang et al. 2004; Campbell et al. 2006; Higgins et al. 2010; Lui et al. 2015), as does the limited body of ILD-response data from human neuroimaging studies (Ungan et al. 2001; Palomäki et al. 2005; Stecker et al. 2015).1 In contrast, however, AC tuning to ITD appears less consistent. Reale and Brugge (1990) observed contralateral ITD tuning in cat AC neural responses, but Fitzpatrick et al. (2000) described a variety of comingled response types (i.e., peak-, intermediate-, and trough-type) with wide-ranging ITD preferences. This evidence—together with relatively weak contralateral cortical bias to ITD observed in chinchillas, gerbils, and primates (reviewed in Belliveau et al. 2014)—suggests that there may be fundamental differences in ITD coding across species and across auditory nuclei. In humans, most neuroimaging results indicate contralateral cortical ITD tuning (Krumbholz et al. 2007; von Kriegstein et al. 2008; Johnson and Hautus 2010; Magezi and Krumbholz 2010), but this effect is relatively weak in some instances (Krumbholz et al. 2005a; Palomäki et al. 2005) and absent in others (Woldorff et al. 1999; Ungan et al. 2001).

Further complicating the issue, human neuroimaging studies that do report cortical binaural tuning have consistently reported differences in the degree of contralateral bias across cerebral hemispheres. Some results, notably those based on electroencepalography (EEG) or functional magnetic resonance imaging (fMRI) measures, show left hemisphere (LH) to respond maximally to contralateral sound source location and right hemisphere (RH) more equally to both contralateral and ipsilateral sound (Krumbholz et al. 2005a, 2007; Magezi and Krumbholz 2010; Briley et al. 2013; Stecker et al. 2015). A number of magnetoencephalography (MEG) studies, however, describe an opposite pattern in which auditory space modulates activity in RH more than LH (Tiitinen et al. 2006; Johnson and Hautus 2010; Salminen et al. 2010). These inconsistent results may partly reflect methodological issues, in that EEG and MEG are not equally sensitive to neural populations presenting at different orientations to the scalp. Considering the gyral anatomy of the human auditory cortex, some results—particularly interhemispheric comparisons—could be affected by such differences. As fMRI does not share this issue with EEG and MEG, it may therefore be better suited for such comparisons.

Current understanding of binaural sensitivity in human AC is thus constrained by limited data, conflicting results, and few if any direct comparisons across cue type and experimental approach. fMRI studies have differed in the types and levels of cues presented and cortical regions examined, and have generally relied on univariate analyses with limited capacity to reveal distributed or subvoxel responses. The present study therefore sought to measure the extent and distribution of binaural tuning in human AC by systematically collecting fMRI responses to parametrically modulated ILD and ITD stimuli in circumscribed AC regions, applying both univariate and multivoxel pattern analyses. Parametric modulation of cue values made it possible to derive response functions that served to inform hypotheses about relative balances of contralateral and ipsilateral-preferring neural populations in AC and, by inference, models of binaural coding.

MATERIALS AND METHODS

Subjects

Ten subjects (five female) participated in the ILD experiment (exp. 1) and 10 (six female) in the ITD experiment (exp. 2). All participants were between 18 and 35 years old. Inclusion criteria were as follows: normal hearing (thresholds <20 dB HL between 500 and 8000 Hz), right-handedness (score > +40 on the Edinburgh Handedness Inventory [Oldfield 1971]), no experience with a tonal language, no known history of any neurological disorders, and no contraindications to MR scanning. Informed written consent was obtained from all participants. All procedures followed the guidelines of the University of Washington Human Subjects Division and were reviewed and approved by the cognizant Institutional Review Board.

Stimuli

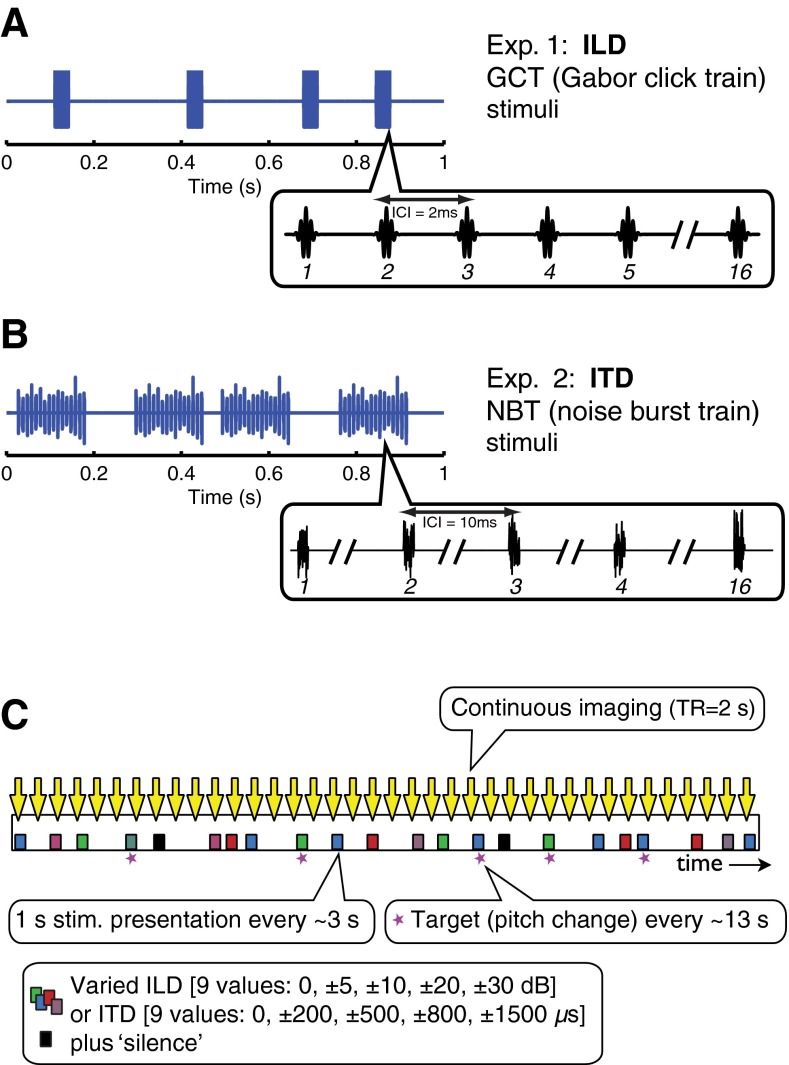
Sounds presented in exp. 1 were trains of Gabor clicks (16 clicks per train) that varied in ILD (Fig. 1A). Clicks were Gaussian-filtered impulses created by multiplying a 4000-Hz carrier frequency cosine by a Gaussian temporal envelope with σ = 221 μs, resulting in a bandwidth of 1800 Hz half-max. The peak-to-peak interclick interval was 2 ms (generating a click rate envelope frequency of 500 Hz) and the presentation rate was 4 trains per second (4 Hz). Sounds carried ILD cues that varied from trial to trial across nine values: ±30, ±20, ±10, ±5, and 0 dB. An equally probable tenth condition presented “silence” (the same underlying stimulus at an average binaural level of −10 dB SPL, which was not audible in the scanner). By convention, negative ILD/ITD values represented sounds that were louder/arrived earlier in the left ear and when played over headphones generated a percept of sound located in the left “intracranial” hemifield, with more negative sounds perceived as further leftward. Likewise, positive cue values represented rightward sounds. The range of ILD values was intended to span the full range of behaviorally relevant values at 4000 Hz. Whereas calculations for spherical heads (Kuhn 1977) suggest an upper limit of 15–20 dB, combinations of pinna effects and the “acoustical bright spot” (Macaulay et al. 2010) can produce much larger values, particularly for nearby sources (Brungart and Rabinowitz 1999).

FIG. 1.

Stimulus waveforms and imaging timeline. Stimulus waveform representations of A Gabor-click-train stimuli (1 s sound comprising 4 trains of 16 Gaussian-filtered impulses, 2 ms interclick interval) and B noise-burst-train stimuli (1 s sound comprising 4 trains of 16 white noise bursts, each 1 ms in duration, presented with 10 ms interburst interval). C Imaging timeline for an example run: event-related continuous imaging of BOLD response to parametrically varied binaural sounds, either ILD cues imposed on Gabor-click train stimuli (see A) or ITD cues imposed on noise burst stimuli (see B). MR scans are represented by downward arrows and stimulus presentation depicted by squares. Symbol color represents different ILD or ITD values.

In order to investigate ITD sensitivity, a different stimulus was used. Gabor click trains as presented in exp. 1 can carry behaviorally salient envelope ITD cues (Stecker 2010); however, initial investigations by McLaughlin (2013) failed to demonstrate blood-oxygenation-level-dependent (BOLD) tuning to that cue in any cortical region, due possibly to the relative weakness of envelope ITD in rapidly modulated narrowband sounds (Salminen et al. 2015a). Exp. 2 of the current study therefore presented trains of noise bursts (Fig. 1B) comprising 16 × 1 ms white noise bursts with 10 ms interburst intervals (i.e., an envelope frequency of 100 Hz). Compared to the stimuli of exp. 1, these present greater low-frequency energy and slower modulations known to render ITD cues more salient. In parallel to exp. 1, nine values of ITD were presented—±1500, ±800, ±500, ±200, and 0 μs—along with a tenth “silent” condition. With the exception of the ITD values of ±1500 μs, the scope of binaural cues presented in exps. 1 and 2 roughly cover the ecologically valid range for ILD and ITD generated by human-sized heads (Blauert 1983). The extreme ITD values of ±1500 μs were included in order to replicate aspects of a previous study by von Kriegstein et al. (2008) in which cortical tuning was observed for ITDs of ±500 but not ±1500 μs. Even for such large disparities, broadband sounds are reliably lateralized to the side of the ITD when applied uniformly across frequency (Trahiotis and Stern 1989; Yost et al. 2007).

All sound stimuli were presented at an average binaural level (ABL) of 80 dB SPL. ILD levels were generated by increasing intensity in one ear while attenuating it by an equal decibel amount in the other, thereby maintaining a constant ABL. Each stimulus presentation consisted of 4 trains delivered over 1 s total duration, occurring randomly within successive 3 s stimulus windows, so that the interstimulus interval ranged randomly from 0 to 5 s. The order of trials was based on a continuous carryover paradigm, with each stimulus condition preceded by every other condition on an equal number of trials, thereby completely balancing for first-order stimulus history effects (Aguirre 2007). Presentation of the binaural cue value conditions was thus pseudorandomly counterbalanced over 201 stimulus trials per run, with two runs acquired per subject. Stimuli were synthesized with Matlab (Matlab 7.4, The MathWorks, Inc., Natick MA) and presented using TDT System 3 signal-processing hardware (Tucker-Davis Technologies, Alachua FL) over piezoelectric insert earphones (Sensimetrics S14, Malden MA), enclosed within circumaural ear defenders to reduce scanner noise by ~40 dB.

Imaging

Magnetic resonance imaging was performed at 3 Tesla (Philips Achieva, Eindhoven, The Netherlands). In each of the two experiments, 10 individuals each participated in one scanning session, lasting ~90 min. In each session, a high-resolution T1-weighted whole-brain structural scan (MPRAGE) was acquired for registration with the functional scans. BOLD data were collected in four runs of echo-planar functional scans (Fig. 1C), each taking ~8 min, using a continuous event-related imaging paradigm (TR = 2 s, 42 slices, 2.75 × 2.75 × 3 mm resolution). Continuous imaging was preferred to “sparse” image acquisition (Hall et al. 1999) for its better sensitivity to the BOLD time course, and because previous comparative studies have indicated only modest differences in results obtained (Woods et al. 2009). Pilot data showed robust sensitivity to modulation of ILD in continuously acquired images, in part presumably because effects of scanner noise were strongly attenuated by the combination of foam insert earphones and ear defenders.

To minimize eye movements, subjects were asked to fixate on a visual center cross displayed on a screen in the scanner via a rear projection system. They were instructed to attend to the sound stimuli and respond by right-handed button press to an infrequent pitch change (created by slightly varying the standard inter-click or inter-burst interval). The task dimension (pitch) was orthogonal to the experimental manipulation. Target pitch changes occurred randomly at an average rate of once per ~13 s. Subjects were familiarized with the stimuli and pitch task in a listening session which took place in a double-walled sound booth 1 to 2 weeks prior to the imaging session. At that time, they also performed a simple lateralization task on the ILD and ITD stimuli to ensure, for each subject, that perceived laterality varied systematically with each cue.

Preprocessing and Analysis

Functional data for each run were pre-processed using FEAT (FSL 4.1, FMRIB, Oxford, UK) (Smith et al. 2004) to perform high-pass filtering (100 s), motion correction, B0 unwarping, and skull-stripping. To identify noise components, linear decomposition of the data from each run was performed using independent component analysis (FSL MELODIC) (Beckmann and Smith 2004). The spatial map, time-course, and power spectrum associated with each independent component were visually inspected by two raters trained to identify noise components based on criteria described by Kelly et al. (2010). Components identified independently by both raters as noise (e.g., due to the presence of large single spikes in the time-course or activation only around the peripheral rim of the brain or in the ventricles) were removed from the data using fsl_regfilt, FSL’s command-line tool for removing regressors. The pre-processed and denoised data for each run were then entered into an experimental model in which the predictors were the nine levels of binaural cue value presented, silence, pitch target, and response to target. The predictor time-courses were convolved with a gamma function and submitted to a first-level general linear model (GLM) analysis in FEAT to obtain beta weights for each predictor variable.

Using this individual first-level data, two main types of univariate analyses were conducted for each experiment: region-of-interest (ROI)-based averaging (Poldrack 2007) (Figs. 2 and 3) and voxel-wise mapping (Friston et al. 1995) (Fig. 4). For ROI analyses, three broad ROIs were created, based on data suggesting differential specialization for auditory spatial processing across posterior and anterior AC (Warren and Griffiths 2003; Krumbholz et al. 2005a; Ahveninen et al. 2006). In each hemisphere, AC was parcellated into three regions: Heschl’s gyrus (HG), anterior superior temporal gyrus (aSTG), and posterior superior temporal gyrus (pSTG). Regions were defined on the cortical surface using Freesurfer 4.1 (Martinos Center for Biomedical Imaging, MGH, Boston MA) and the included Desikan-Killiany atlas (Desikan et al. 2006). The transverse temporal gyrus region defined by Desikan et al. was used for HG, and their superior temporal region (which excludes HG) was subdivided into anterior and posterior STG regions at its intersection with HG. To create subject-specific ROIs, template ROIs were defined on the Freesurfer average surface, mapped to each individual’s cortical surface, and projected to his/her functional 3-D volume (Fig. 2A). Using Matlab, mean BOLD response to each ILD or ITD condition in the three ROIs was calculated for each individual by averaging beta weights across sound-responsive voxels (z > 2.3, uncorrected) in each ROI. Group average beta weights and standard error of the mean across subjects were calculated in each ROI.

FIG. 2.

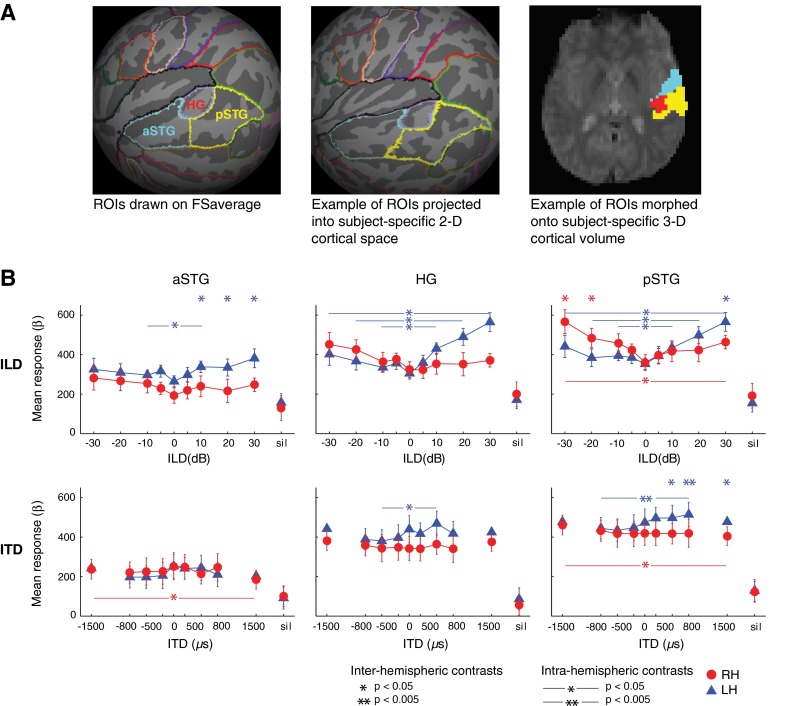
Definition and analyses of cortical regions of interest (ROIs). A Anterior superior temporal gyrus (aSTG), Heschl’s gyrus (HG), and posterior superior temporal gyrus (pSTG) ROIs drawn onto Freesurfer’s population-based 2-D surface atlas, FSaverage (left), morphed into subject-specific 2-D space (middle), and onto the subject-specific 3-D volume (right). Example subject depicted in middle and right panels. B Group response-ILD (top) and response-ITD (bottom) functions plotted in aSTG, HG, and pSTG, with significant differences in response contrasts indicated by ∗p < 0.05 and ∗∗p < 0.005. Stars above graph indicate significant differences between LH versus RH responses to stimulation at a given binaural cue level (“inter-hemispheric contrasts”). Horizontal starred lines indicate significant differences in a given hemisphere’s response to matched leftward (negative cue values) versus rightward (positive cue values) stimulation (“intra-hemispheric contrasts”). Error bars represent standard error of the mean across subjects. Blue triangles = LH responses; red circles = RH responses.

FIG. 3.

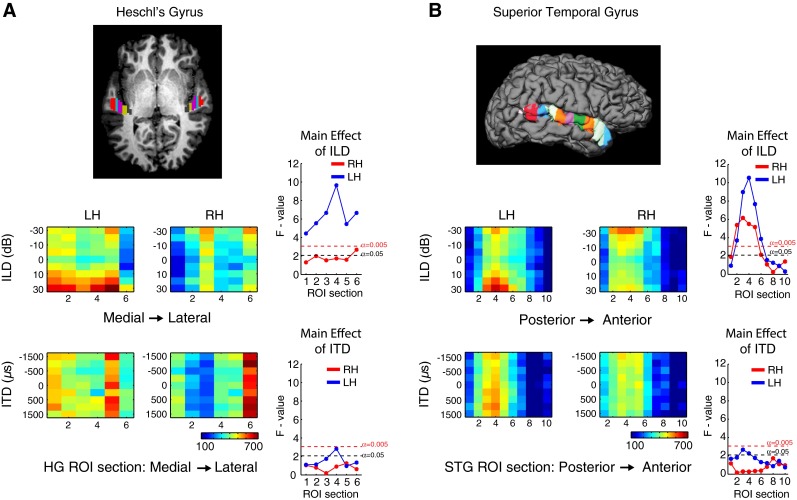
Organization of BOLD response to ILD and ITD in sectional ROIs in Heschl’s gyrus (HG) and superior temporal gyrus (STG). A Brain image showing the division of HG into six equal sections; B brain image showing the division of STG into 10 equal sections. Below each cortical image, heat maps and ANOVA diagrams plot responses in HG to ILD (upper) and ITD (lower), and in STG to ILD (upper) and ITD (lower). Colors in heat maps represent average beta weight for sound-sensitive voxels across subjects in a given sectional ROI for each ILD or ITD condition. ANOVA diagrams plot the main effect of ILD or ITD in each ROI subsection, based on single-factor repeated-measures ANOVA. Red dotted lines represent F values corresponding to significance threshold following Bonferroni correction for multiple comparisons (α = 0.005). Black dotted lines represent p < 0.05.

FIG. 4.

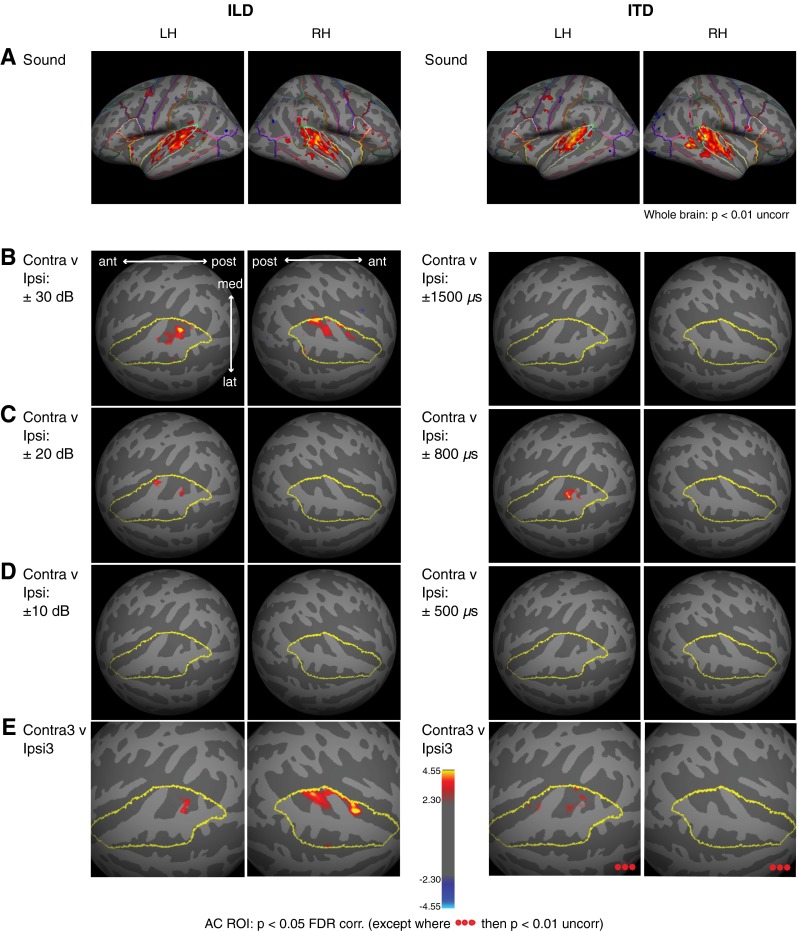
Brain maps of BOLD responses to ILD and ITD. A Group BOLD response to sound (collapsed across all binaural-level combinations), displayed on Freesurfer's template 2-D surface, FSaverage. Whole-brain response is shown at p < 0.01, uncorrected. Desikan-Killiany parcellation (Freesurfer 4.1) indicated by colored outlines for reference. B–D Group BOLD response differences in AC ROI (outlined in yellow) to contralateral versus ipsilateral stimulation at: B ±30 dB ILD; ±1500 μs ITD; C ±20 dB ILD; ±800 μs ITD; and D ±10 dB ILD; ±500 μs ITD. E Group BOLD difference contrast to contralateral 3 versus ipsilateral 3 cues. Suprathreshold voxels responding more (p < 0.05, FDR corrected in AC, except where noted) to contralateral than ipsilateral stimulation are designated by red/yellow shading.

More finely parcellated “sectional” ROI analyses, agnostic to any hypotheses about areal distribution of AC sensitivity to auditory space, were also conducted to allow for more precise localization of regions of binaural sensitivity. STG and HG regions were sectioned into evenly distributed non-overlapping areas for each individual functional dataset. Custom Matlab routines were used to define the most anterior and posterior voxels encompassing STG and this range subdivided into 10 equal sections. A similar procedure was carried out in HG by defining the medial-lateral range and subdividing into six sections. Beta weights corresponding to sound-responsive voxels within each section were averaged together for each ILD or ITD condition. The result of this analysis was an average beta weight for each subject, stimulus condition, and section. Subsequent statistical analyses of these measures were run across subjects.

For voxel-wise mapping analyses (Fig. 4), the first-level results were combined across runs for each subject by implementing higher-level fixed-effects analyses in FEAT. Each subject’s cross-run 3-D functional data were projected to his/her individual 2-D cortical surface in Freesurfer and then morphed onto the template FSaverage 2-D surface. Once the data were in a common 2-D space, Gaussian spatial smoothing (5mm FWHM) was applied, and a higher-level random-effects group GLM analysis implemented in Freesurfer on the 2-D surface. Except where noted, results are displayed on the FSaverage brain at p < 0.05, corrected for multiple comparisons based on false discovery rate (FDR) (Genovese et al. 2002) across either the whole brain or across right and left hemisphere AC ROIs (defined on FSaverage by merging the superior temporal and transverse temporal gyrus regions specified in the Desikan-Killiany atlas).

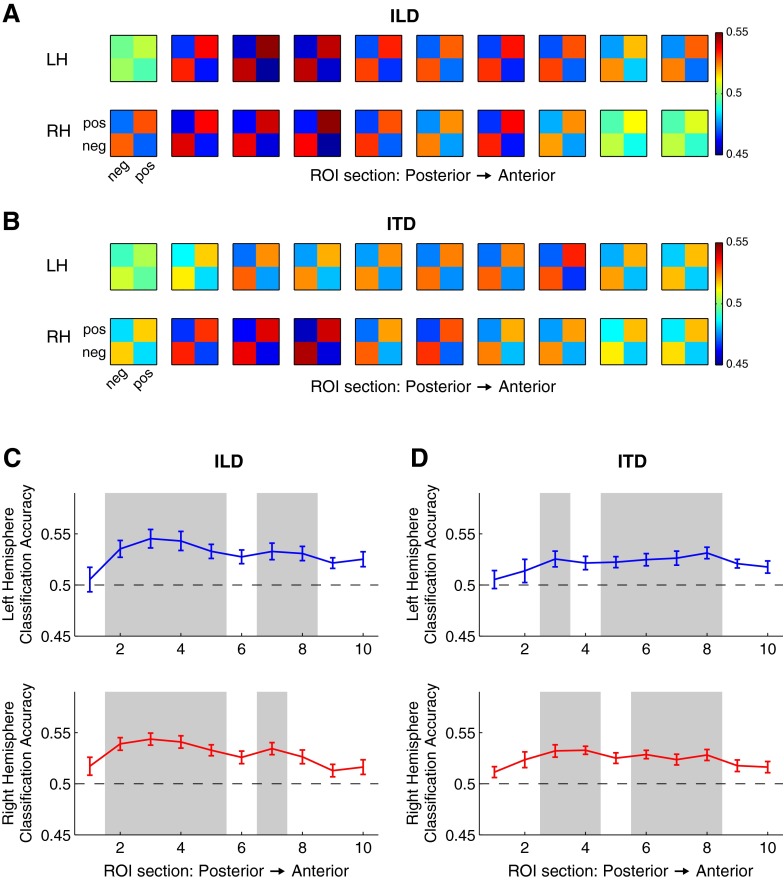
Lastly, in order to identify reliable ILD- or ITD-dependent patterns of voxel activity not described by simple increases in average voxel response, a mulitvoxel pattern analysis (MVPA) was implemented (Fig. 6). For each subject, hemisphere, and ROI subsection, trial-by-trial voxel patterns were extracted and subjected to principal component analysis (PCA). The first eight principal components were retained,2 resulting in an eight-element vector describing the activation pattern on each trial. These data were then subjected to cross-validated classification analysis. Half of the trials were randomly selected and used to train a linear support-vector machine (MATLAB svmtrain) on left-versus-right stimulus classification. Classification performance was assessed using the other (non-trained) half of trials (MATLAB svmclassify). This procedure was repeated for 1000 iterations, with random assignment of trials to training and classification datasets on each iteration. Sets of “leftward” and “rightward” stimuli each included the three most unambiguous values of each cue type (±10, 20, 30 dB ILD or ±200, 400, 800 μs ITD). Classification performance was averaged across iterations and subjects to produce mean confusion matrices (Fig. 6A, B) and metrics of classification accuracy (percent correct; Fig. 6C, D). Classification accuracy was evaluated statistically via 1000-fold permutation test (Nichols and Holmes 2002). Briefly, the sampling distribution of classification performance under the null hypothesis (50 % correct) was estimated by conducting an additional 1000 iterations per subject, in which classifiers were trained on multivoxel patterns after random reshuffling of target labels. The inter-subject mean was computed for each iteration to estimate the sampling distribution of mean classification accuracy in each ROI section. The proportion of iterations with performance equal to or better than the actual mean score (i.e., the p value) was then computed and assessed for statistical significance using the procedure of Benjamini and Hochberg (1995) to control FDR at 0.05 across all 40 comparisons (10 ROI sections × 2 hemispheres × 2 cues).

FIG. 6.

Multivoxel pattern classification across ILD and ITD in sectional STG ROIs. Confusion matrices representing probability of classification for rightward (positive) and leftward (negative) A ILD and B ITD, averaged across subjects. Matrices are arranged by ROI sections from posterior (left column) to anterior (right column). Color represents probability of classification, with chance at 0.5. Mean classification accuracy for C ILD and D ITD averaged across subjects as a function of ROI section for left (blue) and right (red) hemispheres. Error bars represent ±1 SEM; shaded areas signify significant difference from chance performance (permutation test, FDR < 0.05).

RESULTS

Univariate BOLD Tuning to ILD and ITD

Response Functions in pSTG, HG, and aSTG

BOLD tuning functions for ILD and ITD are plotted across ROIs in Figure 2B. Responses to “silent” trials are plotted on the right side of each panel. Paired t tests indicate that group average response to sound (averaged across all binaural cue values) was significantly greater (p < 0.00001) than response to silence in STG for both cues, in both LH and RH (for ILD: LH t₉ = 23.73, RH t₉ = 15.04; for ITD: LH t₉ = 16.65, RH t₉ = 10.49). Hemispheric tuning to binaural cue levels (i.e., contralateral bias) was assessed for significance using paired t tests between group average responses to particular binaural cue values. “Inter-hemispheric” contrasts compared LH and RH responses to a given cue value (significant differences represented by asterisks along the top border of each panel in Figure 2B), and “intra-hemispheric” contrasts compared responses within a given hemisphere to matched cue values across left and right hemispace (significant differences represented by horizontal lines within each panel in Figure 2B). Numerical results are displayed in Table 1.

TABLE 1.

Average group BOLD response to varied ILD and ITD values, and inter- and intrahemispheric comparisons of responses. Mean beta-weight response across sound-responsive voxels to leftward (negative values) and rightward (positive values) sounds in pSTG, HG, and aSTG ROIs, and results of paired-sample t tests for between- (inter) and within- (intra) hemisphere comparisons of responses to binaural cues

			ILD						ITD
			−30	−20	−10	10	20	30	−1500	−800	−500	500	800	1500
pSTG	Mean response	LH	441.5	383.8	394.4	434.5	498.2	565.5	477.7	444.6	434.3	497.4	514.4	477.1
		RH	566.6	483.8	457.5	417.1	423.0	463.7	461.3	431.9	417.5	416.9	419.4	404.7
	LH v RH (inter)	t (9 df )	2.961	2.708	1.919	0.543	2.241	2.751	0.599	0.577	0.640	3.485	3.916	3.152
		p	0.016*	0.024*	0.087	0.600	0.052	0.022*	0.564	0.578	0.538	0.007*	0.004**	0.012*
	Contra v ipsi LH (intra)			±30 v 30		±20 v 20		±10 v 10		±1500 v 1500		±800 v 800		±500 v 500
		t (9 df )		3.312		2.786		2.689		0.021		4.018		1.688
		p		0.009*		0.021*		0.025*		0.984		0.003**		0.130
	RH (intra)	t (9 df )		2.413		1.778		2.175		2.328		0.954		0.014
		p		0.039*		0.109		0.058		0.045*		0.365		0.989
HG	Mean response	LH	402.2	366.9	335.4	430.5	490.0	564.3	442.3	388.6	380.4	468.8	417.8	424.3
		RH	451.6	426.1	363.6	354.2	351.6	371.1	381.9	358.6	344.3	364.0	341.4	374.8
	LH v RH (inter)	t (9 df )	0.562	0.650	0.367	0.924	1.416	2.023	0.845	0.399	0.464	1.508	0.969	0.603
		p	0588	0.532	0.722	0.380	0.190	0.074	0.420	0.700	0.653	0.166	0.358	0.561
	Contra v ipsi LH (intra)			±30 v 30		±20 v 20		±10 v 10		±1500 v 1500		±800 v 800		±500 v 500
		t (9 df )		3.099		2.711		3.128		0.562		1.182		2.313
		p		0.013*		0.024*		0.012*		0.588		0.267		0.046*
	RH (intra)	t (9 df )		1.895		1.653		0.494		0.280		1.405		0.415
		p		0.091		0.133		0.633		0.786		0.194		0.688
aSTG	Mean response	LH	325.6	309.1	296.5	338.1	334.7	381.5	242.5	197.4	197.5	244.7	210.6	202.6
		RH	281.8	266.8	253.8	239.5	216.7	247.4	237.4	220.6	225.6	214.0	246.6	184.2
	LH v RH (inter)	t (9 df )	0.989	1.198	1.053	2.726	2.579	3.055	0.118	0.481	0.460	0.603	0.517	0.367
		p	0.348	0.262	0.320	0.023*	0.030*	0.014*	0.909	0.642	0.656	0.561	0.618	0.722
	Contra v ipsi LH (intra)			±30 v 30		±20 v 20		±10 v 10		±1500 v 1500		±800 v 800		±500 v 500
		t (9 df )		1.314		0.649		3.208		1.175		0.486		1.785
		p		0.221		0.532		0.011*		0.270		0.638		0.108
	RH (intra)	t (9 df )		0.821		1.310		0.627		3.720		1.209		0.303
		p		0.433		0.223		0.546		0.005**		0.257		0.769

Significant t statistics are denoted by italics

∗p < 0.05; ∗∗p < 0.005

The response-ILD curves in Figure 2B demonstrate contralaterally biased responses in left and right pSTG and in left HG, with some additional ILD tuning evident in left aSTG (although aSTG response levels were consistently lower than those in HG and pSTG). Inter-hemispheric contrasts in pSTG reveal contralateral ILD tuning in both hemispheres with RH responding more than LH to leftward −30 and −20 dB, and LH more than RH to rightward +30 dB. In HG, there were no significant differences between LH and RH responses for any of the ILD values presented, while in aSTG, there was a greater LH than RH response for all rightward ILD values. The intra-hemispheric contrasts for ILD also indicated contralateral tuning, primarily in LH. LH responses to rightward ILD stimuli were significantly greater than to leftward stimuli at all levels tested in pSTG and HG, and also in aSTG for +10 > −10 dB. In contrast, a significantly greater RH response to leftward than rightward stimulation was seen only in pSTG for the −30 > +30 dB contrast. The ILD functions observed in HG and pSTG are consistent with ILD data previously collected using a sparse imaging paradigm (Stecker et al. 2015). Also consistent with those previous results, ILD-response functions were non-monotonic, growing with increases in both contralateral and ipsilateral ILD. In both studies, contralateral bias to ILD was greater in LH than RH. Although Stecker et al. (2015) found response minima for moderate ipsilateral ILD values to be 10–20 dB, response-ILD minima in the present study are close to 0 dB ILD.

In contrast to the results for ILD, response-ITD functions did not demonstrate clear tuning to ITD except in left pSTG (and to a lesser extent left HG), where responses exhibited a positive monotonic relationship to increasingly contralateral ITD cues. Inter-hemispheric contrasts showed greater responses in left than right pSTG to rightward ITD values of +1500, +800, and +500 μs, while neither right pSTG nor HG responded significantly more to leftward sound than did their LH homologues. Intra-hemispheric contrasts for ITD in LH were significant at particular cue values in pSTG (rightward +800 μs > leftward −800 μs,) and HG (+500 > −500 μs). In RH, no inter-hemispheric contrasts reached significance, and intra-hemispheric contrasts were significant only for −1500 > +1500 μs, in both pSTG and aSTG. It should be noted that ITD values of ±1500 μs lie beyond the physiological range of delays for direct sounds for humans; such stimuli may thus be perceived in a categorically different manner than sounds with smaller ITDs.

Sectional ROI Analyses

Heat maps of average BOLD response to each ILD and ITD condition within smaller, evenly distributed ROI sections in HG and STG are displayed in Figure 3. The main effect of response modulation by cue is further summarized by repeated-measures analyses of variance (ANOVA) with binaural cue value as the single factor; the resulting F statistics are plotted against section to the right of each corresponding heat map. The ANOVA results indicate a significant main effect of ILD (critical F value: F_8,72 = 3.06, p = 0.005) in all six sections of left HG, although the strongest effects were in sections 3 and 4, situated centrally along the medial/lateral axis of HG. In both left and right HG, the strongest responses were to contralateral 30 and 20 dB ILD; however, they were relatively weaker in RH than LH, crossing a significance threshold of p < 0.05 but not reaching the Bonferroni corrected threshold of p < 0.005. In STG, there was a significant effect of ILD (p < 0.005) in both hemispheres in posterior/central areas (sections 2–6) and this effect was contralaterally tuned, as evidenced by the heat maps. Greater response modulation by ILD was observed in left than in right STG.

For ITD stimulation, though there were robust sound-driven responses in both left and right lateral HG3 (sections 5 and 6), the main effect of ITD modulation showed a significant trend only in LH, exceeding a p < 0.05 threshold in individual sections in both HG and STG, but never reaching the corrected threshold of p < 0.005. It is worth noting that the ROI section in left HG (section 4) displaying the largest main effect for ITD was the same section that exhibited a maximum main effect of ILD. Section 4 (and 3) roughly cover the central portion of HG, which in nearly all HG parcellation schemes corresponds to core or primary auditory cortex (see Baumann et al. 2013). A similar pattern was seen in specific posterior sections of left STG (sections 3–5) in which the largest main effect was seen for both ILD and ITD stimulation. Posterior areas of STG have been hypothesized to correspond to belt or parabelt regions (reviewed in Nourski et al. 2014) analogous to those in the non-human primate model proposed by Hackett et al. (1998) and Kaas and Hackett (2000). This posterior distribution of sensitivity to binaural cue modulation supports the hypothesis that posterior AC is specialized for spatial processing (Rauschecker and Tian 2000; Warren and Griffiths 2003; Ahveninen et al. 2006).

Voxel-Wise Response Maps

Suprathreshold voxel responses obtained from group GLM analyses are displayed on the cortical surface in Figure 4. Uncorrected whole-brain maps of overall response collapsed across all binaural-level combinations (Fig. 4A) show that sound-evoked responses were essentially confined to HG and surrounding regions of STG (i.e., putative human AC) in both experiments. Figure 4B–D plots statistical contrast maps within left and right AC ROIs, comparing responses to contralateral versus ipsilateral sound stimulation at each binaural cue value. Figure 4E (“Contra3>Ipsi3”) contrasts the average response to the three leftward-most physiologically realistic cue values versus the three rightward-most cue values (for ILD: ±30, 20, 10 dB; and for ITD: ±800, 500, 200 μs). The contrast maps, despite reduced sensitivity compared to ROI-based averaging analyses, are consistent in showing contralateral tuning to ILD (particularly at extreme values of ±30 dB ILD) in both hemispheres, primarily in posterior superior temporal regions, but more modest contralateral ITD tuning only in LH, at +800 > −800 μs but not +1500 > −1500 μs.

Comparison of Contralateral Bias Across Cue Type

The present univariate analyses in pSTG show BOLD tuning to both ILD (in LH and RH) and ITD (only in LH), with greater contralateral bias to ILD. Maximal response modulation was observed in the same cortical regions for both cues, notably sections 3–5 of STG, immediately posterior to HG and presumably corresponding to planum temporale (PT). It cannot be ascertained with certainty whether these data represent an underestimate of ITD sensitivity, an overestimate of ILD sensitivity, or a fundamental difference in cortical representations of the two cues. To our knowledge, this is the first study to directly and quantitatively compare tuning to ITD and ILD using fMRI. The two most equivalent previous studies of AC BOLD sensitivity to static ITD values (Krumbholz et al. 2005a; von Kriegstein et al. 2008) did not report response magnitudes that can be directly compared to the current data. Statistical contrasts from these studies, however, suggest that—as in the present data—responses varied weakly but significantly across ITD. Krumbholz et al. (2005a) reported no significant effect of static ITD (±500 μs) except within a restricted region of PT and at a relatively lenient voxel-wise criterion (t = 3.09, p < 0.001 uncorrected); the peak activated voxel gave t = 3.65 in left PT and t = 4.42 in right PT. Similarly, von Kriegstein et al. (2008) reported significant contralateral responses to ±500 μs versus 0 μs ITD with peak voxel responses of Z = 3.0 in left PT and Z = 3.75 in right PT. Bearing in mind the differences of peak-voxel versus ROI-averaged responses, the present results in left pSTG (t₉ = 1.7 and t₉ = 4.0 at ±500 and ±800 μs ITD, respectively) are not inconsistent with this literature.

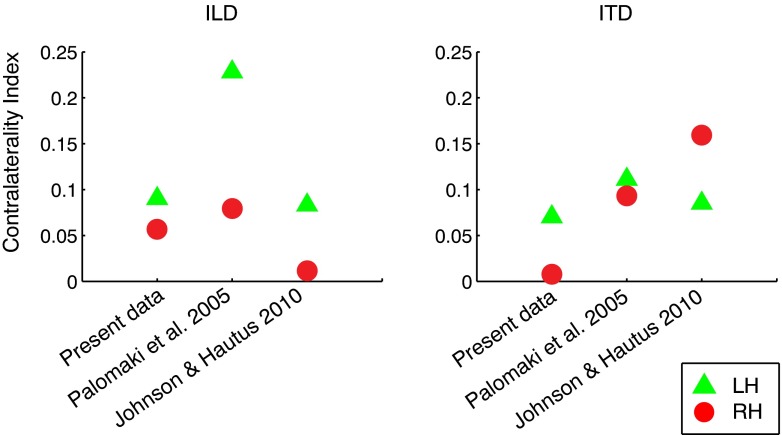
Two additional studies have used MEG to quantitatively compare human AC tuning to ITD and ILD. Palomäki et al. (2005) presented broadband noise bursts with ITD or ILD cues corresponding to a range of sound azimuths that included 0 °, ±45 °, and ±90 ° (ITD values = 0, ±390, ±700 μs; ILD values ≃0, ±11, and ±12 dB). Johnson and Hautus (2010) presented narrowband noise bursts (centered at 600 Hz) with ±500 μs ITD or with ILD values adjusted to produce subjectively similar lateralization. Both studies reported the amplitude of the N1m/M100 evoked response across ITD and ILD values. Although the units are not directly comparable to BOLD response magnitudes, they can be converted to a meaningful index of normalized contralateral bias, which we define as

$$\mathrm{C}\mathrm{I}=\left(c-i\right)/\left(c+i\right)$$ 1

where c and i are response magnitudes to matched contralateral and ipsilateral stimuli, respectively. CI values can range from −1 (ipsilateral bias) to +1 (contralateral bias), with CI = 0 indicating no lateral bias (c = i). CI values computed from the present data (in pSTG) are plotted alongside corresponding values from the two MEG studies in Figure 5, and reveal roughly similar magnitudes in the range 0.05–0.2. For ILD, all three studies show greater contralateral bias for LH than RH responses. For ITD, the results are less consistent; although CI values in LH were quite similar across studies, RH values were quite variable. That difference may be due in part to methodological differences (e.g., MEG versus. fMRI, inclusion of a spatial task by Johnson and Hautus but not others) or could suggest greater variability in the organization of ITD-sensitive activity in RH. For example, ITD sensitivity could be limited to a restricted subregion of right pSTG that varies in position and orientation across listeners. The results of Krumbholz et al. (2005a) and von Kriegstein et al. (2008), which—in contrast to the present data—reported slightly greater t statistics and Z scores in RH than in LH for peak voxels, lend support to this idea.

FIG. 5.

Contralaterality indices across studies comparing cortical response magnitudes for ILD and ITD. Normalized contralateral bias or contralaterality index (CI) is defined as CI = (c − i) / (c + i), where c and i = response magnitudes to matched contralateral and ipsilateral stimuli, respectively. CI ranges from −1 (complete ipsilateral bias) to +1 (complete contralateral bias). CI values for the present study are plotted for pSTG data (left), along with MEG data from Palomäki et al. (2005, center) and Johnson and Hautus (2010, right). Green triangles and red circles indicate values for left and right hemispheres, respectively.

Multivoxel Pattern Classification of ILD and ITD

One possible scenario limiting apparent ITD sensitivity would be if divergent responses to ITD were finely distributed across AC neurons. In this case, it would be possible for single voxels or regions to encompass an amalgam of responses that both increase and decrease with ITD such that the voxel-wise average response appears insensitive to ITD. Alternatively, weak neural and voxel-wise sensitivity to ITD modulation could hide systematic changes in the pattern of responses across voxels with ITD. In either case, voxel-wise univariate average response magnitudes would not reveal sensitivity to ITD, but the response pattern would. To investigate this possibility, linear support vector machines (SVM) were trained to classify trial-to-trial response patterns according to ITD or ILD within each sectional ROI in STG (identical sections as in Figure 4). Mean classification of leftward (negative) versus rightward (positive) cue values is illustrated by confusion matrices in Figure 6A, B, for each hemisphere and ROI section, illustrating the degree to which contralateral and ipsilateral stimuli evoke distinct response patterns. Confusion matrices are plotted according to posterior-to-anterior position along STG. For both cues, clear classification is apparent in most of the middle (2–8) sections, but reduced in far posterior (1) or anterior (9 and 10) sections. Panels C (ILD) and D (ITD) of Figure 6 plot the corresponding classification accuracy (mean proportion correct ±1 s.e.m.), also averaged across listeners, as a function of ROI section for left (blue) and right (red) hemispheres. Shaded areas indicate sections exhibiting significantly better-than-chance classification, based on permutation tests and controlling for FDR of 0.05.

DISCUSSION

Differing Patterns of Tuning to ILD and ITD in the AC BOLD Response

Contralateral ILD tuning was observed in pSTG of both hemispheres, consistent with neurophysiological findings in mammalian AC (Phillips and Irvine 1981; Zhang et al. 2004; Campbell et al. 2006; Higgins et al. 2010; Lui et al. 2015), and in a limited number of human neuroimaging investigations (Ungan et al. 2001; Palomäki et al. 2005; Stecker et al. 2015). The enhanced contralateral bias to ILD in left versus right pSTG replicates our previous study (Stecker et al. 2015), although to a greater degree given that contralateral bias in HG was observed only in LH in the present data.

ILD tuning may be partly mediated by monaural as well as binaural effects. Intensity-dependent responses to contralateral monaural stimulation contribute, for example, to many AC neurons’ preference for sounds on the acoustical axis of the contralateral pinna (Harrington et al. 2008) and to ILD sensitivity in human AC (Stecker et al. 2015). But evidence suggests that true binaural interactions also contribute. The U-shaped response-ILD curves observed in the present study and in Stecker et al. (2015) argue against a strictly contralateral monaural response. Moreover, studies in human listeners have consistently revealed stronger BOLD responses to contralateral monaural stimulation than to binaural stimulation of matched intensity, indicating inhibition of the BOLD response by ipislateral stimulation (Jäncke et al. 2002; Krumbholz et al. 2005b; Langers et al. 2007; Stecker et al. 2015). Additionally, Salminen (2015) observed robust cortical adaptation to ILD that could not be attributed to monaural level effects. These data suggest that the ILD tuning observed in the present study is based in part on binaural processes.

Contralateral preference for ITD in the univariate response was less clearly evident than for ILD, although statistically comparable to past studies (Palomäki et al. 2005; Krumbholz et al. 2005a; von Kriegstein et al. 2008; Johnson and Hautus 2010, see “Comparison of Contralateral Bias Across Cue Type”). Univariate analyses revealed ITD tuning only in left pSTG and (to a more limited extent) left HG, where BOLD responses grew monotonically with contralateral ITD across the range of physiologically plausible values (≤800 μs). These results appear consistent with neurophysiological data in gerbils demonstrating a uniform distribution of preferred ITDs in primary auditory cortex (A1), in contrast to lateralized ITD tuning in the inferior colliculus (Belliveau et al. 2014). Consistent with the hemispheric asymmetries in the present data, Belliveau and colleagues reported a higher percentage of ITD-responsive cells in left (65 %) than right (46 %) A1, although no contralateral bias was observed in either hemisphere. Other studies have demonstrated greater spatial sensitivity in posterior fields as compared to A1 (Stecker et al. 2003; Woods et al. 2006), consonant with the current finding of contralateral ITD tuning primarily in pSTG. RH responses failed to exhibit clear univariate tuning to ITD overall, yet features of the RH data do suggest some form of ITD sensitivity. RH responses significantly differed between ±1500 μs ITD, demonstrating sensitivity to binaural spatial features (e.g., apparent source width), if not to physiologically plausible correlates of location (see von Kriegstein et al. 2008).

Multivoxel analyses demonstrated better than chance classification of ITD-mediated sound location in multiple regions of STG in both hemispheres. In no case did performance approach perfect classification, suggesting that ILD- and ITD-dependent patterns remain partly obscured by other factors such as responses to other stimulus features, within-voxel mixtures of tuned and untuned responses, BOLD signal noise, and limitations of the PCA+SVM classification procedure (i.e., other approaches might be more sensitive). Nevertheless, above-chance performance indicates significant binaural information in the BOLD response pattern. That is, BOLD responses were, in fact, sensitive to ITD. In LH, that result is consistent with the ITD sensitivity that was observed with univariate analyses. In RH, univariate analyses did not reveal ITD sensitivity, suggesting that heterogeneous populations of ITD-selective responses are sufficient to subserve left/right classification in RH but comingled within closely clustered AC voxels and therefore not resolved via univariate analyses (Kamitani and Tong 2005; Haynes and Rees 2005). Thus, sensitivity to ITD (particularly in RH) might not reflect whole-population tuning to contralateral versus ipsilateral sound, but a more distributed representation with diverse response functions. That representation could take several potential forms. For example, the population could comprise a truly unbiased sample of preferred ITDs, either through uniform sampling by sharply tuned responses (Belliveau et al. 2014), coarse sampling by balanced contralateral and ipsilateral populations, or coding of location by response timing rather than magnitude (Furukawa and Middlebrooks 2002). Alternatively, strong spatial biases could be present in the population but obscured by the nature of the stimulus. For example, AC neurons with sharp frequency tuning might be driven by “slipped-cycle” interaural phase differences that disagree with the overall broadband ITD, or different spatial preferences for envelope and fine-structure cues might obscure sensitivity to the overall ITD when voxels contain populations responsive to both temporal configurations of ITD. Future research could aim to differentiate these possibilities using techniques like multivoxel analyses paired with careful stimulus design to isolate the contributions of spatially biased subpopulations.

Other factors could conceivably have led to underestimating the true degree of ITD tuning, particularly in RH. Stimulus history effects—i.e., the effect of prior stimulation on the current stimulus response—may be particularly influential in RH, in which increased sensitivity to novel or unexpected “oddball” stimuli has been observed across modalities (Downar et al. 2000; Stevens et al. 2005; Corbetta et al. 2008; Kucyi et al. 2012). In the present study, first-order stimulus history effects were controlled so that each stimulus condition was preceded by every other condition on an equal number of trials (Aguirre 2007). As reported in the first author’s Ph.D. dissertation (McLaughlin 2013), such effects do alter the responses to subsequent sound in a binaurally specific manner. Future studies which aim to directly capture responses to sound location change might reveal greater RH sensitivity.

Another way in which ITD tuning may have been underestimated is that highly selective (sharply tuned) voxels could have been excluded from ROI analyses defined by overall sound response (although follow-up analyses did not reveal clear evidence of greater tuning in such voxels). Additionally, the combined sensitivity to both cues (ITD and ILD) could have biased responses in the direction of the fixed 0-dB ILD. High-frequency components of the broadband ITD stimuli could be particularly relevant in this regard, although low-frequency ITD cues are thought to dominate (Macpherson and Middlebrooks 2002). A control experiment by McLaughlin (2013) removed those components by low-pass filtering but still failed to detect robust ITD tuning. A final consideration is that tuning to ITD in human AC could also depend on top-down or context-dependent influences. Several neuroimaging studies have reported contralateral bias to ITD when listeners engaged in a spatial task (Johnson and Hautus 2010) or listened to virtually moving stimuli (Krumbholz et al. 2005a, 2007), but only weak contralateral bias has been observed for static ITD (Krumbholz et al. 2005a; Palomäki et al. 2005). Spatial representations in AC may also be significantly altered by the grouping of auditory objects or “streams” (Middlebrooks and Bremen 2013; Salminen et al. 2015b). Future work should aim to address these issues directly.

Cortical Representations of ILD and ITD

Joint Versus Separate Cortical Processing of ILD and ITD

The areal distributions of AC BOLD sensitivity to ILD and ITD included regions of pSTG and HG, consistent with the hypothesis of spatial specialization in posterior AC (Rauschecker and Tian 2000; Stecker et al. 2003; Warren and Griffiths 2003; Krumbholz et al. 2005a; Ahveninen et al. 2006; Deouell et al. 2007; von Kriegstein et al. 2008). Sectional ROI analyses indicated that, at least in LH, sensitivity to both cues colocalized to the same regions of posterior/central STG and central HG. That result is consistent with cue-independent processing of auditory space, although it cannot distinguish whether sensitivity arises from the same or different populations of ILD- and ITD-sensitive neurons within these ROI sections.

Despite the similar areal distributions of ILD and ITD sensitivity, however, differences in the pattern of results for the two cues suggest incomplete integration of ITD and ILD at the level of human AC. For example, response functions in pSTG were asymmetrically U-shaped in response to ILD, but relatively monotonic in response to ITD. Strong evidence for ILD tuning was observed in both univariate and multivoxel analyses. For ITD, however, evidence for univariate tuning was much weaker, and evident only in LH, yet multivoxel pattern responses revealed reliable classification of left versus right hemifield location in both hemispheres. The current results are thus in close agreement with studies demonstrating differences in cortical evoked responses to ITD and ILD (Schröger 1996; Ungan et al. 2001; Tardif et al. 2006; Johnson and Hautus 2010), and particularly with recent studies demonstrating that cortical representations of ITD and ILD are neither completely independent nor fully integrated (Edmonds and Krumbholz 2014; Altmann et al. 2014; Salminen et al. 2015c). These data thus beg the question: at what level of auditory processing are the cues integrated to form a single representation of auditory space? The evidence suggests that complete integration occurs very late, if at all, and perhaps not even within the auditory system per se. Behavioral advantages of this arrangement could include more flexible weighting of the cues across various environmental or multi-sensory contexts. Another possibility is suggested by analogy to vision: retinal inputs from the two eyes are not integrated early in the visual pathway because they do not redundantly encode locations in the visual field; rather, they are integrated via cortical mechanisms that are sensitive to binocular disparity. It is possible that disparities between ITD and ILD provide similarly useful information about the auditory scene (e.g., depth or reverberation).

Neural and Hemispheric Codes for ILD and ITD

The graded response increases observed with increasing contralateral ILD and ITD are consistent with a rate code for contralateral auditory space in AC (Werner-Reiss and Groh 2008), in which response magnitudes, rather than the locus of activity within a topographic map, encode binaural differences. For ILD, the asymmetrically U-shaped response functions observed additionally suggest both contralateral and ipsilateral contributions to BOLD responses to ILD (see Stecker et al. 2015), consistent with reports of broadly tuned contralateral and ipsilateral sub-populations of binaural AC neurons within each hemisphere (Imig and Adrián 1977; Stecker and Middlebrooks 2003; Nakamoto et al. 2004; Werner-Reiss and Groh 2008). These observations support an “opponent-channel” model in which ILD is encoded by response differences between contralateral and ipsilateral neurons (Wise and Irvine 1985; McAlpine et al. 2001; Phillips and Hall 2005; Stecker et al. 2005b). Specifically, the lateral symmetry of response-ILD functions in the two hemispheres suggests a four-channel model comprising a dominant contralateral and lesser ipsilateral channel within each hemisphere (Stecker et al. 2005b).

A four-channel representation of auditory space would seem to suggest that either hemisphere is capable of representing sound locations in both hemifields, and thus would predict few if any spatial deficits due to unilateral AC lesions. Yet experiments in animal models have consistently shown significant sound-localization deficits mainly in the hemifield contralateral to the affected hemisphere (Jenkins and Masterton 1982; Thompson and Cortez 1983; Kavanagh and Kelly 1987; Heffner 1997; Malhotra et al. 2004; Lomber et al. 2007). Because these studies have employed free-field stimulation, however, they do not differentiate the contributions of ILD and ITD processing, which the current results suggest may be distributed differently across hemispheres. To our knowledge, only one experimental lesion study has separately investigated the effects of unilateral AC lesions on ILD and ITD processing. Boester (1994) found that neither LH nor RH lesions significantly affected ILD discrimination in macaques. Likewise, human patients with unilateral damage in either hemisphere exhibit mild to no ILD-related lateralization deficits in clinical lesion studies (Bisiach et al. 1984; Spierer et al. 2009). Thus, it seems possible that either hemisphere is indeed capable of subserving ILD-based localization in both hemifields.

For ITD, response functions were monotonic and contralateral in LH, but essentially flat in RH. These results support the three-channel model proposed by Magezi and Krumbholz (2010), in which right AC contains both contralateral and ipsilateral ITD channels, and left AC only a contralateral channel. That model is consistent with human clinical lesion data in which LH damage is associated with generally mild localization deficits for ITD-mediated (or free-field) sound sources while RH damage is associated with localization deficits in both left and right hemispace (Altman et al. 1979; Ruff et al. 1981; Zatorre and Penhune 2001; Spierer et al. 2009). Although the flat univariate response across ITD values observed in RH would seem to suggest a lack of tuning to ITD, physiological data suggest that BOLD tuning might reflect a more uniform distribution of contralateral and ipsilateral responses in the neuronal population, which nevertheless is capable of subserving accurate decoding of ITD by opponent comparisons (Belliveau et al. 2014).

Taken together, the BOLD responses to ILD and ITD observed in the present study indicate (1) a relatively straightforward model for cortical representation of ILD by the responses of a larger contralateral- and a smaller ipsilateral-responding population within the AC of each cerebral hemisphere (Stecker et al. 2005b), and (2) a more complex model for ITD potentially involving multiple types of codes which may differ across hemispheres.

Conflict of Interest

The authors declare that they have no conflict of interest.