Skip to main content
PMC home page
Nature Communications logoLink to Nature Communications
. 2016 Jan 13;7:10343. doi: 10.1038/ncomms10343

Widely tunable two-colour seeded free-electron laser source for resonant-pump resonant-probe magnetic scattering

Eugenio Ferrari 1,2,*, Carlo Spezzani 1,3,*, Franck Fortuna 4, Renaud Delaunay 5, Franck Vidal 6, Ivaylo Nikolov 1, Paolo Cinquegrana 1, Bruno Diviacco 1, David Gauthier 1, Giuseppe Penco 1, Primož Rebernik Ribič 1, Eleonore Roussel 1, Marco Trovò 1, Jean-Baptiste Moussy 7, Tommaso Pincelli 8, Lounès Lounis 6,9, Michele Manfredda 1, Emanuele Pedersoli 1, Flavio Capotondi 1, Cristian Svetina 1,10, Nicola Mahne 1, Marco Zangrando 1,11, Lorenzo Raimondi 1, Alexander Demidovich 1, Luca Giannessi 1,12, Giovanni De Ninno 1,13, Miltcho Boyanov Danailov 1, Enrico Allaria 1,a, Maurizio Sacchi 6,14,b
PMCID: PMC4735510  PMID: 26757813

Abstract

The advent of free-electron laser (FEL) sources delivering two synchronized pulses of different wavelengths (or colours) has made available a whole range of novel pump–probe experiments. This communication describes a major step forward using a new configuration of the FERMI FEL-seeded source to deliver two pulses with different wavelengths, each tunable independently over a broad spectral range with adjustable time delay. The FEL scheme makes use of two seed laser beams of different wavelengths and of a split radiator section to generate two extreme ultraviolet pulses from distinct portions of the same electron bunch. The tunability range of this new two-colour source meets the requirements of double-resonant FEL pump/FEL probe time-resolved studies. We demonstrate its performance in a proof-of-principle magnetic scattering experiment in Fe–Ni compounds, by tuning the FEL wavelengths to the Fe and Ni 3p resonances.

Inline graphicTwo-colour X-ray free electron laser is a powerful tool for pump–probe measurements, but currently constrained by limited tunability. Here, Ferrari et al. develop a configuration that allows tuning both the pump and the probe to specific electronic excitations, providing element selectivity.

Free-electron laser (FEL) sources covering the wide spectral range from extreme ultraviolet to hard X-rays represent a breakthrough in photon science, with applications in physics, chemistry and biology. Many aspects of the spectral and temporal characteristics of the FEL pulses can be tailored to specific experimental needs by an accurate control of the lasing process, in the so-called beam by design approach1. The ability to run the FEL source in two-colour configuration, that is, to create two synchronized FEL pulses of differing wavelengths, has enormous potential for femtosecond time-resolved studies2,3 as it opens up unique opportunities for studying the dynamic response in atomic, molecular and solid state systems by selectively tuning electron resonances in atoms. As a consequence it has engendered major research4,5,6,7,8 and development9,10,11,12,13,14,15 efforts at all FEL facilities worldwide, with the ambition of attaining wide-ranging colour tunability and timing control.

Various two-colour schemes have been proposed, both for seeded2,9,10,14 and for self-amplified spontaneous emission (SASE)11,12,13 FEL sources. Initial configurations delivered two short FEL pulses with a controlled temporal separation in the range of a few hundred femtoseconds and a small photon wavelength separation (∼1%). Such configurations, where a single electron bunch generates the two FEL pulses, have served users for experiments both at seeded2 and at SASE11,12,13 facilities. In the case of SASE, differing photon wavelengths are obtained by dividing the radiator in two slightly detuned sections11. In the case of external seeding, the FEL wavelength separation is controlled by acting on the seed laser wavelength and by taking advantage of a residual controllable energy chirp on the electron beam2,10. For self-seeding schemes, it has been demonstrated14 that two seeded FEL pulses can be generated using two distinct Bragg diffraction lines in the self-seeding crystal recombined within the taper-tuned undulators. The possibility of producing two colours with a wider spectral separation (up to 30%) has been demonstrated recently at the SACLA hard X-ray SASE source by using the capabilities of a variable gap undulator13.

Until now, no configuration that generates two pulses with independently tunable wavelengths over a wide spectral range had been designed for externally seeded FELs. A whole new class of pump–probe experiments that require both pump and probe to be element selective is created by combining the full coherence of seeded FELs with a broad and independent tunability of the two colours.

Over the last decade, time-resolved studies made frequent use of short X-ray pulses as a probe that is coupled to an optical laser pump. Femto-slicing at synchrotrons16,17,18,19,20,21,22, high harmonic generation in gases23,24,25,26 and FEL sources27,28,29,30,31,32 deliver extreme ultraviolet and X-ray pulses with sub-100-femtoseconds duration that have been used for studying the ultrafast dynamics of magnetic16,17,18,19,20,21,22,23,24,25,26,27,29,31 and structural20,28,29,30 order in optical-laser pump/X-ray probe experiments. Tuning the wavelength to an atomic resonance provides the probe with element selectivity, which is of considerable interest especially for magnetic studies.

Developing FEL sources that can produce two pulses with independently selectable wavelengths for the pump and the probe and with a well-defined time separation obviously widens the potential of FEL radiation for studying the dynamics of a process and makes it possible to associate the pump energy to a specific electronic excitation of a given element. One field that will surely profit from this new tool is magnetization dynamics in 3d-transition-metal and rare-earth based oxides and compounds18,19,22,33,34,35,36,37: the presence of highly localized 3d and 4f orbitals and of mediated exchange interactions suggests that associating the pump energy to a specific electronic excitation will influence the magnetization dynamics profoundly, compared with using a non-resonant pump.

In the proof-of-principle time-resolved scattering experiment on Fe–Ni compounds described here, we use the new two-colour configuration of the externally seeded FERMI FEL source to generate, from the same electron bunch, two synchronized pulses with up to 30% spectral separation. The pump FEL pulse excites the Fe 3p→3d transition resonantly, while the second FEL pulse, tuned to the Ni 3p→3d resonance, probes the ultrafast Ni magnetization dynamics. The experiment successfully reveals the potential of this new source for investigating structural, electronic and magnetization dynamics in the fields of condensed matter as well as atomic and molecular physics.

Results

Two-colour seeded FEL with wide wavelength tunability

The experiment was performed at the FERMI facility38,39, which is a seeded FEL operated in the high-gain harmonic generation (HGHG) mode40,41. The chosen configuration (see Methods) provided a relatively long (∼1 ps) electron bunch interacting with a short (∼100 fs) ultraviolet laser pulse (seed laser) in the first undulator section called the modulator (Mod in Fig. 1a). As a consequence of this interaction, the electron beam energy is modulated with a periodicity imposed by the seed laser wavelength λseed. Following a magnetic chicane that works as a dispersive section (DS in Fig. 1a), the energy modulation is converted into a density modulation (bunching), which has strong harmonic components. Finally, in a second long undulator section called the radiator (Rad in Fig. 1a), the bunched electrons generate coherent FEL emission at one of the harmonics of the seed laser which is selected by setting the undulator gap. The advantages of HGHG with respect to SASE FEL stem from the fine control of the initial bunching, making it possible to generate FEL pulses with a high degree of longitudinal coherence42. Moreover, since only electrons interacting with the seed laser are bunched, this scheme provides a good control of the FEL temporal properties43.

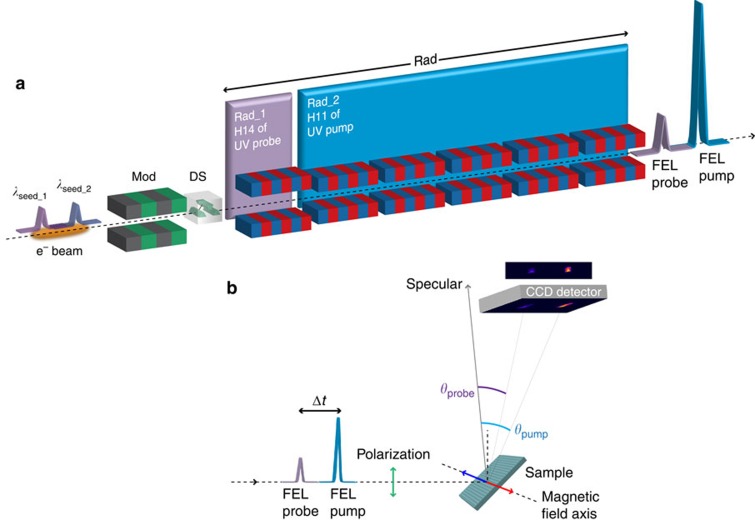
Figure 1. Schematic setup for a two-colour double resonance FEL experiment.

Figure 1

Open in a new tab

(a) Two-colour seeded FEL source configuration: the modulator (Mod), dispersive (DS) and radiator (Rad) sections of the FEL source are outlined. In the modulator section, two ultraviolet (UV) laser pulses of wavelength λseed_1 and λseed_2 delayed by Δt interact with the same electron bunch, imposing an energy modulation that is converted into density modulation in DS. The first radiator subsection Rad_1 is tuned to the 14th harmonic of λseed_1 and the second subsection Rad_2 is tuned to the 11th harmonic of λseed_2, generating the FEL probe and pump pulses, respectively (see Methods). (b) Magnetic scattering experiment: the two linear p-polarized FEL pulses reach the magnetic grating sample and diffract at different angles according to their wavelengths. The diffracted intensities are recorded by a two-dimensional detector (CCD camera). The wavelength separation between pump and probe is detected as a spatial separation at the CCD, while their time separation Δt is defined by the delay between the two seed pulses.

Two FEL pulses with a controlled delay can be produced by seeding the same electron bunch with two seed pulses2. Since in the HGHG seeding process the final FEL wavelength is determined mainly by λseed and it must be close to one of its harmonics, a way for delivering two-colour FEL pulses with very different wavelengths (>10% separation) relies on seeding the electron beam with two laser pulses and on sustaining the amplification process at both wavelengths independently (Fig. 1a).

To achieve this, some constraints have to be dealt with. Both seed wavelengths λseed_1 (for the probe) and λseed_2 (for the pump) have to modulate the electron energy in the interaction region efficiently so their separation must be within the modulator working bandwidth. The two seed pulses modulate the energy in distinct regions of the electron beam. For each region, the dispersive section converts the energy modulation into an electron density modulation that carries all the harmonic components of the corresponding seed wavelength, either λseed_1 or λseed_2. The electron beam is now ready for the amplification of one of these harmonics, selected by the resonance condition of the radiator (undulator gap). A large separation between the two colours can be obtained by dividing the radiator into two subsections (Rad_1 and Rad_2 in Fig. 1a), one resonant at λFEL_1=λseed_1/m and the other at λFEL_2=λseed_2/n, with m and n integers. Since the radiator bandwidths are markedly narrower than the modulator one, we can emit efficiently the pump (or the probe) beam from one radiator subsection only, while suppressing its amplification in the other, selectively (see Methods). Finally, constraints on the temporal separation Δt between the two FEL pulses are set by the need to avoid interference between the laser seeds (lower limit) and by the electron bunch duration (upper limit). In the example reported below, we spanned delays ranging from 300 to 800 fs.

The Fe-3p resonant-pump and Ni-3p resonant-probe test experiment (Fig. 1b) used two FEL pulses tuned to λFEL_2=23.2 nm and λFEL_1=18.7 nm, corresponding to the 11th harmonic of λseed_2=255 nm and to the 14th harmonic of λseed_1=261.5 nm, respectively. To this purpose, a special configuration of the FERMI seed laser was implemented based on the combined use of two ultraviolet pulses originating from a common infra-red source through two separated generation channels. One made use of an optical parametric amplifier (OPA) for producing the 255 nm seed, the other of a third harmonic generation (THG) setup for the 261.5 nm seed. This approach made the twin seeding possible at two different ultraviolet wavelengths, one of them tunable via the OPA (see Methods). We tested different distributions of the six undulator modules over the Rad_1 and Rad_2 radiator subsections (see Fig. 1a). We obtained different power distributions between pump and probe by going from one module in Rad_1 and five in Rad_2 to three modules in each subsection. All the configurations provided satisfactory stable conditions for producing two-colour FEL pulses. Since in our test experiment the pump is required to be more energetic than the probe, five of the six available radiator modules were tuned to produce 23.2 nm pulses (Rad_2 in Fig. 1a), while the remaining module (Rad_1) was tuned to the probe wavelength. It was important, in this configuration, that Rad_1 was the first of the undulator modules, to prevent the smearing of the electron density modulation along the radiator section to degrade its performance. We verified also that one can switch readily the FEL pump and probe wavelengths, by reversing the time delay between OPA and THG generated seed pulses and inverting the gap settings of the Rad_1 and Rad_2 radiator subsections.

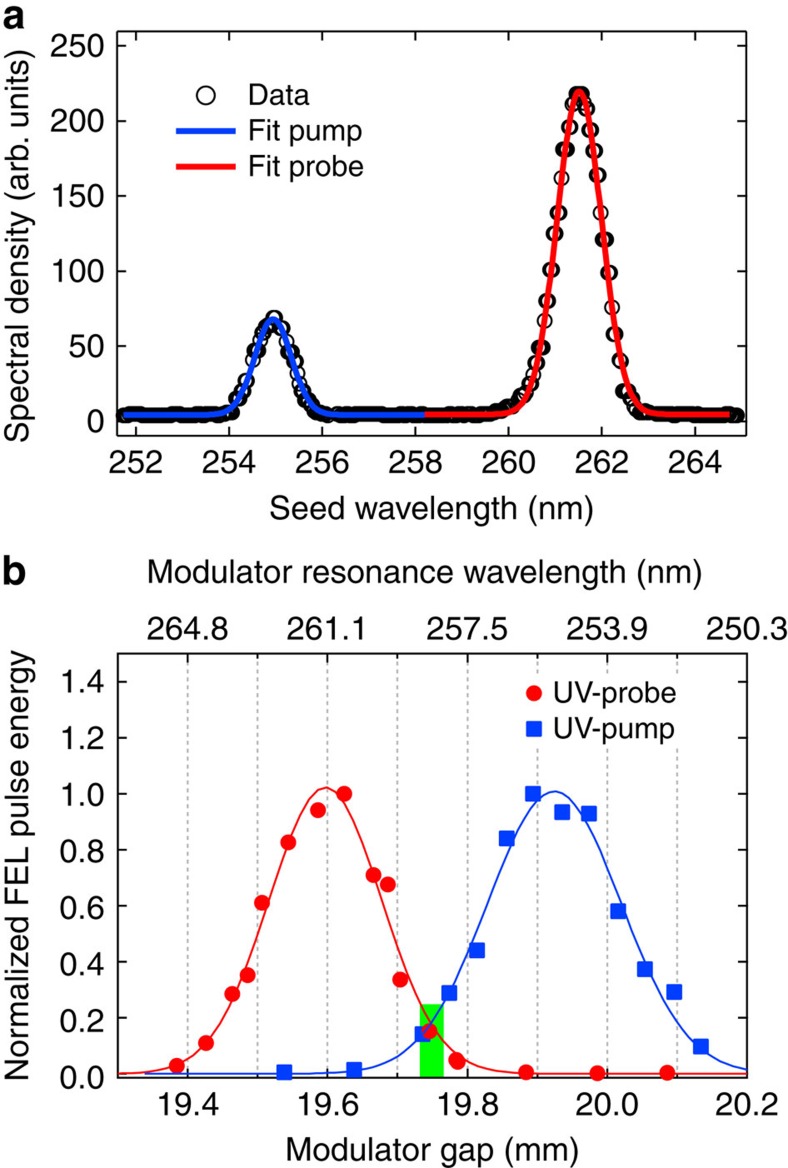
Figure 2a shows the spectral distribution of the two ultraviolet seed laser pulses and Fig. 2b shows the FEL pulse energy as a function of the modulator gap, when using only the ultraviolet -probe or only the ultraviolet -pump seeds. The two curves of Fig. 2b, which are normalized to the same amplitude, illustrate at each wavelength the extreme sensitivity of the FEL intensity to the modulator setting. A modulator gap of 19.94 mm optimizes the FEL pump emission when seeding at λseed_2, while a gap of 19.60 mm is best when seeding at λseed_1 to produce the FEL probe pulse. The gap can be used as an adjustable parameter for the fine control of the relative efficiency in the generation of the pump and probe FEL pulses, thanks to the ∼3% resonance bandwidth of the modulator (Supplementary Fig. 1). In our case, a good compromise was found at a gap of 19.75 mm, which made it possible to generate both λFEL_1=18.7 nm and λFEL_2=23.2 nm pulses, albeit with a reduced intensity. For the Fe–Ni experiment, the FERMI FEL source was characterized by pulse energies of up to ∼10 μJ at the pump wavelength and ∼1 μJ at the probe wavelength using these parameters. Once converted into a fluence F at the sample surface (see Methods), these values were sufficient to reach, in our experiment, the damage threshold and single shot detection conditions for the pump and the probe pulses, respectively.

Figure 2. Seed pulses and modulator setting.

Figure 2

Open in a new tab

(a) Spectral properties of the ultraviolet (UV) laser twin-seed source. Lines are Gaussian fits to the λseed_1=261.5 nm (red line) and λseed_2=255 nm (blue line) probe and pump contributions, respectively. (b) Modulator gap dependence of the FEL output for the two seed wavelengths. Circles and squares refer to seeding at 261.5 and 255 nm, respectively. Each point is the average of 100 consecutive FEL shots. Lines represent Gaussian fits to the intensity distributions. The curves are normalized to the same average maximum, showing that tuning the modulator gap to 19.75 mm (vertical green bar) makes it possible to seed with two colours simultaneously, preserving a fraction of the maximum pulse energy.

Resonant-pump/resonant-probe magnetic scattering experiment

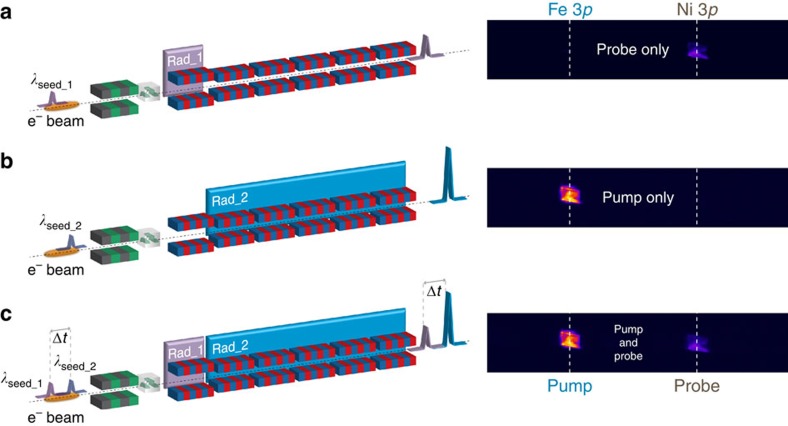
We tested the two-colour twin-seeded FEL source by studying the resonant-pump/resonant-probe magnetization dynamics in Fe–Ni samples, using the IRMA reflectometer44 installed at the DiProI beamline45,46. The samples were a 20-nm-thick permalloy (Ni0.81Fe0.19 alloy) film deposited on a Si grating and a 12.5-nm-thick NiFe2O4 layer epitaxially grown on MgAl2O4(001). Both samples were structured as line gratings with a period of ∼600 nm (see Methods). They worked as dispersive elements, separating different wavelengths at the level of the two-dimensional in-vacuum charge-coupled device (CCD) detector23,24. All Bragg peaks generated by the grating samples at different wavelengths fell within the angular acceptance of the detector and could be collected simultaneously (see Fig. 3).

Figure 3. FEL source configuration and scattering data recording.

Figure 3

Open in a new tab

Diffracted intensity from the 20-nm-thick permalloy grating sample at 46.3° incidence. Data are collected under different seeding conditions (schematics on the left) using a position-sensitive CCD detector (images on the right); the 1,025 × 202 pixel images correspond to 13.84 × 2.73 mm2 and cover ∼1.48° in scattering angle. (a) The λseed_1=261.5 nm laser pulse is sent through the modulator, turning on the Ni-3p resonant FEL emission at λFEL_1=18.7 nm in Rad_1 (14th harmonic) and no emission from Rad_2. (b) The λseed_2=255 nm laser pulse generates the Fe-3p resonant FEL emission at λFEL_2=23.2 nm in the radiator section Rad_2 (11th harmonic) and no emission from Rad_1. (c) Both seed laser pulses, delayed by Δt, interact with the electron bunch, generating Fe-3p resonant pump and Ni-3p resonant probe FEL pulses, also delayed by Δt.

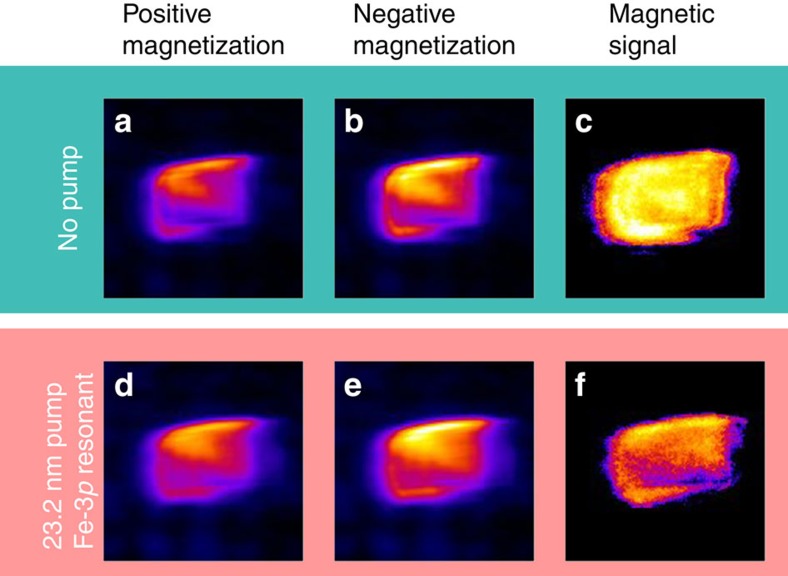
The FEL polarization was set to linear vertical to optimize the sensitivity to the sample magnetization in transverse geometry23,24,47,48,49, that is, with the external magnetic field applied normal to the scattering plane and parallel to the lines of the grating sample (see Fig. 1b). After an initial 80 mT magnetic pulse, the scattered intensity was collected in an applied field of 20 mT, guaranteeing the sample magnetic saturation (see Methods). In the following, the magnetic signal is defined as an asymmetry ratio, that is, as the difference between scattered intensities measured for opposite signs of the applied field divided by their sum, as shown in Fig. 4. At each given delay Δt, the Ni magnetic signal was measured as a function of the pump fluence F (see Methods for the relationship between FEL pulse energy and fluence at the sample). The pump wavelength was tuned either to the Fe-3p resonance (λFEL_2=23.2 nm) or off-resonance (λFEL_2=25.5 nm), the latter being obtained simply by tuning the radiator subsection Rad_2 to the 10th harmonic of the λseed_2 seed laser wavelength, instead of the 11th. It is worth underlining that, according to calculations based on tabulated optical constants50 (see also http://henke.lbl.gov/optical_constants/), the fraction of pump energy absorbed by the sample at 23.2 nm and at 25.5 nm differs by less than 2% for both permalloy and ferrite films.

Figure 4. Magnetic signal in the scattering data.

Figure 4

Open in a new tab

Diffracted intensity at the Ni-3p resonant probe wavelength with no pump (a–c) and following a Fe-3p resonant pump pulse (d–f). The pump fluence is F=8 mJ cm−2, the delay Δt is 450 fs. (a,d) and (b,e) Diagrams refer to a positive and negative saturating magnetic field, respectively. The magnetic signal, expressed as the asymmetry ratio, is shown in c and f. Each picture is 128 × 128 pixels, corresponding to 1.73 × 1.73 mm2.

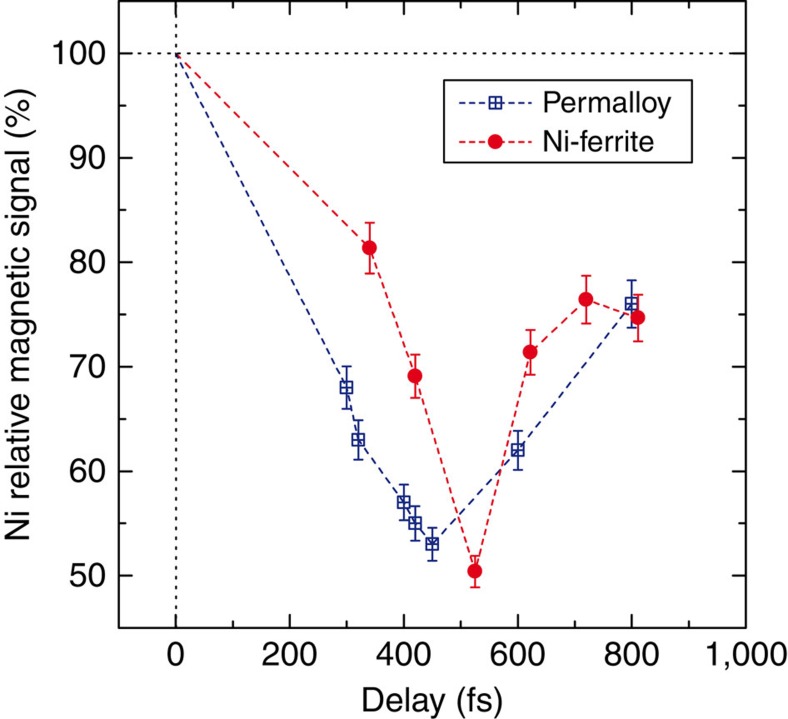
First, we explored the ultrafast Ni demagnetization while varying the delay Δt between the FEL probe and pump by adjusting the delay between the corresponding seed laser pulses. An example of delay dependence spanning the 300–800 fs range is shown in Fig. 5 where the Ni magnetic signal is reported after a Fe-3p resonant pump pulse with fluence F=10 mJ cm−2 (dots and squares refer to Ni-ferrite and permalloy samples, respectively). The asymmetry ratio in the Bragg peak intensity is calculated over a limited detector area of ∼100 × 100 μm2 to ensure homogeneous pump fluence and the Ni magnetic signal is normalized to its static value measured with no pump.

Figure 5. Time-dependent magnetic signal.

Figure 5

Open in a new tab

Ni demagnetization in the permalloy (blue squares) and the Ni-ferrite (red circles) samples at several delays Δt between probe and pump pulses (F=10 mJ cm−2). Vertical error bars represent s.d. (see Methods). The maximum fluctuation in the pump–probe delay over the measurement duration (±5 fs, see Methods) is smaller than the point width. Lines are a guide to the eye.

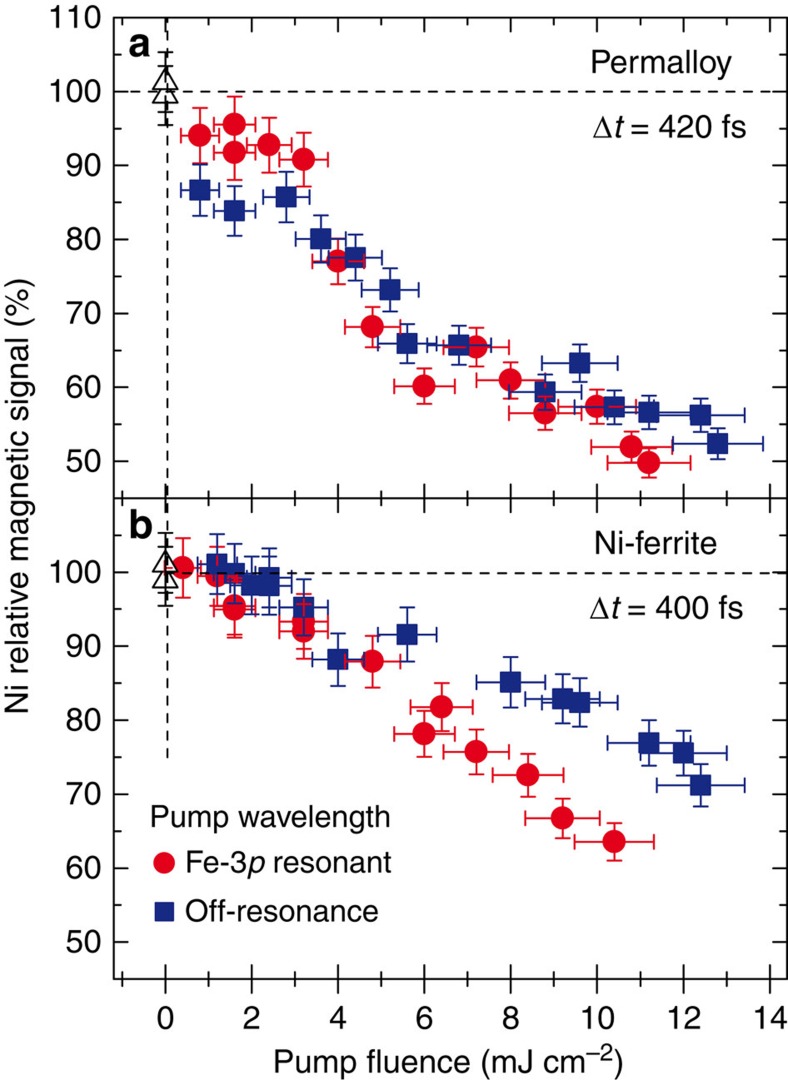
The main advantage of this novel two-colour scheme over those developed previously at the FERMI seeded source2 is its ability to tune both λFEL_1 and λFEL_2 to selected values over a broad range. It is also important to stress that this scheme makes the switching between on- and off-resonance pumping fast and easy. As mentioned before, this can be achieved simply by changing the gap of the Rad_2 radiator subsection for selecting a different harmonic of the λseed_2 wavelength. An example of on/off-resonance pumping is given in Fig. 6. It shows the Ni magnetic signal (normalized to its static value) measured at a fixed time delay of ∼400 fs for a FEL pump wavelength tuned to the Fe-3p resonance (λFEL_2=23.2 nm, red circles) or off-resonance (λFEL_2=25.5 nm, blue squares) as a function of the pump fluence F. The permalloy results (Fig. 6a) do not reveal a measurable effect of the pump wavelength: both curves show the same F-dependence of the Ni magnetic signal, which attains a ∼50% reduction at F∼10 mJ cm−2. On the contrary, pumping at the two on/off-resonance wavelengths results in an apparent difference in Ni demagnetization behaviour when F exceeds ∼5 mJ cm−2 in the case of Ni-ferrite (Fig. 6b).

Figure 6. Resonant versus non-resonant pumping.

Figure 6

Open in a new tab

Pump fluence dependence of the Ni demagnetization in permalloy (a) and Ni-ferrite (b) at ∼400 fs delay, comparing the results for Fe-3p resonant (λFEL_2=23.2 nm, red circles) and non-resonant (λFEL_2=25.5 nm, blue squares) FEL pump pulses. The Ni magnetic signal is reported as the asymmetry ratio in the Bragg peak intensity, normalized to the value measured with no pump (open triangles). Error bars represent s.d. (see Methods).

Although a detailed discussion of the results reported in Figs 5 and 6 is not within the scope of this communication, the observed differences between ferrite and permalloy behaviour can be ascribed to the direct hybridization of delocalized Fe and Ni 3d orbitals in ferromagnetic permalloy versus indirect exchange (via oxygen) of more localized 3d orbitals in ferrimagnetic NiFe2O4. These early results are intriguing and more studies are under consideration to shed light on the observed pump wavelength dependence.

Discussion

We have developed and tested a new FEL setup capable of delivering two-colour time-delayed pulses with independent wavelength tunability over a wide spectral range (18.7–25.5 nm). Combined with the seeded nature of the FERMI source39, this provides improved conditions for two-colour FEL experiments that require tuning both the pump and the probe to selected atomic resonances. The potential of this two-colour scheme has been demonstrated by a scattering experiment that probes the magnetization dynamics in systems containing two magnetic elements, Fe and Ni. Undoubtedly, it can find original applications in many other fields of condensed matter, atomic and molecular physics.

From a technical point of view, the solution that we propose is based on seeding the same electron bunch with two independent laser pulses and on splitting the FEL radiator into two subsections. On one hand, this solution offers the possibility of selectively tuning the two FEL colours over a very wide range. It may go well beyond the 30% bandwidth demonstrated here, by amplifying different harmonics of the seed wavelengths in each radiator subsection. On the other hand, using two laser seeds that modulate the same electron bunch, and two radiators impose some constraints on the relationship between the λFEL_1 and λFEL_2 wavelengths, both in terms of FEL intensity and of possible gaps in the range of wavelengths that can be spanned.

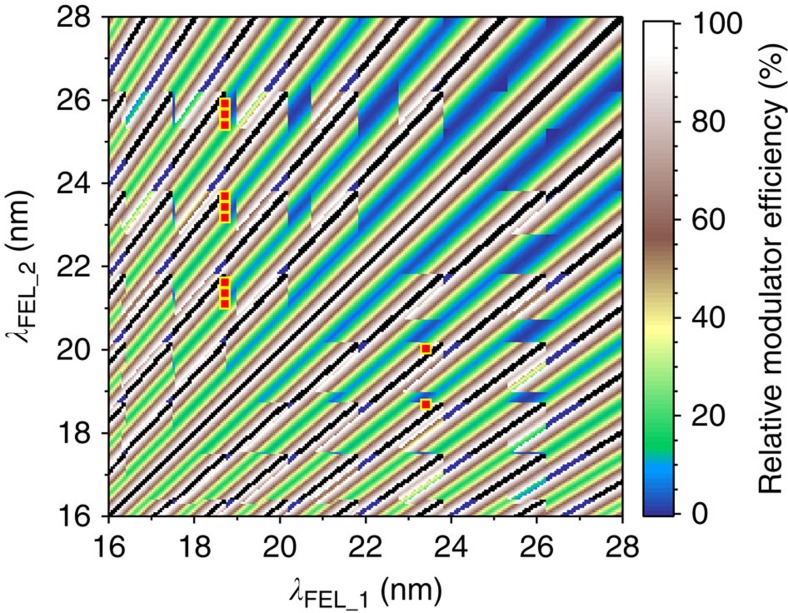
Figure 7 summarizes the calculated source performance when λFEL_1 and λFEL_2 span the 16–28 nm range, showing that marked intensity variations are present. The colour code represents the relative modulator efficiency for each couple of wavelengths, calculated assuming that the modulator resonance is set to the average value of λFEL_1 and λFEL_2. Both seed wavelengths are allowed to span the 228–262 nm range covered by the OPA, and radiator harmonics from 9 to 16 are considered. The finite modulator bandwidth defines the maximum intensity that can be obtained for each (λFEL_1, λFEL_2) combination, hence the efficiency of the two-colour process. The radiator bandwidth imposes limitations on the independent tunability of λFEL_1 and λFEL_2: black dots forming diagonal lines in Fig. 7 mark couples of wavelengths whose corresponding λseed values are close enough to be amplified in both radiator subsections. In this case, four FEL pulses, and not two, would be generated and the proposed two-colour scheme does not work properly.

Figure 7. Calculated seeding efficiency over the 16–28 nm range.

Figure 7

Open in a new tab

The colour code represents the relative modulator efficiency at λFEL_1 and λFEL_2 when the modulator gap is set to resonate with their average value. The calculation uses radiator harmonics from 9 to 16 and λseed values between 228 and 262 nm. Black dots correspond to (λFEL_1, λFEL_2) couples whose λseed values are within the radiator bandwidth and cannot be produced using the proposed source scheme. Red squares identify the couples of wavelengths explored during the test experiment (Supplementary Figs 2 and 3).

Figure 7 shows the tuning capabilities and limitations of the adopted two-colour FEL scheme over the 16–28 nm range that broadly covers the wavelengths used in our test experiment. The red squares in Fig. 7 indicate the pairs of FEL wavelengths that were actually explored for the Fe–Ni double-resonant pump–probe measurements (Supplementary Fig. 2 and Supplementary Fig. 3). In principle, a much wider range of λFEL values extending up to 90 nm can be covered by using the full set of harmonics available at FERMI39,51. At wavelengths longer than ∼45 nm, though, the limited range of the OPA and the low radiator harmonic numbers introduce gaps in the (λFEL_1, λFEL_2) values that can be covered by this two-colour FEL scheme (Supplementary Fig. 4).

The accessible delay range between the pump and the probe is limited by the generation of the two FEL pulses from the same electron bunch of finite temporal length. In our experiment, we spanned the 300–800 fs range and an extension to 200–1,000 fs can be envisaged. This remains a strong constraint on the class of dynamic phenomena that can be addressed. Concerning ultra-fast demagnetization, in particular, many systems of interest feature response times of the order of 200 fs (refs 21, 26, 52), at the limit of the accessible range.

Further developments can be envisaged for improving the source characteristics, such as the twin-bunch mode recently demonstrated in SASE configuration15. The implementation of a similar scheme at FERMI would provide a more efficient bunching at the two wavelengths, a more efficient coupling in the radiator sections and, in fine, a significant increase in the energy per pulse, which could attain tens of microjoules for both the pump and the probe. Moreover, using two independent bunches would provide additional flexibility for tuning the two λseed wavelengths and would soften the constraints on the temporal separation between pump and probe pulses. Another significant improvement, already planned at FERMI, implies a second OPA for tuning both λseed wavelengths independently, as used for computing the tuning range reported in Fig. 7 and Supplementary Fig. 4. The desired resonant condition for both the pump and the probe FEL pulses could be finely matched.

Finally, it is worth remembering that the FERMI radiator section is composed of Apple-II type undulators53 delivering radiation of selectable polarization, either circular (right/left) or linear (vertical/horizontal). Therefore our two-colour source offers the possibility of choosing the polarization state of each pulse independently, which may be especially important in atomic and molecular physics studies.

The two-colour extreme ultraviolet source that we have developed at FERMI already has potential for many interesting and original studies in magnetization dynamics and beyond. For instance, it can cover the 3p resonances of any couple of elements among Mn, Fe, Co and Ni, making a wide class of relevant magnetic materials accessible to resonant FEL pump/resonant FEL probe experiments. More generally, it enables the excitation of a particular energy and polarization-selected resonance on a well-defined atomic site in a complex system and makes it possible to study its dynamics with the second FEL pulse, by choosing for the probe another electronic subshell or another atomic site. This new source will provide unprecedented opportunities for probing in a highly selective way the dynamics of complex relaxation processes, such as Auger cascades or sequential multiple ionization, and of charge transfer processes in large molecules and clusters.

Methods

Accelerator

The FERMI linac54 was operated at 1.3 GeV electron beam energy and 700 pC nominal charge. A moderate compression produced almost flat 500 A current electron bunches. The bunch length provided the conditions for an effective twin seeding with temporal separation of up to ∼900 fs. The longitudinal phase space of the electron beam (energy versus time) was characterized by a chirp with both linear and quadratic components55 that can be exploited to further enhance the difference between the resonant wavelengths in the two parts of the beam.

Seed lasers

The special twin-seed laser configuration for wide tunability two-colour FEL was based on the standard FERMI seed laser system described earlier56,57,58. The output of a Ti:Sapphire amplifier (5–7 mJ per pulse, 100 fs pulse duration, 784 nm central wavelength) was shared between an infrared OPA and a THG setup. Inside the OPA box, the signal pulses delivered by a two-stage white-light-seeded OPA process were frequency mixed with a residual pump pulse and the generated visible light was further up-converted by second harmonic generation to obtain ultraviolet pulses in the 228–262 nm range. The second ultraviolet pulse, generated in a time-plate-type BBO crystal-based THG setup, was adjusted to generate pulses with a central wavelength of 261.5 nm. The intensities of the two pulses could be varied independently through remotely controlled waveplates. The time delay between the two seed pulses was measured using an optical cross-correlator, where each ultraviolet pulse was cross-correlated with an IR pulse derived from the ultrafast oscillator that seeds the Ti:Sapphire amplifier. A remotely controlled delay stage on the THG path was used to set the time delay between the two seed pulses, before recombining them through a 50% beam splitter. Both seed pulses originate from the same source (laser oscillator and regenerative amplifier) and their relative time delay is very stable56,57. It has been verified that once set, the relative time delay between the two ultraviolet pulses (hence between the two FEL pulses) remains stable within less than ±5 fs over a time span of 2 h. This includes both short-term timing jitter and slow timing drifts. The adjustment and long-term stabilization of the spatial coincidence and collinearity of the two seed beams inside the FEL undulator, which are essential for obtaining the coincidence of the two FEL pulses on the sample, were obtained by using a dedicated feedback loop based on independent steering optics for each beam.

Undulators

The modulator is a 100 mm period 3 m long planar undulator with ∼3% nominal resonance bandwidth. The radiator comprises six independent 55 mm period 2.42 m long undulators based on the APPLE–II design53 that provide adjustable polarization59,60. The radiator was divided into two subsections, Rad_1 and Rad_2, set to resonate with harmonic 14 of λseed_1 and harmonic 11 of λseed_2, respectively (Fig. 1a). The λseed_2=255 nm OPA seed produces a localized bunching at all the harmonics including the 11th that matches the resonance in Rad_2, generating 23.2 nm coherent emission which is amplified along the radiator. However, the beam has also bunching at the 18.2 nm 14th harmonic close to the resonant wavelength of Rad_1 (18.7 nm), which may produce unwanted emission. Similarly, the λseed_1=261.5 nm THG seed induces a bunching at 18.7 nm (14th harmonic), which generates the FEL probe pulse in Rad_1, but also at 23.8 nm (11th harmonic) which may excite emission from Rad_2 tuned at 23.2 nm. In both cases, though, the separation between the undesired bunching wavelength and the radiator resonant wavelength is >2%, that is, larger than the ∼0.7% gain bandwidth measured for the radiators (Allaria et al. FEL-1 current status and recent achievements, FERMI Machine Advisory Committee, Sincrotrone Trieste, April 2014, unpublished). It is the narrow bandwidth of the radiators compared with the modulator that makes it possible to produce time-delayed single-frequency pump and probe FEL pulses from the same electron bunch.

Samples

The 20 nm permalloy film was sputter-deposited from a Fe19Ni81 target onto a commercial Si grating (605 nm period, 190 nm groove depth), with 3 nm Al buffer and capping layers. Room temperature magneto-optical Kerr effect measurements showed 100% remanence and ∼8 mT coercive field along the grating lines. The 12.5-nm-thick NiFe2O4 layer was grown on MgAl2O4(001) by molecular beam epitaxy in atomic oxygen plasma. A 100 × 400 μm2 area of the Ni-ferrite layer was ruled by focused ion beam etching with a set of ∼350 nm wide stripes with a ∼600 nm period. The magnetic signal at the Fe-3p resonance measured on the patterned area at the FEL source showed an ∼50 mT coercive field with 100% remanence along the stripes.

Scattering setup

The experiment was performed using the IRMA vertical-scattering-plane reflectometer44. A horseshoe electromagnet applied variable fields (±150 mT) parallel to the sample surface and normal to the scattering plane (Fig. 1b). The FEL beam was refocused at the sample position by two bendable mirrors in Kirkpatrick–Baez configuration, using an extreme ultraviolet imager at the sample position. The final spot size (∼80 μm) was estimated by scanning a movable pin-hole while measuring the transmitted intensity. The reflectometer allowed for a precise alignment of the sample with respect to the FEL beam using a slitted photodiode mounted on the detector arm. The vertically scattered intensity was detected by an in-vacuum CCD camera (2,048 × 2,048 pixels, pixel size 13.5 × 13.5 μm2) shielded from visible light by a 100-nm-thick Al filter. The CCD was mounted at 90° from the incoming FEL beam and at 535 mm from the sample. The pump fluence F at the sample was evaluated by correcting the pump energy measured at the source for the transport-line transmission (six reflections and a 200-nm-thick Al filter), focal spot size (∼80 × 80 μm2) and angle of incidence (46.5°). Error bars on fluence (Fig. 6) account for both the pump energy measurement accuracy and for the source intensity fluctuations. The maximum fluence at the sample was ∼40 and ∼3.5 mJ cm−2 for the pump and the probe, respectively. F values could be adjusted rapidly and continuously by attenuating the pump seed laser. The scattering of the p-polarized FEL radiation was measured near the Brewster extinction condition, reducing non-magnetic contributions and maximizing the magnetic contrast23,24,47,48,49. All the data reported here were collected at 46.5° incidence of the FEL radiation. Magnetization-dependent data were collected following the same protocol for both samples and for all the measurements: (a) application of +80 mT pulse of ∼10 ms duration, exceeding the saturation field; (b) +20 mT applied while collecting the scattered intensity at the CCD detector during a given acquisition time (1–10 s per frame); (c) repeat (a,b) for negative field values; (d) repeat the whole (a–c) sequence 50 times. The magnetic signal was then defined as an asymmetry ratio, that is, as the difference divided by the sum of two images collected for opposite signs of the applied field. Data reported in Figs 5 and 6 represent average values taken over a 7 × 7 pixels area.

Additional information

How to cite this article: Ferrari, E. et al. Widely tunable two-colour seeded free-electron laser source for resonant-pump resonant-probe magnetic scattering. Nat. Commun. 7:10343 doi: 10.1038/ncomms10343 (2016).

Supplementary Material

Supplementary Information

Supplementary Figures 1-4

ncomms10343-s1.pdf (579.3KB, pdf)

Acknowledgments

We are grateful to Maya Kiskinova (Sincrotrone Trieste), Jan Vogel (Institut Néel, Grenoble), Giancarlo Panaccione (CNR-IOM, Trieste), Fausto Sirotti (Synchrotron SOLEIL), Nicolas Moisan (LPS, Orsay), Michael Meyer (European XFEL, Hamburg) and Coryn F. Hague (LCPMR, Paris) for useful discussions and suggestions. This research received financial support from the European Community 7th Framework Programme under grant agreement n° 312284, and from CNRS (France) via the PEPS_SASLELX program. The FERMI project at Elettra—Sincrotrone Trieste is supported by MIUR under grants FIRB-RBAP045JF2 and FIRB-RBAP06AWK3.

Footnotes

Author contributions E.F., C.Sp., G.D.N., M.B.D., E.A. and M.S. devised and coordinated the experiment. E.F., C.Sp., L.G., G.D.N., M.B.D. and E.A. designed and optimized the two-colour FEL source. I.N., P.C., A.D. and M.B.D. reconfigured and operated the twin-seed laser source. E.F., B.D., D.G., G.P., P.R.R., E.R., M.T., L.G., G.D.N. and E.A. operated the FEL source during the experiment. F.F., R.D., F.V., J.B.M. and L.L. fabricated and characterized the samples. M.M., E.P., F.C., C.Sv., N.M., M.Z. and L.R. contributed to the integration of the IRMA experimental chamber at the DIPROI beamline. C.Sp., F.F., R.D., T.P. and M.S. performed the scattering experiment. E.F., C.Sp., F.V., M.B.D., G.D.N., E.A. and M.S. analysed the data and wrote the manuscript, with contributions from all the authors.

References

  1. Hemsing E., Stupakov G. & Xiang D. Beam by design: laser manipulation of electrons in modern accelerators. Rev. Mod. Phys. 86, 897–941 (2014). [Google Scholar]
  2. Allaria E. et al. Two-colour pump–probe experiments with a twin-pulse-seed extreme ultraviolet free-electron laser. Nat. Commun. 4, 2476 (2013). [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Bencivenga F. et al. Multi-colour pulses from seeded free-electron-lasers: towards the development of non-linear core-level coherent spectroscopies. Faraday Discuss. 171, 487–503 (2014). [DOI] [PubMed] [Google Scholar]
  4. Ciocci F. et al. Two color free-electron laser and frequency beating. Phys. Rev. Lett. 111, 264801 (2013). [DOI] [PubMed] [Google Scholar]
  5. Marcus G., Penn G. & Zholents A. A. Free electron laser design for four-wave mixing experiments with soft X-ray pulses. Phys. Rev. Lett. 113, 024801 (2014). [DOI] [PubMed] [Google Scholar]
  6. Campbell L. T., McNeil B. W. J. & Reiche S. Two-colour free electron laser with wide frequency separation using a single monoenergetic electron beam. New J. Phys. 16, 103019 (2014). [Google Scholar]
  7. Chiadroni E. et al. Two color FEL driven by a comb-like electron beam distribution. Phys. Procedia 52, 27–35 (2014). [Google Scholar]
  8. Dattoli G., Mirian N. S., DiPalma E. & Petrillo V. Two-color free-electron laser with two orthogonal undulators. Phys. Rev. Spec. Top. Accel. Beams 17, 050702 (2014). [Google Scholar]
  9. De Ninno G. et al. Chirped seeded free-electron lasers: self-standing light sources for two-color pump-probe experiments. Phys. Rev. Lett. 110, 064801 (2013). [DOI] [PubMed] [Google Scholar]
  10. Mahieu B. et al. Two-colour generation in a chirped seeded free-electron laser: a close look. Opt. Express 21, 22728–22741 (2013). [DOI] [PubMed] [Google Scholar]
  11. Lutman A. A. et al. Experimental demonstration of femtosecond two-color X-ray free-electron lasers. Phys. Rev. Lett. 110, 134801 (2013). [DOI] [PubMed] [Google Scholar]
  12. Marinelli A. et al. Multicolor operation and spectral control in a gain-modulated X-ray free-electron laser. Phys. Rev. Lett. 111, 134801 (2013). [DOI] [PubMed] [Google Scholar]
  13. Hara T. et al. Two-colour hard X-ray free-electron laser with wide tunability. Nat. Commun. 4, 2919 (2013). [DOI] [PubMed] [Google Scholar]
  14. Lutman A. A. et al. Demonstration of single-crystal self-seeded two-color X-ray free-electron lasers. Phys. Rev. Lett. 113, 254801 (2014). [DOI] [PubMed] [Google Scholar]
  15. Marinelli A. et al. High-intensity double-pulse X-ray free-electron laser. Nat. Commun. 6, 6369 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  16. Stamm C. et al. Femtosecond modification of electron localization and transfer of angular momentum in nickel. Nat. Mater. 6, 740–743 (2007). [DOI] [PubMed] [Google Scholar]
  17. Boeglin C. et al. Distinguishing the ultrafast dynamics of spin and orbital moments in solids. Nature 465, 458–461 (2010). [DOI] [PubMed] [Google Scholar]
  18. Radu I. et al. Transient ferromagnetic-like state mediating ultrafast reversal of antiferromagnetically coupled spins. Nature 472, 205–208 (2011). [DOI] [PubMed] [Google Scholar]
  19. Wietstruk M. et al. Hot-electron-driven enhancement of spin-lattice coupling in Gd and Tb 4f ferromagnets observed by femtosecond X-ray magnetic circular dichroism. Phys. Rev. Lett. 106, 127401 (2011). [DOI] [PubMed] [Google Scholar]
  20. Mariager S. O. et al. Structural and magnetic dynamics of a laser induced phase transition in FeRh. Phys. Rev. Lett. 108, 087201 (2012). [DOI] [PubMed] [Google Scholar]
  21. Eschenlohr A. et al. Ultrafast spin transport as key to femtosecond demagnetization. Nat. Mater. 12, 332–336 (2013). [DOI] [PubMed] [Google Scholar]
  22. Bergeard N. et al. Ultrafast angular momentum transfer in multisublattice ferrimagnets. Nat. Commun. 5, 3466 (2014). [DOI] [PubMed] [Google Scholar]
  23. La-O-Vorakiat C. et al. Ultrafast demagnetization dynamics at the M edges of magnetic elements observed using a tabletop high-harmonic soft X-ray source. Phys. Rev. Lett. 103, 257402 (2009). [DOI] [PubMed] [Google Scholar]
  24. La-O-Vorakiat C. et al. Ultrafast demagnetization measurements using extreme ultraviolet light: comparison of electronic and magnetic contributions. Phys. Rev. X 2, 011005 (2012). [Google Scholar]
  25. Mathias S. et al. Probing the timescale of the exchange interaction in a ferromagnetic alloy. Proc. Natl Acad. Sci. USA 109, 4792–4797 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. Günther S. et al. Testing spin-flip scattering as a possible mechanism of ultrafast demagnetization in ordered magnetic alloys. Phys. Rev. B 90, 180407 R (2014). [Google Scholar]
  27. Pfau B. et al. Ultrafast optical demagnetization manipulates nanoscale spin structure in domain walls. Nat. Commun. 3, 1100 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Zhang W. et al. Tracking excited-state charge and spin dynamics in iron coordination complexes. Nature 509, 345–348 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  29. Beaud P. et al. A time-dependent order parameter for ultrafast photoinduced phase transitions. Nat. Mater. 13, 923–927 (2014). [DOI] [PubMed] [Google Scholar]
  30. Clark J. N. et al. Imaging transient melting of a nanocrystal using an X-ray laser. Proc. Natl Acad. Sci. USA 112, 7444–7448 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  31. Först M. et al. Spatially resolved ultrafast magnetic dynamics initiated at a complex oxide heterointerface. Nat. Mater. 14, 883–888 (2015). [DOI] [PubMed] [Google Scholar]
  32. Wernet P. et al. Orbital-specific mapping of the ligand exchange dynamics of Fe(CO)5 in solution. Nature 520, 78–81 (2015). [DOI] [PubMed] [Google Scholar]
  33. Ostler T. A. et al. Ultrafast heating as a sufficient stimulus for magnetization reversal in a ferrimagnet. Nat. Commun. 3, 666 (2012). [DOI] [PubMed] [Google Scholar]
  34. Graves C. E. et al. Nanoscale spin reversal by non-local angular momentum transfer following ultrafast laser excitation in ferrimagnetic GdFeCo. Nat. Mater. 12, 293–298 (2013). [DOI] [PubMed] [Google Scholar]
  35. Finazzi M. et al. Laser-induced magnetic nanostructures with tunable topological properties. Phys. Rev. Lett. 110, 177205 (2013). [DOI] [PubMed] [Google Scholar]
  36. Mangin S. et al. Engineered materials for all-optical helicity-dependent magnetic switching. Nat. Mater. 13, 286–292 (2014). [DOI] [PubMed] [Google Scholar]
  37. Le Guyader L. et al. Nanoscale sub-100 picosecond all-optical magnetization switching in GdFeCo microstructures. Nat. Commun. 6, 5839 (2015). [DOI] [PubMed] [Google Scholar]
  38. Bocchetta C. J. et al. FERMI@Elettra FEL conceptual design report (Sincrotrone Trieste, (2007).
  39. Allaria E. et al. Highly coherent and stable pulses from the FERMI seeded free-electron laser in the extreme ultraviolet. Nat. Photon. 6, 699–704 (2012). [Google Scholar]
  40. Yu L. H. Generation of intense UV radiation by sub-harmonically seeded single-pass free-electron lasers. Phys. Rev. A 8, 5178–5193 (1991). [DOI] [PubMed] [Google Scholar]
  41. Yu L. H. et al. High-gain harmonic-generation free-electron laser. Science 289, 932–934 (2000). [DOI] [PubMed] [Google Scholar]
  42. De Ninno G. et al. Single-shot spectro-temporal characterization of XUV pulses from a seeded free-electron laser. Nat. Commun. 6, 8075 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  43. Gauthier D. et al. Spectrotemporal shaping of seeded free-electron laser pulses. Phys. Rev. Lett. 115, 114801 (2015). [DOI] [PubMed] [Google Scholar]
  44. Sacchi M. et al. Ultra-high vacuum soft X-ray reflectometer. Rev. Sci. Instrum. 74, 2791–2795 (2003). [Google Scholar]
  45. Pedersoli E. et al. Multipurpose modular experimental station for the DiProI beamline of Fermi@Elettra free electron laser. Rev. Sci. Instrum. 82, 043711 (2011). [DOI] [PubMed] [Google Scholar]
  46. Capotondi F. et al. Coherent imaging using seeded free-electron laser pulses with variable polarization: first results and research opportunities. Rev. Sci. Instrum. 84, 051301 (2013). [DOI] [PubMed] [Google Scholar]
  47. Spezzani C. et al. Magnetization and microstructure dynamics in Fe/MnAs/GaAs(001): Fe magnetization reversal by a femtosecond laser pulse. Phys. Rev. Lett. 113, 247202 (2014). [DOI] [PubMed] [Google Scholar]
  48. Sacchi M., Panaccione G., Vogel J., Mirone A. & van der Laan G. Magnetic dichroism in reflectivity and photoemission using linearly polarized light: 3p core level of Ni(110). Phys. Rev. B 58, 3750–3754 (1998). [Google Scholar]
  49. Hecker M., Oppeneer P. M., Valencia S., Mertins H. C. & Schneider C. M. Soft X-ray magnetic reflection spectroscopy at the 3p absorption edges of thin Fe films. J. Electron Spectros. Relat. Phenomena 144-147, 881–884 (2005). [Google Scholar]
  50. Henke B. L., Gullikson E. M. & Davis J. C. X-ray interactions: photoabsorption, scattering, transmission, and reflection at E=50-30,000 eV, Z=1-92. Atom. Data Nucl. Data Tables 54, 181–342 (1993). [Google Scholar]
  51. Allaria E. et al. The FERMI free-electron lasers. J. Synchrotron Radiat. 22, 485–491 (2015). [DOI] [PubMed] [Google Scholar]
  52. Vodungbo B. et al. Laser-induced ultrafast demagnetization in the presence of a nanoscale magnetic domain network. Nat. Commun. 3, 999 (2012). [DOI] [PubMed] [Google Scholar]
  53. Sasaki S. Analyses for a planar variably-polarizing undulator. Nucl. Instrum. Methods A 347, 83–86 (1994). [Google Scholar]
  54. Di Mitri S. et al. Design and simulation challenges for FERMI@ELETTRA. Nucl. Instrum. Methods A 608, 19–27 (2009). [Google Scholar]
  55. Penco G. et al. Experimental demonstration of electron longitudinal-phase-space linearization by shaping the photoinjector laser pulse. Phys. Rev. Lett. 112, 044801 (2014). [DOI] [PubMed] [Google Scholar]
  56. Danailov M. B. et al. Design and first experience with the FERMI seed laser. Proceedings of the 33rd International Free Electron Laser Conference (FEL 2011), (eds Zhao, Z. & Wang, D.) 183–186 (SINAP, Shanghai, China TUOC4, 2012).
  57. Danailov M. B. et al. Towards jitter-free pump-probe measurements at seeded free electron laser facilities. Opt. Express 22, 12869–12879 (2014). [DOI] [PubMed] [Google Scholar]
  58. Cinquegrana P. et al. Optical beam transport to a remote location for low jitter pump-probe experiments with a free electron laser. Phys. Rev. Spec. Top. Accel. Beams 17, 040702 (2014). [Google Scholar]
  59. Schmidt T. & Zimoch D. About APPLE II Operation. AIP Conf. Proc. 879, 404–407 (2007). [Google Scholar]
  60. Allaria E. et al. Control of the polarization of a vacuum-ultraviolet, high-gain, free-electron laser. Phys. Rev. X 4, 041040 (2014). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-4

ncomms10343-s1.pdf (579.3KB, pdf)

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES