Learning to balance on one leg: motor strategy and sensory weighting

Abstract

We investigated motor and sensory changes underlying learning of a balance task. Fourteen participants practiced balancing on one leg on a board that could freely rotate in the frontal plane. They performed six, 16-s trials standing on one leg on a stable surface (2 trials without manipulation, 2 with vestibular, and 2 with visual stimulation) and six trials on the balance board before and after a 30-min training. Center of mass (COM) movement, segment, and total angular momenta and board angles were determined. Trials on stable surface were compared with trials after training to assess effects of surface conditions. Trials pretraining and posttraining were compared to assess rapid (between trials pretraining) and slower (before and after training) learning, and sensory manipulation trials were compared with unperturbed trials to assess sensory weighting. COM excursions were larger on the unstable surface but decreased with practice, with the largest improvement over the pretraining trials. Changes in angular momentum contributed more to COM acceleration on the balance board, but with practice this decreased. Visual stimulation increased sway similarly in both surface conditions, while vestibular stimulation increased sway less on the balance board. With practice, the effects of visual and vestibular stimulation increased rapidly. Initially, oscillations of the balance board occurred at 3.5 Hz, which decreased with practice. The initial decrease in sway with practice was associated with upweighting of visual information, while later changes were associated with suppression of oscillations that we suggest are due to too high proprioceptive feedback gains.

postural balance is maintained by muscle activation patterns that keep the body center of mass (COM) within safe boundaries above the base of support or bring it back above the base of support after perturbations (Nashner 1977; Torres-Oviedo and Ting 2010). While across multiple conditions these activation patterns reflect a consistent set of synergies, i.e., invariant patterns of activation across muscles, task-specific muscle synergies are recruited in addition to the preexisting ones when balance demands are high, as for example in one-legged standing (Torres-Oviedo and Ting 2010). In mechanical terms, these muscle activity patterns control the COM: by shifting the center of pressure of the ground reaction force (COP) within the base of support, by changing the body's angular momentum around the COM, or by creating a new base of support, for example, by stepping or grabbing a hand rail (Hof 2007). Successful balancing is usually defined as the ability to maintain upright body posture without having to step or grab support, so the latter strategy will not be considered here. If balance is controlled by shifting the COP, the body moves more or less as a single segment, often modeled as a single inverted pendulum, rotating around the ankle(s), due to which it has been coined “ankle strategy” (Horak and Nashner 1986). Changing the angular momentum of body segments around the COM involves relative movements of body segments and hence a deviation from inverted pendulum behavior. Given the large movements around the hip in this strategy, it has been coined “hip strategy” (Horak and Nashner 1986). In postural control, multijoint movements appear to be present (Creath et al. 2005; Riemann et al. 2003), and even isolated movements in hip and ankle would require coordinated muscle activity around other joints, as is reflected in the muscle synergies observed (Torres-Oviedo and Ting 2010). Therefore, the terms ankle and hip strategy may be simplifications, but they will be used in the following for brevity.

To generate the appropriate muscle activation patterns to control balance, the central nervous system (CNS) uses feedback from the somatosensory, visual, and vestibular sensory systems (Redfern et al. 2001). Information provided by these modalities is usually consistent. However, in specific conditions, it may be inconsistent and selection or adapted weighting of information is required. This process is called sensory (re-)weighting (Zupan et al. 2002) or sensory (re-)organization (Sober and Sabes 2003).

Training of balance control has been proven to be beneficial in preventing falls in the elderly (Duque et al. 2013; Halvarsson et al. 2013) and decreasing injury risk (Hupperets et al. 2009) and improving sports performance (Cressey et al. 2007) in athletes. Such training commonly involves balancing on an unstable surface like a balance board, foam mat, or gymnastic ball. After initial failures, most people learn to maintain balance on such unstable surfaces, but it is unknown what underlies this improved performance. Changes in motor strategy and changes in sensory weighting may both contribute to this learning process.

Motor Strategy and Support Surface

In balancing on a rigid surface, moments around the ankle joint instantaneously and proportionally change the position of the COP of the ground reaction and therewith cause moments that accelerate the COM (Hof 2007). On an unstable surface, moments around the ankle joint also change the COP position, now by moving (in case of, e.g., a balance board) or deforming (in case of, e.g., a foam mat) the support surface. However, due to the dynamics of the surface, the relation between the ankle moment and the COM acceleration is different than on a rigid surface, with changes in scaling of the effect of changes in ankle moment as well as in the temporal relation between the moment and the resulting COM acceleration. Balancing on an unstable surface might thus be expected to coincide with a shift from the ankle to the hip strategy. Indeed, while the ankle strategy appears to be dominant in the control of normal unperturbed standing, the hip strategy is used more when balancing on narrow supports (Horak and Nashner 1986; Otten 1999) and on compliant surfaces (Patel et al. 2008; Riemann et al. 2003). While it has been suggested that the hip strategy involves movements of the upper body relative to the hip as a single segment (Horak and Nashner 1986; Otten 1999), the actual movement patterns may be more complicated and the contribution of different segments to the change in angular momentum has to our knowledge not been studied.

Motor Strategy and Training

Training possibly also induces changes in the mechanical strategy to control balance. Honegger et al. (2013) reported that tightrope walkers when balancing in tandem stance on a foam mat showed more trunk and arm movements than untrained participants, which may indicate that the trained participants used the hip strategy more. However, to our knowledge, direct effects of training on motor strategy in balancing have not been reported.

Sensory Weighting and Support Surface

With respect to the use of sensory information for balance control, on a rigid surface, different sensory modalities usually provide consistent information on body orientation relative to gravity. While standing on an unstable surface, information derived from the somatosensory system becomes ambiguous, because changes in length of muscles in the lower extremity are not coherent with changes in body orientation relative to gravity (Kiers et al. 2012; Nashner 1982). Also the input into the somatosensory system arising from the contact with the support surface is altered (Pasma et al. 2012). Larger disturbances of upright standing posture by surface manipulations have been associated with upweighting of vestibular information (Maurer et al. 2006; van der Kooij and Peterka 2011) and visual information (Assländer and Peterka 2014; Fransson et al. 2007; Polastri et al. 2012) relative to somatosensory information. Consequently, on an unstable surface, artificial input into the somatosensory system has a smaller effect than on a solid surface (Ivanenko et al. 1999; Kiers et al. 2012; Trimble and Koceja 2001), while the effects of blocking visual input (Andreopoulou et al. 2015; Fransson et al. 2007) and of vestibular stimulation (Andreopoulou et al. 2015) are larger. All in all, there is clear evidence for sensory reweighting for balance control in unstable environments.

Sensory Weighting and Training

Balance control training also appears to coincide with reweighting of sensory information. Full-time ballet dance students showed briefer vestibular reflexes than controls, and this was associated with structural changes in the vestibular cerebellum (Nigmatullina et al. 2015). Well-trained gymnasts have been reported to show smaller effects of closing the eyes on balance control than untrained participants (Robertson et al. 1994; Vuillerme et al. 2001). Although such effects appear not to be very consistent (Asseman et al. 2008; Lamoth et al. 2009), they do suggest a reduced weighting of visual information after gymnastics training. However, these studies do not necessarily indicate that gymnasts use visual information less when it is available, but rather that they can compensate better for its absence. Gautier et al. (2008) bypassed this limitation by using a virtual optic flow to manipulate visual information. They found smaller effects of this flow on head movement velocity in gymnasts than in untrained controls, hence providing more direct evidence of reduced weighting of visual information in gymnasts. Gautier et al. (2008) concluded, however, that their results may still indicate that gymnasts, rather than having downweighted visual information, are better able to exploit multimodal information to compensate for nonveridical visual input. More importantly, gymnastic training involves much more than dealing with unstable surfaces. It would therefore be useful to assess effects of training to balance on an unstable surface more directly. Studies that have done so have shown decreases in proprioceptive reflex gains after balance training (Gruber et al. 2007; Keller et al. 2012; Taube et al. 2007b; Trimble and Koceja 2001), suggesting increased reliance on visual and/or vestibular information for balance control.

Aims and Hypotheses

In summary, balancing on an unstable surface may elicit changes in motor strategy and changes in the use of sensory information, compared with balancing on a rigid surface. With training these adaptations are likely to become more pronounced. The aim of the present study was to comprehensively characterize balance control on an unstable surface. This was achieved in multiple steps. First, we investigated differences between standing on one leg on a rigid vs. an unstable surface. We compared motor strategies used and the influence of perturbations of sensory information between surface conditions. We then studied rapid learning effects by comparing repeated trials on the unstable surface. Subsequently, the subjects trained on the unstable surface for 30 min and we determined long-term effects on motor strategies and on the influence of sensory perturbations. We hypothesized that participants rely more on the hip strategy, involving larger trunk movements relative to the stance leg, on the unstable surface than on the rigid surface. In addition, we aimed to provide insight in the movement pattern underlying the hip strategy or the control of COM through changes in angular momentum. Finally, we hypothesized that vestibular and visual feedback would be upweighted on the unstable compared with the rigid surface and that training would improve performance, coinciding with a further shift in motor strategy and upweighting of visual and vestibular feedback.

METHODS

Participants

Fourteen healthy participants, five males and nine females (age: 22.8 yr; SD: 2.2; weight: 67.6 kg; SD: 10.1; height: 175.6 cm; SD: 7.6), participated in this study. Exclusion criteria were known neurological disorders, vestibular impairments, or any impairment in the lower extremity. Also, people who practiced sports that specifically challenge balance were excluded, as this could limit training gains in the experiment (Kiers et al. 2013).

Ethics Statement

All participants provided written informed consent and the protocol had been approved by the ethical committee of the Faculty of Human Movement Sciences of the VU University Amsterdam (ECB 2013-25).

Instrumentation

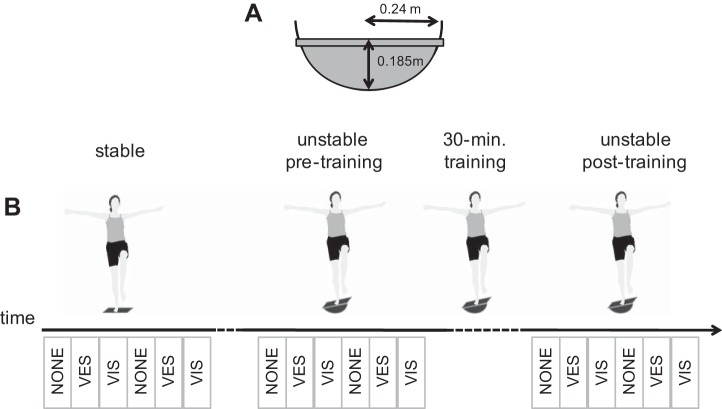
The unstable surface was created with a balance board consisting of a wooden board mounted on a section of a cylinder, creating an unstable support in the frontal plane. The radius of the cylinder was 24 cm and the height of the surface of the board above the point of contact was 18.5 cm (Fig. 1A). Handrails were placed such that participants could grab one of the rails in case of balance loss.

Fig. 1.

Overview of the experimental conditions. A: illustration of the unstable object. B: illustration of the posture of the subjects and the procedure with the 3 experimental conditions: stable surface, unstable surface pretraining, and unstable surface posttraining, intermitted by 30 min of training. In between trials on stable and unstable surfaces, subjects performed 2 practice trials of 16 s on the unstable surface. The total period between measurements on stable and unstable surfaces was ∼5 min. Rest periods between trials within each block of 6 trials lasted 20–30 s. The blocks below the time line represent the 18 trials, with NONE referring to no sensory manipulations, VES referring to vestibular manipulation, and VIS to visual manipulation. Note that the order of the latter 2 conditions varied over subjects. Motor strategies used in balancing were analyzed based on the trials without manipulations. Surface effects were analyzed based on averages over the trials on the stable surface and averages over the trials in the unstable posttraining condition. Training effects were analyzed based on separate trials of the unstable pretraining and unstable posttraining conditions.

An Optotrak camera (Northern Digital, Waterloo, ON, Canada) recorded the 3D-positions of 21 LED markers on the body and two on the lateral ends of the board at a sample rate of 300 samples/s. The markers were placed on the frontal aspects of the ankles, knees, hips, shoulders, elbows, wrists, and forehead. Three markers were placed over the elbows and wrists to increase the probability that at least one marker would be visible at all times.

EMG of the main hip abductor and adductor muscles was measured, as these are thought to play a key role in balance control in the frontal plane (Otten 1999; Rietdyk et al. 1999; Winter et al. 1996). Pairs of surface Ag-AgCl EMG electrodes were attached to the skin over the muscle bellies of the m. adductor longus and m. gluteus medius after shaving and cleaning of the skin. EMG data were sampled at 2,000 sample/s (Porti 17, TMSi; Enschede, The Netherlands; 22 bits AD conversion after 20 times amplification, input impedance >10¹² Ω, CMRR >90 dB for the relevant range of frequencies).

The weighting of vestibular input was assessed by galvanic vestibular stimulation (GVS) by a linear isolated stimulator (Stmisola, Biopac, Goleta CA) set to produce a sinusoidally alternating current with a 4.5-mA amplitude and a frequency of 0.125 Hz through electrodes over the mastoid processes. The weighting of visual input was assessed using a moving scenery created with a data projector. Alternating vertical black and white bars (0.3-m width) were projected on a screen (2.5 by 1.5 m) on the wall 4 m in front of the participant and moved horizontally and sinusoidally at 0.125 Hz with an amplitude of 2.4 m in the trials with visual manipulation.

Experimental Procedure

A measurement consisted of 18 trials, 6 in each of 3 conditions (rigid surface, unstable surface before training, unstable surface after training). For every trial, participants were instructed to stand as still as possible on their preferred leg, with their arms abducted 90° and the unsupported leg hanging free (Fig. 1B). Also, they were asked to look at the middle of the projection screen in front of them. Every trial lasted 16 s. Trial duration was kept this short because pilot studies indicated that subjects starting grabbing the support at an increasing rate during longer trials, reportedly related to fatigue and associated balance loss. In between trials on stable and unstable surfaces, subjects performed two practice trials on the unstable surface of 16 s and the total period between measurements on stable and unstable surfaces was ∼5 min. Rest periods between trials within each block of six trials were given to prevent fatigue and restart measurements, each break lasted 20–30 s. Pilot measurements indicated a substantial learning effect within the first trials on the balance board. To avoid frequent balance loss during the measurements and to attenuate but not efface the fast learning effects, participants were allowed to practice standing on the balance board before the experiment only twice for 16 s and were not allowed to stand on the balance board in between trials.

During each trial kinematic data were recorded continuously. In the first trial of each set of three (Fig. 1B), EMG data were recorded and no manipulation was applied. Subsequently, the two sensory manipulations were applied, the sequence of which was balanced over participants but stayed the same within participants. These manipulations were used to assess the weighting of vestibular and visual information by comparison to the unperturbed trial. To assess fast learning effects, these three trials were repeated once within each condition. In the stable condition, which was performed first, participants balanced on one leg on a rigid platform, flush with the floor of the laboratory; in the other conditions (before and after training) they were standing on the balance board described above. After the first six trials on the board (2 repetitions of 3 trials), participants had 30 min to practice balancing on the board. No instructions or feedback were given, but it was made sure that participants were actively training during this period. Subsequently, the six trials on the balance board were repeated once more.

Data Analysis

During each trial, the three-dimensional coordinates of LED markers on anatomical landmarks and on the balance board were recorded. For every trial, the marker that was within the view of the camera for most of the samples for each wrist and elbow was chosen for analysis. Missing samples were interpolated by cubic spline interpolation. Based on the frontal plane positions of the thirteen body segments and an anthropometrical model (Zatsiorsky 2002), the times series of the frontal position of the body COM was calculated. The position of the hands and feet were extrapolated, assuming fixed wrist and ankle angles. The total excursion of the COM in the mediolateral direction during one trial, or path, was used as a measure of performance and was calculated as:

$$\text{path}=\sum \left|C\dot{O}{\text{M}}_y\right|$$ (1)

in which CȮM_y is the mediolateral velocity of the COM. Given the standardized length of the data series, this measure is equivalent to the mean absolute velocity of the COM. It was used to quantify performance in view of its high reliability and sensitivity as a measure of postural sway (Cholewicki et al. 2000; Raymakers et al. 2005; van Dieen et al. 2010). The time series of COM acceleration in the left-right direction was calculated as the second derivative of the COM time series. In addition, the orientation of the balance board relative to the horizontal was determined.

The EMG signals of the m. adductor longus and m. gluteus medius were band-stop filtered (4th order Butterworth, with cut-off frequencies of 49.5 and 50.5 Hz), high-pass filtered at 10 Hz (2nd order Butterworth) to remove movement artifacts and then rectified and smoothed (bidirectionally applied 2nd order Butterworth filter with a cut-off frequency of 5 Hz). The means and the coefficients of variation of the linear envelope were calculated to obtain measures of the level of activity and of the variance in activity of both muscles. Pearson's correlation coefficient between EMG time series of the antagonistic muscle pair was calculated to assess whether reciprocal activation (negative correlations) or coactivation (positive correlations) was present.

Frequency domain analyses of kinematic data and EMG linear envelopes were performed using Welch's averaged modified periodogram method, with Hamming windows of 1,500 samples overlapping for 50% and with 6,000 points fast Fourier transform to obtain a frequency resolution of 0.05 Hz. The resulting power spectra were normalized to total power to allow straightforward comparison of the of the distribution of power irrespective of the total power of the signals.

Angular momenta of the total body and of individual segments around the body COM were calculated using the following equation (Elftman 1939):

$$H=\sum _{j=1}^n{h}_j=\sum _{j=1}^n{I}_j{\varpi }_j+m_j{r}_j\times v_j$$ (2)

in which H is total angular momentum, n is the number of segments, h_j is angular momentum of segment j, I_j is the segment's moment of inertia relative to its center of gravity (estimated based on Zatsiorsky 2002), ω_j is the segment's angular velocity, r_j is the position vector of the segment's COM m_j relative to the body's COM, and v_j is the velocity vector of the segment's COM relative to the body's COM. The first derivatives of the angular momenta in the frontal plane (Ḣ and ḣ) were calculated.

Ignoring changes in the base of support by grabbing the handrail, the contributions of the ankle strategy and hip strategy to COM acceleration are given by (Hof 2007):

$$m\cdot {\text{COM}}_z\left(t\right)\cdot {\text{CÖM}}_y\left(t\right)=m\cdot g\left({\text{COP}}_y\left(t\right){\text{COM}}_y\left(t\right)\right)-\dot{H}\left(t\right)$$ (3)

in which m is body mass, COM_z is the vertical position of the COM, $\text{C}Ö{\text{M}}_y$ is acceleration of the COM in the mediolateral direction, t is time, g is the gravitational acceleration, COM_y is the mediolateral position of the center of pressure, and COM_y is the mediolateral position of the COM. Here the first part of the right-hand term refers to the ankle strategy and Ḣ, the second part, is proportional to the ML COM acceleration induced by the hip strategy. Root mean squared (RMS) values of the derivatives of the angular momentum of the arms (after summation over upper arm, forearm, and hand), legs (after summation, over thigh, shank and foot), head, and trunk (ḣ) were calculated. To assess how these segments contributed to the total Ḣ. RMS values of ḣ were expressed relative to the RMS of Ḣ.

The contribution of the hip strategy to balance control was quantified as:

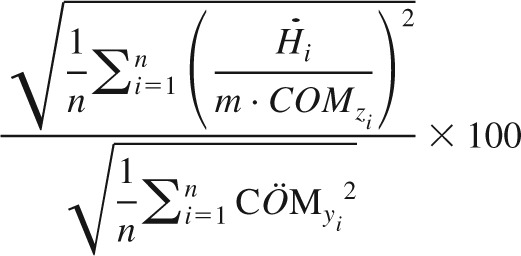(4)

in which i is sample number and n the total number of samples. The denominator in this equation represents the total amount of acceleration of the COM and the numerator represents the amount of acceleration of the COM caused by changes in angular momentum. The ratio thus can be thought of as the contribution of angular momentum changes to COM acceleration. This ratio can exceed 100 when a negative covariance exists between the effect of angular momentum changes and the effect of changes in COP. Note that the second component contributing to COM acceleration, i.e., shifting the COP relative to the COM, was not measured directly, as its contribution can be inferred from Eq. 3. In addition, when standing on the balance board, COP location is directly related to the measured angle of the board.

To further assess the coordinative strategy underlying the generation of Ḣ, principal component analysis (PCA) was performed on the time series of ḣ of arms, legs, trunk, and head. PCA was first performed on the data of each trial and participant separately, subsequently on data pooled over trials per participant and finally over all data pooled. The number of principal components to retain was selected based on the eigenvalues, and varimax rotation of selected components was applied to facilitate interpretation of the structure of the data.

Statistics

All statistical analyses were performed with SPSS 20 (IBM Software, Armonk, NY).

To compare balance performance and motor strategy in one-legged balancing between rigid and unstable surfaces, the mean values over the two trials without manipulations on rigid surface were compared with the mean values of the two trials without manipulations on the balance board after training, using paired tests. We used posttraining trials for the surface condition comparison, as the initial trials on the unstable surface showed rapid changes in motor behavior. Paired t-tests were used, except for EMG data, which were clearly nonnormally distributed and were therefore tested using a Wilcoxon-matched pairs test.

To evaluate training effects, we used two-way repeated measures ANOVAs to test for effects of trial to assess fast learning over repeated trials and for effects of the training period to assess more gradual skill acquisition. In view of their nonnormal distribution, EMG data were tested with Wilcoxon matched pairs tests, comparing adjacent trials, i.e., pretraining trials 1 and 2, pretraining trial 2 and posttraining trial 1, and posttraining trials 1 and 2.

To compare the effects of sensory manipulations between surface conditions, we again used the average values over the trials on the rigid surface and the trials on the unstable surface posttraining. We used two-way repeated measures ANOVAs with as dependent variable the COM mediolateral path length and as independent variables surface and either vestibular or visual manipulation. In addition, because the unstable surface would be expected to amplify effects of any perturbation including the sensory manipulations applied, the relative effects of the sensory manipulations were compared between surface conditions using paired t-tests. To assess effects of training on sensory weighting, three-way ANOVAs with sensory manipulations, trial, and training as independent variables were performed.

Finally, associations between changes in performance over trials and changes in the motor strategy used and in the effects of the sensory manipulations over trials were assessed by means of Pearson's correlation coefficients.

RESULTS

Balance Performance

In the stable surface condition without sensory manipulations, none of the participants touched the support rail, while one or more participants made brief contact with the rail in all other conditions (Table 1). The median number of contacts was zero for all conditions on the stable surface; it increased in the first set of pretraining trials to decrease again over subsequent trials.

Table 1.

Overview of number of times that participants made contact with the support rail in each condition

	Median	Range
Stable
First set
None	0	0
VES	0	0–4
VIS	0	0–6
Second set
None	0	—
VES	0	0–1
VIS	0	0–3
Unstable pretraining
First set
None	1	0–6
VES	1	0–5
VIS	0.5	0–7
Second set
None	0	0–1
VES	0.5	0–4
VIS	0	0–6
Unstable posttraining
First set
None	0	0–1
VES	0	0–2
VIS	0	0–2
Second set
None	0	0–2
VES	0	0–3
VIS	0	0–1

None, no sensory manipulation; VES, vestibular manipulation (galvanic vestibular stimulation); VIS, visual manipulation (moving scenery).

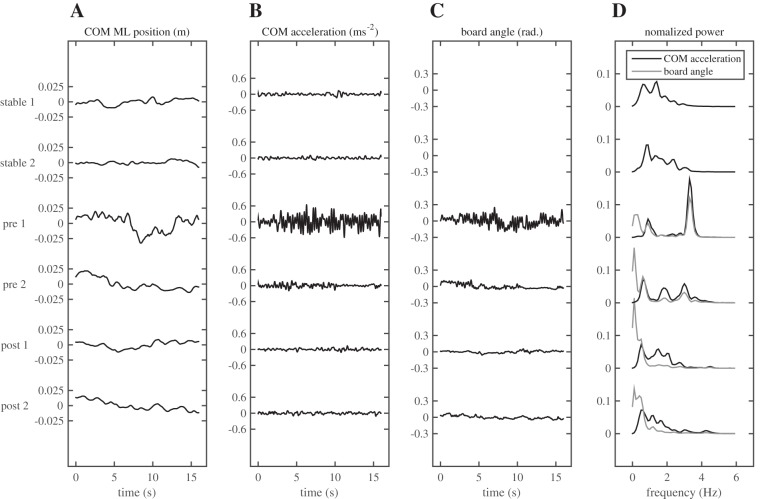
Figure 2 gives a typical example of the COM trajectories and the angle of the balance board of a single participant in the trials without sensory manipulations. Clearly, COM excursion was larger on the unstable surface, especially in the first trial before training. Unanticipated, the COM displacement showed an oscillatory pattern at a relatively high frequency that was more readily apparent in the COM acceleration and its normalized power spectrum and was also present in the time series and spectrum of the board angle. Since the board angle is directly related to the mediolateral position of the COP, which in turn drives COM acceleration (Eq. 3), ankle moments driving the board oscillations can be considered causal in this relation. The peak in the power spectrum was found at ∼3.5 Hz and was still clearly visible in the second trial without sensory manipulation before training but not after training. Also, the frequency of the peak appeared to decrease slightly over trials. The oscillations in the COM acceleration illustrated in Fig. 2 were clearly observable in all participants, albeit at slightly different frequencies. Therefore, we calculated the relative power of the COM acceleration and board angle between 2.5 and 4.5 Hz and compared this between conditions.

Fig. 2.

Typical example of the times series of the mediolateral (ML) position of the center of mass (COM; A), its acceleration (B), the angle of the board with the horizontal (C), and of the normalized power spectra of the latter 2 variables (D) for all trials without sensory manipulations. Note the much larger COM excursion in the 1st pretraining trial and the peaks in the power spectra at ∼3.5 Hz in both pretraining trials.

Effects of surface condition on balance performance.

To assess effects of surface condition, standing on a rigid surface was compared with standing on the unstable surface after training (Fig. 3, bar clusters at left and right). As expected, the mediolateral COM path was significantly longer on the unstable surface than on the rigid surface (Fig. 3A, P < 0.001) illustrating the more demanding nature of balancing on the board. The relative power of the COM acceleration between 2.5 and 4.5 Hz was significantly higher on the unstable surface compared with the stable surface (Fig. 3B, P = 0.033).

Fig. 3.

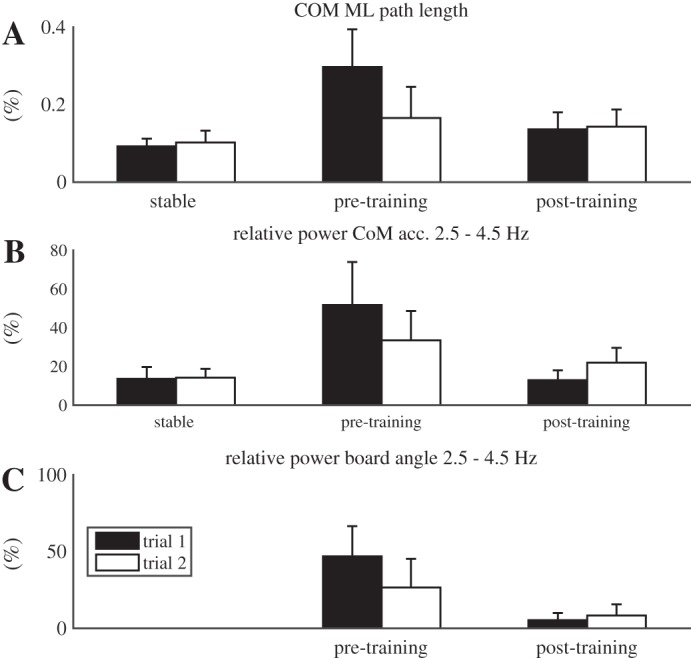
Mean values of the total excursion of the body COM in ML direction (A), the relative power of COM acceleration between 2.5 and 4.5 Hz (B), and the relative power of the board angle between 2.5 and 4.5 Hz (C) for all trials without sensory manipulations. Error bars indicate 1 SD.

Effects of training on balance performance.

We tested for effects of trial to assess fast learning effects over repeated trials and for effects of 30 min of training to assess more gradual training effects (Fig. 3, bar clusters at middle and right). Performance improved with training on both short and long time scales, as evidenced by significant main effects of trial (P = 0.003) and training (P < 0.001) and a significant interaction effect (P < 0.001), with a significant post hoc difference between pretraining trials 1 and 2 (P = 0.003) and a borderline effect between pretraining trial 2 and posttraining trial 1 (P = 0.053) but not between posttraining trials (Fig. 3A).

For the relative power of COM acceleration at 2.5–4.5 Hz, the effect of training was significant (Fig. 3B, P < 0.001) while the effect of trial (P = 0.111) was not. However, a significant interaction was found (P = 0.001), with a decrease between trials before training and a slight increase after training. Post hoc differences between all adjacent trials were significant (P = 0.009, P < 0.001, and P = 0.001, respectively). For the relative power of the board angle, significant main effects of training (Fig. 3C, P = 0.009) and trial (P < 0.001) and a significant interaction were found (P = 0.001), with the pattern of change similar to that observed for the relative power of the COM acceleration at 2.5–4.5 Hz. Again post hoc differences between all adjacent trials were significant (P = 0.006, P < 0.001, and P = 0.043, respectively).

Muscle Activity

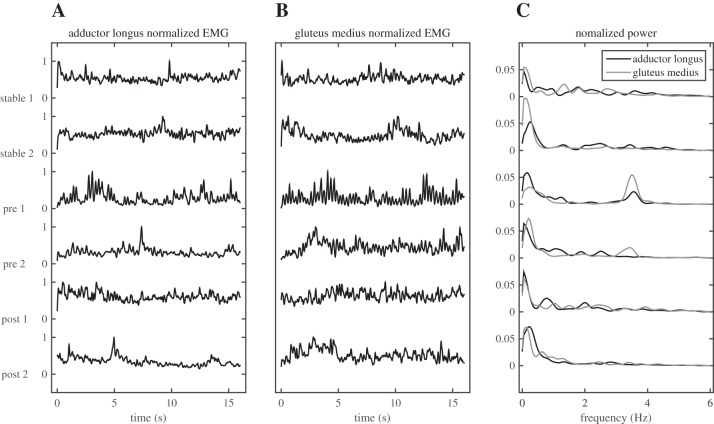
Activity of the adductor longus and gluteus medius muscles consisted of tonic activity with superimposed phasic bursts as illustrated in Fig. 4. Visual inspection suggested that these bursts sometimes reflected reciprocal activation and sometimes reflected coactivation of the antagonistic muscle pair measured. Accordingly, correlations between the EMG linear envelopes of both muscles were overall low; coefficients of correlation ranged from −0.69 to +0.66 with 77% having an absolute value <0.3. In all but one of the participants, the EMG data in the first trials on the unstable surface showed oscillations at the same frequency as observed in the kinematics of the COM and the balance board.

Fig. 4.

Typical example of EMG data in trials without sensory manipulations, showing the linear envelopes normalized to their maximum values (A and B) and the power spectra of the linear envelopes (C) for all trials without sensory manipulations. Note the strong increase in muscle activity in the 1st pretraining trial or the unstable surface and the peaks in power at ∼3.5 Hz in the pretraining trials on the unstable surface.

Effects of surface condition on muscle activity.

Muscle activity was in general higher on the unstable than on the stable surface after training, with adductor longus activity having a 40% higher median (P = 0.026) and gluteus medius activity having a 34% higher median (P = 0.003) on the unstable surface. The coefficient of variation of muscle activity was not different between surface conditions for the adductor longus but was slightly higher on the unstable surface for gluteus medius (median 24 vs. 25%, P = 0.048). The relative power of the EMG linear envelopes between 2.5 and 4.5 Hz was not different between the stable condition and the unstable condition after training. Correlations between EMG linear envelopes of both muscles were equally low in both conditions.

Effects of training on muscle activity.

Muscle activity of the adductor longus and gluteus medius decreased significantly between the two pretraining trials by 36% (P = 0.005) and 26% (P = 0.016), respectively, but did not change significantly between later trials (post 1 vs. post 2). The coefficient of variation of the EMG linear envelopes decreased between pretraining trials, from a median of 42 to 25% for the adductor longus and from 41 to 27% for the gluteus medius (P = 0.008 for both muscles), and decreased from 27 to 26% between pretraining 2 and posttraining 1 for gluteus medius (P = 0.030). Between the pretraining trials, the relative power between 2.5 and 4.5 Hz decreased not significantly for adductor longus (from 22 to 20%, P = 0.094) and significantly for gluteus medius (from 50 to 34%, P = 0.009) and decreased further between pretraining 2 and posttraining 1 for gluteus medius alone (from 34 to 26% P = 0.006).

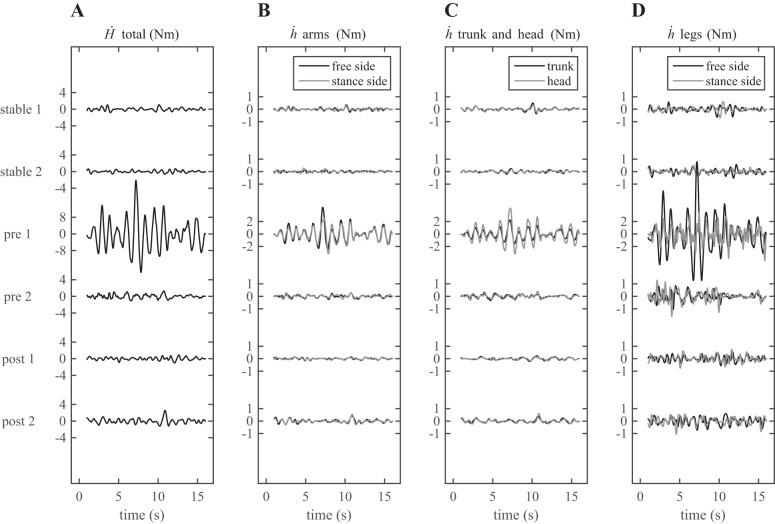
Angular Momentum Control

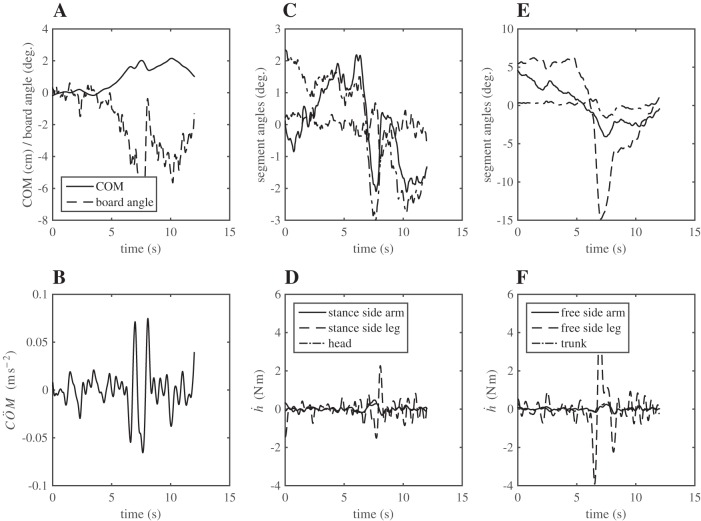
Typical examples of the time series of the derivatives of the total angular momentum (Ḣ) and of the angular momenta of the separate segments (ḣ) are presented in Fig. 5. Angular momenta were clearly more variable in the trials on the unstable surface compared with the trials on the rigid surface, especially in the first trial on the unstable surface. Variations in ḣ of the arms, head, and trunk occurred in phase, while the leg movements appeared to be more independent. The ḣ of the nonstance or free leg was relatively high in all trials. The derivative of the stance leg angular momentum had a relatively high-frequency content, most likely associated with the oscillations of the balance board described above. However, the power of Ḣ in the frequency band concerned (2.5–4.5 Hz) was below 6% of the total power across all conditions and participants.

Fig. 5.

Typical examples of the time series of the derivatives of the total angular momentum (Ḣ; A) and of the angular momenta (ḣ) of both arms (B), trunk and head (C), and both legs (D) for all trials without sensory manipulations. Note that the y-axes scales have been adjusted for the 1st trial pretraining to improve readability.

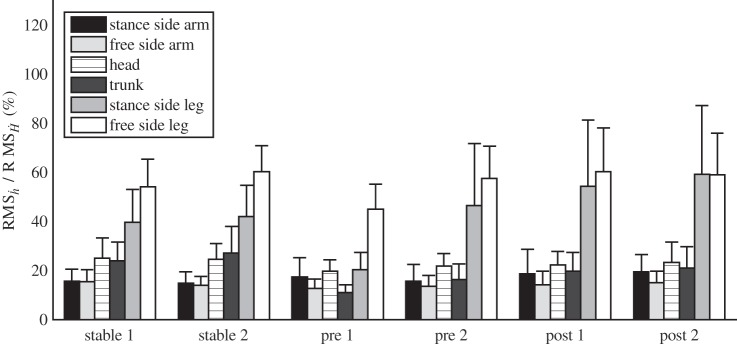
The large effect of the legs on Ḣ was confirmed at the group level by comparison of the relative RMS values of ḣ between segments (Fig. 6). Note, in particular for the stance leg this is also the result of movements of the COM relative to the leg. The sum of the relative RMS values over segments usually exceeded 100% indicating that counterrotations of segments occurred. The effects of the segments on Ḣ were quite consistent over conditions, with some notable exceptions, which will be reported in Effects of surface condition on angular momentum control and Effects of training on angular momentum control.

Fig. 6.

Average root mean squared (RMS) values of the derivatives of the angular momenta (ḣ) of the separate segments expressed as percentage of the RMS values of the total angular momentum (Ḣ) for all trials without sensory manipulations. Error bars indicate 1 SD.

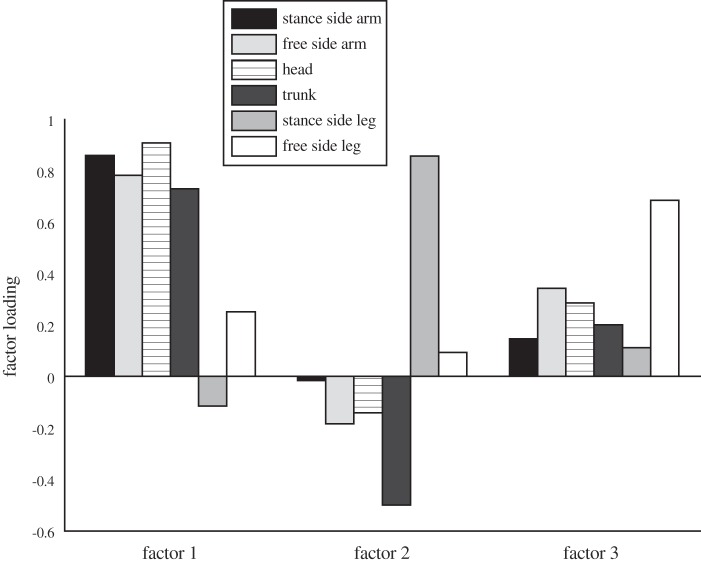
The PCA performed on the time series of ḣ confirmed the consistency of how angular momentum control was used across conditions and even across participants. When applying PCA per participant and per trial, three modes accounted for on average 93% (SD 3%) of the total variance in ḣ. With PCA on data per participant across conditions, three modes accounted for on average 91 SD 2% of total variance in ḣ and finally when applying PCA over all participants and trials, three modes accounted for 90% of the total variance in ḣ. Varimax rotation of the PCA results showed that arms, head, and trunk covaried, while both legs moved largely independently (Fig. 7). The independent variations in angular momentum of the free, nonstance leg (see Fig. 7, factor 3), relative to the trunk and more cranial segments, indicates that the label “hip strategy” does not adequately reflect the motor strategy used.

Fig. 7.

Factor loading of the time series of ḣ after varimax rotation of principal component analysis (PCA) results on data of all participants and all trials. Factor 1 mainly describes ḣ of the arms, head, and trunk. Factor 2 describes out-of-phase variations of ḣ of the stance leg, and factor 3 mainly describes variations in ḣ of the free (nonstance) leg.

Effects of surface condition on angular momentum control.

Between surface conditions, i.e., comparing the average over the two rigid surface conditions to that over the two posttraining trials, the relative effects of segments on Ḣ changed as indicated by a lower relative RMS value of ḣ for the trunk on the unstable surface (P = 0.037) and higher values for the stance leg (P = 0.012) and ipsilateral arm (P = 0.048; Fig. 6).

Confirming our hypothesis, the effect of Ḣ on the acceleration of the body COM was significantly larger on the unstable surface than on the rigid surface (Fig. 8, bar clusters at right and left, P = 0.011).

Fig. 8.

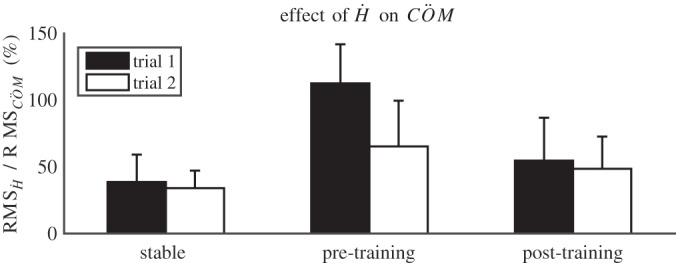
Mean values of the effect of the derivative of body angular momentum (Ḣ) or the hip strategy on the COM acceleration (calculated according to Eq. 3). Note that the effect of Ḣ on the acceleration exceeds 100% when the ankle strategy counteracts effects of Ḣ on the control of the COM (i.e., of the hip strategy). Error bars indicate 1 SD.

Effects of training on angular momentum control.

With trial and training, effects of the stance leg (P = 0.012 and P = 0.001), swing leg (P = 0.028 and P = 0.049), and trunk (P = 0.025 and p 0.003) on Ḣ increased (Fig. 6). The relative contributions of the segments were overall lower in the first trial pretraining, which implies less counterrotations of segments and may imply more strict coordination between segments.

The contribution of the hip strategy to COM acceleration decreased significantly with training (Fig. 8, bar clusters at middle and right, P < 0.001) and trial (P = 0.002) and was affected by their interaction (P = 0.049). The interaction indicated a larger decrease between pretraining trials than between posttraining trials. On post hoc paired comparisons of adjacent trials, only the first two trials were significantly different (P = 0.004).

Sensory Weighting

Figure 9 illustrates the effect of the sensory manipulations in the stable and unstable surface conditions (after training). As can be seen, the sensory manipulations induced sway at the frequency of stimulation in both conditions.

Fig. 9.

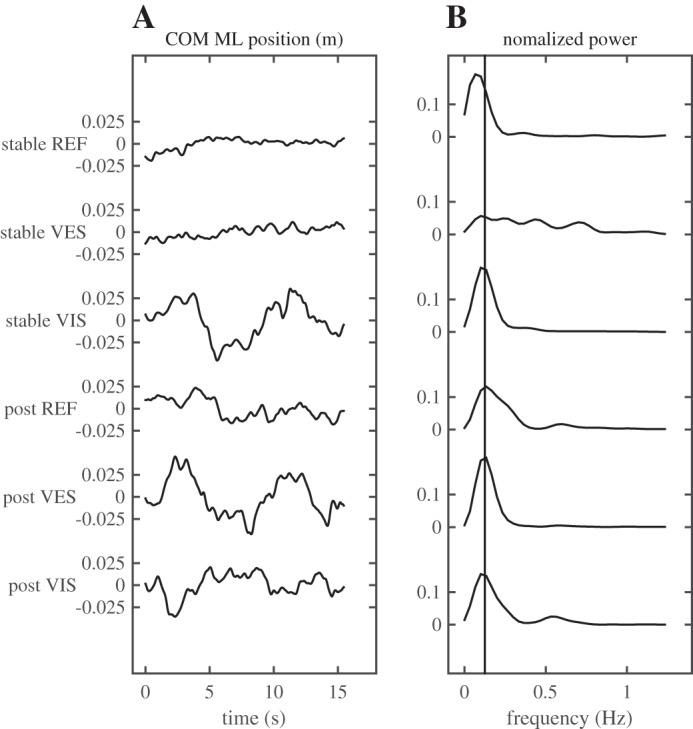
Typical example of the times series of the ML position of the COM (A) and its normalized power spectrum (B), comparing trials with and without sensory manipulations. The last three trials on the rigid and on the unstable surface are plotted here. Note the increase in COM excursion and the peaks in the power spectra at the 0.125 Hz frequency in trials with sensory manipulations. REF, reference; VES, vestibular manipulation (galvanic vestibular stimulation); VIS, visual manipulation (moving scenery).

At the group level, both sensory manipulations significantly increased the excursion of the COM (Figs. 10 and 11, P < 0.001, for both manipulations).

Fig. 10.

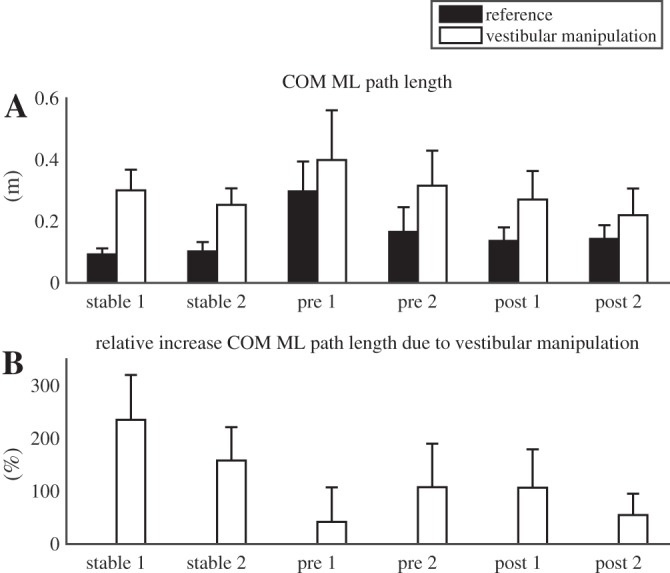
Mean values of the total excursion of the body COM in ML direction (A) and the relative effects (B) of the vestibular manipulation, surface condition and training on the ML excursion of the COM. Error bars indicate 1 SD.

Fig. 11.

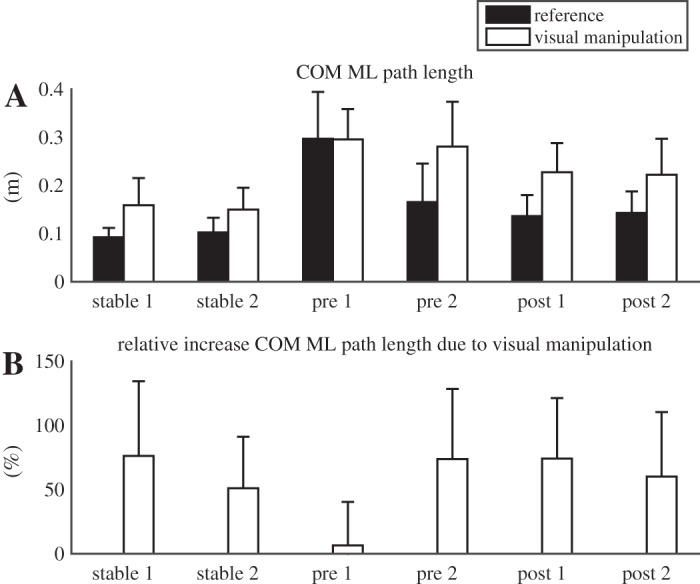
Mean values of the total excursion of the body COM in ML direction (A) and the relative effects (B) of the visual manipulation, surface condition, and training on the ML excursion of the COM. Error bars indicate 1 SD.

Sensory weighting and surface condition.

In contrast with our hypothesis, the effect of vestibular manipulation was smaller on the unstable surface after training than on the stable surface, as evidenced by a significant interaction of surface and vestibular manipulation (Fig. 10A; P < 0.001) and by a significantly smaller relative effect of vestibular manipulation on the unstable surface than on the stable surface (Fig. 10B, P < 0.001).

The effect of visual manipulation was not significantly dependent on the surface condition, i.e., the interaction effect was not significant (Fig. 11A; P = 0.111) nor was the difference in relative effect (Fig. 11B; P = 0.825).

Sensory weighting and training.

The effect of vestibular manipulation was dependent on training and trial, as evidenced by a significant three-way interaction (Fig. 10A, P = 0.044). We therefore compared adjacent trials in terms of absolute and relative effects of vestibular manipulation. Between the pretraining trials, a nonsignificant difference was found for the absolute effect of vestibular manipulation (P = 0.080) and a significant effect for the relative effect (P = 0.015), with a smaller effect in the first trial. No differences were found between the last trial pretraining and the first trial posttraining, while there were nonsignificant differences between the posttraining trials in absolute (P = 0.095) and relative (P = 0.063) effect of vestibular manipulation, with a smaller effect in the final trial.

Also, the effect of visual manipulation was dependent on training and trial as indicated by a three-way interaction (Fig. 11A, P = 0.011). Both absolute (P = 0.010) and relative (P = 0.004) effects of visual manipulation were different only between the pretraining trials, with the smaller effect on the first trial.

Associations Between Training Effects

As reported in Effects of training on balance performance, performance improved with training on both short and long time scales as evidenced by significant main effects of trial and training on the COM excursion. We had hypothesized that improvements in performance would be related to changes in either the motor strategy, reflected by the contribution of the hip strategy to COM acceleration and/or to upweighting of vestibular and/or visual information. To test these hypotheses, we correlated changes in performance between both pretraining trials and between the last pretraining trial and the first posttraining trial to concomitant changes in the contribution of Ḣ and in the effects of the sensory manipulations. In view of the unanticipated effects detected on the oscillations of the balance board, we performed the same analysis for this variable as a potential cause of improvements in performance. For changes between pretraining trials, the coefficient of correlation between decrease in COM excursion and the increase in effect of visual manipulation was significant (R = −0.80, Fig. 12). For the changes over the 30-min training period, the correlation between the improvement in performance and the decrease of the relative power of the board angle between 2.5 and 4.5 Hz was significant (R = 0.73, Fig. 12). These results indicate that the fast initial improvements are mainly due to upweighting of visual information, while the slower progress achieved over the 30-min training period is mainly due to suppression of the oscillatory ankle and balance board movements.

Fig. 12.

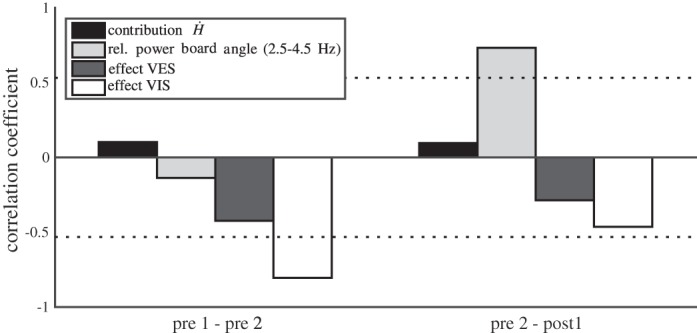
Pearson's correlation coefficients between changes between trials in balance performance (COM mediolateral path length) and changes between trials in the contribution of the derivative of the angular momentum (Ḣ) to COM acceleration in the relative power between 2.5 and 4.5 Hz of the board angle and in the effects of the vestibular and visual manipulation on COM excursion. Pre 1-pre 2 refers to the differences between the 2 pretraining trials. Pre 2-post 1 refers to differences between the 2nd pretraining trial and the 1st posttraining trial. The dashed horizontal line indicates the threshold for significance at α < 0.05.

DISCUSSION

The aims of this study were to assess motor strategies and use of sensory information in balancing on one leg on either a rigid or an unstable surface and to assess the changes that occur with learning to balance on the unstable surface. On the unstable surface, we expected participants to rely more on the hip strategy to control their COM than on the stable surface. In addition, we hypothesized that vestibular and visual manipulations would have stronger effects on the unstable compared with the rigid surface and that training would induce an increase in the use of the hip strategy and of visual and vestibular feedback. Balancing on one leg on the unstable surface was clearly more difficult than on the rigid surface, as evidenced by increased COM excursions and higher activity of the hip abductor and adductor muscles. Over the pretraining trials and the 30-min training period, participants showed a substantial decrease in COM excursions, while hip muscle activity decreased, indicating decreased effort. The largest part of the improvement in performance was achieved over the first set of six trials, which each lasted 16 s only. As hypothesized, an increase in the contribution of the changes in angular momentum to COM accelerations was found on the unstable surface, but in contrast with our hypothesis, with further training, the contribution of angular momentum changes to balance control decreased. Also in contrast with our hypothesis, weighting of visual information was not different between the stable surface and the unstable surface posttraining, while the vestibular information was even downweighted on the unstable surface compared with the stable surface. With practice, however, the effect of visual and vestibular manipulations increased rapidly, suggesting upweighting of these sensory modalities relative to the initial trials on the unstable surface. In addition, in the unstable condition, oscillatory movements of the balance board were seen, which, as will be discussed below, may be due to too high gains of proprioceptive feedback used to generate ankle moments. These oscillations disappeared with practice, suggesting downweighting of proprioceptive signals with learning to balance on the unstable surface. The improvement in balance performance over the initial trials was correlated with the increase in the effect of visual manipulation only, whereas the improvement in balance performance over the 30-min training period was correlated with the decrease in the relative power of the oscillations of the balance board only.

Motor Strategy

To our knowledge, this study is the first to provide insight into how the hip strategy, or more exactly, variations in angular momentum contribute to the control of mediolateral COM accelerations in balancing on one leg. It was found that this strategy was used in a consistent manner across trials and participants, as evidenced by the PCA and the limited effects of the experimental conditions on the relative contributions of the segments to total angular momentum. Previously, it has been suggested that the hip strategy in single-leg stance involves movements around the hip joint of the stance leg, with the upper body and the other leg moving as a single segment (Otten 1999). Here we showed that trunk momenta were to some extent inversely correlated with those of the stance leg and that the angular momenta of trunk, head, and arms were positively correlated. However, independent variations occurred in angular momentum of the free, nonstance leg, relative to the upper body, indicating a contribution by hip movements in the nonstance leg. Similarly for COM control in the anteroposterior direction, it has been shown that the hip strategy in fact involves rotations around multiple joints (Riemann et al. 2003) and consequently the label hip strategy may be a misnomer also from a kinematic perspective.

The hip and ankle strategy are not extremes of a behavioral continuum but rather two coexisting strategies used for balance control (Creath et al. 2005), which are mechanically independent (Hof 2007). In line with this, the COM accelerations were not fully accounted for by angular momentum changes. In fact, in the initial trials on the unstable surface, the two strategies co-existed and produced at least partially opposite effects on the COM, leading to the estimated effect of the hip strategy exceeding 100%. We suggest that changes in angular momentum may be used to control balance specifically when control actions exerted through ankle torques are inadequate. This would be in line with the dominance of the ankle strategy in less challenging conditions, as found here and in previous studies (Creath et al. 2005; Patel et al. 2008).

The PCA revealed independent contributions of the stance leg, of the swing leg, and of all other segments combined to the total angular momentum. Angular momentum changes of the stance leg are in part coupled to ankle torques and in part to movements of the COM relative to the leg and thus could be assumed not to be part of the hip strategy. This is confirmed by the negative covariance of the angular momentum of the stance leg with the other segments, especially the trunk (Fig. 7) and by the fact that the angular momentum of the stance leg reflected the high-frequency oscillations of the balance board in the initial conditions on this unstable surface (Figs. 2 and 5). Angular momentum of the other segments covaried positively, but occasional independent variations in angular momentum of the nonstance leg occurred, which we suggest are used to apply large and fast corrections in case of impending balance loss (Fig. 13). Future studies could address the question whether the use of the free leg to control balance is related to recruitment of muscle synergies that were shown to be used in more challenging balancing tasks only (Torres-Oviedo and Ting 2010).

Fig. 13.

Example of a participant decelerating COM movement with a sudden movement of the nonstance leg. The COM can be seen to start moving towards the right (positive) side after ∼3 s in the trial, coinciding with gradual tilting of the balance board (A). After 6 s in the trial the COM is decelerated, as reflected in a negative peak in the COM acceleration (B). This peak is the result of a left, nonstance leg movement initiated after ∼6 s as illustrated in C and D, which shows the orientation of the segments after subtraction of their mean values. This movement gives rise to the negative angular momentum of the free leg (E and F), which causes the negative acceleration of the COM that limits further right COM movement.

Motor strategy and surface condition.

On the unstable surface, the stance leg contributed slightly but significantly more to the total angular momentum than on the rigid surface, which could be due to the fact that the stance leg could move with the support surface and that large COM movements relative to the stance leg occurred. Also, the ipsilateral arm contributed slightly more, suggesting some covariance of the ipsilateral extremities, which was, however, not strong as it did not show in the results of the PCA.

As hypothesized and in line with previous literature (Creath et al. 2005; Otten 1999; Patel et al. 2008; Riemann et al. 2003), we observed an increase of the effect of the hip strategy, i.e., of changes in the body's angular momentum, on the mediolateral COM acceleration, when standing on the unstable surface. It cannot be ascertained that the changes in angular momentum were corrective. The COM acceleration represents the sum of corrective actions and perturbations of balance and these cannot be separated without imposing known perturbations, well exceeding the amplitude of naturally occurring perturbations. However, especially in the pretraining trials on the unstable surface, the RMS derivative of the angular momentum exceeded that of the COM acceleration, indicating that the hip and ankle strategies had opposing effects. It seems that in these cases angular momentum changes were used to correct accelerations arising from the ankle strategy, which appeared disruptive to balance given their oscillatory nature.

Oscillations between 2.5 and 4.5 Hz in board angle and COM acceleration occurred, in the pretraining trials and to a lesser extent in the post training trials on the unstable surface. To our knowledge such oscillations have not been described before, but they appear to be similar to oscillations that have anecdotally been reported occur in novices attempting to stand on a slackline (Keller et al. 2012). These oscillations can be observed in the COM position time series but are more readily apparent in the acceleration, due to the fact that these relatively high frequency components have a lower power in the position signal than in its second derivative, the acceleration signal. Mechanical considerations show that oscillations of the board orientation are causal in this chain of events, as tilting the board causes the COP to shift sideward and the distance between COM and COP causes a proportional COM acceleration (Hof 2007).

Depending on gain and delay, feedback loops can cause oscillatory behavior. Since both the gain and the phase (delay) introduced by the relation between ankle moments and COP position are dependent on the geometry and the dynamics of the unstable object, the ankle strategy needs to be adapted when standing on this object. Apparently, participants were not completely successful in doing so, especially not during the initial trials before training.

An oscillatory pattern was also visible in the EMG linear envelopes during the early trials on the balance board. This may indicate coordinated activation of hip and ankle muscles, as would be required to obtain single inverted pendulum behavior, or in other words, a pure ankle strategy. Alternatively, given the fact that stance leg, thigh, and trunk movements occurred almost in counterphase, the oscillatory hip muscle activity could be generated to produce hip moments driving upper body angular momentum changes to counteract ankle actions. However, since the relative power of the derivative of the total angular momentum between 2.5 and 4.5 Hz was below 6% in all conditions this appears unlikely. Mechanical simulations suggest that stance leg hip abduction and adduction moments are crucial for balance control in one-legged stance, especially when support conditions require the use of changes in angular momentum to control COM acceleration (Otten 1999). The phasic bursts in EMG data collected and the much higher variability of EMG amplitudes in the pretraining trials on the unstable surface may reflect such modulations in hip moments. Future studies should address the coordination between muscles around multiple joints to understand how muscle activity is organized to control COM accelerations in the frontal plane when balancing on one leg.

Motor strategy and training.

In contrast with our hypothesis, we found a gradual decrease in the contribution of the hip strategy over trials. This would fit with a preference for the ankle strategy and the use of the hip strategy for control when the ankle strategy is inadequate, due to high demands or erroneous control actions. Less corrective actions by changing angular momentum are needed when the ankle strategy is used more appropriately after learning the changed transfer function between ankle torques and COM accelerations. The increase of the ratios of the RMS of the segment angular momenta and the total angular momentum after the initial trials on the unstable surface (Fig. 6) indicates a movement pattern with more counterrotations. This coincided with a decrease in the total angular momentum and its contribution to COM accelerations (Fig. 8). This may reflect less coordinated movement of the individual segments, in line with reduced use of these movements as a strategy to control balance.

The use of the ankle strategy appeared to change with practice, as oscillations of the balance board and the COM were attenuated, indicative of a decreasing feedback gain. Also, the frequency of oscillations appeared to decrease over trials, again indicative of a reduced gain. However, the frequency of the peaks could not be identified with sufficient certainty in posttraining trials, so we refrained from quantitative analysis.

When a novel task in an unstable environment is learned, the central nervous system has to find a motor strategy that reduces errors and yet is energetically efficient (Mitrovic et al. 2010). Over trials and training muscle activity significantly decreased. These results match the results of studies on motor learning in response to environmental challenges in other tasks, for example, in making straight arm-movements under unstable conditions (Franklin et al. 2003). In the latter study, as in the present study, exposure to the unstable environment initially caused an increase in muscle activity, which has been interpreted as an attempt to reduce the kinematic error by increasing joint impedance (Franklin et al. 2007; Selen et al. 2006). With practice, as motor control becomes more accurate, muscle activity decreased, presumably minimizing energetic costs. In the present study, both the mean muscle activity and its coefficient of variation decreased with practice. While decreases in tonic activity, reflected in the decreased mean activity observed here, could be related to decreased hip joint impedance, changes in phasic activity, as reflected in the coefficient of variation, are likely related to decreased use of hip moments to generate changes in angular momentum.

Sensory Weighting

In the present study, we used vestibular and visual manipulations to evaluate to what extent these sensory modalities were used for balance control. Both manipulations increased COM excursions in the frontal plane as intended, and during the manipulations COM movement occurred predominantly at the frequency of the input signals (Fig. 9). It should be noted that the magnitudes of the effects of the vestibular and visual manipulations cannot be compared, since the amplitudes of the input signals were not scaled.

Sensory weighting and surface condition.

We hypothesized that vestibular and visual manipulations would have stronger effects on the unstable surface than on the rigid surface. However, a smaller effect of vestibular manipulation and no change in effect of visual manipulation were found. Ceiling effects are not a likely explanation for this finding, because COM displacements were larger in the pretraining trials than in the posttraining trials used for this comparison, and because balance loss was not more common on the unstable surface after training than on the rigid surface.

We suggest that participants increased proprioceptive feedback gains when first standing on the unstable surface, giving rise to oscillatory behavior when performing this novel and challenging balancing task (c.f. Davis et al. 2011; Horslen and Carpenter 2011), concomitant with downweighting of vestibular and visual information. Interestingly, oscillations increased and the effects of vestibular manipulation and visual stimulation tended to decrease between the posttraining trials. It could be that exposure to the manipulations led to some downweighting again of vestibular and visual information relative to proprioceptive information.

The decrease in GVS effects at the unstable surface may also have been due to the fact that head movements were in part self-initiated to generate changes in body angular momentum. A study in primates indicated that vestibular input arising from self-initiated movement is suppressed (Carriot et al. 2013). However, the information arising from the GVS did not arise from but merely coincided with self-initiated movements in the unstable surface condition.

Previous studies showed smaller backward displacements of the COM or COP when vibrating the calf muscles, to stimulate proprioceptive afferents, in stance on unstable surfaces compared with stable surfaces (Ivanenko et al. 1999; Kiers et al. 2012). These findings were interpreted as indicative of downweighting of proprioceptive information, which appears to be at odds with the findings and the rationale presented here. However, it should be noted that these postural shifts were measured well after the onset of muscle vibration. Initial COP shifts after switching on vibration are similar between standing on a rigid surface and on foam (Kiers 2014), which in view of the compliance of the foam even indicates a stronger response in the latter condition, in line with the increased proprioceptive feedback gain that we suggested above. Gains of short-latency responses based on proprioceptive feedback could be modulated independently of the weighting of proprioceptive information relative to other modalities in slower feedback loops, leading to differential effects of vibration on early and late postural responses, in line with models of postural control as presented, for example, by Goodworth and Peterka (2009). Such parallel feedback loops with independent gain setting could lead to a variable overall gain of the balance control loop. A reciprocal weighting of sensory information is often assumed to exist to avoid such changes in the overall feedback gain. However, empirical data do not consistently support a reciprocal nature of sensory reweighting at the level of the overall control loop (Andreopoulou et al. 2015; Polastri et al. 2012). It should be noted that sensory modalities not studied in the present or these previous studies may play a role. Specifically, sensation of the ground reaction force through pressure sensors in the skin can contribute to balance control (Maurer et al. 2006; Pasma et al. 2012). The signal-to-noise ratio of this source of information may be attenuated on an unstable surface and consequently its weighting may decrease, possibly maintaining a constant overall feedback gain. In this case, the oscillations observed would be due to an increase in the delay of the feedback loop due to the introduction of the unstable surface without a change in feedback gain.

Direct evidence for reduced gains of proprioceptive reflexes when balancing on unstable surfaces has also been reported (Chalmers and Knutzen 2002; Llewellyn et al. 1990; Trimble and Koceja 2001). The present results imply that such findings are dependent on the practice that participants have had on the unstable surface before taking the measurements and hence likely also on task difficulty. This may also explain why sitting on the same balance board as used in the present study, which is a much easier task, led to an immediate increase in effects of visual and vestibular manipulations (Andreopoulou et al. 2015).

Sensory weighting and training.

Over the initial trials before the 30-min training period, evidence was found for upweighting of visual and vestibular information, although this did not lead to larger effects of the sensory manipulations compared with standing on the rigid surface. One might suspect that small effects of vestibular and visual manipulations on the first pretraining trials were due to ceiling effects, since sway in these trials was large and possibly could not increase any further without balance loss. This would lead to an overestimation of training effects. However, sway was substantially larger in the trials with the vestibular manipulation than in the trials with the visual manipulation. Therefore, a ceiling effect can only be assumed for the vestibular manipulations in the first trials pretraining. The number of contacts with the support rail for these trials did not clearly increase with the vestibular manipulation, which argues against such a ceiling effect. Most importantly, upweighting of visual information with learning to balance on the unstable surface appeared most effective and is less likely to be overestimated.

Nevertheless, these training effects indicate that practice led to fast reweighting of sensory information for balance control in the expected direction, i.e., upweighting of visual and vestibular inputs. More indirectly, the decrease in the oscillations of the balance board indicates that the gain of proprioceptive feedback decreased with practice. This occurred both on a short and on a longer time scale as evidenced by differences among pretraining trials and between pre- and posttraining trials, with one of the participants even showing clear oscillations posttraining. In line with decreased proprioceptive reflex gains, reductions in H reflex as well as stretch reflex amplitudes were shown after long-term (weeks) balance training (Gruber et al. 2007; Keller et al. 2012; Taube et al. 2007b; Trimble and Koceja 2001). These reductions in reflex gains have been argued to be presynaptic and probably supraspinal in nature (Gruber et al. 2007; Keller et al. 2012; Taube et al. 2007b). In the present study, substantial reductions in EMG activity occurred with training and postsynaptic effects can thus not be excluded. While presynaptic inhibition of proprioceptive afferent would offer an elegant explanation for both the decrease in the oscillations and the changes in effects of the sensory manipulations, these processes appeared to occur on different time scales, with the former changing during the initial trials to remain constant thereafter and the latter still changing during the 30-min training.

Interestingly, during the last set of trials the effect of vestibular manipulation tended to decrease, while oscillations reappeared. It might be that the preceding trial with galvanic stimulation led to an upweighting of proprioceptive and downweighting of vestibular input.

Associations Between Training Effects

We found no significant correlations between changes in sway path and changes in the use of the hip strategy and in fact correlation coefficients were positive, indicating lower performance with more use of the hip strategy. As outlined above, we suggest that the hip strategy is used when balance cannot adequately be controlled by ankle moments. The preference for using the ankle strategy could be based on energetic efficiency, and the decrease in the effect of the derivative of the angular momentum on COM acceleration might be associated with decreasing effort. More EMG data than measured here or data on oxygen consumption would be required to test such a hypothesis.

The initial improvement in balance performance, occurring before the 30-min training period, was positively correlated with the change in magnitude of the effect of the visual manipulation, suggesting that the latter may cause this fast training effect. The changes in performance over the 30-min training period were positively correlated with the decrease in the oscillations of the balance board, again as argued above, an indication of a change in the use of sensory information as a causal factor underlying improvement of balance control. Taube et al. (2007a) reported significant positive correlations of improvements in balance performance with decreases transcranial magnetic stimulation-conditioned H reflexes but not with normal H reflexes, suggesting that cortical changes underlie improved performance through inhibitory effects on proprioceptive afferents. Participants who were exposed to muscle vibration during training on a balance board outperformed participants who were not (Nguyetnat 2011), suggesting that the former, not being able to use proprioceptive information for maintaining balance due to the vibration, were forced to use the more pertinent information and therewith achieved more robust training results. All in all, these findings indicate that balance training effects are to a large extent dependent on changes in the use of sensory information in balance control and not directly on changes in motor strategies. It should be kept in mind that our participants most likely were able to improve performance more than achieved within the short time frame of this study, and a role of changes in motor strategies at longer time scales cannot be excluded.

Limitations

During the measurements, participants could grab a rail on either side of them to prevent falls. The number of times that the rail was touched was recorded (Table 1) but was not taken into account when expressing performance as COM path length. Participants touched the support rail more frequently in the unstable condition and when sensory manipulations were applied, which would have caused an underestimation of surface and manipulation effects. On the other hand, the dynamics of the unstable support are likely to amplify effects of sensory manipulations.

To avoid fatigue and unwanted training effects, we used very short data series. This limited interpretation of in particular the EMG linear envelopes and also had a negative effect on reliability and hence on study power for all frequency domain measures that were used.

The effects of surface condition were analyzed based on data collected after a training period of arbitrary length. As discussed above, this may be critical for some of the findings on effects of surface condition as performance and the underlying mechanisms were probably still evolving. On the other hand, these comparisons were more conservative than comparisons to the initial trials on the unstable surface would have been.

Conclusion

In one-legged balancing on a surface, which was unstable in the frontal plane, participants used changes in angular momentum to control balance more than on a stable surface. Surface conditions did not change the effects of a manipulation of visual input, while the effects of vestibular information were decreased on the unstable surface. With practice in balancing on the unstable surface, participants rapidly improved balance performance. Initial improvements were associated with upweighting of visual information, while later improvements were associated with the suppression of oscillatory ankle movement that we suggest may be attributable to excessive proprioceptive feedback gains.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the author(s).

AUTHOR CONTRIBUTIONS

Author contributions: J.H.v.D. and M.v.L. conception and design of research; J.H.v.D., M.v.L., and G.S.F. analyzed data; J.H.v.D., M.v.L., and G.S.F. interpreted results of experiments; J.H.v.D. prepared figures; J.H.v.D. drafted manuscript; J.H.v.D., M.v.L., and G.S.F. edited and revised manuscript; J.H.v.D., M.v.L., and G.S.F. approved final version of manuscript; M.v.L. performed experiments.