1. Introduction
Emergency physicians face numerous questions regarding proper management of patients and selection of the best laboratory test or imaging every day. Knowledge on screening performance characteristics of the diagnostic tools used in this department plays an important role in finding the answers to these questions. We assessed these characteristics in the previous parts of educational article series in Emergency journal (-). In the present manuscript, we will describe how to use these screening characteristics for selecting the right diagnostic tools.
Overall, in managing a patient in emergency department (ED), commonly the most probable cause of the problem is considered and diagnostic and treatment measures are based on that. There are various sources for determining the initial probability of a cause, called pre-test probability in this article. Usually, the examiner’s experience, prevalence of the disease at the time of patient presentation, and clinical decision rules are the 3 most important and major sources in determining pre-test probability.
Pre-test probability is either high enough to guarantee the initiation of treatment, or low enough to disprove the presence of the disease. A problem arises when this probability is average and one or more diagnostic tools are required for confirmation. Therefore, our aim when selecting a suitable test, is practically converting an average pre-test probability to a higher post-test probability that guarantees initiation of treatment or intervention. The most important characteristic among screening performance characteristics of a test used for this purpose is likelihood ratio. Theoretically, post-test probability is calculated by multiplying pre-test probability and likelihood ratio. Yet practically, it is not that simple, because probability cannot be divided and multiplied by nature.
Therefore, first we should convert probability to a dividable and multipliable measure called odds. In the present article, we will discuss how to convert probability to odds and vice versa, and calculate post-test probability.
2. Definition
2.1 Probability : It is a measurement tool for expression of likeliness. It is quantified from 0 to 1 and tells us that how certainly the event will occur (4, 5).
2.2. Odds : It is defined as “the ratio of the probability of an event happening to that of it not happening”. Odds is quantified from zero to infinity (4, 6).
2.3. Pre-test probability : It is defined as the probability of the screened person having the disease (7).
2.4. Pre-test odds = Pre-test probability/ [1 – Pre-test probability]
2.5. Post-test probability : It is the probability of the patient having a disease after obtaining the test results (7).
2.6. Example 1: If the prevalence of disease X among a certain population is 25%, the pre-test probability of this disease will be 0.25. From this, we can calculate the pre-test odds as follows:
Pre-test probability = 0.25
Pre-test odds = 0.25/ (1-0.25) = 0.25/ 0.75 = 0.33
We should know the likelihood ratio of the test selected for rule in/out of probable disease.
Post-test odds = Pre-test odds × Likelihood ratio
If the likelihood ratio of this test was 10, the post-test odds can be calculated as follows:
Post-test odds = 0.33 × 10 = 3.3
After calculation of post-test odds, using the same equation, we should convert it to post-test probability.
Post-test probability = Post-test odds / [Post-test odds + 1]
Post-test probability = 3.3 / (3.3 + 1) = 0.76
This means that, if the result of the assumed test was positive for this patient, probability of disease rises from 0.25 to 0.76, which may guarantee initiation of treatment.
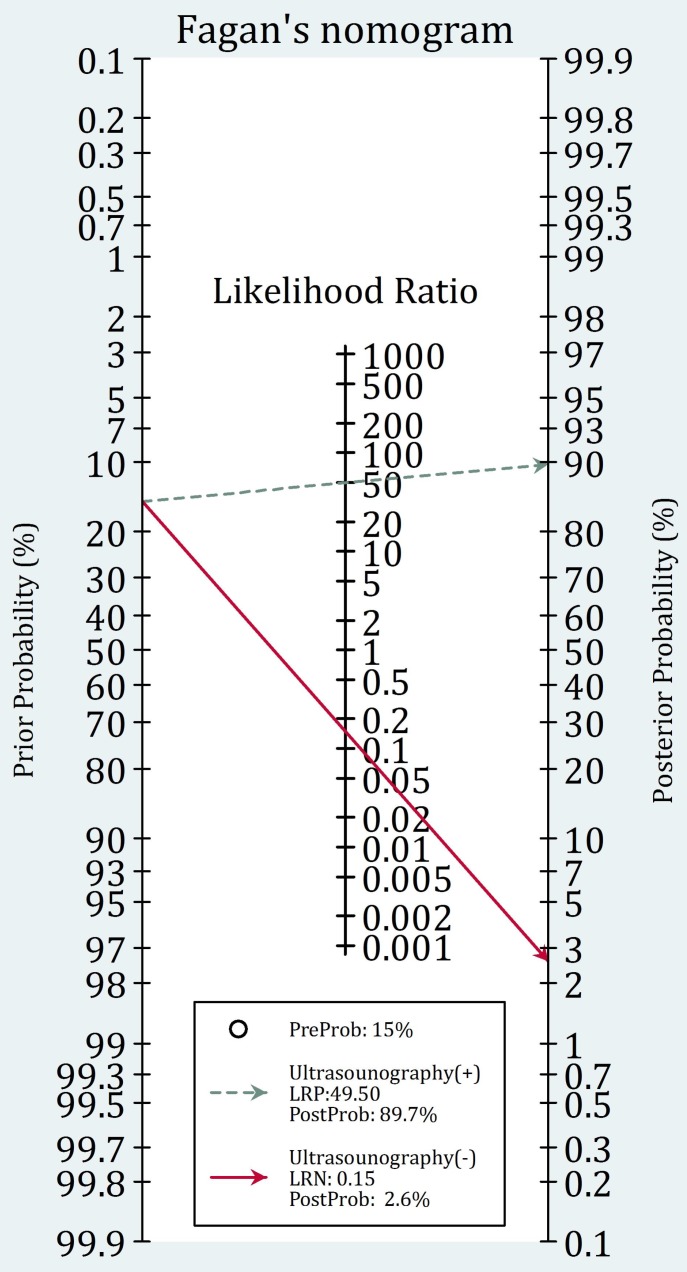
2.7 Example 2: The likelihood ratio of ultrasonography in detection of traumatic lens dislocation was estimated to be 49.5 in a study by Haghighi et al. (8). Considering 15% prevalence of lens dislocation in an example population, please calculate post-test probability of lens dislocation in patients with unilateral blindness following direct eye trauma and positive finding in ultrasonography for lens dislocation.
Pre-test probability of lens dislocation = 0.15
Pre-test odds = 0.15 / (1-0.15) = 0.15 / 0.85 = 0.18
Post-test odds = 0.18 × 49.5 = 8.91
Post-test probability = 8.91 / (8.91 + 1) = 0.90
This means that, ultrasonography is a good choice for rule in of lens dislocation in traumatic patients, because it could raise a low probability of 0.15 to 0.90.
3. An alternative way for calculating post-test probability
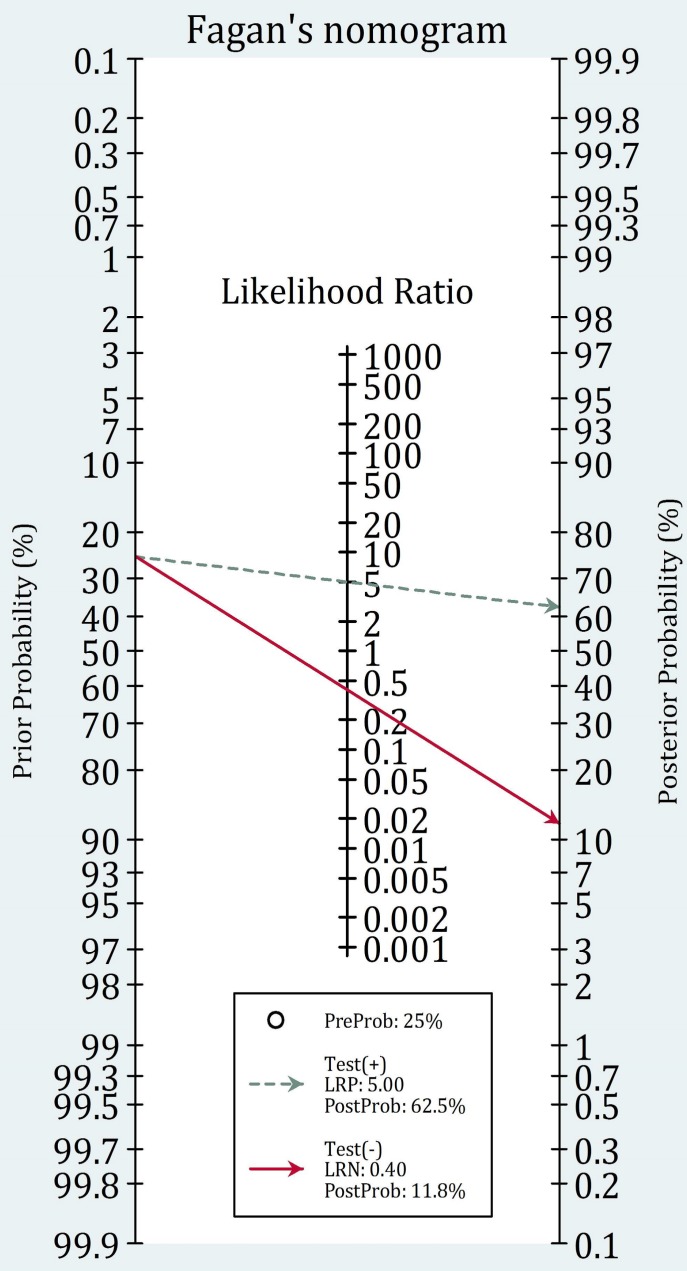
We can also use a shortcut for calculating post-test probability without converting probability to odds. This alternative solution is Fagan’s nomogram (figure 1). It is a graphical tool used for calculating post-test probability, knowing pre-test probability and likelihood ratio (9, 10).
Figure 1.
Fagan’s nomogram
4. Interpretation of Fagan’s Nomogram
In this nomogram the left axis represents the pre-test probability, middle axis represents likelihood ratio, and the right axis shows post-test probability. Initially, we find and mark pre-test probability and likelihood ratio values on the left and middle axes, respectively. Then a straight line is drawn from the two marked points along to the right axis. The point at which the line crosses the left axis is the value of post-test probability.
4.1. Example 3: Assume that the prevalence of a certain disease is 25% and the positive and negative likelihood ratios of the chosen test are 5 and 0.4, respectively. As shown in figure 2, post-test probability will be 62.5 and 11.7 as revealed by Fagan’s nomogram.
Figure 2.
Fagan’s nomogram of example 3
For confirmation, we will calculate the post-test probability through the equations discussed before in this article:
Pre-test probability = 25% = 0.25
Pre-test odds = 0.25/ (1-0.25) = 0.25/ 0.75 = 0.33
Post-test odds = 0.33 × 5 = 1.65 (for positive likelihood ratio)
0.33 × 0.4 = 0.132 (for negative likelihood ratio)
Post-test probability = 1.65 / (1.65 + 1) = 0.625 = 62.5%
0.132 / (0.132 + 1) = 0.117 = 11.7%
4.2. Example 4: Let’s estimate the post-test probability of example 2 using Fagan’s nomogram, figure 3:
Figure 3.
Fagan’s nomogram of example 4
References
- 1.Baratloo A, Hosseini M, Negida A, El Ashal G. Part 1: Simple Definition and Calculation of Accuracy, Sensitivity and Specificity. Emergency. 2015;3(2):48–9. [PMC free article] [PubMed] [Google Scholar]
- 2.Safari S, Baratloo A, Elfil M, Negida AS. Part 2: Positive and negative predictive values of diagnostic tests. EMERGENCY-An Academic Emergency Medicine Journal. 2015;3(3):87–8. [PMC free article] [PubMed] [Google Scholar]
- 3.Baratloo A, Elfil M, Negida A. Part 3: Positive and Negative Likelihood Ratios of Diagnostic Tests. Emergency-An Academic Emergency Medicine journal. 2015;3(4):170–1. [PMC free article] [PubMed] [Google Scholar]
- 4.Fulton LV, Mendez FA, Bastian ND, Musal RM. Confusion Between Odds and Probability, a Pandemic? Journal of Statistics Education. 2012;20(3):n3. [Google Scholar]
- 5.Wassertheil-Smoller S, Smoller J. A Little Bit of ProbabilA Little Bit of Probability. Biostatistics and Epidemiologyity. Springer; 2015. pp. 17–25. [Google Scholar]
- 6.Bland JM, Altman DG. The odds ratio. Bmj. 2000;320(7247):1468. doi: 10.1136/bmj.320.7247.1468. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 7.Parikh R, Parikh S, Arun E, Thomas R. Likelihood ratios: clinical application in day-to-day practice. Indian journal of ophthalmology. 2009;57(3):217. doi: 10.4103/0301-4738.49397. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 8.Haghighi SHO, Begi HRM, Sorkhabi R, Tarzamani MK, Zonouz GK, Mikaeilpour A, et al. Diagnostic Accuracy of Ultrasound in Detection of Traumatic Lens Dislocation. Emergency. 2014;2(3):121–4. [PMC free article] [PubMed] [Google Scholar]
- 9.Akobeng AK. Understanding diagnostic tests 2: likelihood ratios, pre‐and post‐test probabilities and their use in clinical practice. Acta Paediatrica. 2007;96(4):487–91. doi: 10.1111/j.1651-2227.2006.00179.x. [DOI] [PubMed] [Google Scholar]
- 10.Caraguel CG, Vanderstichel R. The two-step Fagan's nomogram: ad hoc interpretation of a diagnostic test result without calculation. Evidence Based Medicine. 2013;18(4):125–8. doi: 10.1136/eb-2013-101243. [DOI] [PubMed] [Google Scholar]