Abstract
Background:
Controversies about the rational positioning of the tibial component in unicompartmental knee arthroplasty (UKA) still exist. Previous finite element (FE) studies were rare, and the results varied. This FE study aimed to analyze the influence of the tibial component coronal alignment on knee biomechanics in mobile-bearing UKA and find a ration range of inclination angles.
Methods:
A three-dimensional FE model of the intact knee was constructed from image data of one normal subject. A 1000 N compressive load was applied to the intact knee model for validating. Then a set of eleven UKA FE models was developed with the coronal inclination angles of the tibial tray ranging from 10° valgus to 10° varus. Tibial bone stresses and strains, contact pressures and load distribution in all UKA models were calculated and analyzed under the unified loading and boundary conditions.
Results:
Load distribution, contact pressures, and contact areas in intact knee model were validated. In UKA models, von Mises stress and compressive strain at proximal medial cortical bone increased significantly as the tibial tray was in valgus inclination >4°, which may increase the risk of residual pain. Compressive strains at tibial keel slot were above the high threshold with varus inclination >4°, which may result in greater risk of component migration. Tibial bone resection corner acted as a strain-raiser regardless of the inclination angles. Compressive strains at the resected surface slightly changed with the varying inclinations and were not supposed to induce bone resorption and component loosening. Contact pressures and load percentage in lateral compartment increased with the more varus inclination, which may lead to osteoarthritis progression.
Conclusions:
Static knee biomechanics after UKA can be greatly affected by tibial component coronal alignment. A range from 4° valgus to 4° varus inclination of tibial component can be recommended in mobile-bearing UKA.
Keywords: Finite Element Analysis, Inclination, Mobile-bearing, Strain, Unicompartmental Knee Arthroplasty
INTRODUCTION
Unicompartmental knee arthroplasty (UKA) has regained popularity in recent years as an excellent treatment for anteromedial osteoarthritis of the knee. With more functional anatomy maintained, UKA can offer more rapid recovery and better restore the kinetics of knee than total knee arthroplasty (TKA).[1] Due to refined surgical techniques and strict patient selection, long-term survivorship of UKA has been greatly enhanced.[2]
However, good outcomes of UKA can be compromised by the postoperative complications. Persistent medial knee pain after UKA has bothered many patients. Although in most cases the pain settles during the 1st year after surgery, in a few patients, it will continue or worsen with time. Another issue – aseptic loosening of the tibial component, will often make the revision unavoidable.[3] The two main complications have been attributed to abnormal tibial bone stress/strain, which are supposed to be greatly related with the coronal mal-alignments of the tibial component, according to previous finite element (FE) studies.[4,5,6]
Although these studies gave some advice about the coronal positioning of the tibial tray, their results varied. Based on an FE model of fix-bearing UKA, Iesaka et al.[4] found that the stress at the proximal medial cortex of tibia increased as the tibial component was turned from valgus to varus; Sawatari et al.[5] found that slight valgus position may get more even stress distribution of cancelous bone and reduce the contact pressure. So they both prefer the slight valgus inclination. While Simpson et al.[6] found the inclination angles had minimal influence on the bone strain in Oxford UKA except for the 2° varus inclination. Besides, these studies all had some important limitations. The baseline FE models used in these studies had not included the femur bone, and some of them were not validated. Meanwhile, separated loads were applied to the self-defined points or areas on the tibial plateau in these studies, which were not the physiological loading conditions of the knee joint.
The aim of this FE study was to analyze the influence of the tibial component coronal alignment on the static knee biomechanics in mobile-bearing UKA, and try to find a rational range for the inclination angles, based on the self-developed UKA FE models that contained main structures of knee joint.
METHODS
Intact knee model
The intact knee model geometry was developed from computed tomography (CT) and magnetic resonance imaging (MRI) scans of the left knee joint of a 40-year-old healthy male volunteer (height 175 cm, weight 70 kg). CT (setting: 120 kV, 150 mA, slice thickness: 1 mm) was used to identify bone structure, and MRI (setting: Echo time 36 ms, repetition time 1300 ms, slice thickness: 1 mm, flip angle 90°) was used to identify cartilage, menisci, and four principal ligaments: Anterior cruciate ligament (ACL) and posterior cruciate ligament (PCL), lateral collateral ligament (LCL), and medial collateral ligament (MCL). The image data were then imported into the image processing software Mimics 17.0 (Materialise Ltd., Leuven, Belgium) to extract the geometry and to generate three-dimensional (3D) models of all structures. The standard tessellation language format files exported from Mimics were entered into Rapidform 2006 (INUS Technology, Inc., Seoul, South Korea) to form solid models. Then the solid models were imported into the FE analysis software Abaqus/Standard 6.10 (Dassault Systemes Simulia Corp., Providence, RI, USA) for assembling [Figure 1a].
Figure 1.
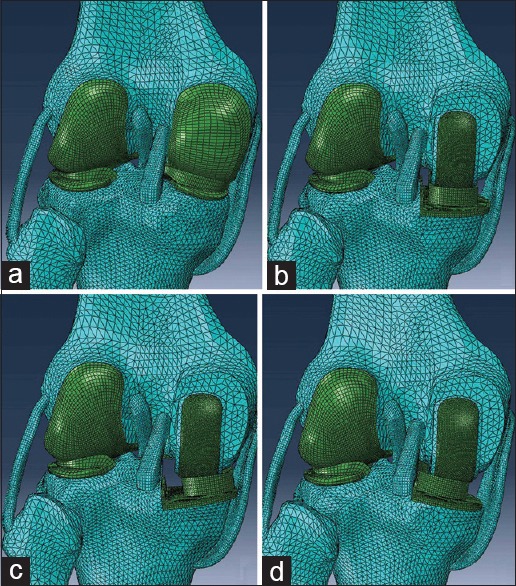
Four major finite element models used in analyses. (a) Intact knee model. (b) Unicompartmental knee arthroplasty model with neutrally aligned tibial tray. (c) Unicompartmental knee arthroplasty model with 10° valgus tibial tray. (d) Unicompartmental knee arthroplasty model with 10° varus tibial tray.
Cartilage, menisci, cortical, and cancelous bone were all considered as linear elastic isotropic material [Table 1].[7,8,9] Ligaments were considered isotropic and hyperelastic material, represented by an incompressible Neo-Hookean behavior with the energy density function: Ψ = C1 (I1 − 3), being C1 the initial shear modulus and I1 the first modified invariant of the right Cauchy–Green strain tensor. C1 values were 6.06, 6.43, 5.83, and 6.06 MPa for the LCL, MCL, ACL, and PCL, respectively.[10] Interfaces between cartilage and bones were modeled as fully bonded.[11] Both menisci were attached to the tibia at the horns.[12]
Table 1.
Material properties incorporated into the FE models
| Items | Modulus of elasticity (MPa) | Poisson’s ratio |
|---|---|---|
| Cortical bone | 17,000 | 0.30 |
| Cancelous bone | 350 | 0.25 |
| Cartilage | 15 | 0.46 |
| Meniscus | 27.5 | 0.33 |
| CoCrMo ally | 195,000 | 0.30 |
| UHMWPE | 685 | 0.40 |
CoCrMo ally: Cobalt–chromium–molybdenum ally; UHMWPE: Ultra-high-molecular-weight-polyethylene; FE: Finite element.
Six contact pairs were set in the intact knee model: Three at the medial compartment and three at the lateral (femoral cartilage-meniscus, meniscus-tibial cartilage, and femoral cartilage-tibial cartilage). Contact condition in all articulations adopted a finite sliding frictionless hard contact algorithm with no penetration.[13]
Unicompartmental knee arthroplasty models
Solid models of Oxford UKA (Biomet UK Ltd., Swindon, UK) offered by the manufacturer were imported into the intact knee model. Size “M” was chosen for both the femoral component and the insert, and size “C” was chosen for the tibial tray. Bones were trimmed and implanted with the prostheses virtually according to the standard surgical techniques in Abaqus/Standard 6.10.[14] The neutrally aligned tibial tray was defined as having a square (0°) inclination in the coronal plane incorporating a 7° posterior slope [Figure 1b]. A rotating axis was defined that paralleled to the lateral edge of the tibial tray and passed through the center of the femoral component peg. Based on the neutral position, tibial tray and bearing were rotated about the axis, and a total of 10 varus-valgus mal-alignments of the tibial tray in the coronal plane were modeled while maintaining 7° slope, without changing the height of joint surface: Up to 10°, in 2° increments, in valgus, and up to 10°, in 2° increments, in varus [Figure 1c and 1d]. For unifying boundary conditions, mal-alignments of femoral component were not modeled, nor were the changes of lower limb alignment after UKA.
The femoral component and tibial tray in these models were fully bonded to the femur and tibia bone, respectively, simulating the use of cement.[15] Medial tibia plateau in each UKA model was fully covered by the same size of the tibial tray, and the component overhang was all <3 mm. The mobile-bearing was free to translate and rotate with respect to the surface of the tibial tray.[16]
The material of the femoral component and tibial tray was cobalt–chromium–molybdenum ally, and the bearing was considered as ultra-high-molecular-weight-polyethylene. All the materials were assumed to be linear elastic isotropic [Table 1].[16] A friction coefficient of 0.07 was used for contact between the bearing and metal components.[17]
Mesh definition
Bone structures in all defined models were meshed by tetrahedral elements, and the other structures were meshed by hexahedral elements. Convergence test was conducted on element size for the tibial bone to ensure that peak von Mises stresses did not change by over 5%. This criterion was met by a mesh size of 2.0 mm, giving 212,128 elements for intact knee model and 217,287–226,821 elements for UKA models [Figure 1].
Loading and boundary conditions
To validate the intact knee model, a compressive axis load of 1000 N, which was consistent with the load magnitude in former studies,[4,5] was applied to the mid-point of the transepicondylar axis in the femur.[18] The femur was constrained only in flexion-extension while the tibia and fibula were completely fixed at their distal ends.[18,19]
Then the intact knee model and eleven UKA models were applied the same load as above, with the femurs only free to rotate in varus-valgus referring to previous in vitro experiment,[20] other boundary conditions remaining the same.
Analyses
In the step of validation, the results of load distribution, contact pressures, and contact areas in intact knee model were extracted and compared with previous studies.
For evaluating bone stress/strain quantitatively, we defined five regions of interest (ROIs) on the proximal tibia [Figure 2]. ROI 1 was defined for investigating the source of residual pain. It was located on the proximal anteromedial cortical bone surface in all models, which was 15 mm below the tibial medial condylar articular surface of intact knee, with the same size of 1450 mm2, and its geometry was not affected by the different bone resection levels. ROI 2 was defined at the resection corner between the sagittal and transverse tibia bone cuts. Another three ROIs were located on the cancelous bone surface below the tibial tray, with ROI 3 medial to the keel slot, ROI 4 lateral to the keel slot, and ROI 5 at the keel slot.
Figure 2.

Locations of five regions of interests defined in the study.
Bone stresses/strains at the ROIs in each model were extracted and analyzed, as well as the load percentage and contact pressures in bilateral compartments. All the FE simulations were performed in Abaqus/Standard 6.10.
RESULTS
Validation of intact knee model
Load distributions in the intact knee model were very close to the results of in vitro experiments.[21] Under 1000 N compressive load, the lateral and medial compartment carried 44.9% and 55.1% of the total load respectively. About 69% of the total load was transferred by the menisci. The lateral meniscus carried 75% of the load present in the lateral compartment while the medial meniscus carried 64% of the load present in the medial compartment.
The results of contact pressures were similar to previous studies.[18] The peak tibial contact pressure, which occurred in uncovered cartilage at the lateral and medial tibial plateau, was 2.43 and 2.68 MPa respectively. While the peak contact pressure of the lateral and medial meniscal was 2.47 and 2.55 MPa respectively.
The FE results of contact area were all in the range of the experimental average deviations.[22] The magnitude of the total tibial contact area in the intact knee model was 1015.8 mm2, and the contact area between menisci and tibia accounted for 74% of the total contact area. Under the compressive loads of 200, 500, and 1000 N, the contact areas in the medial side of tibial plateau were 424.7, 506.8, and 580.3 mm2, respectively; and the contact areas in the lateral side of tibial plateau were 321.6, 372.3, and 435.5 mm2, respectively.
Unicompartmental knee arthroplasty models
Except for the resected surface of the tibia, both the peak von Mises stress and minimum principal (compressive) strain of medial cortical bone surface occurred at ROI 1 [Figure 3]. The peak values of von Mises stress and compressive strain were 6.01 MPa and 362 με at ROI 1 in the intact knee model, which increased by 18.9% (7.14 MPa) and 18.4% (428 με), respectively in the neutrally implanted UKA model. In the range from 10° varus to 10° valgus, the peak von Mises stress at ROI 1 gradually increased from 5.19 MPa to 12.53 MPa, and the peak compressive strain increased from 312 με to 754 με [Figure 4]. Valgus inclinations >4° led to a substantial increase of 68% and 42% in both the peak von Mises stress and compressive strain, compared with the intact knee model and the 0° inclination UKA model respectively.
Figure 3.
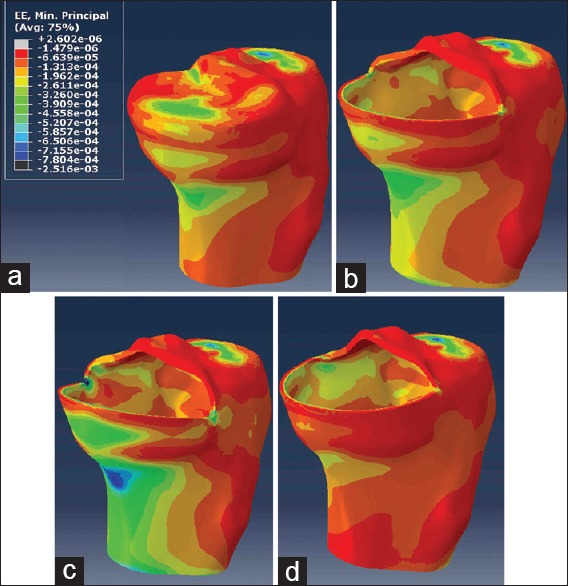
Minimum principal strains of tibial cortical bone in four major models. (a) Intact knee model. (b) Unicompartmental knee arthroplasty model with neutrally aligned tibial tray. (c) Unicompartmental knee arthroplasty model with 10° valgus tibial tray. (d) Unicompartmental knee arthroplasty model with 10° varus tibial tray.
Figure 4.
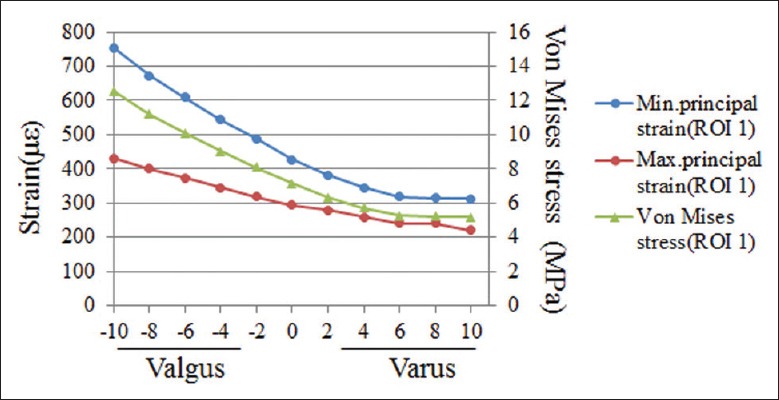
Peak von Mises stresses and minimum principal strains at regions of interest 1 in all unicompartmental knee arthroplasty models.
In all UKA models, ROI 2 had the peak maximum principal (tensile) strain of cancelous bone surface, which increased from 4211 με to 9412 με and showed a general upward trend in the range from 10° varus to 10° valgus, while the peak compressive strain at ROI 2 did not change obviously [Figures 5 and 6]. Although the tensile and compressive strains at ROI 3 and ROI 4 had the same changing trends, the two regions were mainly dominated by the compressive strain [Figures 5 and 6]. In the range from 0° to 10° valgus, the peak compressive strain at ROI 3 increased from 1241 με to 1377 με, and it increased from 1241 με to 1418 με as the tray was turned to 10° varus. As for ROI 4, the peak compressive strain increased from 317 με to 715 με in the range from 10° valgus to 10° varus [Figure 6]. ROI 5 was mainly dominated by the compressive strain, the peak value of which increased from 3046 με to 4183 με with the increasing varus inclination. The peak compressive strains at ROI 5 were all >4000 με with the varus angles >4° [Figure 6].
Figure 5.
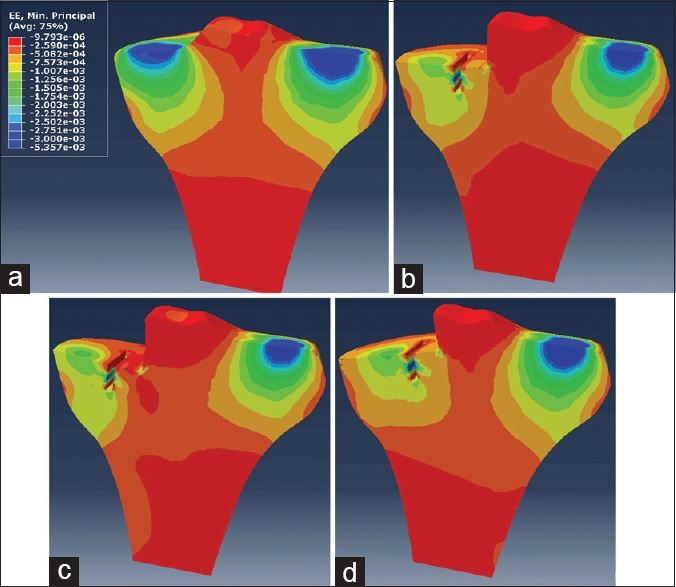
Minimum principal strains of tibial cancelous bone in four major models. (a) Intact knee model. (b) Unicompartmental knee arthroplasty model with neutrally aligned tibial tray. (c) Unicompartmental knee arthroplasty model with 10° valgus tibial tray. (d) Unicompartmental knee arthroplasty model with 10° varus tibial tray.
Figure 6.
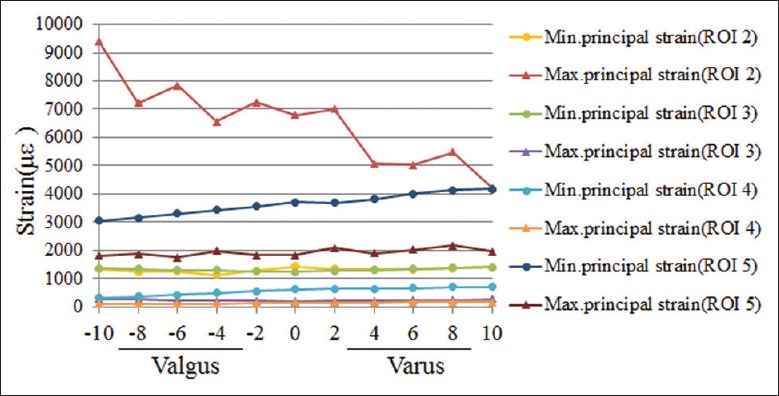
Peak maximum and minimum principal strains at regions of interest 2–5 in all unicompartmental knee arthroplasty models.
The load percentage in the medial compartment of UKA models all increased over the value of 54.6% in the intact knee. In the range from 10° valgus to 10° varus, the load percentage value decreased from 60.9% to 58.5% gradually, and the peak contact pressures of femoral and tibial cartilage in the lateral compartment slightly increased from 2.48 and 2.55 MPa to 3.02 and 3.08 MPa, respectively. Peak contact pressures of the bearing increased from 8.4 MPa to 19.5 MPa and 23.1 MPa, respectively, as the tibial tray was turned from 0° to 10° valgus and to 10° varus inclination.
DISCUSSION
Accurate mechanical axis restoration and correct implant positioning have been shown to be major contributors to improving the implant longevity and clinical outcomes of UKA.[23] There is still no general agreement on the optimal position of the tibial component. This FE study suggested that the static knee biomechanics changed a lot after mobile-bearing UKA, and it can be greatly affected by the coronal alignments of the tibial component.
Being the foundation of this study, the intact knee model had undertaken a series of rigorous validation steps and the results correlated well with previous experimental or FE studies. Therefore, the UKA models founded in this study and the following analyses can be considered as reasonable.
Tibial bone stress and strain after UKA were the first concerns of this study, which were believed to affect greatly on the bone modeling/remodeling process and the performance of the tibial component. According to former studies, critical damage strain thresholds above 2500 με in tensile strain and 4000 με in compressive strain may reduce the capacity for bone remodeling, leading to bone degeneration.[24] While bone strains lower than 100 με, resulting from stress-shielding effect will induce the disuse-mode remodeling and cause bone loss.[25]
Cortical bone always bears most of the load transferred by the tibial tray as its elastic modulus is much larger than that of cancelous bone. Abnormally high stress/strain of proximal medial cortical bone have been used to explain the persistent pain after UKA.[6] In this study, we found the implantation of a neutrally implanted tibial tray increased the peak von Mises stress and compressive strain within ROI 1 by nearly 20%, similar to Pegg's study.[26] Meanwhile, the peak stress/strain at ROI 1 showed the trend of rapid growth with the more valgus inclination, especially when the valgus angles were >4°, which appeared contrary to previous studies.[4,5,6] Our results may be explained by the changing of load location and transmission due to bearing movement.[27] The mobile-bearing always follows the position of the femoral component due to the spherical articulating surfaces. With valgus inclinations of tibial tray, the femoral component will sublux on the bearing, so the bearing will tip and move away from the tray wall in response, thus the load will be shifted medially and cortical bone strain will be increased, which are just the opposite to the conditions of varus inclinations.
Although the strain values under the 1000 N axial load were not supposed to cause intense bone remodeling and degeneration, we could infer that valgus inclinations >4° would induce dramatically increase in bone strains at ROI 1 and the risk of anteromedial pain during higher intensity activities, like step-up or stair climbing. Therefore, we thought that it should be discreet to make the valgus placement of tibial component not to exceed 4° intraoperatively. Besides, our results were found to correlate with the Liddle's study,[28] which reported six patients who underwent cementless Oxford UKA developed increasing anteromedial pain. The tibial components on the radiographs of the last follow-up were all found to be loose and subsidenced into valgus position. Based on our study, it can be assumed that the final valgus position of the tibial tray may have played an important role in initiating or further intensifying the postoperative pain.
Aseptic loosening of tibial component has been attributed to the abnormally low or high stress/strain of cancelous bone, both of which would induce the supporting bone resorption.[25,29] In this study, we mainly analyzed the cancelous bone strains at the bone-implant interface and had some findings. First, we found the tensile strains at ROI 2 were above the 2500 με threshold in all UKA models, which correlated well with previous studies.[20,26] Degenerative remodeling can be assumed to occur at the resection corner regardless of the inclination angles, which may be related to subsidence of the tibial component. Second, although the compressive strains at ROI 3 and ROI 4 changed with the varying inclinations, they were all higher than the low threshold of 100 με that would induce bone resorption, so we thought the valgus-varus mal-alignments of tibial tray would not obviously alter the risk of component loosening secondary to bone resorption. Besides, our finding was in good conformity with former studies,[30,31] which claimed that the peri-prosthetic bone density was preserved well for at least 2 years after Oxford UKA and suggested a much lower stress-shielding effect than TKA. Third, we found the peak compressive strains at ROI 5 were all above the 4000 με threshold when the tray was in >4° varus, which would result in great risk of fatigue failure of adjacent cancelous bone and migration of the tibial keel. Therefore, we did not recommend varus inclination that >4°.
What's more, our data indicated that the load percentage of medial compartment slightly increased in all UKA models. This may be explained by the notable difference in stiffness between the medial and lateral compartment of the knee after UKA, which made more load transfer through the medial compartment. Meanwhile, we found the cartilage contact pressures and load percentage in lateral compartment both slightly increased with the increasing varus inclinations of tibial tray, which may result in higher risk of cartilage degeneration and osteoarthritis progression in the lateral compartment. Besides, the femoral component contacted with the bearing at the edge in excessive valgus or varus inclinations, which induced the increased contact pressures of bearing and may contribute to increased wear.
In consideration of all of our results, valgus inclinations of the tibial component that >4° were not recommended for avoiding the extreme high stress/strain and pain occurred at proximal medial tibia. Although varus inclinations were not likely to induce residual pain, varus angles >4° should be avoided to reduce the risk of tibial keel migration and progressive gonarthrosis of lateral compartment. Thus, the range of coronal inclination from 4° valgus to 4° varus may seem most suitable in terms of bone stress/strain, contact pressures, and load distribution.
Some limitations to the predictive power of this study should be addressed. First, the structures of the FE models were constructed from the image data that were specific to the volunteer, which may affect the extrapolation of the results. Second, the material properties of the bone structures were assumed linear elastic and homogeneous for simplification. Third, the static loading condition in this study represented only normal gait in the stance phase near full extension. The dynamic simulation of the knee joint at varying knee flexion angles will be the subjects of further study.
In conclusion, our study suggested that a range of tibial component coronal inclination from 4° valgus to 4° varus can be recommended in mobile-bearing UKA for reducing the postoperative complications and enhancing long-term survivorship of implants as far as possible.
Financial support and sponsorship
This work was funded by a grant from National Natural Science Foundation of China (No. 81273972).
Conflicts of interest
There are no conflicts of interest.
Footnotes
Edited by: Yi Cui
REFERENCES
- 1.Mochizuki T, Sato T, Tanifuji O, Kobayashi K, Koga Y, Yamagiwa H, et al. In vivo pre- and postoperative three-dimensional knee kinematics in unicompartmental knee arthroplasty. J Orthop Sci. 2013;18:54–60. doi: 10.1007/s00776-012-0322-9. [DOI] [PubMed] [Google Scholar]
- 2.Pandit H, Jenkins C, Gill HS, Barker K, Dodd CA, Murray DW. Minimally invasive Oxford phase 3 unicompartmental knee replacement: Results of 1000 cases. J Bone Joint Surg Br. 2011;93:198–204. doi: 10.1302/0301-620X.93B2.25767. [DOI] [PubMed] [Google Scholar]
- 3.Furnes O, Espehaug B, Lie SA, Vollset SE, Engesaeter LB, Havelin LI. Failure mechanisms after unicompartmental and tricompartmental primary knee replacement with cement. J Bone Joint Surg Am. 2007;89:519–25. doi: 10.2106/JBJS.F.00210. [DOI] [PubMed] [Google Scholar]
- 4.Iesaka K, Tsumura H, Sonoda H, Sawatari T, Takasita M, Torisu T. The effects of tibial component inclination on bone stress after unicompartmental knee arthroplasty. J Biomech. 2002;35:969–74. doi: 10.1016/s0021-9290(01)00244-5. [DOI] [PubMed] [Google Scholar]
- 5.Sawatari T, Tsumura H, Iesaka K, Furushiro Y, Torisu T. Three-dimensional finite element analysis of unicompartmental knee arthroplasty – The influence of tibial component inclination. J Orthop Res. 2005;23:549–54. doi: 10.1016/j.orthres.2004.06.007. [DOI] [PubMed] [Google Scholar]
- 6.Simpson DJ, Price AJ, Gulati A, Murray DW, Gill HS. Elevated proximal tibial strains following unicompartmental knee replacement – A possible cause of pain. Med Eng Phys. 2009;31:752–7. doi: 10.1016/j.medengphy.2009.02.004. [DOI] [PubMed] [Google Scholar]
- 7.Shepherd DE, Seedhom BB. The ‘instantaneous’ compressive modulus of human articular cartilage in joints of the lower limb. Rheumatology (Oxford) 1999;38:124–32. doi: 10.1093/rheumatology/38.2.124. [DOI] [PubMed] [Google Scholar]
- 8.Yao J, Snibbe J, Maloney M, Lerner AL. Stresses and strains in the medial meniscus of an ACL deficient knee under anterior loading: A finite element analysis with image-based experimental validation. J Biomech Eng. 2006;128:135–41. doi: 10.1115/1.2132373. [DOI] [PubMed] [Google Scholar]
- 9.Ashman RB, Rho JY, Turner CH. Anatomical variation of orthotropic elastic moduli of the proximal human tibia. J Biomech. 1989;22:895–900. doi: 10.1016/0021-9290(89)90073-0. [DOI] [PubMed] [Google Scholar]
- 10.Peña E, Calvo B, Martínez MA, Palanca D, Doblaré M. Finite element analysis of the effect of meniscal tears and meniscectomies on human knee biomechanics. Clin Biomech (Bristol, Avon) 2005;20:498–507. doi: 10.1016/j.clinbiomech.2005.01.009. [DOI] [PubMed] [Google Scholar]
- 11.Mootanah R, Imhauser CW, Reisse F, Carpanen D, Walker RW, Koff MF, et al. Development and validation of a computational model of the knee joint for the evaluation of surgical treatments for osteoarthritis. Comput Methods Biomech Biomed Engin. 2014;17:1502–17. doi: 10.1080/10255842.2014.899588. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Moglo KE, Shirazi-Adl A. On the coupling between anterior and posterior cruciate ligaments, and knee joint response under anterior femoral drawer in flexion: A finite element study. Clin Biomech (Bristol, Avon) 2003;18:751–9. doi: 10.1016/s0268-0033(03)00140-2. [DOI] [PubMed] [Google Scholar]
- 13.Mesfar W, Shirazi-Adl A. Biomechanics of the knee joint in flexion under various quadriceps forces. Knee. 2005;12:424–34. doi: 10.1016/j.knee.2005.03.004. [DOI] [PubMed] [Google Scholar]
- 14.Pandit H, Jenkins C, Barker K, Dodd CA, Murray DW. The Oxford medial unicompartmental knee replacement using a minimally-invasive approach. J Bone Joint Surg Br. 2006;88:54–60. doi: 10.1302/0301-620X.88B1.17114. [DOI] [PubMed] [Google Scholar]
- 15.Hopkins AR, New AM, Rodriguez-y-Baena F, Taylor M. Finite element analysis of unicompartmental knee arthroplasty. Med Eng Phys. 2010;32:14–21. doi: 10.1016/j.medengphy.2009.10.002. [DOI] [PubMed] [Google Scholar]
- 16.Kwon OR, Kang KT, Son J, Kwon SK, Jo SB, Suh DS, et al. Biomechanical comparison of fixed- and mobile-bearing for unicomparmental knee arthroplasty using finite element analysis. J Orthop Res. 2014;32:338–45. doi: 10.1002/jor.22499. [DOI] [PubMed] [Google Scholar]
- 17.Crockett R, Roba M, Naka M, Gasser B, Delfosse D, Frauchiger V, et al. Friction, lubrication, and polymer transfer between UHMWPE and CoCrMo hip-implant materials: A fluorescence microscopy study. J Biomed Mater Res A. 2009;89:1011–8. doi: 10.1002/jbm.a.32036. [DOI] [PubMed] [Google Scholar]
- 18.Bao HR, Zhu D, Gong H, Gu GS. The effect of complete radial lateral meniscus posterior root tear on the knee contact mechanics: A finite element analysis. J Orthop Sci. 2013;18:256–63. doi: 10.1007/s00776-012-0334-5. [DOI] [PubMed] [Google Scholar]
- 19.Peña E, Calvo B, Martínez MA, Doblaré M. A three-dimensional finite element analysis of the combined behavior of ligaments and menisci in the healthy human knee joint. J Biomech. 2006;39:1686–701. doi: 10.1016/j.jbiomech.2005.04.030. [DOI] [PubMed] [Google Scholar]
- 20.Chang TW, Yang CT, Liu YL, Chen WC, Lin KJ, Lai YS, et al. Biomechanical evaluation of proximal tibial behavior following unicondylar knee arthroplasty: Modified resected surface with corresponding surgical technique. Med Eng Phys. 2011;33:1175–82. doi: 10.1016/j.medengphy.2011.05.007. [DOI] [PubMed] [Google Scholar]
- 21.Kurosawa H, Fukubayashi T, Nakajima H. Load-bearing mode of the knee joint: Physical behavior of the knee joint with or without menisci. Clin Orthop Relat Res. 1980;149:283–90. [PubMed] [Google Scholar]
- 22.Fukubayashi T, Kurosawa H. The contact area and pressure distribution pattern of the knee. A study of normal and osteoarthrotic knee joints. Acta Orthop Scand. 1980;51:871–9. doi: 10.3109/17453678008990887. [DOI] [PubMed] [Google Scholar]
- 23.Keene G, Simpson D, Kalairajah Y. Limb alignment in computer-assisted minimally-invasive unicompartmental knee replacement. J Bone Joint Surg Br. 2006;88:44–8. doi: 10.1302/0301-620X.88B1.16266. [DOI] [PubMed] [Google Scholar]
- 24.Pattin CA, Caler WE, Carter DR. Cyclic mechanical property degradation during fatigue loading of cortical bone. J Biomech. 1996;29:69–79. doi: 10.1016/0021-9290(94)00156-1. [DOI] [PubMed] [Google Scholar]
- 25.Frost HM. A 2003 update of bone physiology and Wolff's Law for clinicians. Angle Orthod. 2004;74:3–15. doi: 10.1043/0003-3219(2004)074<0003:AUOBPA>2.0.CO;2. [DOI] [PubMed] [Google Scholar]
- 26.Pegg EC, Walter J, Mellon SJ, Pandit HG, Murray DW, D’Lima DD, et al. Evaluation of factors affecting tibial bone strain after unicompartmental knee replacement. J Orthop Res. 2013;31:821–8. doi: 10.1002/jor.22283. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 27.Small SR, Berend ME, Ritter MA, Buckley CA. Bearing mobility affects tibial strain in mobile-bearing unicompartmental knee arthroplasty. Surg Technol Int. 2010;19:185–90. [PubMed] [Google Scholar]
- 28.Liddle AD, Pandit HG, Jenkins C, Lobenhoffer P, Jackson WF, Dodd CA, et al. Valgus subsidence of the tibial component in cementless Oxford unicompartmental knee replacement. Bone Joint J. 2014;96-B:345–9. doi: 10.1302/0301-620X.96B3.33182. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 29.Taylor M, Tanner KE. Fatigue failure of cancellous bone: A possible cause of implant migration and loosening. J Bone Joint Surg Br. 1997;79:181–2. doi: 10.1302/0301-620x.79b2.7461. [DOI] [PubMed] [Google Scholar]
- 30.Hooper GJ, Gilchrist N, Maxwell R, March R, Heard A, Frampton C. The effect of the Oxford uncemented medial compartment arthroplasty on the bone mineral density and content of the proximal tibia. Bone Joint J. 2013;95-B:1480–3. doi: 10.1302/0301-620X.95B11.31509. [DOI] [PubMed] [Google Scholar]
- 31.Richmond BI, Hadlow SV, Lynskey TG, Walker CG, Munro JT. Proximal tibial bone density is preserved after unicompartmental knee arthroplasty. Clin Orthop Relat Res. 2013;471:1661–9. doi: 10.1007/s11999-013-2784-2. [DOI] [PMC free article] [PubMed] [Google Scholar]


