Sufficient oxygen for animal respiration 1,400 million years ago

Significance

How have environmental constraints influenced the timing of animal evolution? It is often argued that oxygen first increased to sufficient levels for animal respiration during the Neoproterozoic Eon, 1,000 million to 542 million years ago, thus explaining the timing of animal evolution. We report geochemical evidence for deep-water oxygenation below an ancient oxygen minimum zone 1,400 million years ago. Oceanographic modeling constrains atmospheric oxygen to a minimum of ∼4% of today’s values, sufficient oxygen to have fueled early-evolved animal clades. Therefore, we suggest that there was sufficient atmospheric oxygen for animals long before the evolution of animals themselves, and that rising levels of Neoproterozoic oxygen did not contribute to the relatively late appearance of animal life on Earth.

Abstract

The Mesoproterozoic Eon [1,600–1,000 million years ago (Ma)] is emerging as a key interval in Earth history, with a unique geochemical history that might have influenced the course of biological evolution on Earth. Indeed, although this time interval is rather poorly understood, recent chromium isotope results suggest that atmospheric oxygen levels were <0.1% of present levels, sufficiently low to have inhibited the evolution of animal life. In contrast, using a different approach, we explore the distribution and enrichments of redox-sensitive trace metals in the 1,400 Ma sediments of Unit 3 of the Xiamaling Formation, North China Block. Patterns of trace metal enrichments reveal oxygenated bottom waters during deposition of the sediments, and biomarker results demonstrate the presence of green sulfur bacteria in the water column. Thus, we document an ancient oxygen minimum zone. We develop a simple, yet comprehensive, model of marine carbon−oxygen cycle dynamics to show that our geochemical results are consistent with atmospheric oxygen levels >4% of present-day levels. Therefore, in contrast to previous suggestions, we show that there was sufficient oxygen to fuel animal respiration long before the evolution of animals themselves.

Some aspects of the history of atmospheric oxygen on Earth are well understood. For example, before about 2,300 million years ago (Ma), atmospheric oxygen was likely less than 0.001% of present atmospheric levels (PAL) (1, 2), whereas, after about 550 Ma, levels have been greater than about 20% PAL (3–5), sufficient to sustain large-animal respiration. The intervening history, however, has been both poorly studied and poorly constrained. This history is of critical importance as it allows one to establish possible links between changing oxygen levels and animal evolution, where molecular clock estimates showing an evolution of crown-group metazoans (including the ancestors of modern Porifera and Placozoa) sometime during the Cryogenian Period (720–635 Ma) (6, 7). Indeed, there is a long-standing suggestion that rising atmospheric oxygen concentrations in the late Neoproterozoic Eon (1,000–542 Ma) (8–11) enabled animal respiration, thus explaining the timing of animal evolution.

The oxygen levels required for early animal respiration were lower than those needed to sustain large motile animals and were probably ≤1% PAL (10, 12, 13). Recent chromium isotope results suggest oxygen levels of <0.1% PAL through the Mesoproterozoic Eon (1,600–1,000 Ma) and until about 700 Ma, when rising levels then spurred animal evolution (11). In contrast, we present evidence that oxygen was ≥3.8% PAL at 1,390 Ma, sufficient to fuel early animal respiration.

Study Site and Sample Collection

The Xiamaling Formation (see Fig. S1) was deposited below storm wave base in a tropical setting between 10°N and 30°N (14, 15). Pre-Xiamaling sediments were deposited on a passive margin, but occasional ash layers in the Xiamaling Formation have led to suggestions of a back-arc setting (15). Sediments of the Xiamaling Formation, however, are highly laminated with no evidence for mass flows or turbidites, and volcanoclastics are rare. Therefore, deposition in a tectonically quiet environment is indicated, consistent with continued deposition on a passive margin. There is also no evidence for storm wave interaction with the sediment, so a water depth of >100 m is likely. The sediment package was never heated to above 90 °C, thus preserving organic biomarkers (16). High-precision zircon data yield an age of 1,384.4 ± 1.4 Ma for a tuff layer 210 m below the top of the formation and 1,392.2 ± 1.0 Ma for a bentonite layer 52 m below the tuff layer (16).

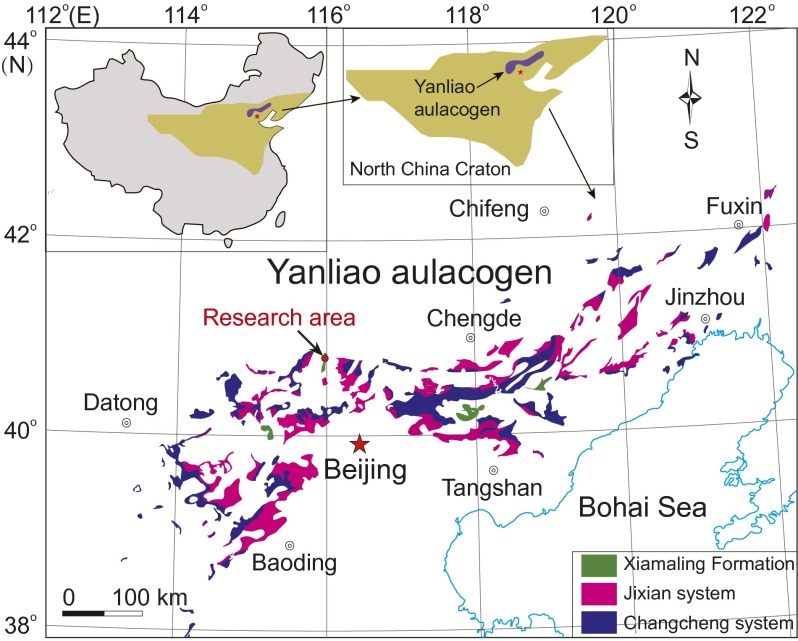
Fig. S1.

The distribution of the Xiamaling Formation on the North China Platform, including the location of our research area (15).

As one of our objectives was to study biomarker distributions within the Xiamaling sediments, most of our data come from cores obtained with fresh water as drilling fluid. In some cases, we compared our inorganic geochemical results to results obtained from outcrop samples, where the outer weathered layer was first removed. Details of our sediment sampling procedures and analytical methods are described in Supporting Information.

Evidence for Bottom Water Oxygenation

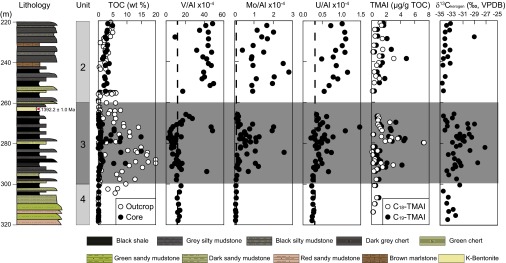
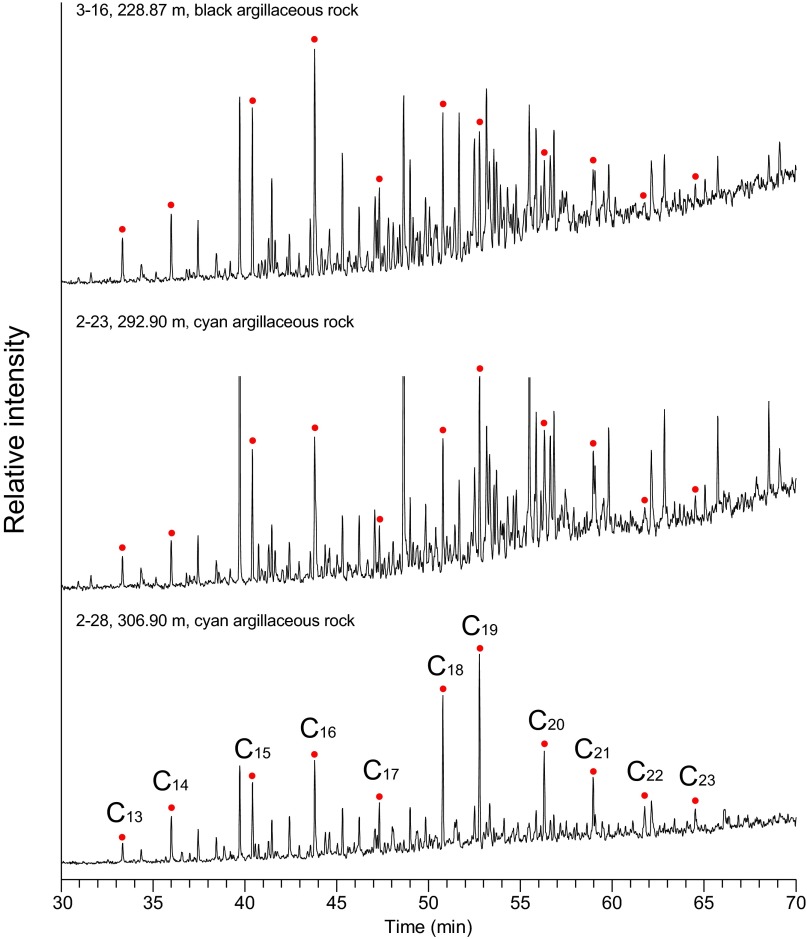
In a zone from 260 to 300 m depth, shales with high total organic carbon (TOC) alternate with low-TOC cherts (Fig. 1 and Fig. S2). These sediments are distinct from the low-TOC sediments deposited below this interval and the intermediate-TOC sediments deposited above. The high-TOC zone is best expressed in outcrop samples, due to the low sample recovery of shales during coring (Fig. 1). The alteration between the high-TOC shales and low-TOC cherts likely represents orbitally forced changes in trade wind intensity, as this influenced the strength of coastal upwelling (16).

Fig. 1.

Geochemistry, biomarker, and δ¹³C of kerogen for the Xiamaling Formation from the top of unit 4 to the bottom of unit 2. TOC, from both the core and outcrop samples are presented. Data include both black shales and other sediment types like cherts. Vertical dotted lines represent concentration ratios relative to crustal average (see Supporting Information and Tables S1 and S2).

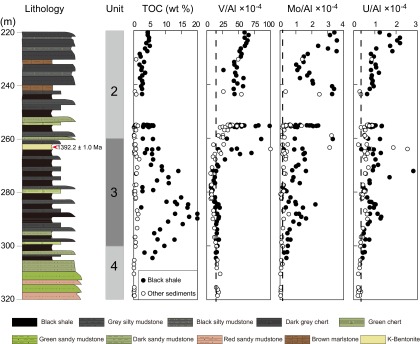
Fig. S2.

Geochemical results for outcrop samples. Vertical dashed lines represent metal/Al ratios of average upper crust (61).

The high-TOC shales within the high-TOC zone, and particularly in the depth range of 270–295 m, are enriched in the redox-sensitive elements molybdenum (Mo) and uranium (U) (Fig. 1 and Fig. S2) but are either depleted or unenriched in vanadium (V). The gray shales in this interval show small to negligible enrichments in Mo and U, but, like the black shales, many also show depletion in V. Our focus, however, will be on the geochemical environment surrounding black shale deposition. In modern environments, V is commonly released from sediments depositing under low-oxygen (but still oxygenated) conditions (17, 18) and under normal bottom water oxygen levels where oxygen only penetrates a few millimeters into the sediment. Vanadate is transported to sediments as the vanadate ion [H₂V(VI)O₄⁻] adsorbed onto Mn oxides. The vanadate ion is released as the Mn oxides are reduced to Mn²⁺ (18), and, where oxygen is limiting, Mn oxides do not readily reform at the sediment surface (18–20), allowing vanadate to escape to the overlying water.

Vanadate is also released into anoxic waters following Mn oxide reduction, but in the absence of oxygen, the vanadate is reduced to the vanadyl form [V(IV)O²⁺] (18). This form is highly surface-reactive and removed to the sediment together with vanadyl ions formed from vanadate ions transported across the chemocline into the anoxic waters. Thus, in oxygen minimum zones (OMZs) where anoxic waters overlay sediments, and in euxinic basins, V is enriched together with Mo and U (17, 21–23). In summary, coenrichments of V, Mo, and U occur under anoxic water column conditions, whereas V release from sediments only occurs under bottom water oxygenation (see Supporting Information for further discussion).

Thus, trace metal patterns demonstrate bottom water oxygenation during deposition of unit 3 of the Xiamaling Formation. Our results complement a previous study from the 1,410 Ma Kaltasy Formation, Volgo-Ural region, Russia, where Fe speciation and trace metal abundances (these sediments also indicate V release relative to crustal average values) indicate bottom water oxygenation in sediments deposited deeper than storm wave base (likely >150 m depth) (24).

Evidence for an Oxygen Minimum Zone Setting

The chemical environment of the Xiamaling Formation is further constrained by exploring the abundance of 2,3,6-trimethyl aryl isoprenoids (2,3,6-TMAI). These biomarkers are breakdown products of isorenieratane, whose precursors are isorenieratene and β-isorenieratene, which are themselves carotenoid pigments associated with “brown” strains of green sulfur bacteria (GSB) (Chlorobiaceae) (25). These organisms are obligate anaerobic phototrophs that frequent modern and ancient sulfidic (and likely also ferruginous) water columns, using the oxidation of sulfide and ferrous iron to gain energy for building cell biomass (26–30). Elevated abundances of 2,3,6-TMAI’s (Fig. 1 and Figs. S3 and S4) suggest that phototrophic low-light-adapted GSB populations occupied the Xiamaling water column during much of unit 3 deposition. Thus, biomarker evidence, combined with trace metal dynamics, suggest that unit 3 of the Xiamaling Formation deposited in a true OMZ setting with deep oxygenated water overlain by anoxic water with either H₂S or Fe²⁺ in the photic zone.

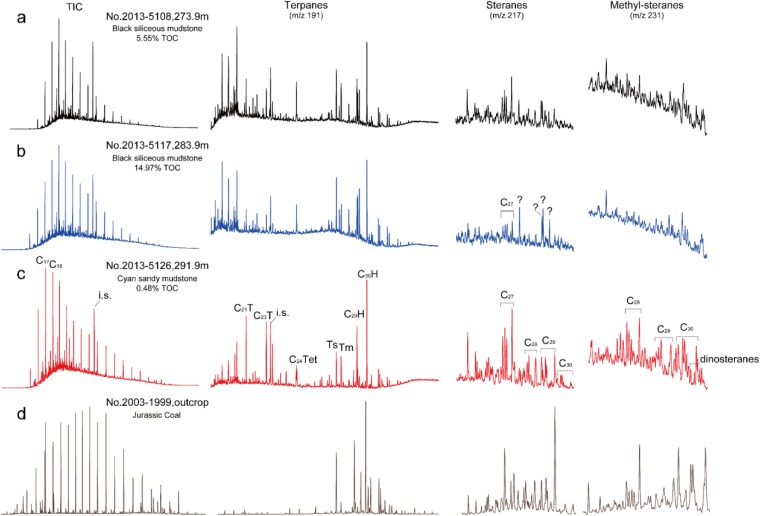
Fig. S3.

Total ion chromatograms (TIC; from GC-MS) and biomarker distributions in the saturated fractions from selected depths in the Xiamaling Formation: (A) 273.9 m (upper layer of the OMZ), (B) 283.9 m (within the OMZ), and (C) 291.9 m (underlying the OMZ). These chromatograms are compared with overlying coals from the Jurassic Xiahuayuan Formation (D). Steranes and methyl steranes were either not detected or were in very low abundance in the sediments from OMZ, and steranes and methyl steranes were both found in the underlying and upper layers of OMZ. The peaks with question marks are interference peaks.

Fig. S4.

GC-MS chromatograms (m/z 134) reveals the distributions of 2,3,6-TMAI in the aromatic fractions from different depths in the Xiamaling Formation.

There is some evidence, however, that β-isorenieratane can form from the late diagenetic aromatization of partially hydrogenated β-carotene (31), with the β-carotene sourced from algae or cyanobacteria. The source of β-isorenieratane, and ultimately the 2,3,6-TMAIs, can, in principle, be tested through the δ¹³C of the 2,3,6-TMAI compounds as GSB produce relatively ¹³C-enriched biomass through the reductive citric acid cycle in carbon fixation (see review in ref. 32). Unfortunately, we were unable to measure the isotopic compositions of the 2,3,6-TMAIs, but the δ¹³C of insoluble organic matter (kerogen) tends to heavier values in sync with peak abundances of C₁₈-TMAI or C₁₉-TMAI (Fig. 1). This observation is consistent with the addition of GSB biomass to the carbon pool. Therefore, we are confident that our C₁₈-TMAI and C₁₉-TMAI biomarkers represent the presence of GSB in the ancient Xiamaling Formation water column. We note, however, that although illuminating the geochemical environment, the recognition of an OMZ setting is not critical to constraining atmospheric oxygen concentrations as described immediately below. The most critical point is the recognition that Xiamaling Formation sediments deposited in oxygenated deep waters as constrained from trace metal distributions as described above.

Constraining Atmospheric Oxygen Levels

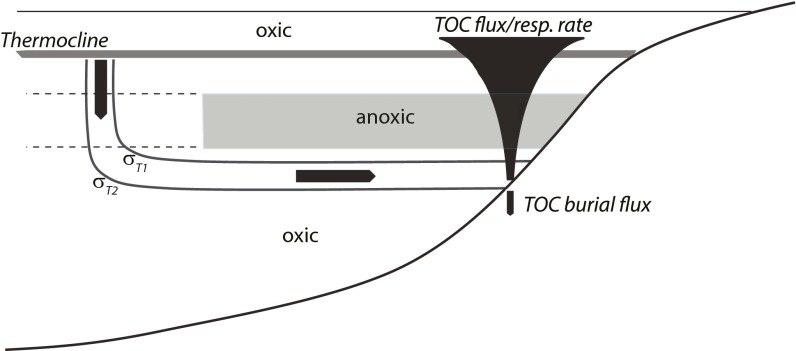
The presence of oxygenated bottom waters during Xiamaling Formation deposition allows constraints on levels of atmospheric oxygen. The water supplied to OMZs originates as oxygen-saturated surface waters that are mixed during winter months into the thermocline in extratropical latitudes (33). This water loses oxygen to respiration as it flows along isopycnal surfaces to the OMZ (Fig. 2). There is sufficient respiration to consume all of the oxygen flowing to the anoxic portion of an OMZ, but insufficient respiration to consume the oxygen from water flowing to the deeper depths. Our goal is to determine the minimum amounts of atmospheric oxygen required to allow oxygenated waters to persist in these deeper waters. Knowing this, we derive a lower limit for atmospheric oxygen levels, assuming that the upper mixed layer of the ocean was in oxygen equilibrium with the atmosphere.

Fig. 2.

Cartoon representation of our oxygen respiration model. The cartoon shows the origin of OMZ water at the thermocline, and its transit to the OMZ along layers of constant density σ_t. The cartoon also shows how both the flux of organic carbon and its respiration rate attenuate with depth in the water column. Finally, the cartoon shows our convergence criteria where depth is determined by matching the TOC flux through the water column with the TOC burial flux in Xiamaling Formation sediments.

The oxygen loss to respiration is obtained by combining the transit time of water from its place of ventilation and the rate of oxygen respiration in the water. The transit time of water to the OMZ is approximated by calculating the so-called “water age,” which is assessed as the volume of water confined within adjacent layers of constant density (isopycnal surfaces) ratioed by the flux of water into this volume (34). The shortest water ages give the lowest estimates for atmospheric oxygen (see below), and these are found in the South Atlantic, with ages of 5–6 y in the upper ∼100 m of the water column, increasing to about 25 y by 400 m water depth (34). In contrast, water ages in the North Pacific range from 7−20 y in the upper 100 m to well over 100 y by 400 m depth (34). Water ages for restricted and semirestricted marine water bodies generally fall within these ranges (see Supporting Information for summary of water ages).

The rate of respiration of marine organic matter decreases as a function of water depth (34), and the rate at a given depth depends on the export flux of organic matter from the upper water column (also called new production), the settling rate of the organic matter, and its degradability. In our modeling, we explored export production values ranging from 10% to 200% of present-day Equatorial Atlantic average values (Supporting Information). Of this range, we view 20–150% as a good estimate for the Xiamaling Formation, recognizing that during the Mesoproterozoic Eon, marine carbon isotope values suggest a carbon cycle operating at rates broadly similar to today (35).

We also explored in our modeling a wide range of particle settling rates. We highlight that the settling rates of small, predominantly prokaryote-derived, organic particles 1.39 Ga were likely much lower than today (36) where dense, eukaryote-derived, algal tests and animal-derived fecal material dominate the settling flux (37). Settling velocities of between 1 m⋅d⁻¹ and 6 m⋅d⁻¹ encompass the range predicted for micrometer-sized planktonic cells, and their aggregates, through a planktonic bloom (38). We focus on this range of settling velocities in interpreting our results.

Organic matter degradability in the modern ocean is assessed from the attenuation of particle-settling fluxes with water depth as revealed through sediment trap experiments. Recent studies, accounting for particle capture efficiency, reveal that upper water column particles degrade more rapidly in low latitudes with higher temperatures than in high latitudes with lower temperatures (39). We least-squares fit the low latitude, the high latitude, and the combined trap data with a continuous reaction model, generating parameters describing the aging of particles as they settle. From these parameters, we calculate the decomposition rate of settling organics with depth as a function of new production and particle settling rate (see Supporting Information for details).

In our calculations, new production is equal to the export flux of organic matter at the base of a nominal mixed-layer depth of 50 m, and organic matter is consumed as the particles sink. At a unique water depth for each calculation, the carbon-settling flux matches the burial flux of organic matter into Xiamaling Formation sediments as constrained from precise sediment chronology and sediment TOC content, which varies from 10 wt% to 20 wt% in the black shales (Fig. 1). The rate of oxygen respiration at this depth is then combined with the appropriate water age for the depth to give the total amount of oxygen respired.

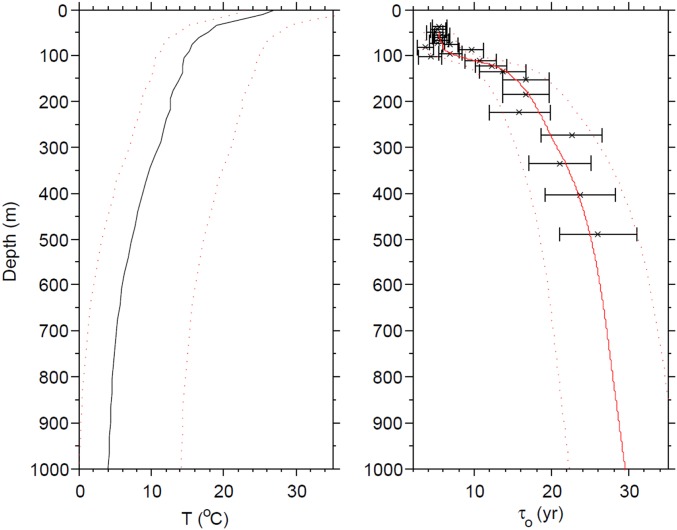
Atmospheric oxygen values are calculated from this amount of respired oxygen assuming water saturation with the atmosphere at the temperature corresponding to the diagnosed water depth, where we use the average temperature−depth distribution as found in the tropical to subtropical South Atlantic (see Supporting Information). The Xiamaling Formation formed during a prolonged ice-free period of Earth history, and ocean temperatures during this time may have been higher than those in the modern ocean. Higher temperatures ultimately yield higher estimates of atmospheric O₂, as explored below, so focusing on present-day temperatures provides a conservative minimum estimate of atmospheric O₂ concentration. Our estimates of atmospheric O₂ are also minimum values, as our calculation of oxygen levels does not account for excess oxygen in the water as required by the geochemical data.

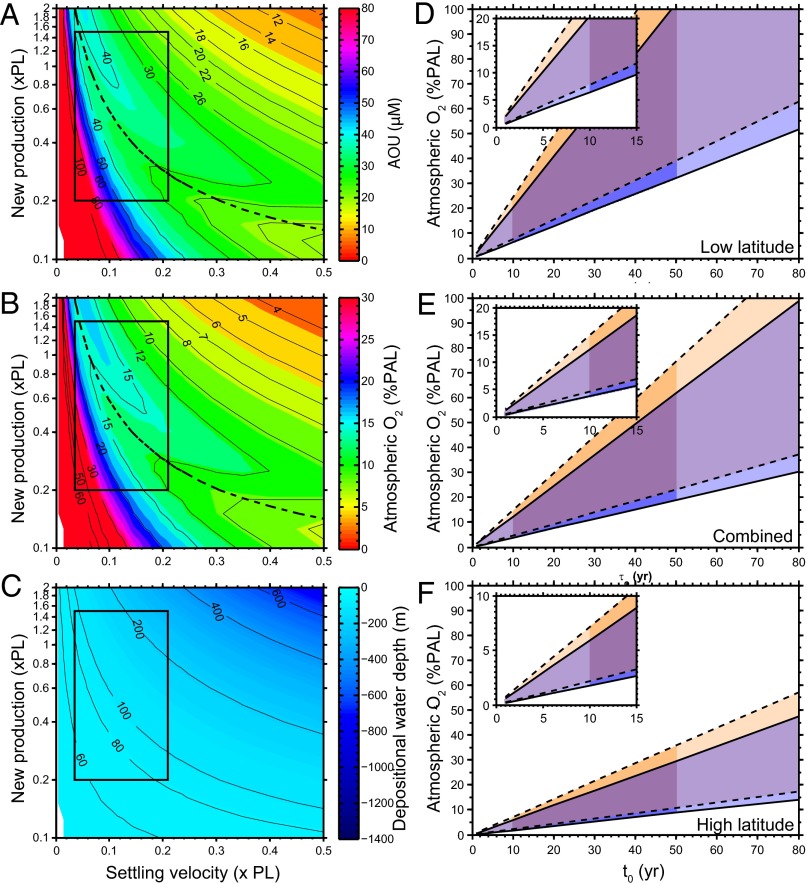
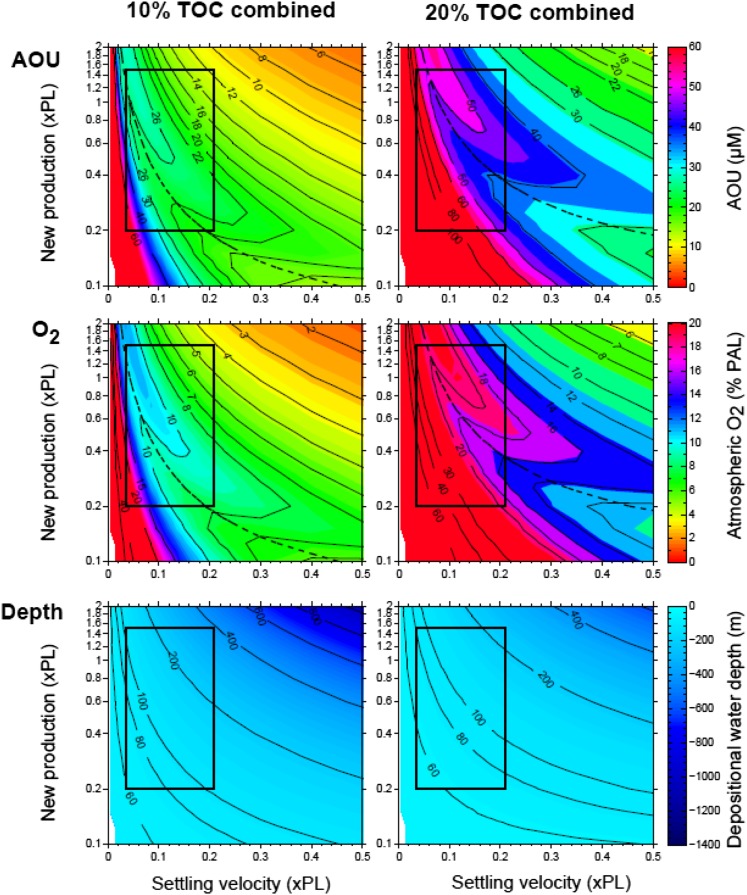
An example of model results is shown in Fig. 3 A−C. These results were generated with a Xiamaling Formation TOC content of 15 wt%, organic reactivity from the combined sediment trap data, and water age and temperature depth distributions for the South Atlantic (Supporting Information). Within the most likely Mesoproterozoic ranges of new production and particle settling rates (as shown in the outlined box in Fig. 3 A−C), and for water depths of >100 m (dotted lines in Fig. 3 A and B), as is likely for the Xiamaling Formation depositional environment, our calculations show oxygen consumption of between about 21 μM and 40 μM, translating into minimum atmospheric oxygen levels of 8–15% PAL.

Fig. 3.

(A) Calculated minimum oxygen utilization (AOU) for water masses circulating to oxygenated waters beneath the anoxic portion of an OMZ. This calculation is calibrated to a Xiamaling Formation organic carbon burial flux calculated from 15 wt% TOC, with organic matter reactivity as calculated from the mean of all sediment trap data, and a temperature−depth distribution as for the present tropical Atlantic Ocean. The black box outlines new production rates ranging from 0.2 to 1.5 times present values (xPL), with the present value as observed in the equatorial Atlantic (Supporting Information), and particle settling rates between 1 m⋅d⁻¹ and 6 m⋅d⁻¹ (they are presented as a fraction, xPL-present level, of the diagnosed particle settling rates from the sediment trap data as described in Supporting Information). The dotted line indicates the solutions for the 100 m depth contour. (B) Values of AOU converted to percent present atmospheric oxygen levels (%PAL) assuming water saturation with air at the temperature for the water depth diagnosed in the calculation. (C) Water depths diagnosed by matching the particle settling flux with the burial flux of organic matter into Xiamaling Formation sediments. The stippled line represents water depths of less than 100 m, which we consider unlikely given the deep-water depositional setting of the Xiamaling Formation. (D) Sensitivity analyses depicting the high and low oxygen estimates for a series of calculations with variable water residence times (τ_o) assuming low organic matter reactivity as found in modern low latitudes. Calculations with the present-day temperature−depth distributions are shown in blue shades, and calculations with this temperature profile +10 °C are shown in brown. A large overlap is observed. The darkened area outlines the most likely range of water ages. (E) As in D, but assuming organic matter reactivity from the combined sediment trap data. (F) As in D, but assuming high organic matter reactivity as found in modern high latitudes. See Constraining Atmospheric Oxygen Levels and Figs. S5–S8 and Tables S3–S7 for details.

Variations in water age, organic matter reactivity, and temperature will change these results, as shown in a series of sensitivity analyses (Fig. 3 D−F). Here we document high and low estimates of atmospheric oxygen with variations in water age−depth distributions (Supporting Information), temperature (present day and +10 °C), and organic matter reactivity as revealed in the high, low, and combined sediment trap data. Higher water temperatures, as might be expected during this apparently ice-free time in Earth history, yield higher estimates for minimum atmospheric oxygen levels, whereas lower water ages yield lower estimates. We view 10 y as a conservative minimum water age; this is less than or equal to the water age found in the modern open ocean at water depths of >100 m, and similar to or less than those found in modern semienclosed basins (see Supporting Information). Higher organic matter reactivity (Fig. 3F) produces higher oxygen estimates, whereas lower reactivity (Fig. 3D) produces lower estimates.

With a water age of 10 y, minimum oxygen estimates range from 1.3% PAL (low reactivity, high latitude, Fig. 3D) to 6.2% PAL (high reactivity, low latitude, Fig. 3F) with 3.8% PAL for the combined data (Fig. 3E). As temperature is considered the prime variable controlling the latitudinal distribution of organic matter reactivities, and because the Xiamaling Formation deposited in a low-latitude setting, we favor the model results from the combined or low-latitude reactivities. Therefore, our sensitivity analysis reveals 3.8–6.2% PAL as a likely minimum atmospheric oxygen level, whereas 1.3% PAL is an unlikely but possible minimum estimate.

Our most likely minimum estimate of 3.8–6.2% PAL is very different from the <0.1% PAL oxygen suggested for this time from Cr isotope results (11). This result arises, ultimately, from a general lack of fractionated Cr (with δ⁵³Cr values in the range of −0.25‰ to 0‰, compared with crustal values estimated at −0.1‰ to −0.2‰) in pre-Neoproterozoic iron-enriched marine sediments. Fractionations are imparted during the oxidative weathering of Cr(III) minerals on land, and fractionated Cr is transported to the sea and is believed to be captured in the iron-enriched sediments (40). Therefore, no fractionation would indicate no oxidative weathering on land and low levels of atmospheric oxygen. We note, however, that other data sets report evidence for fractionated Cr in pre-Neoproterozoic sediments, and, in particular, many banded iron formations in the time window from 1,800 Ma to 3,000 Ma have δ⁵³Cr values ranging up to 0.2–0.3‰ (40, 41). The discrepancy between these data sets has yet to be evaluated, but we note that no standard procedures are used to correct for a detrital Cr component. Therefore, we believe that the terrestrial and marine geochemical behavior of Cr is still poorly understood, and, in particular, it is unclear how chromium isotope signals are transferred from the land to marine sediments and which sediments best preserve such isotope signals.

Consequences of Elevated Mesoproterozoic Oxygen Levels

Atmospheric oxygen levels of 3.8–6.2% PAL (or even 1.3% PAL) are sufficient to support the respiration of sponges, considered good candidates for early evolved animals, whose oxygen requirements are in the range of ≤1–4% PAL (12). Such levels are also sufficient to support small motile animals such as annelid worms, which may require even less oxygen (13). Thus, it appears that sufficient oxygen existed to support animal metabolism long before the evolution of stem-group animals themselves, which, as noted above, from molecular phylogenetic evidence, occurred around 780 Ma (13). Thus, our results support the idea that oxygen itself did not limit the late emergence of animal life (1, 42).

Further Geological Background

The Xiamaling Formation is part of a Paleoproterozoic to Neoproterozoic sediment package deposited on what is now the North China Platform (Fig. S1) (15). The Xiamaling Formation began its deposition about 1,400 Ma onto the stromatolite-rich limestone of the Tieling Formation (15, 16, 43). The Xiamaling Formation forms a transgressive sequence and was deposited in deeper waters below storm wave base through the whole sequence analyzed here (15). In the bottom unit (unit 4) of the Xiamaling Formation, green and red sandy mudstones alternate in repeating bundles, with a low organic carbon abundance of less than 1% (16). These sediments grade upward into alternating layers of black shale and interlayered chert, with high TOC values of over 10 wt% and reaching up to 20 wt% in the black shales (unit 3). The Xiamaling Formation grades further upward into predominantly black shale alternating with gray and black silty mudstones (unit 2) with TOC values of 2–5 wt% (16). Ash layers occur in two horizons in the Xiamaling Formation. Near the middle of unit 2, there are a series of four closely spaced ash layers, and two prominent bentonite layers occur near the top of unit 3. Other than these, there is no evidence for volcanoclastic sediments in the Xiamaling Formation. The sediments from the present study include the upper part of unit 4, all of unit 3, and the lower part of unit 2.

Sample Collection

Samples were collected using a diamond drill coring tube lubricated with fresh water to minimize biomarker contamination from drill fluids. Altogether, three cores were collected within 1 km of each other to provide as complete coverage as possible of the Xiamaling Formation. Stratigraphic depths were calibrated to adjacent outcrops, with calibrations based on both drill angle and core depth as well as geochemical comparisons.

Geochemical Methods

Bulk fresh core samples were washed with purified water, dried, and then crushed to a fine powder (less than 200 mesh) using a stainless steel puck mill, which was cleaned between samples by grinding baked quartz sand multiple times. All of the geochemical data were analyzed from the homogeneous powders.

TOC.

All of the TOC analyses were performed at the Nordic Center for Earth Evolution (NordCEE), University of Southern Denmark. Samples of powdered rocks were acid-treated to remove carbonates (1M HCl for 2 h) and then dried and analyzed for TOC using a Thermo Analytical elemental analyzer, Flash EA 2000, with an analytical uncertainty of less than 5%.

Trace Metals.

X-ray fluorescent and inductively coupled plasma mass spectrometry analysis at the Key Laboratory of Petroleum Geochemistry in China.

Samples with prefixes 1-, 2-, or 3- were analyzed for trace metal elements with high-resolution inductively coupled plasma mass spectrometry (ICP-MS) (Finnigan MAT, Element I) or major elements with X-ray fluorescence (PW2404; Philips Electronics) at the Key Laboratory of Petroleum Geochemistry (KLPG) at the China National Petroleum Corporation, Beijing, China.

For major elements analysis (Al is reported here), rock powders were oven-dried overnight at 105 °C. Then, 2.0 g of each sample was precisely weighed and then ignited for 20 min at 1,100 °C in a Pt(5%)Au crucible and then reweighed to determine the loss on ignition (LOI). Each powdered sample was then prepared following the steps outlined below and analyzed by X-ray fluorescence (PW2404; Philips Electronics). (i) First, 0.5 g of each ignited sample was precisely weighed and carefully mixed with Li₂B₄O₇-LiBO₂-LiF (4.5:1:0.4, wt %) in an unignited sample-to-flux ratio of 1:9. (ii) The ignited powder and the flux were fused in a porcelain crucible for 20 min at 1,100 °C in a muffle furnace, where the molten mixture was constantly swirled to completely disperse the flux. (iii) Cooled samples were reweighed, and any weight lost was made up by adding extra flux. (iv) Samples were fused for a second time over a Meker burner, swirling the molten mixture during heating to ensure homogenization, and samples were then cast on a graphite mold on a 250 °C hotplate. (v) The sample was then pressed with an aluminum plunger to create a flattened disk. All of the pressed powder pellets were analyzed by X-ray fluorescent (XRF) and the accuracies were tested with shale standard material (GBW 03014). The relative standard deviation (SD) was less than 1.0%.

For the determination of trace metals (reported here: U, Mo, and V), dried powders of whole-rock samples were dissolved using a triacid digestion involving HNO₃, HClO₄, and HF following the steps outlined below and then analyzed with a high-resolution ICP-MS (Finnigan MAT, Element I). The preparation steps involved the following: (i) First, 0.5 g of each ignited powder was precisely weighed and transferred into Teflon crucibles. (ii) Then, 7–8 mL of concentrated HF and 5 mL of 50% HNO₃ were added to the sample and left on a hotplate to dissolve overnight. (iii) Samples were then boiled to near dryness on a 250 °C hotplate. (iv) After a few minutes of cooling, 7–8 mL of concentrated HF were again added, and the sample was boiled to near dryness. (v) After this, 5 mL of 50% HNO₃ was added to each dry sample and left on the hotplate to dissolve overnight. (vi) The cooled samples were reboiled the next day with 1 mL of HClO₄ until the white smoke completely disappeared. (vii) These samples were cooled and then heated with 5 mL of 50% HNO₃ until the solution became transparent. (viii) Each sample was then diluted to 50 mL with 5% HNO₃.

Trace metal concentrations were quantified by using calibration solutions containing the elements of interest (Mo, U, and V), and the relative SD of each of these trace metals (precision) was less than 1.5%. Accuracies were tested with the shale standard (GBW 03014) that was measured along with the samples. The accuracy (percent) of the elements of interest (Mo, U, and V) compared with known values for the shale standard (GBW 03014) was 3% (V), 11% (Mo), and 17% (U).

ICP-MS analysis at the Geological Survey of Denmark and Greenland.

Individual samples 2-6, 2-7, S-18, 2#-3, 2#-4, 2-9, 2-10, 2#-5, 2#-6, 2#7, S-19, 2-11, 2#-8, 2-12, S-20, 2#-9, 2-13, 2#-10, 2–14, 2#-11, 2-15, S-21, 2#-12, 2-16, 2-17, 2-18, S-22, 2-20, and 2#-13 (Table S1) were analyzed for elemental composition using ICP-MS (PerkinElmer Elan 6100DRC) at the Geological Survey of Denmark and Greenland (GEUS) in Copenhagen, Denmark.

Table S1.

Lithology and geochemical data of Xiamaling Formation

Sample	Lithology	Depth, m	TOC, wt%	Al2O3, %	V, μg/g	V EF	V/Al, ×10−4	Mo, μg/g	Mo EF	Mo/Al, ×10−4	U, μg/g	U/Al, ×10−4	U EF	C18-TMAI, μg/g TOC	C19-TMAI, μg/g TOC	δ13C, ‰
S-10	black argillaceous rock	221.42	3.34	10.21	261.0	3.28	48.30	21.24	29.12	3.93	5.50	1.02	3.07	1.19	1.59	−33.87
3-14	black argillaceous rock	222.35	5.00	11.68	255.0	2.80	41.26	10.30	12.35	1.67	4.46	0.72	2.18	1.37	1.68
S-11	black argillaceous rock	224.66	3.16	11.06	203.8	2.36	34.82	6.83	8.64	1.17	<LOD			0.44	0.80	−34.11
3-15	black argillaceous rock	225.61	3.01	9.35	217.0	2.98	43.86	9.97	14.93	2.01	5.37	1.09	3.28
S-12	black argillaceous rock	227.94	2.43	10.21	50.4	0.63	9.33	7.58	10.40	1.40	<LOD			0.48	0.83	−34.18
3-16	black argillaceous rock	228.87	2.90	12.81	299.0	3.00	44.11	11.50	12.57	1.70	7.52	1.11	3.35	1.49	2.14	−34.11
3-17	black argillaceous rock	232.13	3.17	10.57	244.0	2.96	43.63	5.35	7.09	0.96	5.70	1.02	3.08	0.30	0.64
S-13	black argillaceous rock	234.46	3.07	11.99	201.8	2.16	31.82	2.53	2.95	0.40				1.55	2.74	−34.35
3-18	black argillaceous rock	235.39	2.51	10.99	275.0	3.21	47.29	8.76	11.16	1.51	6.34	1.09	3.29	0.18	0.39
S-14	black argillaceous rock	237.72	1.87	12.34	249.9	2.60	38.28	5.81	6.59	0.89				1.07	1.47	−34.42
3-19	black argillaceous rock	238.65	1.51	14.46	323.0	2.87	42.22	6.62	6.41	0.87	3.72	0.49	1.47	3.08	4.84
S-15	black argillaceous rock	240.98	3.83	12.34	287.3	2.99	44.00	16.44	18.65	2.52				0.99	1.49	−34.50
3-20	black argillaceous rock	241.91	2.48	13.87	345.0	3.19	47.01	6.98	7.05	0.95	6.34	0.86	2.61	0.70	1.18	−35.15
3-21	black argillaceous rock	245.17	3.47	12.63	261.0	2.65	39.06	18.80	20.84	2.81	7.73	1.16	3.49	0.37	0.75
S-16	black argillaceous rock	247.50	2.23	12.34	260.7	2.71	39.93	13.40	15.20	2.05				0.59	0.93	−35.32
3-22	black argillaceous rock	248.43	2.44	11.53	228.0	2.54	37.37	6.85	8.32	1.12	5.51	0.90	2.73	1.07	1.82
S-17	black argillaceous rock	250.76	3.03	13.66	356.5	3.35	49.33	14.16	14.51	1.96	<LOD			0.35	1.08	−35.31
3-23	black argillaceous rock	251.69	2.01	13.39	324.0	3.11	45.73	6.30	6.59	0.89	4.81	0.68	2.05			−34.41
3-24	black argillaceous rock	254.54	2.52	10.49	96.1	1.18	17.31	5.18	6.91	0.93	3.06	0.55	1.66	0.10	0.38	−32.58
2-1	kelly siliceous rock	265.90	0.14	12.09	132.0	1.40	20.64	0.94	1.08	0.15	2.26	0.35	1.07			−34.41
2-2	kelly siliceous rock	266.90	0.19	12.39	159.0	1.65	24.25	1.29	1.46	0.20	4.02	0.61	1.85	0.92	0.76	−32.86
2-3	kelly siliceous rock	267.90	0.14	11.62	168.0	1.86	27.33	0.55	0.66	0.09	2.47	0.40	1.21	1.01	0.80	−34.05
2-4	kelly siliceous rock	268.90	0.19	11.30	103.0	1.17	17.23	1.05	1.30	0.18	2.14	0.36	1.08	0.88	0.90	−33.78
2-5	gray siliceous rock	269.90	0.81	7.56	58.6	0.99	14.65	0.63	1.16	0.16	2.05	0.51	1.55	2.54	3.36
2#-1	gray siliceous rock	270.70	1.47	9.50	80.6	1.09	16.03	4.68	6.89	0.93	<LOD					−33.74
2-6	gray siliceous rock	270.90	0.12	7.77	45.4	0.75	11.04	1.19	2.14	0.29	1.85	0.45	1.36	0.87	1.14	−32.04
2-7	gray siliceous rock	271.90	0.37	9.44	36.3	0.49	7.26	0.91	1.36	0.18	3.20	0.64	1.93	1.07	1.37
2#-2	gray siliceous rock	272.05	0.65	7.69	190.7	3.18	46.86	8.22	14.95	2.02	6.00	1.47	4.45
S-18	black shale	273.05	5.53	11.87	34.5	0.37	5.49	4.87	5.74	0.77	2.51	0.40	1.21	1.21	2.60	−34.16
2-8	black shale	273.90	5.55	11.55	295.0	3.28	48.27	11.90	14.42	1.95	6.72	1.10	3.32	1.44	2.72
2#-3	gray siliceous rock	274.45	1.00	7.49	15.9	0.27	4.01	2.02	3.78	0.51	1.10	0.28	0.84
2#-4	gray siliceous rock	275.81	0.84	8.64	20.0	0.30	4.39	2.65	4.30	0.58	1.47	0.32	0.97			−29.92
2-9	black shale	275.90	4.44	9.09	54.4	0.77	11.32	1.51	2.33	0.31	2.71	0.56	1.70	0.20	0.29	−30.81
2-10	black shale	276.90	14.90	7.35	61.4	1.07	15.78	4.93	9.39	1.27	3.04	0.78	2.36	1.36	2.37
2#-5	gray siliceous rock	276.96	0.78	7.11	16.0	0.29	4.24	2.90	5.72	0.77	1.07	0.28	0.86
2#-6	chert	277.40	0.06	8.23	17.1	0.27	3.93	1.51	2.58	0.35	1.20	0.28	0.83
2#-7	black shale	277.41	7.80	5.77	7.6	0.17	2.48	30.86	74.88	10.11	0.63	0.21	0.62
S-19	black siliceous rock	277.45	0.63	10.60	42.0	0.51	7.48	6.15	8.13	1.10	2.82	0.50	1.52	2.78	3.86	−31.37
2-11	black shale	277.90	2.76	7.11	38.8	0.70	10.31	0.97	1.91	0.26	2.13	0.57	1.71	3.64	4.65
2#-8	chert	277.96	0.64	8.86	19.9	0.29	4.25	2.02	3.19	0.43	1.44	0.31	0.93			−31.04
2-12	black shale	278.90	4.28	8.16	43.2	0.68	9.99	2.49	4.28	0.58	2.77	0.64	1.94	2.54	4.48
S-20	black shale	279.25	7.49	10.62	31.6	0.38	5.62	6.22	8.20	1.11	2.49	0.44	1.34	1.89	2.86
2#-9	black siliceous rock	279.40	1.60	7.00	22.0	0.40	5.95	3.03	6.07	0.82	1.38	0.37	1.12			−32.85
2-13	black siliceous rock	279.90	1.00	5.52	26.7	0.62	9.15	1.56	3.96	0.54	1.41	0.48	1.46	7.29	7.22
2#-10	chert	281.30	0.44	6.66	21.2	0.41	6.02	2.02	4.24	0.57	1.13	0.32	0.96			−32.17
2-14	black siliceous rock	281.90	0.96	6.69	29.2	0.56	8.24	1.10	2.30	0.31	1.44	0.41	1.23	0.19	0.49
2#-11	chert	282.40	0.03	8.73	26.2	0.39	5.68	1.39	2.22	0.30	1.69	0.37	1.11			−29.80
2-15	black shale	283.90	14.96	9.22	62.4	0.87	12.79	5.20	7.90	1.07	3.02	0.62	1.87	0.82	1.15
S-21	black shale	284.50	7.88	12.04	83.2	0.89	13.07	16.34	19.00	2.56	4.70	0.74	2.23	0.05	0.23
2#-12	chert	285.35	0.75	8.29	30.3	0.47	6.92	2.78	4.69	0.63	1.79	0.41	1.23			−33.57
2-16	black siliceous rock	285.90	1.01	7.42	44.2	0.76	11.25	0.44	0.83	0.11	1.62	0.41	1.25	0.13	0.75	−32.50
2-17	black siliceous rock	287.90	1.05	7.69	43.7	0.73	10.75	0.75	1.36	0.18	1.84	0.45	1.36	0.46	0.90	−31.83
2-18	black siliceous rock	288.90	1.66	11.72	78.7	0.86	12.70	1.56	1.86	0.25	2.02	0.33	0.98	0.41	1.24	−31.57
2-19	black shale	289.90	9.46	10.65	93.6	1.13	16.61	6.05	7.95	1.07	2.79	0.50	1.49	1.30	2.12
S-22	black shale	289.95	5.39	6.90	32.8	0.61	8.98	0.63	1.27	0.17	1.91	0.52				−32.43
2-20	gray siliceous rock	290.90	0.84	12.30	83.9	0.88	12.89	0.87	0.99	0.13	2.30	0.35	1.07	0.18	0.56
2#-13	gray siliceous rock	291.40	0.75	6.97	22.7	0.42	6.14	4.55	9.13	1.23	1.40	0.38	1.15			−32.26
2-21	gray siliceous rock	291.90	0.32	13.35	99.8	0.96	14.13	0.67	0.70	0.09	1.95	0.28	0.83	3.02	4.23	−32.75
2-22	cyan argillaceous rock	292.90	0.48	13.41	113.0	1.08	15.93	1.59	1.66	0.22	2.32	0.33	0.99	1.53	1.95	−33.83
2-23	cyan argillaceous rock	293.90	0.20	13.20	84.2	0.82	12.06	4.93	5.23	0.71	1.90	0.27	0.82	0.73	0.96	−33.49
2-24	cyan argillaceous rock	295.90	0.24	13.28	79.0	0.76	11.24	0.78	0.82	0.11	1.79	0.25	0.77	0.30	0.53	−32.14
2-25	cyan argillaceous rock	297.90	0.12	13.51	76.7	0.73	10.73	0.53	0.55	0.07	1.85	0.26	0.78	0.65	1.01	−31.58
2-26	cyan argillaceous rock	300.90	0.21	14.72	121.0	1.05	15.54	0.48	0.46	0.06	1.77	0.23	0.69	0.40	0.55	−34.45
2-27	cyan argillaceous rock	303.90	0.20	12.79	94.4	0.95	13.95	0.58	0.64	0.09	1.78	0.26	0.79	7.56	14.72	−33.57
2-28	cyan argillaceous rock	306.90	0.08	14.22	87.3	0.79	11.60	0.59	0.58	0.08	1.96	0.26	0.79	0.71	1.00	−33.57
2-29	cyan argillaceous rock	309.90	0.17	14.87	102.0	0.88	12.96	0.36	0.34	0.05	2.03	0.26	0.78	0.39	0.48	−33.84
2-30	cyan argillaceous rock	312.90	0.13	15.54	118.0	0.97	14.35	0.37	0.33	0.04	2.32	0.28	0.85			−32.72
2-31	cyan argillaceous rock	313.90	0.15	16.10	103.0	0.82	12.09	0.53	0.46	0.06	2.36	0.28	0.84	0.19	0.23	−33.50
2-32	red argillaceous rock	315.90	0.09	16.49	121.0	0.94	13.87	0.50	0.43	0.06	2.13	0.24	0.74			−32.30
2-33	red argillaceous rock	317.90	0.09	16.55	109.0	0.85	12.45	0.38	0.32	0.04	2.06	0.24	0.71			−33.68

Enrichment factors (EF) are relative to average upper crust. LOD, limit of detection.

About 0.1 g of finely crushed material was treated with hydrofluoric and nitric acid in a closed Savillex vessel (polytetrafluorethylene polymer) on a hotplate at 130 °C. After at least 24 h, the sample was evaporated to dryness on a hotplate at 100 °C. Nitric acid was added, and the sample was evaporated to dryness again. This procedure was repeated once more. Nitric acid and water was then added, and the vessel was closed and placed on the hotplate at 130 °C for at least 12 h. The sample was then diluted to 50 mL with ultrapure water (type 1, Milli-Q). Before measurement, the sample was further diluted by a factor of 11 with 0.7% nitric acid. For analysis, PerkinElmer’s Total Quant method was used. The setup, data acquisition, calibration, and calculations were done using Elan software version 2.3.2. In PerkinElmer’s Total Quant method, calibration is based on a solution containing a limited number of elements (for example Al, V, Mo, and U) with a precision (%RSD, relative standard deviation) of trace element concentrations reported here of 3% (V), 15% (Mo), and 7% (U). After calibration with the elements solutions, performance was monitored using certified rock standards (44–47) with an accuracy (percent) relative to known values of 10% (V), 12% (Mo), and 15% (U) (PACS-2 excluded for U).

HHXRF analysis at NordCEE at Department of Geosciences and Natural Resource Management (IGN), University of Copenhagen, Denmark.

Trace metals and major elements in samples S-13, S-14, S-15 and S-16 (Table S1) were measured with a handheld energy-dispersive XRF spectrometer (HHXRF) model Delta DP-6000 with Rh anode from Olympus. The X-ray absorption was identified with the Olympus software where fundamental parameters were used to convert counts to concentrations by calibration to a standardized metal alloy coin with known trace element composition (supplied by manufacturer) (48). Powdered rock samples in vials were covered with thin plastic kitchen wrap (for details, see ref. 49) and measured upside down in the XRF stand. Powder thickness was generally above 5 mm to obtain the optimal noise normalization. The counts we made for 120 s on both 10-KHz and 40-KHz beams, for a total of 4 min. We measured a certified reference material (PACS-2) after every five samples, and the relative SD for all of the analyzed major elements was generally smaller than 5%. Of the trace metals, the relative SD for V was <5%, 14% for U, and 12% for Mo. Our final concentrations were determined by machine calibration to a database of samples (concentrations determined by ICP-MS) and standards of known concentration. Powders of certified standards (sediment NIST2702, sediment NIST2781, sediment MAG-1, sediment JDO-1, sediment SCO-1, sediment SDO-1, igneous rock ASK-2, and igneous rock ZGI-TS) were thus measured with both HHXRF and ICP-MS in Denmark (GEUS), and split samples (n = 54) were measured with both HHXRF [Denmark (DK)] and ICP-MS (China). Calibration curves typically have R² > 0.89. After calibration, major element concentrations for PACS-2 were generally within 4% of the certified values, within 33% for V, and within 10% for Mo. Estimating the accuracy of U concentrations is compromised, as U concentration in the standard PACS-2 (3 ppm) is only informal. Furthermore, the four samples measured with only HHXRF have U values around the detection limit for U, and the accuracy is unknown. Therefore, we omit these U values from the dataset. A summary of the accuracy of the various trace metal methods used is shown in Table S2.

Table S2.

Accuracy of trace element concentrations (percent) obtained with ICP-MS (China and DK) and HHXRF

Elements	ICP-MS (China)*	ICP-MS (DK)†	HHXRF (DK)‡
V	3	10	33
Mo	11	12	10
U	17	15	—

^*Relative to rock standard GBW 03014.

^†Relative to rock standards BCR-2, CLB-1, PACS-2 (not for U) and SGR-1b.

^‡Relative to rock standard PACS-2.

Results from Outcrop.

Samples were also collected from outcrop material generated from road cuts made within 2–3 y of sample collection. For outcrop samples, the surface several centimeters of the most weathered surface material were removed, exposing fresher material below. These samples were analyzed using the same methods as described for the core material, and results are shown in Fig. S2.

Carbon Isotopes.

The isotopic composition of carbon in extracted kerogen (δ¹³C_kerogen) was measured with a Flush EA 1112 HT O/H-N/C combined with a Delta V Advantage mass spectrometer (Thermo Scientific Co. Ltd.). The protocol for kerogen extraction included (i) taking 50 g of organic-extracted samples into acid reaction containers, and soaking with water for 2–4 h, then removing the upper liquid and other impurities; (ii) acid treatment with 6 mol/L HCl and 40% HF, then stirring at 60–70 °C for 1–2 h; (iii) washing with water to remove the acid, then centrifuging and removing the supernatant; (iv) flotation with heavy liquid (ZnBr₂-KBr solution with a relative density should be 2.0–2.1 g cm⁻³) to obtain the kerogen; (v) freeze-drying in a refrigerator at −5 °C for >6 h and then drying in an oven at 70 °C. (vi) If the LOI of kerogen is lower than 75%, pyrite must be removed. To do this, we added 6 mol/L HCl into the enriched kerogen, and added a small amount of zinc tablets until no hydrogen sulfide gas as released. Flotation with heavy liquid was used remove the impurities, and the kerogen was then washed with distilled water until halide ions were undetectable (test with 1% AgNO₃ solution), dried in an oven at 70 °C, and then bottled and sealed.

Biomarkers.

The biomarker analyses were performed at KLPG, China. All of the glass vessels used for bitumen extraction were combusted at 700 °C in a muffle furnace to remove any organics and ultrasonically washed with purified water. Then 200 g of powders were precisely weighed and extracted by Soxhlet extraction with chloroform for 8 h. The extracts (chloroform bitumen “A”) were collected in beakers (100 mL) and covered with aluminum foil, until the chloroform was completely evaporated in a fume hood. Then 25 mg of bitumen “A” was dissolved in an appropriate amount of n-hexane, and D₁₀-anthracene, 5α-androstane, and C₂₄D₅₀ as internal standards were added. Approximately 12 h later, the asphaltene precipitate was separated by filtration, and the filtrate was divided into saturated hydrocarbon, aromatic hydrocarbon, and polar fractions subsequently using 10 mL hexane and 20 mL dichloromethane:n-hexane (2:1), respectively, as elute in a silica gel glass column (∼100−200 mesh, activated at 200 °C for 4 h). The GC-MS analyses of the hydrocarbon fractions were performed using a Thermo Scientific TRACE GC Ultra-DSQ II mass spectrometer.

An HP-5 chromatographic column (60 m × 0.25 mm × 0.25 µm) was used to separate the saturated and aromatic hydrocarbon fractions. The oven temperature was initially set at 70 °C for 5 min, programmed to reach 220 °C at 4 °C/min increments, followed by 2 °C/min to 320 °C held for 20 min for the saturated hydrocarbons, and programmed from 70 °C for 5 min to reach 320 °C at 3 °C/min increments, then isothermal for 20 min for the aromatic hydrocarbons. Helium was used as a carrier gas with a constant flow rate of 1 mL/min. Both interface temperature and injection temperatures were 300 °C. The transfer line temperature was 250 °C, and the ion source temperature was 230 °C. The ion source was operated in the electron impact mode at 70 eV, and selected ion monitoring was performed.

Molecular biomarkers can often provide crucial information on microbial ecosystems and environmental evolution, but the syngenecity of Precambrian biomarkers has been doubted (50). We present the following evidence to demonstrate the syngenecity of the Mesoproterozoic molecular biomarkers from the Xiamaling Formation. Firstly, the organic matter has experienced only low levels of thermal maturity (equivalent vitrinite reflectance ≈ 0.6%, T_max < 450 °C) (16, 51). The high TOC and low maturity enhanced the prospects of preserving extractable biomarker molecules, otherwise a challenge for sediments with high thermal maturity. Secondly, the biomarker features of the Xiamaling Formation black shales and overlying Jurassic coal measure strata are very different (Fig. S3). Thus, the Xiamaling Formation does not contain younger Jurassic biomarkers, or any other demonstrable biomarkers from the Phanerozoic Eon. This control shows that the biomarkers we analyzed from the Xiamaling Formation are not from younger migrated hydrocarbons or from contamination during either sampling or analysis procedures. Lastly, the Xiamaling Formation itself displays significant differences in biomarker abundance and compositions between the different depths. For example, there are significant differences in the abundances of terpanes, steranes, and methyl steranes when comparing biomarkers from the depths of 273.9 m (upper layer of the OMZ), 283.9 m (within the OMZ), and 291.9 m (underlying the OMZ) (Fig. S3). Overall, the biomarkers of Xiamaling Formation sediments appear to represent indigenous organic signatures, and to record the marine biological community from the time of Xiamaling Formation deposition.

Alkylated trimethyl benzenes with dominantly 2,3,6-methylation were identified from the aromatic fraction, with the fragment ion of m/z 134 as the base peak (Fig. S4). The concentrations of C₁₈-TMAI and C₁₉-TMAI of Xiamaling Formation samples for different depths are presented in Table S1.

Further Details on Trace Metal Sediment Geochemistry

A critical aspect of our data interpretation involves comparing the geochemistry of V to Mo and U in modern environments. The main principles involving V transport and diagenesis and transport are covered in Evidence for Bottom Water Oxygenation. In what follows, we will focus on V behavior in a variety of environmental settings, and we compare and contrast the behavior of V with the behavior of Mo and U.

As mentioned in the main text, V is enriched together with Mo and U in euxinic settings. To provide specific examples, V is enriched together with Mo and U in sediments depositing in the euxinic waters of the Black Sea, the Cariaco Basin, and Saanich Inlet. Summaries of sediment geochemistries for these sites are shown in Table S3.

Table S3.

Trace metal geochemistry of euxinic sediments

Site	Al, wt%	Mo, ppm	U, ppm	V, ppm	Mo/Al, ×10−4	U/Al, ×10−4	V/Al, ×10−4	Reference
Black Sea Sta 6 (380 m)
Unit 1	4.07	69.7	13.1	116	16.6	3.23	28.1	(63)
Unit 2a	6.21	152	17.2	136	24.5	2.77	21.9	(63)
Black Sea Sta 7 (1,176 m)
Unit 1	3.29	39.4	13.1	102	12.0	3.99	30.9	(63)
Unit 2a	4.86	108	17.9	124	22.3	3.67	48.2	(63)
Cariaco Basin (Holocene average)	4.90	75.0	—	149	15.3	—	30.3	(23)
Saanich Inlet (top 10 m)	5.24	44.9	—	141	8.6	—	26.9	(64)
Crustal average	8.15	1.1	2.7	97	0.135	0.331	11.9	(61)

The concentrations of V, U, and Mo also have been explored in a variety of OMZ settings. For example, and as briefly mentioned in the main text, a detailed study was undertaken of the OMZ in the eastern tropical North Pacific off the Mexican coast, looking at sediment traps, surface sediment, and deep sediment (10–20 cm) (17). The study explored a transect extending from the outer shelf (100 m water depth) above the OMZ, through sediments deposited in the anoxic OMZ waters, and into deep waters of 1,020 m. At this deep site, bottom oxygen accumulated 17 μM, whereas the overlying waters were still oxygen-free.

Sediment traps were deployed at the sites with water depths of 510 m and 1,020 m, and, in both sites, trace metal behavior was similar. Molybdenum was enriched compared with crustal average values for traps from all depths at both sites, and U and V either maintained concentrations relative to crustal average values or displayed some loss.

For sediments collected from the top of sediment cores, Mo showed enrichments relative to crustal average values for sites depositing within the anoxic waters of the OMZ, while displaying crustal average values for sediments depositing above the OMZ, and at the deepest site (1,020 m) where the oxygenated water began to accumulate above the sediment. Uranium showed the most pronounced enrichments in the shallower anoxic OMZ sites (200–400 m depth), and V displayed crustal average values or slight enrichments within the anoxic part of the OMZ, with depletions in the deepest site where oxygen was found above the sediment surface.

Deeper within the sediment cores, at depths of 10–20 cm, U showed enrichments (about a factor of 4) at all sites. For Mo, enrichments were most pronounced for sediments deposited within the anoxic OMZ waters (about a factor of 8–10), and sediments deposited in oxygenated waters both above and below the anoxic OMZ waters were also enriched compared with crustal average (about a factor of 2), but less so than for sediments depositing in the anoxic waters. For V, sediments above and below anoxic OMZ waters were depleted relative to crustal average (by 10–40%), and sediments depositing within the anoxic waters were enriched (by about a factor of 2) compared with crustal average.

For sediments depositing within the Peruvian OMZ, somewhat similar trends were observed (21, 52). Sediments deposited both within anoxic OMZ waters and below where oxygen began to accumulate in the bottom water showed enrichments in U compared with crustal average. In this case, the enrichments were largest (up to a factor of 20) for sediments depositing within the anoxic waters, and lower (up to a factor of 3 or 4) for sediments in the deeper sites where oxygenated waters overlaid the sediment surface. Large enrichments were also found for Mo (up to a factor of 90) and V (up to a factor of 8) for sediments depositing in the anoxic OMZ waters. For sediments depositing in deeper water with oxygen overlying the sediment surface, but still below overlying oxygen-free waters, V returned to crustal average values and molybdenum enrichments reduced to a factor of 2–4.

These two OMZs share in common the following characteristics: U is generally well-enriched in sediments depositing above, within, and below anoxic OMZ waters, but the enrichments tend to be greatest within the anoxic waters. The behavior of Mo is similar, except that enrichments are less pronounced compared with U for sediments depositing in the oxygenated waters of the OMZ. Vanadium is enriched in sediments depositing in anoxic OMZ waters, but is either lost from the sediment or deposited at crustal values for sediments depositing in oxygenated OMZ waters.

As already indicated above from OMZ settings, V is generally either retained at crustal average values or released from sediments depositing under oxygenated conditions. In addition to the studies already indicated, sediments off the Washington coast were all deposited under oxygenated waters (19). Vanadium accumulated at crustal average values at all of the sites, and surface enrichments in V at some of the sites demonstrated the coupling between the V and manganese cycles. However, these sites converged on crustal average values for V with sediment depth. Pore-water profiles of dissolved V showed consistent release of V during Mn oxide reduction, but, for these sites, the V was efficiently captured in the surface sediments by reforming manganese oxides. For other sites depositing in oxygenated waters, as indicated above, and as highlighted in ref. 20, V may be lost from sediments during diagenesis. This seems to occur when oxygen penetration into the sediment is shallow (<1 cm) and where Mn oxides are not readily reformed from the reoxidation of dissolved pore water Mn²⁺ at the sediment surface.

To summarize, there are no modern sediments where V is lost from sediments depositing in anoxic waters, and, indeed, all modern sediments so far explored simultaneously gain U, Mo, and V under such conditions. Thus, sediments experiencing V loss uniquely express deposition under oxygenated water column conditions, as do sediments with Mo and U enrichments but lacking enrichments in V.

PAL O₂ Model

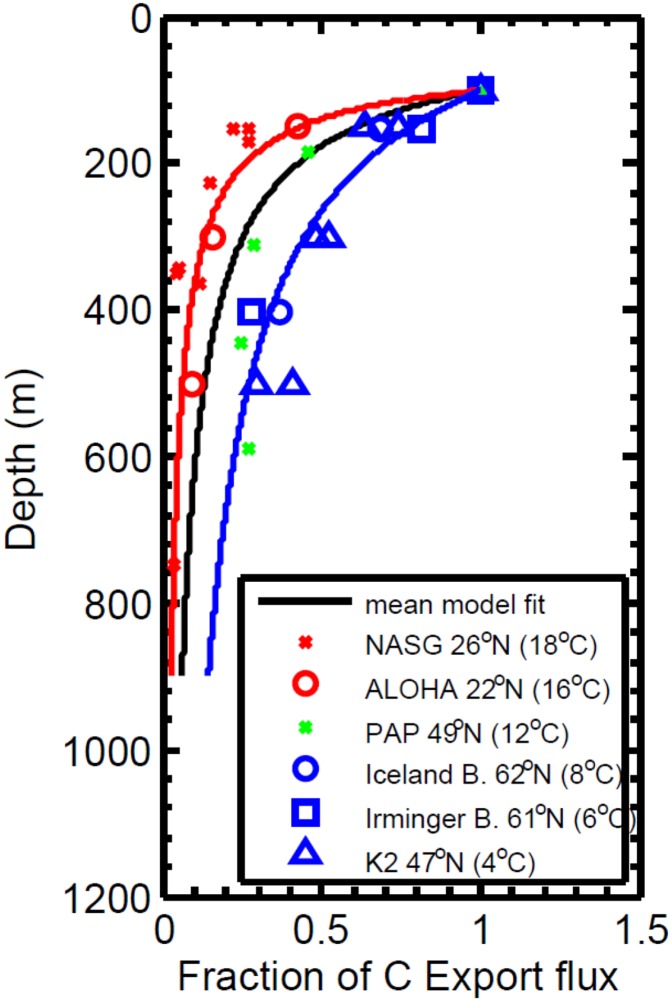
The basics of our oxygen model are described in the main text. Here we will offer details of the calculations and data used in constructing the model. As mentioned in the main text, a central parameter used in driving the model is the oxidation kinetics associated with sinking particulate organic matter in the oceans. In exploring these kinetics, we modeled the depth distribution of sediment trap particle fluxes as summarized in refs. 39 and 53. These sediment trap data have been collected using floating neutrally buoyant traps, and the results have been carefully evaluated for particle trap efficiency and the influence of “swimmers” on particle capture. These authors report strong latitudinal gradients in the dynamics of organic matter remineralization that they attribute to the influence of temperature on, among other things, the oxidation kinetics. Thus, sediment traps trapping particles from warmer waters show higher rates of mineralization compared with traps collecting water from higher, colder latitudes. The trap and model fits (see below) are reproduced in Fig. S5.

Fig. S5.

Sediment trap results from refs. 39 and 53 together with model fits as explained in Constraining Atmospheric Oxygen Levels. Model fits were conducted separately for the low-latitude warmer sites (red line), the high-latitude colder sites (blue line), and a mean fit to all of the data (black line).

In modeling these results, we used a reaction continuum model that assumes a broad continuous distribution of organic matter reactivities associated with the sinking particulates (54). As a result, more labile organic matter is used first, and the remaining organic material becomes more refectory as it ages while settling through the water column. As a result, remineralization rate decreases with increasing time and increasing depth in the ocean. We capture the aging process, in a simplified way, by using the gamma distribution to represent the reactivities in the reactive continuum model (55–57). In this model, the gamma distribution represents the integral of reactivity over the range of degradation rate constants (k) for the total amount of organic matter. Following ref. 55, a simple equation for the rate of organic matter degradation in the water column can be derived based on the gamma distribution,

$$R=-\nu {(a_{t=0}+a_{ocean}\left(z\right))}^{-1}\hspace{0.17em}\text{POC}\left(z\right)$$

where POC is the concentration of particulate organic carbon in the water column, a_t=0 is the mean lifetime of the fastest decaying components, ν defines the distribution near k = 0 (55, 57), and a_ocean is the age of the organic carbon as it settles through the water column,

$$a_{ocean}\left(z\right)=z{\left(w_s\right)}^{-1}.$$

With the value of w_s assumed to be much greater than vertical advection rate of water, our steady-state model one-dimensional conservation equation for POC is

$$w_s\frac{dPOC}{dz}-R=0.$$

We solve this numerically from the base of the mixed layer down through the water column with the present-day new production (NP₀) out of the mixed layer [NP₀(z₀) = −w_s POC] used as upper boundary condition. We identify the free parameters, w_s, a_t=0, and ν, by optimization of the vertical particle flux (F_org = −w_s POC) to the observed present-day particle flux data as collected from sediment traps at different latitudes (39, 53). We assume that 1.5 > ν > 1, which is equivalent to the apparent reaction order for R of less than 2 but greater than 1 (55). With this optimization by least-squares error to the log-transformed data, we find that in warm lower-latitude waters, the settling organic matter remineralizes at shallower depths with slower settling rates than at higher latitudes, in agreement with recent hypotheses (39, 58). The model-derived parameters for the different ocean regions are shown in Table S4.

Table S4.

Model parameters from best fit to sediment trap data from different regions of the ocean

Parameters	Mean of all data	Low latitude	High latitude
ws, m/d	29	20	44
at=0, y	0.010	0.007	0.014
ν	1.3	1.3	1.3

As explained in the main text, there are good reasons to believe that organic matter settling rates were much lower during the Mesoproterozoic Eon in a prokaryote-dominated ecosystem. We have followed the work of ref. 38, who calculate that micrometer-sized phytoplankton would sink at rates varying between 1 m⋅d⁻¹ and 6 m⋅d⁻¹ depending on the phase of a phytoplankton bloom. Higher settling rates (6 m⋅d⁻¹) would be experienced during peak bloom when higher particle densities allow cell aggregation and larger effective particles, whereas, after the bloom, the settling rate decreases to 2–3 m⋅d⁻¹. In our modeling, we focus on settling velocities of between 1 m⋅d⁻¹ and 6 m⋅d⁻¹, where higher settling velocities yield lower oxygen estimates as discussed in the main text.

The flux of organic matter decreases through the water column in our modeling, and, at a unique depth for each calculation, this flux equals the paleoburial flux of TOC (PF_org) as calculated for the Xiamaling Formation, and as explained in the main text. This flux is calculated to 0.136 mol C⋅m⁻²⋅y⁻¹ with a unit 3 sedimentation rate of 0.66 ± 0.14 cm⋅ky⁻¹ (16) and a TOC concentration of 10 wt% in the black shales of unit 3, a flux of 0.201 mol C⋅m⁻²⋅y⁻¹ with a TOC concentration of 15 wt%, and a flux of 0.272 mol C⋅m⁻²⋅y⁻¹ with a TOC concentration of 20 wt% (Fig. 1 and Fig. S5).

We use the factor PL to generalize our calculations both below and beyond present-day values of NP, so that NP = PL × NP₀. Likewise, we scale the settling velocity (w_s) in our calculations to the settling velocity obtained from our model fits to the sediment trap data (Table S4). A compilation of new production values for the global oceans is shown in Table S5. Our standard model case uses equatorial Atlantic values.

Table S5.

New production from various ocean regions

	Org C flux, mol⋅m−2⋅y−1
Latitude band	Atlantic	Indian	Pacific
Subpolar gyre >45°N	7.20		6.68
Subtropic gyre 15°N−45°N	2.54	9.52	2.07
Equatorial 15°N−15°S*	3.48*	2.18	1.59
Subtropic gyre 15°S−45°S	2.96	1.62	1.18
Southern Ocean <45°S	1.96	1.32	1.25
Average	3.21	2.04	1.83

Data are from ref. 65.

^*Used in model base case.

The oxygen utilization rate (OUR) at any depth z_pal is OUR = ηR, where η is the Redfield ratio of oxygen consumption to organic matter carbon remineralization; η is typically taken to be 150/106, but recent evaluations point toward η = (118 ± 30)/106 in equatorial and upwelling regions (59). Conservatively, we take η = 1, which will minimize our minimum O₂ estimates, recognizing that the estimates could be higher by about 30–90% if η is as high as 1.9 as in subtropical parts of the ocean (i.e., 200/106) (59).

From OUR, the total amount of oxygen respired, also called apparent oxygen utilization (AOU), is calculated by multiplying the OUR by the water turnover time (or water age) as a function of depth [τ_o(z)].

$$\text{AOU}\left({\text{z}}_{\text{pal}}\right)=\text{OUR}\left({\text{z}}_{\text{pal}}\right){\tau }_{\text{o}}\left({\text{z}}_{\text{pal}}\right)\text{.}$$

In the main text, we highlight our model using a water age distribution for the South Atlantic Ocean (Fig. S6), which gives the shortest open ocean waters ages in the modern oceans and, therefore, the lowest estimates for atmospheric oxygen. We note that the water transmitted to an OMZ between isopyncal surfaces can mix with water from adjacent isopycnal layers of different water age, providing a spectrum of water ages for a given parcel of water (34). Generally, water with older ages will have experienced more respiration, and, in our calculations, this will yield, ultimately, higher estimates for minimum oxygen concentrations. In Table S6, we summarize water ages from various restricted and semirestricted marine basins. These show similar ranges in water ages to those from the open ocean. The Sea of Japan (∼35°N–45°N) has relatively short turnover times at shallow depths because it is influenced by convection and deep water cascading by cold monsoonal winds from the continent at the extratropical latitudes (60). This is a clearly different dynamic than expected from the paleotropical latitudes of the Xiamaling Formation.

Fig. S6.

Potential temperature (T) and water turnover time (τ₀) of the South Atlantic used in the main model (Fig. 3). Solid lines are robust loess fits (quadratic fit), and dashed lines are lower and upper range estimates used in the model based on icehouse and greenhouse conditions. Potential temperature is from the Global Data Analysis Project data set (62), and τ₀ is from ref. 34.

Table S6.

Water ages for enclosed and semienclosed basins

Basin	Method	Depth, m	Water age, y	Reference
Sea of Japan	3He	298	5–7	(66)
		496	13–14	(66)
		743	16–19	(66)
Black Sea central basin	chlorofluorocarbon	80	4.8	(67)
		120	12.5	(67)
		150	21.1	(67)
		180	30.8	(67)
		200	44.4	(67)
		240	59.7	(67)
Arabian Sea	modeling	100–1,000	6.5	(68)
	modeling	200–1,000	11	(69)
Bay of Bengal	modeling	100–1,000	12	(70)

Sensitivity Analyses

We generated a variety of sensitivity analyses of our model. Our variables include (i) new production rate, (ii) particle settling velocity, (iii) organic matter reactivity, (iv) carbon burial flux in the Xiamaling Formation, (v) water age, and (vi) temperature. Each model run we performed involved setting variables iii–vi, and exploring variables i and ii over ranges that we consider most reasonable for the Mesoproterozoic Eon. For each model run, our main interest was to find the minimum level of oxygen within the modeling space represented by the most reasonable range of variables i and ii. Each of variables iii–vi changed these minimum values. For example, higher temperatures increased minimum values of atmospheric oxygen (for example, see Fig. 3 D−F). Increasing organic matter reactivity (iii) also increased our estimates of atmospheric oxygen, whereas decreasing reactivity decreased these estimates, which is explored in more detail below (Fig. 3 D−F). As mentioned in the main text, higher, rather than lower, organic matter reactivity better suits the paleoenvironmental setting of the Xiamaling Formation, as the higher reactivities in the modern ocean are associated with higher temperatures.

Lower organic matter burial fluxes (vi) in the Xiamaling Formation generate lower values of atmospheric oxygen, whereas higher burial fluxes generate higher concentrations. Because bottom water oxygenation was a persistent feature of the Xiamaling Formation regardless of the range of TOC burial fluxes, we can argue that the burial fluxes yielding the highest oxygen concentrations are the most relevant, as bottom water oxygenation persisted even with these. Nevertheless, we have chosen to be conservative and use a mean TOC concentration of 15 wt% (for the black shales in unit 3) for our base case modeling. In Fig. S7, we explore how variability in the TOC burial flux, determined from TOC concentrations of 10 wt% and 20 wt%, influence estimates of atmospheric oxygen. These results can be compared with the 15 wt% TOC used in Fig. 3.

Fig. S7.

Contours of AOU, water depth, and atmospheric oxygen (%PAL) calculated with Xiamaling Formation burial flux as determined from both 10 wt% and 20 wt% TOC concentrations. Otherwise, these results are generated from the same parameters used for the “base case” scenario presented in Fig. 3.

As mentioned in the main text, and above, water age (v) has a direct influence on our estimates of atmospheric oxygen concentration. In our sensitivity analysis, we explored a range of water age profiles as shown in Fig. S8. We also used, in our analyses, a temperature−depth distribution increased by 10 °C from the present tropical Atlantic, as shown in Fig. S6.

Fig. S8.

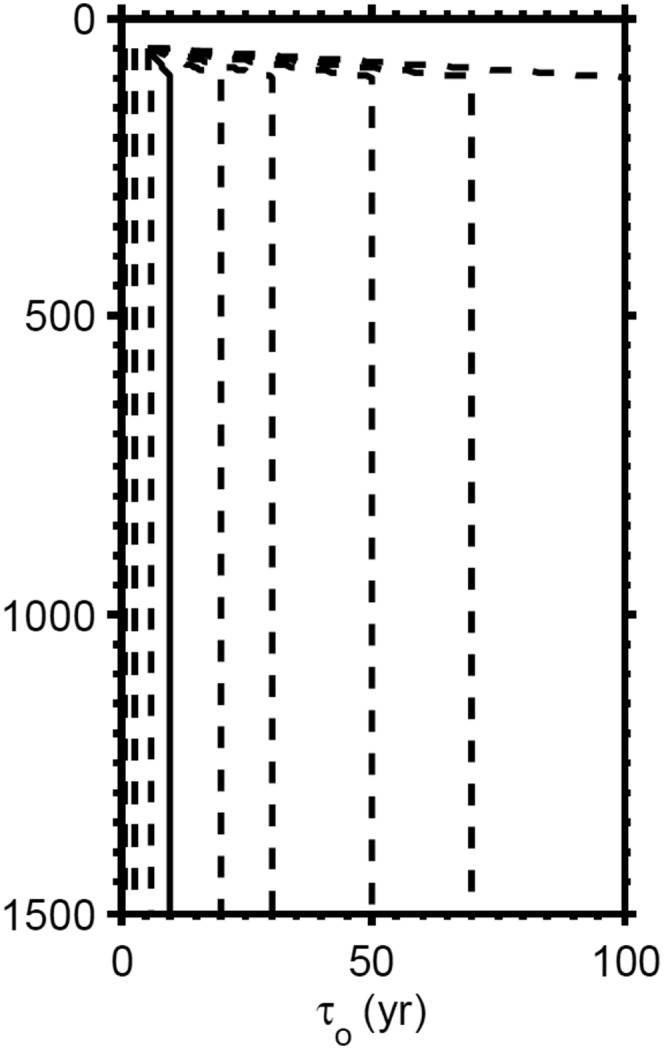
Spectrum of water age distributions used in sensitivity analysis.

We extracted minimum levels of atmospheric oxygen from a series of sensitivity analyses (of the type presented in Fig. 3) with carbon burial rates generated from 10 wt%, 15 wt%, and 20 wt% TOC, and with organic matter reactivities representing the low-latitude high-temperature case, the high-latitude low-temperature case and the combined data. Additional model parameters included modern temperature−depth distribution and minimum water age of 10 y. These results are shown in Table S7. As mentioned above, the results generated from the low carbon flux case (10 wt %TOC) are not relevant because bottom water oxygen persisted in the Xiamaling Formation at higher levels of TOC. Other aspects of this sensitivity analysis are discussed in the main text.

Table S7.

Minimum oxygen levels in %PAL for different model scenarios

TOC contents, wt%	Low latitude	Combined	High latitude
10	3.0	1.8	0.6
15	6.2	3.8	1.34
20	10.5	6.5	2.3

All values calculated with modern mean tropical Atlantic temperature distribution and new production and with minimum water age of 10 y below the mixed layer.