Quantifying the spatial extent of the corollary discharge benefit to transsaccadic visual perception

Abstract

Extraretinal information, such as corollary discharge (CD), is hypothesized to help compensate for saccade-induced visual input disruptions. However, support for this hypothesis is largely for one-dimensional transsaccadic visual changes, with little comprehensive information on the spatial characteristics. Here we systematically mapped the two-dimensional extent of this compensation by quantifying the insensitivity to different displacement metrics. Human subjects made saccades to targets positioned at different amplitudes (4° or 8°) and directions (rightward, oblique, or upward). After the saccade the initial target disappeared and, after a blank period, reappeared at a shifted location—a collinear, diagonal, or orthogonal displacement. Subjects reported the perceived shift direction, and we determined the displacement detection based on the perceptual judgments. The two-dimensional insensitivity fields resulting from the perceptual thresholds had spatial features similar to the saccadic eye movement variability: 1) scaled with movement amplitude, 2) oriented (less sensitive to the change) along the saccade vector, and 3) approximately constant in shape when normalized by movement amplitude. In addition, comparing the postsaccadic perceptual estimate of the presaccadic target location to that based solely on the postsaccade visual error showed that overall the perceptual estimate was approximately 50% more accurate and 35% less variable than estimates based solely on this visual information. However, this relationship was not uniform: The benefit of extraretinal information was observed largely for displacements with a component parallel to the saccade vector. These results suggest a graded use of extraretinal information when forming the postsaccadic perceptual evaluation of transsaccadic environmental changes.

humans sample their surrounding environment with saccades, quick eye movements that bring the fovea to objects of interest. The neural origin of the respective motor commands can vary from saccade to saccade because of differences in the movement metrics (amplitude and direction). For example, the motor signals for horizontal and vertical saccades are generated by distinct neural areas (Leigh and Zee 2006). In addition, these movements are inherently variable. Numerous experimental paradigms have shown that saccades largely fall short of intended targets (Collewijn et al. 1988; de Bie et al. 1987; Leigh and Zee 2006) and movement end-point variability scales with saccade amplitude (Abrams et al. 1989; van Beers 2007).

Because of the brief disruptions to visual input that accompany each saccade and the high frequency of saccades required to sample the environment, these movement imprecisions should impact visual perception. Specifically, the imprecision of each movement (and the resulting visual error between the fovea and the targeted object) creates a difficult credit assignment problem: Was the completed movement incorrect, or was there a change in the environment during the movement? Despite this challenge, multiple studies have shown that visual perception remains largely accurate despite the movement undershoot, end-point variability, and associated visual input disruptions (Wurtz 2008). There are also compelling, and generally complementary, theories on how the brain compensates for movement-induced disruptions to visual perception and maintains space constancy across saccades and over time: calibration of the visual environment based on reference objects (Deubel et al. 1998, 2002), comparison of the scene before and after the saccade to determine a mismatch (Sperry 1950; von Holst and Mittelstaedt 1950), construction of spatiotopic reference frames for visual memory (Burr and Morrone 2011; Zimmermann et al. 2013a, 2013b, 2014), and predictive remapping of objects during the eye movement (Jonikaitis et al. 2013; Rolfs et al. 2011; Szinte et al. 2015). Most perceptual studies and subsequent theories are based on the ability to detect a change in the environment that occurs during the eye movement. For example, humans accurately perceive small (<1°) transsaccadic displacements to saccade targets, both when the target is blanked before the displacement and when the target shift is not proceeded by target blanking (horizontal movements: Bridgeman et al. 1975; Collins et al. 2009; Deubel et al. 1996, 1998, 2002; Joiner et al. 2013a; Ostendorf et al. 2010; vertical movements: Bansal et al. 2015). (Note that the ability to detect the target shift is generally higher when the target is not blanked due to saccadic suppression of displacement.) This ability is hypothesized to be facilitated by a corollary discharge (CD) signal, a duplicate of the motor command used to estimate the sensory consequences of the movement (Gottlieb et al. 1998; Guthrie et al. 1983; Hall and Colby 2011; Sommer and Wurtz 2006; Sperry 1950; von Holst and Mittelstaedt 1950). The contribution of CD to visual perception has been assessed by examining postmovement estimates of transsaccadic target displacements (Bridgeman et al. 1975; Collins et al. 2009; Deubel et al. 1996, 1998, 2002; Ostendorf et al. 2010) and actual and apparent object motion (Szinte et al. 2013; Szinte and Cavanagh 2011). In displacement detection tasks subjects are required to make a saccade to a peripheral target. The target is extinguished during the movement and reappears (either immediately or after a delay) at a displaced location. These target shifts have typically been studied in one of two directions (along 1 axis) with respect to the movement vector (e.g., aligned with or orthogonal to the saccade direction). Subjects are required to report the target displacement direction, and, based on these decisions, the resulting psychometric function provides estimates of perception: the perceptual threshold (the smallest displacement amount that is detected at a level greater than chance) and bias (the target displacement at which the subject reports both shift directions at chance level—the postsaccadic judgment of the presaccadic target location).

Previous studies have confirmed that humans are sensitive to one-dimensional displacements to the saccade target (i.e., changes parallel to the eye movement) despite the variability of the saccadic end points, and that this sensitivity scales with movement amplitude (Bansal et al. 2015; Bridgeman et al. 1975; Collins et al. 2009; Li and Matin 1990, 1997; Niemeier et al. 2007; Ostendorf et al. 2010). Niemeier et al. (2003, 2007) and recently Wexler and Collins (2014) demonstrated that perception is also influenced by the metrics of the target shift with respect to the movement vector; detection thresholds were lower for target shifts that had an orthogonal component than for displacements purely parallel to the saccade direction. Based on the above studies, there is increasing, but isolated knowledge on how the metrics of both the saccade and the visual change influence the purported ability of the CD to compensate for saccade-induced visual input disruptions. Moreover, except for a few reports (e.g., Wexler and Collins 2014), the majority of previous studies have largely focused on perception comparisons for one-dimensional transsaccadic visual changes for a single movement amplitude and direction. Therefore, the complete spatial characteristics of the CD-based compensation remain unclear. That is, there is a lack of information on how CD is possibly utilized to compensate for the combination of saccade metrics (amplitude and direction) and the orientation and magnitude of internal (saccade end-point variability) and external (saccade target displacements) variations.

Here we addressed two questions. First, what is the two-dimensional spatial range of the perceptual insensitivity to the target displacement, and how is this perceptual insensitivity field (PIF) near the saccade end point influenced by movement amplitude and direction? Second, to what extent does extraretinal information (such as the saccade CD) benefit the postsaccadic estimation of the presaccadic target location, and what influence do the target displacement direction and the saccade amplitude and direction have on this capacity? Our results demonstrate for the first time that the two-dimensional spatial insensitivity to transsaccadic target displacement is a near-constant percentage of the saccade length—the perceptual thresholds scaled with movement amplitude at a near-constant percentage that was modulated by the direction of the target shift with respect to the saccade vector. In addition, extending the original analysis of Deubel et al. (1996), we found that overall the perceptual judgment of target location was on average 50% more accurate and 35% less variable than estimates based purely on the difference between the fovea location and the displaced target. However, the largest benefit of extraretinal information over solely postsaccade visual error information was observed for displacements with a component parallel to the saccade vector, while target shifts purely orthogonal to this vector exhibited less of an improvement. This reflects a limitation of the benefit of extraretinal information to perception due to the spatial properties of the inherent saccade variability.

METHODS

Twelve healthy human subjects (7 men and 5 women; 18–24 yr of age) were recruited for this study. Participants reported normal or corrected-to-normal vision and were unaware of the purpose of the study, which consisted of making perceptual judgments about the location of presented visual stimuli. Subjects did not obtain any training in the tasks prior to collection of experimental data and received payment after completing multiple test sessions over 4 days. Experimental protocols were approved by the Institutional Review Board of George Mason University, and informed consent was obtained from each participant.

Apparatus and measurement.

Eye movements were recorded with the Eyelink II eye tracker (head-mounted binocular eye tracker, 500-Hz temporal resolution, 0.2° spatial resolution; SR Research, Mississauga, ON, Canada). Stimuli were presented on a 19-in. CRT monitor (screen resolution 1,024 × 768 pixels; refresh rate 110 Hz) at a viewing distance of 62.5 cm. The fixation cross and targets were white with a luminance of 56 cd/m² viewed against a black background of luminance of <0.1 cd/m². Subjects were seated in a dimly lit room in a stationary chair with their head stabilized by a chinrest. Stimulus presentation, eye movement, and manual keyboard response data acquisition were achieved with real-time experimental control software (Experiment Builder, SR Research). At the start of each experiment session, a 9-point gaze calibration was performed, followed by a 9-point validation. Calibration and/or validation were repeated until the error was smaller than 1° on average, as previously done in studies conducted under these standard calibration settings (Peterson and Wong 2008; Wong and Peterson 2011).

Task and procedure.

In this experiment, each trial began with a central fixation cross (0.3° in extent). Subjects were required to maintain fixation on this cross for a variable period (random duration between 1,200 and 1,800 ms; Fig. 1A). After the fixation period, an initial target was presented at one of two amplitudes (4° or 8°) and three directions [upward, oblique (45°), or rightward] from the fixation cross—six possible locations (Fig. 1B). Subjects were required to make a saccade to the initial target. Once the eye position exceeded a virtual square window (3.2° in width, ±1.6° from fixation) around the fixation point, the cross and target were extinguished and followed by a 250-ms blank period to reduce saccade suppression of displacement, similar to the blank-step condition initially performed by Deubel et al. (1996). The target was extinguished on average 6.8 ms after peak saccade velocity. The initial target then reappeared at a shifted randomized position between ±2.5° (0.5° increments) (Fig. 1C). The target shift was randomly drawn from a Gaussian distribution centered at 0°, with the smaller, less prominent shifts being sampled more than the larger, more detectable shifts (Fig. 1C). After the reappearance of the target, the subject made a manual response, using a keyboard to indicate the direction in which the target shifted. The numbers on the 3 × 3 keypad corresponded to the directions of the possible shifts (1 for 225°, 2 for 270°, 3 for 315°, 4 for 180°, 6 for 0°, 7 for 135°, 8 for 90°, and 9 for 45°) (Fig. 1D). Within a given session there were only two possible manual response choices: 1 or 9, 2 or 8, 3 or 7, and 4 or 6. Subjects had to make this manual response within 3,000 ms but were given no instructions on reaction time. (Note that all subjects responded within this allotted time period.) No feedback was given to subjects to indicate correct or incorrect responses. Each subject completed four sessions of 468 trials each. The amplitude and direction of the initial target were randomly and equally distributed among all six possible locations (4° and 8°, rightward, upward, and oblique). For each session the target shift direction was fixed at horizontal (0° and 180°), vertical (90° and 270°), diagonal left (135° and 315°), or diagonal right (45° and 225°). We counterbalanced the order of the sessions across subjects.

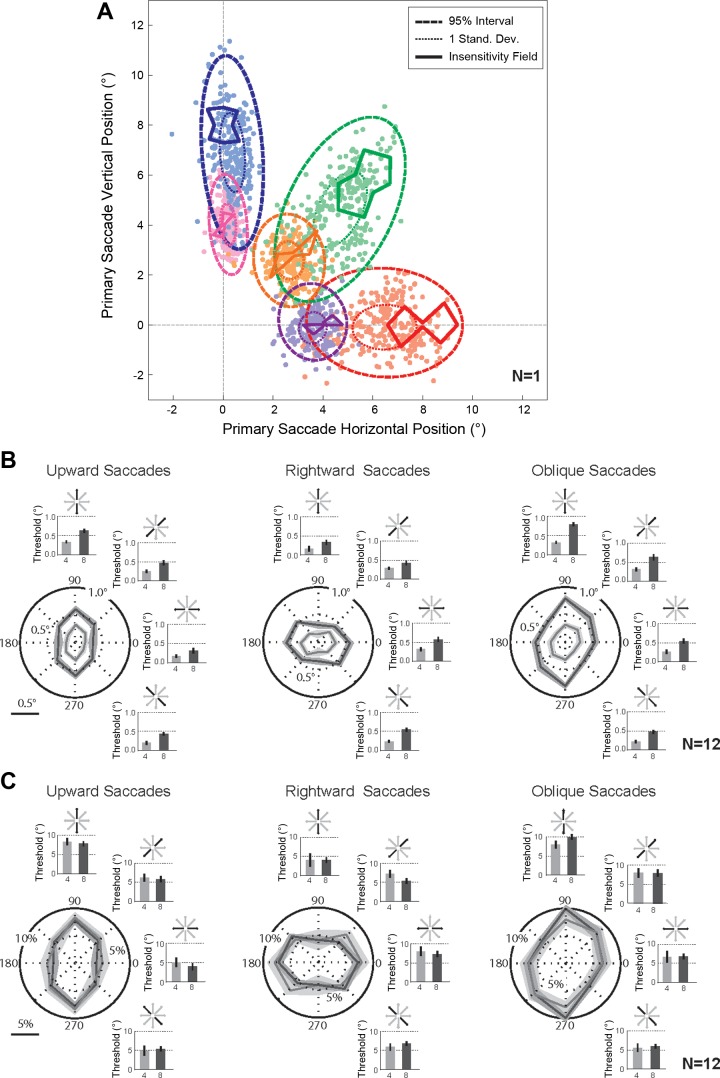
Fig. 1.

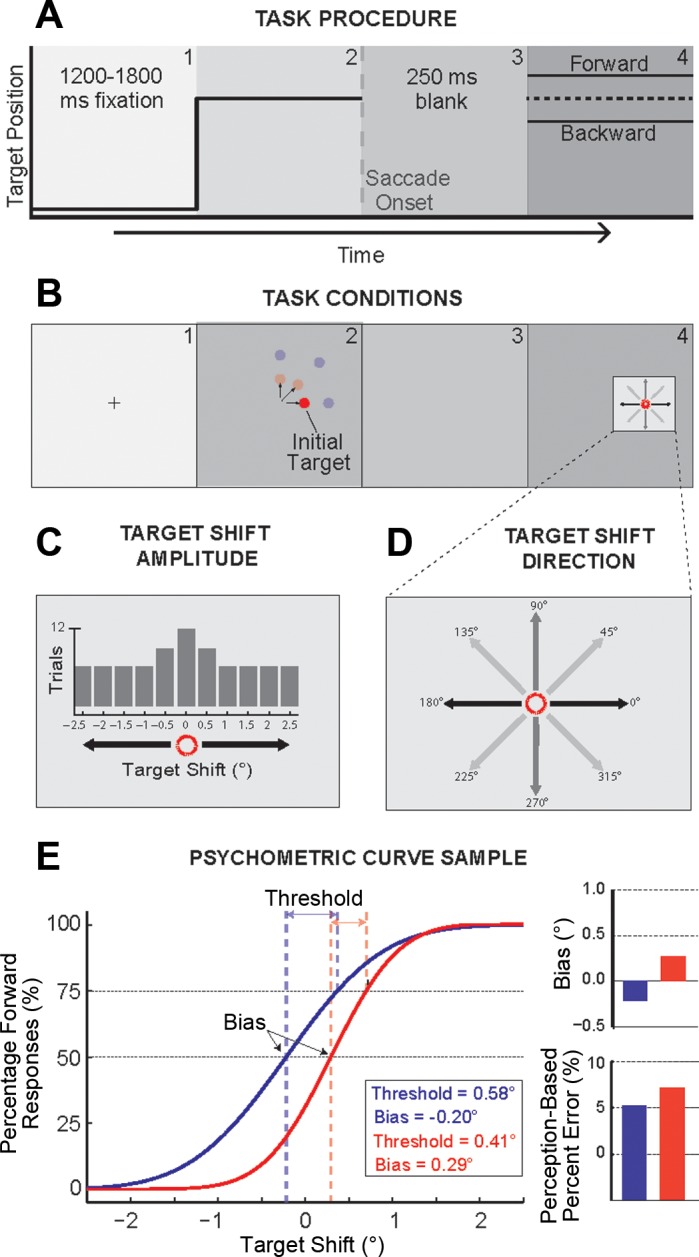
Transsaccadic shift detection task. A: task procedure. Each trial began with a central fixation cross appearing for a variable period (1,200-1,800 ms), followed by the appearance of an initial target. Subjects were required to make a saccadic eye movement toward this initial target, and the target was displaced during the saccade, reappearing at a new location after a 250-ms blank. B: task conditions. The initial target appeared randomly at 4° or 8° in the horizontal, oblique, (45°), or vertical direction from the central fixation point. C and D: the displaced target appeared randomly at shifted positions according to a Gaussian distribution ranging from −2.5° to 2.5° shifts (C) where the displacement was collinear, orthogonal, or diagonal to the initial saccade direction (D) (note that only 1 shifted direction was used per experimental session). After the appearance of the target at the shifted location, subjects were required to indicate the direction of the displacement (backward or forward) using numbered keys on the keyboard (4 or 6, 2 or 8, 3 or 7, 1 or 9 depending on the test session). E: psychometric functions. Frequency of forward responses on the y-axis is plotted as a function of target displacement on the x-axis. These manual response data were fitted to a cumulative Gaussian distribution to determine the psychometric function. Two example psychometric curves are shown. Two perceptual measures are derived from each psychometric function: the perceptual threshold and bias. The threshold is computed as the difference in target displacement between the 50% and 75% points of the sample psychometric curves. The perceptual bias (bar graph, top right) is the perceptual null location and was taken as the displacement from zero at the point where the percentages of forward and backward responses were equal to 50%. This measure is the postsaccadic estimate of the presaccadic target location. The % error of this estimate is the absolute value of the bias divided by the target amplitude, scaled by 100. Note that % error for the example psychometric curves increases with the magnitude of the bias (bar graph, bottom right).

Saccade measures.

Horizontal and vertical movements of both eyes were recorded, and the resulting data were visualized, filtered, and analyzed off-line with the MATLAB v 8.1.0 environment (MathWorks, Natick, MA). During the task, saccade initiation was detected when the saccade left the 3.2° square fixation window. For off-line analyses, an eye movement was classified as a saccade if both eye velocity and acceleration exceeded 50°/s and 2,000°/s², respectively. These proved to be reasonable criteria in order to both correctly detect saccades and meet the inclusion criteria across the different movement amplitudes and directions. Saccade onset and offset were determined when eye velocity and acceleration rose above and fell below these criteria. Saccadic end points were examined for every amplitude and direction. Only trials in which 1) the saccade was initiated within the fixation window, 2) its distance exceeded one-third of the initial target amplitude, and 3) the primary saccade end point was within the average eye position ± 2 standard deviations (SDs) were analyzed. On average 85.6 ± 2.2% of trials for each subject were included in the analysis.

Psychometric curves.

We determined the postsaccadic estimate of the presaccadic target location and the insensitivity to the target shift based on fitted psychometric curves to the experimental data. As in our previous work (Bansal et al. 2015; Joiner et al. 2013a), we estimated these parameters for perception-based judgments (which we refer to as Visual Error + Extraretinal based) by plotting the percentage of forward keyboard responses as a function of target shift. [We use the term Visual Error + Extraretinal to reflect that both the postsaccade visual error and extraretinal information could be used to form the perpetual decision. Although CD is the likely extraretinal signal utilized, we cannot rule out the contribution of a slowly developing proprioception signal providing eye position information (Xu et al. 2011).] Similar to the analysis of Deubel et al. (1996), to estimate these parameters based on the postsaccade visual error information (which we refer to as Visual Error based), on every trial we first determined the difference between the eye position at the time of target reappearance and the shifted target location. The direction of the resulting error vector was used as the basis for the judgment of the target shift. The percentage of these Visual Error-based forward judgments was again plotted as a function of target shift. That is, we determined the hypothetical psychometric function if subjects assumed that the saccade correctly brought the fovea to the target, and the visual error experienced represented the shifted target direction. In this case we assume that subjects have no extraretinal information of the metrics of the completed saccade and perceive the target shift in the direction of where the target now reappeared. Although this is not the case, perceptual measures based on this assumption provide a baseline to determine the extent to which the use of extraretinal information improved perception.

In both cases (Visual Error + Extraretinal and Visual Error) the data were fitted with a cumulative Gaussian distribution as shown by the example curves in Fig. 1E. From these curves, the perceptual bias and threshold were derived. The perceptual bias is the perceptual null location (PNL): the shift amount where the forward and backward judgments occur with equal frequency (50%). We took this point as the postsaccadic estimation of the presaccadic target location. A positive bias indicated that the perceived target location was ahead of the actual target position; a negative bias indicated that the target location was perceived behind the actual position. The difference in shift size between the 50% and 75% points on the psychometric curve represented the perceptual threshold. This measure quantified the ability to perceive the target displacement; larger thresholds or smaller slopes represented an increase in difficulty for accurately perceiving the target shift (Fig. 1E). We used these perceptual thresholds to determine the PIF: the amount of displacement required for the perception of a specific directional change in the target position (Fig. 2, A and B). To determine whether there was a systematic relationship between the saccade amplitude and the perceptual threshold, we compared the normalized thresholds (Fig. 2C, Fig. 3, and Fig. 4). For each subject, we scaled each threshold by the mean saccade amplitude, defining the threshold as a percentage of movement length. For a direct comparison between collinear and orthogonal cases, we plotted the respective thresholds (Fig. 3A) and normalized thresholds (Fig. 3C). We also examined movement variability in the same way and compared the SD of the saccade scatter in the collinear and orthogonal directions (Fig. 3B) and when normalized by the saccade amplitude (Fig. 3D).

Fig. 2.

Perceptual thresholds and insensitivity fields. A: primary saccade variability and perceptual insensitivity fields (n = 1). Primary saccades (filled circles) are plotted for each target amplitude and direction for 1 sample subject for each target (rightward 4°, purple; rightward 8°, red; oblique 4°, orange; oblique 8°, green; upward 4°, pink; upward 8°, blue); thick dashed ellipses show the 95% confidence interval, and thin dotted ellipses represent the 1 SD extent of the saccade variability. Perceptual thresholds for each shifted target location are represented as perceptual insensitivity fields (thick solid lines) for each target amplitude and direction (for 1 sample subject) with the same color code as the movement variability. B: perceptual insensitivity fields and respective perceptual thresholds (n = 12). Polar plots display the perceptual thresholds for each target shift direction for each movement direction and amplitude (saccades to 4° targets, light gray; saccades to 8° targets, dark gray). The respective perceptual thresholds are also represented as bar graphs following the same color code. The schematic above each bar graph depicts the direction of the target shift. C: normalized perceptual insensitivity fields and respective perceptual thresholds (n = 12). Polar plots display the normalized perceptual thresholds for each target shift direction for each movement direction and amplitude (saccades to 4° targets, light gray; saccades to 8° targets, dark gray). The respective normalized perceptual thresholds are also represented as bar graphs following the same color code. Again, the schematic above each bar graph depicts the direction of the target shift.

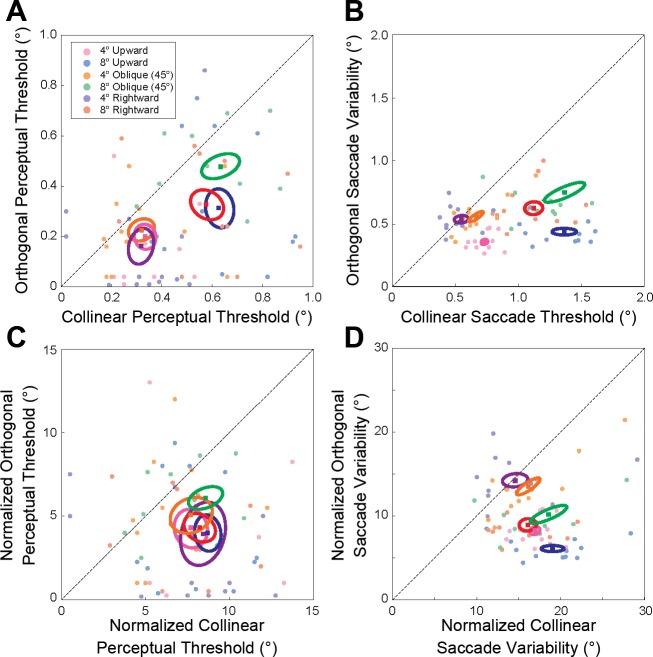
Fig. 3.

Comparison of the perceptual sensitivity to collinear and orthogonal target displacement and the collinear and orthogonal components of saccade variability. A and C: actual (A) and normalized (C) thresholds for orthogonal shifts of the saccade target plotted as a function of the respective thresholds for collinear target displacements for all subjects. B and D: actual (B) and normalized (D) orthogonal SD of the saccade variability plotted as a function of the respective collinear SD for all subjects. In each plot filled circles represent individual subject data for saccades to the different targets (rightward 4°, purple; rightward 8°, red; oblique 4°, orange; oblique 8°, green; upward 4°, pink; upward 8°, blue). Larger filled squares and ellipses represent mean and 1 SE confidence interval across subjects, respectively.

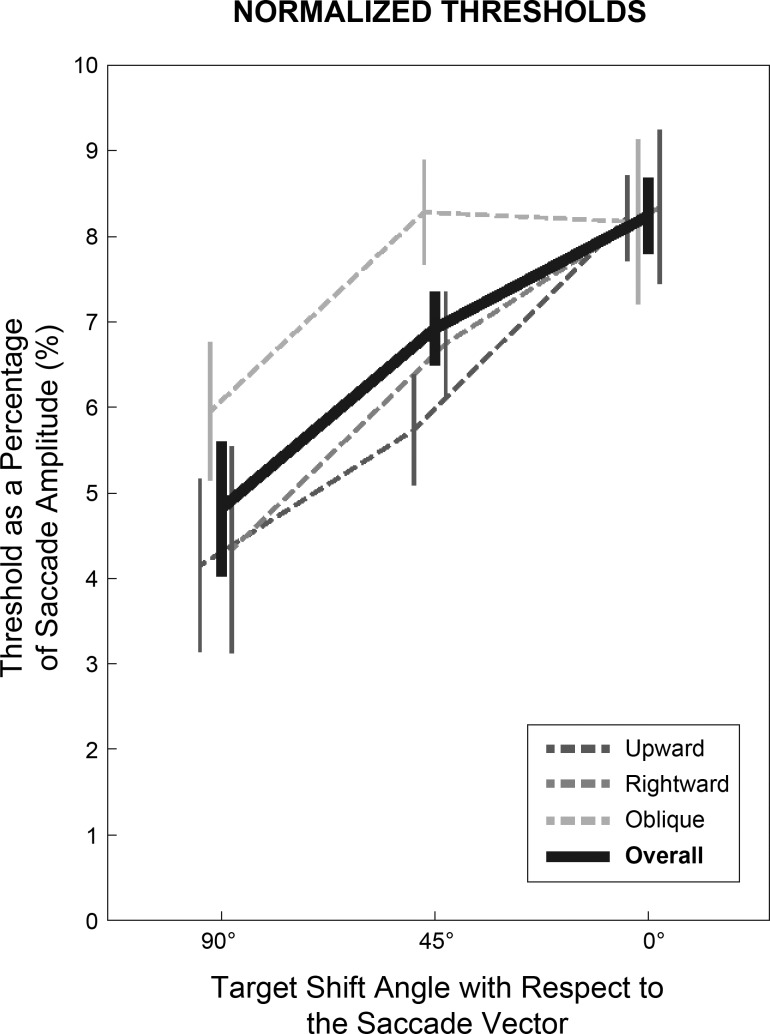
Fig. 4.

Comparison of orthogonal, oblique, and collinear normalized perceptual thresholds. Average normalized (with respect to saccade length) thresholds for orthogonal, oblique, and collinear shifts of the saccade target across movement amplitudes are shown for the 3 saccade directions (upward, rightward, oblique). Overall normalized thresholds across all saccade amplitudes and directions are represented by the thick black line. Respective vertical lines represent the SE across subjects.

In our analysis we examined the target shift on every trial and determined the decision the subject would have made if just reliant on this postsaccade visual error between the fovea and the target. We then plotted the percentage of forward responses based on this visual error criterion as a function of the target shift (Fig. 5). The original analysis of Deubel et al. (1996) plotted the percentage of forward responses as a function of visual error and qualitatively showed that performance was better described by the target shift. Although subtle, this difference (analyzing performance based on the visual error as a function of target shift vs. analyzing performance as a function of visual error) has a substantial impact on the results and interpretation. Specifically, unlike Deubel et al. (1996), our analysis can directly compare the bias and SD of the fitted psychometric functions and make an equivalent comparison between decisions based on only the postsaccade visual error and the actual perceptual responses of the subject.

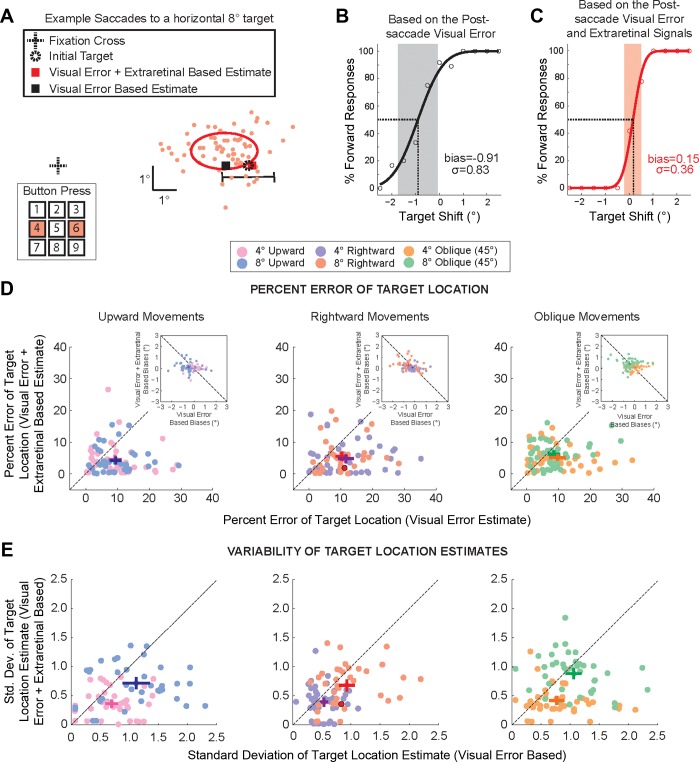
Fig. 5.

Comparison of the postsaccadic target location estimation based solely on postsaccade visual information or with additional extraretinal information. A: eye movements of an example subject for saccades to a rightward target at 8°. Primary saccade end points are displayed as filled red circles. The initial target is marked by a dashed circle, and the solid ellipse represents the spatial extent of the 1 SD confidence interval of saccade end-point variability. The black horizontal line marks the range (±2.5° of displacement) of the target shift. Subjects were required to report the direction of the perceived target shift by pressing numbers on a keypad, in this example either the 4 or the 6 for the horizontal displacement direction. Red and black squares represent the perceptual (Visual Error + Extraretinal based) and Visual Error-based estimates of the target location, respectively. B and C: Visual Error-based (B) and Visual Error + Extraretinal-based (C) psychometric functions of perceived target displacement. Cumulative Gaussian distributions are plotted for both Visual Error + Extraretinal-based and Visual Error-based judgments of shift direction. Shaded regions represent the respective 1 SD range, and dashed lines indicate the perceptual bias; the corresponding values are given in each plot. D: individual data points (light filled circles) and average (dark filled symbols) % errors of target location (Visual Error + Extraretinal- vs. Visual Error-based estimates) are plotted for each target amplitude and direction (rightward 4°, purple; rightward 8°, red; oblique 4°, orange; oblique 8°, green; upward 4°, pink; upward 8°, blue). Crosses represent respective means and SE across subjects. Insets compare the respective Visual Error + Extraretinal-based and Visual Error-based bias measures. E: SD of target location estimates. Individual data points (light filled circles) and average (dark filled symbols) SDs of target location (Visual Error + Extraretinal- vs. Visual Error-based estimates) are plotted for each amplitude and direction. Crosses represent means and SE across subjects. Data for the example subject in A–C are represented by the red filled circle in D and E, center.

Rather than the threshold, we based the confidence of the postsaccadic estimate of the presaccadic target (the bias) on 1 SD of the fitted cumulative Gaussian distribution. We used the threshold measurement to determine the visual displacement required to detect a change in the presaccadic target location and to stay consistent with our previous studies (Bansal et al. 2015; Joiner et al. 2013a). However, we quantified the confidence of the postsaccadic target location estimate with 1 SD rather than the threshold measure because in this case we were interested in quantifying the variability of this estimate rather than the amount of shift required to detect displacement. A relatively smaller SD represents more confidence in the estimation of the presaccadic target position (see Fig. 5, B, C, and E). Taken another way, this comparison of the respective SDs contrasts the noise associated with the eye movements (variability that largely influenced the width of the Visual Error-based psychometric curves) to the variability in the actual perception. For an accurate comparison between the Visual Error and Visual Error + Extraretinal analyses we only compared the data (the mean and SD of the fitted psychometric functions) from psychometric curves that were not significantly different from a normal distribution (Jarque-Bera test, P > 0.05). For each subject and experimental condition (saccade direction, amplitude, and target displacement direction) we applied this test separately to the Visual Error and the Visual Error + Extraretinal data. On the basis of this analysis 8% (23 of 288 total cases) of the Visual Error-based data (and subsequently the corresponding Visual Error + Extraretinal-based data) was removed from the analysis in Fig. 5. The removal of these data did not have any quantitative effects on the analysis or conclusions.

We determined the percent error of the estimated postsaccadic target location for all movement amplitudes and directions (see Fig. 5D). The percent error was the ratio (scaled by 100) between the absolute value of the perceptual bias and the initial target amplitude (examples shown in Fig. 1D). For example, a perceptual bias of 0.4° for a 4° target location is a 10% error in target location. A lower percent error indicates a more accurate postsaccadic judgment of the presaccadic target location. Note that the comparisons in Fig. 5 were made within an experimental session. That is, the Visual Error and Visual Error + Extraretinal conditions were only compared for data obtained within an experimental session in order to minimize the effects of possible differences in eye tracking calibration between sessions.

Statistical analysis.

The experimental group data across subjects were not significantly different from a normal distribution (Jarque-Bera test, P > 0.05). Statistical significance of multiple effects such as target eccentricity (4° or 8°), saccade direction (rightward, oblique, or upward), and target shift direction (horizontal, vertical, or diagonal) on perceptual measures was determined by an ANOVA. For significance, one- or two-tailed t-tests and paired t-tests were used to compare results between conditions. All statistical analyses were performed with MATLAB. For all tests the significance level was 0.05.

RESULTS

Transsaccadic perceptual insensitivity fields.

In this study we investigated the detection of transsaccadic shifts of visual target positions and compared this transsaccadic perception to simulated judgments based purely on the postsaccade visual error resulting from the corresponding saccade variability and the metrics of the target shifts. Perception was assessed for two movement amplitudes (4° and 8°) and three movement directions (rightward, oblique, and upward). The transsaccadic target shift was between ±2.5° in 0.5° increments in eight directions (Fig. 1, C and D): horizontal (0° and 180°), vertical (90° and 270°), diagonal left (135° and 315°), and diagonal right (45° and 225°). We determined the perceptual threshold for detection from derived psychometric curves: cumulative Gaussian functions fit to the percentage of reported forward responses for the target shift range (see methods). The spatial range of perceptual insensitivity (the transsaccadic PIF) was estimated based on the resulting threshold measurements for each direction of the target shift.

The PIFs and saccadic end points for an example subject are presented in Fig. 2A. We selected this subject as a representative of the sample because the majority of this subject's data (thresholds for 20 of the 24 conditions, 83%) was an accurate example of the average behavior. The PIFs are represented as thick solid lines in Fig. 2A, and the end points for individual saccades are presented as filled colored circles for each amplitude and direction; two ellipses representing saccadic movement variability are shown for the 95% confidence intervals (thick dashed lines) and 1 SD (thin dotted lines) for the respective saccade end points for all six conditions. (Note that some of the variability in the saccade end points is due to differences in calibration over the 4 separate experimental sessions.) Consistent with previous reports, saccade end-point variability was significantly greater in the movement direction than in the direction orthogonal to the saccade vector (van Beers 2007; van Opstal and van Gisbergen 1989). This saccade variability also scaled with amplitude (Abrams et al. 1989; Collewijn et al. 1988; de Bie et al. 1987; Leigh and Zee 2006; van Beers 2007), but there was not a significant effect across saccade directions [3-way ANOVA, P < 0.05 for the main effect of variability direction (collinear vs. orthogonal), P < 0.05 for the main effect of saccade amplitude, P = 0.64 for the main effect of saccade direction].

In Fig. 2B we represent the perceptual thresholds for each target shift direction in polar coordinates for each condition (each saccade amplitude and direction). The PIFs and corresponding perceptual thresholds across all subjects are summarized; the three columns show the results for vertical, horizontal and oblique saccades, and the gray and black traces represent the results for saccades to the 4° and 8° targets, respectively. The polar plots in Fig. 2B show the average PIFs as a function of shift direction, and the shaded region represents the SE; the respective thresholds are also depicted by the bar graphs to the right and above each plot. (The schematic above each bar graph depicts the respective direction of the target shift.) First note that the PIFs for saccades to the 8° targets are larger than the PIFs for movements to the 4° targets, following the one-dimensional perceptual threshold results from several previous human studies (Bansal et al. 2015; Li and Matin 1990, 1997; Niemeier et al. 2003, 2007). Second, most PIFs are oriented along the respective movement axis; the thresholds are largest in the direction of the saccade for upward and rightward movements and oblique saccades to the 4° target. These results show that the corresponding perceptual thresholds scaled with saccade amplitude, and that the threshold magnitude was influenced by the direction of the target shift with respect to the movement vector (as demonstrated previously by Niemeier et al. 2003, 2007). In agreement, threshold measurements were significantly different between the two saccade amplitudes and between the different shift directions (2-way ANOVA, P < 0.01 for the main effect of saccade amplitude and P < 0.01 for the main effect of target shift direction).

To determine whether the thresholds (and the corresponding PIFs) were a near-constant percentage of the movement length we normalized each threshold by the respective saccade amplitude (Fig. 2C). We observed that these normalized thresholds were approximately constant for 4° and 8° saccades; the normalized thresholds were not significantly different across saccade amplitudes but were significantly different across movement directions (2-way ANOVA, P = 0.73 for the main effect of saccade amplitude and P < 0.01 for saccade direction). This suggests that the ability to detect a given target shift direction (e.g., a shift in the 90° and 270° direction) was directly influenced by the orientation of the primary saccade (rightward, oblique, or upward). For example, a horizontal target shift was easier to detect (lower normalized threshold) after an upward saccade (orthogonal target shift with respect to the initial saccade vector) than after a rightward saccade (collinear target shift with respect to the initial saccade vector).

To further investigate this relationship between saccade and target shift vectors (i.e., the orientation of the PIFs) we examined the perceptual thresholds and movement variability with respect to the saccade direction. We should note that our aim was not to determine correlations between saccade variability and perception as in Niemeier et al. (2003). Rather, we wanted to determine whether relationships between collinear and orthogonal components of saccade variability and perceptual performance were similar across the different saccade amplitudes and directions tested. In Fig. 3A we plot the perceptual threshold for orthogonal target shifts (with respect to the movement vector) as a function of the respective threshold for collinear target shifts for each subject. Figure 3B shows the same relationship for saccade variability (the orthogonal SD of the saccade end points plotted as a function of the respective collinear SD). Note that both Fig. 3, A and B, show that, overall, collinear thresholds and saccade variability were larger than the respective orthogonal measures for the three saccade directions, and that both the threshold and saccade variability measures scaled with amplitude. Threshold and saccade variability measures were significantly different between movement amplitudes and the two component directions (collinear or orthogonal target shifts and collinear or orthogonal components of the saccade variability), but these measures were not significantly different between the three movement directions [3-way ANOVA, P < 0.05 for main effect of saccade amplitude, P < 0.05 for main effect of direction (collinear or orthogonal), and P > 0.38 for main effect of movement direction, for both perceptual threshold and saccade variability]. To determine whether there was an approximate constant scaling between saccade amplitude and saccade variability and perceptual threshold, we further compared the normalized thresholds and SDs (Fig. 3, C and D). That is, we normalized the data in Fig. 3, A and B, by the respective movement length. These results show that the normalized collinear and orthogonal thresholds were significantly different from each other but were not significantly different across saccade amplitude or direction [3-way ANOVA, P < 0.05 for main effect of target shift direction (collinear or orthogonal), P = 0.31 for saccade amplitude, and P = 0.49 for saccade direction]. Similarly, the normalized collinear SDs were significantly different from the normalized variability orthogonal to the movement vector but were not significantly different across saccade amplitude and direction [3-way ANOVA, P < 0.01 for main effect of variation direction (collinear or orthogonal), P = 0.63 for saccade amplitude, and P = 0.61 for saccade direction].

We did not find a consistent relationship between saccade scatter and perceptual threshold as in Niemeier et al. (2003). The authors previously showed a near-linear relationship between the ratio of saccade scatter (collinear to orthogonal variability) and the ratio of the perceptual thresholds to detect collinear and orthogonal shifts of the target. This inconsistency could be attributed to two differences in the experimental design. First, the saccade amplitudes examined in the present study were ∼25–50% the movement amplitude tested in Niemeier et al. (2003). It is possible that the saccade scatter ratios between the collinear and orthogonal saccade variability for these smaller movement amplitudes were not sufficient to reveal the relationship between perceptual performance and movement variability. For example, in our data the largest ratio in saccade scatter was ∼3 (eye position was 3 times more variable in the direction of the saccade than in the orthogonal direction). This is at the low end of the range of the saccade scatter reported by Niemeier et al. (2.5–4.5). Second, to the best of our knowledge, the study by Niemeier et al. did not blank the target after saccade onset. Blanking the target reduces the saccadic suppression of displacement and correspondingly lowers the perceptual thresholds for both collinear and orthogonal displacements (Deubel et al. 1996; Wexler and Collins 2014). These reduced thresholds resulted in perceptual ratios (>10) that far exceeded the ratios reported in Niemeier et al. (between 2 and 6). Thus differences in our experimental data may prevent the demonstration of the same relationship between perception and movement variability. Nonetheless, our data do show that several characteristics (scaling with saccade amplitude and systematic differences between the collinear and orthogonal directions) are consistent between movement variability and perceptual performance across saccade directions and amplitudes, suggesting a strong link between the two.

The results in Figs. 2 and 3 suggest that the normalized thresholds were similar across movement amplitude and direction but were strongly influenced by the orientation of the shift with respect to the saccade vector. For a direct comparison, Fig. 4 plots the normalized thresholds for orthogonal (90°), oblique (45°), and collinear (0°) target shifts for the three saccade directions (averaged across movement amplitude, thin dashed traces) and the overall normalized threshold across both saccade amplitude and direction (thick black trace). As shown in the figure, the normalized threshold systematically changed with the direction of the target shift. The average threshold for collinear displacements was 8.2 ± 0.5% of the movement amplitude (consistent with previous studies: Li and Matin 1990, 1997; Bansal et al. 2015), while the thresholds for oblique and orthogonal shifts were 6.9 ± 0.4% and 4.8 ± 0.8% of the movement amplitude, respectively. This demonstrates that, independent of saccade direction and amplitude, there was a systematic increase in the perceptual threshold as the target shift became more aligned with the movement vector.

Postsaccadic estimation of presaccadic target location: the benefit of extraretinal information.

Numerous studies have confirmed that the postsaccade visual errors in this displacement detection task do not influence perceptual performance, suggesting that a combination of visual and extraretinal information (provided by the saccade CD and other sources) is used to form the perceptual decision (Bansal et al. 2015; Collins et al. 2009; Deubel 2004; Joiner et al. 2013a; Ostendorf et al. 2010; Ostendorf and Dolan 2015). [Note that the proprioceptive information coming from the eye muscles is unlikely to provide this extraretinal information but cannot be ruled out in the present experiment (Guthrie et al. 1983; Lewis et al. 2001; Poletti et al. 2013; Wang et al. 2007). Thus we refer in general to the benefit of extraretinal information but propose that this information is largely due to a CD signal.] Although these previous studies have shown that trial-to-trial variations in the saccade end point do not correlate with changes in perception of the target shift, this analysis 1) was performed largely for collinear shifts of the saccade target and, importantly, 2) cannot quantify the extent to which the additional extraretinal information possibly assists in forming the accurate perceptual report. Here we were specifically interested in quantifying the extent to which extraretinal information assists accurate perception (postsaccadic estimation of the presaccadic target location) and how the benefit of extraretinal information is affected by the saccade and target displacement metrics (amplitude and direction). To be clear, we did not seek to show that perceptual performance is independent of the saccade variability and the subsequent postsaccade visual error but rather to quantify the capacity extraretinal information improved perceptual performance over the available visual information following the postsaccadic target shift. To directly compare the accuracy and confidence of the combined postsaccade Visual Error and Extraretinal signal-based perceptual performance (Visual Error + Extraretinal for short) to that based solely on the available postsaccade Visual Error information (Visual Error for short) we determined the psychometric curves when the shift direction judgment was dependent on the postsaccadic visual error experienced at target reappearance (see methods). Based on the subsequent psychometric functions we computed the estimate of the presaccadic target location (the bias) and confidence of this estimate (the 1 SD extent of the fitted cumulative Gaussian distribution). These estimates of the target location and confidence of the location estimates were then compared when based only on the postsaccade visual error (Visual Error based; Fig. 5B) or on the actual perceptual judgments (Visual Error + Extraretinal based; Fig. 5C). We determined the bias and SD extent for all 24 conditions (2 saccade amplitudes, 3 saccade directions, and 4 target shift directions) for each subject.

Figure 5A displays the data for an example subject for saccades to the 8° rightward target. In this example the target was displaced along the movement vector (collinear shifts of the target). The filled red circles in Fig. 5A represent the saccade end points, and the solid line ellipse represents the 1 SD confidence interval of the saccade variability. As demonstrated by this example subject, the majority of saccades undershot the target location. The mean saccade gains across subjects for movements to the 4° (8°) targets were 80.8 ± 4.0% (83.6 ± 4.7%), 89.0 ± 2.7% (80.4 ± 3.1%), and 83.8 ± 3.7% (83.5 ± 4.4%) for rightward, upward, and oblique movements, respectively. However, despite regularly producing saccades that undershot the target, subjects typically overestimated the target location (a positive perceptual bias). The mean perceptual biases (PNL) across subjects for movements to the 4° (8°) targets across all saccade directions were 0.08 ± 0.08 (0.19 ± 0.12), 0.03 ± 0.09 (0.02 ± 0.08), and 0.10 ± 0.07 (0.40 ± 0.13) for collinear, orthogonal, and oblique target shifts, respectively. This is consistent with a saccade CD with a gain < 1. That is, based on the saccade CD, subjects expected a greater undershoot than that experienced and subjects perceived the target as stationary (PNL) when there was a small forward shift of the target.

This overestimation of the target location is exemplified by the example subject presented in Fig. 5. In Fig. 5A the red square represents the Visual Error + Extraretinal-based estimate of the presaccadic target location and the black square represents the Visual Error-based estimate. For the same example subject, the Visual Error-based and Visual Error + Extraretinal-based psychometric functions are shown in Fig. 5, B and C, respectively; the shaded regions represent the 1 SD range, and the dashed lines indicate the bias. The corresponding values for each measure are given in each plot. As demonstrated by the example subject, the SD of the Visual Error + Extraretinal-based report was less than the SD of the Visual Error-based (0.36° vs 0.83°) and the Visual Error + Extraretinal-based bias was more accurate than that based on the postsaccade visual errors (0.15° compared to −0.91°). Note that because of the saccade undershoot the majority of the Visual Error-based reports of the target shift are in the forward direction, resulting in a negative bias (underestimation of the target location). Thus the postsaccadic estimate of the presaccadic target would be closer to the mean saccade end point if only reliant on postsaccade visual errors. However, the bias for the Visual Error + Extraretinal-based estimate is positive, indicating an overestimation of the presaccadic target location. In addition, the saccade variability results in a broader psychometric function than the actual perceptual results. That is, reports of the shift based only on postsaccade visual information would provide a noisier and less reliable estimate of the presaccadic target location. We were interested in quantifying the extent to which extraretinal information improved the accuracy and confidence of the location estimate over the use of purely postsaccade visual information.

To directly compare the accuracy of the Visual Error + Extraretinal-based and Visual Error-based postsaccadic estimates of the presaccadic target location we calculated the percent error of the bias measurements (see methods). The actual perceptual bias measures for the Visual Error and Visual Error + Extraretinal conditions are shown in Fig. 5D, insets. For all saccade directions and amplitudes, there was no linear relationship between the Visual Error + Extraretinal-based and Visual Error-based perceptual biases (P > 0.05). We were interested in the magnitude of the estimation error rather than the direction of the respective errors. For example, the percent errors of the estimated target location for the psychometric functions presented in Fig. 5, B and C, were 11.4% (Visual Error based) and 1.9% (Visual Error + Extraretinal based), respectively. The percent error of the target location was calculated from the bias measures of the psychometric functions for each subject and summarized in Fig. 5D. The Visual Error + Extraretinal-based percent error in target location is plotted as a function of the corresponding Visual Error-based percent error. First, the average Visual Error + Extraretinal-based and Visual Error-based percent errors were not significantly different for saccades made to the 4° and 8° target locations (P > 0.05 for all 6 conditions, paired 2-tailed t-test). Second, for all saccades to the 4° and 8° targets, the average Visual Error + Extraretinal-based percent error was significantly less than the corresponding Visual Error-based percent error (P < 0.01 for all 6 cases, paired 2-tailed t-test). For oblique target shifts, the percent error in the perceptual estimate of the target location was 52 ± 7% less for movements to the 4° targets and 36 ± 17% less for saccades to the 8° targets. These respective values were 53 ± 7% less and 41 ± 12% less for collinear displacements to the 4° and 8° targets, 30 ± 30% less and 33 ± 12% less for orthogonal shifts. Note that the percent decrease in the relative percent error measures for all shift directions was significantly greater than 0 (P < 0.05, 1-tailed t-test) except for the orthogonal shift condition for saccades to the 4° targets (P = 0.07). Overall, the percent error of the Visual Error + Extraretinal-based estimate of the target location was ∼50% less than estimation based on the visual error. Specifically, the percent error for the Visual Error + Extraretinal-based biases was 52 ± 7% less than that for the Visual Error-based biases for movements to the 4° targets and 47 ± 10% less for movements to the 8° targets.

We used the extent of the 1 SD range of the cumulative Gaussian distributions (the psychometric functions) as a measure of the confidence in the estimate of the presaccadic target location. The SDs of the respective cumulative Gaussian distributions for each subject are summarized in Fig. 5E. The Visual Error + Extraretinal-based SD is plotted as a function of the corresponding Visual Error-based variability. First, both Visual Error + Extraretinal-based and Visual Error-based variability were significantly greater for saccades made to the 8° targets than those made to the 4° targets for all three saccade directions (upward, rightward, and oblique) (P < 0.05 for all 6 cases, paired 2-tailed t-test). This suggests greater confidence in the overall estimates of the target location for smaller-amplitude saccades. Second, for rightward and upward saccades to the 4° and 8° targets, the Visual Error + Extraretinal-based variability was significantly less than the Visual Error-based variability (P < 0.05 for all cases, paired 2-tailed t-test). This was also true for oblique saccades to the 4° target (P < 0.05, paired 2-tailed t-test). However, we found no significant difference between Visual Error + Extraretinal-based and Visual Error-based variability for oblique saccades to the 8° target (P = 0.11, paired 2-tailed t-test). Across the different movement directions, for oblique target shifts, the perceptual variability was 38 ± 10% less for movements to the 4° targets and 21 ± 17% less for saccades to the 8° targets. These respective values were 30 ± 12% less and 21 ± 14% less for collinear displacements to the 4° and 8° targets and 15 ± 32% less and 9 ± 28% less for orthogonal target shifts. Note that, similar to the above results for the percent error, the percent decreases in the variability for all collinear and oblique target shifts were significantly greater than 0 (P < 0.05, 1-tailed t-test). However, this was not the case for the orthogonal target shifts for both saccade amplitudes (P > 0.05 in both cases). Overall, the variability of the Visual Error + Extraretinal-based estimate of target location was ∼35% less than that based on the visual error. The variability for the Visual Error + Extraretinal-based estimate of target location was 45 ± 20% less than the Visual Error-based variability for movements to the 4° targets and 30 ± 17% less for movements to the 8° targets.

DISCUSSION

Understanding how the metrics of both saccades and visual changes influence perception provides insights into the neural mechanisms and strategies that enable visual stability despite the visual information interruptions and variability that accompany the respective eye movements. In this study, we designed a target shift detection task that examined the perceptual ability to detect transsaccadic target location changes for different movement amplitudes (4° and 8°), directions [upward, oblique (45°), and rightward], and shifts of the target (collinear, orthogonal, or diagonal with respect to the saccade vector). We quantified the spatial range of the resulting transsaccadic PIFs—the perceptual threshold as a function of the target shift direction. Consistent with previous studies that examined one (Bansal et al. 2015; Joiner et al. 2013a; Li and Matin 1990, 1997; Niemeier et al. 2007)- and two (Niemeier et al. 2003; Wexler and Collins 2014)-directional transsaccadic detection, we found that the PIFs 1) scaled with saccade amplitude, 2) were oriented along the movement direction, and 3) were approximately constant in shape when normalized by movement amplitude. The normalized perceptual thresholds changed as a function of the target shift direction but were a near-constant percentage of the movement length across the different saccade directions and amplitudes. In addition, we quantified the accuracy and the amount of variation in the postsaccadic estimation of the presaccadic target location when determined from Visual Error + Extraretinal-based perceptual judgments or the postsaccade visual error (Visual Error based) experienced at the reappearance of the displaced target. We report that the error in the estimation of the target location was on average 50% less and 35% less variable for Visual Error + Extraretinal-based estimates than estimates based purely on visual error information. Consistent with previous studies, our experimental data show that perception is highly accurate despite movement variability. However, we further demonstrate that these perceptual reports are strongly influenced by the direction of the visual change with respect to the movement orientation, suggesting a trade-off between the use of postsaccade visual error and extraretinal information as the shift direction becomes more aligned with the saccade vector. Although it is possible that other sources of extraretinal information may contribute to the quantified perceptual benefit in our task, we focus our discussion on the possible role of the saccade CD.

Perceptual benefit of the saccade CD.

The estimated PIFs from our study exhibited two features similar to the spatial variability of the saccadic eye movements. First, both PIFs and saccade variability scaled in proportion with movement amplitude, in agreement with several previous human and monkey studies (Bansal et al. 2015; Joiner et al. 2013a; Li and Matin 1990, 1997; Niemeier et al. 2003, 2007). Second, both PIFs and saccade variability were significantly greater in magnitude along the movement direction (less sensitive) compared with the orthogonal direction (Fig. 3), as previously reported (Niemeier et al. 2003, 2007; van Beers 2007; van Opstal and van Gisbergen 1989; Wexler and Collins 2014). The combined influence of the saccade and target shift direction on perception is consistent with a CD-based detection of a transsaccadic variation through the comparison of the initial and remapped target location (Jonikaitis et al. 2013; Rolfs et al. 2011; Sperry 1950; Szinte et al. 2015; von Holst and Mittelstaedt 1950). In addition, the shared features between perceptual insensitivity and eye movement variability for different saccade directions suggest that there is a limit to the extent the CD can assist perception of this transsaccadic variation due to the neural variability of the motor commands (Niemeier et al. 2003, 2007). This is similar to neurons in frontal cortex that exhibit a perpendicular tuning bias—better detection of displacements perpendicular to the saccade vector than of displacements parallel to the movement (Crapse and Sommer 2012). This is also supported by the approximately constant relationship reported for perceptual threshold across different saccade amplitudes (Bansal et al. 2015; Li and Matin 1990, 1997; Niemeier et al. 2003, 2007); the perceptual threshold for collinear target shifts is an approximately constant percentage of the movement amplitude, which may reflect the signal-dependent noise previously demonstrated for saccade generation (Goossens and van Opstal 2012). Here we report that this relationship between movement amplitude and perceptual insensitivity was also observed for the different shift directions we tested. The thresholds for collinear, oblique, and orthogonal target shifts across movement directions and amplitudes were 8.2 ± 0.5%, 6.9 ± 0.4%, and 4.8 ± 0.8% of the saccade length, resulting in a near-constant shape in the PIFs when normalized by movement amplitude.

Collectively, these observed systematic changes in perceptual threshold with movement amplitude and target shift direction may reflect the corresponding neural variability associated with the respective motor commands and subsequently different perceptual strategies dependent upon the transsaccadic detection requirements (Niemeier et al. 2003, 2007). For example, the least benefit of the extraretinal information occurred for orthogonal target shifts. The Visual Error + Extraretinal-based estimates of the presaccadic target location and the confidence of this estimation were not significantly different from the measures made based on only the postsaccade visual error information. This suggests a ceiling effect that may be due to the characteristics of the saccade variability (variance along the movement vector compared with the variance orthogonal to the trajectory); it would be difficult to distinguish environmental variations from saccade-induced changes for target displacements in the direction of movement, but less so for orthogonal changes in the target location. That is, because of the lower saccade end-point variability in the orthogonal direction, the perception of the orthogonal shift direction was sufficiently accurate when just reliant on the available visual error information. However, as the target shift aligned with the direction of the saccade, we report that there was an increase in the benefit of the additional extraretinal (likely CD) information for both the 1) accuracy and 2) confidence of the postsaccadic target location estimate (Fig. 5). Thus extraretinal information may play a small role in guiding perception when the motor noise and corresponding visual error are the smallest (orthogonal target shifts) but may provide a greater contribution when these errors are a less reliable indicator of the visual change due to greater noise in the motor commands (collinear target shifts).

Temporal and spatial properties of CD: influence on CD-based neural mechanisms.

The presented behavioral results may relate to the subsequent properties of neural mechanisms that rely on the saccade CD. For example, the experiments of Sommer and Wurtz (2004a, 2004b, 2006) established that the saccade CD plays a role in the anticipatory visual sensitivity demonstrated by frontal eye field (FEF) neurons. FEF neurons that receive CD information of the impending eye movement respond to a peripheral visual stimulus before a saccade brings that visual stimulus into their receptive field (Crapse and Sommer 2012; Joiner et al. 2011, 2013b; Shin and Sommer 2012; Sommer and Wurtz 2004a, 2004b, 2006; Umeno and Goldberg 1997). Importantly, inactivation of the CD pathway from the brain stem, through the thalamus to frontal cortex (delineated by Sommer and Wurtz 2006), reduces this sensitivity. This anticipatory activity has been demonstrated in other visual and visual-motor areas, strengthening the hypothesis that this predictive mechanism underlies the maintenance of stable visual perception across saccades (Duhamel et al. 1992; Nakamura and Colby 2002; Umeno and Goldberg 1997; Walker et al. 1995; see also Zirnsak et al. 2014). Recent behavioral work has aided in inferring the spatial and temporal features of the saccade CD and the implication for this predictive CD-based mechanism (Binda et al. 2009; Cicchini et al. 2013; Collins et al. 2009; Joiner et al. 2010, 2013a; Jonikaitis et al. 2013; Ostendorf et al. 2010; Rolfs et al. 2011; Szinte et al. 2015; Wexler and Collins 2014; Zimmermann et al. 2013a, 2013b). The present results suggest that the spatial extent of this anticipatory visual activity will likely be greater in the direction of movement than orthogonal to the saccade vector. Similar to the work of Crapse and Sommer (2012), it would be of interest to compare this mapping of the predictive visual sensitivity for different saccade metrics (amplitudes and directions) and the effect of CD inactivation on the detection of different transsaccadic target shifts. For example, if postsaccade visual error information is primarily used for detecting orthogonal shifts of the target, one hypothesis is that reducing the saccade CD will not affect perceptual performance in this direction but a deficit will be observed for collinear target shifts. Such physiological investigations may help determine the relationships between the spatial properties of the saccade variability, CD-based anticipatory neural activity, and perceptual sensitivity, as well as the different strategies and extraretinal information used for stable visual perception.

Limitations in understanding natural transsaccadic sensorimotor integration.

The transsaccadic target displacement task utilized in the present study, as introduced by Deubel et al. (1996), provides an effective method for studying extraretinal, and likely CD-mediated, contributions to sensorimotor integration—the combination of the actual and predicted sensory consequences of movement. However, there is considerable evidence that suggests that in normal vision transsaccadic change detection is poor because of saccadic suppression (Deubel et al. 1996, 1998, 2002). Other factors, such as relational information within the environment, likely also assist sensory integration and perceptual stability across saccades (Deubel 2004; Ostendorf and Dolan 2015). For example, when visual reference information is also provided with transsaccadic target changes, perceptual performance is more accurate than when this reference information is absent (Ostendorf and Dolan 2015). It is unlikely that other visual information could be used for the perceptual response in our task because of the lack of any visual information on the screen other than the target and fixation cross. The screen border is also likely not to contribute because the targets (with the maximum displacements taken into account) were positioned far enough so that there was no relative information that could reasonably be used to determine the target shift direction (>11°). However, the limited visual information and target blanking we utilized in the present study is a somewhat artificial condition and may only provide limited information on the various mechanisms that support natural vision. Nonetheless, these types of perceptual paradigms are an effective method for estimating the properties of the movement-related extraretinal signals that contribute to visual stability.

Actual vs. predicted sensory error due to movement.

Theoretical and experimental work has suggested that the brain uses the CD of motor commands with an internal model of the body's forward motions to predict the sensory consequences of executed movements (Kawato 1999; Miall and Wolpert 1996; Wolpert et al. 1995). Based on this framework, CD provides a means to distinguish self-caused sensations from those due to external fluctuation in the environment (Sommer and Wurtz 2008). In this task the accurate perception of the visual scene change is subject to at least two noise sources: that due to the saccadic eye movements that move the fovea and that due to changes in the environment (the amplitude and direction of the actual target shift). Here we directly compared the extent to which the extraretinal information could assist in distinguishing between these internal and external fluctuations. We determined the estimated target location based purely on sensory information (the visual error resulting from the eye movement and displaced target reappearance) and compared this to estimates based on the postsaccade visual error and the possible CD of the saccade (the perceptual reports of the displacement direction made by the subjects). Overall, for target shifts with components in the direction of the saccade, the estimated target location based on the postsaccade visual error and extraretinal information was significantly more accurate and less variable than that based solely on the postsaccade visual error. This analysis of the movement and perceptual data may provide an insightful method to examine deficits in CD; determining the perceptual estimates when only reliant on sensory information provides a behavioral prediction that can be compared to the actual estimates based on the perceptual reports. This framework may provide testable predictions to systematically evaluate visual perceptual deficits in patients with psychiatric disorders purported to have impaired CD transmission (Ford et al. 2001; Ford and Mathalon 2004; Frith 1992; Rösler et al. 2015; Thakkar et al. 2015).

GRANTS

This work was supported by National Eye Institute Grant R00 EY-021252 to W. M. Joiner.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the author(s).

AUTHOR CONTRIBUTIONS

Author contributions: L.C.J.B., S.B., and W.M.J. analyzed data; L.C.J.B. and W.M.J. interpreted results of experiments; L.C.J.B., S.B., and W.M.J. prepared figures; L.C.J.B. and W.M.J. drafted manuscript; L.C.J.B., S.B., and W.M.J. edited and revised manuscript; L.C.J.B., S.B., and W.M.J. approved final version of manuscript; S.B. performed experiments; W.M.J. conception and design of research.