Skip to main content
PMC home page
Nature Communications logoLink to Nature Communications
. 2016 Apr 25;7:11377. doi: 10.1038/ncomms11377

A modular design of molecular qubits to implement universal quantum gates

Jesús Ferrando-Soria 1, Eufemio Moreno Pineda 1, Alessandro Chiesa 2, Antonio Fernandez 1, Samantha A Magee 1, Stefano Carretta 2, Paolo Santini 2, Iñigo J Vitorica-Yrezabal 1, Floriana Tuna 1, Grigore A Timco 1, Eric JL McInnes 1, Richard EP Winpenny 1,a
PMCID: PMC4848482  PMID: 27109358

Abstract

The physical implementation of quantum information processing relies on individual modules—qubits—and operations that modify such modules either individually or in groups—quantum gates. Two examples of gates that entangle pairs of qubits are the controlled NOT-gate (CNOT) gate, which flips the state of one qubit depending on the state of another, and the 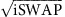Inline graphic gate that brings a two-qubit product state into a superposition involving partially swapping the qubit states. Here we show that through supramolecular chemistry a single simple module, molecular {Cr7Ni} rings, which act as the qubits, can be assembled into structures suitable for either the CNOT or Inline graphic gate by choice of linker, and we characterize these structures by electron spin resonance spectroscopy. We introduce two schemes for implementing such gates with these supramolecular assemblies and perform detailed simulations, based on the measured parameters including decoherence, to demonstrate how the gates would operate.

Inline graphicThe physical implementation of quantum information processing requires individual qubits and entangling gates. Here, the authors demonstrate a modular implementation through chemistry, assembling molecular {Cr7Ni} rings acting as qubits, with supramolecular structures realizing gates by choice of the linker.

The inability of conventional computers to solve certain problems efficiently, such as the simulation of quantum systems1,2, is one of the main driving forces for the implementation of quantum computing and quantum information processing (QIP)3,4, which exploit the laws of quantum mechanics. At the theoretical level, several algorithms have been shown to outperform classical computers in certain computational tasks such as factoring large numbers into primes5 and searching of unsorted directories6. A variety of quantum systems have shown an excellent performance as the basic units for quantum information (qubits)7,8,9,10,11. However, they are difficult to link controllably into useful arrays producing the two-qubit entangling quantum gates (QGs) crucial for any quantum algorithm. Among the most important entangling QGs are the controlled NOT-gate (CNOT) and the 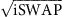Inline graphic gate. The 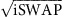Inline graphic gate brings the two-qubit state 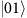Inline graphic to the superposition 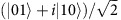Inline graphic. The CNOT gate flips the state of the target qubit if, and only if, the control qubit is in the 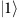Inline graphic state; this implies that each qubit has to respond inequivalently to an external stimulus. All other quantum gates, however complex, can be constructed from these two-qubit gates and single-qubit gates.

Therefore the major challenge for the physical implementation of QIP is bringing together qubits in an organized, scalable and addressable way to make such QGs3,4. Here we demonstrate that supramolecular chemistry could have a major impact in addressing this challenge.

Molecular nanomagnets have been proposed as qubits12,13,14,15,16,17,18,19,20,21,22,23,24,25,26 and coherence times of individual molecular qubits have been studied and have improved24,25. Supramolecular chemistry27 allows us to bring together, with great control, complex molecules into arrays, and recently this has been exploited to tune two-qubit interactions built from molecular nanomagnets28. Hence supramolecular chemistry is a promising tool to build multi-qubit devices; if this could be made scalable this is a competitive route towards the realization of a quantum computer.

Demonstrations of CNOT gates based on molecular nanomagnets have been published18,19,23. The interaction between two dissimilar qubits produces a splitting in the low-lying two-qubit states that allows selective addressing of the transition by means of resonant EPR pulses, while keeping the other components of the wave-function frozen. In this way, the excitation of the target depends on the state of the control qubit and a CNOT gate is implemented. The drawback of this approach is the permanent direct coupling between the qubits, which makes these proposals hard to scale as with a permanent coupling the state of the qubits experiences an unwanted many-body spontaneous evolution in time (as in NMR QIP schemes29), whose harmful effects increase with the number of qubits. Conversely, a switchable indirect coupling between the two qubits would make the register much more easily scalable as when the switch is in the off state the unwanted spontaneous evolution is suppressed.

We have proposed using {Cr7Ni} heterometallic rings as qubits14. They are two-level systems (S=1/2 ground state) that have sufficiently long phase memory times to allow many gate operations before state degradation occurs25, and assemblies of {Cr7Ni} rings have been made which show a permanent coupling between the spins26. Here we report two-qubit assemblies which include switchable links, that allow us to propose a fully modular supramolecular design strategy27,28 towards quantum computation schemes based on either the CNOT or 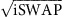Inline graphic gate. The strategy uses supramolecular chemistry to tailor the individual components, spatial configuration and hence the properties of the resulting supramolecules. These supramolecules have been studied by electrochemistry, continuous-wave and pulsed EPR spectroscopy to understand their static and dynamic spin properties. These measured parameters have been used to perform detailed simulations of the performance of both the CNOT and 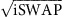Inline graphic gates including the effects of decoherence. The two gates we propose are based on either global or local control of the qubit–qubit interaction. The first proposal exploits uniform magnetic pulses to manipulate two inequivalent Cr7Ni qubits in an asymmetric supramolecule, which would implement the CNOT gate. The second exploits the local electric control of a redox-active linker, which might be addressed by a tip to reversibly switch on and off the qubit–qubit interaction, to implement the 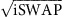Inline graphic gate. The scalability of these approaches is discussed in the last part of the paper.

Results

Syntheses and structural characterization

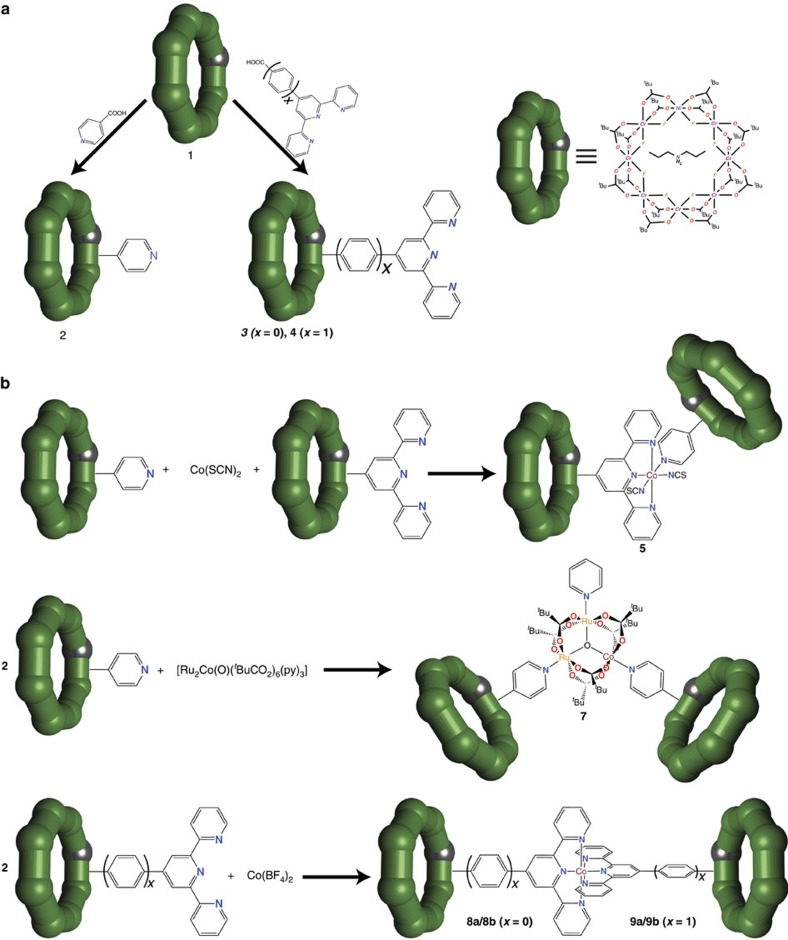
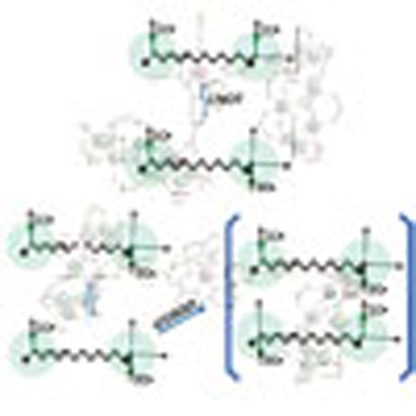
We have earlier reported the selective functionalization of [nPr2NH2][Cr7NiF8(O2CtBu)16] 1 (ref. 30) with iso-nicotinate (O2C-py) to obtain [nPr2NH2][Cr7NiF8(O2CtBu)15(O2C-py)] 2 (ref. 26) (Supplementary Fig. 1a). This synthetic method can be extended to produce [nPr2NH2][Cr7NiF8(O2CtBu)15(O2C-terpy)] 3 and [nPr2NH2][Cr7NiF8(O2CtBu)15(O2C-Ph-terpy)] 4 (Supplementary Fig. 1b,c), from the controlled reaction of 1 with 4-carboxy-2,2′:6′,2″-terpyridine (O2C-terpy) and 4′-(4-carboxyphenyl)-2,2′:6′,2″-terpyridine (O2C-Ph-terpy), respectively (full experimental details are given in the Supplementary Methods)31. 2, 3 and 4 are hereafter abbreviated as {Cr7Ni-O2C-py}, {Cr7Ni-O2C-terpy} and {Cr7Ni-O2C-Ph-terpy}, respectively. They consist of CrIII7NiII rings, containing an octagon of metal centres, with the inner rim bridged by fluoride ions and the outer rim by carboxylates: the functionalized O2C-py 2, O2C-terpy 3 and O2C-Ph-terpy 4 ligands sit on a Cr…Ni edge of the octagon. This provides us with three supramolecular qubits with two different denticities, which allow us to assemble different QGs by appropriate choice of the central node (Fig. 1).

Figure 1. Supramolecular design strategy for the construction of two-qubits assemblies.

Figure 1

Open in a new tab

(a) Synthesis of functionalized {Cr7Ni} rings (where x=0 and 1). (b) Linkage of {Cr7Ni} coordination cages to different central nodes.

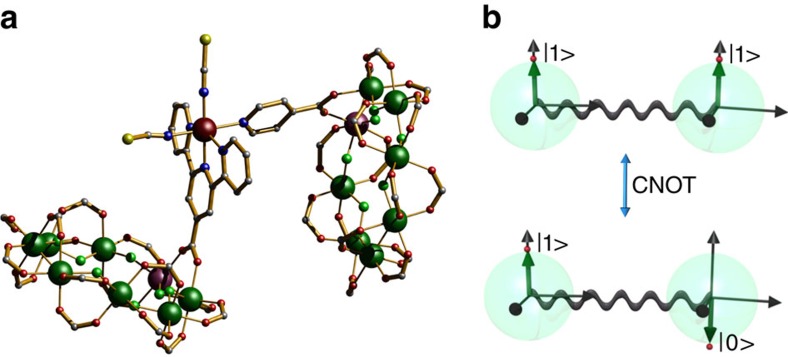
Reaction of equimolar quantities of 2 and 3 with cobalt(II) thiocyanate in a mixture of Et2O/acetone leads to [{Cr7Ni-O2C-py}→Co(SCN)2←{Cr7Ni-O2C-terpy}] 5 in good yield, which has been characterized by X-ray single crystal diffraction (Fig. 2a; for all crystallographic information see Supplementary Data 1). Compound 5 contains two inequivalent Cr7Ni qubits coordinated to a central cobalt(II) ion, which has a six-coordinate CoN6 octahedral environment with a cis-arrangement of the two thiocyanate N atoms. The Co-N bond distances are typical of high spin cobalt(II) ions32, with the bonds to the thiocyanate ligands shorter (average 2.056(12) Å) than those to terpy or pyridine N-donors (2.137(6) to 2.224(4)  Å). Hence the d7 CoII site (SCo=3/2) has a 4T1g ground term (using Oh symmetry labels)33, which leads to a well-isolated effective Seff=1/2 ground state at low temperature (see Characterization of assemblies via EPR spectroscopy below). The cis coordination geometry at the CoII node means the {Cr7Ni-O2C-terpy}- and {Cr7Ni-O2C-py}-based qubits are arranged in an almost orthogonal orientation. Therefore the two qubits in 5 are symmetry inequivalent, as required for implementing a CNOT gate (Fig. 2b).

Figure 2. Molecular structure of the asymmetric two-qubit assembly for proposed implementation of a CNOT gate.

Figure 2

Open in a new tab

(a) Molecular structure of [{Cr7Ni-O2C-py}→Co(SCN)2←{Cr7Ni-O2C-terpy}] 5. nPr2NH2+ cations are not shown (H atoms and tert-butyl groups are omitted for clarity). Colour code: Co, dark red; Cr, green; Ni, purple; Ru, brown; N, cyan; O, red; S, yellow; C, grey; F, pale green. 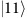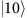(b) Schematic representation of the effect of the CNOT gate on a pair of qubits, initialized in the computational basis states Inline graphic and Inline graphic, respectively. 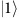The CNOT flips the target qubit if the control is set to Inline graphic.

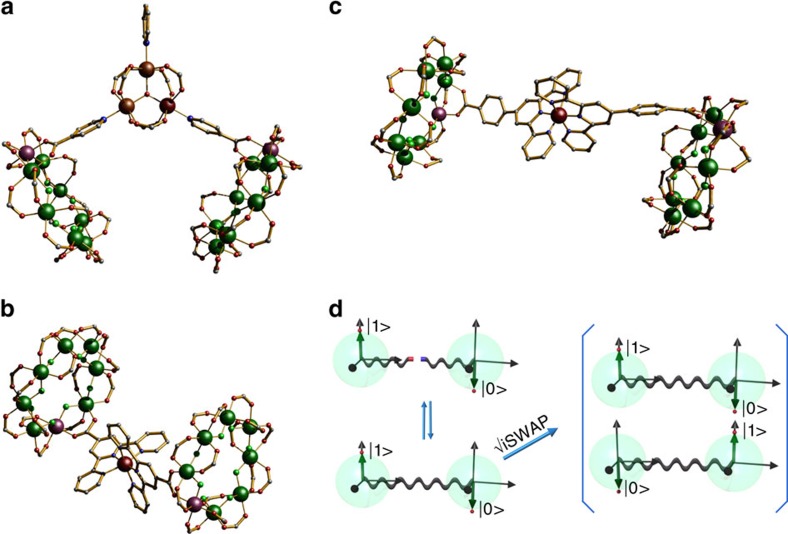
Reaction of two equivalents of 2 with a preformed oxo-centred pivalate-bridged triangular cluster with terminal pyridine groups [RuIII2CoIIO(O2CtBu)6(py)3] 6, hereafter abbreviated as [RuIII2CoII], in acetone gives [{Cr7Ni-O2C-py}→[RuIII2CoIIO(tBuCO2)6(py)]←{Cr7Ni-O2C-py}] 7 (Fig. 3a), where two of the terminal pyridine ligands of 6 were replaced by the iso-nicotinate group of 2. Reaction of two equivalents of 3 or 4 and either cobalt(II) perchlorate or tetrafluoroborate gives [{Cr7Ni-O2C-terpy}→Co←{Cr7Ni-O2C-terpy}][X]2 [X=ClO4− 8a or BF4− 8b] and [{Cr7Ni-O2C-Ph-terpy}→Co←{Cr7Ni-O2C-Ph-terpy}][X]2 [X=ClO4− 9a or BF4− 9b] (Fig. 3b,c) (full experimental details are given in the Supplementary Methods). The architecture in 7, 8 and 9 contains two equivalent qubits, separated by a redox-switchable centre34,35, which makes it suitable for implementation of a ✓iSWAP gate (Fig. 3d).

Figure 3. Molecular structures of the redox-active two-qubit assemblies for proposed implementation of an ✓iSWAP gate.

Figure 3

Open in a new tab

(a) [{Cr7Ni-O2C-py}→[RuIII2CoIIO(tBuCO2)6(py)]←{Cr7Ni-O2C-py}] 7. (b) the cation of [{Cr7Ni-O2C-terpy}→Co←{Cr7Ni-O2C-terpy}][ClO4]2 8a. (c) the cation of [{Cr7Ni-O2C-Ph-terpy}→Co←{Cr7Ni-O2C-Ph-terpy}][ClO4]2 9a. nPr2NH2+ cations and ClO4− anions are not shown (H atoms and tert-butyl groups are omitted for clarity). Colour codes as Fig. 2. (d) Schematic representation of the effect of the ✓iSWAP gate on a pair of qubits, initialized in the computational basis state 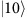Inline graphic. The gate brings 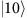Inline graphic to the equal-weight superposition Inline graphic. In the scheme proposed below, it operates as soon as the inter-qubit interaction is turned on (double arrow).

The crystal structure of 7 consists of two {Cr7Ni-O2C-py} rings linked through the iso-nicotinate groups to a [RuIII2CoIIO(tBuCO2)6(py)] triangle (Fig. 3a)34,36. The metal ions are statistically disordered over the three sites within the triangular M3O unit. They have a six-coordinate, octahedral MO5N environment formed by four carboxylate oxygen atoms from the bridging pivalate ligands in the equatorial plane, with the central oxide and one nitrogen atom from iso-nicotinate or pyridine groups occupying the axial positions. The three metal ions are positioned at the corners of an isosceles triangle with two distinct intermetallic distances of 3.296 and 3.315 Å. The central oxide lies within the M3 plane of the metal atoms, while the six pivalate bridging ligands lie above and below the M3O plane and the pyridine N-donors rest perpendicular to this plane.

The crystal structure of 8a and 9a are made up of cationic [{Cr7Ni-O2C-terpy}CoII{Cr7Ni-O2C-terpy}] and [{Cr7Ni-O2C-Ph-terpy}CoII{Cr7Ni-O2C-Ph-terpy}] units together with counterbalancing ClO4− anions (Fig. 3b,c). Both compounds have a central cobalt(II) ion with a six-coordinate CoN6 octahedral environment, with the six Co-N bonds in the range expected for a low spin cobalt(II) ion37. The average value of the two N-donors from the central pyridines of the terpy are shorter (1.923(6) 8a and 1.920(8) 9a Å) than the other Co-N contacts (2.118(7) 8a and 1.986(9) 9a Å). This feature leads to an axially compressed octahedral environment for the low-spin d7 CoII ions giving a SCo=1/2 ground state. Compounds 8a and 9a differ in the shortest Co…M(ring) contact (8.675(2) 8a and 10.979(5) 9a Å) and the staggered 8a or eclipsed 9a arrangement of rings in the assemblies.

The two Cr7Ni qubits in each of 7–9 are linked by a redox-switchable central node34,38. Cyclic voltammetry on 7–9, and on the central nodes in isolation, show a one-electron reversible oxidation in each case (measured in CH2Cl2, 0.1 M nBu4NPF6). For 6 and 7 the half-wave potential (E1/2)=−0.31 (6), −0.35 (7) V versus Fc+/Fc (Fc=ferrocene)39, and this oxidation is assigned to the [RuIII2CoII] to [RuIII,IV2CoII] couple34. For 8b, 9b and reference complexes [Co(HO2C-terpy)2][BF4]2 10 and [Co(HO2C-Ph-terpy)2][BF4]2 11, E1/2=−0.30 (8b), −0.27 (9b), −0.27 (10) and −0.24 (11) V, which are assigned to the CoII/III couple. The anodic to cathodic peak separation values are similar to those of ferrocene under the same conditions (see Electrochemistry section in Supplementary Methods, Supplementary Fig. 4 and Supplementary Table 3).

Characterization of assemblies via EPR spectroscopy

To study the interactions between the molecular components in the supramolecular structures 5, 7, 8b and 9b we performed magnetometry and multi-frequency continuous wave EPR studies. The measured magnetometry data are essentially the sum of the Cr7Ni qubits and the linking nodes (Supplementary Figs 5–9), and therefore are uninformative other than confirming that the interactions are very weak. EPR spectroscopy is much more sensitive to such weak interactions.

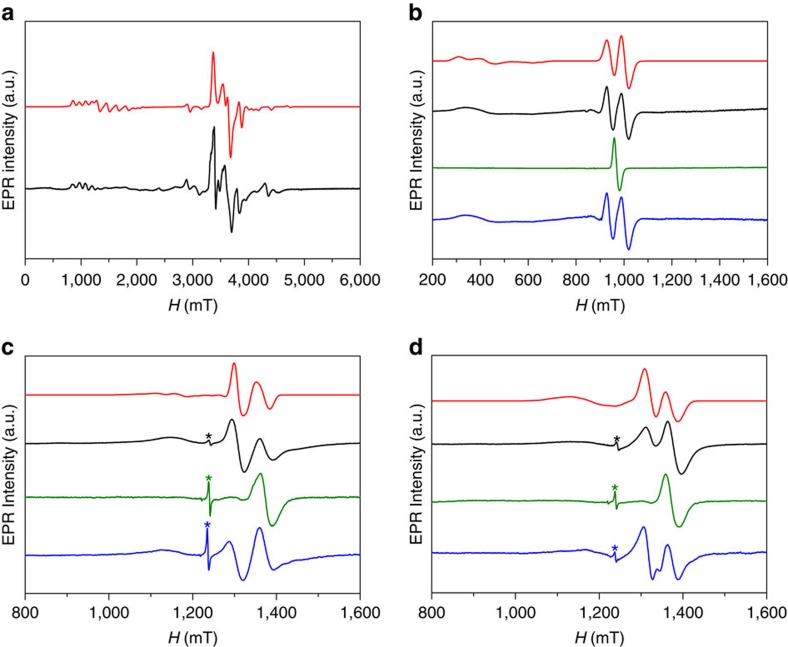
The EPR spectra of a powder of 5 at 5K (Fig. 4a and Supplementary Fig. 10) have a complex multiplet structure because the Seff=1/2 of the Co(II) node has a very anisotropic effective g-tensor and also gives rise to very anisotropic exchange tensors (J) with the {Cr7Ni} rings. This structure is best resolved at W-band (94 GHz; Fig. 4a). The spectra can be simulated40 with the effective three-spin Hamiltonian (1), with two different anisotropic exchange interactions (J12 and J23) between the Seff=½ of the Co(II) ion (S2) and the two S=½ of {Cr7Ni-O2C-terpy} (S1) and {Cr7Ni-O2C-py} (S3) rings, respectively (Fig. 4a).

Figure 4. EPR spectroscopy of QG assemblies.

Figure 4

Open in a new tab

(a) Experimental powder W-band (94 GHz) EPR spectrum of 5 at 5K (black) and simulation (red) using Hamiltonian (1) and parameters in Table 1. (b) Experimental K-band (24 GHz) EPR spectra of 7 frozen solution (black), and simulation (red) using Hamiltonian (2) and parameters given in the text: experimental spectra after oxidation of 7 to 7box with [FeCp2](PF6) (ca. 3 mM, 1:1) (green), and after reducing 7box with cobaltocene (3 mM, 1:1) (blue). (c,d) Experimental Q-band (34 GHz) EPR spectra of 8b and 9b, respectively, in frozen solution (black), and simulations (red) using Hamiltonian (2) and parameters given in the text; experimental spectra after oxidation to 8box and 9box with AgBF4 (ca. 3 mM, 1:1) (green), and after reducing using with cobaltocene (3 mM, 1:1) (blue). The sharp peak marked * is a trace radical impurity.

graphic file with name ncomms11377-m11.jpg

The multiplets centred at ca. 1, 1.5 and 3.5 T (Fig. 4a) mark the effective g values of the Co(II) ion as g=6.50, 4.25 and ca. 2, while the Cr7Ni rings have well defined g=1.78, 1.78 and 1.74 (the unique value being for the orientation perpendicular to the ring)30. The large difference (Δg) between the g=6.5 and 4.3 orientations of the Co(II) ion and those of the rings, and the weak exchange interactions 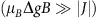Inline graphic is useful as it allows us to treat the problem as an ABX spin system. The multiplet structure of the g=6.5 and 4.3 features is then due to weak exchange with two different S=1/2, giving doublets-of-doublets from which we can read the Jy and Jz components of J12 and J23. The high field region is more complicated, due to the much smaller difference between the third Co(II) g value and those of the {Cr7Ni} rings. The remaining parameters (the final components of J12, J23 and g2) were obtained by simulation (Fig. 4a). The parameters in Table 1 are labelled to a common reference frame, that is, taking into account the orthogonal orientation of the two {Cr7Ni} rings.

Table 1. Spin-Hamiltonian parameters used for simulation of the W-band EPR spectra of 5.

  gx gy gz   Jx (cm−1) Jy (cm−1) Jz (cm−1)
{Cr7Ni-O2C-terpy} 1.78 1.78 1.74 J12 −0.14 0.34 0.17
{Cr7Ni-O2C-py} 1.74 1.78 1.78 J23 −0.07 0.17 0.34
Co(II) 1.78 4.25 6.50        
Open in a new tab

J12, J23 and g={gx,gy,gz} are diagonal tensors.

7, 8b and 9b, contain redox-active linking nodes and their EPR spectra change with the oxidation state. Hence, EPR was performed on both the reduced and oxidized forms at 5K as frozen solutions, allowing cycling of the redox state (Fig. 4b–d). Spectra were measured on the as prepared samples, then solutions were warmed to room temperature and the oxidized forms 7ox, 8box and 9box generated in situ by addition of [FeCp2](PF6) or AgBF4 followed by freezing and measurement. To complete the cycle, the solutions were thawed again and 7, 8b and 9b regenerated by reduction with cobaltocene. We have also measured spectra of the isolated nodes in the paramagnetic Co(II) form, viz. complex 6, and [Co(HO2C-terpy)2](BF4)2 10 and [Co(HO2C-Ph-terpy)2](BF4)2 11 (Supplementary Figs 2 and 3). Like 5, 6 also contains a 4T high-spin Co(II) ion with an Seff=1/2 ground state, giving g=5.61, 4.05, 2.77 (Supplementary Fig. 11), while 10 and 11 have low-spin Co(II) hence S=1/2, giving g=2.047, 2.076, 2.195 (Supplementary Fig. 12) and g=2.022, 2.111 and 2.215 (Supplementary Fig. 13), respectively.

For the supramolecular structures 7, 8b and 9b, the exchange coupling is nicely resolved in K- and Q-band (24 and 34 GHz) EPR spectra (Fig. 4b–d). In each case there is weak coupling with respect to the difference in Zeeman energies Inline graphic41. This, and the equivalence of the two Cr7Ni rings, gives AB2 spin systems. The {Cr7Ni} ring resonances (the ‘B' spins) are split into doublets (Fig. 4b–d), giving a direct measure of J (the exchange splittings of the central node resonances are not resolved). The spectra can be simulated with the simple Hamiltonian (2) using an isotropic exchange interaction between the central nodes (S2) and the two {Cr7Ni} rings (S1 and S3), even for 7, which has an effective spin 1/2 centre at the node.

graphic file with name ncomms11377-m14.jpg

Simulations with the isotropic exchange interaction J as the only variable gives J=−0.026, −0.026 and −0.024 cm−1 for 7, 8b and 9b, respectively; the g values were fixed to those measured for individual components. The EPR spectra of 7ox, 8box and 9box are very simple, resembling isolated {Cr7Ni} rings, because the oxidized forms of the central nodes are diamagnetic. Reduction of 7ox, 8box and 9box back to 7, 8b and 9b regenerates the original spectrum.

Implementing interesting quantum algorithms requires qubits with long phase memory times, such that they can be manipulated many times without errors. Individual {Cr7Ni} heterometallic rings and simpler paramagnetic centres have shown long enough phase memory times (TM)21,22,24,25 to perform coherent electron spin manipulations before state degradation occurs. To check that this key property is preserved when they are incorporated in the supramolecular assemblies, we have performed pulsed EPR measurements at resonances corresponding to both the central nodes and the {Cr7Ni} rings (Supplementary Figs 14–26). For the latter we find similar values for all compounds, and in both oxidation states where relevant (TM=683, 749, 767, 790, 984, 750 and 1,031 ns for 5, 7, 7ox, 8b, 8box, 9b and 9box, respectively, measured at Q-band and 3K; Supplementary Table 4), demonstrating that the phase memory times are not strongly influenced by structural or magnetic differences in the linkage of the rings. In addition, values of TM measured at resonances fields corresponding to the central node (in the paramagnetic state) are also similar for all compounds (500–700 ns at Q-band and 3K; Supplementary Tables 5–12). Hence, the phase memory time of the central node does not represent a limitation (see also the Discussion below) for the applicability of our schemes for implementing a universal set of quantum gates. These results are used in the simulations below, and are extremely promising, as these TM times are sufficiently long for spin manipulation even when they are integrated in a supramolecular assembly.

CNOT gate with uniform magnetic pulses

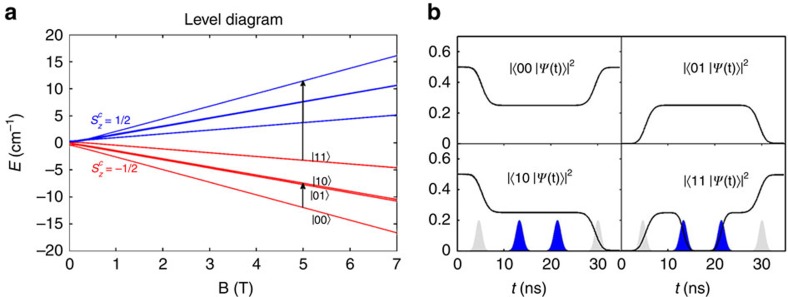
In the following, we introduce two quantum computation schemes, based on either local or non-local control of the inter-qubit interaction employing the structures described and the parameters obtained by EPR spectroscopy. First, we show that compound 5 is suitable for a CNOT gate, using uniform magnetic pulses as the only manipulation tool42. As we are treating three interacting inequivalent doublets this produces 23 energy levels. We define the computational basis within the low-energy subspace where CoII is frozen into its Sz=−1/2 state, which corresponds to the four lowest levels shown in red in Fig. 5a. The four levels correspond to arrangements of the spins of the two qubits, S1 and S3, having the relative orientations |↓↓>, |↓↑>, |↑↓> and |↑↑>, respectively, which we label as |00>, |01>, |10> and |11> in Fig. 5a.

Figure 5. A CNOT gate based on the structure of 5.

Figure 5

Open in a new tab

(a) Field-dependence of the energy levels of 5 resulting from the Hamiltonian (1). The low-energy group of levels (red), where Co is frozen into its Sz=−1/2 state, defines the computational basis. The high-energy group of levels, where the Co spin is inverted, is exploited to perform two-qubit gates. (b) Simulation of the pulse sequence implementing CNOT as Ry(π/2)CZ Ry(−π/2), where Ry(α) is a rotation of the target qubit by an angle α around the y axis and CZ is a controlled-Z gate. We illustrate the gate by starting at time t=0 with a superposition state 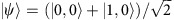Inline graphic, which transforms under a CNOT gate (with the left qubit acting as control) into Inline graphic. The latter state is actually obtained by the pulse sequence implementing Ry(π/2)CZRy(−π/2), with a fidelity of 99.7%. The envelope of the pulses implementing the two Ry rotations and the CZ are outlined at the bottom. Note that performing the CZ gate (two central pulses) requires temporarily leaving the computational subspace. The intensity of the oscillating field at the pulse maximum is 50 G, and we assume a static field of 5 T directed along z.

In a field of a few Teslas the eigenstates are factorized, that is, the eigenfunctions of the rings and the CoII are not entangled. Hence it is possible to implement high-fidelity single-qubit rotations by EPR pulses resonant with low-energy gaps (see, for example, the shorter arrow in Fig. 5a). The combination of the inequivalent and anisotropic ring-Co exchange interaction and of the perpendicular arrangement of the two rings makes the two qubits significantly inequivalent. In particular, there is a sizeable gap (about 33 μeV in a field of 5 T) between the |00>→|01> and |00>→|10> transitions, which enables independent single-qubit rotations. We note that a distribution of the spin Hamiltonian parameters arising from a reasonable g or J strain (∼1%, see ref. 43) would yield a broadening of the energy levels with s.d. ∼5 μeV, thus keeping the two transitions distinguishable in realistic conditions, possibly with the help of narrow and/or composite pulses44,45.

A controlled phase-shift (Cφ) gate is obtained by a pulse resonant with the transition corresponding to the longer arrow in Fig. 5a where the cobalt(II) ion is temporarily in its Sz=+1/2 state (blue energy levels), followed by a repetition of the same pulse that would bring the state back with an additional phase φ. The value of φ is controlled by the phase difference between the first and the second pulse. For a pulse with field along the y direction, the implementation of this gate is fast, owing to the large value of gy for CoII ion. Moreover, the relatively large exchange interaction in equation (1) allows us to employ large oscillating fields (50 G), since the desired transition is spectroscopically well resolved from all the others. Consequently, the Cφ gate can be performed in only about 12 ns, with fidelities close to 99.99 % (see Computational Details section in Supplementary Methods).

The CNOT gate can then be obtained by the sequence of gates Ry(π/2) CZ Ry(−π/2), where Ry(α) is a rotation of the target Cr7Ni qubit by an angle α around the y axis (a single-qubit operation) and where CZ is the phase-shift gate described above with φ=π. We have numerically solved the time-dependent Schrödinger equation for the Hamiltonian (1) in presence of this pulse sequence. Results are reported in Fig. 5b and show that this two-qubit gate can be obtained with very high fidelity in only 30 ns. As noted above, the two qubits are significantly inequivalent, even if the g tensor of the rings is nearly isotropic. This makes this complex well suited for the quantum simulation of antisymmetric Hamiltonians (Supplementary Figs 28 and 29 for an illustrative example).

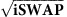Inline graphic gate with local electric control

The redox properties of the central node in 7, 8 and 9 can be exploited to perform the universal 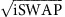Inline graphic gate on the {Cr7Ni} qubits, whose effect on the computational basis is given by:

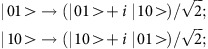
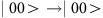Inline graphic

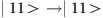Inline graphic and Inline graphic.

When the central node is in the diamagnetic state ([RuIII,IV2CoII] 7 or CoIII 8–9), the two qubits are decoupled and only single-qubit operations can be performed. Conversely, when the paramagnetic oxidation state, [RuIII2CoII] 7 or CoII 8–9, is present the system behaves as a trimer described by the Hamiltonian (2). Previously, Lehmann et al.16 have proposed that two spins S=1/2 connected by a redox-active unit can be exploited for the implementation of the 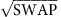Inline graphic gate by switching the redox unit with a scanning tunnelling microscope (STM) tip at an appropriate potential. In this way one electron can be added or removed from the redox unit very quickly. It was demonstrated that particular sets of parameters of the trimer Hamiltonian lead to a pure 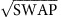Inline graphic evolution of the two qubits after specific time intervals. However, high fidelity for the gate is guaranteed only for fixed ratios between the qubit–qubit exchange (Jqq) and the qubit-redox unit exchange (Jqr). For the measured parameters of 7, 8 and 9 (Jqr≡J and Jqq=0 in equation (2)) the fidelity of the Inline graphic gate would be low.

Here we propose a more flexible scheme for the 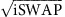Inline graphic gate based on our measured parameters, which works if the two qubits have the same Zeeman energy but one different to that of the switch in the ‘on' state. This is the case here, where the g values of the qubits and switches are very different, g1z=g3z=1.74 for {Cr7Ni} rings and g2z=2.77 (7), 2.195 (8) and 2.215 (9) for the central node. This difference in the g values means that in magnetic fields of a few T, the ring-central node exchange J is small compared with the difference between the Zeeman energies of the ring and the central node. Hence, the spin state of the central node is nearly frozen in the Sz=−1/2 state and has only tiny virtual fluctuations that lead to an effective interaction between the two {Cr7Ni} qubits given by

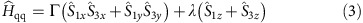graphic file with name ncomms11377-m24.jpg

where the field is along z, S1 and S3 refer to the spins of the first and second Cr7Ni qubits and

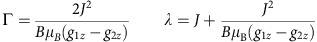graphic file with name ncomms11377-m25.jpg

The feasibility of the scheme relies only on the equivalence of the qubits and on the hierarchy of the interactions 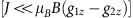Inline graphic (see Supplementary Methods for more details).

Therefore when the central node is paramagnetic, the state of the two qubits evolves according to (3), with negligible entanglement with the central node. For specific times, this evolution coincides with the Inline graphic gate apart from single-qubit rotations along z due to the second term in (3).

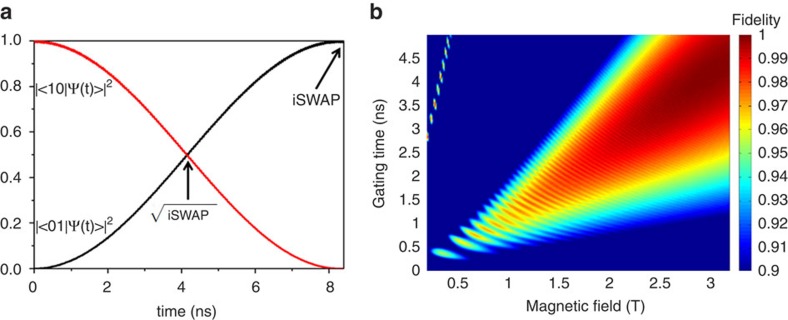
This perturbative picture is confirmed by the results of detailed calculations for compound 9 using the full Hamiltonian (2) (Fig. 6). Starting from the |10> logical state, we report in Fig. 6a the time evolution of the trimer wavefunction that would implement the Inline graphic gate. In a magnetic field of 3 T, after 4 ns the wavefunction has equal-weight contributions from |10> and |01>, which is the Inline graphic gate, while after 8 ns the states of the two spins are exchanged, that is, we have the |01> state. An extremely good fidelity F (larger than 0.99) for compound 9 is obtained for fields of the order of about 2.5 T or larger, after a suitable gating time tf of the order of a few nanoseconds (Fig. 6b). For such fields the perturbative picture of equation (3) holds very well, and tf is proportional to B (Fig. 6b), which is consistent with the form of the effective qubit–qubit coupling Γ∝1/B.

Figure 6. Simulation of the 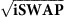Inline graphic gate.

Figure 6

Open in a new tab

(a) For 9, taking |10>≡|1/2,−1/2>⊗|−1/2>Co as the initial state and an applied field of 3 T, we calculate the time-dependence of the oscillation of the trimer wavefunction between |10> and |01>≡|−1/2,1/2> ⊗|−1/2>Co. Other components are negligible. (b) Calculated average fidelity 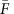Inline graphic for 9 as a function of the magnetic field B and of the gating time, that is, the time the Co switch is in the on state. The fidelity is defined by Inline graphic, where for a given starting logical state, Inline graphic is the final state after an ideal gate, whereas 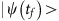Inline graphic is the actual final state. The average has been made over four random starting states. For each value of the field, the optimal gating time tf is the one maximizing 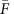Inline graphic ca. 4 ns for 3 T as shown in a. The oscillations corresponding to the fringes in the picture are associated with fluctuations of the Co spin state. As long as the frequency of these fluctuations is much larger than 1/tf these fluctuations are negligible, that is, the perturbative description of equation (3) is valid.

Analogous or better fidelities can be obtained with the parameters derived for compounds 7 and 8. Indeed, larger values of |g1z−g2z| (as in 7) increase the validity of the perturbative picture (3), even for smaller values of B. Hence, we can exploit the modular strategy and optimize the performance of the gates by targeted chemical manipulations.

Discussion

Our initial simulations above did not include decoherence. To gain a deeper insight into the performance of the proposed quantum gates, we have performed further simulations that include the effect of both relaxation and pure dephasing, allowing for the finite and measured values of T1 and TM, respectively. For the values of T1 measured by pulsed EPR (>10 μs in all compounds, see the Supplementary Tables 4–12), the effect of relaxation is found to be completely negligible.

Pure dephasing is accounted for by numerically solving the Lindblad master equation for the system density matrix ρ (refs 46, 47):

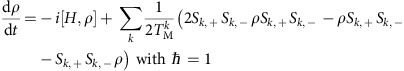graphic file with name ncomms11377-m30.jpg

Here the commutator describes the coherent evolution induced by the full system Hamiltonian H, while the second term describes pure dephasing mechanisms. The subscript k=1, 2 and 3 labels the spins of the qubits and of the switch, while 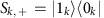Inline graphic and Inline graphic are spin 1/2 raising and lowering operators.

Using the values of TM of the Cr7Ni qubits measured by pulsed-EPR spectroscopy (between 700 and 1,000 ns, see above) we still find very high fidelities: 99.3% for the implementation of the CNOT gate on compound 5 and 99.6% for the 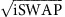Inline graphic gate on 9. As expected, the fidelities are only marginally affected by decoherence because gating times are much shorter than TM.

It is also worth discussing the role of the decoherence of the switch in the present schemes. As far as the CNOT gate scheme (compound 5) is concerned, the contribution of the central Co switch to decoherence is similar to that of the rings, because the switch is temporarily excited to the SZ=+1/2 state during the CNOT gates. 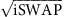Conversely, only virtual excitations of the switch are involved in the implementation of the Inline graphic gate exploiting the redox-active linker. Hence, the fidelity remains very high even for dephasing time of the switch of the order of the gating time (some ns). This difference is shown by the two colour maps reported in Fig. 7. They show the dependence of the fidelity of a Inline graphic (left) and of a CNOT gate (right) on the dephasing times TM of the rings and of the switch. It is evident that the fidelity of the 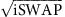Inline graphic is practically independent on 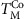Inline graphic whereas its dependence on 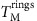Inline graphic is much more pronounced. Conversely, the implementation of the CNOT on compound 5 leads to a similar dependence of the fidelity on Inline graphic and Inline graphic.

Figure 7. Effect of decoherence on quantum gates.

Figure 7

Open in a new tab

(a) Fidelity of the 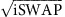Inline graphic as a function of the dephasing times of the rings and of the switch. Remarkably, the fidelity is nearly independent on the Inline graphic

The chemistry described above can be extended to make one-dimensional (1D) chains incorporating the two-Cr7Ni supramolecules, linked by either single cobalt sites or oxo-centred metal triangles. Such 1D chains have already been made involving single Cr7Ni units and [2]- and [3]-rotaxanes and the principles established, especially for 1D chains of rotaxanes48, should work well. The key steps are to include two functional groups per Cr7Ni ring, which has already been done for iso-nicotinate acid, and by functionalizing the termini of the central organic thread of rotaxanes. Inclusion of two different functionalities is more taxing but entirely feasible (see the detailed schemes in Supplementary Figs 30 and 31). Therefore the chemistry is potentially scalable.

While this challenging chemistry proceeds, it is possible to show that the schemes are theoretically scalable. The extension of a two-qubit QG to a multi-qubit register raises important issues concerning the propagation of errors. In such a register, we can identify two main sources of errors, whose effect increases with the number of qubits: pure dephasing and imperfect operation of the switch arising from a residual inter-qubit interaction still present in the ‘off' state.

Errors induced by pure dephasing (decoherence) increase with the overall computational time. However, a finite chain of qubits with interposed switches would allow simultaneous (parallel) manipulation of non-overlapping parts of the register, which drastically reduces the computation time, and hence decoherence-induced errors, with respect to a serial implementation. For instance, a setup based on compound 5 can be manipulated in parallel for interesting classes of quantum simulation algorithms17. This requires inclusion of two distinct switches in the structure—SwitchA and SwitchB—with different responses to external stimuli, such that the 1D chain has as a repeat pattern -Qubit-SwitchA-Qubit-SwitchB-.

For compounds 7, 8 and 9, the scheme requires a local addressability of individual switches on the chain, which are separated by about 3 nm. For a first proof-of-principle experiment with a single assembly, an STM tip could be used to provide the best control. A parallel implementation of gates would be possible if different Co switches could be addressed individually at the same time. To achieve a scalable structure, a molecular chain of qubits might be layered onto a surface and addressed by means of a cross-bar architecture similar to that proposed in refs 16, 49. It is worth noting that Cr7Ni rings have already been deposited on surfaces, without significant modification of their magnetic properties50.

We now examine the scaling of the errors with the number of qubits in the register. As a first step, we consider the effect of pure dephasing on a set of non-interacting qubits subject to Lindblad (Markovian) dynamics. It can be shown (see ref. 51 and Supplementary Information) that the decoherence error ɛ=1−F2 on N qubits scales at most as 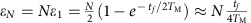Inline graphic, valid for tf<<TM (where tf is the optimal gating time). In the parallel quantum-simulator implementation considered in ref. 17, manipulating a chain of N qubits takes the same time as manipulating the shortest chain that contains the two distinct switches (qubit-SwitchA-qubit-SwitchB-qubit). Therefore tf is limited by the value it assumes for a chain of three qubits, while in a serial scheme tf increases linearly with N. By chemical engineering of Cr7Ni qubits, a TM of 15 μs has been obtained25; this should allow the implementation of around twenty 2-qubit gate operations on a chain consisting of 10 qubits, while keeping the error below 0.1.

Finally, we analyse the consequences of an imperfect operation of the switch and their effect on scalability. For an ideal 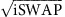Inline graphic gate the inter-qubit exchange interaction would be completely turned off when the central node is in its diamagnetic state. Double electron–electron resonance measurements (Supplementary Fig. 27) reveal a very weak, residual interaction between the qubits. The resulting oscillations are on a time scale (ca. 0.2 μs) much longer than our gating times (a few ns) and could be corrected by means of refocusing techniques29. In the case of the CNOT scheme (compound 5), the always ‘on' exchange interaction between the rings and the Co ion yields a small residual qubit–qubit coupling (Supplementary Methods). As a consequence, an unwanted slow evolution of the qubits occurs on a timescale TUE of the order of a few hundreds of ns. Although this is longer than the CNOT gate time (about 30 ns), larger values of TUE would be needed for performing sequences of many gates. This can be obtained by modifying the molecule to decrease the Co-ring exchange interaction. For instance, TUE can be increased by a factor of 50 by reducing the Co-ring exchange interaction by a factor of three, which could be achieved chemically by adding an extra phenyl group between the ring and the central node. A similar reduction of the residual qubit–qubit coupling can be obtained by increasing the static magnetic field.

In summary, we have described two different schemes for universal quantum information processing, based on either local or global control of the qubit–qubit interaction. We have demonstrated that the flexibility of molecular {Cr7Ni} qubits makes them suitable for the implementation of each of these two schemes, if properly functionalized and linked by means of a supra-molecular design strategy. The two-qubit units can be controlled either magnetically or electrically, and implement either the CNOT or Inline graphic perfectly entangling gates. Our realistic simulations, based on experimental parameters and including decoherence, show that these gates can be performed with remarkably high fidelity. The modular strategy proposed here offers a degree of control in terms of the magnitude of the coupling between molecular spin qubits, the spatial orientation of the modules and the possibility to have a switchable interaction that represents a significant step forward with respect to previous achievements on the assembly of qubits.

Since future developments of quantum technology are unpredictable, we emphasize the importance of pursuing both these two parallel roads towards the actual realization of a quantum computer.

Additional information

Accession codes: The X-ray crystallographic coordinates for structures reported in this Article have been deposited at the Cambridge Crystallographic Data Centre (CCDC), under deposition number CCDC 1029608–1029613 and 1415380–1415383. These data can be obtained free of charge from The Cambridge Crystallographic Data Centre via www.ccdc.cam.ac.uk/data_request/cif.

How to cite this article: Ferrando-Soria, J. et al. A modular design of molecular qubits to implement universal quantum gates. Nat. Commun. 7:11377 doi: 10.1038/ncomms11377 (2016).

Supplementary Material

Supplementary Information

Supplementary Figures 1-31, Supplementary Tables 1-12, Supplementary Methods and Supplementary References

ncomms11377-s1.pdf (3.3MB, pdf)
Supplementary Data 1

Cif files for compounds 3 to 11

ncomms11377-s2.zip (8.3MB, zip)

Acknowledgments

This work was supported by the EPSRC (UK), the European Commission (Marie Curie Intra-European Fellowship to J.F.-S. (622659) and A.F. (300402)). E.M.P. thanks the Panamanian agency SENACYT-IFARHU for funding. R.E.P.W. thanks the Royal Society for a Wolfson Merit Award. We also thank EPSRC (UK) for funding an X-ray diffractometer (grant number EP/K039547/1) and the National EPR Facility. We thank Diamond Light Source for access to synchrotron X-ray facilities. A.C., S.C. and P.S. acknowledge financial support from the FIRB Project No. RBFR12RPD1 of the Italian Ministry of Education and Research.

Footnotes

Author contributions J.F.S., E.M.P. and A.F. synthesized the majority of the compounds discussed; S.A.M. synthesized compound 6. G.T. was involved in design of the synthetic strategies. E.M.P. and I.J.V.-Y. carried out the X-ray single crystal diffraction studies. E.M.P., J.F.-S. and F.T. performed the EPR spectroscopic studies, and J.F.-S. carried out the electrochemical studies reported here. E.J.L.M. helped interpret the EPR spectra. A.C., S.C. and P.S. designed the schemes for quantum gates and A.C. carried out their detailed simulations. R.E.P.W. oversaw the project and wrote the paper with J.F.S., S.C. and P.S., with significant input from all other authors.

References

  1. Feynman R. P. Simulating physics with computers. Int. J. Theor. Phys. 21, 467–488 (1982). [Google Scholar]
  2. Lloyd S. Universal quantum simulators. Science 273, 1073–1078 (1996). [DOI] [PubMed] [Google Scholar]
  3. Nielsen M. A. & Chuang I. L. Quantum Computation and Quantum Information Cambridge University Press (2000). [Google Scholar]
  4. Ladd T. D. et al. Quantum computers. Nature 464, 45–53 (2010). [DOI] [PubMed] [Google Scholar]
  5. Shor P. W. Polynomial-time algorithms for prime factorization and discrete logarithms on a quantum computer. SIAM J. Comput. 26, 1484–1509 (1997). [Google Scholar]
  6. Grover L. K. Quantum computers can search arbitrarily large databases by a single query. Phys. Rev. Lett. 79, 4709–4712 (1997). [Google Scholar]
  7. Burkard G., Loss D. & DiVincenzo D. P. Coupled quantum dots as quantum gates. Phys. Rev. B 59, 2070–2078 (1999). [Google Scholar]
  8. Hanson R. & Awschalom D. D. Coherent manipulation of single spins in semiconductors. Nature 453, 1043–1049 (2008). [DOI] [PubMed] [Google Scholar]
  9. Balasubramanian G. et al. Ultralong spin coherence time in isotopically engineered diamond. Nat. Mater. 8, 383–387 (2009). [DOI] [PubMed] [Google Scholar]
  10. Knowles H. S., Kara D. M. & Atatüre M. Observing bulk diamond spin coherence in high-purity nanodiamonds. Nat. Mater. 13, 21–25 (2014). [DOI] [PubMed] [Google Scholar]
  11. Georgescu I. M., Ashhab S. & Nori F. Quantum simulation. Rev. Mod. Phys. 86, 153 (2014). [Google Scholar]
  12. Leuenberger M. & Loss D. Quantum computing in molecular magnets. Nature 410, 789–793 (2001). [DOI] [PubMed] [Google Scholar]
  13. Meier F., Levy J. & Loss D. Quantum computing with spin cluster qubits. Phys. Rev. Lett. 90, 47901–47904 (2003). [DOI] [PubMed] [Google Scholar]
  14. Troiani F. et al. Molecular engineering of antiferromagnetic rings for quantum computation. Phys. Rev. Lett. 94, 207208 (2005). [DOI] [PubMed] [Google Scholar]
  15. Troiani F., Affronte M., Carretta S., Santini P. & Amoretti G. Proposal for quantum gates in permanently coupled antiferromagnetic spin rings without need of local fields. Phys. Rev. Lett. 94, 190501 (2005). [DOI] [PubMed] [Google Scholar]
  16. Lehmann J., Gaita-Ariño A., Coronado E. & Loss D. Spin qubits with electrically gated polyoxometalate molecules. Nat. Nanotechnol. 2, 312–317 (2007). [DOI] [PubMed] [Google Scholar]
  17. Santini P., Carretta S., Troiani F. & Amoretti G. Molecular nanomagnets as quantum simulators. Phys. Rev. Lett. 107, 230502 (2011). [DOI] [PubMed] [Google Scholar]
  18. Nakazawa S. et al. A synthetic two-spin quantum bit: g-engineered exchange-coupled biradical designed for controlled-NOT gate operations. Angew. Chem. Int. Ed. 51, 9860–9864 (2012). [DOI] [PubMed] [Google Scholar]
  19. Luis F. et al. Molecular prototypes for spin-based CNOT and SWAP quantum gates. Phys. Rev. Lett. 107, 117203 (2011). [DOI] [PubMed] [Google Scholar]
  20. Aromí G., Aguilà D., Gamez P., Luis F. & Roubeau O. Design of magnetic coordination complexes for quantum computing. Chem. Soc. Rev. 41, 537–546 (2012). [DOI] [PubMed] [Google Scholar]
  21. Warner M. et al. Potential for spin-based information processing in a thin-film molecular semiconductor. Nature 503, 504–509 (2013). [DOI] [PubMed] [Google Scholar]
  22. Graham M. J. et al. Influence of electronic spin and spin-orbit coupling on decoherence in mononuclear transition metal complexes. J. Am. Chem. Soc. 136, 7623–7626 (2014). [DOI] [PubMed] [Google Scholar]
  23. Aguilà D. et al. Heterodimetallic [LnLn'] lanthanide complexes: toward a chemical design of two-qubit molecular spin quantum gates. J. Am. Chem. Soc. 136, 14215–14222 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  24. Bader K. et al. Room temperature quantum coherence in a potential molecular qubit. Nat. Commun. 5, 5304 (2014). [DOI] [PubMed] [Google Scholar]
  25. Wedge C. J. et al. Chemical engineering of molecular qubits. Phys. Rev. Lett. 108, 107204(1)–107204(5) (2012). [DOI] [PubMed] [Google Scholar]
  26. Timco G. A. et al. Engineering the coupling between molecular spin qubits by coordination chemistry. Nat. Nanotechnol. 4, 173–178 (2008). [DOI] [PubMed] [Google Scholar]
  27. Lehn J.-M. Supramolecular Chemistry: Concepts and Perspectives Wiley-VCH (1995). [Google Scholar]
  28. Ardavan A. et al. Engineering coherent interactions in molecular nanomagnet dimers. NPJ Quantum Inf. 1, 15012 (2015). [Google Scholar]
  29. Jones J. A. Quantum computing with NMR. Prog. Nucl. Magn. Reson. Spectrosc. 59, 91 (2011). [DOI] [PubMed] [Google Scholar]
  30. McInnes E. J. L., Timco G. A., Whitehead G. F. S. & Winpenny R. E. P. Heterometallic rings as a playground for physics and supramolecular building blocks. Angew. Chem., Int. Ed. 54, 14244–14269 (2015). [DOI] [PubMed] [Google Scholar]
  31. Constable E. C. et al. Expanded ligands: bis(2,2':6',2''-terpyridine carboxylic acid)ruthenium(II) complexes as metallosupramolecular analogues of dicarboxylic acids. Dalton Trans. 38, 4323–4332 (2007). [DOI] [PubMed] [Google Scholar]
  32. Hayami S., Komatsu Y., Shimizu T., Kamihata H. & Hoon Lee Y. Spin-crossover in cobalt(II) compounds containing terpyridine and its derivatives. Coord. Chem. Rev. 255, 1981–1990 (2011). [Google Scholar]
  33. Lloret F., Julve M., Cano J., Ruiz-García R. & Pardo E. Magnetic properties of six-coordinated high-spin cobalt(II) complexes: theoretical background and its application. Inorg. Chim. Acta 361, 3432–3445 (2008). [Google Scholar]
  34. Ohto A., Sasaki Y. & Ito T. Mixed-metal trinuclear complexes containing two ruthenium(III) ions and a divalent metal ion, [Ru2M(μ3-O)(μ-CH3COO)6(L)3] (M=Mg, Mn, Co, Ni, Zn; L=H2O, Pyridine). Inorg. Chem. 33, 1245–1246 (1994). [Google Scholar]
  35. Schubert U. S., Hofmeier H. & Newkome G. R. Modern Terpyridine Chemistry Wiley-VCH (2006). [Google Scholar]
  36. Cannon R. D. & White R. P. Chemical properties of triangular bridged metal complexes. Prog. Inorg. Chem. 36, 195–298 (1988). [Google Scholar]
  37. Murray K. S. in Spin-Crossover Materials: Properties and Applications (ed. Halcrow, M. A.), Ch. 1, 1–54 (John Wiley and Sons, 2013).
  38. Aribia K. B., Moehl T., Zakeeruddin S. M. & Grätzel M. Tridentate cobalt complexes as alternative redox couples for high-efficiency dye-sensitized solar cells. Chem. Sci. 4, 454–459 (2013). [Google Scholar]
  39. Connelly N. G. & Geiger W. E. Chemical redox agents for organometallic chemistry. Chem. Rev. 96, 877–910 (1996). [DOI] [PubMed] [Google Scholar]
  40. Stoll S. & Schweiger A. EasySpin, a comprehensive software package for spectral simulation and analysis in EPR. J. Magn. Reson. 178, 42–55 (2006). [DOI] [PubMed] [Google Scholar]
  41. Fernandez A. et al. g-engineering in hybrid rotaxanes to create AB and AB2 electron spin systems: EPR studies of weak interactions between dissimilar electron spin qubits. Angew. Chem. Int. Ed. 54, 10858–10861 (2015). [DOI] [PubMed] [Google Scholar]
  42. Chiesa A. et al. Molecular nanomagnets with switchable coupling for quantum simulation. Sci. Rep. 4, 7423 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  43. Park K., Novotny M. A., Dalal N. S., Hill S. & Rikvold P. A. Effects of D-strain, g-strain, and dipolar interactions on EPR linewidths of the molecular magnets Fe8 and Mn12. Phys. Rev. B 65, 014426 (2001). [Google Scholar]
  44. Wesenberg J. & Mølmer K. Robust quantum gates and a bus architecture for quantum computing with rare-earth-ion-doped crystals. Phys. Rev. A 68, 012320 (2003). [Google Scholar]
  45. Cummins H. K., Llewellyn G. & Jones J. A. Tackling systematic errors in quantum logic gates with composite rotations. Phys. Rev. A 67, 042308 (2003). [Google Scholar]
  46. Tempel D. G. & Aspuru-Guzik A. Relaxation and dephasing in open quantum systems time-dependent density functional theory: properties of exact functionals from an exactly-solvable model system. Chem. Phys. 391, 130 (2011). [Google Scholar]
  47. Breuer H. P. & Petruccione F. The Theory of Open Quantum Systems Oxford University Press (2002). [Google Scholar]
  48. Whitehead G. F. S. et al. Rings and threads as linkers in metal-organic frameworks and poly-rotaxanes. Chem. Commun. 49, 7195–7197 (2013). [DOI] [PubMed] [Google Scholar]
  49. Green J. E. et al. A 160-kilobit molecular electronic memory patterned at 1011 bits per square centimeter. Nature 445, 414–417 (2007). [DOI] [PubMed] [Google Scholar]
  50. Corradini V. et al. Magnetic anisotropy of Cr7Ni spin clusters on surfaces. Adv. Funct. Mat 22, 3706 (2012). [Google Scholar]
  51. Jing J. & Hu X. Scaling of decoherence for a system of uncoupled spin qubits. Sci. Rep. 5, 17013 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-31, Supplementary Tables 1-12, Supplementary Methods and Supplementary References

ncomms11377-s1.pdf (3.3MB, pdf)
Supplementary Data 1

Cif files for compounds 3 to 11

ncomms11377-s2.zip (8.3MB, zip)

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES