Skip to main content
PMC home page
Nature Communications logoLink to Nature Communications
. 2016 May 9;7:11516. doi: 10.1038/ncomms11516

Intrinsic correlation between β-relaxation and spatial heterogeneity in a metallic glass

F Zhu 1,2, H K Nguyen 2, S X Song 1, Daisman P B Aji 2, A Hirata 2, H Wang 2, K Nakajima 2, M W Chen 1,2,a
PMCID: PMC4865810  PMID: 27158084

Abstract

β-relaxation has long been attributed to localized motion of constituent molecules or atoms confined to isolated regions in glasses. However, direct experimental evidence to support this spatially heterogeneous scenario is still missing. Here we report the evolution of nanoscale structural heterogeneity in a metallic glass during β-relaxation by utilizing amplitude-modulation dynamic atomic force microscopy. The successive degeneration of heterogeneity during β-relaxation can be well described by the Kohlrausch–Williams–Watts equation. The characteristic relaxation time and activation energy of the heterogeneity evolution are in accord with those of excess enthalpy release by β-relaxation. Our study correlates β-relaxation with nanoscale spatial heterogeneity and provides direct evidence on the structural origins of β-relaxation in metallic glasses.

Inline graphicBeta-relaxation in glasses is commonly attributed to the confined motions of constituent atoms in nanosized domains, but there is no direct evidence so far. Here, Zhu et al. show the correlation between the evolution of spatial heterogeneity at nanoscale and beta-relaxation below glass transition point.

When a glass-forming liquid is cooled down to the moderately supercooled regime, the high-temperature single-relaxation process splits into α- and β-relaxations1,2,3,4,5. The α-relaxation is related to glass transition and vanishes at a glass transition temperature (Tg), whereas the β-relaxation or Johari–Goldstein relaxation remains as the principal source of dynamics in glasses4,5,6. Recent studies have demonstrated that the β-relaxation plays important roles in structural relaxation7, accelerated partial devitrification8 and deformation of metallic glasses9,10, showing both scientific and practical significances in glass dynamics. However, microscopic origins of β-relaxation have not been fully understood and theoretical models remain evolving2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17. It has been originally suggested by Johari11 that the β-relaxation in mechanically rigid glasses, especially in metallic glasses, should be processed by the translational motion of atoms localized in loosely packed regions. This scheme certainly assumes that β-relaxation intrinsically correlates with and structurally originates from structural heterogeneity of glasses. Although structural heterogeneity has been observed in metallic glasses at different length scales by experiments8,18,19,20,21,22,23,24,25,26 and simulations27,28,29,30,31, the direct evidence on the intrinsic correlation between structural heterogeneity and β-relaxation in metallic glasses is still missing. Consequently, the structural origins of β-relaxation are still under debate despite many efforts devoted to this long-standing problem.

In this study, we utilize amplitude-modulation dynamic atomic force microscopy (AM-AFM)20,32,33 to characterize the successive changes of structural heterogeneity in a metallic glass regulated by the sub-Tg enthalpy relaxation. It is found that the characteristic relaxation time and activation energy of the heterogeneity dynamics are approximately equal to those of β-relaxation measured by excess enthalpy release using a hyper-quenching–annealing–calorimetric scan approach7. The quantitative measurements of the dynamics for both spatial heterogeneity and β-relaxation in one system provide direct evidence on the intrinsic correlation between β-relaxation and heterogeneous structure in metallic glasses, which reveals the structural origin of sub-Tg relaxation of metallic glasses.

Results

Sub-T g relaxation

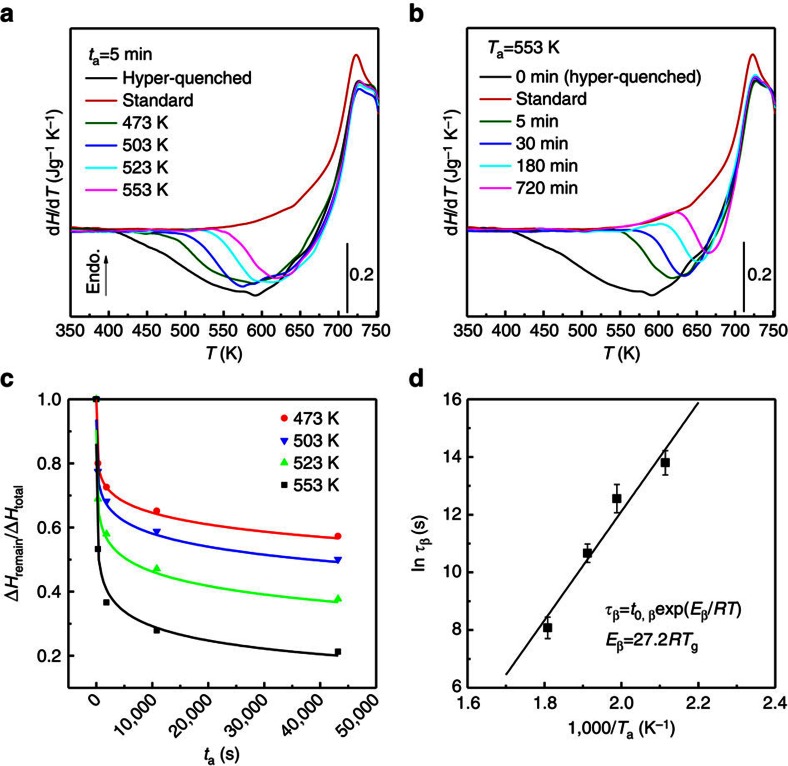
To successively change the local structure of the hyper-quenched metallic glass, the glass samples are relaxed by annealing at sub-Tg temperatures from Ta=473 K (∼0.68Tg, Tg=695 K) to 553 K (∼0.8Tg) for different durations from ta=5 min to 720 min. For reference, the hyper-quenched metallic glass without annealing is marked with ta=0 min. Figure 1 shows the differential scanning calorimeter (DSC) profiles of the sub-Tg relaxation. Compared with the standard sample, the hyper-quenched sample exhibits a broad exothermic peak below Tg, indicative of the abundant excess enthalpy trapped in the glass with a structure far away from equilibrium16. At Ta=553 K, the excess enthalpy is sequentially released with annealing time (Fig. 1a). At a constant annealing time ta=5 min, more excess enthalpy is released at higher Ta (Fig. 1b). As demonstrated by the previous DSC measurements7,16, the β-relaxation appears as the tails at the low-temperature side of the broad sub-Tg exothermic peaks in the DSC traces. Our enthalpy relaxation is controlled below 0.8Tg at which the sub-Tg relaxation shows irrelevant influence on the glass transition as the DSC traces above Tg are almost identical before and after annealing (see Fig. 1a and Supplementary Fig. 1). Thus, it can be concluded that the sub-Tg relaxation below 0.8Tg is mainly processed by the β-relaxation. The remaining excess enthalpy ΔHremain for the samples annealed below 553 K is normalized by the total excess enthalpy ΔHtotal measured from the hyper-quenched sample and plotted against ta as shown in Fig. 1c. The time dependence of the normalized enthalpy release at each annealing temperature can be well fitted by the Kohlrausch–Williams–Watts (KWW) function: 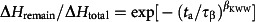Inline graphic, where τβ is the characteristic relaxation time and βKWW is the stretch parameter for the fitting. The KWW function is often used to describe the temporal behaviour of disordered systems in response to external stimulus, such as high temperature, applied electric field, stress and imposed deformation1. The good fitting of the normalized sub-Tg enthalpy release confirms the previous suggestion that the KWW function is appropriate for time-related processes of β-relaxation1,7. Since the β-relaxation also obeys the Arrhenius law1,7, the temperature dependence of the relaxation time, τβ, derived from the KWW fittings, follows the Arrhenius equation: 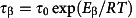Inline graphic. The activation energy Eβ for the sub-Tg relaxation can be obtained by plotting the logarithm of the relaxation time τβ versus the reciprocal annealing temperature (1/Ta). The linear relationship in Fig. 1d gives a value of Eβ around (27.2±4.3)RTg, which is in accord with the empirical value Eβ=∼26RTg of β-relaxation in metallic glasses, polymers, rigid molecules and other glasses, but much smaller than that of α-relaxation4,6,7,16,34. Particularly, this value is consistent with the activation energy for β-relaxation of rapidly quenched metallic glasses in previous thermal measurements7,16 and dynamic mechanical analyses35. Therefore, the β-relaxation dominates the excess enthalpy release and the structural changes during the sub-Tg relaxation of the hyper-quenched metallic glass.

Figure 1. Structural relaxation of hyper-quenched metallic glass below Tg.

Figure 1

Open in a new tab

(a) Heat flow traces of the glass samples relaxed at Ta=553 K for different durations; and (b) the samples annealed at different temperatures Ta for ta=5 min. (c) Annealing time ta dependence of the normalized remaining enthalpy for the samples annealed at 473, 503, 523 and 553 K and their corresponding fittings by KWW function. (d) Dependence of the characteristic relaxation time τβ on the reciprocal annealing temperature (1/Ta), surrendering the activation energy of sub-Tg relaxation. The error bars indicate standard deviation. Endo., endothermic.

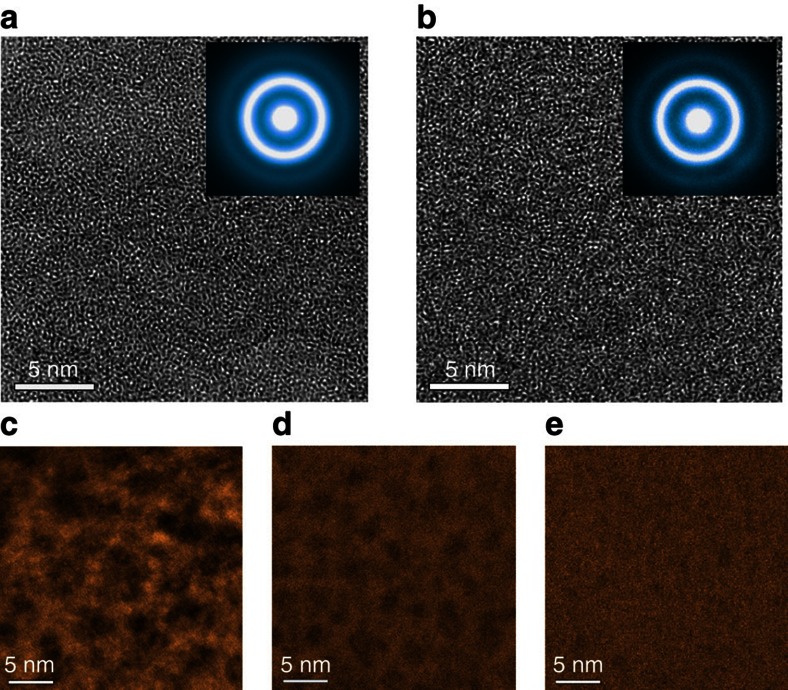
The glass samples before and after the annealing are inspected by X-ray diffraction (Supplementary Fig. 2), X-ray energy dispersive spectroscopy (Supplementary Fig. 3) and X-ray photoelectron spectroscopy (XPS) (Supplementary Fig. 4). No evident changes in amorphous structure and chemistry, particularly oxygen concentration, can be seen, suggesting that the annealing does not cause detectable crystallization or oxidation. The detected oxygen in the X-ray photoelectron spectroscopic spectra is mostly from the surface adsorption of carbonates, as well as from the topmost surface oxidation after the samples are exposed to an atmosphere. Phase-contrast high-resolution transmission electron microscopy (TEM) images show the homogeneously amorphous structures of the hyper-quenched (Fig. 2a) and relaxed samples (Fig. 2b). The corresponding diffraction halos (insets in Fig. 2a,b) confirm their amorphous natures. However, obvious contrast variation can be observed in the hyper-quenched metallic glass by high-angle annular dark-field scanning TEM (Fig. 2c). The size of dark regions, corresponding to low density domains, is ranging from 5 to 7 nm. After the sub-Tg relaxation at 553 K, the dark regions become weaker and smaller with the increase of annealing time (Fig. 2d,e). Since all TEM samples are prepared by ion milling with the same conditions, such contrast difference may be associated with the nanoscale structural heterogeneity of metallic glass. More detailed TEM analysis of the inhomogeneous structure in metallic glasses will be reported elsewhere.

Figure 2. Microstructure of the hyper-quenched and sub-Tg relaxed metallic glass.

Figure 2

Open in a new tab

HRTEM images of (a) the hyper-quenched metallic glass and (b) the metallic glass relaxed at 553 K for 720 min. High-angle annular dark-field scanning TEM (HAADF-STEM) images of (c) the hyper-quenched metallic glass, (d) the metallic glass relaxed at 553 K for 5 min and (e) relaxed at 553 K for 720 min. All the samples were prepared using gentle ion milling.

Amplitude-modulation AFM

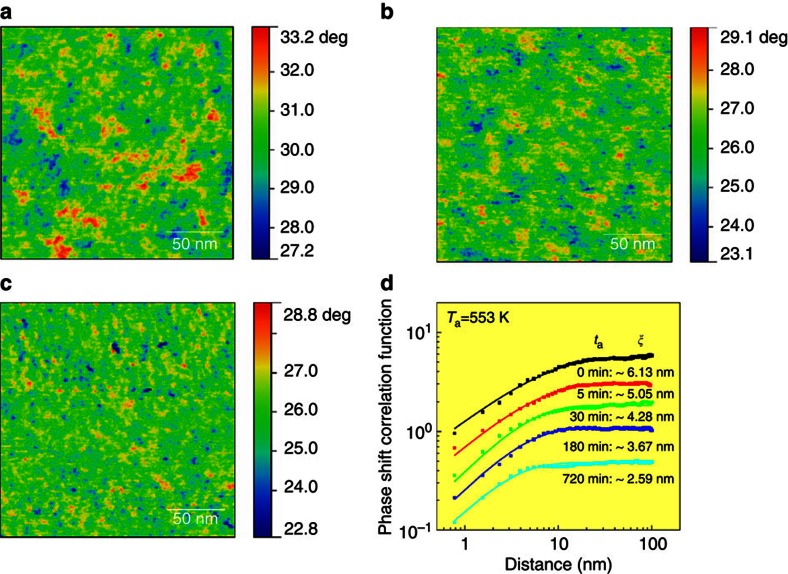
Figure 3a presents the AM-AFM phase shift image of the hyper-quenched metallic glass before and after the sub-Tg relaxation at 553 K. Since the phase lags in the AM-AFM image arise from the energy dissipation during tip–sample interactions, the viscoelastic origin of the phase shift can be determined by measuring the normalized dissipated energy versus the amplitude ratio (Supplementary Note 1)20,32. The inhomogeneous intensity distribution in the phase shift image corresponds to position-dependent viscoelasticity in the glassy sample, originating from spatial heterogeneity caused by local structure variation20. Importantly, the phase shift in the images is independent of the surface roughness (Supplementary Figs 5 and 6). The colour range in the image is set according to the Gaussian distributions of phase shift angles (Supplementary Fig. 7). After structural relaxation at Ta=553 K for 5 min, the characteristic length of the spatial heterogeneity becomes smaller, accompanying with a more dispersed distribution of high phase shift regions in the matrix (Fig. 3b). For a longer annealing duration of 720 min at Ta=553 K, the β-relaxation leads to a further decrease in size of the domains which correspond to the spatial heterogeneity (Fig. 3c). The correlation function P(r)=2σ2[1−exp(−(r/ξ)2α)] is applied to quantitatively evaluate the characteristic length of the spatial heterogeneity (Fig. 3d), where σ is the standard deviation of phase shift values, α is the exponent and ξ is the lateral correlation length and defines the characteristic lengths between two correlated points in the phase shift images20,32. The correlation length of the spatial heterogeneity in the hyper-quenched metallic glass is measured to be ∼6.13 nm. Although this value appears to be much smaller than the sizes of the prominent features of phase shift image, the zoom-in AM-AFM micrograph verifies that the correlation length represents the mean sizes of spatial heterogeneity in local regions over the entire image (Supplementary Fig. 8). After annealing for 5, 30, 180 and 720 min at Ta=553 K, the correlation length sequentially decreases to around 5.05, 4.28, 3.67 and 2.59 nm. The samples annealed at lower Ta of 473, 503 and 523 K show slower processes of the correlation length shrinkage (Supplementary Figs 9 and 10). From the phase shift and actual amplitude images, the distribution of dissipation energy can be obtained, which directly reflects the heterogeneity of structure and mechanical properties of the glass samples20. After sub-Tg relaxation, the amplitudes of the dissipation energy Edis. become smaller and the distribution profiles of Edis. turn out to be sharper (Supplementary Fig. 11), implying that the structure of the metallic glass develops more uniform with the disappearance and shrinkage of the high-energy-dissipated domains.

Figure 3. Evolution of spatial heterogeneity during sub-Tg relaxation at Ta=553 K.

Figure 3

Open in a new tab

Phase shift images of (a) the hyper-quenched metallic glass, (b) the metallic glass relaxed at 553 K for 5 min and (c) relaxed at 553 K for 720 min. (d) Correlation function curves of the samples annealed at 553 K for different durations. The correlation lengths of spatial heterogeneity in phase shift images can be determined by the correlation function curves. Note that the curves were shifted vertically for clear identification.

Dynamics of heterogeneity evolution

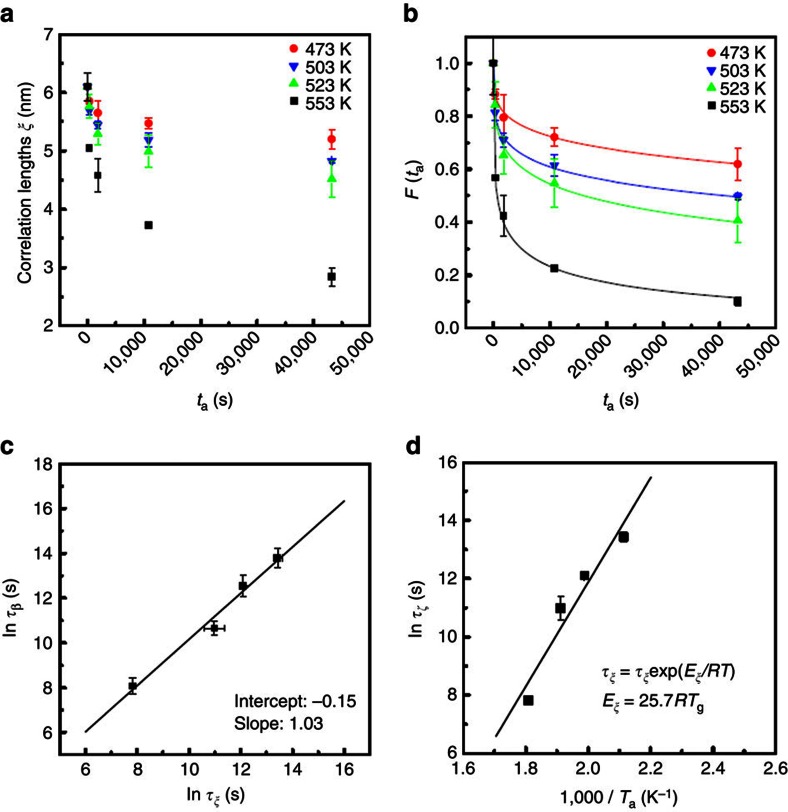
We analyse the dynamics of the spatial heterogeneity evolution during the sub-Tg relaxation at 473, 503, 523 and 553 K. The mean values of correlation lengths are plotted with ta for all samples in Fig. 4a, showing a Ta-dependent relaxation behaviour. We use the KWW function 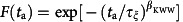Inline graphic to describe the relaxation behaviour of the spatial heterogeneity, where τξ is the characteristic relaxation time for the evolution of spatial heterogeneity. Since the spatial heterogeneity evolves in a three-dimensional space, the normalized correlation length cubed, F(ta)=(ξ/ξ0)3, where ξ0 is the correlation length of the hyper-quenched metallic glass, is used to fit the structure evolution at each annealing temperature. The nearly perfect fittings for the data acquired at four annealing temperatures (Fig. 4b) imply that the degeneration of the spatial heterogeneity is related to the sub-Tg dynamics of the metallic glass1,17. Similar to sub-Tg enthalpy release, the relaxation time τξ, derived from the KWW fitting, becomes shorter at higher Ta. Importantly, as shown in Fig. 4c and Table 1, the relaxation time τξ is approximately equal to the characteristic relaxation time τβ of the sub-Tg enthalpy relaxation at the same annealing temperatures, indicating the inherent relationship between sub-Tg enthalpy release and the evolution of spatial heterogeneity. According to the Arrhenius equation, the activation energy Eξ for the structural evolution is given to be (25.7±5.2)RTg (Fig. 4d). Again, this value is close to the activation energy Eβ for β-relaxation4,6,7,16,34,35. The relatively large variation of the measured activation energy values for both enthalpy release and spatial heterogeneity evolution is mainly due to the experimental error. Especially, the high-temperature data points (553 K) tend to form a curve in the linear Arrhenius plots (Fig. 1d and Fig. 4d), indicating a higher activation energy required for the high-temperature structure relaxation. As shown in Supplementary Fig. 1d, one can see that after annealing at 553 K for 720 min, the heat flow trace above the glass transition temperature has a slight deviation from the hyper-quenched one, suggesting that insignificant α-relaxation, which requires a much higher activation energy than β-relaxation, may be involved into the long-term sub-Tg relaxation and leads to the deviation of the 553 K data points from the linear Arrhenius plots.

Figure 4. Dynamics of the nanoscale spatial heterogeneity.

Figure 4

Open in a new tab

(a) The evolution of the correlation lengths ξ of the samples relaxed at 473 K, 503 K, 523 K and 553 K for different durations. (b) Annealing duration ta dependences of the normalized correlation length cubed F(ta). (c) A comparison between the characteristic relaxation times τβ and τξ derived from the sub-Tg enthalpy relaxation and the evolution of spatial heterogeneity, respectively. (d) The activation energy of the evolution of spatial heterogeneity derived from the dependence of characteristic relaxation time τξ of the spatial heterogeneity volumes on the reciprocal annealing temperature (1/Ta). The error bars indicate standard deviation.

Table 1. The characteristic relaxation times τ ξ and τ β .

Ta (K) ln τξ (s) ln τβ (s)
473 13.43±0.16 13.80±0.42
503 12.16±0.12 12.56±0.49
523 10.98±0.40 10.66±0.32
553 7.82±0.03 8.08±0.37
Open in a new tab

Discussion

The equivalences in relaxation time and activation energy between spatial heterogeneity evolution and sub-Tg enthalpy relaxation suggest that the underlying physical processes for the two different phenomena are dynamically identical. In fact, the dynamic-structure correspondence also unveils the micromechanisms of β-relaxation. Apparently, the β-relaxation cannot be attributed to all the atoms in mechanically rigid metallic glasses because a permanent macroscopic deformation has not been observed during mechanical or thermal stimulations at β-relaxation timescale. Thus, one reasonable scenario for β-relaxation in metallic glasses is local atomic motions or short-range diffusion confined to isolated small regions, which are energetically unstable and give rise to high energy dissipation during AM-AFM scanning and enthalpy release during thermal relaxation. The evolution of spatial heterogeneity with β-relaxation provides direct evidence on the scenario, that is, the β-relaxation is realized by very local structure changes, rather than global atomic motion. On the other hand, the local atomic re-arrangement through short-range diffusion or cooperative atomic motion14,16, driven by the release of the excess enthalpy, leads to the structure evolution of the hyper-quenched metallic glass and thereby the degeneration of the spatial heterogeneity.

The origins of the spatial heterogeneity in glasses have not been completely understood17. Apparently, it inherits from low-temperature supercooled liquids and represents the frozen state of supercooled liquids during glass transition36. Therefore, the spatial heterogeneity could be the result of heterogeneous dynamics of supercooled liquids, associated with density fluctuations11,37, frustration-limited domains38,39 or the structural basis of fragility40. It may also be created as relics of the liquid–liquid transition when the cooling rate is high enough, such as hyper-quenching, to completely freeze the liquid structure41. As revealed by Angstrom-beam electron diffraction42, the local atomic dense packing in metallic glasses, such as icosahedra, experiences geometric frustration because the free-energy-preferred atomic configurations cannot fill space efficiently and fails to extend indefinitely, giving birth to the frustration-limited domains or the spatial heterogeneity in glasses. As evidenced by the local energy dissipation and enthalpy release, the spatial heterogeneity observed in this study is related to the formation of a high-energy ‘phase' in comparison with the relatively stable matrix. Therefore, after the sub-Tg annealing, the strength of β-relaxation gradually decreases, together with the degeneration of the high-energy-dissipated domains. This structure change may lead to the loss of active sites that act as shear transformation zones to respond to the applied external forces for plastic deformation10,25,28,43,44 and thereby results in the annealing-induced embrittlement45, one of the major challenges for commercialization of metallic glasses46. The intrinsic correlation between spatial heterogeneity and β-relaxation revealed by this study may also explain the rejuvenation47 and temperature-dependent fast secondary relaxation48 of metallic glasses by non-affine thermal strains. The mismatch in local thermal expansion caused by spatial heterogeneity can lead to increased geometric frustration and high-energy domains with the growth of spatial heterogeneity at low temperatures and during the thermal cycling.

In summary, we report the evolution of spatial heterogeneity in a hyper-quenched metallic glass during sub-Tg β-relaxation. The characteristic relaxation times and activation energy of the spatial heterogeneity dynamics are in well accordance with those of β-relaxation, evidencing the intrinsic correlation between local structure evolution and sub-Tg β-relaxation. The microscopic connection between structure and dynamics of metallic glasses provides compelling evidence on the structural origin of β-relaxation and has important implications in understanding the mechanical properties and dynamics of metallic glasses.

Methods

Sample preparation

A hyper-quenched metallic glass with a composition of Zr53Cu36Al11 (atomic %) is prepared using RF magnetron sputtering with a deposition rate of∼0.2 nm s-1 at room temperature49. The thickness of the glass films on silicon (100) substrates is about 4 μm. By carefully controlling the deposition conditions, a mirror-like smooth surface with a sub-nanoscale roughness are achieved from the as-deposited films. The nominal cooling rate is evaluated to be ∼2.4 × 107 K s−1 according to the scaled Arrhenius plot of fictive temperatures versus cooling rates16.

Sub-T g relaxation

The sub-Tg relaxation is performed using the hyper-quenching–annealing–calorimetric scan approach7. The samples are annealed in a Pt furnace with flowing pure Ar gas to prevent possible surface oxidation. The thermal properties of the glass are measured using DSC (Perkin-Elmer 8500). The heating and cooling rates for thermal scanning are set to be 20 K min−1. The standard sample is prepared by slowly cooling the hyper-quenched sample from the supercooled liquid region at 20 K min−1 and then subjected to the second up-scan to obtain the standard heat flow trace.

Amplitude-modulation AFM

The hyper-quenched and relaxed samples are directly used for the AM-AFM testing without any polishing or surface processing to avoid damage and contamination to the sub-nanoscale smooth surfaces. The AM-AFM measurements are performed by a scanning probe microscope (Bruker MultiMode) at a tapping mode with a Nanoscope V controller. A pyramidal silicon tip with a sharp diamond-like spike of∼1 nm and spring constant around 5 N m-1 is driven vibrating near the resonant frequency (∼ 160 kHz) of the Si cantilever to scan across the top surfaces of samples. Surface height, phase shift and amplitude images are recorded simultaneously during the scanning. At least three different locations are scanned for each sample to get the mean values. The amplitude ratio is set to be around 0.85 during the scanning to avoid the damage of the sharp spike.

Electron microscopy

Transmission electron microscopy samples are carefully prepared by the ion milling with 3 keV Ar ions at the liquid nitrogen temperature. High-resolution TEM and high-angle annular dark-field scanning TEM observations are conducted using a Cs-corrected TEM (JEM-2100 F, JEOL) with double spherical aberration (Cs) correctors for both the probe-forming and image-forming objective lenses.

Additional information

How to cite this article: Zhu, F. et al. Intrinsic correlation between β-relaxation and spatial heterogeneity in a metallic glass. Nat. Commun. 7:11516 doi: 10.1038/ncomms11516 (2016).

Supplementary Material

Supplementary Information

Supplementary Figures 1-12, Supplementary Note 1 and Supplementary References

ncomms11516-s1.pdf (1,010.9KB, pdf)

Acknowledgments

This work was sponsored by MOST 973 of China (Grant No. 2015CB856800), National Natural Science Foundation of China (Grant No. 11327902, 51271113), and the fusion research program of ‘World Premier International Research Center (WPI) Initiative' by MEXT, Japan.

Footnotes

Author contributions M.W.C. conceived and supervised this study. F.Z., S.X.S. and H.W. prepared the hyper-quenched samples. F.Z. and D.P.B.A. conducted the DSC measurements. F.Z. and A.H. performed TEM characterization. F.Z., H.K.N. and K.N. contributed to AM-AFM experiments. M.W.C. and F. Z. wrote the manuscript. All the authors contributed to data analysis and discussion.

References

  1. Debenedetti P. G. & Stillinger F. H. Supercooled liquids and the glass transition. Nature 410, 259–267 (2001). [DOI] [PubMed] [Google Scholar]
  2. Johari G. P. Intrinsic mobility of molecular glasses. J. Chem. Phys. 58, 1766–1770 (1973). [Google Scholar]
  3. Johari G. P. & Goldstein M. Viscous liquids and the glass transition. II. Secondary relaxations in glasses of rigid molecules. J. Chem. Phys. 53, 2372–2388 (1970). [Google Scholar]
  4. Ngai K. L. Relaxation and Diffusion in Complex Systems Springer (2011). [Google Scholar]
  5. Angell C. A., Ngai K. L., McKenna G. B., McMillan P. F. & Martin S. W. Relaxation in glassforming liquids and amorphous solids. J. Appl. Phys. 88, 3113–3157 (2000). [Google Scholar]
  6. Yu H. B., Wang W. H., Bai H. Y. & Samwer K. The β-relaxation in metallic glasses. Natl. Sci. Rev. 1, 429–461 (2014). [Google Scholar]
  7. Hu L. N. & Yue Y. Z. Secondary relaxation in metallic glass formers: its correlation with the genuine Johari-Goldstein relaxation. J. Phys. Chem. C 113, 15001–15006 (2009). [Google Scholar]
  8. Ichitsubo T. et al. Microstructure of fragile metallic glasses inferred from ultrasound-accelerated crystallization in Pd-based metallic glasses. Phys. Rev. Lett. 95, 245501 (2005). [DOI] [PubMed] [Google Scholar]
  9. Wang W. H. Correlation between relaxations and plastic deformation, and elastic model of flow in metallic glasses and glass-forming liquids. J. Appl. Phys. 110, 053521 (2011). [Google Scholar]
  10. Yu H. B. et al. Tensile plasticity in metallic glasses with pronounced β relaxations. Phys. Rev. Lett. 108, 015504 (2012). [DOI] [PubMed] [Google Scholar]
  11. Johari G. P. Localized molecular motions of beta-relaxation and its energy landscape. J. Non Cryst. Solids 307, 317–325 (2002). [Google Scholar]
  12. Thayyil M. S., Capaccioli S., Prevosto D. & Ngai K. L. Is the Johari-Goldstein beta-relaxation universal? Philos. Mag. 88, 4007–4013 (2008). [Google Scholar]
  13. Stevenson J. D. & Wolynes P. G. A universal origin for secondary relaxations in supercooled liquids and structural glasses. Nat. Phys. 6, 63–68 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Yu H. B., Samwer K., Wu Y. & Wang W. H. Correlation between beta relaxation and self-diffusion of the smallest constituting atoms in metallic glasses. Phys. Rev. Lett. 109, 095508 (2012). [DOI] [PubMed] [Google Scholar]
  15. Yu H. B., Samwer K., Wang W. H. & Bai H. Y. Chemical influence on β-relaxations and the formation of molecule-like metallic glasses. Nat. Commun. 4, 2204 (2013). [DOI] [PubMed] [Google Scholar]
  16. Liu Y. H., Fujita T., Aji D. P. B., Matsuura M. & Chen M. W. Structural origins of Johari-Goldstein relaxation in a metallic glass. Nat. Commun. 5, 3238 (2014). [DOI] [PubMed] [Google Scholar]
  17. Ediger M. D. Spatially heterogeneous dynamics in supercooled liquids. Annu. Rev. Phys. Chem 51, 99–128 (2000). [DOI] [PubMed] [Google Scholar]
  18. He L., Zhang P., Besser M. F., Kramer M. J. & Voyles P. M. Electron correlation microscopy: a new technique for studying local atom dynamics applied to a supercooled liquid. Microsc. Microanal. 21, 1026–1033 (2015). [DOI] [PubMed] [Google Scholar]
  19. Wang Z., Sun B. A., Bai H. Y. & Wang W. H. Evolution of hidden localized flow during glass-to-liquid transition in metallic glass. Nat. Commun. 5, 5823 (2014). [DOI] [PubMed] [Google Scholar]
  20. Liu Y. H. et al. Characterization of nanoscale mechanical heterogeneity in a metallic glass by dynamic force microscopy. Phys. Rev. Lett. 106, 125504 (2011). [DOI] [PubMed] [Google Scholar]
  21. Wagner H. et al. Local elastic properties of a metallic glass. Nat. Mater. 10, 439–442 (2011). [DOI] [PubMed] [Google Scholar]
  22. Dmowski W., Iwashita T., Chuang C. P., Almer J. & Egami T. Elastic heterogeneity in metallic glasses. Phys. Rev. Lett. 105, 205502 (2010). [DOI] [PubMed] [Google Scholar]
  23. Ye J. C., Lu J., Liu C. T., Wang Q. & Yang Y. Atomistic free-volume zones and inelastic deformation of metallic glasses. Nat. Mater. 9, 619–623 (2010). [DOI] [PubMed] [Google Scholar]
  24. Huo L. S., Zeng J. F., Wang W. H., Liu C. T. & Yang Y. The dependence of shear modulus on dynamic relaxation and evolution of local structural heterogeneity in a metallic glass. Acta Mater. 61, 4329–4338 (2013). [Google Scholar]
  25. Pan D., Inoue A., Sakurai T. & Chen M. W. Experimental characterization of shear transformation zones for plastic flow of bulk metallic glasses. Proc. Natl Acad. Sci. USA 105, 14769–14772 (2008). [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. Yang Y. et al. Fractal growth of the dense-packing phase in annealed metallic glass imaged by high-resolution atomic force microscopy. Acta Mater. 60, 5260–5272 (2012). [Google Scholar]
  27. Ding J., Patinet S., Falk M. L., Cheng Y. & Ma E. Soft spots and their structural signature in a metallic glass. Proc. Natl Acad. Sci. USA 111, 14052–14056 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Cheng Y. Q. & Ma E. Atomic-level structure and structure-property relationship in metallic glasses. Prog. Mater. Sci. 56, 379–473 (2011). [Google Scholar]
  29. Murali P. et al. Atomic scale fluctuations govern brittle fracture and cavitation behavior in metallic glasses. Phys. Rev. Lett. 107, 215501 (2011). [DOI] [PubMed] [Google Scholar]
  30. Fujita T. et al. Atomic-scale heterogeneity of a multicomponent bulk metallic glass with excellent glass forming ability. Phys. Rev. Lett. 103, 075502 (2009). [DOI] [PubMed] [Google Scholar]
  31. Fujita T. et al. Coupling between chemical and dynamic heterogeneities in a multicomponent bulk metallic glass. Phys. Rev. B 81, 140204 R (2010). [Google Scholar]
  32. Garcia R. et al. Identification of nanoscale dissipation processes by dynamic atomic force microscopy. Phys. Rev. Lett. 97, 016103 (2006). [DOI] [PubMed] [Google Scholar]
  33. Nguyen H. K., Wang D., Russell T. P. & Nakajima K. Observation of dynamical heterogeneities and their time evolution on the surface of an amorphous polymer. Soft Matter 11, 1425–1433 (2015). [DOI] [PubMed] [Google Scholar]
  34. Ngai K. L. & Capaccioli S. Relation between the activation energy of the Johari-Goldstein β relaxation and Tg of glass formers. Phys. Rev. E 69, 031501 (2004). [DOI] [PubMed] [Google Scholar]
  35. Hachenberg J. et al. Merging of the α and β relaxations and aging via the Johari–Goldstein modes in rapidly quenched metallic glasses. Appl. Phys. Lett. 92, 131911 (2008). [Google Scholar]
  36. Egami T. & Srolovitz D. Local structural fluctuations in amorphous and liquid metals: a simple theory of the glass transition. J. Phys. F Met. Phys. 12, 2141–2163 (1982). [Google Scholar]
  37. Ediger M. D. Can density or entropy fluctuations explain enhanced translational diffusion in glass-forming liquids? J. Non Cryst. Solids 235, 10–18 (1998). [Google Scholar]
  38. Kivelson D., Kivelson S. A., Zhao X., Nussinov Z. & Tarjus G. A. thermodynamic theory of supercooled liquids. Phys. A 219, 27–38 (1995). [Google Scholar]
  39. Hu Y. C., Li F. X., Li M. Z., Bai H. Y. & Wang W. H. Five-fold symmetry as indicator of dynamic arrest in metallic glass-forming liquids. Nat. Commun. 6, 8310 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  40. Mauro N. A., Blodgett M., Johnson M. L., Vogt A. J. & Kelton K. F. A structural signature of liquid fragility. Nat. Commun. 5, 4616 (2014). [DOI] [PubMed] [Google Scholar]
  41. Xu W. et al. Evidence of liquid-liquid transition in glass-forming La50Al35Ni15 melt above liquidus temperature. Nat. Commun. 6, 7696 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  42. Hirata A. et al. Geometric frustration of icosahedron in metallic glasses. Science 341, 376–379 (2013). [DOI] [PubMed] [Google Scholar]
  43. Falk M. L. & Langer J. S. Dynamics of viscoplastic deformation in amorphous solids. Phys. Rev. E 57, 7192 (1998). [Google Scholar]
  44. Perepezko J. H., Imhoff S. D., Chen M. W., Wang J. Q. & Gonzalez S. Nucleation of shear bands in amorphous alloys. Proc. Natl Acad. Sci. USA 111, 3938–3942 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  45. Murali P. & Ramamurty U. Embrittlement of a bulk metallic glass due to sub-Tg annealing. Acta Mater. 53, 1467–1478 (2005). [Google Scholar]
  46. Plummer J. & Johnson W. L. Is metallic glass poised to come of age? Nat. Mater. 14, 553–555 (2015). [DOI] [PubMed] [Google Scholar]
  47. Ketov S. V. et al. Rejuvenation of metallic glasses by non-affine thermal strain. Nature 524, 200–203 (2015). [DOI] [PubMed] [Google Scholar]
  48. Wang Q. et al. Unusual fast secondary relaxation in metallic glass. Nat. Commun. 6, 7876 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  49. Liu Y. H. et al. Deposition of multicomponent metallic glass films by single-target magnetron sputtering. Intermetallics 21, 105–114 (2012). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-12, Supplementary Note 1 and Supplementary References

ncomms11516-s1.pdf (1,010.9KB, pdf)

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES