Abstract
The ongoing activity of neurons generates a spatially and time-varying field of extracellular voltage (Ve). This Ve field reflects population-level neural activity, but does it modulate neural dynamics and the function of neural circuits? We provide a cable theory framework to study how a bundle of model neurons generates Ve and how this Ve feeds back and influences membrane potential (Vm). We find that these “ephaptic interactions” are small but not negligible. The model neural population can generate Ve with millivolt-scale amplitude, and this Ve perturbs the Vm of “nearby” cables and effectively increases their electrotonic length. After using passive cable theory to systematically study ephaptic coupling, we explore a test case: the medial superior olive (MSO) in the auditory brain stem. The MSO is a possible locus of ephaptic interactions: sounds evoke large (millivolt scale) Ve in vivo in this nucleus. The Ve response is thought to be generated by MSO neurons that perform a known neuronal computation with submillisecond temporal precision (coincidence detection to encode sound source location). Using a biophysically based model of MSO neurons, we find millivolt-scale ephaptic interactions consistent with the passive cable theory results. These subtle membrane potential perturbations induce changes in spike initiation threshold, spike time synchrony, and time difference sensitivity. These results suggest that ephaptic coupling may influence MSO function.
Keywords: ephaptic coupling, medial superior olive, cable theory, field potential, coincidence detection
neurons are bathed in a shared extracellular voltage (Ve) that is generated by voltage-gated ionic currents, synaptic currents, and other transmembrane currents (see Buzsáki et al. 2012 for review). This endogenous Ve can be recorded in vivo with depth electrodes (e.g., the local field potential) and is detectable on the surface of the scalp (e.g., electroencephalography). It has long been known that current delivered via a stimulating electrode can produce an applied (i.e. “exogenous”) Ve that modulates neural activity (e.g., Strumwasser and Rosenthal 1960). Indeed, this is the basic mechanism by which neural prostheses such as cochlear implants and deep brain stimulation provide therapeutic benefits. The functional consequences of endogenous Ve, however, remain a subject of investigation.
In this work, we present a model for assessing neuronal coupling via endogenous Ve. Intracellular and extracellular domains in the model are dynamically coupled via the flow of transmembrane current so that Ve is generated by simulated neural activity. This spatially nonuniform endogenous Ve can, in turn, influence the activity of model neurons embedded in the extracellular space. We adopt standard terminology and describe this mechanism of neuron-to-neuron interaction (which occurs solely via generation of and response to endogenous Ve) as ephaptic coupling (Arvanitaki 1942).
In her pioneering study of ephaptic coupling, Arvanitaki expressed “no doubt that the activity of an element in the midst of a cell agglomeration can influence that of its neighbors [via ephaptic coupling]” (Arvanitaki 1942). Classical studies of interactions between side-by-side axons supported her assertion (Arvanitaki 1942; Katz and Schmitt 1940; Ramón and Moore 1978), and recent studies have demonstrated that relatively weak and oscillatory applied Ve (designed to be “endogenous-like”) can enhance spike time synchrony in vitro (Anastassiou et al. 2011; Frölich and McCormick 2010; Radman et al. 2007).
In this work, we present a systematic study of ephaptic coupling in a bundle of model neurons using methods that are an extension of classical cable theory (Rall 1977). By invoking assumptions of cable theory and by considering a population of identical neurons (see materials and methods), we solve the ephaptic coupling problem by computing intra- and extracellular voltages in coupled one-dimensional domains. Using the cable model, we show how millivolt-scale endogenous Ve can induce millivolt-scale perturbations in membrane potential. We then apply these insights to the medial superior olive (MSO) in the auditory brain stem and find that ephaptic coupling can alter (simulated) spike activity in these specialized coincidence detector neurons.
Our approach differs from standard “line-source” or “point-source” approximations in which Ve is computed from simulated neural activity without including any feedback between Ve and neural activity (Holt and Koch 1999; Klee and Rall 1977; Lindén et al. 2011; Reimann et al. 2013). Our method is substantially simplified compared with solvers that couple membrane dynamics of three-dimensional neuron models to Maxwell's equations for extracellular electric and magnetic fields (Agudelo-Toro and Neef 2013; Malik 2011).
We consider a population of identical model neurons that receive identical inputs and that are arranged with spatial symmetry (i.e., equally spaced and oriented parallel to one another). These assumptions appear restrictive, but the approach is inspired by pioneering analyses of endogenous Ve in the olfactory bulb (Rall and Shepherd 1968) and cerebellum (Nicholson and Llinás 1971). We have found this idealized modeling approach useful to describe sound-evoked extracellular voltages recorded in vivo in the auditory brain stem (Goldwyn et al. 2014).
The auditory brain stem is an intriguing test case for studying ephaptic interactions. Neurons in the MSO are believed to generate the auditory brain stem Ve (Biedenbach and Freeman 1964; Clark and Dunlop 1968; Galambos et al. 1959; Goldwyn et al. 2014; Mc Laughlin et al. 2010; Moushegian et al. 1964; Tsuchitani and Boudreau 1964). The dense packing of MSO neurons' dendrites and the presence of a prominent, sound-evoked extracellular voltage field have led to suggestions that ephaptic interactions may be at work in this nucleus (Ashida and Carr 2011; Schwartz 1977). MSO neurons perform a known computation: sound localization via temporally precise coincidence detection of dendritic inputs (see Grothe et al. 2010 for review). We can evaluate the functional consequences of ephaptic interactions in this system by simulating coincidence detection in MSO neuron models in the presence of (simulated) endogenous Ve.
MSO neurons are bipolar (two dendrites with modest branching, extending away from a central soma; Rautenberg et al. 2009). Back-propagating action potentials in the soma are small (Franken et al. 2015; Scott et al. 2007) and are difficult to detect with extracellular and juxtacellular electrodes (van der Heijden et al. 2013; Yin and Chan 1990). It is reasonable, therefore, to begin our study with a passive cable model and neglect contributions of spike-generating Na currents. In this initial analysis we gain insights into ephaptic interactions in the MSO and dendritic bundles in general. We find that the cable population can generate millivolt-scale Ve and that this Ve can induce a millivolt-scale perturbation in the membrane potential of a neuron embedded in the Ve bath. These ephaptic interactions are largest for electrotonically compact cables (i.e., large cable space constant).
The passive cable results illustrate that Ve, because of its spatially distributed nature, can hyperpolarize or depolarize different portions of a “nearby” neuron. As a corollary, when we model ephaptic interactions in the MSO and include spike-generating Na currents, we find that ephaptic coupling can have either “excitatory” or “inhibitory” effects depending on the location of spike initiation in the spatially varying Ve. The relatively modest (millivolt scale) perturbations of membrane potential due to endogenous Ve alter spike initiation threshold, spike timing, and the sensitivity of MSO neurons to arrival times of bilateral inputs. Specifically, we find that ephaptic coupling suppresses spiking activity if spikes are generated near the soma of the MSO neuron model and promotes spiking activity if spikes are generated at locations distant from the soma. Our results establish, in principle, that ephaptic coupling can influence neural processing in this early stage of the auditory pathway.
MATERIALS AND METHODS
Ephaptic Coupling in a Population of Passive Cables
Model formulation.
In the standard (passive) cable theory, spatiotemporal dynamics of membrane voltage Vm(x,t) are governed by the balance of capacitive, leak, and applied currents crossing the cell membrane and diffusion of current within the cell (Rall 1977):
| (1) |
cm is the membrane capacitance per unit length (mF/cm), rm is the resistance across a unit length of membrane (Ωcm), ri is intracellular (axial) resistance per unit length (Ω/cm), Elk is the leak current reversal potential (mV), and iin is input current per unit length (mA/cm). At the ends of the cable, we impose a “sealed-end” (zero axial current) boundary condition by requiring ∂Vi/∂x = 0 for x = 0 or l, where l is the physical length of the cable. In all cable model simulations, we present Vm as its deviation from resting potential (or, equivalently, set Elk = 0 mV). We provide a circuit diagram schematic to illustrate the balance between intracellular current and membrane current for a small patch of membrane in Fig. 1B.
Fig. 1.
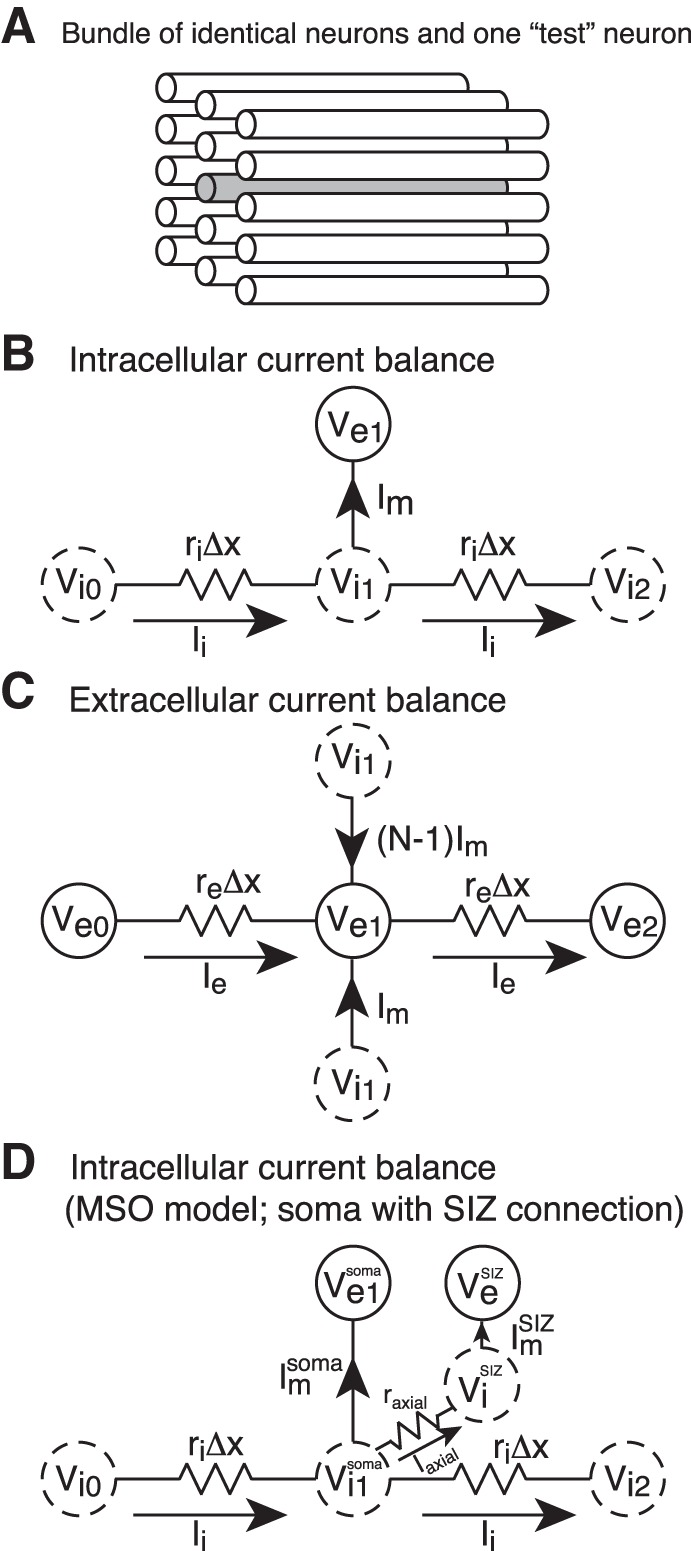
A: a population of identical neurons arranged with spatial symmetry (white cables) generates extracellular voltage (Ve). An additional “test neuron” (gray cable) is embedded in the population. The test neuron activity does not contribute to Ve, but its transmembrane potential (Vm) can be influenced by it. Voltage dynamics are described by a passive cable equation or by a model for medial superior olive (MSO) neurons (see text for details). B: circuit diagram schematic for current balance relations in a local patch of membrane. Locations in the intracellular domain are marked with dotted circles; locations in the extracellular domain are marked with solid circles. Conservation of current inside a cable segment of a model neuron requires a balance between intracellular current (Ii) and transmembrane current (Im). In the passive cable model, Im is composed of capacitive and leak current. In the MSO model, Im also includes Ih and voltage-gated IKLT. C: conservation of current outside the model neuron requires a balance between extracellular current (Ie) and Im. Key assumptions in the model are that Ie is confined to a 1-dimensional volume conductor and that Ve is generated from N identical neurons; thus Ie is balanced by N × Im in the schematic. D: in some simulations a spike initiation zone (SIZ compartment) is added to a test neuron (see results in Fig. 11 and Fig. 12). In these cases, intracellular current in the test neuron is modified relative to the schematic in A to also include axial current flow between the soma of the test neuron and the SIZ. We allow the voltage exterior to the SIZ (VeSIZ) to depend on the location of the SIZ, but we do not vary the axial resistance between soma and SIZ (raxial) (see text for details). The test neuron does not contribute to Ve, so the extracellular circuit is unchanged.
To gauge the effect of Ve on Vm, one can substitute Vm + Ve for Vi in Eq. 1:
| (2) |
In this formulation, the effect of Ve on the dynamics of Vm appears as a spatially distributed current source proportional to the second spatial derivative of Ve. Positive curvature of Ve acts locally as a depolarizing (“excitatory”) current. This is the basis for the activating function method (Rattay 1986), a heuristic used to approximate the effect of applied extracellular fields on neurons (Rattay 1999). Holt and Koch (1999) refer to the second spatial derivative of Ve divided by ri as a “fictitious distributed current (the ephaptic current).”
A common modeling assumption is that extracellular voltage has a negligible impact on the cell's voltage dynamics. In that case, since Vm = Vi − Ve, one sets Ve = 0 for all x (no “ephaptic current”) and lets Vi = Vm. We are specifically investigating how Ve affects neuronal dynamics via ephaptic interactions, so we retain Vi in Eq. 1 as a quantity distinct from Vm.
We now describe how the activity of neurons generates Ve and how this Ve feeds back and influences Vm in an idealized model of N identical and parallel cables. Figure 1A illustrates the idealized population of neurons (drawn as cables). If all cables receive similar input iin (in terms of temporal dynamics and spatial location), then the spatiotemporal distribution of membrane currents will be similar across the population. As a consequence, Ve in the region surrounding any one cable will be similar to Ve surrounding any neighboring cable in the population. In other words, the gradient of Ve (which is proportional to the current flow in the extracellular region) will be directed, for the most part, parallel to the orientation of the cables. We thus make the assumption that the extracellular space can be described as a one-dimensional volume conductor. This reduces the problem of modeling extracellular interactions to two, coupled one-dimensional domains: the inside of the cable (intracellular core conductor) and a thin layer surrounding the cable (extracellular volume conductor).
In the one-dimensional extracellular region, there is a current balance relationship composed of the sum of all membrane currents in the population of N cables
and current flow along the one-dimensional extracellular pathway , where re is the resistance per unit length (Ω/cm) in the extracellular region in the direction parallel to the cables. The superscript (n) is the index of neurons in the population. We provide a circuit diagram schematic to illustrate the balance between extracellular current and membrane current for a small patch of membrane in Fig. 1C.
Under the assumption described above, iin(n) (and, therefore, Vm(n)) are similar for all N cables, so we divide by N and obtain the population-averaged current balance relation
| (3) |
Vm and iin represent population-averaged quantities, for instance . Note that the intracellular current balance relation in Eq. 1 still holds if Vm, Vi, and iin are population-averaged quantities, so going forward we will maintain this mean-field perspective. A more general formulation would allow the applied current iin to differ in the intracellular and extracellular domains (cf. Tuckwell 1988). We consider iin to be transmembrane current (synaptic current, for example), so we require the applied intra- and extracellular currents to be identical.
Extracellular space extends beyond the ends of the cables, and volume conduction allows Ve to spread to a distant electric ground at which Ve = 0 mV. We impose a mixed boundary condition that describes the flow of current to electric ground along a one-dimensional current pathway of length dg. These boundary conditions are dg ∂Ve/∂x − Ve = 0 at x = 0 and dg ∂Ve/∂x + Ve = 0 at x = l.
Using a standard reparameterization, we define the time and space constants of the cable (Rall 1977): τ = cmrm and λ2 = rm/ri. In addition, we introduce a coupling parameter κ = Nre/ri. The governing equations for the coupled system of intracellular and extracellular voltages are
| (4) |
| (5) |
| (6) |
| (7) |
We will often report results in terms of the nondimensional spatial variable X = x/λ and the cable length L = x/λ. Note that Vi and Ve are independent variables and Vm, the membrane potential, is defined as the difference of these: Vm = Vi − Ve.
To further investigate ephaptic coupling, we embed an additional neuron, with possibly different cable properties and input current, into the surrounding Ve (Fig. 1A). We ignore its O(1/N) contribution to Ve and the population-averaged Vm, but since membrane potential is the difference between intracellular and extracellular voltage, Ve perturbs this additional neuron's membrane potential. We refer to this as a “test neuron,” and its membrane and intracellular voltages satisfy Eq. 4 and the boundary condition in Eq. 6. We use the ^ accent to indicate parameter values for the test neuron that differ from the cable population. We note that there is no spike generating mechanism in the passive cable model. We view this as a subthreshold model and are neglecting any contributions of spiking activity to Ve and ephaptic interactions.
Solution method.
Equations 4–7 form a system of partial differential algebraic equations (PDAEs) and, in general, require special solution methods (Lucht et al. 1997a, 1997b). For simple cases (constant or sinusoidal input current iin injected at a single point on the cable), we reformulate and solve these equations numerically as a boundary value problem. For general current waveforms (and voltage-gated membrane currents), numerical solution methods are available to integrate these equations in time (see solution method for MSO model below).
In response to a constant current input, the system will reach a steady state with ∂Vm/∂t = 0. This eliminates the time dependence in Eqs. 4 and 5. Steady-state spatial profiles of Vi and Ve satisfy a linear, constant coefficient system of ordinary differential equations:
| (8) |
| (9) |
| (10) |
with boundary conditions given in Eqs. 6 and 7. Derivatives in these equations are with respect to the spatial variable x, and the ^ accent indicates parameters and variables associated with the test neuron. We solve this boundary value problem with the function bvp4c in MATLAB (R2012b, MathWorks).
We can also formulate a boundary value problem to describe the frequency-response characteristics of the coupled intracellular-extracellular system. In response to the stimulus iin = i0exp(i2πft)δ(x − x0), intracellular and extracellular voltages are of the form Vi(x) = Ui(x)exp(i2πft) + Elk and Ve(x) = Ue(x)exp(i2πft) and solve the following ordinary differential equations:
| (11) |
| (12) |
| (13) |
The boundary conditions in Eqs. 6 and 7 are applied to the amplitude variables U(x), and we solve these equations with bvp4c in MATLAB. We report the amplitude of the oscillatory response to iin as the absolute value of U(x) and use the phase command in MATLAB to recover the phase of the response. Note that the intracellular amplitude Ui measures the deviation of the intracellular voltage from the resting potential Elk. In all simulations, we present cable model Vm responses in terms of their deviation from rest (equivalent to setting Elk = 0 mV in above equations).
Ephaptic Coupling in an Idealized Model of the Medial Superior Olive
Model formulation.
Neurons of the MSO are thought to generate prominent extracellular voltages in response to acoustic stimuli (e.g., Galambos et al. 1959). In previous work, we developed an MSO model that predicted spatiotemporal features of these extracellular voltage responses (Goldwyn et al. 2014). Here we adapt this model to test ephaptic interactions among MSO neurons.
The construction of the MSO model is similar to the cable model introduced above. We use one neuron to represent a population of neurons that generate Ve and are dynamically coupled to Ve. We include a second neuron, referred to as a “test neuron,” to highlight how the membrane potential of a neuron is perturbed when it is embedded in an endogenously generated Ve. The MSO neuron model differs from the passive cable discussed above because it includes voltage-gated membrane current in addition to the leak current and a nonuniform morphology (two dendrites, connected to a soma). The properties of the test neuron and the neuron that generates Ve are identical, with one exception: in some simulations, we add a compartment to the test neuron that includes spike-generating Na current. This allows us to probe the effects of ephaptic coupling on spike initiation and spike timing in the MSO neuron model.
These nonlinearities and inhomogeneity interfere with the construction of a population-averaged model. However, we have argued previously that a mean-field perspective is justified for describing in vivo MSO responses to pure-tone stimuli (Goldwyn et al. 2014). Specifically, we noted that MSO neurons have a relatively simple morphology (bipolar dendrites with minimal branching) and are oriented roughly in parallel. Moreover, early stages of the auditory pathway are specialized to deliver inputs to MSO neurons with high levels of phase locking (e.g., Joris et al. 1994). We idealize these anatomical and physiological observations to argue that MSO neurons are arranged with spatial symmetry and their inputs arrive in synchrony with one another across a local subpopulation. These conditions of synchrony and symmetry justify the use of a simplified one-dimensional volume conductor model (Rall and Shepherd 1968).
The current balance relations for the intra- and extracellular domains of the MSO model (in terms of current density) are
| (14) |
| (15) |
with the same boundary conditions as the passive model (Eqs. 6 and 7). These equations and the dynamics of the gating variables w and z are adapted from a biophysically based model of an MSO neuron first presented by Mathews et al. (2010) (see Eqs. 16xref>–19 below). Vm, Vi, and gating variables in these equations are representative of the activity of a population of N neurons (mean-field perspective), but we use the coupling coefficient κ in Eq. 15 as we did in Eq. 5 of the passive cable model to avoid the introduction of unknown parameters for extracellular resistance and population size. We discuss plausible values for κ below (see Fig. 2 and explanation in results).
Fig. 2.
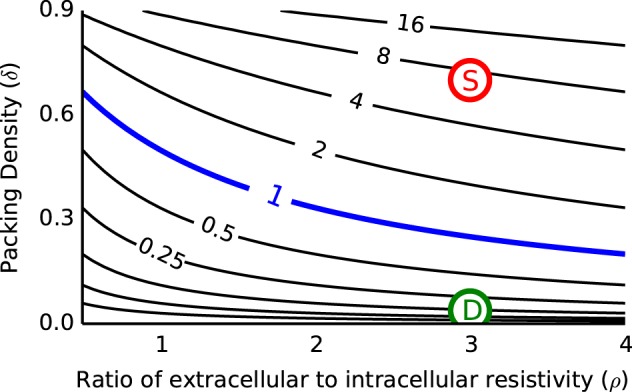
Contour plot of the coupling parameter κ for plausible values of packing density (δ) and resistance ratio (ρ). Coupling parameter is κ = ρδ/(1 − δ), contour lines have logarithmic spacing (powers of 2). We use κ = 1 (blue contour) in simulations of the cable model with ephaptic coupling. Estimates for κ in the MSO model are marked with colored circles. κ is larger surrounding the soma (7, red S) than the dendrite (0.12, green D) since the larger diameter of the soma implies a larger packing density (δ = 0.7 for soma and 0.038 for dendrites; ρ = 3 throughout).
The neuron model consists of two dendrites extending away from a central soma. Each dendrite is a 150-μm-long cylinder with diameter d = 3.5 μm. The soma is a cylinder of length 20 μm and diameter d = 20 μm. Membrane capacitance is Cm = 0.9 μF/cm2, intracellular (axial) resistivity is Ri = 200 Ωcm, and leak conductance density is Glk = 0.3 mS/cm2. The neuron model includes low-threshold K (KLT) current and hyperpolarization-activated cation (h) current. Maximal KLT conductance density is 17 mS/cm2 in the soma and 3.58 mS/cm2 in the dendrites, and maximal h conductance density is 0.86 mS/cm2 in the soma and 0.18 mS/cm2 in the dendrites. These parameters correspond to the “step-gradient” model in Mathews et al. (2010).
The KLT current has a voltage-gated activation variable w and an inactivation variable z that evolve according to du/dt = [u∞(Vm) − u]/τu(Vm), where u = w or z and
| (16) |
| (17) |
| (18) |
| (19) |
In these and all equations time is in units of milliseconds and Vm is in units of millivolts. The gating variable for the h current evolves slowly (timescale on the order of hundreds of milliseconds; see Khurana et al. 2011). We make the simplification, therefore, that Gh remains at a constant value in all simulations.
In most simulations the input current Jin is a simulated synaptic input with alpha-function conductance:
| (20) |
Gsyn is the maximal synaptic conductance density (mS/cm2), t0 is the onset time of the synaptic event, τsyn is the synaptic time constant, and Esyn is the reversal potential. Excitatory inputs to the MSO neurons are fast (Golding and Oertel 2012) and primarily target dendrites (Couchman et al. 2012). We set the synaptic time constant in Eq. 20 to τsyn = 0.2 ms, consistent with in vitro physiology and previous modeling studies (Fischl et al. 2012; Jercog et al. 2010; Myoga et al. 2014), and the reversal potential is 0 mV. In some simulations the onset times t0 of synaptic events are fixed (with a specific timing difference between inputs on the two dendrites, for instance), and in other simulations the onset times are drawn from Poisson processes to approximate more realistic input patterns. Exceptions are the simulations of spike time synchrony and time-difference tuning curves (see Fig. 12) in which the alpha-function conductance in Eq. 20 is replaced with a half-wave rectified sine function that is meant to approximate the population-averaged conductance input to MSO in response to pure-tone stimuli. We place excitatory inputs on either dendrite ∼125 μm from the soma. MSO neurons also receive inhibitory inputs (Grothe and Sanes 1993, 1994) that primarily target the soma (Couchman et al. 2012). We omit these in the present study. We have explored the contribution of inhibition to simulated Ve responses in an MSO model in previous work (Goldwyn et al. 2014).
Fig. 12.
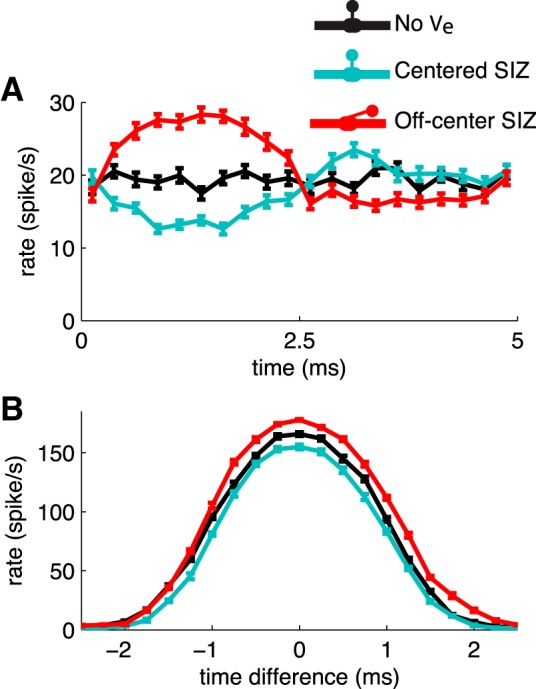
Ephaptic effects on spike timing and coincidence detection. A: cycle histogram of test neuron response to bilateral trains of excitatory synaptic events. Event times of input trains are generated from independent homogeneous Poisson processes. Average firing rates calculated in 0.25-ms bins from 50 simulations of responses to 10-s-long input trains. Error bars are SE. B: time-difference tuning curves for test neuron's response to bilateral trains of excitatory synaptic events. Event times of input trains are generated from independent inhomogeneous Poisson processes. Poisson rate for the underlying Poisson process is a 200-Hz half-wave rectified sine function with an average event rate of 100 events/s. The timing difference of the half-wave rectified sine Poisson rates is shown on the x-axis with 0-ms time difference representing rate functions to both dendrites that are in phase relative to one another and relative to the input to the MSO population. Firing rates were computed from responses to 10-s-long inputs. Mean and SE (error bars) were obtained by dividing these responses into 10 segments and counting spikes in each 1-s-long subintervals. Model configurations and color code are same as in Fig. 11D. Input to MSO population (generators of Ve) is 200-Hz half-wave rectified sine function excitation with same bilateral time differences as input to test neuron.
To highlight ephaptic coupling in the MSO model we embed an additional “test” neuron in the Ve field. This single neuron's contribution to Ve is O(1/N), so it can be neglected. We keep the properties of this test neuron the same as the properties of the neurons that generate the endogenous Ve (see Eqs. 14 and 15), but in some simulations we attach an additional compartment to the soma that contains spike-generating Na current. We use this test neuron to evaluate how ephaptic interactions alter spiking activity in the MSO neuron model.
The additional compartment represents the putative spike initiation zone (SIZ). This likely corresponds to the axon initial segment and/or a proximal node of Ranvier (see Lehnert et al. 2014 for a recent computational study of spike initiation in an MSO neuron model). The membrane dynamics in the SIZ are
| (21) |
ViSIZ is the membrane potential in the SIZ, VmSIZ is the SIZ membrane potential, and Visoma is the intracellular potential at the soma. The SIZ is influenced by the local extracellular voltage because VmSIZ = ViSIZ − Ve, where Ve is the extracellular voltage at the location of the SIZ.
The dynamics of the Na current (m and h variables) are modified from the Rothman and Manis model (2003). They are adjusted for a temperature of 35°C (Khurana et al. 2011), and the gating properties of the Na inactivation variable h are “left-shifted” by 6 mV. This modification is motivated by in vitro measurements (Scott et al. 2010) and enhances the phasic character of the model neuron (Huguet et al. 2012).
| (22) |
| (23) |
| (24) |
| (25) |
The SIZ is assumed to be a small patch of membrane (1-μm diameter and 1-μm length, surface area is SSIZ = 3.14 μm2) with a dense concentration of Na channels. The capacitance per unit area is Cm = 0.9 μF/cm2. The leak conductance density is GlkSIZ = 200 mS/cm2; the maximum Na conductance density is GNaSIZ = 75,000 mS/cm2. These parameter values seem large, but we view this compartment as a phenomenological representation of a spatially extended structure: the axon and axon initial segment containing multiple possible sites of spike initiation. The parameter values were chosen so that spiking dynamics in the model (including the amplitude of the backpropagating action potential in the soma) are similar to in vitro recordings (Scott et al. 2007).
The current balance equation for the soma of the test neuron is also altered to account for axial current flow to and from the SIZ with gaxial = 60 nS. We provide a circuit diagram schematic in Fig. 1D to illustrate this modified current balance relation. Reasonable assumptions are that the initial segment's diameter is ∼1–1.5 μm (Lehnert et al. 2014) and the axial resistance connecting the SIZ to the soma is 200 Ωcm. For these values and gaxial = 60 nS, the implied length of the soma-to-SIZ connection is ∼6–15 μm, consistent with the anatomy of the initial segment reported by Lehnert et al. (2014). In our simulations we take a phenomenological view of the SIZ and allow its location in the Ve field to be more distant from the soma. In particular, we compare two configurations: a “centered” SIZ model in which the SIZ is aligned with the soma and an “off-center” SIZ model in with the SIZ is aligned with a location on the right dendrite that is 117.5 mm from the soma center. The intracellular connection between the SIZ and the soma (i.e., gaxial) remains the same in all simulations regardless of the location of the SIZ in the extracellular domain.
We assume that spikes do not contribute significantly to Ve. We do not, therefore, include spikes in the MSO population and do not take into account spikes generated in the SIZ of the test neuron when computing Ve. This assumption is based on a consensus that postsynaptic membrane currents in the MSO generate the prominent, ongoing sound-evoked Ve in the auditory brain and spikes do not significantly contribute to it (e.g., Galambos et al. 1959; Mc Laughlin et al. 2010). Physiological observations support the assumption that spikes do not contribute to Ve. Back-propagating action potentials in the soma of MSO neuron are small (∼20 mV) when measured in vitro (Scott et al. 2007) and in vivo (Franken et al. 2015) and can be difficult to detect in extracellular recordings (Yin and Chan 1990) and juxtacellular recordings (van der Heijden et al. 2013).
Solution method.
As mentioned above, Eqs. 14 and 15 represent a system of PDAEs. Because of the voltage-gated ion currents and the synaptic (conductance) input, the coupling between intra- and extracellular voltages is nonlinear and Vm dependent. To solve these equations, we discretize the spatial domain in small bins of length Δx. This converts the PDAEs into a system of differential algebraic equations (DAEs) that can be solved with appropriate software (we use SUNDIALS, available at http://computation.llnl.gov/casc/sundials/main.html; Hindmarsh et al. 2005). The discretization is analogous to the compartmental method for computing Vm dynamics in a spatially extended neuron model (Segev and Burke 1998), but in our formulation, intracellular and extracellular compartments reside at each point in discretized space and are coupled to one another. The numerical method is designed to conserve the flow of current in and out of each compartment (see Fig. 1, B–D), so it is necessary to define intracellular, extracellular, and membrane currents (Ii, Ie, Im) (units of mA).
Let x identify the spatial location of a compartment of width Δx. Then we denote the intracellular (axial) current flow from an adjacent compartment into the compartment at x as
| (26) |
and extracellular current flow as
| (27) |
For notational simplicity we have substituted ri for 4Ri/πd2 in these and subsequent equations. Note that, unlike the cable model, the diameter d of each compartment is not uniform (soma is larger than dendrites). As a result, ri and the coupling parameter κ are larger in the soma compartments than in the dendrite compartments. For ease of notation we do not explicitly indicate this x-dependence of ri and κ.
The net flows of intra- and extracellular currents at location x satisfy current balance relations with the transmembrane current Im(x,t):
| (28) |
| (29) |
The transmembrane current Im(x,t) at location x (by convention, outward flow of positive ions is positive current) consists of capacitive, leak, ionic, and input currents:
| (30) |
S denotes surface area of the MSO compartment, and it depends on x; it is larger in soma compartments than in dendrite compartments. Iin is the input current in units of milliamperes.
By identifying the right side of Eq. 30 with the second-order differences in Eqs. 28 and 29, we obtain a set of equations that dynamically couple intracellular and extracellular voltage via the membrane potential. We impose boundary conditions as in the cable model: a “sealed end” for Vi in the intracellular domain and a linear decay of Ve to ground (0 mV) at a distance of 1 mm from the ends of the dendrites. To include the test neuron with SIZ, we solve two versions of the intracellular model, one for the population MSO response (the generators of Ve) and one for the test neuron. The test neuron includes coupling to its SIZ given by Eq. 21. The SUNDIALS numerical solver steps forward in time while maintaining these current balance relations by using a variable order, variable coefficient implicit method (Hindmarsh et al. 2005). We used a relative tolerance of 10−6 and an absolute error tolerance of 10−8 in the solver and obtained the solution at 1-μs time steps.
MATLAB code is available on the ModelDB repository (accession no. 183948) and includes example solutions of the MSO model and user-friendly simulation code for the passive cable model.
RESULTS
Ephaptic Interactions in Passive Cables
Remarks on coupling parameter κ.
The coupling parameter κ = Nre/ri dictates the strength of interactions between Ve and Vm. The standard cable theory assumes that Ve is spatially uniform and negligible. This is the limiting case of κ → 0. To estimate a range of plausible nonzero values of κ, we introduce two new parameters: the packing density δ of the population of N cables and the ratio of extracellular to intracellular volume resistivities ρ = Re/Ri. Let Ai be the cross-sectional area of each cable and Ae the cross-sectional area of the extracellular space (i.e. the total cross-sectional area of the brain region under consideration is NAi + Ae). Then, the relations ru = Ru/Au (u = i, e) and δ = NAi/(NAi + Ae) allow us to express the coupling parameter as κ = ρδ(1 − δ). The advantage of this formulation is that we can estimate bounds on ρ and δ.
Extracellular resistivity Re has been measured in a number of biological tissues and animals (e.g., Geddes and Baker 1967). Based on these and other experiments, modeling studies typically use values of ρ that range from ∼1 to 4. In simulations of neuron-Ve interactions, Holt and Koch (1999) used ρ = 2.2 (Ri = 150 Ωcm and Re = 330 Ωcm). Recent modeling studies of cortical local field potentials have used similar values: ρ = 2.2 in Lindén et al. (2010) and ρ = 3.5 in Reimann et al. (2013), for example.
The packing density δ of a local population of neurons depends on their spatial arrangement and morphology. For the idealized case of uniform cables oriented in parallel to one another we can provide a theoretical upper bound by treating the population of cables as an example of circle packing in a plane (when viewed in cross section). In this case, the theoretical upper bound for δ is ∼0.9 (Weisstein). If the extracellular domain is a circle, then the upper bound for δ would be closer to 0.8 for a moderate (<100) number of cables (Graham et al. 1998). Figure 2 shows a contour plot of κ as a function of ρ and δ in these parameter ranges. For small packing densities and small ρ the coupling strength approaches 0, but κ exceeds 1 in over half of the parameter space and can reach values as large as ∼15. We include ephaptic effects in the cable model by setting the coupling parameter to an intermediate value in the plausible range of values shown in Fig. 2. Specifically, we set κ = 1 for simulations that include ephaptic effects, unless otherwise stated. Our estimates for κ in the MSO model are also within this plausible range. They are marked in Fig. 2 and discussed below.
Responses to constant current: initial observations.
We begin our investigation of ephaptic interactions by studying responses to constant current applied at a single point along the cable. Responses in this scenario are solutions to the boundary value problem for the coupled intra- and extracellular voltages at steady state given in Eqs. 8–10. The space constant in MSO neurons is roughly the length of one dendrite (Mathews et al. 2010). We use the cable model to gain initial insights that can be applied to the MSO, so we set the space constant λ in the cable model to be one-half the physical length of the cable, unless otherwise indicated. Steady-state solutions do not depend on the cable time constant τ.
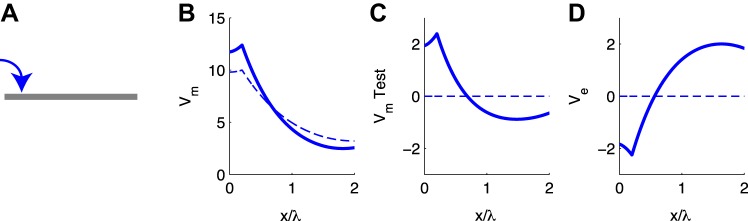
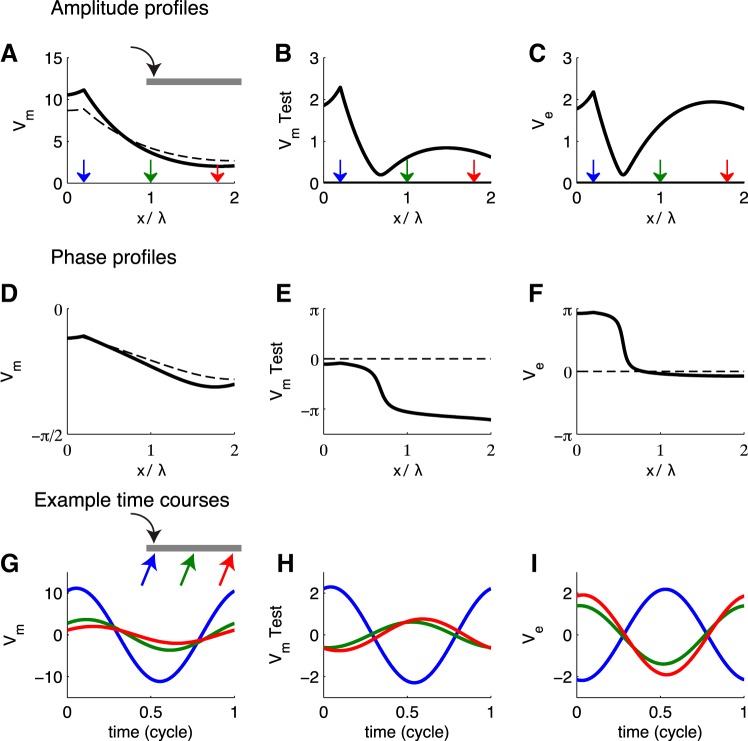
We first injected a constant, depolarizing current to a site near the left end of the cables in the population (input location is x = 0.2λ; see schematic in Fig. 3A). The population-averaged Vm is maximal at the site of the input and attenuates with distance along the cable (Fig. 3B). The stimulus amplitude in these simulations is arbitrary; response amplitude scales linearly with stimulus amplitude for current injection to passive cables.
Fig. 3.
Passive cable response to constant input current. Responses without ephaptic coupling (κ = 0) are shown with dashed line; responses with ephaptic coupling (κ = 1) are shown with solid line. A: input location is near 1 end of cable. B: steady-state depolarization of population-averaged Vm. C: steady-state V̂m response of “test neuron” that is coupled to population of cables via the extracellular voltage. Vm in B and C are plotted as deviations from resting potential. D: steady-state Ve response produced by population of cables. x-Axis in all panels is distance along the cable normalized by the cable space constant λ (half the physical length of the cable).
The effect of coupling all cables in the population via Ve is evident in the difference between the population-averaged Vm response in the absence of coupling (κ = 0; Fig. 3B) and the response with ephaptic coupling (κ = 1; Fig. 3B). Ephaptic coupling increases the membrane depolarization Vm near the stimulation site, acting to increase the input resistance. At locations distant from the input site, ephaptic coupling decreases the depolarization of Vm. The net result of ephaptic coupling is to increase the rate at which Vm attenuates with distance along the cable. In other words, ephaptic coupling decreases the cable space constant, effectively increasing the electrotonic length of the cable. This effect of Vm-to-Ve coupling was noted by Rall in his example of an “axon in oil” (Rall 1977). In that analysis of an infinite cable surrounded by a thin extracellular layer, Rall noted that the space constant of the cable decreases as extracellular resistance increases according to , or equivalently , where d is the cable diameter.
We highlight the ephaptic effect by showing the response of a “test neuron” embedded in the Ve field generated by all neurons in the population. The test neuron's membrane potential V̂m (more precisely, the deviation of V̂m from rest) displays the same changes discussed above: a local depolarization near the site of input current and hyperpolarization at more distant locations on the cable (Fig. 3C). This cable has properties identical to the cables whose population-averaged Vm is shown in Fig. 3B. The test neuron does not receive any current injection. The Ve surrounding the test neuron is the sole “input” that determines the spatial profile of V̂m in Fig. 3C. We point out that ephaptic coupling hyperpolarizes V̂m at the center of the cable (V̂m < 0 mV at x/λ = 1). This anticipates a main finding in our MSO simulations: Ve produced by dendritic excitation can have a hyperpolarizing or “inhibitory” effect on the soma of a “nearby” MSO neuron.
We can understand the ephaptic effects in these simulations by examining the spatial profile of Ve in Fig. 3D. The input current is a transfer of positive ions from the extracellular domain into the interior of the population-averaged cable, and thus Ve is negative near the input site at x/λ = 0.2; the input acts as a current sink to the surrounding extracellular domain. Recall from Eq. 2 the heuristic that the second spatial derivative of Ve acts as a distributed “ephaptic current” to perturb membrane potential (neglecting boundary effects). The second spatial derivative of Ve at x/λ = 0.2 is positive, and thus the effect of the spatially nonuniform Ve is to depolarize V̂m near the input location. Conservation of current in the system requires that the flow of current from the extracellular domain into the cables (i.e., the stimulus current) must be returned back to the extracellular domain at other locations along the cable. As a result, Ve is positive at spatial locations distant from the stimulus site. At these distant locations the extracellular space draws current out of the test neuron and hyperpolarizes V̂m; the efflux acts as a current source to the extracellular surround.
In these simulations, a relatively modest input current and coupling parameter (∼12-mV maximum depolarization in population-averaged response, κ = 1) produces Ve amplitudes of ±2 mV. The amplitude of the response of the test neuron is similar to Ve: approximately 2.5-mV maximum depolarization and approximately −1-mV hyperpolarization. This result is consistent with the observation made by Anastassiou et al. (2010) that the spatial frequency of Ve must be sufficiently large, relative to the electric and physical lengths of the cable, to perturb V̂m. More precisely, they showed that a spatially inhomogeneous Ve with spatial frequency fs has an O(1) effect on Vm of a passive cable if the dimensionless angular spatial frequency of Ve (Ω = 2πfsλ) is larger than 1 and 1/L, where L is the cable's physical length normalized by its space constant λ. Both conditions are satisfied in these simulations because L in our cable model is twice the cable space constant. This implies that 1/L is one-half (less than one). In addition, the spatial profile of Ve in Fig. 3D (although not exactly a sine wave) appears somewhat like a half-cycle of a periodic waveform. We can say, therefore, that fsλ ≈ 1 and thus Ω > 1.
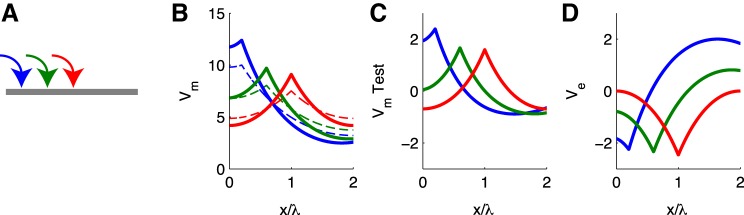
If we move the stimulation site to different locations along the cable, the spatial angular frequency of Ve appears to always be about one-half to one times the length of the cable (Fig. 4D). Thus the effect of Ve on V̂m remains roughly O(1) in all cases, as shown in the responses of the test neuron in Fig. 4C. This generic feature of the Ve spatial profile generated by the cable population is due to conservation of current and the geometry of the one-dimensional volume conductor. Depolarizing input current reduces Ve near the input site, and the return of current back to the extracellular space restores Ve to zero or positive values at locations of the cable distant from the input site. These Ve effects can also be seen in the population-averaged Vm responses by comparing responses that include ephaptic coupling to those that do not include ephaptic coupling (Fig. 4B).
Fig. 4.
Passive cable response to constant input current for varying input location. Input location is near end of cable (blue, same as Fig. 3), intermediate (green), or at center of cable (red). Format same as in Fig. 3.
For the different input locations in Fig. 4, the spatial pattern of Ve changes dramatically in the extracellular region beyond the ends of the cable (not shown). In these simulations, the distance from the ends of the cable to electric ground is two times the space constant (i.e., same as physical length of cable). For off-center inputs, Ve is nonzero beyond the ends of the cable as it decays linearly to 0 mV at electric ground. For inputs to the center of the cable, Ve is 0 mV at the ends of the cable and remains zero at all spatial locations beyond the ends of the cable. This is reminiscent of a “closed-field” configuration. The responses to off-center inputs have Ve spatial profiles that extend broadly beyond the ends of the cables and would be classified as an “open-field” configuration (Lorente de Nò 1947). We note that for this linear problem (passive cable with current input), a more complicated spatial pattern of inputs can be constructed by taking the appropriate sum of responses to “point current” inputs.
Ephaptic interactions are greatest for electrotonically compact cables.
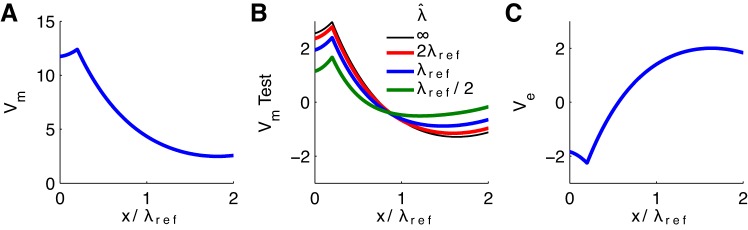
The cable properties of a neuron model influence the amount to which its membrane potential is perturbed by a fixed, applied Ve. In particular, previous studies of passive cables embedded in Ve found that the effect of Ve on Vm increases with the cable space constant (sinusoidal Ve in Anastassiou et al. 2010; linear Ve in Frölich and McCormick 2010). We show in Fig. 5 that the same result holds for endogenous Ve generated in response to constant current input.
Fig. 5.
Passive cable response to constant input current for varying space constant λ̂ of test neuron. A: steady-state depolarization of population-averaged Vm; same as Fig. 3B. B: steady-state response of “test neuron” V̂m. Space constant λ̂ of test neuron is varied so that it is smaller than (green), the same as (blue), or larger than (red) space constant of cables in population. Space constant for cables in population is λref (half the physical length of the cable) in all simulations. C: steady-state Ve response produced by population of cables; same as Fig. 3D. x-Axis shows dimensionless distance along cable relative to the reference space constant (half the physical length of all cables).
We keep the space constant of the cable population identical in all simulations (twice the physical length of the cables in the population) and obtain population-averaged Vm and Ve responses to constant, depolarizing current injection (Fig. 5, A and C). This value of the space constant is our “reference” space constant λref. The x-axis in all panels of Fig. 5 shows distance along the cables normalized by λref.
Keeping the properties of the cable population unchanged, we vary the space constant λ̂ of the test neuron and observe changes in the test neuron's response to endogenous Ve. Specifically, we show that compact cables are more susceptible to ephaptic interactions (Fig. 5B). The value for the space constant used in previous simulations is half the physical length of the cable (denoted as λref). If the space constant of the test neuron is reduced to half this value, i.e., an electrically longer cable, the ephaptic effect on V̂m decreases. If the space constant is twice as large as the reference value, i.e., an electrically shorter cable, the ephaptic effect on V̂m increases.
These changes of V̂m with space constant reflect that the test neuron's response to Ve is a balance between local membrane currents that drive the membrane potential back to rest (0 mV in these figures since V̂m is plotted as deviation from rest) and axial intracellular currents that drive V̂i toward a constant spatial profile. In the limit of an electrotonically compact test neuron (large space constant), V̂i approaches a uniform spatial profile because intracellular current is easily redistributed along the cable. More precisely, for large λ̂ the deviation of V̂m from Elk for the test neuron approaches . This value, which represents the upper bound on how much Ve can perturb V̂m of the test neuron, is shown as a thin black line in Fig. 5B.
In the opposite limit of an electrotonically long test neuron (small space constant), the local membrane currents dominate (relative to axial current flow). V̂m remains near its resting potential over most of the cable but is perturbed near the input site and cable terminals. These observations regarding the sensitivity of the test neuron to Ve also match our intuition from thinking of Ve as a distributed “ephaptic current.” Recall Eq. 2: an increase of ri (by reducing axial conductance, for instance) decreases the amplitude of the term . Thus neurons with small space constants due to large internal resistance ri are unresponsive to Ve.
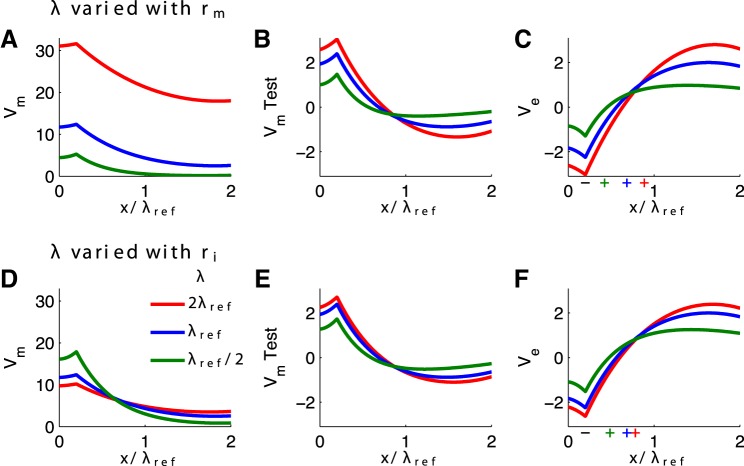
A novel feature of our model is that Ve is not imposed as an exogenous input. Ve is generated endogenously by the activity of a population of cables. We can also explore, therefore, how the spatial profile of Ve depends on the space constant λ of the population that generates Ve. We vary λ in two ways: by altering the membrane resistance rm (Fig. 6, A–C) or by altering the intracellular (axial) resistance ri (Fig. 6, D–F). Recall that the coupling strength is inversely proportional to ri, so for Fig. 6, D–F, we changed κ to 1/4 for the case of small λ and κ = 4 for the case of large λ.
Fig. 6.
Passive cable response to constant input current for varying space constant of the cable population. The test neuron space constant is fixed at the reference value (half the physical length of the cable, denoted λref) in all simulations. Space constants of the cable population are varied by changing rm (A–C) or ri (D–F). A and D: steady-state depolarization of population-averaged Vm. B and E: steady-state V̂m response of test neuron. C and F: steady-state Ve response produced by population of cables. Minus signs mark the location of the current sink (current injection). Plus signs mark the center of mass of source currents (see text for explanation). x-Axis shows dimensionless distance along cable relative to the reference space constant.
We find that the population-averaged Vm responses change dramatically depending on which parameter is manipulated (compare red curves in Fig. 6, A and D, for instance, and note that the input current is identical in all simulations). These changes in Vm are consistent with differences in the input resistance to these cables. In Fig. 6, A–C, increases of λ are associated with increases of rm and, consequently, increases of the cable input resistance (Rall 1977). In the Fig. 6, D–F, increases of λ are associated with decreases of ri and, consequently, decreases of the cable input resistance.
Although population-averaged Vm responses change with these parameter manipulations, the test neuron V̂m responses (Fig. 6, B and E) and the extracellular Ve responses (Fig. 6, C and F) remain similar for equal values of λ. We expect Ve responses to be similar for identical λ values because λ determines the spatial distribution of membrane current, which, in turn, generates the Ve response. In other words, λ determines the steady-state spatial profile of Ve, not the individual values of rm or ri.
The amplitude of Ve increases with λ, as seen in Fig. 6, C and F. We provide an intuitive explanation by thinking of the cable population as a collection of current dipoles: current injection at one site on a cable is a sink, and the rest of the cable acts as a source to return current to the extracellular space. The analogy to a current dipole is loose because the return “source” currents are not localized; they are distributed across the entire cable. To illustrate this analogy, we marked the location of the current sink (site of current injection) by a black minus sign in Fig. 6, C and F. We then computed the center of mass of source currents, where we defined source currents by . We marked these locations with colored plus signs. For increasing values of λ, the resistance of the intracellular (axial) pathway decreases relative to membrane resistance. As a result, the center of mass of source currents moves away from the location of the current sink with increases to the cable space constant. By analogy to a current dipole, we say that the dipole moment increases with λ.
In these simulations, the space constant of the test neuron is set to the reference value (half the physical length of the cable). Nonetheless, because of changes in the Ve amplitude discussed above, the perturbation of V̂m from rest increases for larger values of the cable population space constant (Fig. 6, B and E). If we were to allow the test neuron space constant to covary with the cable population, we would see a “double effect” of ephaptic coupling. As the space constant of the population and test neurons increased together, the test neuron would be more susceptible to the effects of Ve (recall Fig. 5B) and the amplitude of Ve would increase (Fig. 6, C and F).
Responses to sinusoidal current: initial observations.
We have gained helpful initial insights by studying steady-state responses to constant current injection, but ultimately we are interested in dynamic ephaptic interactions (e.g., responses to trains of synaptic events). As a next step, therefore, we investigate responses of the cable population to sinusoidal current injection (Fig. 7). Vm, V̂m, and Ve in this case are solutions to the boundary value problem Eqs. 11–13. We visualize these solutions by plotting amplitude (Fig. 7, A–C) and phase (Fig. 7, D–F) as functions of normalized distance along the cable. Phase is in units of radians, with zero-phase equal to the starting phase of the sinusoidal stimulus. We plot time courses of Vm, V̂m, and Ve selected from three locations along the cable in Fig. 7, G–I.
Fig. 7.
Passive cable response to sine-wave input current. Input frequency in dimensionless units is fτ = 0.1. A–C: amplitude profiles of cable population (Vm), test neuron (V̂m), and extracellular voltage response (Ve). Membrane potentials are plotted as deviation from rest (units: mV). D–F: phase profiles of cable population (Vm), test neuron (V̂m), and extracellular voltage responses (Ve). Phase is in units of radians, and zero-phase is referenced to the stimulus phase. In A–F, x-axis is the dimensionless distance along the cable (distance normalized by the space constant). Dashed lines show responses without ephaptic coupling (κ = 0), and solid lines show responses with ephaptic coupling (κ = 1). G–I: time courses of Vm, V̂m, and Ve plotted at 3 locations along the cable. x-Axis is 1 cycle of oscillations; y-axis is in units of millivolts (deviation from rest for population-averaged and test neuron). Schematic in G shows input location (black arrow). Time course locations are marked by colored arrows in A–C and G. They are X = 0.2, 1, and 1.8, where X = x/λ is a dimensionless measure of distance along the cable.
Responses to time-varying stimuli depend on the time constant τ = cmrm of the cable. In these simulations we set fτ = 0.1, where f is the stimulus frequency. This is an example in which the stimulus frequency is slow relative to the cable time constant. MSO neurons have exceptionally fast membranes (time constant ∼300 μs in gerbil MSO; Scott et al. 2005). We expect, therefore, that these simulations can provide intuition for MSO responses to stimulus frequencies as high as several hundred hertz.
In the absence of ephaptic effects, the population-averaged Vm attenuates along the length of the cable (κ = 0; Fig. 7A). The speed at which Vm propagates along the cable is evident in the roughly linear decay of phase along the cable (Fig. 7D). If ephaptic coupling is included (κ = 1), the test neuron membrane potential and the extracellular voltage are nonzero. We remarked above that ephaptic coupling effectively decreases the space constant of the cable (Rall 1977). This can be seen in the steeper attenuation of Vm amplitude for κ = 1. A smaller space constant is also associated with slower propagation of voltage along a cable (Koch 1998). In our simulations, the phase of oscillations in the voltage response to sinusoidal inputs changes more steeply with distance along the cable when ephaptic coupling is included (compare results for κ = 1 and κ = 0 in Fig. 7D). This indicates that, as expected, voltage propagates more slowly along the cable when ephaptic coupling is included.
The Ve amplitude profile has two peaks (Fig. 7C) that correspond to two (roughly) antiphase oscillations (note the abrupt, half-cycle phase transition in Fig. 7F). These antiphase oscillations can be seen in time courses of Ve by comparing responses near the input site to responses distant from the input site (Fig. 7I). This response profile is an indication that the cable population acts like a collection of synchronized current dipoles (Mc Laughlin et al. 2010). The spatial location of the minimum of the Ve amplitude profile is similar to the location of the half-cycle phase transition. For very low frequencies it would align with the location of the zero-crossing of the steady-state Ve response in Fig. 3D.
The V̂m response of the test neuron (Fig. 7, B, E, and H) has characteristics similar to the Ve response. In particular, it primarily comprises two antiphase oscillations (note the 2 peaks in the amplitude profile and the corresponding half-cycle phase transition). It can be helpful to distinguish these two “modes” by which ephaptic coupling drives V̂m in the test neuron. On the proximal side, the test neuron membrane potential oscillates nearly in phase with the population-averaged membrane potential (compare blue time courses in Fig. 7, G and H). In contrast, the central and more distant regions of the test neuron oscillate antiphase relative to the left side of the test neuron (compare green and red lines to blue line in Fig. 7H). In the transition region between these two oscillatory “modes,” there is a minimum in the V̂m amplitude profile. For very low frequencies, this minimum would approach 0 mV at the location of the zero-crossing in the stationary response (Fig. 3C). In response to time-varying inputs, however, there is spread of voltage along the cable and V̂m is not equal to 0 mV at one fixed location for all time.
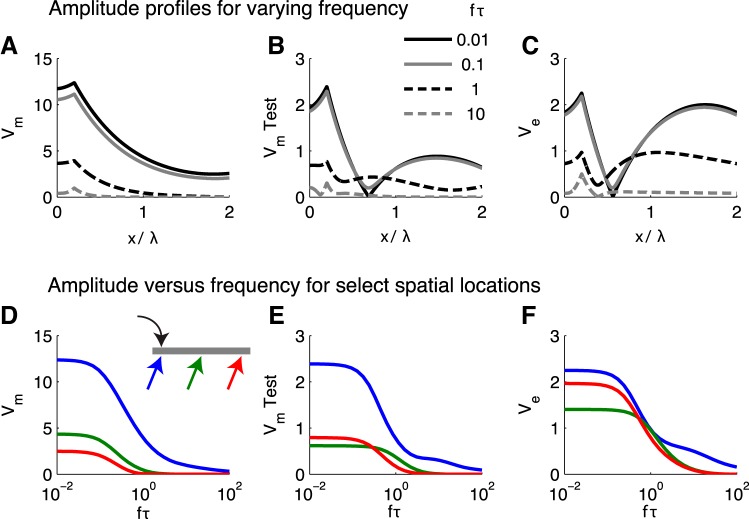
Attenuation of high-frequency responses due to low-pass cable dynamics.
Responses to higher-frequency stimuli are attenuated by capacitive filtering of the passive cable (Fig. 8). The amplitude of population-averaged Vm decreases with increasing frequency at all locations along the cable (Fig. 8, A and D).
Fig. 8.
Attenuation of high-frequency responses. A–C: amplitude profiles of cable population (Vm), test neuron (V̂m), and extracellular voltage responses (Ve). Membrane potentials are plotted as deviation from rest (units: mV). Different lines represent responses to different stimulus frequencies (see key in B). D–F: amplitudes of Vm, V̂m, and Ve responses plotted against stimulus frequency for 3 locations along the cable. Input location (black arrows) and response locations (colored arrows) are indicated by the schematic in D; they are X = 0.2, 1, and 1.8.
Ve (Fig. 8, C and F) and the test neuron V̂m (Fig. 8, B and E) responses exhibit slightly more complex changes with stimulus frequency. We remarked above that the Ve responses to low-frequency inputs are dipolelike and that the ephaptic interaction in these cases evokes two antiphase “modes” of oscillation in test neuron V̂m of the test neuron. As stimulus frequency increases, these dipolelike response features are distorted. In particular, when the timescale of the stimulus and the test neuron's cable dynamics are similar (fτ = 1), then the two “modes” of oscillation interact via spread of membrane potential along the cable. We have provided user-friendly simulation code to the ModelDB repository (accession no. 183948) so that the interested reader can view movies of these time-varying solutions.
We remark that at specific positions along the cable (say, the point aligned with the minimum of the black curve in Fig. 8C), Ve amplitude has a nonmonotonic dependence on stimulus frequency. For the three locations we plot in Fig. 8F, however, Ve amplitude attenuates monotonically with stimulus frequency.
Ephaptic Interactions in a Model of Medial Superior Olive
Initial simulations using a passive cable model have provided a basic understanding of the spatial and temporal patterning of ephaptic interactions in dendrite bundles. We next investigate ephaptic interactions in a biophysically based model of the MSO to determine possible effects of Ve coupling in a specialized nucleus in the auditory brain stem.
Remarks on coupling parameter κ.
Recall that the coupling parameter depends on the ratio of extracellular to intracellular resistivity (ρ) and the packing density of neurons δ according to the relationship κ = ρδ/(1 − δ). Extracellular resistivity Re in the auditory brain stem has not been measured, to our knowledge. As noted above, typical values of ρ in models of local field potentials in cortex are often in the range of 2.2–3.5 (Holt and Koch 1999; Lindén et al. 2011; Reimann et al. 2013). It is plausible that ρ in the MSO is larger because of the dense packing of myelinated fibers passing through the auditory brain stem, but we will use ρ = 3 as a reasonable estimate of the resistivity ratio.
We can estimate the packing density of neurons from an anatomical study of the MSO neurons in the gerbil (Rautenberg et al. 2009). In that study, the mean soma diameter was 13 μm and the density of MSO neurons in a mature MSO slice was 7 cells per 100 μm. Consider, then, an idealized cross section of MSO containing one column of 7 MSO somata (i.e., 100 μm length by 13 μm width). The packing density in this column is 0.715. In our simulations, we take δ = 0.7 so that κ = 7 around the soma. Rautenberg et al. reported that the diameter of dendrites in mature MSO slices was ∼3 μm. The packing density of dendrites, and consequently the value of κ in regions surrounding dendrites, is smaller than the values estimated above for somata. If we again consider 7 cells distributed in a 100 μm × 13 μm column, then the packing density of dendrites is δ = 0.038. We set ρ = 3 (the same value as we used for the soma) and estimate the coupling strength for dendrites to be κ = 0.12. These estimated values of κ near the soma and dendrites are marked in Fig. 2.
Responses to monolateral synaptic excitation.
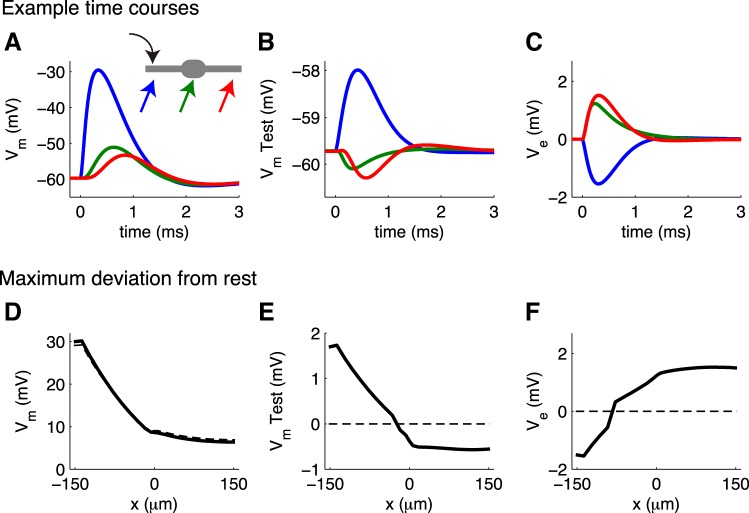
MSO neurons receive excitatory inputs that predominantly target their dendrites (Couchman et al. 2012). We begin our investigation of ephaptic coupling in MSO, therefore, by examining responses to a single excitatory synaptic event on one dendrite. The synaptic input depolarizes Vm in the dendrites of the population of MSO neurons (∼30 mV near the input site). Vm amplitude attenuates as it spreads away from the site of synaptic input (Fig. 9D, and evident in time courses at 3 locations along the neuron in Fig. 9A). Vm in the soma is ∼9 mV, and the finite propagation speed is evident as the peak of Vm is increasingly delayed as the postsynaptic potential propagates through the neuron.
Fig. 9.
MSO response to monolateral synaptic excitation; test neuron receives no synaptic stimulation. A–C: example time courses of Vm in MSO population, V̂m in the test neuron, and extracellular Ve at 3 locations along the neuron. Schematic in A shows these locations (left dendrite near the input site, soma, and right dendrite) and input location (left dendrite). D–F: spatial profiles of maximum deviation from resting voltage for Vm, V̂m, and Ve. y-Axis is deviation from resting voltage and can be compared to steady-state passive cable responses in Fig. 3. Results for simulations without ephaptic coupling are dashed lines; results for simulations that include ephaptic coupling are solid lines.
The Ve response (Fig. 9C) is negative near the input site because of the local transfer of positive ions from the extracellular domain into the intracellular domain (current sink). Near the soma and opposite dendrite, Ve is positive because of combined contributions of return currents distributed across the neuron (current sources). Recall that the coupling parameter in the dendrites is small (κ = 0.12). Nonetheless, the stimulation strength used in this simulation (maximal conductance is 27 mS/cm2) suffices to generate Ve amplitudes of ±0.8 mV in the extracellular domain surrounding the dendrites.
The “test” MSO neuron receives no direct synaptic input; its membrane potential V̂m (Fig. 9B) is perturbed by the spatiotemporal pattern of the surrounding extracellular voltage. V̂m increases near the stimulation site and decreases in the soma and distal dendrite. The peak ephaptic “excitation” is ∼1 mV. This illustrates that the millivolt-scale Ve responses observed in vivo in the MSO (e.g., Mc Laughlin et al. 2010) could, in principle, represent a nonsynaptic mechanism by which MSO neurons could induce millivolt-scale perturbations in membrane potential of neighboring neurons.
These simulations illustrate the dynamics of Vm, V̂m, and Ve responses to simulated synaptic inputs. Many of the main qualitative features, however, were already present in the steady-state passive cable simulations presented at the outset. To highlight the useful insights provided by the steady-state cable model, we plot spatial profiles of the maximum deviation from rest for the MSO neuron model in Fig. 9, D–F. These results can be compared to steady-state passive cable responses (Fig. 3). Simulations without ephaptic coupling are shown with dashed lines and simulations with ephaptic coupling with solid lines.
The deviations from resting voltage of the MSO neuron and passive cable models share many of the same qualitative features. For instance, in both cases the test neuron V̂m amplitude in the soma (or center of passive cable) is negative. This indicates that ephaptic coupling (in this scenario of monolateral dendritic excitation) diminishes the response at the soma and may raise the threshold for MSO spiking. We explore this in more detail below (see Fig. 11 and Fig. 12).
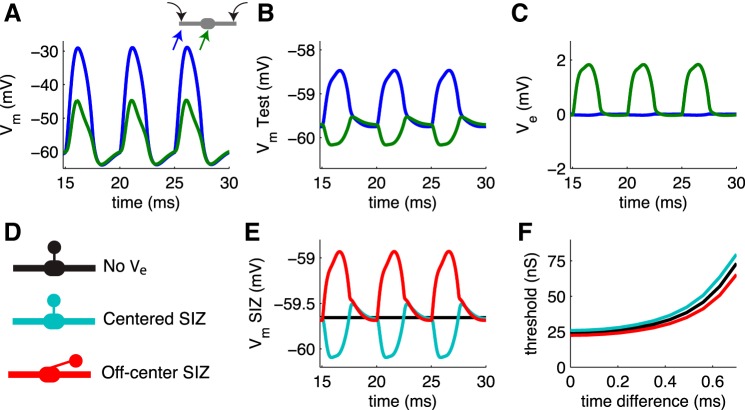
Fig. 11.
Response of MSO to periodic bilateral excitation. Test neuron includes a spike initiation zone (SIZ). A–C: time courses of Vm in the MSO population, V̂m in the test neuron, and extracellular Ve at 2 locations along neuron. Schematic in A shows these locations: left dendrite near the input site (blue) and soma (green). Responses on right dendrite are identical to those on left dendrite and are not shown. Waveform of excitatory conductance to MSO population is 200 Hz half-wave rectified sine function (identical on both dendrites). D: schematic diagram illustrating the different SIZ and Ve configurations tested: no ephaptic coupling (black), ephaptic coupling and SIZ located in alignment with the soma at x = 0 μm (cyan), and ephaptic coupling and SIZ located ∼100 μm away from soma (red). E: membrane potential response in SIZ for 3 different model configurations. F: conductance threshold of test neuron in response to bilateral excitation with time difference varied (x-axis).
There are, of course, quantitative differences. There is an order of magnitude difference between the coupling coefficient κ near the input site for the cable model (κ = 1) and the MSO neuron (κ = 0.12; see Fig. 2). As a consequence, Ve perturbs V̂m of the test neuron more in the cable model than in the MSO neuron model (compare solid lines in Fig. 9D and Fig. 3B). Based on this local measure of coupling strength, one may be surprised that the V̂m response of the test neuron is larger near the site of the synaptic input (κ = 0.12) than in the soma (κ = 7). We recall, however, that the effect of Ve on the test neuron can be understood as a “fictitious distributed current” [in the words of Holt and Koch (1999)] that is proportional to the second spatial derivative of Ve (see Eq. 2). The absolute value of this “ephaptic current” is maximal near the site of synaptic input, and thus we expect the ephaptic effect on V̂m to be largest there.
The weaker ephaptic effect in the MSO model is likely due to the small coupling coefficient κ for MSO dendrites. Perhaps surprisingly, the V̂m responses of the test neuron are largest in the dendrite (near the site of synaptic input) despite the small κ value there.
Responses to bilateral synaptic excitation.
In natural listening conditions, MSO neurons receive excitation on both dendrites (from sounds arriving in both ears). In Fig. 10 we show responses to coincident bilateral inputs (simulated excitatory synaptic events that arrive simultaneously on both dendrites). Example voltage time courses are shown in Fig. 10, A–C, and the Fig. 10, D–F, show the maximal deviation from resting voltage.
Fig. 10.
MSO response to bilateral synaptic excitation; test neuron receives no synaptic stimulation. A–C: example time courses of Vm in the MSO population, V̂m in the test neuron, and extracellular Ve at 2 locations along the neuron. Schematic in A shows these locations (left dendrite near the input site, soma) and input locations (both dendrites). Responses on right dendrite are identical to those on left dendrite and are not shown. D–F: spatial profiles of maximum deviation from resting voltage for Vm, V̂m, and Ve. Results for simulations without ephaptic coupling are dashed lines; results for simulations that include ephaptic coupling are solid lines.
The synaptic inputs depolarize the Vm by ∼30 mV near the synaptic site and a summed depolarization of ∼15 mV in the soma (Fig. 10, A and D). Responses to bilateral inputs differ slightly from the linear superposition of monolateral responses due to the presence of voltage-gated low-threshold K current in the dendrites and soma.
A striking difference in these simulations compared with responses to monolateral inputs shown in Fig. 9 above is that Ve is spatially localized (Fig. 10, C and F). Ve reaches a maximum value of ∼2.5 mV near the soma, but near the distal reaches of the dendrites Ve decreases to 0 mV. The symmetrical arrangement of membrane currents produces a “closed field” with no volume conduction beyond the dendrites' terminal ends. Given the small Ve and small coupling parameter surrounding the dendrite, ephaptic effects on the dendrites might not be expected. This is not the case. The depolarization of V̂m in the dendrites of the test neuron is, in fact, roughly twice as large (in amplitude) as the hyperpolarization in the test neuron soma (compare blue curve to green curve in Fig. 10B).
Ephaptic coupling influences MSO spike initiation.
We have shown that endogenously generated Ve can perturb the membrane potential of a “test” MSO neuron embedded in the extracellular bath. Does this ephaptic interaction suffice to alter spiking outputs of MSO neurons? By adding a spike-initiation zone (SIZ) to the test neuron model (see materials and methods), we can investigate how SIZ membrane potential and spiking activity are influenced by ephaptic interactions.
We created a situation in which Ve is generated from a MSO neuron model that does not include a SIZ. We then embedded a test neuron with a SIZ into this Ve field (see materials and methods). Ve influences the membrane potential of the test neuron (including in its SIZ) because membrane potential is a voltage difference: V̂m = V̂i − Ve. The test neuron's contribution to Ve is assumed to be negligible. We are treating this one neuron's contribution to Ve as sufficiently small that it can be ignored.
For all simulations shown in Fig. 11 we stimulate the MSO population that generates Ve with identical excitatory inputs on both dendrites. The input current at each input site is of the form G(t)(Vm − Vsyn), where the input conductance density G(t) is modeled as a 200-Hz half-wave rectified sine function, Vm is the membrane potential at the input site, and Vsyn = 0 mV is the reversal potential for excitation. The conductance density waveform G(t) is identical on both dendrites, with peak amplitude of 20 mS/cm2. We use the half-wave rectified sine function as a simplified, population-averaged representation of excitatory drive to MSO in response to a pure-tone stimulus. The input sites were on both dendrites located 137.5 μm from the soma center.
The Vm response to these inputs is a large ∼30-mV depolarization in the dendrites that attenuates to a ∼15-mV depolarization in the soma (Fig. 11A). The MSO population generates a periodic Ve response with ∼1.8-mV positive-going oscillations around the soma and much smaller negative-going oscillations around the distal ends of the dendrites (Fig. 11C). In response to this endogenous Ve field, the test neuron exhibits ∼1.5-mV positive-going oscillations in V̂m in the dendrites and smaller ∼0.5-mV negative-going oscillations in the soma (Fig. 11B). Note that the test neuron does not receive any synaptic input in these simulations, so these V̂m changes are strictly due to ephaptic coupling.
The test neuron includes a SIZ in these simulations, and Ve perturbs the SIZ's membrane potential (Fig. 11E). In these and subsequent simulations, we compare three SIZ configurations illustrated in Fig. 11D: the “control” condition of no ephaptic coupling, ephaptic coupling and SIZ aligned with the soma, and ephaptic coupling and SIZ aligned with a position 117.5 μm from the soma. If the SIZ is located near the soma, its membrane potential exhibits negative-going oscillations similar to V̂m in the soma of the test neuron (compare cyan line in Fig. 11E and green line in Fig. 11B). If the SIZ is located away from the soma, its membrane potential exhibits positive-going oscillations similar to the response of the test neuron dendrite (compare red line in Fig. 11E and blue line in Fig. 11B). Note that in both cases the SIZ is connected via the same internal, axial resistance to the soma of the MSO neuron model. The SIZ position does affect the axial current flow between the SIZ and the soma in the test neuron. This current is proportional to the voltage difference Visoma − ViSIZ = (Vmsoma + Vesoma) − (VmSIZ + VeSIZ), and VeSIZ is calculated with regard to the spatial location of the SIZ.
These simulations reveal that Ve can increase or decrease the SIZ membrane potential depending on the position of the SIZ. Do ephaptic interactions alter spiking activity in MSO? We used conductance threshold as a measure of neuron excitability and found that ephaptic effects modulate the threshold curve depending on the location of the SIZ (Fig. 11F). If the SIZ is aligned with the soma, we see in Fig. 11E that Ve hyperpolarizes the SIZ membrane potential. This translates to an increase in threshold (diminished excitability) for all time differences tested. In contrast, the depolarizing effect of Ve on the off-center SIZ translates to a decrease in threshold, i.e., enhanced excitability.
The test neuron in these simulations received synaptic excitation in the form of excitatory (alpha function) synaptic events arriving on the two dendrites 15 ms after the start of the periodic input to the MSO population. The 15-ms delay ensured that any transient onset dynamics are avoided. We varied the difference in the timing of the two synaptic inputs to the test neuron (x-axis). A time difference of 0 ms represents coincident bilateral inputs to the test neuron. In this case the synaptic event times in the test neuron match the onset of one cycle of the half-wave rectified sine conductance input to the MSO population. Time differences larger than 0 ms (positive values on the x-axis of Fig. 11F) represent bilateral inputs to the test neuron that are not coincident. The synaptic event on one dendrite arrives earlier than the onset of the half-wave rectified sine input to the MSO population, and the other synaptic event trails the onset of the half-wave rectified sine.
Conductance threshold is the smallest peak conductance needed to generate a spike in the SIZ. In the absence of ephaptic effects, thresholds increase with submillisecond increases in synaptic time difference (Fig. 11F). This is confirmation that the model neuron, like MSO neurons, acts as a coincidence detector. For the time differences tested in these simulations, ephaptic interactions decrease spike threshold by ∼10% for off-center SIZ and increase threshold by ∼10% for centered SIZ.
Ephaptic coupling can entrain MSO spike timing.
We next show that the small changes in spike threshold measured above can result in changes in spike timing.
We conducted a set of simulations in which we measured spike timing in the test neuron's responses to random trains of excitatory synaptic events. To construct the input to each dendrite of the test neuron, we combined 10 independent realizations of a homogeneous Poisson process (100-Hz event rate) to generate 10 independent input trains of “event times.” We then convolved these event times with an alpha function (0.2 ms time constant, see Eq. 20). Each “unitary” synaptic event had a peak conductance of 10 mS/cm2, was located 137.5 μm from the center of the soma, and increased the SIZ membrane potential by 4.5 mV in the soma. The simultaneous arrival of four such events (2 per dendrite) can evoke a spike in the SIZ, as can the arrival of six such events on a single dendrite.
This random input train caused the test neuron to fire spontaneously at a rate of 20 spikes/s, on average, in the absence of ephaptic coupling. The firing rate is presented as a cycle histogram in Fig. 12A. In the absence of ephaptic interactions, there is no 200-Hz “rhythm” to entrain spike times; thus the cycle histogram is flat for the spontaneously firing neuron.
We repeated the simulation in the presence of an endogenously generated Ve that is the same as shown in Fig. 11; it is the response to bilateral input currents (inputs at 1 location on each dendrite) of the form G(t)(Vm − Vsyn), where G(t) is a 200-Hz half-wave rectified sine function. The phase of the half-wave rectified sine function is identical for the inputs to the two dendrites. As in the previous simulations, we investigated ephaptic effects when the SIZ was aligned with the soma (centered configuration) and when it was located 117.5 μm from the soma center (off-center configuration).
We found that ephaptic interactions temporally modulate spike timing (Fig. 12A). Consistent with our previous results, the effect of Ve differs depending on the location of the SIZ. For the centered SIZ, ephaptic coupling reduces firing rates during the first half of the 5-ms cycle and increases firing rates during the second half of the cycle. The cumulative effect of this Ve-induced firing rate modulation is a decrease of ∼1.4 spike/s. For the off-center SIZ, the effect is opposite. Ephaptic coupling increases firing rates during the first half of the 5-ms period and decreases firing rates thereafter. The cumulative change is an extra ∼1.7 spikes/s due to ephaptic coupling. Simulations that include ephaptic coupling and the off-center SIZ produce spikes that are more likely to occur in phase with the periodic input to the MSO population. Thus the ephaptic interaction can entrain the test neuron's spikes, even though inputs to the test neuron are random and do not have a periodic structure.
Ephaptic coupling influences MSO coincidence detection.
As a final test of ephaptic interactions, we simulated the standard measure of MSO neuron tuning to sound location: time-difference tuning curves (Fig. 12B). Inputs to the test neuron were constructed from inhomogeneous Poisson processes, with Poisson event rate given by a 200-Hz half-wave rectified sine function that produced (on average) 100 inputs events/s. Ten independent realizations (trains) of event times were generated per dendrite, and input event times were convolved, as previously described, with an alpha function (0.2 ms time constant).
The relative timing of the half-wave rectified sine wave functions that defined the Poisson event rates on the two dendrites of the test neuron varied, as indicated on the x-axis of Fig. 12B. A time difference of 0 ms indicates Poisson rates that are identical on the two dendrites. A nonzero time difference indicates a phase difference between the half-wave rectified sine wave Poisson rate functions.
The test neuron responds maximally when the Poisson rate functions are in phase on the two dendrites (0 ms time difference). This is another indication that the MSO model neuron acts as a coincidence detector with submillisecond temporal precision. In the absence of ephaptic coupling, the maximal firing rate is 166 Hz and decreases for larger time differences (Fig. 12B).
We included ephaptic coupling as in Fig. 12A by using the MSO model to generate endogenous Ve. As above, we used bilateral input currents of the form G(t)(Vm − Vsyn), where G(t) is a 200-Hz half-wave rectified sine function to generate Ve. The phase of the half-wave rectified sine function G(t) varied for the two dendrites so that Ve was generated by inputs with the same bilateral time difference as inputs to the test neuron (as given on the x-axis). In other words, the input current to each dendrite of the test neuron was constructed to follow the same time course (roughly) as the input currents to each dendrite of the neurons generating Ve.
When ephaptic coupling is included, the effect on time-difference tuning depends on the SIZ location in a manner consistent with our previous tests. Ephaptic coupling and the centered SIZ combine to have an “inhibitory” effect that reduces firing rates. The firing rate at 0 ms time difference is 155 spikes/s (6.5% decrease), and we observed statistically significant decreases in firing rates over a wide range of time differences (P < 0.03 using Welch's t-test for time differences between −1.75 ms and 1.5 ms). Ephaptic coupling and the off-center SIZ combine to have an “excitatory” effect that increases firing rates. The firing rate at 0 ms time difference is 178 spikes/s (7.3% increase), and we observed statistically significant increases in firing rate over a wide range of time differences (P < 0.01 using Welch's t-test for time differences between −1 ms and 2.25 ms). Taken together, the results in Fig. 12 illustrate that the nonlinear nature of spike generation can amplify small changes in membrane potential. Millivolt-scale ephaptic interactions can plausibly alter spike activity in neurons and circuits.
DISCUSSION
Summary of Main Findings
Neural activity generates transmembrane currents that, in turn, generate spatiotemporal patterns of extracellular voltage. Nearby neurons are embedded in this shared, endogenously generated Ve. Ve can provide, therefore, a channel for nonsynaptic communication between neurons. Following the insightful early work of Arvanitaki (1942), we refer to this phenomenon as ephaptic coupling.
We have developed and analyzed a model of ephaptic interactions in an idealized one-dimensional setting (computer code available on ModelDB, accession no. 183948). We considered a population of identical passive cables as an idealized model of uniform, unbranched neurons receiving identical inputs. We illustrated how ephaptic coupling in this system can be described with an extension of cable theory that accounts for dynamical coupling between one-dimensional intra- and extracellular domains. We identified the strength of extracellular coupling by introducing a new dimensionless parameter κ = ρδ(1 − δ), where ρ is the ratio of volume resistivities Re/Ri and δ is the packing density of neurons, and offered plausible estimates for κ.
We found that the idealized neuron population could generate millivolt-scale Ve, and this Ve could induce millivolt-scale perturbations in the membrane potential of an additional “test” neuron embedded in the endogenously generated Ve. We varied the spatial profile of Ve by varying the localized stimulus input site and found that the amplitude of the Vm perturbation remained relatively constant (Fig. 4). We also found that electrotonically compact cables (large space constant) experience larger changes in their Vm due to coupling to Ve than electrotonically long cables (small space constant) (Fig. 5) and compact cables generate larger-amplitude Ve responses (Fig. 6). For sinusoidal inputs, ephaptic effects at low frequencies were similar to steady-state responses (Fig. 7). The low-pass dynamics imposed by the cable's passive membrane (and quantified by the parameter τ) limit Vm responses and the generation of endogenous Ve in response to higher-frequency inputs (Fig. 8). Ephaptic effects are relevant over a large frequency range, therefore, for populations of neurons with fast passive dynamics (small time constant τ).
We applied the same one-dimensional idealization to model Ve generated by simultaneously activated and spatially aligned neurons in the MSO of the auditory brain stem. These neurons have a relatively simple structure: a soma and two dendrites extending away from the soma with minimal branching. The qualitative features of ephaptic coupling in the passive cable model and this more biophysically detailed model were broadly similar. Our simulations used physiologically plausible parameters and fast synaptic conductances and demonstrated that MSO neurons can generate millivolt-scale Ve responses that induce millivolt-scale perturbations in a “test” MSO neuron (Fig. 9 and Fig. 10).
The MSO is a critical early stage of binaural processing, and it can extract information regarding the location of sound sources in the environment. MSO neurons receive inputs arriving from both ears, and they are sensitive to submillisecond timing differences in these inputs. We were particularly interested, therefore, to determine whether ephaptic effects and their instantaneous nature can alter the precise coincidence detection computation performed by MSO neurons. We tested ephaptic effects on MSO spiking by adding a spike initiation zone (SIZ) to a test neuron embedded in the endogenously generated (simulated) Ve. We found that millivolt-scale ephaptic effects can change the spike output of MSO neurons. The location of the SIZ in the spatially distributed Ve is critical. We compared two configurations: a “centered” SIZ aligned with the soma and an “off-center” SIZ located ∼100 μm away from the soma (see schematic in Fig. 11D). Ve can act in an “excitatory” or “inhibitory” manner depending on the SIZ position. In particular, for an SIZ located away from the soma, Ve produced by in-phase bilateral inputs to the MSO population depolarized Vm in the SIZ (Fig. 11E), decreased thresholds for spike generation (Fig. 11F), entrained spike times to the ongoing Ve oscillation (Fig. 12A), and increased the gain of a simulated time-difference tuning curve (Fig. 12B). If the SIZ is aligned with the soma, Ve has opposite effects and suppresses spiking activity. We demonstrated these effects for 200-Hz input frequency and found that Ve affected firing rates similarly for input frequencies as high as ∼1 kHz (simulations not shown).
Relation to Previous Work
Our model relies on the idealization that neural dynamics in the presence of ephaptic interactions can be described by coupling a one-dimensional intracellular domain (cable core conductor) to a one-dimensional extracellular volume conductor. We arrived at this simplified geometry by taking a mean-field view of a large population of identical cables organized in parallel to one another and receiving identical inputs. This construction may appear overly simplistic, but it is inspired by pioneering studies of endogenously generated Ve (Nicholson and Llinás 1971; Rall and Shepherd 1968) and we have recently used a similar formulation to study in vivo Ve in the auditory brain stem of cats (Goldwyn et al. 2014). Alternative methods have been formulated that include more realistic neural morphologies and extracellular volume conductors (Agudelo-Toro and Neef 2013; Malik 2011), but these methods have the drawback of requiring substantial computing resources. In addition, the one-dimensional intracellular and extracellular domains facilitate visualization and analyses of simulation results. The validity of the one-dimensional volume conductor model could be evaluated in future studies with these more sophisticated computational methods.
A number of our observations concur with earlier studies of Ve effects on passive cables. Rall (1977) pointed out that coupling to Ve decreases the cable space constant from to . Recent studies have pointed out that increasing the space constant of a cable (larger rm and/or smaller ri) increases the effect of Ve on Vm (Anastassiou et al. 2010; Frölich and McCormick 2010). We identified the upper bound of the ephaptic effect for steady-state responses to constant localized input as (see Fig. 5B).
We showed that a periodic Ve can alter spike timing in MSO model neurons (Fig. 12A). Recent articles have reported similar results in vitro and in simulation studies (Anastassiou et al. 2011; Frölich and McCormick 2010). Specifically, these studies applied periodic extracellular fields to cortical slice preparations and demonstrated that spike timing can be entrained to the “rhythm” of ongoing, periodic Ve. A novel finding in our study is that the location of the SIZ matters when considering the effect of Ve on spike timing. Ve can indeed entrain neurons and enhance spike synchrony (Fig. 12A, off-center SIZ). It is also possible, however, that the SIZ could be located in a region of extracellular space where Ve decreases the SIZ membrane potential and suppresses spike output (Fig. 12A, centered SIZ). This could reduce spike time synchrony across a population. We did not consider the contribution of spikes to Ve because they are not prominent in MSO field potentials. A recent modeling study of layer V pyramidal cells has demonstrated that Ve generated by spikes is capable of altering spike timing in simulations (Stacey et al. 2015).
Implications for Functional Ephaptic Coupling
Applied Ve affects cell-level and circuit-level neural activity; this is the principle by which neural prostheses such as cochlear implants, retinal implants, and deep brain stimulation operate. We are concerned with a complementary question: is endogenously generated Ve a form of nonsynaptic, global coupling that can alter neural dynamics?
Studies in diverse neural structures have identified ephaptic coupling as a means of fast, nonsynaptic inhibition (teleost Mauthner cell system in goldfish, Weiss et al. 2008; olfactory receptor neurons in insects, Su et al. 2012; pinceau structure surrounding axon initial segment of cerebellar Purkinje cell, Blot and Barbour 2014). Figure 3 illustrates how ephaptic coupling could operate in an inhibitory manner in a bundle of neurons. Excitatory inputs arriving near the terminal of a neural structure (e.g., a distal dendrite site) hyperpolarize the opposite end (e.g., soma and initial segment) of neighboring neural structures through the ephaptic interaction.
Figure 3 also illustrates that ephaptic interactions could promote local excitation. Excitatory inputs arriving near the end, say the proximal end, of a neural structure locally depolarize the proximal end of nearby neurons through the ephaptic interaction. This local depolarization could be enhanced further in dendritic bundles with regenerative currents and provide a nonsynaptic mechanism for simultaneously exciting dendrites in a local population. Bokil et al. (2001) demonstrated this type of “excitatory” ephaptic coupling in simulations of a bundle of axons. They found, using a mean field formulation similar to ours, that spikes in an axon could promote spike generation and spike time synchrony in nearby axons. The capacity for Ve to depolarize nearby neurons via an “excitatory” ephaptic effect has also been proposed as a mechanism for epileptogenesis in the hippocampus (Traub et al. 1985; Zhang et al. 2014).
Our MSO simulations that included a SIZ in the test neuron demonstrated that even small ephaptic effects (millivolt scale) can alter spiking activity (Fig. 12). The nonlinear nature of spike generation can amplify ephaptic coupling (see also Anastassiou et al. 2011; Radman et al. 2007). We found that the anatomy of the spike generator matters for neurons embedded in a spatially varying Ve. Ephaptic coupling can have opposing “excitatory” or “inhibitory” effects depending on the orientation of axons and the site of spike generation. We are not aware of a comprehensive study of axon anatomy in the MSO. This makes it difficult for us to make specific predictions regarding possible functional effects of ephaptic coupling in MSO. A recent study has examined spike generation in MSO axons and the anatomy of the MSO axon initial segment (Lehnert et al. 2014). Further work in this direction would contribute to our understanding of ephaptic coupling the MSO. Auditory brain stem neurons in the chick exhibit plasticity in the length of the axon initial segment (specifically, the distribution of Na channels) (Kuba et al. 2010). This raises the possibility that ephaptic effects could be modulated over time.
Our cable theory-based study of ephaptic interactions among passive cables has informed our understanding of ephaptic interactions in MSO neurons. We view the MSO as a useful “model system” to explore these effects because Ve is large and sound-evoked in vivo, spatiotemporal features of the Ve response can be modeled with the mean-field approximation and a one-dimensional volume conductor (Goldwyn et al. 2014), and MSO neurons perform a known computation. That computation—coincidence detection of binaural inputs—is typically studied by recording (or simulating) time-difference tuning curves analogous to Fig. 12B. We found that ephaptic coupling could increase or decrease the gain of the time-difference tuning curve, depending on the location of the spike generator (but the tuning curve width was not changed appreciably). Our simulations establish a “proof of principle” that ephaptic interactions can alter binaural processing in the MSO. We cannot yet identify a functional role for ephaptic coupling in the MSO, but this remains an intriguing avenue for future research.
GRANTS
This research has been supported by funding from the National Institute on Deafness and Other Communication Disorders: Grants F32 DC-012978 (J. H. Goldwyn) and R01 DC-008543 (J. Rinzel).
DISCLOSURES
No conflicts of interest, financial or otherwise, are declared by the author(s).
AUTHOR CONTRIBUTIONS
Author contributions: J.H.G. and J.R. conception and design of research; J.H.G. performed experiments; J.H.G. analyzed data; J.H.G. and J.R. interpreted results of experiments; J.H.G. prepared figures; J.H.G. drafted manuscript; J.H.G. and J.R. edited and revised manuscript; J.H.G. and J.R. approved final version of manuscript.
ACKNOWLEDGMENTS
Large-scale simulations were supported by computing resources at the New York University High Performance Computing Center and an allocation of computing time from the Ohio Supercomputer Center.
GlossaryGeneral
- t
Time (ms)
- x
Distance (cm)
- Vi
Intracellular voltage (mV)
- Ve
Extracellular voltage (mV)
- Vm = Vi − Ve
Transmembrane potential (mV)
- Elk
Reversal potential of leak current (mV)
- ri
Intracellular (axial) resistance per unit length (Ω/cm)
- re
Extracellular resistance per unit length (Ω/cm)
- N
Number of neurons in population
- κ = Nre/ri
Coupling constant
- ρ = Re/Ri
Resistivity ratio
- δ
Packing density
- Ai, Ae
Cross-sectional area of intra- and extracellular domains (cm2)
- ^
Parameter or variable associated with “test” neuron (e.g., V̂m)
Passive Cable Model
- cm
Capacitance per unit length (mF/cm)
- rm
Resistance across a unit length (Ωcm)
- τ = cmrm
Membrane time constant (ms)
- λ =
Cable space constant (cm)
- λref
“Reference value” of cable space constant (cm)
- T = t/τ
Nondimensional time variable
- X = x/λ
Nondimensional space variable
- L = l/λ
Nondimensional length of cable
- iin
Input current per unit length (mA/cm)
- im
Membrane current per unit length (mA/cm)
- l
Physical length of cable (cm)
- dg
Distance from end of cable to electric ground (cm)
- f
Frequency of sinusoidal current input (Hz)
- Um, Ui, Ue
Voltage in Fourier domain
Medial Superior Olive Model
- Cm
Capacitance (mF/cm2)
- Ri
Intracellular (axial) resistivity (Ωcm)
- Re
Extracellular resistivity (Ωcm)
- Ii, Ie, Im, Iin
Intracellular, extracellular, transmembrane, and input current (mA)
- Jin
Input current density (mA/cm2)
- Glk, GKLT, Gh, GNa
Maximal conductance density for leak, KLT, h, and Na currents (mS/cm2)
- EK, Eh, ENa
Reversal potential for K, h, and Na currents (mV)
- w, z
Gating variables for KLT current
- m, h
Gating variables for Na current
- u∞
Steady-state functions for gating variables (where u = w, z, m, or h)
- τu
Time constants for activation and inactivation variables (where u = w, z, m, or h)
- Gsyn
Maximal synaptic conductance density (mS/cm2 per input event)
- Esyn
Reversal potential of synaptic current (mV)
- τsyn
Synaptic conductance time constant (ms)
- t0
Onset time of synaptic event (ms)
- gaxial
Axial conductance between soma and spike initiation zone (nS)
- d
Diameter of MSO neuron model (cm)
- Δx
Length of compartment in discretized MSO neuron model (cm)
- S
Surface area of compartment (cm2)
REFERENCES
- Agudelo-Toro A, Neef A. Computationally efficient simulation of electrical activity at cell membranes interacting with self-generated and externally imposed electric fields. J Neural Eng 10: 026019, 2013. [DOI] [PubMed] [Google Scholar]
- Anastassiou CA, Montgomery SM, Barahona M, Buzsáki G, Koch C. The effect of spatially inhomogeneous extracellular electric fields on neurons. J Neurosci 30: 1925–1936, 2010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Anastassiou CA, Perin R, Markram H, Koch C. Ephaptic coupling of cortical neurons. Nat Neurosci 14: 217–223, 2011. [DOI] [PubMed] [Google Scholar]
- Arvanitaki A. Effects evoked in an axon by the activity of a contiguous one. J Neurophysiol 5: 89–108, 1942. [Google Scholar]
- Ashida G, Carr CE. Sound localization: Jeffress and beyond. Curr Opin Neurobiol 21: 745–751, 2011. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Biedenbach MA, Freeman WJ. Click-evoked potential map from the superior olivary nucleus. Am J Physiol 206: 1408–1414, 1964. [DOI] [PubMed] [Google Scholar]
- Blot A, Barbour B. Ultra-rapid axon-axon ephaptic inhibition of cerebellar Purkinje cells by the pinceau. Nat Neurosci 17: 289–295, 2014. [DOI] [PubMed] [Google Scholar]
- Bokil H, Laaris N, Blinder K, Ennis M, Keller A. Ephaptic interactions in the mammalian olfactory system. J Neurosci 21: RC173, 2001. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Buzsáki G, Anastassiou CA, Koch C. The origin of extracellular fields and currents—EEG, ECoG, LFP and spikes. Nat Rev Neurosci 13: 407–420, 2012. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Clark GM, Dunlop CW. Field potentials in the cat medial superior olivary nucleus. Exp Neurol 20: 31–42, 1968. [DOI] [PubMed] [Google Scholar]
- Couchman K, Grothe B, Felmy F. Functional localization of neurotransmitter receptors and synaptic inputs to mature neurons of the medial superior olive. J Neurophysiol 107: 1186–1198, 2012. [DOI] [PubMed] [Google Scholar]
- Fischl MJ, Combs TD, Klug A, Grothe B, Burger RM. Modulation of synaptic input by GABAB receptors improves coincidence detection for computation of sound location. J Physiol 590: 3047–3066, 2012. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Franken TP, Roberts MT, Wei L, Golding NL, Joris PX. In vivo coincidence detection in mammalian sound localization generates phase delays. Nat Neurosci 18: 444–452, 2015. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Frölich F, McCormick DA. Endogenous electric fields may guide neocortical network activity. Neuron 67: 129–143, 2010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Galambos R, Schwartzkopff J, Rupert A. Microelectrode study of superior olivary nuclei. Am J Physiol 197: 527–536, 1959. [DOI] [PubMed] [Google Scholar]
- Geddes LA, Baker LE. The specific resistance of biological material—a compendium of data for the biomedical engineer and physiologist. Med Biol Eng 5: 271–293, 1967. [DOI] [PubMed] [Google Scholar]
- Golding NL, Oertel D. Synaptic integration in dendrites: exceptional need for speed. J Physiol 590: 5563–5569, 2012. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Goldwyn JH, Mc Laughlin M, Verschooten E, Joris PX, Rinzel J. A model of the medial superior olive explains spatiotemporal features of the local field potentials. J Neurosci 34: 11705–11722, 2014. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Graham RL, Lubachevsky BD, Nurmela KJ, Östergård PR. Dense packings of congruent circles in a circle. Discrete Math 181: 139–154, 1998. [Google Scholar]
- Grothe B, Pecka M, McAlpine D. Mechanisms of sound localization in mammals. Physiol Rev 90: 983–1012, 2010. [DOI] [PubMed] [Google Scholar]
- Grothe B, Sanes DH. Bilateral inhibition by glycinergic afferents in the medial superior olive. J Neurophysiol 69: 1192–1196, 1993. [DOI] [PubMed] [Google Scholar]
- Grothe B, Sanes DH. Synaptic inhibition influences the temporal coding properties of medial superior olivary neurons: an in vitro study. J Neurosci 14: 1701–1709, 1994. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hindmarsh AC, Brown PN, Grant KE, Lee SL, Serban R, Shumaker DE, Woodward CS. SUNDIALS: SUite of Nonlinear and DIfferential/ALgebraic Equation Solvers. ACM Trans Math Software 31: 363–396, 2005. [Google Scholar]
- Holt GR, Koch C. Electrical interactions via the extracellular potential near cell bodies. J Comput Neurosci 6: 169–184, 1999. [DOI] [PubMed] [Google Scholar]
- Huguet G, Meng X, Rinzel J. Phasic firing, by divisive or subtractive feedback, can perform coincidence detection—but how well (Abstract)? Neuroscience Meeting Planner 2012: 540 26, 2012. [Google Scholar]
- Jercog PE, Svirskis G, Kotak VC, Sanes DH, Rinzel J. Asymmetric excitatory synaptic dynamics underlie interaural time difference processing in the auditory system. PLoS Biol 8: e1000406, 2010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Joris PX, Carney LH, Smith PH, Yin TC. Enhancement of neural synchronization in the anteroventral cochlear nucleus. I. Responses to tones at the characteristic frequency. J Neurophysiol 71: 1022–1036, 1994. [DOI] [PubMed] [Google Scholar]
- Katz B, Schmitt OH. Electric interaction between two adjacent nerve fibers. J Physiol 97: 471–488, 1940. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Khurana S, Remme MW, Rinzel J, Golding NL. Dynamic interaction of Ih and IK-LVA during trains of synaptic potentials in principal neurons of the medial superior olive. J Neurosci 31: 8936–8947, 2011. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Klee M, Rall W. Computed potentials of cortically arranged populations of neurons. J Neurophysiol 40: 647–666, 1977. [DOI] [PubMed] [Google Scholar]
- Koch C. Biophysics of Computation: Information Processing in Single Neurons. New York: Oxford Univ. Press, 1998, p. 47. [Google Scholar]
- Kuba H, Oichi Y, Ohmori H. Presynaptic activity regulates Na+ channel distribution at the axon initial segment. Nature 465: 1075–1078, 2010. [DOI] [PubMed] [Google Scholar]
- Lehnert S, Ford MC, Alexandrova O, Hellmundt F, Felmy F, Grothe B, Leibold C. Action potential generation in an anatomically constrained model of medial superior olive axons. J Neurosci 34: 5370–5384, 2014. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lindén H, Tetzlaff T, Potjans TC, Pettersen KH, Grün S, Diesmann M, Einevoll GT. Modeling the spatial reach of the LFP. Neuron 72: 859–872, 2011. [DOI] [PubMed] [Google Scholar]
- Lorente de Nò R. Action potential of the motoneurons of the hypoglossus nucleus. J Cell Comp Physiol 29: 207–287, 1947. [DOI] [PubMed] [Google Scholar]
- Lucht W, Strehmel K, Eichler-Liebenow C. Linear Partial Differential Algebraic Equations. Part I: Indexes, Consistent Boundary/Initial Conditions (Report 17). Halle, Germany: Fachbereich Mathematik und Informatik, Martin-Luther-Universität, 1997a. [Google Scholar]
- Lucht W, Strehmel K, Eichler-Liebenow C. Linear Partial Differential Algebraic Equations. Part II: Numerical Solution (Report 18). Halle, Germany: Fachbereich Mathematik und Informatik, Martin-Luther-Universität, 1997b. [Google Scholar]
- Malik NA. Frequency-Dependent Response of Neurons to Oscillating Electric Fields (PhD dissertation) Coventry, UK: University of Warwick, 2011. [Google Scholar]
- Mathews PJ, Jercog PE, Rinzel J, Scott LL, Golding NL. Control of submillisecond synaptic timing in binaural coincidence detectors by Kv1 channels. Nat Neurosci 13: 601–609, 2010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mc Laughlin M, Verschooten E, Joris PX. Oscillatory dipoles as a source of phase shifts in field potentials in the mammalian auditory brainstem. J Neurosci 30: 13472–13487, 2010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Moushegian G, Rupert A, Whitcomb MA. Medial superior-olivary-unit response patterns to monaural and binaural clicks. J Acoust Soc Am 36: 196–202, 1964. [DOI] [PubMed] [Google Scholar]
- Myoga MH, Lehnert S, Leibold C, Felmy F, Grothe B. Glycinergic inhibition tunes coincidence detection in the auditory brainstem. Nat Commun 5: 3790, 2014. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Nicholson C, Llinás R. Field potentials in the alligator cerebellum and theory of their relationship to Purkinje cell dendritic spikes. J Neurophysiol 34: 509–531, 1971. [DOI] [PubMed] [Google Scholar]
- Radman T, Su Y, An JH, Parra LC, Biksom M. Spike timing amplifies the effect of electric fields on neurons: implications for endogenous field effects. J Neurosci 27: 3030–3036, 2007. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rall W. Core conductor theory and cable properties of neurons. In: Handbook of Physiology. The Nervous System. Cellular Biology of Neurons. Bethesda, MD: Am. Physiol. Soc, 1977, sect. 1, vol. I, p. 39–97. [Google Scholar]
- Rall W, Shepherd GM. Theoretical reconstruction of field potentials and dendrodendritic synaptic interactions in olfactory bulb. J Neurophysiol 31: 884–915, 1968. [DOI] [PubMed] [Google Scholar]
- Ramón F, Moore JW. Ephaptic transmission in squid giant axons. Am J Physiol Cell Physiol 234: C162–C169, 1978. [DOI] [PubMed] [Google Scholar]
- Rattay F. Analysis of models for external stimulation of axons. IEEE Trans Biomed Eng 33: 974–977, 1986. [DOI] [PubMed] [Google Scholar]
- Rattay F. The basic mechanism for the electrical stimulation of the nervous system. Neuroscience 89: 335–346, 1999. [DOI] [PubMed] [Google Scholar]
- Rautenberg PL, Grothe B, Felmy F. Quantification of the three-dimensional morphology of coincidence detector neurons in the medial superior olive of gerbils during late postnatal development. J Comp Neurol 517: 385–396, 2009. [DOI] [PubMed] [Google Scholar]
- Reimann MW, Anastassiou CA, Perin R, Hill SL, Markram H, Koch C. A biophysically detailed model of neocortical local field potentials predicts the critical role of active membrane currents. Neuron 79: 375–390, 2013. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rothman JS, Manis PB. The roles potassium currents play in regulating the electrical activity of ventral cochlear nucleus neurons. J Neurophysiol 89: 3097–3113, 2003. [DOI] [PubMed] [Google Scholar]
- Scott LL, Hage TA, Golding NL. Weak action potential backpropagation is associated with high-frequency axonal firing capability in principal neurons of the gerbil medial superior olive. J Physiol 583: 647–661, 2007. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Scott LL, Mathews PJ, Golding NL. Posthearing development refinement of temporal processing in principal neurons of the medial superior olive. J Neurosci 25: 7887–7895, 2005. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Schwartz IR. Dendritic arrangements in the cat medial superior olive. Neuroscience 2: 81–101, 1977. [DOI] [PubMed] [Google Scholar]
- Segev I, Burke RE. Compartmental models of complex neurons. In: Methods in Neuronal Modeling: from Ions to Networks, edited by Koch C, Segev I. Cambridge, MA: MIT Press, 1998. [Google Scholar]
- Stacey RG, Hilbert L, Quail T. Computational study of synchrony in fields and microclusters of ephaptically coupled neurons. J Neurophysiol 113: 3229–3241, 2015. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Strumwasser F, Rosenthal S. Prolonged and patterned direct extracellular stimulation of single neurons. Am J Physiol 198: 405–413, 1960. [DOI] [PubMed] [Google Scholar]
- Su CY, Menuz K, Reisert J, Carlson JR. Non-synaptic inhibition between grouped neurons in an olfactory circuit. Nature 492: 66–71, 2012. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Traub RD, Dudek FE, Taylor CP, Knowles WD. Simulation of hippocampal afterdischarges synchronized by electrical interactions. Neuroscience 14: 1033–1038, 1985. [DOI] [PubMed] [Google Scholar]
- Tsuchitani C, Boudreau JC. Wave activity in the superior olivary complex of the cat. J Neurophysiol 27: 814–827, 1964. [DOI] [PubMed] [Google Scholar]
- Tuckwell HC. Introduction to Theoretical Neurobiology. Vol. 1, Linear Cable Theory and Dendritic Structure. New York: Cambridge Univ. Press, 1988, p. 130. [Google Scholar]
- van der Heijden M, Lorteije JA, Plauska A, Roberts MT, Golding NL, Borst JG. Directional hearing by linear summation of binaural inputs at the medial superior olive. Neuron 78: 936–948, 2013. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Weiss SA, Preuss T, Faber DS. A role of electrical inhibition in sensorimotor integration. Proc Natl Acad Sci USA 105: 18047–18052, 2008. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Weisstein EW. Circle Packing. Wolfram MathWorld http://mathworld.wolfram.com/CirclePacking.html [3 March 2015].
- Yin TC, Chan JC. Interaural time sensitivity in medial superior olive of cat. J Neurophysiol 64: 465–488, 1990. [DOI] [PubMed] [Google Scholar]
- Zhang M, Ladas TP, Qui C, Shivacharan RS, Gonzales-Reyes LE, Durand DM. Propagation of epileptiform activity can be independent of synaptic transmission, gap junctions, or diffusion and is consistent with electrical field transmission. J Neurosci 34: 1409–1419, 2014. [DOI] [PMC free article] [PubMed] [Google Scholar]