Posterior Corneal Aberrations and Their Compensation Effects on Anterior Corneal Aberrations in Keratoconic Eyes

Abstract

Purpose

To characterize posterior corneal aberrations in keratoconic (KC) eyes and investigate compensatory effects between anterior and posterior corneal surfaces.

Methods

The corneal topography of 113 eyes (37 advanced KC, 31 moderate KC, 14 mild KC, and 31 normal eyes) was used to compute the corneal aberrations. The central 6-mm diameter of both anterior and posterior corneal topographies was decomposed into Zernike polynomials. The magnitude and the orientation of each posterior corneal aberration were calculated by vector analysis. The compensation effects between anterior and posterior corneal aberrations were also assessed quantitatively with a linear regression model.

Results

The average higher order RMS wavefront errors for the posterior corneas were 1.04, 0.54, 0.24, and 0.19 µm in the advanced, moderate, and mild KC and normal eyes, respectively. In the advanced KC eyes, posterior corneal coma was oriented in the superior–nasal direction with a mean orientation angle of 75° ± 19° OD and 78° ± 20° OS. On average, 22%, 24%, and 14% of the anterior corneal coma were compensated by the posterior cornea in the advanced, moderate, and mild KC eyes, respectively. However, no significant higher order aberration (HOA) compensation effects were found in normal corneas.

Conclusions

Significantly larger amounts of posterior corneal aberrations and stronger compensation effects were observed in KC eyes than in normal eyes. The uncorrected posterior corneal aberration in KC eyes was substantial and degraded retinal image quality. This may explain the relatively poor visual acuity obtained in eyes with rigid gas permeable (RGP) lenses, which correct only anterior corneal aberrations.

Keratoconus is a noninflammatory corneal disorder characterized by increased anterior corneal aberrations.^1,2 By masking the patient’s irregular anterior cornea with a regular spherical lens surface, rigid gas permeable (RGP) lenses provide aberration correction. Reduction of higher order aberrations (HOAs) was observed in KC eyes with these lenses.^3–5 However, compared to normal eyes, a relatively larger amount of HOA was still observed in KC eyes, with RGP lenses leading to worse visual performance.^6,7 The measured residual aberration could be attributed to internal ocular aberrations generated by both the posterior corneal surface and crystalline lens.^8–11 Assuming that there is no significant difference in the aberration of the crystalline lens between normal and KC eyes, the larger residual aberration is more likely caused by the posterior cornea. Using the back surface customized soft contact lens to neutralize KC eyes’ anterior corneal aberration, Chen et al.⁸ observed that the measured internal ocular aberration was mainly attributable to posterior corneal aberrations. The irregular posterior cornea in KC eyes could generate amounts of aberrations significantly larger than those in normal eyes, thus playing a major role in the internal optics aberrations. Investigating the posterior corneal aberrations provides a better understanding of the contributions of posterior aberration to the internal optical aberrations and how they differ between normal and KC eyes. It also has been reported that the internal ocular aberrations are opposite to anterior corneal aberrations, showing a compensation mechanism between the two.^8,12 Since posterior corneal aberrations are the major source of internal ocular aberrations in KC eyes,⁸ a similar compensation effect may exist between anterior and posterior corneal aberrations. The compensation mechanism between the anterior and posterior corneal surfaces would be especially significant in KC eyes, because of the substantial increment of both anterior and posterior corneal aberrations.

Despite its significance, however, there have been no studies focused on posterior corneal aberrations and its compensatory effect. In this study, we systematically investigated the amplitude and orientation of the posterior corneal aberrations in the advanced, moderate, and mild KC eyes and compared them with those in normal eyes. The posterior corneal aberration’s compensatory effects on the anterior corneal aberrations were also quantitatively assessed in KC eyes with different degrees of severity.

Methods

The corneal topography of 31 normal eyes and 82 KC eyes (37 advanced, 31 moderate, 14 mild, classified based on the CLEK [Collaborative Longitudinal Evaluation of Keratoconus] recommendation¹³) was included in the study. Informed consent was obtained from all patients and the tenets of the Declaration of Helsinki were followed for all study procedures. Both anterior and posterior corneal topographies were measured with a corneal analysis system (Orbscan IIz; Bausch and Lomb, Rochester, NY). Previous studies^14–16 indicated that corneal topography in general centered on the corneal apex, whereas Shack-Hartmann measurements used the center of the entrance pupil of the eye as the origin for wavefront calculation. To make the measured topography data comparable with other wavefront experiment results, all the corneal topography calculations were realigned with the corneal sighting center as the elevation data origin, which was the intersection between the line of sight and the corneal surface. For the corneal analysis system, the anterior and posterior corneal sighting center could be identified as the projection of the pupil center on the anterior and posterior corneal surfaces, respectively. Using a program written in commercial software (MatLab; The MathWorks, Natick, MA) program, only the central 6-mm of both the anterior and posterior corneal raw elevation data were fitted with a Cartesian oval (or spherical aberration free) surface,¹⁷ which was aligned with the sighting center. Then, the difference map was decomposed with Zernike polynomials. Although the system provides a 10-mm diameter zone by default, we used only the center 6-mm diameter of the elevation data to calculate the Cartesian oval surface with our custom program. Wavefront aberrations were calculated from the anterior and posterior corneal elevation difference map multiplied by the refractive index difference between the cornea and two media (air for the anterior cornea and aqueous for the posterior cornea). We used refractive index differences of 0.376 and −0.04 for the anterior and posterior corneal aberrations computation, respectively. The Zernike defocus mode (Z₂⁰) was excluded from the analysis, because it varies with the curvature of the reference surface that was used to fit the elevation data. Since bilateral symmetry exists between right and left eyes,¹⁸ according to the recommendation of Thibos et al.,¹⁹ the Zernike coefficients for the right eye and left eye were expressed with a right-hand and left-hand Cartesian coordinate system, respectively, in this article. By this definition, the positive x-axis direction is toward the nasal direction for both eyes, which allowed the bilateral symmetry of wavefront aberrations to be compared directly. All Zernike coefficients were finally reduced to Zernike vectors according to methods proposed by Campbell²⁰ and used in previous studies.^10,21 With this method, each pair of Zernike modes (Z_n^m and Z_n^−m) were combined into a vector V⃗(M,θ) represented by its magnitude (M) and orientation angle (θ).

The magnitude of each aberration expressed as the root mean square (RMS) wavefront error was calculated by the formula

$$M=\sqrt{{({C_n}^{-m})}^2+{C_n}^m{)}^2}\hspace{1em}(\text{if }m\ne 0)$$ (1)

and

$$M=|{C_n}^0|\hspace{1em}(\text{if }m=0),$$ (2)

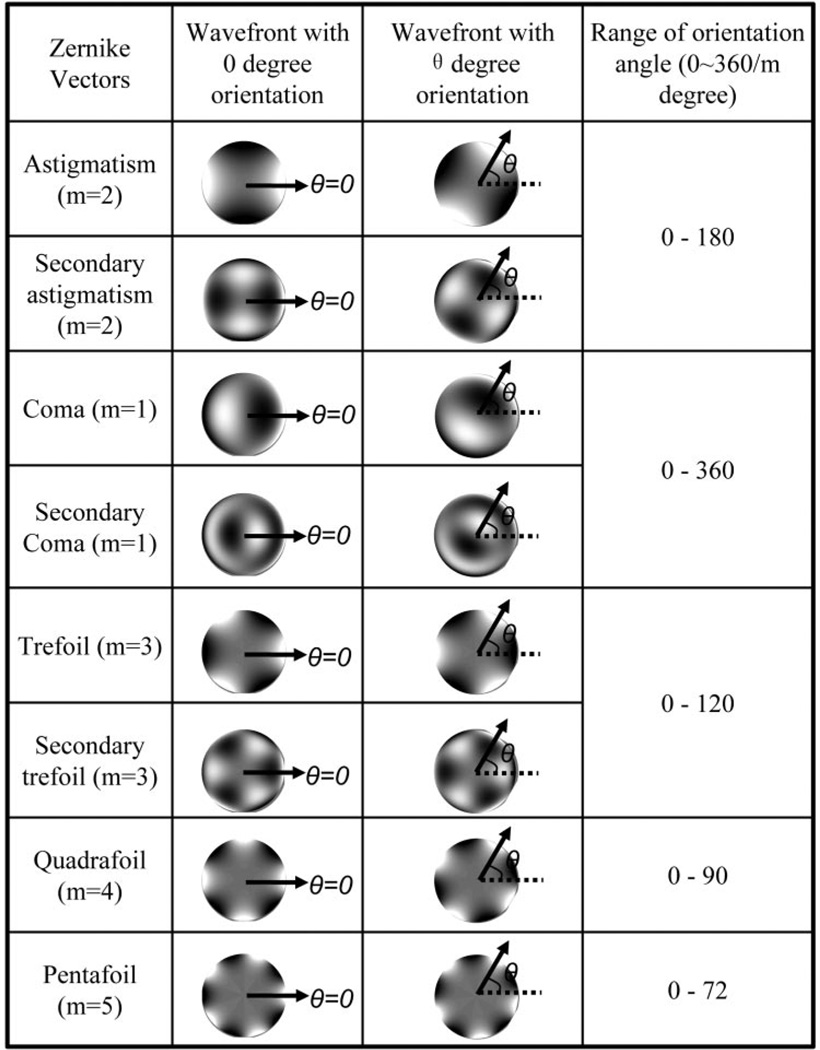
where C_n^m, C_n^−m and C_n⁰ are the Zernike coefficients of the corresponding Zernike modes Z_n^m, Z_n^−m and Z_n⁰, respectively, and M is the magnitude of the aberration. The orientation angle (θ) calculated using the following formula is illustrated in Figure 1 for different aberrations.

$$\theta =\{\begin{array}{c}\frac{1}{m}{\text{tan}}^{-1}\left(\frac{{C_n}^{-m}}{{C_n}^m}\right)\hspace{1em}\text{if }({C_n}^m>0)\hfill \\ \frac{1}{m}\left({\text{tan}}^{-1}\right(\frac{{C_n}^{-m}}{{C_n}^m})+\text{180}°)\hspace{1em}\text{if }({C_n}^m<0)\hfill \\ \frac{1}{m}90°\hspace{1em}\text{if }({C_n}^m=0,{C_n}^{-m}>0)\hfill \\ \frac{1}{m}270°\hspace{1em}\text{if }({C_n}^m=0,{C_n}^{-m}<0).\hfill \end{array}$$ (3)(4)(5)(6)

if m = 0 (spherical aberrations), corresponding aberrations are radially symmetric and there is no azimuthal orientation associated with it.

Figure 1.

The definition of the orientation angle (θ) for each aberration vector. Arrows: the orientation of each aberration and the angle (θ) between the arrow and x-axis (dashed line) are defined as the orientation angle for each aberration vector. For different aberrations, the orientation angle covers different ranges (from 0 to 360/m degree) because of rotational symmetry. (Bright regions indicate a phase-advanced wavefront.)

Using these formulas, we can compute the orientation angle of both anterior and posterior corneal aberrations. Therefore, the orientation angle difference between anterior and posterior corneal aberrations can be calculated. In this article, Δβ, defined as the angular difference, is used to represent the orientation angle difference between the anterior aberration vector (A⃗) and the posterior aberration vector (P⃗). The angular difference was calculated and rescaled in the range of 0° to 180° by the following formula:

$$\mathrm{\Delta }\beta =\{\begin{array}{c}m·|{\theta }_{\mathrm{A}}-{\theta }_{\mathrm{P}}|\hspace{1em}\text{if }m·|{\theta }_{\mathrm{A}}-{\theta }_{\mathrm{P}}|\le 180°\hfill \\ 360-m·|{\theta }_{\mathrm{A}}-{\theta }_{\mathrm{P}}|\hspace{1em}\text{if }m·|{\theta }_{\mathrm{A}}-{\theta }_{\mathrm{P}}|>180°\hfill \end{array},$$ (7)

where θ_A and θ_P are the orientation angles of anterior aberration vector (A⃗) and posterior aberration vector (P⃗), respectively. The use of Δβ to represent the angular difference between the two vectors allowed uniformity of range from 0 to 180° for different sets of aberrations, thus facilitating easy comparison. As an example, for two aberrations with opposite directions, although |θ_A − θ_P| equals 90°, 60°, 180°, 45°, 90°, 36°, 60°, and 180° for astigmatism (m = 2), trefoil (m = 3), coma (m = 1), quadrafoil (m = 4), secondary astigmatism (m = 2), pentafoil (m = 5), secondary trefoil (m = 3), and secondary coma (m = 1) respectively, the value of Δβ is always 180°.

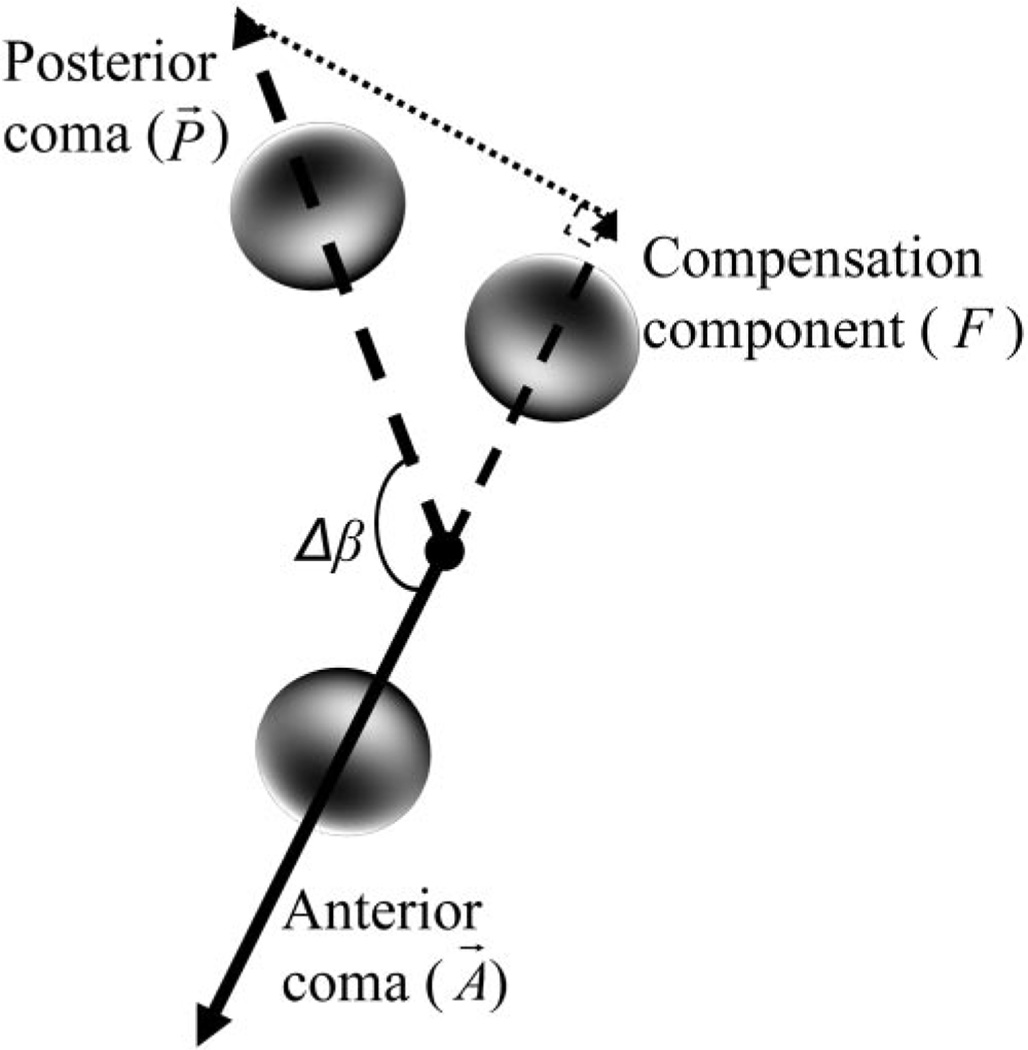
To calculate the compensation effect between anterior and posterior corneal aberration, we decomposed the posterior corneal aberration (P⃗) into a component (F), defined as the compensation component, whose direction was parallel to the direction of the anterior corneal aberration vector (A⃗). The following formula was used to calculate the compensation component:

$$F=M_{\mathrm{P}}·\text{cos}(\mathrm{\Delta }\beta )\hspace{1em}\text{if }m\ne 0$$ (9)

and

$$F={({C_n}^0)}_{\mathrm{p}}\hspace{1em}\text{if }m=0,$$ (10)

where F was the compensation component, M_P was the magnitude of the posterior corneal aberration vector, and (C_n⁰)_p was the Zernike coefficients of the posterior corneal aberration. For spherical aberration (m = 0), both anterior and posterior aberrations were not vectors and F was equal to the Zernike coefficient of posterior corneal aberration. Using coma as an example, Figure 2 explains the method for calculating the compensation component (F). For each patient and specific aberration, the percentage of the anterior corneal aberration compensated by posterior cornea was obtained by calculating the ratio of F and the magnitude of the anterior corneal aberration, M_A (when m ≠ 0), or the ratio of F and the anterior corneal aberration Zernike coefficient, (C_n⁰)_A (when m = 0). To get the average compensation factor (k), we computed the the linear regression (with 0 y-axis interception) between F and the anterior corneal aberration M_A (for aberrations with m ≠ 0) or between F and the anterior corneal aberration Zernike coefficient (C_n⁰)_A (for aberrations with m = 0) for all subjects. The slope (k) of the fitted line indicated that the average compensation factor and the determination factor (R²) was a measure of how strictly this percentage was followed in each subject in the investigated group.

Figure 2.

The method of calculating the compensation component (F) for Zernike coma (m = 1). Δβ is the angular difference between the anterior coma and posterior coma. (Bright regions indicate a phase-advanced wavefront.)

Results

Posterior Corneal Aberrations

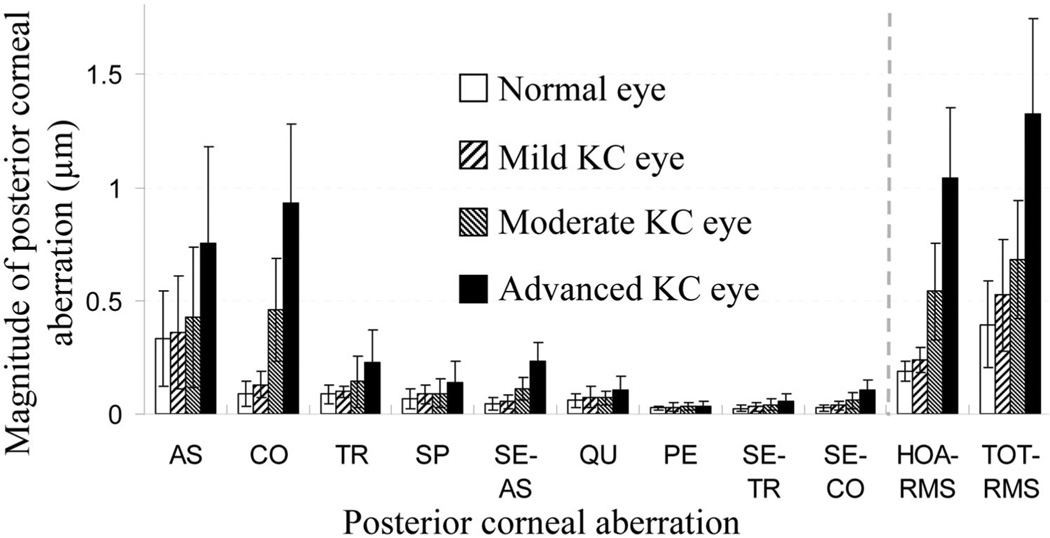
Figure 3 compares the magnitude of the posterior corneal aberrations among different groups of eyes (defocus term was excluded). Significantly larger amounts of posterior corneal aberrations were observed in the KC eyes than in the normal eyes. Average astigmatism was 0.76, 0.43, 0.36, and 0.33 µm in the advanced, moderate, and mild KC and normal eyes, respectively. The average (±SD) HOA RMS value in normal eyes was 0.19 ± 0.05 µm. This value was increased to 1.04 ± 0.31, 0.54 ± 0.21, and 0.24 ± 0.05 µm for advanced, moderate, and mild KC eyes, respectively. The average RMS value of coma, the most dominant posterior corneal HOA in KC eyes, was 0.93 ± 0.35, 0.46 ± 0.23, 0.12 ± 0.06, and 0.09 ± 0.06 µm, respectively, for advanced, moderate, and mild KC and normal eyes, respectively. A more than 10-fold RMS increase was observed between advanced KC and normal eyes. In normal eyes, the RMS errors of trefoil, spherical aberration, secondary astigmatism, and quadrafoil were 0.09, 0.07, 0.04, and 0.06 µm, respectively. Whereas in the advanced KC eyes, the RMS values of these aberrations increased to 0.23, 0.14, 0.23, and 0.10 µm. The similar RMS value increasing in KC eyes was also found in fifth-order aberrations. The average RMS value for pentafoil, secondary trefoil, and secondary coma was 0.02, 0.02, and 0.03 µm, respectively, in normal eye group and increased to 0.03, 0.06, and 0.10 µm, respectively, in the advanced KC eyes. As shown in Table 1, the Student’s t-test was performed to compare the magnitude of aberrations among the four groups of eyes. For all aberrations, a general trend could be observed with the comparisons between advanced KC and normal eyes and between mild KC and normal eyes. This trend was that a significant difference (P < 0.05) was found between advanced KC and the normal eyes, whereas there was no significant difference between the mild KC and normal eye groups. For all other comparisons that were not covered by this result, coma, trefoil, secondary astigmatism, and secondary coma showed a significant difference, whereas there was no significant difference observed with pentafoil. This indicated that, compared with other aberrations, pentafoil had the least change with the progression of keratoconus. Besides the nonsignificant difference observed between mild KC and normal eyes, astigmatism, secondary trefoil, and spherical aberration showed a nonsignificant difference for moderate KC versus mild KC, and spherical aberration also showed a nonsignificant difference for moderate KC versus normal eyes. Although for quadrafoil, besides a significant difference observed between the advanced KC and normal eyes, only advanced and moderate KC eyes showed a significant difference.

Figure 3.

The average magnitude with SD of posterior corneal aberrations, including astigmatism (AS), coma (CO), trefoil (TR), spherical aberration (SP), secondary astigmatism (SE-AS), quadrafoil (QU), pentafoil (PE), secondary trefoil (SE-TR), and secondary coma (SE-CO). The defocus term was not included in the total RMS.

Table 1.

Student t-Test Results, Comparing the Magnitude of Posterior Corneal Aberrations

	Aberration
	AS	CO	TR	SP	SE-AS	QU	PE	SE-TR	SE-CO
AD vs. MO	*	*	*	*	*	*	NS	*	*
AD vs. MI	*	*	*	*	*	NS	NS	*	*
AD vs. NO	*	*	*	*	*	*	*	*	*
MO vs. MI	NS	*	*	NS	NS	NS	NS	NS	*
MO vs. NO	*	*	*	NS	NS	NS	NS	*	*
MI vs. NO	NS	NS	NS	NS	NS	NS	NS	NS	NS

AD, advanced KC; AS, astigmatism; CO, coma; MI, mild KC; MO, moderate KC; NO, normal; PE, pentafoil; QU, quadrafoil; SE-AS, secondary astigmatism; SE-CO, secondary coma; SE-TR, secondary trefoil; SP, spherical aberration; TR, trefoil.

^*P < 0.05; NS, P ≥ 0.05.

The orientation of each aberration vector is listed in Table 2. Astigmatism had the similar average orientation angle and SD in both KC and normal eyes. However, coma had obviously different features in KC and normal eyes. In the advanced KC eyes, the average orientation angle of posterior corneal coma was 76°, indicating a positive coma dominant aberration that was opposite the negative coma dominant aberration found in the anterior cornea. In normal eyes, coma oriented randomly from 0° to 360° with an average of 192° and a wide range of intersubject variability, which was seven times larger than the variability of the advanced KC eyes. A similar feature of intersubject variability reduction in more severe KC eyes was also observed with secondary coma. Comparing with normal eyes, its average orientation angle was about two times larger in the advanced KC eyes, but the intersubject variability was less than half of that in normal eyes. Trefoil had a larger orientation angle in the advanced and moderate KC eyes, and the intersubject variability was also slightly larger in comparison to that in normal eyes. All other aberrations had the similar orientation angles and intersubject variability among the four groups of eyes. We also investigated orientation angle symmetry for both right and left eyes, and clear mirror symmetry was observed only with coma and secondary coma in the advanced KC eyes. Posterior corneal coma was oriented in a superior–nasal direction for both right and left eyes with an orientation angle of 75 ± 19° OD and 78 ± 20° OS, respectively. Secondary coma was oriented toward the inferior–temporal direction with an orientation angle of 238 ± 39° OD and 237 ± 40° for OD, respectively.

Table 2.

The Averaged Orientation Angle ± SD of Posterior Corneal Aberrations among the Four Groups of Eyes

	Aberration
	AS	CO	TR	SE-AS	QU	PE	SE-TR	SE-CO
AD	93 ± 42	76 ± 19	54 ± 43	104 ± 53	55 ± 25	34 ± 24	77 ± 23	238 ± 39
MO	83 ± 57	101 ± 67	61 ± 35	104 ± 52	47 ± 29	32 ± 23	82 ± 32	227 ± 68
MI	86 ± 41	107 ± 80	35 ± 20	88 ± 53	38 ± 22	30 ± 24	71 ± 42	199 ± 97
NO	83 ± 60	192 ± 133	33 ± 28	105 ± 53	46 ± 28	31 ± 22	77 ± 33	100 ± 88

Values are listed in degrees. Abbreviations are as in Table 1.

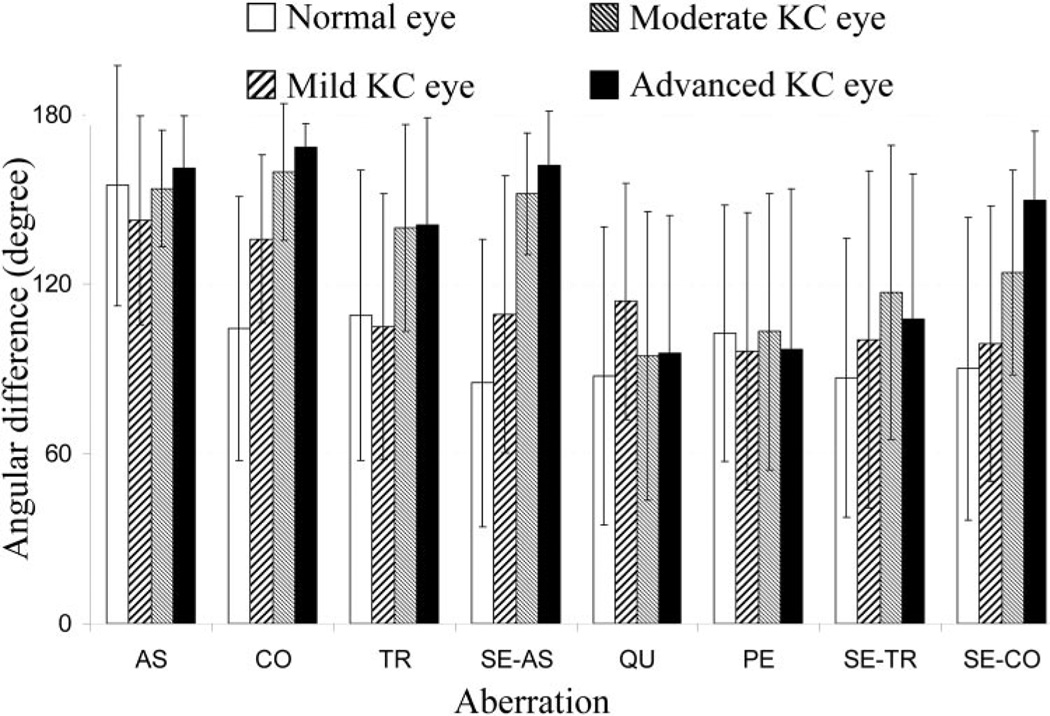
For each eye, the angular difference between anterior and posterior corneal aberration was calculated by the method described earlier. Figure 4 shows the angular difference between the anterior and posterior corneal aberrations and Table 3 summarizes the Student t-test comparison of the angular difference among all groups of eyes. As described earlier, the angular difference between anterior and posterior aberrations always ranged between 0° and 180°. For astigmatism, the average angular difference ± SD was 161 ± 18°, 154 ± 21°, 143 ± 37°, and 154 ± 42° for the advanced, moderate, and mild KC and normal eyes, respectively. The Student t-test did not show a significant difference (P > 0.05) in angular difference with astigmatism among KC and normal eye groups, as shown in Table 3. The angular difference of coma aberration showed a significant difference among all groups. The average angular difference was 168 ± 9°, 160 ± 26°, 122 ± 32°, and 111 ± 47° in the advanced, moderate, and mild KC and normal eyes, respectively. With an increase of severity of keratoconus, the average angular difference for coma tended to be closer to 180° (opposite direction). This trend was also observed in trefoil, secondary astigmatism, and secondary coma. The trend was not observed for quadrafoil, pentafoil, and secondary trefoil. For astigmatism, coma, trefoil, secondary astigmatism, and secondary coma, smaller intersubject variability (SD) of angular difference was observed in the advanced and moderate KC eyes than in mild KC and normal eyes. Clinically, the comparison of the angular difference between mild KC and normal eyes was especially important, since it might be able to provide preliminary insight to identify early stage KC eyes. A significant difference was found in coma (P = 0.01) and secondary astigmatism (P = 0.02), which indicated that the angular difference in these aberrations may be used for the early diagnosis of keratoconus.

Figure 4.

The angular difference (and its SD) between anterior and posterior corneal aberrations. AS, astigmatism; CO, coma; PE, pentafoil; QU, quadrafoil; SE-AS, secondary astigmatism; SE-CO, secondary coma; SE-TR, secondary trefoil; SP, spherical aberration.

Table 3.

The t-Test Comparison of the Angular Difference between Anterior and Posterior Corneal Aberrations

	Aberration
	AS	CO	TR	SE-AS	QU	PE	SE-TR	SE-CO
AD vs. MO	NS	*	NS	*	NS	*	NS	*
AD vs. MI	NS	*	*	*	NS	NS	NS	*
AD vs. NO	NS	*	*	*	NS	NS	NS	*
MO vs. MI	NS	*	*	*	NS	NS	NS	*
MO vs. NO	NS	*	*	*	NS	NS	*	*
MI vs. NO	NS	*	NS	*	NS	NS	NS	NS

Abbreviations are as in Table 1.

^*P < 0.05 and NS, P ≥ 0.05.

Compensation between the Anterior and Posterior Cornea

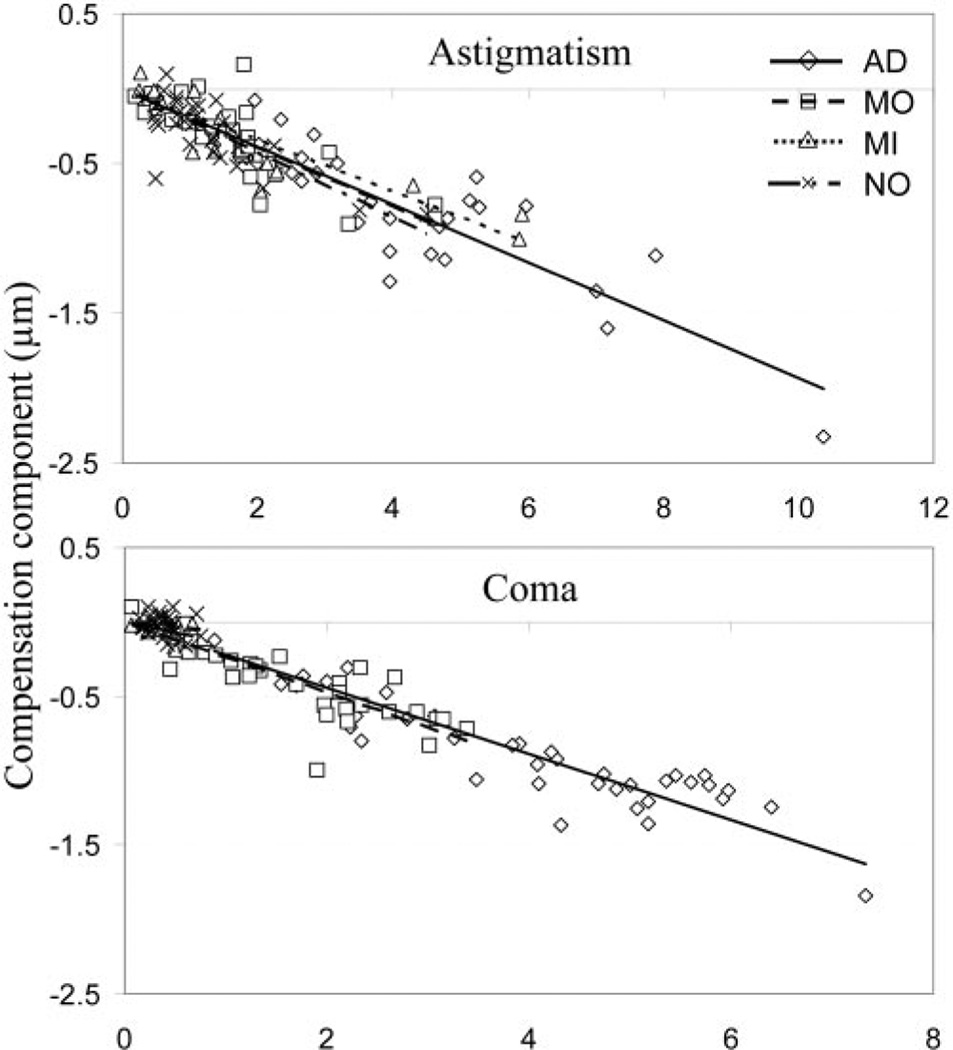
We next examined the average percentage of the anterior corneal aberration compensated by the posterior corneal aberrations. In Figure 5, the compensation component (F) is plotted as a function of the magnitude of the anterior corneal aberration for astigmatism and coma. By fitting the data with a linear regression model, we obtained the average percentage of the anterior corneal aberration compensated by the posterior corneal aberration, as the slope of the regression line. Table 4 lists the slopes (k) and determination factors (R²) for all the aberrations within the four groups of eyes. Negative k values indicate a compensatory effect existing between the two corneal surfaces. Whereas a positive slope (k) indicates that the posterior corneal aberration augments the anterior corneal aberration.

Figure 5.

The linear regression of the relation between the compensation component (F) and the magnitude of anterior corneal aberration for both astigmatism and coma. AD, advanced KC; MI, mild KC; MO, moderate KC; NO, normal.

Table 4.

The Slopes and Determination Factors for All the Aberrations within the Four Groups of Eyes

	Aberration
	AS	CO	TR	SP	SE-AS	QU	PE	SE-TR	SE-CO
AD
k	−0.19	−0.22	−0.17	−0.20	−0.21	−0.05	−0.02	−0.05	−0.16
(R2)	(0.79)	(0.82)	(0.40)	(0.74)	(0.33)	(0.04)	(0.01)	(0.01)	(0.25)
MO
k	−0.20	−0.24	−0.19	−0.19	−0.24	0.05	−0.05	−0.1	−0.15
(R2)	(0.64)	(0.64)	(0.49)	(0.20)	(0.17)	(0.03)	(0.01)	(3e-3)	(0.44)
MI
k	−0.17	−0.14	−0.11	−0.33	−0.11	−0.03	−0.08	−0.07	−0.08
(R2)	(0.78)	(0.20)	(0.13)	(2e-3)	(2e-3)	(0.03)	(0.05)	(0.02)	(0.04)
NO
k	−0.21	−0.06	−0.12	−0.18	0.01	0.01	−0.05	0.01	−0.01
(R2)	(0.57)	(0.07)	(0.06)	(0.02)	(1e-3)	(3e-3)	(0.03)	(2e-5)	(0.01)

The slope (k) and determination factor (R²) of the linear fitting of compensation component against the magnitude of anterior corneal aberration (when m ≠ 0), or the fitting of compensation component against the anterior corneal Zernike coefficients (when m = 0).

Abbreviations are as in Table 1.

On average, 19%, 20%, 17%, and 21% of anterior corneal astigmatism was compensated by posterior corneal astigmatism in the advanced, moderate, and mild KC and normal eyes with a determination factor equaling 0.79, 0.64, 0.78, and 0.57 for the four groups of eyes, respectively. Advanced, moderate, and mild KC had 22%, 24%, and 14%, respectively, of anterior corneal coma compensated by the posterior cornea. However, significant variability was found among the normal eyes (R² = 0.07), indicating that the compensation effect for coma was not consistent within the normal eye group. As indicated by determination factor (R²), the general trend for coma was that the compensation effect had increasingly stronger correlation with the increase of the severity of keratoconus. A similar trend was found with the trefoil, spherical aberration, secondary astigmatism, and secondary coma. For these aberrations, in general, larger R² values were observed in more severe KC eyes than in those of normal eyes. No significant compensation effect was observed for quadrafoil, pentafoil, and secondary trefoil in all KC and normal eyes since R² were rather small (≤0.05) for all groups of eyes.

Discussion

Corneal Surface Irregularity and Its Compensation Effects

Keratoconus is a condition, in which the cornea assumes a conical shape due to localized thinning of the corneal stroma.^1,22 Corneal thinning induces significant surface irregularities^23–25 and HOAs.^26,27 The induced anterior and posterior corneal surface irregularities contribute to the corneal HOA,²⁶ which was computed by using the surface profile multiplied by the refractive index difference between the cornea and the media. That the sign of refractive index changing from the aqueous to the cornea (n_aqueous − n_cornea = −0.04) was opposite that from the cornea to air (n_cornea − n_air = 0.376) showed that there was an aberration compensation effect between the anterior and posterior cornea if their irregular surface profiles had the same direction. Because the radius of corneal curvature was much larger than corneal thickness, assuming that the corneal thickness was uniform (anterior and posterior corneal surfaces are parallel with each other), both the anterior and posterior cornea would have similar surface profile. Thus, approximately 10.8% (0.04/0.376, representing the anteriorposterior corneal aberration ratio equaling the ratio of the refractive index difference between the cornea and aqueous and the cornea and air) of the anterior corneal aberrations were compensated by the posterior corneal aberrations. Our experiment showed that, in KC eyes, several major corneal HOAs had more than 10.8% anterior corneal aberration compensated by posterior cornea. This result indicated that the posterior corneal surface profile was more irregular than that of the anterior corneal surface in KC eyes.

One hypothesis to explain this phenomenon is that, as keratoconus progresses, both the anterior and posterior corneal surfaces protrude, forming a conelike structure with significantly increased irregularities. However, because of the local thinning effect in the central corneal area, the posterior cornea had a shaper surface profile than that of the anterior cornea. Thus, more irregularities developed in the posterior cornea than in the anterior cornea.

Corneal surface parallelism also depends on the magnitude of individual lower and higher order aberrations. Recent studies²⁸ of the corneal thickness spatial profile have shown KC eyes to have a more abrupt increase in corneal thickness than that of the normal eye group from the thinnest point toward its periphery. Thus, the KC cornea may have worse parallelism than the normal cornea.

Furthermore, because the anterior corneal aberration would be partially cancelled by posterior corneal aberration because of the compensation effects, if a compensation effect existed, we would expect to observe a reduction in total corneal aberrations comparing with the anterior corneal aberration. In our experiments, an average lower order aberration (astigmatism) compensation effect was observed in both the KC and the normal eyes. As for HOAs, there is no average HOA compensation effect found within the normal eyes. However, in the KC eyes, the compensation effect was observed with several major HOAs, and this effect was especially stronger (as indicated by R²) in the more severe KC eye groups. We calculated the magnitude of HOAs for both the anterior cornea and the total cornea. For the anterior cornea, 4.50 ± 1.30, 2.08 ± 0.83, 0.59 ± 0.18 and 0.53 ± 0.1 µm of HOA RMSs were observed in the advanced, moderate, and mild KC and normal eyes, respectively, whereas for the total cornea these values were reduced to 3.54 ± 1.2, 1.64 ± 0.67, 0.54 ± 0.17, and 0.51 ± 0.10 µm. Using the Student t-test, we also compared the anterior corneal and total corneal HOA. Significant HOA reduction in total cornea was observed in the advanced and moderate KC groups (P = 0.003 and 0.024, respectively) because of the posterior corneal HOA compensation effect. In the group with less severe keratoconus, the total corneal HOA reduction was not significant (P = 0.43 and 0.51 for mild KC and normal eyes, respectively) because of the reduced posterior corneal compensation effect in these groups of eyes.

Although the cornea has a layered structure, in our calculations we used the single uniform refractive index of the entire cornea. We verified whether the corneal epithelium has a significant impact on the corneal aberration. We computed the total corneal spherical aberration when assuming a 40-µm thick-epithelium (n = 1.401) and a 460-µm thick stroma (n = 1.376) with a 7-mm radius of curvature. The spherical aberration of this model cornea demonstrated negligibly small differences (2.7e-4 µm) from that calculated assuming the singlelayer cornea (500-µm stroma). However, it is important to note that the nonuniform distribution²⁹ of the epithelial layer could cause the larger difference due to other asymmetric higher order aberrations.

Clinical Implication

Previous research^6,7 has shown significantly larger total ocular HOA and lower visual performance in KC eyes with RGP lenses than that in normal eyes. From the present study, we found that the significantly large amounts of posterior corneal aberrations contributed to the large HOAs, which were not corrected by RGP lenses in the KC eyes. This may explain the clinical observation that KC eyes with RGP lenses have poorer visual acuity than that in normal eyes. Since RGP lenses correct only anterior corneal lower and higher order aberrations, significant amounts of residual posterior corneal HOAs, in KC eyes would limit the visual benefit obtainable with conventional RGP lenses.

The magnitude of posterior corneal aberrations is much smaller than that of the anterior cornea due to the smaller refractive index change between the aqueous and cornea than between the cornea and air. This factor is the major reason that the posterior corneal HOAs have insignificant contributions to the total ocular aberrations in normal eyes. However, the average posterior corneal HOA RMS for advanced KC eyes was 1.04 µm for 6-mm pupil which was more than five times larger than that of normal eyes. Moreover, this value was about two times larger than the average HOA RMS (~0.51 µm) of total corneal HOAs of naked normal eyes. This finding suggests that when correcting advanced KC eyes with a conventional RGP lens, visual performance would be significantly worse than normal eyes due to the uncorrected posterior corneal aberrations.

With an RGP lens on the keratoconic cornea, a small amount of residual aberrations could still be induced from the anterior corneal surface due to the refractive index difference between the cornea (n_cornea = 1.376) and tear (n_tear = 1.336). These residual aberrations compensate for some of the posterior aberrations. When taking this into consideration, the total residual HOA RMS in the patients with RGP lenses was 0.80, 0.50, and 0.34 µm for advanced, moderate, and mild KC, respectively. These results still suggest that the impact of the posterior corneal aberrations on visual performance is more significant with an increase in degrees of keratoconus, and at least advanced KC eyes would have poorer visual performance than normal eyes. Other factors, such as lens decentration could induce the total ocular wavefront aberration in KC eyes with RGP lenses. More studies are needed to investigate the impact of posterior corneal aberration on visual function.

Another potential clinical application is the use of angular difference between anterior and posterior aberrations for both coma and secondary astigmatism, to diagnose keratoconus in its early stage since a significant difference was found between the mild KC and normal eyes. It was also interesting to note that the magnitude of the anterior and posterior corneal aberrations did not distinguish the mild KC and normal eye group. Further investigation on the keratoconus suspect patients is needed to confirm this observation.

In conclusion, significantly larger amounts of posterior corneal aberrations were found in keratoconic corneas than in normal corneas. The posterior coma and secondary coma showed mirror symmetry between the left and right eyes in the advanced KC group. The posterior corneal aberration compensation effect, which partially cancelled anterior corneal aberrations, was also observed and was especially stronger in the advanced KC eyes. These aberrations contributed to the uncorrected residual aberrations in the KC eyes with RGP lenses.