Skip to main content
PMC home page
PLOS ONE logoLink to PLOS ONE
. 2016 May 25;11(5):e0155246. doi: 10.1371/journal.pone.0155246

An Integrated View of Whole-Tree Hydraulic Architecture. Does Stomatal or Hydraulic Conductance Determine Whole Tree Transpiration?

Juan Rodríguez-Gamir 1, Eduardo Primo-Millo 1, María Ángeles Forner-Giner 1,*
Editor: Manuel Reigosa2
PMCID: PMC4880183  PMID: 27223695

Abstract

Hydraulic conductance exerts a strong influence on many aspects of plant physiology, namely: transpiration, CO2 assimilation, growth, productivity or stress response. However we lack full understanding of the contribution of root or shoot water transport capacity to the total water balance, something which is difficult to study in trees. Here we tested the hypothesis that whole plant hydraulic conductance modulates plant transpiration using two different seedlings of citrus rootstocks, Poncirus trifoliata (L.) Raf. and Cleopatra mandarin (Citrus reshni Hort ex Tan.). The two genotypes presented important differences in their root or shoot hydraulic conductance contribution to whole plant hydraulic conductance but, even so, water balance proved highly dependent on whole plant conductance. Further, we propose there is a possible equilibrium between root and shoot hydraulic conductance, similar to that between shoot and root biomass production, which could be related with xylem anatomy.

Introduction

Transpiration in plants plays a critical role in plant physiological processes, affecting carbon uptake by leaves, growth and productivity and depends on plant hydraulics, stomatal conductance and/or environment conditions [1, 2, 3, 4]. Plant hydraulics constrains ecosystem productivity by setting physical limits to water transport [5, 6], thus its study is essential to understanding plant water-use regulation and its associated impact on water balance.

Hydraulic conductance can be variable throughout the day [7] or in response to environmental conditions [8, 9]. Besides, maximum transpiration rates have been related to maximum values of whole plant hydraulic conductance [10]. Studies suggest that hydraulic conductance of different plant organs can regulate the opening and closing of stomata in response to vapor pressure deficits [11, 12], regulating, thus, plant water relations. In this respect, there are existing studies that link hydraulic conductance of leaf [13, 14, 15, 16, 17, 18, 19], shoot [20], stems [21, 22] or root [23, 24, 25] with transpiration or stomatal conductance. However, there is a lack of understanding about the integration of whole woody plant hydraulics and its implication in water relations. As [26] state, few studies have examined all the organ components of the hydraulic pathway to evaluate how they relate to whole plant conductance and most work on hydraulic transport of woody plants has been conducted on lateral stems or branches. Regarding different organ contribution, [11, 27, 28] propose that approximately 50–60% of the whole-plant hydraulic resistances are located in the root system. [10] found higher values of shoot hydraulic conductance compared with root hydraulic conductance values in sunflower. However on kiwifruit plants, root hydraulic conductance was higher than shoot hydraulic conductance [29]. It is evident that there is inconsistent information on the contribution of root or shoot hydraulic conductance to total hydraulic conductance and, more so, to plant transpiration.

Most studies on whole plant hydraulic architecture parameters with exchange parameters use the evaporative flux method [30]. This method assumes the hydraulic conductance of the soil/root/leaf pathway is K = E/Δp (Kg s-1 MPa-1), where Δp is the difference in the water potential between the two considered points (e.g. between soil water potential and leaf water potential), and E is the amount of water lost through transpiration at the time plant water potential is determined. The method has been validated [31], however, it makes possible to establish better relationships between gas exchange parameters and hydraulic conductance. In our study, we measured hydraulic conductance with a high-pressure flow meter (HPFM), therefore hydraulic trait measurements were totally independent of gas exchange measurements.

Furthermore, plant hydraulics is a complex system and its regulation is complex as well. It can depend on root anatomy, interactions between water and solute flow or on aquaporin activity in cell membranes [9, 32, 33, 34]. Many studies indicate stem or root hydraulic conductance in trees are related with xylem structure [35, 36]. And also, plants have the ability to adjust their water uptake capacity to changing environmental conditions by regulating aquaporins in the plasma membrane [37].

Taking all this into account, we hypothesized that whole plant hydraulic conductance modulates plant transpiration. Accordingly, we tested whether plants that differ in their contribution of root and shoot hydraulic conductance to whole plant hydraulic conductance also differ in their hydraulic conductance-transpiration relationship. We also studied the influence of xylem anatomy on root and shoot hydraulic conductance, as well as its possible effect on different plant-part contribution to plant hydraulic conductance.

To do so, we used seedlings of two different citrus rootstocks Poncirus trifoliata (L.) Raf. (PT) and Cleopatra mandarin (Citrus reshni Hort ex Tan.) (CM). Citrus rootstocks present different hydraulic characteristics that determine tree behavior, water relations or response to different stresses. Poncirus trifoliata and CM differ greatly at both physiological and morphological levels. Cleopatra mandarin is characterized by simple leaves versus PT that presents trifoliate leaves, smaller than those of CM. In previous studies, PT showed higher transpiration rates than CM, both in seedlings and in varieties grafted on them [23, 38, 39], and higher root hydraulic conductance values than CM [23, 38, 40]. To date studies of shoot hydraulic conductance of these genotypes are lacking.

Material and Methods

Plant Material and growth conditions

Ten-month-old seedlings of Poncirus trifoliata (PT) and Cleopatra mandarin (CM) were used in the experiment. PT and CM seeds were harvested from the mother seed trees held in the germplasm collection at IVIA, Valencia, Spain. Seeds were sown on 55x40 cm trays containing a mixture of peat and siliceous sand (3:2 v:v) in an aphid-proof greenhouse with a cooling system that kept temperatures between 15°C and 18°C and 80% relative humidity. Plants were grown with supplementary light (<50 μmol m-2 s-1, 400–700 nm) to extend the photoperiod to 16 h. Five-month-old plants were transplanted into 3 L pots with a mixture of peat and siliceous sand (3:2 v:v) and were watered twice a week with the following nutrient solution: 3 mMCa(NO3)2, 3 mM KNO3, 2 mM MgSO4, 2.3 mM H3PO4, 17.9 mM Fe-EDDHA and micronutrients as described by [41]. The pH of the nutrient solution was adjusted to 6.0 with 1 M KOH or 1 M H2SO4.

At the beginning of the experiment, sixteen plants of each genotype with no branches, (i.e., all leaves were on the main stem) and with different levels of development, were selected. Plants were selected for the experiment according to individual whole plant transpiration (measured gravimetrically before to the experiment, as described below) as we wanted to have a wide range of whole plant transpiration values and wanted this range to be similar for both genotypes. Selected CM plants had between 17 and 77 leaves and PT plants had 15 to 80 leaves. The experiments were conducted at the IVIA, Valencia, Spain (39.28 N—0.22 W) during late summer, with a photoperiod of 14 daylight hours. The plants were outdoors where average temperature was 24 ± 1°C and relative humidity, 80%.

During the experiment, plants were contained individually in the aforementioned 3 L pots and irrigated with 1.5 L water daily. The experiment lasted 5 days and plants were randomly distributed and surrounded by a guard row (buffer) not included in the experiment.

Whole plant transpiration (Tp)

From day 1 to 3, to measure whole plant transpiration, after each irrigation pots were covered with a sheet of plastic with a hole of similar diameter to that of the plant stem, through which the plant protruded. This system prevented evaporation from the substrate. The daily transpiration of each plant (Tp) was calculated as the difference between the weight of the watered pot (after draining) and the weight of the pot before watering the following day. The mean of three determinations (one per day) on each plant was considered as representative of each individual plant

Gas exchange and vapor pressure deficit

Transpiration rate (E, mmolH2O m-2 s-1) and stomatal conductance (gs, mmolH2Om-2 s-1) were measured on days 2 and 3, recording conditions of ambient light, temperature, relative humidity and CO2 concentration with an LCi Portable Photosynthesis System (ADC, herts, UK). The measurements were taken from 8:00 am to 6:00 pm. Air and leaf temperatures and photosynthetic photon flux density, provided by the LCi ranged from 19.1 to 32.2°C and 101.5 to 1971.7 μmol m-2 s-1, respectively. Both parameters reached their maximum values between 10:00 am and 3:00 pm. The E and gs were measured in fully expanded leaves taken from the central part of each plant. At each sampling time, six different leaves were measured in six different plants for each type plant.

Leaf-to-air vapor pressure deficit (VPD) is given by VPD = es−eair, where eair is the partial pressure of water vapor in the air, while es is the saturation water vapour pressure as it is assumed that water vapor pressure in the substomatal cavity is close to 100% [42]. eair was provided by the LCi. es was calculated by: es = 6,1373 10−3 e^(Tleaf*(18,568-Tleaf/254)/(Tleaf+255,57)), obtained by the numerical integration of Clausius-Clapeyron equation, where es is the saturation water vapour pressure (bar) and Tleaf is the leaf temperature (°C) measured with the LCi.

Root, Shoot and Plant hydraulic conductance

On day 4 and 5 of the experiment, root hydraulic conductance (Kr, KgH2O MPa-1 s-1) and shoot hydraulic conductance (Ks, KgH2O MPa-1 s-1) were measured in each experimental plant with a High Pressure Flow Meter (HPFM) (Dynamax Inc., Houston, TX). We followed the method described by [43]. To minimize the potential impact of diurnal periodicity on hydraulic conductance [10], all measurements were taken between 10:00 am and 2:00 pm at ambient temperature (24 ± 1°C).

The plants were cut 5 cm above the soil surface and the stumps of shoots and roots were connected to the HPFM with a water-tight seal, and the conductance of these plant parts (Ks and Kr) was determined using the transient measurement mode. Plant hydraulic conductance (Kp) was calculated by the equation 1/Kp = 1/Kr+1/Ks.

At the end of the measurements, the leaf areas of each plant were measured with a Li-Cor Li-3100 Area Meter (Li-Cor, inc. Lincoln, Nebraska, USA). All plant fractions were dried in a forced-draft oven at 60°C for 48 hours and weighed, and we calculated the Leaf dry weight/Root dry weight ratio (L/R).

We calculated leaf-specific root hydraulic conductance (Kr-l) and leaf-specific shoot hydraulic conductance (Ks-l) by dividing Kr or Ks, respectively, by the total plant leaf area. Root biomass-specific root hydraulic conductance (Kr-r) was calculated by dividing Kr by the root dry weight.

Light microscopy

Samples of about 3 mm of pioneer roots and 3 × 2 mm of wood from basal stem and taproot wood from seedlings were fixed, dehydrated and embedded in LR White (London Resin Co., Woking, Surrey, UK) according to [44]. Semi-thin transverse sections (1 μm thickness) were cut with a Leica RM2165 Rotary Microtome (Leica Instruments, Heidelberg, Germany) and stained with toluidine blue 0 (CI 52040, Merck, Darmstad, Germany) according to O’Brien et al. (1964). Representative sections of two tissue samples per plant and organ part (basal stem and taproot wood) from six independent plants of each seedling were examined and photographed with an Olympus BX-51 microscope (Olympus Imaging Corp., Tokyo, Japan), with a digital camera DP-12 and the Analysis program (Soft Imaging System GmbH, Munster, Germany). The anatomical data correspond to the mean of six independent plants on each rootstock. Three samples per tissue and plant were studied, and the average values were considered as representative of each individual plant. For each sample, values are the mean of three visual fields from three sections.

Statistical Analyses

Although plant development differed, data for biomass, whole plant transpiration and hydraulic conductance showed a normal distribution. Therefore, in a first analysis, parameters were tested by analysis of variance (ANOVA) and comparisons of means were determined by the least significant differences (LSD) method, at 95% confidence level with Statgraphics Plus ver. 5.1 (Statistical Graphics, Englewood Cliffs, NJ, USA). Additionally, slopes and intercepts of the performed linear regressions were compared between genotypes using the same software application.

Results

Plant biomass and whole plant transpiration

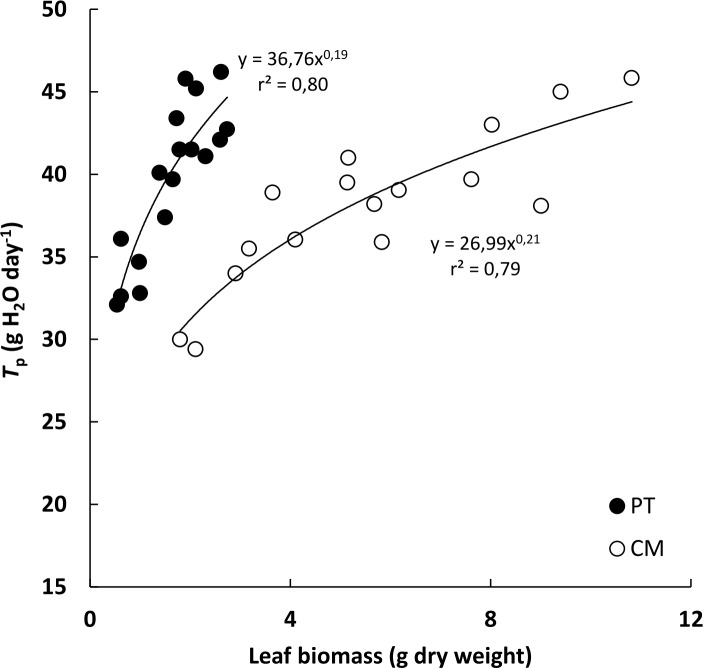
We found morphological differences between the two types of plant (Table 1). This was due, in part, to the fact that Cleopatra mandarin (CM) is characterized by simple leaves while P. trifoliata (PT) presents smaller trifoliate leaves. Both genotypes were similar in terms of root system development. However, significant differences in foliar biomass values were found, being 3.65-fold higher for CM than for PT plants, which increased the Leaf dry weight/Root dry weight ratio (L/R) for CM compared with PT (3.72-fold higher in CM). Although CM had a higher foliar biomass than PT, whole plant transpiration (Tp) values were similar in both plant groups. There was a positive and curvilinear correlation between leaf biomass and Tp for both genotypes (Fig 1).

Table 1. Dry weight of leaf (DW leaves) and roots (DW roots), leaf dry weight/root dry weight ratio (L/R), and whole plant transpiration (Tp) in P. trifoliata (PT) and Cleopatra mandarin (CM).

DW Leaves DW Roots L/R Tp
(g) (g) g d-1
PT 1.55 ± 0.19 b 3.70 ± 0.31 a 0.44 ± 0.05 b 39.71 ± 1.20 a
CM 5.66 ± 0.68 a 3.68 ± 0.46 a 1.65 ± 0.15 a 38.07 ± 1.16 a
Open in a new tab

For each parameter, means (n = 16) with different letters show statistically significant differences (P<0.05).

Fig 1. Relationship between foliar biomass and whole plant transpiration (Tp) in P. trifoliata (PT) and Cleopatra mandarin (CM).

Fig 1

Open in a new tab

Each point represents the mean of three measurements of Tp.

Gas exchange and vapor pressure deficit

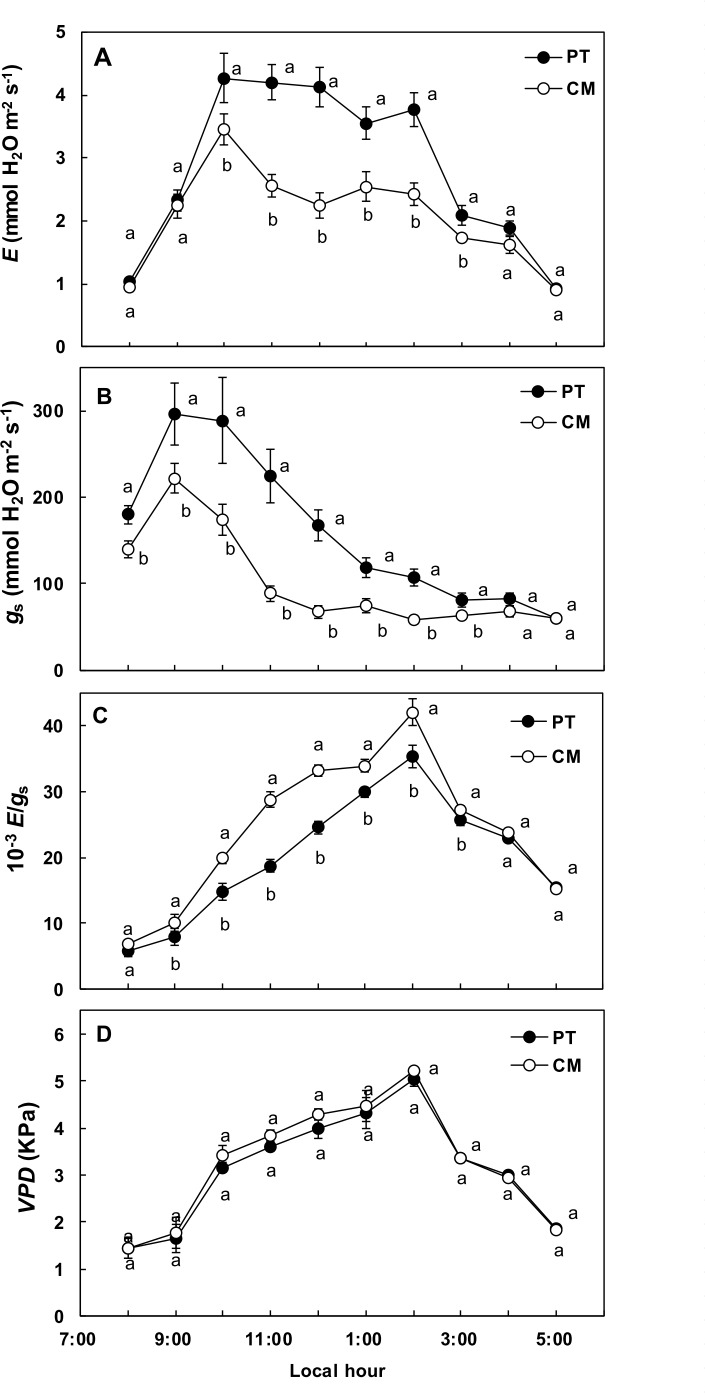
Fig 2A shows the diurnal variations of transpiration rate (E) in leaves from plants of the two genotypes. Transpiration rate displayed typical behavior, increasing in the early morning (8:00 to 10:00 am) and then decreasing after 2:00 pm. Leaves from PT showed significantly higher E values throughout the day than leaves from CM (average E value between 10:00 am and 2:00 pm was 3.98 mmol H2O m-2 s-1 for PT and 2.68 mmol H2O m-2 s-1 for CM). Stomatal conductance (gs) (Fig 2B) differed with respect to E trend. Maximum gs values were reached at 9:00 am in both genotypes and stomatal closure occurred from 10:00 am. CM presented significantly lower gs values than PT and there was not a constant relationship between E and gs during the day (ratio E/gs) (Fig 2C), which also differed between genotypes. CM had higher E/gs values than PT between 9.00 am and 3.00 pm, despite the vapor pressure deficit (VPD), driving force for water loss by via transpiration, showed a similar pattern throughout the day for both genotypes with no statistical differences between them (Fig 2D). This suggests transpiration was not solely regulated by stomatal conductance.

Fig 2. Diurnal time courses of stomatal conductance (gs), transpiration (E), E/gs ratio and vapor pressure deficit (VPD) in P. trifoliata (PT) and Cleopatra mandarin (CM).

Fig 2

Open in a new tab

Values are means of six replicates ± SE (n = 6). For each time, different letters indicate statistically significant differences (P <0.05) (LSD test).

Root, shoot and plant hydraulic conductance

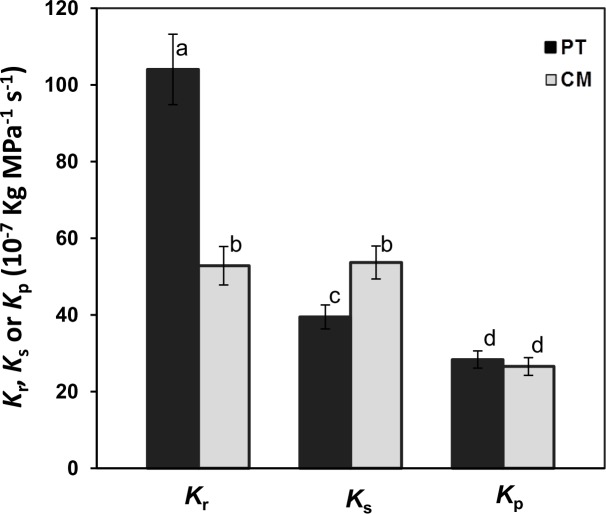
The average value of root hydraulic conductance (Kr) was significantly higher in PT than in CM (104.03 10−7 and 52.87 10−7 Kg MPa-1 s-1, respectively) (Fig 3). This, together with the results presented in Table 1, which show a similar root-system biomass for both genotypes, demonstrates the higher water transport capacity of PT than CM at similar root biomass levels. However, shoot hydraulic conductance (Ks) was significantly higher in CM than in PT (53.69 10−7 and 39,50 10−7 Kg MPa-1 s-1, respectively). Moreover, in PT seedlings, Kr was significantly higher than Ks, while CM presented no significant differences between Kr and Ks. Despite differences between Kr and Ks values between CM and PT, whole plant hydraulic conductance (Kp) was similar in both plant types.

Fig 3. Root, shoot and plant hydraulic conductance (Kr, Ks and Kp, respectively), of P. trifoliata (PT) and Cleopatra mandarin (CM).

Fig 3

Open in a new tab

The mean (n = 16) with different letters show statistically significant differences (P <0.05).

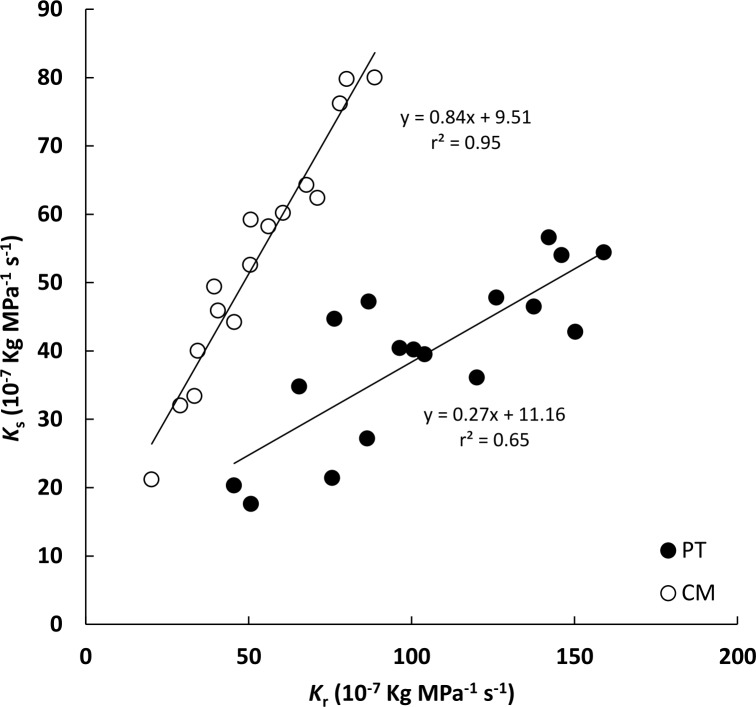
When the Ks value of each individual plant was plotted versus its respective Kr value, these two parameters were linearly related in both genotypes (r2 = 0.95 for CM, and r2 = 0.65 for PT) (Fig 4). However this relationship differed in each plant type. For a given Kr value, Ks was higher in CM than in PT plants.

Fig 4. Relationship between root hydraulic conductance (Kr) and shoot hydraulic conductance (Ks) in P. trifoliata (PT) and Cleopatra mandarin (CM).

Fig 4

Open in a new tab

Relationship between hydraulic conductance and plant biomass

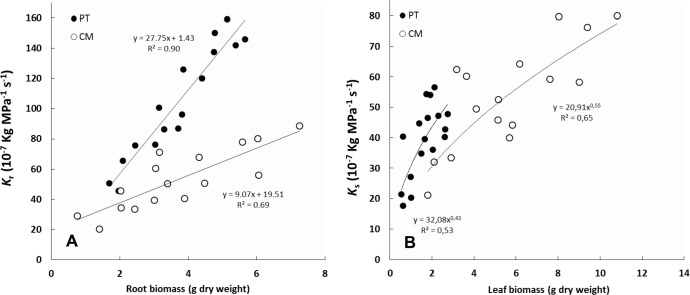
In PT, root hydraulic conductance values measured (Kr) ranged from 45.60 10−7 to 159.00 10−7 Kg MPa-1 s-1 for roots which their dry weight ranged between 1.69 and 5.67 g dry weight (Fig 5A). In CM, Kr values ranged from 20.20 10−7 to 88.70 10−7 kg MPa-1 s-1 for roots between 0.76 and 7.25 g dry weight. Kr increased linearly both for CM and for PT with increased root biomass, with r2 values of 0.90 and 0.69 for PT and CM, respectively. For a given root biomass, Kr values were higher in PT than in CM.

Fig 5. Relationship between root hydraulic conductance (Kr) and root biomass and between shoot hydraulic conductance (Ks) and the leave biomass expressed in g of dry weight in P. trifoliata (PT) and Cleopatra mandarin (CM).

Fig 5

Open in a new tab

Leaves of PT plants of the experiment showed values between 0.54 and 2.74 g dry weight (Fig 5B). Ks values of PT were between 17.60 10−7 and 56.60 10−7 Kg MPa-1 s-1. In CM, whose foliar biomass ranged from 1.80 to 10.82 g dry weight, Ks values were in a range 21.21 10−7 to 80.03 10−7 kg MPa-1 s-1. There was a curvilinear increase in Ks with increased foliar biomass presenting r2 values of 0.53 and 0.65 for PT and CM, respectively. For similar foliar biomass, PT presented higher Ks values.

Relationship between whole plant transpiration and root, shoot and plant hydraulic conductance

Whole plant transpiration (Tp), measured gravimetrically, increased linearly with increasing Kr and Ks values in both genotypes (Fig 6). In CM, Tp was affected in a similar way by Kr and Ks (Fig 6B) (there were no differences in the slope and the ordinate of the regression line between both regression lines). However, in PT plants, there was a greater Tp increase when Ks rose than when Kr rose (Fig 6A).

Fig 6. Relationship between root hydraulic conductance (Kr) and shoot hydraulic conductance (Ks) with whole plant transpiration (Tp) in P. trifoliata (PT) and Cleopatra mandarin (CM).

Fig 6

Open in a new tab

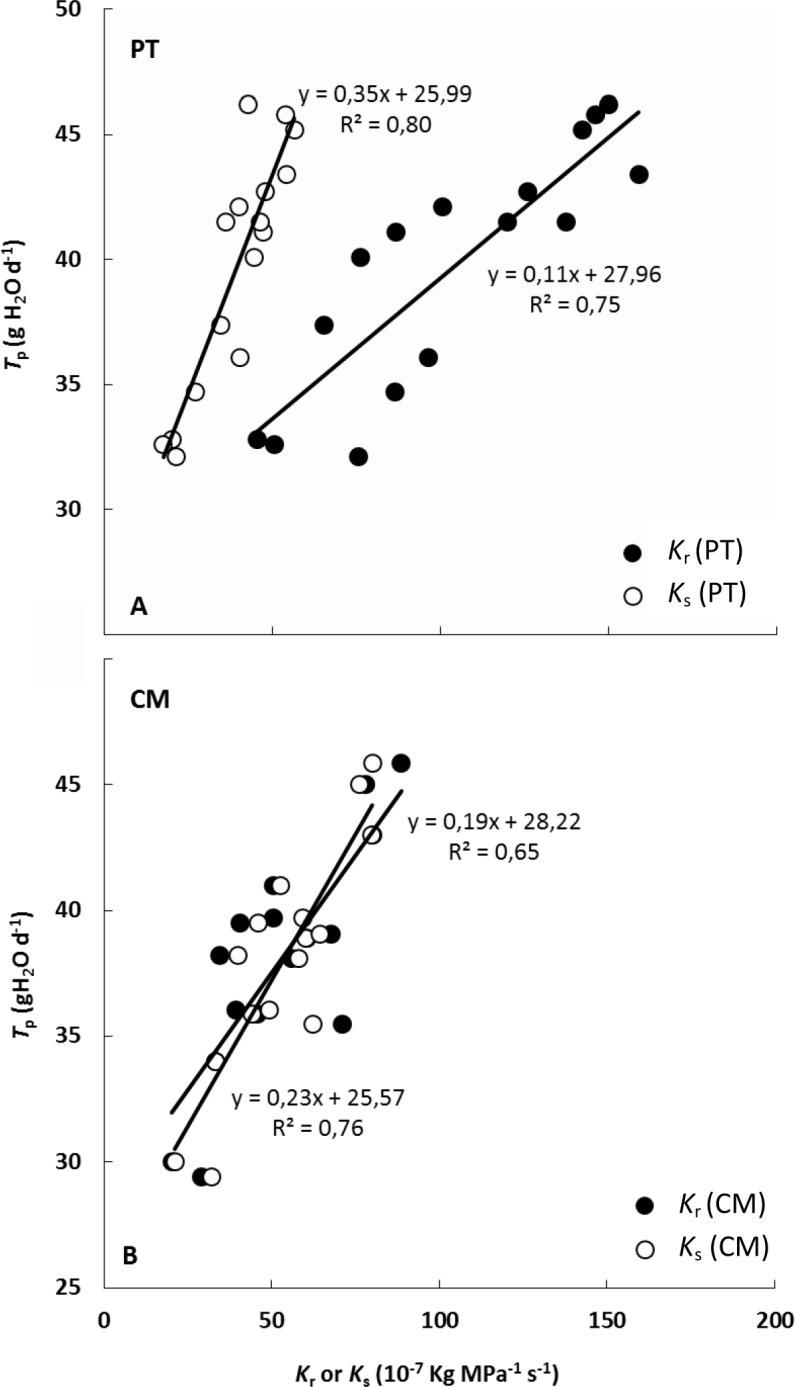
Each point represents the mean of three measurements of Tp.

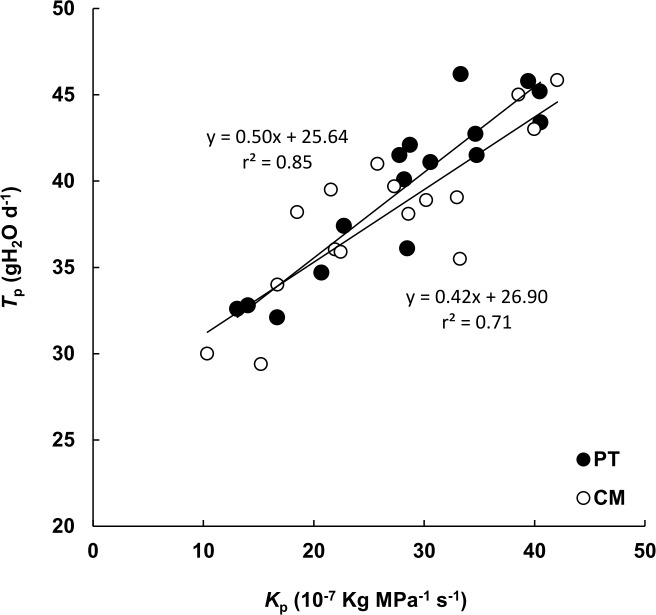
Moreover, the hydraulic conductance of the whole plant (Kp) was linearly related to Tp (Fig 7). This relationship was identical in both genotypes (slopes and intercepts were not significantly different) despite morphological, physiological and hydraulic traits these genotypes presented.

Fig 7. Relationship between plant hydraulic conductance (Kp) and whole plant transpiration (Tp) in P. trifoliata (PT) and Cleopatra mandarin (CM) in sixteen independent plants for each seedling.

Fig 7

Open in a new tab

Each point represents the mean of three measurements of Tp for each plant.

Xylem anatomy

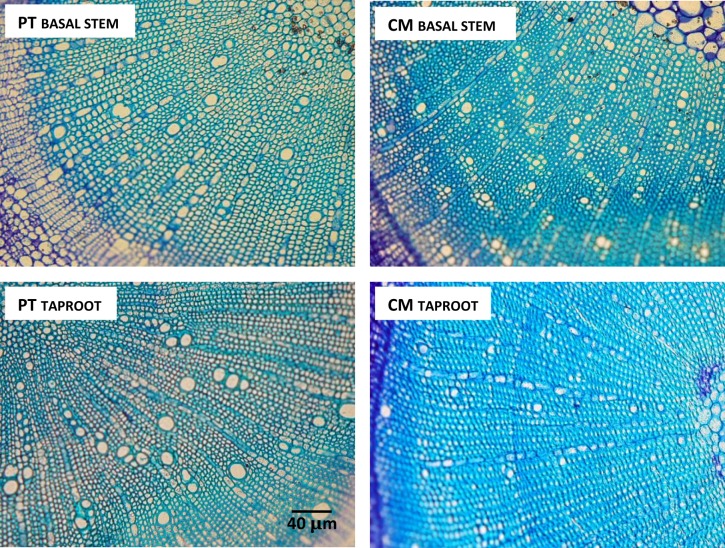
Xylem anatomical differences existed between the two genotypes (Figs 8 and 9). There were no observed changes in xylem vessel diameter in PT between taproot and basal stem while CM presented larger vessel diameter in basal stem than in taproot (Fig 8A). Despite this increasing diameter in the CM basal stem, xylem vessel diameter was larger in PT than in CM, both in taproot and basal stem.

Fig 8.

Fig 8

Open in a new tab

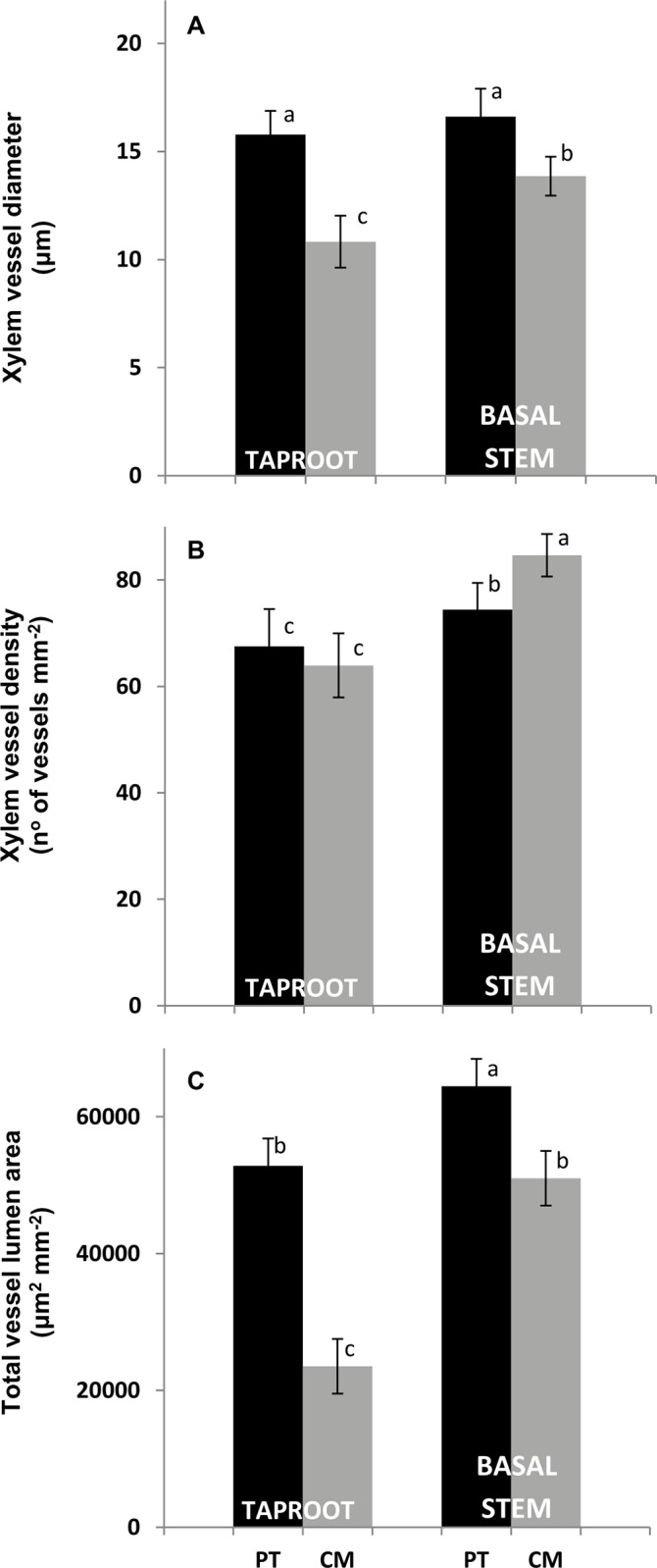
(A) Diameter, (B) density and (C) total lumen area of xylem vessels of taproot and basal stem in cross sections of Poncirus trifoliata (PT) and Cleopatra mandarin (CM) seedlings. Histological data correspond to the mean of six independent plants (n = 6) of each rootstock. The value for each plant is the mean of three visual fields of three sections from three samples per root and stem. Different letters indicate statistically significant differences (P <0.05) (LSD test).

Fig 9. Light micrograph of secondary xylem from basal stem and taproot of P. trifoliata (PT) and Cleopatra mandarin (CM).

Fig 9

Open in a new tab

Sections were cut at 20–25 mm under and above to soil surface.

On the other hand, xylem vessel density was higher in the basal stem than in the taproot in both genotypes although this difference was greater in CM (Fig 8B). In CM, the increased vessel diameter and vessel density in basal stem compared to their values in taproot resulted in a total vessel lumen area 2.17-fold higher in basal stem than in taproot. PT showed a 1.22-fold higher total vessel lumen area in basal stem compared to that of taproot (Fig 8C).

Discussion

The main objective of this work was to determine the influence of hydraulic conductance on transpiration in citrus. Apparently, transpiration rate (E) was not regulated solely by the degree of stomatal opening (Fig 2). However, results showed a linear relationship, identical for both studied genotypes (P. trifoliata [PT] and Cleopatra mandarin [CM]), between whole plant transpiration (Tp) and plant hydraulic conductance (Kp) (Fig 7).

Leaf biomass and Leaf dry weight/Root dry weight ratio (L/R) were higher in CM than PT. Both the transpiration rate (E) and the average root hydraulic conductance (Kr) were higher in PT than in CM (Figs 2 and 3). However, CM presented higher average shoot hydraulic conductance (Ks) value (Fig 3).

In both genotypes Ks and Kr were related positively with foliar and root biomass, respectively, as reported by other authors (e.g., [45]). When both parameters are scaled by dividing between leaf surface to obtain leaf-specific root hydraulic conductance (Kr-l) and leaf-specific shoot hydraulic conductance (Ks-l) (Table 2), Kr-l and Ks-l values were higher in PT. Both could determine the higher transpiration rate, stomatal conductance and net CO2 assimilation that PT presents, compared with CM [39, 40, 46].

Table 2. Root-specific root hydraulic conductance (Kr-r), leaf-specific root hydraulic conductance (Kr-l) and leaf-specific shoot hydraulic conductance (Ks-l) in P. trifoliata (PT) and Cleopatra mandarin (CM).

Kr-r Kr-l Ks-l
(10−7 x Kg MPa-1 s-1 g-1) 10−5 x Kg MPa-1 s-1 cm-2) 10−5 x Kg MPa-1 s-1 cm-2)
PT 28.12 ± 2.23 a 177.58 ± 9.18 a 66.80 ± 7.10 a
CM 14.37 ± 2.06 b 23.93 ± 3.01 b 24.44 ± 2.31 b
Open in a new tab

For each parameter, means (n = 16) with different letters show statistically significant differences (P<0.05).

A linear relationship between Kr and Ks was found in both genotypes, although this relationship was not the same for both types of plants. For equal Kr values, CM presented higher Ks than PT (Fig 4). Similar relationships between different plant hydraulic parameters have been described by other authors. [47] showed a linear regression between leaf hydraulic conductance and whole plant hydraulic conductance while [21] reported relationships between whole-plant and shoot hydraulic conductance. In our study, interestingly, for an individual plant independent of its genotype, Ks was always similar or lower than Kr. Also [48] observed that whole leaf hydraulic conductance was lower than Kr in nineteen grass species. This could be an evolutionary trait to avoid cavitation. If Ks was greater than Kr, and water flow in shoots was close to its possible peak flow, roots could not provide all the water claimed by the air component. This could result in increased water tension that would facilitate air bubble formation in the xylem. The lack of literature integrating the study of whole root and shoot systems in tree species does not enable us to pose this hypothesis. However, our results support the assumption that the design of plant hydraulic conductance and resistance to cavitation within a plant is optimized to deal with the conflicting balance between evaporative demand and protection from hydraulic failure [49].

The higher Ks/Kr ratio in CM could be attributed to the anatomical features of the xylem of the main root and stem base. In CM seedlings, values of lumen diameter and density of vessels in the base of the stem were 27.9% and 32.4% higher compared with the values of these parameters in the taproot, resulting in a two-fold increase in total lumen vessel area compared with this area in the taproot, while in PT this increase was much lower (Fig 8). Therefore, the same as with xylem traits and L/R ratio, the Ks/Kr ratio could be genetically determined. On the other hand, the higher diameter in xylem vessels in PT could be attributed to higher aquaporin activity. According to [50], aquaporins play a role in mediating water transport to support xylogenesis because plants with higher aquaporin expression achieve a greater final cell diameter in xylem vessels. In other studies, we have observed more aquaporin expression in roots of PT than in CM [24, 51], which could be partially responsible for the high root hydraulic conductance of PT plants, and also for their greater vessel diameter.

Clearly root and shoot growth must be coordinated somehow during the life of a plant [52]. The relationship between both Kr and Ks with plant biomass (Fig 5) and the relationship between both conductances (Fig 4) suggests that hydraulic traits should influence Shoot/Root ratio and plant growth, in accordance with other authors [36, 53, 54, 55]. It is known that growth of the aerial component in olive trees is related to Kr [53] and also that Kr is responsible for the vigor of grafted peach varieties [54]. However, although many studies associate high values of Kr-l or Kr-r (leaf or root-specific root hydraulic conductance) with strong shoot development, in our experiment CM showed a higher L/R ratio even though PT presented higher Kr-l and Kr-r (Table 2). Therefore, root hydraulic conductance may apparently determine the gas exchange features of leaves rather than shoot growth rate. [54] observed that non-vigorous peach varieties grafted on vigorous rootstocks presented lower Ks, and less vigorous development than vigorous varieties grafted on the same rootstocks, indicating that genetic factors probably determine the Ks and growth of these varieties. Therefore, these could explain the higher L/R ratio in CM. Accordingly, the effect of rootstocks hydraulic traits on shoot growth would be more evident when rootstocks were grafted with same variety. Taking all this into account, in our study, the highest L/R ratio in CM could be more closely related with the Ks/Kr ratio and xylem anatomy traits than with differences in water transport capacity of the root system.

On the other hand, E stayed practically constant between 10:00 am– 4:00 pm, even though stomatal closure was observed after 11:00 am. The reduction in gs was probably due to the high temperature increase in late morning, which resulted in a high VPD (Fig 2D). By reducing gs, plants minimize water loss and maintain plant cell hydration as VPD increases. Despite reduced stomatal conductance in response to increasing vapor pressure deficit, transpiration rate can increase [56, 57], as occurred in this experiment, since E/gs ratio increased (Fig 2C). This response varies among species and genotypes [58, 59, 60]. Our results showed that at early morning (from 8:00 am to 10:00 am E was limited by VPD and not by gs (Fig 2A, 2B and 2D), which presented its maximum values in this period and was not completely related to the increasing E. At mid-range values of VPD (approximately from 10:00 am to 12:00 am, stomata progressively closed as VPD increased. However, E remained constant as the increase in the driving force (VPD) compensated the reduction in gs. It suggested that hydraulic limitation more than stomatal limitation were related to the maximum E values reached at midday. A number of studies have indicated a functional relationship between gs and hydraulic conductance [18, 52]. Accordingly, as VPD increased, the rate of stomatal closure (which controls water losses) could be modulated by the plant hydraulic system (which controls water supply to the leaves). Therefore E would be the result of the balance between gs, VPD and hydraulic conductance.

The relationship between whole plant transpiration (Tp) and Kr, presented in Fig 6, has also been reported in citrus by [38]. The latter authors found a significant correlation (r2 = 0.63) between these two parameters, noting that rootstocks with low Kr values showed lower Tp values than rootstocks with higher Kr values. However, our study shows that apparently Ks also influences plant transpiration (Fig 6B). Previous studies on citrus rootstocks suggest that differences in the root size or in the crown volume of grafted varieties affect the plant-soil-water relationship [61, 62, 63, 64, 65]. Moreover, Tp in both genotypes were strongly associated with foliar biomass (Fig 1). This suggests that foliar biomass is probably an important factor determining transpiration, which in turn appears to be related to the fact that increases in foliar biomass are related to Ks increments (Fig 5B). Our results support the dependence of Tp on foliar biomass, in accordance with previous studies in citrus suggesting that the crown size influences the rate of water absorbed by trees grafted on different rootstocks [61] but also indicate that this is associated with the higher hydraulic conductance values reached with higher crown size.

The relationships between Kr and Ks with Tp differed between CM and PT (Fig 6), but the relationship between whole plant hydraulic conductance (Kp) and Tp was identical for both genotypes (Fig 7). Shoot and root contribution to whole plant hydraulic conductance differed greatly between the two genotypes, even so, the relationship between Kp and Tp remained constant, suggesting that plant transpiration is regulated directly by Kp, which in turn depends on Kr and Ks [10, 66]. Several studies have highlighted the coordination between plant water transport capacity and leaf-level gas exchange or photosynthetic capacity [67, 68, 69, 70, 71]. It is therefore assumed that a high plant hydraulic efficiency (i.e. high leaf-specific hydraulic conductance) which modulates transpiration is an essential prerequisite for a high productivity in tree species [3]. However, a decrease in future precipitation and an increase in the temperature have been predicted for many Citrus growing regions, affecting the plant water relations and potentially constraining its productivity [72]. Both CM and PT are widely used as citrus rootstocks. The ability of the rootstock to supply water and nutrients to the plant is a main factor influencing fruit development in citrus trees, determining the strength of the grafted variety and tolerance to water stress [73, 74]. When comparing grafted varieties onto CM and PT rootstocks, grafted varieties on CM presented less net CO2 assimilation, transpiration rate and reduced stomatal conductance than when are grafted in PT [23, 24]. However, grafted varieties on CM present higher tolerance to water stress probably attributed to the relatively low values of Kr-r and Kr-l that limits sap flow from roots to leaves promoting conservative water use and favoring better water balance in the plant [24, 75]. Therefore, in areas where water availability can be a limiting factor the use of CM could be a strategy for reducing water stress.

This work shows the need for integration of whole plant hydraulic system when attempting to understand the plant water relations and we conclude that transpiration in citrus is strongly determined by the hydraulic system of the whole plant and the differences in root and shoot contribution to Kp could be related to the leaf/root ratio, as well as to xylem anatomy characteristics.

Supporting Information

S1 Table. Number of leaves, dry weight of stem, leaves and root, leave surface and shoot, root and plant hydraulic conductance of the sixteen plants of Cleopatra Mandarin and Poncirus trifoliata used in the experiment.

(XLSX)

Click here for additional data file. (12.6KB, xlsx)
S2 Table. Stomatal conductance (gs), transpiration (E), E/gs ratio and vapor pressure deficit (VPD) in P. trifoliata (PT) and Cleopatra mandarin (CM).

At each hour of the day, values are means of six replicates for each type of plant.

(XLSX)

Click here for additional data file. (27.8KB, xlsx)
S3 Table. Histological data correspond to the mean of six independent plants of Poncirus trifoliata and six independent plants of Cleopatra mandarin.

(XLSX)

Click here for additional data file. (8.9KB, xlsx)

Acknowledgments

This work was funded by the Instituto Nacional de Investigación y Tecnología Agraria y Alimentaria (RTA2014-00059), Generalitat Valenciana and FEDER funds.

Data Availability

All relevant data are within the paper and its Supporting Information files.

Funding Statement

This work was funded by the Instituto Nacional de Investigación y Tecnología Agraria y Alimentaria (RTA2014-00059), Generalitat Valenciana and FEDER funds.

References

  • 1.Lo Gullo MA, Salleo S, Rosso R, Trifilò P. Drought resistance of 2-year-old saplings of Mediterranean forest trees in the field: relations between water relations, hydraulics and productivity. Plant Soil. 2003;250: 259–272. [Google Scholar]
  • 2.Mencuccini M. The ecological significance of long-distance water transport: short-term regulation, long-term acclimation and the hydraulic costs of stature across plant life forms. Plant Cell Environ. 2003;26: 163–182. [Google Scholar]
  • 3.Tyree M. Hydraulic limits on tree performance: transpiration, carbon gain and growth of trees. Trees. 2003;17: 95–100. [Google Scholar]
  • 4.Solari LI, Johnson S, DeJong TM. Hydraulic conductance characteristics of peach (Prunus persica) trees on different rootstocks are related to biomass production and distribution. Tree Physiol. 2006;26: 1343–1350. [DOI] [PubMed] [Google Scholar]
  • 5.Manzoni S, Vico G, Katul G, Palmroth S, Jackson RB, Porporato A. Hydraulic limits on maximum plant transpiration and the emergence of the safety-efficiency trade-off. New Phytol. 2013;198: 169–78. 10.1111/nph.12126 [DOI] [PubMed] [Google Scholar]
  • 6.Quentin AG, O'Grady AP, Beadle CL, Mohammed C, Pinkard EA. Interactive effects of water supply and defoliation on photosynthesis, plant water status and growth of Eucalyptus globulus Labill. Tree Physiol. 2012;32: 958–67. 10.1093/treephys/tps066 [DOI] [PubMed] [Google Scholar]
  • 7.Õunapuu E, Sellin A. Daily dynamics of leaf and soil-to-branch hydraulic conductance in silver birch (Betula pendula) measured in situ. Plant Physiol Bioch. 2013;68: 104–110. [DOI] [PubMed] [Google Scholar]
  • 8.Weatherley PE. Water uptake and flow into roots In: Lange OL, Nobel PS, Osmond CB, Zeigler H, eds. Encyclopedia of plant physiology, Vol. 12B Berlin: Springer Verlag; 1982. pp. 79–109. [Google Scholar]
  • 9.Steudle E, Peterson CA. How does water get through roots. J Exp Bot. 1998;49: 775–788. [Google Scholar]
  • 10.Tsuda M, Tyree MT. Plant hydraulic conductance measured by the high pressure flow meter in crop plants. J Exp Bot. 2000;51: 823–828. [PubMed] [Google Scholar]
  • 11.Domec JC, Noormets A, King JS, Sun G, McNulty SG, Gavazzi MJ, et al. (2009a) Decoupling the influence of leaf and root hydraulic conductances on stomatal conductance and its sensitivity to vapor pressure deficit as soil dries in a drained loblolly pine plantation. Plant Cell Environ. 2009;32: 980–991. 10.1111/j.1365-3040.2009.01981.x [DOI] [PubMed] [Google Scholar]
  • 12.Meinzer FC, McCulloh KA, Lachenbruch B, Woodruff DR, Johnson DM. The blind men and the elephant: the impact of context and scale in evaluating conflicts between plant hydraulic safety and efficiency. Oecologia. 2010;164: 287–296. 10.1007/s00442-010-1734-x [DOI] [PubMed] [Google Scholar]
  • 13.Sack L, Cowan PD, Jaikumar N, Holbrook NM. The ‘hydrology’ of leaves: co-ordination of structure and function in temperate woody species. Plant Cell Environ. 2003;26: 1343–1356. [Google Scholar]
  • 14.Sack L, Tyree MT, Holbrook NM. Leaf hydraulic architecture correlates with regeneration irradiance in tropical rainforest trees. New Phytol. 2005;167: 403–413. [DOI] [PubMed] [Google Scholar]
  • 15.Dewar RC. The Ball–Berry–Leuning & Tardieu–Davies stomatal models: synthesis and extension within a spatially aggregated picture of guard cell function. Plant Cell Environ. 2002;25: 1383–1398. [Google Scholar]
  • 16.Buckely TN. The control of stomata by water balance. New Phytol. 2005;168: 275–292. [DOI] [PubMed] [Google Scholar]
  • 17.Brodribb TJ, Jordan GJ. Internal coordination between hydraulics and stomatal control in leaves. Plant Cell Environ. 2008;31: 1557–1564. 10.1111/j.1365-3040.2008.01865.x [DOI] [PubMed] [Google Scholar]
  • 18.Brodribb TJ, Holbrook NM, Zwieniecki MA, Palma B. Leaf hydraulic capacity in ferns, conifers and angiosperms: impacts on photosynthetic maxima. New Phytol. 2005;165: 839–846. [DOI] [PubMed] [Google Scholar]
  • 19.Sadok W, Sinclair TR. Transpiration response of ‘slow-wilting’ and commercial soybean (Glycine max (L.) Merr.) genotypes to three aquaporin inhibitors. J Exp Bot. 2010;61: 821–829. 10.1093/jxb/erp350 [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 20.Yang Y, Tyree MT. Hydraulic resistance in the shoots of Acer saccharum and its influence on leaf water potential and transpiration. Tree Physiol. 1993;12: 231–242. [DOI] [PubMed] [Google Scholar]
  • 21.Nardini A, Salleo S. Limitation of stomatal conductance by hydraulic traits: sensing or preventing xylem cavitation? Trees. 2000;15: 14–24. [Google Scholar]
  • 22.Martorell S, Diaz-Espejo A, Medrano H, Ball MC, Choat B. Rapid hydraulic recovery in Eucalyptus pauciflora after drought: linkages between stem hydraulics and leaf gas exchange. Plant Cell Environ. 2014;37: 617–626. 10.1111/pce.12182 [DOI] [PubMed] [Google Scholar]
  • 23.Rodríguez-Gamir J, Intrigliolo DS, Primo-Millo E, Forner-Giner MA. Relationships between xylem anatomy, root hydraulic conductivity, leaf/root ratio and transpiration rates in citrus trees on different rootstocks. Physiol plant. 2010;139: 159–169. 10.1111/j.1399-3054.2010.01351.x [DOI] [PubMed] [Google Scholar]
  • 24.Rodríguez-Gamir J, Ancillo G, Aparicio F, Bordas M, Primo-Millo E, Forner-Giner MA Water-deficit tolerance in citrus is mediated by the down regulation of PIP gene expression in the roots. Plant Soil. 2011;347: 91–104. [Google Scholar]
  • 25.Perrone I, Gambino G, Chitarra W, Vitali M, Pagliarani C, Riccomagno N, et al. The grapevine root-specific aquaporin VvPIP2;4N controls root hydraulic conductance and leaf gas exchange under well-watered conditions but not under water stress. Plant Physiol. 2012;160: 965–77. 10.1104/pp.112.203455 [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 26.Pratt RB, North GB, Jacobsen AL, Ewers FW, Davis SD. Xylem root and shoot hydraulics is linked to life history type in chaparral seedlings. Funct Ecol. 2010; 24: 70–81. [Google Scholar]
  • 27.Domec JC, Scholz FG, Bucci SJ, Meinzer FC, Goldstein G, Villalobos-Vega R. Diurnal and seasonal variation in root xylem embolism in neotropical savanna woody species: impact on stomatal control of plant water status. Plant Cell Environ. 2006;29: 26–35. [DOI] [PubMed] [Google Scholar]
  • 28.Domec JC, Palmroth S, Ward E, Maier CA, Thérézien M, Oren R. Acclimation of leaf hydraulic conductance and stomatal conductance of Pinus taeda (loblolly pine) to long term growth in elevated CO2 (FACE) and N-fertilization. Plant Cell Environ. 2009;32: 1500–1512. 10.1111/j.1365-3040.2009.02014.x [DOI] [PubMed] [Google Scholar]
  • 29.Marykate ZB, Patterson KJ, Minchin PEH, Gould KS, Clearwater MJ. Hydraulic responses of whole vines and individual roots of kiwifruit (Actinidia chinensis) following root severance. Tree Physiol. 2013;31: 508–518. [DOI] [PubMed] [Google Scholar]
  • 30.Cochard H, Bréda N, Granier A. Whole tree hydraulic conductance and water loss regulation in Quercus during drought: evidence for stomatal control of embolism? Ann Sci For. 1996;53: 197–206. [Google Scholar]
  • 31.Tsuda M, Tyree MT. Whole-plant hydraulic resistance and vulnerability segmentation in Acer saccharinum. Tree Physiol. 1997;17: 351–357. [DOI] [PubMed] [Google Scholar]
  • 32.Steudle E. Water uptake by roots: effects of water deficits. J Exp Bot. 2000;51: 1531–1542. [DOI] [PubMed] [Google Scholar]
  • 33.Steudle E. The cohesion-tension mechanism and the acquisition of water by plant roots. Annu Rev Plant Physiol Plant Mol Biol. 2001;52: 847–875. [DOI] [PubMed] [Google Scholar]
  • 34.Henzler T, Waterhouse RN, Smyth AJ, Carvajal M, Cooke DT, Schaffner AR, et al. Diurnal variations in hydraulic conductivity and root pressure can be correlated with the expression of putative aquaporins in the roots of Lotus japonicus. Planta. 1999;210: 50–60. [DOI] [PubMed] [Google Scholar]
  • 35.Olmstead MA, Lang NS, Ewers FW, Owens SA. Xylem vessel anatomy of sweet cherries grafted onto dwarfing and non dwarfing rootstocks. J Am Soc Hortic Sci. 2006;131: 577–585. [Google Scholar]
  • 36.Gonçalves B, Correia CM, Silva AP, Bacelar EA, Santos A, Ferreira H, et al. Variation in xylem structure and function in roots and stems of scion-rootstock combinations of sweet cherry tree (Prunus avium L.). Trees. 2007;21: 121–130. [Google Scholar]
  • 37.Laur J, Hacke UG. Transpirational demand affects aquaporin expression in poplar roots. J Exp Bot. 2013;64: 2283–2293. 10.1093/jxb/ert096 [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 38.Syvertsen JP, Graham JH. Hydraulic conductivity of roots, mineral nutrition, and leaf gas exchange of Citrus rootstocks. J Am Soc Hortic Sci. 1985;110: 865–869. [Google Scholar]
  • 39.Forner-Giner MA, Primo-Millo E, Forner JB. Performance of Forner-Alcaide 5 and Forner-Alcaide 13, hybrids of Cleopatra mandarin × Poncirus trifoliata, as salinity-tolerant citrus rootstocks. J Am Pomol Soc. 2009;63: 72–80. [Google Scholar]
  • 40.Zekri M, Parsons LR. Growth and root hydraulic conductivity of several citrus rootstocks under salt and polyethylene glycol stresses. Physiol plant. 1989;77: 99–106. [Google Scholar]
  • 41.Hoagland DR, Arnon DI. The water culture method for growing plants without soil. Calif Agric Exp Sta Circular. 1950;347: 1–32. [Google Scholar]
  • 42.Pearcy RW, Schulze E-D, Zimmerman R. Measurement of transpiration and leaf conductance In Plant Physiological Ecology. Eds. Pearcy R.W., Ehleringer J., Mooney H.A. and Rundel P.W.. Chapman and Hall, London; 1989. pp. 137–160. [Google Scholar]
  • 43.Tyree MT, Patiño S, Bennink J, Alexander J. Dynamic measurements of root hydraulic conductance using a high-pressure flowmeter in the laboratory and field. J Exp Bot. 1995;46: 83–94. [Google Scholar]
  • 44.Tadeo FR, Ben-Cheikh W, Gomez-Cadenas A, Talon M, Primo-Millo E. Gibberellin-ethylene interaction controls radial expansion in citrus roots. Planta. 1997;202: 370–378. [Google Scholar]
  • 45.Tyree MT, Vélez V, Dalling JW. Growth dynamics of root and shoot hydraulic conductance in seedlings of five neotropical tree species: scaling to show possible adaptation to differing light regimes. Oecologia. 1998;114: 293–298. [DOI] [PubMed] [Google Scholar]
  • 46.Syvertsen JP, Yelenosky G. Salinity can enhance freeze tolerance of citrus rootstock seedlings by modifying growth, water relations, and mineral nutrition. J Am Soc Hortic Sci. 1988;113: 889–893. [Google Scholar]
  • 47.Fichot R, Chamaillard S, Depardieu C, Le Thiec D, Cochard H, Barigah T, et al. Hydraulic efficiency and coordination with xylem resistance to cavitation, leaf function and growth performance among eight unrelated Populus deltoids x Populus nigra hybrids. J Exp Bot. 2011;62: 2093–2106. 10.1093/jxb/erq415 [DOI] [PubMed] [Google Scholar]
  • 48.Ocheltree TW, Nippert JB, Prasad PVV. Stomatal responses to changes in vapour pressure deficit reflect tissue-specific differences in hydraulic conductance. Plant Cell Environ. 2014;37: 132–139. 10.1111/pce.12137 [DOI] [PubMed] [Google Scholar]
  • 49.Tyree MT, Davis SD, Cochard H. Biophysical perspectives of xylem evolution: is there a trade-off of hydraulic efficiency for vulnerability to dysfunction? IAWA J. 1994;15: 335–360. [Google Scholar]
  • 50.Hacked UG, Plavcová L, Almeida-Rodriguez A, King-Jones S, Zhou W, Cooke JEK. Influence of nitrogen fertilization on xylem traits and aquaporin expression in stems of hybrid poplar. Tree Physiol. 2010;30: 1016–1025. 10.1093/treephys/tpq058 [DOI] [PubMed] [Google Scholar]
  • 51.Rodríguez-Gamir J, Ancillo G, Legaz F, Primo-Millo E, Forner-Giner MA Influence of salinity on PIP gene expression in citrus roots and its relationship with root hydraulic conductance, transpiration and chloride exclusion from leaves. Environ Exp Bot. 2012;78: 163–166. [Google Scholar]
  • 52.Meinzer F, Grantz D. Stomatal and hydraulic conductance in growing sugarcane: stomatal adjustment to water transport capacity. Plant Cell Environ. 1990;13: 383–388. [Google Scholar]
  • 53.Nardini A, Gascó A, Raimondo F, Gortan E, Lo Gullo MA, Caruso T, et al. Is rootstock-induced dwarfing in olive an effect of reduced plant hydraulic efficiency?. Tree Physiol. 2006;26: 1137–1144. [DOI] [PubMed] [Google Scholar]
  • 54.Tombesi S, Johnson RS, Day KR, DeJong TM. Relationships between xylem vessel characteristics, calculated axial hydraulic conductance and size controlling capacity of peach rootstocks. Ann Bot. 2010;105: 327–331 10.1093/aob/mcp281 [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 55.Martínez-Alcántara B, Rodríguez-Gamir J, Martínez-Cuenca MR, Iglesias DJ, Primo-Millo E, Forner-Giner MA. Relationship between hydraulic conductance and citrus dwarfing by the Flying Dragon rootstock (Poncirus trifoliata L. Raft var. Monstrosa). Trees. 2012;27: 629–638. [Google Scholar]
  • 56.Moteith JL. A reinterpretation of stomatal responses to humidity. Plant Cell Environ. 1995;18: 357–364. [Google Scholar]
  • 57.Bunce JA. How do leaf hydraulics limit stomatal conductance at high water vapour pressure deficits?. Plant Cell Environ. 2006;29: 1644–1650. [DOI] [PubMed] [Google Scholar]
  • 58.Maherali L, Johnson HB, Jackson RB. Stomatal sensitivity to vapour pressure difference over a subambient to elevated CO2 gradient in a C-3/C-4 grassland. Plant Cell Environ. 2003;26: 1297–1306. [Google Scholar]
  • 59.Fletcher AL, Sinclair T, Allen LH. Transpiration responses to vapour pressure deficit in well watered ‘slow-wilting’ and commercial soybean. Environ Exp Bot. 2007;61: 145–151. [Google Scholar]
  • 60.Sinclair T, Zwieniecki M, Holbrook N. Low leaf hydraulic conductance associated with drought tolerance in soybean. Physiol Plant. 2008;132: 446–451 10.1111/j.1399-3054.2007.01028.x [DOI] [PubMed] [Google Scholar]
  • 61.Castle WS, Krezdorn AH. Soil water use and apparent root efficiencies of citrus trees on four rootstocks. J Am Soc Hortic Sci. 1977;102: 403–406. [Google Scholar]
  • 62.Cohen S, Naor A. The effect of three rootstocks on water use, canopy conductance and hydraulic parameters of apple trees and predicting canopy from hydraulic conductance. Plant Cell Environ. 2002;25: 17–28. [Google Scholar]
  • 63.Romero P, Navarro JM, Pérez-Pérez J, García-Sánchez F, Gómez-Gómez A, Porras I, et al. Deficit irrigation and rootstock: their effects on water relations, vegetative development, yield, fruit quality and mineral nutrition of Clemenules mandarin. Tree Physiol. 2006;26: 1537–1548. [DOI] [PubMed] [Google Scholar]
  • 64.Cohen S, Naor A, Bennink J, Avraham G, Tyree M. Hydraulic resistance components of mature apple trees on rootstocks of different vigours. J Exp Bot. 2007;58: 4213–4224. 10.1093/jxb/erm281 [DOI] [PubMed] [Google Scholar]
  • 65.Clearwater MJ, Blattmann ZL, Lowe RG. Control of scion vigour by kiwifruit rootstocks is correlated with spring root pressure phenology. J Exp Bot. 2007;58: 1741–1751. [DOI] [PubMed] [Google Scholar]
  • 66.Brisson N, Olioso A, Clastre P. Daily transpiration of field soybeans as related to hydraulic conductance, root distribution, soil potential and midday leaf potential. Plant Soil. 1993;154: 227–237. [Google Scholar]
  • 67.Brodribb T, Feild T. Stem hydraulic supply is linked to leaf photosynthetic capacity: evidence from New Caledonian and Tasmanian rainforests. Plant Cell Environ. 2000;23: 1381–1388. [Google Scholar]
  • 68.Brodribb T, Holbrook NM, Gutierrez M. Hydraulic and photosynthetic co‐ordination in seasonally dry tropical forest trees. Plant Cell Environ. 2002;25: 1435–1444. [Google Scholar]
  • 69.Hubbard R, Ryan M, Stiller V, Sperry J. Stomatal conductance and photosynthesis vary linearly with plant hydraulic conductance in ponderosa pine. Plant Cell Environ. 2001;24: 113–121. [Google Scholar]
  • 70.Santiago L, Goldstein G, Meinzer F, Fisher J, Machado K, Woodruff D, et al. Leaf photosynthetic traits scale with hydraulic conductivity and wood density in Panamanian forest canopy trees. Oecologia. 2004;140: 543–550. [DOI] [PubMed] [Google Scholar]
  • 71.Sperry J, Alder N, Eastlack S. The effect of reduced hydraulic conductance on stomatal conductance and xylem cavitation. J Exp Bot. 1993;44: 1075–1082. [Google Scholar]
  • 72.IPCC. Climate change 2013: the physical basis In: Summary for Policymakers. Contribution of Working Group I to the fifth assessment report of the Intergovernmental Panel on Climate Change. Cambridge, UK & New York, NY, USA: Cambridge University Press; 2013. [Google Scholar]
  • 73.Syvertsen JP, Lloyd JJ. Citrus In: Schaffer BA, Andersen PC (eds) Handbook of environmental physiology of fruits crops. Vol II. Subtropical and tropical crops. CRC Press, Boca Raton, FL; 1994. pp. 65–99. [Google Scholar]
  • 74.Medina CL, Machado FC. Gas exchange and water relations of Valencia orange tree grafted on Rangpur lime and Poncirus trifoliata submitted to a water deficit. Bragantia, Campinas. 1998;57: 15–22. [Google Scholar]
  • 75.Yamada S, Katsuhara M, Kelly WB, Michalowski CB, Bohnert HJ. A family of transcripts encoding water channel proteins: tissue-specific expression in common ice plant. Plant Cell. 1995;7: 1129–1142. [DOI] [PMC free article] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

S1 Table. Number of leaves, dry weight of stem, leaves and root, leave surface and shoot, root and plant hydraulic conductance of the sixteen plants of Cleopatra Mandarin and Poncirus trifoliata used in the experiment.

(XLSX)

Click here for additional data file. (12.6KB, xlsx)
S2 Table. Stomatal conductance (gs), transpiration (E), E/gs ratio and vapor pressure deficit (VPD) in P. trifoliata (PT) and Cleopatra mandarin (CM).

At each hour of the day, values are means of six replicates for each type of plant.

(XLSX)

Click here for additional data file. (27.8KB, xlsx)
S3 Table. Histological data correspond to the mean of six independent plants of Poncirus trifoliata and six independent plants of Cleopatra mandarin.

(XLSX)

Click here for additional data file. (8.9KB, xlsx)

Data Availability Statement

All relevant data are within the paper and its Supporting Information files.

Articles from PLoS ONE are provided here courtesy of PLOS

RESOURCES