Lunisolar tidal force and its relationship to chlorophyll fluorescence in Arabidopsis thaliana

Abstract

The yield of chlorophyll fluorescence Ft was measured in leaves of Arabidopsis thaliana over periods of several days under conditions of continuous illumination (LL) without the application of saturating light pulses. After linearization of the time series of the chlorophyll fluorescence yield (ΔFt), oscillations became apparent with periodicities in the circatidal range. Alignments of these linearized time series ΔFt with the lunisolar tidal acceleration revealed high degrees of synchrony and phase congruence. Similar congruence with the lunisolar tide was obtained with the linearized quantum yield of PSII (ΔФII), recorded after application of saturating light pulses. These findings strongly suggest that there is an exogenous timekeeper which is a stimulus for the oscillations detected in both the linearized yield of chlorophyll fluorescence (ΔFt) and the linearized quantum yield of PSII (ΔФII).

Abbreviations

PSII

Photosystem II

ΔFt

linearized yield of chlorophyll fluorescence

ΔФII

linearized quantum yield of PSII

LL

continuous illumination.

Introduction

Early text books in plant biology can be traced back to Theophrastus of Eresos (371 – 287 BC) which constitute the most important contribution to botanical science during antiquity and the Middle Ages. His “Enquiries into plants and minor works on odours and weather signs” contains the first systematization of the botanical world; on the strength of these works some call Theophrastus the “father of botany.”¹ A similar milestone in the history of botanical literature was set nearly 2000 years later by Julius Sachs in the year 1865. His “Handbuch der Experimental-Physiologie der Pflanzen” covers a wide range of issues in plant physiology, many of which are still unresolved.² Specific issues with major impact for the present study focus on photosynthesis. In chapter 1 of this monumental Handbuch, Sachs described several home-made devices used to investigate chlorophyll fluorescence.² In this context, he emphasized the enormous potential of his methodology to obtain important insights into the mechanisms of major plant processes involved in CO₂ assimilation and its relationship to water movement within the plant.

More than 60 years after Sachs's publication of 1865, Kautsky & Hirsch in the year 1931 observed that changes in chlorophyll fluorescence induced by illumination of dark-adapted leaves were qualitatively correlated with changes in CO₂ assimilation.³ Later, it became apparent that, under some circumstances, chlorophyll fluorescence emissions in photosynthetic organisms could be correlated with their photosynthetic rates. Due to their operational convenience, the application of chlorophyll fluorescence devices to monitor photosynthetic performance in plants is now widespread. Presently, chlorophyll fluorescence parameters are used to analyze changes in photosystem II (PSII) photochemistry, linear electron flux, and CO₂ assimilation in vivo.⁴ Correlation between Photosystem II activity and water flux-mediated leaf movement was suggested by Huang et al.⁵ To follow up on their observations, these authors examined chlorophyll fluorescence, the chlorophyll fluorescence-derived parameter maximum quantum yield of PSII (Fv/Fm) and diurnal changes in leaf angle in the liana, Bauhinia tenuiflora, grown in an open field. During the daytime, the minimum values of Fv/Fm amounted to 0.75.⁵ In the early morning, high Fv/Fm was accompanied by large leaf angle. At noon, when Fv/Fm was <0.8, B. tenuiflora raised its leaves and leaf angle decreased.⁵ In the afternoon, when Fv/Fm was >0.8, the leaves were lowered and leaf angle gradually increased. After treatment with 3-(3, 4-dichlorophenyl)-1,1-dimethylurea (DCMU, an inhibitor of PSII), both Fv/Fm and leaf angle decreased significantly.⁵ Based on these results, Huang et al. suggested that PSII activity, as detected by chlorophyll fluorescence methods, could be involved in the regulation of leaf movement as controlled by water fluxes in the pulvini of B. tenuiflora.^5,6

Due to the availability of new geophysical methodology, various rhythmic phenomena in the circatidal range associated with plants have been demonstrated to be strongly, and probably causally, correlated with the lunisolar gravitational force.^7-9 This weak tidal force is the result of the rotation of the Earth around its axis in conjunction with the orbital motions of the Earth and its Moon around the Sun. Within the framework of general relativity, tidal forces reflect specific curvatures in the 4-dimensional space time continuum.¹⁰ As a consequence, this curvature continually modulates the gravitational field of the Earth an effect that is most pronounced and readily measurable in relation not only to the tidal movements of seas and oceans but also to small variable and elastic deformations of the Earth's crust.^11,12 These latter are measurable by gravimetry.^13,14 In particular, the recently developed ‘Etide’ program enables local variations of the Earthly gravitational acceleration, δg, due to the lunisolar tidal force, to be estimated for any given date, past or future.^7,15 These estimates therefore provide an opportunity to search for variations within biological systems that may be synchronized with geophysical timecourses.

The idea that the unforced leaf movements of bean plants which are mediated by influx and efflux of water within the pulvinus might be regulated by the lunisolar tidal force was introduced by Barlow et al.^16,17 Examples of the proposed relationship between these 2 variables, one biological the other geophysical, could be established for bean plants, Canavalia ensiformis, which were subject to 2 different sets of natural conditions.⁷ In addition, attention was drawn to an apparent concordance between the daily variation in tree stem diameter, δD, and the variation in the gravimetric tide, δg; ^18,19 and, similarly, with the aid of the Etide program, Fisahn et al. observed that roots of Arabidopsis thaliana appeared to perceive the lunisolar tidal acceleration, this perception being expressed in a tide-like variation in the rate of root elongation. ²⁰

In continuation of work by Fisahn et al on chlorophyll fluorescence,²¹ as initiated by Sachs,² the present study demonstrates a close correlation between the yield of chlorophyll fluorescence Ft derived from Photosystem II in Arabidopsis thaliana and the lunisolar tidal force. In particular, we tested the hypothesis that oscillations in the yield of chlorophyll fluorescence Ft emerging from the reaction centers of PSII under conditions of continuous illumination exhibit phase congruence and period synchrony with the lunisolar tidal force, as estimated by the Etide software application.

Results

To investigate the suitability of the Imaging-Pam technique for the detection of circatidal rhythms in chlorophyll fluorescence, outputs from the leaves of several plants of Arabidopsis thaliana were measured in parallel over 25-h periods. Prior to the measurements, the experimental leaves were mounted between thin nylon threads (Fig. 1) and continuously exposed to photon flux densities of 100 µmol quanta/ m²s over the entire recording period. Images of the leaf chlorophyll fluorescence yield Ft were taken from pre-selected areas of interest every 5 min and summed up. Figure 2 depicts typical chlorophyll fluorescence Ft records, after drift elimination, obtained from 2 leaves of 2 different plants measured in parallel. These linearized timecourses of the yield of chlorophyll fluorescence emitted from PSII were aligned with the estimated timecourses of the lunisolar tidal acceleration provided by the Etide software application. It is clear that the linearized timecourses of the chlorophyll fluorescence Ft of both leaves exhibited oscillations in the circatidal range (Fig. 2). Moreover, the peaks and troughs in the chlorophyll fluorescence records of 2 different leaves from 2 distinct Arabidopsis plants were in synchrony (Fig. 2). Alignment with the profile of the lunisolar tidal acceleration revealed additional synchrony of the chlorophyll fluorescence records, in this case with the lunisolar tidal force (Fig. 2). Based on these encouraging results, our newly developed method of linearized long-term chlorophyll fluorescence kinetics detection was further applied to more extended observational time periods.

Figure 1.

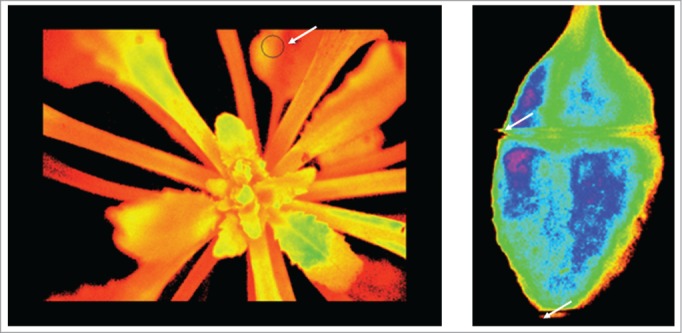
Chlorophyll fluorescence images of a 32-day old Arabidopsis thaliana rosette and a single leaf. Colors encode fluorescence intensity or yield. To numerically quantify the amount of fluorescence, areas of interest can be preselected, indicated by the black circle on the left panel and marked by a white arrow. As leaves are moving throughout extended observational periods of several days, individual leaves were gently fixed between thin nylon threads, as indicated in the right panel. Nylon threads are marked by the white arrows.

Figure 2.

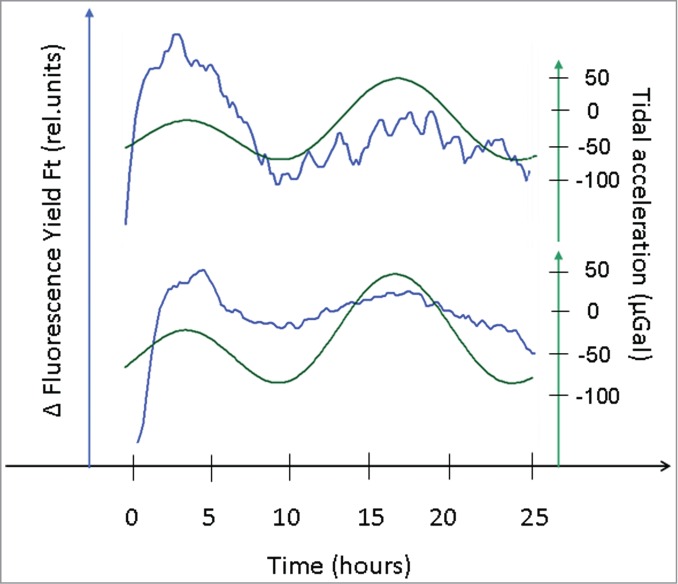
Linearized chlorophyll fluorescence yield (ΔFt, blue traces) recordings derived from 2 separate (representative) leaves of distinct plants measured in parallel over a period of 25 h (LL). These traces of ΔFt were aligned with the lunisolar tidal acceleration profile (green lines).

Timecourses of linearized chlorophyll fluorescence were prepared over extended periods of 4 days under conditions of continuous illumination (Fig. 3, upper trace). Rhythmicity with a circatidal periodicity continued throughout the entire 4-d measuring interval (Fig. 3). Alignment of these linearized chlorophyll fluorescence timecourses with the profiles of the contemporaneous lunisolar tidal acceleration again suggested (cf. Fig. 2) the expected congruence of both timecourses (Fig. 3, 2 upper traces). During the observational period, the shape of the lunisolar tidal acceleration is developing a bimodal configuration; it seems that only one of 2 troughs in the bimodal pattern of the lunisolar tidal acceleration is picked up by the chlorophyll fluorescence kinetics. Similar findings have been reported by Barlow and Fisahn, and Fisahn et al, to occur in leaf movement records of bean leaves and Arabidopsis thaliana.^7,9 Due to the bimodal phase of the lunisolar tidal profile, it is rather optimistic to estimate the actual periodicity immanent within the linearized chlorophyll fluorescence timecourse. Therefore, a 24-h and a 24.8-h sine wave was fitted to the linearized record of the chlorophyll fluorescence yield (Fig. 3, lower trace; compare also Fisahn et al.²⁰). The degree of correlation between the 24-h and the 24.8-h sine waves and the linearized chlorophyll fluorescence profile was quantified by a cross-correlation technique (Fig. 3). The cross-correlation function identified a periodicity of 24.8 h immanent within the linearized chlorophyll fluorescence timecourses. A 24.8-h periodicity is also immanent within the Etide timecourses.

Figure 3.

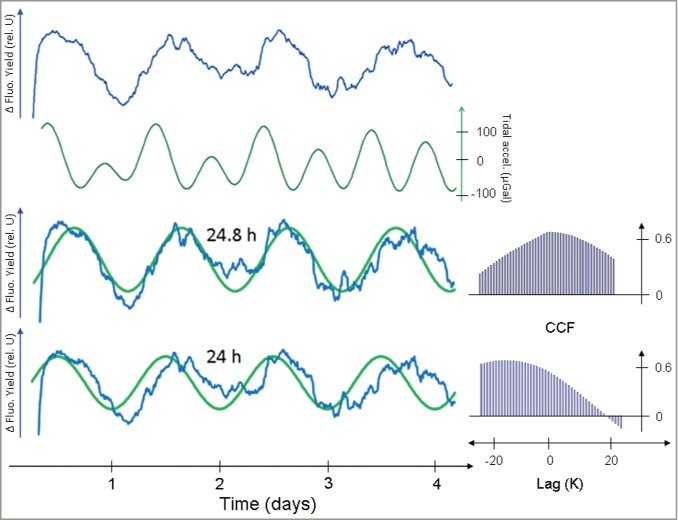
Linearized chlorophyll fluorescence yield (ΔFt) recorded over several days (upper blue line) under conditions of continuous illumination (LL). Lunisolar tidal acceleration (green line) was calculated with the aid of the Etide software application and aligned with the ΔFt trace. In the lower part of the figure the linearized chlorophyll fluorescence yield timecourses (blue lines) were aligned with a sine wave of 24.8 h and 24 h (green lines), respectively. The degree of correlation between these alignments was quantified by application of the cross correlation function depicted to the right of the sine wave alignments.

To underline the statistical significance of the newly developed linearized chlorophyll fluorescence detection method, we averaged 20 time series recorded over a span of 7 days with a sampling period of 5 minutes (Fig. 4, upper trace), Subsequent alignment of this averaged timecourse of linearized chlorophyll fluorescence yields also suggests phase congruence and period synchrony with the lunisolar tidal acceleration (Fig. 4, upper 2 traces). As a consequence of the development of a bimodal tidal during the measurement period the amplitude in the tidal acceleration decreases in the positive regime (Fig. 4). A parallel decrease emerged in the averaged linearized chlorophyll fluorescence signal (Fig. 4). Notable positive peaks, e.g. above the zero line, in the profile of tidal accelerations decreased to almost zero during the 7-day observational period (Fig. 4). To demonstrate the impact of the lunar gravitational acceleration on the total tidal force and the chlorophyll fluorescence kinetics, we depicted the times of moon rise, moon set and the declination of the Moon with regard to the horizon (Fig. 4, lower trace). In this situation of development of a bimodal pattern in lunisolar tidal acceleration, moonrise correlated with the increase in the averaged linearized chlorophyll fluorescence yield.

Figure 4.

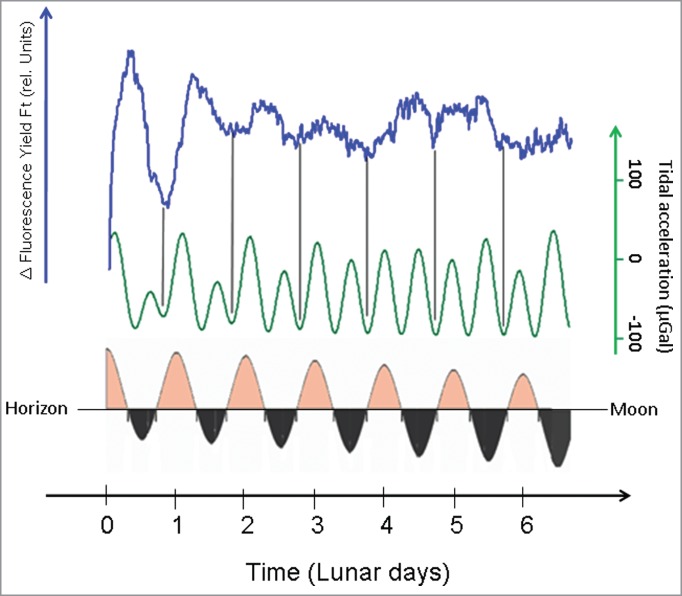
Average of 20 timecourses of the linearized chlorophyll fluorescence yield (ΔFt) recorded over a period of 7days (blue line) under conditions of continuous illumination (LL) aligned with the lunisolar tidal acceleration (green line). The lower part of the figure depicts the times of moon rise and set as well as the relative position of the moon with regard to the horizon.

Mechanisms involved in reception and processing of the lunisolar gravitational signal are presently unknown. To obtain further insights into the putative mediators of weak gravity perception, it is important to analyze events that interfere with the standard responses, as described in Figs 2–4. As already described in Barlow and Fisahn⁷ and Fisahn et al⁹ the elementary mode in the leaf movement response patterns of either 24.8 h, for leaf movements on Earth, or 90 min, for leaf movements in International Space Station (ISS), could be extended and shortened in synchrony with the lunisolar tidal acceleration. In the present investigation of linearized chlorophyll fluorescence timecourses, the bimodal lunisolar tidal profile severely declines (Fig. 5) and, as a consequence, the initial 24.8-periodicity extends to a periodicity of 24.8 h + 12.4 h = 37.2 h (Fig. 5). Subsequently an oscillation with a period of 12.4 h was observed in the linearized chlorophyll fluorescence time series (Fig. 5). Eventually, the elementary mode of 24.8 h is regained in the fluorescence records (Fig. 5). Similar findings were described for the ultradian oscillations in leaf movement detected in the ISS.⁹ In particular, periods of 45 min, 90 min and 135 min in leaf movement were reported by Solheim et al.²² However, these oscillations exactly matched the oscillations of the in-orbit lunisolar tidal profiles.⁹

Figure 5.

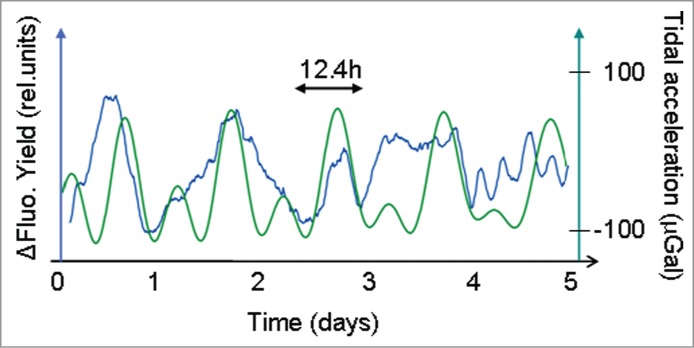
Period deviations of the linearized chlorophyll fluorescence yield (ΔFt, blue line) from the elemental mode of 24.8 h under continuous illumination (LL). ΔFt time courses were aligned with the profile of the lunisolar tidal acceleration (green line).

Saturating light pulses were applied to investigate the quantum yield of PSII (ФII) in the Crassulacean acid metabolism plant Kalanchoe daigremontiana under continuous illumination for several days.²³ Control plants exhibited weak oscillations in the circatidal range,²³ but neither the phase nor the periodicity remained constant in the prevailing environmental conditions (Wyka et al.²³, their Fig. 5c). It could be speculated that the repeated application of high-intensity light pulses for several days might have interfered with the various light receptors of the plant and thus induced an uncontrolled reset of the circadian system. To test the applicability of saturating light pulses for the detection of circatidal rhythms in Arabidopsis thaliana, we exposed the experimental plants for several days to continuous illumination and additionally provided saturating light pulses with a 10-min repeat period. Surprisingly, the linearized yield of chlorophyll fluorescence exactly followed the lunisolar tidal profile, even under these conditions of repeated light flashing (Fig. 6, upper trace). Based on the detected fluorescence parameters associated with the saturating light pulses, the linearized quantum yield of PSII (ΔФII) was calculated. Phase congruence and period synchrony with the inverted lunisolar tidal acceleration was revealed (Fig. 6). During the observational recording period a predominantly near-unimodal lunisolar tidal profile prevailed (Fig. 6). In this situation of prevailing unimodality in the lunisolar tidal acceleration, decreasing phases in the linearized quantum yield of PSII (ΔФII) coincided with the strong lunar and solar phases related to positions below the horizon (Fig. 6). Moreover, both Sun and Moon have short times of visibility as measurements were performed in winter time. Noteworthy, the linearized quantum yield of PSII (ΔФII) exhibited no immediate phase congruence with the subjective LD conditions used in the entraining period prior to the application of continuous light (LL) (Fig. 6).

Figure 6.

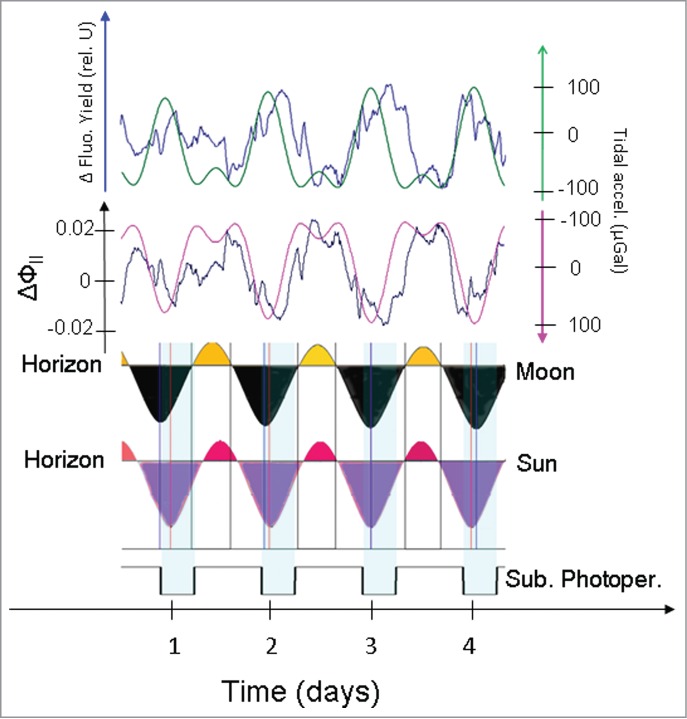
Comparison of records of the linearized chlorophyll fluorescence yield (ΔFt) and the linearized quantum yield of PSII (ΔФII). To test the applicability of saturating light pulses for the detection of circatidal rhythms in Arabidopsis thaliana, the experimental plants were exposed to continuous illumination. Saturating light pulses with a 10-min repeat period were applied to quantify the linearized quantum yield of PSII (ΔФII). The linearized chlorophyll fluorescence yield (ΔFt, blue line) was aligned with the timecourse of the lunisolar tidal acceleration (green line, upper panel). The linearized quantum yield of PSII (ΔФII) was aligned with the inverted profile of the lunisolar tidal acceleration (pink line, second alignment from top). Both parameters ΔFt and ΔФII exhibited high degrees of synchrony with the lunisolar tidal acceleration. The lower part of the figure depicts the times of Moon rise and set, as well as the times of Sun rise and set. Relative positions of the Moon and the Sun are indicated above and below the horizon. The bottom trace depicts subjective light/dark cycles.

Discussion

Based on early observations of chlorophyll fluorescence pioneered by Sachs already in the year 1865,² we developed a novel extension of this method to answer one of the many issues in plant physiology addressed by Sachs,² namely, whether endogenous or exogenous origin of circatidal rhythms could be detected in the various plant-associated parameters.^7,9 Linearized chlorophyll fluorescence yield (ΔFt) recordings were established as a representative output of these rhythms.

Distinction between the exogenous or endogenous origin of the detected oscillations in the linearized yield of chlorophyll fluorescence (ΔFt) emerged from alignments with the lunisolar tidal profiles (Fig. 2–6) estimated by the Etide software application.⁷ Synchrony in periodicity and phase congruence between the lunisolar tidal acceleration and the linearized yield of chlorophyll fluorescence (ΔFt) (Figs 2–6) strongly suggests an environmental cause, reflected in the Etide values, of the rhythmicity in the Photosystem II- associated parameter. Similar conclusions were derived from leaf movement studies performed in Earth-bound laboratories^7,8 and during in-orbit investigations performed in the ISS.⁹

In previous models, circadian ion fluxes across the thylakoid membrane were proposed to generate reversible conformational changes which couple/uncouple entire photosynthetic units in the thylakoid membrane and thereby induce a circadian rhythmicity in the photosynthetic capacity of the cell.²⁴ Circadian oscillations in membrane properties of Gonyaulax have been documented and these include rhythms in both membrane electrical potential²⁵ and thylakoid orientation.²⁶ Chl a fluorescence, which also displays a circadian rhythmicity in Gonyaulax, is a sensitive probe of structural changes in the thylakoid and can be particularly responsive to changes in cation concentrations and lipid composition.²⁴ The general effect of cations on the structural and energetic state of chloroplast membranes has been well established. Specifically related to photosynthetic activity, protons are liberated as a result of photosystem II-dependent oxidation of water, and the free protons are transported into the thylakoid membrane or inner thylakoid space. The uptake of protons has been shown to be closely linked to K⁺, Mg²⁺, and water efflux.²⁴ Based on our present results, we suggest that the lunisolar tidal force affects the ion and water fluxes across the thylakoids of Arabidopsis thaliana and thereby controls the energetic state of the membrane and thus its photosynthetic activity. This flux of water could be part of a more general tidally modulated flux of water into the leaf and petiole that drives oscillatory leaf movement.

The mechanisms involved in the perception and processing of the strength of the lunisolar field by plants are neither understood nor identified at present. Nevertheless, to complement explanations derived from classical Newtonian mechanics or general relativity, quantum gravitational models have been developed by Dorda which could account for the described causal relationship between the lunisolar gravity profile and the circatidal rhythms.²⁷ Central to these models are coherent assemblies of water molecules that interact in a quantum physical manner with the lunisolar gravitational field. As a consequence of this interaction, the water volume within susceptible cells or organelles is modulated in a lunisolar-force specific manner, and an initial movement of water due to a change in the gravitational field, as envisaged by Dorda²⁷, may lead to a cascade of ion fluxes which translate into, and amplify, the osmotic changes necessary to bring about a rapid alteration of the thylakoid environment. In support of the quantum gravitational model suggested by Dorda,²⁷ techniques of 2-dimensional electronic spectroscopy have been recently applied to explore photosynthetic–light–harvesting complexes and revealed the existence of long lived quantum coherence among the electronic excited states of the multiple pigments in pigment protein complexes.^28-30 Therefore, based on the framework provided by Dorda²⁷ it can be speculated that this long-lived quantum coherence within the pigment protein complexes is modulated by the lunisolar tidal force and thus modulates the yield of chlorophyll fluorescence in a lunisolar-acceleration associated manner.

In conclusion, the present study traces back to early pioneering experiments on chlorophyll fluorescence performed by Sachs in the year 1865.² As envisioned by Sachs,² technical equipment has now been developed to automatize long-term recordings of chlorophyll fluorescence. Nowadays, computerized detections of time series in the yield of chlorophyll fluorescence has enabled, 150 years after the publication of Sachs's Handbuch, answers to a still controversial issue in plant biology. The exogenous or endogenous origin of rhythmic phenomena in the circatidal range exhibited by plants was an issue already raised by Sachs.² In summary, our present study revealed the exogenous origin of circatidal oscillations in the linearized yield of chlorophyll fluorescence (ΔFt) and the linearized quantum yield of PSII (ΔФII). Potentially these rhythms represent a whole set of output parameters associated with the flow of water within the plant.

Methods

Plant material

Seedlings of Arabidopsis thaliana (L.) Heyhn. ecotype Columbia (Col-0) were grown within a regime of white light and darkness (LD 16 : 8 h) in a green house. Temperature was held constant at 20° ± 2° C. Humidity was adjusted to 60%. After 4 weeks, plantlets were transferred to a phytochamber for chlorophyll fluorescence measurements. Conditions in the phytochamber were set to a temperature of 20° ± 0.5° C, 60% humidity and continuous illumination at a photon flux density of 100 µmol quanta / m²s generated by metal halogen lamps (HPI-T 400W-645; Philips, Hamburg, Germany).

Chlorophyll fluorescence imaging

Chlorophyll fluorescence imaging was performed by an ‘Imaging-Pam’ chlorophyll fluorometer (Heinz Walz GmbH, Effeltrich, Germany) as described in Wulff-Zottele et al,³¹ at photon flux densities of 100 µmol quanta / m²s (Fig. 1). To avoid artifacts induced by leaf movements during the extended measuring periods of several days, the experimental leaves were gently fixed between 2 thin nylon threads (Fig. 1).^21,32

Continuous records of the current fluorescence yield Ft

The current fluorescence yield, Ft, is continuously monitored in the Measure-mode of the Imaging-Pam when the measuring light (ML) is switched on. Ft images could be saved without the application of a saturating light pulse by operation of the “Ft only clock” option.

To quantify the information contained in the Ft images, areas of interest were defined at various locations of an experimental leaf (Fig. 1). Ft images of several leaves maintained under continuous illumination were saved every 5 min for several days.

Continuous records of the ФII quantum yield

To determine the quantum yield of photochemical energy conversion in PS II, a series of saturating light pulses was applied at 10 min intervals for several days at continuous photon flux densities of 100 µmol quanta / m²s.^21,31-34

Drift elimination

To amplify the actual small amplitudes of the circatidal kinetics superimposed upon the recorded chlorophyll fluorescence signals during the observational period, polynomials up to sixth-order were fitted to the measured kinetics and subsequently subtracted from the experimentally obtained results.^9,35 These linearized leaf chlorophyll fluorescence timecourses were then aligned with the estimated timecourse of the lunisolar gravity profile derived from the Etide software.

Lunisolar gravity profile

Standing proxy for a direct measurement of the lunisolar tidal force is an estimate of the gravimetric tide provided by the software application ‘Etide’.⁷ The program, using the algorithm of Longman,¹⁵ is based upon the 50 parameters which compute the vertical component of the lunisolar tidal force (the horizontal component of which is negligible) and is coupled with an elasticity factor of 1.16 for the body of the Earth. The input to Etide consists of the latitude, longitude and altitude of the location in question, together with the calendar dates for which local gravimetric tidal estimates are required. The output is a timecourse of δg (a gravity profile), the increase and decrease of the Earth's gravitational acceleration at any particular location brought about by the combined gravitational forces of the Sun and Moon. Numerically, it consists of gravimetric values in units of µGals (the gravitational acceleration at the Earth's surface is 1 g = 9.81 × 10⁸ µGals, where 1 Gal = 1 cm s⁻² and 1 g = 9.81 m /s²) which are estimated at specified intervals over the required period. The δg time courses are prepared with reference to Coordinated Universal Time (UTC) and therefore need to be matched to the local times at which the biological data were recorded. When required, times of sunrise, sunset, moonrise and moonset, for any date at a given location were obtained from:http://aa.usno.navy.mil/data/docs/RS_OneYear.php or http://www.timeanddate.com/moon/phases/germany/berlin.

Statistical methods

Cross-correlation methods were used according to the WESSA program available on the Internet.³⁶

Disclosure of Potential Conflicts of Interest

No potential conflicts of interest were disclosed.

Acknowledgments

We would like to acknowledge valuable discussions on coherent particle assemblies and quantum coherence phenomena with Prof. Dr. R. Menzel (University of Potsdam, Department of Experimental Physics).