Role of I_h in differentiating the dynamics of the gastric and pyloric neurons in the stomatogastric ganglion of the lobster, Homarus americanus

Abstract

The hyperpolarization-activated inward cationic current (I_h) is known to regulate the rhythmicity, excitability, and synaptic transmission in heart cells and many types of neurons across a variety of species, including some pyloric and gastric mill neurons in the stomatogastric ganglion (STG) in Cancer borealis and Panulirus interruptus. However, little is known about the role of I_h in regulating the gastric mill dynamics and its contribution to the dynamical bifurcation of the gastric mill and pyloric networks. We investigated the role of I_h in the rhythmic activity and cellular excitability of both the gastric mill neurons (medial gastric, gastric mill) and pyloric neurons (pyloric dilator, lateral pyloric) in Homarus americanus. Through testing the burst period between 5 and 50 mM CsCl, and elimination of postinhibitory rebound and voltage sag, we found that 30 mM CsCl can sufficiently block I_h in both the pyloric and gastric mill neurons. Our results show that I_h maintains the excitability of both the pyloric and gastric mill neurons. However, I_h regulates slow oscillations of the pyloric and gastric mill neurons differently. Specifically, blocking I_h diminishes the difference between the pyloric and gastric mill burst periods by increasing the pyloric burst period and decreasing the gastric mill burst period. Moreover, the phase-plane analysis shows that blocking I_h causes the trajectory of slow oscillations of the gastric mill neurons to change toward the pyloric sinusoidal-like trajectories. In addition to regulating the pyloric rhythm, we found that I_h is also essential for the gastric mill rhythms and differentially regulates these two dynamics.

the stomatogastric ganglion (STG) contains two central pattern generators (CPGs) that produce distinct rhythms in vitro: a slow gastric mill CPG that generates flexible rhythmic outputs (5∼20 s) that controls the movement of teeth in the stomach and a fast pyloric CPG that generates more consistent rhythmic outputs (0.5∼2 s) that controls the filtering movements of the pylorus (Selverston 2005). In the pyloric network, the motor neuron pyloric dilator (PD) and interneuron anterior burster (AB) are pacemaker neurons (Hartline and Russell 1984; Miller and Selverston 1982). In the gastric mill network, interneuron 1 (Int1) is the pacemaker neuron; and, the medial gastric (MG), lateral gastric (LG), and dorsal gastric (DG) neurons are endogenous bursters that are coordinated by the Int1 neuron through excitatory and inhibitory synaptic inputs (Elson and Selverston 1992; Selverston et al. 2009). For both rhythms to operate in vitro, they must receive neuromodulatory inputs from the two commissural ganglia (CoGs) and the esophageal ganglion (Selverston et al. 1976). During early embryonic stages, the lobster stomatogastric nervous system is first organized into a single embryonic network that generates a single rhythmic pattern. The system then bifurcates into two distinct functional adult networks, the pyloric and gastric mill networks (Fenelon et al. 2003; Lavalli and Ayers 1994; Le Feuvre et al. 1999). To date, one of the most intriguing questions remaining is what underlying mechanisms differentiate the dynamics of the fast pyloric and slow gastric mill CPGs. Different ion channel conductances contribute to the properties of individual neurons and therefore to the overall dynamics of both the pyloric and gastric mill CPGs (Harris-Warrick 2002; Marder and Bucher 2007; Selverston 2005). The goal of this study is to evaluate the role of the hyperpolarization-activated inward cationic current (I_h) in mediating the difference in the dynamics of the two rhythms. We used specific blockers for I_h that block its effect in vitro and evaluated quantitatively the changes to both rhythms.

The hyperpolarization-activated inward cationic current (I_h) is mediated predominately by Na⁺ and K⁺ and typically activates at −45 to approximately −60 mV, which is close to the resting membrane potential (Pape 1996; Robinson and Siegelbaum 2003). I_h has multiple functions in different cell types across species. The most recognized function is the pacing function that was described in heart cells, motor neurons, and other neuron types (DiFrancesco 1993; McCormick and Pape 1990; Pape 1996). I_h depolarizes the membrane potential to facilitate the onset of consecutive bursts or action potentials, which is essential for spontaneous oscillatory activity. I_h also modulates neuronal excitability (Thoby-Brisson et al. 2000) and dendrite excitability (Beaumont and Zucker 2000; Magee 1998). The kinetics and other properties of I_h vary in different cell types (Santoro and Baram 2003). Blocking I_h with CsCl or ZD-7288 decreases the pacemaker burst period in the inspiratory pre-Bötzinger complex neurons in mice (Thoby-Brisson et al. 2000). In Cancer borealis and Panulirus interruptus, I_h regulates postinhibitory rebound (PIR) and neuronal excitability in the PD and lateral pyloric (LP) neurons (Golowasch et al. 1992; Ouyang et al. 2007; Peck et al. 2006; Zhang et al. 2003). Studies have shown that I_h exists in most of the pyloric and gastric mill neurons (Kiehn and Harris-Warrick 1992; Schulz et al. 2007); however, little is known about the function of I_h in regulating the dynamics of the gastric mill neurons.

We asked 1) how does I_h regulate the gastric mill dynamics and 2) does I_h contribute to differentiating between the distinct dynamics of the gastric mill and pyloric rhythms? We bath applied the selective channel blocker CsCl to pharmacologically block I_h in only the STG and recorded individual neuronal activity. We did not synaptically isolate individual neurons because we wanted to investigate the neuronal dynamics in an active network and evaluate the contributions of I_h to the dynamics. Studies on P. interruptus show that I_h is localized in close proximity to synapses but not in the presynaptic terminals in the STG (Goeritz et al. 2011). Therefore, the effects of blocking I_h in the STG can be considered a result of decreasing I_h in the neurons within the STG. We conclude that I_h expressed in the STG shapes the dynamics of the gastric and pyloric neurons differently. Specifically, it affects their burst periods and oscillatory trajectories differently and regulates the intraburst spike frequency and duty cycle of both the gastric and pyloric neurons.

MATERIALS AND METHODS

Animals and solutions.

American lobsters, Homarus americanus of either sex, weighting between 0.5 and 1 kg were obtained from Nahant Fish & Lobster (Nahant, MA) and F/V Jacqueline Bess (Swampscott, MA). The animals were kept in aerated ambient running seawater at 10–15°C. Before dissection, the animals were anesthetized by cooling at 4°C for 30 min. The H. americanus physiological saline that we used was composed of (in mM) 479.12 NaCl, 12.74 KCl, 13.67 CaCl₂, 20 MgSO₄, 3.91 NaSO₄, and 5 HEPES; pH was set to 7.4–7.5. The saline was supplemented with 1 g/l glucose. The saline was filtered through a 0.2-μM filter, and we added 20 μg/ml penicillin and streptomycin before use. CsCl solutions were directly made with sterile saline solution and kept at −80°C before use. All chemicals were purchased from Sigma-Aldrich.

Preparation.

The stomatogastric nervous system containing the STG, a pair of commissural ganglia, the esophageal ganglion, and output motor nerves were separated from the stomach and pinned down in a Sylgard-lined petri dish (Maynard and Dando 1974) with chilled saline (14°C). The sheath of the STG was removed using a pair of sharp forceps to facilitate access to the soma of neurons. The STG was enclosed in a small well made of petroleum jelly that served as a separate chamber of ∼1 ml volume. CsCl solutions were applied to the STG while the anterior ganglia and output motor nerves were bathed in normal physiological saline. The stomatogastric nervous system tissue was perfused with the physiological saline throughout the experiment.

Electrophysiology and data acquisition.

In this study, we used both the extracellular and intracellular recording methods. Extracellular voltage races were measured using model 1700 Differential AC Amplifiers (A-M Systems, Carlsborg, WA). Intracellular voltage traces were measured using model 1600 Neuroprobe Amplifiers (A-M Systems) in bridge mode. Each individual soma was impaled with glass microelectrodes filled with 3 M KCl with a resistance of 10–30 MΩ. Cell identification was achieved by comparing intracellular recording traces with simultaneous extracellular recordings from the output motor nerves and by observing the phase relationship among neurons. To hyperpolarize cells, −10 nA current was applied to the cell through manually pressing the hyperpolarization button on model 1600 Neuroprobe Amplifiers. Voltage traces were acquired and digitized by a computer equipped with a PCI-MIO-16E4 data acquisition board (National Instruments, Austin, TX). Data were recorded and saved using Axoscope (Axon Instruments, Foster City, CA).

Data analysis.

The voltage traces were analyzed in MATLAB (Mathworks) using self-written and modified scripts from Dr. Daniel Wagenaar's MBL course package (2008–2011) (http://www.its.caltech.edu/~daw/teach.html). The burst period was calculated primarily by determining the peak frequency using fast-Fourier transform (FFT). We also calculated it by averaging the individual burst period of all bursts on a spike train to eliminate potential errors during FFT analysis. Both methods produce nearly identical results. The intraburst spike frequency was the average instantaneous spike frequency within bursts. The instantaneous spike frequency was calculated based on the time delay between two consecutive spikes within bursts. The duty cycle is defined as the burst duration divided by the burst period. The burst duration was defined as the time between the first and last spike of a burst. The intraburst spike frequency and duty cycle were calculated by averaging the results from at least 20 cycles of bursts on a spike train. Both intracellular and extracellular recordings were used in extracting the spikes. For the oscillation trajectory analysis, slow oscillation traces were generated by applying a second-order Butterworth lowpass filter to original voltage traces to filter out all action potentials; the cut-off frequency was 5 Hz for the pyloric neuron traces and 1.5 Hz for the gastric mill neuron traces. The phase-plane analysis used the voltage, as well as the first and second derivatives of voltage. The cell membrane acts like a capacitor; I_Cap = dQ/dt = d(CV)/dt = C(dV/dt), where I_Cap is capacitive current, dQ is the rate of change of charge, C is the capacitance of the cell membrane, V is the membrane potential, and t is time. Because we simply recorded from a native neuron without external manipulations such as injecting external current or holding voltage, I_Cap is equal to the net membrane ionic current (I_Ion) but in the opposite direction. Therefore, the first derivative of voltage (dV/dt) is proportional to the current, and the second derivative of voltage (d²V/dt²) is proportional to the rate of change of current. The membrane capacitance (C) is determined by the thickness, area, and dielectric constant of the membrane (Niebur 2008), which are not altered by pharmacologically blocking I_h and thus can be considered constant. We compared dV/dt and d²V/dt² to indicate changes in current and the rate of change of current before and after blocking I_h. In the analysis to examine the PD modulation of the gastric mill neurons, two consecutive PD burst cycles were plotted in each graph to show the PD modulatory effect on the gastric mill neurons. The voltage of the gastric mill neurons from the recording data was first put through a lowpass filter at 10 Hz to eliminate the spikes. The filtered voltage data were then normalized by dividing each PD burst cycle into 400 time bins. The mean value for each time bin was used to represent the voltage of the gastric mill neurons over the two consecutive PD burst cycles. Each voltage trace shown in the analysis plots is the average value of three selected sections in the recording of each trial. In the MG and gastric mill (GM) neurons, only the PD bursts during the gastric interburst intervals were selected. In the lateral posterior gastric (LPG) neurons, only the PD bursts during the LPG bursts were selected. For statistic analysis, all data used showed normal distribution according to the Anderson-Darling normality test. We used the Student's t-test or one-way ANOVA followed by Tukey's honest significant difference (HSD) post hoc test for statistical analysis. Error bars are represented as SD.

RESULTS

I_h was sufficiently blocked by 30 mm CsCl in H. americanus.

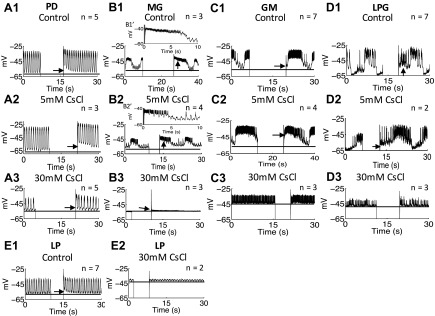
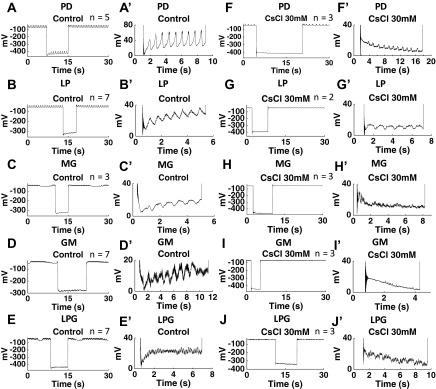
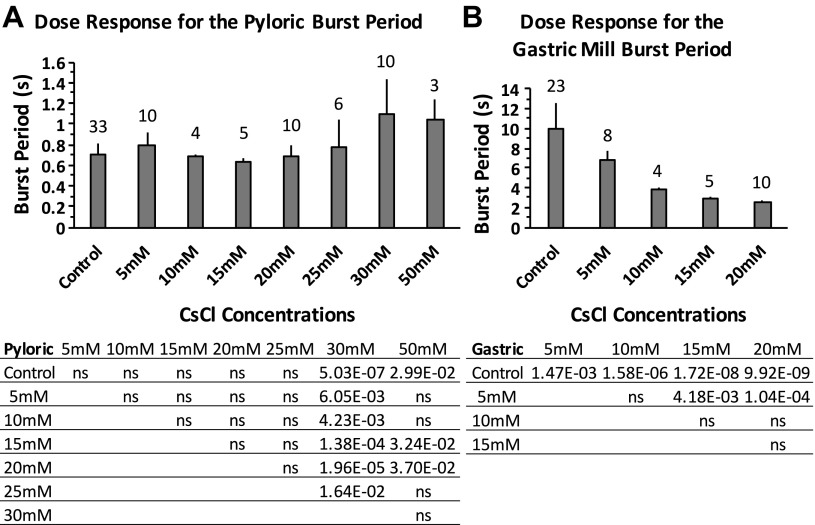
We sought to determine the optimal inhibitory concentration of CsCl. I_h induces a signature voltage sag upon hyperpolarization and a signature PIR upon releasing from hyperpolarization; low concentrations of Cs⁺ potently block the voltage sag and the PIR in mammalian cells (Pape 1996). CsCl (5 mM) can block most I_h in C. borealis and P. interruputs (Kiehn and Harris-Warrick 1992; Peck et al. 2006; Zhang et al. 2003) but does not block the I_h-induced PIR in pyloric neurons of H. americanus (Ballo and Bucher 2009). Our results show that 5 mM CsCl did not completely block the PIR induced by I_h in the PD, MG, GM, and LPG neurons in H. americanus (Fig. 1). Therefore, we tested CsCl concentrations ranging from 5 to 50 mM. The concentrations were applied in increasing sequence. We washed with normal saline for between 10 and 30 min to reverse the effects in between concentrations. Measurements were taken 10 min after bath applying CsCl. Through the measurement of the burst period, as well as the elimination of PIR and voltage sag in response to the injection of 10 nA hyperpolarizing current for >3 s, we found that 30 mM CsCl sufficiently blocked I_h-induced PIR (Fig. 1) and voltage sag (Fig. 2) in all of the investigated pyloric and gastric mill neurons. The I_h-induced voltage sag was present in all of the investigated neurons in the control conditions (Fig. 2, A′-E′). The voltage sag was completely abolished after application of 30 mM CsCl (Fig. 2, F′-J′). The dose-response results indicate that the pyloric burst period was affected by blocking I_h with CsCl applications, and the burst period increased at 30 and 50 mM CsCl applications [1-way ANOVA with Tukey's HSD post hoc test, F(7,73) = 7.981, P < 0.0001; Fig. 3A]. The change of the pyloric burst period from the control condition was significantly bigger at 30 and 50 mM CsCl applications than at concentrations lower than 30 mM [1-way ANOVA with Tukey's HSD post hoc test, F(6,41) = 8.535, P < 0.0001; between 30 mM and lower concentrations: P < 0.01; between 50 mM and concentrations lower than 30 mM: P < 0.05, n = 10 (5 mM), 4 (10 mM), 5 (15 mM), 10 (20 mM), 6 (25 mM), 10 (30 mM), and 3 (50 mM)]. The gastric mill burst period decreased when the CsCl concentration increased [1-way ANOVA with Tukey's HSD post hoc test, F(4,45) = 38.654, P < 0.0001; Fig. 3B]. Compared with the decrease of burst period at 5 mM CsCl application, the decrease of burst period was more significant at 15 and 20 mM CsCl applications [1-way ANOVA with Tukey's HSD post hoc test, F(3,23) = 11.518, P < 0.0001; between 5 and 15 mM CsCl applications: P = 0.0003; between 5 and 20 mM CsCl applications: P = 0.0002, n = 8 (5 mM), 4 (10 mM), 5 (15 mM), and 10 (20 mM)]. The MG, GM, and LPG neurons fired tonically or became silent when the CsCl concentration reached above 20 mM [data not shown, n = 6 (25 mM), and 10 (30 mM), the MG and GM neurons fired tonically in all trials at 25 mM CsCl application, and the LPG fired tonically in all trials at 30 mM CsCl application]. Therefore, we only plotted the gastric mill burst period between 5 and 20 mM CsCl and used results from application of 20 mM CsCl for the analysis of the gastric mill neurons. The blocking by CsCl is reversible after a 10- to 30-min wash with fresh saline. The pyloric burst period fully recovered to the control level (0.80 ± 0.29 s in the control condition, 0.89 ± 0.16 s after wash, paired Student's t-test, n = 10, P > 0.05). The gastric burst period recovered to 7.02 ± 0.61 s compared with 9.15 ± 1.82 s in the control condition (paired Student's t-test, n = 8, P = 0.023). The recovered gastric mill period is within the typical normal range of 5–10 s (Weimann et al. 1991). In addition, both the pyloric and gastric mill neurons recovered to normal oscillations [data not shown, n = 6 (PD), 3 (LP), 2 ventricular dilator (VD), 4 (MG), 6 (GM), and 5 (LPG)]. Thus we consider the CsCl application reversible. We are aware that using CsCl has the potential drawback of blocking some other potassium currents (Coggan et al. 1994; Pape 1996; Thoby-Brisson et al. 2000; Wischmeyer and Karschin 1997). Therefore, we also blocked I_h using 50, 100, and 150 μM ZD-7288. The results are consistent with our results from application of CsCl and are included below.

Fig. 1.

Postinhibitory rebound (PIR) in the pyloric dilator (PD), medial gastric (MG), lateral pyloric (LP), gastric mill (GM), and lateral posterior gastric (LPG) neurons was blocked by 30 mM CsCl. Arrowheads show PIR after releasing from injecting 10-nA hyperpolarizing current. A1: the PIR was present in the PD neurons in the control condition. A2: the PIR was present in the PD neurons after application of 5 mM CsCl. A3: the PIR was blocked in the PD neurons after application of 30 mM CsCl. B1′ and B2′ are magnified images to show PIR in B1 and B2, respectively. B1 and B1′: the PIR was present in the MG neuron in the control condition and caused the temporary increase in burst duration and inraburst spike frequency. B2 and B2′: the PIR was present in the MG neuron after application of 5 mM CsCl and caused the temporary increase in burst duration and intraburst spike frequency. B3: the PIR was blocked in the MG neuron after application of 30 mM CsCl. C1: PIR was present in the control condition in the GM neurons. C2: PIR was present in the GM neurons after application of 5 mM CsCl. C3: the PIR was blocked in the GM neurons after application of 30 mM CsCl. D1: the PIR was present in the LPG neurons in the control condition. D2: the PIR was present in the LPG neurons after application of 5 mM CsCl. D3: the PIR was blocked in the LPG neurons after application of 30 mM CsCl. E1: the PIR was present in the LP neuron in the control condition. E2: PIR was blocked in the LP neuron after application of 30 mM CsCl. The number of trials (n) is indicated.

Fig. 2.

CsCl (30 mM) eliminated the voltage “sag” induced by hyperpolarization-activated inward cationic current (I_h) in the PD, LP, MG, GM, and LP neurons. A′, B′, C′, D′, E′, F′, G′, H′, I′, and J′ are magnified images from A, B, C, D, E, F, G, H, I, and J, respectively. A, A′, B, B′, C, C′, D, D′, E, E′: in the control condition, the PD, LP, MG, GM and LPG neurons all showed voltage sag after a −10-nA hyperpolarization. F, F′, G, G′, H, H′, I, I′, J, J′: after application of 30 mM CsCl, the PD, LP, MG, GM, and LPG neurons did not show voltage sag after a −10-nA hyperpolarization. n Values are indicated in each plot. The large negative voltage value during hyperpolarization (A-J) was due to the unbalanced bridge when using the single microelectrode recording. Balancing the bridge is an adjustment of the output signal; it has no effect on the cells.

Fig. 3.

Changes of the burst period of pyloric and gastric neurons after blocking I_h with a series of concentrations of CsCl ranging from 5 to 50 mM. A: the increase in the pyloric burst period reached significance at application of 30 and 50 mM CsCl. One-way ANOVA followed by Tukey's honest significant difference (HSD) post hoc test, F(7,73) = 7.981, P < 0.0001. B: the gastric mill burst period decreased after application of CsCl at 5, 10, 15, and 20 mM concentrations. One-way ANOVA followed by Tukey's HSD post hoc test, F(4,45) = 38.654, P < 0.0001. ns, Nonsignificant. Error bars are represented as SD. The no. of trials (n) is indicated.

Blocking I_h decreased the difference between the gastric and pyloric burst period.

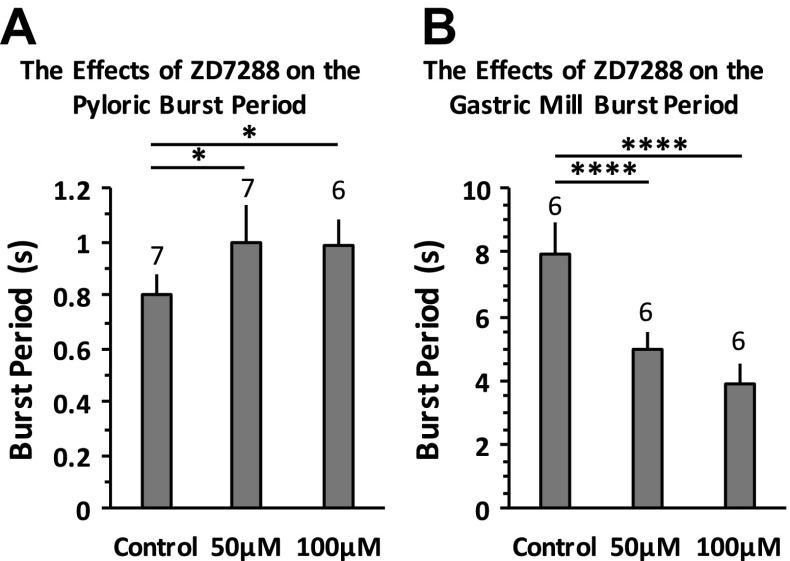
We examined the changes in burst periods of both the pyloric and gastric mill rhythms. Our results show that the pyloric burst period increased to 1.09 ± 0.34 s after application of 30 mM CsCl compared with 0.80 ± 0.29 s in the control condition (paired Student's t-test, n = 10, P = 0.002). In contrast, the gastric mill burst period decreased to 2.48 ± 0.30 s after application of 20 mM CsCl compared with 9.88 ± 2.22 s in the control condition (paired Student's t-test, n = 10, P < 0.0001). When blocking I_h with ZD-7288, we found an increased pyloric burst period after application of 50 and 100 μM [1-way ANOVA with Tukey's HSD post hoc test, F(2,17) = 6.933, P = 0.006; Fig. 4A]. The pyloric burst period at 150 μM ZD-7288 increased in all three trials. However, the increase did not show statistical significance (n = 3, paired Student's t-test, P = 0.196). This may be due to the relatively small sample size. We also found a decreased gastric burst period after application of 50 and 100 μM ZD-7288 [1-way ANOVA with Tukey's HSD post hoc test, F(2,15) = 49.546, P < 0.0001; Fig. 4B]. After application of 150 μM ZD-7288 (n = 3), the LG neuron became silent, the MG neuron became mostly silent with occasional tonic spikes, and the GM and LPG neurons changed to tonic firing. This is consistent with the results seen after application of 30 mM CsCl (n = 10): the LG neuron became silent, the MG neuron became tonic firing or silent, and the GM and LPG neurons became tonic firing. Our results indicate that 1) the gastric mill and pyloric CPGs responded to the global blocking of I_h in the STG differently, 2) I_h plays a major role in differentiating the gastric mill burst period from the pyloric burst period because the difference in burst period between the two CPGs decreased to ∼2 s after blocking I_h compared with ∼8 s in the control condition, and 3) when reducing PIR, the burst period of a neuron would normally increase because it would take a longer time to depolarize the membrane potential to initiate the next burst, which explains the increased burst period of the PD neurons after blocking I_h. However, unlike the PD neurons, the burst period of the gastric mill neurons decreased, suggesting that there should be additional pathways for I_h to modulate the gastric mill burst period in the STG, and I_h-induced PIR in the gastric mill neurons is not a primary factor in modulating the burst period.

Fig. 4.

Blocking I_h with ZD-7288 increased the pyloric burst period and decreased the gastric mill burst period. A: the pyloric burst period increased after 50 and 100 μM ZD-7288 applications. One-way ANOVA followed by Tukey's HSD post hoc test, F(2,17) = 6.933, P = 0.006. B: the gastric mill burst period decreased after 50 and 100 μM ZD-7288 applications. One-way ANOVA followed by Tukey's HSD post hoc test, F(2,15) = 49.546, P < 0.0001. Error bars are represented as SD. The no. of trials (n) is indicated. *P < 0.05 and ****P < 0.00001.

Blocking I_h decreased the intraburst spike frequency of both the pyloric and gastric mill neurons.

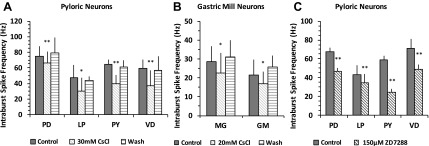
We measured the average intraburst spike frequency within bursts from both the pyloric neurons [PD, LP, pyloric (PY), and VD] and gastric neurons (MG and GM). We did not distinguish between the early PYs and late PYs because the data were pooled from both extracellular and intracellular recordings. After application of 5 mM CsCl, we did not find significant differences in the intraburst spike frequency of either the pyloric neurons or GM neurons [paired Student's t-test, P > 0.05, n = 8 (PD), 5 (LP), 8 (PY), 6 (VD), 6 (MG), and 6 (GM)]. When I_h was blocked with 30 mM CsCl in the pyloric neurons (paired Student's t-test, PD: n = 10, P = 0.006; LP: n = 7, P = 0.033; VD: n = 7, P = 0.003; PY: n = 7, P = 0.007; Fig. 5A) and with 20 mM CsCl in the gastric neurons (paired Student's t-test, MG: n = 9, P = 0.024; GM: n = 10, P = 0.019; Fig. 5B), the intraburst spike frequency of all investigated neurons was significantly decreased. Blocking I_h with 150 μM ZD-7288 also resulted in a decreased pyloric intraburst spike frequency (paired Student's t-test, PD: n = 3, P = 0.005; PY: n = 3, P = 0.001; LP: n = 3, P = 0.001; VD: n = 3, P = 0.024; Fig. 5C). However, lower ZD-7288 concentrations (50 and 100 μM) did not show significant changes (data not shown). Our results suggest that I_h plays a role in maintaining the intraburst spike frequency of both the pyloric and gastric mill neurons. In addition, we found that the oscillation amplitudes of the pyloric follower neurons LP and VD were greatly reduced, which were more severely affected than the PD neurons after blocking I_h [data not shown, n = 6 (PD), 3 (LP), and 2 (VD)], suggesting that I_h is important in maintaining the excitability of pyloric neurons.

Fig. 5.

Blocking I_h decreased the intraburst spike frequency of both pyloric and gastric neurons. A: the intraburst spike frequency of the pyloric neurons decreased after application of 30 mM CsCl, paired Student's t-test, PD: n = 10, P = 0.006; LP: n = 7, P = 0.033; ventricular dilator (VD): n = 7, P = 0.003; pyloric (PY): n = 7, P = 0.007. B: the intraburst spike frequency of the gastric mill neurons decreased after application of 20 mM CsCl, paired Student's t-test, MG: n = 9, P = 0.024; GM: n = 10, P = 0.019. C: the intraburst spike frequency of the pyloric neurons decreased after application of 150 μM ZD-7288, paired Student's t-test, PD: n = 3, P = 0.005; PY: n = 3, P = 0.001; LP: n = 3, P = 0.001; VD: n = 3, P = 0.024. A, B, and C: *P < 0.05 and **P < 0.01. Error bars are represented SD.

Blocking I_h altered the duty cycle of both the pyloric and gastric mill neurons.

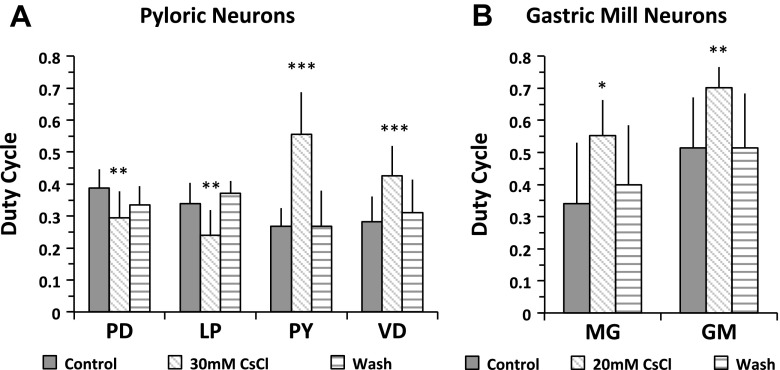
The duty cycle is calculated by dividing the burst duration by burst period. Our results show that the duty cycle of the PD and MG neurons changed after application of 5 mM CsCl, but the rest of the neurons did not show significant changes [paired Student's t-test, P > 0.05, n = 8 (PD), 5 (LP), 8 (PY), 6 (VD), 6 (MG), and 6 (GM)]. When I_h was blocked with 20 mM CsCl, the duty cycle of both the MG and GM neurons increased. The duty cycle of the MG neuron increased to 0.55 ± 0.10 from 0.34 ± 0.19 in the control condition (n = 9, paired Student's t-test, P = 0.015; Fig. 6B); the duty cycle of the GM neurons increased to 0.70 ± 0.06 from 0.52 ± 0.16 in the control condition (n = 10, paired Student's t-test, P = 0.001; Fig. 6B). The gastric mill burst period decreased dramatically after blocking I_h (Fig. 3B), which contributed to the increased duty cycle (Fig. 6B). The duty cycle of the pyloric neurons changed differently when I_h was blocked with 30 mM CsCl: the duty cycle of the PD and LP neurons decreased while that of the PY and VD neurons increased (paired Student's t-test, PD: n = 10, P = 0.003; LP: n = 7, P = 0.004; PY: n = 7, P = 0.0003; VD: n = 7, P = 0.0003; Fig. 6A). In the pyloric network, blocking the I_h-induced PIR increased the burst period (Fig. 3A) (Zhang et al. 2003), which contributed to the decreased duty cycle of the PD neurons. However, the duty cycle of pyloric follower neurons did not always decrease, even when the burst period increased, suggesting that, in addition to the I_h-induced PIR, the duty cycle of the pyloric follower neurons LP, PY, and VD may be regulated through additional pathways, such as synaptic regulation from the PD/AB pacemaker core.

Fig. 6.

Blocking I_h altered the duty cycle of the pyloric and gastric mill neurons. A: the duty cycle of the PD and LP neurons decreased, and the duty cycle of the PY and VD neurons increased, paired Student's t-test, PD: n = 10, P = 0.003; LP: n = 7, P = 0.004; PY: n = 7, P = 0.0003; VD: n = 7, P = 0.0003. B: the duty cycle of the MG and GM neurons increased, paired Student's t-test, MG: n = 9, P = 0.015; GM: n = 10, P = 0.001. A and B: *P < 0.05, **P < 0.01, and ***P < 0.001. Error bars are represented as SD.

Phase-plane diagrams showed decreased d²V/dt² at the onset of the depolarizing phase of PD neurons when I_h was blocked.

Rhythm generation in the stomatogastric neurons relies extensively on nonspiking synaptic transmission and has rate-sensitive components (Raper 1979). A phase-plane diagram is a sensitive method to describe the trajectory of bursting activity independent of time. This is achieved by plotting one time-dependent variable against another time-dependent variable. In practice, the voltage is plotted against dV/dt to describe the spiking and bursting activity (Chagnaud et al. 2011; Felix et al. 2013; Pinsker and Bell 1981). To visualize the changes in the slow oscillation before and after blocking I_h, we applied a lowpass filter on voltage traces to filter out all action potentials, and then plotted voltage against dV/dt and dV/dt against d²V/dt² using filtered slow oscillation traces. The cell membrane acts like a capacitor: when there are no external voltage and current manipulations, dV/dt is proportional to the net membrane ionic current, and d²V/dt² is proportional to the rate at which the current changes. The positive half of the dV/dt trajectory shows the rising slope (depolarizing phase); the negative half of the dV/dt trajectory shows the falling slope (repolarizing phase). When dV/dt and d²V/dt² have opposite signs, it means that the rate of change of current is slowing down, which happens at the end of the depolarizing and repolarizing phases. When they have the same sign, it means that the rate of change of current is speeding up, which happens at the beginning of the depolarizing (dV/dt > 0 and d²V/dt² > 0) and repolarizing (dV/dt < 0 and d²V/dt² < 0) phases. All phase-plane trajectories travel clockwise in these diagrams.

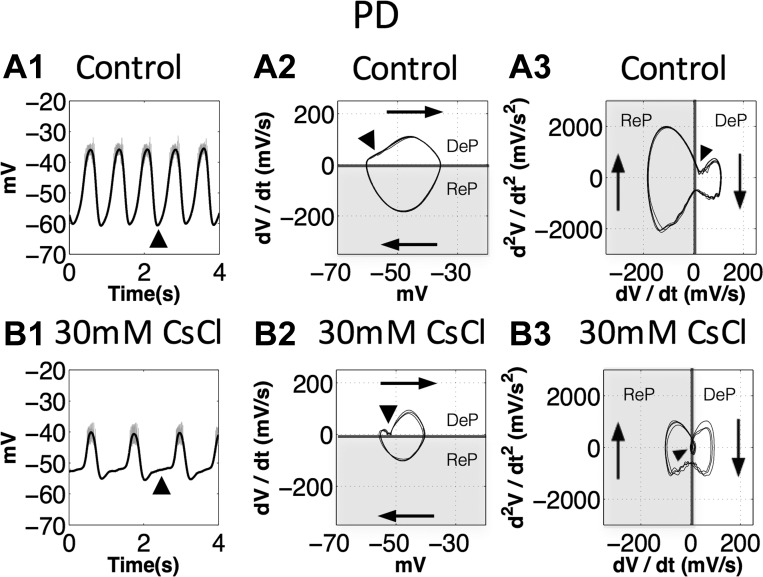
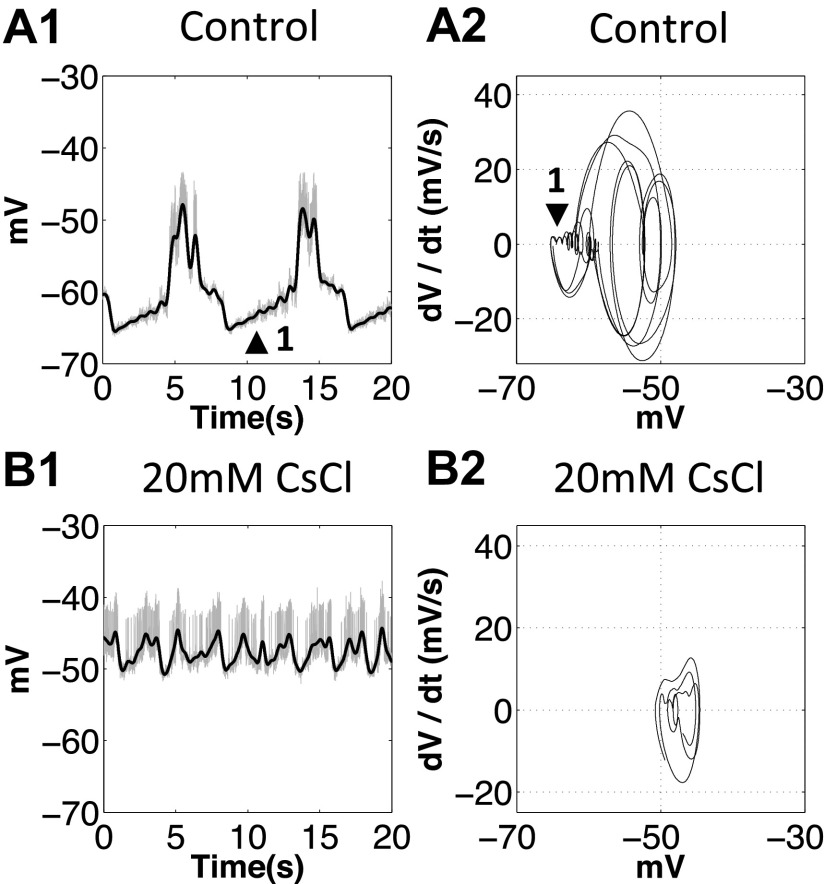
I_h is activated near the resting potential and induces PIR in the PD neurons (Zhang et al. 2003), which corresponds to the onset of the depolarizing phase in the PD neurons. We plotted phase-plane diagrams for the PD neurons in the control condition and after application of 30 mM CsCl (n = 6, all trials showed the same changes to their oscillatory trajectories; Fig. 7). In the control condition, the slow oscillation of the PD neurons is sinusoidal like; the trajectory is close to an elliptical shape in the voltage vs. dV/dt diagram (Fig. 7A2). The dV/dt vs. d²V/dt² diagram clearly shows that the repolarizing phase is steeper (changing faster) than the depolarizing phase (Fig. 7A3), which is difficult to distinguish from the original voltage trace (Fig. 7A1). When I_h was blocked with 30 mM CsCl, phase-plane diagrams showed both dV/dt (proportional to the current) and d²V/dt² (proportional to the rate of change of current) decreased at the onset of the depolarizing phase of the PD neurons (Fig. 7, B1, B2, and B3). More specifically, application of 30 mM CsCl causes the rate of depolarization to decelerate (d²V/dt² < 0) at the onset of depolarization (Fig. 7B3), leading to a much slower depolarization phase (Fig. 7, B2 and B1) than in the control condition (Fig. 7, A2 and A1). Our results suggest that 1) the phase-plane diagram enables us to directly visualize details and detect subtle changes in oscillatory trajectories, and 2) the decrease of d²V/dt² at the onset of the depolarizing phase after application of 30 mM CsCl is consistent with our previous results indicating that I_h was blocked.

Fig. 7.

The second derivative of voltage (d²V/dt²) at the onset of the depolarizing phase of the PD neurons decreased when I_h was blocked. DeP, depolarization phase; ReP, repolarization phase. The shaded sections show the repolarization phases. Arrowheads indicate the onset of the depolarizing phase. A1 and B1: black traces, the slow oscillations; gray traces, the original voltage traces. A2 and B2: voltage vs. the first derivative of voltage (dV/dt) diagrams, traces travel clockwise, dV/dt at the onset of the depolarizing phase decreased after application of 30 mM CsCl. A3 and B3: dV/dt vs. d²V/dt² diagrams, black arrowhead shows d²V/dt² at the onset of the depolarizing phase. Note that, after the onset, application of 30 mM CsCl (B3) causes the rate of depolarization to decelerate (d²V/dt² < 0), leading to a much slower depolarization phase (B2 and B1).

Slow oscillations of the gastric mill neurons became pyloric like when I_h was blocked.

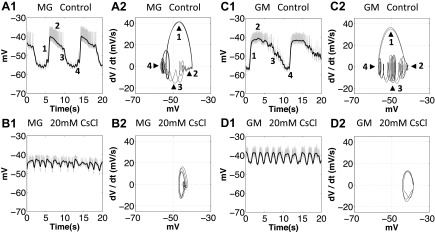
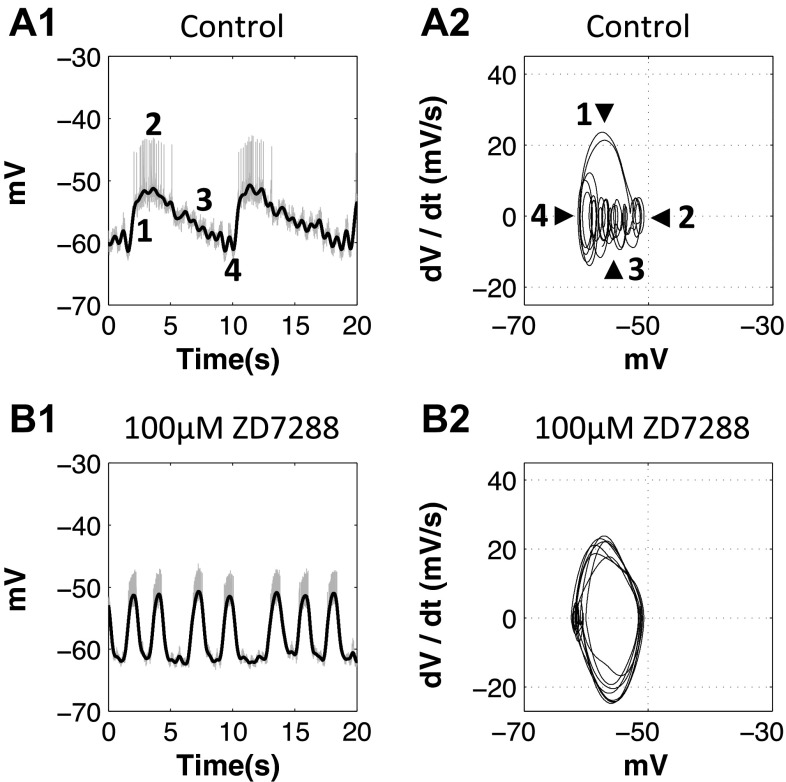
In H. americanus, pyloric modulation is present in the oscillations of all gastric mill motor neurons, which is likely due to inhibition from the pyloric pacemaker AB/PD neuron to the gastric mill pacemaker Int1 neuron and MG neuron (Clemens et al. 1998; Marder and Bucher 2007). We plotted phase-plane diagrams of the MG, GM, and LPG neurons in the control condition and after application of 20 mM CsCl [n = 4 (MG), 6 (GM), and 5 (LPG), all trials showed the same changes to their oscillatory trajectories; Figs. 8 and 9]. In the control condition, the oscillatory trajectory of the MG and GM neurons is often characterized by 1) a steep depolarizing phase (Fig. 8, no. 1), 2) a long plateau phase (Fig. 8, no. 2), and 3) a gradual repolarizing phase and valley where pyloric modulation is superimposed (Fig. 8, nos. 3 and 4). The LPG neurons are inhibited by the MG neuron and are also strongly modulated by the pyloric neurons (Marder and Bucher 2007); the oscillation is often characterized by a long and flat interburst interval due to the inhibition from the MG neuron (Fig. 9, no. 1) and multiple peaks due to the pyloric modulation (Fig. 9).

Fig. 8.

When I_h was blocked with 20 mM CsCl, the oscillatory trajectory of the MG and GM neurons became pyloric like. A1, A2, C1, and C2: 1, depolarizing phase; 2, plateau phase; 3, repolarizing phase; 4, valley. A2, B2, C2, and D2: after application of 20 mM CsCl, the oscillatory trajectory of the MG and GM neurons changed toward sinusoidal like, and the pyloric modulation was diminished. A1, B1, C1, and D1: gray traces show the orignal voltage traces, and black traces show the slow oscillations after filtering.

Fig. 9.

When I_h was blocked with 20 mM CsCl, the oscillatory trajectory of the LPG neuron changed. A1 and A2: 1, voltage valleys. After application of 20 mM CsCl, the pyloric modulation was diminished.

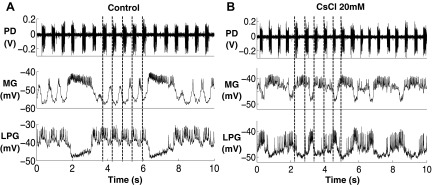
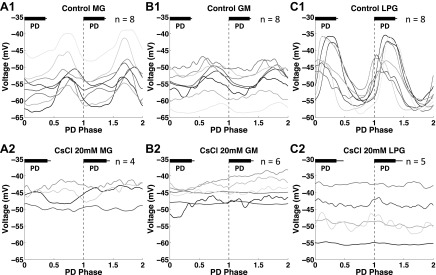
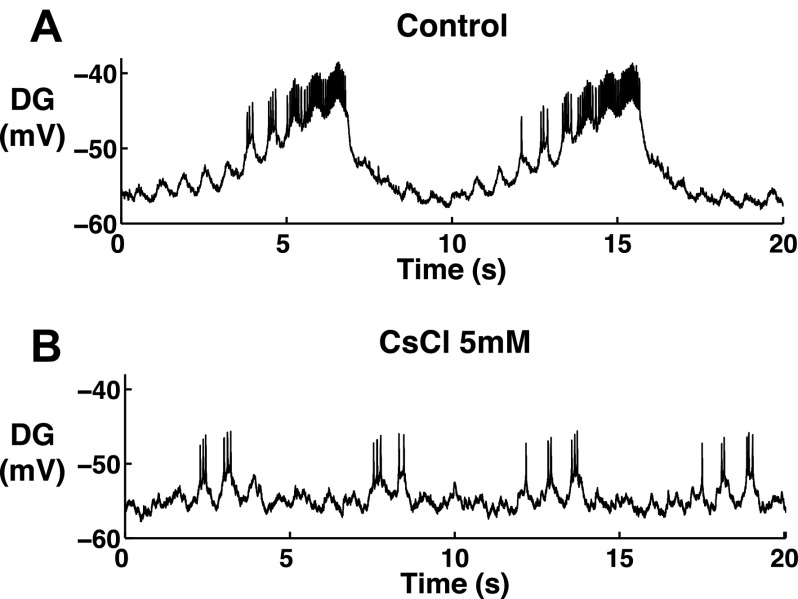
After application of 20 mM CsCl, we found that the oscillatory trajectory of the MG and GM neurons lost their oscillatory characteristics that were shown in controls. First, the oscillation became faster, and second, the pyloric modulation during interburst intervals was diminished (Fig. 10, n = 4, Fig. 11). A purely sinusoidal oscillation will trace a perfect ellipse in voltage vs. dV/dt, and the observed gastric mill trajectories approach this behavior (Fig. 8, B2 and D2). Because the oscillatory trajectory of the pyloric neurons in controls is sinusoidal like (Fig. 7, A1 and A2), our results suggest that the altered MG and GM trajectories after blocking I_h change toward the sinusoidal-like oscillatory trajectories of the pyloric neurons. The LPG neuron experienced similar changes to the MG and GM neurons after application of 20 mM CsCl while the oscillation became more irregular than the MG and GM neurons (Figs. 8 and 9). We further quantified the PD modulation of the MG, GM, and LPG neurons in both the control and 20 mM CsCl conditions (Fig. 11). We used the extracellularly recorded PD neuron burst starts in selected cycles. For the MG and GM neurons, the PD modulation is strong during interburst intervals. Thus only pyloric burst cycles during the gastric interburst intervals were selected. For the LPG neurons, the PD modulation is strong during the LPG bursts. Thus only the pyloric burst cycles during the LPG bursts were selected. In each plot, two consecutive PD burst cycles were plotted in each graph to show the modulatory effect on the gastric mill neurons. To illuminate the low-frequency components, the intracellular recordings were low-pass filtered above 10 Hz to eliminate the action potential transients. The filtered voltage data were then normalized by dividing each PD burst cycle into 400 time bins over two consecutive PD burst cycles. To minimize the variability, we averaged three sections (2 PD cycles for each section) in each trial to represent the average change in membrane potential of the gastric mill neurons in relation to the PD burst phase. Our results showed that, in the control condition, the MG and GM neurons (during interburst intervals) were consistently hyperpolarized during PD bursts [n = 8 (MG) and 8 (GM); Fig. 11, A1 and B1], whereas the LPG neurons (during bursts) were consistently depolarized during PD bursts [n = 8 (LPG); Fig. 11C1]. Our results in the control condition are consistent with the results from a previous study on H. americanus (Bucher et al. 2006). After application of 20 mM CsCl, the voltage traces of the MG, GM, and LPG neurons no longer consistently oscillated with the PD bursts [n = 4 (MG), 6 (GM), and 5 (LPG); Fig. 11, A2, B2, and C2]. This suggests that the PD modulations of the MG, GM, and LPG neuron were all diminished.

Fig. 10.

Pyloric modulation of the MG and LPG neurons diminished after application of 20 mM CsCl. The dark gray lines indicate the onset of PD bursts. A: the PD modulation was present in the MG and LPG oscillations in the control condition. B: the PD modulation was no longer present in the MG and LPG oscillations after application of 20 mM CsCl, n = 4.

Fig. 11.

PD modulation on the MG, GM, and LPG neurons diminished after I_h was blocked with 20 mM CsCl. Black bars in each graph represent the PD burst in each burst cycle. A1: in the control condition, the MG interburst membrane potential was hyperpolarized during PD bursts and depolarized during PD interburst intervals. A2: after application of 20 mM CsCl, the MG interburst membrane potential no longer oscillated with the PD phase. B1: in the control condition, the GM interburst membrane potential was hyperpolarized during PD bursts and depolarized during PD interburst intervals. B2: after application of 20 mM CsCl, the GM interburst membrane potential no longer oscillated with the PD phase. C1: in the control condition, the LPG burst membrane potential was depolarized during PD bursts and hyperpolarized during PD interburst intervals. B2: after application of 20 mM CsCl, the LPG burst membrane potential no longer oscillated with the PD phase.

The results of altered oscillatory trajectories of GM neurons were confirmed by blocking I_h with 100 μM ZD-7288: the oscillatory trajectory of the GM neurons became elliptical in the voltage vs. dV/dt diagram, suggesting it changed toward the typical sinusoidal-like pyloric oscillations (n = 3, all 3 trials showed the same changes to their oscillations; Fig. 12B2). The strong pyloric modulation during interburst interval diminished (Fig. 12B1). Taken together, our results show that, after blocking I_h, the oscillations of the gastric neurons became faster and had diminished pyloric modulation. These changes lead the GM oscillations changed toward typical sinusoidal-like pyloric oscillations. Therefore, we conclude that I_h plays a major role in differentiating the oscillations of the gastric neurons from those of the pyloric neurons.

Fig. 12.

When I_h was blocked with 100 μM ZD-7288, the oscillatory trajectory of the GM neurons became pyloriclike. A1 and A2: 1, depolarizing phase; 2, plateau phase; 3, repolarizing phase; 4, valley. B1 and B2: after application of 100 μM ZD-7288, the oscillatory trajectory neurons changed toward sinusoidal like, and the pyloric modulation was diminished.

DISCUSSION

In this study, we demonstrate that I_h in the STG differentially regulates the burst period and oscillatory dynamics of the pyloric and gastric neurons. I_h also plays a role in maintaining the excitability and regulating the duty cycle of both the pyloric and gastric neurons.

Specificity of the I_h blockade.

CsCl can block several potassium currents in addition to I_h, for example, Cs⁺ can weakly block M current in vertebrate systems (Coggan et al. 1994; Rudy 1988). M current is an outward potassium current that is more likely to open during the depolarization phase than at the resting potential (Brown and Adams 1980). However, there is no direct evidence to confirm the existence of M current in crustaceans. Even if M current does exist in the STG neurons, blocking it would likely result in increased depolarizing amplitude, which is inconsistent with our results. Cs⁺ can also block inward-rectifying potassium current (I_Kir) (Wischmeyer and Karschin 1997) in mammalian cells. Similar to I_h, I_Kir also contributes to maintaining the resting potential; however, no study has shown the existence of or has characterized the kinetics of I_Kir in the STG neurons. In our experiments, even if we partially block other potassium currents with CsCl, such as M current and I_Kir, the effects would be physiologically insignificant because our results from application of ZD-7288 are consistent with the results from application of CsCl. ZD-7288 does not block M current, I_Kir, or leak current. Therefore, we argue that the effects described in this study are primarily attributable to the blocking of I_h.

I_h-induced PIR is not the sole factor regulating the duty cycle of the pyloric follower neurons.

Our results show that the duty cycle of the PD and LP neurons decreases while that of the PY and VD neurons increases, which suggests that I_h-induced PIR is not the sole factor regulating the duty cycle of the pyloric follower neurons. One explanation is that the duty cycle of the pyloric follower neurons is determined by different synaptic strengths between them; inhibition from PD to LP, PD to PY, LP to VD, and PY to LP is strong, whereas inhibition from LP to PY is weak (Miller and Selverston 1982). When the duty cycle of the PD neurons decreases, the duration of inhibition from PD to LP and PY decreases. At the same time, inhibition from PY to LP is stronger than inhibition from LP to PY. Altogether, this can lead to the increased PY duty cycle and decreased LP duty cycle. VD receives strong inhibition from LP; once the duty cycle of LP decreases, the duty cycle of VD increases.

Role of I_h in regulating the gastric mill burst period.

In contrast to the pyloric neurons, blocking I_h decreases the burst period of the gastric mill neurons, suggesting that I_h regulates the gastric mill period differently from the manner in which it regulates the pyloric period. Because we know that the function of PIR is to facilitate the depolarization of the next burst, blocking PIR would prolong the burst period. Therefore, our results suggest that I_h-induced PIR is not the sole or dominant mechanism in regulating the gastric mill burst period. Instead, I_h expressed in the STG regulates the gastric mill burst period through additional pathways. Although mammalian studies have shown that blocking I_h in the presynaptic terminals of GABAergic interneurons directly reduces presynaptic GABA release (Aponte et al. 2006; Lupica et al. 2001; Southan et al. 2000), I_h is not found in presynaptic terminals in the STG (Goeritz et al. 2011). However, it is highly expressed in fine synaptic neuropil throughout the STG in P. interruptus (Goeritz et al. 2011). Thus, blocking I_h in the STG is unlikely to directly affect the presynaptic terminals of the projection neurons. However, blocking I_h in the STG could indirectly affect projection neurons by affecting the STG neurons that send axons to them. Although there is a lack of studies that show how the STG neurons send synaptic inputs to projection neurons in Homarus (Combes et al. 1999), this type of interaction is shown in both P. interruptus (Russell 1976; Selverston et al. 1976) and C. borealis (Coleman et al. 1995).

In both H. gammarus and P. interruptus, the MG/LG neurons reciprocally inhibit the Int1 neuron and DG neuron (Clemens et al. 1998; Selverston et al. 2009; Thuma and Hooper 2002). Previous studies in P. interruptus have shown that the burst period of the Int1 neuron decreases to <5 s when its membrane potential is hyperpolarized after injecting up to 1 nA hyperpolarization current (Selverston et al. 2009). Thus it is possible that I_h regulates the gastric burst period by regulating the membrane potential of the Int1 neuron. Our preliminary observations show that the burst period of the DG neuron decreases to ∼6 s while its burst duration and intraburst spike frequency are greatly reduced when partially blocking I_h with 5 mM CsCl (n = 2; Fig. 13), indicating an increased inhibition from the LG/MG neurons. In addition, the MG neuron has an increased duty cycle when blocking I_h, suggesting prolonged inhibition from the MG to Int1. In P. interruptus, the two excitatory neurons in the CoGs fire spontaneously in a tonic firing fashion and can burst at the gastric rhythm frequency when receiving inhibition from the pacemaker Int1 neuron. The excitatory neurons also excite the GM, LPG, MG, and LG neurons (Russell 1976). When the Int1 neuron is shut off, both the E neurons and gastric motor neurons fire tonically (Russell 1976). Our results show that, when blocking I_h with 25–30 mM CsCl, the GM, MG, and LPG neurons change to tonic firing, which indicates that the Int1 neuron is strongly inhibited and likely shut off. Therefore, if similar connections exist in H. americanus, our results support the explanation that the Int1 neuron is inhibited and bursts at a decreased period (Selverston et al. 2009) when blocking I_h with between 5 and 20 mM CsCl, until it gets shut off when blocking I_h with 25–30 mM CsCl. The rest of the gastric network follows the burst period of the Int1 neuron.

Fig. 13.

When blocking I_h with 5 mM CsCl, the burst period, burst duration, and intraburst spike frequency of the dorsal gastric (DG) neuron decreased, n = 2.

In C. borealis, the modulatory commissural neuron 1 (MCN1) is known to activate and modulate the gastric rhythm through the Int1, LG, and DG neurons using different neurotransmitters (Coleman et al. 1995; Stein et al. 2007; Wood et al. 2000). The MCN1 terminals in the STG are electrically coupled with the LG neuron (Coleman et al. 1995). The gastric burst period is negatively correlated with the MCN1 firing frequency (Bartos et al. 1999); thus, the change in the LG activity after blocking I_h would affect the activity of the MCN1 terminals in the STG. However, it is unknown if similar connections also exist in H. americanus. If so, it is possible to indirectly increase the firing frequency of the MCN1 terminals in the STG by blocking I_h. This effect would be mediated through the electrical coupling with the LG neuron and lead to a decreased gastric burst period.

Other than these explanations, one study has shown that blocking I_h decreases the burst period of the inspiratory neurons in mice, but the mechanism remains unclear (Thoby-Brisson et al. 2000). It could also be possible that the regulatory function of I_h in the gastric network shares some similarities to its function in the inspiratory neurons. Studies in H. gammarus have shown that P (pyloric modulatory neurons that originate in the commissural ganglion and modulated in pylorc time by the AB neuron) and commissural pyloric neurons in the CoGs receive synaptic inputs from the AB neuron and excite the pyloric neurons (Nagy et al. 1994). However, it is unknown if these neurons also modulate gastric neurons. Studies have shown that the commissural gastric and gastric inhibitor neurons directly modulate certain gastric neurons in H. gammarus (Combes et al. 1999); however, it is unknown if there are feedback pathways from the STG neurons. More studies on the connectivity between STG and projection neurons in Homarus are necessary for us to understand the pathways for regulating the gastric network.

Role of I_h in regulating the pyloric modulation of the oscillations of gastric mill neurons.

The oscillations of the gastric mill neurons are subject to modulation from the pyloric neurons. Our results show that, when I_h is blocked with 20–30 mM CsCl or 100–150 μM ZD-7288, the superimposed pyloric modulation of the gastric mill oscillations is diminished. In both lobsters and crabs, the pyloric pacemaker AB/PD neurons inhibit the MG/LG neurons (Clemens et al. 1998; Thuma and Hooper 2002; Weimann and Marder 1994). We assume that the AB neuron remains oscillating in phase with the PD neurons when I_h is blocked and consider them together as a functional group because they are pacemakers and are electrically coupled to each other. Our results show that the activity of the PD neurons is weakened after I_h is blocked. The weakened PD activity can result in weaker modulatory effects on the MG/LG neurons. In addition, the PD/AB pacemaker kernel also excites the gastric pacemaker Int1 neuron (Mulloney 1977; Selverston 2010). The weakened PD activity after blocking I_h can lead to the reduction of the pyloric excitation of the Int1 neuron, thereby reducing the pyloric modulation of the gastric rhythm.

Influence of the gastric mill neurons on the pyloric burst period.

Studies in H. gammarus show that the gastric rhythm modulates the pyloric rhythm through the inhibition from the MG neuron to the PD neurons (Clemens et al. 1998). Our results show that, after application of 30 mM CsCl, the PD burst period decreases, whereas the MG neuron changes to the silent state. Thus the inhibition from the MG to PD neurons is not present, and the increased PD burst period can be explained by the effect of blocking I_h-induced PIR. Interestingly, the PD burst period does not show significant changes after application of 5–20 mM CsCl when the gastric burst period decreases significantly. Studies have shown that the pyloric neurons burst faster when the gastric neurons also burst faster (Bartos et al. 1999). This suggests that the fast gastric rhythm possibly influences the PD to burst faster when blocking I_h with 5–20 mM CsCl, which dampens the effect of increasing burst period from reducing PIR.

I_h regulates the gastric mill and pyloric CPGs through different pathways.

Our results show that the change in the dynamics of the pyloric neurons is different from that of the gastric motor neurons when blocking I_h, which strongly suggests that I_h regulates the pyloric and gastric mill CPGs through different pathways. For the pyloric CPG, our results suggest that I_h regulates the neuronal dynamics by affecting PIR, especially the PIR in the PD neurons. In addition, the pyloric rhythm receives some influence from the gastric mill network. For the gastric mill CPG, our results suggest that I_h regulates the dynamics at both the network and cellular levels, including modulating the activity of the MG/LG and Int1 neurons in the gastric mill network, as well as the activity of the AB/PD neurons in the pyloric network.

Previous studies have shown that the dynamics of the STG neurons are shaped by a number of ionic currents (reviewed by Harris-Warrick 2002), for example: 1) the transient outward potassium current I_A delays the onset of action potentials and increases the pyloric period (Kloppenburg et al. 1999), 2) the calcium-activated nonselective cation current I_CAN maintains the plateau potential in the gastric mill neurons (Zhang and Harris-Warrick 1995), and 3) the calcium-activated potassium current I_K(Ca) is essential for the plateau potential termination (Kiehn and Harris-Warrick 1992). Previous work on spiny lobsters show that I_h facilitates the onset of the burst and decreases the burst period in the pyloric neurons (Kiehn and Harris-Warrick 1992; Zhang et al. 2003). Our work expands our understanding of the functions of I_h from the pyloric network to the gastric mill network. We show that I_h plays an important role in shaping gastric mill oscillations. This is an addition to the previous knowledge that calcium-related currents regulate the gastric mill oscillations mentioned above. Moreover, we show that I_h differentially regulates the pyloric and gastric mill rhythms, which opens the door for future studies on dissecting the mechanisms through which I_h differentially regulates the two rhythms.

GRANTS

This work was funded by Schlumberger/Doll.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the authors.

AUTHOR CONTRIBUTIONS

L.Z. performed experiments; L.Z. analyzed data; L.Z., A.I.S., and J.A. interpreted results of experiments; L.Z. prepared figures; L.Z. drafted manuscript; L.Z., A.I.S., and J.A. edited and revised manuscript; L.Z., A.I.S., and J.A. approved final version of manuscript; A.I.S. and J.A. conception and design of research.