Biomechanics of cervical tooth region and noncarious cervical lesions of different morphology; three-dimensional finite element analysis

Abstract

Objective:

The present study aims to investigate the influence of presence and shape of cervical lesions on biomechanical behavior of mandibular first premolar, subjected to two types of occlusal loading using three-dimensional (3D) finite element method (FEM).

Materials and Methods:

3D models of the mandibular premolar are created from a micro computed tomography X-ray image: model of sound mandibular premolar, model with the wedge-shaped cervical lesion (V lesion), and model with saucer-shaped cervical lesion (U lesion). By FEM, straining of the tooth tissues under functional and nonfunctional occlusal loading of 200 (N) is analyzed. For the analysis, the following software was used: CTAn program 1.10 and ANSYS Workbench (version 14.0). The results are presented in von Mises stress.

Results:

Values of calculated stress in all tooth structures are higher under nonfunctional occlusal loading, while the functional loading is resulted in homogeneous stress distribution. Nonfunctional load in the cervical area of sound tooth model as well as in the sub-superficial layer of the enamel resulted with a significant stress (over 50 [MPa]). The highest stress concentration on models with lesions is noticed on the apex of the V-shaped lesion, while stress in saucer U lesion is significantly lower and distributed over wider area.

Conclusion:

The type of the occlusal teeth loading has the biggest influence on cervical stress intensity. Geometric shape of the existing lesion is very important in the distribution of internal stress. Compared to the U-shaped lesions, V-shaped lesions show significantly higher stress concentrations under load. Exposure to stress would lead to its progression.

INTRODUCTION

Occlusal biomechanical forces cause microstructural loss of tissue in the cervical tooth region. This type of tissue loss is marked as abfraction by Grippo.[1] Tensile and compressive forces of cervical tooth area lead to bonds interrupting among hydroxylapatite crystals of enamel and dentin, allowing penetration of small molecules, which are preventing re-establishment of bonds.[2] Abfraction lesions are characterized by a wedge-shaped defect of tissue, bordered with sharp internal and external edges [Figure 1].

Figure 1.

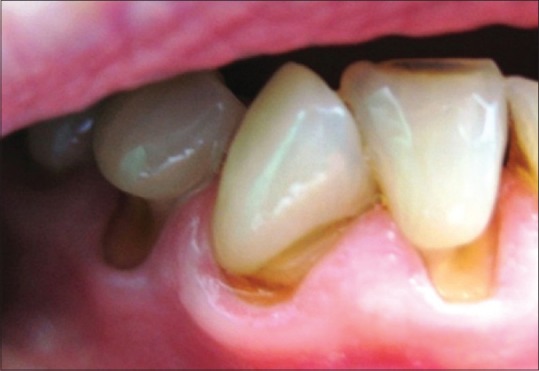
Abfraction lesions [5]

Each tooth tissue has different mechanical properties and morphology, which makes analysis of distribution process and stress concentration alongside these structures very complex.[3]

Yettram et al. describe stress distribution alongside the enamel “as a cap around the tooth,” which is finally concentrated at the cement-enamel junction.[4]

Incidence of noncarious cervical lesion increases with patient's age.[5] The highest prevalence of noncarious cervical lesions is identified on mandibular first premolar.[5,6,7]

The aim of this study was to explore stress distribution inside tooth structures and abfraction lesions with different morphologies under various loading conditions.

MATERIALS AND METHODS

Mandibular first premolar was scanned by micro computed tomography (µCT) scanner (SkyScan 1076 Kontich, Belgium)[8] and the obtained images were reconstructed into transaxial sections using Nrecon and CTAn program software (SkyScan). Approximately, 570 horizontal sections (resolution up to 758 × 758 [px]) were chosen for the reconstruction of three-dimensional (3D) models [Figure 2].

Figure 2.

Micro computed tomography images of the mandibular first premolar[5]

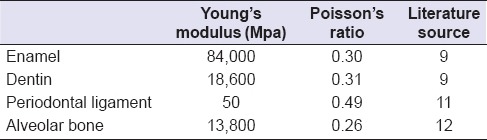
A volumetric 3D CAD tooth model was created using program packages MATLAB (MathWorks, Inc., Natick, USA) and Creo Parametric 1.0 CAD software. The resulting model consisted of enamel, dentin, pulp, periodontal ligament, and reconstructed segment of alveolar bone [Figure 3]. In comparison to adjacent structures, Young's modulus of pulp is negligibly small, therefore pulp was modeled as an empty space. Periodontal ligament was created as a membrane of 0.3 mm thin.[9]

Figure 3.

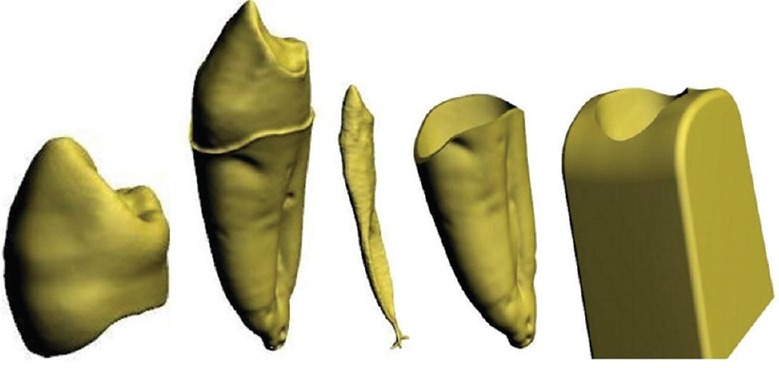
Three-dimensional volumetric tooth tissue models reconstructed from micro computed tomography images; enamel, dentin, pulp, periodontal ligament, and reconstructed segment of the alveolar bone[6]

To compare the stress distribution in lesions with different geometry, two types of cervical lesions were modeled; the wedge-shaped lesion – V lesion [Figure 4] and saucer-shaped lesion – U lesion [Figure 5].[10]

Figure 4.

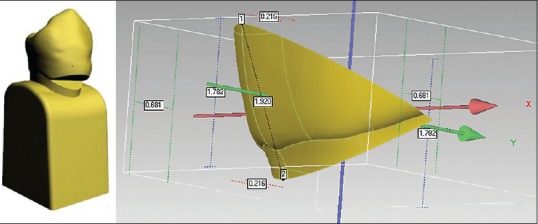
V-shaped lesion, appearance on the three-dimensional tooth model, and lesion dimensions

Figure 5.

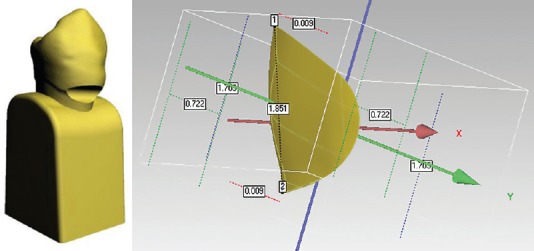
U-shaped lesion, appearance on the three-dimensional tooth model, and lesion dimensions

The models were exported to finite element analysis software ANSYS Workbench (14.0) and a finite element mesh of the models is made [Figure 6]. Properties of the tooth tissues used in the research are given in Table 1.[9,11,12]

Figure 6.

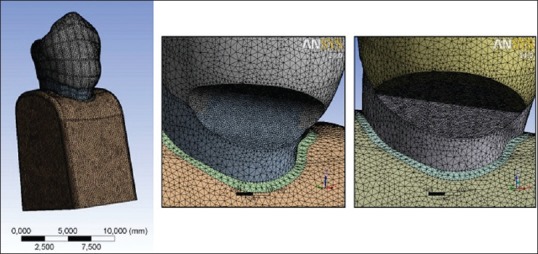
Three-dimensional meshed models

Table 1.

Tooth tissue properties

Since the intensity of masticatory force and teeth contact surface is extremely variable, two occlusal contact types were chosen to show the effects of a favorable and unfavorable situation for the tooth and surrounding tissues.

For the purpose of presenting the action of functional occlusal load, occlusal contacts are simulated on regions that imitate teeth contacts in central occlusion [Figure 7a]. In addition to this, nonfunctional load is simulated on the external buccal cusp side, at 40° angle [Figure 7b].[12,13] Values of the mentioned load were 200 (N).[14,15,16]

Figure 7.

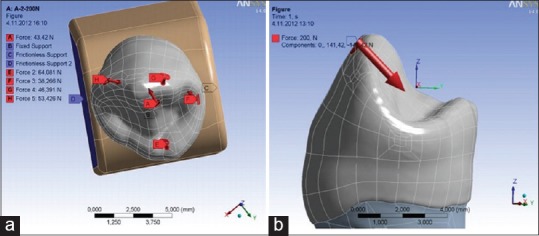
Type of simulated occlusal contacts in (a) central occlusion (b) laterotrusion[5]

Following steps were processing (finite elements method [FEM] calculation of tooth tissue straining) and postprocessing (result analysis by stress distribution criteria). To record the complex stress measured in (MPa), von Mises stress (VMS) - the hypothesis of the highest distortion energy was used. VMS determines the total of resulting stress in every point of the observed object – tooth element.

RESULTS

Results are presented in the form of images with the stress distribution presented as a color scale and numeric values obtained in (MPa).

The highest stress values were seen in the crown and the cervical tooth region. Calculated stress values were higher with eccentric occlusal forces in all tooth tissues.

The calculated VMS on the sound tooth in the cervical region under functional load measured up to 12 (MPa) [Figure 8a], while the stresses in the same area under eccentric load are significantly higher and were over 50 (MPa) [Figure 8b].

Figure 8.

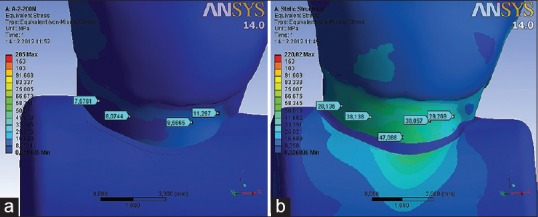
Distribution of von Mises stress at the cervical part of the tooth under (a) functional and (b) nonfunctional load of 200 (N)[5]

On the sagittal section of the cervical tooth area, the highest stress was observed along the dentino-enamel junction (DEJ). The values of calculated VMS in the region of sub-superficial enamel under nonfunctional load of 200 (N) range up to 60 (MPa), which is 5 times higher in comparison to the values measured in superficial enamel [Figure 9].

Figure 9.

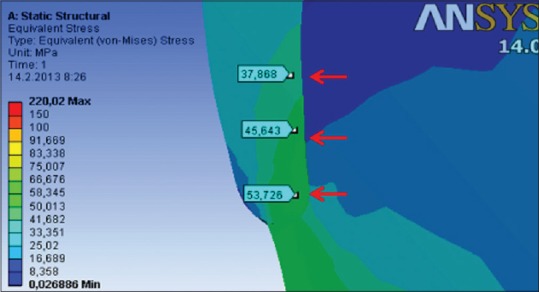
Distribution of von Mises stress on the sagittal section of the superficial and sub-superficial enamel of sound tooth model under nonfunctional load of 200 (N)[5]

The sound model showed higher stress distribution pattern for both loading types compared with models with lesions. On the model with V-shaped lesions, discontinuity of the enamel and dentin resulted in a strain increase, as well as stress concentration around the apex/bottom of the lesion. Values of the VMS on the apex/bottom of the wedge lesion are extremely high and measured up to 266.13 (MPa) under nonfunctional load [Figures 10b and 11a] and 93.24 (MPa) under functional load [Figures 10a].

Figure 10.

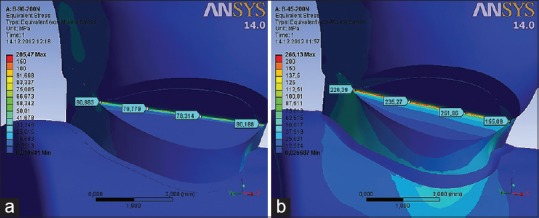
Distribution of von Mises stress in the V-shaped lesions under (a) functional and (b) nonfunctional load of 200 (N)

Figure 11.

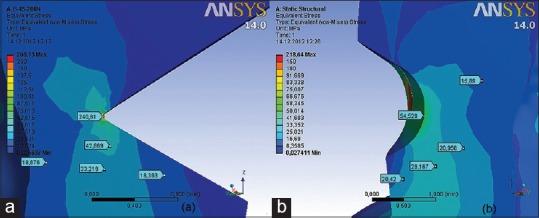
Distribution of von Mises stress in the U-shaped lesions under (a) functional and (b) nonfunctional load of 200 (N)

Values of VMS on the bottom of U-shaped lesion were up to 16 (MPa) under functional load [Figure 12a] and 55 (MPa) under nonfunctional load [Figures 11b and 12b]. Higher values of strain up to 55 MPa under functional load and 180 (MPa) under nonfunctional load were measured with the saucer lesion only on the mesial and distal side of the lesion, where the enamel inserts with its sharp edges.

Figure 12.

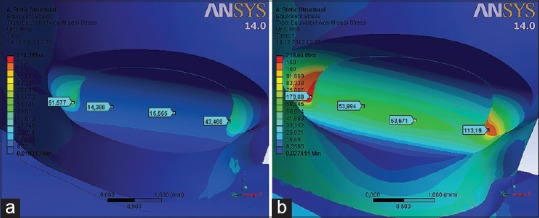
Distribution of von Mises stress on the sagittal section of (a) V-shaped lesion and (b) U-shaped lesion and surrounding tooth tissues under nonfunctional load of 200 (N)

DISCUSSION

The biomechanics of complex systems, such as a tooth, requires application of complex research methods. Structure analyses of such systems must fulfill high criteria of accuracy and preciseness. The FEM used in this research is an adequate method for the biomechanical behavior prediction of the tooth under load.[17] The models in this research are complex and accurate, not only because of the 3D structure, but also due to the large number of elements and nodes of which they are composed, as well as the fact that they are gained from a µCT image that allowed a reproduction of the smallest morphological details [Figure 2].

The research tested the action of two types of occlusal loading on a model of sound tooth, model with wedge-shaped cervical lesion, i.e., V lesion [Figure 4] and model with saucer-shaped cervical lesion, i.e., U lesion [Figure 5].

This research showed that under eccentric forces, stress values on tested models were higher in all tooth tissues.

Occlusal stress is commonly reflected in the cervical tooth region, which is especially noticeable with the action of nonfunctional occlusal forces. Calculated VMS in the cervical tooth area under axial load measured up to 12 (MPa), [Figure 8a], whereas the stresses in the same part of the tooth under paraxial load are significantly higher and measured up to 60 (MPa) [Figure 8b]. Studies of Borcic et al.,[14] Yaman et al.,[18] and Rees and Hammadeh[19] reported that tensile stress values on the buccal aspect of the cervical region range from 60 to 90 (MPa).

Rees and Hammadeh analyzed the mechanisms of forming abfraction lesions and presented a theory of a possible undermining of the enamel due to stress concentration on the DEJ.[19] Results of our research confirmed the aforementioned theory, since the values of calculated stress in the cervical part of the tooth were 5 times higher in the sub-superficial compared to the superficial region of the enamel [Figure 9]. This indicates that breaking of the bonds between enamel prisms might occur in these layers exactly. Afterward, cervical lesion can progress through erosion and abrasion.

The results of this study demonstrated that the geometric shape of the lesions is very important in the distribution of internal stress in the tooth. On the model with V-shaped lesion, the enamel and dentin discontinuity resulted in strain increase, as well as in stress concentration around the apex of the lesion. Stress values on the apex/bottom of the V lesion are extremely high and measures up to 266.13 (MPa) under paraxial load [Figures 10b and 12a] and 93.24 [MPa] under axial load [Figures 10a and 12b]. The results of analyses showed that the bottom of cervical lesions concentrated stress under load, which clearly indicates that their further exposure to stress would lead to its deepening.

Stress values on the bottom of the U-shaped lesion under nonfunctional load measures up to 55 (MPa) [Figures 11b and 12b], which is 5 times lower comparing with V-shaped lesions. Higher values of strain, up to 180 (MPa), were calculated with the saucer lesion only in the areas where the enamel inserts with its sharp edges.

According to these results, it can be concluded that the geometric shape of the lesion plays an important role in the distribution of the internal stress of the tooth. Lesions with emphasized geometric discontinuity (V-shaped lesions) result in high strain concentration, whereas lesions with rounded contours (U-shaped lesions) concentrate less strain.

The results obtained in this study can be important in clinical practice because they open a possibility of considering lesions remodeling with extremely sharp geometry into rounded lesions during their restoration. A use of timely therapy could prevent further tooth tissue loss.

CONCLUSIONS

1. The type of the teeth loading has the biggest influence on stress intensity. Eccentric occlusal forces in all tooth tissues caused higher stress values on the tested models

2. Occurrence of significant stress in the cervical part of the sound tooth model is caused by eccentric occlusal load. Stress is almost 5 times higher in the sub-superficial layer of the cervical enamel in comparison to the superficial enamel

3. Geometric shape of the lesion is very important in the distribution of internal stress in hard dental tissues. Compared to the U-shaped lesions, V-shaped lesions show significantly higher stress concentrations under load. Therefore, it could be concluded that their further exposure to stress would lead to its progression

4. This research provides a good insight into stress distribution in one moment of tooth loading. Limitation of this study is that the acting force is assumed as a static, not as a dynamic process, what it is in reality.

Financial support and sponsorship

Nil.

Conflicts of interest

There are no conflicts of interest.