Skip to main content
PMC home page
Journal of Visualized Experiments : JoVE logoLink to Journal of Visualized Experiments : JoVE
. 2016 May 9;(111):53588. doi: 10.3791/53588

A Microfluidic Model of Biomimetically Breathing Pulmonary Acinar Airways

Rami Fishler 1, Josué Sznitman 1
PMCID: PMC4942038  PMID: 27214269

Abstract

Quantifying respiratory flow characteristics in the pulmonary acinar depths and how they influence inhaled aerosol transport is critical towards optimizing drug inhalation techniques as well as predicting deposition patterns of potentially toxic airborne particles in the pulmonary alveoli. Here, soft-lithography techniques are used to fabricate complex acinar-like airway structures at the truthful anatomical length-scales that reproduce physiological acinar flow phenomena in an optically accessible system. The microfluidic device features 5 generations of bifurcating alveolated ducts with periodically expanding and contracting walls. Wall actuation is achieved by altering the pressure inside water-filled chambers surrounding the thin PDMS acinar channel walls both from the sides and the top of the device. In contrast to common multilayer microfluidic devices, where the stacking of several PDMS molds is required, a simple method is presented to fabricate the top chamber by embedding the barrel section of a syringe into the PDMS mold. This novel microfluidic setup delivers physiological breathing motions which in turn give rise to characteristic acinar air-flows. In the current study, micro particle image velocimetry (µPIV) with liquid suspended particles was used to quantify such air flows based on hydrodynamic similarity matching. The good agreement between µPIV results and expected acinar flow phenomena suggest that the microfluidic platform may serve in the near future as an attractive in vitro tool to investigate directly airborne representative particle transport and deposition in the acinar regions of the lungs.

Keywords: Bioengineering, Issue 111, Microfluidics, lungs, breathing, pulmonary acinus, respiratory flows, flow visualization, particle dynamics.

Download video file (29.9MB, mp4)
Open in a new tab

Introduction

A detailed quantification of respiratory flow dynamics in the distal, alveolated regions of the lungs is paramount towards understanding airflow mixing in the pulmonary acinus and predicting the fate of inhaled aerosols in the deepest airways1–3. This latter aspect is of particular concern when addressing on the one hand the hazards of inhaled pollutant particles or conversely in seeking novel strategies for improved and targeted drug delivery of inhaled therapeutics to localized lung sites4, 5 as well as for systemic delivery.

To date, respiratory flows in the deep pulmonary acinar regions have been typically investigated in silico using computational fluid dynamics (CFD) or alternatively in vitro with scaled-up experimental models following hydrodynamic similarity matching. In the past few decades, CFD methods have been increasingly applied to study acinar flow phenomena, from single alveolar models6, 7 and alveolated ducts8–12 to more elaborate in silico models that capture anatomically-realistic acinar tree structures with multiple generations of alveolated ducts and up to several hundreds of individual alveoli13–15.

Together, numerical efforts have been pivotal in shedding light on the role and influence of wall motion during breathing movements on ensuing acinar airflow patterns. In the absence of breathing motion, static alveoli feature recirculating flows within their cavities that exhibit no convective exchange of air between the acinar duct and the alveolus6, 7; in other words, alveolar flows would be entirely isolated from flows within the acinar trees and exchange of air would result uniquely from diffusive mechanisms. With the existence of cyclic expansions of the alveolar domain, however, alveolar flow topologies are drastically modified and the resulting flow patterns inside alveoli are intimately tied to the location of an alveolus along the acinar tree (e.g., proximal vs. distal generations).

In particular, it has been hypothesized in simulations that alveolar flow patterns are strongly influenced by the ratio of alveolar to ductal flow rates such that proximal generations of the pulmonary acinar tree, where this ratio is relatively large following mass conservation across a tree structure, feature complex recirculating flows inside the alveolar cavities with irreversible fluid pathlines. With each deeper acinar generation, the ratio of alveolar to ductal flow rates gradually decreases such that distal acinar generations exhibit more radial-like streamlines that are reminiscent of simple inflations and deflations of a balloon. With advances in modern imaging modalities, lung imaging data16, 17 of rodents, including rat and mouse, have given rise to some of the first CFD simulations of anatomically-reconstructed acinar flows in reconstructed alveoli. Despite such promising progress, these recent studies are still limited to addressing airflow phenomena in terminal alveolar sacs only18, 19 or a few alveoli surrounding a single duct20. As a result, state-of-the-art investigations of respiratory flow phenomena in the acinus remain dominated by studies focusing on generic anatomically-inspired geometries of the acinar environment2.

On the experimental side, various setups featuring an airway with one or several alveoli have been developed over the years21–24. Yet, there exists no experimental models of bifurcating alveolated airways that are capable of mimicking physiological respiration by expanding and contracting in a breathing-like fashion. Given a lack of attractive experimental platforms at hand, the study of acinar transport phenomena remains limited with regards to validating computational studies and critically, there remains a dearth of experimental data available. In recent years, Ma et al. (2009) have constructed a scaled-up rigid-wall model of an acinus consisting of three acinar generations; however, the lack of wall motion in this model limited its capability to capture realistic alveolar flow patterns under breathing conditions.

Other scaled-up experiments including a moving wall model based on anatomical data from cast replica were recently introduced25; however, since the model only captured the last two acinar generations (i.e., terminal sacs), it failed to capture the complex recirculating flows that characterize more proximal acinar generations. These latter examples of scaled-up experiments further underline the ongoing limitations with such approaches. Specifically, no existing experiment has thus far demonstrated the hypothesized transition from recirculating to radial flows along the acinus and thereby confirm numerical predictions of flow topologies hypothesized to exist in real pulmonary acinar trees7, 15. Perhaps most critically, scaled-up experiments are extremely limited in investigating inhaled particle transport and deposition dynamics26 due to difficulties in matching all relevant non-dimensional parameters (e.g., particle diffusion, a critical transport mechanism for sub-micron particles, is completely neglected).

With ongoing experimental challenges, new experimental platforms that permit investigations of respiratory air flows and particle dynamics in complex moving walls acinar networks are sought. Here, an anatomically-inspired in vitro acinar model is introduced. This microfluidic platform mimics pulmonary acinar flows directly at the representative acinar scale, and broadens the growing range of pulmonary microfluidic models27, including bronchial liquid plug-flows28–30 and the alveolar-capillary barrier31.

Namely, the present design features a simplified five generation alveolated airway tree with cyclically expanding and contracting walls, where cyclic motions are achieved by controlling pressure inside a water chamber which surrounds the thin PDMS lateral walls and where the top wall is deformed by an additional water chamber sitting directly above the acinar structure. Unlike common multilayer microfluidic devices, this chamber is simply formed by embedding the barrel section of a syringe inside the PDMS Device, and does not require preparation of an additional PDMS mold.

The miniaturized approach presented here offers a simple and versatile means for reproducing complicated acinar structures with moving walls as compared to scaled-up models while capturing the underlying characteristics of the acinar flow environment. This platform can be used for flow visualization using fluid-suspended particles inside the airways (see Representative Results below). In the near future, the model will be used with airborne particles for studying inhaled acinar particle dynamics.

Protocol

1. Master Fabrication

  1. Use deep reactive ion etching (DRIE) of a silicon on insulator (SOI) wafer to fabricate a master silicon wafer as described in former works32, 33. NOTE: DRIE is preferred to standard SU-8 micromachining due to the high aspect ratio features (40 µm wide and 90 µm deep trenches).

2. Casting and Sealing of the Microfluidic Device

  1. Mix PDMS and curing agent at a 10:1 weight ratio inside a clean small container such as a plastic weighing dish.

  2. Degas the mixture in a desiccator under vacuum until all air bubbles are removed. NOTE: Prepare enough PDMS for all subsequent steps. Here below, the acronym "PDMS" refers always to the degassed 10:1 PDMS:curing-agent mixture that was prepared in steps 2.1 and 2.2.

  3. Pour the degassed-mixture to a height of approximately 1 mm above the master wafer. Degas once again for at least 40 min to remove all the air bubbles above the wafer and minimize the bubbles below the wafer. NOTE: Make sure that the wafer is as close as possible to the bottom of the plate. If necessary press the wafer gently to the bottom using 2 stirring sticks and degas once again.

  4. Bake at 65 °C for 20 min in a natural convection oven. NOTE: After 20 min the PDMS is hardened and almost fully cured. While a longer baking time is possible baking for 20 min saves time and improves the adherence of the second PDMS layer (see below) to the first one.

  5. File the barrel section of a plastic 2 ml syringe using a fine grit sand paper to improve adherence to PDMS. In addition, use the sand paper to flatten the base of the syringe barrel by placing the sand paper on a flat surface and sliding the base of the syringe barrel on top of it. Clean the syringe using pressurized air.

  6. Place the barrel section of the syringe on top of the first PDMS layer with the large opening facing the surface of the PDMS, and pour a second layer of PDMS on top of the first one to a height of ~5 mm, and degas the PDMS once again in a desiccator. NOTE: The second PDMS layer should be poured from the small container around the barrel, and should not enter within it. 

  7. Bake the entire setup at 65° C for at least 2 hr in a natural convection oven. NOTE: There is no need to hold the barrel in place during the curing processes since the weight of the PDMS pressing against the wide base of the barrel holds the barrel firmly in place.

  8. Cut through the PDMS mold around the patterned region of the master wafer using a scalpel. While cutting, the scalpel should weakly touch the surface of the wafer. Then, gently insert a thin tool such as wafer forceps in the notch created by the scalpel, and peel off the PDMS cast from the master wafer.

  9. Place the cast on a soft surface covered with aluminum foil with the patterned side facing up (i.e., the barrel should hang from the edge of the table), and punch a hole in the PDMS at the chamber inlet and channel inlet using a 1 mm biopsy punch. 

  10. Coat a clean glass slide with a (degassed) 10:1 PDMS:curing-agent mixture using a spin coater programmed at 3,000 rpm for 30 sec, and bake for >1 hr at 65 °C. Then, clean the slide and PDMS cast using clear tape.

  11. Treat the surface of the PDMS mold and PDMS coated glass slide with O2 plasma (e.g., using a hand-held corona treater) for 1 min, and then gently press the surfaces together and bake at 65 °C overnight (O/N).

3. Device Filling and Actuation

  1. Mix water suspended fluorescent polystyrene particles with water and glycerol in a glass vial to obtain a 64/36 (v/v) glycerol/water mixture with 0.25% (w/w) particles..

  2. Place a drop of the glycerol solution on top of the channel inlet and a drop of DI water on the chamber inlet, then place the apparatus inside a desiccator and vacuum for ~5 min. NOTE: Before releasing the vacuum wait for the bubbles that form in the drops of glycerol solution and DI water to pop. Upon vacuum release the liquids are sucked into the voids inside the device. If residual air remains inside the channels, eliminate it by applying external pressure on the fluids (e.g., using a syringe) and allowing the air to diffuse into the PDMS.

  3. Inject ~2 ml of DI water into the top chamber (i.e., the syringe barrel, Fig. 2b) until it is fully filled with water. Then cover the top chamber with a 19 gauge blunt syringe tip, cut the tip of another blunt 19 gauge syringe tip and insert this tip to the side chamber inlet. Connect both syringe tips to a 1 ml syringe via thin Teflon tubing and a T-shaped connector. NOTE: Make sure that the 1 ml syringe, Teflon tubing, T-shaped connector and top chamber (2 ml syringe barrel) are all filled with water without bubbles. This may be achieved by opening connection points, pushing water through empty sections of tubing and reconnecting the connection points.

  4. Connect the 1 ml syringe to a syringe pump pre-programmed to mimic for example a quiet tidal breathing cycle (with a period of T = 4 sec) constructed of linear ramps, i.e., from zero to 1.8 ml/min in 1 sec, from 1.8 ml/min to -1.8 ml/min in 2 sec and from -1.8 ml/min back to zero in 1 sec.

4. Flow Visualization Experiments: Micro-particle Image Velocimetry (µPIV)

  1. While the device is being actuated, obtain a series of 9 - 12 phase-locked, double-frame images of the particle-seeded flow using a micro-particle image velocimetry (µPIV) system consisting for example of a dual frame-multiple exposure CCD camera (e.g., 1,600 × 1,200 pixels to achieve sufficient resolution), a double pulsed Nd-YAG laser (wavelength: 532 nm, output energy: 400 mJ, pulse duration: 4 nsec), and an inverted microscope. NOTE: Such a system is capable of obtaining frame pairs with a time lag of down to a few microseconds between the first and second frames. To achieve phase-locked double frame images, it is useful to acquire a double frame series at e.g., 10 Hz (frame pairs are separated by 0.1 sec from one another). Then, the data may be reorganized so that all frame pairs that are separated by a full cycle time (here T = 4 sec) form a new time series. Image acquisition should be repeated several times while modifying the lag time between the first and second frames in each frame pair (i.e., 100 µsec to 0.1 sec) for resolving different flow regions inside the alveolar cavity. Note: alternative setups with regards to best combinations of image acquisition systems (i.e., camera) and illumination sources (i.e., lasers) to image such microflows are also available34, 35.

  2. Use a sum-of-correlation algorithm to compute phase-locked velocity vector maps of the resulting flow field from the image series for each time lag used. Repeat this process several times with varying lag times between the first and second frames in each frame pair for resolving different flow regions inside the alveolar cavity. Next, use a data analysis program to stitch together the individual flow maps into a complete and high-detailed map of flow patterns by averaging overlapping data points33.

Representative Results

Computer-aided design (CAD) and microscope images of the in vitro acinar platform are presented in Fig. 1. The biomimetic acinar model features five generations of branching rectangular channels lined with alveolar-like cylindrical cavities (Fig. 1). Here, the model generations are numbered from generation 1 (for the most proximal generation) to generation 5 (for the most distal generation). Note that only the channel inlet leading to generation 1 is open to the outer environment by means of an opening in the PDMS. The 16 ducts leading away from generation 5 are left closed to air (Fig. 1a). By modulating periodically the water pressure within the chambers, the thin walls constituting the alveolar cavities and ducts are cyclically deformed. At the same time, the ceiling of the airways is deformed vertically by means of an additional water chamber located above the ducts; to create this top chamber in a simple manner without preparation of an additional microfluidic layer the barrel of a syringe was submerged inside the PDMS before cross-linking. This resulted in a PDMS layer of approximately 1 mm separating the alveolated ducts and the top water chamber (see Fig. 2).

The water chambers are connected to a syringe pump programmed to repeat a series of linearly ramped flow rates to mimic a normal to heavy tidal breathing scenario of an average human adult with a 4 sec cycle time (T). This results in a periodical decrease and increase of airway volume; since the outlets are sealed and only the inlet is open to the environment, the fluid inside the ducts is inhaled and exhaled from the device through the inlet, in analogy to a natural breathing process. Here, the airway ducts were filled with a glycerol solution seeded with fluorescent particles (see Protocol) and micro particle image velocimetry (µPIV) was used to map the resulting flow fields across the airways tree33.

The normalized velocity magnitude (ux/ux,max) in the streamwise (i.e., axial) direction across the width of the channels is shown in Fig. 3. Results are presented at peak inhalation velocity for each of the 5 device generations, and represent the 2D projection of the flow within a thin slab near the duct midplane. For comparison, the analytical solution of the steady-state laminar flow for an infinitely long channel36 is also presented in Fig. 3.

Figure 4 shows streamline patterns and velocity magnitudes within alveolar cavities at the midplane of the airways at peak inhalation. Figures 4a,b and c depict acinar generations 1, 3 and 5, respectively.

graphic file with name jove-111-53588-0.jpg

Figure 1: Microfluidic Model of the Acinar Tree Network. (a) CAD drawing of the full device. (b) Close-up snapshots of the acinar tree structure showing the channels, the chambers, and the thin walls separating them. Purple arrows indicate the corresponding locations and positive y-directions of the flow profiles presented in Fig. 3. Adapted with permission from ref. 33.

graphic file with name jove-111-53588-1.jpg

Figure 2: CAD Design of the Microfluidic Device. (a) Broken lines indicate the tubes leading from the lateral and top chambers to the syringe pump via a T-shaped connector. (b) side cut through the center of the device illustrating the location of the syringe within the PDMS cast. Adapted with permission from ref. 33.

graphic file with name jove-111-53588-2.jpg

Figure 3: Acinar Flow Velocities. Normalized ductal velocity profiles (ux/ux,max) extracted from PIV along the width of the channel for generations 1 through 5 at the locations illustrated in Fig. 1; y = 0 coincides with the midpoint location across the channel and ux,max = 0.0104 m/sec corresponds here to the peak streamwise velocity measured in device generation 1. PIV measurements are shown here at peak inhalation (t = 0.6 sec) and the black line corresponds to the analytical velocity profile for creeping flow inside a rectangular channel with Wd = 345 µm and h = 92 µm. Adapted with permission from ref. 33.

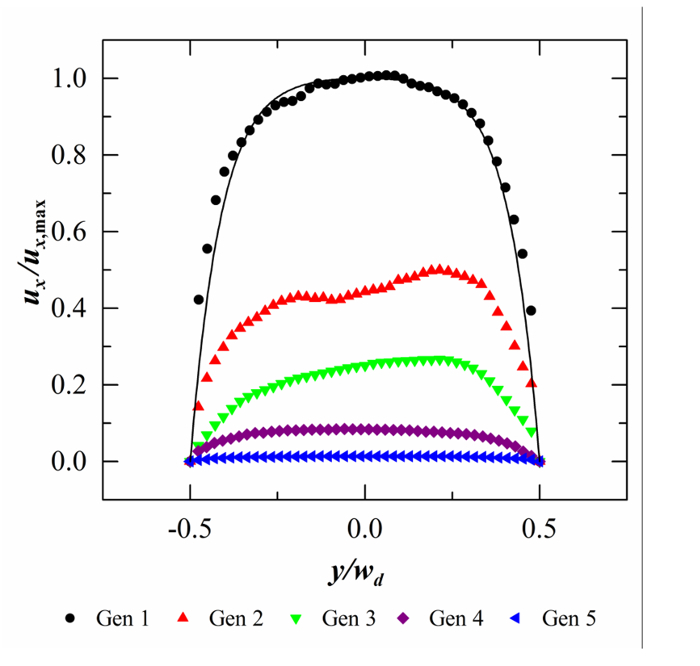
graphic file with name jove-111-53588-3.jpg

Figure 4: Velocity Magnitudes and Corresponding Streamline Patterns. Data are obtained from micro-PIV for a projection of the flow extracted at the midplane of an alveolus located at device generations 1, 3 and 5. Flow fields are shown at approximately peak inhalation (t = 0.6 sec). Velocity magnitudes are shown on a logarithmic scale. Adapted with permission from ref. 33.

Discussion

A critical feature of the microfluidic acinar platform presented here is its ability to reproduce physiologically-realistic breathing motions that give rise to physiological flow profiles and velocities within acinar ducts and within alveoli. Since the microfluidic channels are produced with a relatively low aspect ratio (i.e., wd/h ≈ 3.9, where wd is the duct width and h is the duct height), the measured flows show more plug-like flow characteristics compared to the anticipated parabolic flow profiles that would exist in circular channels. Nevertheless, the measured velocities are well within the physiological range; it is found that the characteristic dimensionless Reynolds number, comparing inertial to viscous forces, yields a maximum of approximately 0.01 corresponding to mid- to distal acinar regions, following semi-empirical estimates2.

Here, the Reynolds number is defined as Re = ūx,maxDh/νglycerol solution, where ūx,max is the average streamwise velocity across the duct midplane at the instant of maximal flow rate, Dh is the hydraulic diameter of the duct and νglycerol solution is the kinematic viscosity of the glycerol solution used for flow visualization which was matched to the kinematic viscosity of air at ~24 °C (νair = 1.55 × 10-5 m2/sec, νglycerol solution = 1.51 × 10-5 m2/sec). In addition, a decrease in flow magnitude by about a factor of two is observed after each bifurcation as expected from the dichotomous branching patterns of the acinar model. Namely, this cascade of flow velocities is an important feature of acinar flows in airway trees.

Flow profiles near and within alveolar cavities (Fig. 4) show that ductal velocities are gradually decreasing towards deeper acinar generations. In addition, flow magnitudes drop steeply along the opening of alveoli resulting in flow velocities that are two to three orders of magnitude slower inside alveoli compared to the ducts; such flow topologies were previously reported in several numerical studies1, 9, 15. In addition, flow patterns change significantly from one acinar generation to another, as predicted in simulations7, 15: while generation 1 features a recirculation zone which roughly coincides with the center of the alveolus (Fig. 4, left), generation 3 is characterized by a recirculation zone which is shifted towards the proximal side of the alveolus with a more open streamline pattern (Fig. 4, middle). Finally, radial streamlines with no recirculation zone are observed in device generation 5 (Fig. 4, right). To the best of the authors' knowledge, this is the first time that the existence of a wide range of alveolar flow patterns is captured experimentally.

The success of the presented method depends on a few critical steps in the microfabrication protocol. First, to prevent the thin PDMS walls from tearing upon release from the master wafer the etched pattern on the surface of the wafer should have straight walls and must not adhere to the cured PDMS. It is therefore highly recommended to produce the wafers using DRIE of a SOI wafer as described in Fishler et al. (2013). Such a master wafer is durable and can be easily coated with an anti-sticking layer by either silanizing the surface as described in Fishler et al. (2013) or by ensuring that the last step in the DRIE process is that of passivation with CF4. Another critical step is filing (step 2.5) and embedding (step 2.6) the syringe barrel to create the top chamber. Air bubbles caught between the syringe base and the first PDMS layer can greatly reduce the integrity and durability of the manufactured device. To prevent bubble formation, it is critical that the base of the syringe barrel is flat and uniformly filed.

While the current design allows fabrication of a two-layer-device using only one master wafer, a modified method may include creating an additional PDMS layer containing a circular indentation to form the top chamber. For this second PDMS layer an additional master wafer featuring a circular ridge can be fabricated using standard SU-8 photolithography. An additional modification of the protocol may include a different method for PDMS bonding that does not require a corona treater. To adhere the PDMS mold to the glass slide, first coat the glass slide as described in step 2.10 of the Protocol but use a 5:1 instead of a 10:1 PDMS:curing-agent weight ratio. Bake the coated glass for 15 min at 65 °C in a natural convection oven, press the PDMS mold to the PDMS coated glass, and bake overnight at 65 °C in a natural convection oven.

On the occasion of liquid leaking from the bonding surface between PDMS mold and glass the following measures may be taken: (1) make sure that the corona treater is producing electric sparks during the treatment, if not, increase the output voltage, (2) prolong treating time with corona treater and (3) use alternative method for bonding the PDMS mold to the glass (see paragraph above). Often water may leak through the connection of the thin Teflon tubing to the chamber inlet. To circumvent such leaking, make sure that 19-gauge blunt syringe tip is used to connect the Teflon tubing to the inlet. If water leaks between the PDMS mold and the top chamber (the 2 ml syringe barrel) make sure that the base of the syringe barrel was properly filed (see step 2.5 in Protocol), and that the second layer of PDMS was poured high enough (~5 mm above the first PDMS layer).

Note that the extent of wall deformation is highly dependent on PDMS mechanical properties. Slight changes in the preparation procedure of the devices may result in considerable variability of the measured velocities between different devices. To ensure maximal repeatability use constant preparation conditions (humidity, baking times etc.). In addition, fine tuning of the volume change during device actuation may be achieved by visualizing the top surface of the channels using phase contrast microscopy and adjusting the velocity ramps of the syringe pump so that the top surface of the channel is deflected to the desired distance as measured by the z-motion of the microscope stage.

An important limitation of the current technique is that the exact morphological characteristics (e.g., anatomy, morphometry) of the lungs cannot be accurately reproduced. Indeed, the planar design of the acinar model does not capture for example out-of-plane acinar bifurcations and the ratio of alveolar to ductal volume is much lower than measured in vivo values37. In addition, the simplified microfluidic geometry only captures a small portion of a full acinus. Despite these limitations, the present model is able to reproduce anticipated flow patterns and velocities directly at the true anatomical length scales, and therefore represents a valuable testing platform for acinar transport phenomena.

To conclude, the featured microfluidic models of the pulmonary acinus show great promise as an in vitro tool for quantitative investigations of respiratory acinar flows mimicking breathing patterns. Here, the simple acinar model consists of five generations of expanding and contracting alveolated ducts, thus reproducing some of the important underlying flow properties anticipated to exist within the acinar region of the lungs. Flow visualization, using micro-PIV, within alveolar cavities provides for the first time experimental evidence of the range of complex recirculating and radial alveolar flows along the acinar tree. This microfluidic approach allows fabrication of complex acinar structures with moving walls following a relatively simple procedure and offers an attractive alternative to scaled-up acinar models. In particular, with the main advantage of delivering a model at a one-to-one scale, true inhaled acinar particle dynamics can be investigated without further need for dynamic similarity matching.

Disclosures

The authors have nothing to disclose.

Acknowledgments

This work was supported in part by the European Commission (FP7 Program) through a Career Integration Grant (PCIG09-GA-2011-293604), the Israel Science Foundation (Grant nr. 990/12) and the Technion Center of Excellence in Environmental Health and Exposure Science (TCEEH). Microfabrication of microfluidic chips was conducted at the Micro-Nano Fabrication Unit (MNFU) of the Technion and supported by a seed grant from the Russel Berrie Institute of Nanotechnology (RBNI) at Technion. The authors thank Avshalom Shai for assistance during deep reactive ion etching (DRIE) and Molly Mulligan and Philipp Hofemeier for helpful discussions.

References

  1. Kleinstreuer C, Zhang Z. Airflow and Particle Transport in the Human Respiratory System. Annu. Rev. Fluid Mech. 2010;42(1):301–334. [Google Scholar]
  2. Sznitman J. Respiratory microflows in the pulmonary acinus. J. Biomech. 2013;46(2):284–298. doi: 10.1016/j.jbiomech.2012.10.028. [DOI] [PubMed] [Google Scholar]
  3. Tsuda A, Henry FS, Butler JP. Gas and aerosol mixing in the acinus. Respir. Physiol. Neurobiol. 2008;163(1-3):139–149. doi: 10.1016/j.resp.2008.02.010. [DOI] [PMC free article] [PubMed] [Google Scholar]
  4. Kleinstreuer C, Zhang Z, Donohue JF. Targeted Drug-Aerosol Delivery in the Human Respiratory System. Annu. Rev. Biomed. Eng. 2008;10(1):195–220. doi: 10.1146/annurev.bioeng.10.061807.160544. [DOI] [PubMed] [Google Scholar]
  5. Semmler-Behnke M, Kreyling WG, Schulz H, Takenaka S, Butler JP, Henry FS, Tsuda A. Nanoparticle delivery in infant lungs. Proc. Natl. Acad. Sci. 2012;109(13):5092–5097. doi: 10.1073/pnas.1119339109. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Sznitman J, Heimsch F, Heimsch T, Rusch D, Rosgen T. Three-Dimensional Convective Alveolar Flow Induced by Rhythmic Breathing Motion of the Pulmonary Acinus. J. Biomech. Eng. 2007;129(5):658–665. doi: 10.1115/1.2768109. [DOI] [PubMed] [Google Scholar]
  7. Tsuda A, Henry FS, Butler JP. Chaotic mixing of alveolated duct flow in rhythmically expanding pulmonary acinus. J. Appl. Physiol. 1995;79(3):1055–1063. doi: 10.1152/jappl.1995.79.3.1055. [DOI] [PubMed] [Google Scholar]
  8. Henry FS, Butler JP, Tsuda A. Kinematically irreversible acinar flow: a departure from classical dispersive aerosol transport theories. J. Appl. Physiol. 2002;92(2):835–845. doi: 10.1152/japplphysiol.00385.2001. [DOI] [PubMed] [Google Scholar]
  9. Kumar H, Tawhai MH, Hoffman EA, Lin CL. The effects of geometry on airflow in the acinar region of the human lung. J. Biomech. 2009;42(11):1635–1642. doi: 10.1016/j.jbiomech.2009.04.046. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Lee DY, Lee JW. Characteristics of particle transport in an expanding or contracting alveolated tube. J. Aerosol Sci. 2003;34(9):1193–1215. [Google Scholar]
  11. Tsuda A, Butler JP, Fredberg JJ. Effects of alveolated duct structure on aerosol kinetics. I. Diffusional deposition in the absence of gravity. J. Appl. Physiol. 1994;76(6):2497–2509. doi: 10.1152/jappl.1994.76.6.2497. [DOI] [PubMed] [Google Scholar]
  12. Tsuda A, Butler JP, Fredberg JJ. Effects of alveolated duct structure on aerosol kinetics. II. Gravitational sedimentation and inertial impaction. J. Appl. Physiol. 1994;76(76):2510–2516. doi: 10.1152/jappl.1994.76.6.2510. [DOI] [PubMed] [Google Scholar]
  13. Ma B, Darquenne C. Aerosol bolus dispersion in acinar airways—influence of gravity and airway asymmetry. J. Appl. Physiol. 2012;113(3):442–450. doi: 10.1152/japplphysiol.01549.2011. [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Ma B, Darquenne C. Aerosol deposition characteristics in distal acinar airways under cyclic breathing conditions. J. Appl. Physiol. 2011;110(5):1271–1282. doi: 10.1152/japplphysiol.00735.2010. [DOI] [PMC free article] [PubMed] [Google Scholar]
  15. Heimsch J, Sznitman T, Wildhaber JH, Tsuda A, Rösgen T. Respiratory Flow Phenomena and Gravitational Deposition in a Three-Dimensional Space-Filling Model of the Pulmonary Acinar Tree. J. Biomech. Eng. 2009;131(3):031010. doi: 10.1115/1.3049481. [DOI] [PubMed] [Google Scholar]
  16. Litzlbauer HD, Korbel K, Kline TL, Jorgensen SM, Eaker DR, Bohle RM, Ritman EL, Langheinrich AC. Synchrotron-Based Micro-CT Imaging of the Human Lung Acinus. Anat. Rec. Adv. Integr. Anat. Evol. Biol. 2010;293(9):1607–1614. doi: 10.1002/ar.21161. [DOI] [PMC free article] [PubMed] [Google Scholar]
  17. Tsuda A, Filipovic N, Haberthür D, Dickie R, Matsui Y, Stampanoni M, Schittny JC. Finite element 3D reconstruction of the pulmonary acinus imaged by synchrotron X-ray tomography. J. Appl. Physiol. 2008;105(3):964–976. doi: 10.1152/japplphysiol.90546.2008. [DOI] [PMC free article] [PubMed] [Google Scholar]
  18. Berg EJ, Weisman JL, Oldham MJ, Robinson RJ. Flow field analysis in a compliant acinus replica model using particle image velocimetry (PIV) J. Biomech. 2010;43(6):1039–1047. doi: 10.1016/j.jbiomech.2009.12.019. [DOI] [PubMed] [Google Scholar]
  19. Sznitman J, Sutter R, Altorfer D, Stampanoni M, Rösgen T, Schittny JC. Visualization of respiratory flows from 3D reconstructed alveolar airspaces using X-ray tomographic microscopy. J. Vis. 2010;13(4):337–345. [Google Scholar]
  20. Henry FS, Haber S, Haberthür D, Filipovic N, Milasinovic D, Schittny JC, Tsuda A. The Simultaneous Role of an Alveolus as Flow Mixer and Flow Feeder for the Deposition of Inhaled Submicron Particles. J. Biomech. Eng. 2012;134(12):121001. doi: 10.1115/1.4007949. [DOI] [PMC free article] [PubMed] [Google Scholar]
  21. Chhabra S, Prasad AK. Flow and Particle Dispersion in Lung Acini: Effect of Geometric and Dynamic Parameters During Synchronous Ventilation. J. Fluids Eng. 2011;133(7):071001. doi: 10.1115/1.4004362. [DOI] [PMC free article] [PubMed] [Google Scholar]
  22. Cinkotai FF. Fluid flow in a model alveolar sac. J. Appl. Physiol. 1974;37(2):249–251. doi: 10.1152/jappl.1974.37.2.249. [DOI] [PubMed] [Google Scholar]
  23. Karl A, Henry FS, Tsuda A. Low reynolds number viscous flow in an alveolated duct. J. Biomech. Eng. 2004;126(4):420–429. doi: 10.1115/1.1784476. [DOI] [PubMed] [Google Scholar]
  24. Tippe A, Tsuda A. recirculating flow in an expanding alveolar model: experimental evidence of flow-induced mixing of aerosols in the pulmonary acinus. J. Aerosol Sci. 2000;31(8):979–986. [Google Scholar]
  25. Berg EJ, Robinson RJ. Stereoscopic particle image velocimetry analysis of healthy and emphysemic alveolar sac models. J. Biomech. Eng. 2011;133(6):061004. doi: 10.1115/1.4004251. [DOI] [PubMed] [Google Scholar]
  26. Ma B, Ruwet V, Corieri P, Theunissen R, Riethmuller M, Darquenne C. CFD simulation and experimental validation of fluid flow and particle transport in a model of alveolated airways. J. Aerosol Sci. 2009;40(5):403–414. doi: 10.1016/j.jaerosci.2009.01.002. [DOI] [PMC free article] [PubMed] [Google Scholar]
  27. Kumar Mahto S, Tenenbaum-Katan J, Sznitman J. Respiratory Physiology on a Chip. Scientifica. 2012;2012:e364054. doi: 10.6064/2012/364054. [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Huh D, Fujioka H, Tung YC, Futai N, Paine R, Grotberg JB, Takayama S. Acoustically detectable cellular-level lung injury induced by fluid mechanical stresses in microfluidic airway systems. Proc. Natl. Acad. Sci. 2007;104(48):18886–18891. doi: 10.1073/pnas.0610868104. [DOI] [PMC free article] [PubMed] [Google Scholar]
  29. Song Y, Baudoin M, Manneville P, Baroud CN. The air–liquid flow in a microfluidic airway tree. Med. Eng. Phys. 2011;33(7):849–856. doi: 10.1016/j.medengphy.2010.10.001. [DOI] [PubMed] [Google Scholar]
  30. Tavana H, Huh D, Grotberg JB, Takayama S. Microfluidics, Lung Surfactant, and Respiratory Disorders. Lab Med. 2009;40(4):203–209. [Google Scholar]
  31. Huh D, Matthews BD, Mammoto A, Montoya-Zavala M, Hsin HY, Ingber DE. Reconstituting Organ-Level Lung Functions on a Chip. Science. 2010;328(5986):1662–1668. doi: 10.1126/science.1188302. [DOI] [PMC free article] [PubMed] [Google Scholar]
  32. Pihl J, Sinclair J, Sahlin E, Karlsson M, Petterson F, J Olofsson, Orwar O. Microfluidic Gradient-Generating Device for Pharmacological Profiling. Anal. Chem. 2005;77(13):3897–3903. doi: 10.1021/ac050218+. [DOI] [PubMed] [Google Scholar]
  33. Fishler R, Mulligan MK, Sznitman J. Acinus-on-a-chip: A microfluidic platform for pulmonary acinar flows. J. Biomech. 2013;46(16):2817–2823. doi: 10.1016/j.jbiomech.2013.08.020. [DOI] [PubMed] [Google Scholar]
  34. Lindken R, Rossi M, Grosse S, Westerweel J. Micro-Particle Image Velocimetry (microPIV): recent developments, applications, and guidelines. Lab. Chip. 2009;9(17):2551–2567. doi: 10.1039/b906558j. [DOI] [PubMed] [Google Scholar]
  35. Wereley ST, Meinhart CD. Recent Advances in Micro-Particle Image Velocimetry. Annu. Rev. Fluid Mech. 2010;42(1):557–576. [Google Scholar]
  36. Bruus H. Theoretical Microfluidics. Oxford Master Series in Condensed Matter Physics. 2008.
  37. Haefeli-Bleuer B, Weibel ER. Morphometry of the human pulmonary acinus. Anat. Rec. 1988;220(4):401–414. doi: 10.1002/ar.1092200410. [DOI] [PubMed] [Google Scholar]

Articles from Journal of Visualized Experiments : JoVE are provided here courtesy of MyJoVE Corporation

RESOURCES