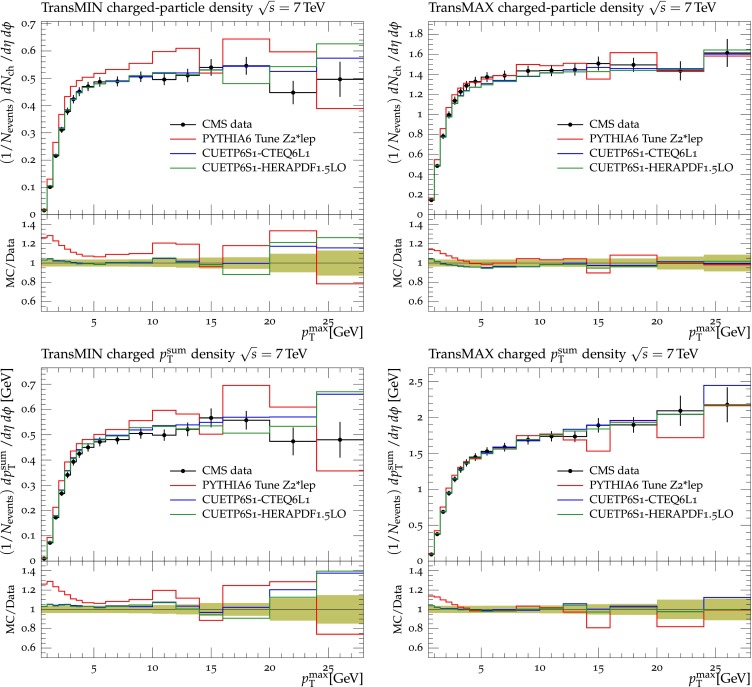
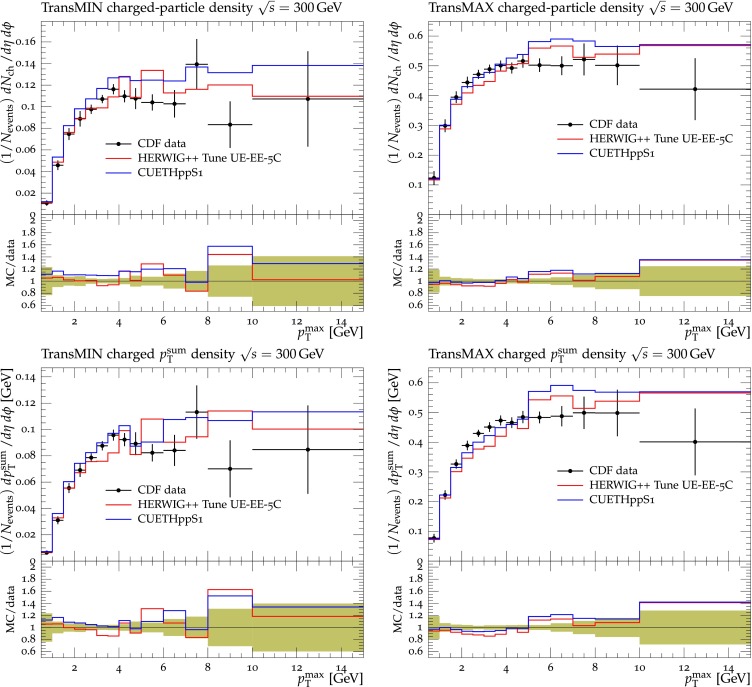
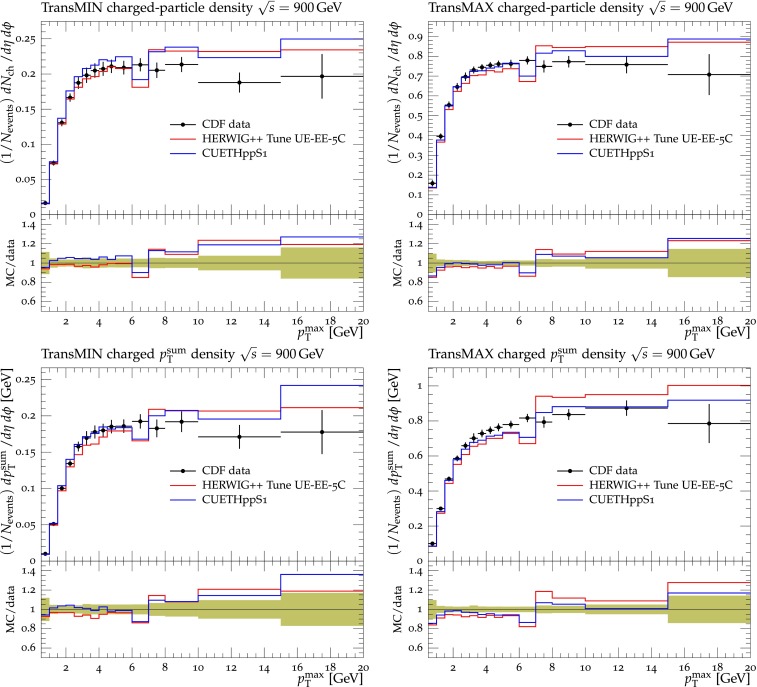
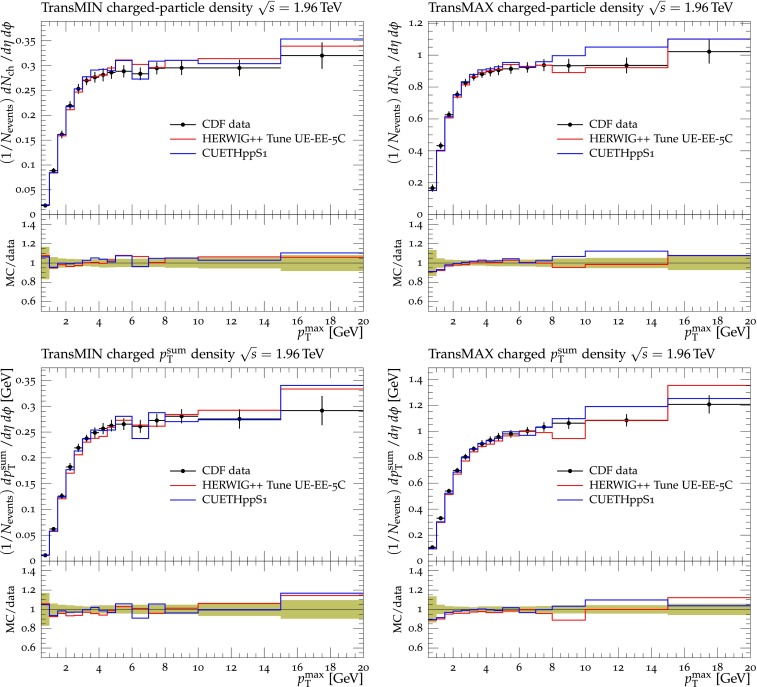
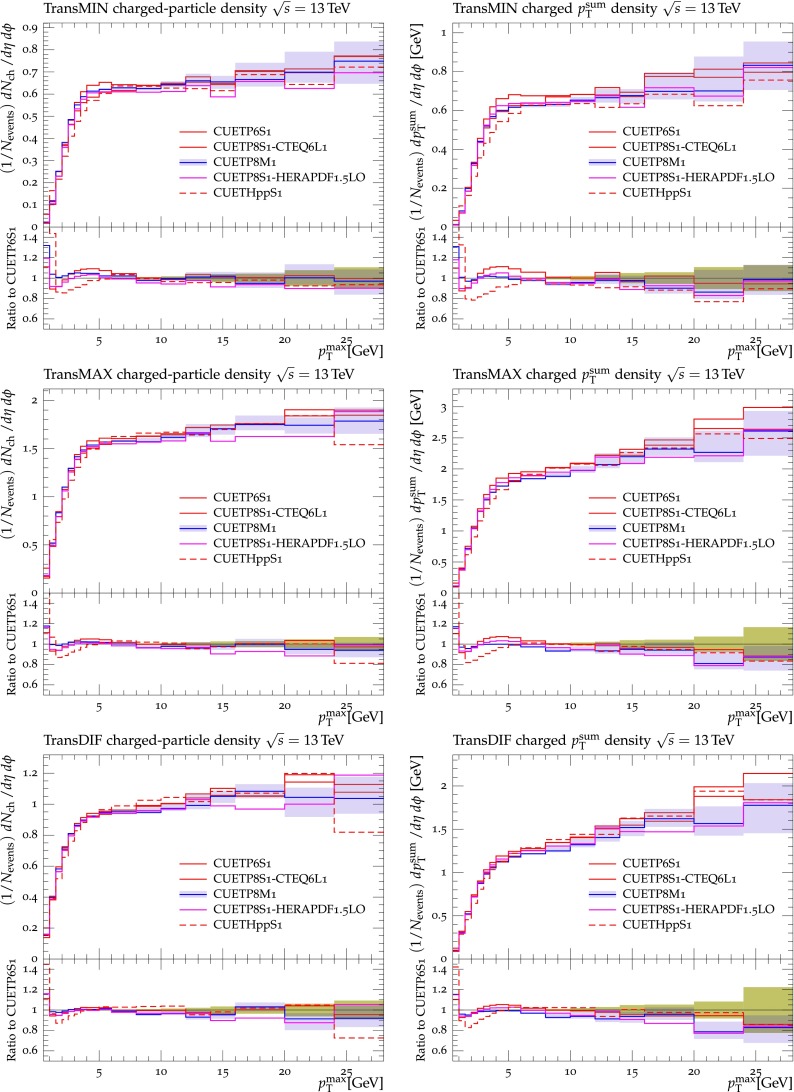
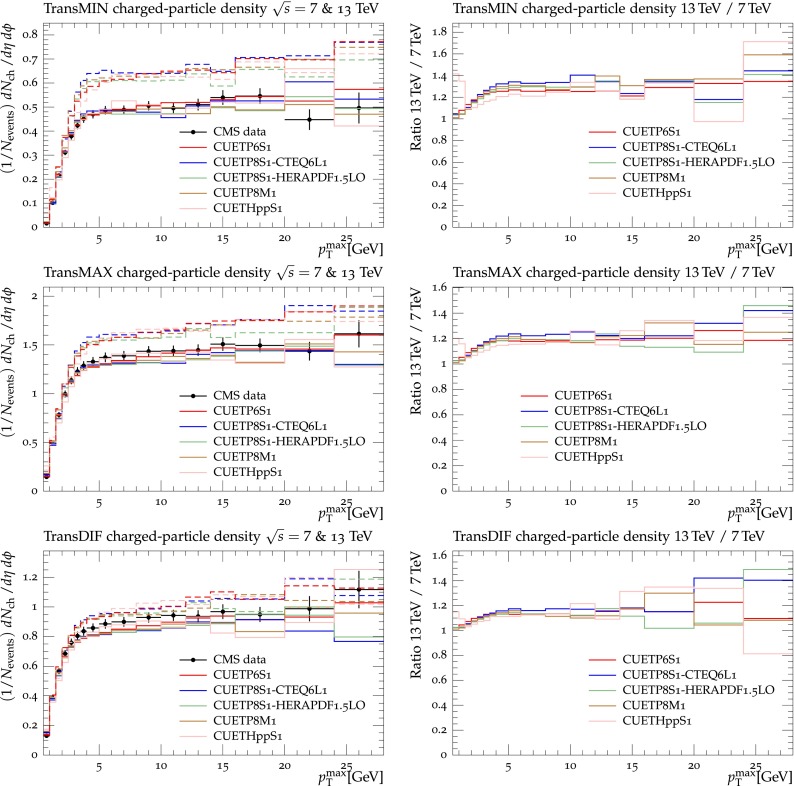
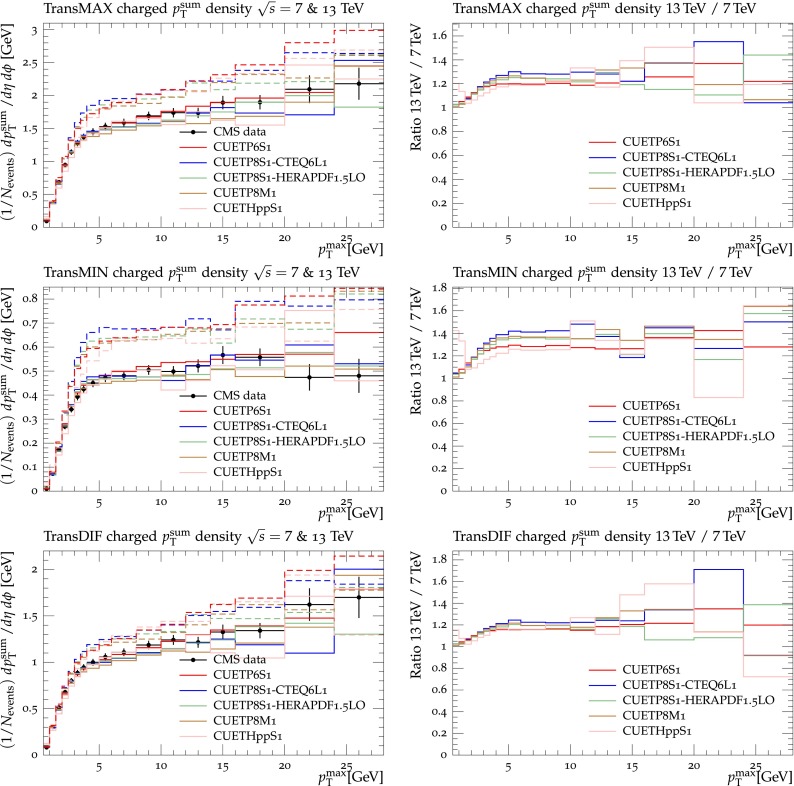
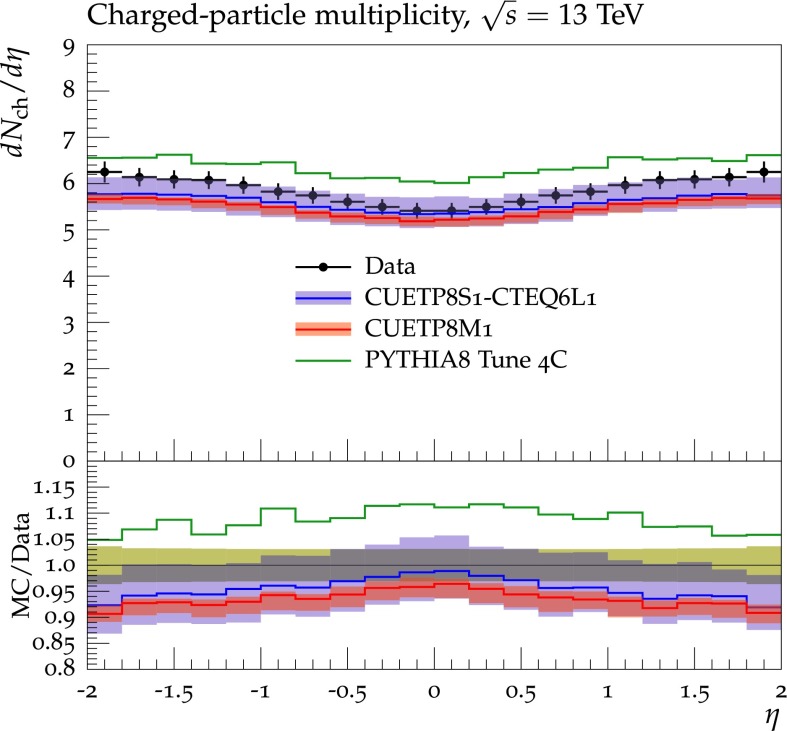
V Khachatryan
V Khachatryan
1Yerevan Physics Institute, Yerevan, Armenia
1,
A M Sirunyan
A M Sirunyan
1Yerevan Physics Institute, Yerevan, Armenia
1,
A Tumasyan
A Tumasyan
1Yerevan Physics Institute, Yerevan, Armenia
1,
W Adam
W Adam
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
E Asilar
E Asilar
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
T Bergauer
T Bergauer
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
J Brandstetter
J Brandstetter
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
E Brondolin
E Brondolin
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
M Dragicevic
M Dragicevic
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
J Erö
J Erö
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
M Friedl
M Friedl
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
R Frühwirth
R Frühwirth
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
V M Ghete
V M Ghete
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
C Hartl
C Hartl
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
N Hörmann
N Hörmann
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
J Hrubec
J Hrubec
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
M Jeitler
M Jeitler
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
V Knünz
V Knünz
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
A König
A König
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
M Krammer
M Krammer
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
I Krätschmer
I Krätschmer
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
D Liko
D Liko
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
T Matsushita
T Matsushita
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
I Mikulec
I Mikulec
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
D Rabady
D Rabady
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
B Rahbaran
B Rahbaran
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
H Rohringer
H Rohringer
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
J Schieck
J Schieck
1Yerevan Physics Institute, Yerevan, Armenia
1,
R Schöfbeck
R Schöfbeck
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
J Strauss
J Strauss
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
W Treberer-Treberspurg
W Treberer-Treberspurg
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
W Waltenberger
W Waltenberger
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
C-E Wulz
C-E Wulz
2Institut für Hochenergiephysik der OeAW, Vienna, Austria
2,
V Mossolov
V Mossolov
3National Centre for Particle and High Energy Physics, Minsk, Belarus
3,
N Shumeiko
N Shumeiko
3National Centre for Particle and High Energy Physics, Minsk, Belarus
3,
J Suarez Gonzalez
J Suarez Gonzalez
3National Centre for Particle and High Energy Physics, Minsk, Belarus
3,
S Alderweireldt
S Alderweireldt
4Universiteit Antwerpen, Antwerp, Belgium
4,
T Cornelis
T Cornelis
4Universiteit Antwerpen, Antwerp, Belgium
4,
E A De Wolf
E A De Wolf
4Universiteit Antwerpen, Antwerp, Belgium
4,
X Janssen
X Janssen
4Universiteit Antwerpen, Antwerp, Belgium
4,
A Knutsson
A Knutsson
4Universiteit Antwerpen, Antwerp, Belgium
4,
J Lauwers
J Lauwers
4Universiteit Antwerpen, Antwerp, Belgium
4,
S Luyckx
S Luyckx
4Universiteit Antwerpen, Antwerp, Belgium
4,
M Van De Klundert
M Van De Klundert
4Universiteit Antwerpen, Antwerp, Belgium
4,
H Van Haevermaet
H Van Haevermaet
4Universiteit Antwerpen, Antwerp, Belgium
4,
P Van Mechelen
P Van Mechelen
4Universiteit Antwerpen, Antwerp, Belgium
4,
N Van Remortel
N Van Remortel
4Universiteit Antwerpen, Antwerp, Belgium
4,
A Van Spilbeeck
A Van Spilbeeck
4Universiteit Antwerpen, Antwerp, Belgium
4,
S Abu Zeid
S Abu Zeid
5Vrije Universiteit Brussel, Brussels, Belgium
5,
F Blekman
F Blekman
5Vrije Universiteit Brussel, Brussels, Belgium
5,
J D’Hondt
J D’Hondt
5Vrije Universiteit Brussel, Brussels, Belgium
5,
N Daci
N Daci
5Vrije Universiteit Brussel, Brussels, Belgium
5,
I De Bruyn
I De Bruyn
5Vrije Universiteit Brussel, Brussels, Belgium
5,
K Deroover
K Deroover
5Vrije Universiteit Brussel, Brussels, Belgium
5,
N Heracleous
N Heracleous
5Vrije Universiteit Brussel, Brussels, Belgium
5,
J Keaveney
J Keaveney
5Vrije Universiteit Brussel, Brussels, Belgium
5,
S Lowette
S Lowette
5Vrije Universiteit Brussel, Brussels, Belgium
5,
L Moreels
L Moreels
5Vrije Universiteit Brussel, Brussels, Belgium
5,
A Olbrechts
A Olbrechts
5Vrije Universiteit Brussel, Brussels, Belgium
5,
Q Python
Q Python
5Vrije Universiteit Brussel, Brussels, Belgium
5,
D Strom
D Strom
5Vrije Universiteit Brussel, Brussels, Belgium
5,
S Tavernier
S Tavernier
5Vrije Universiteit Brussel, Brussels, Belgium
5,
W Van Doninck
W Van Doninck
5Vrije Universiteit Brussel, Brussels, Belgium
5,
P Van Mulders
P Van Mulders
5Vrije Universiteit Brussel, Brussels, Belgium
5,
G P Van Onsem
G P Van Onsem
5Vrije Universiteit Brussel, Brussels, Belgium
5,
I Van Parijs
I Van Parijs
5Vrije Universiteit Brussel, Brussels, Belgium
5,
P Barria
P Barria
6Université Libre de Bruxelles, Brussels, Belgium
6,
H Brun
H Brun
6Université Libre de Bruxelles, Brussels, Belgium
6,
C Caillol
C Caillol
6Université Libre de Bruxelles, Brussels, Belgium
6,
B Clerbaux
B Clerbaux
6Université Libre de Bruxelles, Brussels, Belgium
6,
G De Lentdecker
G De Lentdecker
6Université Libre de Bruxelles, Brussels, Belgium
6,
G Fasanella
G Fasanella
6Université Libre de Bruxelles, Brussels, Belgium
6,
L Favart
L Favart
6Université Libre de Bruxelles, Brussels, Belgium
6,
A Grebenyuk
A Grebenyuk
6Université Libre de Bruxelles, Brussels, Belgium
6,
G Karapostoli
G Karapostoli
6Université Libre de Bruxelles, Brussels, Belgium
6,
T Lenzi
T Lenzi
6Université Libre de Bruxelles, Brussels, Belgium
6,
A Léonard
A Léonard
6Université Libre de Bruxelles, Brussels, Belgium
6,
T Maerschalk
T Maerschalk
6Université Libre de Bruxelles, Brussels, Belgium
6,
A Marinov
A Marinov
6Université Libre de Bruxelles, Brussels, Belgium
6,
L Perniè
L Perniè
6Université Libre de Bruxelles, Brussels, Belgium
6,
A Randle-conde
A Randle-conde
6Université Libre de Bruxelles, Brussels, Belgium
6,
T Seva
T Seva
6Université Libre de Bruxelles, Brussels, Belgium
6,
C Vander Velde
C Vander Velde
6Université Libre de Bruxelles, Brussels, Belgium
6,
R Yonamine
R Yonamine
6Université Libre de Bruxelles, Brussels, Belgium
6,
P Vanlaer
P Vanlaer
6Université Libre de Bruxelles, Brussels, Belgium
6,
R Yonamine
R Yonamine
6Université Libre de Bruxelles, Brussels, Belgium
6,
F Zenoni
F Zenoni
6Université Libre de Bruxelles, Brussels, Belgium
6,
F Zhang
F Zhang
6Université Libre de Bruxelles, Brussels, Belgium
6,
V Adler
V Adler
7Ghent University, Ghent, Belgium
7,
K Beernaert
K Beernaert
7Ghent University, Ghent, Belgium
7,
L Benucci
L Benucci
7Ghent University, Ghent, Belgium
7,
A Cimmino
A Cimmino
7Ghent University, Ghent, Belgium
7,
S Crucy
S Crucy
7Ghent University, Ghent, Belgium
7,
D Dobur
D Dobur
7Ghent University, Ghent, Belgium
7,
A Fagot
A Fagot
7Ghent University, Ghent, Belgium
7,
G Garcia
G Garcia
7Ghent University, Ghent, Belgium
7,
M Gul
M Gul
7Ghent University, Ghent, Belgium
7,
J Mccartin
J Mccartin
7Ghent University, Ghent, Belgium
7,
A A Ocampo Rios
A A Ocampo Rios
7Ghent University, Ghent, Belgium
7,
D Poyraz
D Poyraz
7Ghent University, Ghent, Belgium
7,
D Ryckbosch
D Ryckbosch
7Ghent University, Ghent, Belgium
7,
S Salva
S Salva
7Ghent University, Ghent, Belgium
7,
M Sigamani
M Sigamani
7Ghent University, Ghent, Belgium
7,
M Tytgat
M Tytgat
7Ghent University, Ghent, Belgium
7,
W Van Driessche
W Van Driessche
7Ghent University, Ghent, Belgium
7,
E Yazgan
E Yazgan
7Ghent University, Ghent, Belgium
7,
N Zaganidis
N Zaganidis
7Ghent University, Ghent, Belgium
7,
S Basegmez
S Basegmez
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
C Beluffi
C Beluffi
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
O Bondu
O Bondu
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
S Brochet
S Brochet
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
G Bruno
G Bruno
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
A Caudron
A Caudron
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
L Ceard
L Ceard
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
G G Da Silveira
G G Da Silveira
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
C Delaere
C Delaere
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
D Favart
D Favart
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
L Forthomme
L Forthomme
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
A Giammanco
A Giammanco
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
J Hollar
J Hollar
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
A Jafari
A Jafari
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
P Jez
P Jez
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
M Komm
M Komm
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
V Lemaitre
V Lemaitre
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
A Mertens
A Mertens
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
M Musich
M Musich
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
C Nuttens
C Nuttens
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
L Perrini
L Perrini
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
A Pin
A Pin
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
K Piotrzkowski
K Piotrzkowski
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
A Popov
A Popov
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
L Quertenmont
L Quertenmont
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
M Selvaggi
M Selvaggi
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
M Vidal Marono
M Vidal Marono
8Université Catholique de Louvain, Louvain-la-Neuve, Belgium
8,
N Beliy
N Beliy
9Université de Mons, Mons, Belgium
9,
G H Hammad
G H Hammad
9Université de Mons, Mons, Belgium
9,
W L Aldá Júnior
W L Aldá Júnior
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
F L Alves
F L Alves
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
G A Alves
G A Alves
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
L Brito
L Brito
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
M Correa Martins Junior
M Correa Martins Junior
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
M Hamer
M Hamer
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
C Hensel
C Hensel
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
A Moraes
A Moraes
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
M E Pol
M E Pol
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
P Rebello Teles
P Rebello Teles
10Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro, Brazil
10,
E Belchior Batista Das Chagas
E Belchior Batista Das Chagas
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
W Carvalho
W Carvalho
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
J Chinellato
J Chinellato
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
A Custódio
A Custódio
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
E M Da Costa
E M Da Costa
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
D De Jesus Damiao
D De Jesus Damiao
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
C De Oliveira Martins
C De Oliveira Martins
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
S Fonseca De Souza
S Fonseca De Souza
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
L M Huertas Guativa
L M Huertas Guativa
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
H Malbouisson
H Malbouisson
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
D Matos Figueiredo
D Matos Figueiredo
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
C Mora Herrera
C Mora Herrera
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
L Mundim
L Mundim
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
H Nogima
H Nogima
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
W L Prado Da Silva
W L Prado Da Silva
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
A Santoro
A Santoro
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
A Sznajder
A Sznajder
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
E J Tonelli Manganote
E J Tonelli Manganote
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
A Vilela Pereira
A Vilela Pereira
11Universidade do Estado do Rio de Janeiro, Rio de Janeiro, Brazil
11,
S Ahuja
S Ahuja
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
C A Bernardes
C A Bernardes
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
A De Souza Santos
A De Souza Santos
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
S Dogra
S Dogra
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
T R Fernandez Perez Tomei
T R Fernandez Perez Tomei
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
E M Gregores
E M Gregores
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
P G Mercadante
P G Mercadante
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
C S Moon
C S Moon
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
S F Novaes
S F Novaes
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
Sandra S Padula
Sandra S Padula
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
D Romero Abad
D Romero Abad
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
J C Ruiz Vargas
J C Ruiz Vargas
12Universidade Estadual Paulista, Universidade Federal do ABC, São Paulo, Brazil
12,
A Aleksandrov
A Aleksandrov
13Institute for Nuclear Research and Nuclear Energy, Sofia, Bulgaria
13,
R Hadjiiska
R Hadjiiska
13Institute for Nuclear Research and Nuclear Energy, Sofia, Bulgaria
13,
P Iaydjiev
P Iaydjiev
13Institute for Nuclear Research and Nuclear Energy, Sofia, Bulgaria
13,
M Rodozov
M Rodozov
13Institute for Nuclear Research and Nuclear Energy, Sofia, Bulgaria
13,
S Stoykova
S Stoykova
13Institute for Nuclear Research and Nuclear Energy, Sofia, Bulgaria
13,
G Sultanov
G Sultanov
13Institute for Nuclear Research and Nuclear Energy, Sofia, Bulgaria
13,
M Vutova
M Vutova
13Institute for Nuclear Research and Nuclear Energy, Sofia, Bulgaria
13,
A Dimitrov
A Dimitrov
14University of Sofia, Sofia, Bulgaria
14,
I Glushkov
I Glushkov
14University of Sofia, Sofia, Bulgaria
14,
L Litov
L Litov
14University of Sofia, Sofia, Bulgaria
14,
B Pavlov
B Pavlov
14University of Sofia, Sofia, Bulgaria
14,
P Petkov
P Petkov
14University of Sofia, Sofia, Bulgaria
14,
M Ahmad
M Ahmad
14University of Sofia, Sofia, Bulgaria
14,
J G Bian
J G Bian
15Institute of High Energy Physics, Beijing, China
15,
G M Chen
G M Chen
15Institute of High Energy Physics, Beijing, China
15,
H S Chen
H S Chen
15Institute of High Energy Physics, Beijing, China
15,
M Chen
M Chen
15Institute of High Energy Physics, Beijing, China
15,
T Cheng
T Cheng
15Institute of High Energy Physics, Beijing, China
15,
R Du
R Du
15Institute of High Energy Physics, Beijing, China
15,
C H Jiang
C H Jiang
15Institute of High Energy Physics, Beijing, China
15,
R Plestina
R Plestina
15Institute of High Energy Physics, Beijing, China
15,
F Romeo
F Romeo
15Institute of High Energy Physics, Beijing, China
15,
S M Shaheen
S M Shaheen
15Institute of High Energy Physics, Beijing, China
15,
A Spiezia
A Spiezia
15Institute of High Energy Physics, Beijing, China
15,
J Tao
J Tao
15Institute of High Energy Physics, Beijing, China
15,
C Wang
C Wang
15Institute of High Energy Physics, Beijing, China
15,
Z Wang
Z Wang
15Institute of High Energy Physics, Beijing, China
15,
H Zhang
H Zhang
15Institute of High Energy Physics, Beijing, China
15,
C Asawatangtrakuldee
C Asawatangtrakuldee
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
Y Ban
Y Ban
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
Q Li
Q Li
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
S Liu
S Liu
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
Y Mao
Y Mao
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
S J Qian
S J Qian
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
D Wang
D Wang
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
Z Xu
Z Xu
16State Key Laboratory of Nuclear Physics and Technology, Peking University, Beijing, China
16,
C Avila
C Avila
17Universidad de Los Andes, Bogota, Colombia
17,
A Cabrera
A Cabrera
17Universidad de Los Andes, Bogota, Colombia
17,
L F Chaparro Sierra
L F Chaparro Sierra
17Universidad de Los Andes, Bogota, Colombia
17,
C Florez
C Florez
17Universidad de Los Andes, Bogota, Colombia
17,
J P Gomez
J P Gomez
17Universidad de Los Andes, Bogota, Colombia
17,
B Gomez Moreno
B Gomez Moreno
17Universidad de Los Andes, Bogota, Colombia
17,
J C Sanabria
J C Sanabria
17Universidad de Los Andes, Bogota, Colombia
17,
N Godinovic
N Godinovic
18Faculty of Electrical Engineering, Mechanical Engineering and Naval Architecture, University of Split, Split, Croatia
18,
D Lelas
D Lelas
18Faculty of Electrical Engineering, Mechanical Engineering and Naval Architecture, University of Split, Split, Croatia
18,
I Puljak
I Puljak
18Faculty of Electrical Engineering, Mechanical Engineering and Naval Architecture, University of Split, Split, Croatia
18,
P M Ribeiro Cipriano
P M Ribeiro Cipriano
18Faculty of Electrical Engineering, Mechanical Engineering and Naval Architecture, University of Split, Split, Croatia
18,
Z Antunovic
Z Antunovic
19Faculty of Science, University of Split, Split, Croatia
19,
M Kovac
M Kovac
19Faculty of Science, University of Split, Split, Croatia
19,
V Brigljevic
V Brigljevic
20Institute Rudjer Boskovic, Zagreb, Croatia
20,
K Kadija
K Kadija
20Institute Rudjer Boskovic, Zagreb, Croatia
20,
J Luetic
J Luetic
20Institute Rudjer Boskovic, Zagreb, Croatia
20,
S Micanovic
S Micanovic
20Institute Rudjer Boskovic, Zagreb, Croatia
20,
L Sudic
L Sudic
20Institute Rudjer Boskovic, Zagreb, Croatia
20,
A Attikis
A Attikis
21University of Cyprus, Nicosia, Cyprus
21,
G Mavromanolakis
G Mavromanolakis
21University of Cyprus, Nicosia, Cyprus
21,
J Mousa
J Mousa
21University of Cyprus, Nicosia, Cyprus
21,
C Nicolaou
C Nicolaou
21University of Cyprus, Nicosia, Cyprus
21,
F Ptochos
F Ptochos
21University of Cyprus, Nicosia, Cyprus
21,
P A Razis
P A Razis
21University of Cyprus, Nicosia, Cyprus
21,
H Rykaczewski
H Rykaczewski
21University of Cyprus, Nicosia, Cyprus
21,
M Bodlak
M Bodlak
22Charles University, Prague, Czech Republic
22,
M Finger
M Finger
22Charles University, Prague, Czech Republic
22,
M Finger Jr
M Finger Jr
22Charles University, Prague, Czech Republic
22,
A A Abdelalim
A A Abdelalim
23Academy of Scientific Research and Technology of the Arab Republic of Egypt, Egyptian Network of High Energy Physics, Cairo, Egypt
23,
A Awad
A Awad
23Academy of Scientific Research and Technology of the Arab Republic of Egypt, Egyptian Network of High Energy Physics, Cairo, Egypt
23,
A Mahrous
A Mahrous
23Academy of Scientific Research and Technology of the Arab Republic of Egypt, Egyptian Network of High Energy Physics, Cairo, Egypt
23,
Y Mohammed
Y Mohammed
23Academy of Scientific Research and Technology of the Arab Republic of Egypt, Egyptian Network of High Energy Physics, Cairo, Egypt
23,
A Radi
A Radi
23Academy of Scientific Research and Technology of the Arab Republic of Egypt, Egyptian Network of High Energy Physics, Cairo, Egypt
23,
B Calpas
B Calpas
24National Institute of Chemical Physics and Biophysics, Tallinn, Estonia
24,
M Kadastik
M Kadastik
24National Institute of Chemical Physics and Biophysics, Tallinn, Estonia
24,
M Murumaa
M Murumaa
24National Institute of Chemical Physics and Biophysics, Tallinn, Estonia
24,
M Raidal
M Raidal
24National Institute of Chemical Physics and Biophysics, Tallinn, Estonia
24,
A Tiko
A Tiko
24National Institute of Chemical Physics and Biophysics, Tallinn, Estonia
24,
C Veelken
C Veelken
24National Institute of Chemical Physics and Biophysics, Tallinn, Estonia
24,
P Eerola
P Eerola
25Department of Physics, University of Helsinki, Helsinki, Finland
25,
J Pekkanen
J Pekkanen
25Department of Physics, University of Helsinki, Helsinki, Finland
25,
M Voutilainen
M Voutilainen
25Department of Physics, University of Helsinki, Helsinki, Finland
25,
J Härkönen
J Härkönen
26Helsinki Institute of Physics, Helsinki, Finland
26,
V Karimäki
V Karimäki
26Helsinki Institute of Physics, Helsinki, Finland
26,
R Kinnunen
R Kinnunen
26Helsinki Institute of Physics, Helsinki, Finland
26,
T Lampén
T Lampén
26Helsinki Institute of Physics, Helsinki, Finland
26,
K Lassila-Perini
K Lassila-Perini
26Helsinki Institute of Physics, Helsinki, Finland
26,
S Lehti
S Lehti
26Helsinki Institute of Physics, Helsinki, Finland
26,
T Lindén
T Lindén
26Helsinki Institute of Physics, Helsinki, Finland
26,
P Luukka
P Luukka
26Helsinki Institute of Physics, Helsinki, Finland
26,
T Mäenpää
T Mäenpää
26Helsinki Institute of Physics, Helsinki, Finland
26,
T Peltola
T Peltola
26Helsinki Institute of Physics, Helsinki, Finland
26,
E Tuominen
E Tuominen
26Helsinki Institute of Physics, Helsinki, Finland
26,
J Tuominiemi
J Tuominiemi
26Helsinki Institute of Physics, Helsinki, Finland
26,
E Tuovinen
E Tuovinen
26Helsinki Institute of Physics, Helsinki, Finland
26,
L Wendland
L Wendland
26Helsinki Institute of Physics, Helsinki, Finland
26,
J Talvitie
J Talvitie
27Lappeenranta University of Technology, Lappeenranta, Finland
27,
T Tuuva
T Tuuva
27Lappeenranta University of Technology, Lappeenranta, Finland
27,
M Besancon
M Besancon
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
F Couderc
F Couderc
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
M Dejardin
M Dejardin
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
D Denegri
D Denegri
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
B Fabbro
B Fabbro
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
J L Faure
J L Faure
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
C Favaro
C Favaro
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
F Ferri
F Ferri
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
S Ganjour
S Ganjour
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
A Givernaud
A Givernaud
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
P Gras
P Gras
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
G Hamel de Monchenault
G Hamel de Monchenault
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
P Jarry
P Jarry
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
E Locci
E Locci
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
M Machet
M Machet
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
J Malcles
J Malcles
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
J Rander
J Rander
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
A Rosowsky
A Rosowsky
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
M Titov
M Titov
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
A Zghiche
A Zghiche
28DSM/IRFU, CEA/Saclay, Gif-sur-Yvette, France
28,
I Antropov
I Antropov
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
S Baffioni
S Baffioni
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
F Beaudette
F Beaudette
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
P Busson
P Busson
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
L Cadamuro
L Cadamuro
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
E Chapon
E Chapon
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
C Charlot
C Charlot
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
T Dahms
T Dahms
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
O Davignon
O Davignon
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
N Filipovic
N Filipovic
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
R Granier de Cassagnac
R Granier de Cassagnac
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
M Jo
M Jo
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
S Lisniak
S Lisniak
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
L Mastrolorenzo
L Mastrolorenzo
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
P Miné
P Miné
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
I N Naranjo
I N Naranjo
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
M Nguyen
M Nguyen
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
C Ochando
C Ochando
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
G Ortona
G Ortona
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
P Paganini
P Paganini
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
P Pigard
P Pigard
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
S Regnard
S Regnard
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
R Salerno
R Salerno
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
J B Sauvan
J B Sauvan
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
Y Sirois
Y Sirois
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
T Strebler
T Strebler
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
Y Yilmaz
Y Yilmaz
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
A Zabi
A Zabi
29Laboratoire Leprince-Ringuet, Ecole Polytechnique, IN2P3-CNRS, Palaiseau, France
29,
J-L Agram
J-L Agram
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
J Andrea
J Andrea
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
A Aubin
A Aubin
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
D Bloch
D Bloch
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
J-M Brom
J-M Brom
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
M Buttignol
M Buttignol
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
E C Chabert
E C Chabert
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
N Chanon
N Chanon
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
C Collard
C Collard
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
E Conte
E Conte
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
X Coubez
X Coubez
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
J-C Fontaine
J-C Fontaine
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
D Gelé
D Gelé
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
U Goerlach
U Goerlach
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
C Goetzmann
C Goetzmann
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
A-C Le Bihan
A-C Le Bihan
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
J A Merlin
J A Merlin
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
K Skovpen
K Skovpen
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
P Van Hove
P Van Hove
30Institut Pluridisciplinaire Hubert Curien, Université de Strasbourg, Université de Haute Alsace Mulhouse, CNRS/IN2P3, Strasbourg, France
30,
S Gadrat
S Gadrat
31Centre de Calcul de l’Institut National de Physique Nucleaire et de Physique des Particules, CNRS/IN2P3, Villeurbanne, France
31,
S Beauceron
S Beauceron
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
C Bernet
C Bernet
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
G Boudoul
G Boudoul
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
E Bouvier
E Bouvier
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
C A Carrillo Montoya
C A Carrillo Montoya
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
R Chierici
R Chierici
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
D Contardo
D Contardo
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
B Courbon
B Courbon
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
P Depasse
P Depasse
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
H El Mamouni
H El Mamouni
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
J Fan
J Fan
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
J Fay
J Fay
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
S Gascon
S Gascon
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
M Gouzevitch
M Gouzevitch
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
B Ille
B Ille
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
F Lagarde
F Lagarde
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
I B Laktineh
I B Laktineh
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
M Lethuillier
M Lethuillier
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
L Mirabito
L Mirabito
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
A L Pequegnot
A L Pequegnot
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
S Perries
S Perries
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
J D Ruiz Alvarez
J D Ruiz Alvarez
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
D Sabes
D Sabes
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
L Sgandurra
L Sgandurra
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
V Sordini
V Sordini
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
M Vander Donckt
M Vander Donckt
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
P Verdier
P Verdier
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
S Viret
S Viret
32Institut de Physique Nucléaire de Lyon, Université de Lyon, Université Claude Bernard Lyon 1, CNRS-IN2P3, Villeurbanne, France
32,
T Toriashvili
T Toriashvili
33Georgian Technical University, Tbilisi, Georgia
33,
D Lomidze
D Lomidze
34Tbilisi State University, Tbilisi, Georgia
34,
C Autermann
C Autermann
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
S Beranek
S Beranek
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
M Edelhoff
M Edelhoff
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
L Feld
L Feld
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
A Heister
A Heister
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
M K Kiesel
M K Kiesel
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
K Klein
K Klein
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
M Lipinski
M Lipinski
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
A Ostapchuk
A Ostapchuk
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
M Preuten
M Preuten
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
F Raupach
F Raupach
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
S Schael
S Schael
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
J F Schulte
J F Schulte
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
T Verlage
T Verlage
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
H Weber
H Weber
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
B Wittmer
B Wittmer
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
V Zhukov
V Zhukov
35I. Physikalisches Institut, RWTH Aachen University, Aachen, Germany
35,
M Ata
M Ata
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
M Brodski
M Brodski
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
E Dietz-Laursonn
E Dietz-Laursonn
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
D Duchardt
D Duchardt
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
M Endres
M Endres
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
M Erdmann
M Erdmann
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
S Erdweg
S Erdweg
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
T Esch
T Esch
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
R Fischer
R Fischer
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
A Güth
A Güth
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
T Hebbeker
T Hebbeker
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
C Heidemann
C Heidemann
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
K Hoepfner
K Hoepfner
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
S Knutzen
S Knutzen
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
P Kreuzer
P Kreuzer
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
M Merschmeyer
M Merschmeyer
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
A Meyer
A Meyer
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
P Millet
P Millet
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
M Olschewski
M Olschewski
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
K Padeken
K Padeken
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
P Papacz
P Papacz
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
T Pook
T Pook
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
M Radziej
M Radziej
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
H Reithler
H Reithler
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
M Rieger
M Rieger
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
F Scheuch
F Scheuch
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
L Sonnenschein
L Sonnenschein
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
D Teyssier
D Teyssier
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
S Thüer
S Thüer
36III. Physikalisches Institut A, RWTH Aachen University, Aachen, Germany
36,
V Cherepanov
V Cherepanov
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
Y Erdogan
Y Erdogan
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
G Flügge
G Flügge
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
H Geenen
H Geenen
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
M Geisler
M Geisler
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
F Hoehle
F Hoehle
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
B Kargoll
B Kargoll
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
T Kress
T Kress
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
Y Kuessel
Y Kuessel
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
A Künsken
A Künsken
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
J Lingemann
J Lingemann
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
A Nehrkorn
A Nehrkorn
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
A Nowack
A Nowack
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
I M Nugent
I M Nugent
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
C Pistone
C Pistone
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
O Pooth
O Pooth
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
A Stahl
A Stahl
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
M Aldaya Martin
M Aldaya Martin
37III. Physikalisches Institut B, RWTH Aachen University, Aachen, Germany
37,
I Asin
I Asin
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
N Bartosik
N Bartosik
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
O Behnke
O Behnke
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
U Behrens
U Behrens
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A J Bell
A J Bell
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
K Borras
K Borras
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Burgmeier
A Burgmeier
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Campbell
A Campbell
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
S Choudhury
S Choudhury
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
F Costanza
F Costanza
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
C Diez Pardos
C Diez Pardos
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
G Dolinska
G Dolinska
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
S Dooling
S Dooling
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
T Dorland
T Dorland
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
G Eckerlin
G Eckerlin
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
D Eckstein
D Eckstein
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
T Eichhorn
T Eichhorn
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
G Flucke
G Flucke
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
E Gallo
E Gallo
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
J Garay Garcia
J Garay Garcia
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Geiser
A Geiser
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Gizhko
A Gizhko
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
P Gunnellini
P Gunnellini
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
J Hauk
J Hauk
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
M Hempel
M Hempel
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
H Jung
H Jung
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Kalogeropoulos
A Kalogeropoulos
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
O Karacheban
O Karacheban
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
M Kasemann
M Kasemann
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
P Katsas
P Katsas
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
J Kieseler
J Kieseler
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
C Kleinwort
C Kleinwort
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
I Korol
I Korol
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
W Lange
W Lange
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
J Leonard
J Leonard
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
K Lipka
K Lipka
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Lobanov
A Lobanov
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
W Lohmann
W Lohmann
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
R Mankel
R Mankel
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
I Marfin
I Marfin
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
I-A Melzer-Pellmann
I-A Melzer-Pellmann
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A B Meyer
A B Meyer
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
G Mittag
G Mittag
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
J Mnich
J Mnich
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Mussgiller
A Mussgiller
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
S Naumann-Emme
S Naumann-Emme
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Nayak
A Nayak
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
E Ntomari
E Ntomari
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
H Perrey
H Perrey
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
D Pitzl
D Pitzl
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
R Placakyte
R Placakyte
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
A Raspereza
A Raspereza
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
B Roland
B Roland
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
M Ö Sahin
M Ö Sahin
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
P Saxena
P Saxena
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
T Schoerner-Sadenius
T Schoerner-Sadenius
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
M Schröder
M Schröder
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
C Seitz
C Seitz
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
S Spannagel
S Spannagel
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
K D Trippkewitz
K D Trippkewitz
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
R Walsh
R Walsh
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
C Wissing
C Wissing
38Deutsches Elektronen-Synchrotron, Hamburg, Germany
38,
V Blobel
V Blobel
39University of Hamburg, Hamburg, Germany
39,
M Centis Vignali
M Centis Vignali
39University of Hamburg, Hamburg, Germany
39,
A R Draeger
A R Draeger
39University of Hamburg, Hamburg, Germany
39,
J Erfle
J Erfle
39University of Hamburg, Hamburg, Germany
39,
E Garutti
E Garutti
39University of Hamburg, Hamburg, Germany
39,
K Goebel
K Goebel
39University of Hamburg, Hamburg, Germany
39,
D Gonzalez
D Gonzalez
39University of Hamburg, Hamburg, Germany
39,
M Görner
M Görner
39University of Hamburg, Hamburg, Germany
39,
J Haller
J Haller
39University of Hamburg, Hamburg, Germany
39,
M Hoffmann
M Hoffmann
39University of Hamburg, Hamburg, Germany
39,
R S Höing
R S Höing
39University of Hamburg, Hamburg, Germany
39,
A Junkes
A Junkes
39University of Hamburg, Hamburg, Germany
39,
R Klanner
R Klanner
39University of Hamburg, Hamburg, Germany
39,
R Kogler
R Kogler
39University of Hamburg, Hamburg, Germany
39,
N Kovalchuk
N Kovalchuk
39University of Hamburg, Hamburg, Germany
39,
T Lapsien
T Lapsien
39University of Hamburg, Hamburg, Germany
39,
T Lenz
T Lenz
39University of Hamburg, Hamburg, Germany
39,
I Marchesini
I Marchesini
39University of Hamburg, Hamburg, Germany
39,
D Marconi
D Marconi
39University of Hamburg, Hamburg, Germany
39,
M Meyer
M Meyer
39University of Hamburg, Hamburg, Germany
39,
D Nowatschin
D Nowatschin
39University of Hamburg, Hamburg, Germany
39,
J Ott
J Ott
39University of Hamburg, Hamburg, Germany
39,
F Pantaleo
F Pantaleo
39University of Hamburg, Hamburg, Germany
39,
T Peiffer
T Peiffer
39University of Hamburg, Hamburg, Germany
39,
A Perieanu
A Perieanu
39University of Hamburg, Hamburg, Germany
39,
N Pietsch
N Pietsch
39University of Hamburg, Hamburg, Germany
39,
J Poehlsen
J Poehlsen
39University of Hamburg, Hamburg, Germany
39,
D Rathjens
D Rathjens
39University of Hamburg, Hamburg, Germany
39,
C Sander
C Sander
39University of Hamburg, Hamburg, Germany
39,
C Scharf
C Scharf
39University of Hamburg, Hamburg, Germany
39,
H Schettler
H Schettler
39University of Hamburg, Hamburg, Germany
39,
P Schleper
P Schleper
39University of Hamburg, Hamburg, Germany
39,
E Schlieckau
E Schlieckau
39University of Hamburg, Hamburg, Germany
39,
A Schmidt
A Schmidt
39University of Hamburg, Hamburg, Germany
39,
J Schwandt
J Schwandt
39University of Hamburg, Hamburg, Germany
39,
V Sola
V Sola
39University of Hamburg, Hamburg, Germany
39,
H Stadie
H Stadie
39University of Hamburg, Hamburg, Germany
39,
G Steinbrück
G Steinbrück
39University of Hamburg, Hamburg, Germany
39,
H Tholen
H Tholen
39University of Hamburg, Hamburg, Germany
39,
D Troendle
D Troendle
39University of Hamburg, Hamburg, Germany
39,
E Usai
E Usai
39University of Hamburg, Hamburg, Germany
39,
L Vanelderen
L Vanelderen
39University of Hamburg, Hamburg, Germany
39,
A Vanhoefer
A Vanhoefer
39University of Hamburg, Hamburg, Germany
39,
B Vormwald
B Vormwald
39University of Hamburg, Hamburg, Germany
39,
C Barth
C Barth
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
C Baus
C Baus
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
J Berger
J Berger
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
C Böser
C Böser
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
E Butz
E Butz
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
T Chwalek
T Chwalek
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
F Colombo
F Colombo
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
W De Boer
W De Boer
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
A Descroix
A Descroix
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
A Dierlamm
A Dierlamm
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
S Fink
S Fink
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
F Frensch
F Frensch
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
R Friese
R Friese
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
M Giffels
M Giffels
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
A Gilbert
A Gilbert
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
D Haitz
D Haitz
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
F Hartmann
F Hartmann
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
S M Heindl
S M Heindl
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
U Husemann
U Husemann
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
I Katkov
I Katkov
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
A Kornmayer
A Kornmayer
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
P Lobelle Pardo
P Lobelle Pardo
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
B Maier
B Maier
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
H Mildner
H Mildner
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
M U Mozer
M U Mozer
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
T Müller
T Müller
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
Th Müller
Th Müller
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
M Plagge
M Plagge
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
G Quast
G Quast
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
K Rabbertz
K Rabbertz
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
S Röcker
S Röcker
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
F Roscher
F Roscher
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
G Sieber
G Sieber
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
H J Simonis
H J Simonis
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
F M Stober
F M Stober
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
R Ulrich
R Ulrich
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
J Wagner-Kuhr
J Wagner-Kuhr
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
S Wayand
S Wayand
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
M Weber
M Weber
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
T Weiler
T Weiler
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
S Williamson
S Williamson
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
C Wöhrmann
C Wöhrmann
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
R Wolf
R Wolf
40Institut für Experimentelle Kernphysik, Karlsruhe, Germany
40,
G Anagnostou
G Anagnostou
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
G Daskalakis
G Daskalakis
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
T Geralis
T Geralis
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
V A Giakoumopoulou
V A Giakoumopoulou
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
A Kyriakis
A Kyriakis
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
D Loukas
D Loukas
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
A Psallidas
A Psallidas
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
I Topsis-Giotis
I Topsis-Giotis
41Institute of Nuclear and Particle Physics (INPP), NCSR Demokritos, Aghia Paraskevi, Greece
41,
A Agapitos
A Agapitos
42University of Athens, Athens, Greece
42,
S Kesisoglou
S Kesisoglou
42University of Athens, Athens, Greece
42,
A Panagiotou
A Panagiotou
42University of Athens, Athens, Greece
42,
N Saoulidou
N Saoulidou
42University of Athens, Athens, Greece
42,
E Tziaferi
E Tziaferi
42University of Athens, Athens, Greece
42,
I Evangelou
I Evangelou
43University of Ioánnina, Ioánnina, Greece
43,
G Flouris
G Flouris
43University of Ioánnina, Ioánnina, Greece
43,
C Foudas
C Foudas
43University of Ioánnina, Ioánnina, Greece
43,
P Kokkas
P Kokkas
43University of Ioánnina, Ioánnina, Greece
43,
N Loukas
N Loukas
43University of Ioánnina, Ioánnina, Greece
43,
N Manthos
N Manthos
43University of Ioánnina, Ioánnina, Greece
43,
I Papadopoulos
I Papadopoulos
43University of Ioánnina, Ioánnina, Greece
43,
E Paradas
E Paradas
43University of Ioánnina, Ioánnina, Greece
43,
J Strologas
J Strologas
43University of Ioánnina, Ioánnina, Greece
43,
G Bencze
G Bencze
44Wigner Research Centre for Physics, Budapest, Hungary
44,
C Hajdu
C Hajdu
44Wigner Research Centre for Physics, Budapest, Hungary
44,
A Hazi
A Hazi
44Wigner Research Centre for Physics, Budapest, Hungary
44,
P Hidas
P Hidas
44Wigner Research Centre for Physics, Budapest, Hungary
44,
D Horvath
D Horvath
44Wigner Research Centre for Physics, Budapest, Hungary
44,
F Sikler
F Sikler
44Wigner Research Centre for Physics, Budapest, Hungary
44,
V Veszpremi
V Veszpremi
44Wigner Research Centre for Physics, Budapest, Hungary
44,
G Vesztergombi
G Vesztergombi
44Wigner Research Centre for Physics, Budapest, Hungary
44,
A J Zsigmond
A J Zsigmond
44Wigner Research Centre for Physics, Budapest, Hungary
44,
N Beni
N Beni
45Institute of Nuclear Research ATOMKI, Debrecen, Hungary
45,
S Czellar
S Czellar
45Institute of Nuclear Research ATOMKI, Debrecen, Hungary
45,
J Karancsi
J Karancsi
45Institute of Nuclear Research ATOMKI, Debrecen, Hungary
45,
J Molnar
J Molnar
45Institute of Nuclear Research ATOMKI, Debrecen, Hungary
45,
Z Szillasi
Z Szillasi
45Institute of Nuclear Research ATOMKI, Debrecen, Hungary
45,
M Bartók
M Bartók
45Institute of Nuclear Research ATOMKI, Debrecen, Hungary
45,
A Makovec
A Makovec
46University of Debrecen, Debrecen, Hungary
46,
P Raics
P Raics
46University of Debrecen, Debrecen, Hungary
46,
Z L Trocsanyi
Z L Trocsanyi
46University of Debrecen, Debrecen, Hungary
46,
B Ujvari
B Ujvari
46University of Debrecen, Debrecen, Hungary
46,
P Mal
P Mal
47National Institute of Science Education and Research, Bhubaneswar, India
47,
K Mandal
K Mandal
47National Institute of Science Education and Research, Bhubaneswar, India
47,
D K Sahoo
D K Sahoo
47National Institute of Science Education and Research, Bhubaneswar, India
47,
N Sahoo
N Sahoo
47National Institute of Science Education and Research, Bhubaneswar, India
47,
S K Swain
S K Swain
47National Institute of Science Education and Research, Bhubaneswar, India
47,
S Bansal
S Bansal
47National Institute of Science Education and Research, Bhubaneswar, India
47,
S B Beri
S B Beri
48Panjab University, Chandigarh, India
48,
V Bhatnagar
V Bhatnagar
48Panjab University, Chandigarh, India
48,
R Chawla
R Chawla
48Panjab University, Chandigarh, India
48,
R Gupta
R Gupta
48Panjab University, Chandigarh, India
48,
U Bhawandeep
U Bhawandeep
48Panjab University, Chandigarh, India
48,
A K Kalsi
A K Kalsi
48Panjab University, Chandigarh, India
48,
A Kaur
A Kaur
48Panjab University, Chandigarh, India
48,
M Kaur
M Kaur
48Panjab University, Chandigarh, India
48,
R Kumar
R Kumar
48Panjab University, Chandigarh, India
48,
A Mehta
A Mehta
48Panjab University, Chandigarh, India
48,
M Mittal
M Mittal
48Panjab University, Chandigarh, India
48,
J B Singh
J B Singh
48Panjab University, Chandigarh, India
48,
G Walia
G Walia
48Panjab University, Chandigarh, India
48,
Ashok Kumar
Ashok Kumar
49University of Delhi, Delhi, India
49,
A Bhardwaj
A Bhardwaj
49University of Delhi, Delhi, India
49,
B C Choudhary
B C Choudhary
49University of Delhi, Delhi, India
49,
R B Garg
R B Garg
49University of Delhi, Delhi, India
49,
A Kumar
A Kumar
49University of Delhi, Delhi, India
49,
S Malhotra
S Malhotra
49University of Delhi, Delhi, India
49,
M Naimuddin
M Naimuddin
49University of Delhi, Delhi, India
49,
N Nishu
N Nishu
49University of Delhi, Delhi, India
49,
K Ranjan
K Ranjan
49University of Delhi, Delhi, India
49,
R Sharma
R Sharma
49University of Delhi, Delhi, India
49,
V Sharma
V Sharma
49University of Delhi, Delhi, India
49,
S Bhattacharya
S Bhattacharya
50Saha Institute of Nuclear Physics, Kolkata, India
50,
K Chatterjee
K Chatterjee
50Saha Institute of Nuclear Physics, Kolkata, India
50,
S Dey
S Dey
50Saha Institute of Nuclear Physics, Kolkata, India
50,
S Dutta
S Dutta
50Saha Institute of Nuclear Physics, Kolkata, India
50,
Sa Jain
Sa Jain
50Saha Institute of Nuclear Physics, Kolkata, India
50,
N Majumdar
N Majumdar
50Saha Institute of Nuclear Physics, Kolkata, India
50,
A Modak
A Modak
50Saha Institute of Nuclear Physics, Kolkata, India
50,
K Mondal
K Mondal
50Saha Institute of Nuclear Physics, Kolkata, India
50,
S Mukherjee
S Mukherjee
50Saha Institute of Nuclear Physics, Kolkata, India
50,
S Mukhopadhyay
S Mukhopadhyay
50Saha Institute of Nuclear Physics, Kolkata, India
50,
A Roy
A Roy
50Saha Institute of Nuclear Physics, Kolkata, India
50,
D Roy
D Roy
50Saha Institute of Nuclear Physics, Kolkata, India
50,
S Roy Chowdhury
S Roy Chowdhury
50Saha Institute of Nuclear Physics, Kolkata, India
50,
S Sarkar
S Sarkar
50Saha Institute of Nuclear Physics, Kolkata, India
50,
M Sharan
M Sharan
50Saha Institute of Nuclear Physics, Kolkata, India
50,
A Abdulsalam
A Abdulsalam
51Bhabha Atomic Research Centre, Mumbai, India
51,
R Chudasama
R Chudasama
51Bhabha Atomic Research Centre, Mumbai, India
51,
D Dutta
D Dutta
51Bhabha Atomic Research Centre, Mumbai, India
51,
V Jha
V Jha
51Bhabha Atomic Research Centre, Mumbai, India
51,
V Kumar
V Kumar
51Bhabha Atomic Research Centre, Mumbai, India
51,
A K Mohanty
A K Mohanty
51Bhabha Atomic Research Centre, Mumbai, India
51,
L M Pant
L M Pant
51Bhabha Atomic Research Centre, Mumbai, India
51,
P Shukla
P Shukla
51Bhabha Atomic Research Centre, Mumbai, India
51,
A Topkar
A Topkar
51Bhabha Atomic Research Centre, Mumbai, India
51,
T Aziz
T Aziz
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Banerjee
S Banerjee
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Bhowmik
S Bhowmik
52Tata Institute of Fundamental Research, Mumbai, India
52,
R M Chatterjee
R M Chatterjee
52Tata Institute of Fundamental Research, Mumbai, India
52,
R K Dewanjee
R K Dewanjee
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Dugad
S Dugad
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Ganguly
S Ganguly
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Ghosh
S Ghosh
52Tata Institute of Fundamental Research, Mumbai, India
52,
M Guchait
M Guchait
52Tata Institute of Fundamental Research, Mumbai, India
52,
A Gurtu
A Gurtu
52Tata Institute of Fundamental Research, Mumbai, India
52,
G Kole
G Kole
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Kumar
S Kumar
52Tata Institute of Fundamental Research, Mumbai, India
52,
B Mahakud
B Mahakud
52Tata Institute of Fundamental Research, Mumbai, India
52,
M Maity
M Maity
52Tata Institute of Fundamental Research, Mumbai, India
52,
G Majumder
G Majumder
52Tata Institute of Fundamental Research, Mumbai, India
52,
K Mazumdar
K Mazumdar
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Mitra
S Mitra
52Tata Institute of Fundamental Research, Mumbai, India
52,
G B Mohanty
G B Mohanty
52Tata Institute of Fundamental Research, Mumbai, India
52,
B Parida
B Parida
52Tata Institute of Fundamental Research, Mumbai, India
52,
T Sarkar
T Sarkar
52Tata Institute of Fundamental Research, Mumbai, India
52,
N Sur
N Sur
52Tata Institute of Fundamental Research, Mumbai, India
52,
B Sutar
B Sutar
52Tata Institute of Fundamental Research, Mumbai, India
52,
N Wickramage
N Wickramage
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Chauhan
S Chauhan
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Dube
S Dube
52Tata Institute of Fundamental Research, Mumbai, India
52,
A Kapoor
A Kapoor
52Tata Institute of Fundamental Research, Mumbai, India
52,
K Kothekar
K Kothekar
52Tata Institute of Fundamental Research, Mumbai, India
52,
S Sharma
S Sharma
53Indian Institute of Science Education and Research (IISER), Pune, India
53,
H Bakhshiansohi
H Bakhshiansohi
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
H Behnamian
H Behnamian
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
S M Etesami
S M Etesami
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
A Fahim
A Fahim
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
R Goldouzian
R Goldouzian
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
M Khakzad
M Khakzad
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
M Mohammadi Najafabadi
M Mohammadi Najafabadi
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
M Naseri
M Naseri
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
S Paktinat Mehdiabadi
S Paktinat Mehdiabadi
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
F Rezaei Hosseinabadi
F Rezaei Hosseinabadi
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
B Safarzadeh
B Safarzadeh
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
M Zeinali
M Zeinali
54Institute for Research in Fundamental Sciences (IPM), Tehran, Iran
54,
M Felcini
M Felcini
55University College Dublin, Dublin, Ireland
55,
M Grunewald
M Grunewald
55University College Dublin, Dublin, Ireland
55,
M Abbrescia
M Abbrescia
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
C Calabria
C Calabria
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
C Caputo
C Caputo
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
A Colaleo
A Colaleo
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
D Creanza
D Creanza
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
L Cristella
L Cristella
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
N De Filippis
N De Filippis
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
M De Palma
M De Palma
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
L Fiore
L Fiore
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
G Iaselli
G Iaselli
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
G Maggi
G Maggi
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
G Miniello
G Miniello
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
M Maggi
M Maggi
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
S My
S My
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
S Nuzzo
S Nuzzo
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
A Pompili
A Pompili
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
G Pugliese
G Pugliese
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
R Radogna
R Radogna
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
A Ranieri
A Ranieri
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
G Selvaggi
G Selvaggi
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
L Silvestris
L Silvestris
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
R Venditti
R Venditti
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
P Verwilligen
P Verwilligen
56INFN Sezione di Bari, Università di Bari, Politecnico di Bari, Bari, Italy
56,
G Abbiendi
G Abbiendi
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
C Battilana
C Battilana
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
A C Benvenuti
A C Benvenuti
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
D Bonacorsi
D Bonacorsi
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
S Braibant-Giacomelli
S Braibant-Giacomelli
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
L Brigliadori
L Brigliadori
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
R Campanini
R Campanini
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
P Capiluppi
P Capiluppi
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
A Castro
A Castro
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
F R Cavallo
F R Cavallo
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
S S Chhibra
S S Chhibra
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
G Codispoti
G Codispoti
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
M Cuffiani
M Cuffiani
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
G M Dallavalle
G M Dallavalle
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
F Fabbri
F Fabbri
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
A Fanfani
A Fanfani
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
D Fasanella
D Fasanella
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
P Giacomelli
P Giacomelli
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
C Grandi
C Grandi
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
L Guiducci
L Guiducci
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
S Marcellini
S Marcellini
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
G Masetti
G Masetti
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
A Montanari
A Montanari
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
F L Navarria
F L Navarria
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
A Perrotta
A Perrotta
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
A M Rossi
A M Rossi
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
F Primavera
F Primavera
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
T Rovelli
T Rovelli
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
G P Siroli
G P Siroli
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
N Tosi
N Tosi
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
R Travaglini
R Travaglini
57INFN Sezione di Bologna, Università di Bologna, Bologna, Italy
57,
G Cappello
G Cappello
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
M Chiorboli
M Chiorboli
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
S Costa
S Costa
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
A Di Mattia
A Di Mattia
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
F Giordano
F Giordano
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
R Potenza
R Potenza
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
A Tricomi
A Tricomi
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
C Tuve
C Tuve
58INFN Sezione di Catania, Università di Catania, Catania, Italy
58,
G Barbagli
G Barbagli
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
V Ciulli
V Ciulli
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
C Civinini
C Civinini
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
R D’Alessandro
R D’Alessandro
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
E Focardi
E Focardi
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
S Gonzi
S Gonzi
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
V Gori
V Gori
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
P Lenzi
P Lenzi
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
M Meschini
M Meschini
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
S Paoletti
S Paoletti
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
G Sguazzoni
G Sguazzoni
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
A Tropiano
A Tropiano
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
L Viliani
L Viliani
59INFN Sezione di Firenze, Università di Firenze, Florence, Italy
59,
L Benussi
L Benussi
60INFN Laboratori Nazionali di Frascati, Frascati, Italy
60,
S Bianco
S Bianco
60INFN Laboratori Nazionali di Frascati, Frascati, Italy
60,
F Fabbri
F Fabbri
60INFN Laboratori Nazionali di Frascati, Frascati, Italy
60,
D Piccolo
D Piccolo
60INFN Laboratori Nazionali di Frascati, Frascati, Italy
60,
F Primavera
F Primavera
60INFN Laboratori Nazionali di Frascati, Frascati, Italy
60,
V Calvelli
V Calvelli
61INFN Sezione di Genova, Università di Genova, Genoa, Italy
61,
F Ferro
F Ferro
61INFN Sezione di Genova, Università di Genova, Genoa, Italy
61,
M Lo Vetere
M Lo Vetere
61INFN Sezione di Genova, Università di Genova, Genoa, Italy
61,
M R Monge
M R Monge
61INFN Sezione di Genova, Università di Genova, Genoa, Italy
61,
E Robutti
E Robutti
61INFN Sezione di Genova, Università di Genova, Genoa, Italy
61,
S Tosi
S Tosi
61INFN Sezione di Genova, Università di Genova, Genoa, Italy
61,
L Brianza
L Brianza
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
M E Dinardo
M E Dinardo
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
S Fiorendi
S Fiorendi
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
S Gennai
S Gennai
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
R Gerosa
R Gerosa
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
A Ghezzi
A Ghezzi
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
P Govoni
P Govoni
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
S Malvezzi
S Malvezzi
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
R A Manzoni
R A Manzoni
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
B Marzocchi
B Marzocchi
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
D Menasce
D Menasce
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
L Moroni
L Moroni
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
M Paganoni
M Paganoni
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
D Pedrini
D Pedrini
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
S Ragazzi
S Ragazzi
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
N Redaelli
N Redaelli
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
T Tabarelli de Fatis
T Tabarelli de Fatis
62INFN Sezione di Milano-Bicocca, Università di Milano-Bicocca, Milan, Italy
62,
S Buontempo
S Buontempo
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
N Cavallo
N Cavallo
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
S Di Guida
S Di Guida
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
M Esposito
M Esposito
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
F Fabozzi
F Fabozzi
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
A O M Iorio
A O M Iorio
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
G Lanza
G Lanza
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
L Lista
L Lista
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
S Meola
S Meola
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
M Merola
M Merola
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
P Paolucci
P Paolucci
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
C Sciacca
C Sciacca
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
F Thyssen
F Thyssen
63INFN Sezione di Napoli, Università di Napoli ‘Federico II’, Napoli, Italy, Università della Basilicata, Potenza, Italy, Università G. Marconi, Rome, Italy
63,
P Azzi
P Azzi
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
N Bacchetta
N Bacchetta
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
L Benato
L Benato
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
D Bisello
D Bisello
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
A Boletti
A Boletti
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
A Branca
A Branca
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
R Carlin
R Carlin
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
P Checchia
P Checchia
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
M Dall’Osso
M Dall’Osso
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
T Dorigo
T Dorigo
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
U Dosselli
U Dosselli
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
S Fantinel
S Fantinel
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
F Fanzago
F Fanzago
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
F Gasparini
F Gasparini
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
U Gasparini
U Gasparini
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
A Gozzelino
A Gozzelino
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
K Kanishchev
K Kanishchev
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
S Lacaprara
S Lacaprara
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
M Margoni
M Margoni
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
A T Meneguzzo
A T Meneguzzo
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
J Pazzini
J Pazzini
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
N Pozzobon
N Pozzobon
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
P Ronchese
P Ronchese
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
F Simonetto
F Simonetto
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
E Torassa
E Torassa
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
M Tosi
M Tosi
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
M Zanetti
M Zanetti
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
P Zotto
P Zotto
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
A Zucchetta
A Zucchetta
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
A Braghieri
A Braghieri
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
A Magnani
A Magnani
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
P Montagna
P Montagna
64INFN Sezione di Padova, Università di Padova, Padova, Italy, Università di Trento, Trento, Italy
64,
S P Ratti
S P Ratti
65INFN Sezione di Pavia, Università di Pavia, Pavia, Italy
65,
V Re
V Re
65INFN Sezione di Pavia, Università di Pavia, Pavia, Italy
65,
C Riccardi
C Riccardi
65INFN Sezione di Pavia, Università di Pavia, Pavia, Italy
65,
P Salvini
P Salvini
65INFN Sezione di Pavia, Università di Pavia, Pavia, Italy
65,
I Vai
I Vai
65INFN Sezione di Pavia, Università di Pavia, Pavia, Italy
65,
P Vitulo
P Vitulo
65INFN Sezione di Pavia, Università di Pavia, Pavia, Italy
65,
L Alunni Solestizi
L Alunni Solestizi
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
G M Bilei
G M Bilei
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
D Ciangottini
D Ciangottini
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
L Fanò
L Fanò
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
P Lariccia
P Lariccia
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
G Mantovani
G Mantovani
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
M Menichelli
M Menichelli
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
A Saha
A Saha
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
A Santocchia
A Santocchia
66INFN Sezione di Perugia, Università di Perugia, Perugia, Italy
66,
K Androsov
K Androsov
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
P Azzurri
P Azzurri
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
G Bagliesi
G Bagliesi
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
J Bernardini
J Bernardini
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
T Boccali
T Boccali
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
R Castaldi
R Castaldi
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
M A Ciocci
M A Ciocci
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
R Dell’Orso
R Dell’Orso
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
S Donato
S Donato
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
G Fedi
G Fedi
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
F Fiori
F Fiori
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
L Foà
L Foà
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
A Giassi
A Giassi
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
M T Grippo
M T Grippo
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
F Ligabue
F Ligabue
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
T Lomtadze
T Lomtadze
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
L Martini
L Martini
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
A Messineo
A Messineo
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
F Palla
F Palla
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
A Rizzi
A Rizzi
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
A Savoy-Navarro
A Savoy-Navarro
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
A T Serban
A T Serban
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
P Spagnolo
P Spagnolo
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
R Tenchini
R Tenchini
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
G Tonelli
G Tonelli
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
A Venturi
A Venturi
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
P G Verdini
P G Verdini
67INFN Sezione di Pisa, Università di Pisa, Scuola Normale Superiore di Pisa, Pisa, Italy
67,
L Barone
L Barone
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
F Cavallari
F Cavallari
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
G D’imperio
G D’imperio
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
D Del Re
D Del Re
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
M Diemoz
M Diemoz
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
S Gelli
S Gelli
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
C Jorda
C Jorda
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
E Longo
E Longo
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
F Margaroli
F Margaroli
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
P Meridiani
P Meridiani
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
G Organtini
G Organtini
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
R Paramatti
R Paramatti
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
F Preiato
F Preiato
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
S Rahatlou
S Rahatlou
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
C Rovelli
C Rovelli
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
F Santanastasio
F Santanastasio
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
P Traczyk
P Traczyk
68INFN Sezione di Roma, Università di Roma, Rome, Italy
68,
N Amapane
N Amapane
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
R Arcidiacono
R Arcidiacono
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
S Argiro
S Argiro
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
M Arneodo
M Arneodo
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
R Bellan
R Bellan
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
C Biino
C Biino
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
N Cartiglia
N Cartiglia
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
M Costa
M Costa
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
R Covarelli
R Covarelli
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
A Degano
A Degano
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
N Demaria
N Demaria
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
L Finco
L Finco
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
B Kiani
B Kiani
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
C Mariotti
C Mariotti
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
S Maselli
S Maselli
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
E Migliore
E Migliore
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
V Monaco
V Monaco
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
E Monteil
E Monteil
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
M M Obertino
M M Obertino
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
L Pacher
L Pacher
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
N Pastrone
N Pastrone
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
M Pelliccioni
M Pelliccioni
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
G L Pinna Angioni
G L Pinna Angioni
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
F Ravera
F Ravera
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
A Potenza
A Potenza
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
A Romero
A Romero
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
M Ruspa
M Ruspa
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
R Sacchi
R Sacchi
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
A Solano
A Solano
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
A Staiano
A Staiano
69INFN Sezione di Torino, Università di Torino, Torino, Italy, Università del Piemonte Orientale, Novara, Italy
69,
S Belforte
S Belforte
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
V Candelise
V Candelise
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
M Casarsa
M Casarsa
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
F Cossutti
F Cossutti
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
G Della Ricca
G Della Ricca
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
B Gobbo
B Gobbo
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
C La Licata
C La Licata
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
M Marone
M Marone
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
A Schizzi
A Schizzi
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
A Zanetti
A Zanetti
70INFN Sezione di Trieste, Università di Trieste, Trieste, Italy
70,
T A Kropivnitskaya
T A Kropivnitskaya
71Kangwon National University, Chunchon, Korea
71,
S K Nam
S K Nam
71Kangwon National University, Chunchon, Korea
71,
D H Kim
D H Kim
72Kyungpook National University, Daegu, Korea
72,
G N Kim
G N Kim
72Kyungpook National University, Daegu, Korea
72,
M S Kim
M S Kim
72Kyungpook National University, Daegu, Korea
72,
M S Kim
M S Kim
72Kyungpook National University, Daegu, Korea
72,
D J Kong
D J Kong
72Kyungpook National University, Daegu, Korea
72,
S Lee
S Lee
72Kyungpook National University, Daegu, Korea
72,
Y D Oh
Y D Oh
72Kyungpook National University, Daegu, Korea
72,
A Sakharov
A Sakharov
72Kyungpook National University, Daegu, Korea
72,
D C Son
D C Son
72Kyungpook National University, Daegu, Korea
72,
J A Brochero Cifuentes
J A Brochero Cifuentes
73Chonbuk National University, Jeonju, Korea
73,
H Kim
H Kim
73Chonbuk National University, Jeonju, Korea
73,
T J Kim
T J Kim
73Chonbuk National University, Jeonju, Korea
73,
S Song
S Song
74Chonnam National University, Institute for Universe and Elementary Particles, Kwangju, Korea
74,
S Choi
S Choi
75Korea University, Seoul, Korea
75,
Y Go
Y Go
75Korea University, Seoul, Korea
75,
D Gyun
D Gyun
75Korea University, Seoul, Korea
75,
B Hong
B Hong
75Korea University, Seoul, Korea
75,
H Kim
H Kim
75Korea University, Seoul, Korea
75,
Y Kim
Y Kim
75Korea University, Seoul, Korea
75,
B Lee
B Lee
75Korea University, Seoul, Korea
75,
K Lee
K Lee
75Korea University, Seoul, Korea
75,
K S Lee
K S Lee
75Korea University, Seoul, Korea
75,
S Lee
S Lee
75Korea University, Seoul, Korea
75,
S Lee
S Lee
75Korea University, Seoul, Korea
75,
S K Park
S K Park
75Korea University, Seoul, Korea
75,
Y Roh
Y Roh
75Korea University, Seoul, Korea
75,
H D Yoo
H D Yoo
76Seoul National University, Seoul, Korea
76,
M Choi
M Choi
77University of Seoul, Seoul, Korea
77,
H Kim
H Kim
77University of Seoul, Seoul, Korea
77,
J H Kim
J H Kim
77University of Seoul, Seoul, Korea
77,
J S H Lee
J S H Lee
77University of Seoul, Seoul, Korea
77,
I C Park
I C Park
77University of Seoul, Seoul, Korea
77,
G Ryu
G Ryu
77University of Seoul, Seoul, Korea
77,
M S Ryu
M S Ryu
77University of Seoul, Seoul, Korea
77,
Y Choi
Y Choi
78Sungkyunkwan University, Suwon, Korea
78,
J Goh
J Goh
78Sungkyunkwan University, Suwon, Korea
78,
D Kim
D Kim
78Sungkyunkwan University, Suwon, Korea
78,
E Kwon
E Kwon
78Sungkyunkwan University, Suwon, Korea
78,
J Lee
J Lee
78Sungkyunkwan University, Suwon, Korea
78,
I Yu
I Yu
78Sungkyunkwan University, Suwon, Korea
78,
V Dudenas
V Dudenas
78Sungkyunkwan University, Suwon, Korea
78,
A Juodagalvis
A Juodagalvis
79Vilnius University, Vilnius, Lithuania
79,
J Vaitkus
J Vaitkus
79Vilnius University, Vilnius, Lithuania
79,
I Ahmed
I Ahmed
79Vilnius University, Vilnius, Lithuania
79,
Z A Ibrahim
Z A Ibrahim
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
J R Komaragiri
J R Komaragiri
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
M A B Md Ali
M A B Md Ali
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
F Mohamad Idris
F Mohamad Idris
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
W A T Wan Abdullah
W A T Wan Abdullah
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
M N Yusli
M N Yusli
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
W A T Wan Abdullah
W A T Wan Abdullah
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
E Casimiro Linares
E Casimiro Linares
80National Centre for Particle Physics, Universiti Malaya, Kuala Lumpur, Malaysia
80,
H Castilla-Valdez
H Castilla-Valdez
81Centro de Investigacion y de Estudios Avanzados del IPN, Mexico City, Mexico
81,
E De La Cruz-Burelo
E De La Cruz-Burelo
81Centro de Investigacion y de Estudios Avanzados del IPN, Mexico City, Mexico
81,
I Heredia-De La Cruz
I Heredia-De La Cruz
81Centro de Investigacion y de Estudios Avanzados del IPN, Mexico City, Mexico
81,
A Hernandez-Almada
A Hernandez-Almada
81Centro de Investigacion y de Estudios Avanzados del IPN, Mexico City, Mexico
81,
R Lopez-Fernandez
R Lopez-Fernandez
81Centro de Investigacion y de Estudios Avanzados del IPN, Mexico City, Mexico
81,
A Sanchez-Hernandez
A Sanchez-Hernandez
81Centro de Investigacion y de Estudios Avanzados del IPN, Mexico City, Mexico
81,
S Carrillo Moreno
S Carrillo Moreno
82Universidad Iberoamericana, Mexico City, Mexico
82,
F Vazquez Valencia
F Vazquez Valencia
82Universidad Iberoamericana, Mexico City, Mexico
82,
I Pedraza
I Pedraza
83Benemerita Universidad Autonoma de Puebla, Puebla, Mexico
83,
H A Salazar Ibarguen
H A Salazar Ibarguen
83Benemerita Universidad Autonoma de Puebla, Puebla, Mexico
83,
A Morelos Pineda
A Morelos Pineda
84Universidad Autónoma de San Luis Potosí, San Luis Potosí, Mexico
84,
D Krofcheck
D Krofcheck
85University of Auckland, Auckland, New Zealand
85,
P H Butler
P H Butler
86University of Canterbury, Christchurch, New Zealand
86,
A Ahmad
A Ahmad
87National Centre for Physics, Quaid-I-Azam University, Islamabad, Pakistan
87,
M Ahmad
M Ahmad
87National Centre for Physics, Quaid-I-Azam University, Islamabad, Pakistan
87,
Q Hassan
Q Hassan
87National Centre for Physics, Quaid-I-Azam University, Islamabad, Pakistan
87,
H R Hoorani
H R Hoorani
87National Centre for Physics, Quaid-I-Azam University, Islamabad, Pakistan
87,
W A Khan
W A Khan
87National Centre for Physics, Quaid-I-Azam University, Islamabad, Pakistan
87,
T Khurshid
T Khurshid
87National Centre for Physics, Quaid-I-Azam University, Islamabad, Pakistan
87,
M Shoaib
M Shoaib
87National Centre for Physics, Quaid-I-Azam University, Islamabad, Pakistan
87,
H Bialkowska
H Bialkowska
88National Centre for Nuclear Research, Swierk, Poland
88,
M Bluj
M Bluj
88National Centre for Nuclear Research, Swierk, Poland
88,
B Boimska
B Boimska
88National Centre for Nuclear Research, Swierk, Poland
88,
T Frueboes
T Frueboes
88National Centre for Nuclear Research, Swierk, Poland
88,
M Górski
M Górski
88National Centre for Nuclear Research, Swierk, Poland
88,
M Kazana
M Kazana
88National Centre for Nuclear Research, Swierk, Poland
88,
K Nawrocki
K Nawrocki
88National Centre for Nuclear Research, Swierk, Poland
88,
K Romanowska-Rybinska
K Romanowska-Rybinska
88National Centre for Nuclear Research, Swierk, Poland
88,
M Szleper
M Szleper
88National Centre for Nuclear Research, Swierk, Poland
88,
P Zalewski
P Zalewski
88National Centre for Nuclear Research, Swierk, Poland
88,
G Brona
G Brona
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
K Bunkowski
K Bunkowski
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
A Byszuk
A Byszuk
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
K Doroba
K Doroba
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
A Kalinowski
A Kalinowski
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
M Konecki
M Konecki
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
J Krolikowski
J Krolikowski
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
M Misiura
M Misiura
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
M Olszewski
M Olszewski
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
M Walczak
M Walczak
89Institute of Experimental Physics, Faculty of Physics, University of Warsaw, Warsaw, Poland
89,
P Bargassa
P Bargassa
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
C Beir ao Da Cruz E Silva
C Beir ao Da Cruz E Silva
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
A Di Francesco
A Di Francesco
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
P Faccioli
P Faccioli
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
P G Ferreira Parracho
P G Ferreira Parracho
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
M Gallinaro
M Gallinaro
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
N Leonardo
N Leonardo
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
L Lloret Iglesias
L Lloret Iglesias
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
F Nguyen
F Nguyen
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
J Rodrigues Antunes
J Rodrigues Antunes
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
J Seixas
J Seixas
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
O Toldaiev
O Toldaiev
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
D Vadruccio
D Vadruccio
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
J Varela
J Varela
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
P Vischia
P Vischia
90Laboratório de Instrumentação e Física Experimental de Partículas, Lisbon, Portugal
90,
S Afanasiev
S Afanasiev
91Joint Institute for Nuclear Research, Dubna, Russia
91,
P Bunin
P Bunin
91Joint Institute for Nuclear Research, Dubna, Russia
91,
M Gavrilenko
M Gavrilenko
91Joint Institute for Nuclear Research, Dubna, Russia
91,
I Golutvin
I Golutvin
91Joint Institute for Nuclear Research, Dubna, Russia
91,
I Gorbunov
I Gorbunov
91Joint Institute for Nuclear Research, Dubna, Russia
91,
A Kamenev
A Kamenev
91Joint Institute for Nuclear Research, Dubna, Russia
91,
V Karjavin
V Karjavin
91Joint Institute for Nuclear Research, Dubna, Russia
91,
V Konoplyanikov
V Konoplyanikov
91Joint Institute for Nuclear Research, Dubna, Russia
91,
A Lanev
A Lanev
91Joint Institute for Nuclear Research, Dubna, Russia
91,
A Malakhov
A Malakhov
91Joint Institute for Nuclear Research, Dubna, Russia
91,
V Matveev
V Matveev
91Joint Institute for Nuclear Research, Dubna, Russia
91,
P Moisenz
P Moisenz
91Joint Institute for Nuclear Research, Dubna, Russia
91,
V Palichik
V Palichik
91Joint Institute for Nuclear Research, Dubna, Russia
91,
V Perelygin
V Perelygin
91Joint Institute for Nuclear Research, Dubna, Russia
91,
M Savina
M Savina
91Joint Institute for Nuclear Research, Dubna, Russia
91,
S Shmatov
S Shmatov
91Joint Institute for Nuclear Research, Dubna, Russia
91,
S Shulha
S Shulha
91Joint Institute for Nuclear Research, Dubna, Russia
91,
V Smirnov
V Smirnov
91Joint Institute for Nuclear Research, Dubna, Russia
91,
A Zarubin
A Zarubin
91Joint Institute for Nuclear Research, Dubna, Russia
91,
V Golovtsov
V Golovtsov
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
Y Ivanov
Y Ivanov
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
V Kim
V Kim
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
E Kuznetsova
E Kuznetsova
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
P Levchenko
P Levchenko
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
V Murzin
V Murzin
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
V Oreshkin
V Oreshkin
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
I Smirnov
I Smirnov
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
V Sulimov
V Sulimov
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
L Uvarov
L Uvarov
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
S Vavilov
S Vavilov
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
A Vorobyev
A Vorobyev
92Petersburg Nuclear Physics Institute, Gatchina, St. Petersburg, Russia
92,
Yu Andreev
Yu Andreev
93Institute for Nuclear Research, Moscow, Russia
93,
A Dermenev
A Dermenev
93Institute for Nuclear Research, Moscow, Russia
93,
S Gninenko
S Gninenko
93Institute for Nuclear Research, Moscow, Russia
93,
N Golubev
N Golubev
93Institute for Nuclear Research, Moscow, Russia
93,
A Karneyeu
A Karneyeu
93Institute for Nuclear Research, Moscow, Russia
93,
M Kirsanov
M Kirsanov
93Institute for Nuclear Research, Moscow, Russia
93,
N Krasnikov
N Krasnikov
93Institute for Nuclear Research, Moscow, Russia
93,
A Pashenkov
A Pashenkov
93Institute for Nuclear Research, Moscow, Russia
93,
D Tlisov
D Tlisov
93Institute for Nuclear Research, Moscow, Russia
93,
A Toropin
A Toropin
93Institute for Nuclear Research, Moscow, Russia
93,
V Epshteyn
V Epshteyn
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
V Gavrilov
V Gavrilov
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
N Lychkovskaya
N Lychkovskaya
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
V Popov
V Popov
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
l Pozdnyakov
l Pozdnyakov
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
G Safronov
G Safronov
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
A Spiridonov
A Spiridonov
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
E Vlasov
E Vlasov
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
A Zhokin
A Zhokin
94Institute for Theoretical and Experimental Physics, Moscow, Russia
94,
A Bylinkin
A Bylinkin
95National Research Nuclear University ‘Moscow Engineering Physics Institute’(MEPhI), Moscow, Russia
95,
V Andreev
V Andreev
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
M Azarkin
M Azarkin
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
I Dremin
I Dremin
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
M Kirakosyan
M Kirakosyan
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
A Leonidov
A Leonidov
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
G Mesyats
G Mesyats
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
S V Rusakov
S V Rusakov
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
A Baskakov
A Baskakov
96P. N. Lebedev Physical Institute, Moscow, Russia
96,
A Belyaev
A Belyaev
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
E Boos
E Boos
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
M Dubinin
M Dubinin
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
L Dudko
L Dudko
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
A Ershov
A Ershov
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
A Gribushin
A Gribushin
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
V Klyukhin
V Klyukhin
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
O Kodolova
O Kodolova
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
I Lokhtin
I Lokhtin
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
I Myagkov
I Myagkov
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
S Obraztsov
S Obraztsov
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
S Petrushanko
S Petrushanko
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
V Savrin
V Savrin
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
A Snigirev
A Snigirev
97Skobeltsyn Institute of Nuclear Physics, Lomonosov Moscow State University, Moscow, Russia
97,
I Azhgirey
I Azhgirey
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
I Bayshev
I Bayshev
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
S Bitioukov
S Bitioukov
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
V Kachanov
V Kachanov
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
A Kalinin
A Kalinin
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
D Konstantinov
D Konstantinov
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
V Krychkine
V Krychkine
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
V Petrov
V Petrov
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
R Ryutin
R Ryutin
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
A Sobol
A Sobol
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
L Tourtchanovitch
L Tourtchanovitch
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
S Troshin
S Troshin
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
N Tyurin
N Tyurin
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
A Uzunian
A Uzunian
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
A Volkov
A Volkov
98State Research Center of Russian Federation, Institute for High Energy Physics, Protvino, Russia
98,
P Adzic
P Adzic
99Faculty of Physics and Vinca Institute of Nuclear Sciences, University of Belgrade, Belgrade, Serbia
99,
P Cirkovic
P Cirkovic
99Faculty of Physics and Vinca Institute of Nuclear Sciences, University of Belgrade, Belgrade, Serbia
99,
J Milosevic
J Milosevic
99Faculty of Physics and Vinca Institute of Nuclear Sciences, University of Belgrade, Belgrade, Serbia
99,
V Rekovic
V Rekovic
99Faculty of Physics and Vinca Institute of Nuclear Sciences, University of Belgrade, Belgrade, Serbia
99,
J Alcaraz Maestre
J Alcaraz Maestre
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
C Battilana
C Battilana
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
E Calvo
E Calvo
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
M Cerrada
M Cerrada
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
M Chamizo Llatas
M Chamizo Llatas
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
N Colino
N Colino
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
B De La Cruz
B De La Cruz
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
A Delgado Peris
A Delgado Peris
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
A Escalante Del Valle
A Escalante Del Valle
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
C Fernandez Bedoya
C Fernandez Bedoya
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
J P Fernández Ramos
J P Fernández Ramos
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
J Flix
J Flix
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
M C Fouz
M C Fouz
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
P Garcia-Abia
P Garcia-Abia
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
O Gonzalez Lopez
O Gonzalez Lopez
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
S Goy Lopez
S Goy Lopez
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
J M Hernandez
J M Hernandez
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
M I Josa
M I Josa
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
E Navarro De Martino
E Navarro De Martino
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
A Pérez-Calero Yzquierdo
A Pérez-Calero Yzquierdo
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
J Puerta Pelayo
J Puerta Pelayo
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
A Quintario Olmeda
A Quintario Olmeda
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
I Redondo
I Redondo
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
L Romero
L Romero
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
J Santaolalla
J Santaolalla
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
M S Soares
M S Soares
100Centro de Investigaciones Energéticas Medioambientales y Tecnológicas (CIEMAT), Madrid, Spain
100,
C Albajar
C Albajar
101Universidad Autónoma de Madrid, Madrid, Spain
101,
J F de Trocóniz
J F de Trocóniz
101Universidad Autónoma de Madrid, Madrid, Spain
101,
M Missiroli
M Missiroli
101Universidad Autónoma de Madrid, Madrid, Spain
101,
D Moran
D Moran
101Universidad Autónoma de Madrid, Madrid, Spain
101,
J Cuevas
J Cuevas
102Universidad de Oviedo, Oviedo, Spain
102,
J Fernandez Menendez
J Fernandez Menendez
102Universidad de Oviedo, Oviedo, Spain
102,
S Folgueras
S Folgueras
102Universidad de Oviedo, Oviedo, Spain
102,
I Gonzalez Caballero
I Gonzalez Caballero
102Universidad de Oviedo, Oviedo, Spain
102,
E Palencia Cortezon
E Palencia Cortezon
102Universidad de Oviedo, Oviedo, Spain
102,
J M Vizan Garcia
J M Vizan Garcia
102Universidad de Oviedo, Oviedo, Spain
102,
I J Cabrillo
I J Cabrillo
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
A Calderon
A Calderon
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
J R Castiñeiras De Saa
J R Castiñeiras De Saa
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
P De Castro Manzano
P De Castro Manzano
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
M Fernandez
M Fernandez
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
J Garcia-Ferrero
J Garcia-Ferrero
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
G Gomez
G Gomez
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
A Lopez Virto
A Lopez Virto
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
J Marco
J Marco
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
R Marco
R Marco
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
C Martinez Rivero
C Martinez Rivero
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
F Matorras
F Matorras
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
J Piedra Gomez
J Piedra Gomez
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
T Rodrigo
T Rodrigo
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
A Y Rodríguez-Marrero
A Y Rodríguez-Marrero
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
A Ruiz-Jimeno
A Ruiz-Jimeno
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
L Scodellaro
L Scodellaro
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
N Trevisani
N Trevisani
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
I Vila
I Vila
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
R Vilar Cortabitarte
R Vilar Cortabitarte
103Instituto de Física de Cantabria (IFCA), CSIC-Universidad de Cantabria, Santander, Spain
103,
D Abbaneo
D Abbaneo
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
E Auffray
E Auffray
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
G Auzinger
G Auzinger
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Bachtis
M Bachtis
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
P Baillon
P Baillon
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A H Ball
A H Ball
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
D Barney
D Barney
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Benaglia
A Benaglia
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
J Bendavid
J Bendavid
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
L Benhabib
L Benhabib
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
J F Benitez
J F Benitez
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
G M Berruti
G M Berruti
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
P Bloch
P Bloch
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Bocci
A Bocci
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Bonato
A Bonato
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
C Botta
C Botta
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
H Breuker
H Breuker
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
T Camporesi
T Camporesi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
R Castello
R Castello
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
G Cerminara
G Cerminara
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M D’Alfonso
M D’Alfonso
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
D d’Enterria
D d’Enterria
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Dabrowski
A Dabrowski
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
V Daponte
V Daponte
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A David
A David
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M De Gruttola
M De Gruttola
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
F De Guio
F De Guio
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A De Roeck
A De Roeck
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
S De Visscher
S De Visscher
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
E Di Marco
E Di Marco
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Dobson
M Dobson
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Dordevic
M Dordevic
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
B Dorney
B Dorney
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
T du Pree
T du Pree
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
D Duggan
D Duggan
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Dünser
M Dünser
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
N Dupont
N Dupont
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Elliott-Peisert
A Elliott-Peisert
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
G Franzoni
G Franzoni
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
J Fulcher
J Fulcher
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
W Funk
W Funk
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
D Gigi
D Gigi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
K Gill
K Gill
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
D Giordano
D Giordano
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Girone
M Girone
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
F Glege
F Glege
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
R Guida
R Guida
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
S Gundacker
S Gundacker
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Guthoff
M Guthoff
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
J Hammer
J Hammer
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
P Harris
P Harris
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
J Hegeman
J Hegeman
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
V Innocente
V Innocente
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
P Janot
P Janot
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
H Kirschenmann
H Kirschenmann
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M J Kortelainen
M J Kortelainen
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
K Kousouris
K Kousouris
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
K Krajczar
K Krajczar
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
P Lecoq
P Lecoq
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
C Lourenço
C Lourenço
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M T Lucchini
M T Lucchini
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
N Magini
N Magini
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
L Malgeri
L Malgeri
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Mannelli
M Mannelli
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Martelli
A Martelli
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
L Masetti
L Masetti
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
F Meijers
F Meijers
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
S Mersi
S Mersi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
E Meschi
E Meschi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
F Moortgat
F Moortgat
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
S Morovic
S Morovic
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Mulders
M Mulders
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M V Nemallapudi
M V Nemallapudi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
H Neugebauer
H Neugebauer
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
S Orfanelli
S Orfanelli
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
L Orsini
L Orsini
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
L Pape
L Pape
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
E Perez
E Perez
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Peruzzi
M Peruzzi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Petrilli
A Petrilli
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
G Petrucciani
G Petrucciani
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Pfeiffer
A Pfeiffer
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
D Piparo
D Piparo
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Racz
A Racz
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
T Reis
T Reis
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
G Rolandi
G Rolandi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Rovere
M Rovere
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Ruan
M Ruan
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
H Sakulin
H Sakulin
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
C Schäfer
C Schäfer
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
C Schwick
C Schwick
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Seidel
M Seidel
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Sharma
A Sharma
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
P Silva
P Silva
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Simon
M Simon
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
P Sphicas
P Sphicas
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
J Steggemann
J Steggemann
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
B Stieger
B Stieger
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
M Stoye
M Stoye
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
Y Takahashi
Y Takahashi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
D Treille
D Treille
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Triossi
A Triossi
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Tsirou
A Tsirou
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
G I Veres
G I Veres
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
N Wardle
N Wardle
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
H K Wöhri
H K Wöhri
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
A Zagozdzinska
A Zagozdzinska
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
W D Zeuner
W D Zeuner
104CERN, European Organization for Nuclear Research, Geneva, Switzerland
104,
W Bertl
W Bertl
105Paul Scherrer Institut, Villigen, Switzerland
105,
K Deiters
K Deiters
105Paul Scherrer Institut, Villigen, Switzerland
105,
W Erdmann
W Erdmann
105Paul Scherrer Institut, Villigen, Switzerland
105,
R Horisberger
R Horisberger
105Paul Scherrer Institut, Villigen, Switzerland
105,
Q Ingram
Q Ingram
105Paul Scherrer Institut, Villigen, Switzerland
105,
H C Kaestli
H C Kaestli
105Paul Scherrer Institut, Villigen, Switzerland
105,
D Kotlinski
D Kotlinski
105Paul Scherrer Institut, Villigen, Switzerland
105,
U Langenegger
U Langenegger
105Paul Scherrer Institut, Villigen, Switzerland
105,
D Renker
D Renker
105Paul Scherrer Institut, Villigen, Switzerland
105,
T Rohe
T Rohe
105Paul Scherrer Institut, Villigen, Switzerland
105,
F Bachmair
F Bachmair
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
L Bäni
L Bäni
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
L Bianchini
L Bianchini
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
B Casal
B Casal
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
G Dissertori
G Dissertori
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
M Dittmar
M Dittmar
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
M Donegà
M Donegà
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
P Eller
P Eller
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
C Grab
C Grab
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
C Heidegger
C Heidegger
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
D Hits
D Hits
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
J Hoss
J Hoss
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
G Kasieczka
G Kasieczka
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
W Lustermann
W Lustermann
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
B Mangano
B Mangano
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
M Marionneau
M Marionneau
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
P Martinez Ruiz del Arbol
P Martinez Ruiz del Arbol
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
M Masciovecchio
M Masciovecchio
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
D Meister
D Meister
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
F Micheli
F Micheli
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
P Musella
P Musella
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
F Nessi-Tedaldi
F Nessi-Tedaldi
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
F Pandolfi
F Pandolfi
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
J Pata
J Pata
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
F Pauss
F Pauss
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
L Perrozzi
L Perrozzi
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
M Quittnat
M Quittnat
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
M Rossini
M Rossini
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
A Starodumov
A Starodumov
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
M Takahashi
M Takahashi
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
V R Tavolaro
V R Tavolaro
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
K Theofilatos
K Theofilatos
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
R Wallny
R Wallny
106Institute for Particle Physics, ETH Zurich, Zurich, Switzerland
106,
T K Aarrestad
T K Aarrestad
107Universität Zürich, Zurich, Switzerland
107,
C Amsler
C Amsler
107Universität Zürich, Zurich, Switzerland
107,
L Caminada
L Caminada
107Universität Zürich, Zurich, Switzerland
107,
M F Canelli
M F Canelli
107Universität Zürich, Zurich, Switzerland
107,
V Chiochia
V Chiochia
107Universität Zürich, Zurich, Switzerland
107,
A De Cosa
A De Cosa
107Universität Zürich, Zurich, Switzerland
107,
C Galloni
C Galloni
107Universität Zürich, Zurich, Switzerland
107,
A Hinzmann
A Hinzmann
107Universität Zürich, Zurich, Switzerland
107,
T Hreus
T Hreus
107Universität Zürich, Zurich, Switzerland
107,
B Kilminster
B Kilminster
107Universität Zürich, Zurich, Switzerland
107,
C Lange
C Lange
107Universität Zürich, Zurich, Switzerland
107,
J Ngadiuba
J Ngadiuba
107Universität Zürich, Zurich, Switzerland
107,
D Pinna
D Pinna
107Universität Zürich, Zurich, Switzerland
107,
P Robmann
P Robmann
107Universität Zürich, Zurich, Switzerland
107,
F J Ronga
F J Ronga
107Universität Zürich, Zurich, Switzerland
107,
D Salerno
D Salerno
107Universität Zürich, Zurich, Switzerland
107,
Y Yang
Y Yang
107Universität Zürich, Zurich, Switzerland
107,
M Cardaci
M Cardaci
108National Central University, Chung-Li, Taiwan
108,
K H Chen
K H Chen
108National Central University, Chung-Li, Taiwan
108,
T H Doan
T H Doan
108National Central University, Chung-Li, Taiwan
108,
Sh Jain
Sh Jain
108National Central University, Chung-Li, Taiwan
108,
R Khurana
R Khurana
108National Central University, Chung-Li, Taiwan
108,
M Konyushikhin
M Konyushikhin
108National Central University, Chung-Li, Taiwan
108,
C M Kuo
C M Kuo
108National Central University, Chung-Li, Taiwan
108,
W Lin
W Lin
108National Central University, Chung-Li, Taiwan
108,
Y J Lu
Y J Lu
108National Central University, Chung-Li, Taiwan
108,
S S Yu
S S Yu
108National Central University, Chung-Li, Taiwan
108,
Arun Kumar
Arun Kumar
108National Central University, Chung-Li, Taiwan
108,
R Bartek
R Bartek
109National Taiwan University (NTU), Taipei, Taiwan
109,
P Chang
P Chang
109National Taiwan University (NTU), Taipei, Taiwan
109,
Y H Chang
Y H Chang
109National Taiwan University (NTU), Taipei, Taiwan
109,
Y Chao
Y Chao
109National Taiwan University (NTU), Taipei, Taiwan
109,
K F Chen
K F Chen
109National Taiwan University (NTU), Taipei, Taiwan
109,
P H Chen
P H Chen
109National Taiwan University (NTU), Taipei, Taiwan
109,
C Dietz
C Dietz
109National Taiwan University (NTU), Taipei, Taiwan
109,
F Fiori
F Fiori
109National Taiwan University (NTU), Taipei, Taiwan
109,
U Grundler
U Grundler
109National Taiwan University (NTU), Taipei, Taiwan
109,
W-S Hou
W-S Hou
109National Taiwan University (NTU), Taipei, Taiwan
109,
Y Hsiung
Y Hsiung
109National Taiwan University (NTU), Taipei, Taiwan
109,
Y F Liu
Y F Liu
109National Taiwan University (NTU), Taipei, Taiwan
109,
R-S Lu
R-S Lu
109National Taiwan University (NTU), Taipei, Taiwan
109,
M Miñano Moya
M Miñano Moya
109National Taiwan University (NTU), Taipei, Taiwan
109,
E Petrakou
E Petrakou
109National Taiwan University (NTU), Taipei, Taiwan
109,
J f Tsai
J f Tsai
109National Taiwan University (NTU), Taipei, Taiwan
109,
Y M Tzeng
Y M Tzeng
109National Taiwan University (NTU), Taipei, Taiwan
109,
B Asavapibhop
B Asavapibhop
110Department of Physics, Faculty of Science, Chulalongkorn University, Bangkok, Thailand
110,
K Kovitanggoon
K Kovitanggoon
110Department of Physics, Faculty of Science, Chulalongkorn University, Bangkok, Thailand
110,
G Singh
G Singh
110Department of Physics, Faculty of Science, Chulalongkorn University, Bangkok, Thailand
110,
N Srimanobhas
N Srimanobhas
110Department of Physics, Faculty of Science, Chulalongkorn University, Bangkok, Thailand
110,
N Suwonjandee
N Suwonjandee
110Department of Physics, Faculty of Science, Chulalongkorn University, Bangkok, Thailand
110,
A Adiguzel
A Adiguzel
111Cukurova University, Adana, Turkey
111,
M N Bakirci
M N Bakirci
111Cukurova University, Adana, Turkey
111,
S Cerci
S Cerci
111Cukurova University, Adana, Turkey
111,
Z S Demiroglu
Z S Demiroglu
111Cukurova University, Adana, Turkey
111,
C Dozen
C Dozen
111Cukurova University, Adana, Turkey
111,
E Eskut
E Eskut
111Cukurova University, Adana, Turkey
111,
F H Gecit
F H Gecit
111Cukurova University, Adana, Turkey
111,
S Girgis
S Girgis
111Cukurova University, Adana, Turkey
111,
G Gokbulut
G Gokbulut
111Cukurova University, Adana, Turkey
111,
Y Guler
Y Guler
111Cukurova University, Adana, Turkey
111,
Y Guler
Y Guler
111Cukurova University, Adana, Turkey
111,
E Gurpinar
E Gurpinar
111Cukurova University, Adana, Turkey
111,
I Hos
I Hos
111Cukurova University, Adana, Turkey
111,
E E Kangal
E E Kangal
111Cukurova University, Adana, Turkey
111,
G Onengut
G Onengut
111Cukurova University, Adana, Turkey
111,
M Ozcan
M Ozcan
111Cukurova University, Adana, Turkey
111,
K Ozdemir
K Ozdemir
111Cukurova University, Adana, Turkey
111,
A Polatoz
A Polatoz
111Cukurova University, Adana, Turkey
111,
D Sunar Cerci
D Sunar Cerci
111Cukurova University, Adana, Turkey
111,
H Topakli
H Topakli
111Cukurova University, Adana, Turkey
111,
M Vergili
M Vergili
111Cukurova University, Adana, Turkey
111,
C Zorbilmez
C Zorbilmez
111Cukurova University, Adana, Turkey
111,
I V Akin
I V Akin
112Physics Department, Middle East Technical University, Ankara, Turkey
112,
B Bilin
B Bilin
112Physics Department, Middle East Technical University, Ankara, Turkey
112,
S Bilmis
S Bilmis
112Physics Department, Middle East Technical University, Ankara, Turkey
112,
B Isildak
B Isildak
112Physics Department, Middle East Technical University, Ankara, Turkey
112,
G Karapinar
G Karapinar
112Physics Department, Middle East Technical University, Ankara, Turkey
112,
M Yalvac
M Yalvac
112Physics Department, Middle East Technical University, Ankara, Turkey
112,
M Zeyrek
M Zeyrek
112Physics Department, Middle East Technical University, Ankara, Turkey
112,
E Gülmez
E Gülmez
113Bogazici University, Istanbul, Turkey
113,
M Kaya
M Kaya
113Bogazici University, Istanbul, Turkey
113,
O Kaya
O Kaya
113Bogazici University, Istanbul, Turkey
113,
E A Yetkin
E A Yetkin
113Bogazici University, Istanbul, Turkey
113,
T Yetkin
T Yetkin
113Bogazici University, Istanbul, Turkey
113,
A Cakir
A Cakir
113Bogazici University, Istanbul, Turkey
113,
K Cankocak
K Cankocak
114Istanbul Technical University, Istanbul, Turkey
114,
S Sen
S Sen
114Istanbul Technical University, Istanbul, Turkey
114,
F I Vardarlı
F I Vardarlı
114Istanbul Technical University, Istanbul, Turkey
114,
B Grynyov
B Grynyov
115Institute for Scintillation Materials of National Academy of Science of Ukraine, Kharkov, Ukraine
115,
L Levchuk
L Levchuk
116National Scientific Center, Kharkov Institute of Physics and Technology, Kharkov, Ukraine
116,
P Sorokin
P Sorokin
116National Scientific Center, Kharkov Institute of Physics and Technology, Kharkov, Ukraine
116,
R Aggleton
R Aggleton
117University of Bristol, Bristol, UK
117,
F Ball
F Ball
117University of Bristol, Bristol, UK
117,
L Beck
L Beck
117University of Bristol, Bristol, UK
117,
J J Brooke
J J Brooke
117University of Bristol, Bristol, UK
117,
E Clement
E Clement
117University of Bristol, Bristol, UK
117,
D Cussans
D Cussans
117University of Bristol, Bristol, UK
117,
H Flacher
H Flacher
117University of Bristol, Bristol, UK
117,
J Goldstein
J Goldstein
117University of Bristol, Bristol, UK
117,
M Grimes
M Grimes
117University of Bristol, Bristol, UK
117,
G P Heath
G P Heath
117University of Bristol, Bristol, UK
117,
H F Heath
H F Heath
117University of Bristol, Bristol, UK
117,
J Jacob
J Jacob
117University of Bristol, Bristol, UK
117,
L Kreczko
L Kreczko
117University of Bristol, Bristol, UK
117,
C Lucas
C Lucas
117University of Bristol, Bristol, UK
117,
Z Meng
Z Meng
117University of Bristol, Bristol, UK
117,
D M Newbold
D M Newbold
117University of Bristol, Bristol, UK
117,
S Paramesvaran
S Paramesvaran
117University of Bristol, Bristol, UK
117,
A Poll
A Poll
117University of Bristol, Bristol, UK
117,
T Sakuma
T Sakuma
117University of Bristol, Bristol, UK
117,
S Seif El Nasr-storey
S Seif El Nasr-storey
117University of Bristol, Bristol, UK
117,
S Senkin
S Senkin
117University of Bristol, Bristol, UK
117,
D Smith
D Smith
117University of Bristol, Bristol, UK
117,
V J Smith
V J Smith
117University of Bristol, Bristol, UK
117,
K W Bell
K W Bell
118Rutherford Appleton Laboratory, Didcot, UK
118,
A Belyaev
A Belyaev
118Rutherford Appleton Laboratory, Didcot, UK
118,
C Brew
C Brew
118Rutherford Appleton Laboratory, Didcot, UK
118,
R M Brown
R M Brown
118Rutherford Appleton Laboratory, Didcot, UK
118,
L Calligaris
L Calligaris
118Rutherford Appleton Laboratory, Didcot, UK
118,
D Cieri
D Cieri
118Rutherford Appleton Laboratory, Didcot, UK
118,
D J A Cockerill
D J A Cockerill
118Rutherford Appleton Laboratory, Didcot, UK
118,
J A Coughlan
J A Coughlan
118Rutherford Appleton Laboratory, Didcot, UK
118,
K Harder
K Harder
118Rutherford Appleton Laboratory, Didcot, UK
118,
S Harper
S Harper
118Rutherford Appleton Laboratory, Didcot, UK
118,
E Olaiya
E Olaiya
118Rutherford Appleton Laboratory, Didcot, UK
118,
D Petyt
D Petyt
118Rutherford Appleton Laboratory, Didcot, UK
118,
C H Shepherd-Themistocleous
C H Shepherd-Themistocleous
118Rutherford Appleton Laboratory, Didcot, UK
118,
A Thea
A Thea
118Rutherford Appleton Laboratory, Didcot, UK
118,
I R Tomalin
I R Tomalin
118Rutherford Appleton Laboratory, Didcot, UK
118,
T Williams
T Williams
118Rutherford Appleton Laboratory, Didcot, UK
118,
S D Worm
S D Worm
118Rutherford Appleton Laboratory, Didcot, UK
118,
M Baber
M Baber
119Imperial College, London, UK
119,
R Bainbridge
R Bainbridge
119Imperial College, London, UK
119,
O Buchmuller
O Buchmuller
119Imperial College, London, UK
119,
A Bundock
A Bundock
119Imperial College, London, UK
119,
D Burton
D Burton
119Imperial College, London, UK
119,
S Casasso
S Casasso
119Imperial College, London, UK
119,
M Citron
M Citron
119Imperial College, London, UK
119,
D Colling
D Colling
119Imperial College, London, UK
119,
L Corpe
L Corpe
119Imperial College, London, UK
119,
N Cripps
N Cripps
119Imperial College, London, UK
119,
P Dauncey
P Dauncey
119Imperial College, London, UK
119,
G Davies
G Davies
119Imperial College, London, UK
119,
A De Wit
A De Wit
119Imperial College, London, UK
119,
M Della Negra
M Della Negra
119Imperial College, London, UK
119,
P Dunne
P Dunne
119Imperial College, London, UK
119,
A Elwood
A Elwood
119Imperial College, London, UK
119,
A Elwood
A Elwood
119Imperial College, London, UK
119,
W Ferguson
W Ferguson
119Imperial College, London, UK
119,
D Futyan
D Futyan
119Imperial College, London, UK
119,
G Hall
G Hall
119Imperial College, London, UK
119,
G Iles
G Iles
119Imperial College, London, UK
119,
M Kenzie
M Kenzie
119Imperial College, London, UK
119,
R Lane
R Lane
119Imperial College, London, UK
119,
R Lucas
R Lucas
119Imperial College, London, UK
119,
L Lyons
L Lyons
119Imperial College, London, UK
119,
A-M Magnan
A-M Magnan
119Imperial College, London, UK
119,
S Malik
S Malik
119Imperial College, London, UK
119,
J Nash
J Nash
119Imperial College, London, UK
119,
A Nikitenko
A Nikitenko
119Imperial College, London, UK
119,
J Pela
J Pela
119Imperial College, London, UK
119,
M Pesaresi
M Pesaresi
119Imperial College, London, UK
119,
K Petridis
K Petridis
119Imperial College, London, UK
119,
D M Raymond
D M Raymond
119Imperial College, London, UK
119,
A Richards
A Richards
119Imperial College, London, UK
119,
A Rose
A Rose
119Imperial College, London, UK
119,
C Seez
C Seez
119Imperial College, London, UK
119,
A Tapper
A Tapper
119Imperial College, London, UK
119,
K Uchida
K Uchida
119Imperial College, London, UK
119,
M Vazquez Acosta
M Vazquez Acosta
119Imperial College, London, UK
119,
T Virdee
T Virdee
119Imperial College, London, UK
119,
S C Zenz
S C Zenz
119Imperial College, London, UK
119,
J E Cole
J E Cole
120Brunel University, Uxbridge, UK
120,
P R Hobson
P R Hobson
120Brunel University, Uxbridge, UK
120,
A Khan
A Khan
120Brunel University, Uxbridge, UK
120,
P Kyberd
P Kyberd
120Brunel University, Uxbridge, UK
120,
D Leggat
D Leggat
120Brunel University, Uxbridge, UK
120,
D Leslie
D Leslie
120Brunel University, Uxbridge, UK
120,
I D Reid
I D Reid
120Brunel University, Uxbridge, UK
120,
P Symonds
P Symonds
120Brunel University, Uxbridge, UK
120,
L Teodorescu
L Teodorescu
120Brunel University, Uxbridge, UK
120,
M Turner
M Turner
120Brunel University, Uxbridge, UK
120,
A Borzou
A Borzou
121Baylor University, Waco, USA
121,
K Call
K Call
121Baylor University, Waco, USA
121,
J Dittmann
J Dittmann
121Baylor University, Waco, USA
121,
K Hatakeyama
K Hatakeyama
121Baylor University, Waco, USA
121,
H Liu
H Liu
121Baylor University, Waco, USA
121,
N Pastika
N Pastika
121Baylor University, Waco, USA
121,
T Scarborough
T Scarborough
121Baylor University, Waco, USA
121,
Z Wu
Z Wu
121Baylor University, Waco, USA
121,
O Charaf
O Charaf
122The University of Alabama, Tuscaloosa, USA
122,
S I Cooper
S I Cooper
122The University of Alabama, Tuscaloosa, USA
122,
C Henderson
C Henderson
122The University of Alabama, Tuscaloosa, USA
122,
P Rumerio
P Rumerio
122The University of Alabama, Tuscaloosa, USA
122,
D Arcaro
D Arcaro
122The University of Alabama, Tuscaloosa, USA
122,
A Avetisyan
A Avetisyan
123Boston University, Boston, USA
123,
T Bose
T Bose
123Boston University, Boston, USA
123,
C Fantasia
C Fantasia
123Boston University, Boston, USA
123,
D Gastler
D Gastler
123Boston University, Boston, USA
123,
P Lawson
P Lawson
123Boston University, Boston, USA
123,
D Rankin
D Rankin
123Boston University, Boston, USA
123,
C Richardson
C Richardson
123Boston University, Boston, USA
123,
J Rohlf
J Rohlf
123Boston University, Boston, USA
123,
J St John
J St John
123Boston University, Boston, USA
123,
L Sulak
L Sulak
123Boston University, Boston, USA
123,
D Zou
D Zou
123Boston University, Boston, USA
123,
J Alimena
J Alimena
124Brown University, Providence, USA
124,
E Berry
E Berry
124Brown University, Providence, USA
124,
S Bhattacharya
S Bhattacharya
124Brown University, Providence, USA
124,
D Cutts
D Cutts
124Brown University, Providence, USA
124,
N Dhingra
N Dhingra
124Brown University, Providence, USA
124,
A Ferapontov
A Ferapontov
124Brown University, Providence, USA
124,
A Garabedian
A Garabedian
124Brown University, Providence, USA
124,
J Hakala
J Hakala
124Brown University, Providence, USA
124,
U Heintz
U Heintz
124Brown University, Providence, USA
124,
E Laird
E Laird
124Brown University, Providence, USA
124,
G Landsberg
G Landsberg
124Brown University, Providence, USA
124,
Z Mao
Z Mao
124Brown University, Providence, USA
124,
M Narain
M Narain
124Brown University, Providence, USA
124,
S Piperov
S Piperov
124Brown University, Providence, USA
124,
S Sagir
S Sagir
124Brown University, Providence, USA
124,
R Syarif
R Syarif
124Brown University, Providence, USA
124,
R Breedon
R Breedon
125University of California, Davis, Davis, USA
125,
G Breto
G Breto
125University of California, Davis, Davis, USA
125,
M Calderon De La Barca Sanchez
M Calderon De La Barca Sanchez
125University of California, Davis, Davis, USA
125,
S Chauhan
S Chauhan
125University of California, Davis, Davis, USA
125,
M Chertok
M Chertok
125University of California, Davis, Davis, USA
125,
J Conway
J Conway
125University of California, Davis, Davis, USA
125,
R Conway
R Conway
125University of California, Davis, Davis, USA
125,
P T Cox
P T Cox
125University of California, Davis, Davis, USA
125,
R Erbacher
R Erbacher
125University of California, Davis, Davis, USA
125,
G Funk
G Funk
125University of California, Davis, Davis, USA
125,
M Gardner
M Gardner
125University of California, Davis, Davis, USA
125,
W Ko
W Ko
125University of California, Davis, Davis, USA
125,
R Lander
R Lander
125University of California, Davis, Davis, USA
125,
M Mulhearn
M Mulhearn
125University of California, Davis, Davis, USA
125,
D Pellett
D Pellett
125University of California, Davis, Davis, USA
125,
J Pilot
J Pilot
125University of California, Davis, Davis, USA
125,
F Ricci-Tam
F Ricci-Tam
125University of California, Davis, Davis, USA
125,
S Shalhout
S Shalhout
125University of California, Davis, Davis, USA
125,
J Smith
J Smith
125University of California, Davis, Davis, USA
125,
M Squires
M Squires
125University of California, Davis, Davis, USA
125,
D Stolp
D Stolp
125University of California, Davis, Davis, USA
125,
M Tripathi
M Tripathi
125University of California, Davis, Davis, USA
125,
S Wilbur
S Wilbur
125University of California, Davis, Davis, USA
125,
R Yohay
R Yohay
125University of California, Davis, Davis, USA
125,
C Bravo
C Bravo
125University of California, Davis, Davis, USA
125,
R Cousins
R Cousins
126University of California, Los Angeles, USA
126,
P Everaerts
P Everaerts
126University of California, Los Angeles, USA
126,
C Farrell
C Farrell
126University of California, Los Angeles, USA
126,
A Florent
A Florent
126University of California, Los Angeles, USA
126,
J Hauser
J Hauser
126University of California, Los Angeles, USA
126,
M Ignatenko
M Ignatenko
126University of California, Los Angeles, USA
126,
D Saltzberg
D Saltzberg
126University of California, Los Angeles, USA
126,
C Schnaible
C Schnaible
126University of California, Los Angeles, USA
126,
V Valuev
V Valuev
126University of California, Los Angeles, USA
126,
M Weber
M Weber
126University of California, Los Angeles, USA
126,
K Burt
K Burt
127University of California, Riverside, Riverside, USA
127,
R Clare
R Clare
127University of California, Riverside, Riverside, USA
127,
J Ellison
J Ellison
127University of California, Riverside, Riverside, USA
127,
J W Gary
J W Gary
127University of California, Riverside, Riverside, USA
127,
G Hanson
G Hanson
127University of California, Riverside, Riverside, USA
127,
J Heilman
J Heilman
127University of California, Riverside, Riverside, USA
127,
M Ivova PANEVA
M Ivova PANEVA
127University of California, Riverside, Riverside, USA
127,
P Jandir
P Jandir
127University of California, Riverside, Riverside, USA
127,
E Kennedy
E Kennedy
127University of California, Riverside, Riverside, USA
127,
F Lacroix
F Lacroix
127University of California, Riverside, Riverside, USA
127,
O R Long
O R Long
127University of California, Riverside, Riverside, USA
127,
A Luthra
A Luthra
127University of California, Riverside, Riverside, USA
127,
M Malberti
M Malberti
127University of California, Riverside, Riverside, USA
127,
M Olmedo Negrete
M Olmedo Negrete
127University of California, Riverside, Riverside, USA
127,
A Shrinivas
A Shrinivas
127University of California, Riverside, Riverside, USA
127,
H Wei
H Wei
127University of California, Riverside, Riverside, USA
127,
S Wimpenny
S Wimpenny
127University of California, Riverside, Riverside, USA
127,
B R Yates
B R Yates
127University of California, Riverside, Riverside, USA
127,
J G Branson
J G Branson
128University of California, San Diego, La Jolla, USA
128,
G B Cerati
G B Cerati
128University of California, San Diego, La Jolla, USA
128,
S Cittolin
S Cittolin
128University of California, San Diego, La Jolla, USA
128,
R T D’Agnolo
R T D’Agnolo
128University of California, San Diego, La Jolla, USA
128,
M Derdzinski
M Derdzinski
128University of California, San Diego, La Jolla, USA
128,
A Holzner
A Holzner
128University of California, San Diego, La Jolla, USA
128,
R Kelley
R Kelley
128University of California, San Diego, La Jolla, USA
128,
D Klein
D Klein
128University of California, San Diego, La Jolla, USA
128,
J Letts
J Letts
128University of California, San Diego, La Jolla, USA
128,
I Macneill
I Macneill
128University of California, San Diego, La Jolla, USA
128,
D Olivito
D Olivito
128University of California, San Diego, La Jolla, USA
128,
S Padhi
S Padhi
128University of California, San Diego, La Jolla, USA
128,
M Pieri
M Pieri
128University of California, San Diego, La Jolla, USA
128,
M Sani
M Sani
128University of California, San Diego, La Jolla, USA
128,
V Sharma
V Sharma
128University of California, San Diego, La Jolla, USA
128,
S Simon
S Simon
128University of California, San Diego, La Jolla, USA
128,
M Tadel
M Tadel
128University of California, San Diego, La Jolla, USA
128,
Y Tu
Y Tu
128University of California, San Diego, La Jolla, USA
128,
A Vartak
A Vartak
128University of California, San Diego, La Jolla, USA
128,
S Wasserbaech
S Wasserbaech
128University of California, San Diego, La Jolla, USA
128,
C Welke
C Welke
128University of California, San Diego, La Jolla, USA
128,
F Würthwein
F Würthwein
128University of California, San Diego, La Jolla, USA
128,
A Yagil
A Yagil
128University of California, San Diego, La Jolla, USA
128,
G Zevi Della Porta
G Zevi Della Porta
128University of California, San Diego, La Jolla, USA
128,
J Bradmiller-Feld
J Bradmiller-Feld
129University of California, Santa Barbara, Santa Barbara, USA
129,
C Campagnari
C Campagnari
129University of California, Santa Barbara, Santa Barbara, USA
129,
A Dishaw
A Dishaw
129University of California, Santa Barbara, Santa Barbara, USA
129,
V Dutta
V Dutta
129University of California, Santa Barbara, Santa Barbara, USA
129,
K Flowers
K Flowers
129University of California, Santa Barbara, Santa Barbara, USA
129,
M Franco Sevilla
M Franco Sevilla
129University of California, Santa Barbara, Santa Barbara, USA
129,
P Geffert
P Geffert
129University of California, Santa Barbara, Santa Barbara, USA
129,
C George
C George
129University of California, Santa Barbara, Santa Barbara, USA
129,
F Golf
F Golf
129University of California, Santa Barbara, Santa Barbara, USA
129,
L Gouskos
L Gouskos
129University of California, Santa Barbara, Santa Barbara, USA
129,
J Gran
J Gran
129University of California, Santa Barbara, Santa Barbara, USA
129,
J Incandela
J Incandela
129University of California, Santa Barbara, Santa Barbara, USA
129,
N Mccoll
N Mccoll
129University of California, Santa Barbara, Santa Barbara, USA
129,
S D Mullin
S D Mullin
129University of California, Santa Barbara, Santa Barbara, USA
129,
S D Mullin
S D Mullin
129University of California, Santa Barbara, Santa Barbara, USA
129,
J Richman
J Richman
129University of California, Santa Barbara, Santa Barbara, USA
129,
D Stuart
D Stuart
129University of California, Santa Barbara, Santa Barbara, USA
129,
I Suarez
I Suarez
129University of California, Santa Barbara, Santa Barbara, USA
129,
C West
C West
129University of California, Santa Barbara, Santa Barbara, USA
129,
J Yoo
J Yoo
129University of California, Santa Barbara, Santa Barbara, USA
129,
D Anderson
D Anderson
130California Institute of Technology, Pasadena, USA
130,
A Apresyan
A Apresyan
130California Institute of Technology, Pasadena, USA
130,
A Bornheim
A Bornheim
130California Institute of Technology, Pasadena, USA
130,
J Bunn
J Bunn
130California Institute of Technology, Pasadena, USA
130,
Y Chen
Y Chen
130California Institute of Technology, Pasadena, USA
130,
J Duarte
J Duarte
130California Institute of Technology, Pasadena, USA
130,
A Mott
A Mott
130California Institute of Technology, Pasadena, USA
130,
H B Newman
H B Newman
130California Institute of Technology, Pasadena, USA
130,
C Pena
C Pena
130California Institute of Technology, Pasadena, USA
130,
M Pierini
M Pierini
130California Institute of Technology, Pasadena, USA
130,
M Spiropulu
M Spiropulu
130California Institute of Technology, Pasadena, USA
130,
J R Vlimant
J R Vlimant
130California Institute of Technology, Pasadena, USA
130,
S Xie
S Xie
130California Institute of Technology, Pasadena, USA
130,
R Y Zhu
R Y Zhu
130California Institute of Technology, Pasadena, USA
130,
M B Andrews
M B Andrews
130California Institute of Technology, Pasadena, USA
130,
V Azzolini
V Azzolini
131Carnegie Mellon University, Pittsburgh, USA
131,
A Calamba
A Calamba
131Carnegie Mellon University, Pittsburgh, USA
131,
B Carlson
B Carlson
131Carnegie Mellon University, Pittsburgh, USA
131,
T Ferguson
T Ferguson
131Carnegie Mellon University, Pittsburgh, USA
131,
M Paulini
M Paulini
131Carnegie Mellon University, Pittsburgh, USA
131,
J Russ
J Russ
131Carnegie Mellon University, Pittsburgh, USA
131,
M Sun
M Sun
131Carnegie Mellon University, Pittsburgh, USA
131,
H Vogel
H Vogel
131Carnegie Mellon University, Pittsburgh, USA
131,
I Vorobiev
I Vorobiev
131Carnegie Mellon University, Pittsburgh, USA
131,
J P Cumalat
J P Cumalat
132University of Colorado Boulder, Boulder, USA
132,
W T Ford
W T Ford
132University of Colorado Boulder, Boulder, USA
132,
A Gaz
A Gaz
132University of Colorado Boulder, Boulder, USA
132,
F Jensen
F Jensen
132University of Colorado Boulder, Boulder, USA
132,
A Johnson
A Johnson
132University of Colorado Boulder, Boulder, USA
132,
M Krohn
M Krohn
132University of Colorado Boulder, Boulder, USA
132,
T Mulholland
T Mulholland
132University of Colorado Boulder, Boulder, USA
132,
U Nauenberg
U Nauenberg
132University of Colorado Boulder, Boulder, USA
132,
K Stenson
K Stenson
132University of Colorado Boulder, Boulder, USA
132,
S R Wagner
S R Wagner
132University of Colorado Boulder, Boulder, USA
132,
J Alexander
J Alexander
133Cornell University, Ithaca, USA
133,
A Chatterjee
A Chatterjee
133Cornell University, Ithaca, USA
133,
J Chaves
J Chaves
133Cornell University, Ithaca, USA
133,
J Chu
J Chu
133Cornell University, Ithaca, USA
133,
S Dittmer
S Dittmer
133Cornell University, Ithaca, USA
133,
N Eggert
N Eggert
133Cornell University, Ithaca, USA
133,
N Mirman
N Mirman
133Cornell University, Ithaca, USA
133,
G Nicolas Kaufman
G Nicolas Kaufman
133Cornell University, Ithaca, USA
133,
J R Patterson
J R Patterson
133Cornell University, Ithaca, USA
133,
A Rinkevicius
A Rinkevicius
133Cornell University, Ithaca, USA
133,
A Ryd
A Ryd
133Cornell University, Ithaca, USA
133,
L Skinnari
L Skinnari
133Cornell University, Ithaca, USA
133,
L Soffi
L Soffi
133Cornell University, Ithaca, USA
133,
W Sun
W Sun
133Cornell University, Ithaca, USA
133,
S M Tan
S M Tan
133Cornell University, Ithaca, USA
133,
W D Teo
W D Teo
133Cornell University, Ithaca, USA
133,
J Thom
J Thom
133Cornell University, Ithaca, USA
133,
J Thompson
J Thompson
133Cornell University, Ithaca, USA
133,
J Tucker
J Tucker
133Cornell University, Ithaca, USA
133,
Y Weng
Y Weng
133Cornell University, Ithaca, USA
133,
P Wittich
P Wittich
133Cornell University, Ithaca, USA
133,
S Abdullin
S Abdullin
134Fermi National Accelerator Laboratory, Batavia, USA
134,
M Albrow
M Albrow
134Fermi National Accelerator Laboratory, Batavia, USA
134,
G Apollinari
G Apollinari
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Banerjee
S Banerjee
134Fermi National Accelerator Laboratory, Batavia, USA
134,
L A T Bauerdick
L A T Bauerdick
134Fermi National Accelerator Laboratory, Batavia, USA
134,
A Beretvas
A Beretvas
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J Berryhill
J Berryhill
134Fermi National Accelerator Laboratory, Batavia, USA
134,
P C Bhat
P C Bhat
134Fermi National Accelerator Laboratory, Batavia, USA
134,
G Bolla
G Bolla
134Fermi National Accelerator Laboratory, Batavia, USA
134,
K Burkett
K Burkett
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J N Butler
J N Butler
134Fermi National Accelerator Laboratory, Batavia, USA
134,
H W K Cheung
H W K Cheung
134Fermi National Accelerator Laboratory, Batavia, USA
134,
F Chlebana
F Chlebana
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Cihangir
S Cihangir
134Fermi National Accelerator Laboratory, Batavia, USA
134,
V D Elvira
V D Elvira
134Fermi National Accelerator Laboratory, Batavia, USA
134,
I Fisk
I Fisk
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J Freeman
J Freeman
134Fermi National Accelerator Laboratory, Batavia, USA
134,
E Gottschalk
E Gottschalk
134Fermi National Accelerator Laboratory, Batavia, USA
134,
L Gray
L Gray
134Fermi National Accelerator Laboratory, Batavia, USA
134,
D Green
D Green
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Grünendahl
S Grünendahl
134Fermi National Accelerator Laboratory, Batavia, USA
134,
O Gutsche
O Gutsche
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J Hanlon
J Hanlon
134Fermi National Accelerator Laboratory, Batavia, USA
134,
D Hare
D Hare
134Fermi National Accelerator Laboratory, Batavia, USA
134,
R M Harris
R M Harris
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Hasegawa
S Hasegawa
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J Hirschauer
J Hirschauer
134Fermi National Accelerator Laboratory, Batavia, USA
134,
Z Hu
Z Hu
134Fermi National Accelerator Laboratory, Batavia, USA
134,
B Jayatilaka
B Jayatilaka
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Jindariani
S Jindariani
134Fermi National Accelerator Laboratory, Batavia, USA
134,
M Johnson
M Johnson
134Fermi National Accelerator Laboratory, Batavia, USA
134,
U Joshi
U Joshi
134Fermi National Accelerator Laboratory, Batavia, USA
134,
A W Jung
A W Jung
134Fermi National Accelerator Laboratory, Batavia, USA
134,
B Klima
B Klima
134Fermi National Accelerator Laboratory, Batavia, USA
134,
B Kreis
B Kreis
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Lammel
S Lammel
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J Linacre
J Linacre
134Fermi National Accelerator Laboratory, Batavia, USA
134,
D Lincoln
D Lincoln
134Fermi National Accelerator Laboratory, Batavia, USA
134,
R Lipton
R Lipton
134Fermi National Accelerator Laboratory, Batavia, USA
134,
T Liu
T Liu
134Fermi National Accelerator Laboratory, Batavia, USA
134,
R Lopes De Sá
R Lopes De Sá
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J Lykken
J Lykken
134Fermi National Accelerator Laboratory, Batavia, USA
134,
K Maeshima
K Maeshima
134Fermi National Accelerator Laboratory, Batavia, USA
134,
J M Marraffino
J M Marraffino
134Fermi National Accelerator Laboratory, Batavia, USA
134,
V I Martinez Outschoorn
V I Martinez Outschoorn
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Maruyama
S Maruyama
134Fermi National Accelerator Laboratory, Batavia, USA
134,
D Mason
D Mason
134Fermi National Accelerator Laboratory, Batavia, USA
134,
P McBride
P McBride
134Fermi National Accelerator Laboratory, Batavia, USA
134,
P Merkel
P Merkel
134Fermi National Accelerator Laboratory, Batavia, USA
134,
K Mishra
K Mishra
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Mrenna
S Mrenna
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Nahn
S Nahn
134Fermi National Accelerator Laboratory, Batavia, USA
134,
C Newman-Holmes
C Newman-Holmes
134Fermi National Accelerator Laboratory, Batavia, USA
134,
V O’Dell
V O’Dell
134Fermi National Accelerator Laboratory, Batavia, USA
134,
K Pedro
K Pedro
134Fermi National Accelerator Laboratory, Batavia, USA
134,
O Prokofyev
O Prokofyev
134Fermi National Accelerator Laboratory, Batavia, USA
134,
G Rakness
G Rakness
134Fermi National Accelerator Laboratory, Batavia, USA
134,
E Sexton-Kennedy
E Sexton-Kennedy
134Fermi National Accelerator Laboratory, Batavia, USA
134,
A Soha
A Soha
134Fermi National Accelerator Laboratory, Batavia, USA
134,
W J Spalding
W J Spalding
134Fermi National Accelerator Laboratory, Batavia, USA
134,
L Spiegel
L Spiegel
134Fermi National Accelerator Laboratory, Batavia, USA
134,
N Strobbe
N Strobbe
134Fermi National Accelerator Laboratory, Batavia, USA
134,
L Taylor
L Taylor
134Fermi National Accelerator Laboratory, Batavia, USA
134,
S Tkaczyk
S Tkaczyk
134Fermi National Accelerator Laboratory, Batavia, USA
134,
N V Tran
N V Tran
134Fermi National Accelerator Laboratory, Batavia, USA
134,
L Uplegger
L Uplegger
134Fermi National Accelerator Laboratory, Batavia, USA
134,
E W Vaandering
E W Vaandering
134Fermi National Accelerator Laboratory, Batavia, USA
134,
C Vernieri
C Vernieri
134Fermi National Accelerator Laboratory, Batavia, USA
134,
M Verzocchi
M Verzocchi
134Fermi National Accelerator Laboratory, Batavia, USA
134,
R Vidal
R Vidal
134Fermi National Accelerator Laboratory, Batavia, USA
134,
H A Weber
H A Weber
134Fermi National Accelerator Laboratory, Batavia, USA
134,
A Whitbeck
A Whitbeck
134Fermi National Accelerator Laboratory, Batavia, USA
134,
D Acosta
D Acosta
135University of Florida, Gainesville, USA
135,
P Avery
P Avery
135University of Florida, Gainesville, USA
135,
P Bortignon
P Bortignon
135University of Florida, Gainesville, USA
135,
D Bourilkov
D Bourilkov
135University of Florida, Gainesville, USA
135,
A Carnes
A Carnes
135University of Florida, Gainesville, USA
135,
M Carver
M Carver
135University of Florida, Gainesville, USA
135,
D Curry
D Curry
135University of Florida, Gainesville, USA
135,
S Das
S Das
135University of Florida, Gainesville, USA
135,
R D Field
R D Field
135University of Florida, Gainesville, USA
135,
I K Furic
I K Furic
135University of Florida, Gainesville, USA
135,
S V Gleyzer
S V Gleyzer
135University of Florida, Gainesville, USA
135,
J Hugon
J Hugon
135University of Florida, Gainesville, USA
135,
J Konigsberg
J Konigsberg
135University of Florida, Gainesville, USA
135,
A Korytov
A Korytov
135University of Florida, Gainesville, USA
135,
K Kotov
K Kotov
135University of Florida, Gainesville, USA
135,
J F Low
J F Low
135University of Florida, Gainesville, USA
135,
P Ma
P Ma
135University of Florida, Gainesville, USA
135,
K Matchev
K Matchev
135University of Florida, Gainesville, USA
135,
H Mei
H Mei
135University of Florida, Gainesville, USA
135,
P Milenovic
P Milenovic
135University of Florida, Gainesville, USA
135,
G Mitselmakher
G Mitselmakher
135University of Florida, Gainesville, USA
135,
D Rank
D Rank
135University of Florida, Gainesville, USA
135,
R Rossin
R Rossin
135University of Florida, Gainesville, USA
135,
L Shchutska
L Shchutska
135University of Florida, Gainesville, USA
135,
M Snowball
M Snowball
135University of Florida, Gainesville, USA
135,
D Sperka
D Sperka
135University of Florida, Gainesville, USA
135,
N Terentyev
N Terentyev
135University of Florida, Gainesville, USA
135,
L Thomas
L Thomas
135University of Florida, Gainesville, USA
135,
J Wang
J Wang
135University of Florida, Gainesville, USA
135,
S Wang
S Wang
135University of Florida, Gainesville, USA
135,
J Yelton
J Yelton
135University of Florida, Gainesville, USA
135,
S Hewamanage
S Hewamanage
136Florida International University, Miami, USA
136,
S Linn
S Linn
136Florida International University, Miami, USA
136,
P Markowitz
P Markowitz
136Florida International University, Miami, USA
136,
G Martinez
G Martinez
136Florida International University, Miami, USA
136,
J L Rodriguez
J L Rodriguez
136Florida International University, Miami, USA
136,
J R Adams
J R Adams
136Florida International University, Miami, USA
136,
A Ackert
A Ackert
136Florida International University, Miami, USA
136,
T Adams
T Adams
137Florida State University, Tallahassee, USA
137,
A Askew
A Askew
137Florida State University, Tallahassee, USA
137,
S Bein
S Bein
137Florida State University, Tallahassee, USA
137,
J Bochenek
J Bochenek
137Florida State University, Tallahassee, USA
137,
B Diamond
B Diamond
137Florida State University, Tallahassee, USA
137,
J Haas
J Haas
137Florida State University, Tallahassee, USA
137,
S Hagopian
S Hagopian
137Florida State University, Tallahassee, USA
137,
V Hagopian
V Hagopian
137Florida State University, Tallahassee, USA
137,
K F Johnson
K F Johnson
137Florida State University, Tallahassee, USA
137,
A Khatiwada
A Khatiwada
137Florida State University, Tallahassee, USA
137,
H Prosper
H Prosper
137Florida State University, Tallahassee, USA
137,
M Weinberg
M Weinberg
137Florida State University, Tallahassee, USA
137,
M M Baarmand
M M Baarmand
137Florida State University, Tallahassee, USA
137,
V Bhopatkar
V Bhopatkar
137Florida State University, Tallahassee, USA
137,
S Colafranceschi
S Colafranceschi
137Florida State University, Tallahassee, USA
137,
M Hohlmann
M Hohlmann
138Florida Institute of Technology, Melbourne, USA
138,
H Kalakhety
H Kalakhety
138Florida Institute of Technology, Melbourne, USA
138,
D Noonan
D Noonan
138Florida Institute of Technology, Melbourne, USA
138,
T Roy
T Roy
138Florida Institute of Technology, Melbourne, USA
138,
F Yumiceva
F Yumiceva
138Florida Institute of Technology, Melbourne, USA
138,
M R Adams
M R Adams
139University of Illinois at Chicago (UIC), Chicago, USA
139,
L Apanasevich
L Apanasevich
139University of Illinois at Chicago (UIC), Chicago, USA
139,
D Berry
D Berry
139University of Illinois at Chicago (UIC), Chicago, USA
139,
R R Betts
R R Betts
139University of Illinois at Chicago (UIC), Chicago, USA
139,
I Bucinskaite
I Bucinskaite
139University of Illinois at Chicago (UIC), Chicago, USA
139,
R Cavanaugh
R Cavanaugh
139University of Illinois at Chicago (UIC), Chicago, USA
139,
O Evdokimov
O Evdokimov
139University of Illinois at Chicago (UIC), Chicago, USA
139,
L Gauthier
L Gauthier
139University of Illinois at Chicago (UIC), Chicago, USA
139,
C E Gerber
C E Gerber
139University of Illinois at Chicago (UIC), Chicago, USA
139,
D J Hofman
D J Hofman
139University of Illinois at Chicago (UIC), Chicago, USA
139,
P Kurt
P Kurt
139University of Illinois at Chicago (UIC), Chicago, USA
139,
C O’Brien
C O’Brien
139University of Illinois at Chicago (UIC), Chicago, USA
139,
l D Sandoval Gonzalez
l D Sandoval Gonzalez
139University of Illinois at Chicago (UIC), Chicago, USA
139,
C Silkworth
C Silkworth
139University of Illinois at Chicago (UIC), Chicago, USA
139,
P Turner
P Turner
139University of Illinois at Chicago (UIC), Chicago, USA
139,
N Varelas
N Varelas
139University of Illinois at Chicago (UIC), Chicago, USA
139,
Z Wu
Z Wu
139University of Illinois at Chicago (UIC), Chicago, USA
139,
M Zakaria
M Zakaria
139University of Illinois at Chicago (UIC), Chicago, USA
139,
B Bilki
B Bilki
140The University of Iowa, Iowa City, USA
140,
W Clarida
W Clarida
140The University of Iowa, Iowa City, USA
140,
K Dilsiz
K Dilsiz
140The University of Iowa, Iowa City, USA
140,
S Durgut
S Durgut
140The University of Iowa, Iowa City, USA
140,
R P Gandrajula
R P Gandrajula
140The University of Iowa, Iowa City, USA
140,
M Haytmyradov
M Haytmyradov
140The University of Iowa, Iowa City, USA
140,
V Khristenko
V Khristenko
140The University of Iowa, Iowa City, USA
140,
J-P Merlo
J-P Merlo
140The University of Iowa, Iowa City, USA
140,
H Mermerkaya
H Mermerkaya
140The University of Iowa, Iowa City, USA
140,
A Mestvirishvili
A Mestvirishvili
140The University of Iowa, Iowa City, USA
140,
A Moeller
A Moeller
140The University of Iowa, Iowa City, USA
140,
J Nachtman
J Nachtman
140The University of Iowa, Iowa City, USA
140,
H Ogul
H Ogul
140The University of Iowa, Iowa City, USA
140,
Y Onel
Y Onel
140The University of Iowa, Iowa City, USA
140,
F Ozok
F Ozok
140The University of Iowa, Iowa City, USA
140,
A Penzo
A Penzo
140The University of Iowa, Iowa City, USA
140,
C Snyder
C Snyder
140The University of Iowa, Iowa City, USA
140,
E Tiras
E Tiras
140The University of Iowa, Iowa City, USA
140,
J Wetzel
J Wetzel
140The University of Iowa, Iowa City, USA
140,
K Yi
K Yi
140The University of Iowa, Iowa City, USA
140,
I Anderson
I Anderson
140The University of Iowa, Iowa City, USA
140,
I Anderson
I Anderson
140The University of Iowa, Iowa City, USA
140,
B A Barnett
B A Barnett
141Johns Hopkins University, Baltimore, USA
141,
B Blumenfeld
B Blumenfeld
141Johns Hopkins University, Baltimore, USA
141,
N Eminizer
N Eminizer
141Johns Hopkins University, Baltimore, USA
141,
D Fehling
D Fehling
141Johns Hopkins University, Baltimore, USA
141,
L Feng
L Feng
141Johns Hopkins University, Baltimore, USA
141,
A V Gritsan
A V Gritsan
141Johns Hopkins University, Baltimore, USA
141,
P Maksimovic
P Maksimovic
141Johns Hopkins University, Baltimore, USA
141,
C Martin
C Martin
141Johns Hopkins University, Baltimore, USA
141,
M Osherson
M Osherson
141Johns Hopkins University, Baltimore, USA
141,
J Roskes
J Roskes
141Johns Hopkins University, Baltimore, USA
141,
A Sady
A Sady
141Johns Hopkins University, Baltimore, USA
141,
U Sarica
U Sarica
141Johns Hopkins University, Baltimore, USA
141,
M Swartz
M Swartz
141Johns Hopkins University, Baltimore, USA
141,
M Xiao
M Xiao
141Johns Hopkins University, Baltimore, USA
141,
Y Xin
Y Xin
141Johns Hopkins University, Baltimore, USA
141,
C You
C You
141Johns Hopkins University, Baltimore, USA
141,
M Xiao
M Xiao
141Johns Hopkins University, Baltimore, USA
141,
P Baringer
P Baringer
142The University of Kansas, Lawrence, USA
142,
A Bean
A Bean
142The University of Kansas, Lawrence, USA
142,
G Benelli
G Benelli
142The University of Kansas, Lawrence, USA
142,
C Bruner
C Bruner
142The University of Kansas, Lawrence, USA
142,
R P Kenny III
R P Kenny III
142The University of Kansas, Lawrence, USA
142,
D Majumder
D Majumder
142The University of Kansas, Lawrence, USA
142,
D Majumder
D Majumder
142The University of Kansas, Lawrence, USA
142,
M Malek
M Malek
142The University of Kansas, Lawrence, USA
142,
M Murray
M Murray
142The University of Kansas, Lawrence, USA
142,
S Sanders
S Sanders
142The University of Kansas, Lawrence, USA
142,
R Stringer
R Stringer
142The University of Kansas, Lawrence, USA
142,
Q Wang
Q Wang
142The University of Kansas, Lawrence, USA
142,
A Ivanov
A Ivanov
143Kansas State University, Manhattan, USA
143,
K Kaadze
K Kaadze
143Kansas State University, Manhattan, USA
143,
S Khalil
S Khalil
143Kansas State University, Manhattan, USA
143,
M Makouski
M Makouski
143Kansas State University, Manhattan, USA
143,
Y Maravin
Y Maravin
143Kansas State University, Manhattan, USA
143,
A Mohammadi
A Mohammadi
143Kansas State University, Manhattan, USA
143,
L K Saini
L K Saini
143Kansas State University, Manhattan, USA
143,
N Skhirtladze
N Skhirtladze
143Kansas State University, Manhattan, USA
143,
S Toda
S Toda
143Kansas State University, Manhattan, USA
143,
D Lange
D Lange
144Lawrence Livermore National Laboratory, Livermore, USA
144,
F Rebassoo
F Rebassoo
144Lawrence Livermore National Laboratory, Livermore, USA
144,
D Wright
D Wright
144Lawrence Livermore National Laboratory, Livermore, USA
144,
C Anelli
C Anelli
144Lawrence Livermore National Laboratory, Livermore, USA
144,
A Baden
A Baden
145University of Maryland, College Park, USA
145,
O Baron
O Baron
145University of Maryland, College Park, USA
145,
A Belloni
A Belloni
145University of Maryland, College Park, USA
145,
B Calvert
B Calvert
145University of Maryland, College Park, USA
145,
S C Eno
S C Eno
145University of Maryland, College Park, USA
145,
C Ferraioli
C Ferraioli
145University of Maryland, College Park, USA
145,
J A Gomez
J A Gomez
145University of Maryland, College Park, USA
145,
N J Hadley
N J Hadley
145University of Maryland, College Park, USA
145,
S Jabeen
S Jabeen
145University of Maryland, College Park, USA
145,
S Jabeen
S Jabeen
145University of Maryland, College Park, USA
145,
R G Kellogg
R G Kellogg
145University of Maryland, College Park, USA
145,
T Kolberg
T Kolberg
145University of Maryland, College Park, USA
145,
J Kunkle
J Kunkle
145University of Maryland, College Park, USA
145,
Y Lu
Y Lu
145University of Maryland, College Park, USA
145,
A C Mignerey
A C Mignerey
145University of Maryland, College Park, USA
145,
Y H Shin
Y H Shin
145University of Maryland, College Park, USA
145,
A Skuja
A Skuja
145University of Maryland, College Park, USA
145,
M B Tonjes
M B Tonjes
145University of Maryland, College Park, USA
145,
S C Tonwar
S C Tonwar
145University of Maryland, College Park, USA
145,
A Apyan
A Apyan
146Massachusetts Institute of Technology, Cambridge, USA
146,
R Barbieri
R Barbieri
146Massachusetts Institute of Technology, Cambridge, USA
146,
A Baty
A Baty
146Massachusetts Institute of Technology, Cambridge, USA
146,
K Bierwagen
K Bierwagen
146Massachusetts Institute of Technology, Cambridge, USA
146,
S Brandt
S Brandt
146Massachusetts Institute of Technology, Cambridge, USA
146,
K Bierwagen
K Bierwagen
146Massachusetts Institute of Technology, Cambridge, USA
146,
W Busza
W Busza
146Massachusetts Institute of Technology, Cambridge, USA
146,
I A Cali
I A Cali
146Massachusetts Institute of Technology, Cambridge, USA
146,
Z Demiragli
Z Demiragli
146Massachusetts Institute of Technology, Cambridge, USA
146,
L Di Matteo
L Di Matteo
146Massachusetts Institute of Technology, Cambridge, USA
146,
G Gomez Ceballos
G Gomez Ceballos
146Massachusetts Institute of Technology, Cambridge, USA
146,
M Goncharov
M Goncharov
146Massachusetts Institute of Technology, Cambridge, USA
146,
D Gulhan
D Gulhan
146Massachusetts Institute of Technology, Cambridge, USA
146,
Y Iiyama
Y Iiyama
146Massachusetts Institute of Technology, Cambridge, USA
146,
G M Innocenti
G M Innocenti
146Massachusetts Institute of Technology, Cambridge, USA
146,
M Klute
M Klute
146Massachusetts Institute of Technology, Cambridge, USA
146,
D Kovalskyi
D Kovalskyi
146Massachusetts Institute of Technology, Cambridge, USA
146,
Y S Lai
Y S Lai
146Massachusetts Institute of Technology, Cambridge, USA
146,
Y-J Lee
Y-J Lee
146Massachusetts Institute of Technology, Cambridge, USA
146,
A Levin
A Levin
146Massachusetts Institute of Technology, Cambridge, USA
146,
P D Luckey
P D Luckey
146Massachusetts Institute of Technology, Cambridge, USA
146,
A C Marini
A C Marini
146Massachusetts Institute of Technology, Cambridge, USA
146,
C Mcginn
C Mcginn
146Massachusetts Institute of Technology, Cambridge, USA
146,
C Mironov
C Mironov
146Massachusetts Institute of Technology, Cambridge, USA
146,
S Narayanan
S Narayanan
146Massachusetts Institute of Technology, Cambridge, USA
146,
X Niu
X Niu
146Massachusetts Institute of Technology, Cambridge, USA
146,
C Paus
C Paus
146Massachusetts Institute of Technology, Cambridge, USA
146,
D Ralph
D Ralph
146Massachusetts Institute of Technology, Cambridge, USA
146,
C Roland
C Roland
146Massachusetts Institute of Technology, Cambridge, USA
146,
G Roland
G Roland
146Massachusetts Institute of Technology, Cambridge, USA
146,
J Salfeld-Nebgen
J Salfeld-Nebgen
146Massachusetts Institute of Technology, Cambridge, USA
146,
G S F Stephans
G S F Stephans
146Massachusetts Institute of Technology, Cambridge, USA
146,
K Sumorok
K Sumorok
146Massachusetts Institute of Technology, Cambridge, USA
146,
M Varma
M Varma
146Massachusetts Institute of Technology, Cambridge, USA
146,
D Velicanu
D Velicanu
146Massachusetts Institute of Technology, Cambridge, USA
146,
J Veverka
J Veverka
146Massachusetts Institute of Technology, Cambridge, USA
146,
J Wang
J Wang
146Massachusetts Institute of Technology, Cambridge, USA
146,
T W Wang
T W Wang
146Massachusetts Institute of Technology, Cambridge, USA
146,
B Wyslouch
B Wyslouch
146Massachusetts Institute of Technology, Cambridge, USA
146,
M Yang
M Yang
146Massachusetts Institute of Technology, Cambridge, USA
146,
V Zhukova
V Zhukova
146Massachusetts Institute of Technology, Cambridge, USA
146,
B Dahmes
B Dahmes
147University of Minnesota, Minneapolis, USA
147,
A Evans
A Evans
147University of Minnesota, Minneapolis, USA
147,
A Finkel
A Finkel
147University of Minnesota, Minneapolis, USA
147,
A Gude
A Gude
147University of Minnesota, Minneapolis, USA
147,
P Hansen
P Hansen
147University of Minnesota, Minneapolis, USA
147,
S Kalafut
S Kalafut
147University of Minnesota, Minneapolis, USA
147,
S C Kao
S C Kao
147University of Minnesota, Minneapolis, USA
147,
K Klapoetke
K Klapoetke
147University of Minnesota, Minneapolis, USA
147,
Y Kubota
Y Kubota
147University of Minnesota, Minneapolis, USA
147,
Z Lesko
Z Lesko
147University of Minnesota, Minneapolis, USA
147,
J Mans
J Mans
147University of Minnesota, Minneapolis, USA
147,
S Nourbakhsh
S Nourbakhsh
147University of Minnesota, Minneapolis, USA
147,
N Ruckstuhl
N Ruckstuhl
147University of Minnesota, Minneapolis, USA
147,
R Rusack
R Rusack
147University of Minnesota, Minneapolis, USA
147,
N Tambe
N Tambe
147University of Minnesota, Minneapolis, USA
147,
J Turkewitz
J Turkewitz
147University of Minnesota, Minneapolis, USA
147,
J G Acosta
J G Acosta
148University of Mississippi, Oxford, USA
148,
S Oliveros
S Oliveros
148University of Mississippi, Oxford, USA
148,
E Avdeeva
E Avdeeva
149University of Nebraska-Lincoln, Lincoln, USA
149,
K Bloom
K Bloom
149University of Nebraska-Lincoln, Lincoln, USA
149,
S Bose
S Bose
149University of Nebraska-Lincoln, Lincoln, USA
149,
D R Claes
D R Claes
149University of Nebraska-Lincoln, Lincoln, USA
149,
A Dominguez
A Dominguez
149University of Nebraska-Lincoln, Lincoln, USA
149,
C Fangmeier
C Fangmeier
149University of Nebraska-Lincoln, Lincoln, USA
149,
R Gonzalez Suarez
R Gonzalez Suarez
149University of Nebraska-Lincoln, Lincoln, USA
149,
R Kamalieddin
R Kamalieddin
149University of Nebraska-Lincoln, Lincoln, USA
149,
J Keller
J Keller
149University of Nebraska-Lincoln, Lincoln, USA
149,
D Knowlton
D Knowlton
149University of Nebraska-Lincoln, Lincoln, USA
149,
I Kravchenko
I Kravchenko
149University of Nebraska-Lincoln, Lincoln, USA
149,
F Meier
F Meier
149University of Nebraska-Lincoln, Lincoln, USA
149,
J Monroy
J Monroy
149University of Nebraska-Lincoln, Lincoln, USA
149,
F Ratnikov
F Ratnikov
149University of Nebraska-Lincoln, Lincoln, USA
149,
J E Siado
J E Siado
149University of Nebraska-Lincoln, Lincoln, USA
149,
G R Snow
G R Snow
149University of Nebraska-Lincoln, Lincoln, USA
149,
M Alyari
M Alyari
149University of Nebraska-Lincoln, Lincoln, USA
149,
J Dolen
J Dolen
150State University of New York at Buffalo, Buffalo, USA
150,
J George
J George
150State University of New York at Buffalo, Buffalo, USA
150,
A Godshalk
A Godshalk
150State University of New York at Buffalo, Buffalo, USA
150,
C Harrington
C Harrington
150State University of New York at Buffalo, Buffalo, USA
150,
I Iashvili
I Iashvili
150State University of New York at Buffalo, Buffalo, USA
150,
J Kaisen
J Kaisen
150State University of New York at Buffalo, Buffalo, USA
150,
A Kharchilava
A Kharchilava
150State University of New York at Buffalo, Buffalo, USA
150,
A Kumar
A Kumar
150State University of New York at Buffalo, Buffalo, USA
150,
S Rappoccio
S Rappoccio
150State University of New York at Buffalo, Buffalo, USA
150,
B Roozbahani
B Roozbahani
150State University of New York at Buffalo, Buffalo, USA
150,
G Alverson
G Alverson
151Northeastern University, Boston, USA
151,
E Barberis
E Barberis
151Northeastern University, Boston, USA
151,
D Baumgartel
D Baumgartel
151Northeastern University, Boston, USA
151,
M Chasco
M Chasco
151Northeastern University, Boston, USA
151,
A Hortiangtham
A Hortiangtham
151Northeastern University, Boston, USA
151,
A Massironi
A Massironi
151Northeastern University, Boston, USA
151,
D M Morse
D M Morse
151Northeastern University, Boston, USA
151,
D Nash
D Nash
151Northeastern University, Boston, USA
151,
T Orimoto
T Orimoto
151Northeastern University, Boston, USA
151,
R Teixeira De Lima
R Teixeira De Lima
151Northeastern University, Boston, USA
151,
D Trocino
D Trocino
151Northeastern University, Boston, USA
151,
R-J Wang
R-J Wang
151Northeastern University, Boston, USA
151,
D Wood
D Wood
151Northeastern University, Boston, USA
151,
J Zhang
J Zhang
151Northeastern University, Boston, USA
151,
K A Hahn
K A Hahn
152Northwestern University, Evanston, USA
152,
A Kubik
A Kubik
152Northwestern University, Evanston, USA
152,
N Mucia
N Mucia
152Northwestern University, Evanston, USA
152,
N Odell
N Odell
152Northwestern University, Evanston, USA
152,
B Pollack
B Pollack
152Northwestern University, Evanston, USA
152,
A Pozdnyakov
A Pozdnyakov
152Northwestern University, Evanston, USA
152,
M Schmitt
M Schmitt
152Northwestern University, Evanston, USA
152,
S Stoynev
S Stoynev
152Northwestern University, Evanston, USA
152,
K Sung
K Sung
152Northwestern University, Evanston, USA
152,
M Trovato
M Trovato
152Northwestern University, Evanston, USA
152,
M Velasco
M Velasco
152Northwestern University, Evanston, USA
152,
A Brinkerhoff
A Brinkerhoff
153University of Notre Dame, Notre Dame, USA
153,
N Dev
N Dev
153University of Notre Dame, Notre Dame, USA
153,
M Hildreth
M Hildreth
153University of Notre Dame, Notre Dame, USA
153,
C Jessop
C Jessop
153University of Notre Dame, Notre Dame, USA
153,
D J Karmgard
D J Karmgard
153University of Notre Dame, Notre Dame, USA
153,
N Kellams
N Kellams
153University of Notre Dame, Notre Dame, USA
153,
K Lannon
K Lannon
153University of Notre Dame, Notre Dame, USA
153,
N Marinelli
N Marinelli
153University of Notre Dame, Notre Dame, USA
153,
F Meng
F Meng
153University of Notre Dame, Notre Dame, USA
153,
C Mueller
C Mueller
153University of Notre Dame, Notre Dame, USA
153,
Y Musienko
Y Musienko
153University of Notre Dame, Notre Dame, USA
153,
M Planer
M Planer
153University of Notre Dame, Notre Dame, USA
153,
A Reinsvold
A Reinsvold
153University of Notre Dame, Notre Dame, USA
153,
R Ruchti
R Ruchti
153University of Notre Dame, Notre Dame, USA
153,
G Smith
G Smith
153University of Notre Dame, Notre Dame, USA
153,
S Taroni
S Taroni
153University of Notre Dame, Notre Dame, USA
153,
N Valls
N Valls
153University of Notre Dame, Notre Dame, USA
153,
M Wayne
M Wayne
153University of Notre Dame, Notre Dame, USA
153,
M Wolf
M Wolf
153University of Notre Dame, Notre Dame, USA
153,
A Woodard
A Woodard
153University of Notre Dame, Notre Dame, USA
153,
L Antonelli
L Antonelli
154The Ohio State University, Columbus, USA
154,
J Brinson
J Brinson
154The Ohio State University, Columbus, USA
154,
B Bylsma
B Bylsma
154The Ohio State University, Columbus, USA
154,
L S Durkin
L S Durkin
154The Ohio State University, Columbus, USA
154,
S Flowers
S Flowers
154The Ohio State University, Columbus, USA
154,
A Hart
A Hart
154The Ohio State University, Columbus, USA
154,
C Hill
C Hill
154The Ohio State University, Columbus, USA
154,
R Hughes
R Hughes
154The Ohio State University, Columbus, USA
154,
W Ji
W Ji
154The Ohio State University, Columbus, USA
154,
T Y Ling
T Y Ling
154The Ohio State University, Columbus, USA
154,
B Liu
B Liu
154The Ohio State University, Columbus, USA
154,
W Luo
W Luo
154The Ohio State University, Columbus, USA
154,
D Puigh
D Puigh
154The Ohio State University, Columbus, USA
154,
M Rodenburg
M Rodenburg
154The Ohio State University, Columbus, USA
154,
B L Winer
B L Winer
154The Ohio State University, Columbus, USA
154,
H W Wulsin
H W Wulsin
154The Ohio State University, Columbus, USA
154,
O Driga
O Driga
155Princeton University, Princeton, USA
155,
P Elmer
P Elmer
155Princeton University, Princeton, USA
155,
J Hardenbrook
J Hardenbrook
155Princeton University, Princeton, USA
155,
P Hebda
P Hebda
155Princeton University, Princeton, USA
155,
S A Koay
S A Koay
155Princeton University, Princeton, USA
155,
P Lujan
P Lujan
155Princeton University, Princeton, USA
155,
D Marlow
D Marlow
155Princeton University, Princeton, USA
155,
T Medvedeva
T Medvedeva
155Princeton University, Princeton, USA
155,
M Mooney
M Mooney
155Princeton University, Princeton, USA
155,
J Olsen
J Olsen
155Princeton University, Princeton, USA
155,
C Palmer
C Palmer
155Princeton University, Princeton, USA
155,
P Piroué
P Piroué
155Princeton University, Princeton, USA
155,
H Saka
H Saka
155Princeton University, Princeton, USA
155,
D Stickland
D Stickland
155Princeton University, Princeton, USA
155,
C Tully
C Tully
155Princeton University, Princeton, USA
155,
A Zuranski
A Zuranski
155Princeton University, Princeton, USA
155,
S Malik
S Malik
156University of Puerto Rico, Mayagüez, USA
156,
V E Barnes
V E Barnes
157Purdue University, West Lafayette, USA
157,
D Benedetti
D Benedetti
157Purdue University, West Lafayette, USA
157,
D Bortoletto
D Bortoletto
157Purdue University, West Lafayette, USA
157,
L Gutay
L Gutay
157Purdue University, West Lafayette, USA
157,
M K Jha
M K Jha
157Purdue University, West Lafayette, USA
157,
M Jones
M Jones
157Purdue University, West Lafayette, USA
157,
K Jung
K Jung
157Purdue University, West Lafayette, USA
157,
D H Miller
D H Miller
157Purdue University, West Lafayette, USA
157,
N Neumeister
N Neumeister
157Purdue University, West Lafayette, USA
157,
F Primavera
F Primavera
157Purdue University, West Lafayette, USA
157,
B C Radburn-Smith
B C Radburn-Smith
157Purdue University, West Lafayette, USA
157,
X Shi
X Shi
157Purdue University, West Lafayette, USA
157,
I Shipsey
I Shipsey
157Purdue University, West Lafayette, USA
157,
D Silvers
D Silvers
157Purdue University, West Lafayette, USA
157,
J Sun
J Sun
157Purdue University, West Lafayette, USA
157,
A Svyatkovskiy
A Svyatkovskiy
157Purdue University, West Lafayette, USA
157,
F Wang
F Wang
157Purdue University, West Lafayette, USA
157,
W Xie
W Xie
157Purdue University, West Lafayette, USA
157,
L Xu
L Xu
157Purdue University, West Lafayette, USA
157,
N Parashar
N Parashar
158Purdue University Calumet, Hammond, USA
158,
J Stupak
J Stupak
158Purdue University Calumet, Hammond, USA
158,
A Adair
A Adair
159Rice University, Houston, USA
159,
B Akgun
B Akgun
159Rice University, Houston, USA
159,
Z Chen
Z Chen
159Rice University, Houston, USA
159,
K M Ecklund
K M Ecklund
159Rice University, Houston, USA
159,
F J M Geurts
F J M Geurts
159Rice University, Houston, USA
159,
M Guilbaud
M Guilbaud
159Rice University, Houston, USA
159,
W Li
W Li
159Rice University, Houston, USA
159,
B Michlin
B Michlin
159Rice University, Houston, USA
159,
M Northup
M Northup
159Rice University, Houston, USA
159,
B P Padley
B P Padley
159Rice University, Houston, USA
159,
R Redjimi
R Redjimi
159Rice University, Houston, USA
159,
J Roberts
J Roberts
159Rice University, Houston, USA
159,
J Rorie
J Rorie
159Rice University, Houston, USA
159,
Z Tu
Z Tu
159Rice University, Houston, USA
159,
J Zabel
J Zabel
159Rice University, Houston, USA
159,
B Betchart
B Betchart
160University of Rochester, Rochester, USA
160,
A Bodek
A Bodek
160University of Rochester, Rochester, USA
160,
P de Barbaro
P de Barbaro
160University of Rochester, Rochester, USA
160,
R Demina
R Demina
160University of Rochester, Rochester, USA
160,
Y Eshaq
Y Eshaq
160University of Rochester, Rochester, USA
160,
T Ferbel
T Ferbel
160University of Rochester, Rochester, USA
160,
M Galanti
M Galanti
160University of Rochester, Rochester, USA
160,
M Galanti
M Galanti
160University of Rochester, Rochester, USA
160,
A Garcia-Bellido
A Garcia-Bellido
160University of Rochester, Rochester, USA
160,
J Han
J Han
160University of Rochester, Rochester, USA
160,
A Harel
A Harel
160University of Rochester, Rochester, USA
160,
O Hindrichs
O Hindrichs
160University of Rochester, Rochester, USA
160,
O Hindrichs
O Hindrichs
160University of Rochester, Rochester, USA
160,
A Khukhunaishvili
A Khukhunaishvili
160University of Rochester, Rochester, USA
160,
G Petrillo
G Petrillo
160University of Rochester, Rochester, USA
160,
P Tan
P Tan
160University of Rochester, Rochester, USA
160,
M Verzetti
M Verzetti
160University of Rochester, Rochester, USA
160,
S Arora
S Arora
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
A Barker
A Barker
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
J P Chou
J P Chou
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
C Contreras-Campana
C Contreras-Campana
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
E Contreras-Campana
E Contreras-Campana
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
D Ferencek
D Ferencek
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
Y Gershtein
Y Gershtein
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
R Gray
R Gray
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
E Halkiadakis
E Halkiadakis
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
D Hidas
D Hidas
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
E Hughes
E Hughes
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
S Kaplan
S Kaplan
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
R Kunnawalkam Elayavalli
R Kunnawalkam Elayavalli
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
A Lath
A Lath
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
K Nash
K Nash
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
S Panwalkar
S Panwalkar
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
M Park
M Park
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
S Salur
S Salur
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
S Schnetzer
S Schnetzer
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
D Sheffield
D Sheffield
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
S Somalwar
S Somalwar
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
R Stone
R Stone
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
S Thomas
S Thomas
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
P Thomassen
P Thomassen
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
M Walker
M Walker
161Rutgers, The State University of New Jersey, Piscataway, USA
161,
M Foerster
M Foerster
162University of Tennessee, Knoxville, USA
162,
G Riley
G Riley
162University of Tennessee, Knoxville, USA
162,
K Rose
K Rose
162University of Tennessee, Knoxville, USA
162,
S Spanier
S Spanier
162University of Tennessee, Knoxville, USA
162,
A York
A York
162University of Tennessee, Knoxville, USA
162,
O Bouhali
O Bouhali
163Texas A&M University, College Station, USA
163,
A Castaneda Hernandez
A Castaneda Hernandez
163Texas A&M University, College Station, USA
163,
A Celik
A Celik
163Texas A&M University, College Station, USA
163,
M Dalchenko
M Dalchenko
163Texas A&M University, College Station, USA
163,
M De Mattia
M De Mattia
163Texas A&M University, College Station, USA
163,
A Delgado
A Delgado
163Texas A&M University, College Station, USA
163,
S Dildick
S Dildick
163Texas A&M University, College Station, USA
163,
S Dildick
S Dildick
163Texas A&M University, College Station, USA
163,
R Eusebi
R Eusebi
163Texas A&M University, College Station, USA
163,
J Gilmore
J Gilmore
163Texas A&M University, College Station, USA
163,
T Huang
T Huang
163Texas A&M University, College Station, USA
163,
T Kamon
T Kamon
163Texas A&M University, College Station, USA
163,
V Krutelyov
V Krutelyov
163Texas A&M University, College Station, USA
163,
V Krutelyov
V Krutelyov
163Texas A&M University, College Station, USA
163,
R Mueller
R Mueller
163Texas A&M University, College Station, USA
163,
I Osipenkov
I Osipenkov
163Texas A&M University, College Station, USA
163,
Y Pakhotin
Y Pakhotin
163Texas A&M University, College Station, USA
163,
R Patel
R Patel
163Texas A&M University, College Station, USA
163,
R Patel
R Patel
163Texas A&M University, College Station, USA
163,
A Perloff
A Perloff
163Texas A&M University, College Station, USA
163,
A Rose
A Rose
163Texas A&M University, College Station, USA
163,
A Safonov
A Safonov
163Texas A&M University, College Station, USA
163,
A Tatarinov
A Tatarinov
163Texas A&M University, College Station, USA
163,
K A Ulmer
K A Ulmer
163Texas A&M University, College Station, USA
163,
N Akchurin
N Akchurin
164Texas Tech University, Lubbock, USA
164,
C Cowden
C Cowden
164Texas Tech University, Lubbock, USA
164,
J Damgov
J Damgov
164Texas Tech University, Lubbock, USA
164,
C Dragoiu
C Dragoiu
164Texas Tech University, Lubbock, USA
164,
P R Dudero
P R Dudero
164Texas Tech University, Lubbock, USA
164,
J Faulkner
J Faulkner
164Texas Tech University, Lubbock, USA
164,
S Kunori
S Kunori
164Texas Tech University, Lubbock, USA
164,
K Lamichhane
K Lamichhane
164Texas Tech University, Lubbock, USA
164,
S W Lee
S W Lee
164Texas Tech University, Lubbock, USA
164,
T Libeiro
T Libeiro
164Texas Tech University, Lubbock, USA
164,
S Undleeb
S Undleeb
164Texas Tech University, Lubbock, USA
164,
I Volobouev
I Volobouev
164Texas Tech University, Lubbock, USA
164,
E Appelt
E Appelt
165Vanderbilt University, Nashville, USA
165,
A G Delannoy
A G Delannoy
165Vanderbilt University, Nashville, USA
165,
S Greene
S Greene
165Vanderbilt University, Nashville, USA
165,
A Gurrola
A Gurrola
165Vanderbilt University, Nashville, USA
165,
R Janjam
R Janjam
165Vanderbilt University, Nashville, USA
165,
W Johns
W Johns
165Vanderbilt University, Nashville, USA
165,
C Maguire
C Maguire
165Vanderbilt University, Nashville, USA
165,
Y Mao
Y Mao
165Vanderbilt University, Nashville, USA
165,
A Melo
A Melo
165Vanderbilt University, Nashville, USA
165,
H Ni
H Ni
165Vanderbilt University, Nashville, USA
165,
P Sheldon
P Sheldon
165Vanderbilt University, Nashville, USA
165,
B Snook
B Snook
165Vanderbilt University, Nashville, USA
165,
S Tuo
S Tuo
165Vanderbilt University, Nashville, USA
165,
J Velkovska
J Velkovska
165Vanderbilt University, Nashville, USA
165,
Q Xu
Q Xu
165Vanderbilt University, Nashville, USA
165,
M W Arenton
M W Arenton
166University of Virginia, Charlottesville, USA
166,
B Cox
B Cox
166University of Virginia, Charlottesville, USA
166,
B Francis
B Francis
166University of Virginia, Charlottesville, USA
166,
J Goodell
J Goodell
166University of Virginia, Charlottesville, USA
166,
R Hirosky
R Hirosky
166University of Virginia, Charlottesville, USA
166,
A Ledovskoy
A Ledovskoy
166University of Virginia, Charlottesville, USA
166,
H Li
H Li
166University of Virginia, Charlottesville, USA
166,
C Lin
C Lin
166University of Virginia, Charlottesville, USA
166,
C Neu
C Neu
166University of Virginia, Charlottesville, USA
166,
T Sinthuprasith
T Sinthuprasith
166University of Virginia, Charlottesville, USA
166,
X Sun
X Sun
166University of Virginia, Charlottesville, USA
166,
Y Wang
Y Wang
166University of Virginia, Charlottesville, USA
166,
E Wolfe
E Wolfe
166University of Virginia, Charlottesville, USA
166,
J Wood
J Wood
166University of Virginia, Charlottesville, USA
166,
F Xia
F Xia
166University of Virginia, Charlottesville, USA
166,
C Clarke
C Clarke
167Wayne State University, Detroit, USA
167,
R Harr
R Harr
167Wayne State University, Detroit, USA
167,
P E Karchin
P E Karchin
167Wayne State University, Detroit, USA
167,
C Kottachchi Kankanamge Don
C Kottachchi Kankanamge Don
167Wayne State University, Detroit, USA
167,
P Lamichhane
P Lamichhane
167Wayne State University, Detroit, USA
167,
J Sturdy
J Sturdy
167Wayne State University, Detroit, USA
167,
D A Belknap
D A Belknap
167Wayne State University, Detroit, USA
167,
D Carlsmith
D Carlsmith
168University of Wisconsin, Madison, USA
168,
M Cepeda
M Cepeda
168University of Wisconsin, Madison, USA
168,
S Dasu
S Dasu
168University of Wisconsin, Madison, USA
168,
L Dodd
L Dodd
168University of Wisconsin, Madison, USA
168,
S Duric
S Duric
168University of Wisconsin, Madison, USA
168,
B Gomber
B Gomber
168University of Wisconsin, Madison, USA
168,
M Grothe
M Grothe
168University of Wisconsin, Madison, USA
168,
R Hall-Wilton
R Hall-Wilton
168University of Wisconsin, Madison, USA
168,
M Herndon
M Herndon
168University of Wisconsin, Madison, USA
168,
A Hervé
A Hervé
168University of Wisconsin, Madison, USA
168,
P Klabbers
P Klabbers
168University of Wisconsin, Madison, USA
168,
A Lanaro
A Lanaro
168University of Wisconsin, Madison, USA
168,
A Levine
A Levine
168University of Wisconsin, Madison, USA
168,
K Long
K Long
168University of Wisconsin, Madison, USA
168,
R Loveless
R Loveless
168University of Wisconsin, Madison, USA
168,
A Mohapatra
A Mohapatra
168University of Wisconsin, Madison, USA
168,
I Ojalvo
I Ojalvo
168University of Wisconsin, Madison, USA
168,
T Perry
T Perry
168University of Wisconsin, Madison, USA
168,
G A Pierro
G A Pierro
168University of Wisconsin, Madison, USA
168,
G Polese
G Polese
168University of Wisconsin, Madison, USA
168,
T Ruggles
T Ruggles
168University of Wisconsin, Madison, USA
168,
T Sarangi
T Sarangi
168University of Wisconsin, Madison, USA
168,
A Savin
A Savin
168University of Wisconsin, Madison, USA
168,
A Sharma
A Sharma
168University of Wisconsin, Madison, USA
168,
N Smith
N Smith
168University of Wisconsin, Madison, USA
168,
W H Smith
W H Smith
168University of Wisconsin, Madison, USA
168,
D Taylor
D Taylor
168University of Wisconsin, Madison, USA
168,
N Woods
N Woods
168University of Wisconsin, Madison, USA
168;
CMS Collaboration169