Temperature and atmospheric CO₂ concentration estimates through the PETM using triple oxygen isotope analysis of mammalian bioapatite

Significance

Our data suggest that the sudden rise in atmospheric temperature during the Paleocene–Eocene transition was not accompanied by highly elevated carbon dioxide concentrations >∼2,500 ppm. Instead, the low ¹³C/¹²C isotope ratios during the Paleocene–Eocene Thermal Maximum were most likely caused by a significant contribution of methane to the atmosphere. We present data applying a newly developed partial pressure of CO₂ proxy.

Abstract

The Paleocene–Eocene Thermal Maximum (PETM) is a remarkable climatic and environmental event that occurred 56 Ma ago and has importance for understanding possible future climate change. The Paleocene–Eocene transition is marked by a rapid temperature rise contemporaneous with a large negative carbon isotope excursion (CIE). Both the temperature and the isotopic excursion are well-documented by terrestrial and marine proxies. The CIE was the result of a massive release of carbon into the atmosphere. However, the carbon source and quantities of CO₂ and CH₄ greenhouse gases that contributed to global warming are poorly constrained and highly debated. Here we combine an established oxygen isotope paleothermometer with a newly developed triple oxygen isotope paleo-CO₂ barometer. We attempt to quantify the source of greenhouse gases released during the Paleocene–Eocene transition by analyzing bioapatite of terrestrial mammals. Our results are consistent with previous estimates of PETM temperature change and suggest that not only CO₂ but also massive release of seabed methane was the driver for CIE and PETM.

The Paleocene–Eocene transition at about 56 Ma (1–3) is marked by an abrupt climate change in conjunction with a large negative carbon isotope excursion (CIE), ocean acidification, and enhanced terrestrial runoff—during a period lasting less than 220,000 y (e.g., refs. 4–7). During the CIE a global temperature increase of 5–8 °C has been inferred from a variety of marine and terrestrial proxies (e.g., refs. 3 and 8–21), hence the name “Paleocene–Eocene Thermal Maximum,” or PETM is frequently applied to the transition interval.

The CIE is well-documented by proxy data, and it is largely accepted that it was caused by the addition of a large amount of ¹³C-depleted carbon into the exogenic carbon cycle (ref. 22 and references therein). Considering the atmospheric CIE to be between –4 and –5‰ (ref. 22 and references therein and refs. 23 and 24), the suggested amount of carbon needed to cause this negative carbon isotope shift ranges between 2,200 and 153,000 Gt, a range depending on the isotope composition of the hypothesized carbon source and secondarily on the model approach used (22, 24–27). Possible carbon sources are biogenic methane (i.e., methane clathrates, δ¹³C ∼–60‰), thermogenic methane or permafrost soil carbon (δ¹³C ∼–30‰), carbon released due to wildfires or through desiccation and oxidation of organic matter due to drying of epicontinental seas (δ¹³C ∼–22‰), and mantle CO₂ (δ¹³C ∼–5‰) (22, 28).

A massive release of methane clathrates by thermal dissociation (29) has been the most convincing hypothesis to explain the CIE since it was first identified. However, a methane clathrate source is still debated, and an involvement of other carbon sources—exclusively or additionally—is widely discussed (e.g., refs. 22, 26, and 28).

Global warming during the CIE is attributed to radiative forcing due to increased concentrations of greenhouse gases, most likely CO₂ and/or CH₄, and to accessory phenomena such as aerosol forcing and reduced cloudiness (26, 30).

Substantially different CO₂ scenarios have been discussed for the PETM. Mass balance estimates range from an increase in atmospheric CO₂ concentration of less than 100 ppm to an increase of several 10,000 ppm, depending on the proposed magnitude of the CIE and the associated carbon source (10, 22, 24, 25, 27, 31–34).

Existing proxy data for CO₂ concentrations in the time interval surrounding the PETM (50–60 Ma) suggest background partial pressure of CO₂ (pCO₂) levels between 100 and 1,900 ppm (ref. 35 and references therein). Only a single estimate (36) of the previously published data is questionably correlated to PETM strata (670 ppm).

From increased insect herbivory during the PETM Currano et al. (37, 38) suggested a three- to fourfold increase in atmospheric CO₂ but left open the possibility that increased herbivory was an effect of higher insect diversity and density due to the elevated temperature.

Here we bring evidence to bear on both temperatures and CO₂ concentrations through the Paleocene–Eocene transition based on triple oxygen isotope measurements (δ¹⁷O, δ¹⁸O) of bioapatite in mammalian tooth enamel.

The oxygen isotopic composition of mammalian body water is determined by its oxygen sources and sinks (Figs. S1 and S2). The main oxygen input sources for mammals are drinking water, food water, and inhaled air O₂. Sinks are feces, sweat, water loss due to transpiration, as well as exhaled CO₂ and moisture. The fractionation between δ¹⁸O of body water and δ¹⁸O of bioapatite in mammals is constant due to a constant body temperature of ∼37 °C, and it has been demonstrated that it is possible to relate the δ¹⁸O of mammalian bioapatite to the oxygen isotope composition of drinking water (i.e., environmental surface water, δ¹⁸O_SW) by empirically developed calibration equations or by modeling approaches (39–44). Due to the strong correlation between δ¹⁸O_SW and mean annual (air) temperatures (MAT) (45), it is in turn possible to infer paleotemperatures using the oxygen isotope composition of δ¹⁸O_SW reconstructed from δ¹⁸O of mammalian bioapatite. This was first applied extensively by Bryant et al. (46). These studies rely solely on δ¹⁸O because, in nearly all terrestrial fractionation processes, δ¹⁸O is correlated to δ¹⁷O by the equation δ¹⁷O ≈ 0.52 × δ¹⁸O (e.g., ref. 47 and references therein).

Fig. S1.

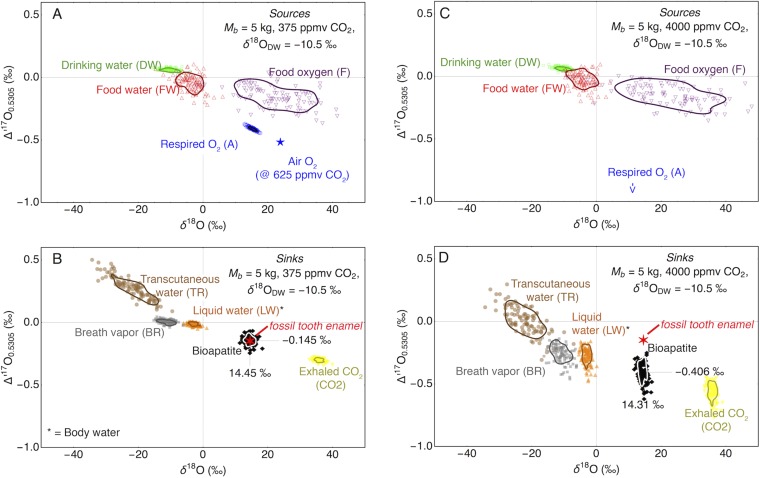
Plot of Δ¹⁷O vs. δ¹⁸O for sources (A) and sinks, BW, and bioapatite (B) for a scenario with 375 ppm CO₂. The mass balance was calculated for M_b = 5 kg and model δ¹⁸O_DW = –10.5 ‰. The ellipses outline the 1σ SD that was computed calculated by Monte Carlo simulation (n = 100). The uncertainties of the individual input parameters were taken from Pack et al. (50). The composition of fossil tooth enamel [sample E. osbornianus (Wa-2, locality SC-2), red star] is shown for comparison. (B and C) The same scenario as in A with an atmospheric CO₂ mixing ratio of 4,000 ppm. The model bioapatite (D, black diamonds) has much lower Δ¹⁷O than the analyzed bioapatite (red star).

Fig. S2.

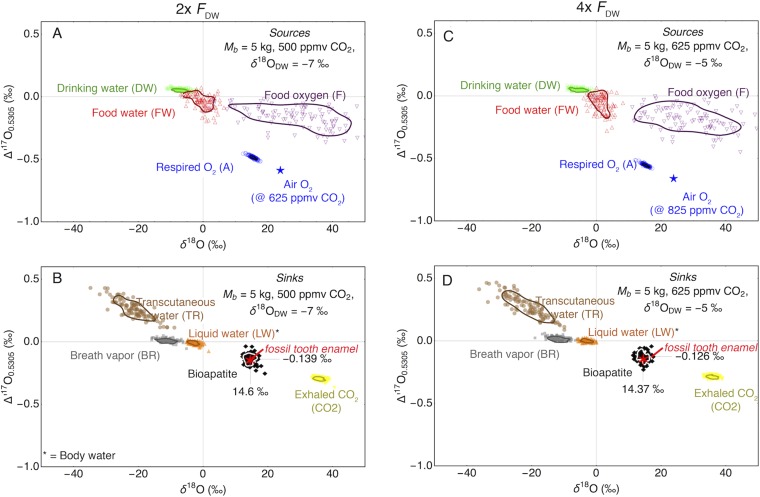
Mass balance calculation for enhanced water flow (2×, A and B and 4×, C and D) higher than inferred from the allometric scaling; all other parameters were the same as used in the model illustrated in Fig. S1). The main difference is the model δ¹⁸O_DW, which increases with increasing F_DW. This reflects the increasing dominance of drinking water as controlling factor for the isotope composition of BW and bioapatite.

However, air O₂, which is one of the main oxygen sources of mammalian body water, carries an isotope anomaly in ¹⁷O (48) that is related to mass independent processes in the stratosphere. The isotope anomaly of inhaled air O₂ is transferred to the body water and hence to mammalian bioapatite. The ¹⁷O anomaly of air O₂ scales with atmospheric CO₂ level and gross primary productivity (GPP) (49), and thus triple oxygen isotope analyses of bioapatite from fossil mammals can be used as a paleo-CO₂ proxy (see ref. 50 for more details).

The magnitude of the ¹⁷O anomaly in mammalian bioapatite is connected to the fraction of inhaled anomalous air O₂ in relation to the other oxygen sources. Due to higher specific metabolic rates (i.e., higher specific O₂ respiration), smaller mammals carry a higher portion of anomalous oxygen in their bioapatite than larger mammals (50).

Studies relying on the relationship of paleotemperature to δ¹⁸O in mammalian bioapatite already exist for the Paleocene–Eocene transition in the Clarks Fork and Bighorn Basin (Wyoming) based on various mammalian taxa, targeting either the carbonate oxygen component (δ¹⁸O_CO3) of mammalian tooth enamel, the phosphate oxygen component (δ¹⁸O_PO4), or both in a combined approach (8, 17, 20, 21, 51–53).

In the present study, we combine this well-established δ¹⁸O paleothermometer (e.g., refs. 39 and 40) with the recently published discovery of a connection between the triple oxygen isotope composition of mammalian bioapatite and atmospheric CO₂ concentration (50).

Materials and Methods

Samples.

The most suitable taxa for an application of the approach described above should have a body weight as small as possible (to ensure a high proportion of inhaled anomalous air oxygen in respect to the other oxygen sources) but large enough (with respect to fossil samples) to provide an adequate amount of diagenetically unaltered sample material (i.e., tooth enamel) (e.g., refs. 54 and 55).

We sampled tooth enamel from teeth from jaws and isolated teeth of 11 individual specimens of the phenacodontid genus Ectocion (Ectocion osbornianus and Ectocion parvus) from the Clarks Fork Basin (Sand Coulee area) in northwestern Wyoming (e.g., refs. 56 and 57). The enamel was carefully hand-picked under a binocular microscope before pretreatment. The samples cover a time interval of several mammalian biozones, from the late Clarkforkian (Cf-3) to the early Wasatchian (Wa-2), encompassing the PETM. All samples were acquired from the collections of the Museum of Paleontology at the University of Michigan, Ann Arbor (localities SC-2, SC-11, SC-67, SC-107, SC-138, and SC-161). All were molded and cast before destructive sampling. Only second molars (M₂) were used for the present study to obtain the highest possible comparability.

Sample Pretreatment.

To ensure analysis was limited to the phosphate oxygen isotope composition (δ¹⁸O_PO4), other oxygen-bearing components of the sample material (e.g., organic matter, sorbed water, structural carbonate, and OH^– groups) were removed by treating the tooth enamel in an Ar-flushed horizontal tube furnace for 10–15 min at 1,000 °C. Subsequently, the samples were cooled to below 100 °C while remaining in an Ar atmosphere, followed by immediate storage in a desiccator until analysis (50). This procedure follows Lindars et al. (58), who demonstrated safe removal of all of the unwanted compounds at temperatures between 850 and 1,000 °C. Ar is used to avoid exchange with atmospheric water, which was reported to be a problem by Lindars et al. (58) when heating samples to 1,000 °C in ambient air.

Triple Oxygen Isotope Analysis.

Variations in the oxygen isotope ratios (¹⁷O/¹⁶O, ¹⁸O/¹⁶O) are reported relative to the international isotope reference standard Vienna Standard Mean Ocean Water 2 in the form of the δ¹⁷O and δ¹⁸O notation (59). In mass-dependent (equilibrium or kinetic) fractionation processes, variations in δ¹⁷O broadly correlate with variations in δ¹⁸O. To better display small deviations from an otherwise good correlation, the Δ¹⁷O value has been introduced. We follow the definition scheme for Δ¹⁷O as presented in Pack and Herwartz (60) with Δ¹⁷O = 1,000*ln(δ¹⁸O/1,000 + 1) – 0.5305*1,000*ln(δ¹⁸O/1,000 + 1). The Δ¹⁷O has been normalized to a revised composition of NBS 28 quartz with δ¹⁷O = 5.03‰ and δ¹⁸O = 9.60‰ (Δ¹⁷O = −0.05‰).

A slope derived from analytical data of terrestrial rocks and minerals as done in previous studies (50, 55) has not been used because of its arbitrary selection depending on the specific samples analyzed to obtain it. However, the data can easily be converted for comparison with results from other studies and/or other laboratories.

Oxygen was released from the samples by infrared laser fluorination (61) and analyzed by gas chromatography isotope ratio monitoring gas mass spectrometry in continuous flow mode (e.g., refs. 50, 55, and 62). Typically, ∼0.3 mg of pretreated fossil tooth enamel was loaded into an 18-pit polished Ni sample holder along with ∼0.3 mg of pretreated tooth enamel from a modern African Elephant (Loxodonta africana) as an internal standard and ∼0.2 mg NBS 28 quartz. Following evacuation and heating of the sample chamber to 70 °C for at least 12 h, fluorination was implemented by heating the samples with a SYNRAD 50 W CO₂ laser in a ∼20–30 mbar atmosphere of F₂ gas, purified according to Asprey (63). Liberated excess F₂ was reacted with heated NaCl (∼180 °C) to NaF and Cl₂. The latter was cryotrapped by liquid N₂ until all samples were analyzed. Sample O₂ was cryofocused on a molecular sieve trap at –196 °C (liquid N₂) then expanded into a stainless steel capillary and transported with He as carrier gas through a second molecular sieve trap, where a fraction of the sample gas was again cryofocused at –196 °C. After releasing this back into the He carrier gas stream by heating it in a hot water bath (∼92 °C), the sample O₂ was purified by passing it through a 5-Å molecular sieve GC column of a Thermo Scientific GasBench II and then injected via an open split valve of the GasBench II into the source of a Thermo MAT 253 gas mass spectrometer. The signals of ¹⁶O¹⁶O, ¹⁷O¹⁶O, and ¹⁸O¹⁶O were simultaneously monitored on three Faraday cups. Reference O₂ was injected before each sample measurement through a second open split valve of the GasBench II. Sample and reference gas peaks (m/z = 32) had an amplitude of 20–30 V.

The SD for replicates of the same bioapatite samples from different runs is typically better than ±0.5‰ in Δ¹⁸O and ± 0.04‰ in Δ¹⁷O for a single analysis.

A total of 72 single laser fluorination analyses were made using 14 different Ectocion tooth enamel samples (from the 11 Ectocion specimens used for the present study, 3 were sampled twice to have a reproducibility control between different analytical runs) (Table 1).

Table 1.

Oxygen isotope composition (δ¹⁸O_PO4, Δ¹⁷O_PO4) of tooth enamel from second molars of Ectocion osbornianus and Ectocion parvus from the Clarks Fork Basin in north-western Wyoming

Species	Biozone	Level, m	Locality	Sample no.	δ18OPO4, ‰	Δ17OPO4, ‰	N	pCO2 (model), ppm by volume		pCO2 (model, 2.3× GPP), ppm by volume
E. osbornianus	Wa-2	1,720	SC-2	85906	13.0 ± 0.4	−0.17 ± 0.04	5	500	−400 +600	1,150	−1,000 +1,400
E.osbornianus	Wa-2	1,720	SC-2	66572 (sample A)	12.4 ± 0.3	−0.18 ± 0.02	8	625	−400 +600	1,440	−1,000 +1,400
E.osbornianus	Wa-2	1,720	SC-2	66572 (sample B)	13.0 ± 0.5	−0.19 ± 0.03	7	750	−400 +600	1,730	−1,000 +1,400
Mean					12.8 ± 0.2	-0.18 ± 0.02	20	630	−230 +350	1,450	−500 +800
E.osbornianus	Wa-2	1,665	SC-161	80705	13.5 ± 0.4	−0.14 ± 0.02	3	225	−200 +400	520	−500 +1,000
E.osbornianus	Wa-2	1,665	SC-161	98404	13.2 ± 0.3	−0.13 ± 0.03	4	200	−200 +400	460	−500 +1,000
E.osbornianus	Wa-2	1,665	SC-161	68200	12.3 ± 0.3	−0.15 ± 0.03	4	250	−250 +500	580	−600 +1,200
Mean					13.0 ± 0.2	-0.14 ± 0.02	11	230	−200 +320	530	−460 +750
E.parvus	Wa-0	1,520	SC-67	83478	14.3 ± 0.3	−0.15 ± 0.04	2	250	−200 +400	580	−500 +1,000
E.parvus	Wa-0	1,520	SC-67	87354	14.3 ± 0.6	−0.18 ± 0.03	5	625	−400 +600	1,440	−1,000 +1,400
E.parvus	Wa-0	1,520	SC-67	86572 (sample A)	15.1 ± 0.2	−0.17 ± 0.03	7	625	−400 +600	1,440	−1,000 +1,400
E.parvus	Wa-0	1,520	SC-67	86572 (sample B)	14.4 ± 0.3	−0.15 ± 0.03	2	250	−200 +400	580	−500 +1,000
Mean					14.5 ± 0.2	-0.16 ± 0.02	16	440	−160 +250	1,010	−400 +600
E.osbornianus	Cf-3	1,502	SC-107	66621	11.0 ± 0.5	−0.14 ± 0.03	11	250	−250 +500	580	−600 +1,200
E.osbornianus	Cf-3	1,495	SC-138	67243 (sample A)	13.7 ± 0.3	−0.16 ± 0.03	7	500	−400 +500	1,150	−1,000 +1,200
E.osbornianus	Cf-3	1,495	SC-138	67243 (sample B)	13.8 ± 0.6	−0.19 ± 0.05	4	825	−400 +600	1,900	−1,000 +1,400
E.osbornianus	Cf-3	1,485	SC-11	64726	12.1 ± 0.3	−0.14 ± 0.02	3	225	−200 +500	520	−500 +1,200
Mean					12.7 ± 0.2	−0.16 ± 0.02	25	450	−200 +260	1,040	−450 +600

The reported pCO₂ data were calculated for modern gross primary productivity (GPP) and 2.3× elevated GPP on basis of the mass balance model (for details, see Supporting Information). Uncertainty estimates are ±1σ SD.

Results and Discussion

Temperature Change Across the PETM Inferred from δ¹⁸O of Ectocion Tooth Enamel.

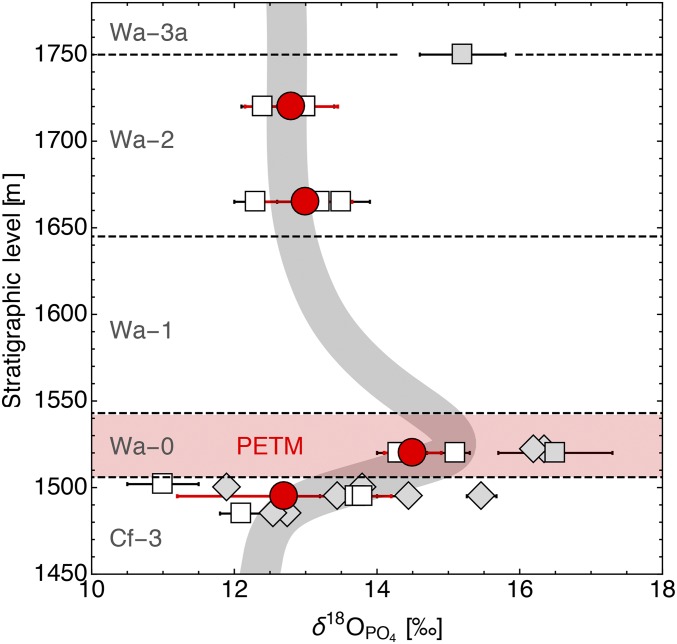
The mean oxygen isotope composition of tooth enamel phosphate (δ¹⁸O_PO4) in the Ectocion specimens investigated increases by 2.2‰ from the late Clarkforkian (Cf-3) to the CIE in the early Wasatchian (Wa-0), considering the mean values for each mammalian biozone. From Wa-0 to Wa-2, a decrease in mean δ¹⁸O_PO4 of 1.6‰ is observed (different meter levels within Wa-2 do not show any difference in δ¹⁸O_PO4, so they were combined (Fig. 1 and Tables 1 and 2). This is in agreement with previous results from δ¹⁸O_PO4 (17, 20), as well as δ¹⁸O_CO3 (17, 20, 21) for mammals covering the same biozones in the same study area. To estimate the change of δ¹⁸O_SW from δ¹⁸O_PO4, we used the relationship for modern herbivorous mammals (δ¹⁸O_SW = 1.32 × δ¹⁸O_PO4), which Secord et al. (20) adapted from Kohn et al. (64).

Fig. 1.

Oxygen isotope composition (δ¹⁸O_PO4, ±1σ SD) of Ectocion (white squares, this study; Table 1), Phenacodus [gray diamonds; Secord et al. (20)], and Coryphodon specimens [gray squares; Fricke et al. (17)]. Red circles are mean values for Ectocion (this study). Missing error bars indicate single analyses. The stratigraphic level is expressed in meters above the K-T boundary in the Clarks Fork Basin. Age of Ectocion samples is in the range of −56 to −55 Ma (see Fig. 2).

Table 2.

Estimated change in δ¹⁸O_SW and temperature at the Paleocene–Eocene transition in the Clarks Fork and Bighorn Basin

Biozone interval	Δ18OPO4, ‰	Δ18OSW, ‰	ΔT (0.58‰/°C)	ΔT (0.39‰/°C)	ΔT (0.36‰/°C)
This study
Wa-0 to Wa-2	−1.6	−2.1	−3.6	−5.4	−5.8
Cf-3 to Wa-0	+2.2	+2.9	+5.0	+7.4	+8.1
Secord et al. (20)
Cf-3 to Wa-0	+2.1	+2.8	+4.8	+7.2	+7.8
Fricke et al. (17)
Wa-0 to Wa-3a	−1.4	−1.8	−3.1	−4.6	−5.0
Cf-2 to Wa-0	+1.8	+2.4	+4.1	+6.2	+6.7

Based on δ¹⁸O_PO4 of Ectocion (this study), Phenacodus [Secord et al. (20)] and Coryphodon [Fricke et al. (17)], for three different MAT/δ¹⁸O_SW slopes [the modern and two calculated values for early Eocene conditions from Secord et al. (20)].

Applying this to our results, δ¹⁸O_SW became enriched by 2.9‰ from Cf-3 to Wa-0 followed by a depletion of 2.1‰ to Wa-2 (Table 2).

The δ¹⁸O_SW/MAT slope is sensitive to the latitudinal gradient and other factors (e.g., ref. 65), so an important precondition for estimating the corresponding change in temperature is to adjust this slope as closely as possible to early Cenozoic conditions. The modern temperature dependence for a MAT of 0–20 °C ranges between 0.5 and 0.6‰ per degree Celsius (66–68). During the Paleocene–Eocene transition in the Bighorn Basin, Secord et al. (20) proposed δ¹⁸O_SW/MAT slopes of 0.39 and 0.36 (per mille per degree Celsius), based on two different approaches.

For the Ectocion tooth enamel data of the present study, this implies an increase of 7.4 °C (8.1 °C) from Cf-3 to Wa-0 and a decrease of 5.4 °C (5.8 °C) from Wa-0 to Wa-2 (the value in parentheses refers to the slope for 0.36). These results are in general agreement with previous studies from Fricke et al. (17) and Secord et al. (20) based on tooth enamel δ¹⁸O_PO4 of Coryphodon and Phenacodus, respectively. Calculated on the same basis, the data from Fricke et al. (17) indicate a temperature increase of 6.2 °C (6.7 °C) from Cf-2 to Wa-0 and a decrease of 4.6 °C (5.0 °C) from Wa-0 to Wa-3. The data from Secord et al. (20) suggest a temperature increase of 7.2 °C (7.8 °C) from Cf-3 to Wa-0, if mean biozone values are used (Table 2).

Atmospheric CO₂ Levels Across the PETM Inferred from δ¹⁷O of Ectocion Tooth Enamel.

Tooth enamel of the investigated Ectocion specimens has a distinctly negative ¹⁷O anomaly, with Δ¹⁷O ranging between –0.19 and –0.13‰ (Table 1). The anomaly is due to the portion of oxygen in the bioapatite that comes from inhaled isotopically anomalous air O₂ (50). To estimate the Δ¹⁷O of PETM air oxygen from the tooth enamel measurements, we used the oxygen mass balance model of Pack et al. (50), developed for terrestrial herbivorous mammals (for details see Supporting Information). The body weights of E. osbornianus and E. parvus are estimated to have been 9 and 5 kg, respectively (e.g., refs. 69 and 70).

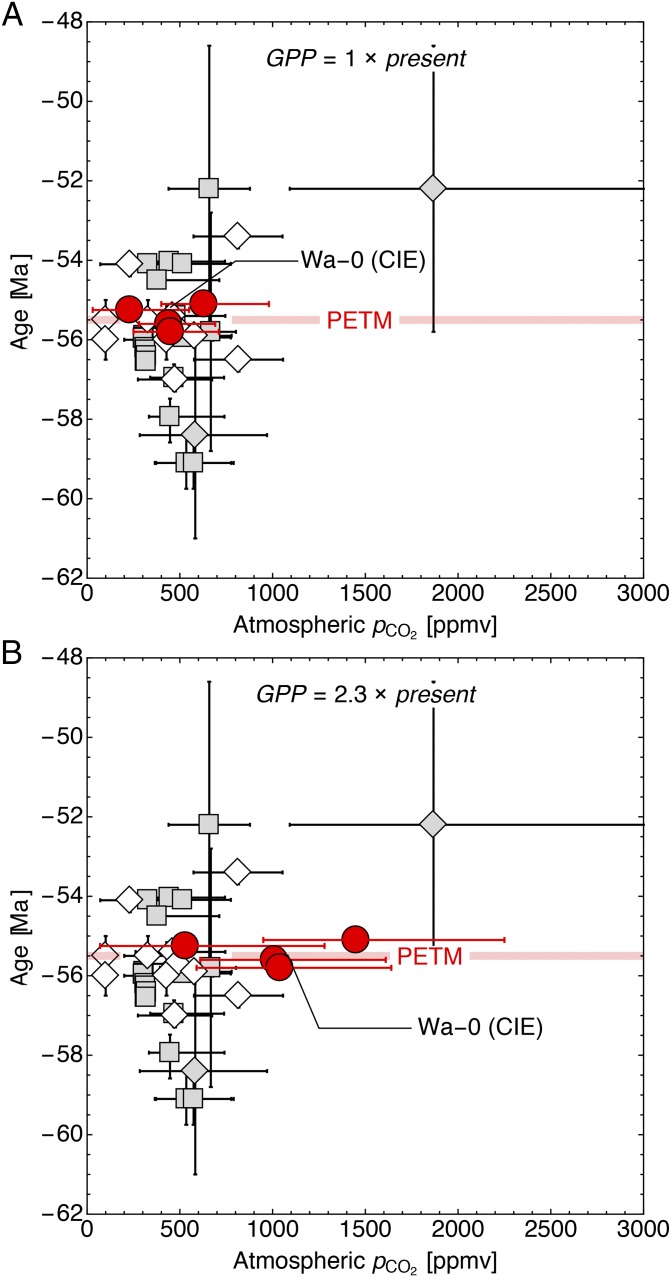
Combining the approach of Pack et al. (50) with the linear relationship between Δ¹⁷O_air and pCO₂ given by Bao et al. (49), the estimated mean pCO₂ values are 450, 440, 230, and 630 ppm for Cf-3 (1,485- to 1,502-m level), Wa-0 (1,520-m level), Wa-2 (1,665-m level), and Wa-2 (1,720-m level), respectively (Fig. 2A and Table 1). The uncertainty estimate is in the range of ± several hundred ppm. However, these numbers are based on modern O₂ and GPP conditions. Whereas pO₂ remains more or less unchanged within the last 60 Ma (71), a considerably different GPP of 2.3 times the modern value has been estimated by Beerling (72) for the early Eocene. Because a doubling of GPP results in a halving of Δ¹⁷O_air (49, 50), a 2.3 GPP would imply pCO₂ mean values of 1,040, 1,010, 530, and 1,450 ppm for Cf-3 (1,485- to 1,502-m level), Wa-0 (1,520-m level), Wa-2 (1,665-m level), and Wa-2 (1,720-m level), respectively (Fig. 2B and Table 1).

Fig. 2.

Existing proxy data for pCO₂ between 50 and 60 Ma adapted from the compilation of Beerling and Royer (35), compared with pCO₂ estimates from Δ¹⁷O of Ectocion bioapatite (red circles, this study). pCO₂ has been calculated for (A) modern global GPP conditions and (B) GPP 2.3 times the modern value, as proposed by Berner (71). White diamonds, paleosols; gray squares, leaf stomata; white squares, marine phytoplankton; gray diamonds, liverworts. pCO₂ results here are the first to our knowledge to come unquestionably from the PETM interval. Note that pCO₂ results for the PETM here are not elevated but consistent with earlier and later pCO₂ values reported by Beerling and Royer (35).

The results for the modern GPP scenario agree well with other proxy data for the time slice between 50 and 60 Ma from δ¹³C of paleosols (1, 36, 73), leaf stomata (36, 74–76), and δ¹³C of marine phytoplankton (77), which nearly all range between 300 and 800 ppm (Fig. 2B). A few paleosol estimates propose a considerably lower pCO₂ of ∼100 ppm [ref. 78, reevaluated by Breecker et al. (73)], and a single estimate from δ¹³C of fossil liverworts (79) suggests a considerably higher pCO₂ of ∼1,900 ppm for the Early Eocene.

Following Beerling and Royer (35), Paleocene and Eocene pCO₂ estimates from δ¹¹B of planktonic foraminifera were not considered for the present comparison due to several uncertainties (e.g., regarding diagenetic alteration and unknown vital effects of the analyzed foraminifera). Geochemical modeling (GEOCARBSULFvolc, refs. 80–82) predicts slightly higher pCO₂ than nearly all proxy results. However, the low resolution (10-Ma time steps) precludes tracking pCO₂ fluctuations within shorter time intervals.

Nearly all previously published proxy data represent either pre- or post-PETM estimates of pCO₂. Only Royer et al. [ref. 36, updated by Beerling et al. (76); compare also Beerling and Royer (35)] report a single stomatal index estimate revised to 670 ppm. This estimate is based on material from the Isle of Mull, United Kingdom, which was initially stated to correlate potentially to the PETM, within a data series of slightly older late Paleocene and younger early Eocene pCO₂ estimates ranging between 300 and 570 ppm.

Thus, the pCO₂ data presented here from the Wa-0 biozone, which to our knowledge are the first to track CO₂ levels directly through the CIE and PETM, are of particular interest and provide independent information on the potential carbon source generating the CIE.

Even when considering the large uncertainty in pCO₂ and a 2.3 times higher bioproductivity than today, all mean biozone estimates suggest a peak pCO₂ (upper error limit) not higher than about 2,500 ppm before and during the PETM and CIE, from Cf-3 to the start of Wa-2 (Fig. 2B and Table 1).

In the spectrum of pCO₂ values associated with different carbon sources considered as generating a global CIE in a range of –4 to –5‰, our results are compatible with dissociation of ∼2,500 to ∼4,500 Gt of highly ¹³C-depleted marine methane clathrates, as initially proposed by Dickens et al. (29), which would require only a moderate pCO₂ increase (e.g., refs. 22, 24, and 27).

The model of Zeebe et al. (27) requires an increase in pCO₂ from a pre-PETM 1,000 ppm baseline to ∼1,700 ppm during the PETM main phase, based on an initial input of 3,000 Gt C from methane clathrates with a δ¹³C lighter than –50‰. Furthermore, Zeebe et al. (27) claim that this 70% increase in pCO₂ is largely independent from the initial pre-PETM pCO₂, arguing for an increase from 500 to ∼850 ppm as likewise possible. A similar result was obtained by Cui et al. (24) when 2,500 Gt C from methane clathrates with a δ¹³C of –60‰ was assumed as the potential carbon source (increase in pCO₂ from a baseline of 835 ppm to ∼1,500 ppm). In addition, the mass balance presented by McInerney and Wing (22) estimated a pCO₂ increase to ∼1,500 ppm, potentially triggered by a release of 4,300 Gt C from methane clathrates required to generate a CIE of –4.6‰. In a recent approach, Schubert and Jahren (83) calculated initial and peak-PETM CO₂ levels using the difference between the magnitude of the marine and terrestrial CIE (ΔCIE) and proposed a pCO₂ increase from 670 to 1,380 for the methane clathrate scenario.

All other sources in discussion for the CIE (thermogenic methane, permafrost soil carbon, carbon released due to wildfires or through desiccation and oxidation of organic matter due to drying of epicontinental seas as well as mantle CO₂) would have led to significantly higher CO₂ levels than suggested by our results.

If the observed CIE would have been induced by the release of thermogenic methane or permafrost soil carbon (10,000 Gt C, δ¹³C ∼–30‰), McInerney and Wing (22) proposed a pCO₂ increase to ∼3,000 ppm. Using ΔCIE, Schubert and Jahren (83) calculated an increase from 920 to 2,480 ppm until the peak of the PETM for this scenario (Fig. 2). Such a scenario would still be within the uncertainty range of our data and cannot be completely excluded here.

Higher pCO₂ estimates have been proposed for carbon input from wildfires and/or desiccation and oxidation of organic matter from drying epicontinental seas (with a δ¹³C of ∼–22‰). The mass balance of McInerney and Wing (22) suggests CO₂ levels >4,000 ppm induced by a release of ∼15,400 Gt of carbon. This is nearly identical to the model of Cui et al. (24), who proposed a release of 13,000 Gt C from the same source, resulting in a pCO₂ increase from 835 to 4,200 ppm. The proposed CO₂ concentrations are considerably higher than estimated from the present study.

An alternative model (25) suggests a minimum pulse of carbon with a δ¹³C of –22‰ to be 6,800 Gt to trigger a CIE of –4‰, associated to an increase in pCO₂ to levels considerably above 2,000 ppm. The approach of Schubert and Jahren (83) suggests for the same carbon sources an increase from an initial value of 1,030 ppm to 3,340 ppm atmospheric CO₂ during the PETM.

Thus, the present study supports the initial idea of Dickens et al. (29) that massive releases of methane from clathrates caused the positive temperature excursion and the CIE during the PETM, at least to a considerable amount. Some criticisms to the methane clathrate scenario proposed in the last decade have turned out to be poorly constrained. One widely discussed argument is that pCO₂ values associated with the methane clathrate hypothesis are insufficient to explain the PETM temperature increase. However, alternative mechanisms, such as direct radiative forcing due to an increased pCH₄, and associated indirect effects (26, 27, 30, 31) may overrule this objection. The assumption that early Paleogene methane clathrate reservoirs were too small (84) has recently been discredited (26, 85). The idea that the amount of carbon released from methane clathrates would have been insufficient to explain observed shoaling of the carbonate compensation depth (86) has also been criticized (26). A massive release of CO₂ from soils, wildfires, or by oxidation of organic matter from drying epicontinental seas is not supported by the results of this study.

Conclusions

Triple oxygen isotope analysis of fossil mammalian bioapatite has the potential to trace fluctuations in temperature and CO₂ levels simultaneously, representing a powerful new tool for paleoclimatological research. Our Ectocion bioapatite data agree well with previous estimates of temperature change from the oxygen isotope composition of mammalian tooth enamel and from other proxies across the Paleocene–Eocene transition. Our results support existing proxy data for late Paleocene and early Eocene pCO₂. Our samples originate directly from pre-, peak- and post-PETM/CIE strata, making it possible for the first time to our knowledge to recognize any substantial change in CO₂ concentration within the respective time interval. Our data suggest that CO₂ levels during the PETM/CIE remained, within uncertainty of a few hundreds of parts per million, at pre- and post-PETM/CIE levels. Our data hence support the hypothesis that the CIE was mainly caused by a massive release of seabed methane to the atmosphere, and carbon emissions from other sources have made a subordinate contribution.

Oxygen Mass Balance Model

The mass balance model used in this study is based on the model presented in Pack et al. (50). The following in and out fluxes F_i were considered:

• •Sources

• o F_DW: Drinking water

• o F_A: Respired air O₂

• o F_FW: Moisture in food

• o F_F: Oxygen bond in carbohydrates, fats, and proteins

• •Sinks

• o F_LW: Liquid water in excrements

• o F_CO2: Oxygen in exhaled CO₂

• o F_TR: Transcutaneous water loss

• o F_BR: Vapor loss in breath

The fluxes (moles of O₂ per day) were taken from Pack et al. (50). The steady-state oxygen isotope composition of body water (BW) is given by

$${\delta }^{\mathrm{17,18}}{\mathrm{O}}_{\mathrm{BW}}={\displaystyle \sum \frac{F_i}{{\displaystyle \sum F_{i,\mathrm{in}}}}}\cdot {\delta }^{\mathrm{17,18}}{\mathrm{O}}_{\mathit{i}}$$

with

$${\displaystyle \sum F_{i,\text{in}}+{\displaystyle \sum F_{i,\text{out}}=0}}.$$

The isotope compositions of the influxes (F_DW, F_A, F_FW, and F_F) are independent of the composition of the BW. The isotope compositions of the outfluxes (F_LW, F_CO2, F_TR, and F_BR) are function of δ^17,18O_BW and the fractionation associated with the outflux with

$${\delta }^{\mathrm{17,18}}{\text{O}}_{i,\text{out}}={\alpha }_{i,\text{out}-\text{BW}}^{\mathrm{17,18}}\cdot \left(\mathrm{1,000}+{\delta }^{\mathrm{17,18}}{\text{O}}_{\text{BW}}\right)-\mathrm{1,000.}$$

The fractionation factor α¹⁷ for ¹⁷O/¹⁶O is related to the fractionation factor α¹⁸ for ¹⁸O/¹⁶O by the triple isotope fractionation exponent θ with

$$\log {\alpha }_{i,\text{out}-\text{BW}}^{17/16}={\mathit{\theta }}_{i,\text{out}-\text{BW}}\cdot \text{log}{\alpha }_{i,\text{out}-\text{BW}}^{18/16}.$$

The Δ¹⁷O of any flux F_i and BW is then calculated from the individual δ¹⁷O_i–δ¹⁸O_i pairs. The composition of the bioapatite is assumed to be in equilibrium with BW at a temperature of 37 °C (see ref. 50 for details).

The only anomalous component is air O₂ with negative Δ¹⁷O. Several studies have been undertaken to determine the Δ¹⁷Ο of tropospheric O₂ (87–91). The reported Δ¹⁷O values vary between −566 (89) and −432 ppm (91). The anomaly in Δ¹⁷O scales with the atmospheric CO₂ mixing ratio (49) and is an important input parameter for this study. We have extracted O₂ from air following a protocol similar to that described by Young et al. (91). We obtained Δ¹⁷O = −469 ppm for air O₂, which is in good agreement with the data reported by Thiemens et al. (−462 ppm) (87), and Young et al. (91) normalized to the revised Δ¹⁷O of San Carlos olivine of −51 ppm (−460 ppm). For this study we adopt a value of Δ¹⁷O = −465 ppm for air O₂. This value is tied to the preindustrial CO₂ mixing ratio of 270 ppm by volume.

Because of the restricted body mass M_b range in this study (5 and 9 kg), allometric scaling for the total water flux (FTW; data from ref. 92) and field metabolic rates (FMR; data from ref. 93) were calculated from data with 1 kg ≤ M_b ≤ 10 kg (FTW = 3.520 M_b^0.787 mol O₂⋅d^–1) and 0.1 kg ≤ M_b ≤ 10 kg (FMR = 1.434 M_b^0.638 mol O₂⋅d^–1).

Examples for the results of the mass balance are given in Figs. S1 and S2.

For the pre- and post-PETM fossils, the mass balance model gives δ¹⁸O_DW is −14.8‰. During the PETM (Wa-0), an elevated mean model δ¹⁸O_DW of −10.5‰ is calculated (Fig. S1B). This is slightly lower than the range of −12 to −8‰ suggested by Koch et al. (21) but higher than suggested by measurements of soil hematite by Bao et al. (94). The discrepancy between our model δ¹⁸O_DW data and the suggested meteoric water data by Koch et al. (21) could be interpreted in terms of a higher F_DW/F_A flux ratio (F_DW and F_A are the main oxygen sources in mammals). This would lead to a dilution of the anomalous oxygen isotope signal and erroneously low pCO₂ estimates. We thus calculated the pCO₂ for a two- and fourfold increased intake of drinking water (F_DW) (Fig. S2).

The model with 1× F_DW gives 375 ppm CO₂ (Fig. S1). For elevated drinking water fluxes (2× and 4×; Fig. S2), elevated CO₂ concentrations of 500 and 625 ppm are inferred from the model. Dilution of the isotope anomaly of respired air O₂ by isotopically normal drinking water leads to an underestimate of the atmospheric CO₂ mixing ratios. The effect of a fourfold increased water flux, however, is still within the uncertainty limits given for a scenario with average (in this case for mammals of M_b = 5 kg) F_DW. The scenario with 4× enhanced drinking water flux, however, gives a δ¹⁸O_DW = –5 ‰, which is higher than suggested for the Bighorn Basin during the Eocene/Paleocene boundary (21, 94). Higher F_DW will give even higher δ¹⁸O values for the meteoric water. From these results we conclude that the E. parvus likely had a water balance typical of the average land-living mammal.