Skip to main content
PMC home page
Reports of Practical Oncology and Radiotherapy logoLink to Reports of Practical Oncology and Radiotherapy
. 2016 Jul 16;21(5):473–479. doi: 10.1016/j.rpor.2016.06.003

Validation and clinical implementation of commercial secondary check software with heterogeneity corrections

Vijayalakshmi Kuppusamy 1,⁎, Vivekanandan Nagarajan 1, Lavanya Murugan 1
PMCID: PMC4956914  PMID: 27482153

Abstract

Aim

To validate and implement PTW diamond secondary check software (SCS) in a routine clinical use.

Background

The secondary independent monitor unit or dose calculation verifications have led to a significant increase in the workflow associated with QA treatments. Modelling, validation and commissioning are necessary steps thereby making it a useful tool for QA.

Materials and methods

PTW Diamond SCS is capable of calculating VMAT fields, based on modified Clarkson integration, accounting for multi-leaf collimators (MLC) transmission and measured collimator scatter factors. Validation for heterogeneity corrections is made using circular phantom with inserts of various density materials. 150 VMAT plans were compared using (i) plans calculated in homogeneous cylindrical phantom and (ii) VMAT plans calculated with heterogeneity corrections using electron density values for each organ.

Results

Diamond SCS calculated dose for homogeneous cylindrical phantom resulted in average deviation of (0.1 ± 2.14%) with Eclipse TPS calculated dose and (−2.0 ± 1.66%) with absolute measured dose. PTW's OCTAVIUS-4D phantom with 729 ion chamber detector array measurements agreed well with Eclipse TPS calculated dose showing an average deviation of (−1.69 ± 1.56%). Diamond SCS dose calculations were performed with heterogeneity corrections for 124 VMAT plans with isocentre at a region above −350 HU. The overall MU variations between Diamond SCS and TPS Acuros-XB algorithms were within ±5%.

Conclusion

Hence, the Diamond SCS can be used as an additional tool along with phantom measurements for patient specific quality assurance of VMAT plans with heterogeneity corrections having isocentre at a region above −350 HU.

Keywords: Diamond SCS, VMAT, Heterogeneity corrections

1. Background

The main goal of a secondary MU (monitor unit) calculation is to prevent serious errors during the MU calculation by Treatment Planning System (TPS). In radiotherapy, a significant proportion of errors are related to TPS.1 Potential errors affect not only conventional 3D treatments, but also all other treatments, even though the ultimate source of error remains the same (errors on data input for modelling, on the geometric parameters of the unit, wedge factors, tray, multi-leaf collimator (MLC) transmission, etc.). An alternative calculation method is therefore recommended in order to verify the accuracy of the TPS, regardless of a treatment technique used. In the case of complex 3D (3-dimensional) conformal or intensity modulated radiation therapy (IMRT) or volumetric modulated arc therapy (VMAT) treatments, the manual method of MU calculation is too complicated and time consuming. Therefore, subsequent quality control (QC) requires the use of tools that minimize the time necessary for MU calculations.2, 3, 4 Algorithms used for such independent monitor unit calculations are simpler and make it possible for most calculations of conventional 3D fields to be manually performed using dosimetric data from radiation units.5 In contrast, independent monitor unit verification calculation (MUVC) for complex MLC shaped 3D fields,6 sliding windows, step and shoot IMRT7, 8, 9, 10 and tomotherapy11, 12, 13 must be performed with the aid of software applications, commercial or in-house developed. Some publications describe the modelling based on measured geometric and dosimetric data before the implementation of MUVC in clinical use. Validation of independent dose calculation software with dose differences should fall in the range ±3%. Similar research describing point dose calculation methods for validating independent dose calculation software for both conventional and VMAT fields are published.14, 15 In our work, validation of independent secondary dose calculation (Diamond, Secondary check software, version-6, PTW, Germany) was used to calculate MU for clinically approved VMAT plans. PTW's diamond secondary check software (SCS) uses modified Clarkson's integration technique16 to calculate MUs for irregular MLC field segments. Percentage depth dose (PDD), total scatter factor (Sc,p), collimator scatter factor (Sc), phantom scatter factor (Sp), off axis factor (OAF) were measured and modelled in Diamond SCS.

2. Aim

The aim of the present work is also to perform patient specific QA using independent dose calculation software with and without heterogeneity corrections for VMAT plans.

3. Material and methods

VMAT plans were generated in a treatment planning system (TPS) Eclipse version-11, for TrueBeam linear accelerator supplied by VARIAN. The dose calculation algorithms used were AAA (analytical anisotropic algorithm) and Acuros-XB. Secondary, independent dose calculations were performed using Diamond SCS version-6 provided by PTW. Acuros-XB algorithm calculates dose by implementing linear Boltzmann transport equations. Dose distributions calculated by Acuros-XB have been reported to be accurate and to be in good agreement with BEAMnrc/DOSXYZnrc Monte Carlo dose calculations.17 In the literature, comparisons between AXB and AAA algorithms have been reported by Bush et al.17 and Kroon et al.18 Prakash et al. developed an in-house excel spread sheet based MUVC program for volumetric modulated arc therapy (VMAT) using Clarkson's integration technique19 with water equivalent depth (WED), calculated using the isocentre CT image section and an in-house developed MATLAB program was used for each segment.

3.1. Diamond SCS – modified Clarkson's integration calculation algorithm

Diamond SCS provides two algorithms as a function of complexity of the field. In conventional photon fields, a simple algorithm is used, based on equivalent squares and TPR/TMR tables obtained from PDD and Sc/Sp data. For IMRT/VMAT field calculations, integration was performed using the Clarkson method.16 A ‘point-eye-view’ algorithm was used to integrate scattering from the linear accelerating head to the calculation point. This algorithm includes the source and flattening filter position, and the aperture modified by the MLC, using Sc measurements.20 Points-eye-view refers to the calculation method of Sc by projecting back into the collimation elements the view of the source, primary collimators, flattening filter and ion chamber as seen by the point taking into account the upper and lower collimators and the shaping effect of the MLC. This method is described in the literature as detectors-eye-view (DEV).21 Diamond SCS integrates the head scatter through an algorithm that takes into account the unique scatter from the collimator jaws and the MLC by the use of measured scatter values. For collimator (or) jaw configuration in Diamond SCS, the Cunningham and Johns (C and J) penumbra model22 is used. The coefficients for jaws are a1, a2 and source diameter are used to describe the penumbra with an analytical curve. The source size for the linear accelerator is the size of the beam at the flattening filter. Coefficient a1 described the slope inside the field and a2, under the collimator or block. When the MLC is used, the collimator and jaw calculation under the MLC is replaced by the MLC leaf transmission. The AcuTrack algorithm is a unique MLC profile model that allows precise tracking of the measured MLC profile (MLC shape), which is particularly important for small field segments. In Diamond SCS, MLC Leaf parameters were modelled using the jaw profile and MLC profile measured in True Beam linear accelerator which are particularly important for small field segments. The values input into the software for both jaws and MLCs are adjusted to exactly align the measured profile. In Eclipse TPS, the ‘isocentre’ or a ‘reference point’ is the point of calculation for monitor units. This ensures that the correct point coordinates are contained in the exported DICOM file. The RT plan is exported using the DICOM export functionality within Eclipse, which contains all necessary plan information for Diamond SCS calculations.

3.2. Validation of conventional fields

It is essential to perform a validation of Diamond SCS for conventional fields prior to any procedure for VMAT fields. This will allow the detection of substantial errors in modelling. The set of fields used includes simple symmetrical fields defined by collimators with depth Dmax of up to 30 cm, following the guidelines recommended in ESTRO documentation.19 For dose measurements, PTW, TN 30013 Farmer Type (0.6 cc) ionization chamber, PTW UNIDOS-E electrometer were used. A slab phantom of 40 cm × 40 cm created in Eclipse, assigning CT value equivalent to water was used for calculations. Fields were generated with the isocentre (corresponding to the calculation point) placed at the centre of the sensitive volume of chamber, at a depth of Dmax, 5, 10, 15, 20, 25 and 30 cm. The next step involved exporting and calculating these fields in Diamond SCS. The plan was exported generating a DICOM file which was imported directly by Diamond SCS. Finally, all experimental measurements were compared with the calculations performed using Diamond SCS.

3.3. Validation of heterogeneity corrections

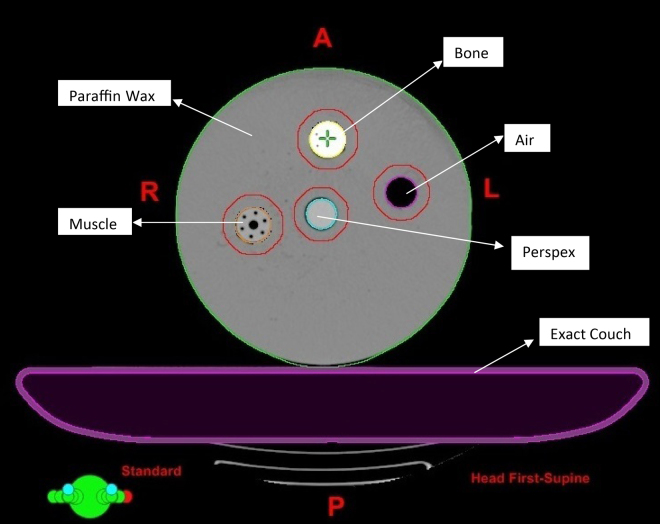
It is also essential to perform a validation for heterogeneity corrections of Diamond SCS prior to any procedure for VMAT fields, which allows the detection of substantial errors in modelling. The phantom used includes a circular cylindrical phantom sized 25 cm × 25 cm × 19 cm having insets of various densities material equivalent to the bone, perspex, muscle and air as shown in Fig. 1. The phantom was CT scanned with a slice spacing of 1.25 mm and the CT data were exported to Eclipse. Different types of beam entry, such as ANT, AP/PA, 4F, 7F IMRT, 9F IMRT and VMAT were generated for the circular phantom consisting of various density material inserts with the isocentre (corresponding to the calculation point) placed at the centre of the sensitive volume of the chamber. The next step involved exporting and calculating these fields in Diamond SCS. The plan was exported generating a DICOM file which was imported directly by Diamond SCS. Finally, all experimental measurements were compared with the calculations performed using TPS. For dose measurements at the isocentre section, PTW, TN 30013 Farmer Type (0.6 cc) ionization chamber, PTW UNIDOS-E electrometer were used. The perceptive of the dose changes due to the presence of the bone, muscle, perspex, air and its variation with increasing field numbers. A tissue equivalent circular phantom was constructed to investigate the dose calculations due to Acuros-XB algorithm. The dose variations in the middle of the bone, muscle, perspex and air from a single small field of 10 cm × 10 cm were calculated and compared between AXB (vs.) Diamond, AXB (vs.) Measured. Each heterogeneity cavity and the surrounding tissue equivalent material with 5 mm margin were subsequently contoured as different organs. The ratio of mean dose to each heterogeneity material calculated by AXB to that by Diamond was estimated for plans with increasing number of fields, ranging from 1 to 4, using the same field size (10 cm × 10 cm) from evenly distributed directions. The same ratio was also calculated for 7 field and 9 field IMRT plans and one RA plan for the circular phantom. The isocentre of each plan was located at the centre of the bone material below the phantom surface. The dose variation due to muscle, perspex and air were also studied by repeating the same procedures by replacing the bone cavity.

Fig. 1.

Fig. 1

Open in a new tab

The tissue equivalent circular phantom with bone, muscle, perspex and air cavity insert for investigation of dose variation due to the use of the TPS Acuros-XB algorithm.

3.4. Diamond SCS calculations for homogeneous cylindrical phantom

For the plans under consideration, absolute dose measurements were performed as part of patient specific quality assurance using PTW Octavius-4D phantom with 729 ion chamber array detector. In all VMAT plans, dose calculations were done at the isocentre using the Acuros-XB algorithm. The phantom was CT scanned with a slice spacing of 2 mm and the CT data were exported to Eclipse TPS. A total of 150 clinically accepted VMAT plans were recalculated and exported in DICOM format to Diamond SCS and point dose calculations at the isocentre were carried out. Results were analyzed for Diamond SCS calculations versus measured dose, Eclipse TPS versus measured dose and Diamond SCS calculations versus Eclipse TPS calculations in the homogeneous phantom.

3.5. Diamond SCS calculations for VMAT plans with heterogeneity corrections

In this study, 150 VMAT (Rapid Arc) plans were grouped into the head and neck, thorax and pelvic regions, consisting of 50, 39 and 61 plans, respectively. All these clinically accepted plans were calculated in Eclipse TPS version 11 and exported in DICOM format to Diamond SCS. It includes two features related to the algorithm, (i) body contour importation and (ii) heterogeneity corrections. The option to import the body contour for VMAT plans makes it possible to assign a source to surface distance (SSD) and depth of calculation to each control point (a VMAT field is composed of 177 or 178 control points), otherwise averaged values of SSD and depth must be assigned to all control points. In the previous studies4, 15, 23 using PTW's Diamond software (version 5.01.02.131 or higher), the heterogeneity corrections were made by setting an effective depth different from the geometric one. For IMRT and 3D plans, it can be done easily by using values obtained from TPS and setting them manually in Diamond SCS. For VMAT this is not possible as TPS only gives an averaged effective depth for the 177 or 178 control points, and if this value is set for all control points, an averaged SSD is used thereby losing all information relating to the body contour. Similar papers for 3D and IMRT plans use the same approximation.8, 24 In our present work, using Diamond SCS, version-6, the heterogeneity corrections were done by assigning relative electron density values to each organ. The structure sets are imported from TPS along with the RT plan into Diamond. Each structure set referring to particular organs is assigned a particular relative density value which is obtained from the CT calibration curve inbuilt in TPS and are shown in Table 1. The geometric and effective depth for the 177 control points is obtained from relative electron density values of each organ for the point dose measurements. In this work, using Diamond SCS, version-6, dose calculations were performed for VMAT plans with heterogeneity corrections.

Table 1.

The CT values of each structure with their mass density and relative electron density values obtained from TPS.

S. No. Structure CT value Mass density Relative electron density
1 Bone 1000 or more 1.86 1.87
2 Muscle (skeletal) 45–100 1.05 1.0505
3 Adipose tissue 0–50 0.92 0.8867
4 Air 0 0.0012 0.0074
5 Prespex 100–150 1.07 1.0701
6 Couch surface −300 HU 0.0071 0.0071
7 Couch interior −1000 HU 0.0011 0.0011
Open in a new tab

3.6. Statistical analysis

To appraise the difference between the techniques, descriptive statistics and Analysis of Variance (ANOVA) statistical test were done. ANOVA is a kind of parametric method for means comparison and is an extension of a t-test. When there are more than two groups to be compared, a pairwise t-test is not appropriate and ANOVA should be used. ANOVA requires normality and equal variance.

4. Results

4.1. Conventional field calculations

The results of conventional field calculations Diamond SCS showed good agreement (within ±0.5%) with measured and Eclipse TPS (Acuros-XB algorithm). For large field sizes, the deviations were within ±1%. The percentage depth dose (PDD), tissue phantom ratio (TPR), collimator scatter factor (Sc), phantom scatter factor (Sp) and off-axis factor (OAF) for different field sizes at various depths were calculated in Diamond SCS. The calculated doses in Diamond SCS were compared with the measured ones and the Acuros-XB algorithm for TrueBeam linear accelerator.

4.2. Heterogeneity corrections calculations

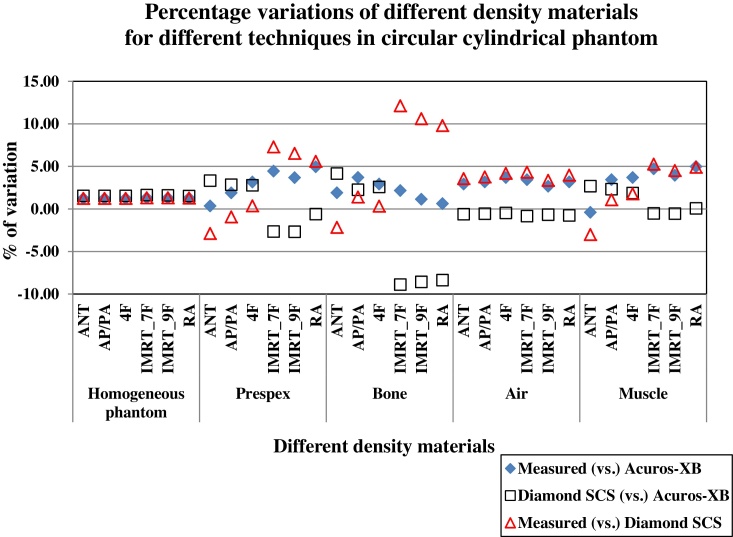
Heterogeneity corrections calculations were made using a circular cylindrical phantom made-up of paraffin wax material with various inserts having different densities equivalent to the bone, perspex, muscle and air. The validation results of heterogeneity correction calculations using Diamond SCS showed a good agreement (within ±5%) with measured and Eclipse TPS (Acuros-XB algorithm) for a homogeneous cylindrical phantom, air and muscle inserts. Since Diamond SCS uses modified Clarkson's integration algorithm, results of dose calculations for the perspex and bone inserts showed higher variation with dose measurements and the TPS Acuros-XB algorithm. The percentage variations between measured dose (vs.) TPS dose and Diamond dose (vs.) TPS dose are shown in Fig. 2.

Fig. 2.

Fig. 2

Open in a new tab

Percentage variations of different density materials using for heterogeneity corrections calculations between absolute dose measured (vs.) eclipse TPS calculated dose, Diamond SCS calculated dose (vs.) eclipse TPS calculated dose.

4.3. Diamond SCS calculations for homogeneous cylindrical phantom

For the 150 VMAT plans, the comparisons of Eclipse TPS (vs.) Absolute dose measurements, Diamond SCS (vs.) Absolute dose measurements, and Eclipse TPS dose (vs.) Diamond SCS dose are made in a homogeneous phantom as shown in Fig. 3. For VMAT plan calculations with a homogenous cylindrical phantom, Diamond SCS showed reasonable agreement with Eclipse TPS. Overall percentage variation between measured dose and Eclipse TPS calculated doses for all sites were between −4.92% and 4.26% with mean deviation of −1.47 ± 2.13%. The overall percentage variation between measured dose and Diamond SCS calculated doses for all sites were between -4.99% and 3.82% with mean deviation of −2.00% ± 1.66%. The overall percentage variation between Diamond SCS dose and Eclipse TPS calculated dose for all sites were between −4.60% and 4.67% with mean deviation of −0.10 ± 2.14%.

Fig. 3.

Fig. 3

Open in a new tab

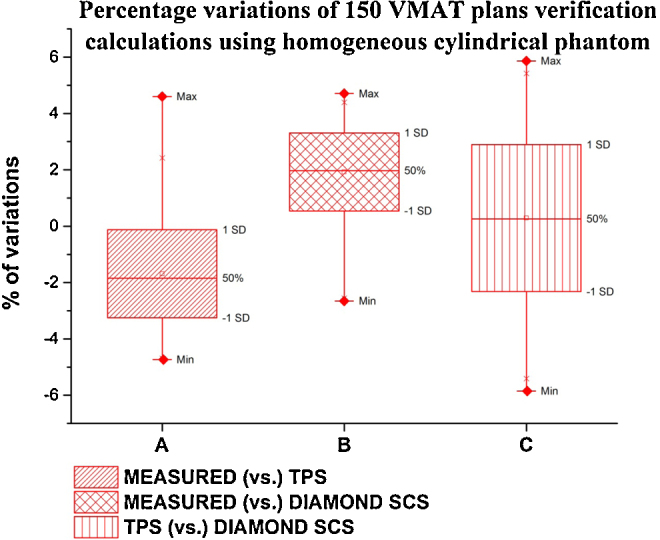
Percentage variations of VMAT plans calculated in homogeneous cylindrical phantom for all sites between absolute dose measured (vs.) eclipse TPS calculated dose, absolute dose measured (vs.) Diamond SCS calculated dose and eclipse TPS calculated dose (vs.) Diamond SCS calculated dose.

4.4. Diamond SCS calculations for VMAT plans with heterogeneity corrections

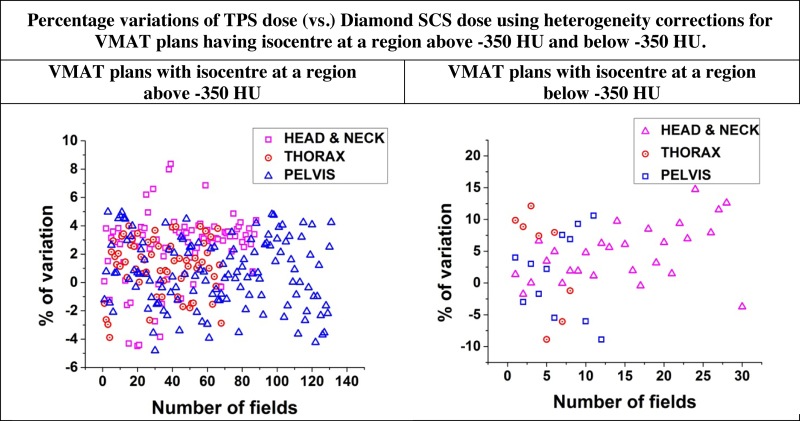
Dose calculated by Diamond SCS software version-6 for VMAT plans with heterogeneity corrections, when compared with the Acuros-XB algorithm, showed similar higher variations when the isocentre was at a region below −350 HU. 34 head and neck, 35 thorax and 55 pelvic plans had isocentre at a region above −350 HU. For this group of plans, the overall MU variations between Diamond SCS and TPS Acuros-XB algorithms were within ±5% and are shown in Fig. 4. For the remaining 26 plans, isocentre was at a region below −350 HU. For some of these plans Diamond SCS calculated much higher doses up to 18.52% compared to TPS dose.

Fig. 4.

Fig. 4

Open in a new tab

Percentage variations between eclipse TPS calculated dose (vs.) Diamond SCS calculated dose with heterogeneous corrections for patient anatomy plans with isocentre at a region above −350 HU and below −350 HU.

5. Discussions

In this study, the results of conventional field calculations Diamond SCS showed good agreement (within ±0.5%) with measured dose as well as TPS calculations. For plan verification using homogenous cylindrical phantom, Diamond SCS calculations resulted in a variation of ±5% compared to measured values and Eclipse TPS calculations. For the clinically approved VMAT treatment plan verification with heterogeneity corrections using electron density values and isocentre at a region above −350 HU, the variations between Diamond SCS, dose measurements and TPS calculations were also found to be within ±5%.

But for plans with isocentre at a region below −350 HU, it has been shown by Bush et al.20 and Kroon et al.21 that the Acuros-XB algorithm is more accurate in low density regions. However, in a low density region similar to Prakash et al.,22 verification calculations by Diamond SCS showed large variations of −8.90% and 18.52% when compared with TPS Acuros-XB algorithm calculations. Diamond SCS dose calculation using modified Clarkson's integration algorithm cannot predict doses at low density regions accurately. Hence, the Diamond SCS cannot be justified for verification for plans with isocentre at regions below −350 HU. The statistical analysis of Diamond dose (vs.) TPS dose calculations show that VMAT plans with isocentre at a region above −350 HU, the population means are not significantly different, but for isocentre at a region below −350 HU the population means are significantly different. Furthermore Diamond SCS cannot be used, if the isocentre is near the edges of the PTV, where there is a rapid dose fall off, or at the low dose regions outside the PTV. If the isocentre lies at any of the above stated regions, a suitable off-axis reference point may be chosen for MU verification according to ICRU reference point selection guidelines.

6. Conclusions

In this study, TrueBeam linear accelerator was modelled in Diamond SCS and validated. The validation results are in good agreement with Eclipse TPS data and hence it was accepted for routine clinical use. The results show that the Diamond SCS can be used as an additional tool along with phantom measurements for patient specific quality assurance of VMAT plans with heterogeneity corrections having isocentre at a region above −350 HU. The study can be further extended by comparing the dose map of Diamond SCS with TPS for further detailed analysis of plans.

Conflict of interest

None declared.

Financial disclosure

None declared.

References

  • 1.International Atomic Energy Agency . IAEA; Vienna: 2000. Lessons learned from accidental exposures in radiotherapy. Safety Reports Series No. 17. [Google Scholar]
  • 2.Stelczer G., Pesznyak C., Major T., Kontra G. Independent MU calculations with PTW DIAMOND. Poster presentation, 5th Alpe-Adria Medical Physics Meeting; Trieste, Italy, May 3–5; 2012. [Google Scholar]
  • 3.Kutcher G., Coia L., Gillin M. “Comprehensive QC for radiation oncology”, a report of AAPM radiation therapy committee task group. Med Phys. 1994;40 doi: 10.1118/1.597316. [DOI] [PubMed] [Google Scholar]
  • 4.Mata Colodro F., Serna Berná A., Puchades Puchades V. Dosimetric validation of a redundant independent calculation software for VMAT fields. Phys Med. 2013;29:341. doi: 10.1016/j.ejmp.2012.05.003. [DOI] [PubMed] [Google Scholar]
  • 5.Dutreix A, Svensson H, Bjärngard BE, Bridier A. Monitor unit calculation for high energy photon beams, Physics for Clinical Radiotherapy, ESTRO booklet no. 3.
  • 6.Sellakumar P., Arun C., Sanjay S.S., Ramesh S.B. Comparison of monitor units calculated by radiotherapy treatment planning system and an independent monitor unit verification software. Phys Med. 2011;27:21–29. doi: 10.1016/j.ejmp.2010.01.006. [DOI] [PubMed] [Google Scholar]
  • 7.Dsa Chen X., Nath R. Independent monitor unit calculation for intensity modulated radiotherapy using the MIMiC multileaf collimator. Med Phys. 2002:29. doi: 10.1118/1.1500397. [DOI] [PubMed] [Google Scholar]
  • 8.Haslam J., Bonta D., Lujan A., Rash C., Jackson W., Roeske J. Comparison of dose calculated by an intensity modulated radiotherapy treatment planning system and an independent monitor unit verification program. J Appl Clin Med Phys. 2003:4. doi: 10.1120/jacmp.v4i3.2519. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 9.Kung Chen, Kuchnir F.K. A monitor unit verification calculation in intensity modulated radiotherapy as a dosimetry quality assurance. Med Phys. 2000:27. doi: 10.1118/1.1286553. [DOI] [PubMed] [Google Scholar]
  • 10.Sivakumar S., Krishnamurthy K., Davis C.A. Clinical implementation of dynamic intensity-modulated radiotherapy: dosimetric aspects and initial experience. J Med Phys. 2008:33. doi: 10.4103/0971-6203.41195. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 11.Gibbons J.P., Smith K., Cheek D., Rosen I. Independent calculation of dose from a helical tomotherapy unit. J Appl Clin Med Phys. 2009:10. doi: 10.1120/jacmp.v10i1.2772. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 12.Papanikolaou N., He W., Vazquez L.A. MU-Tomo: independent dose validation software for helical tomotherapy. J Cancer Sci Ther. 2010;2:145–152. [Google Scholar]
  • 13.Tsai J., Engler M.J., Liu J. Quasi-independent monitor unit calculation for intensity modulated sequential tomotherapy. J Appl Clin Med Phys. 2002:3. doi: 10.1120/jacmp.v3i2.2577. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 14.Amri I.A., Ravichandran R., Sivakumar S.S. Radiotherapy pre-treatment dose validation: a second verification of monitor units with a commercial software. J Med Phys. 2012;37(4):235–239. doi: 10.4103/0971-6203.103610. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 15.Colodro J.F.M., Berna A.S., Puchades V., Amores D.R., Banos M.A. Results of 1 year of clinical experience with independent dose calculation software for VMAT fields. J Med Phys. 2014;39(4):219–224. doi: 10.4103/0971-6203.144485. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 16.Clarkson J.R. A note on depth doses in fields of irregular shape. Br J Radiol. 1941;14:265. [Google Scholar]
  • 17.Bush K., Gagne I.M., Zavgorodni S., Ansbacher W., Beckham W. Dosimetric validation of Acuros XB with Monte Carlo methods for photon dose calculations. Med Phys. 2011;38(4):2208–2221. doi: 10.1118/1.3567146. [DOI] [PubMed] [Google Scholar]
  • 18.Kroon P.S., Hol S., Essers M. Dosimetric accuracy and clinical quality of Acuros XB and AAA dose calculation algorithm for stereotactic and conventional lung volumetric modulated arc therapy plans. Radiat Oncol. 2013;8(1):149. doi: 10.1186/1748-717X-8-149. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 19.Jeevanandam P., Rajasekaran D., Sukumar P., Nagarajan V. In-house spread sheet based monitor unit verification program for volumetric modulated arc therapy. Phys Med. 2014;30:509–512. doi: 10.1016/j.ejmp.2014.01.001. [DOI] [PubMed] [Google Scholar]
  • 20.Ahnesjo A., Aspradakis M.M. Dose calculations for external photon beams in radiotherapy. Phys Med Biol. 1999;44:R99–R155. doi: 10.1088/0031-9155/44/11/201. [DOI] [PubMed] [Google Scholar]
  • 21.Kim S., Palta J.R., Zhu T.C. A generalized solution for the calculation of in-air output factors in irregular fields. Med Phys. 1998;25(9):169. doi: 10.1118/1.598350. [DOI] [PubMed] [Google Scholar]
  • 22.Johns H.E., Cunningham J.R. fourth ed. C.C. Thomas; 1983. The physics of radiology. [Google Scholar]
  • 23.Dose Calculation Management Software . DIAMOND edition. Setup 2000. K&S Associated, Inc.; Nashville, TN, USA: 2009. User's manual. [Google Scholar]
  • 24.Chan J., Russell D., Peters V.G., Farrell T.J. Comparison of monitor unit calculations performed with a 3D computerized planning system and independent hand calculations: results of three years clinical experience. J Appl Clin Med Phys. 2002;3:293–301. doi: 10.1120/jacmp.v3i4.2553. [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Reports of Practical Oncology and Radiotherapy are provided here courtesy of Via Medica sp. z o.o. sp. k.

RESOURCES