The mechanistic bases of the power–time relationship: muscle metabolic responses and relationships to muscle fibre type

Abstract

Key points

• The power‐asymptote (critical power; CP) of the hyperbolic power–time relationship for high‐intensity exercise defines a threshold between steady‐state and non‐steady‐state exercise intensities and the curvature constant (W′) indicates a fixed capacity for work >CP that is related to a loss of muscular efficiency.

• The present study reports novel evidence on the muscle metabolic underpinnings of CP and W′ during whole‐body exercise and their relationships to muscle fibre type.

• We show that the W′ is not correlated with muscle fibre type distribution and that it represents an elevated energy contribution from both oxidative and glycolytic/glycogenolytic metabolism.

• We show that there is a positive correlation between CP and highly oxidative type I muscle fibres and that muscle metabolic steady‐state is attainable <CP but not >CP.

• Our findings indicate a mechanistic link between the bioenergetic characteristics of muscle fibre types and the power–time relationship for high‐intensity exercise.

Abstract

We hypothesized that: (1) the critical power (CP) will represent a boundary separating steady‐state from non‐steady‐state muscle metabolic responses during whole‐body exercise and (2) that the CP and the curvature constant (W′) of the power–time relationship for high‐intensity exercise will be correlated with type I and type IIx muscle fibre distributions, respectively. Four men and four women performed a 3 min all‐out cycling test for the estimation of CP and constant work rate (CWR) tests slightly >CP until exhaustion (T _lim), slightly <CP for 24 min and until the >CP T _lim isotime to test the first hypothesis. Eleven men performed 3 min all‐out tests and donated muscle biopsies to test the second hypothesis. Below CP, muscle [PCr] [42.6 ± 7.1 vs. 49.4 ± 6.9 mmol (kg d.w.)⁻¹], [La⁻] [34.8 ± 12.6 vs. 35.5 ± 13.2 mmol (kg d.w.)⁻¹] and pH (7.11 ± 0.08 vs. 7.10 ± 0.11) remained stable between ∼12 and 24 min (P > 0.05 for all), whereas these variables changed with time >CP such that they were greater [[La⁻] 95.6 ± 14.1 mmol (kg d.w.)⁻¹] and lower [[PCr] 24.2 ± 3.9 mmol (kg d.w.)⁻¹; pH 6.84 ± 0.06] (P < 0.05) at T _lim (740 ± 186 s) than during the <CP trial. The CP (234 ± 53 W) was correlated with muscle type I (r = 0.67, P = 0.025) and inversely correlated with muscle type IIx fibre proportion (r = −0.76, P = 0.01). There was no relationship between W′ (19.4 ± 6.3 kJ) and muscle fibre type. These data indicate a mechanistic link between the bioenergetic characteristics of different muscle fibre types and the power–duration relationship. The CP reflects the bioenergetic characteristics of highly oxidative type I muscle fibres, such that a muscle metabolic steady‐state is attainable below and not above CP.

Key points

• The power‐asymptote (critical power; CP) of the hyperbolic power–time relationship for high‐intensity exercise defines a threshold between steady‐state and non‐steady‐state exercise intensities and the curvature constant (W′) indicates a fixed capacity for work >CP that is related to a loss of muscular efficiency.

• The present study reports novel evidence on the muscle metabolic underpinnings of CP and W′ during whole‐body exercise and their relationships to muscle fibre type.

• We show that the W′ is not correlated with muscle fibre type distribution and that it represents an elevated energy contribution from both oxidative and glycolytic/glycogenolytic metabolism.

• We show that there is a positive correlation between CP and highly oxidative type I muscle fibres and that muscle metabolic steady‐state is attainable <CP but not >CP.

• Our findings indicate a mechanistic link between the bioenergetic characteristics of muscle fibre types and the power–time relationship for high‐intensity exercise.

Abbreviations

CP

critical power

Cr

creatine

CWR

constant work rate

GET

gas exchange threshold

Gly

glycogen

La⁻

lactate

PCr

phosphocreatine

³¹P‐MRS

³¹phosphorous magnetic resonance spectroscopy

T_lim

time to exhaustion

${\dot{V}}_{\mathrm{E}}$

minute ventilation

$\dot{\mathrm{V}}{\mathrm{O}}_2$

rate of pulmonary oxygen uptake

W′

curvature constant of the power–time relationship for high‐intensity exercise

Introduction

Attainment of the maximal rate of O₂ uptake (${\dot{V}}_{{\mathrm{O}}_2\max }$) during exhaustive, constant work rate (CWR) exercise above the critical power (CP) defines the range of the severe exercise intensity domain (Poole et al. 1988; Hill et al. 2002). The consistent attainment of ${\dot{V}}_{{\mathrm{O}}_2\max }$ in the severe domain enables very accurate prediction of exercise tolerance based on the hyperbolic relationship between time‐to‐exhaustion (T _lim) and work‐rate (P) (Jones et al. 2010). The curvature constant of this hyperbola, termed the W′, represents a fixed amount of work that can be performed >CP before exhaustion ensues (Chidnok et al. 2013). The observation that the magnitude of the W′ remains fixed irrespective of the rate of its expenditure has given rise to an all‐out 3 min sprint protocol for a time‐efficient method to estimate the CP and W′ (Burnley et al. 2006). After ∼2 min of all‐out cycling against a fixed resistance, the W′ is essentially reduced to zero such that the greatest power output that can be maintained by maximum effort over the final 30 s of the 3 min test closely approximates the CP (Vanhatalo et al. 2007).

The CP has been defined as a critical threshold of intramuscular metabolic control on the basis of ³¹phosphorous magnetic resonance spectroscopy (³¹P‐MRS) assessment of muscle phosphorous‐containing metabolites during single‐leg knee‐extension exercise (Jones et al. 2008). Just below the CP, muscle phosphocreatine (PCr), inorganic phosphate (P_i) and pH reached stable values within ∼2 min of the onset of exercise, whereas, above the CP, these variables exhibited non‐steady‐state responses (Jones et al. 2008; Vanhatalo et al. 2010). Although the ³¹P‐MRS one‐legged knee‐extension model has several advantages, it does not enable the measurement of muscle lactate ([La⁻]) or glycogen ([Gly]) and the supine body position and small active muscle mass may limit its translational value. To date, muscle metabolic responses during whole‐body exercise performed just below and just above CP have not been determined. This is an important consideration because muscle perfusion is higher during small muscle mass exercise compared to whole‐body exercise (Calbet & Lundby, 2012). Given that the ${\dot{V}}_{{\mathrm{O}}_2\max }$ and CP are both sensitive to interventions that alter O₂ delivery (Vanhatalo et al. 2010; Simpson et al. 2015), the characterization of muscle metabolic responses to exercise below and above the CP during whole‐body exercise is prerequisite for the validation of the critical power concept.

The mechanistic bases of the W′ are complex and remain equivocal. The W′ was originally described as an anaerobic work capacity (Monod & Scherrer, 1965) but was subsequently shown to be sensitive to manipulation of O₂ delivery (Vanhatalo et al. 2010). We have shown that the W′ estimated in a 3 min all‐out cycling test is proportional to the amplitude of the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component (Vanhatalo et al. 2011), which represents the delayed onset increase in ${\dot{V}}_{{\mathrm{O}}_2}$ during CWR exercise above the lactate threshold. The ${\dot{V}}_{{\mathrm{O}}_2}$ slow component signifies an increased O₂ cost of force production and therefore a loss of muscle efficiency (Krustrup et al. 2003; 2004). Although it appears intuitive that a link exists between the utilization of the W′, the development of the ${\dot{V}}_{{\mathrm{O}}_2}$slow component and the accumulation of muscle fatigue, direct evidence of muscle metabolic responses during whole‐body exercise where the W′ is reduced to zero and the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component amplitude drives ${\dot{V}}_{{\mathrm{O}}_2}$ to its maximum is currently lacking. Specifically, it is not known whether the significantly greater ${\dot{V}}_{{\mathrm{O}}_2}$ slow component during the 3 min all‐out test compared to a work‐matched CWR test (Vanhatalo et al. 2011) is associated with greater perturbation of muscle metabolism [i.e. depletion of PCr and/or accumulation of creatine (Cr), H⁺ and La⁻].

The ${\dot{V}}_{{\mathrm{O}}_2}$ slow component is more pronounced in individuals who possess a large proportion of type II muscle fibres, whereas a fast ‘primary’ rise in ${\dot{V}}_{{\mathrm{O}}_2}$ at exercise onset (rapid ‘phase II’ kinetics) is associated with a high proportion of type I muscle fibres and is indicative of high level of endurance fitness (Barstow et al. 1996; Pringle et al. 2003). Given that the W′ estimated from a 3 min all‐out test is proportional to the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component amplitude (Vanhatalo et al. 2011) and the CP derived from CWR prediction trial data is inversely correlated with the phase II ${\dot{V}}_{{\mathrm{O}}_2}$ time constant (τ) (Murgatroyd et al. 2011), it is possible that the W′ might be related to a large proportion of type II (a+x) muscle fibres, whereas a high CP might be associated with a high proportion of type I fibres. However, the relationship between muscle fibre type and the parameters of the power–time relationship has never been determined.

The present study therefore aimed to characterize the muscle metabolic responses to exercise in close proximity below and above CP and to clarify the mechanistic bases of the power–time parameters (CP and W′) in relation to muscle metabolism and fibre type distribution. We tested the hypotheses that: (1) the CP established in a 3 min all‐out cycling test will represent a threshold below which muscle [PCr], [La⁻] and pH will attain a steady‐state, whereas no steady‐state in these variables will be attained during cycling >CP; (2) the CP will be positively correlated with muscle type I fibre distribution (%), and the W′ will be positively correlated with muscle type II (a+x) fibre distribution; and (3) the changes in muscle [PCr], [La⁻] and pH relative to change in work rate (‘gain’) will be greater during all‐out compared to work‐matched CWR exercise.

Methods

Ethical approval

The protocols were approved by the host institution's Research Ethics Committee and conducted in accordance with the code of the ethical principles of the World Medical Association (Declaration of Helsinki). Subjects provided their written informed consent to participate after the experimental procedures, associated risks and potential benefits of participation had been explained.

Subjects

The data collection was separated into two studies with distinct subject recruitment. Four men (mean ± SD, age 24 ± 2 years; height 1.73 ± 0.08 m; body mass 70.1 ± 11.3 kg) and four women (28 ± 5 years; 1.75 ± 0.10 m; 68.4 ± 15.4 kg) volunteered to participate in Study 1 to test hypotheses 1 and 3. To test hypothesis 2, the data for Study 2 were collated from three distinct studies in the same laboratory (A. Vanhatalo, M.I. Black, C. Thomspon, L.J. Wylie, A.M. Jones, unpublished results) where the participants had performed a 3 min test and donated a muscle sample for fibre typing within a 6 month period. In all, data from 11 men (27 ± 8 years, 1.78 ± 0.07 m, 85.6 ± 15.5 kg) were available for Study 2. Only male subjects were included in Study 2 because differences in body composition between sexes would skew any potential relationship between fibre type (expressed as a percentage of fibres counted) and power–time parameters. On all occasions, participants were instructed to arrive in the laboratory adequately hydrated and not to consume alcohol for 24 h, as well as food or caffeine for 3 h before testing. Participants refrained from strenuous physical activity for 24 h before each laboratory visit.

Study 1: Muscle metabolic bases of CP and W′ during cycling

Exercise protocols

All exercise testing was conducted using an electrically‐braked cycle ergometer (Lode Excalibur Sport, Groningen, The Netherlands) and visits were separated by a minimum of 24 h of rest. The ergometer seat and handlebars were adjusted for comfort during the first visit and settings were recorded and replicated for subsequent tests. The preliminary tests included a ramp incremental test for the assessment of ${\dot{V}}_{{\mathrm{O}}_2\max }$ and gas exchange threshold (GET) (Whipp et al. 1981), which were used to normalize the fixed resistance for the all‐out tests, and a 3 min all‐out familiarization trial. On a subsequent visit, subjects performed a 3 min all‐out test for estimation of the CP and the W′, and these values were then used to calculate a ‘constant work rate predicted to result in exhaustion in 3 min’ (CWR3) according to the power–time relationship:

$$\mathrm{CWR}3=\left({\mathit{W}}^{\prime }/180\mathrm{s}\right)+\mathrm{CP}$$ (1)

During subsequent visits, subjects performed the following protocols: (1) 5 s and 30 s of all‐out sprints separated by 30 min of passive rest; (2) a 90 s all‐out sprint; (3) a 3 min all‐out sprint; (4) a CWR3 trial until exhaustion; (5) 5 s and 90 s at CWR3 separated by 30 min of passive rest; (6) a CWR test at CP + 5% of ramp test peak power (CP+5%), which was continued until exhaustion or up to 24 min; (7) a CWR test at CP – 5% of ramp test peak power (CP‐5%) until exhaustion or up to 24 min; and (8) a CWR test at CP – 5% of ramp test peak power (CP‐5%), which was continued until the T _lim measured during the CP+5% trial (‘CP+5% isotime’). The range of ± 5% of ramp test peak power (tests 6, 7 and 8) was chosen based on the original protocol by Poole et al. (1988). These seven visits were administered in a randomized order, except for the requirement that the CP+5% T _lim isotime trial had to be performed after the CP+5% trial.

The ramp incremental protocol for the assessment of ${\dot{V}}_{{\mathrm{O}}_2\max }$ and the GET consisted of 3 min of unloaded baseline pedalling, followed by a ramp increase in power output of 30 W min⁻¹ until the subject reached exhaustion. Subjects were instructed to maintain their preferred cadence (80 rpm, n = 3; 75 rpm, n = 1, 70 rpm, n = 4) for as long as possible. The test was terminated when the pedal rate fell more than 10 rpm below the chosen cadence, despite strong verbal encouragement.

The all‐out sprint tests commenced from a 3 min unloaded baseline, followed by an all‐out effort of 5, 30, 90 or 180 s. Prior to the start of the test, subjects were informed that the maximum duration of the test was 180 s and they were instructed to cycle all‐out until the test administrator asked them to stop. Subjects were therefore unaware of the test duration to be performed on any given visit. Subjects were asked to accelerate to 110–120 rpm over the last 5 s of the baseline period. The resistance on the pedals during the all‐out effort was set using the linear mode of the Lode Excalibur Sport ergometer such that the subject would attain the power output halfway between the GET and ${\dot{V}}_{{\mathrm{O}}_2\max }$ work rates (50% ∆) on reaching their preferred cadence (linear factor = power/preferred cadence²). Strong verbal encouragement was provided throughout the tests but subjects were not informed about their elapsed time. Subjects were instructed to attain their peak power output as quickly as possible from the start of the test and to maintain the cadence as high as possible at all times throughout the test.

The CWR tests began with 3 min of unloaded baseline pedalling after which the work rate abruptly increased to CWR3, CP+5% or CP‐5%. Subjects were instructed to maintain their preferred cadence (i.e. the same cadence as in the ramp incremental test) for as long as possible during the test. A test was terminated when cadence fell more than 10 rpm below preferred cadence. Strong verbal encouragement was provided throughout the test and time to exhaustion was recorded to the nearest second. Subjects were not informed of the work rates, the time to exhaustion or their performance in any of the tests until the entire study had been completed.

Blood sampling

A catheter (Insyte‐W TM; Becton‐Dickinson, Franklin Lakes, NJ, US) was inserted in an antecubital vein. Blood samples were drawn during the final 60 s of the baseline, at the end of exercise and 5 min post‐exercise for all tests. During the CP+5% and CP‐5% tests, blood samples were also drawn every 4 min during exercise. From these samples, 200 μl was immediately haemolysed in 200 μl of buffer solution (Triton X‐100; Amresco, Salon, OH, USA) and analysed for blood [La⁻] and [glucose] (YSI 2300; Yellow Springs Instruments, Yellow Springs, OH, USA). The remaining sample was centrifuged at 4000 rpm for 8 min and the extracted plasma was analysed for [K⁺] and [Na⁺] (9180 Electrolyte Analyser; F. Hoffman‐La Roche, Basel, Switzerland).

Muscle biopsies

Incisions (∼0.6 cm) were made through the skin and fascia over the medial part of musculus vastus lateralis muscle under local anaesthesia (2 ml; 20 mg l⁻¹ lidocaine without adrenalin) and covered with sterile gauze. Muscle samples (∼150 mg wet weight) were taken at rest and as soon as possible (within ∼10 s) after each exercise bout using the needle biopsy technique with suction (Bergström, 1975). The tissue sample was immediately frozen in liquid nitrogen and stored at −80°C for subsequent analyses of muscle metabolite concentrations.

The frozen muscle samples from each biopsy were weighed before and after freeze‐drying to determine water content (XP6U; Mettler Toledo, Greifensee, Switzerland). After freeze‐drying, the muscle samples were dissected free from blood, fat and connective tissue. Prior to muscle metabolite analysis, 200 μl of 3 m perchloric acid was added to approximately 2.5 mg d.w. muscle. The solution was then centrifuged and incubated on ice for 30 min. It was subsequently neutralized to pH 7.0 with 255 μl of cooled KHCO₃ and centrifuged (10,000 g). The supernatant was analysed for [PCr], creatine ([Cr]), [ATP] and [La⁻] by fluorometric assays (Lowry & Passonneau, 1976). An aliquot containing 1–2 mg d.w. muscle was extracted in 1 m HCl and hydrolysed at 100°C for 3 h and glycogen content was determined using the hexokinase method (Lowry & Passonneau, 1976). Muscle pH was measured using a glass electrode following the homogenization of approximately 1 mg d.w. of muscle in in 100 μl of non‐buffering solution containing 145 mm KCl, 10 mm NaCl and 5 mm iodoacetic acid.

Pulmonary gas exchange

Pulmonary gas exchange was measured breath‐by‐breath during all exercise tests with subjects wearing a nose clip and breathing through a low dead space (90 ml), low resistance (0.75 mm Hg l⁻¹ s⁻¹ at 15 l s⁻¹) mouthpiece and impeller turbine assembly (Jaeger Triple V; Jaeger GmbH, Hoechberg, Germany). The inspired and expired gas volume and gas concentration signals were sampled continuously at 100 Hz (Jaeger Oxycon Pro, Jaeger GmbH) via a capillary line connected to the mouthpiece. These analysers were calibrated before each test with gases of known concentration, and the turbine volume transducer was calibrated using a 3 litre syringe (Hans Rudolph, Kansas City, MO, USA). The volume and concentration signals were time aligned by accounting for the delay in capillary gas transit and analyser rise time relative to the volume signal. Rates of oxygen uptake, carbon dioxide output (${\dot{V}}_{\mathrm{C}{\mathrm{O}}_2}$) and minute ventilation (${\dot{V}}_{\mathrm{E}}$) were calculated using standard formulae and displayed breath‐by‐breath.

Data analysis

The ${\dot{V}}_{{\mathrm{O}}_2\max }$ was determined as the highest 10 s mean value recorded during the ramp incremental test. The GET was established from the gas exchange data averaged in 10 s time bins using the following criteria: (1) the first disproportionate increase in ${\dot{V}}_{\mathrm{C}{\mathrm{O}}_2}$from visual inspection of individual plots of ${\dot{V}}_{\mathrm{C}{\mathrm{O}}_2}$ vs. ${\dot{V}}_{{\mathrm{O}}_2}$; (2) an increase in ${\dot{V}}_{\mathrm{E}}$/${\dot{V}}_{\mathrm{C}{\mathrm{O}}_2}$ with no increase in ${\dot{V}}_{\mathrm{E}}$/${\dot{V}}_{\mathrm{C}{\mathrm{O}}_2}$; and (3) the first increase in end‐tidal O₂ tension with no fall in end‐tidal CO₂ tension. The data from the initial ramp test were used to calculate the 50%Δ work rate (i.e. the WR at GET plus 50% of the interval between the work rate at GET and ${\dot{V}}_{{\mathrm{O}}_2\max }$) taking into account the lag in ${\dot{V}}_{{\mathrm{O}}_2}$ during incremental exercise by deducting two‐thirds of the ramp rate (i.e. 20 W) from the work rate that was time aligned with the point at which GET was identified.

The CP was estimated as the mean power output recorded over the final 30 s of the 3 min all‐out test and the W′ was calculated as the work done >CP. These parameters were used to predict the power output (P) which could be sustained for 3 min in a CWR test using the linear transformation of the power‐time relationship as shown in eqn (1). The peak ${\dot{V}}_{{\mathrm{O}}_2}$ values during the 3 min all‐out test and the CWR3 test were determined as the highest 10 s rolling mean. The ‘time‐to‐attain‐${\dot{V}}_{{\mathrm{O}}_2}$ peak’ in the all‐out test was determined as the time required for the 5 s rolling‐average ${\dot{V}}_{{\mathrm{O}}_2}$ to rise to a value that was within 1 SD of the ${\dot{V}}_{{\mathrm{O}}_2\max }$ (using the criterion established in the ramp incremental test). The ${\dot{V}}_{{\mathrm{O}}_2}$ slow component gain in the 3 min all‐out test was calculated as the difference between the end‐exercise gain (i.e. (end‐exercise ${\dot{V}}_{{\mathrm{O}}_2}$ – baseline ${\dot{V}}_{{\mathrm{O}}_2}$)/Δ power output) and the gain at the ‘time‐to‐attain${\dot{V}}_{{\mathrm{O}}_2\max }$’ (Vanhatalo et al. 2011). The absolute ${\dot{V}}_{{\mathrm{O}}_2}$ slow component amplitude in the all‐out test (l min⁻¹) was estimated as the difference between the end‐exercise ${\dot{V}}_{{\mathrm{O}}_2}$ and the ${\dot{V}}_{{\mathrm{O}}_2}$ at the CP, which was estimated using linear regression applied to the ${\dot{V}}_{{\mathrm{O}}_2}$–WR relationship established in the ramp incremental test.

The breath‐by‐breath ${\dot{V}}_{{\mathrm{O}}_2}$ data from the CWR test were interpolated into 1 s bins and the first 20 s of data after exercise onset were deleted to eliminate the cardiodynamic ‘phase I’ data from the model fit. A non‐linear least‐squarealgorithm was used to fit the data, as described by:

$${\dot{V}}_{{\mathrm{O}}_2}\left(t\right)={\dot{V}}_{{\mathrm{O}}_2\mathrm{baseline}}+A_{\mathrm{p}}(1-{{\mathrm{e}}^{-\left(\mathrm{t}-\mathrm{TDp}\right)/}}^{\tau \mathrm{p}})$$ (2)

where ${\dot{V}}_{{\mathrm{O}}_2}$ (t) represents the absolute ${\dot{V}}_{{\mathrm{O}}_2}$ at a given time t; ${\dot{V}}_{{\mathrm{O}}_2}$ _baseline represents the mean ${\dot{V}}_{{\mathrm{O}}_2}$in the final 60 s of the baseline period; and A _p, TD _p and Τ_p represent the amplitude,time delay and time constant, respectively, describing the phase II increase in ${\dot{V}}_{{\mathrm{O}}_2}$ above baseline. An iterative process was used to minimize the sum of the squared errors between the fitted function and the observed values and the fitting window was constrained to the time point at which a departure from fundamental mono‐exponentiality occurred (as judged from visual inspection of a plot of the residuals of the fit). The end‐exercise ${\dot{V}}_{{\mathrm{O}}_2}$ was defined as the mean ${\dot{V}}_{{\mathrm{O}}_2}$ measured over the final 10 s of exercise and the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component was calculated as the difference between the asymptotic amplitude of the ${\dot{V}}_{{\mathrm{O}}_2}$ fundamental component and the end‐exercise ${\dot{V}}_{{\mathrm{O}}_2}$. In addition, the gain of the fundamental ${\dot{V}}_{{\mathrm{O}}_2}$ response (G _p) was computed by dividing A _p by the ∆ work rate; the gain of the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component and the entire response (i.e. end‐exercise gain) was calculated in a similar manner. Similarly, the gain values for the muscle [PCr], [La⁻] and pH during the CWR (5 s, 90 s and T _lim) and all‐out tests (5, 30, 90 and 180 s) were calculated as the change from resting baseline relative to ∆ work rate.

Study 2: Muscle fibre type and the power‐time parameters

The CP and W′ were estimated using a 3 min all‐out test protocol as described above for Study 1. On a separate occasion, a resting muscle biopsy was obtained from musculus vastus lateralis as also described for Study 1. Tissue samples (∼ 80 mg wet weight) were cut and oriented in the transverse plane before embedding in Tissue‐Tek OTC compound (Sakura Finetek Europe BV, Zoeterwoude, The Netherlands) and freezing in isopentane pre‐cooled to −160°C. For histochemical determination of muscle fibre type, serial 10 μm sections were cut on a cryostat‐microtome (CryoStar NX50; Thermo Scientific, Waltham, MA, USA) maintained at −16°C, and mounted on three separate slides. Sections were stained using a refined version of Brooke & Kaiser's (1970) ATPase method. Pre‐incubation at pH of 4.37, 4.6 or 10.3 enables differential lability of myosin ATPase to identify myosin heavy chain isoform types I, IIa and IIx. The three slides were placed in 50 ml Coplin staining jars and incubated in solutions adjusted to these pH conditions. Following pre‐incubation, slides were rinsed in an activating calcium solution, and incubated at 37°C with an ATP solution. Myosin ATP‐ase catalyses the conversion to ADP, releasing P_i. The ATP was replaced with a 1% calcium chloride solution, forming calcium phosphate. Next, 2% cobalt chloride was added to displace the calcium, followed by 1% ammonium sulphide to give a final product of cobalt sulphide, a dark pigment that stains the activated cells for quantification. Slides were mounted under a coverslip with an aqueous polyvinylpyrrolidon mounting medium. Type I (slow‐twitch oxidative) fibres are stained with a pre‐incubation pH of 4.37 and 4.6 (Fig. 1 A and B), whereas type II (fast twitch) fibres are stained under alkaline conditions (pH 10.3) (Fig. 1 C). Type IIa, (fast‐oxidative) and IIx (fast‐glycolytic) fibres are identified at pH 4.6 because the latter isoform is also active under those conditions (Fig. 1 B). Fibres were identified and counted under a CKX41 microscope with cellSens Dimension software (Olympus Corp., Tokyo, Japan).

Figure 1. Histological determination of muscle fibre type .

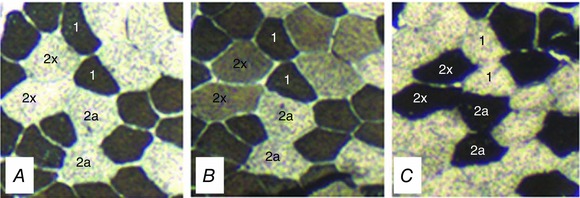
Serial sections stained for myofibrillar ATPase, following pre‐incubation at pH 4.37 (A) showing a checkerboard pattern of myosin heavy chain isoform type I fibres, pH 4.6 (B) showing type I (dark) and type IIx (intermediate) fibres, and pH 10.3 (C) showing type IIa and IIx fibres.

Statistical analysis

Differences in end‐exercise physiological responses between the CP‐5% and CP+5% tests were assessed using paired samples t tests. One‐way ANOVAs with repeated measures were used to assess differences in the ${\dot{V}}_{{\mathrm{O}}_2}$ peak in the all‐out and the CWR tests and the ramp test ${\dot{V}}_{{\mathrm{O}}_2\max }$. Two‐way repeated measures ANOVAs were used to assess differences in physiological responses across condition (all‐out vs. CWR) and time (common time points of 5 s, 90 s and end‐exercise). Relationships between W′ and CP and the muscle metabolic responses and muscle fibre type were assessed using Pearson product moment correlation coefficients. Statistical significance was accepted at P < 0.05 and data are presented as the mean ± SD unless stated otherwise.

Results

Study 1: Muscle metabolic bases of CP and W′ during cycling

The ${\dot{V}}_{{\mathrm{O}}_2\max }$ in the ramp incremental test was 3.54 ± 0.77 l min⁻¹ and the peak power output was 345 ± 61 W. The GET occurred at 1.59 ± 0.39 l min⁻¹ (108 ± 29 W). The CP estimate in the 3 min all‐out test was 225 ± 47 W (attained at 76 ± 6 rpm) and the W′ was 14.9 ± 3.8 kJ. The peak power attained in the all‐out test was 743 ± 194 W, which was associated with a cadence of 133 ± 14 rpm. T _lim in the 3CWR test was 189 ± 18 s and the mean cadence was 72 ± 6 rpm.

The work rates for the CP‐5% and CP+5% tests were 206 ± 42 W and 249 ± 48 W, respectively. None of the subjects reached volitional exhaustion during the CP‐5% test (where, according to the methodology, the test was terminated at 1440 ± 0 s) and the end‐exercise ${\dot{V}}_{{\mathrm{O}}_2}$ (2.84 ± 0.54 l min⁻¹) was lower than the ramp test ${\dot{V}}_{{\mathrm{O}}_2\max }$ (P < 0.05) (Fig. 2 A). T _lim in the CP+5% tests ranged from 465 s to 1016 s (740 ± 186 s) and the end‐exercise ${\dot{V}}_{{\mathrm{O}}_2}$ (3.58 ± 0.65 l min⁻¹) was not different from the ramp test ${\dot{V}}_{{\mathrm{O}}_2\max }$ (P > 0.05) (Fig. 2 A). End‐exercise blood [La⁻] (Fig. 2 B) was higher (P < 0.05), and plasma [K⁺] tended to be higher (P = 0.06; Fig. 2 C) during the CP+5% compared to the CP‐5% test. The muscle [PCr], [Cr], [La⁻] and pH at T _lim during the CP+5% test were different from the CP+5% T _lim isotime (∼12 min) and end‐exercise (24 min) measurements during the CP‐5% test (P < 0.05 for all) (Fig. 3). At the CP‐5% work rate, there were no differences between CP+5% T _lim isotime (∼12 min) and end‐exercise (24 min) in muscle [PCr], [Cr], [Gly], [La⁻] or pH (P > 0.05 for all) (Fig. 3). Muscle [Gly] was not significantly different between CP+5% and CP‐5% tests at any time point.

Figure 2. Physiological responses to exercise above and below CP .

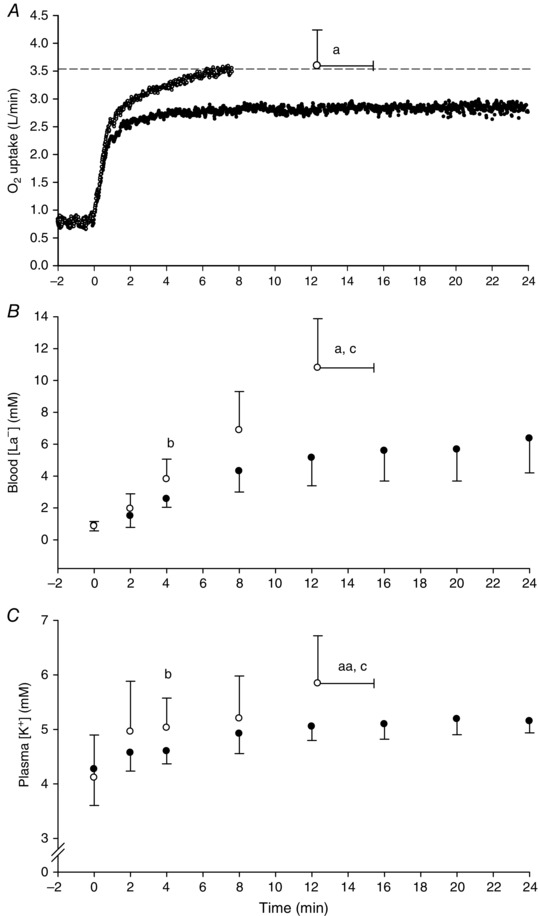
Group mean pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ (A), blood [La⁻] (B) and plasma [K⁺] (C) responses to exercise below (black symbols) and above (white symbols) the critical power. The dashed horizontal line in (A) indicates the ramp test ${\dot{V}}_{{\mathrm{O}}_2}$ peak. The horizontal error bars indicate SD for time to exhaustion and the vertical error bars indicate SD for the y‐axis variable.^aDifferent from end‐exercise value during the <CP test (P < 0.05). ^aaTrend for difference from end‐exercise value during the <CP test (P = 0.06). ^bDifferent from the same time point in the <CP test (P < 0.05). ^cDifferent from the value at 12 min in the <CP test (P < 0.05).

Figure 3. Muscle metabolic responses to exercise above and below CP .

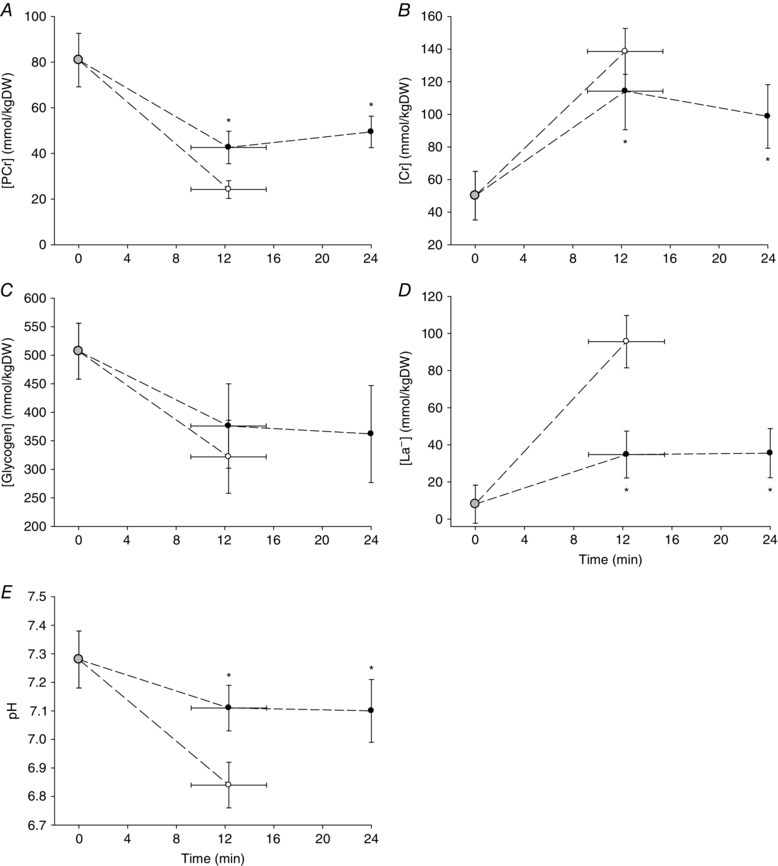
Group mean muscle [PCr] (A), [Cr] (B), [glycogen] (C), [La⁻] (D) and pH (E) during exercise just below (black symbols) and just above (white symbols) the critical power. Grey symbols indicate resting baseline values. *Different from the end‐exercise value during >CP test (P < 0.05).

The mean power outputs over the first 5, 30, 90 and 180 s of the all‐out‐sprints were not different (P > 0.05 for all comparisons). The power output data from these tests were therefore time aligned and averaged to obtain a single power profile for the all‐out test (Fig. 4 A). The ${\dot{V}}_{{\mathrm{O}}_2}$data from the 5, 30, 90 and 180 s all‐out‐sprints were time aligned and averaged in the same way (Fig. 4 B and C). The comparisons between pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$variables in the 3CWR and 3 min all‐out tests are shown in Table 1. There were no differences in ${\dot{V}}_{{\mathrm{O}}_2}$ peak between the 3CWR test (3.51 ± 0.70 l min⁻¹), the 3 min all‐out test (3.52 ± 0.65 l min⁻¹) and the ramp test ${\dot{V}}_{{\mathrm{O}}_2\max }$ (P > 0.05). The W′ was positively correlated with the all‐out test ${\dot{V}}_{{\mathrm{O}}_2}$slow component amplitude (r = 0.75, P < 0.05).

Figure 4. Power output and pulmonary oxygen uptake during 3 min of all‐out exercise and work‐matched, exhaustive CWR exercise .

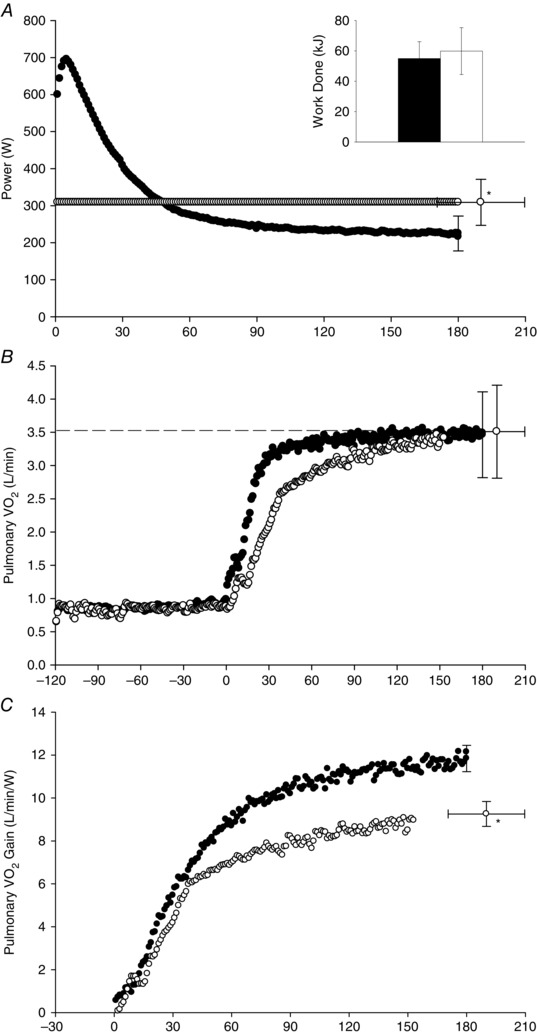
Group mean power output (A), pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ (B) and the pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ ‘gain’ (C) during the 3 min all‐out test (black symbols and bar) and the work‐matched CWR test (white symbols and bar). The dashed line in (B) indicates the ${\dot{V}}_{{\mathrm{O}}_2\max }$ measured in the ramp incremental test. To aid clarity, error bars (SD) are shown for end‐exercise time points only. *Different from the end‐exercise power output (A) and end‐exercise ${\dot{V}}_{{\mathrm{O}}_2}$ gain (C) in the all‐out test (P < 0.05).

Table 1.

Work, power and pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ variables in the 3 min all‐out test and a work‐matched CWR test

	CWR	All‐out
Total work (kJ)	58.9 ± 15.2	55.3 ± 11.4
Mean power (W)	309 ± 62	307 ± 63
Work >CP (kJ)	16.1 ± 4.5	14.9 ± 3.8
${\dot{V}}_{{\mathrm{O}}_2}$ baseline (l min−1)	0.84 ± 0.16	0.86 ± 0.17
${\dot{V}}_{{\mathrm{O}}_2}$ peak (l min−1)	3.51 ± 0.70	3.52 ± 0.65
Time‐to‐${\dot{V}}_{{\mathrm{O}}_2}$peak (s)	117 ± 31	54 ± 29*
End‐exercise gain (ml min−1 W−1)	9.3 ± 0.6	11.8 ± 0.6*
Slow component amplitude	0.4 ± 0.1	0.9 ± 0.1*
(l min−1)
Slow component gain	1.4 ± 0.4	4.1 ± 2.4*
(ml min−1 W−1)

*Different from CWR (P < 0.05).

The muscle metabolic responses for the 3 min all‐out test and the CWR3 test are shown in Fig. 5. At 5 s, the muscle [ATP] and [PCr] were lower and the muscle [La⁻] and plasma [K⁺] were higher in the all‐out test compared to CWR3 (P < 0.05 for all). The end‐exercise muscle metabolic responses and plasma [K⁺] were not different between the all‐out and CWR3 protocols, whereas the blood [La⁻] was higher in the all‐out test compared to CWR3 after 90 s and at end‐exercise. The muscle [PCr] gain was greater at end‐exercise [−277 ± 87 vs. −220 ± 85 μmol (kg d.w.)⁻¹ W⁻¹], the pH gain was greater at 90 s (−0.0015 ± 0.0006 vs. −0.0011 ± 0.0006 1/kgDW/W) and the [La⁻] gain was greater at 5 s [19 ± 18 vs. 1 ± 22 μmol (kg d.w.)⁻¹ W⁻¹] and 90 s [365 ± 109 vs. 229 ± 64 μmol (kg d.w.)⁻¹ W⁻¹] in the all‐out compared to the CWR3 test (P < 0.05 for all) (Fig. 6 A–C). The linearity of the Δ[PCr] vs. Δ ${\dot{V}}_{{\mathrm{O}}_2}$ relationship was preserved, with no differences in slope [−0.02 ± 0.01 vs. −0.02 ± 0.01 mmol (kg d.w.)⁻¹ ml⁻¹ min⁻¹] or intersect [−5.6 ± 22.2 vs. −2.7 ± 0.9 mmol (kg d.w.)⁻¹] between the all‐out and CWR protocols (Fig. 6 D).

Figure 5. Muscle and circulatory variables during 3 min of all‐out exercise and work‐matched CWR exercise .

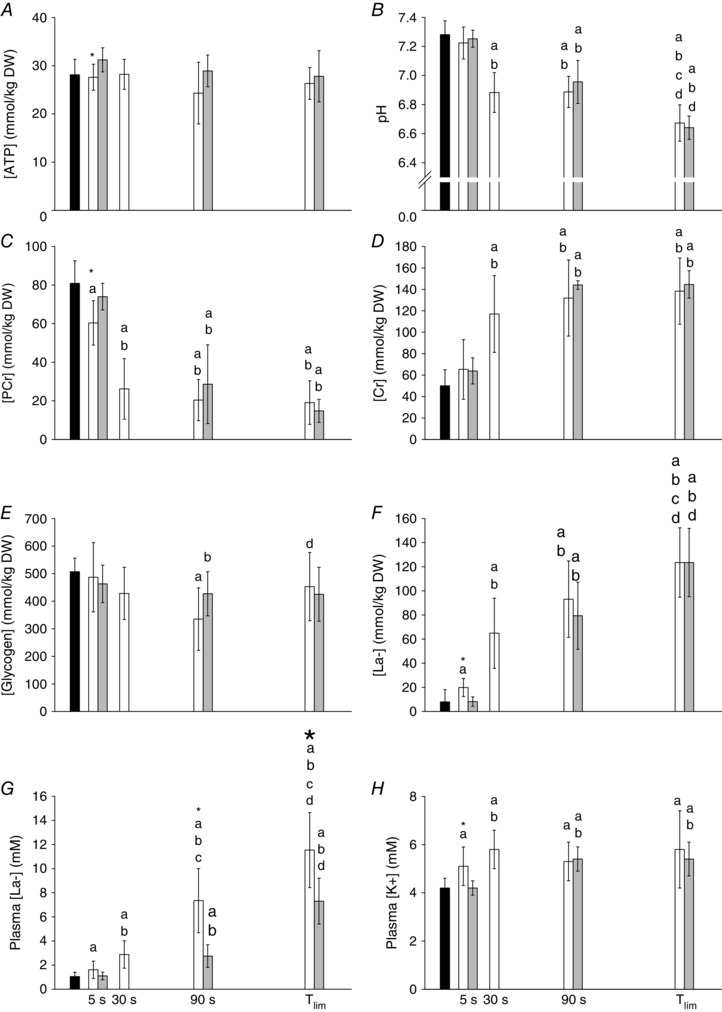
Group mean muscle [ATP] (A), pH (B), [PCr] (C), [Cr] (D), [glycogen] (E) and [La⁻] (F) and blood [La⁻] (G) and [K⁺] (H) responses during the 3 min all‐out test and the work‐matched CWR test. Black bars indicate the resting baseline; white bars indicate all‐out tests; grey bars indicate CWR tests. *Different from CWR at the same time point (P < 0.05). ^aDifferent from resting measurement (P < 0.05). ^bDifferent from 5 s within the same condition (all‐out or CWR) (P < 0.05). ^cDifferent from 30 s within the same condition (all‐out or CWR) (P < 0.05). ^dDifferent from 90 s within the same condition (all‐out or CWR) (P < 0.05).

Figure 6. Muscle metabolic responses relative to power output during all‐out and CWR exercise .

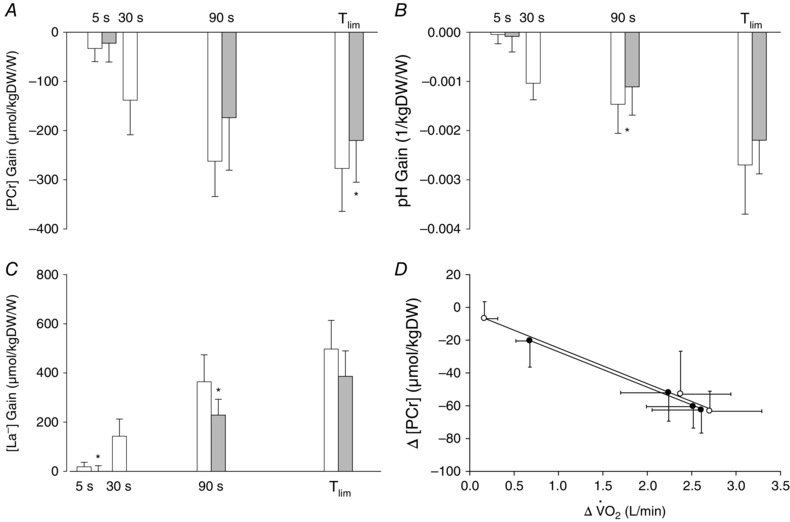
The muscle [PCr] gain (A), [pH] gain (B) and [La⁻] gain during the 3 min all‐out test and the work‐matched CWR test. *Different from all‐out test at the same time point (P < 0.05). D, linear relationships between changes in muscle [PCr] and pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ during the all‐out and CWR protocols.

No muscle tissue was obtained in the end‐exercise biopsy for the 3 min all‐out test for one female subject and therefore, the following correlations are for n = 7. There were positive correlations between the W′ and the end‐exercise muscle [La⁻] [124 ± 29 mmol (kg d.w.)⁻¹] (r = 0.88, P < 0.05) and the W′ and the end‐exercise muscle [Cr] [138 ± 31 mmol (kg d.w.)⁻¹] (r = 0.86, P < 0.05) in the 3 min all‐out test. The relationship between W′ and the end‐exercise [PCr] [19 ± 11 mmol (kg d.w.)⁻¹] was not statistically significant (r = −0.73, P > 0.05). The rates of change in muscle [PCr], [La⁻] and pH relative to the rates of W′ utilization were similar during the 3 min all‐out and CWR3 protocols (Fig. 7).

Figure 7. Muscle metabolic responses relative to W′ utilisation during all‐out and CWR exercise .

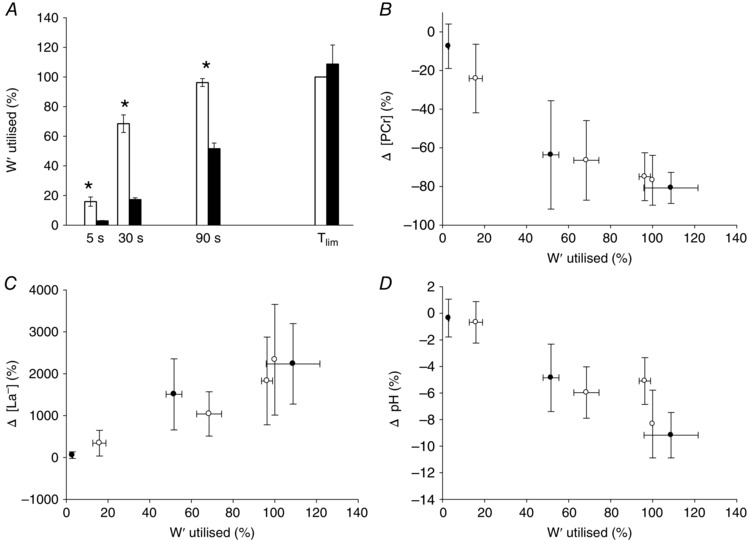
A, W′ utilised (%) after 5, 30 and 90 s and at the end of exercise (T _lim) in the all‐out (white bars) and CWR tests (black bars). The changes in muscle [PCr] (B), [La⁻] (C) and pH (D) relative to the percentage of W′ utilized were similar in the all‐out (black circles) and CWR tests (white circles). *Different from CWR test at the same time point (P < 0.05).

Study 2: Muscle fibre type and the power‐time parameters

The CP was 234 ± 53 W and the W′ was 19.4 ± 6.3 kJ. A representative stain of muscle fibre sample is shown in Fig. 1 A–C. The muscle fibre type distribution was 52 ± 17% type I, 36 ± 17% type IIa and 13 ± 13% type IIx. In one subject (who showed 66% type I and 34% type IIa+x fibres) it was impossible to differentiate between type IIa and IIx fibres due to poor staining and small sample, and therefore type IIa and IIx data are for n = 10. The CP was positively correlated with type I (r = 0.67, P < 0.05, n = 11) and inversely correlated with type IIx fibre proportion (r = −0.76, P < 0.05, n = 10) (Fig. 8 A and B), whereas there was no relationship between CP and type IIa fibre proportion (r = −0.19, P > 0.05, n = 10). In addition, CP was inversely correlated with type II (a+x) (r = −0.67, P < 0.05, n = 11) and positively correlated with type I + IIa (r = 0.75, P < 0.05, n = 10) muscle fibre proportions. The relationships between W′ and type IIx (r = 0.51, P > 0.05, n = 10) (Fig. 8 C), type IIa (r = 0.07, P > 0.05, n = 10) and type II (a+x) (r = 0.47, P > 0.05, n = 11) (Fig. 8 D) muscle fibre proportions were not significant.

Figure 8. Correlations among power‐time parameters and muscle fibre type .

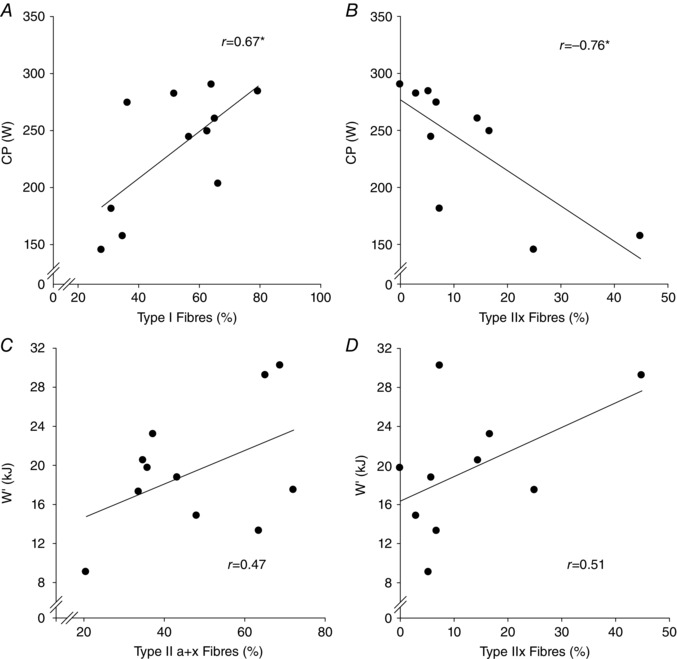
The CP was positively correlated with type I muscle fibre distribution (A) and inversely correlated with type IIx distribution (B). There were no significant relationships between the W′ and type IIa+x (C) or type IIx muscle fibre distribution (D). *P < 0.05.

Discussion

The principal novel findings of the present study are that: (1) the CP during whole‐body exercise demarcated intensities that resulted in steady‐state (<CP) and non‐steady‐state (>CP) muscle metabolic responses; (2) a high CP was associated with a high proportion of type I muscle fibres and a low proportion of type IIx muscle fibres; (3) large W′ values were associated with high end‐exercise muscle [La⁻] and [Cr], which are indices of substrate level phosphorylation; and (4) the muscle metabolic perturbation, as indicated by changes in muscle [PCr], [La⁻] and pH relative to work rate, was greater during all‐out compared to work‐matched CWR exercise. Collectively, these findings provide significant new insight into the mechanistic bases of the power‐time parameters measured during whole‐body exercise.

The primary purpose of the present study was to validate the CP estimate for whole‐body exercise by characterizing the muscle metabolic responses to cycling just below and just above CP. We replicated the protocol of Poole et al. (1988) but included end‐exercise muscle biopsies in the CP‐5% and CP+5% tests and at CP+5% T _lim isotime during a separate CP‐5% test. It was shown that the muscle [PCr], [Cr], [Gly], [La⁻] and pH remained stable between the CP+5% T _lim isotime (∼12 min) and end‐exercise measurements (24 min) when exercise was performed <CP (Fig. 3). In contrast, during exercise >CP, the ${\dot{V}}_{{\mathrm{O}}_2}$ reached maximum in all subjects, exercise tolerance was limited (∼12 min) and the end‐exercise muscle metabolic responses differed significantly from those observed during exercise <CP (Fig. 3). These data are indicative of a considerable contribution to ATP turnover from glycolysis/glycogenolysis during >CP exercise. It has been previously shown that muscle blood flow increases disproportionately during exercise >CP and is preferentially directed to type II muscle fibres that rely more heavily on glycolysis than type I fibres (Copp et al. 2010). Maximal small muscle mass exercise, such as the single‐leg knee extensions used by Jones et al. (2008), allows maximal dilatation of the active vascular bed but it does not necessitate maximal cardiac output (Roach et al. 1999). By contrast, in the whole‐body exercise model employed in the present study, cardiac output probably reached maximum over the latter stages of the CP+5% test (Poole & Richardson, 1997). Considering the plasticity of haemodynamics relative to the size of the active muscle mass (Calbet, 2000) and the lability of the CP to the manipulation of O₂ delivery (Vanhatalo et al. 2010; Simpson et al. 2015), it was necessary to assess the muscle metabolic responses below and above CP during whole‐body exercise. The present study therefore has provided the first evidence indicating that the CP for large muscle mass exercise represents a critical threshold of muscle metabolic control, consistent with previous ³¹P‐MRS data for the single‐leg knee‐extension exercise model (Jones et al. 2008).

The identification of the CP as the boundary between the heavy and severe exercise intensity domains in the present study was prerequisite for drawing inferences regarding the muscle metabolic underpinnings of the W′. The 3 min all‐out cycling test against fixed resistance represents an ideal experimental model that yields maximum ${\dot{V}}_{{\mathrm{O}}_2}$ slow component amplitude, attainment of ${\dot{V}}_{{\mathrm{O}}_2\max }$, significant muscle metabolic perturbation and complete utilization of the W′ (Vanhatalo et al. 2007; 2011). We confirmed the positive correlation between the W′ and the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component amplitude reported previously (Murgatroyd et al. 2011; Vanhatalo et al. 2011) and, by including muscle biopsies in this experimental model, we were able to assess muscle metabolites associated with fatigue and the potential loss of efficiency in all energy metabolic pathways simultaneously with measurements of ${\dot{V}}_{{\mathrm{O}}_2}$ and W′. The muscle [PCr] ‘gain’ (i.e. Δ[PCr] relative to Δ work rate) was significantly greater after ∼3 min of all‐out compared to CWR exercise, and the muscle pH and [La⁻] gains also became progressively greater during all‐out compared to CWR exercise. These data indicate that the muscle metabolic perturbation proceeded at a faster rate together with the development of a more pronounced ${\dot{V}}_{{\mathrm{O}}_2}$ slow component (∼0.93 l min⁻¹) during all‐out exercise compared to work‐matched CWR exercise (∼0.39 l min⁻¹). Collectively, these pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ and muscle metabolic data are consistent with a progressively greater increase with time in ATP resynthesis from both oxidative and glycolytic/glycogenolytic metabolism during the all‐out test compared to CWR exercise.

The proposed mechanistic underpinnings of the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component include a progressive recruitment of higher‐order (fast‐twitch, or type II) muscle fibres, slower ${\dot{V}}_{{\mathrm{O}}_2}$ kinetics of higher‐order fibres compared to slow‐twitch (type I) fibres and/or the O₂ cost of recovery processes in fatigued muscle fibres that are de‐recruited as exhaustive exercise proceeds (i.e. loss of efficiency) (Krustrup et al. 2004; Jones et al. 2011). The progressively increasing O₂ cost of force production during the 3 min all‐out test (as the ${\dot{V}}_{{\mathrm{O}}_2}$ approaches maximum and power output approaches CP) represents a mirror image of the conventional ${\dot{V}}_{{\mathrm{O}}_2}$ slow component that develops during severe CWR exercise, where the work rate remains constant and the ${\dot{V}}_{{\mathrm{O}}_2}$increases (Vanhatalo et al. 2011). We showed that the linearity of the ΔPCr vs. Δ${\dot{V}}_{{\mathrm{O}}_2}$ relationship (Fig. 6 D) was preserved during the maximal all‐out and CWR protocols. Consistent with established models of respiratory control (Mahler, 1985; Meyer, 1988), these data suggest that the O₂ cost of ATP resynthesis did not change during these protocols, which both generated a large ${\dot{V}}_{{\mathrm{O}}_2}$slow component. The present data, therefore, represent novel experimental evidence in a large muscle mass exercise model in support of the notion that the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component derives from elevated ATP cost of force generation as fatiguing exercise proceeds (Tonkonogi et al. 1999; Rossiter et al. 2002).

The definition of W′ as a fixed work capacity (J) that can be utilized before exhaustion occurs during exercise >CP has considerable experimental foundation (Fukuba et al. 2003; Chidnok et al. 2013), although its complex physiological correlates have proven difficult to interpret. Given the plasticity of the W′ in the face of muscle [Gly] and [PCr] manipulation, it was originally postulated that the magnitude of the W′ might be determined by the size of the ‘anaerobic’ energy substrate stores (Smith et al. 1998; Miura et al. 1999; 2000). If the W′ represents a work capacity which derives from substrate level phosphorylation, it would be expected to be related to changes in muscle [PCr] and [Cr] and muscle [La⁻]. We showed that large W′ values were indeed associated with high concentrations of end‐exercise muscle Cr and La⁻, and also tended to be associated with low levels of muscle PCr, at the end of the 3 min all‐out test. These data suggest that the mechanistic bases of the W′ are probably related to muscle glycolytic capacity and the capacity for PCr breakdown. This interpretation is consistent with the reduction in W′ observed following glycogen depletion (Miura et al. 2000).

It is important to consider that the muscle [PCr] may influence the W′ by mechanism(s) other than acting as an ‘anaerobic’ substrate store. The evidence on the effects of Cr loading on the W′ is mixed, with some studies showing an increase in W′ (Smith et al. 1998; Miura et al. 1999) and others showing no significant effects (Eckerson et al. 2005; Vanhatalo & Jones 2009). It should be considered that Cr loading increases not only muscle [PCr], but also muscle [Cr], and changes in PCr/Cr ratio influence the sensitivity of mitochondrial respiration to ADP (Walsh et al. 2001). Amplification of the ADP signal by Cr may occur in highly oxidative muscle fibres (cardiac and type I skeletal muscle) in which the permeability of the outer mitochondrial membrane to cytosolic ADP is low, but not in fast‐twitch (type II) muscle fibres with high outer membrane ADP permeability (Kushmerick et al. 1992; Kuznetzov et al. 1996). In other words, in highly oxidative type I fibres compared to type II fibres, a lesser degree of cytosolic ADP rise (metabolic perturbation) is required to yield a given metabolic rate (${\dot{V}}_{{\mathrm{O}}_2}$), indicative of tighter coupling of ATP demand and supply. This is pertinent, because the sensitivity of the W′ to interventions that alter the ${\dot{V}}_{{\mathrm{O}}_2}$ kinetics and/or the ${\dot{V}}_{{\mathrm{O}}_2\max }$ (Jones et al. 2003; Ferguson et al. 2007; Vanhatalo et al. 2010; Bailey et al. 2011; Simpson et al. 2015) implies that the mechanistic bases of the W′ are intricately linked to respiratory control via the creatine kinase reaction (Kay et al. 2000; Meyer 1988).

We hypothesized that the W′ would be positively correlated with the proportion of type II (a+x) muscle fibres and the CP would be correlated with the proportion of type I muscle fibres, based on the premise that the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component amplitude is related to muscle type II fibre proportion and the phase II kinetics is related to type I fibre proportion (Barstow et al. 1996; Pringle et al. 2003). We showed that a high CP was associated with a high type I and a low type IIx muscle fibre proportion, whereas there was no relationship between CP and the percentage of type IIa muscle fibres. Type IIa ‘oxidative‐glycolytic’ fibres are more fatigue‐resistant and have greater succinate dehydrogenase activity than type IIx fibres (Sant'Ana Pereira et al. 1996). However, type IIa fibres also have a high glycolytic enzyme content and are more easily fatigable than type I fibres (Schiaffino & Reggiani, 2011). The greater fatigue‐resistance of type I muscle fibres stems from greater mitochondrial size and density and better developed cristae, such that they are less reliant on glycolysis than type II (a+x) fibres (Schiaffino et al. 1970; Kugelberg, 1973). At the onset of dynamic all‐out exercise, essentially all available task‐specific motor units are maximally (>95%) recruited (Beelen et al. 1995) such that progressive recruitment of additional fibres cannot occur (Vanhatalo et al. 2011). The highest order fibres (i.e. type IIx) are the primary contributories to external work over the early stages of an all‐out test where there is also a significant proportion of the W′ accumulated. Type II fibres would be expected to become progressively fatigued (first IIx, then IIa fibres) and to stop contributing to external work as all‐out exercise proceeds (Sant'Ana Pereira et al. 1996; Sargeant & de Haan, 2006) such that, over the latter stages where the CP is attained (2.5–3 min), the external work probably derives almost exclusively from the type I, most fatigue‐resistant fibres. The precise muscle fibre recruitment pattern during prolonged all‐out exercise beyond ∼30 s in duration warrants further investigation using single fibre metabolite analyses. The finding that the CP signifies a threshold above which blood [La⁻] and acid–base balance and muscle pH and [La⁻] cannot be stabilized (present study; Poole et al. 1988; Jones et al. 2008), however, is consistent with the positive relationship between CP and highly oxidative muscle type I fibres.

By contrast to our hypothesis, we did not observe significant relationships between the W′ and type IIx, type IIa or type II(a+x) muscle fibre distribution in Study 2. We reasoned that the W′ might be proportional to the type II fibre population because the W′ has been positively correlated with the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component amplitude (Murgatroyd et al. 2011; Vanhatalo et al. 2011) and the slow component is associated with a high proportion of type II muscle fibres (Barstow et al. 1996; Pringle et al. 2003). Study 1 provided some evidence that the W′ may be related to the specific bioenergetic characteristics of type II muscle fibres, given that the PCr and glycogen degradation rates are faster in type II vs. type I muscles during maximal exercise (Greenhaff et al. 1994) and also that the type II fibres have significantly greater [PCr] (Sant'Ana Pereira et al. 1996) and total [Cr] (Edström et al. 1982) compared to type I muscle fibres. High muscle total [Cr] has also been associated with slow muscle [PCr] (and, by extension, ${\dot{V}}_{{\mathrm{O}}_2}$) on‐ and off‐kinetics during transitions to exercise and recovery (Meyer, 1989). Therefore, the positive correlations between the W′ and muscle [Cr] and [La⁻] observed in Study 1 may originate from differences in muscle fibre type distribution, suggesting that individuals with a high W′ tend to have a greater proportion of type II fibres. However, considering the probable fibre type recruitment pattern during the 3 min all‐out test where higher‐order, fatigable fibres are sequentially contributing to power output and then dropping‐out, a more varied mix of different fibre populations probably contributes to W′. In contrast, the CP is measured at the end of the test (2.5–3 min), when type I fibres are deemed to be (almost) the sole contributors to external power output. During the time period over which the W′ is accumulated in a 3 min all‐out test (>95% over the first 90 s), type I muscle fibres also contribute to external work and it is therefore a reasonable assumption that they also contribute to the work measured as W′. Collectively, these observations indicate that the size of the W′ is not proportional to any specific muscle fibre type population but, rather, that there are multifactorial determinants of this parameter probably including mechanisms common to respiratory control via the creatine kinase reaction.

It is important to note that muscle fibre type assessment on the basis of myosin heavy chain characteristics in the present study does not necessarily reflect muscle oxidative capacity. Type IIa ‘oxidative‐glycolytic’ fibres are sensitive to endurance training such that they can exhibit a highly oxidative phenotype resembling type I fibres in the trained state (Schiaffino & Reggiani, 2011). The cross‐sectional area of type II fibres is generally greater than that of type I and the cross‐sectional area of all fibre types tends to be sensitive to training (Folland & Williams, 2007). Comparisons of the relationships between fibre type (proportion and cross‐sectional area), muscle oxidative capacity and the power‐time parameters may help clarify these issues in future studies. Although the results of the present study indicate that fibre type proportion plays a role in determining the CP, previous research has shown that muscle energetics (Jones et al. 2008) and O₂ delivery (Copp et al. 2010; Vanhatalo et al. 2010) also represent key determinants of the CP. The variability in the relationship between CP and type I fibre proportion (Fig. 8 A) may be indicative of the multifactorial determinants of this parameter.

Conclusions

The data reported in the present study indicate that the CP measured during whole‐body exercise reflects the bioenergetic characteristics of highly oxidative type I muscle fibres, such that a muscle metabolic steady‐state is attainable below but not above CP. We further demonstrated that the greater pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ slow component occurred alongside greater muscle metabolic perturbation, as indicated by the gains of muscle [PCr], pH and [La⁻], during all‐out compared to CWR exercise. The ${\dot{V}}_{{\mathrm{O}}_2}$ slow component, which was positively correlated with the W′, therefore represented an increased phosphate cost of force production originating from the elevated energy contribution from both oxidative and glycolytic/glycogenolytic metabolism during the all‐out test. We also showed that, although the W′ was associated with muscle bioenergetic ([Cr], [La⁻]) and systemic (pulmonary ${\dot{V}}_{{\mathrm{O}}_2}$ slow component) characteristics of type II muscle fibres, it was not correlated with any specific fibre type population. Collectively, the present studies have therefore provided novel evidence of a mechanistic link between the bioenergetic characteristics of type I and II muscle fibres and the power–time relationship for high‐intensity exercise. The data reported in the present study provide novel evidence in an in vivo whole‐body exercise model for the utilization of W′, development of the ${\dot{V}}_{{\mathrm{O}}_2}$ slow component and progression of muscle metabolic perturbation being temporally linked.

Additional information

Competing interests

The authors declare that they have no competing interests.

Author contributions

A.V. contributed to the conception and design of the experiment, collection, analysis and interpretation of the data, and writing of this article. M.I.B., F.J.D., J.R.B., J.F.S., C.T., L.J.W. contributed to the design of the experiment, collection and analysis of data, and critical revision of this article. J.B., P.K., M.M. and A.M.J. contributed to the conception and design of the experiment, collection and analysis of data and critical revision of this article. All authors approved approved the final version of the manuscript and agree to be accountable for all aspects of the work. All persons designated as authors qualify for authorship, and all those who qualify for authorship are listed.

Funding

No funding was received for the present study.