Posterior Eye Shape Measurement With Retinal OCT Compared to MRI

Abstract

Purpose

Posterior eye shape assessment by magnetic resonance imaging (MRI) is used to study myopia. We tested the hypothesis that optical coherence tomography (OCT), as an alternative, could measure posterior eye shape similarly to MRI.

Methods

Macular spectral-domain OCT and brain MRI images previously acquired as part of the Singapore Epidemiology of Eye Diseases study were analyzed. The right eye in the MRI and OCT images was automatically segmented. Optical coherence tomography segmentations were corrected for optical and display distortions requiring biometry data. The segmentations were fitted to spheres and ellipsoids to obtain the posterior eye radius of curvature (R_c) and asphericity (Q_xz). The differences in R_c and Q_xz measured by MRI and OCT were tested using paired t-tests. Categorical assignments of prolateness or oblateness using Q_xz were compared.

Results

Fifty-two subjects (67.8 ± 5.6 years old) with spherical equivalent refraction from +0.50 to −5.38 were included. The mean paired difference between MRI and original OCT posterior eye R_c was 24.03 ± 46.49 mm (P = 0.0005). For corrected OCT images, the difference in R_c decreased to −0.23 ± 2.47 mm (P = 0.51). The difference between MRI and OCT asphericity, Q_xz, was −0.052 ± 0.343 (P = 0.28). However, categorical agreement was only moderate (κ = 0.50).

Conclusions

Distortion-corrected OCT measurements of R_c and Q_xz were not statistically significantly different from MRI, although the moderate categorical agreement suggests that individual differences remained. This study provides evidence that with distortion correction, noninvasive office-based OCT could potentially be used instead of MRI for the study of posterior eye shape.

Myopia is a common condition affecting a large percentage of individuals worldwide, ranging from 30% to 90% depending on the age of the population (more common in younger populations), ethnicity (more common in Chinese, Japanese, Koreans), and region (more common in urban cities in Asia).^1–5 It has been shown that eye shape in individuals with myopia—especially those with high myopia and pathologic myopia—differs compared to that in emmetropic individuals.^6–8 Furthermore, different types of posterior eye shape are associated with pathologic forms of myopia with vision-threatening complications.^9–11 These pathologic forms of myopia are a major cause of vision loss, particularly in Asian countries. Understanding structural changes in myopia can provide insight into mechanisms and hence help in development of prevention strategies.

The vast majority of studies investigating posterior eye shape have been performed with magnetic resonance imaging (MRI).^6–8,12,13 While MRI provides a complete three-dimensional (3D) representation of the entire eye free of optical distortions, there are logistical and financial barriers to readily obtaining MRI images of the eye on a routine basis, limiting the current use of posterior eye shape primarily to highly specialized research settings. Developing techniques as alternatives to MRI would make measurement of posterior eye shape more accessible to both researchers and clinicians and may allow better understanding of the structural changes that lead to pathologic myopia.

Since its invention, optical coherence tomography (OCT) has become a readily available and indispensable 3D ocular imaging technique in widespread use in eye clinics and research centers worldwide.^14,15 Optical coherence tomography images of the eye, however, suffer from distortions that affect OCT's ability to accurately represent the shape of the eye.^16–20 Briefly, these distortions result from refraction of OCT light through the eye and from display of nontelecentric scans on a rectangular format. When these optical and display distortions are corrected, the difference between OCT- and MRI-measured posterior eye shape expressed as spherical radius of curvature is decreased.²⁰

The objective of this study was to test the hypothesis that distortion-corrected OCT images of the posterior eye would produce similar measurements of both spherical and aspherical posterior eye shape compared to MRI images of the same eyes. Optical coherence tomography and MRI images previously acquired as part of a national epidemiologic study were used for this study. This was a unique dataset because the OCT data were acquired using a common, commercially available OCT system. Further, instead of a highly controlled acquisition protocol specific for shape, these OCT images were acquired using regular clinical protocols and therefore are broadly applicable as a reflection of general OCT usage.

Methods

Subject Selection

The study dataset was drawn from subjects who had previously enrolled in the Singapore Epidemiology of Eye Diseases (SEED) study and its Epidemiology of Dementia in Singapore (EDIS) substudy.^3,21–24 Both were multiethnic, population-based studies of 40- to 85-year-old Singaporeans. For the SEED and EDIS studies, the research followed the tenets of the Declaration of Helsinki; informed consent was obtained from the subjects after explanation of the nature and possible consequences of the study; and the research was approved by the SingHealth Institutional Review Board (IRB) and the National Healthcare Group Domain-Specific Review Board. For the current retrospective study, the research followed the tenets of the Declaration of Helsinki, and the research was additionally approved by the Duke University Medical Center IRB.

A total of 251 subjects who had undergone both ocular OCT scans and brain MRI scans in the SEED study and EDIS substudy were initially included. From this population, subjects with a spherical equivalent refraction of +0.50 or more myopic were chosen to include emmetropic and myopic individuals (101 subjects from the initial population). Subjects were then excluded if there was no macular OCT scan (macular scans were used for OCT analysis: −13 subjects), if they were pseudophakic (pseudophakic refraction may not reflect the original refractive error of the eye: −23 subjects), or if they lacked ocular biometric values (required for OCT distortion correction in this study: −13 subjects). A final total of 52 subject datasets met all the inclusion and exclusion criteria and proceeded to analysis.

MRI Analysis

The MRI images (3T Magnetom Trio Tim; Siemens, Munich, Germany) were previously acquired using a 32-channel head coil at the Clinical Imaging Research Centre of the National University of Singapore. The standardized protocol included high-resolution, 3D T1-weighted Magnetization Prepared Rapid Gradient Recalled Echo (MPRAGE) sequences with 1.0- × 1.0- × 1.0-mm³ voxels, repetition time (TR) = 7.2 ms, time to echo (TE) = 3.3 ms, inversion time (TI) = 900 ms, flip angle = 9°, and matrix = 256 × 256 × 180 mm³.

For MRI analysis, axial slices were created from the 1-mm isotropic MPRAGE volumes to match the orientation of the axial OCT scans. The slices were exported as bitmap image stacks for analysis. Using active contours²⁵ implemented in MATLAB (MathWorks; Natick, MA, USA), the interface between the dark vitreous and bright eye wall was automatically segmented in each slice containing the right eye; the search region was initially limited by manual designation of two opposite corners of a box encompassing the eye (similar to Fig. 1B) and an arbitrary point within the vitreous. In the axial slice containing both the lens and optic nerve, an optical axis was defined by manual designation and linear fitting of three points: apex of the anterior cornea, apex of the anterior lens, and apex of the posterior lens. Relative to this optical axis, all images were rotated by 3.5° in the axial plane so that the posterior eye shape was estimated with reference to the visual axis that passed through the fovea (z-axis).²⁶ This estimate of “visual axis” was required because there was insufficient resolution in the MRI scans to visualize the fovea directly; the difference between the visual and optical axis in an older population similar to ours was approximately 3.5°.²⁷ The anterior 120° containing the cornea and lens was then removed.²⁸ Hence the posterior 240° of the eye with visual axis coincident with the z-axis was the final form used for shape analysis (Fig. 1). (In this work, the axis designations are x-axis: left–right lateral axis, y-axis: cranial–caudal axis, and z-axis: anterior–posterior axis. Using this axis designation, the axial plane is the xz plane, the coronal plane is the xy plane, and the sagittal plane is the yz plane).

Figure 1.

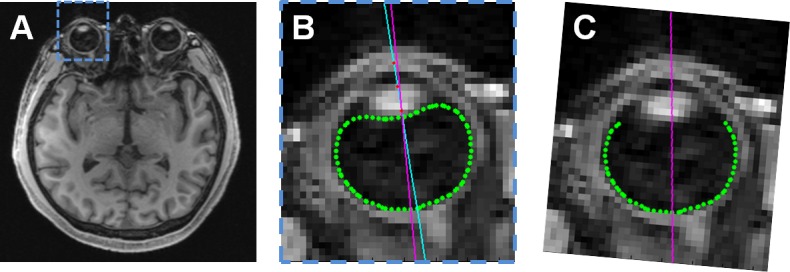
Overview of analysis of MRI images. (A) The right eyes from 1-mm isotropic axial MPRAGE slices were analyzed. (B) Magnified view of blue-bounded box in (A). The vitreous/eye wall boundary was automatically segmented (green) and a user-defined optical axis through the corneal apex, anterior lens apex, and posterior lens apex was created (red dots and light blue line). The visual axis (magenta) was defined as 3.5° temporal to the optical axis relative to the posterior lens apex. (C) The eye was rotated so that the visual axis was parallel to the z (anterior–posterior) axis. The posterior 240° of the segmented volume was then fit to the desired shape. (Segmentations from the other slices comprising the right eye volume are not shown here).

OCT Analysis

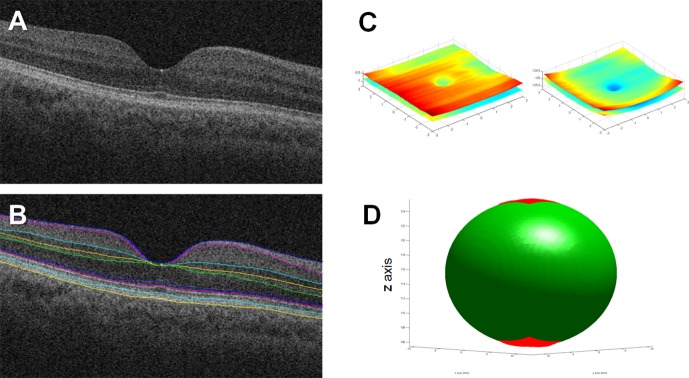
Nominally 6- × 6-mm macular OCT (Cirrus; Zeiss, Jena, Germany) scan volumes of the dilated right eye composed of 1024 depth pixels × 512 A-scans × 128 B-scans were used for OCT analysis. We imported the OCT volumes into a custom, automated layer segmentation software—the Duke OCT Retinal Analysis Program (DOCTRAP). The core automated segmentation algorithm of DOCTRAP is based on the graph theory and dynamic programming framework.²⁹ Using DOCTRAP, the retinal pigment epithelium (RPE) was automatically segmented within each axial B-scan. Optical and display distortions were then removed using a numeric distortion correction algorithm based on an optical model of the OCT scanner and patient eye implemented in OpticStudio Standard (Zemax, LLC, Kirkland, WA, USA).²⁰

Regarding the OCT scanner in the model, an important step was calibrating the scan dimensions of the specific OCT system used to acquire the retinal OCT data. Achromatic focusing lenses (Thorlabs, Inc.; Newton, NJ, USA) were mounted in front of the Cirrus used in the SEED study to focus the retinal scan beam to a micrometer target (Newport Corp., Irvine, CA, USA). A 1024- × 512- × 128-pixel volume was taken to replicate the retinal scans. Using the imaged micrometer target, the scan dimensions were determined and used to optimize the software model of the OCT scanner. This calibration was performed once to represent the base state of the OCT system.

Regarding the patient eye, a schematic model eye was used as a basis,³⁰ and for each subject, the model eye was customized by incorporating the subject's corneal curvature, anterior chamber depth (ACD), and axial length (AL) as measured in the SEED study (Zeiss IOLMaster). The model OCT scanner and eye were then combined, and both were optimized together to minimize the spot size across the retina. From this model, the modeled scan beam positions were used to distortion correct the OCT scan. For optical path length to physical distance conversions in the posterior eye OCT image, a presumed uniform refractive index of 1.34 was used.³¹ The distortion-corrected right eye RPE segmentation volumes were the final form used for shape analysis.

Shape Analysis

Two forms of shape analysis were performed on the eye images: spherical and aspherical. For spherical analysis, we fit the eye segmentations—both MRI and OCT—to the algebraic equation for a sphere using the least squares method.³² The specific form was the spherical form of the general quadric surface equation: A(x² + y² + z²) + 2Gx + 2Hx + 2Iz = 1. The radius of curvature of the fitted sphere (R_c) was used as the spherical shape descriptor.

For aspherical analysis, we fit the eye segmentations to the algebraic equation for an ellipsoid constrained to have its axes aligned to the Cartesian axes using the least squares method.³² The specific form was Ax² + By² + Cz² + 2Gx + 2Hx + 2Iz = 1. However, for the OCT segmentations, there was insufficient information in just the limited, approximately 6- × 6-mm scanned surface to successfully fit an ellipsoid with this equation; instead, the fitting resulted in hyperboloids. To fit to an ellipsoid, additional information was added to further constrain the fitting. Specifically, biometry data available from the distortion correction step was used to create a pilot estimate of the axial radius of curvature: R_xz,pilot = (AL − ACD)/2. The OCT surface was then reflected across the xy plane. This resulted in a mirrored copy of the OCT surface whose center was 2 * R_xz,pilot away from the center of the original OCT surface. This mirroring was done only to enable ellipsoid fitting, which has mirrored symmetry, and was not meant to reflect mirrored biological morphology. With these pilot estimates and constraints, the OCT segmentations could then be fit to an ellipsoid (Fig. 2). For both imaging modalities, the axial asphericity Q_xz was defined as R_x²/R_z² − 1 where R_x and R_z were the radii in the designated axes for the fitted ellipsoid. Categorical descriptors were assigned using Q_xz > 0 as oblate and Q_xz < 0 as prolate. Aspheric descriptors were limited to the xz plane because the segmentations for both OCT and MRI were performed in that plane. While OCT B-scans in the fast axis (xz in this case) are relatively motionless, the orthogonal slow scan axis (yz in this case) would be subject to significant motion artifacts, which could affect shape measurements; OCT scans taken with the fast axis in the yz direction were not available in this dataset.

Figure 2.

Overview of analysis of OCT images. (A) Standard view of one representative B-scan from OCT volume of a right eye with the axial dimension stretched per convention. (B) Retinal layers were automatically segmented. Only the retinal pigment epithelial layer (bottom yellow layer) was used for shape analysis. (C) Original (left) and distortion-corrected (right) retinal surfaces from the volume displayed using isotropic axes. Note the relative flatness of the retina in the original data and the restoration of ocular curvature in the distortion-corrected data. For spherical fitting, these surface data were all that was required. (D) Given the limited area covered by the OCT scan, additional pilot estimates were required for successful ellipsoid fitting. Briefly, the corrected retinal surface from (C), represented as the bottom red surface in (D), was mirrored across the xy plane and placed [axial length minus anterior chamber depth] away from the original surface. The mirrored surface (represented as the top red surface in [D]) and the estimate of the z radius (represented by half the distance between the two surfaces) allowed for ellipsoid fitting of the OCT data.

Statistical Analysis

All statistical analyses were performed in JMP Pro 12.0.1 (SAS Institute, Cary, NC, USA). For numerical comparisons, paired t-tests were performed to compare MRI and OCT measurements of R_c and Q_xz. Paired differences between MRI and OCT measurements for the study population were considered statistically significantly different for P ≤ 0.05. To have 80% power to detect a difference of at least 500 μm between MRI and OCT measures of R_c with α = 0.05, a minimum of 48 eyes needed to be analyzed. This difference was appropriate given that the pixel spacing in the MRI images was 1 mm. Additionally, Bland-Altman plots were generated to provide a visual representation of the distribution and the limits of agreement (±1.96 * standard deviation of paired differences).³³

For categorical comparisons of asphericity (prolate and oblate), overall agreement was calculated as number of matching comparisons divided by the total subjects. Additionally, McNemar's test was performed, and kappa (κ) was used to estimate agreement between MRI and OCT categorical designations of asphericity. Sensitivity and specificity were also calculated for the prolate state using MRI as the gold standard.

Results

The final population consisted of 52 right eyes of 52 subjects (mean ± SD age: 67.8 ± 5.6 years; 16 males, 36 females). The spherical equivalent refraction ranged from +0.50 to −5.38 diopters (D) (mean −0.81 D).

Spherical Shape Analysis

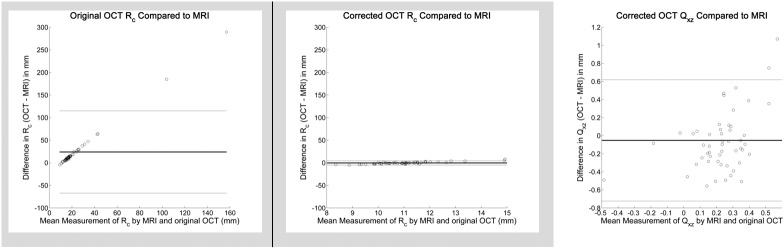
The mean paired difference between MRI and original, non–distortion-corrected OCT measures of R_c was 24.03 ± 46.49 mm (P = 0.0005) with limits of agreement of −67.08 and 115.15 mm. After distortion correction of OCT images, the mean paired difference between MRI and OCT measures of R_c was −0.23 ± 2.47 mm (P = 0.51) with limits of agreement of −5.06 and 4.61 mm. The distribution of differences in Bland-Altman form is shown in Figure 3, left and center.

Figure 3.

Bland-Altman comparison of spherical radius of curvature (R_c) measured by MRI and original uncorrected OCT (left) and corrected OCT (middle) as well as comparison of asphericity (Q_xz) measured by MRI and corrected OCT (right). The differences in R_c in mm (y-axis) for the left and middle plots are set to the same scale to facilitate comparison between original and corrected OCT. Left: R_c for original OCT versus MRI: The mean difference was 24.03 mm. The Bland-Altman limits of agreement (±1.96× standard deviation of differences, gray lines) were −67.08 and 115.15 mm. Middle: R_c for corrected OCT versus MRI: The mean difference was −0.22 mm. The limits of agreement were −5.06 and 4.61 mm. Compared to the uncorrected OCT in the left plot, the difference and distribution are substantially smaller. Right: Q_xz for corrected OCT versus MRI: The mean difference was −0.0515. The limits of agreement were −0.7234 and 0.6204.

Aspherical Shape Analysis

For aspherical analysis, only distortion-corrected OCT images were used because the lower-order spherical fitting (R_c) already showed a substantial difference compared to MRI. The mean paired difference between MRI and distortion-corrected OCT measures of Q_xz was −0.052 ± 0.343 (P = 0.28) with limits of agreement of −0.7234 and 0.6204. The distribution of differences in Bland-Altman form is shown in Figure 3, right.

Categorically, OCT agreed with MRI classification of oblateness (Q_xz > 1) and prolateness (Q_xz < 1) in 47 of 52 eyes for an overall agreement of 90.4%. Within the 49 eyes classified as oblate by MRI, OCT also categorized 44 of those eyes as oblate and misclassified 5 eyes as prolate. Out of 3 eyes that MRI categorized as prolate, OCT also categorized all 3 of those eyes as prolate. Assuming MRI to be the gold standard and “prolate” to be the nonnormal state, the resultant sensitivity of OCT for prolateness is 100%, and the specificity of OCT for prolateness is 89.8%. Overall, κ = 0.50 with 95% bounds of 0.14 to 0.86.

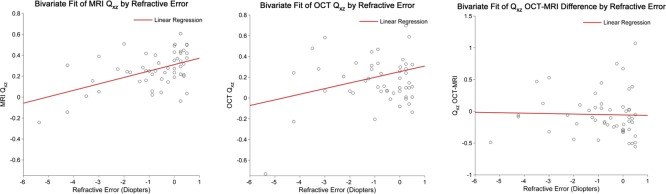
The distributions of Q_xz,MRI, Q_xz,OCT, and their paired difference, Δ(Q_xz,OCT − Q_xz,MRI), as a function of the spherical equivalent refractive error are shown in Figure 4. For MRI, Q_xz showed a trend of becoming more prolate as myopia increased. There was a similar trend for OCT. There was no apparent relationship between Δ(Q_xz,OCT − Q_xz,MRI) and refractive error.

Figure 4.

Relationship between asphericity (Q_xz) and spherical equivalent refractive error as measured by MRI (left) and OCT (center). The red lines are linear regressions with R² of 0.25, 0.07, and 0.0009 from left to right. Rather than an exact fitting of the data, the linear regressions show the general trend of less oblateness as myopia increases in both MRI and OCT measures of Q_xz. The right figure shows the paired differences between OCT measures of Q_xz by MRI and OCT as a function of refractive error. Refractive error did not appear to affect the differences between MRI and OCT (slope = −0.06).

Discussion

Posterior eye shape assessment has been used in the study of myopic refractive error and has been associated with pathologic forms of myopia. The predominant imaging modality used to measure posterior eye shape has been MRI. In contrast to MRI, OCT is far more accessible in eye care settings worldwide, is less invasive, and is less costly than MRI. With correction of optical and display distortions inherent to OCT, we showed that there was no statistically significant difference between MRI- and OCT-measured posterior eye shape as described by spherical radius of curvature. When using higher-order descriptors of posterior eye shape (asphericity, Q), there was similarly no statistically significant difference between the MRI and OCT measures for this population. In prior work,²⁸ Q_xz values by MRI in an emmetropic and myopic population ranged from −0.06 to 0.55, which is consistent with the MRI Q_xz values measured in this study. In that same work and others,⁸ a trend of decreasing oblateness with increasing myopic refractive error was shown, which was also seen in this study for both MRI and OCT measures of Q_xz.

Although there was no statistically significant difference between MRI and OCT measures of asphericity for this population, closer examination of the data revealed important differences if viewed at the individual rather than population level. Even with OCT distortion correction and considering the expected range of Q_xz values reported by others, the scale of the standard deviation of the paired differences between MRI and OCT for Q_xz is half that range. For eyes classified as oblate by MRI, OCT misclassified 10% of those as prolate. Overall, the classification agreement between MRI and OCT was also merely moderate. Given that there were only three prolate eyes (by MRI) in this study population, OCT performance could potentially differ, be better or worse, with more prolate eyes. The number of prolate eyes seen in this study is consistent with that of prior work, however.^8,28

There are important limitations to the use of OCT for measuring posterior eye shape. First, these OCT scans were acquired using standard commercial systems and protocols. Were a different OCT system used, potentially even of the same type, specific calibration measurements and models of the system would likely need to be created on a per system basis. There are also useful parameters that cannot currently be obtained from the commercial system. Altering the distance between the eye and OCT system can affect the OCT displayed eye curvature. Ophthalmic photographers often use this knowledge to flatten the retinal OCT image. Knowing the OCT system to eye distance would remove this source of error in the distortion correction algorithms. Also, OCT scans are sequential in time, and patient motion in the scan introduces higher-frequency artifacts into the scans. Spherical and aspherical fittings serve effectively as low-pass filters to mitigate some of the motion artifacts for our analyses; faster OCT image acquisition rates or a dedicated motion correction solution at the time of capture would minimize captured motion artifacts in the actual imaged surface.³⁴ However, our current use of these global, lower-order shape descriptors (sphere, ellipsoid) does not describe localized deformations. Currently available descriptive classification systems for localized deformations⁹ still play a valuable role until numeric methods for identifying localized deformations are developed. Finally, the OCT scans used in this population covered only a 6- × 6-mm area of the back of the eye. This is a small percentage of the surface area of the posterior eye (only 4.3% of the approximately 831 mm² for the posterior half of a sphere with radius 11.5 mm). Generating Q_xz for OCT in this study required additional biometric data from non-OCT devices and pilot constraints to ensure an ellipsoid fitting; these constraints likely affected the numeric accuracy of the Q_xz measurement because of the constraint this imposes on R_xz. Direct surface comparison was also not possible as unique registration points were not shared between MRI and OCT (foveal pit present in OCT but not seen in MRI; optic nerve present in MRI but not seen in these macular OCT volumes). Future advances in OCT systems with longer and wider scan ranges such as full eye biometers and wide-field systems will help overcome these current limitations in scan range.^35–40 Minor adjustments would need to be considered in wide-field OCT scans for features such as optic nerve head. Automated methods^41,42 can be used to detect and exclude this known, localized deformation prior to global shape fitting of the posterior eye. Overall, though, because the algorithms and analyses used in this study should be conceptually generalizable to any OCT system, they can be adapted to take advantage of future improvements in OCT hardware.

It is important to note that although MRI has been used in multiple studies as the standard for measuring eye shape,^{6–8,12,13,26,28} MRI can itself be subject to inaccuracies besides uncertainties from resolution limits. Geometric distortions in MRI images result from magnetic gradient field nonlinearities and magnetic field inhomogeneities.^43,44 Gradient field nonlinearities create increasing distortions away from the gradient isocenter of the MRI coils; the effect on the image is akin to radial distortions (e.g. pincushion) encountered in optical imaging. Simultaneous excitation of the whole field of view using 3D MRI reduces the presence of some types of nonlinear gradient aberrations that are secondary to 2D slice-based imaging. To compensate for the continued presence of barrel aberration in 3D MRI, calibration and mathematical/software techniques are used in the acquisition process. Effects of gradient nonlinearity can also be reduced by placing the region of interest close to the 3D isocenter of the gradient coils. In regard to magnetic field inhomogeneities, inhomogeneities create geometric distortions as well as artifactual intensity variations in the image. Inhomogeneities originate from the scanner and interactions of the magnetic field with the imaged object. Inhomogeneities are particularly prominent at interfaces with large differences in magnetic susceptibility like air to tissue or paramagnetic substances such as iron in tissue. General scanner inhomogeneities are addressed using shimming, resistive coils that generate compensatory fields to counter magnetic field inhomogeneities that are automatically performed in modern scanners. While there are additional advanced techniques to address magnetic field inhomogeneities outside the scope of this current work, the general idea is that areas with large differences in magnetic susceptibility, such as the anterior eye, are prone to inaccuracies. With respect to the current work, the location of the eye near the z-axis center of the 3D MRI volume and analysis of only the posterior eye surrounded by tissue are advantageous. In the xz plane, the posterior eye is peripheral to the center of the slice, and uncompensated gradient nonlinearities could affect the accuracy of the spatial measurements of our standard. In the stereotactic surgery community, computed tomography (CT) is generally regarded as the more spatially accurate modality.⁴⁵ Studies comparing eye shape with MRI and CT remain to be done.

Other methods besides MRI and OCT to measure posterior eye shape have been described. These include ultrasound, peripheral refraction, and partial coherence interferometry (PCI).^26,46–49 Despite its long history in eye care, there are few studies using ultrasound for measuring posterior eye shape (excepting 1-dimensional length measurements) and no comparisons of this type of measurement to other imaging modalities like MRI. The peripheral refraction method and PCI require dedicated hardware customizations; they also have limited sampling as measurements have to be specifically made at defined points to recreate the posterior eye surface. Aside from those caveats, those methods are acceptable ways to determine posterior eye shape. Comparatively, the OCT data used in this study were from an unmodified commercial system, and the data representing the surface were acquired in a single, seconds-long scan. Further, in addition to prospective acquisitions, OCT datasets acquired in the past under standard clinical conditions, as used in this study, can be analyzed to recover shape measures with the earlier considerations.

In conclusion, we have demonstrated that with careful calibration of the OCT scanner enabling correction of optical and display artifacts, OCT measures of posterior eye shape by spherical and aspherical descriptors are fairly accurate when compared with MRI measures of the same eyes across a population. On an individual level, however, there is not perfect agreement between these modalities for aspherical classification. This is one of the first comparisons of OCT to other medical imaging modalities and may allow posterior eye shape determinations to be more readily available for both researchers and clinicians. This use of OCT imaging to study posterior eye shape can potentially lead to better assessment and understanding of myopia.