Constraint, natural selection, and the evolution of human body form

Significance

Human morphological variation is thought to have been partially shaped by natural selection associated with environmental factors like climate. Patterns of variation in body form correspond with latitude, but evolutionary processes that yielded this variation are not yet established. Examining the traits used in these studies (e.g., limb lengths) independently ignores their genetic covariation, which affects their responses to evolutionary forces. To address this relationship, we estimated the directional selection necessary to evolve correlated traits reflecting body shape across latitudes and examined trait-specific responses. Although most traits appear to be under directional selection, their response is constrained by between-trait covariance. This finding suggests that trait differences among human groups may not directly reflect the forces of selection that shaped them.

Abstract

Variation in body form among human groups is structured by a blend of natural selection driven by local climatic conditions and random genetic drift. However, attempts to test ecogeographic hypotheses have not distinguished between adaptive traits (i.e., those that evolved as a result of selection) and those that evolved as a correlated response to selection on other traits (i.e., nonadaptive traits), complicating our understanding of the relationship between climate and morphological distinctions among populations. Here, we use evolutionary quantitative methods to test if traits previously identified as supporting ecogeographic hypotheses were actually adaptive by estimating the force of selection on individual traits needed to drive among-group differentiation. Our results show that not all associations between trait means and latitude were caused by selection acting directly on each individual trait. Although radial and tibial length and biiliac and femoral head breadth show signs of responses to directional selection matching ecogeographic hypotheses, the femur was subject to little or no directional selection despite having shorter values by latitude. Additionally, in contradiction to ecogeographic hypotheses, the humerus was under directional selection for longer values by latitude. Responses to directional selection in the tibia and radius induced a nonadaptive correlated response in the humerus that overwhelmed its own trait-specific response to selection. This result emphasizes that mean differences between groups are not good indicators of which traits are adaptations in the absence of information about covariation among characteristics.

Among-group variation in human body form is the result of genetic drift, gene flow, and natural selection (1–4). Patterns of variation in human body shape appear to match ecogeographic expectations set out by Bergmann (5) and Allen (6). Comparisons of average body form among groups of humans living at different latitudes reflect these rules. Distal limb segments decrease relative to proximal limb segments, body mass increases, and the pelvis widens as latitude increases (7–16). The prevailing explanation for these patterns is that among-group variation in body proportions was shaped by natural selection acting on thermoregulatory efficacy (8, 9, 11).

Two problems with this interpretation require further investigation. First, there is considerable overlap between the distribution of climate across latitude and the migratory routes of recent range expansions of humans from Africa into other parts of the world (2, 17–19). Thus, patterns of body form variation among groups could reflect random genetic drift and gene flow and mimic the hypothesized effects of climate. Correspondingly, among-group differences in traits strongly associated with Bergmann’s and Allen’s rules—distal limb segment lengths and body breadth—best fit models that include both latitude and random genetic drift (4). In contrast, the among-group variation in proximal limb segment lengths and femoral head diameter (a proxy for body mass) was described best by population structure alone (4). A second, previously unexplored issue is that all studies of ecogeographic variation in body form to date have worked under the assumption that morphological differences among populations are the result of natural selection, rather than having arisen due to a correlated response to selection on other traits.

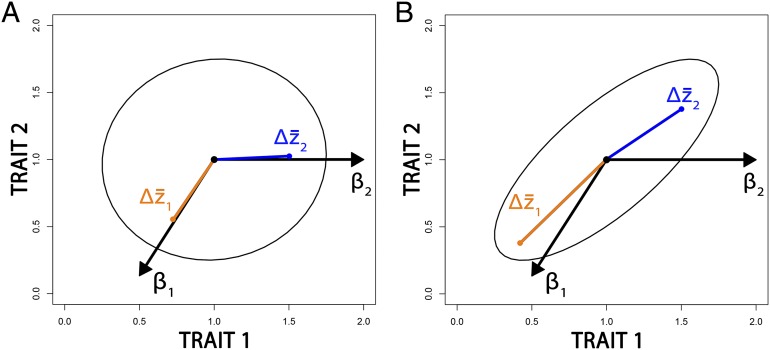
The distinction between the action of selection on a trait (a trait’s influence on fitness) and the evolutionary response of a trait to natural selection is a cornerstone of modern evolutionary theory (20). When natural selection is acting on a set of genetically covarying characteristics, individual responses to selection are a function of the relationship between that characteristic and fitness independent of all other traits, as well as the correlated responses imparted by directional selection acting on genetically covarying traits. Fig. 1 (adapted with permission from ref. 21) shows this contrast graphically: if traits are uncorrelated, then they will respond to selection in the same direction as the force of selection irrespective of what direction in the morphospace selection is acting (Fig. 1A). However, genetic correlations among traits may cause the response to selection not to match the direction of selection (β₂ and $\mathrm{\Delta }{\overline{z}}_2$; Fig. 1B), unless the response to selection closely parallels the main axis of variation (β₁ and $\mathrm{\Delta }{\overline{z}}_1$; Fig. 1B). Distinguishing between the action of selection (the estimated selective force) and the response to selection (the evolutionary change) is important because when there are strong genetic correlations among traits, as there are in those reflecting body form (3, 4), the magnitude of realized differences among groups may not reflect the strength and direction of natural selection. Adaptation will not be apparent from differences in means between groups. Similar studies have demonstrated that this is the case in cranial (22, 23), pelvic (24–26), and hand and foot bones (27).

Fig. 1.

The differences between the force of directional selection (β) and responses to selection $\left(\mathrm{\Delta }\overline{z}\right)$ for two traits that are independent (A) or that strongly covary (B). In both examples, we subject the trait means to two selection gradients (β₁ and β₂). The traits are uncorrelated in A, so responses to selection $\left(\mathrm{\Delta }{\overline{z}}_1,\mathrm{\Delta }{\overline{z}}_2\right)$ will closely match the vectors of the selection gradients (β₁ and β₂). Studies that use the mean differences in traits between groups to indicate how those traits responded to selection implicitly assume this framework for selection. However, in B, the strong covariance between traits affects the ability of the traits to respond to certain selection gradients. A selection gradient (β₁) similar to the major axis of variation will result in responses to selection that closely parallel that axis and yield a larger response than in uncorrelated traits. In contrast, a selection gradient that greatly departs from the major axis (β₂), and which is positive for trait 1 but zero for trait 2, will nonetheless result in positive responses to selection $\left(\mathrm{\Delta }{\overline{z}}_2\right)$ for both traits because of their covariance. Adapted with permission from ref. 21; see box 1 and figure 1 in ref. 21 for a more comprehensive exploration of the effect of trait covariance on responses to selection.

The standard ecogeographic model assumes the mean body form of groups migrating away from the tropics evolved from a tropically adapted ancestral condition (10–16). We take the average morphology of several groups from sub-Saharan Africa to represent the morphological range of variation for putatively tropically adapted groups. We then used groups from regions of higher latitude (including North Africa, temperate Europe, and the circumpolar Arctic) to represent the range of variation in body form that resulted, in part, from climate mediated natural selection. Table 1 presents the sample sizes and measurement means for the groups used in this study. A map of the group locations can be found in SI Appendix, Fig. S1.

Table 1.

Measurement means of study groups (males)

Geographic region	Group	N	HML	RML	FML	TML	FHD	BIB
Sub-Saharan Africa	Uganda	12	333.6	265.6	474.0	407.7	42.5	241.2
	San	15	292.5	228.1	424.9	356.6	39.7	214.8
	West Africa	16	317.5	258.7	456.2	388.3	43.4	233.7
	Kikuyu	15	327.5	258.8	458.5	391.2	43.9	241.8
Northern Africa	Egypt	83	315.1	247.3	447.1	376.6	44.4	257.4
	Nubia	37	313.4	245.5	438.5	375.6	44.2	255.8
Temperate Europe	Ireland	20	329.3	243.3	457.8	368.0	47.6	271.7
	France	25	315.9	238.8	444.5	368.7	46.1	272.7
	Austria	48	317.1	244.4	442.9	367.2	45.8	272.8
	Bosnia	48	328.6	247.3	463.8	385.5	49.0	278.6
Circumpolar Arctic	NeoAleut	37	302.1	230.2	415.5	336.1	45.5	263.0
	Ikogmiut	29	313.1	233.8	425.2	348.4	45.7	264.8
	Kuskowagamiut	14	307.9	230.1	423.0	333.2	45.9	265.2
	Point Hope-Tigara	22	302.0	226.2	432.1	355.8	47.1	280.1

All dimensions are in millimeters. BIB, biiliac breadth; FHD, femoral head diameter; FML, femur maximum length; HML, humerus maximum length; RML, radius maximum length; TML, tibia maximum length.

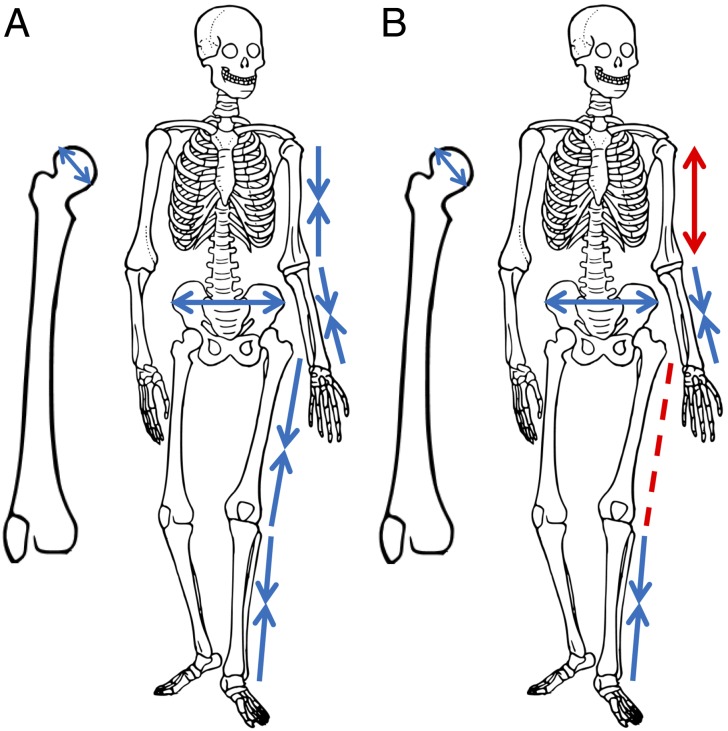
To address these problems, we asked two questions. (i) Which characteristics of human body form (long bone lengths, femoral head breadth, and body breadth) were directly affected by directional selection? (ii) Which traits evolved by correlated responses to directional selection acting on other traits? The traits we examine are all staples of the ecogeographic literature (11, 16, 28): humeral, radial, femoral, and tibial maximum lengths; femoral head diameter; and biiliac breadth. Fig. 2A presents the anticipated direction of natural selection on these traits when evolving from lower to higher latitudes based on ecogeographic expectations. We use a model (27, 29–34) of retrospectively estimated selection gradients to identify those traits that were under directional selection for morphological evolution between different climate regions (e.g., the tropics and the arctic). These selection gradients are partial regression coefficients of relative fitness onto a characteristic holding the effects of other characteristics constant (29, 30). They describe the direction and magnitude of selective pressures on individual traits. Positive selection gradients between groups at different latitudes indicate a net positive relationship with fitness (for long bones to lengthen or biiliac breadth and femoral head diameter to broaden). Alternatively, negative selection gradients between groups at different latitudes indicate a net negative relationship with fitness. We also decompose separate components of the net response to selection into the response of each trait arising from selection acting directly on a trait and the response driven by selection acting on correlated traits (21–27). To reemphasize, it is important to draw a distinction between the force of selection on a trait and the response to selection of that trait. If correlated responses to selection on other traits are larger than the independent response to selection on a characteristic, the sign of the response could be considerably different from the force of selection on that trait (Fig. 1).

Fig. 2.

Directional selection pressures predicted by classic ecogeographic expectations (A) and the consensus pattern of directional selection from our analysis (B) for the six traits investigated in this study. Arrows indicate the direction of selection hypothesized (A) or estimated (B) to have led to trait differences between human groups in sub-Saharan Africa and higher latitudes. Inward-facing arrows indicate negative directional selection (the trait is predicted to shorten/narrow), whereas outward-facing arrows indicate positive directional selection (the trait is predicted to lengthen/widen). The dashed line indicates that estimates of directional selection were not distinct from zero in our consensus pattern. Red lines in B indicate differences between the classic ecogeographic expectations and the consensus pattern of directional selection from our analysis.

Results

Table 1 presents group means and SI Appendix, Figs. S2–S5 present group means and SDs of each of the six traits. The means among these regional groupings follow the general ecogeographic pattern of shorter distal limb segments relative to proximal limb segments, wider biiliac breadths, and wider femoral head diameters at increasing latitudes.

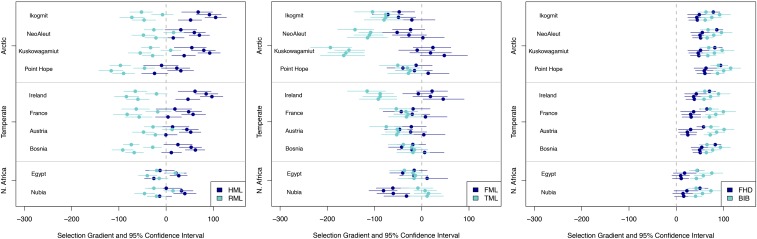
Fig. 3 presents estimated selection gradients for each pairwise comparison between groups and their 95% CIs generated using a nonparametric bootstrap (35). These selection gradients represent the independent action of directional selection on each trait. In these figures, each circle-and-whisker set represents the mean and 95% CI of a pairwise selection gradient estimation between one of four sub-Saharan groups and a group from the given region (Table 1). We interpret selection gradients whose 95% CIs do not encompass zero to show evidence of directional selection on a trait. SI Appendix, Figs. S6–S14 present larger versions of Fig. 3.

Fig. 3.

Selection gradients (dots) and 95% CIs (whiskers) for pairwise comparisons of traits between sub-Saharan African groups and higher latitude groups. See legends in each figure for traits (Table 1). CIs that overlap with zero indicate a selection gradient not discernable from zero (i.e., no directional selection is estimated). See SI Appendix, Figs. S2–S5 for more detailed versions of these figures.

Humeral length tends to demonstrate positive selection gradients and radial length negative selection gradients in comparisons between the sub-Saharan groups and those of the temperate and circumpolar regions (Fig. 3, Left). The majority of CIs for femoral length overlap with zero regardless of interregional comparison, indicating little or no detectable directional selection (Fig. 3, Center). Tibial length displays negative selection gradients in among-group comparisons between the sub-Saharan groups and those of both the temperate and arctic regions (Fig. 3, Center). Femoral head diameter and biiliac breadth generally show positive selection gradients across pairs of groups in different climate areas (Fig. 3, Right). Only the CIs for femoral head gradients estimated for groups in North Africa overlap with zero. The general trends are presented graphically in Fig. 2B, which compares the consensus pattern of directional selection from our analysis against the ecogeographic predictions shown in Fig. 2A.

Although the majority (27 of 40) of between-group comparisons show no directional selection in the femur, when directional selection for femoral length is estimated between groups, it is negative in all but one instance (12 of 13 gradients; Fig. 3). As noted above, more groups exhibit directional selection in humeral length, radial length, and tibial length from temperate to arctic ecogeographic regions. For example, four of eight comparisons between groups in sub-Saharan Africa and those in North Africa show directional selection in the humerus, whereas 10 of 16 comparisons between sub-Saharan and temperate groups and 12 of 16 sub-Saharan and arctic groups demonstrate directional selection in humeral lengths. This trend is not present in the two other dimensions (femoral head diameter and biiliac breadth).

Humeral length is shorter, on average, in groups from temperate and arctic regions than those in sub-Saharan Africa, but the selection gradients for humeral length are positive. Positive directional selection is opposite to ecogeographic expectations (Table 1) (11, 36). These results are illustrated in Table 2, which present examples of pairwise comparisons between groups from sub-Saharan Africa and groups from each of the other regions. All pairwise comparisons between groups are reported in SI Appendix, Tables S1a–S1nn. The direction of net responses to selection generally match the direction of differences in trait means between pairs of groups. Similar opposing gradients and responses in the femur are associated with selection gradients not statistically different from zero, so we do not offer an interpretation of them (Fig. 3). An examination of the trait-specific contribution to the net response to selection in the humerus (Table 2: ∆z_HML) shows that were humerus length independent of the other five traits, it would respond to selection by lengthening among groups from higher latitudes. However, this is clearly not the case.

Table 2.

Examples of directional selection estimated between sub-Saharan African and non–sub-Saharan African groups: differences in trait means between groups, estimated selection gradients (β), net response to selection (∆ $\overline{z}$), and correlated responses to selection on traits that combine to yield the net response (∆z_element)

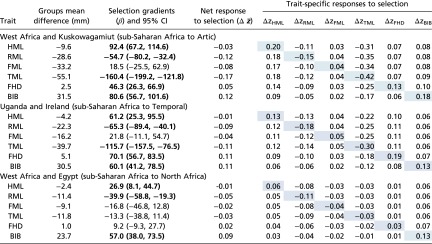Within the trait-specific responses to selection matrix, direct responses to selection are shaded, and indirect (correlated) responses are not. Bold text indicates estimated selection gradients with 95% CIs that do not overlap with zero. All pairwise comparisons between sub-Saharan African and non–sub-Saharan African groups are presented in SI Appendix, Tables S1a–S1nn.

The response to selection in the humerus is greatly attenuated by the correlated response to selection between the tibia and the radius with the humerus, respectively. The shared direction of the selection gradients, net response to selection, and direct response to selection for both the radius and tibia indicate a relationship between fitness and shorter distal limb segment lengths as latitude increases.

Discussion

We show strong evidence for directional selection on many of the traits, which are hypothesized to vary among humans as a result of climatic adaptation under the ecogeographic model. However, the magnitude and orientation of directional selection across the traits often does not match the pattern and magnitude of morphological change. Changes in trait means among regions (Table 1) do not mean selection was acting directly on those traits, or that the force of selection was the same as the direction of difference in means. This difference is especially apparent in the limb segment lengths, where genetic covariation among elements, especially with the tibia, diminishes the response to directional selection acting directly on those traits. Observed mean trait differences between groups may not be good indicators of what aspects of morphology most closely and directly covaried with fitness during the evolution of group differences (23, 24, 27, 29, 37–39). The evolution of morphology is a whole-organism problem (40).

The analysis of covariance among traits is the best predictor for how genetically complex traits have evolved. Limbs have a complex polygenic basis. They are found to be highly genetically correlated and exhibit strong pleiotropy when studied in a quantitative genetic framework (21, 41–43). When sample sizes are sufficient to render precise estimates of genetic correlations for morphological characters, the genetic and phenotypic correlations are nearly identical, implying a high degree of proportionality between genetic and phenotypic covariances (44, 45). Any pattern of covariation among traits can be caused by many different combinations of genetic variants acting through a variety of developmental processes (46, 47). Over short evolutionary time scales, such as those over which recent human diversity evolved, an estimate of covariation gives us our best estimate of the tendency of traits to evolve together in response to processes of evolution and do not require a deep understanding of the developmental processes underlying limb development. Our exclusion of considering these processes in this discussion is not to downplay the important ways in which development and evolutionary forces conspire to change the presentation of covariation in populations over longer evolutionary time scales (48).

Which Characteristics Were Directly Affected by Directional Selection to Produce Differences Among Groups?

Previous ecogeographic studies presumed that differences in trait means between groups reflected the direction of selection gradients. Both femoral head diameter and biiliac breadth are consistent with this assumption, having positive selection gradients and positive net responses to selection (4, 11, 16). Ruff (11) argued that pelvic breadth serves as a proxy dimension for surface area-to-volume ratios and therefore is evolving in response to thermoregulatory requirements. Ecogeographic models also argue that larger body masses at increasing latitude are more fit (11), a trend our results support if femoral head size is a good proxy for body mass (49, 50). We cannot test the mechanistic aspects of these models in our study, although our results support the argument that pelvic breadth and femoral head diameter were under and responded to natural selection, even in comparisons between groups from sub-Saharan Africa and North Africa.

In combination with the results of earlier studies, our findings generally show that three of the four limb segment lengths vary among humans in part because of directional natural selection (4, 11–13). The differences between group means, however, are not the same as what we would expect based on the selection gradients we calculate, especially in the humerus. These results show that, although many of the traits we observe evolved by directional selection, the differences in trait means among groups are not proportional to the force of directional selection on each trait independently. Were it not for the covariance among traits, we might expect greater differences and different patterns of evolution in traits.

Which Traits Evolved by Correlated Responses to Directional Selection Acting on Other Traits?

Correlated responses to directional selection arising from covariance between the tibia and other limb elements dramatically affected responses of limb elements to directional selection. This relationship is particularly notable in the humerus. As shown in Table 2, directional selection on the tibia and radius, in combination with weak selection on the femur, cause a strong correlated negative response on the humerus. This combination overwhelms the response generated by the positive net relationship between fitness and humeral length. Specifically, selection on the distal traits counteracts the response of humeral length to the directional selection that is acting on it. The action and response to selection for the distal elements correspond with expectations from the ecogeographic literature (Fig. 2).

Our findings highlight the ways in which evolutionary trajectories favored by natural selection can be constrained by covariation, even when all directions in a morphospace have some variation (51, 52). Covariances among aspects of body form we include in this study do not covary so tightly that there are directions in their morphospace with no variation (i.e., our covariance matrix is positive definite); thus, we are not presented with a hard variational constraint. Because the prevailing action of directional selection on the humerus (to lengthen) and the radius and tibia (to shorten) have opposite signs, the net response to selection for individual traits are constrained by the action of selection on other traits. Distal limb elements did not evolve as quickly as they might were they independent and the humerus evolved in a direction opposite to its contribution to increasing fitness.

An important point to recognize here is that we do not exhaustively cover all dimensions of body form, some of which might be more directly tied to fitness differences than the ones we do consider. It is entirely possible that the strong selection gradients that we estimate from comparisons of groups in sub-Saharan Africa and those in the arctic are driven by selection on some unmeasured aspect of body form (or physiology) genetically correlated with both humeral length and overall form. Although this is certainly the case, our analyses cannot account for characteristics we did not measure. Nonetheless, our analyses considerably narrow the space of possible models that could be used (e.g., models that go beyond mean trait comparison) and gives a guide as to which traits we might consider in the future (30). Studies of humerus-to-body size allometry suggests a possible direction in which this study might be taken (53).

Our study’s results concerning directional selection for the humerus, femoral head, and femur differ in some respects from those reported by Roseman and Auerbach (4). They showed equivalent performance for models including population structure on one hand, and both population structure and latitude on the other, as the explanation for among-group variance in the humerus. Their study (4) used the global sample of 121 groups, whereas we selected 14 groups from that sample for our study. The consistency of results among the many pairwise comparisons we made between sub-Saharan groups and those from other latitudes (SI Appendix, Tables S1a–S1nn) argues against sampling bias. We attribute much of the discrepancy in results reported in this study with those in Roseman and Auerbach (4) to differences between the univariate approach used by the latter, where each trait was examined in isolation, and the multivariate approach in this study. As we show, the covariation of traits within an evolutionary framework affects the capacity of those traits to respond to directional selection. A multivariate approach avails us of the ability to apprehend otherwise cryptic evolutionary processes.

Conclusions

Directional selection has contributed to the differentiation of the tibia, radius, femoral head, and biiliac breadth among human groups from different ecogeographic contexts. In some respects, these results accord well with previous ecogeographic studies. More importantly, however, we show that differences in trait averages among groups are not good indicators of how traits responded to natural selection. Notably, because selection acted on correlated traits, the humerus shows an evolutionary response to directional selection opposite to its influence on fitness. Patterns of mean difference among groups are not necessarily an accurate reflection of the processes that created that variation.

The ecogeographic literature is silent when it comes to the fitness effects of humeral length, which are only apparent after taking into account covariance among traits. By focusing only on realized differences between groups, traditional adaptationist ways of studying ecogeographic variation have left the ways in which natural selection acted undescribed. Natural selection’s effect on the humerus would not be apparent from a standard adaptationist perspective focused on explaining evolution using group differences on a trait-by-trait basis.

Materials and Methods

We used a global distribution of phenotypic data provided by the Goldman Data Set (50), Americas Data Set (16), and the generosity of Trent Holliday, Department of Anthropology, Tulane University, New Orleans, and Chris Ruff, Center for Functional Anatomy & Evolution, Johns Hopkins University School of Medicine, Baltimore. When compiled, 2,187 individuals from 121 groups were used. These data are the dataset compiled and analyzed by Roseman and Auerbach (4), and more information about them can be found in that publication. These data represent a wide latitudinal dispersion of human populations (SI Appendix, Fig. S1). The groups we chose have a representative range of the means for the six traits under investigation for each latitudinal group, based on the larger sampling of human groups in the datasets from which they were drawn. It is for this reason the San—who are not equatorial but are sub-Saharan—were included in the study; they have the smallest body size and limb dimensions of all of the sub-Saharan groups used by Roseman and Auerbach except the Biaka Pygmy (4), which we excluded due to their very small sample size. We use the maximum lengths of the humerus (HML), radius (RML), femur (FML), and tibia (TML), as well as femoral head diameter (FHD), and biiliac breadth (BIB) to quantify body and limb form (all in millimeters). In an effort to maximize global representation and avoid issues of standardizing for sexual dimorphism, only males were used in this analysis (4).

We used Lande’s equation for multivariate response to selection (29, 54)

$$\mathrm{\Delta }\overline{z}=G\beta ,$$ [1]

where $\mathrm{\Delta }\overline{z}$ represents the change in mean phenotype values, G is the additive genetic variance-covariance matrix, and β is a vector of selection gradients, partial regression coefficients of relative fitness onto the traits. We conducted our analyses on the mean standardized scale (40). Because the phenotypic variance-covariance matrix (P-matrix) is proportional to the genetic variance-covariance matrix (G-matrix) in morphological characteristics (22, 23, 44, 45), we follow standard practice and substitute the mean standardized within-group variance-covariance P-matrix for the G-matrix. This within-group P-matrix was calculated using the full sample of 121 groups in Roseman and Auerbach (4).

For the pairwise comparison of any two groups, the mean standardized difference between groups $\left(\mathrm{\Delta }\overline{z}\right)$ was determined using the equation

$$\mathrm{\Delta }\overline{z}=\frac{{\overline{x}}_1-{\overline{x}}_2}{{\overline{x}}_1},$$ [2]

where ${\overline{x}}_1$ always refers to one of the four sub-Saharan African groups and ${\overline{x}}_2$ to one of the North African, temperate European, or circumpolar arctic groups. This difference in means $\left(\mathrm{\Delta }\overline{z}\right)$ was then multiplied against the inverse of the mean standardized within-group variance-covariance matrix of phenotypes (P) to determine the selection gradients.

The change in mean phenotype values between groups $\left(\mathrm{\Delta }\overline{z}\right)$ and mean standardized global variance-covariance matrix (P) were multiplied to estimate the selection gradients (β) (39)

$$\beta =P^{-1}\mathrm{\Delta }\overline{z}.$$ [3]

To examine relationships across climate regions, we conducted 40 pairwise comparisons between 14 globally distributed groups. We used the six traits above to estimate the directional selection that would have been necessary to evolve the group differences found between four sub-Saharan African groups (Uganda, Kikuyu, a combination of “Western African” groups, and the San) and groups from North Africa (Egypt, Nubia), temperate Europe (Ireland, France, Austria, Bosnia), and the circumpolar arctic (Neo-Aleut, Ikogmiut, Kuskowagamiut, Point Hope Tigara). Groups are listed in Table 1, and SI Appendix, Fig. S1 is a map of their regional distribution.

CIs were calculated using a bootstrap approach that resampled the two groups 1,000 times with replacement (as per their group-specific sample size) and determined the vectors of means for each group from the resampled iterations. These resampled means were then used to calculate the selection gradients that would have allowed the evolution of the observed differences between group 1 and group 2 (Eq. 3). A 95% CI was determined from these bootstrapped vectors of selection gradients.

Trait-specific responses to selection (∆z_element) sum to provide the net response to selection for a given characteristic $\left(\mathrm{\Delta }\overline{z}\right)$ (28, 29). To further examine the relationship between changes in phenotype means between groups (P) and the net response to selection $\left(\mathrm{\Delta }\overline{z}\right)$, we therefore divided each trait’s net response to selection into its component trait-specific responses to selection (∆z_element) (Table 2). These trait-specific responses to selection (∆z_element) consist of direct responses to selection (shaded values), which are calculated by multiplying the trait’s selection gradient (β) against the pooled, mean standardized variance, and indirect responses to selection (unshaded values), which are calculated by multiplying the trait’s selection gradient (β) against the pooled, mean standardized covariance with each other trait independently (28, 29). These indirect responses to selection, therefore, provide information about how the genetic correlation between traits influences each traits’ net response to selection $\left(\mathrm{\Delta }\overline{z}\right)$.

All analyses were performed in the R statistical computing language (https://cran.r-project.org). Data and analysis code are available from the authors.