Abstract
Purpose
To compare measurements of the full-field photopic negative response (PhNR), as well as intra-subject variation in the PhNR, using time and time-frequency domain analyses.
Methods
Full-field ERGs were recorded from 20 normally-sighted subjects (ages 24 to 65 years) elicited by a long-wavelength pulse (3 cd s m−2) presented against a short wavelength adapting field (12.5 cd m−2). Three to 10 waveforms were obtained from each subject and each waveform was analyzed using standard time domain analyses of the PhNR, as well as a discrete wavelet transform (DWT) to extract time-frequency components that correspond to the PhNR. Three different measures of the PhNR were derived and compared: 1) amplitude at the PhNR trough; 2) amplitude at 72 ms following stimulus onset; 3) energy in the 11 Hz, 60 to 120 ms DWT frequency bin that corresponds to the PhNR. In addition, the effect of normalizing the PhNR by the b-wave was evaluated for each of the measures. Coefficients of variation (CVs) were computed for each definition to evaluate intra-subject variation.
Results
PhNR amplitudes measured at the trough and at 72 ms were significantly correlated (r = 0.88, p < 0.001). Additionally, PhNR energy derived by DWT was significantly correlated with the amplitude measured at the trough (r = 0.64, p = 0.002) and at 72 ms (r = 0.60, p = 0.005). Mean (± SD) intra-subject CVs were 26% (15%), 49% (26%), and 30% (15%), for measures at the trough, 72 ms, and DWT, respectively. Normalization by the b-wave amplitude (i.e. PhNR/b) had minimal effect on the intra-subject CVs, whereas normalization by the sum of the b-wave and PhNR amplitudes (i.e. PhNR/[b+PhNR]) substantially reduced the CVs for all three measures (mean CVs were less than 17% for all conditions).
Conclusions
Although each PhNR definition has advantages and disadvantages, all three metrics provide similar estimates of the PhNR. Intra-subject CVs, however, were relatively high for measurements made at 72 ms, indicating that definitions based on a fixed time-point may introduce variability. The substantial decrease in intra-subject variation after normalization by the sum of the PhNR and b-wave amplitudes may be advantageous under some conditions.
Keywords: electroretinogram (ERG), photopic negative response, discrete wavelet analysis
Introduction
The photopic negative response (PhNR) is a slow, negative component of the photopic full-field electroretinogram (ERG) that follows the b-wave. The PhNR is thought to arise primarily from the spiking activity of retinal ganglion cells [1–3]. Evidence for a retinal ganglion cell source comes from animal studies showing that the PhNR is reduced or absent in macaques following intravitreal injection of tetrodotoxin as well as in experimentally-induced glaucoma [2,4]. The inner-retina source of the PhNR has made it a useful tool for assessing dysfunction in conditions including glaucoma [3,5–8], optic atrophy [1,9,10], idiopathic intracranial hypertension [11], and optic glioma [12].
Although nearly all studies elicit the PhNR using a long-wavelength flash presented against a short-wavelength adapting field, two different methods are commonly used to define the PhNR amplitude. Specifically, the PhNR has been defined as the minimum amplitude (trough) following the b-wave [2,3,5,10,11] and as the amplitude at a fixed time-point following the b-wave [3,7,13]. An advantage of measuring the PhNR at the trough is that a measure of implicit time can also be obtained. However, because the PhNR is a slow waveform component, it is not always possible to define a single trough, particularly for patients who have small amplitude responses. Measuring the PhNR at a fixed time-point solves this problem, but has a disadvantage in that a somewhat arbitrary time-point must be defined. Measurements of the PhNR made at the trough and at a fixed time-point are, presumably, correlated, but their relationship has not been investigated systematically.
As an additional approach to quantify the PhNR, several previous studies have normalized the PhNR amplitude by the b-wave amplitude in an effort to minimize inter-subject variability [3,6,7,13,14]. That is, confounding factors such as the axial length of the eye and electrode placement that affect the PhNR amplitude will also likely affect the b-wave amplitude similarly. If the PhNR and b-wave amplitudes are correlated, normalization helps to reduce the effects of these factors. Although some studies have reported a correlation between the PhNR and b-wave amplitudes [13], others have not [3]. Mortlock et al. [13] compared several possible definitions of the PhNR (e.g. measured at the trough, at a fixed time-point, normalized to the b-wave, and non-normalized) and showed that the smallest inter-subject differences in amplitude were obtained when the PhNR was defined at the trough and normalized by the b-wave amplitude. However, previous studies have not addressed the extent to which normalization reduces intra-subject variation (i.e. differences among responses for a given subject within a test session), but this is of potential importance because multiple responses are typically obtained from a subject and averaged prior to analysis.
Although previous studies of the PhNR have analyzed the response in the time domain, analysis of the PhNR in the time-frequency domain is possible and may be advantageous. For example, the discrete wavelet transform (DWT) is a wavelet-based approach to analyze non-stationary signals that has recently been used to extract the a- and b-wave components of the single flash ERG [15,16]. The DWT permits localizing the energy content of the ERG in both time and frequency, and it has been suggested that this approach may be able to identify subtle diagnostic features of the ERG that are less apparent in time domain measures [16]. The DWT may be particularly useful for analysis of the PhNR because it is objective, not based on a single time-point, and is capable of defining slow, small amplitude responses. To date, the DWT has not been used to analyze the PhNR.
The purpose of the present study is to compare measurements of the PhNR using time and time-frequency domain analyses. We sought to determine how the different commonly used measures of the PhNR (at the trough and at a fixed time) compare to the PhNR derived by the DWT. We also evaluated variation in the PhNR within subjects and between subjects by computing coefficients of variation. The results of this study may be of use in guiding the measurement and definition of the PhNR in future studies.
Methods
Subjects
Twenty visually-normal subjects (14 male, 6 female) ages 24 to 65 years participated. The subjects had no history of eye disease and ETDRS best-corrected visual acuity of 0 log MAR or better (equivalent to 20/20 or better Snellen acuity). Informed consent was obtained from all subjects before their participation. Procedures adhered to the tenets of the Declaration of Helsinki, and the protocol was approved by an Institutional Review Board at the University of Illinois at Chicago.
Apparatus, stimuli, and ERG recording procedure
Full-field stimuli were generated by a Diagnosys Espion E3 system and presented in a ColorDome desktop ganzfeld (Diagnosys LLC, Lowell, MA). The stimulus consisted of a long-wavelength LED-generated flash (dominant wavelength of 642 nm; 3 phot. cd s m−2) presented against a short-wavelength adapting field (dominant wavelength of 465 nm; 12.5 cd m−2). This combination of a long-wavelength pulse on a short wavelength background has been shown to elicit large PhNRs in visually-normal subjects [4]. Stimulus wavelength and luminance were measured using a Spectrascan 740 spectroradiometer (Photo Research, Chatsworth, CA).
The procedure and recording conditions used to obtain the PhNR measurements are described elsewhere [11]. In brief, the subjects’ pupils were dilated using 1% tropicamide and 2.5% phenylephrine hydrochloride drops. A fiber DTL-plus recording electrode (Diagnosys LLC, Lowell, MA) was placed above the lower eyelid at the corneal limbus and the signal from this electrode was referenced to the earlobe, with a forehead ground electrode. Signals were acquired at a sampling frequency of 2 kHz then band-pass filtered (0.3 to 300 Hz; two-pole Bessel filter), consistent with ISCEV recommendations. The luminance pulses were presented with an inter-pulse interval of approximately 7 s until a minimum of three ERG responses with minimal eye movement artifacts were recorded (in typical clinical practice, we obtain and average a minimum of three responses).
Data Analysis
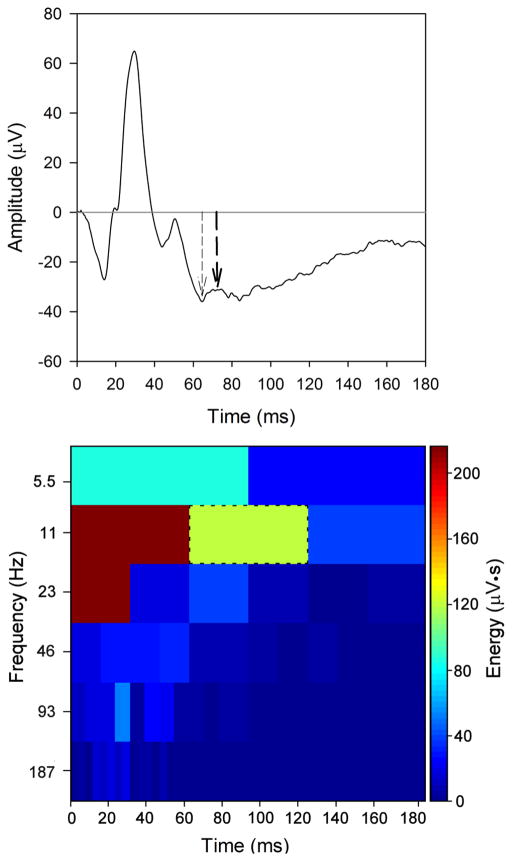
Fig. 1 (top) shows the mean photopic single-flash ERG obtained from the 20 subjects. As illustrated in Fig. 1 (top), the PhNR was defined according to the two typical definitions: at the trough (thin arrow; left) and at 72 ms (thick arrow; right). A fixed time-point of 72 ms was selected based on the results of Viswanathan et al. [3] who showed that the mean PhNR implicit time was approximately 72 ms for subjects in their 40’s (the mean age of our subjects). For the measurements made at the trough, the mean amplitude of 11 consecutive ERG data points (5 ms) centered at the trough of the PhNR was calculated. For the mean waveform, the amplitude of the PhNR measured at the trough (36.0 μV) was similar to that measured at 72 ms (31.4 μV).
Figure 1.
The top panel shows the mean photopic single-flash ERG waveform obtained from the 20 subjects. The PhNR defined at the trough following the b-wave is indicated by the left arrow (thin dashed) and the PhNR defined at 72 ms is indicated by the right arrow (thick dashed). The DWT of the mean waveform is shown in the bottom panel. Energy (given by the scale at right) for each time-frequency bin is shown. The PhNR component corresponds to the 11 Hz, 60 – 120 ms bin (green bin; indicated by the black dashed outline).
The DWT scalogram of the mean response is shown in the bottom panel of Fig. 1. The DWT was computed in MATLAB according to standard techniques that are described in detail elsewhere [16]. In brief, DWTs were obtained using the fast wavelet transform algorithm of Mallat [17]. The db2 wavelet, which was also used in previous work [16], was selected as the mother wavelet; although numerous mother wavelet possibilities are available, preliminary evidence indicated that the db2 resulted in less variation in DWT energy among the subjects, as compared to the Haar and db3 wavelets. Eight levels of decomposition were obtained (i.e. 8 different frequency bands), which spanned the frequency spectrum contained in the single flash ERG. The central frequency of each band is given on the y-axis of Fig. 1 (bottom; note that only the lowest 6 frequency bands are displayed for clarity because the energy above 200 Hz is negligible). In this figure, the energy (μV•s) contained in the response is shown for each time-frequency bin. Previous work has shown that the dominant energy of the a- and b-waves in the DWT occurs at relatively short durations (less than approximately 60 ms) and moderate temporal frequencies (greater than 20 Hz) [16]. Although no previous attempt has been made to define the PhNR component of the DWT, our data indicate that the dominant energy is localized to relatively late durations (60 to 120 ms) and low temporal frequencies (near approximately 11 Hz), as expected. The assignment of the PhNR to the 11 Hz, 60 – 120 ms bin was determined by: 1) setting the energy of this bin to zero, which resulted in the attenuation of the PhNR in the time domain when the inverse DWT was computed; 2) setting the amplitude of the waveform in the time domain to 0 μV after 60 ms, which resulted in low energy in the 11 Hz, 60 – 120 ms time-frequency bin of the DWT (see supplementary data Figure 1). Although there is energy at lower frequencies (e.g. 5.5 Hz) that may correspond to the PhNR, preliminary evidence indicated that including energy in the 5.5 Hz band had minimal effects. As an additional detail to consider, the DWT is not a shift-invariant transform, in that shifting (translating) the complete ERG waveform in time can affect the energy of the presumed PhNR time-frequency bin. However, in contrast to the results reported for analysis of the a- and b-waves [16], preliminary evidence indicated that the effect of shifting the waveform on the PhNR energy (11 Hz, 60 – 120 ms time-frequency bin) was minimal. As such, only non-shifted data (i.e. initial data point at time 0) are presented.
Results
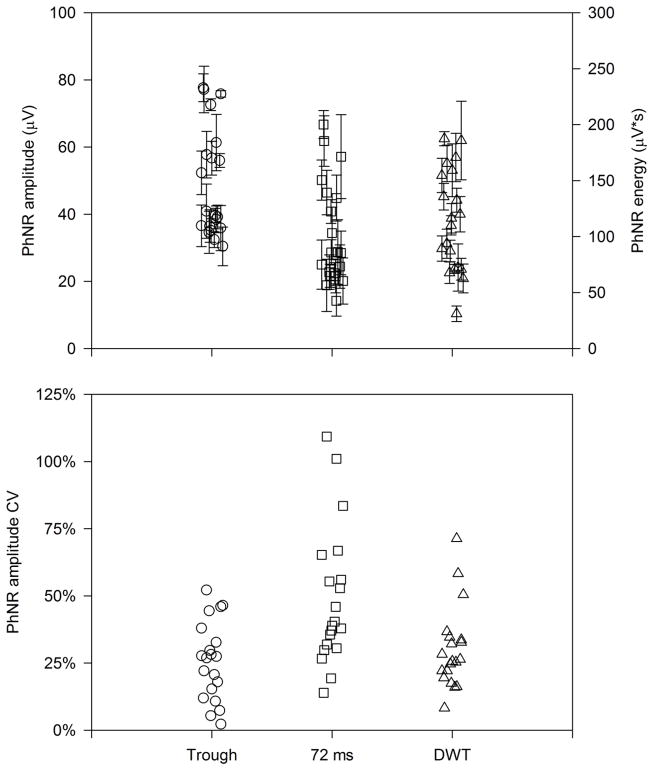
Fig. 2 (top) shows the PhNR amplitude defined at the trough (circles) and at 72 ms (squares). Each data point represents the mean amplitude for a given subject and each error bar represents the standard error of the subject’s mean. Thus, the error bars provide a measure of intra-subject variation and the differences among the data points provide a measure of inter-subject variation. The amplitude for measurements made at the trough is typically greater than those made at 72 ms. This is because, by definition, the trough is the minimum amplitude (largest difference from baseline) following the b-wave. The rightmost data set (triangles) represents the PhNR energy derived by the DWT (i.e. energy in the 11 Hz, 60 – 120 ms bin). Note that these data are plotted according to the right y-axis (μV•s). The PhNR amplitudes measured at the trough and at 72 ms were significantly correlated (r = 0.88, p < 0.001). Additionally, PhNR energy derived by DWT was significantly correlated with the amplitude measured at the trough (r = 0.64, p = 0.002) and at 72 ms (r = 0.60, p = 0.005).
Figure 2.
The top panel shows the PhNR amplitude measured at the trough (left data set; circles), at 72 ms (middle data set; squares), and derived from the DWT (right data set; triangles). Each symbol represents the mean amplitude for a different subject and the error bars represent each subject’s SEM. The bottom panel shows the amplitude CV (standard deviation/mean) for each subject.
Fig. 2 (bottom) shows the CV for each subject (the ratio of mean response amplitude [or energy] to the standard deviation of the response amplitude [or energy]). Since the CV is a ratio, direct comparison among the three measures can be made. For the PhNR amplitude measured at the trough, the intra-subject CV showed the least amount of variation (approximately 26%, on average), whereas the PhNR measured at 72 ms showed higher variability (approximately 49%, on average). The CV obtained from the DWT (approximately 30%, on average) was slightly larger than that obtained from the trough measurements and smaller than that obtained from the measurements at 72 ms. Of note, the relatively high CV for measurements at 72 ms is not due to the use of a single time-point for the calculation; obtaining an average amplitude from 69.5 ms to 74.5 ms (i.e. a 5 ms window, as used in the trough definition) did not meaningfully improve the CV (48%, on average).
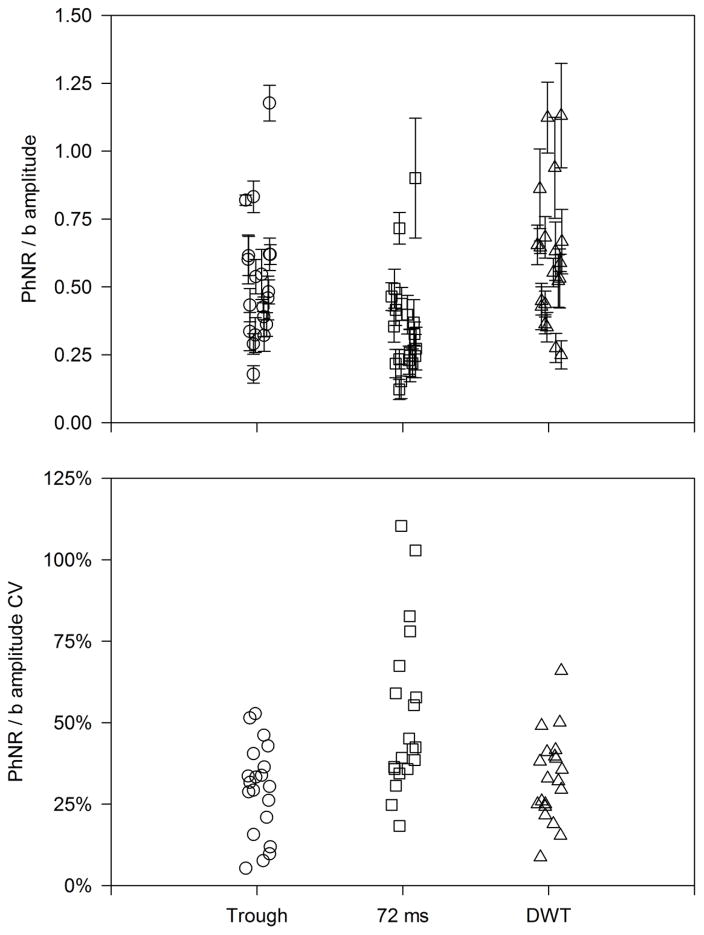
Figure 3 (top) shows the normalized (PhNR/b-wave amplitude) for each subject measured at the trough (circles) and at 72 ms (squares). The triangles represent the DWT energy in the 11 Hz, 60 – 120 ms bin (PhNR component) normalized by the 23 Hz 0 – shown. The PhNR component corresponds to the 11 Hz, 60 measure are approximately similar. Fig. 3 (bottom) shows the CV for the b-wave normalized PhNR. The CV values follow the same pattern as for the non-normalized values (c.f. Fig. 2, bottom). Specifically, the values were approximately similar for measurements made at the trough (29%) and for the DWT (33%), and relatively large for the measurements made at 72 ms (52%). These CV values (29% to 52%) are similar to those obtained for the non-normalized PhNR measurements (26% to 49%), indicating that normalization did not substantially reduce the variance among subjects.
Figure 3.
The top panel shows the PhNR normalized by the b-wave and the bottom panel shows the CV for the b-wave-normalized PhNR amplitude for each subject. Other conventions are as in Fig. 2.
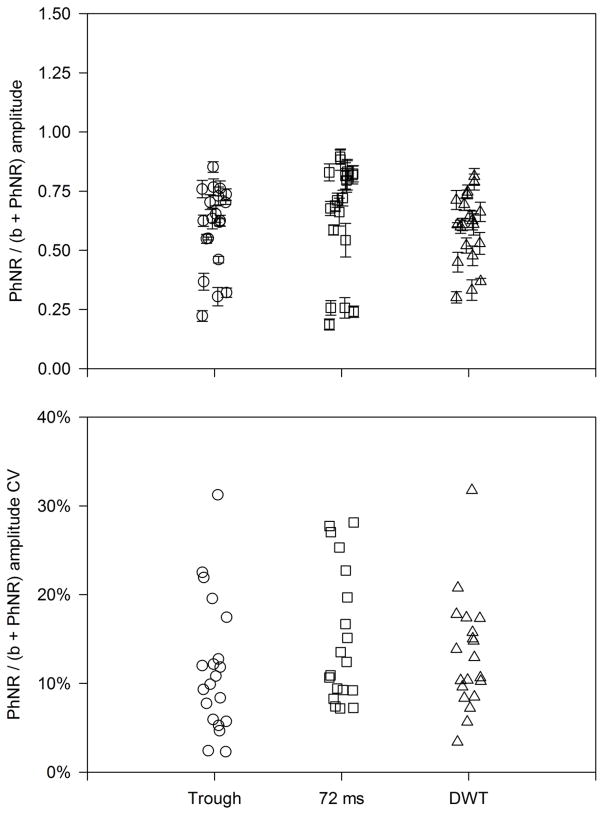
As an alternative approach to normalizing the PhNR by the b-wave, Mortlock et al. [13] recommended inclusion of the b-wave in the numerator and denominator and defined a “peak-to-trough b-wave to PhNR amplitude ratio” (PTR). We propose a related definition that includes the PhNR amplitude in the numerator and denominator: PhNR/(b+PhNR). As outlined in the Discussion, there may be advantages of this definition over the PTR. Fig. 4 shows that normalization by the sum of the PhNR and b-wave amplitudes substantially reduced the variation among and within subjects, compared to the more commonly used PhNR/b ratio. Figure 4 (top) shows that the amplitude ratios for measurements at the trough (circles), 72 ms (squares), and obtained from the DWT (triangles) were approximately similar. The bottom panel of Fig. 4 shows the CV values obtained for the three measurements. The mean CV values were approximately similar for measurements made at the trough (12%), at 72 ms (16%), and for the DWT (13%). These CV values are all substantially smaller than those obtained without normalization or by normalization to the b-wave (PhNR/b).
Figure 4.
The top panel shows the PhNR normalized by the sum of the b-wave and PhNR amplitudes and the bottom panel shows the CV for the b-wave + PhNR normalized amplitude for each subject. Note that the y-axis scale in the bottom panel differs from Figs. 2 and 3; other conventions are as in Fig. 2.
Discussion
The present study sought to compare measurements of the PhNR using time and time-frequency domain analyses. All measures of the PhNR are subject to variability, typically greater than that observed for the a- and b-waves, due to baseline drift, eye movements, and the small, slow nature of the response. By comparing two common time domain measurements (at the trough following the b-wave and at a fixed time-point) and one novel time-frequency domain measure (DWT), we endeavored to identify the least variable measure of the PhNR. In general, measurement at the trough, at 72 ms, and from the DWT provided approximately similar estimates of the PhNR (i.e. they were all significantly correlated). However, variation was smallest for calculations at the trough, largest when calculated at 72 ms, and intermediate for the DWT. This finding is consistent with a previous study [13] that found less variability among subjects for PhNR measurements made at the trough compared to those made at a fixed time-point.
Each of the three definitions of the PhNR evaluated in the present study has advantages and disadvantages. For example, measurements at the trough are easily implemented and provide a measure of response timing. However, for small amplitude responses, such as those observed in some patient populations, a single trough cannot always be identified, resulting in a subjective definition for these individuals. Defining the PhNR at a constant time-point largely obviates this problem, but has the disadvantage that a somewhat arbitrary time-point must be selected. The timing of the PhNR is known to become progressively delayed with age [3], so the use of multiple fixed time-points may be necessary if the age of the subjects spans a broad range. As a further complication, selecting a time-point based on previous literature could possibly be misleading unless care is taken to match stimulus characteristics including luminance, duration, and wavelength. The DWT, which to our knowledge has not been used previously to evaluate the PhNR, has a number of advantages: it is objective, not based on a single time-point, and is capable of defining slow, small amplitude responses. The primary disadvantage of the DWT is that the algorithms needed to extract the energy from the signal are somewhat complex and less intuitive than standard analyses that are performed only in the time domain. Additionally, the specific implementation of the DWT may need to be optimized for the particular signal component of interest by changing parameters such as the shape of the mother wavelet, as well as the amount of translation and padding. Nevertheless, DWT, or other time-frequency domain analyses, could be built into ERG software allowing for easier implementation in the clinic.
To reduce variability among subjects, several previous studies have normalized the PhNR amplitude by the b-wave amplitude [3,6,7,13,14]. However, the PhNR measurements made in the present study were not less variable among (or within) subjects after normalization by the b-wave. The similar results for the absolute and normalized CV values is likely attributable to the non-significant correlation between the b-wave and PhNR amplitudes: the Pearson correlation coefficient describing the relationship between the b-wave amplitude and the PhNR amplitude was 0.12 (p = 0.61) for measurements at the trough and 0.19 (p = 0.43) for measurements at 72 ms. The b-wave and PhNR energies were significantly correlated (r = 0.58, p = 0.01); nevertheless, the effect of normalization by the b-wave energy on the CV was minimal.
Mortlock et al. [13] recommended inclusion of the b-wave in the numerator and denominator when normalizing the PhNR. Specifically, they defined the PTR as the ratio of the b-wave to the PhNR measured from the peak of the b-wave. Although the PTR greatly reduced the between subjects variability (inter-subject CV), it has the counterintuitive property that large PTR values represent small PhNRs. In fact, waveforms that completely lack a PhNR would result in a PTR value greater than one. As an alternative, we included the PhNR in both the numerator and denominator (i.e. PhNR/[b+PhNR]). This definition is more intuitive in that large ratios represent large PhNRs. Additionally, for waveforms that lack a PhNR, this ratio would be zero (rather than a number greater than 1). This definition substantially reduced the variability among and within subjects and may be useful in future studies. That is, PhNR definitions that result in low variation among visually-normal subjects might be useful for comparing control and patient populations, as these definitions may make abnormalities in patient populations more apparent.
In summary, our data suggest that measures of the PhNR made at the trough are an appropriate, simple technique for characterizing the PhNR. In cases where a clear PhNR trough is not apparent, the DWT can be used to extract a time-frequency component that corresponds to the PhNR, as there is a significant correlation between the PhNR defined at the trough and by DWT energy. It is possible that the correlation between these measurements, and the CV derived by DWT, could be improved by further optimizing the DWT parameters by selecting alternative mother wavelets, implementing waveform translation, and/or combining time-frequency bins. Furthermore, other approaches to signal analysis, such as obtaining Fourier series, may be useful for deriving and quantifying the PhNR. Nevertheless, the parameters and approach used in the present study provide a good approximation of the PhNR in the time-frequency domain. Finally, normalization of the PhNR by the sum of the b-wave and PhNR amplitudes (or energies) can reduce variability substantially among subjects, and may be particularly useful for samples in which the correlation between the b-wave and PhNR amplitude (or energy) is weak.
Supplementary Material
Supplementary Figure 1: The DWT of the mean waveform is shown in the top panel (replotted from Fig. 1). The middle panel shows the effects on DWT energy of setting the mean response voltage to 0 μV after 60 ms; the PhNR component is absent. The bottom panel shows the effects on DWT energy of setting the mean response voltage to 0 μV from 0 ms to 60 ms; the PhNR component is present, but the early a-wave and b-wave components are strongly attenuated.
Acknowledgments
This research was supported by National Institutes of Health research grants R01EY026004 (JM) and P30EY001792 (UIC core grant), an unrestricted departmental grant and a Dolly Green Special Scholar Award (JM) from Research to Prevent Blindness.
Funding: The National Institutes of Health and Research to Prevent Blindness provided financial support in the form of funding. The sponsors had no role in the design or conduct of this research.
Footnotes
Conflict of Interest: All authors certify that they have no affiliations with or involvement in any organization or entity with any financial interest (such as honoraria; educational grants; participation in speakers’ bureaus; membership, employment, consultancies, stock ownership, or other equity interest; and expert testimony or patent-licensing arrangements), or non-financial interest (such as personal or professional relationships, affiliations, knowledge or beliefs) in the subject matter or materials discussed in this manuscript.
Ethical approval: All procedures performed in studies involving human participants were in accordance with the ethical standards of the institutional and/or national research committee and with the 1964 Helsinki declaration and its later amendments or comparable ethical standards.
Informed consent: Informed consent was obtained from all individual participants included in the study
References
- 1.Rangaswamy NV, Frishman LJ, Dorotheo EU, Schiffman JS, Bahrani HM, Tang RA. Photopic ergs in patients with optic neuropathies: Comparison with primate ergs after pharmacologic blockade of inner retina. Invest Ophthalmol Vis Sci. 2004;45(10):3827–3837. doi: 10.1167/iovs.04-0458. [DOI] [PubMed] [Google Scholar]
- 2.Viswanathan S, Frishman LJ, Robson JG, Harwerth RS, Smith EL., 3rd The photopic negative response of the macaque electroretinogram: Reduction by experimental glaucoma. Invest Ophthalmol Vis Sci. 1999;40(6):1124–1136. [PubMed] [Google Scholar]
- 3.Viswanathan S, Frishman LJ, Robson JG, Walters JW. The photopic negative response of the flash electroretinogram in primary open angle glaucoma. Invest Ophthalmol Vis Sci. 2001;42(2):514–522. [PubMed] [Google Scholar]
- 4.Rangaswamy NV, Shirato S, Kaneko M, Digby BI, Robson JG, Frishman LJ. Effects of spectral characteristics of ganzfeld stimuli on the photopic negative response (phnr) of the erg. Invest Ophthalmol Vis Sci. 2007;48(10):4818–4828. doi: 10.1167/iovs.07-0218. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 5.Colotto A, Falsini B, Salgarello T, Iarossi G, Galan ME, Scullica L. Photopic negative response of the human erg: Losses associated with glaucomatous damage. Invest Ophthalmol Vis Sci. 2000;41(8):2205–2211. [PubMed] [Google Scholar]
- 6.Machida S, Gotoh Y, Toba Y, Ohtaki A, Kaneko M, Kurosaka D. Correlation between photopic negative response and retinal nerve fiber layer thickness and optic disc topography in glaucomatous eyes. Invest Ophthalmol Vis Sci. 2008;49(5):2201–2207. doi: 10.1167/iovs.07-0887. [DOI] [PubMed] [Google Scholar]
- 7.Preiser D, Lagreze WA, Bach M, Poloschek CM. Photopic negative response versus pattern electroretinogram in early glaucoma. Invest Ophthalmol Vis Sci. 2013;54(2):1182–1191. doi: 10.1167/iovs.12-11201. [DOI] [PubMed] [Google Scholar]
- 8.Wilsey LJ, Fortune B. Electroretinography in glaucoma diagnosis. Curr Opin Ophthalmol. 2016;27(2):118–124. doi: 10.1097/ICU.0000000000000241. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 9.Gotoh Y, Machida S, Tazawa Y. Selective loss of the photopic negative response in patients with optic nerve atrophy. Arch Ophthalmol. 2004;122(3):341–346. doi: 10.1001/archopht.122.3.341. [DOI] [PubMed] [Google Scholar]
- 10.Gowrisankaran S, Genead MA, Anastasakis A, Alexander KR. Characteristics of late negative erg responses elicited by sawtooth flicker. Doc Ophthalmol. 2013;126(1):9–19. doi: 10.1007/s10633-012-9352-z. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Moss HE, Park JC, McAnany JJ. The photopic negative response in idiopathic intracranial hypertension. Invest Ophthalmol Vis Sci. 2015;56(6):3709–3714. doi: 10.1167/iovs.15-16586. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Abed E, Piccardi M, Rizzo D, Chiaretti A, Ambrosio L, Petroni S, Parrilla R, Dickmann A, Riccardi R, Falsini B. Functional loss of the inner retina in childhood optic gliomas detected by photopic negative response. Invest Ophthalmol Vis Sci. 2015;56(4):2469–2474. doi: 10.1167/iovs.14-16235. [DOI] [PubMed] [Google Scholar]
- 13.Mortlock KE, Binns AM, Aldebasi YH, North RV. Inter-subject, inter-ocular and inter-session repeatability of the photopic negative response of the electroretinogram recorded using dtl and skin electrodes. Doc Ophthalmol. 2010;121(2):123–134. doi: 10.1007/s10633-010-9239-9. [DOI] [PubMed] [Google Scholar]
- 14.Fortune B, Bui BV, Cull G, Wang L, Cioffi GA. Inter-ocular and inter-session reliability of the electroretinogram photopic negative response (phnr) in non-human primates. Exp Eye Res. 2004;78(1):83–93. doi: 10.1016/j.exer.2003.09.013. [DOI] [PubMed] [Google Scholar]
- 15.Gauvin M, Lina JM, Lachapelle P. Advance in erg analysis: From peak time and amplitude to frequency, power, and energy. Biomed Res Int. 2014;2014:246096. doi: 10.1155/2014/246096. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Gauvin M, Little JM, Lina JM, Lachapelle P. Functional decomposition of the human erg based on the discrete wavelet transform. J Vis. 2015;15(16):14. doi: 10.1167/15.16.14. [DOI] [PubMed] [Google Scholar]
- 17.Mallat SG. A wavelet tour of signal processing the sparse way. 3. Academic Press; Houston, TX: 2009. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Supplementary Figure 1: The DWT of the mean waveform is shown in the top panel (replotted from Fig. 1). The middle panel shows the effects on DWT energy of setting the mean response voltage to 0 μV after 60 ms; the PhNR component is absent. The bottom panel shows the effects on DWT energy of setting the mean response voltage to 0 μV from 0 ms to 60 ms; the PhNR component is present, but the early a-wave and b-wave components are strongly attenuated.