Skip to main content
PMC home page
Nature Communications logoLink to Nature Communications
. 2016 Sep 29;7:12836. doi: 10.1038/ncomms12836

Engineering island-chain silicon nanowires via a droplet mediated Plateau-Rayleigh transformation

Zhaoguo Xue 1, Mingkun Xu 1, Yaolong Zhao 1, Jimmy Wang 1, Xiaofan Jiang 1, Linwei Yu 1,2,a, Junzhuan Wang 1, Jun Xu 1,a, Yi Shi 1, Kunji Chen 1, Pere Roca i Cabarrocas 2
PMCID: PMC5056411  PMID: 27682161

Abstract

The ability to program highly modulated morphology upon silicon nanowires (SiNWs) has been fundamental to explore new phononic and electronic functionalities. We here exploit a nanoscale locomotion of metal droplets to demonstrate a large and readily controllable morphology engineering of crystalline SiNWs, from straight ones into continuous or discrete island-chains, at temperature <350 °C. This has been accomplished via a tin (Sn) droplet mediated in-plane growth where amorphous Si thin film is consumed as precursor to produce crystalline SiNWs. Thanks to a significant interface-stretching effect, a periodic Plateau-Rayleigh instability oscillation can be stimulated in the liquid Sn droplet, and the temporal oscillation of the Sn droplets is translated faithfully, via the deformable liquid/solid deposition interface, into regular spatial modulation upon the SiNWs. Combined with a unique self-alignment and positioning capability, this new strategy could enable a rational design and single-run fabrication of a wide variety of nanowire-based optoelectronic devices.

Inline graphicThe ability to program periodic morphology into nanowires affords control over photonic and electronic transport properties. Here, the authors stimulate Plateau-Rayleigh transformations in silicon nanowires through an oscillating catalyst droplet, resulting in nanowires with island-chain morphology.

In analogy to Plateau-Rayleigh (P-R) instability in a dribbling fluid thread1, one-dimensional (1D) nanowires are thermally instable in view of surface Gibbs energy. If an efficient mass migration is allowed, the nanowires will tend to develop large diameter variation or just break-up into discrete spheres2,3,4. This kind of morphology evolution, if it happens in a controllable way, could provide an extremely powerful way to engineer the electronic, photonic and phononic transport through the silicon nanowire (SiNW) channels5,6,7,8,9,10,11,12,13,14. So far, such periodically modulated SiNW channels have been mostly manufactured by sophisticated electron beam lithography out of silicon-on-insulator wafer11,15, or by selective chemical etching into the uniform SiNWs trunks16,17. In parallel, diameter and composition-modulated superstructure are widely sought after in III–V or Ge nanowires grown via periodically controlled vapour–liquid–solid (VLS) approach18,19,20,21. Whether a simple self-organization can be tamed to engineer such sophisticated functional building blocks, ideally in a low temperature, reproducible and programmable way, is not only an intriguing fundamental challenge but also of tremendous practical importance.

It has been known that uniform-sized SiNWs experience a complete spheroidization at high temperature (>1000 °C; refs 2, 22, 23). A Plateau-Rayleigh crystal growth has been proposed, only recently, to demonstrate a discrete island-shell growth upon the sidewall of Si or Ge nanowires3,4. However, this P-R instability transformation becomes extremely inefficient at low temperature far below the melting point of Si (1414 °C for c-Si). Seeking a P-R transformation of solid c-SiNWs, at a much lower temperature and in a controllable means, requires a radically new strategy that stems from the unexplored potential of nanoscale locomotion growth.

We here report a large P-R self-transformation phenomenon during in-plane SiNW growth, mediated by low-melting-point tin (Sn) catalyst droplets that take in amorphous Si as precursor and produce crystalline in-plane SiNWs behind. Thanks to an extraordinary large interface-interaction that forces a significant stretching of the liquid catalyst droplet, which is usually unattainable in the typical VLS growth in a gaseous growth environment24,25, a periodic P-R transformation/oscillation can be stimulated in the Sn droplet and transferred to shape highly modulated SiNWs, from straight ones into continuous or discrete island-chains, all in a single-run low temperature fabrication <350 °C. In addition, this unique morphology engineering capability is accompanied with a precise self-positioning capability, and these combined are advantageous for large scale device connection and deployment.

Results

Fabrication of SiNWs with a-Si:H thin film precursor

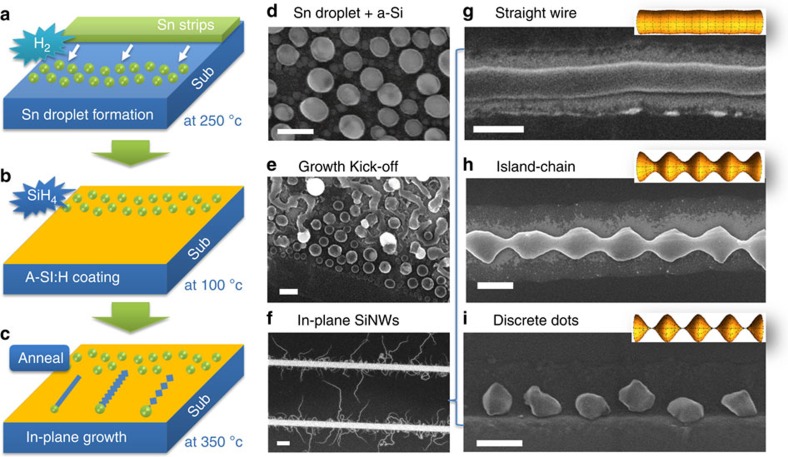
The in-plane SiNWs are grown on SiO2 (300 nm)/n+-Si substrates with pre-patterned Sn stripes prepared by thermal evaporation to 20–40 nm thick. The samples are first loaded into a plasma-enhanced chemical vapour deposition (PECVD) system and treated by H2 plasma at 250 °C (Fig. 1a) to transform the Sn stripes into discrete Sn droplets with diameters ranging from 200 to 400 nm; Then, a thin layer of hydrogenated amorphous silicon (a-Si:H) is coated at 100 °C (Fig. 1b) to a thickness of 30 to 60 nm, as witnessed in the scanning electron microscopy (s.e.m.) image in Fig. 1d; Finally, the samples are annealed at 350 °C in a H2 atmosphere for 20 min, to activate the Sn liquid droplets to kick off the in-plane growth. Interestingly, as the in-plane growth requires no gas feeding and thus can be observed directly in the s.e.m. system equipped with a heating stage (see Methods for more details). In the s.e.m. image of the initial kick-off growth of the Sn droplets (Fig. 1e), the Sn droplets are identified as the bright spots that absorb a-Si:H thin film in front and produce crystalline SiNWs behind. Meanwhile, in situ s.e.m. images recorded during the in-plane growth of SiNWs in real-time provide the most straightforward proof of a nanoscale locomotion led by Sn droplets, as witnessed in the s.e.m. snapshots presented in Supplementary Fig. 1. However, we didnot succeed in triggering and recording an island-chain growth in in situ s.e.m. heating and growth, probably due to the amorphous carbon contamination on the sample surface, which is almost inevitable in our s.e.m. set-up. Usually, only bending but uniform-sized SiNWs can be obtained during an in situ s.e.m. heating growth. A direct comparison of the in-plane SiNWs produced via an annealing in PECVD or s.e.m. chambers are provided in Supplementary Fig. 2, as well as a more detailed discussion of this point in Supplementary Discussion.

Figure 1. Fabrication procedure and illustration of the morphology transformation.

Figure 1

Open in a new tab

(a–c) Illustrate the H2 plasma catalyst formation, a-Si:H layer coating and annealing growth steps during the Sn-catalysed in-plane growth of silicon nanowires (SiNWs), while d shows the scanning electron microscopy (s.e.m.) image of the Sn catalyst droplets after a-Si:H layer coating, (e) the in situ s.e.m. observation of the initial activation and growth of Sn droplets within the Sn pad stripe and (f) the outgrowth of in-plane SiNWs from the Sn seeding stripes; (g–i) Depicts the self-transformation of straight SiNW into continuous or discrete island-chain nanowire structure, in a way equivalent to the morphology evolution due to Plateau-Rayleigh instability. Scale bars, 400 nm in (d,e); Scale bars, 10 μm in (f) and Scale bars, 200 nm in (g–i).

At the end of the growth, the remnant a-Si:H layer can be selectively removed by a low temperature H2 plasma etching at 100 °C, while preserving very well the crystalline SiNWs26. In addition, in order to direct and position the in-plane SiNWs, parallel step-edge lines lying perpendicular to the Sn stripes were etched into the oxide layer to a depth of ∼180 nm before the above-mentioned fabrication procedures. A close s.e.m. image taken at 45 degree (presented in Supplementary Fig. 3) provides a better sideview of the guiding step edge configuration.

Island-chain structure of the in-plane SiNWs

There are three distinct morphologies, as illustrated in Fig. 1c, that the Sn-mediated in-plane automation will produce in a single-run growth, which are respectively the uniform-sized straight, continuous island-chains (ic) SiNWs and the discrete-dot structures, as seen in the s.e.m. imaging in Fig. 1g–i. Particularly, the continuous island-chain SiNWs seen in Fig. 1h features an elegant sinusoidal edge-line, with a large diameter/or width modulation varying from ∼180 nm in the island to only 20–30 nm at the connecting bridges. On both sides of the SiNWs and the region, passed by the Sn droplet, a whiter region appears in the s.e.m. images as the a-Si:H layer has been absorbed. Note that, the large max/min width modulation and the rather smooth edge-lines all indicate a fundamentally different formation mechanism from the shallow corrugation observed on the sidewall of VLS-grown SiNWs27,28. Importantly, the converging trough or bridge necks are well preserved between the islands, which are indeed a prerequisite to allow efficient electric transport for electronic or thermoelectric applications.

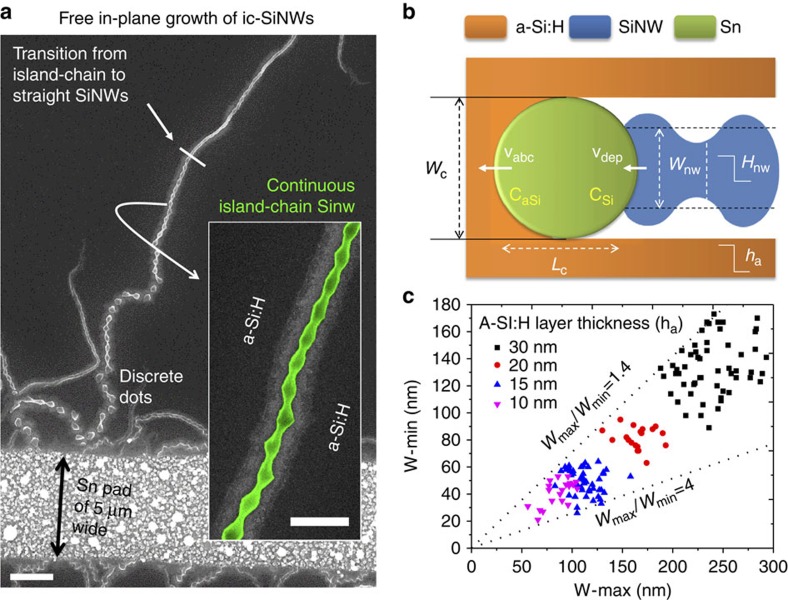
Remarkably, though the outgrowth of the in-plane SiNW can reach as long as >50 μm, as seen in the s.e.m. image in Fig. 1f, a closer scrutiny of single SiNWs reveal, however, three growth stages with clear morphology transition from discrete to continuous island-chain, and to uniform-sized SiNWs, as witnessed in Fig. 2a. Departing from the Sn pad edge, the relatively thick SiNWs are found to be composed of discrete islands/or grains, which then gradually merge with the neighbours into a continuous island-chain structure, as seen in the inset of Fig. 2a, when the in-plane growth proceed further. Later on, even the width modulation amplitude diminishes gradually, and eventually switch into a uniform-sized SiNWs. For instance, this transit from the island-chain growth into uniform-sized ones has been marked by a dashed line in Fig. 2a. Meanwhile, it is also noteworthy that such an island-chain growth are only observed for the Sn-catalysed growth of SiNWs, while the indium-catalysed in-plane growth leads to usually uniform-sized SiNWs29,30. In addition, plotting the max/min width ratio variations for the continuous ic-SiNWs with different initial a-Si:H layer thicknesses ha in Fig. 2c shows that (1) the mean width of the in-plane growth of ic-SiNW scales up linearly with the increase of the a-Si:H layer thickness; and (2) the max/min width ratios share a common linear up-bound, delineated by rd∼140%, while the maximum is obtained for the thinner ones (with proportional thinner ha as well) with a ratio beyond rd >400%.

Figure 2. Morphology and statistics of freely grown island-chain nanowires.

Figure 2

Open in a new tab

(a) Shows an enlarged view of a single island-chain silicon nanowire (ic-SiNW) grown out of the Sn pad edge, with an inset that displays a green colour-highlighted segment of the continuous island-chain structure in the middle of the SiNWs. The width of the Sn stripe is 5 μm wide. Scale bars in a and in the inset are 2 μm and 1 μm, respectively; (b) Depicts the key dimension parameters of control in the nanoscale locomotion growth system, where height of the SiNW and the a-Si:H layer are labeled as Hnw and ha, respectively; (c) Plots the width modulation of the in-plane island chain SiNWs, with different a-Si:H layer thickness of ha=10, 15, 20 and 30 nm

In parallel, reference island-chain (ic) SiNW samples with shorter annealing growth times of 10 min and 2 min are also prepared, with corresponding s.e.m. images shown in Supplementary Fig. 4. Compared with the ic-SiNWs out of 20-min annealing growth as seen in Fig. 2a, we found that the Sn droplets were almost exhausted after the first 10 min annealing growth, leaving behind ic-SiNWs with similar lengths and morphology features. Meanwhile, the Sn catalyst droplets can be observed after only 2-min annealing growth, as shown in Supplementary Fig. 4c,d, where the island-chain structures are produced immediately after the leading Sn catalyst droplets, without the final stage of uniform-sized SiNW. These observations indicate that the island-chain structures are not produced via direct P-R morphology transformation of the uniform SiNWs (after they were produced by the Sn catalyst droplet), rather the large diameter modulation are directly shaped or forged by the leading Sn droplets, as a consequence of large oscillation dynamics during the Sn droplet's in-plane movement, as will be discussed in more details later.

It is also noteworthy that, according to the atomic force microscopy profile reconstruction and the cross-section analysis of a continuous island-chain SiNW segment presented in Supplementary Fig. 5, the in-plane SiNWs typically have a larger width than their height, Wnw>Hnw. This feature can be understood as a consequence of a surface-constraint in-plane growth, where the precursor thin film supply is limited on the lower substrate surface, which is also in a strong contrast to that in gas-feeding VLS growth environment (usually leads to a round cross section).

Self-positioned guided growth of the ic-SiNWs

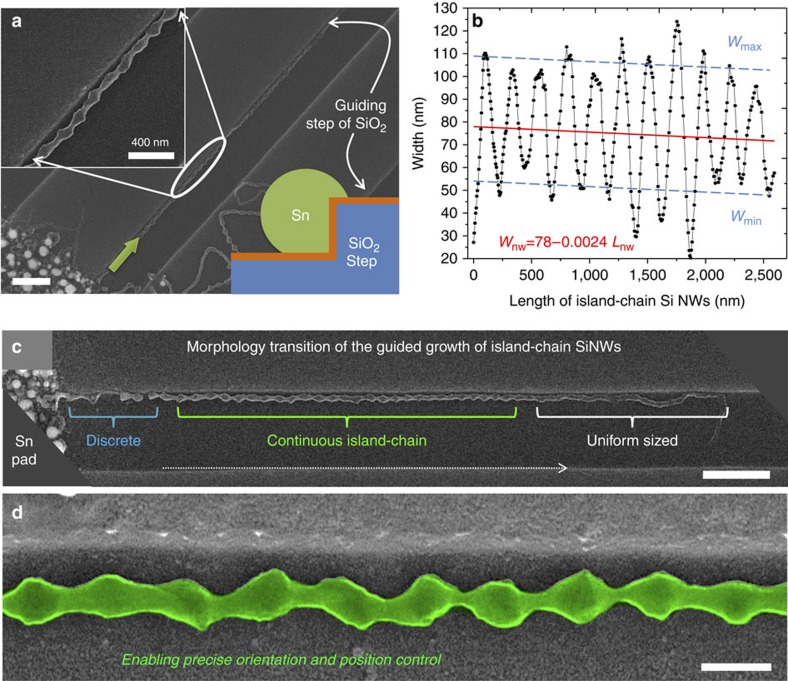
More excitingly, the growth of ic-SiNWs by itself can be directed into precise direction and locations, with the aid of simple step edges formed by etching into the SiO2 dielectric layer and lying perpendicular to the Sn stripes, as seen in Fig. 3a. A schematic illustration of the guided growth along step edge has been placed in the bottom-right inset, depicting a cross-section view of the guided growth SiNWs with an extra contact line on the vertical sidewall that can help to attract the catalyst droplet to move along. This self-positioning or guided growth is indeed a unique capability of the in-plane growth of SiNWs26,31, which is important to deploy SiNW-based functionalities over large scale, without the need of any extra nano-manipulation. A statistics of the width variation along the length of a guided ic-SiNW is plotted in Fig. 3b, with the corresponding s.e.m. image marked in green shown in Fig. 3d. A linear fitting of the width evolution in Fig. 3b reveals a slightly decreasing trend of the mean width of 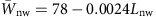Inline graphic along the growth direction.

Figure 3. Morphology and statistics of guided growth island-chain nanowires.

Figure 3

Open in a new tab

(a) Show the s.e.m. images of the guided growth of island-chain silicon nanowires (ic-SiNWs) along the step-edges formed by etching into the SiO2 substrate, with a configuration as depicted by the inset; (b) Summarizes the width variation, as well linear fittings of the average, minimum and maximum width of the guided growth of SiNW, along the length of the ic-SiNW segment marked in green in d,c provides an overview of an ic-SiNWs, starting from the Sn pad edge on the left, which experiences a series of morphology transition, during the guided in-plane growth, from discrete to continuous island-chains and then to uniform-sized ones. Scale bars, 1 μm in (a); scale bars, 1 μm in (c) and scale bars, 200 nm in (d).

Structural analysis

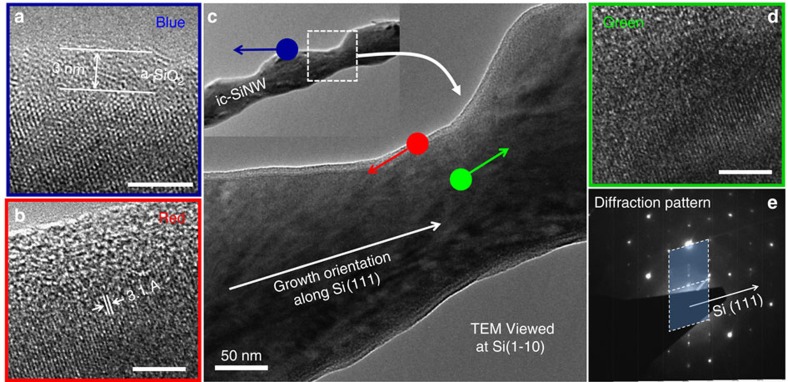
Despite of a large geometry modulation, a high crystallinity has been confirmed within the ic-SiNWs, as witnessed in Fig. 4a–d, where a series of high-resolution transmission electron microscopy (HR-TEM) characterizations are presented. Surrounding by a thin native oxide (a-SiO2) layer of ∼3 nm thick on the sidewall, the HR-TEM lattice images, taken at three different locations, indicated a coherent Si lattice throughout the ic-SiNWs, for example, in the regions marked by the blue spot in Fig. 4a at the edge of the island maximum, and the green and the red ones at the edge and the center of a converging trough in Fig. 4b–d, respectively. Also, for this specific ic-SiNW segment, the growth direction is identified to be along Si[111] orientation, as determined by the electron diffraction pattern presented in Fig. 4e. This is somehow deviated from the conventional Si[211] growth direction of the in-plane SiNWs led by indium droplets26,32.

Figure 4. Structural analysis of an island-chain nanowire segment.

Figure 4

Open in a new tab

(a,b,d) Show the high-resolution transmission electron microscopy lattice images taken three different locations at the island maximum (marked by the blue spot), the edge (red) and the center (green) of the converging trough neck, as indicated in c. According to the electron diffraction pattern recorded in the center in e, the growth direction of this ic-SiNW is determined to be along Si[111] direction. Scale bars, 5 nm in (a); scale bars, 5 nm in (b); scale bars, 5 nm in (d).

Discussion

In order to understand this unique self-automated growth dynamics, we come to formulate the in-plane growth with a set of key parametric dimensions as indicated in Fig. 2b. In general, a higher Gibbs energy of Si atoms in the amorphous state is the key driving force for the Sn catalyst droplet to absorb Si atoms continuously from the front a-Si:H/Sn interface and precipitate c-SiNWs at the rear Sn/SiNW deposition interface. Due to a higher Gibbs energy seen by the Si atoms in the amorphous status compared with that in crystalline phase, δE∼0.11 to 0.15 eV (refs 33, 34), the equilibrium concentration of Si in the liquid Sn droplet at the front a-Si:H/Sn interface (CaSi, in contact to an a-Si:H medium) is higher than that of the rear Sn/c-Si interface (C0, in contact to c-SiNW) with a relation of CaSi=eδE/kTC0. This equilibrium concentration difference will drive a diffusion flux of the dissolved Si atoms front the front interface towards the rear deposition interface, and thus establishing a supersaturation of CSi>C0 that leading to an axial growth rate of

graphic file with name ncomms12836-m2.jpg

where B is assumed to be a constant pre-factor. In the meantime, the front absorption interface advance and move on, with a speed mainly limited by the diffusion transport of Si atoms over the length of the Sn catalyst droplet Lc, as

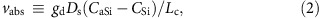graphic file with name ncomms12836-m3.jpg

where Ds is the diffusion constant of Si atoms in Sn droplet, and gd the geometric correction factor accounting for the asymmetric width of the front and rear interfaces.

Actually, a distinctive aspect of the in-plane growth of SiNW is that the front Sn/a-Si:H and the rear SiNW/Sn boundaries are both solid-liquid interfaces, and both of them can exert significant forces upon the liquid catalyst droplet sandwiched in between, which is in strong contrast to the situation of conventional VLS growth where a soft gaseous precursor environment. During the in-plane growth, the droplet always tends to presume a spherical shape while its length is always constrained by the relative positions of the two bounded interfaces, that is, the absorption front interface and the deposition interface contacting the SiNW end. As a consequence, the temporal variation of Lc is determined by the relative speeds of the front absorption and the rear deposition interfaces, as

graphic file with name ncomms12836-m4.jpg

Meanwhile, the evolution of the dissolved Si atom concentration at the rear Sn/c-SiNW interface is governed by the competition between the absorption and deposition terms with

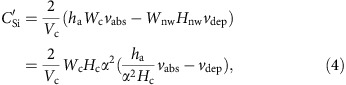graphic file with name ncomms12836-m5.jpg

where Wc and Wnw are the widths of the absorption and the deposition interfaces, as defined in Fig. 2b, 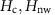Inline graphic and ha the heights of the Sn droplet, the SiNW and the a-Si:H layer, respectively. The width and the height of the SiNWs are always proportional to the dimensions of the Sn droplet, with Wnw=αWc and Hnw=αHc.

According to equation (1), the moving rate of the rear deposition interface can be represented by an blue line in Fig. 5a that increases linearly with higher Si atom concentration of CSi dissolved in the Sn droplet, while the moving rate of the front absorption interface is plotted as the orange line that decreases, according to equation (2), with the increase of CSi and drops to zero when CSi=CaSi. These two lines cross at a balance condition point P1 sought by equation (3) to arrive 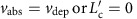Inline graphic. However, this will not always guarantee a balanced state for the evolution of CSi in equation (4), unless the pre-factor before vabs within the parenthesis of equation (4) equals unity 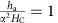Inline graphic. In case of 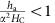Inline graphic with a crossing point between the green absorption line and the blue deposition line at P2, to the left of P1, the deposition term in equation (4) outweighs the absorption and thus the Si concentration will decrease from CSi1→CSi2 to approach P2. When this continues, the front interface will pick up speed and the rear interface will slow down a little bit. As a consequence, the Sn droplet is forced to deform, being elongated in order to bring down the slope of the absorption line, which is ∼1/Lc according to equation (2). In the meantime, a longer Lc causes a thinner Hc (and Wc alike), and enlarges the factor of 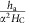Inline graphic as well, to meet the inclining vabs line. In principle, if the initial catalyst diameter is 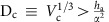Inline graphic, the Sn droplet will be driven into a stretching state with longer Lc and shorter Hc and Wc, otherwise the opposite will take place.

Figure 5. Growth balance point evolution and oscillation during in-plane growth.

Figure 5

Open in a new tab

The blue (or orange) line in a represents the evolution trend of the rear deposition (the front absorption) rate as a function of the Si concentration (CSi), while the green one stands for the product of the absorption flux with a geometry-dependent pre-factor of ha/α2Hc; (b) Illustrates the coincided balance points achieved when the pre-factor term ha/α2Hc=1, and their periodic oscillation around the balance points.

At the end of the evolution in Fig. 5a, the Sn droplet will be stretched to satisfy 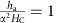Inline graphic, and thus the two balance points (P1 and P2) will coincide as depicted in Fig. 5b. Normally, this balance will be maintained if it is stable against external perturbation. This is, however, not always the case, because in view of the Gibbs surface energy, an elongated geometry is energetically unfavorable. Based merely on a surface tension consideration, the stretched Sn droplet, as illustrated in Fig. 6a, can be viewed as a segment of a continuous liquid rod/thread, where a Plateau-Rayleigh instability/transformation will happen whenever the length of the free droplet segment (Lc) is approaching 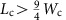Inline graphic, where Wc is the width of the catalyst droplet (see Supplementary Fig. 6 and Supplementary Discussion for the derivation). Note that, this self-transformation, arising from P-R instability will cause a gradual shrinkage of the rear deposition interface, and thus shape a narrowing neck/trough of the as-produced SiNWs, as Wnw=αWc.

Figure 6. The formation of continuous or discrete island-chain nanowires.

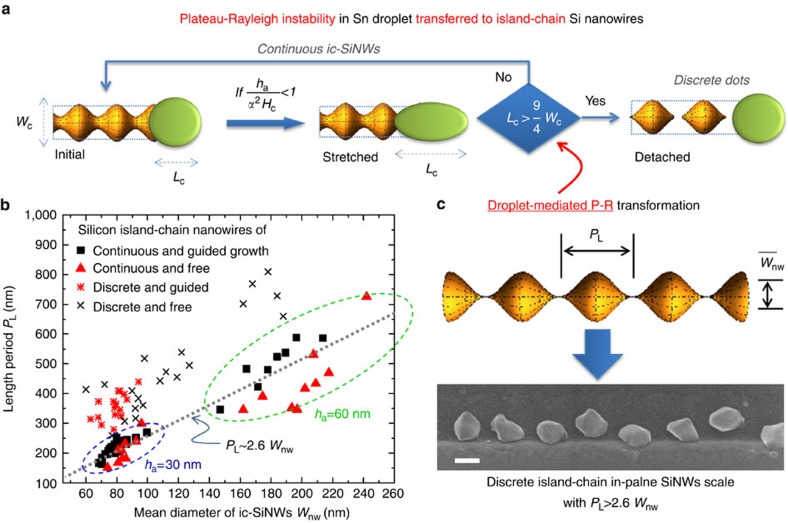
Figure 6

Open in a new tab

(a) Plateau-Rayleigh instability of the Sn catalyst droplets, under stretching, is recorded upon the as-produced SiNWs, leads to continuous or discrete island-chain geometry depending on whether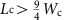Inline graphic, the criterion for P-R transformation; (b) Presents a statistics of the continuous or discrete island-chain SiNWs, with or without guiding step edges, grown with two a-Si:H layer thicknesses of ha=30 and 60 nm, respectively, as marked by the blue and the green dashed circles; (c) Provides the typical s.e.m. of the discrete Si dots structure. Scale bar, 200 nm.

Depending on how fast this nanoscale locomotion system can re-establish the balance, as discussed above, a thinner Hc has a counter effect to speed up the advancement of the rear deposition interface to catch up the front interface, there are two possible eventual geometries, as depicted schematically in Fig. 6a. In the first situation, if the counter mechanism can respond quickly enough, to reverse the elongation of the Sn droplet, continuous island-chain morphology will be produced; On the contrary, if the P-R transform proceed to the end, the Sn droplet can eventually detach from the pointed Si grain behind, in order to restore a spherical shape. Then, a new round of nucleation and growth will initiate and repeat, leaving a chain of discrete Si dots along the moving course, as witnessed for instance in Fig. 6c. This is indeed a remarkably new type of growth dynamics, where a low-melting-point catalyst droplet is confined by a pair of moving and interacting interfaces, and is forced to deform to a large extent that causes an automated P-R instability transformation. In turn, this droplet oscillating dynamics is faithfully recorded by the as-produced SiNWs, enabling an unprecedented morphology control of the functional 1D building blocks.

In order to gain deeper insights of this periodic growth of ic-SiNWs, we write down a very general form of the overall surface Gibbs energy, for the sake of brevity but without loss of generality, as 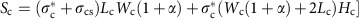Inline graphic, where 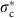Inline graphicis the mean surface energy density of Sn catalyst droplet (that also absorbs the contributions from Sn/SiNW and Sn/a-Si:H interfaces), while σcs stands for the interfacial energy density of the Sn/substrate interface. Under a constant volume constraint of Vc≡Wc(1+α)LcHc/2 and let Lc determined by the relative positions of the two interfaces, the liquid catalyst droplet is allowed to adjust Wc, in order to minimize the Sc by

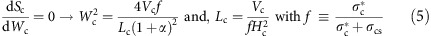graphic file with name ncomms12836-m16.jpg

Combining equation (3) and equation (4), the second temporal derivative of Lc is written as

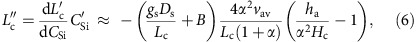graphic file with name ncomms12836-m17.jpg

where the speeds of the two interfaces are replaced by their mean velocity 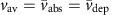Inline graphic at the equilibrium balance point. Assuming a periodic form of 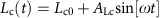Inline graphic, where ALc is the amplitude of oscillation, as well Wc(t)=Wc0+AWcsin[ωt+π] and Hc(t)=Hc0+AHcsin[ωt+π]. Note that, a phase shifting of π is imposed as 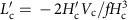Inline graphic according to equation (5). Inserting these into equation (6), with the derivation detailed in Supplementary Discussion, the first criterion to stimulate a periodic dynamics is

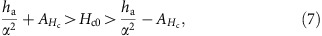graphic file with name ncomms12836-m21.jpg

where Hc0 is proportional to the initial size of the Sn catalyst droplet, as Hc0∼Vc1/3. Specifically, the second inequality in equation (7) set the first constraint for the ic-SiNW growth that

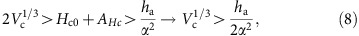graphic file with name ncomms12836-m22.jpg

which tells that the Sn catalyst droplet has to be large enough compared to the a-Si:H layer thickness of ha in order to trigger an oscillating growth dynamics. Otherwise, the oscillation dynamics will quickly damp away, leading to uniform-sized SiNWs as seen in Fig. 1g. This also explains why at the end of the in-plane growth, when the Sn droplet shrinks, an ‘island-chain to uniform-sized' transition will happen as observed in Fig. 2a and Fig. 3c. Actually, approaching to this transition point, the oscillation amplitude AHc will also diminish to AHc≪Hc0, which refines this criterion further to Inline graphic This indicates that, for a larger Sn droplet compared to a given a-Si:H thickness, in order to re-establish the growth balance condition as specified in Fig. 5b of Inline graphic, the Sn droplet will tend to develop a ‘stretching' status, which is exactly the key to trigger a P-R instability engineering of ic-SiNWs, as discussed above.

On the other hand, the first inequality in equation (7) requires that 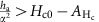Inline graphic. This implies that, when the Sn droplet is large with 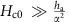Inline graphic, the size oscillation amplitude also has to increase proportionally. To the extreme of very large amplitude, that's AHc∼Hc0, the as-produced SiNWs will become simply discontinuous, as seen in Fig. 1i, though a precise description of this large-deformation dynamics falls beyond the capability of this qualitative analytic model. In short, to the lower end of Sn droplet size, only uniform-sized in-plane SiNWs will be produced, while to the higher end, the ic-SiNWs will tend to become discontinuous. This finding is indeed crucial, as controlling the relative size of the Sn droplet with respect to the given a-Si:H layer thickness (or 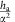Inline graphic) can provide a powerful and convenient means to program the morphology of the Sn-catalysed in-plane SiNWs.

Furthermore, expanding the last term in equation (6) to the first order around 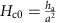Inline graphic, that is, 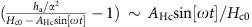Inline graphic, we have

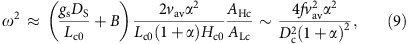graphic file with name ncomms12836-m30.jpg

where the first term on the right is proportional to 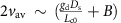Inline graphic and Dc∼Vc1/3. Thus, the length period PL of the island-chain nanowires can be deduced to be

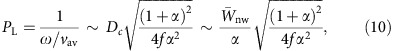graphic file with name ncomms12836-m32.jpg

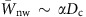where the mean width (Inline graphic) of the island-chain SiNWs is proportional to the cross section dimension of the Sn droplet Inline graphic. equation (10) implies that the length period of the ic-SiNWs (PL) should be proportional to the mean with of ic-SiNWs. And this relation has been examined in Fig. 6b, where the length period PL of the ic-SiNWs, grown freely or being guided along step-edge represented by the red triangles or the black circles, respectively, have been plotted against their mean width 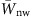Inline graphic. Discrete ic-SiNWs are usually found with a larger length period above the scaling low of continuous ones. Interestingly, though with two different a-Si:H coating layer thicknesses of ha=30 nm or 60 nm, the groups of continuous ic-SiNWs points split into two distinct groups as delineated by two dashed circles in blue and green, their distribution follows clearly a scaling trend of PL∼2.6Wnw, which agrees fairly well with what we expect of the lower-bound of 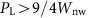Inline graphic for a Plateau-Rayleigh instability dominated self-transformation.

In other words, the island-chain nanowires are cast out of periodically vibrating Sn catalyst droplets under stretching, where the deformable deposition interface serves as a kinetics channel to translate the periodic droplet oscillation into spatial periodicity encoded upon the as produced SiNWs. It is important to emphasize that this self-automated island-chain modulation is fully programmable by means of controlling the a-Si:H layer thickness with respect to the initial catalyst size control. Combined with a unique self-positioning capability, these new findings will pave the way for future rational fabrication and practical device deployment.

In summary, we here demonstrate a unique Plateau-Rayleigh morphology engineering of 1D SiNWs mediated by a nanoscale locomotion of tin (Sn) catalyst droplets. Particularly, we have been able to fabricate well-defined and controllable island-chain SiNW structures via a low temperature (<350 °C) thin film deposition, and have shown that the island-chain SiNWs can be easily positioned along step-edges that enable precise location control. These results emphasize a unique morphology tailoring capability of such a nanoscale locomotion that will eventually enable a straightforward manufacturing of more sophisticated, as well more functional, nanostructures and devices.

Methods

SiNW fabrication

The in-plane SiNWs are fabricated a conventional PECVD system on n+-Si wafer substrate with a thermal SiO2 layer of 300 nm thick. Parallel Sn stripes of of ∼40 nm thick and 2 μm wide were defined by lithography patterning, thermal evaporation and lift-off. The samples are first clean by ultrasonic prior to loading into the PECVD chamber for H2 plasma treatment at 250 °C for 5 min; Then, a thin layer of hydrogenated amorphous silicon (a-Si:H) is deposited at 100 °C by pure silane plasma, with a flow rate of 10 standard cubic centimetre per minute (SCCM) and radio frequency (RF) power of 2W, to 30 nm ∼ 60 nm; After that, the substrate temperature is raised again to 350 °C and a H2 atmosphere annealing is carried out for 20 min, with a chamber pressure of 133 Pa. During this course, the in-plane growth of SiNWs are activated mediated by the surface-moving Sn liquid droplets; Finally, the remnant a-Si:H layer is removed by a low temperature H2 plasma etching at 100 °C.

In situ s.e.m. annealing and observation

The samples are first prepared with the same fabrication procedure in PECVD system as detailed above, except the final annealing step. Then, the samples are unloaded and transferred to s.e.m. (Zeiss Sigma) system, with an air-exposure period <2 min. Within the s.e.m. chamber, equipped with a heating stage (Kammrath & Weiss, Heating Module 800 °C), the substrate temperature is increased to 330 °C for 5 min to activate the catalyst droplets, and then ramped up to 350 °C for in-plane SiNW growth observation. During this course, the chamber vacuum is maintained at <5 × 10−3 Pascal.

Guiding step-edge preparation

For the guided in-plane growth of SiNWs, step edge lines are defined by lithography and subsequence reactive ion etching (RIE) etching into the underlying 300 nm oxide layer to a depth of ∼180 nm. The spacing between the guiding edge-lines are around 20 μm. In the next step, Sn stripes were prepared, with the above-mentioned procedure, but lying perpendicular to the guiding edge lines.

Data availability

All relevant data are available from the corresponding authors on request.

Additional information

How to cite this article: Xue, Z. et al. Engineering island-chain silicon nanowires via a droplet mediated Plateau-Rayleigh transformation. Nat. Commun. 7:12836 doi: 10.1038/ncomms12836 (2016).

Supplementary Material

Supplementary Information

Supplementary Figures 1-6 and Supplementary Discussion.

ncomms12836-s1.pdf (1.1MB, pdf)

Acknowledgments

We acknowledge the financial support from National Basic Research 973 Program under Grant Nos 2013CB632101, 2014CB921101 and NSFC under Nos. 61674075, 11274155 and 61204050, Jiangsu Excellent Young Scholar Program, Scientific and Technological Support Programme in Jiangsu province under No. BE2014147-2, Jiangsu Shuangchuang Team's and Personal Program and the Fundamental Research Funds for the Central Universities.

Footnotes

Author contributions Z.X., M.X. and L.Y. conceived, designed and carried out these experiments; L.Y. wrote the manuscript and prepared the figures; Z.X. and X.J. performed the s.e.m. and HR-TEM analysis; L.Y. and J.X. analysed the data and proposed the model; All the authors participated in discussing and reviewing the manuscript.

References

  1. Eggers J. Nonlinear dynamics and breakup of free-surface flows. Rev. Mod. Phys. 69, 865–930 (1997). [Google Scholar]
  2. Peng H. Y. et al. Temperature dependence of Si nanowire morphology. Adv. Mater. 13, 317–320 (2001). [Google Scholar]
  3. Day R. W. et al. Plateau–Rayleigh crystal growth of periodic shells on one-dimensional substrates. Nat. Nanotechnol. 10, 345–352 (2015). [DOI] [PubMed] [Google Scholar]
  4. Day R. W., Mankin M. N. & Lieber C. M. Plateau–Rayleigh crystal growth of nanowire heterostructures: strain-modified surface chemistry and morphological control in one, two, and three dimensions. Nano Lett. 16, 2830–2836 (2016). [DOI] [PubMed] [Google Scholar]
  5. Boukai A. I. et al. Silicon nanowires as efficient thermoelectric materials. Nature 451, 168–171 (2008). [DOI] [PubMed] [Google Scholar]
  6. Hochbaum A. I. et al. Enhanced thermoelectric performance of rough silicon nanowires. Nature 451, 163–167 (2008). [DOI] [PubMed] [Google Scholar]
  7. Carrete J., Gallego L. J., Varela L. M. & Mingo N. Surface roughness and thermal conductivity of semiconductor nanowires: going below the Casimir limit. Phys. Rev. B 84, 075403 (2011). [Google Scholar]
  8. Chen R. et al. Thermal conductance of thin silicon nanowires. Phys. Rev. Lett. 101, 105501 (2008). [DOI] [PubMed] [Google Scholar]
  9. Martin P., Aksamija Z., Pop E. & Ravaioli U. Impact of phonon-surface roughness scattering on thermal conductivity of thin Si nanowires. Phys. Rev. Lett. 102, 125503 (2009). [DOI] [PubMed] [Google Scholar]
  10. Blanc C., Rajabpour A., Volz S., Fournier T. & Bourgeois O. Phonon heat conduction in corrugated silicon nanowires below the casimir limit. Appl. Phys. Lett. 103, 043109 (2013). [Google Scholar]
  11. Fujiwara A. et al. Double-island single-electron devices. A useful unit device for single-electron logic LSI's. IEEE Transactions on Electron Devices 46, 954–959 (1999). [Google Scholar]
  12. Sutikno Bin M., Uda H. & Zul Azhar Z. J. Fabrication and characterization of Si quantum dots and SiO(2) tunnel barriers grown by a controlled oxidation process. Nanotechnology 19, 369–381 (2008). [DOI] [PubMed] [Google Scholar]
  13. Gorman J., Hasko D. G. & Williams D. A. Charge-qubit operation of an isolated double quantum dot. Phys. Rev. Lett. 95, 090502 (2005). [DOI] [PubMed] [Google Scholar]
  14. Zwanenburg F. A. et al. Silicon quantum electronics. Rev. Mod. Phys. 85, 961–1019 (2012). [Google Scholar]
  15. Takahashi Y. et al. Fabrication technique for Si single-electron transistor operating at room temperature. Electron. Lett. 31, 136–137 (1995). [Google Scholar]
  16. Christesen J. D., Pinion C. W., Grumstrup E. M., Papanikolas J. M. & Cahoon J. F. Synthetically encoding 10 nm morphology in silicon nanowires. Nano Lett. 13, 6281–6286 (2013). [DOI] [PubMed] [Google Scholar]
  17. Luo Z. et al. Atomic gold–enabled three-dimensional lithography for silicon mesostructures. Science 348, 1451–1455 (2015). [DOI] [PubMed] [Google Scholar]
  18. Musin I. R., Boyuk D. S. & Filler M. A. Surface chemistry controlled diameter-modulated semiconductor nanowire superstructures. J. Vac. Sci. Technol. B 31, 020603 (2013). [Google Scholar]
  19. Kallesøe C. et al. Integration, gap formation, and sharpening of III-V heterostructure nanowires by selective etching. J. Vac. Sci. Technol. 28, 21–26 (2010). [Google Scholar]
  20. Lim S. K., Crawford S., Haberfehlner G. & Gradečak S. Controlled modulation of diameter and composition along individual iii–v nitride nanowires. Nano Lett. 13, 331–336 (2013). [DOI] [PubMed] [Google Scholar]
  21. Caroff P. et al. Controlled polytypic and twin-plane superlattices in iii–v nanowires. Nat. Nanotechnol. 4, 50–55 (2009). [DOI] [PubMed] [Google Scholar]
  22. Kim B.-S. et al. Catalyst-free growth of single-crystal silicon and germanium nanowires. Nano Lett. 9, 864–869 (2009). [DOI] [PubMed] [Google Scholar]
  23. Wang H. et al. A surface curvature oscillation model for vapour-liquid-solid growth of periodic one-dimensional nanostructures. Nat. Commun. 6, (2015). [DOI] [PubMed] [Google Scholar]
  24. Wagner R. S. & Ellis W. C. Vapor-liquid-solid mechanism of single crystal growth. Appl. Phys. Lett. 4, 89 (1964). [Google Scholar]
  25. Yu L. et al. Plasma-enhanced low temperature growth of silicon nanowires and hierarchical structures by using tin and indium catalysts. Nanotechnology 20, 225604 (2009). [DOI] [PubMed] [Google Scholar]
  26. Yu L. et al. Growth-in-place deployment of in-plane silicon nanowires. Appl. Phys. Lett. 99, 203104–203103 (2011). [Google Scholar]
  27. Wen C.-Y. et al. Periodically changing morphology of the growth interface in Si, Ge, and GaP nanowires. Phys. Rev. Lett. 107, 1–14 (2011). [DOI] [PubMed] [Google Scholar]
  28. Ross F. M., Tersoff J. & Reuter M. C. Sawtooth faceting in silicon nanowires. Phys. Rev. Lett. 95, 146104 (2005). [DOI] [PubMed] [Google Scholar]
  29. Yu L. et al. In-plane epitaxial growth of silicon nanowires and junction formation on Si(100) substrates. Nano Lett. 14, 6469–6474 (2014). [DOI] [PubMed] [Google Scholar]
  30. Yu L., Alet P.-J., Picardi G. & Roca i Cabarrocas P. An in-plane solid-liquid-solid growth mode for self-avoiding lateral silicon nanowires. Phys. Rev. Lett. 102, 125501 (2009). [DOI] [PubMed] [Google Scholar]
  31. Xu M. et al. Operating principles of in-plane silicon nanowires at simple step-edges. Nanoscale 7, 5197–5202 (2015). [DOI] [PubMed] [Google Scholar]
  32. Chen W. et al. Incorporation and redistribution of impurities into silicon nanowires during metal-particle-assisted growth. Nat. Commun. 5, 4134 (2014). [DOI] [PubMed] [Google Scholar]
  33. Scarontich I., Car R. & Parrinello M. Amorphous silicon studied by ab initio molecular dynamics: preparation, structure, and properties. Phys. Rev. B 44, 11092 (1991). [DOI] [PubMed] [Google Scholar]
  34. Roorda S., Doorn S., Sinke W. C., Scholte P. M. L. O. & van Loenen E. Calorimetric evidence for structural relaxation in amorphous silicon. Phys. Rev. Lett. 62, 1880 (1989). [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-6 and Supplementary Discussion.

ncomms12836-s1.pdf (1.1MB, pdf)

Data Availability Statement

All relevant data are available from the corresponding authors on request.

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES