Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2016 Oct 11;6:35225. doi: 10.1038/srep35225

A new class of optical sensors: a random laser based device

Emilio Ignesti 1, Federico Tommasi 1,a, Lorenzo Fini 1, Fabrizio Martelli 1, Niccolò Azzali 1, Stefano Cavalieri 1
PMCID: PMC5057090  PMID: 27725755

Abstract

In a random laser the optical feedback is provided by scattering rather than by an optical cavity. Then, since its emission characteristics are very susceptible to the scattering details, it is a natural candidate for making active sensors to use as a diagnostic tool for disordered media like biological samples. However, the methods reported up to now, requiring the injection of toxic substances in the sample, have the drawback of altering the physical-chemical composition of the medium and are not suitable for in-vivo measurements. Here we present a random laser based sensor that overcomes these problems by keeping gain and diffusion separated. We provide an experimental characterisation of the sensor by using a reference diffusive liquid phantom and we show that, compared to a passive method, this sensor takes advantage of the gain and spectral properties of the random laser principle.

In the last two decades the development of optical sensors for the study of diffusive materials has made great advances and in particular the interest for the investigation of biological samples has generated a wealth of different techniques and applications1,2,3. In addition, the scattering properties of the materials are not always considered as undesired loss effects and noise sources; indeed, engineered disorder was also introduced in photonic devices in order to increase sensitivity4. While all these different techniques are based on passive optical methods, some researchers have explored the potentialities of stimulated emission to enhance the detection sensitivity by coupling a gain material with an optical microcavity5,6. Measurements of transport mean free path by coupling a diffusive sample with an amplified spontaneous emission beam from a dye solution pumped by a Nd: YAG laser have also been reported7. An other kind of active device can be obtained by exploiting the physical principle of the random laser8. Since the pioneer work of Letokhov9 it has been recognised that a diffusive material, when coupled to a gain medium, can provide the necessary optical feedback to sustain a laser action. Respect to a conventional laser, this light source does not need an optical cavity: the stimulated emission mechanism, that acts along random paths of light inside the disordered medium, can lead to laser-like emission if gain overcomes losses. Since ‘80s different random laser systems have been proposed. Many of them are obtained by embedding in a liquid or solid matrix active centres like dye molecules or Ti:Sa powders, and scatterers like ZnO or TiO2 nanoparticles or powdered glass10,11,12.

Biological tissues form an important class of scattering materials, and this kind of optical media have been found suitable for generating random laser radiation. Random laser action has been reported in several ex-vivo biological structures infiltrated with amplifying dye molecules: chicken tissue and pig fat13, rat muscle14 and chicken breast tissue15, bovine bone16, wing of cicada covered with a layer of a dye doped polymer film17 and butterfly embedded with ZnO nanoparticles18. Moreover, using a drug-dye composite as active medium infiltrated within the tissue, the possibility to develop new combined opto-chemical therapies has been recently reported19.

Notably and from diagnostic point of view, in ex-vivo dye infiltrated human tissue, emission spectrum modifications have been reported in malignant tissue respect to the healthy one20, opening the interesting opportunity to exploit the random laser physics to design new sensors to detect biological tissue diseases. An ex-vivo dye infiltrated human tissue from the same individual has been used for mapping the cancerous and healthy zones21.

The striking point is that the typical emission features of a random laser strongly depend on the scattering properties of the medium22,23,24,25,26,27, as well as the geometry of the active zone28 and the pump profile29. Indeed this emission is extremely sensible to small scale alterations in dimension, distribution and optical response of the scatterers. Hence, the random laser principle has been recognised as a great opportunity to create a new kind of active optical sensors for different scattering media30,31. Since the stimulated emission mechanism alters the emission spectrum shape, the effect of the disorder can be detected by measurements of spectral properties variations, instead of by energy measurements or light path reconstruction as in passive systems. In addition, in a random laser the scattering does not play the role of a loss factor, as in many passive methods, but it actually feeds the signal, leading to more suitable strategies for sensing strong scattering samples. Then, respect to typical passive techniques, a random laser based sensor can potentially take advantage of amplification of weak signals and of a detection strategy with lower experimental and computational difficulties. However, up to now the necessity to inject toxic substances, like dye molecules and solvents in the disordered medium, has hindered the application of random laser sensors for a real competitive utilisation on biological samples, given the impossibility to perform in-vivo measurements.

In the following we describe a random laser based sensor that does not suffer of this limitation. Our idea to avoid the injection of external substances in the scattering sample is to put the active medium out of the disorder. Then, our design consists in a transparent spherical cell with an active medium, leaving the sensor to communicate with the external disordered medium through the emitted and received light (Fig. 1).

Figure 1. The optical fibre ends with a transparent spherical cell containing the active medium.

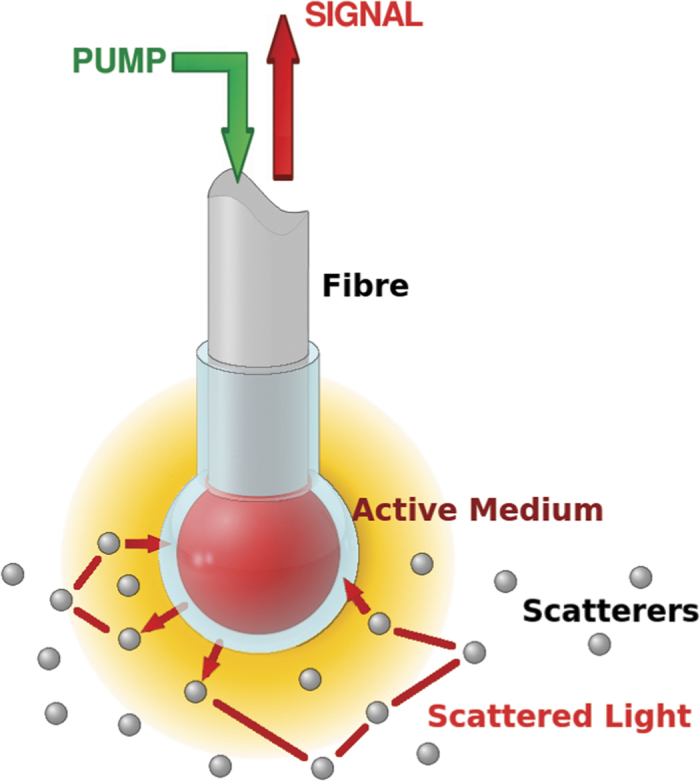
Figure 1

Open in a new tab

The pump beam and the output signal are transported by the same fibre.

The transparent walls of the cell allow the propagation of the spontaneously emitted radiation to the external disordered medium. After a random path through the diffusive medium, an amount of light can go back to the cell, thus undergoing amplification by stimulated emission in the active medium. The external disordered medium provides a feedback mechanism for the spontaneously emitted radiation, in a way dependent on the characteristics of the single scatterers and on their concentration as long as the single scattering approximation holds. Moreover, the optical fibre that carries the pump beam to create the population inversion also collects the output signal. This gives to our device characteristics of high portability and manageability.

Results

Sample preparation

In the measurements reported in this paper the disordered scattering medium is an aqueous dilution of Intralipid® 20%, a fat emulsion composed by suspended particles with size ranging from 50 to 700 nm32. Measurements were performed for several values of the pump energy and several values of the concentrations of scatterers obtained by different dilutions of Intralipid® 20%. This substance is widely used to prepare reference tissue-like phantoms33,34,35.

In order to characterize the scattering properties of the different dilutions, the chosen key parameter is the light transport mean free paths LT, i.e. the average distance beyond which the propagation direction of the propagating photons becomes completely randomized. It differs from the scattering mean free path by a factor that depends on the asymmetry of the scattering phase function. In our prepared samples, the used concentrations correspond to different LTs that cover a very large range of lengths, from a few mm to 60 μm, measured at the reference wavelength of 632.8 nm.

The reference signal was collected from an external sample without Intralipid® 20% (pure water) and it mainly consists in photons directly emitted from the active medium to the optical fibre with the additional contribute of the Fresnel reflections at the cell walls.

Experimental spectra

Figure 2 shows typical spectra of the signal obtained at a fixed pump energy of 4.32 ± 0.04 mJ and for eight different dilutions of Intralipid® 20% in the external medium. Compared to the reference signal measured in pure water, even the weakest scattering medium (LT = 2.75 mm) has the effect of modifying the shape of the spectrum, that appears still broad but with a peak at longer wavelengths (at ~600 nm) respect to the fluorescence peak (at ~590 nm); increasing the scatterers concentration, this peak shifts toward the red wavelengths up to ~625 nm. The increase of concentration leads to two other main features: a large enhancement of the total emission and the narrowing of the spectrum itself. These effects are peculiar of the random laser process. Because of the stimulated emission, at high energy the overall FWHM of the spectra changes from 23 (pure water) to 10.5 nm (LT = 0.06 mm).

Figure 2. Typical spectra for different values of the transport mean free path LT in the external disordered medium at a fixed pump energy of 4.32 ± 0.04 mJ.

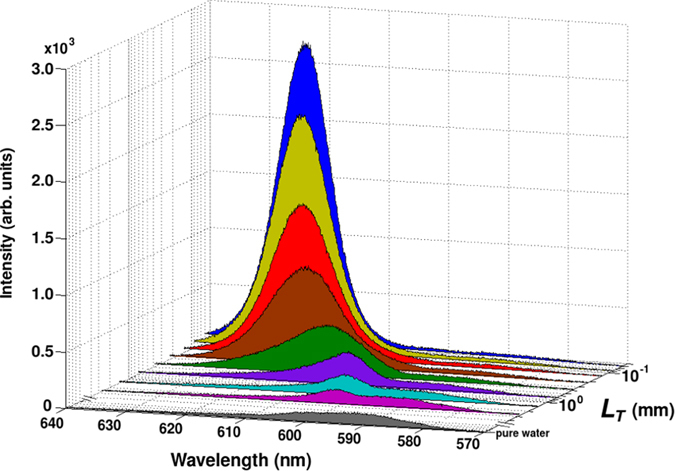
Figure 2

Open in a new tab

As the scatterers concentration increases, i.e. as LT decreases, the peak presents both a monotone enhancement and a red-shift from 590 nm in pure water to 625 nm for LT = 0.06 mm.

In Fig. 3 the peak value of the output spectrum, as a function of the pump energy and for the different Intralipid® 20% dilutions, is reported together with the reference signal (pure water). The signal shows an enhancement in presence of strong scattering with a detection limit of the method that is roughly represented by the sample with LT of 2.75 mm. An even better accuracy is provided by the analysis of the spectral behaviour of the emission. The peak wavelengths as a function of pump energy are reported in Fig. 4 for the same LT values of Fig. 3. The peak redshift is strongly dependent on the concentration and, notably, the redshift reaches a saturation value that solely depends on the scatterers concentrations in the external medium, whatever the intensity of the pump energy.

Figure 3. Intensity of the signal peak as a function of pump energy for different values of the mean free path LT (values shown in legend).

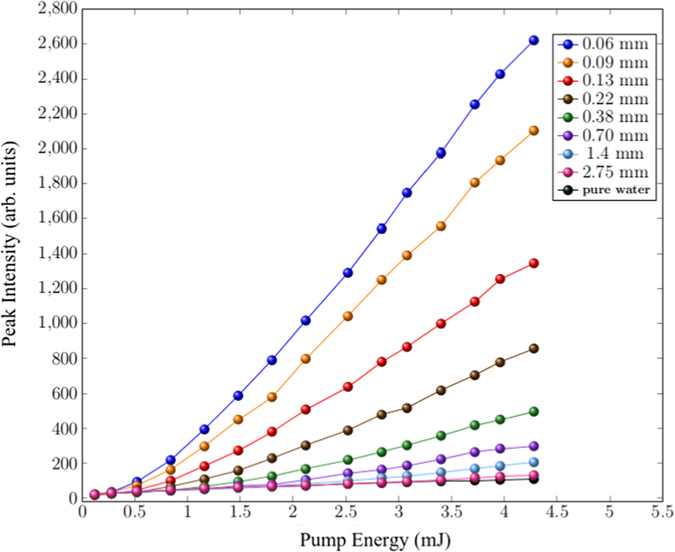
Figure 3

Open in a new tab

The reference signal at zero concentration of scatterers (pure water) is also reported.

Figure 4. Shift of the spectrum peak as a function of pump energy for different scatterers concentrations.

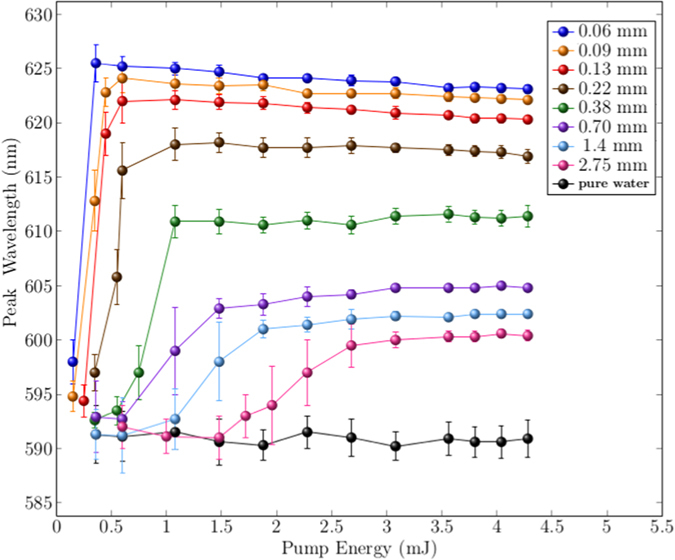
Figure 4

Open in a new tab

The peak shifts toward longer wavelengths reaching a saturation value, that only depends on LT of the external medium. Notably, no red-shift is detected in the case without scatterers (pure water).

Comparison with a passive case

In order to highlight the efficiency of the active random laser sensor, a comparison with a passive one with a similar structure was performed. To simulate a passive device, the sensor was removed from the optical fibre and the pump laser substituted by a diode laser with a broad emission spectrum (20 nm FWHM) centred at the wavelength of 650 nm. Hence, the optical fibre was immersed in the external medium and the collected scattered light was extracted and sent to the spectrometer by a beamsplitter. The measurements were obtained by collecting the cw signal out of the fibre for 17 ms. In this passive case only modifications of the spectrum intensity were observed, with no shift or spectral shape changes, confirming the fact that the shift of the peak is then peculiar of the active sensor.

The comparison between the behaviour of the two systems is shown in Fig. 5, where the measured spectral peak intensities are reported as a function of LT in the external medium. Both curves are normalised to the corresponding reference signal obtained with pure water. The active device presents a much stronger increase of signal when LT becomes shorter, up to more than one order of magnitude. The reason stands in the combined effect of total emission increase and spectral narrowing that is typical of the random laser emission.

Figure 5. Comparison between the random laser active sensor and a passive one.

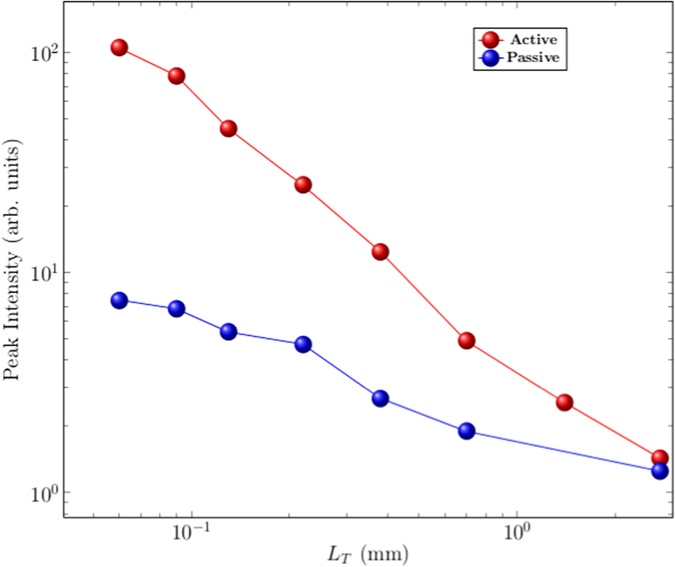
Figure 5

Open in a new tab

The peak values of relative spectra are reported, normalised to the value measured with pure water. The passive system consists of a diode laser beam (with a broad emission spectrum of 20 nm) coupled with an optical fibre whose head is immersed in the external disordered medium. The pump energy for the active case is 4.32 ± 0.04 mJ.

The large modification in the spectral behaviour, due to the interplay between the external feedback from the disordered medium, the losses by re-absorption and the gain by stimulated emission, undoubtedly represents the main effect that distinguishes this random laser based sensor from alternative passive systems.

Discussion

In conclusion we have demonstrated that the gain characteristics and spectral properties of the random laser can be usefully exploited in optical sensing applications. Maintaining the active part of the device separated from the diffusive one allowed us to build a self-contained optical sensor that could be employed in non-invasive diagnostic of biological samples. It is worth to emphasise that the results show an high sensitivity, (modifications of a few tens of μm in LT are well resolved), together with the capability to sense the transport mean free path lengths, with the same sensor, for values ranging for almost two order of magnitude. A further peculiar feature of this active sensor is the redshift of the spectrum peak, which, at high pumping energy, is determined only by the scattering properties of the external medium.

Regarding future applications on tissue optics, it is important to stress that the pumping beam at 532 nm is completely absorbed by the active medium, whereas the light that actually propagates through the medium is a low intensity fluorescence that is safe even for in-vivo applications.

Methods

Experimental set-up

The structure of the sensor is schematically shown in Figs 1 and 6. The sensor head was obtained by a glass capillary with a refractive index@632.8 nm of 1.4709 ± 0.0002, measured by a semi-automatic instrument (COMPASSO)36,37,38,39 and based on a prism coupling technique and dark line spectroscopy.

Figure 6. Scheme of the experimental set-up: Nd:YAG: pump beam source; SP: spectrometer; MP: movable polariser; FP: fixed polariser; SE: semi-transparent plate; EM: Energy Meter; DM: dichroic mirror; M: mirror; L: lens; F: optical fibre; S: sensor.

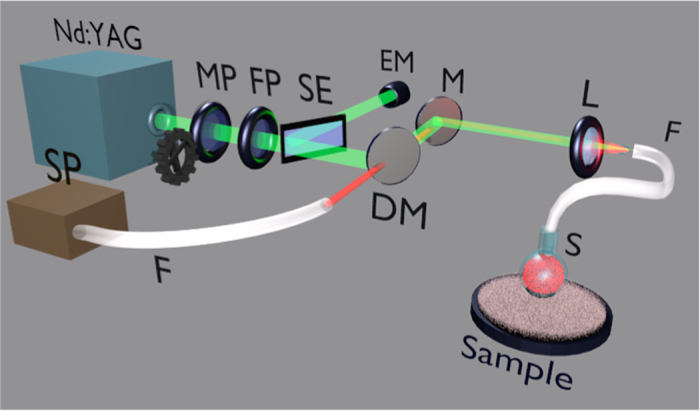
Figure 6

Open in a new tab

The green beam is the pump and the red one the signal.

The hollow sphere of an external diameter of few mm was realised in a closed end of the capillary by glass-blowing. Then the sphere was filled with the active medium and the optical fibre of a core of 900 μm was inserted in the opposite side. The active medium is composed by a suitably designed concentration of dye molecules and scatterers in an alcoholic solution. The whole device is covered by patent40.

In Fig. 6 a schematic diagram of the experimental set-up is shown. The pump beam, provided by a frequency doubled Q-switched Nd:YAG at a repetition rate of 2 Hz, is directed and focalised to the input of the sensor fibre, with a coupling efficiency of about 32%. The pumping energy is tuned by a pair of polarizers, the first of which is movable by a stepper motor connected to a PC, while the second one remain fixed. A reflection from a semi-transparent plate is sent to an energy meter to indirectly measure the pumping energy. The sensor head is immersed inside the sample, that is the external liquid medium, during the measurements.

The radiation emitted by the sensor is collected by the same fibre and sent back to a dichroic mirror towards a spectrometer with resolution of 0.25 nm. The shot-to-shot spectra are then automatically acquired and stored by a PC.

Determination of the transport mean-free-path

The values of LT for different dilutions of Intralipid ® 20% have been obtained from the expression for the reduced scattering coefficient 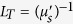Inline graphic (Inline graphic) at the reference He-Ne laser wavelength 632.8 nm41,42:

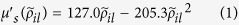graphic file with name srep35225-m3.jpg

where 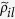Inline graphic is the fractional volume occupied by the scatterers, given the different components of the emulsion33. 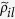Inline graphic is related to the volume concentration of Intralipid®, ρil, i.e. the ratio between the volume of Intralipid® 20% and the total volume of the emulsion (Intralipid® and water), by the relation 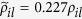Inline graphic42. The equation also accounts for the deviation from the linearity between 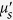Inline graphic and ρil due to the lack of validity of the independent scattering approximation, that strictly holds only when the average inter-particles distance is much larger than the wavelength.

Additional Information

How to cite this article: Ignesti, E. et al. A new class of optical sensors: a random laser based device. Sci. Rep. 6, 35225; doi: 10.1038/srep35225 (2016).

Acknowledgments

Acknowledgements are due to Andrea Barucci, Franco Cosi, Daniele Farnesi, Simone Berneschi and Gualtiero Nunzi Conti (IFAC-CNR) for contributions to fabricating the sensor and to measuring the refractive index of the glass and to Laura Piccini for finding additional materials.

Footnotes

The concept and the structure of the sensor and the method of sensing are patented.

Author Contributions E.I., F.T., L.F., F.M. and S.C. conceived the experiment, E.I., F.T. and N.A. conducted the experiment, E.I. and F.T. analysed the results. E.I., F.T., L.F., F.M. and S.C. wrote the main manuscript text. F.T. and L.F. prepared Figure 1. F.T. prepared Figures 2, 3, 4, 5 and 6. All authors reviewed the manuscript.

References

  1. Durduran T., Choe R., Baker W. B. & Yodh A. G. Diffuse optics for tissue monitoring and tomography. Rep. Prog. Phys. 73, 076701 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  2. Leff D. R. et al. Diffuse optical imaging of the healthy and diseased breast: a systematic review. Breast Cancer Res. Treat. 108, 9–22 (2008). [DOI] [PubMed] [Google Scholar]
  3. Jacques S. L. Optical properties of biological tissues: a review. Phys Med. Biol. 58, R37 (2013). [DOI] [PubMed] [Google Scholar]
  4. Redding B., Liew S. F., Sarma R. & Cao H. Compact spectrometer based on a disordered photonic chip. Nat. Photon. 7, 746–751 (2011). [Google Scholar]
  5. Humar M. & Hyun Yun S. Intracellular microlasers. Nat. Photon. 9, 572–576 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Schubert M. et al. Lasing within live cells containing intracellular optical micro-resonators for barcode-type cell tagging and tracking. Nano Lett (2015). [DOI] [PubMed] [Google Scholar]
  7. Leonetti M. & López C. Measurement of transport mean-free path of light in thin systems. Opt. Lett. 36, 2824–2826 (2011). [DOI] [PubMed] [Google Scholar]
  8. Wiersma D. S. The physics and applications of random lasers. Nat. Phys. 4, 359–367 (2008). [Google Scholar]
  9. Letokhov V. S. Generation of Light by a Scattering Medium with Negative Resonance Absorption. Sov. Phys. JETP 26, 835–840 (1968). [Google Scholar]
  10. Noginov M., Caulfield H., Noginova N. & Venkateswarlu P. Line narrowing in the dye solution with scattering centers. Opt. Commun. 118, 430–437 (1995). [Google Scholar]
  11. Wiersma D. S. & Lagendijk A. Light diffusion with gain and random lasers. Phys. Rev. E 54, 4256 (1996). [DOI] [PubMed] [Google Scholar]
  12. Sha W., Liu C.-H., Liu F. & Alfano R. Competition between two lasing modes of sulforhodamine 640 in highly scattering media. Opt. Lett. 21, 1277–1279 (1996). [DOI] [PubMed] [Google Scholar]
  13. Siddique M., Yang L., Wang Q. & Alfano R. Mirrorless laser action from optically pumped dye-treated animal tissues. Opt. Commun. 117, 475–479 (1995). [Google Scholar]
  14. Wang L., Liu D., He N., Jacques S. L. & Thomsen S. L. Biological laser action. Appl. Opt. 35, 1775–1779 (1996). [DOI] [PubMed] [Google Scholar]
  15. Polson R. & Vardeny Z. Organic random lasers in the weak-scattering regime. Phys. Rev. B 71, 045205 (2005). [Google Scholar]
  16. Song Q. et al. Random lasing in bone tissue. Opt. Lett. 35, 1425–1427 (2010). [DOI] [PubMed] [Google Scholar]
  17. Zhang D., Kostovski G., Karnutsch C. & Mitchell A. Random lasing from dye doped polymer within biological source scatters: The pomponia imperatorial cicada wing random nanostructures. Org. Electron. 13, 2342–2345 (2012). [Google Scholar]
  18. Wang C.-S., Chang T.-Y., Lin T.-Y. & Chen Y.-F. Biologically inspired flexible quasi-single-mode random laser: An integration of pieris canidia butterfly wing and semiconductors. Sci. Rep. 4 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Lahoz F. et al. Random laser in biological tissues impregnated with a fluorescent anticancer drug. Laser Phys. Lett. 12, 045805/1–6 (2015). [Google Scholar]
  20. Polson R. C. & Vardeny Z. V. Random lasing in human tissues. Appl. Phys. Lett. 85, 1289–1291 (2004). [Google Scholar]
  21. Polson R. C. & Vardeny Z. V. Cancerous tissue mapping from random laser emission spectra. J. Opt. 12, 024010/1–4 (2010). [Google Scholar]
  22. Mujumdar S., Ricci M., Torre R. & Wiersma D. S. Amplified extended modes in random lasers. Phys. Rev. Lett. 93, 053903 (2004). [DOI] [PubMed] [Google Scholar]
  23. Wu X. et al. Random lasing in weakly scattering systems. Phys. Rev. A 74, 053812 (2006). [Google Scholar]
  24. Sharma D., Ramachandran H. & Kumar N. Lévy statistical fluctuations from a random amplifying medium. Fluct. Noise Lett. 6, L95–L101 (2006). [DOI] [PubMed] [Google Scholar]
  25. Uppu R., Tiwari A. K. & Mujumdar S. Identification of statistical regimes and crossovers in coherent random laser emission. Opt. Lett. 37, 662–664 (2012). [DOI] [PubMed] [Google Scholar]
  26. El-Dardiry R. G., Mooiweer R. & Lagendijk A. Experimental phase diagram for random laser spectra. New J. Phys. 14, 113031 (2012). [Google Scholar]
  27. Ignesti E. et al. Experimental and theoretical investigation of statistical regimes in random laser emission. Phys. Rev. A 88, 033820 (2013). [Google Scholar]
  28. Tommasi F., Ignesti E., Fini L. & Cavalieri S. Controlling directionality and the statistical regime of the random laser emission. Phys. Rev. A 91, 033820 (2015). [Google Scholar]
  29. Leonetti M. & López C. Active subnanometer spectral control of a random laser. Appl. Phys. Lett. 102, 7 (2013). [Google Scholar]
  30. Cao H. Random lasers: development, features and applications. Opt. Photonics News 16, 24–29 (2005). [Google Scholar]
  31. Choi S. H. & Kim Y. L. The potential of naturally occurring lasing for biological and chemical sensors. Biomed. Eng. Lett. 4, 201–212 (2014). [Google Scholar]
  32. Michels R., Foschum F. & Kienle A. Optical properties of fat emulsions. Opt. Express 16, 5907–5925 (2008). [DOI] [PubMed] [Google Scholar]
  33. Van Staveren H. J., Moes C. J., van Marie J., Prahl S. A. & Van Gemert M. J. Light scattering in lntralipid-10% in the wavelength range of 400–1100 nm. Appl. Opt. 30, 4507–4514 (1991). [DOI] [PubMed] [Google Scholar]
  34. Pogue B. W. & Patterson M. S. Review of tissue simulating phantoms for optical spectroscopy, imaging and dosimetry. J. Biomed. Opt. 11, 041102–041102 (2006). [DOI] [PubMed] [Google Scholar]
  35. Di Ninni P., Martelli F. & Zaccanti G. Intralipid: towards a diffusive reference standard for optical tissue phantoms. Phys. Med. Biol. 56, N21 (2011). [DOI] [PubMed] [Google Scholar]
  36. Monneret S., Huguet-Chantome P. & Flory F. m-lines technique: prism coupling measurement and discussion of accuracy for homogeneous waveguides. J. Opt. A: Pure Appl. Opt. 2, 188–195 (2000). [Google Scholar]
  37. Ulrich R. & Torge R. Measurement of Thin Film Parameters with a Prism Coupler. Appl. Opt. 12, 2901–2908 (1973). [DOI] [PubMed] [Google Scholar]
  38. Berneschi S. et al. Optical and spectroscopic properties of soda-lime alumino silicate glasses doped with Er3+ and/or Yb3 +. Opt. Mater. 28, 1271–1275 (2006). [Google Scholar]
  39. Berneschi S. et al. Aluminum co-doping of soda-lime silicate glasses: Effect on optical and spectroscopic properties. J. Non-Cryst. Solids 351, 1747–1753 (2005). [Google Scholar]
  40. Cavalieri S., Fini L., Ignesti E., Tommasi F. & Martelli F. Sensore ottico basato su random laser. Italian Patent 102016000054453. 2016.
  41. Zaccanti G., Bianco S. D. & Martelli F. Measurements of optical properties of high density media. Appl. Opt. 42, 4023–4030 (2003). [DOI] [PubMed] [Google Scholar]
  42. Martelli F. & Zaccanti G. Calibration of scattering and absorption properties of a liquid diffusive medium at NIR wavelengths. CW method. Opt. Express 15(2), 486–500 (2007). [DOI] [PubMed] [Google Scholar]

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES